Reasoning, Representing, and Generalizing in Geometric Proof Problems among 8th Grade Talented Students
Abstract
:1. Introduction
- Analyze students’ reasoning style and their willingness to use visual or analytical processes in proofs must be determined;
- Detect the role of representation in the justifications put forward by students;
- Organize the level of generality shown by the student in their arguments.
2. Theoretical Framework
2.1. Reasoning Styles
- Analytical: where the logical–verbal dimension prevails over the visual–pictorial component. Scantly developed spatial concepts, inability to use visual support to solve problems, and no need felt for such support;
- Geometric: where the pictorial–visual dimension prevails over the logical–verbal component. Well-developed spatial concepts, and ability to use and acceptance of visual support as necessary for problem solving;
- Harmonic: where the logical–verbal and visual–pictorial components are in balance. Well-developed spatial concepts.
2.2. Representation
2.3. Scope of Argumentation
3. Methodology
3.1. Subjects and Problems
Lesson Components and Sequence
3.2. Analysis of Questionnaires
- Geometric (visual): prevalence of the pictorial–visual component. Argumentation is supported by visual elements only, alluding exclusively to visually perceived equality of areas or lengths.
- Analytical: prevalence of the logical–verbal component. Argumentation is supported by algebraic expressions, deduction of properties, or the use of theorems.
- Harmonic: even balance between the two components. Argumentation is supported by the connection between visualization and analytical reasoning, alluding to area equality in the graphics to deduce algebraic expressions and vice-versa.
- Particular: argumentation focused on a specific case, with allusion to no other.
- Set: argumentation with allusion to a given set of elements.
- Generalization: generalized argumentation valid for all cases.
- Verbal: use of natural language only.
- Algebraic: use of symbolic notation and algebraic expressions.
- Pictorial: use of resources such as drawings, diagrams, and graphics, including manipulation with Geogebra.
4. Results
4.1. Overall Data
4.2. Reasoning Styles
4.2.1. Harmonic Reasoning
S5: This is the proof of the Pythagorean theorem. If the triangles are subtracted from the large square, the result is an area of c2. If the position of the triangles changes, two squares would be left, a2 and b2, which means that c2 = (a + b)2 − 2ab and a2 + b2 = (a + b)2 − 2ab and, therefore, that c2 = a2 + b2.
S13: This proof is for the Pythagorean theorem. You know that because the formula represented is the sum of a + b squared. a and b form triangles because if you formulate an equation with them, you get c2 = (a + b)2 + (a × b + a × b + a × b + a × b)/2. If you simplify that equation, the result is a2 + b2.
Yes, it would be graphic proof because you can see from the drawing that the Pythagorean theorem holds. And you can also derive the formula from the graphic representation. Because in the end all you vary is their length. In other words, in the end [it doesn’t matter] whether the length is larger or smaller in the drawing, it will always be a + b and the area will be a times b divided by 2 and in the end you get the same result.
S18: I think this is the proof of the Pythagorean theorem. I think it’s a proof first of all because if you find for the whole square you get a2 + b2 + 2ab and if you subtract the four right triangles from the square what should remain is the area of the central square, a2 + b2 + 2ab – 4 × ab/2 = a2 + b2, so c is the area of the square. And since the two squares have the same area when you remove the right triangles, which together sum the same area as the ones in the first square, what we have is that the sum of the areas of the squares with sides a and b is the same as the area of the square with side c in the first square, so again a2 + b2 + 2ab.
4.2.2. Visual Reasoning
S4: When you rotate or move (the triangle) the angles remain unaffected so it continues to be a right triangle to which you can apply the Pythagorean theorem. Thanks to symmetry and homotheties it’s the same for similar triangles. The shape (angles) goes unchanged, except that the result is a larger or smaller mirror image.
S8: Yes, they intersect at the circumcenter, which is the point where all perpendicular bisectors for all sides of the triangle intersect. In right triangles that point is located on the hypotenuse; in obtuse triangles outside the triangle and in equilateral tringles inside it (inner point).
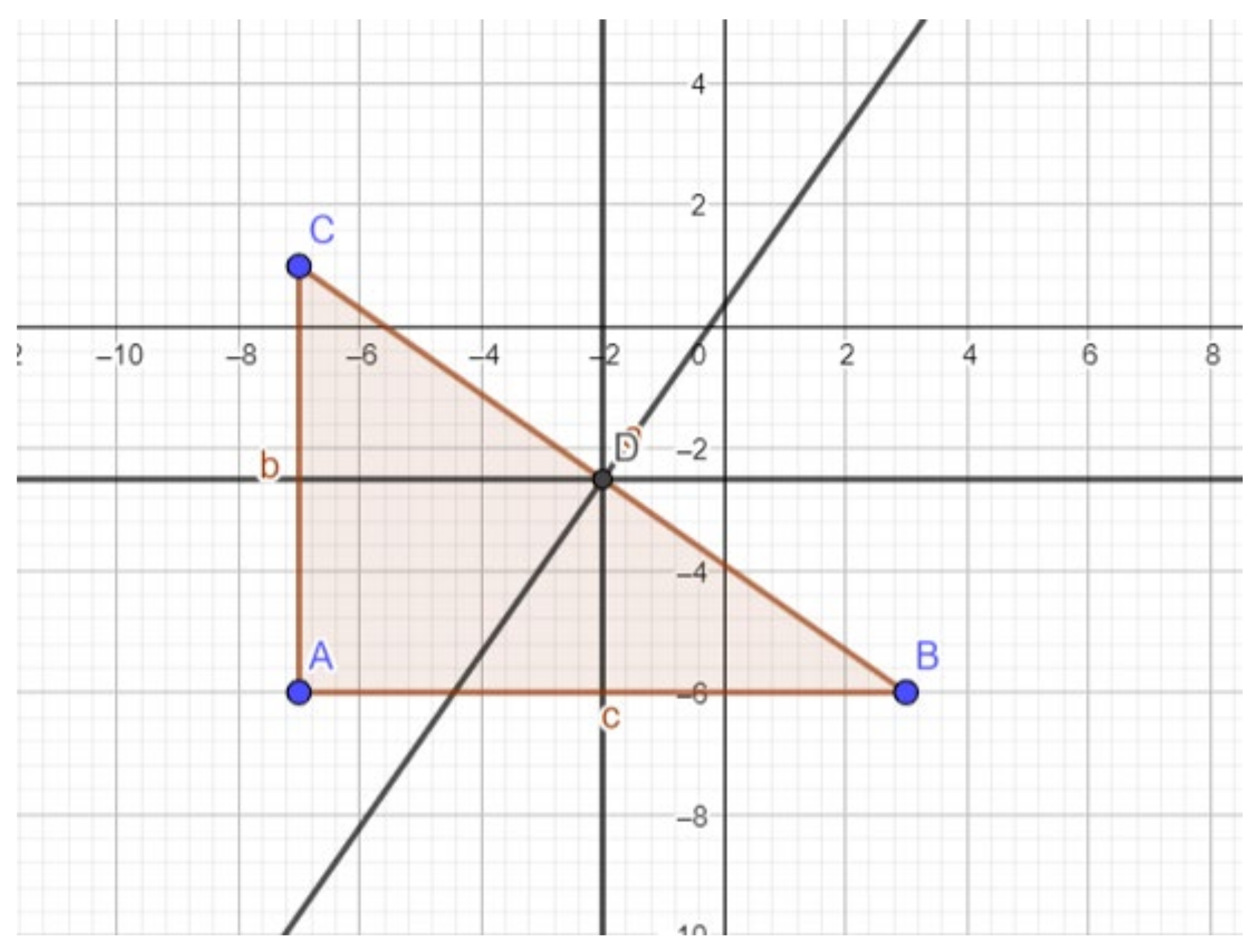
S6: It holds in all [cases] because as long as the triangle has a right angle, it holds. It doesn’t matter if it’s moved or the sides are larger or smaller (Figure 5).
S19: Yes, because the triangle is always the same (and therefore always has a right angle which is why the Pythagorean theorem holds), except that the position changes.
S11: It also holds after applying symmetry because the symmetric triangle would have the same angles and sides as the original, but in different positions.
It also holds after homotheties because if all the sides are multiplied times the same number, the squares [of those products] would comply. If m is the number times which they are multiplied, then m2(a2 + b2) = m2 × c2. If you then divide both sides of the equation by m2 you get the same equation.
4.2.3. Analytical Reasoning
S4: The perpendicular bisectors are a straight line where all the points are at an equal distance from both edges. Each bisector is therefore consistent with the definition. If all the points are at the same distance from both ends on the bisectors, there must be one point that’s at the same distance from all three points and that point would have to be on the three bisectors.
S4: The three bisectors intersect at a point that must be at the same distance from a, b and c. Since the circumcenter is the circumference formed with the points on the triangle, it has to be at that point.
S16: Yes, because it doesn’t matter what the triangle is like because since the point is at the same distance from all the vertices you’ll always be able to draw a circle that touches them all.
S11: Yes, it’s true, because like a classmate said, the perpendicular bisector for a is at the same distance from vertex a as from vertex b; the bisector for b is at the same distance from vertex b as from c and the bisector for c is at the same distance from c as from a. In that case, the point where the three bisectors intersect is at the same distance from all the vertices, and that distance is the radius of the circumcenter.
4.3. Equivalence Class-Based Reasoning
S13: Yes, for all right triangles the circumcenter would be on the hypotenuse. That is so because the perpendicular bisector for one leg is parallel to the other leg, and vice-versa. And they intersect at the midpoint of the leg where they form as well as at the midpoint of the hypotenuse, and the bisector for the hypotenuse, like the bisectors for the legs, springs from its midpoint.
S13: There are infinite equilateral triangles, but they’re all similar.
S13: Yes, but it’s easier to prove than in all cases of right triangles that the circumcenter is on one side. It’s harder to prove than when the circumcenter is on one side, that it’s a right triangle.
S4: If the circumcenter is on one side, that means the point is equidistant from the edges of the triangle. It has to be a right triangle then, because if it were an acute triangle it would be inside and if it were an obtuse triangle, it would be outside it.
S4: The perpendicular bisector is a straight line where all the points are at an equal distance from both edges of the segment. Therefore if we take the three bisectors there must necessarily be in all triangles a point that’s at the same distance from a as it is from b and c, in other words, the three vertices. The bisectors for legs ab, ac and ca will pass through that point. And it’s there they’ll intersect and it has to be inside because otherwise it wouldn’t be at the same distance from the three points.
R1: (…) Does anyone think otherwise?
S13: I don’t fully agree. Because when I uploaded it to Geogebra I found that when a right triangle is involved, the perpendicular bisector for one leg is parallel to the other leg and the same happens with the other leg. And the bisector for the hypotenuse intersects the square formed by the legs and the bisectors diagonally. And if the triangle is obtuse, it would start to shorten… and the point would lie outside. That would contradict what S4 said because the point where all the points are at the same distance doesn’t have to lie inside the triangle. In an obtuse triangle that point would be outside the triangle.
S4: What can I say? (…) You’ve convinced me.
S13: Well… If the triangle is acute, the circumcenter lies inside it. And when it’s obtuse, outside.
R1: And why would that be?
S13: I’d have to think about that.
R1: If when you see in Geogebra that when it moves it falls outside, would that be a valid proof for you?
S13: It would be evidence, but not proof.
5. Discussion and Conclusions
- Harmonic and analytical reasoning proved to be more favorable than visual reasoning to generalized argumentation
- The type of representation was not found to be the sole determinant for the level of generality
- The use of representation systems based on items representative of whole sets may favor the transition from perceptive to general
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
- Problem 1: What is being proven in the Figure A1 below?
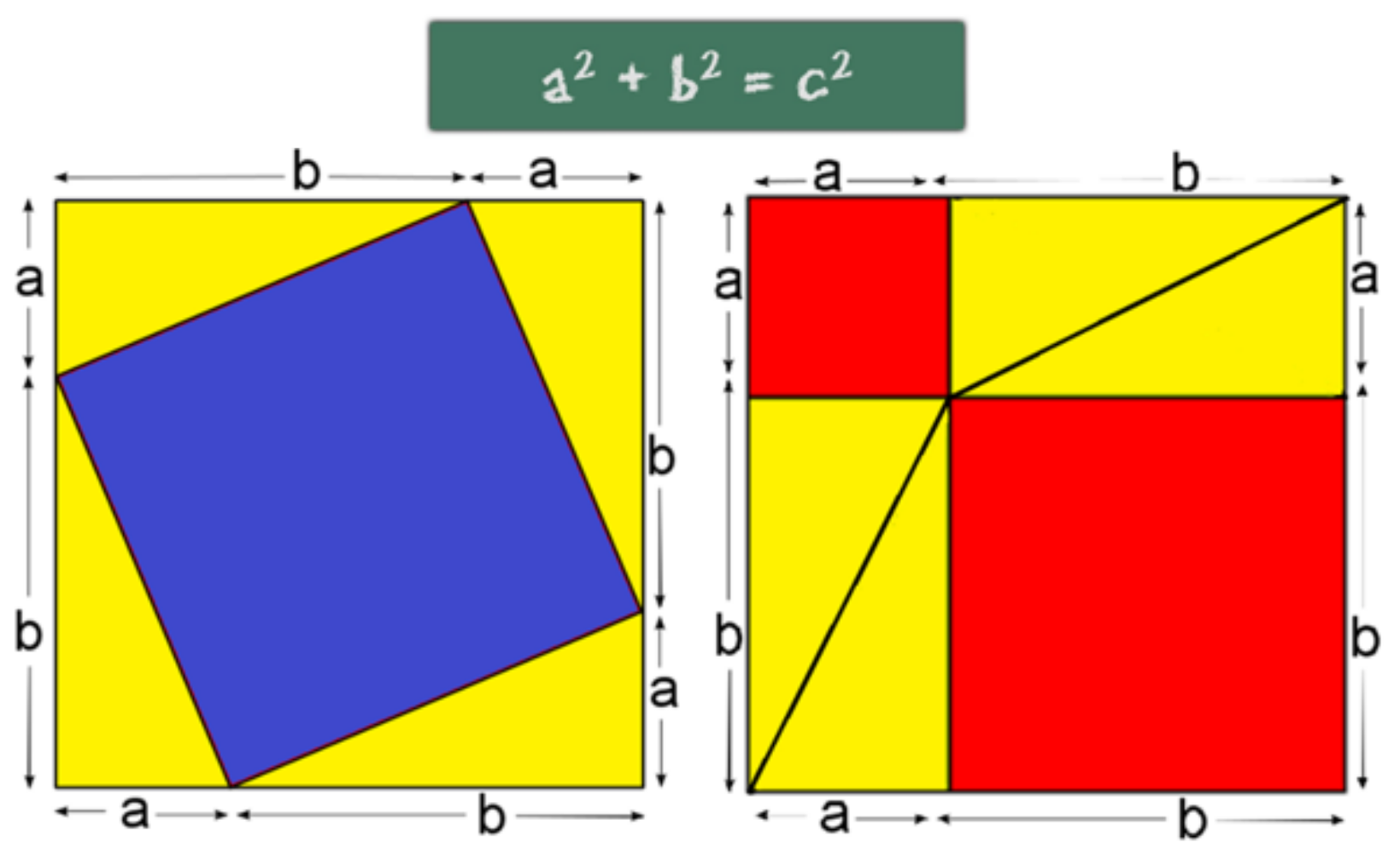
- Problem 2: If the Pythagorean theorem holds in a right triangle:
- Does it hold if the triangle is rotated?
- If it is translated?
- If it is symmetrically transformed?
- If it is homothetically transformed?
- Would it hold in a similar triangle?
- Problem 3: Is the following statement true? Explain your answer.
- Problem 4: Is the following statement true? Explain your answer.
- Problem 5: Is the following statement true? Explain your answer.
References
- Jones, K.; Tzekaki, M. Research on the teaching and learning of geometry. In The Second Handbook of Research on the Psychology of Mathematics Education: The Journey Continues; Gutiérrez, Á., Leder, G.C., Boero, P., Eds.; Sense Publishers: Rotterdam, The Netherlands, 2016; pp. 109–149. [Google Scholar]
- Stylianides, A.J.; Harel, G. Advances in Mathematics Education Research on Proof and Proving: An International Perspective; Springer: Cham, Switzerland, 2018. [Google Scholar]
- Jones, K. Issues in the Teaching and Learning of Geometry. In Aspects of Teaching Secondary Mathematics. Perspectives on Practice; Haggarty, L., Ed.; Routledge Falmer: London, UK, 2002; pp. 121–139. [Google Scholar]
- Meyer, M.; Schnell, S. What counts as a “good” argument in school?—How teachers grade students’ mathematical arguments. Educ. Stud. Math. 2020, 105, 35–51. [Google Scholar] [CrossRef]
- Rocha, H. Mathematical proof: From mathematics to school mathematics. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2019, 377, 20180045. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Lange, M. Aspects of mathematical explanation: Symmetry, unity, and salience. Philos. Rev. 2014, 123, 485–531. [Google Scholar] [CrossRef] [Green Version]
- Schwarz, B.B.; Hershkowitz, R.; Prusak, N. Argumentation and mathematics. In Educational Dialogues: Understanding and Promoting Productive Interaction; Howe, C., Littleton, K., Eds.; Routledge: London, UK, 2010; pp. 115–141. [Google Scholar]
- Sriraman, B.; Umland, K. Argumentation in Mathematics Education. In Encyclopedia of Mathematics Education; Lerman, S., Ed.; Springer: London, UK, 2014; pp. 46–48. [Google Scholar]
- Campbell, T.G.; Boyle, J.D.; King, S. Proof and argumentation in K-12 mathematics: A review of conceptions, content, and support. Int. J. Math. Educ. Sci. 2020, 51, 754–774. [Google Scholar] [CrossRef]
- Common Core State Standards Initiative. Common Core State Standards for Mathematics; National Governors Association Center for Best Practices and the Council of Chief State School Officers: Washington, DC, USA, 2010. [Google Scholar]
- Mulligan, J.; Woolcott, G.; Mitchelmore, M.; Davis, B. Connecting mathematics learning through spatial reasoning. Math. Educ. Res. J. 2018, 30, 77–87. [Google Scholar] [CrossRef]
- Duval, R. Understanding the Mathematical Way of Thinking: The Registers of Semiotic Representations; Springer: Cham, Switzerland, 2017. [Google Scholar]
- Alcock, L.; Weber, K. Undergraduates’ example use in proof production: Purposes and effectiveness. Investig. Math. Learn. 2010, 3, 1–22. [Google Scholar] [CrossRef] [Green Version]
- Zazkis, D.; Weber, K.; Mejía-Ramos, J.P. Bridging the gap between graphical arguments and verbal symbolic proofs in a real analysis context. Educ. Stud. Math. 2016, 93, 155–173. [Google Scholar] [CrossRef]
- Pedemonte, B. How can the relationship between argumentation and proof be analysed? Educ. Stud. Math. 2007, 66, 23–41. [Google Scholar] [CrossRef]
- Pedemonte, B. Argumentation and algebraic proof. ZDM—Int. J. Math. Educ. 2008, 40, 385–400. [Google Scholar] [CrossRef]
- Bartlo, J.R. Why Ask Why: An Exploration of the Role of Proof in the Mathematics Classroom. Ph.D. Thesis, Portland State University, Portland, OR, USA, 2013. [Google Scholar]
- Gulkilik, H.; Kaplan, H.A.; Emul, N. Investigating the relationship between argumentation and proof from a representational perspective. Int. J. Math. Teach. Learn. 2019, 20, 131–148. [Google Scholar]
- Lin, F.-L.; Yang, K.-L.; Lee, K.-H.; Tabach, M.; Stylianides, G. Principles for task design for conjecturing and proving. In Proof and Proving in Mathematics Education, New ICMI Study Series 15; Hanna, G., de Villiers, M., Eds.; Springer: Dordrecht, The Netherlands, 2012; pp. 305–325. [Google Scholar]
- Stylianides, A.J.; Bieda, K.N.; Morselli, F. Proof and argumentation in mathematics education. In The Second Handbook of Research on the Psychology of Mathematics Education; Gutiérrez, A., Leder, G.C., Boero, P., Eds.; Sense Publishers: Rotterdam, The Netherlands, 2016; pp. 315–351. [Google Scholar]
- Balacheff, N. The benefits and limits of social interaction: The case of teaching mathematical proof. In Mathematical Knowledge: Its Growth through Teaching; Bishop, A., Mellin-Olsen, S., Van Dormolen, J., Eds.; Kluwer: Dordrecht, The Netherlands, 1991; Volume 186, pp. 175–192. [Google Scholar]
- Schwarz, B. Argumentation and learning. In Argumentation and Education; Muller Mirza, N., Perret-Clermont, A., Eds.; Springer: New York, NY, USA, 2009; pp. 91–126. [Google Scholar]
- Healy, L.; Hoyles, C. Software tools for geometric problem solving: Potentials and pitfalls. Int. J. Comput. Math. Learn. 2001, 6, 235–256. [Google Scholar] [CrossRef]
- Mariotti, M.A. Justifying and proving in the Cabri environment. Int. J. Comput. Math. Learn. 2001, 6, 257–281. [Google Scholar] [CrossRef]
- Marrades, R.; Gutierrez, A. Proofs produced by secondary school students learning geometry in a dynamic computer environment. Educ. Stud. Math. 2000, 44, 87–125. [Google Scholar] [CrossRef]
- Hanna, G. Challenges to the importance of proof. Learn. Math. 1995, 15, 42–49. [Google Scholar]
- Hanna, G. Mathematical proof, argumentation, and reasoning. In Encyclopedia of Mathematics Education; Lerman, S., Ed.; Springer: London, UK, 2014; pp. 561–566. [Google Scholar]
- Aksoy, Y.; Bayazit, İ.; Soybaş, D. The Effects of GeoGebra in Conjectures and Proofs. In Proceedings of the First North American GeoGebra Conference, Ithaca, NY, USA, 1 April 2010–31 March 2011; Geogebra-NA: Ithaca, NY, USA, 2010; pp. 190–195. [Google Scholar]
- Hohenwarter, M.; Fuchs, K. Combination of Dynamic Geometry, Algebra and Calculus in the Software System GeoGebra. In Computer Algebra Systems and Dynamic Geometry Systems in Mathematics Teaching Conference; Darwish, D., Ed.; 2004; pp. 1–6. Available online: https://www.researchgate.net/publication/228398347_Combination_of_dynamic_geometry_algebra_and_calculus_in_the_software_system_GeoGebra (accessed on 6 February 2022).
- Widder, M.; Berman, A.; Koichu, B. Action strategies in spatial geometry problem solving supported by dynamic geometry software. In Proceedings of the 42nd conference of the International Group for the Psychology of Mathematics Education, Umeå, Sweden, 3–8 July 2018; Bergqwist, E., Österholm, M., Granberg, C., Sumpter, L., Eds.; PME: Umea, Sweden, 2018; Volume 4, pp. 435–442. [Google Scholar]
- Gutiérrez, A. Aspectos metodológicos de la investigación sobre aprendizaje de la demostración mediante exploraciones con software de geometría dinámica. In Actas del IX Simposio de la Sociedad Española de Investigación en Educación Matemática; Maz, A., Gómez, B., Torralbo, M., Eds.; SEIEM: Córdoba, Spain, 2005; pp. 27–44. [Google Scholar]
- Tsujiyama, Y.; Yui, K. Using examples of unsuccessful arguments to facilitate students’ reflection on their processes of proving. In Advances in Mathematics Education Research on Proof and Proving: An International Perspective; Stylianides, A.J., Harel, G.H., Eds.; Springer: Cham, Switzerland, 2018; pp. 269–281. [Google Scholar]
- Subtil, M.; Domingos, A. The instrumental genesis in the demonstration of the pythagorean theorem. In Proceedings of the 42nd Conference of the International Group for the Psychology of Mathematics Education, Umeå, Sweden, 3–8 July 2018; Bergqwist, E., Österholm, M., Granberg, C., Sumpter, L., Eds.; PME: Umea, Sweden, 2018; Volume 5, p. 164. [Google Scholar]
- Battista, M.T. Representations and cognitive objects in modern school geometry. In Research on Technology and the Teaching and Learning of Mathematics; Blume, G., Heid, M.K., Eds.; Information Age Publishing: Charlotte, NC, USA, 2008; Volume 2, pp. 341–362. [Google Scholar]
- Komatsu, K. How do students generalize a conjecture through proving?: The importance of boundary cases between example and counterexample. In Proceedings of the 35th Conference of the International Group for the Psychology of Mathematics Education, Ankara, Turkey, 10–15 July 2011; Ubuz, B., Ed.; PME: Ankara, Turkey, 2011; Volume 3, pp. 89–96. [Google Scholar]
- Knuth, E. Teachers conceptions of proof in the context of secondary school mathematics. J. Res. Math. Educ. 2002, 5, 61–88. [Google Scholar]
- Benedicto, C.; Gutierrez, A.; Jaime, A. Analysis of Mathematically Gifted Students’ Answers to Cognitively Demanding School Tasks. In Proceedings of the 42nd Conference of the International Group for the Psychology of Mathematics Education, Umeå, Sweden, 3–8 July 2018; Bergqwist, E., Österholm, M., Granberg, C., Sumpter, L., Eds.; PME: Umea, Sweden, 2018; Volume 5, p. 18. [Google Scholar]
- National Council of Teachers of Mathematicas. Principles to Actions; NCTM: Reston, VA, USA, 2014. [Google Scholar]
- Zaslavsky, O. Genericity, conviction, and conventions: Examples that prove and examples that don’t prove. In Advances in Mathematics Education Research on Proof and Proving: An International Perspective; Stylianides, A.J., Harel, G., Eds.; Springer: Cham, Switzerland, 2018; pp. 283–298. [Google Scholar]
- Dogan, M.F.; Williams-Pierce, C. The role of generic examples in teachers’ proving activities. Educ. Stud. Math. 2021, 106, 133–150. [Google Scholar] [CrossRef]
- Rott, B. Inductive and deductive justification of knowledge: Epistemological beliefs and critical thinking at the beginning of studying mathematics. Educ. Stud. Math. 2021, 106, 117–132. [Google Scholar] [CrossRef]
- Fujita, T.; Kondo, Y.; Kumakura, H.; Kunimune, S.; Jones, K. Spatial reasoning skills about 2D representations of 3D geometrical shapes in grades 4 to 9. Math. Educ. Res. J. 2020, 32, 235–255. [Google Scholar] [CrossRef]
- Lowrie, T.; Logan, T.; Hegarty, M. The influence of spatial visualization training on students’ spatial reasoning and mathematics performance. J. Cogn. Dev. 2019, 20, 729–751. [Google Scholar] [CrossRef]
- Uygun, T. An inquiry-based design research for teaching geometric transformations by developing mathematical practices in dynamic geometry environment. Math. Educ. Res. J. 2020, 32, 523–549. [Google Scholar] [CrossRef]
- Seah, R.T.K.; Horne, M. The influence of spatial reasoning on analysing about measurement situations. Math. Educ. Res. J. 2020, 32, 365–386. [Google Scholar] [CrossRef]
- Subramaniam, K. Representational coherence in instruction as a means of enhancing students’ access to mathematics. In Proceedings of the 43rd Conference of the International Group for the Psychology of Mathematics Education, Pretoria, South Africa, 7–12 July 2019; Graven, M., Venkat, H., Essien, A., Vale, P., Eds.; PME: Pretoria, South Africa, 2019; Volume 1, pp. 33–52. [Google Scholar]
- Resnick, I.; Harris, D.; Logan, T.; Lowrie, T. The relation between mathematics achievement and spatial reasoning. Math. Educ. Res. J. 2020, 32, 171–174. [Google Scholar] [CrossRef]
- Kollosche, D. Styles of reasoning for mathematics education. Educ. Stud. Math. 2021, 107, 471–486. [Google Scholar] [CrossRef]
- Stylianides, A.J. Proof and proving in school mathematics. J. Res. Math. Educ. 2007, 38, 289–321. [Google Scholar]
- Mariotti, M.A. Proof and proving in mathematics education. In Handbook of Research on the Psychology of Mathematics Education: Past, Present and Future; Gutiérrez, A., Boero, P., Eds.; Sense Publishers: Rotterdam, The Netherlands, 2006; pp. 173–204. [Google Scholar]
- Harel, G.; Sowder, L. Students’ proof schemes: Results from exploratory studies. In Research in Collegiate Mathematics Education III; Schoenfeld, A., Kaput, J., Dubinsky, E., Eds.; Mathematical Association of America: Washington, DC, USA, 1998; pp. 234–283. [Google Scholar]
- Krutetskii, V.A. The psychology of Mathematical Abilities in Schoolchildren; University of Chicago Press: Chicago, IL, USA, 1976. [Google Scholar]
- Lean, G.; Clements, M.A. Spatial ability, visual imagery, and mathematical performance. Educ. Stud. Math. 1981, 12, 267–299. [Google Scholar] [CrossRef]
- Rivera, F.D. Teaching and Learning Patterns in School Mathematics: Psychological and Pedagogical Considerations; Springer: Dordrecht, The Netherlands, 2013. [Google Scholar]
- Cheah, U.H.; Herbst, P.G.; Ludwig, M.; Richard, P.R.; Scaglia, S. Topic study group no. 13: Teaching and learning of Geometry—Secondary Level. In Proceedings of the 13th ICME, Hamburg, Germany, 24–31 July 2017; Kaiser, G., Ed.; Springer: Berlin/Heidelberg, Germany, 2017; pp. 435–438. [Google Scholar]
- Presmeg, N.C. Visualisation and mathematical giftedness. Educ. Stud. Math. 1986, 17, 297–311. [Google Scholar] [CrossRef]
- Lee, K.; Ko, E.; Song, S. The analysis of activity that gifted students construct definition of regular polyhedra. In Proceedings of the 31st Conference of the International Group for the Psychology of Mathematics Education, Seoul, Korea, 8–13 July 2007; Wo, J.H., Lew, H.C., Park, K.S., Seo, D.Y., Eds.; PME: Seoul, Korea, 2007; Volume 3, pp. 153–160. [Google Scholar]
- Neria, D.; Amit, M. Talented middle school students’ strategies and reasoning in solving analytic reasoning problems. In Proceedings of the 34th Conference of the International Group for the Psychology of Mathematics Education, Belo Horizonte, Brazil, 18–23 July 2010; Pinto, M.M., Pinto, F., Kawasaki, T.F., Eds.; PME: Belo Horizonte, Brazil, 2010; Volume 3, pp. 321–328. [Google Scholar]
- Ryu, H.; Chong, Y.; Song, S. Mathematically gifted students’ spatial visualization ability of solid figures. In Proceedings of the 31st Conference of the International Group for the Psychology of Mathematics Education, Seoul, Korea, 8–13 July 2007; Wo, J.H., Lew, H.C., Park, K.S., Seo, D.Y., Eds.; PME: Seoul, Korea, 2007; Volume 4, pp. 137–144. [Google Scholar]
- Gruessing, M. Spatial abilities and mathematics achievement among elementary school children. In Proceedings of the 35th Conference of the International Group for the Psychology of Mathematics Education, Ankara, Turkey, 10–15 July 2011; Ubuz, B., Ed.; PME: Ankara, Turkey, 2011; Volume 1, p. 306. [Google Scholar]
- Rabab’h, B.; Veloo, A. Spatial visualization as mediating between mathematics learning strategy and mathematics achievement among 8th grade students. Int. Educ. Stud. 2015, 8, 1–11. [Google Scholar] [CrossRef] [Green Version]
- Rivera, F.D. Towards a Visually-Oriented School Mathematics Curriculum; Springer: Dordrecht, The Netherlands, 2011. [Google Scholar]
- Van Garderen, D. Spatial visualization, visual imagery, and mathematical problem solving of students with varying abilities. J. Learn. Disabil. 2006, 39, 496–506. [Google Scholar] [CrossRef]
- Liu, Y. Aspects of Mathematical Arguments that Influence Eighth Grade Students’ Judgment of their Validity. Ph.D. Thesis, Ohio State University, Columbus, OH, USA, 2013. [Google Scholar]
- Laamena, C.M.; Nusantara, T.; Irawan, E.B.; Muksar, M. Analysis of the Students’ Argumentation based on the level of Ability: Study on the Process of Mathematical Proof. J. Phys. Conf. Ser. 2018, 1028, 012156. [Google Scholar] [CrossRef]
- Seah, R.; Horne, M. The construction and validation of a geometric reasoning test item to support the development of learning progression. Math. Educ. Res. J. 2020, 32, 607–628. [Google Scholar] [CrossRef]
- Sukirwan; Darhim; Herman, T.; Prahmana, R.C.I. The students’ mathematical argumentation in geometry. J. Phys. Conf. Ser. 2018, 943, 012026. [Google Scholar]
- Beitlich, J.T.; Obsteiner, A.; Reiss, K. How do secondary school students make use of different representation formats in heuristic worked examples? An analysis of eye movements. In Proceedings of the 39th Conference of the International Group for the Psychology of Mathematics Education, Hobart, Australia, 13–18 July 2015; Beswick, K., Muir, T., Fielding-Wells, J., Eds.; PME: Hobart, Australia, 2015; pp. 97–104. [Google Scholar]
- Healy, L.; Hoyles, C. A study of proof conceptions in Algebra. J. Res. Math. Educ. 2000, 31, 396–428. [Google Scholar] [CrossRef]
- Goldin, G.A. Mathematical representations. In Encyclopedia of Mathematics Education; Lerman, S., Ed.; Springer: London, UK, 2014; pp. 409–413. [Google Scholar]
- Dreyfus, H.L. Intelligence without representation–Merleau-Ponty’s critique of mental representation the relevance of phenomenology to scientific explanation. Phenomenol. Cogn. Sci. 2002, 1, 367–383. [Google Scholar] [CrossRef]
- Rodriguez, F.; Gutiérrez, A. Analysis of proofs produced by university mathematics students, and the influence of using Cabri software. In Proceedings of the 30th Conference of the International Group for the Psychology of Mathematics Education, Prague, Czech Republic, 16–21 July 2006; Novotná, J., Moraová, H., Krátká, M., Stehlíková, N., Eds.; PME: Prague, Czech Republic, 2006; Volume 4, pp. 433–440. [Google Scholar]
- Haj-Yahya, A.; Hershkowitz, R. When visual and verbal representations meet-The case of geometrical figures. In Proceedings of the 37th Conference of the International Group for the Psychology of Mathematics Education, Kil, Germany, 28 July–2 August 20; Lindmeier, A.M., Heinze, A., Eds.; PME: Kil, Germany, 2013; Volume 2, pp. 409–416. [Google Scholar]
- Jones, K.; Fujita, T.; Kunimune, S. Representations and reasoning in 3-D geometry in lower secondary school. In Proceedings of the 36th Conference of the International Group for the Psychology of Mathematics Education, Taipei, Taiwan, 18–22 July 2011; Tso, T., Ed.; PME: Taipei, Taiwan, 2012; Volume 2, pp. 339–346. [Google Scholar]
- Ayala-Altamirano, C.; Molina, M. El proceso de generalización y la generalización en acto. Un estudio de casos. PNA 2021, 15, 211–241. [Google Scholar] [CrossRef]
- Mason, J. Expressing Generality and Roots of Algebra. In Approaches to Algebra, Perspectives for Research and Teaching; Bernarz, N., Kieran, C., Lee, L., Eds.; Springer: Dordrecht, The Netherlands, 1996; pp. 65–86. [Google Scholar]
- Balacheff, N. Aspects of proof in pupils’ practice of school mathematics. In Mathematics, Teachers and Children; Pimm, D., Ed.; Hodder and Stoughton: London, UK, 1988; pp. 216–235. [Google Scholar]
- Fiallo, J.; Gutiérrez, A. Analysis of the cognitive unity or rupture between conjecture and proof when learning to prove on a grade 10 trigonometry course. Educ. Stud. Math. 2017, 96, 145–167. [Google Scholar] [CrossRef]
- Mason, J.; Pimm, D. Generic examples: Seeing the general in the particular. Educ. Stud. Math. 1984, 15, 277–287. [Google Scholar] [CrossRef]
- Manouchehri, A.; Sriraman, B. Mathematical Cognition: In Secondary Years [13–18] Part 1. In Encyclopedia of Mathematics Education; Lerman, S., Ed.; Springer: London, UK, 2014; pp. 505–520. [Google Scholar]
- Swafford, J.O.; Langrall, C.W. Grade 6 students’ preinstructional use of equations to describe and represent problem situations. J. Res. Math. Educ. 2000, 31, 89–112. [Google Scholar] [CrossRef]
- Torres, M.D.; Moreno, A.; Cañadas, M.C. Generalization Process by Second Grade Students. Mathematics 2021, 9, 1109. [Google Scholar] [CrossRef]
- Greenes, C. Identifying the Gifted Student in Mathematics. Arith. Teach. 1981, 28, 14–17. [Google Scholar] [CrossRef]
- Miller, R.C. Discovering Mathematical Talent; Office of Educational Research and Improvement: Washington, DC, USA, 1990. [Google Scholar]
- Del Grande, J.J. Spatial sense. Arith. Teach. 1990, 37, 14–20. [Google Scholar] [CrossRef]
- Arcavi, A. The role of visual representations in the learning of mathematics. Educ. Stud. Math. 2003, 52, 215–241. [Google Scholar] [CrossRef]
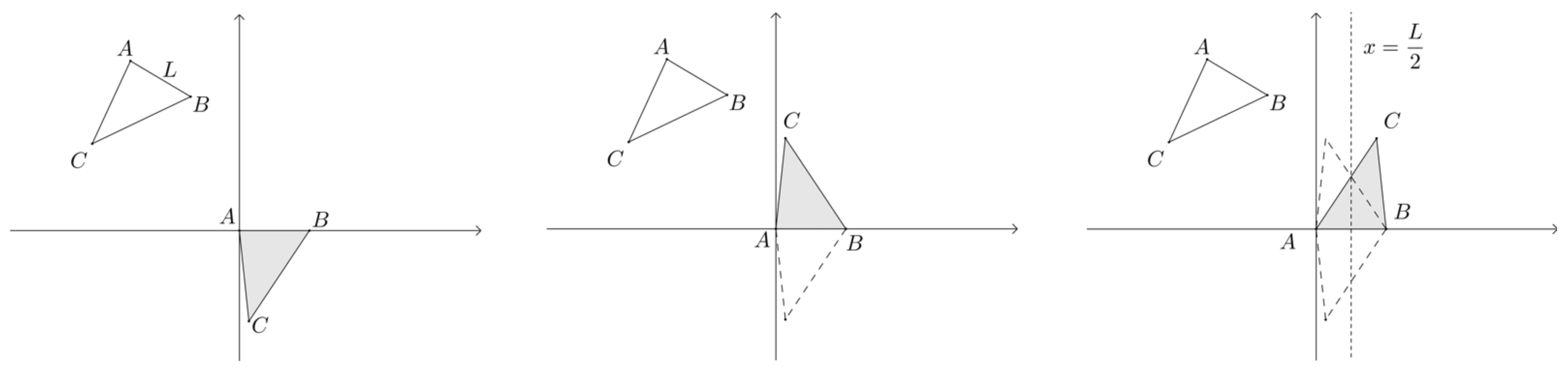

| Problem/Stage | Target/s | Description | Timing |
|---|---|---|---|
| 1/questionnaires | Proof with graphic and algebraic representation | Introduction to the lesson | Start |
| 1/group pooling | Compare proofs | S13: harmonic proof | Minute 29 |
| - | R1: visual proof 1 | - | |
| Use Geogebra to visualize proof elements | Pythagorean theorem in acute and obtuse triangles | Minute 35 | |
| - | Familiarity with Geogebra | Drag tool | - |
| 2/questionnaires | Proof with verbal representation | Explanation of homotheties and translation; definition of similarity | Minute 39 |
| 2/group pooling | Examples of isometric properties | S8’s answer | Minute 56 |
| - | R1: Isometry | - | |
| Distinction between proof and checking | Examples in Pythagorean triples | - | |
| - | Isometric properties | - | |
| - | Similarity in equilateral triangles R2: Distinction between proof and checking (example with paper triangle) | - | |
| 3/questionnaires | Proof with verbal representation and Geogebra | Perpendicular bisector and its properties Construction of perpendicular bisector with Geogebra (command and process) | Minute 69 |
| 3/group pooling | Compare proofs | S4’s proof | Minute 85 |
| - | S13’s rebuttal | - | |
| Break | - | - | - |
| 4/questionnaires | Proof with verbal representation and Geogebra | Shape-dependent properties Definition of circumcenter | Minute 100 |
| 4/group pooling | Compare proofs | S18’s answer | Minute 115 |
| Explanation of equivalence class | In-plane construction of the equivalence class of triangles | R1: Similarity with equivalent fractions R2: Construction process (using paper example and Geogebra) | Minute 119 |
| Use the equivalence class model with Geogebra | Model using Geogebra to manipulate | - | |
| 5/questionnaires | Proof using the equivalence class | Construction of circumcenter with Geogebra | Minute 140 |
| 5/group pooling | Compare proofs | S13’s answer (involving the inverse) S13’s reply (visual) Role of points as representatives in the equivalence class | Minute 165 |
| Student | Reasoning Style | Scope of Argumentation | Representation |
|---|---|---|---|
| S1 | Visual | General | Verbal |
| S2 | Harmonic | General | Algebraic and pictorial |
| S3 | Harmonic | Particular | Verbal and algebraic |
| S4 | Visual | Generalization | Verbal |
| S5 | Harmonic | Generalization | Verbal and algebraic |
| S6 | NA 1 | Generalization | Pictorial |
| S7 | Visual | Generalization | Verbal |
| S8 | Visual | Generalization | Verbal |
| S9 | NA | Generalization | Verbal |
| S10 | Visual | Particular | Pictorial |
| S11 | Visual | Generalization | Verbal |
| S12 | NA | Particular | - |
| S13 | Harmonic | Generalization | Verbal and algebraic |
| S14 | Visual | Generalization | Verbal |
| S15 | NA | Generalization | Verbal |
| S16 | Visual | Generalization | Verbal |
| S17 | Visual | Generalization | Verbal |
| S18 | Harmonic | Generalization | Verbal and algebraic |
| S19 | NA | Generalization | Verbal |
| S20 | NA | - | - |
| S21 | Visual | Generalization | Verbal |
| Student | Reasoning Style | Scope of Argumentation | Representation |
|---|---|---|---|
| S1 | Visual | Particular | Pictorial |
| S2 | Visual | Generalization | Verbal |
| S4 | Visual | Generalization | Verbal |
| S5 | Visual | Generalization | Verbal |
| S6 | Visual | Generalization | Verbal |
| S8 | Visual | Generalization | Verbal |
| S9 | Visual | Generalization | Verbal |
| S10 | Visual | Generalization | Verbal and algebraic |
| S11 | Visual | Generalization | Verbal and algebraic |
| S13 | Visual | Generalization | Verbal |
| S14 | Visual | Generalization | Verbal |
| S15 | Visual | Generalization | Verbal |
| S16 | Visual | Generalization | Verbal |
| S17 | Visual | Generalization | Verbal |
| S18 | Visual | Generalization | Verbal |
| S19 | Visual | Generalization | Verbal |
| Student | Reasoning Style | Scope of Argumentation | Representation |
|---|---|---|---|
| S2 | Counter examples | - | Algebraic and pictorial |
| S4 | Analytical | Generalization | Verbal |
| S6 | Counter examples | - | Pictorial |
| S8 | Counter examples | - | Pictorial |
| S9 | Counter examples | - | Pictorial |
| S10 | Counter examples | - | Pictorial |
| S11 | Counter examples | Set | Verbal |
| S13 | Counter examples | Set | Verbal |
| S16 | Counter examples | - | Verbal |
| S17 | Counter examples | - | Verbal |
| S18 | Counter examples | - | Pictorial |
| S19 | Counter examples | - | Pictorial |
| S21 | Counter examples | - | Pictorial |
| Student | Reasoning Style | Scope of Argumentation | Representation |
|---|---|---|---|
| S4 | Analytical | Generalization | Algebraic and pictorial |
| S6 | Analytical | Generalization | Verbal |
| S8 | Visual | Set | Pictorial |
| S9 | NA 1 | - | - |
| S10 | Analytical | - | Verbal |
| S11 | Analytical | Generalization | Verbal |
| S13 | Visual | Generalization | Pictorial |
| S15 | Analytical | Generalization | Verbal |
| S16 | Analytical | Generalization | Verbal |
| S18 | Analytical | Generalization | Verbal |
| S18 | Analytical | Generalization | Verbal |
| Student | Reasoning Style | Scope of Argumentation | Representation |
|---|---|---|---|
| S4 | Visual | Generalization | Verbal |
| S5 | Visual | Set | Verbal |
| S8 | Visual | Set | Verbal |
| S9 | Visual | Generalization | Verbal |
| S13 | Visual | Set | Verbal |
| S15 | Visual | Generalization | Verbal |
| S16 | Visual | Generalization | Verbal |
| S18 | Visual | Set | Verbal |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ramírez-Uclés, R.; Ruiz-Hidalgo, J.F. Reasoning, Representing, and Generalizing in Geometric Proof Problems among 8th Grade Talented Students. Mathematics 2022, 10, 789. https://doi.org/10.3390/math10050789
Ramírez-Uclés R, Ruiz-Hidalgo JF. Reasoning, Representing, and Generalizing in Geometric Proof Problems among 8th Grade Talented Students. Mathematics. 2022; 10(5):789. https://doi.org/10.3390/math10050789
Chicago/Turabian StyleRamírez-Uclés, Rafael, and Juan F. Ruiz-Hidalgo. 2022. "Reasoning, Representing, and Generalizing in Geometric Proof Problems among 8th Grade Talented Students" Mathematics 10, no. 5: 789. https://doi.org/10.3390/math10050789
APA StyleRamírez-Uclés, R., & Ruiz-Hidalgo, J. F. (2022). Reasoning, Representing, and Generalizing in Geometric Proof Problems among 8th Grade Talented Students. Mathematics, 10(5), 789. https://doi.org/10.3390/math10050789






