Numerical Solutions to Wave Propagation and Heat Transfer Non-Linear PDEs by Using a Meshless Method
Abstract
:1. Introduction
2. GFDM and Newton–Raphson Method
2.1. Explicit Finite Difference Formulae
2.2. Newton–Raphson Method (N–R)
- When a given tolerance is reached, in this paper : .
- When 15 iterations are attained.
3. Numerical Examples for Eikonal Equation
3.1. Numerical Test 1
3.2. Numerical Test 2
4. Numerical Examples for Stationary Non-Linear Heat Transfer Equation
4.1. Numerical Test 3
4.2. Numerical Test 4
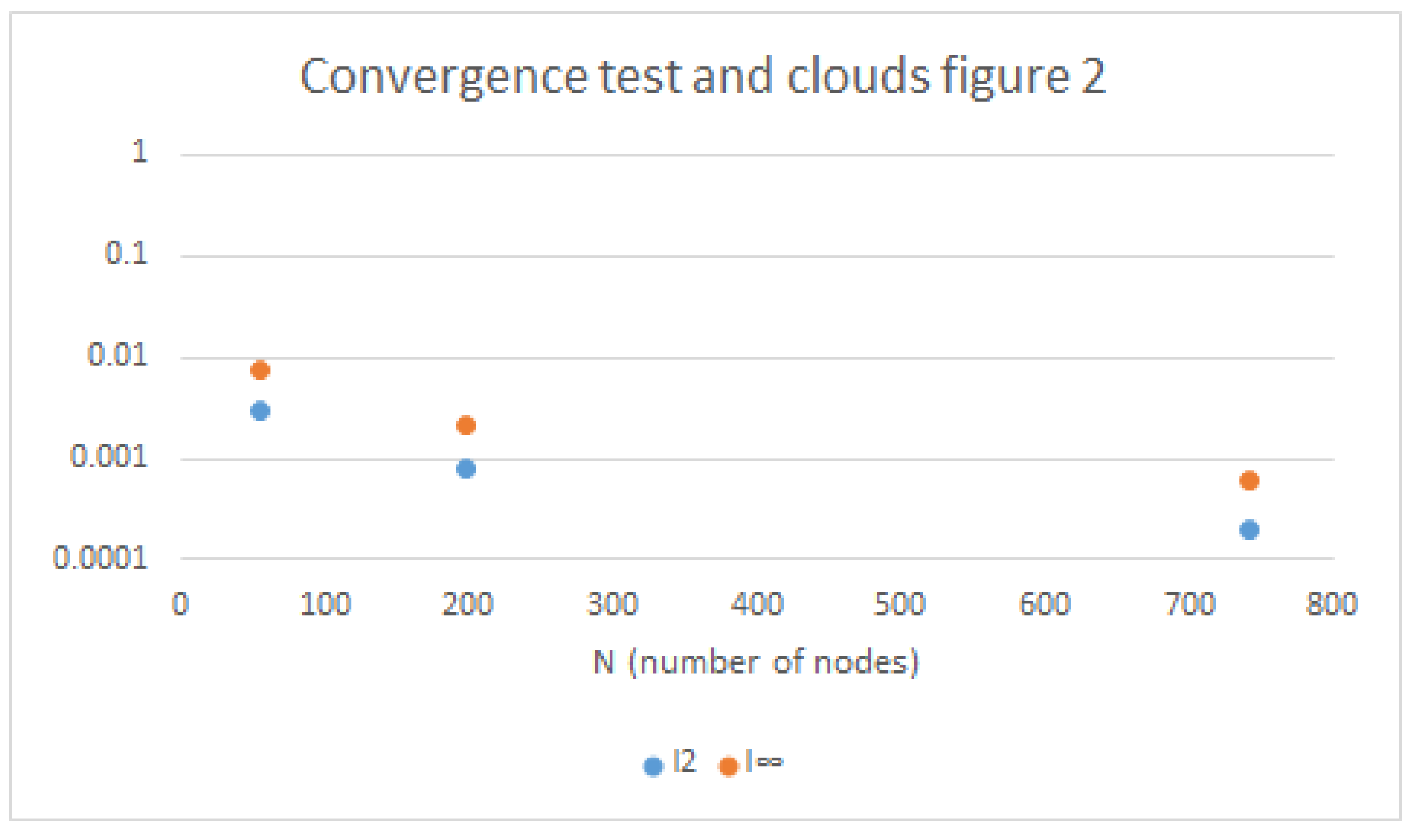
4.3. Convergence Test
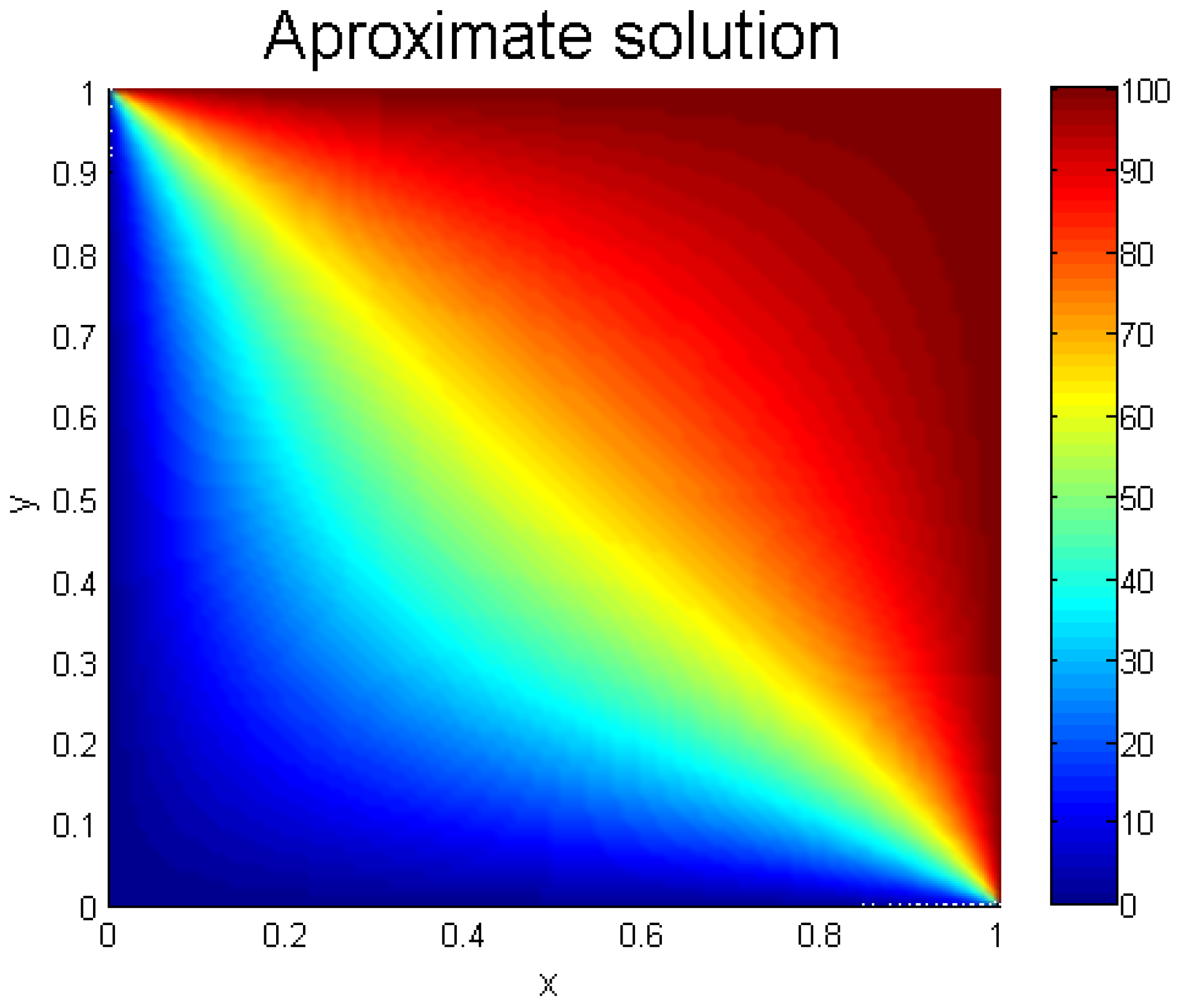
5. Example 5: Physical Example
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Courant, R.; Friedrichs, K.; Lewy, H. On the partial differential eqautions or mathematical physic. Math. Ann. 1928, 100, 32–74. [Google Scholar] [CrossRef]
- Tadmor, A. A review of numerical methods for non-linear partial differential eqautions. Bull. Am. Mthematical Soc. 2012, 42, 507–554. [Google Scholar] [CrossRef] [Green Version]
- Zhao, H. A Fast sweeping Method for Eikonal Equation. Math. Comput. 2004, 74, 603–627. [Google Scholar] [CrossRef] [Green Version]
- Dehghan, M.; Salehi, R. A boundary-only meshless method for numerical solution of the Eikonal equation. Comput. Mech. 2011, 47, 283–294. [Google Scholar] [CrossRef]
- Mehr, M.; Rostamy, D. Computational solutions for Eikonal equation by differential quadrature method. Alex. Eng. J. 2021, 61, 4445–4455. [Google Scholar] [CrossRef]
- Ahmed, S.; Bak, S.; McLaughlin, J.R.; Renzi, D. A Third Order Accurate Fast Marching Method for the Eikonal Equation in Two Dimensions. SIAM J. Sci. Comput. 2011, 33, 2402–2420. [Google Scholar] [CrossRef] [Green Version]
- Cristiani, E.; Falcone, M. Fast Semi-Lagrangian Schemes for the Eikonal Equation and Applications. SIAM J. Numer. Anal. 2007, 45, 1979–2011. [Google Scholar] [CrossRef]
- Crank, J.; Nicolson, E. A practical method for numerical evaluation of solutions of partial differential equations of the heat-conduction type. Adv. Comput. Math. 1996, 6, 207–226. [Google Scholar] [CrossRef]
- Khani, F.; Raji, M.A.; Nejad, H.H. Analytical solutions and efficiency of the nonlinear fin problem with temperature-dependent thermal conductivity and heat transfer coefficient. Commun. Nonlinear Sci. Numer. Simul. 2009, 14, 3327–3338. [Google Scholar] [CrossRef]
- Mokheimer, E.M.A. Heat transfer from extended surfaces subject to variable heat transfer coefficient. Heat Mass Transf. 2003, 39, 131–138. [Google Scholar] [CrossRef]
- Ma, S.W.; Behbahani, A.I.; Tsuei, Y.G. Two-dimensional rectangular fin with variable heat transfer coefficient. Int. J. Heat Mass Transf. 1991, 34, 79–85. [Google Scholar] [CrossRef]
- Gorgisheli, S.; Mrevlishvili, M.; Natroshvili, D. Localized Boundary-Domain Integro-Differential Equation Approach for Stationary Heat Transfer Equation. In Applications of Mathematics and Informatics in Natural Sciences and Engineering; Jaiani, G., Natroshvili, D., Eds.; AMINSE 2019; Springer Proceedings in Mathematics & Statistics; Springer: Cham, Switzerland, 2019; Volume 334. [Google Scholar] [CrossRef]
- Taler, J.; Duda, P. Solving Steady-State Heat Conduction Problems by Means of Numerical Methods. In Solving Direct and Inverse Heat Conduction Problems; Springer: Berlin/Heidelberg, Germany, 2006. [Google Scholar] [CrossRef]
- Shokri, A.; Dehghan, M. A Meshless Method Using Radial Basis Functions for the Numerical Solution of Two-Dimensional Complex Ginzburg-Landau Equation. Comput. Model. Eng. Sci. 2012, 84, 333–358. [Google Scholar]
- Aslefallah, M.; Abbasbandy, S.; Shivanian, E. Numerical Solution of a modified anomalous diffusion equation with nonlinear source term through meshless singular boundary method. Eng. Anal. Bound. Elem. 2019, 107, 198–207. [Google Scholar] [CrossRef]
- Liszka, T.; Orkisz, J. The finite difference method at arbitrary irregular grids and its application in applied mechanics. Comput. Struct. 1980, 11, 83–95. [Google Scholar] [CrossRef]
- Benito, J.J.; Ure na, F.; Gavete, L. Influence of several factors in the generalized finite difference method. Appl. Math. Model. 2001, 25, 1039–1053. [Google Scholar] [CrossRef]
- Vargas, A.M. Finite difference method for solving fractional differential equations at irregular meshes. Math. Comput. Simul. 2022, 193, 204–216. [Google Scholar] [CrossRef]
- Salete, E.; Vargas, A.M.; García, A.; Benito, J.J.; Ureña, F.; Ureña, M. An effective numeric method for different formulations of the elastic wave propagation problem in isotropic medium. Appl. Math. Model. 2021, 96, 480–496. [Google Scholar] [CrossRef]
- Benito, J.J.; García, A.; Gavete, L.; Negreanu, M.; Urena, F.; Vargas, A.M. On the numerical solution to a parabolic-elliptic system with chemotactic and periodic terms using Generalized Finite Differences. Eng. Anal. Bound. Elem. 2020, 113, 181–190. [Google Scholar] [CrossRef]
- Benito, J.J.; Urena, F.; Gavete, L. Solving parabolic and hyperbolic equations by the generalized finite difference method. J. Comput. Appl. Math. 2007, 209, 208–233. [Google Scholar] [CrossRef] [Green Version]

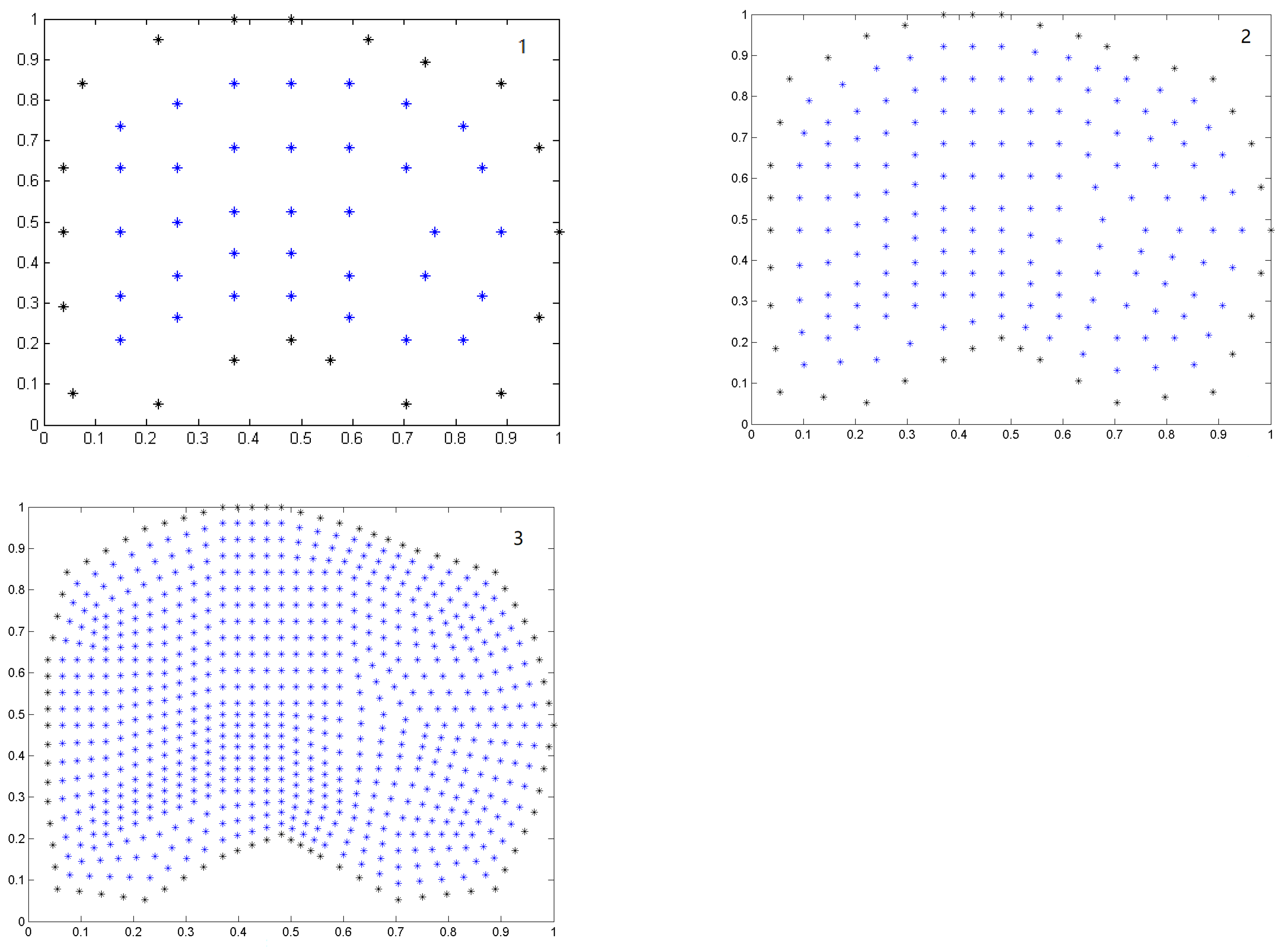
| Cloud of Nodes | Iterations Number | ||
|---|---|---|---|
| Cloud 1 | 2.1354 × 10 | 5.2347 × 10 | 8 |
| Cloud 2 | 5.3784 × 10 | 9.2317 × 10 | 6 |
| Cloud 3 | 6.2513 × 10 | 1.0032 × 10 | 8 |
| Cloud 4 | 1.2231 × 10 | 4.1256 × 10 | 7 |
| Cloud of Nodes | Iterations Number | ||
|---|---|---|---|
| Cloud 1 | 7.4231 × 10 | 2.5717 × 10 | 9 |
| Cloud 2 | 9.3487 × 10 | 4.0196 × 10 | 8 |
| Cloud 3 | 5.2066 × 10 | 8.9902 × 10 | 8 |
| Cloud 4 | 1.2231 × 10 | 5.7086 × 10 | 7 |
| Cloud of Nodes | ||
|---|---|---|
| Cloud 1 | 1.3154 × 10 | 3.4247 × 10 |
| Cloud 2 | 3.33487 × 10 | 5.2914 × 10 |
| Cloud 3 | 5.6244 × 10 | 8.3201 × 10 |
| Cloud 4 | 8.1232 × 10 | 1.2572 × 10 |
| Cloud of Nodes | ||
|---|---|---|
| Cloud 1 | 4.2578 × 10 | 6.7241 × 10 |
| Cloud 2 | 7.1378 × 10 | 1.0058 × 10 |
| Cloud 3 | 8.3254 × 10 | 1.9231 × 10 |
| Cloud 4 | 1.9288 × 10 | 3.4255 × 10 |
| Number of Nodes | ||
|---|---|---|
| 55 | 0.002973 | 0.007653 |
| 197 | 0.000810 | 0.002129 |
| 743 | 0.000206 | 0.000608 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Flores, J.; García, Á.; Negreanu, M.; Salete, E.; Ureña, F.; Vargas, A.M. Numerical Solutions to Wave Propagation and Heat Transfer Non-Linear PDEs by Using a Meshless Method. Mathematics 2022, 10, 332. https://doi.org/10.3390/math10030332
Flores J, García Á, Negreanu M, Salete E, Ureña F, Vargas AM. Numerical Solutions to Wave Propagation and Heat Transfer Non-Linear PDEs by Using a Meshless Method. Mathematics. 2022; 10(3):332. https://doi.org/10.3390/math10030332
Chicago/Turabian StyleFlores, Jesús, Ángel García, Mihaela Negreanu, Eduardo Salete, Francisco Ureña, and Antonio M. Vargas. 2022. "Numerical Solutions to Wave Propagation and Heat Transfer Non-Linear PDEs by Using a Meshless Method" Mathematics 10, no. 3: 332. https://doi.org/10.3390/math10030332
APA StyleFlores, J., García, Á., Negreanu, M., Salete, E., Ureña, F., & Vargas, A. M. (2022). Numerical Solutions to Wave Propagation and Heat Transfer Non-Linear PDEs by Using a Meshless Method. Mathematics, 10(3), 332. https://doi.org/10.3390/math10030332






