Two-Parameter Exponentially Fitted Taylor Method for Oscillatory/Periodic Problems

Abstract

This work presents the construction and implementation of a two-parameter exponentially fitted Taylor method suitable for solving ordinary differential equations that possess oscillatory or periodic behaviour. The methodology is based on a six-step algorithm discussed in the literature. We present the associated truncation error of the method and demonstrate its accuracy using two test cases. The method gave better results compared with its counterparts discussed in the literature.

1. Introduction

One important and interesting class of initial value problems that often arise in practice consists of ordinary differential equations (ODEs), whose solutions are periodic or oscillate with known frequencies. Usually, classical numerical methods produce less accurate results when applied to such problems but they can be adapted to efficiently give better accuracy [1,2,3]. This adaptation is the core of the exponential fitting technique [3,4,5,6,7]. The adapted classical method is modified such that it is exact for the problem whose solution is a linear combination of

$$\begin{array}{c}\hfill 1,t,\dots ,t^K,exp(\pm \omega t),texp(\pm \omega t),\dots ,t^{P}exp(\pm \omega t),\end{array}$$

(1)

where K and P are integers. The adaptation of several classical methods for problems with the fitting space (1) has gained popularity in recent times [5,7,8,9,10,11,12,13]. However, very little has been performed in the construction of exponentially fitted methods for fitting space with multiple frequencies [3,6,14]. Authors in Refs. [15,16,17] constructed a Runge–Kutta-type method for the fitting space

$$\begin{array}{c}\hfill 1,t,\dots ,t^K,exp(\pm \omega t),exp(\pm 2\omega t),\dots ,exp(\pm (P+1)\omega t).\end{array}$$

(2)

In Ref. [6], a multiparameter exponentially fitted Numerov method for the space

$$\begin{array}{c}\hfill 1,t,\cdots ,t^K,exp(\pm {\omega }_{0}t),exp(\pm {\omega }_{1}t),\cdots ,exp(\pm {\omega }_{p}t),\end{array}$$

(3)

for solving periodic problems with more than one frequency was proposed. This work is related to Refs. [6,12,18] and shall provide extension by the construction of a two-parameter exponentially fitted Taylor method for the fitting space

$$\left.\begin{array}{c}\hfill 1,t,\dots ,t^K,exp(\pm {\omega }_{i}t),texp(\pm {\omega }_{i}t),\dots ,t^{P_i}exp(\pm {\omega }_{i}t),\\ \hfill i=1,2.\end{array}\right\}$$

(4)

This work is justified by the fact that there are many problems whose solution lies within the fitting space (4).

2. Construction of Method

The general r-th-order Taylor scheme is given as

$$\begin{array}{c}\hfill u_{j+1}=u_j+h{u}_j^{\prime }+\frac{1}{2}{h}^2{u}_j^{″}+\cdots +\frac{1}{r!}{h}^r{u}_j^{\left(r\right)}.\end{array}$$

(5)

We set $r=4$ in (5) to obtain the classical fourth-order Taylor method (6)

$$\begin{array}{c}\hfill u_{j+1}=u_j+h{u}_j^{\prime }+\frac{1}{2}{h}^2{u}_j^{″}+\cdots +\frac{1}{4!}{h}^4{u}_j^{\left(4\right)},\end{array}$$

(6)

to be fitted exponentially in this work. Following the six-step algorithm proposed in Ref. [3], the base method (6) is first written in a more general form as

$$\begin{array}{c}\hfill u_{j+1}={\alpha }_0{u}_j+{\beta }_{1}h{u}_j^{\prime }+{\beta }_2{h}^2{u}_j^{″}+{\beta }_3{h}^3{u}_j^{\left(3\right)}+{\beta }_4{h}^4{u}_j^{\left(4\right)},\end{array}$$

(7)

and the associated linear difference operator $\mathcal{L}[h,\mathbf{a}]$ is given as

$$\begin{array}{ccc}\hfill \mathcal{L}[h,\mathbf{a}]u\left(t\right)& =& u(t+h)-{\alpha }_{0}u\left(t\right)-{\beta }_{1}h{u}^{\prime }\left(t\right)-{\beta }_2{h}^2{u}^{″}\left(t\right)-{\beta }_3{h}^3{u}^{\left(3\right)}\left(t\right)-{\beta }_4{h}^4{u}^{\left(4\right)}\left(t\right),\hfill \end{array}$$

where $\mathbf{a}:=({\alpha }_0,{\beta }_1,{\beta }_2,{\beta }_3,{\beta }_4)$. Next, we determine the maximum value of M that makes the system

$$\begin{array}{ccc}\hfill L_m^{*}\left(\mathbf{a}\right)& =& h^{-m}\mathcal{L}[h,\mathbf{a}]t^m{|}_{t=0}=0|m=0,1,2,\cdots M-1\hfill \end{array}$$

(8)

compatible. The system obtained from (8) is given as

$$\begin{array}{ccc}\hfill L_0^{*}\left(\mathbf{a}\right)& =& 1-{\alpha }_0=0,\hfill \end{array}$$

(9)

$$\begin{array}{ccc}\hfill L_1^{*}\left(\mathbf{a}\right)& =& 1-{\beta }_1=0,\hfill \end{array}$$

(10)

$$\begin{array}{ccc}\hfill L_2^{*}\left(\mathbf{a}\right)& =& 1-2{\beta }_2=0,\hfill \end{array}$$

(11)

$$\begin{array}{ccc}\hfill L_3^{*}\left(\mathbf{a}\right)& =& 1-6{\beta }_3=0,\hfill \end{array}$$

(12)

$$\begin{array}{ccc}\hfill L_4^{*}\left(\mathbf{a}\right)& =& 1-24{\beta }_4=0,\hfill \end{array}$$

(13)

and is compatible with $M=5$. Solving (9) yields the coefficient of the well-known classical fourth-order Taylor method here referred to as S0. In order to fit (6) exponentially, a six-step algorithm requires that we obtain the expressions for $G^{+}(Z_i,\mathbf{a})$ and $G^{-}(Z_i,\mathbf{a})$, defined, respectively, as

$$\begin{array}{ccc}\hfill G^{+}(Z_i,\mathbf{a})& =& \frac{1}{2}\left(E_0^{*}(z_i,\mathbf{a})+E_0^{*}(-z_i,\mathbf{a})\right),\hfill \end{array}$$

(14)

$$\begin{array}{ccc}\hfill G^{-}(Z_i,\mathbf{a})& =& \frac{1}{2z_i}\left(E_0^{*}(z_i,\mathbf{a})-E_0^{*}(-z_i,\mathbf{a})\right),\hfill \end{array}$$

(15)

where

$$E_0^{*}(\pm z_i,\mathbf{a})=e^{\mp {\omega }_{i}t}\mathcal{L}[h,\mathbf{a}]e^{\pm {\omega }_{i}t}$$

and

$$Z_i=z_i^2,z_i={\omega }_{h_i}={\omega }_{i}h,i=1,2.$$

Now, the respective expressions for $G^{+}(Z_i,\mathbf{a})$ and $G^{-}(Z_i,\mathbf{a})$ are obtained as

$$\begin{array}{ccc}\hfill G^{+}(Z_i,\mathbf{a})& =& -{\alpha }_0-{\beta }_4{\omega }_{h_i}^4-{\beta }_2{\omega }_{h_i}^2+cosh\left({\omega }_{h_i}\right)\hfill \\ \hfill G^{-}(Z_i,\mathbf{a})& =& -{\beta }_1-{\beta }_3{\omega }_{h_i}^2+\frac{sinh\left({\omega }_{h_i}\right)}{{\omega }_{h_i}},\hfill \end{array}$$

where ${\omega }_i$, the frequencies of oscillation, are real or imaginary. Considering the general fitting space (4) with $M=5$, the algorithm gave rise to a two-parameter exponentially fitted variant of (6) referred to as EF2PT and characterized by:

$EF2PT:(K,P_1,P_2)=(0,0,0)$:

The two-parameter exponentially fitted case with the set

$$\left\{1,exp(\pm {\omega }_{1}t),exp(\pm {\omega }_{2}t)\right\}.$$

By solving the nonlinear algebraic system (16), the coefficients of the two-parameter exponentially fitted variant are given in (17)

$$\begin{array}{c}\hfill \left.\begin{array}{c}\hfill 1-{\alpha }_0=0\\ \hfill -{\alpha }_0-{\beta }_4{\omega }_{h_1}^4-{\beta }_2{\omega }_{h_1}^2+cosh\left({\omega }_{h_1}\right)=0\\ \hfill -{\beta }_1-{\beta }_3{\omega }_{h_1}^2+\frac{sinh\left({\omega }_{h_1}\right)}{{\omega }_{h_1}}=0\\ \hfill -{\alpha }_0-{\beta }_4{\omega }_{h_2}^4-{\beta }_2{\omega }_{h_2}^2+cosh\left({\omega }_{h_2}\right)=0\\ \hfill -{\beta }_1-{\beta }_3{\omega }_{h_2}^2+\frac{sinh\left({\omega }_{h_2}\right)}{{\omega }_{h_2}}=0\end{array}\right\}\end{array}$$

(16)

$$\begin{array}{c}\hfill \left.\begin{array}{c}{\alpha }_0=1\hfill \\ {\beta }_1=\frac{Z_1^{3/2}sinh\left(\sqrt{\left|Z_2\right|}\right)-Z_2^{3/2}sinh\left(\sqrt{\left|Z_1\right|}\right)}{Z_1^{3/2}\sqrt{\left|Z_2\right|}-Z_2^{3/2}\sqrt{\left|Z_1\right|}}\hfill \\ {\beta }_2=\frac{Z_1^2\left(cosh\left(\sqrt{\left|Z_2\right|}\right)-1\right)-Z_2^2\left(cosh\left(\sqrt{\left|Z_1\right|}\right)-1\right)}{Z_1\left(Z_1-Z_2\right)Z_2}\hfill \\ {\beta }_3=\frac{\sqrt{\left|Z_2\right|}sinh\left(\sqrt{\left|Z_1\right|}\right)-\sqrt{\left|Z_1\right|}sinh\left(\sqrt{\left|Z_2\right|}\right)}{Z_1^{3/2}\sqrt{\left|Z_2\right|}-Z_2^{3/2}\sqrt{\left|Z_1\right|}}\hfill \\ {\beta }_4=\frac{Z_2\left(cosh\left(\sqrt{\left|Z_1\right|}\right)-1\right)-Z_1\left(cosh\left(\sqrt{\left|Z_2\right|}\right)-1\right)}{Z_1\left(Z_1-Z_2\right)Z_2}\hfill \end{array}\right\}\end{array}$$

(17)

As expected, the exponentially fitted variant reduced to the classical method as $Z\to 0$.

3. Error Analysis: Local Truncation Error (LTE)

The leading term of the local truncation error (lte) for an exponentially fitted method with respect to the basis (4) is of the form

$$\begin{array}{ccc}\hfill LTE\left(t\right)& =& {(-1)}^{{\sum }_{i=1}^I{P}_i+I}{h}^M\frac{{\mathcal{L}}_{K+1}^{*}\left(\mathbf{a}\left(Z_i\right)\right)}{(K+1)!Z_1^{P_1+1}\cdots Z_I^{P_I+1}}\times \hfill \\ & & \prod _{i=1}^I{(D^2-{\omega }_i^2)}^{P_i+1},D^m:=\frac{d^m}{d{t}^m}\hfill \end{array}$$

(18)

with $K,P_1,\cdots ,P_I$ and M satisfying the condition $K+2(P_1+\cdots +P_I)=M-2I-1$ [14]. Using (18), the local truncation error in our exponentially fitted method is obtained as:

$$\begin{array}{ccc}\hfill LTE& =& h^5\frac{\left({\beta }_1-1\right)\left(u^{\left(5\right)}\left(t\right)-{\omega }_2^2{u}^{\left(3\right)}\left(t\right)+{\omega }_1^2\left({\omega }_2^2{u}^{\prime }\left(t\right)-u^{\left(3\right)}\left(t\right)\right)\right)}{Z_1{Z}_2}\hfill \end{array}$$

(19)

4. Convergence and Stability Analysis

Theorem 1

(Dahlquist Theorem). The necessary and sufficient conditions for a linear multistep method to be convergent are that it be consistent and zero-stable [19].

For exponentially fitted algorithms, the Dahlquist Theorem (1) also holds true; however, the concepts of consistency and stability have to be adapted since their coefficients are no longer constants.

Definition 1.

An exponentially fitted method is said to be of exponential order q if q is the maximum value of M, such that the algebraic system $\left\{{\mathcal{L}}_m^{*}\left(\mathbf{a}\right)=0|m=0,\cdots ,M-1\right\}$ is compatible [3].

Definition 2.

A linear multistep method is said to be consistent if it has order $\mathcal{P}\ge 1$ [1].

Since our proposed method, EF2PT, is of order $M=5\ge 1$, the consistency requirement is satisfied. Hence, the constructed scheme is consistent.

Definition 3.

The method, EF2PT, is zero-stable if no root of the first characteristic polynomial has a modulus greater than one and if every root with modulus one is simple [20].

In order to establish the stability of EF2PT, we apply it to the test problems $y^{\prime }=\lambda y$ and obtain the stability function $R\left(q\right)$ of the class of methods as

$$\begin{array}{c}\hfill \frac{y_{n+1}}{y_n}=R\left(q\right)={\alpha }_0+{\beta }_{0}q+{\beta }_1{q}^2+{\beta }_3{q}^3+{\beta }_4{q}^4,\mathrm{with}q=\lambda h.\end{array}$$

(20)

Definition 4.

A region of absolute stability is a region in the complex plane, throughout which $\left|R\left(q\right)\right|<1$. Any closed curve defined by $\left|R\left(q\right)\right|=1$ is an absolute stability boundary. In addition, any interval $(\alpha ,\beta )$ of the real line is said to be the interval of absolute stability if the method is stable for all $q\in (\alpha ,\beta )$ [1,19].

The absolute stability region for the method constructed in this work is given in Figure 1. It has an absolute stability interval of $\left(-2.79,0\right]$.

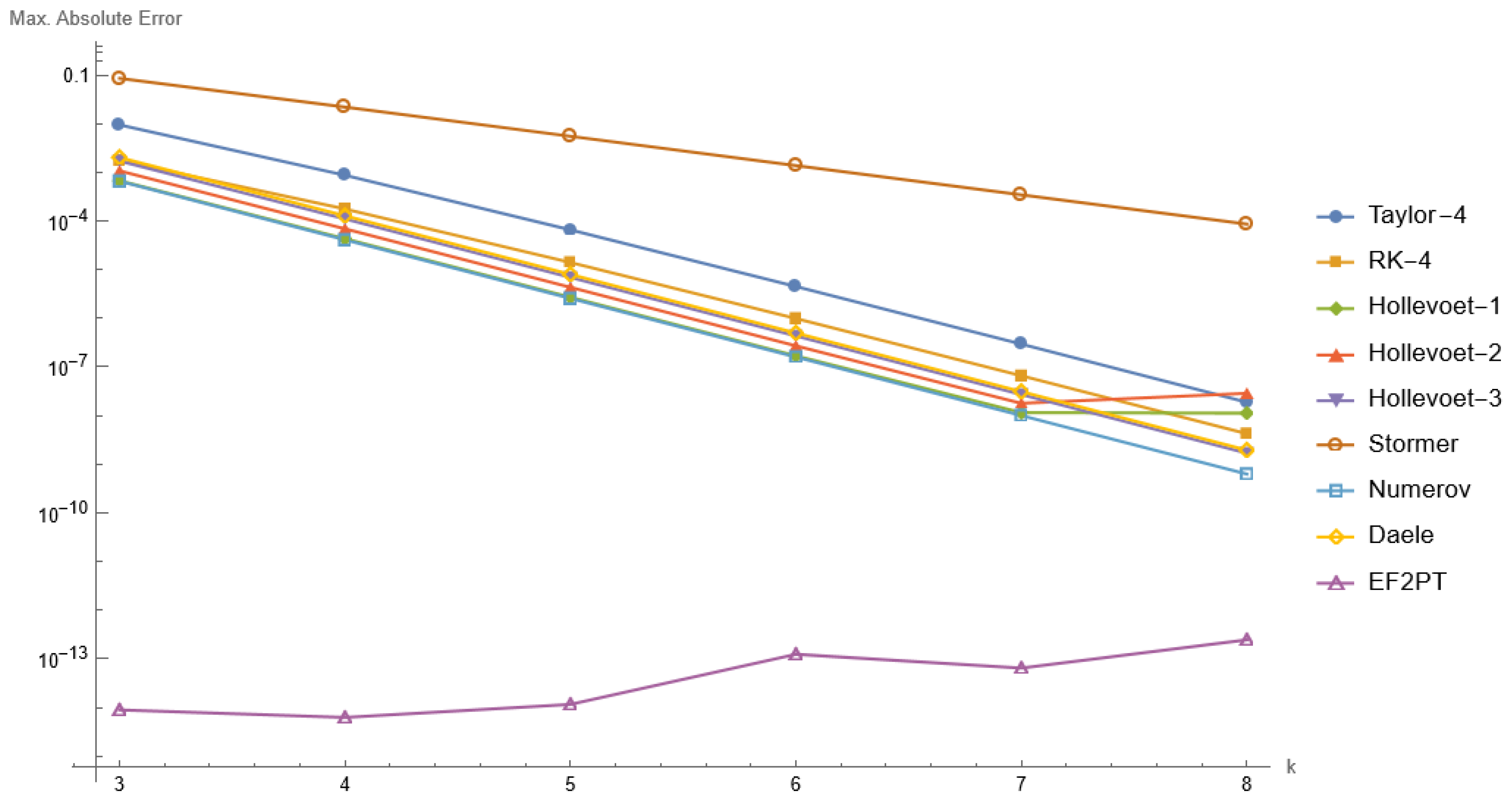
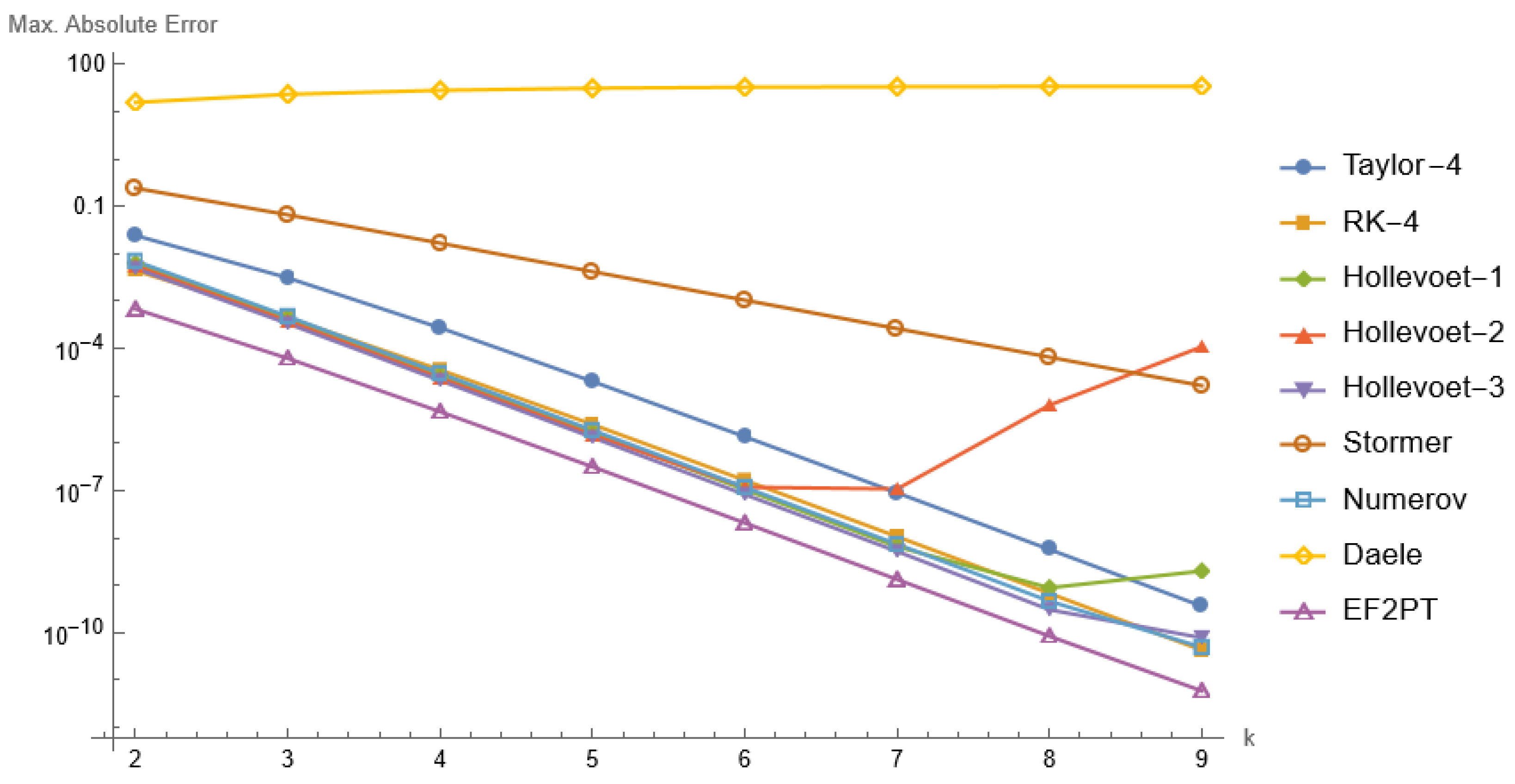
5. Numerical Results

Here, we demonstrate the performance of our method, referred to as EF2PT, using three standard test problems. We implement our method on these test problems and compare the obtained results with those of the classical Taylor, Runge–Kutta, Stomer and Numerov methods, which are methods proposed by the authors in Ref. [21], hereby referred to as Hollevoet-1, Hollevoet-2 and Hollevoet-3. A comparison with the method proposed in Ref. [6] was also carried out.

5.1. Problem 1

Consider the initial value problem given as

$$\begin{array}{ccc}\hfill u^{″}& =& \frac{3}{4}u-exp\left(t\right)sin\left(\frac{t}{2}\right),u\left(0\right)=1,u^{\prime }\left(0\right)=1\hfill \end{array}$$

(21)

whose exact solution is

$$\begin{array}{c}\hfill u\left(t\right)=exp\left(t\right)cos\left(\frac{t}{2}\right)\end{array}$$

(22)

Problem (21) has two complex conjugate frequencies, which are ${\omega }_1=1+\frac{1}{2}i$ and ${\omega }_2=1-\frac{1}{2}i$, and have been studied by Refs. [6,12,21]. Here, we solve this problem using a different steplength h on the interval $[0,\pi ]$, and the maximum absolute error for each steplength is obtained and presented in Figure 2.

Our method performed better compared with the methods discussed in the literature, as seen in Figure 2.

5.2. Problem 2

Consider the equation

$$\begin{array}{c}\hfill y^{″}=y+2exp\left(t\right)-8exp\left(3t\right),y\left(0\right)=-1,y\left(1\right)=exp\left(1\right)-exp\left(3\right)\end{array}$$

(23)

with exact solution

$$\begin{array}{c}\hfill y\left(t\right)=texp\left(t\right)-exp\left(3t\right).\end{array}$$

(24)

This problem was also solved using different stepsizes on the interval $[0,1]$, although the solution lies outside the fitting space of our method; however, our method gave good results compared with its counterparts, as seen in Figure 3.

6. Conclusions

The two-parameter exponentially fitted Taylor method constructed in this work is of algebraic order four and is self-starting. Its local truncation error is of order five. Compared with its classical counterparts, the results obtained from the numerical example showed that the new method is suitable for solving periodic/oscillatory problems.