Hermite Method of Approximate Particular Solutions for Solving Time-Dependent Convection-Diffusion-Reaction Problems

Abstract

This article describes the development of the Hermite method of approximate particular solutions (MAPS) to solve time-dependent convection-diffusion-reaction problems. Using the Crank-Nicholson or the Adams-Moulton method, the time-dependent convection-diffusion-reaction problem is converted into time-independent convection-diffusion-reaction problems for consequent time steps. At each time step, the source term of the time-independent convection-diffusion-reaction problem is approximated by the multiquadric (MQ) particular solution of the biharmonic operator. This is inspired by the Hermite radial basis function collocation method (RBFCM) and traditional MAPS. Therefore, the resultant system matrix is symmetric. Comparisons are made for the solutions of the traditional/Hermite MAPS and RBFCM. The results demonstrate that the Hermite MAPS is the most accurate and stable one for the shape parameter. Finally, the proposed method is applied for solving a nonlinear time-dependent convection-diffusion-reaction problem.

1. Introduction

The convection-diffusion-reaction model is one of the most frequently used models in science and engineering [1]. Applications include biological modelling, chemical reaction and transportation, evolutions of substance in media, transport phenomena in granular flows, mixing of fluids, air pollution simulations, options pricing modelling and other partial differential equations (PDEs) [2,3,4]. Furthermore, convection-diffusion-reaction problems have been numerically studied by the finite difference method [5], finite element method [6], finite volume method [7], boundary element method [8], etc. In addition, meshless numerical methods have been applied to solve convection-diffusion problems [3,9,10,11,12,13,14,15]. In this study, a meshless numerical method is proposed to solve the above-mentioned problem with improved stability and accuracy.

Many meshless numerical methods are based on the radial basis functions (RBF), which are traditionally used for reconstructing scattered data [16,17]. Using the multiquadric (MQ) RBF, the traditional radial basis function collocation method (RBFCM) was developed by Kansa [18] for solving some PDEs. It is well known that the traditional RBFCM is meshless, spectrally accurate and easily implemented for solving different PDEs. However, it has been reported that the traditional RBFCM resulted in singular matrices for some extreme cases [19], and therefore Fasshauer [20] developed the Hermite RBFCM to improve the stability of the numerical solutions. Sequentially, comparisons between the Hermite and traditional RBFCM were made by Power and Barraco [21]. In that study, they found that the Hermite RBFCM was more stable for the shape parameter and the traditional RBFCM was simpler for implementation. Both the traditional [10,22] and Hermite [9,11,12] RBFCMs have successfully solved many engineering and science problems. Additionally, Stevens et al. [23] developed a localized version of the Hermite RBFCM and demonstrated a much-improved reconstruction of partial derivatives.

To improve the numerical accuracy of the RBFCM, Chen and his colleagues [24] proposed the method of approximate particular solutions (MAPS), where the MQ particular solution of the harmonic operator is directly used to approximate the sought solution. Since the traditional MAPS contains some information from the governing operator, it was demonstrated that the traditional MAPS was more accurate than the traditional RBFCM [25,26]. The MQ particular solutions of certain partial differential operators can be found in the literature [26,27]. The proposed method is also utilized to obtain the particular solutions in the applications of boundary-type numerical methods [28,29]. In the traditional MAPS, the source term of the considered PDE is approximated by the MQs. Successful applications of the traditional MAPS include the elasticity problems [30], Poisson problems [31], time-dependent problems [13] and flow problems [32,33].

To have a symmetric system matrix and to approximate the right-hand-side of the considered PDE by the MQs, Chang et al. [34] innovated the Hermite MAPS to solve a class of linear elliptic PDEs. They found that the Hermite MAPS are more accurate and stable than the traditional/Hermite RBFCM and the traditional MAPS, especially for a steady convection-diffusion-reaction problem with larger Péclet numbers. The main focus of this study is to demonstrate the accuracy and stability improvements of the Hermite MAPS over the other three numerical methods for solving linear and nonlinear time-dependent convection-diffusion-reaction problems.

When solving a time-dependent convection-diffusion-reaction problem, time integration methods are applied to convert the problem into time-independent convection-diffusion-reaction problems for consequent time steps. At each time step, the time-independent convection-diffusion-reaction problem is solved by the traditional/Hermite MAPS and RBFCM after the source term of the time-independent governing equation is computed from the results at the previous time step. The prescribed methods have been investigated with the traditional RBFCM [15], the traditional MAPS [13] and the Hermite RBFCM [11,12,14]. In a previous study, La Rocca et al. [12] used the Crank-Nicholson method for the time integration and solved the time-independent problem by the Hermite RBFCM. Following their study, we replace the Hermite RBFCM with the Hermite MAPS in the solution procedure and demonstrate that the Hermite MAPS is the most accurate and stable among these four numerical methods used for solving time-dependent convection-diffusion-reaction problems.

The organization of this paper is listed in the following. The problem definition and the temporal discretizations are addressed in Section 2. Sequentially, the traditional/Hermite MAPS and RBFCM are stated in Section 3. Numerical examples, including a nonlinear time-dependent convection-diffusion-reaction problem, are studied in Section 4. Finally, the conclusions are presented in Section 5.

2. Problem Definition and Temporal Discretizations

In this work, the nonlinear time-dependent convection-diffusion-reaction problem in two dimensions is considered as

$$\frac{\partial C}{\partial t}=D{\nabla }^{2}C+U\cdot \nabla C+kC+S \mathrm{in} \Omega ,$$

(1)

where $C=C\left(x,t\right)$ is the sought solution, $D=D\left(C,x,t\right)$ is the diffusion coefficient, $U=U\left(C,x,t\right)$ is the convection velocity, $k=k\left(C,x,t\right)$ is the reaction coefficient and $S=S\left(x,t\right)$ is the source function, with $x=\left(x,y\right)$ and $t$ being the spatial and temporal coordinates, respectively. In addition, $\Omega$ is the computational domain and $\nabla =\left(\frac{\partial }{\partial x},\frac{\partial }{\partial y}\right)$ is the two-dimensional del operator.

In order to have a well-posed problem, initial and boundary conditions are required, respectively, as

$$C\left(x,t=0\right)=f\left(x\right)$$

(2)

and

$$\left\{\begin{array}{l}C\left(x,t\right)=g\left(x,t\right) \mathrm{for} x\in {\Gamma }_D\\ \frac{\partial C}{\partial n}\left(x,t\right)=h\left(x,t\right) \mathrm{for} x\in {\Gamma }_N,\end{array}\right.$$

(3)

where $\Gamma ={\Gamma }_D+{\Gamma }_N$ is the boundary of $\Omega$, $n$ is the outward normal vector, as well as $f$, $g$ and $h$ are given functions.

Following La Rocca et al. [12], the Crank-Nicholson ($\theta$ weighted) method is applied to integrate Equation (1) in time as

$${\nabla }^2{C}^{n+1}+\frac{U^{n+1}}{D^{n+1}}\cdot \nabla C^{n+1}+\left(\frac{k^{n+1}}{D^{n+1}}-\frac{1}{\theta D^{n+1}\Delta t}\right)C^{n+1}=F\left(C^n,S^{n+1},S^n\right)$$

(4)

with

$$F\left(C^n,S^{n+1},S^n\right)=-\frac{C^n}{\theta D^{n+1}\Delta t}-\frac{\left(1-\theta \right)}{\theta D^{n+1}}{G}^n\left(C^n,S^n\right)-\frac{1}{D^{n+1}}{S}^{n+1}$$

(5)

and

$$G^n\left(C^n,S^n\right)=D^n{\nabla }^2{C}^n+U^n\cdot \nabla C^n+k^n{C}^n+S^n,$$

(6)

where $\Delta t$ is a prescribed time step and $C^{n+1}=C^{n+1}(\mathrm{x})=C(\mathrm{x},t=(n+1)\Delta t)$ is the sought solution at the $n+1$ -th time step. The source term $F\left(C^n,S^{n+1},S^n\right)$ can be calculated from the solution $C^n$ at the previous time step and the given source functions $S^n$ and $S^{n+1}$ at the $n$-th and $n+1$-th time steps, respectively. At the first time step, the $C^0$ in the source term F can be obtained from the initial condition (2). In addition, the weighting parameter $\theta$ is defined as $0<\theta \le 1$. Therefore, Equation (4) reduces to the implicit Euler and standard Crank-Nicholson methods for $\theta =1$ and $\theta =0.5$, respectively.

At each time step, the time-independent convection-diffusion-reaction problem (4) or (7) with boundary conditions (3) can be solved for $C^{n+1}$ by the traditional/Hermite MAPS and RBFCM, which will be addressed in the next section.

If any of D, $U$ or $k$ depends on C, the problem becomes nonlinear and should be solved by the Picard iteration [35] as

$${\nabla }^2{C}^{n+1,s+1}+\frac{1}{D^{n+1,s}}{U}^{n+1,s}\cdot \nabla C^{n+1,s+1}+\left(\frac{k^{n+1,s}}{D^{n+1,s}}-\frac{1}{\theta D^{n+1,s}\Delta t}\right)C^{n+1,s+1}=F\left(C^n,S^{n+1,s},S^n\right),$$

(7)

where $s$ is the index of the Picard iteration. At each Picard iteration, the linear time-independent convection-diffusion-reaction problem (7) with boundary conditions (3) can be solved for $C^{n+1,s+1}$ by the traditional/Hermite MAPS and RBFCM. In this study, the following criteria

$$\frac{\sqrt{{\displaystyle \sum _{i=1}^N{\left(C_i^{n+1,s+1}-C_i^{n+1,s}\right)}^2}}}{\sqrt{{\displaystyle \sum _{i=1}^N{\left(C_i^{n+1,s}\right)}^2}}}<\epsilon$$

(8)

is adopted for the convergence of the Picard iteration, with ε being threshold parameter. The solution procedure can then proceed for the next time step. In Equation (8), $N$ is the total number of spatial discretization points to be introduced in the next section.

In this study, as the main focus is on the stability of the spatial discretization, the Crank-Nicholson method is used the temporal discretization. If increased effectiveness is sought, advanced time integration schemes, such as the Adams-Moulton method [36], can be utilized as

$${\nabla }^2{C}^{n+1}+\frac{U^{n+1}}{D^{n+1}}\cdot \nabla C^{n+1}+\left(\frac{k^{n+1}}{D^{n+1}}-\frac{24}{9\Delta t{D}^{n+1}}\right)C^{n+1}=F_1\left(C^n,C^{n-1},C^{n-2},S^{n+1},S^n,S^{n-1},S^{n-2}\right)$$

(9)

with

$$\begin{array}{l}{F}_1\left(C^n,C^{n-1},C^{n-2},S^{n+1},S^n,S^{n-1},S^{n-2}\right)\\ =-\frac{19}{9D^{n+1}}{G}^n\left(C^n,S^n\right)+\frac{5}{9D^{n+1}}{G}^{n-1}\left(C^{n-1},S^{n-1}\right)-\frac{1}{9D^{n+1}}{G}^{n-2}\left(C^{n-2},S^{n-2}\right)-\frac{24C^n}{9\Delta t{D}^{n+1}}-\frac{S^{n+1}}{D^{n+1}},\end{array}$$

(10)

which can be calculated from the solutions at the previous time steps and the given source functions $S$. Sequentially, the time-independent convection-diffusion-reaction problem (9) with boundary conditions (3) can be solved for $C^{n-1}$ by the traditional/Hermite MAPS and RBFCM. Similarly, the Picard iteration can be applied if a time-dependent convection-diffusion-reaction is considered.

3. Traditional/Hermite MAPS and RBFCM

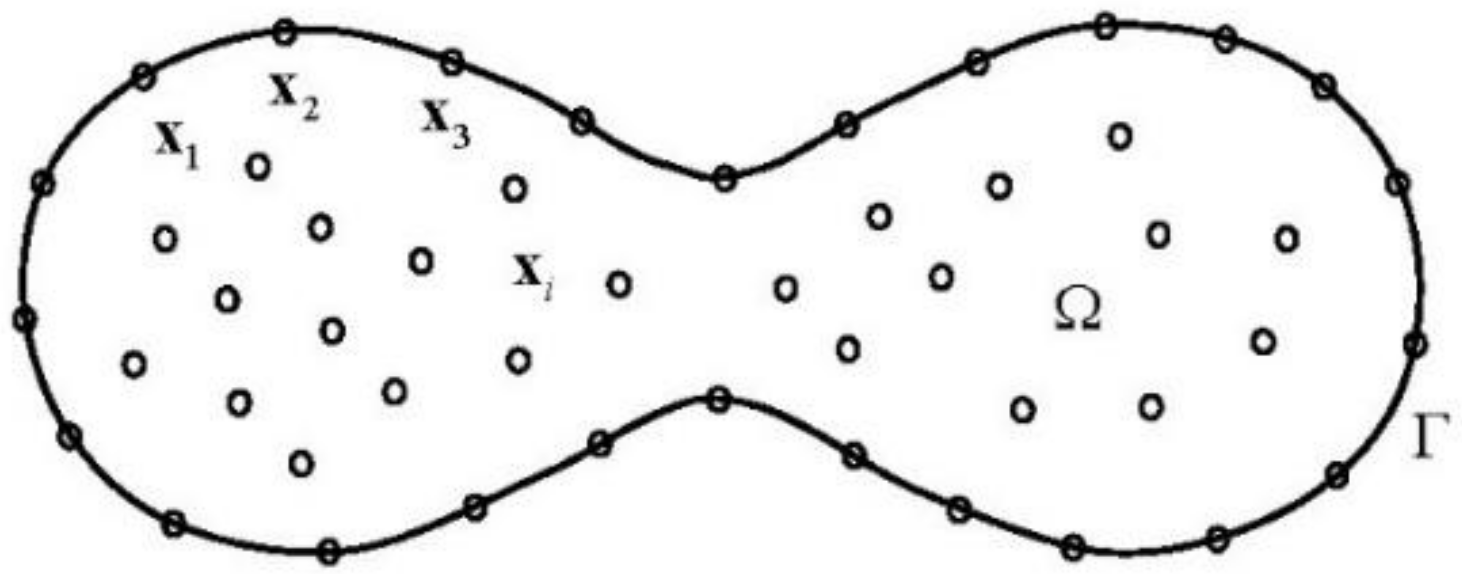
In order to apply the traditional MAPS and RBFCM [18,24], $C^{n+1}$ is approximated by

$$C^{n+1}(x)\approx {\displaystyle \sum _{j=1}^N{\alpha }_j^{n+1}{\psi }_K\left(r_j\right)\hspace{0.17em}}={\displaystyle \sum _{j=1}^N{\alpha }_j^{n+1}{\psi }_K\left(‖x-x_j‖\right)},$$

(11)

where ${\alpha }_j^{n+1}$ are $N$ unknown coefficients at the prescribed points $x_j$, which are located in the domain $\Omega$ as well as the boundary $\Gamma$ as shown in Figure 1. Here, it is assumed that $x_j$’s are numbered so that the first $N_0$ points are inside the domain $\Omega$, $N_1$ points on ${\Gamma }_D$ are sequentially numbered and $N_2$ points on ${\Gamma }_N$ are lastly numbered with $N=N_0+N_1+N_2$. In Equation (11), we have defined

$${\psi }_I\left(r\right)=c\sqrt{1+R^2}$$

(12)

and

$${\psi }_{II}\left(r\right)=\frac{c^3}{9}\left\{\left(R^2+4\right)\sqrt{R^2+1}-3\ln \left(\frac{1+\sqrt{R^2+1}}{2}\right)-4\right\}$$

(13)

with

$$r=cR$$

(14)

and $c$ being the shape parameter for tuning the numerical accuracy [17]. When using the approximation (11), polynomials are sometimes augmented to improve the solvability [21]. In Equations (12) and (13), ${\psi }_I$ is the MQ and ${\psi }_{II}$ is its particular solution of the Laplace operator as

$${\nabla }^2{\psi }_{II}={\psi }_I.$$

(15)

The $N$ unknown coefficients ${\alpha }_j^{n+1}$ can be solved by collocating Equation (11) by the governing Equation (3) and boundary conditions (3) as

$${\left[A\right]}_{N,N}{\psi }_K\left(‖x_i-x_j‖\right){\left[{\alpha }_j^{n+1}\right]}_{N,1}=\left[\begin{array}{c}{\left[F\left(C^n,S^n,S^{n+1}\right)\left(x_i\right)\right]}_{N_0,1}\\ {\left[g\left(x_i,\left(n+1\right)\Delta t\right)\right]}_{N_1,1}\\ {\left[h\left(x_i,\left(n+1\right)\Delta t\right)\right]}_{N_2,1}\end{array}\right]$$

(16)

where ${\left[A\right]}_{N,N}$ with $K=I$ or $K=II$ gives the differential matrix for the traditional RBFCM and MAPS, respectively. Details have been explicitly addressed in the previous study [34]. If the system is nonsingular, it can be used for solving the $N$ unknown coefficients ${\alpha }_j^{n+1}$. Sequentially, Equation (11) can be used for approximating $C^{n+1}$.

For the Hermite MAPS and RBFCM [20,21], $C^{n+1}$ is approximated by

$$\begin{array}{l}{C}^{n+1}(x)\approx {\displaystyle \sum _{j=1}^{N_0}{\beta }_j^{n+1}{\left({\nabla }^2+\frac{1}{D}U\cdot \nabla +\frac{k}{D}-\frac{1}{\theta D\Delta t}\right)}_{x_j}{\psi }_K\left(‖x-x_j‖\right)}\\ +{\displaystyle \sum _{j=N_0+1}^{N_0+N_1}{\beta }_j^{n+1}{\psi }_K\left(‖x-x_j‖\right)}+{\displaystyle \sum _{j=N_0+N_1+1}^{N_0+N_1+N_2}{\beta }_j^{n+1}{\left(\frac{\partial }{\partial n}\right)}_{x_j}{\psi }_K\left(‖x-x_j‖\right)}\end{array}$$

(17)

with $K=I$ and $K=III$ for the Hermite MAPS and RBFCM, respectively. In Equation (17), the subscript $x_j$ indicates that the differential operators are performed with respect to the source point $x_j$ as detailed in the literature [20,21,34]. ${\psi }_{III}$ is the MQ particular solution of the biharmonic operator defined by

$${\psi }_{III}\left(r\right)=\frac{c^5}{900}\left\{\left(4R^4+48R^2-61\right)\sqrt{1+R^2}-\left(75R^2-30\right)\ln \left(\frac{1+\sqrt{R^2+1}}{2}\right)-25R^2+61\right\}$$

(18)

and

$${\nabla }^2{\psi }_{III}={\psi }_{II}.$$

(19)

It needs to be mentioned that all of ${\psi }_I$, ${\psi }_{II}$ and ${\psi }_{III}$ are infinitely differentiable [25]. Similarly, the $N$ unknown coefficients ${\beta }_j^{n+1}$, in Equation (17), can be solved by collocating the governing Equation (4) and boundary conditions (3) as

$${\left[B\right]}_{N,N}{\psi }_K\left(‖x_i-x_j‖\right){\left[{\beta }_j^{n+1}\right]}_{N,1}=\left[\begin{array}{c}{\left[F\left(C^n,S^n,S^{n+1}\right)\left(x_i\right)\right]}_{N_0,1}\\ {\left[g\left(x_i,\left(n+1\right)\Delta t\right)\right]}_{N_1,1}\\ {\left[h\left(x_i,\left(n+1\right)\Delta t\right)\right]}_{N_2,1}\end{array}\right]$$

(20)

where ${\left[B\right]}_{N,N}$ is the differential matrix for the Hermite MAPS and RBFCM [34]. Equation (20) is utilized to obtain the $N$ unknown coefficients ${\beta }_j^{n+1}$ with $K=I$ and $K=III$ for the Hermite MAPS and RBFCM, respectively. Then, Equation (17) can be used for approximating $C^{n+1}$ of the Hermite methods.

After the solution $C^{n+1}$ is obtained by the traditional/Hermite MAPS and RBFCM, the time marching can proceed to a desired final time using Equations (3)–(5).

4. Numerical Results

The traditional/Hermite MAPS and RBFCM are validated by several numerical examples in this section. These examples are essential and natural boundary conditions in rectangular and peanut-shaped domains. Numerical results demonstrate that the Hermite MAPS is the most accurate and stable of these four numerical methods.

In the following analyses, numerical accuracy is evaluated by the maximum absolute error defined by

$$\mathrm{error}=\underset{i}{\mathrm{Max}}\left\{\left|C^{n+1}\left({\overline{x}}_i\right)-C_{exact}\left({\overline{x}}_i,\left(n+1\right)\Delta t\right)\right|\right\},$$

(21)

where $C_{exact}$ and $C^{n+1}$ are the exact and numerical solutions, respectively. Additionally, it is required that the evaluation points ${\overline{x}}_i$ should be sufficiently dense so that the errors are representative.

4.1. Example 1: Heat Equation (Pure Diffusion)

Following La Rocca et al. [12], we study the solution of the time-dependent heat equation (Equation (1) with $U=0$, $k=0$, and $S=0$) as

$$\frac{\partial C}{\partial t}=D{\nabla }^{2}C$$

(22)

with $D=1$. A rectangular computational domain $[0,10]\times [0,6]$ is considered with the following initial and boundary conditions:

$$C\left(x,t=0\right)=0,$$

(23)

$$C\left(x=0,y,t\right)=C\left(x=2L,y,t\right)=C_0,$$

(24)

and

$$\frac{\partial C}{\partial y}\left(x,y=0,t\right)=\frac{\partial C}{\partial y}\left(x,y=6,t\right)=0.$$

(25)

The analytical solution of the above problem is given by

$$C=C_0-{\displaystyle \sum _{n=0}^{\infty }{C}_0\frac{2}{{\lambda }_{n}L}{e}^{-D{\lambda }_n{}^{2}t}\sin {\lambda }_{n}x}$$

(26)

with

$${\lambda }_n=\frac{\left(2n+1\right)\pi }{2L}$$

(27)

and

$$L=5.$$

(28)

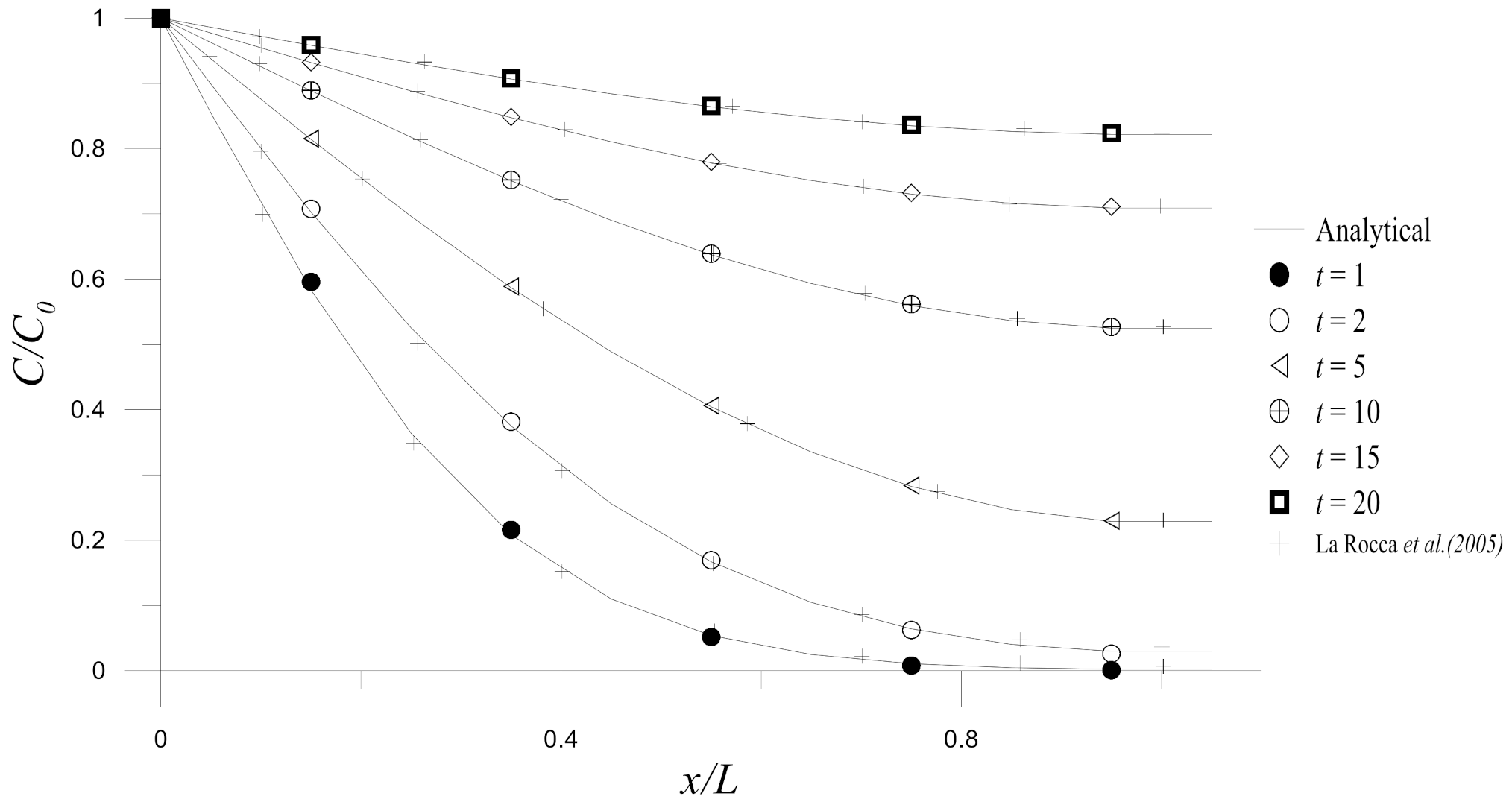
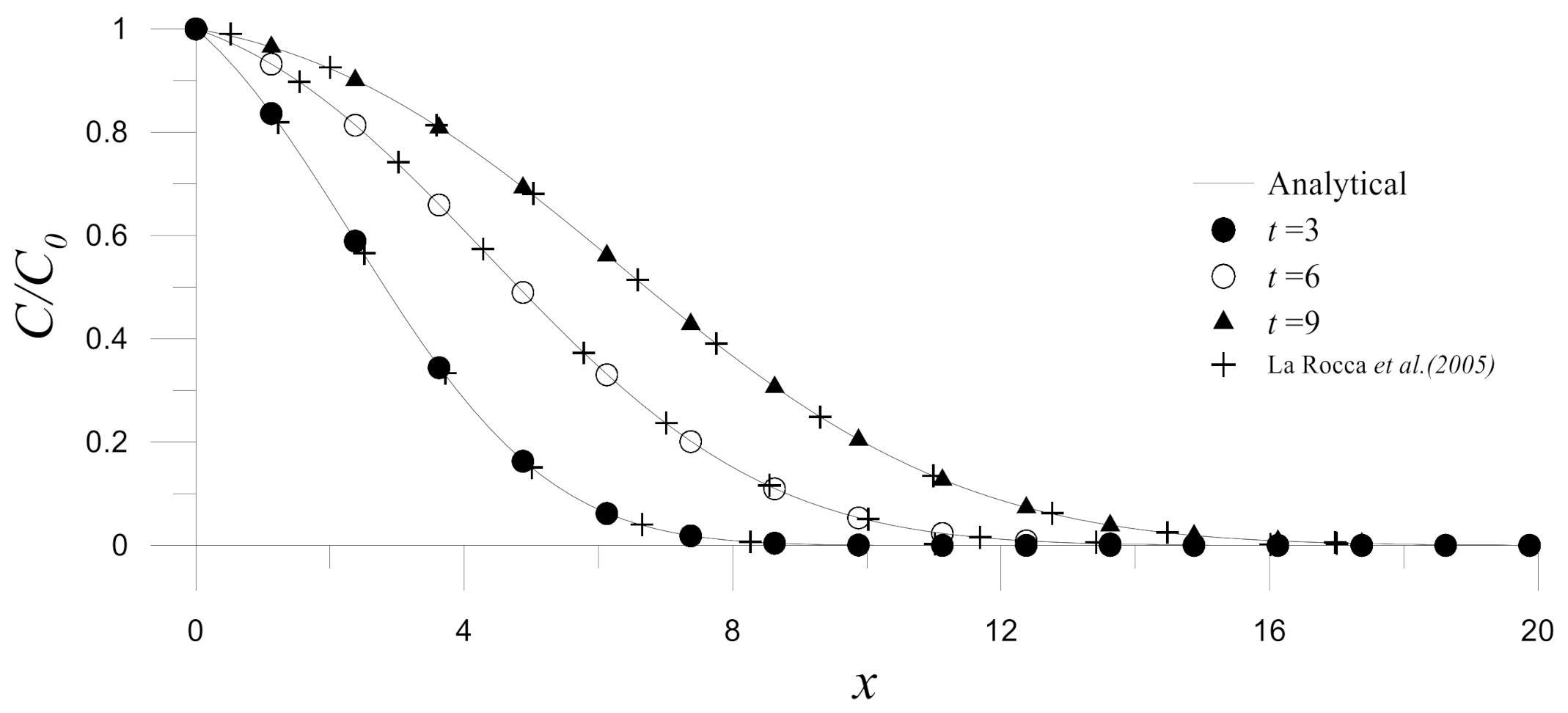
In this example, we typically adopt the implicit Euler method ($\theta =1$) with $\Delta t=0.1$, $c=1$ and $N=N_0+N_1=20\times 12+64$ if not otherwise mentioned. The Hermite MAPS is validated by solving the prescribed problem and comparing it with the results from the Hermite RBFCM and the analytical solutions as depicted in Figure 2. Additionally, Figure 3 shows the errors obtained by the Hermite MAPS for different node numbers. The convergence concerning the node numbers can be observed.

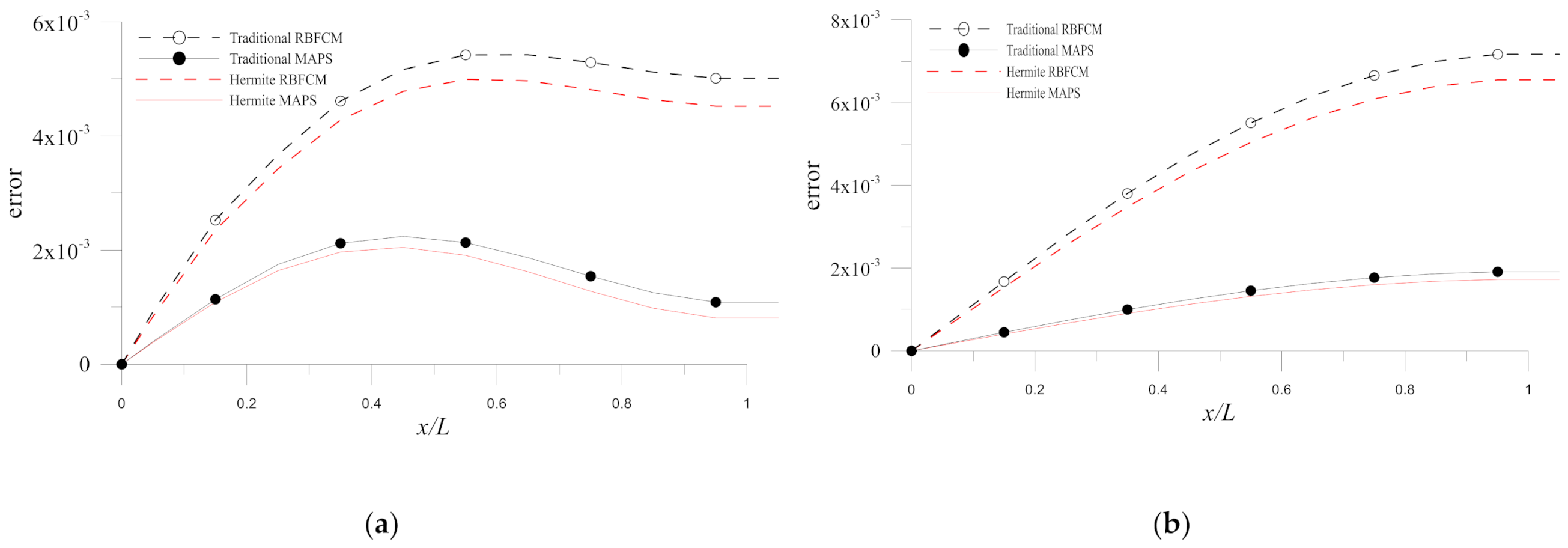
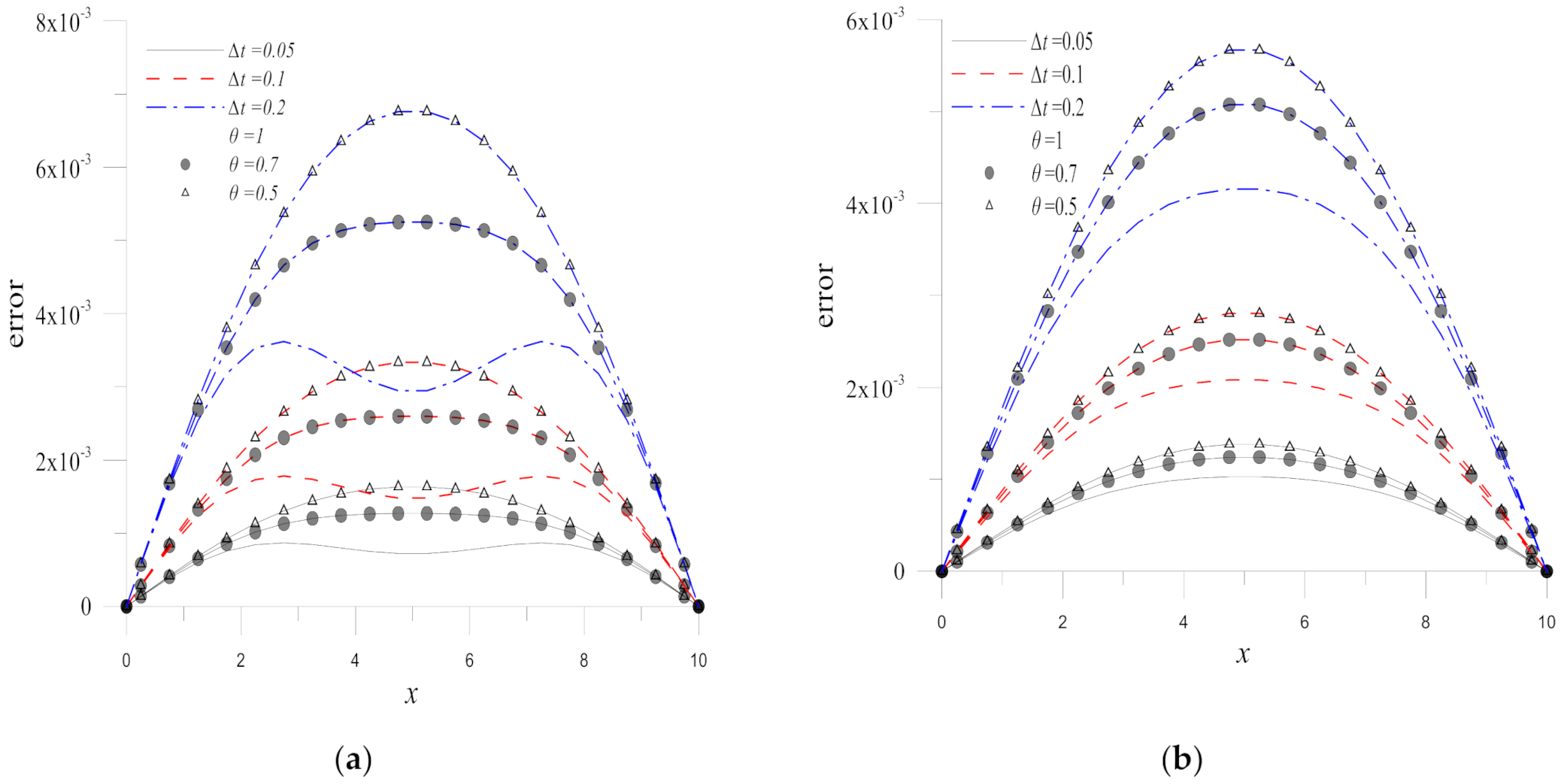
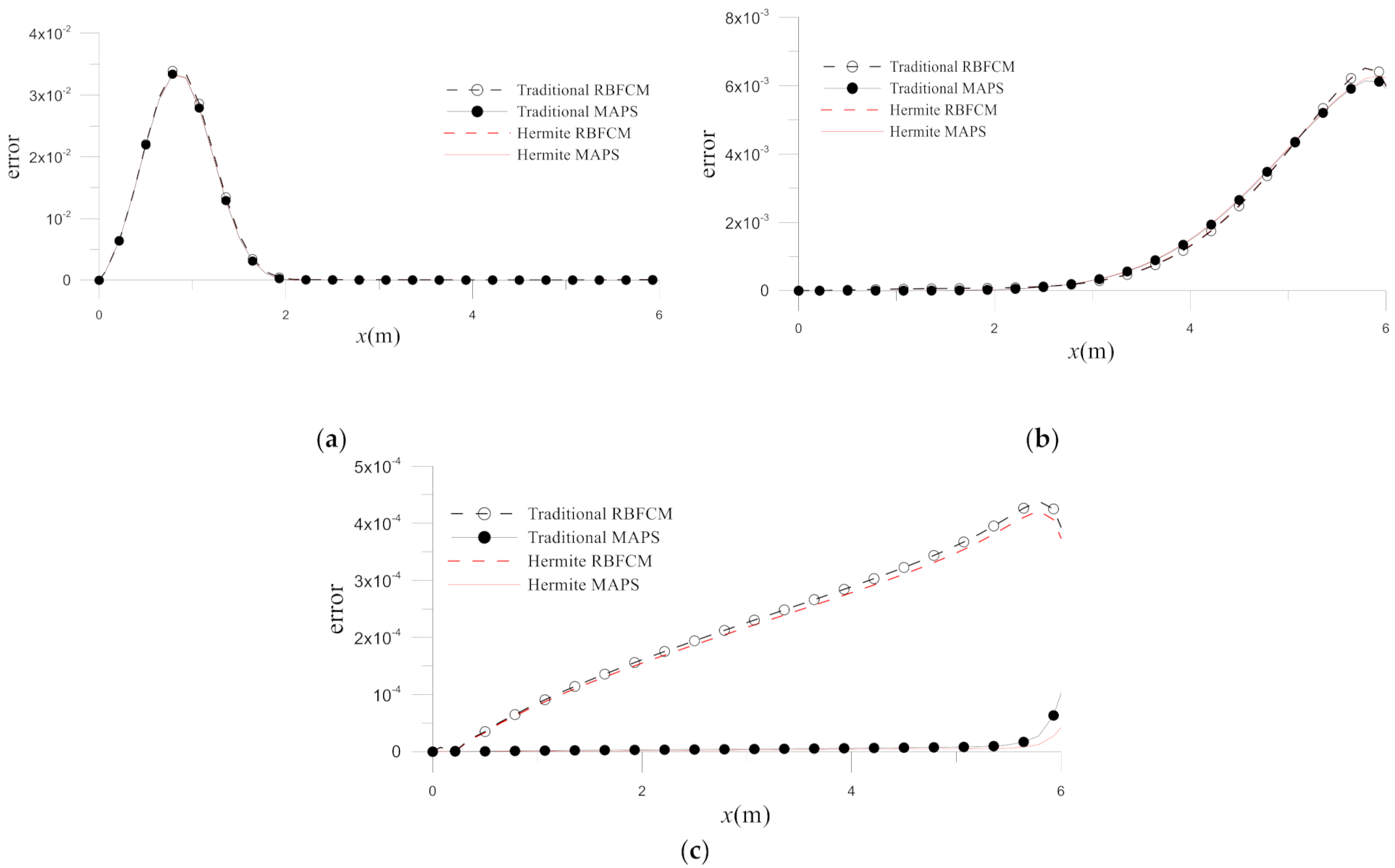
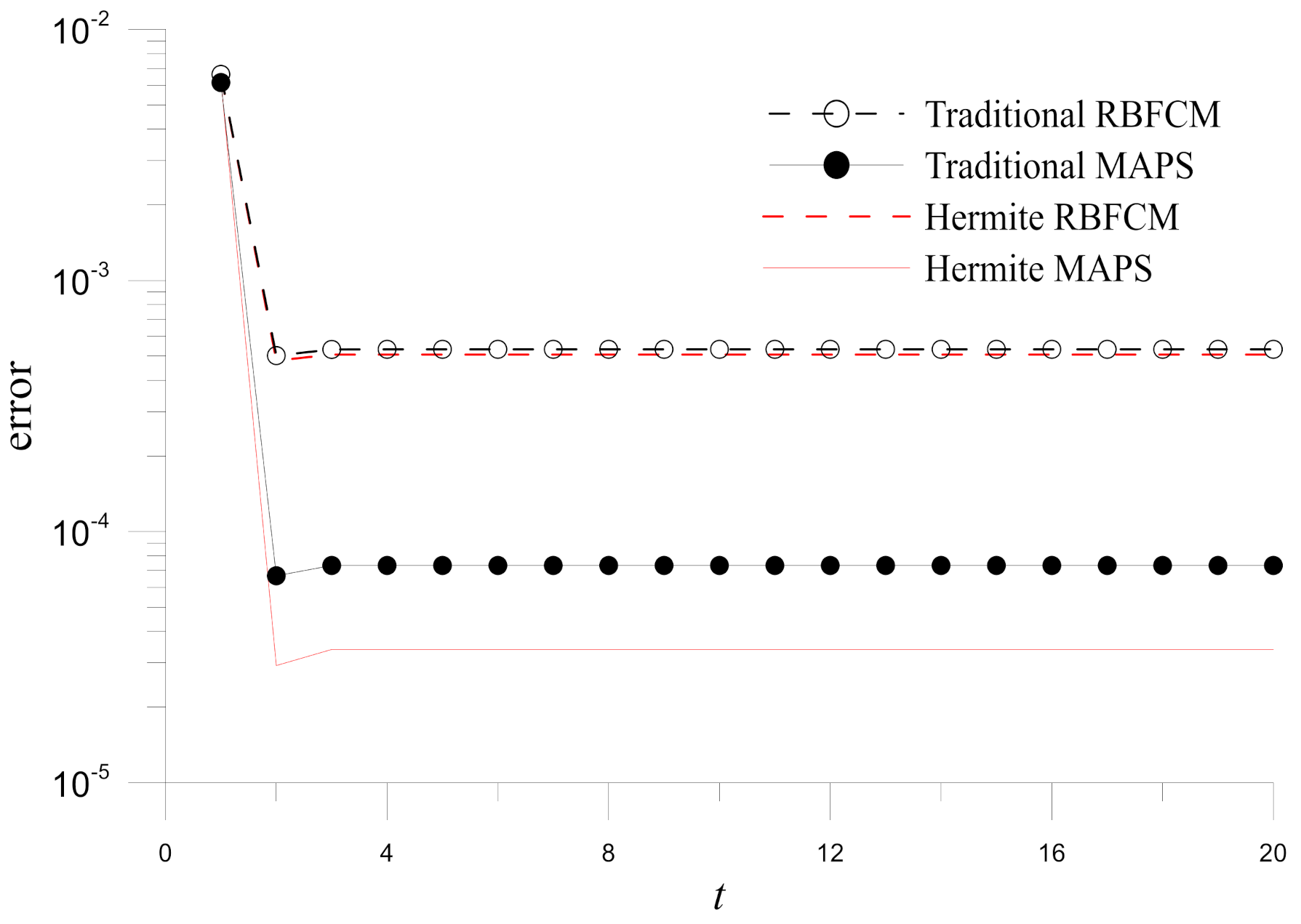
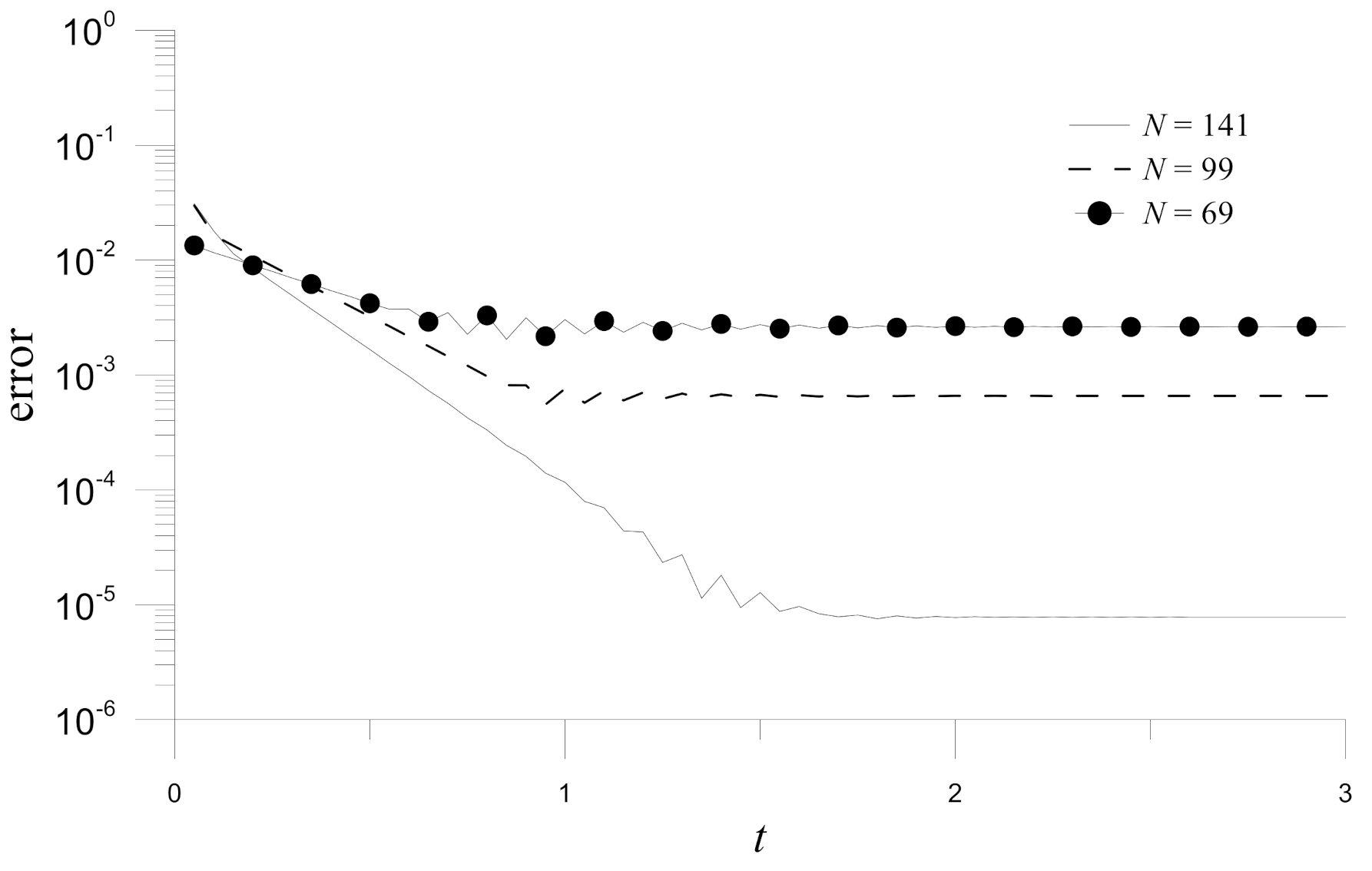
After the Hermite MAPS is validated, comparisons are made between the traditional/Hermite MAPS and RBFCM. Figure 4 depicts the errors on $y=3$ at $t=5$ and $t=20$. It is evident from the figure that the MAPS are more accurate, and the Hermite MAPS is the most accurate in these four numerical methods. The accuracy improvement in the Hermite MAPS over the other three methods is further demonstrated by the time histograms of errors for numerical solutions obtained by the four methods ($t=0~20$) in Figure 5.

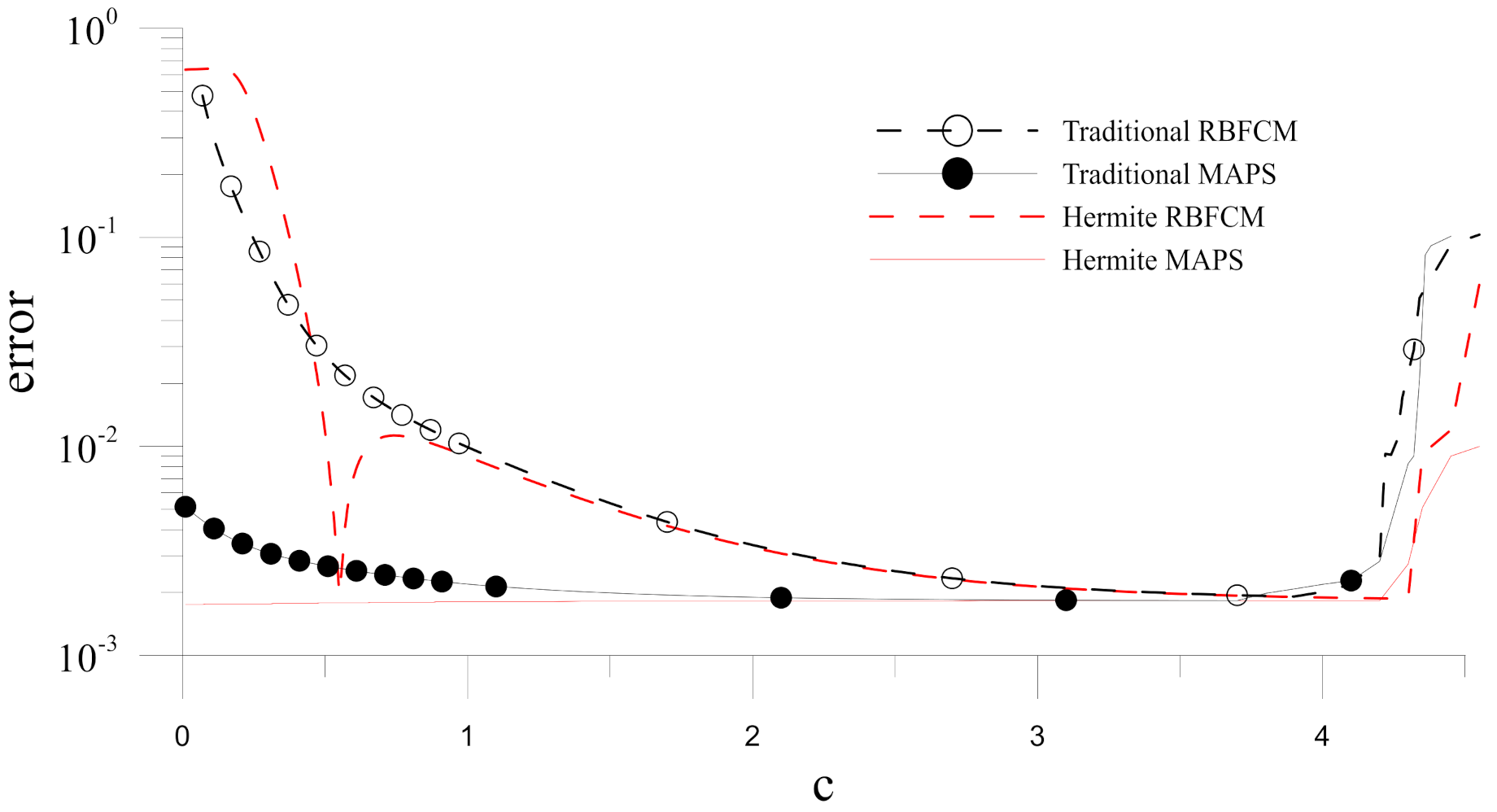
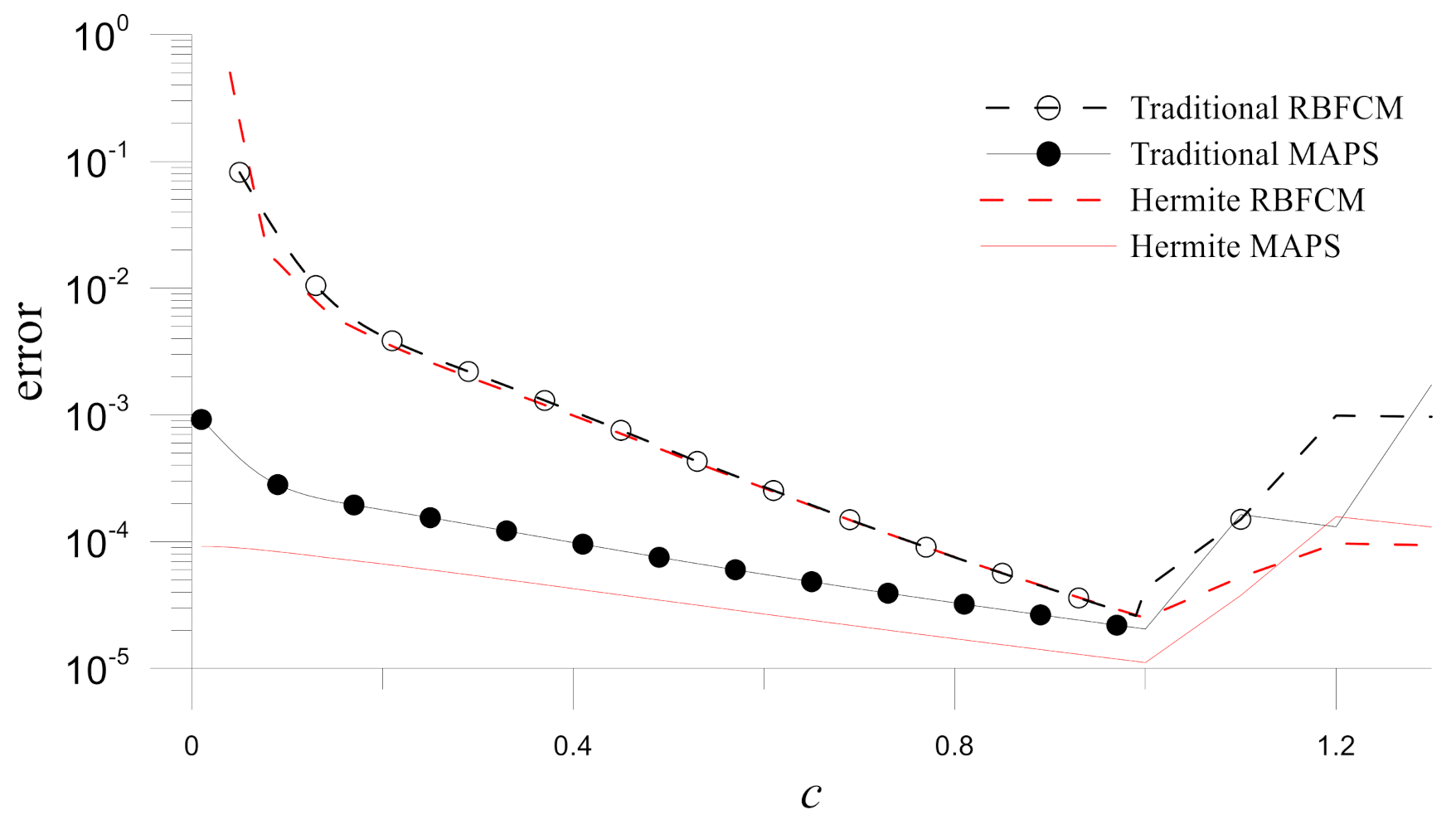
To study the stability of the methods, the numerical errors of the solutions obtained by these four numerical methods are compared for different shape parameters $c$. Figure 6 demonstrates the Hermite MAPS is the most stable concerning the shape parameter in these four numerical methods. Additionally, the computing costs for the four methods are studied in

Table 1. Computing time (s) for numerical solutions by the four methods in Example 1 ($c=1$, $\theta =1$, $\Delta t=0.1$, and $t=0~5$ ).

	N = 92	N = 304	N = 636
Traditional RBFCM	<1	11	68
Traditional MAPS	<1	12	73
Hermite RBFCM	<1	15	85
Hermite MAPS	<1	19	124

. In the table, it can be observed that the traditional RBFCM is more efficient than the other three methods. However, the Hermite MAPS is the most time-consuming method, while its computing time is about twice compared with that of the traditional RBFCM. Overall, the computing time by a typical PC is about several minutes in the presented numerical examples.

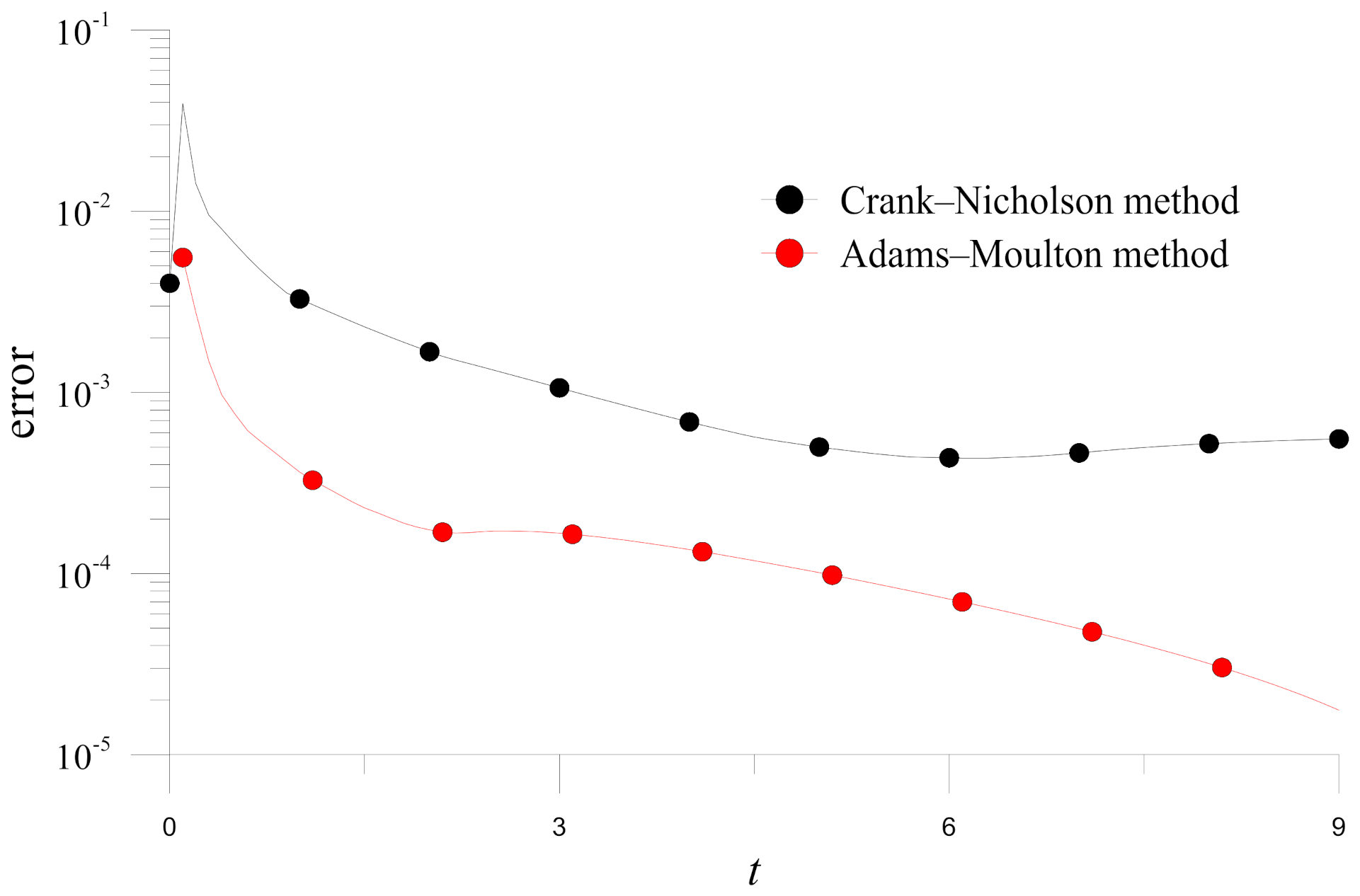
Sequentially, the effects of $\Delta t$ and the weighting parameter $\theta$ are studied as depicted in Figure 7. The figure shows clearly that the solutions obtained by the methods with smaller $\Delta t$ are more accurate. Additionally, the effect of weighting parameter $\theta$ is not significate while the implicit Euler method ($\theta =1$) seems to give slightly more accurate results. Finally, if a more effective temporal discretization is considered, the Adams-Moulton method is applied to solve the present example. Figure 8 depicts the time histograms of errors obtained by the Crank-Nicholson and Adams-Moulton methods. Significant accuracy improvement in the Adams-Moulton method results can be observed.

4.2. Example 2: Convective-Diffusion Equation

We consider the solution of the time-dependent convection-diffusion problem with constant diffusion coefficient and convection velocity (Equation (1) with $k=0$ and $S=0$) as

$$\frac{\partial C}{\partial t}=D{\nabla }^{2}C-\left(u,0\right)\cdot \nabla C$$

(29)

with $D=1$ and $u=0.6$ (Péclet number, $Pe=uL/D=12$) in a rectangular domain $\left[0,20\right]\times \left[0,1\right]$ with initial and boundary conditions as

$$C\left(x,t=0\right)=0,$$

(30)

$$C\left(x=0,y,t\right)=C_0,$$

(31)

and

$$\frac{\partial C}{\partial y}\left(x,y=0,t\right)=\frac{\partial C}{\partial y}\left(x,y=1,t\right)=\frac{\partial C}{\partial x}\left(x=20,y,t\right)=0.$$

(32)

Following La Rocca et al. [12], the exact solution of the problem is

$$C=\frac{C_0}{2}\mathrm{erfc}\left(\frac{x-ut}{2\sqrt{Dt}}\right)+\frac{C_0}{2}{e}^{\frac{2ux}{2D}}\mathrm{erfc}\left(\frac{x+ut}{2\sqrt{Dt}}\right).$$

(33)

In this example, numerical results are obtained by using the standard Crank-Nicholson method ($\theta =0.5$) with $\Delta t=0.025$, $c=1$ and $N=N_0+N_1=60\times 3+126$ if not otherwise addressed. Similar to the previous case, the Hermite MAPS is validated by comparing its results with the Hermite RBFCM results and the analytical solutions depicted in Figure 9. Good agreements can be observed in the figure.

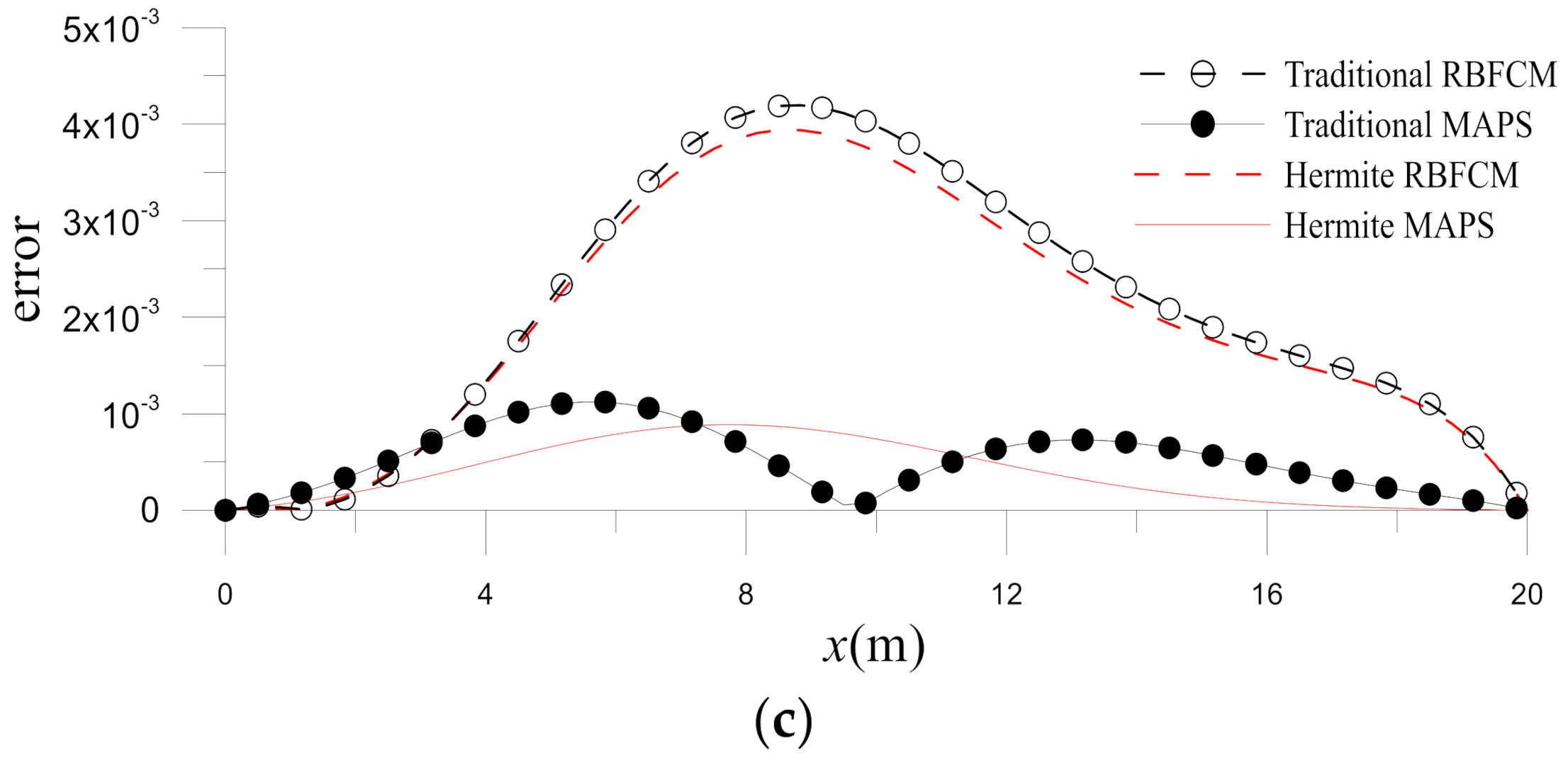
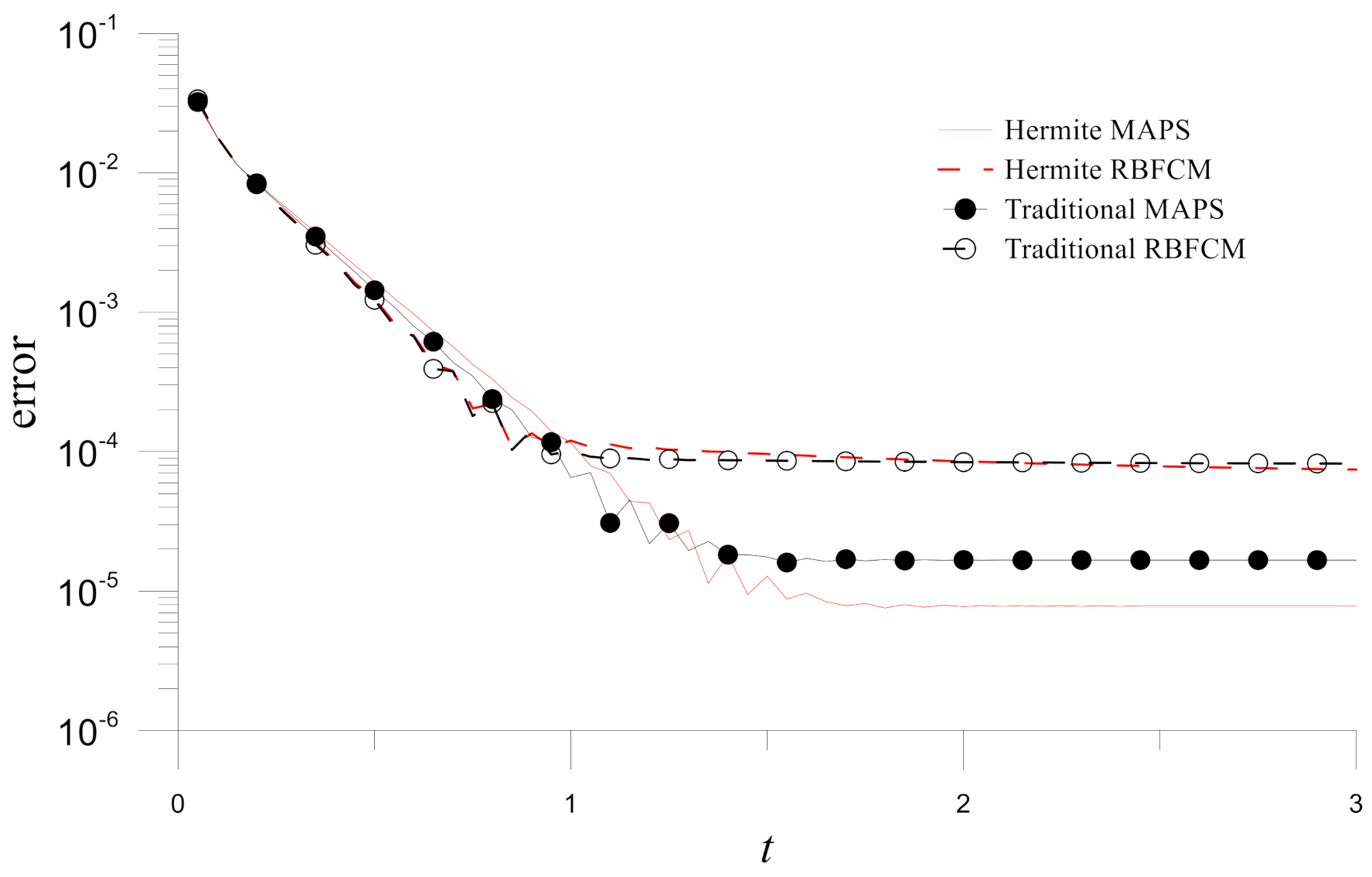
Then, comparisons are made for the traditional/Hermite MAPS and RBFCM. Figure 10 depicts the errors on $y=0.5$ at different times. It is clear from the figure that the MAPS are more accurate. The accuracy improvements are further studied by the time histograms of errors obtained by the four numerical methods. Figure 11 demonstrates that the Hermite MAPS is the most accurate in these four numerical methods for $t=0~20$.

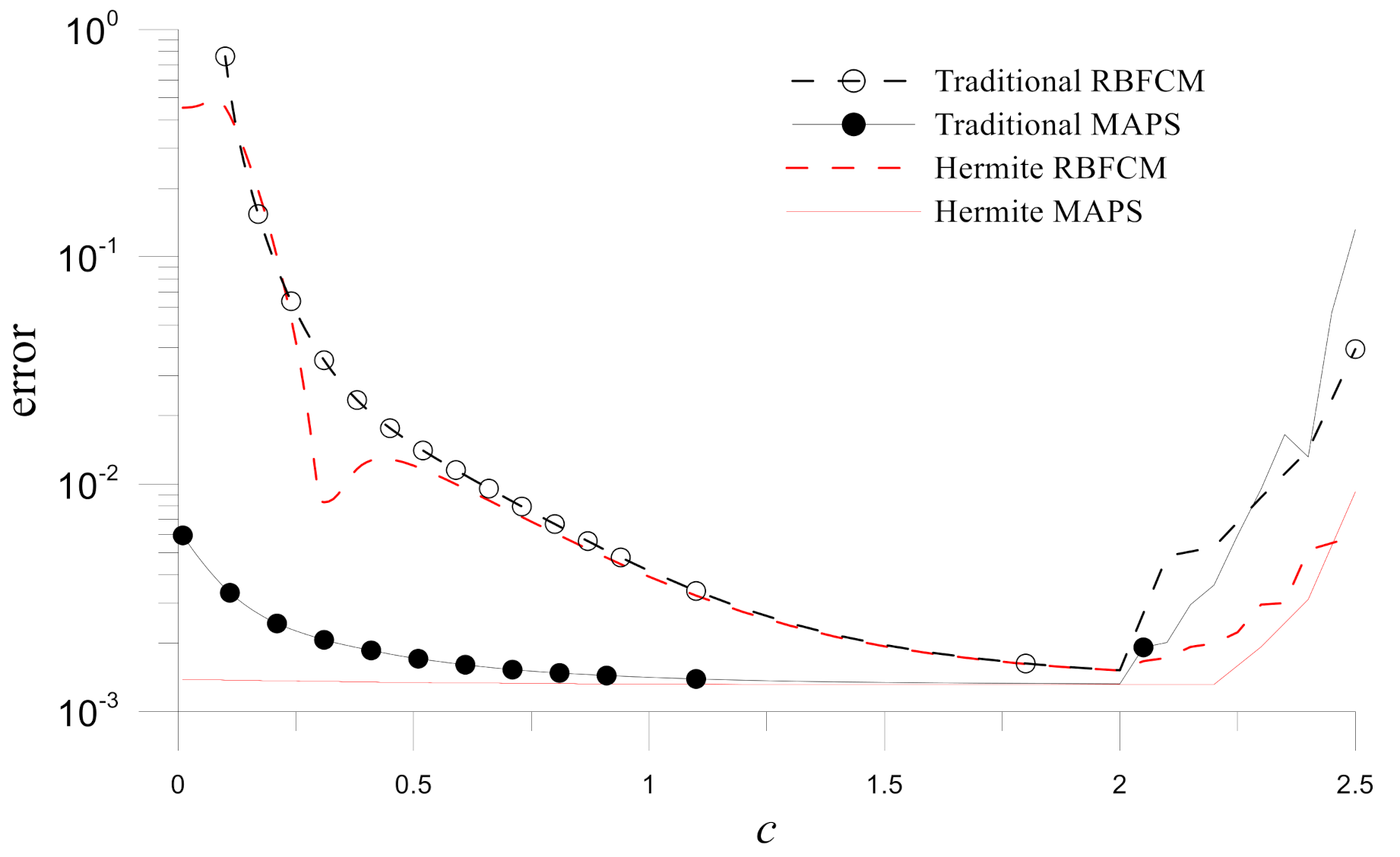
Sequentially, the errors for different shape parameters for the numerical results obtained by the four numerical methods are shown in Figure 12. The figure also demonstrates that the Hermite MAPS is the most stable concerning the shape parameter in these four numerical methods.

Overall, it is evident that the Hermite MAPS is the most accurate and stable method for solving the present time-dependent convection-diffusion problem.

4.3. Example 3: Convection-Diffusion-Reaction Problem

Following La Rocca et al. [12], we consider a time-dependent convection-diffusion-reaction problem (Equation (1) with $S=0$) as

$$\frac{\partial C}{\partial t}=D{\nabla }^{2}C-\left(u,0\right)\cdot \nabla C-kC$$

(34)

with $D=1$, $u=6$, and $k=0.287$ (Péclet number, $Pe=uL/D=36$) in a rectangular domain $\left[0,6\right]\times \left[0,0.7\right]$. The initial and boundary conditions are given by

$$C\left(x,t=0\right)=0,$$

(35)

$$C\left(x=0,y,t\right)=C_0,$$

(36)

and

$$\frac{\partial C}{\partial y}\left(x,y=0,t\right)=\frac{\partial C}{\partial y}\left(x,y=0.7,t\right)=\frac{\partial C}{\partial x}\left(x=6,y,t\right)=0.$$

(37)

The exact solution of the problem can be obtained from [37] as

$$C=\frac{C_{0}G\left(x,t;u,v,D,k\right)}{1+\left(\frac{v-u}{v+u}\right)e^{-\frac{vL}{D}}}$$

(38)

with

$$\begin{array}{l}G\left(x,t;u,v,D,k\right)\\ =\frac{1}{2}{e}^{\frac{\left(u-v\right)x}{2D}}\mathrm{erfc}\left(\frac{x-vt}{2\sqrt{Dt}}\right)+\frac{1}{2}{e}^{\frac{\left(u+v\right)x}{2D}}\mathrm{erfc}\left(\frac{x+vt}{2\sqrt{Dt}}\right)+\frac{v-u}{2\left(v+u\right)}{e}^{\frac{\left(u+v\right)x-2vL}{2D}}\mathrm{erfc}\left(\frac{\left(2L-x\right)-vt}{2\sqrt{Dt}}\right)\\ +\frac{v+u}{2\left(v-u\right)}{e}^{\frac{\left(u-v\right)x+2vL}{2D}}\mathrm{erfc}\left(\frac{\left(2L-x\right)+vt}{2\sqrt{Dt}}\right)-\frac{u^2}{2kD}{e}^{\frac{uL}{D}-kt}\mathrm{erfc}\left(\frac{\left(2L-x\right)+ut}{2\sqrt{Dt}}\right),\end{array}$$

(39)

$$v=u\sqrt{1+\frac{4kD}{u^2}},$$

(40)

and

$$L=6.$$

(41)

In this example, numerical results are obtained by the standard Crank-Nicholson method ($\theta =0.5$) with $\Delta t=0.01$, $c=0.5$ and $N=N_0+N_1=42\times 5+94$ if not additionally stated. Sequentially, the Hermite MAPS is validated by comparing its results with those obtained by the Hermite RBFCM and the analytical solutions, as shown in Figure 13. Similar to the previous examples, good agreements can be observed.

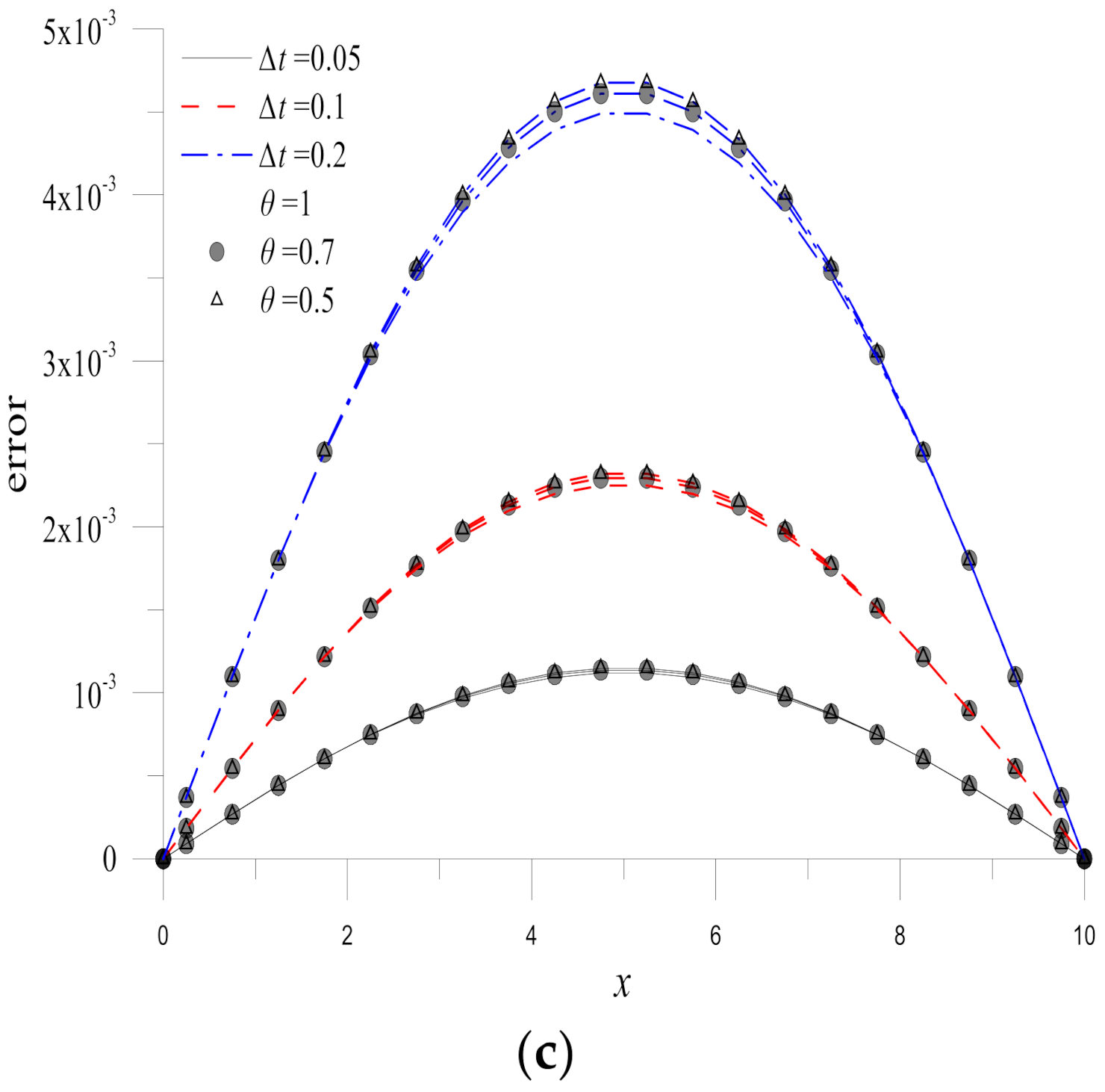
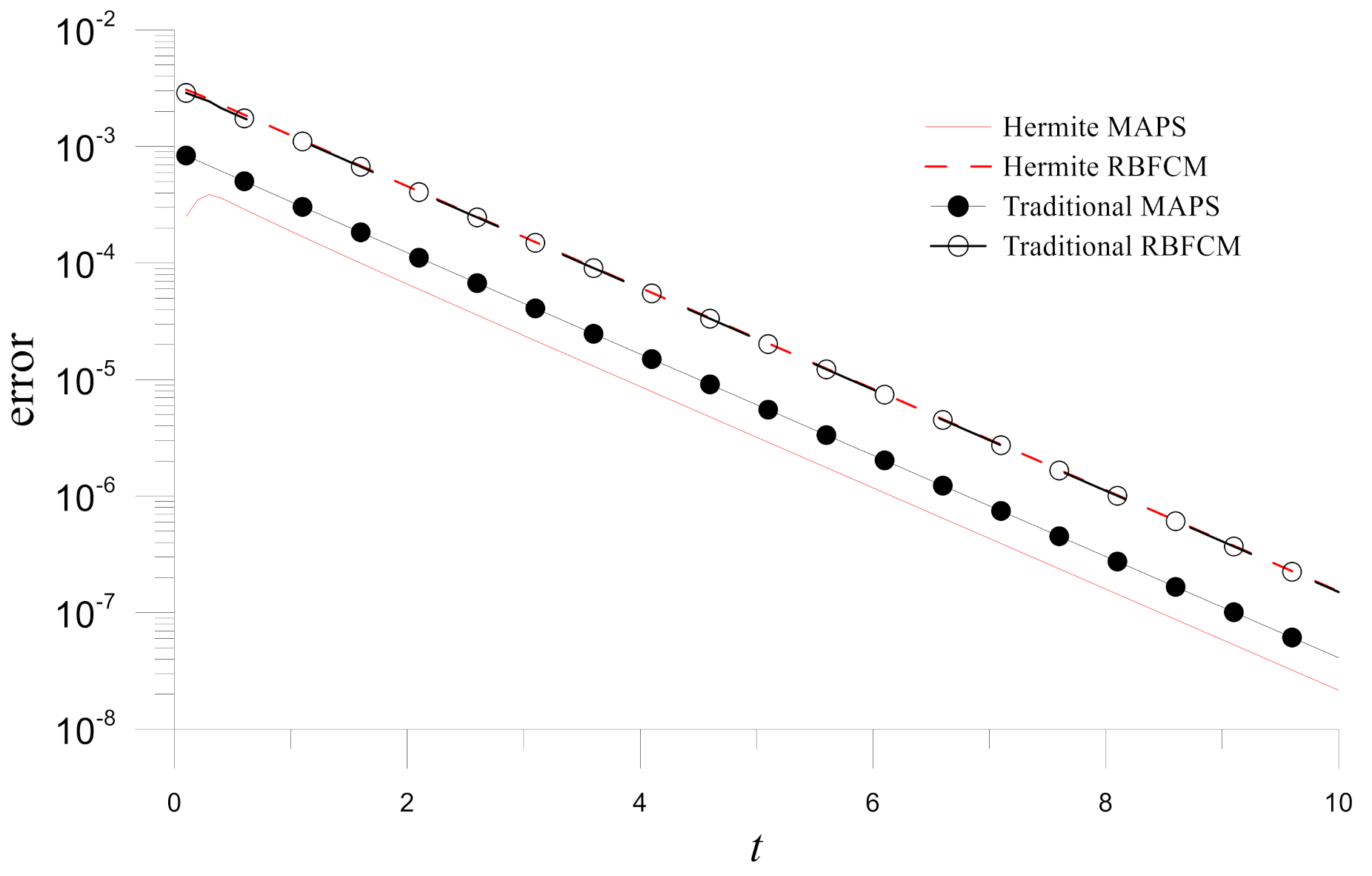
The performance of the traditional/Hermite MAPS and RBFCM is then compared. Figure 14 gives the errors on $y=0.35$ at different times and shows that the MAPS are more accurate, especially for a larger time. This result can be expected, as the MAPS contain information from the governing operator. The accuracy improvements are further studied by the time histograms of errors obtained by the four numerical methods. In Figure 15, it can be found that the Hermite MAPS is the most accurate in these four numerical methods for $t=0\sim 20$.

The stability issue is then studied. Figure 16 demonstrates the errors for different shape parameters corresponding to the numerical results by the four numerical methods. It also demonstrates that the Hermite MAPS is the most stable concerning the shape parameter in these four numerical methods.

Overall, it is evident the Hermite MAPS is the most accurate and stable method for solving the present time-dependent convection-diffusion-reaction problem.

4.4. Example 4: Time-Dependent Convection-Diffusion-Reaction Problem in a Peanut-Shaped Domain

In order to demonstrate the accuracy and stability outperformance of the Hermite MAPS over the other three methods for problems in irregular domains, we consider the time-dependent convection-diffusion-reaction problem governed by

$$\frac{\partial C}{\partial t}=D{\nabla }^{2}C-\left(u,0\right)\cdot \nabla C-kC+S$$

(42)

with

$$S=C_0\left(ucosx+\left(k-1\right)sinx\right)sinh y exp\left(-t\right).$$

(43)

The exact solution of the problem is

$$C=\frac{C_{0}G\left(x,t;u,v,D,k\right)}{1+\left(\frac{v-u}{v+u}\right)e^{-\frac{vL}{D}}}+C_{0}sinxsinhyexp\left(-t\right)$$

(44)

with other definitions given in Equations (39) and (40) and parameters the same as those in the previous example. The initial condition is set based on the prescribed analytical solution. Additionally, the essential boundary conditions are enforced on the peanut-shaped boundary defined in polar coordinates $\left(\rho ,\theta \right)$ as

$$\rho =0.3\sqrt{cos2\theta +\sqrt{1.1-{sin}^{2}2\theta }} for 0\le \theta \le 2\pi .$$

(45)

The Hermite MAPS is validated by comparing its results with the analytical solutions for different collocation points, as shown in Figure 17. The convergence concerning the node numbers is also observed. Similarly, Figure 18 depicts the time histograms of errors obtained by the four numerical methods for $t=0~3$. Figure 18 demonstrates that Hermite MAPS is the most accurate of these four numerical methods for solving the time-dependent convection-diffusion-reaction problem in the peanut-shaped domain.

4.5. Example 5: Nonlinear Time-Dependent Convection-Diffusion-Reaction Problem

Finally, the Hermite MAPS with the Picard iteration (7) is applied for solving a nonlinear time-dependent convection-diffusion-reaction problem in a square domain of $\left[-3,3\right]\times \left[-3,3\right]$, which is governed by

$$\frac{\partial C}{\partial t}={\nabla }^{2}C+\left(U,1\right)\cdot \nabla C+kC+S$$

(46)

with

$$U=10+sinC$$

(47)

and

$$k=C.$$

(48)

The source term $S$ and the Dirchlet boundary condition are defined according to the exact solution as

$$C=e^{-t}\sin x\cos y.$$

(49)

The threshold parameter for the convergence criteria of the Picard iteration (8) is set as $\epsilon ={10}^{-10}$.

First, the Hermite MAPS is validated by comparing its results with the analytical solutions for different collocation points, as depicted in Figure 19. An evident convergence concerning the node numbers can be observed. After the Hermite MAPS is validated, Figure 20 depicts the time histograms of errors obtained by the four numerical methods for $t=0~10$. The figure also shows that the Hermite MAPS is the most accurate among these four numerical methods for solving the nonlinear time-dependent convection-diffusion-reaction problem in the peanut-shaped domain.

Finally, if the more effective Adams-Moulton method is considered, Figure 21 depicts the time histograms of errors obtained by the Crank-Nicholson and Adams-Moulton methods. Accuracy improvement in the Adams-Moulton method results can also be observed.

5. Conclusions

In this article, the Hermite MAPS was developed for solving time-dependent convection-diffusion-reaction problems in two dimensions. Using the Crank-Nicholson or the Adams-Moulton method, the time-dependent convection-diffusion-reaction problem was converted into time-independent convection-diffusion problems for consequent time steps. At each time step, the source term of the time-independent convection-diffusion-reaction problem was computed from the previous time step. The MQ particular solution of the biharmonic operator was used in the Hermite MAPS and, therefore, the source term of the time-independent convection-diffusion-reaction equation is approximated by the MQ. Additionally, the prescribed method results in a symmetric system matrix. The traditional/Hermite MAPS and RBFCM were applied for solving different numerical problems with governing equations, boundary conditions and computational domains. The numerical results were shown to support analytical and numerical solutions in the literature. Furthermore, the numerical results indicate that the Hermite MAPS is the most accurate and stable of these four numerical methods. Additionally, it was demonstrated that the Hermite MAPS was able to be applied for solving a nonlinear time-dependent convection-diffusion-reaction problem by the Picard iteration.