Stability Evaluation of Medium Soft Soil Pile Slope Based on Limit Equilibrium Method and Finite Element Method
Abstract
1. Introduction
- (1)
- Engineering geological analogy method
- (2)
- Rigid body limit equilibrium method
- (3)
- Numerical analysis limit equilibrium method
- (4)
- Probability analysis
- (5)
- Other new methods
2. Engineering Geological Background
2.1. Engineering Geological Characteristics of Slope
2.1.1. Formation Lithology
- (1)
- Yanchang Formation of Upper Triassic system (T3y)
- (2)
- A formation of middle and lower Jurassic(J1-2y)
- (1)
- The first rock section (J1-2y1): From the bottom boundary of the formation to the top boundary of coal formation 5, including three coal formations, 5, 6, and 7, the grain size of the rock in this section gradually becomes finer from bottom to top. The bottom of the lithology is mainly gray-white medium coarse-grained quartz sandstone. Local sections contain gravel. The sandstone is well-sorted and has a high quartz content. It is a regional correlation marker layer. The lithology of the middle and upper parts is interbedded with grayish-white sandstone, dark gray siltstone, and sandy mudstone containing a large number of fragment plant fossils. The surface of the area is only exposed in each valley, the control stratum thickness is about 85m, and it is in pseudointegrated contact with the underlying stratum.
- (2)
- The second rock section (J1-2y2): The area is from the coarse sandstone of the roof of the No. 5 coal formation to the bottom boundary of the sandstone of the roof of the No. 3 coal formation. The exposure in the ore field is incomplete, and only four coal groups remain. Its lithology is mainly sandy mudstone, siltstone, and mudstone intercalated with coarse-, medium-, and fine-grained sandstone, and the lower part is yellow thick-layered coarse sandstone. The rock is mainly composed of quartz, feldspar, and argillaceous cementation and is generally loose. Some sections are calcareous cementation, and the rock is spherical after weathering. The average thickness of the stratum is about 78m, with a small amount of exposure in the east of the ore field, which is in integrated contact with the underlying stratum.
- (3)
- Quaternary system (Q4): This is mainly exposed in the valley of the ore field, and the lithology is composed of an alluvial proluvial gravel layer, eluvial deluvial gravel, and secondary loess. The stratum thickness is 0~21.45m, with an average thickness of 8.49 m, and there is angular unconformity between all old strata.
2.1.2. Geological Structure
2.1.3. Rock Mass Structure Type
2.1.4. Engineering Geological Factors
- (1)
- Engineering geological characteristics
- (2)
- Engineering geological characteristics of coal seam and roof and floor rock
- (3)
- Type of engineering geological exploration
2.2. Determination of Physical and Mechanical Indexes of Rock and Soil Mass
3. Slope Stability Analysis
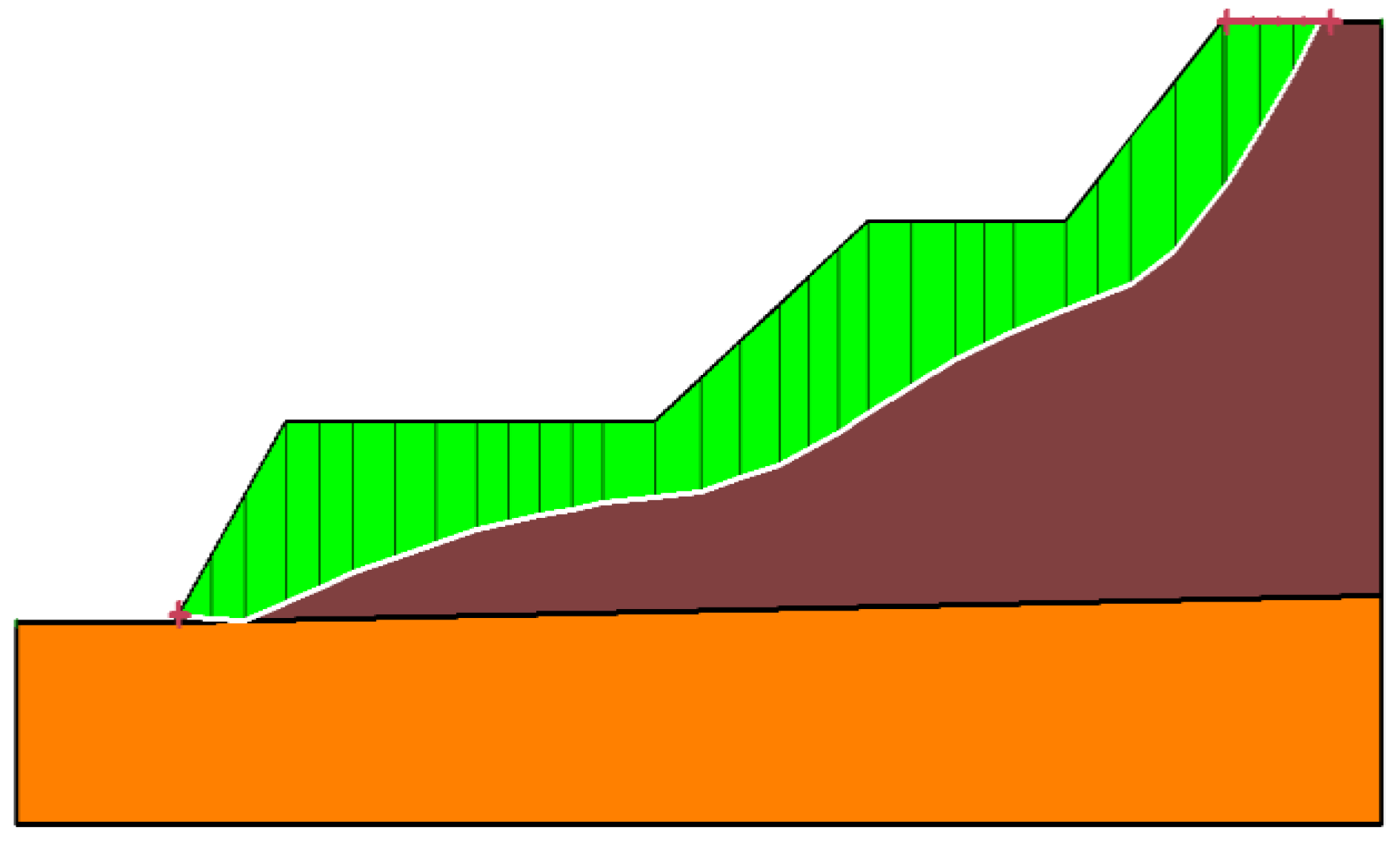
3.1. Limit Equilibrium
3.1.1. Overview of Limit Equilibrium Theory
- (1)
- Definition of safety and stability factor
- (2)
- Molar Coulomb strength criterion
- (3)
- Force balance conditions
3.1.2. Selection of Methods
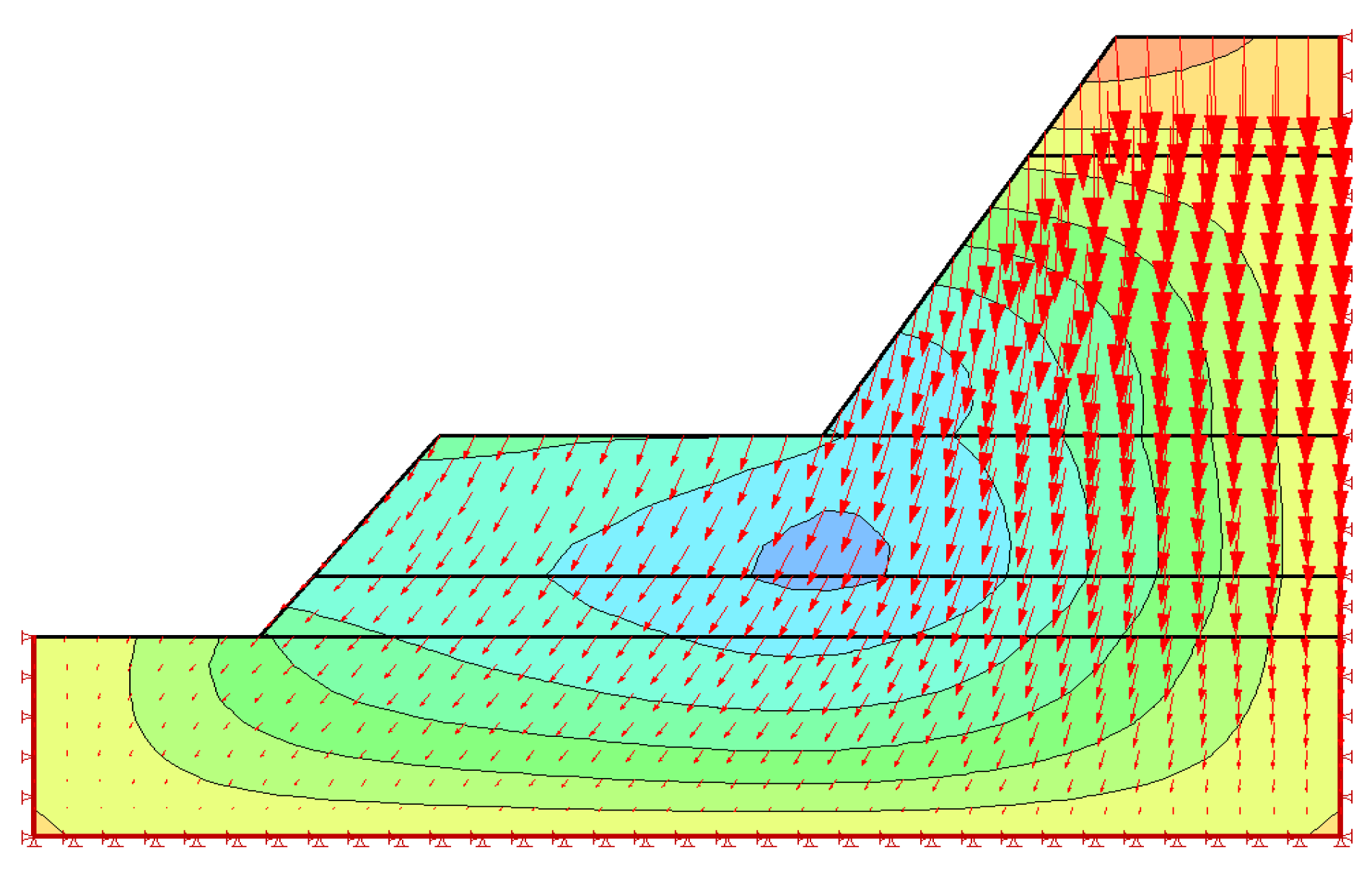
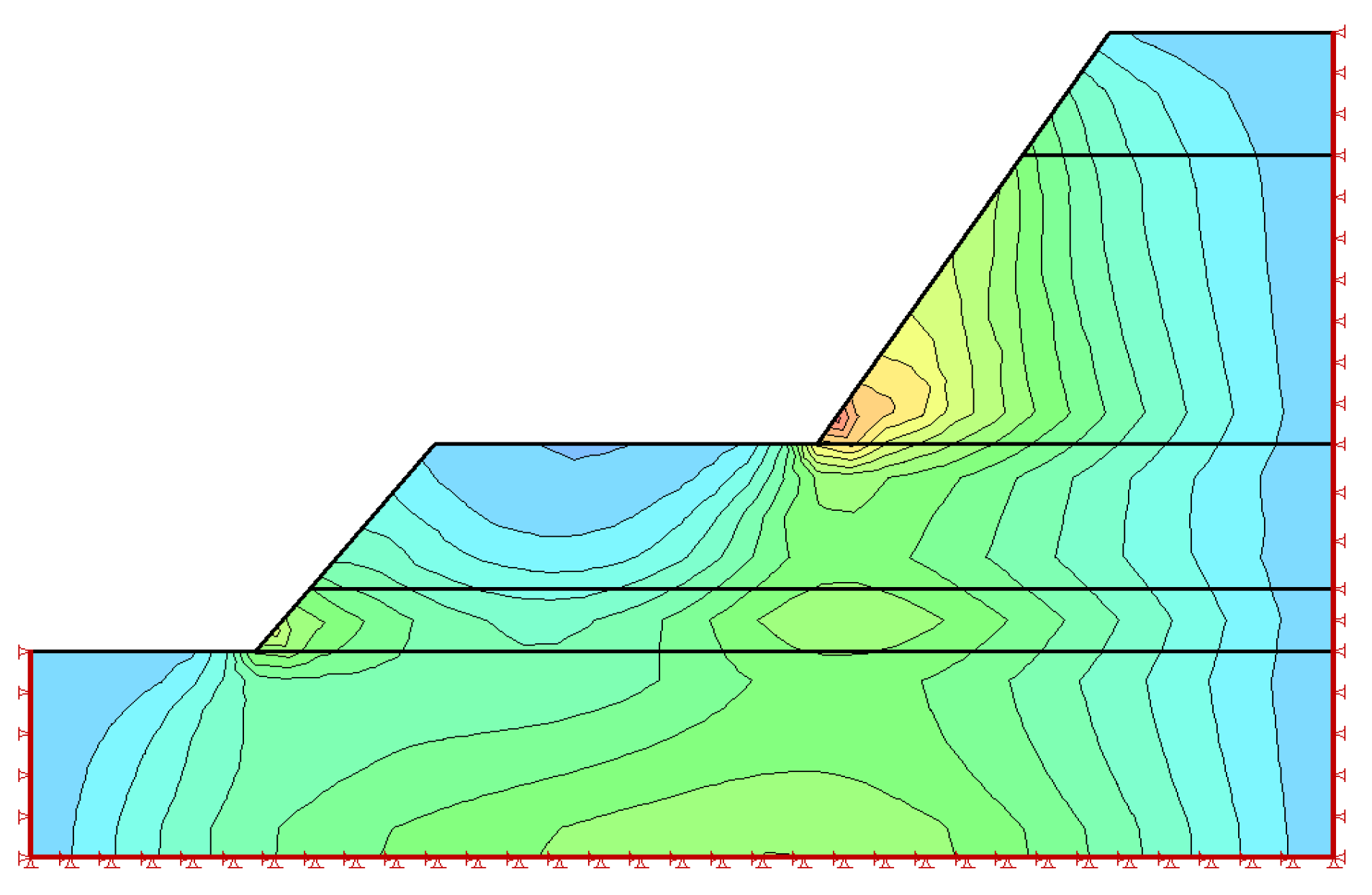
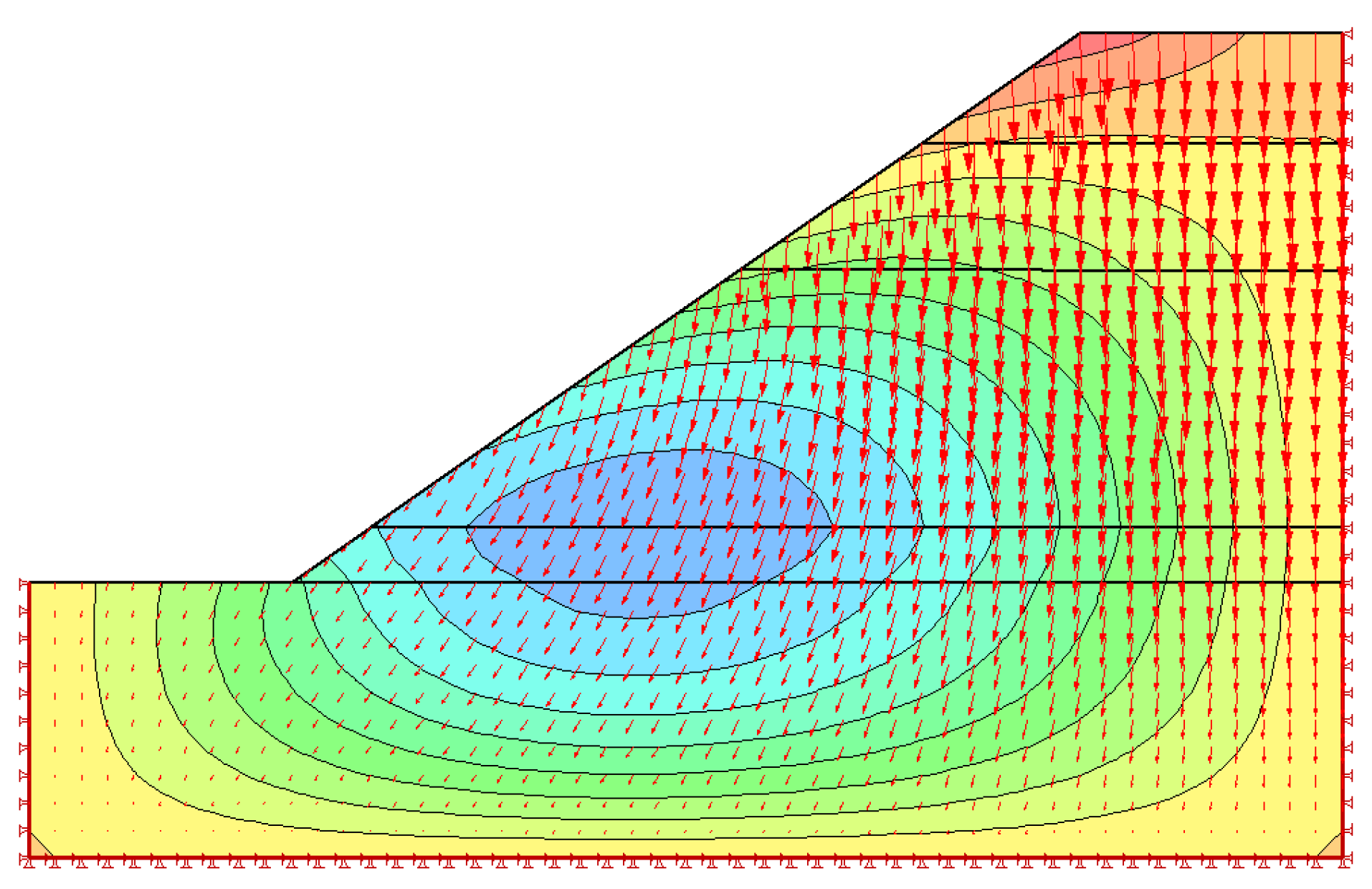
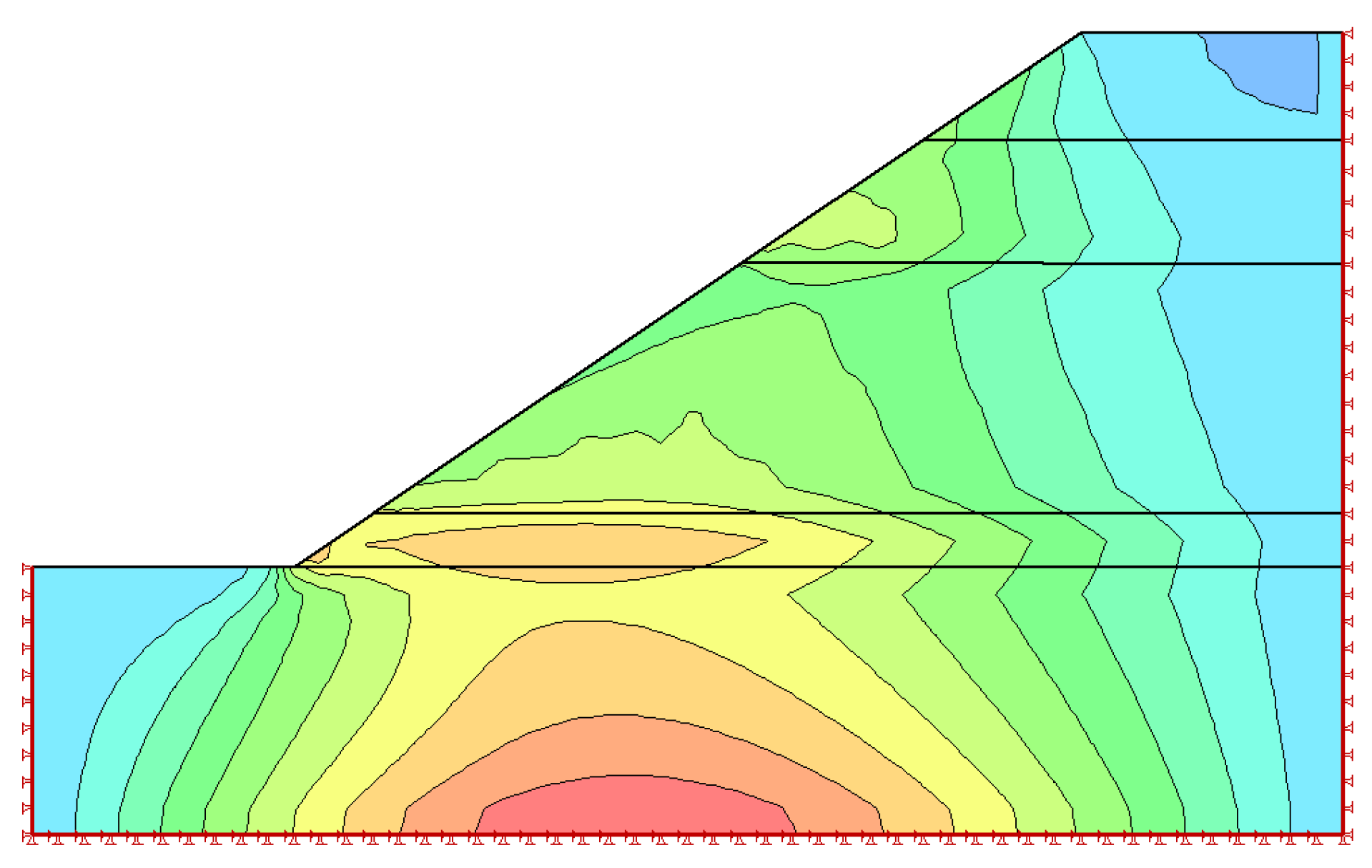
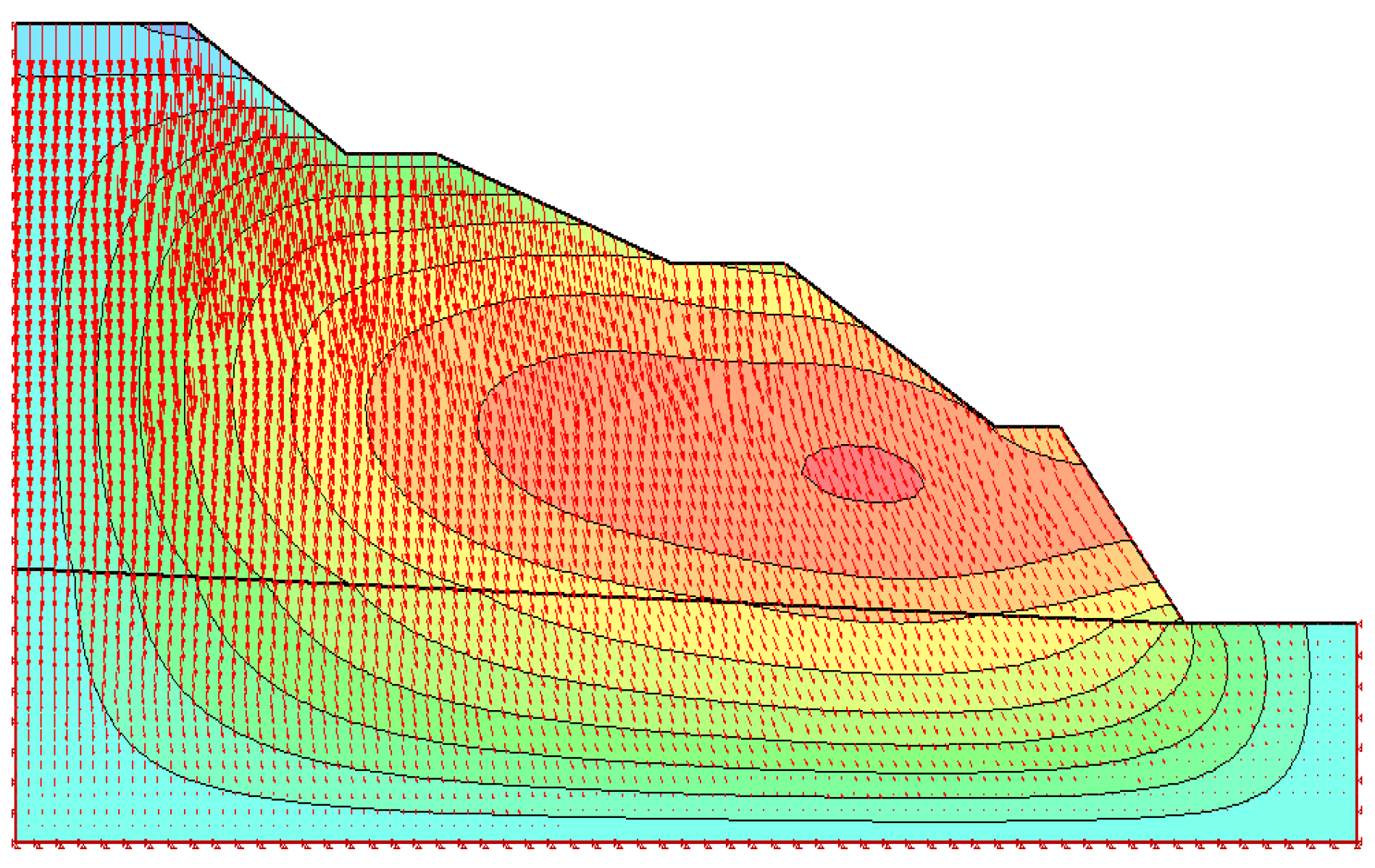
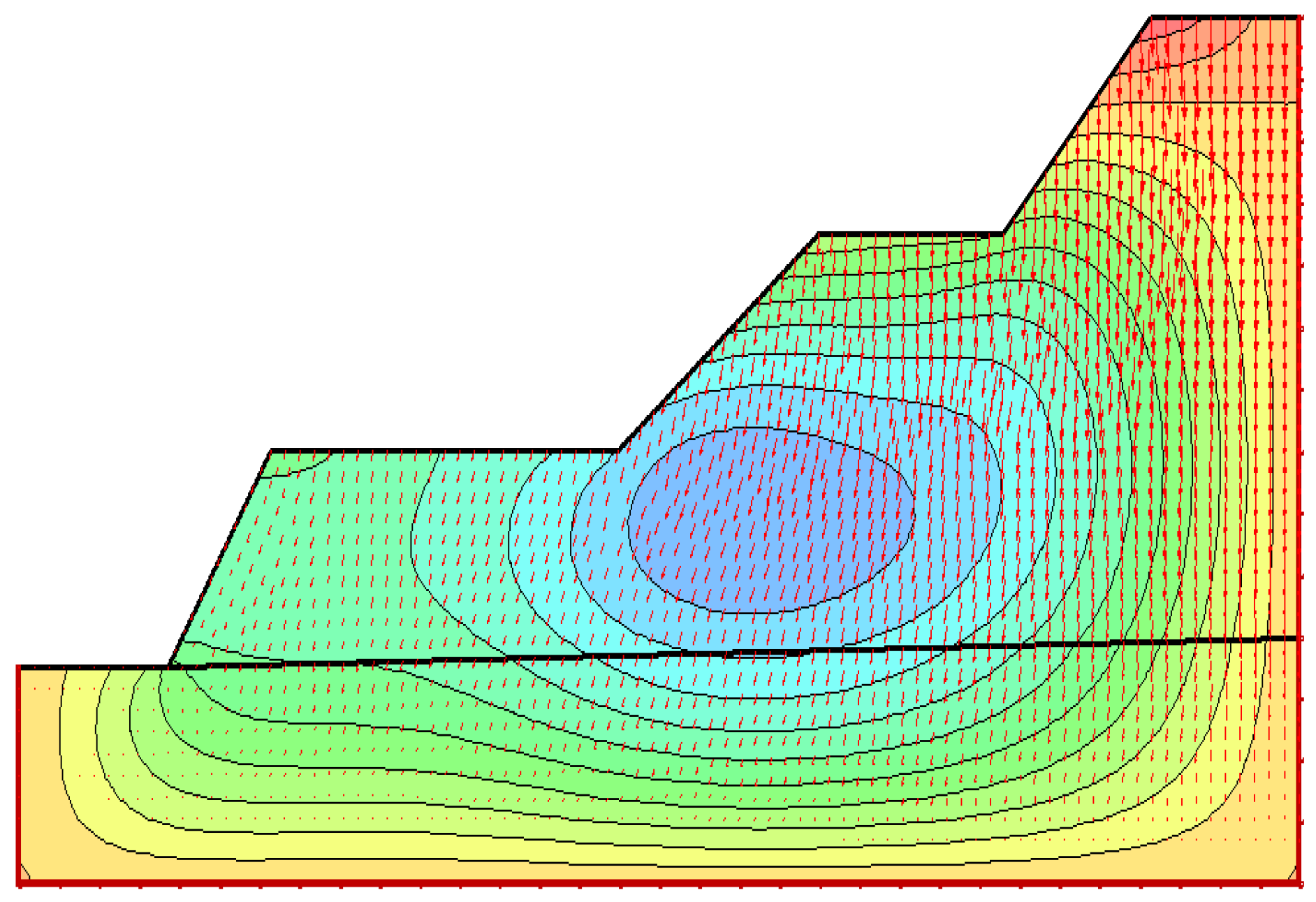
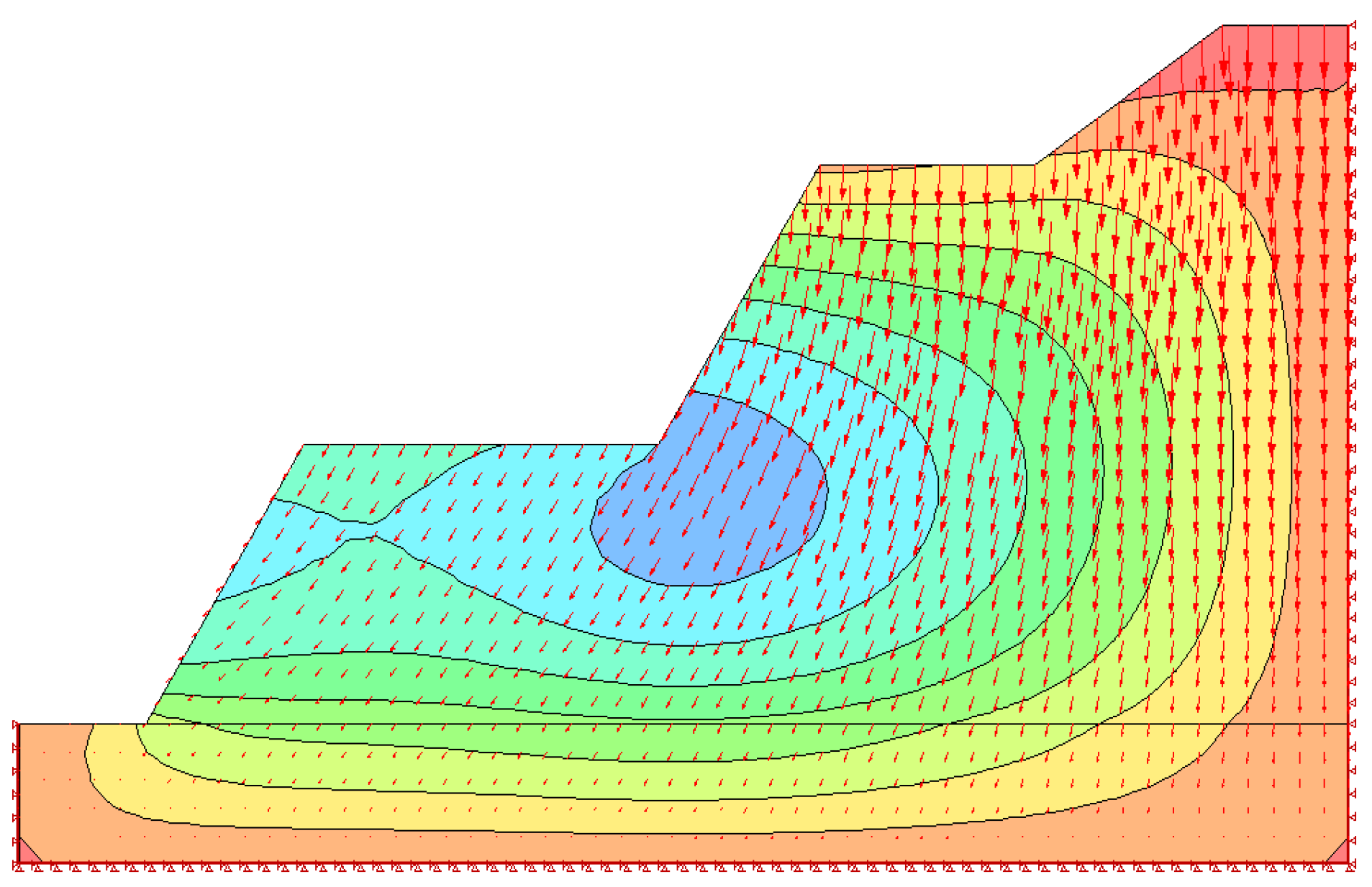
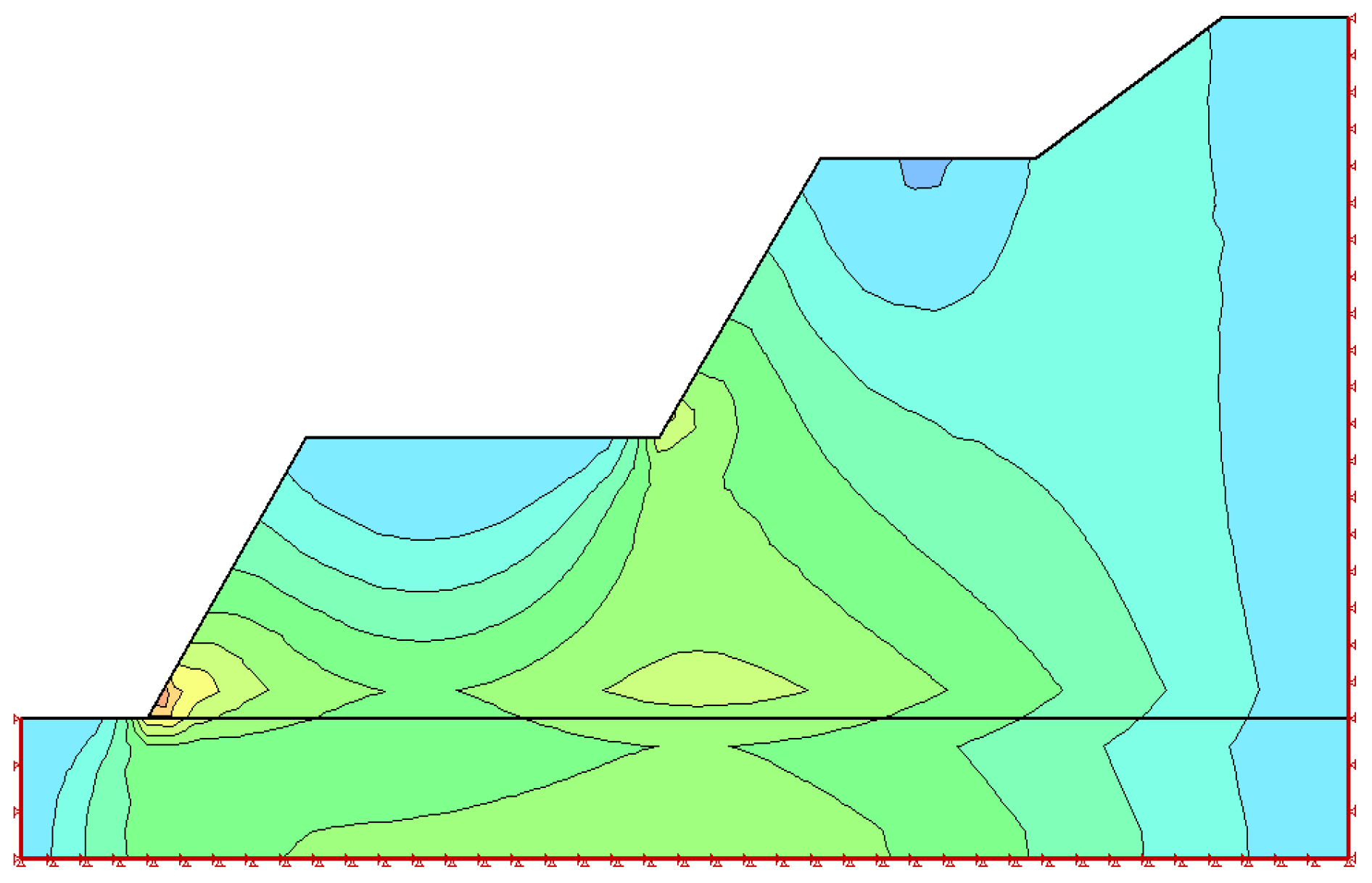
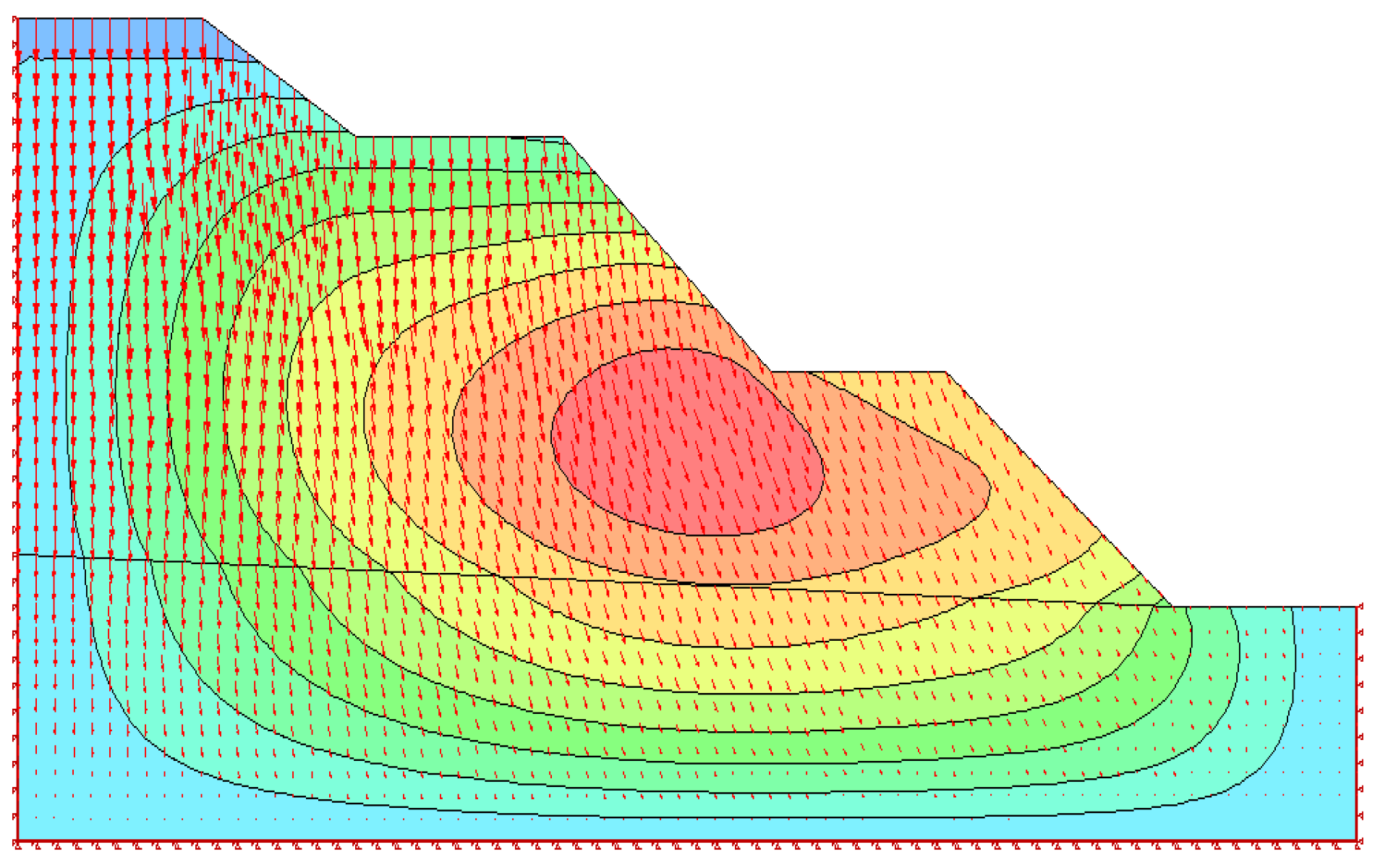
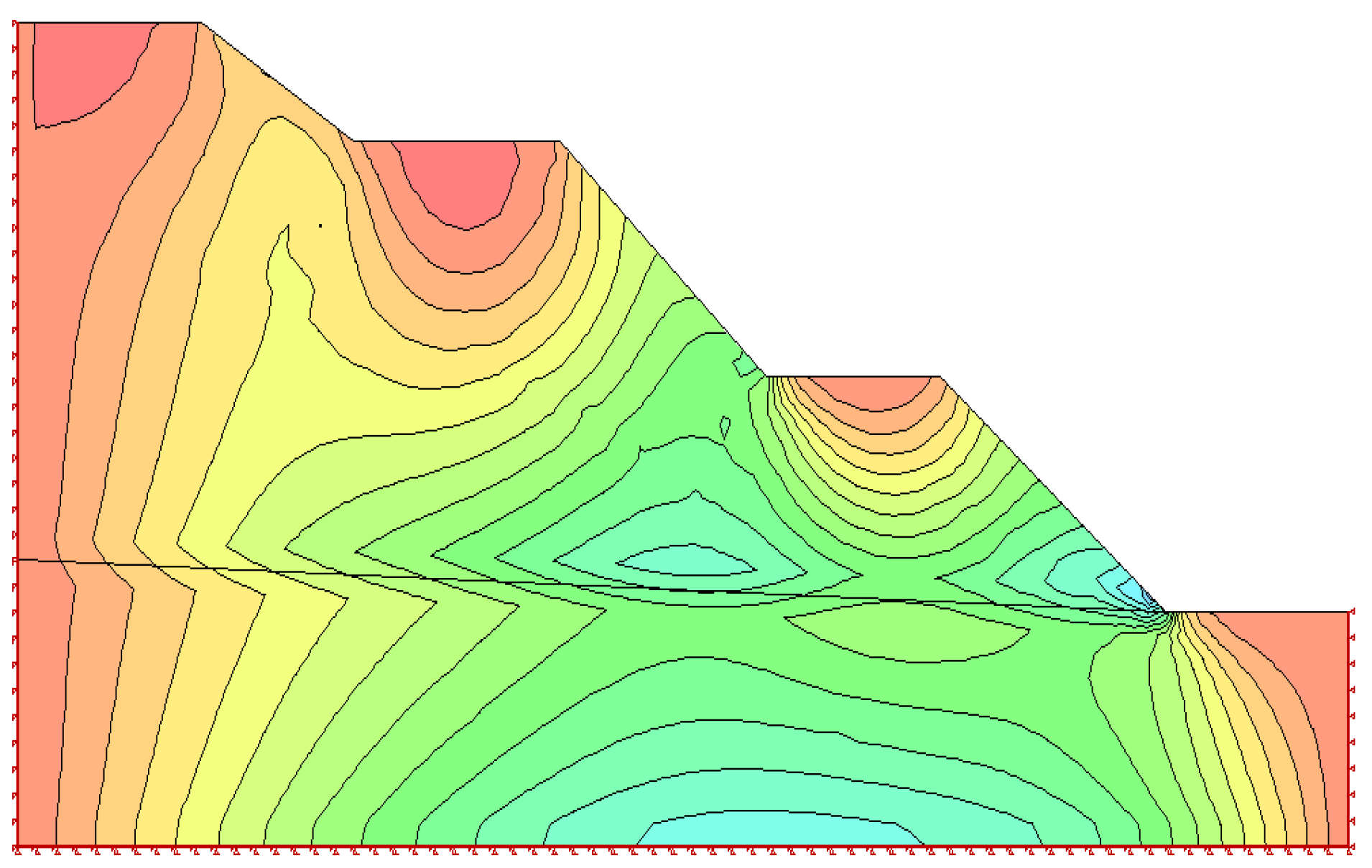
3.2. Numerical Simulation
- (1)
- Mathematics mechanics principle of finite element analysis
3.3. Determination of Stability Reserve Coefficient
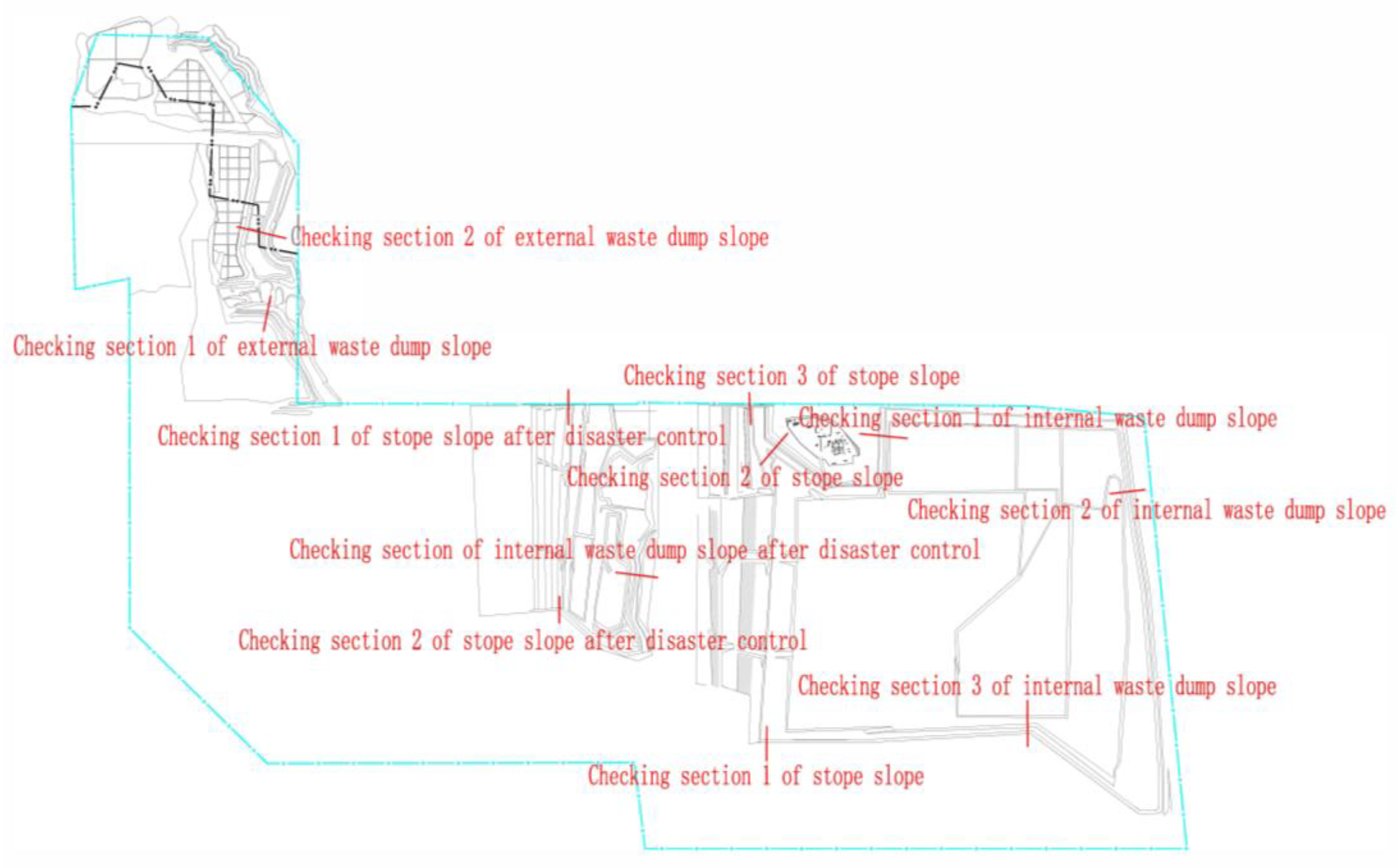
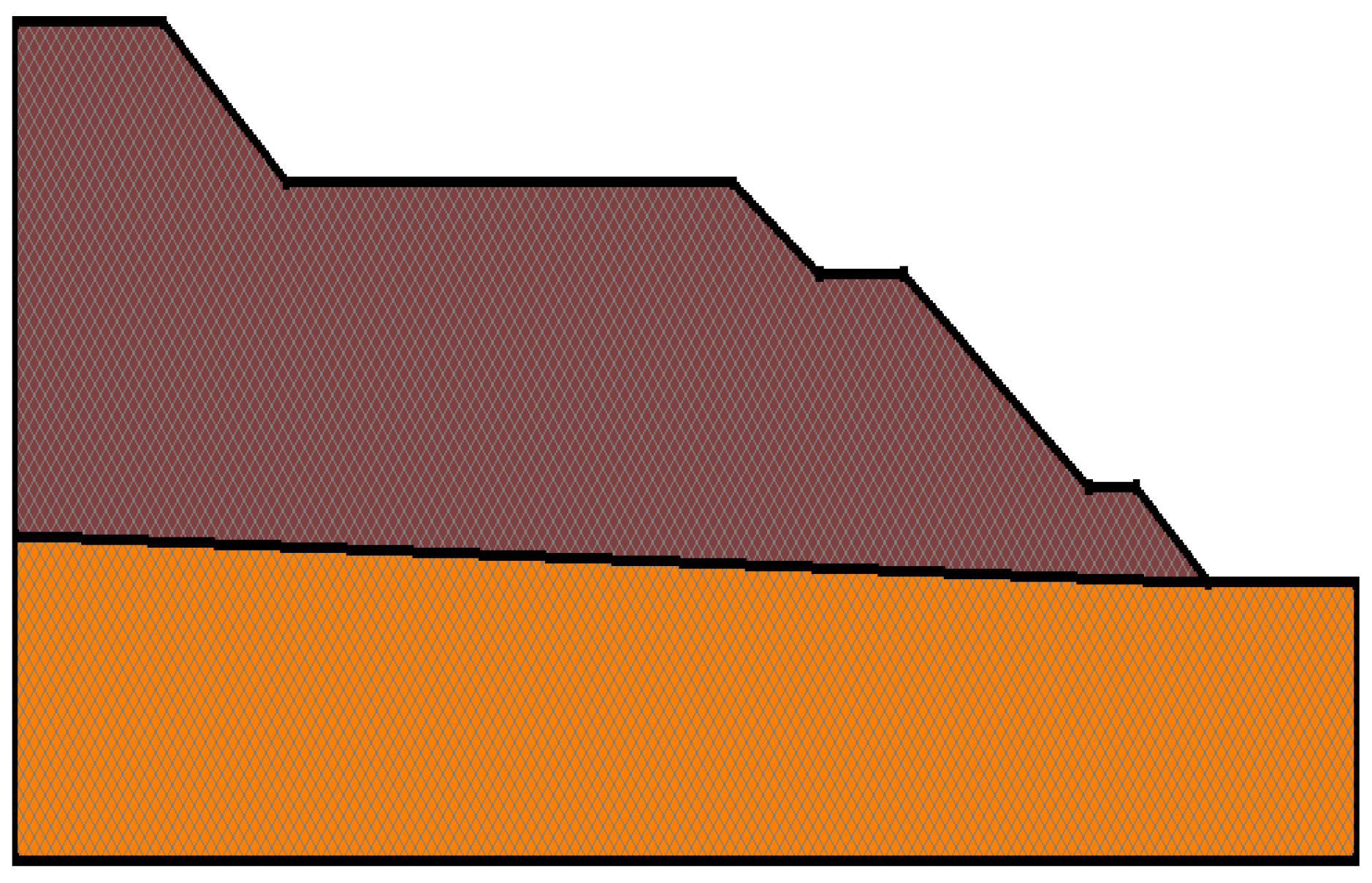
3.4. Selection of Checking Section
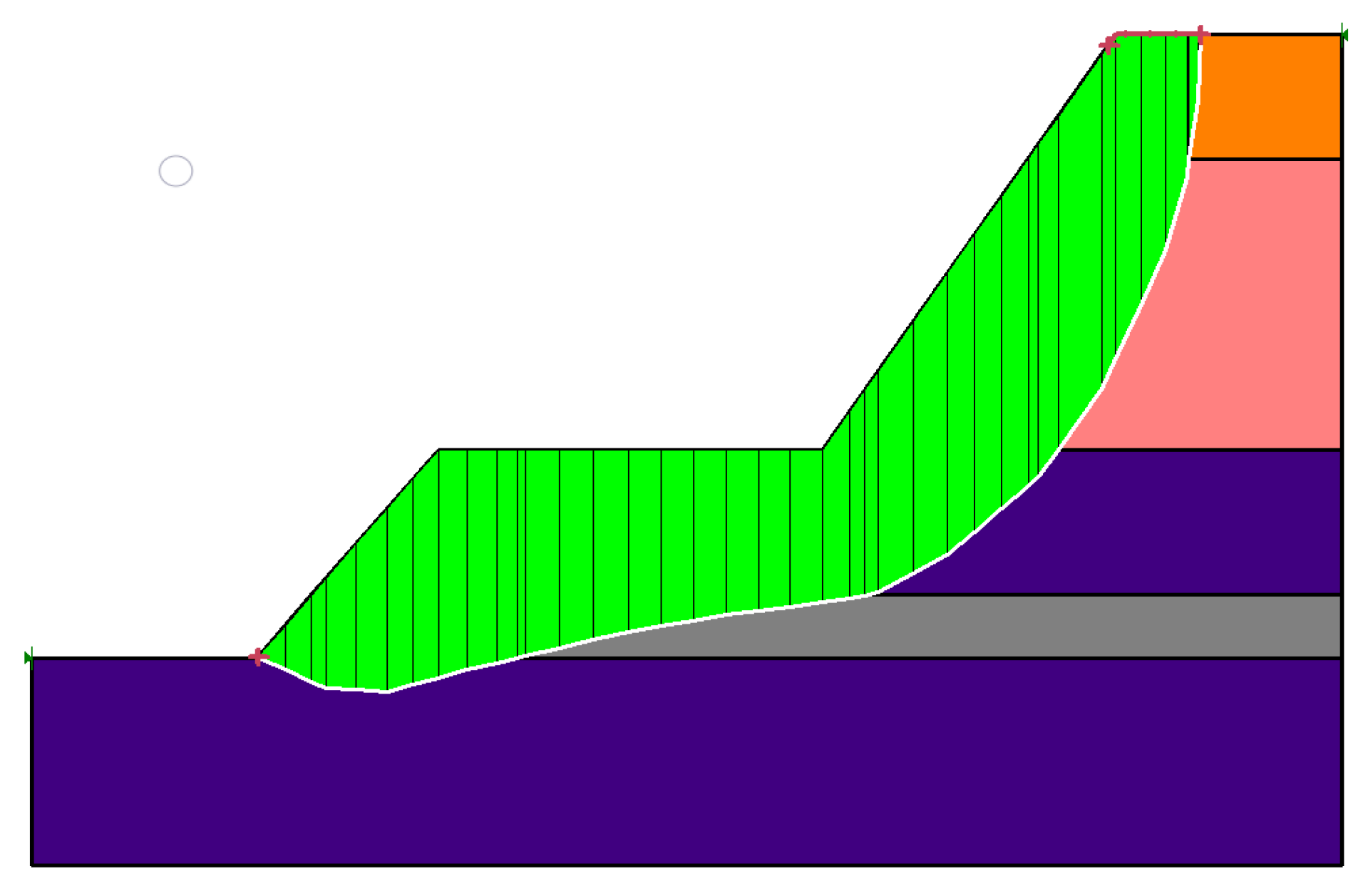
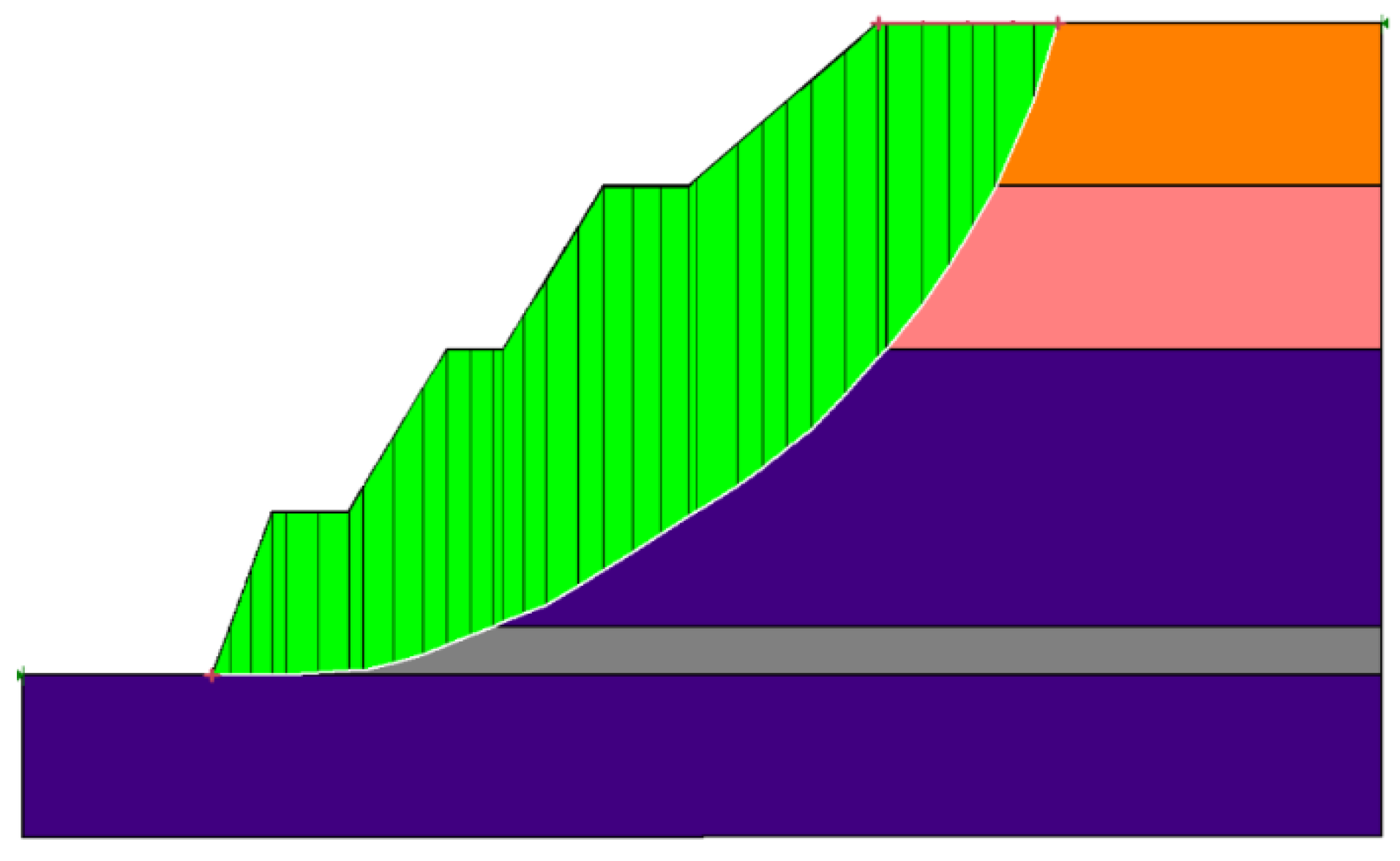
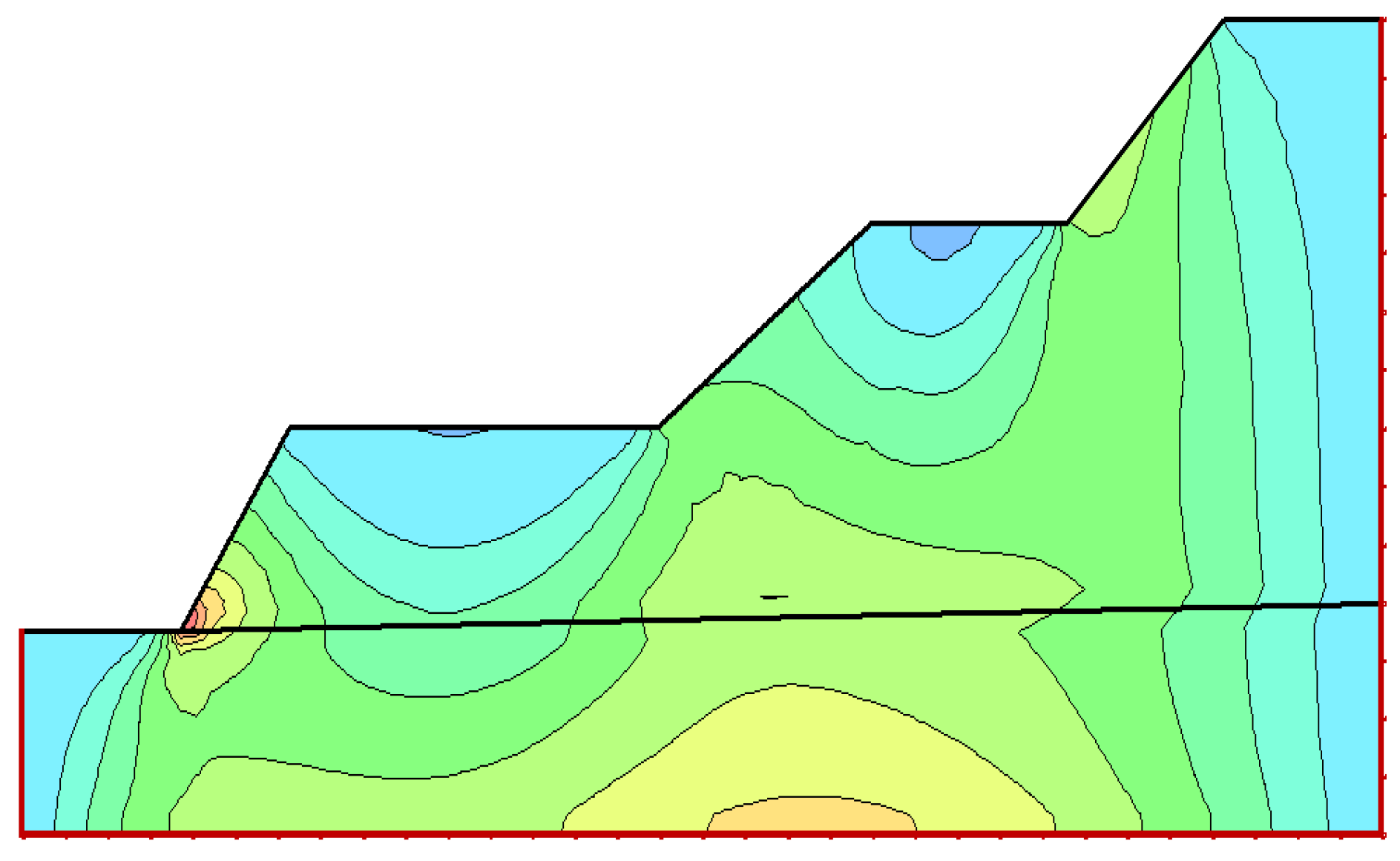
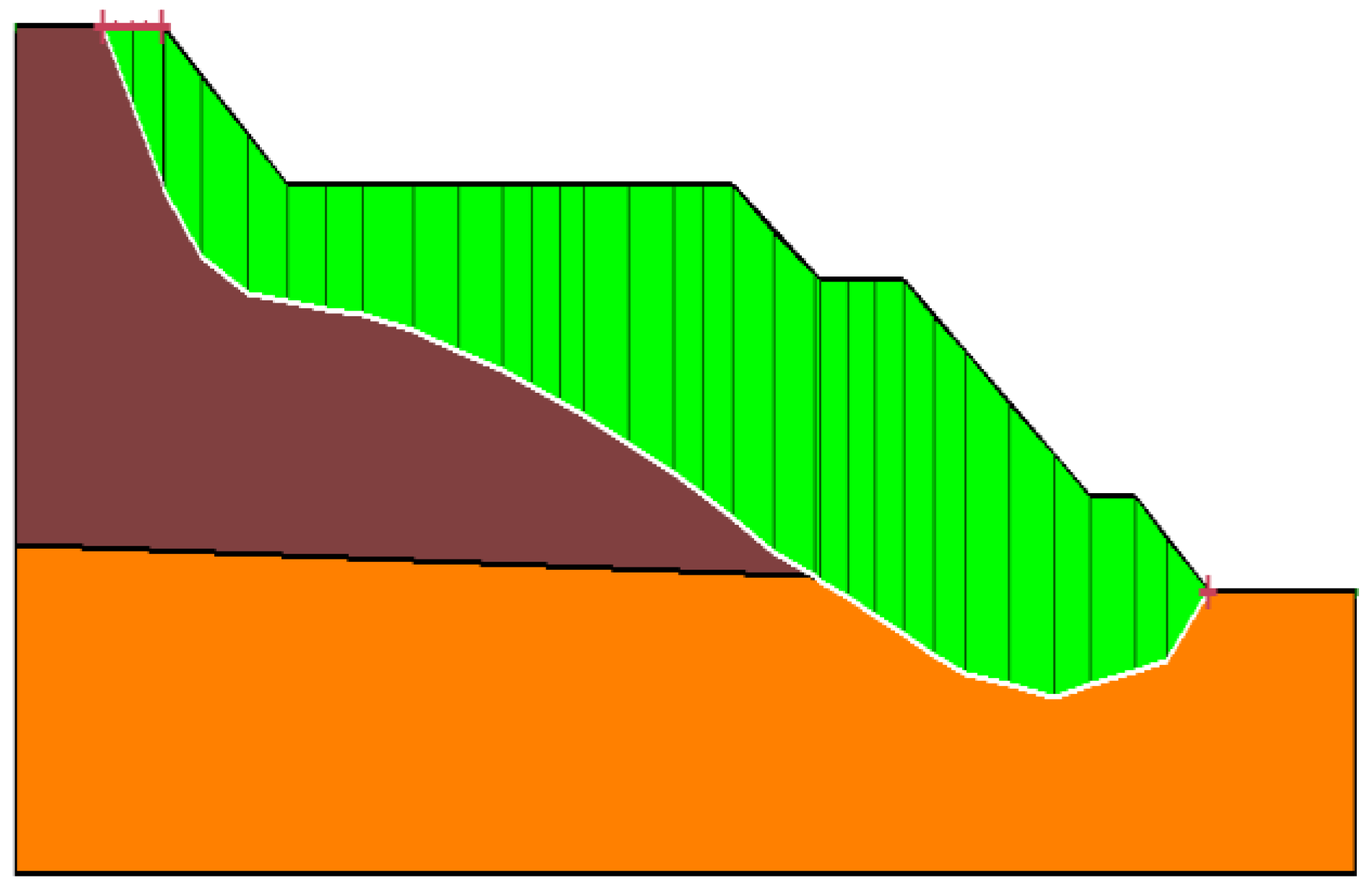
3.5. Checking Calculation of Stope Slope Stability after Disaster Control
- (1)
- Checking section 1 of the stope slope after disaster control
- (2)
- Checking section 2 of the stope slope after disaster control
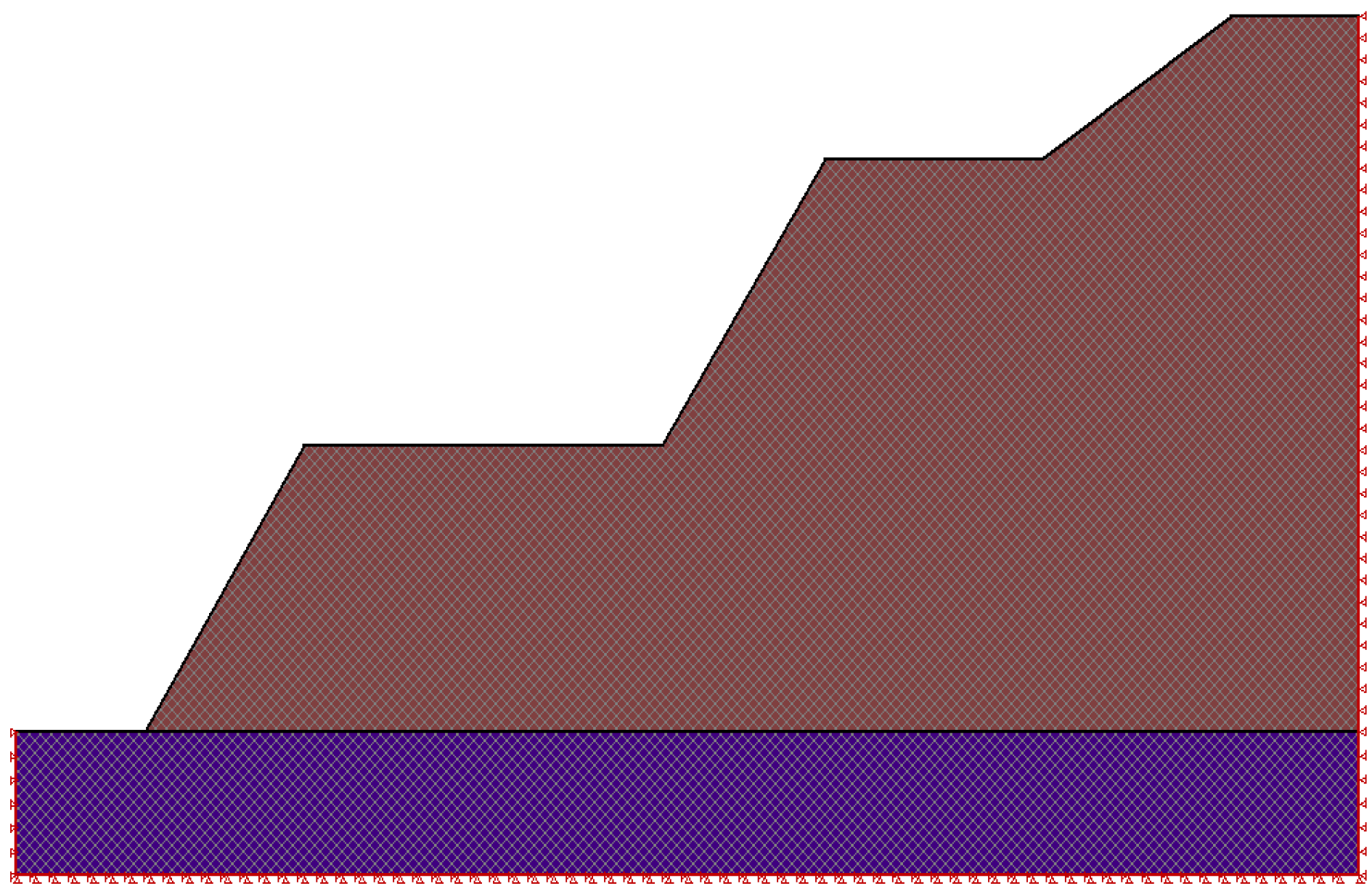
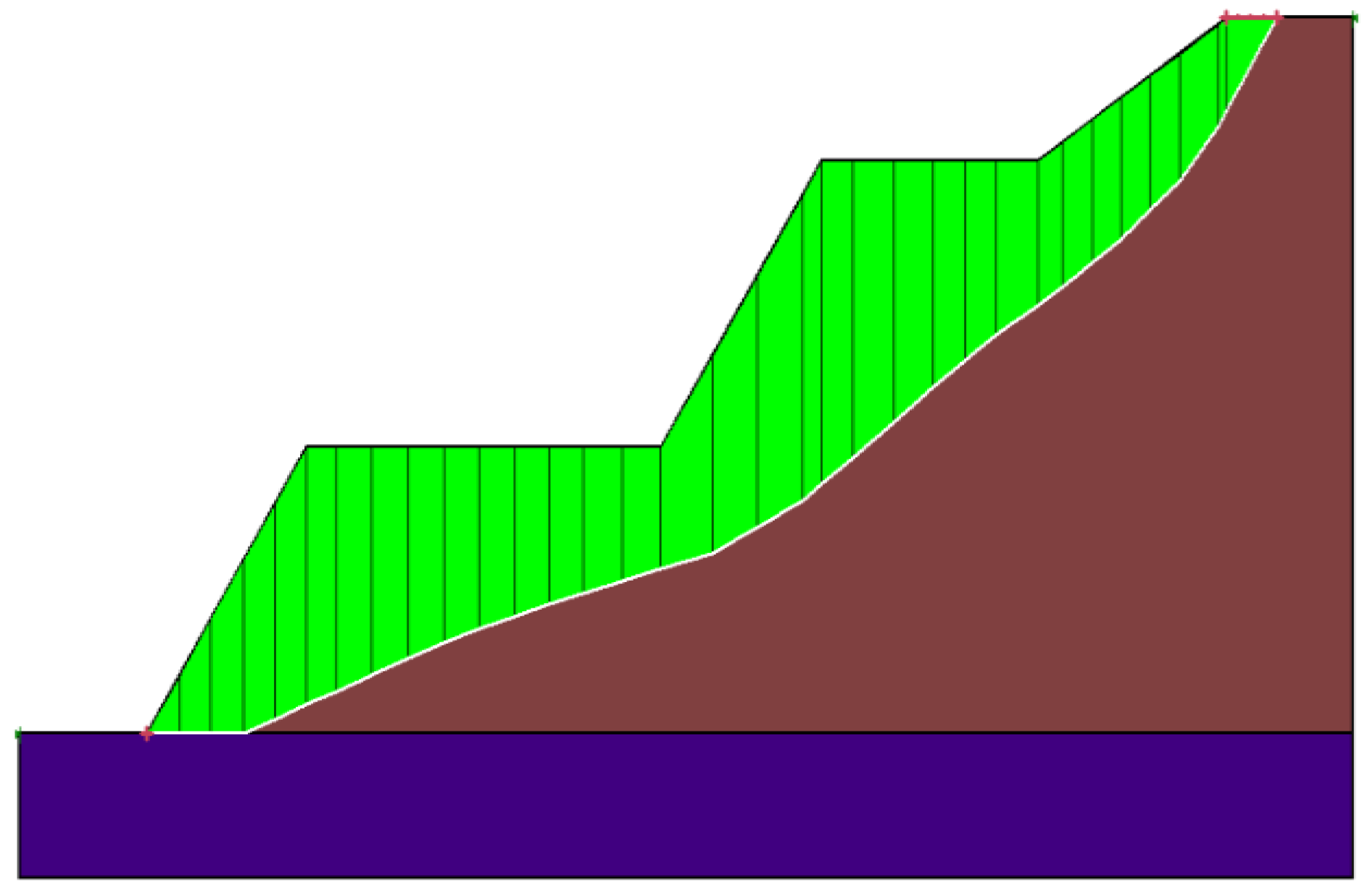
3.6. Checking Calculation of Stope Slope Stability in Open-Pit Mine
- (1)
- Checking section 1 of the stope slope
- (2)
- Checking section 2 of the stope slope
- (3)
- Checking section 3 of the stope slope
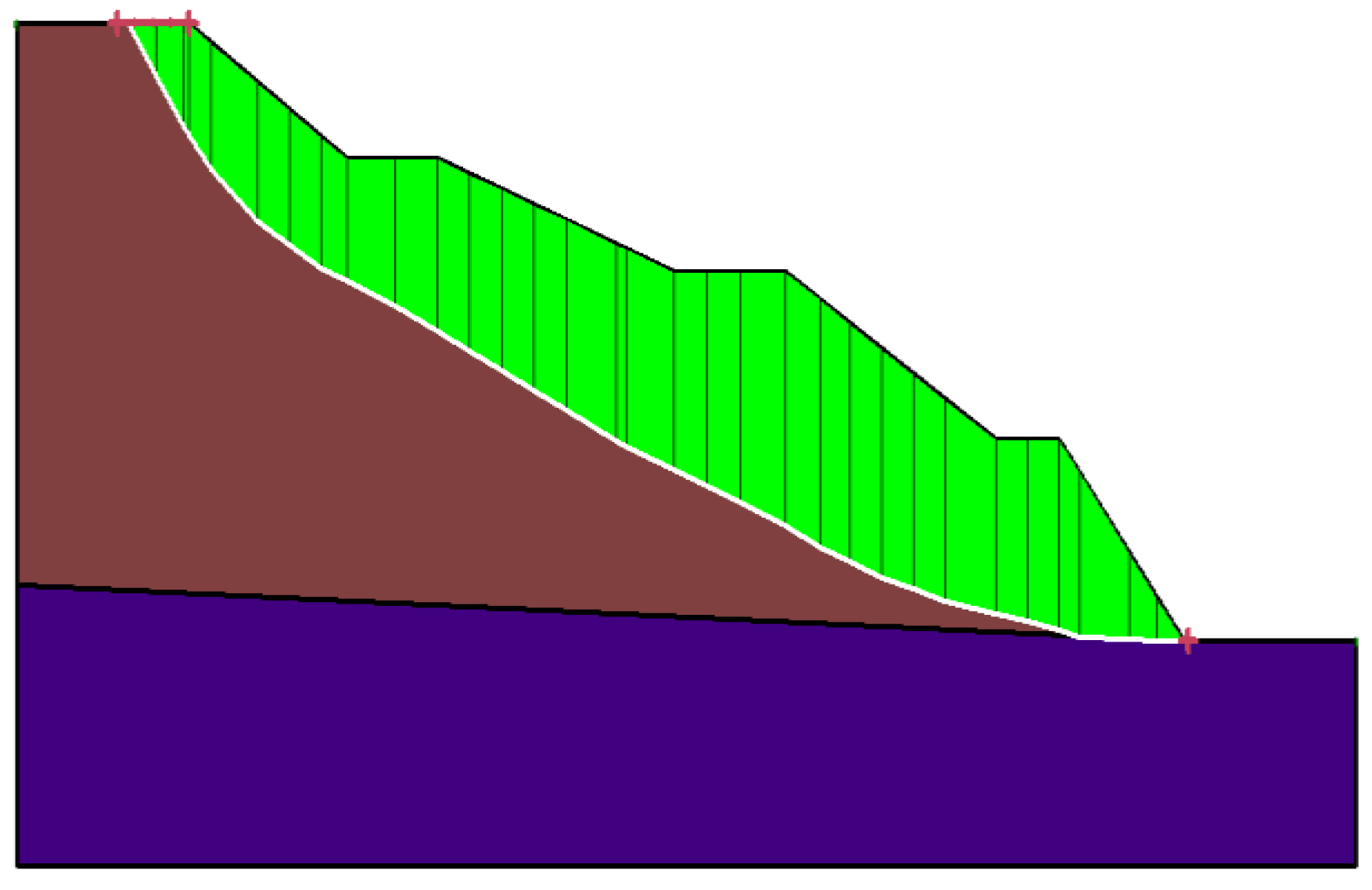
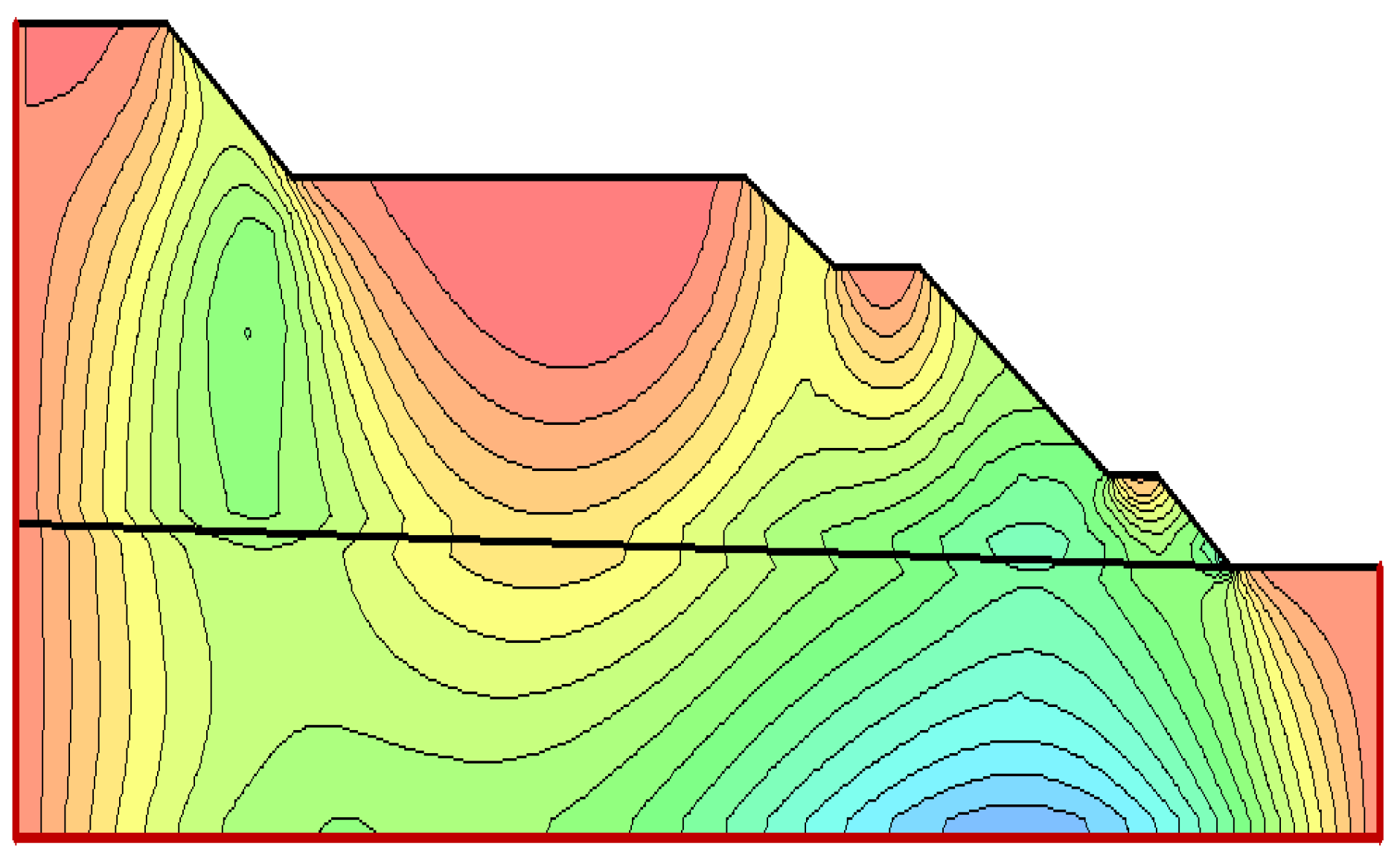
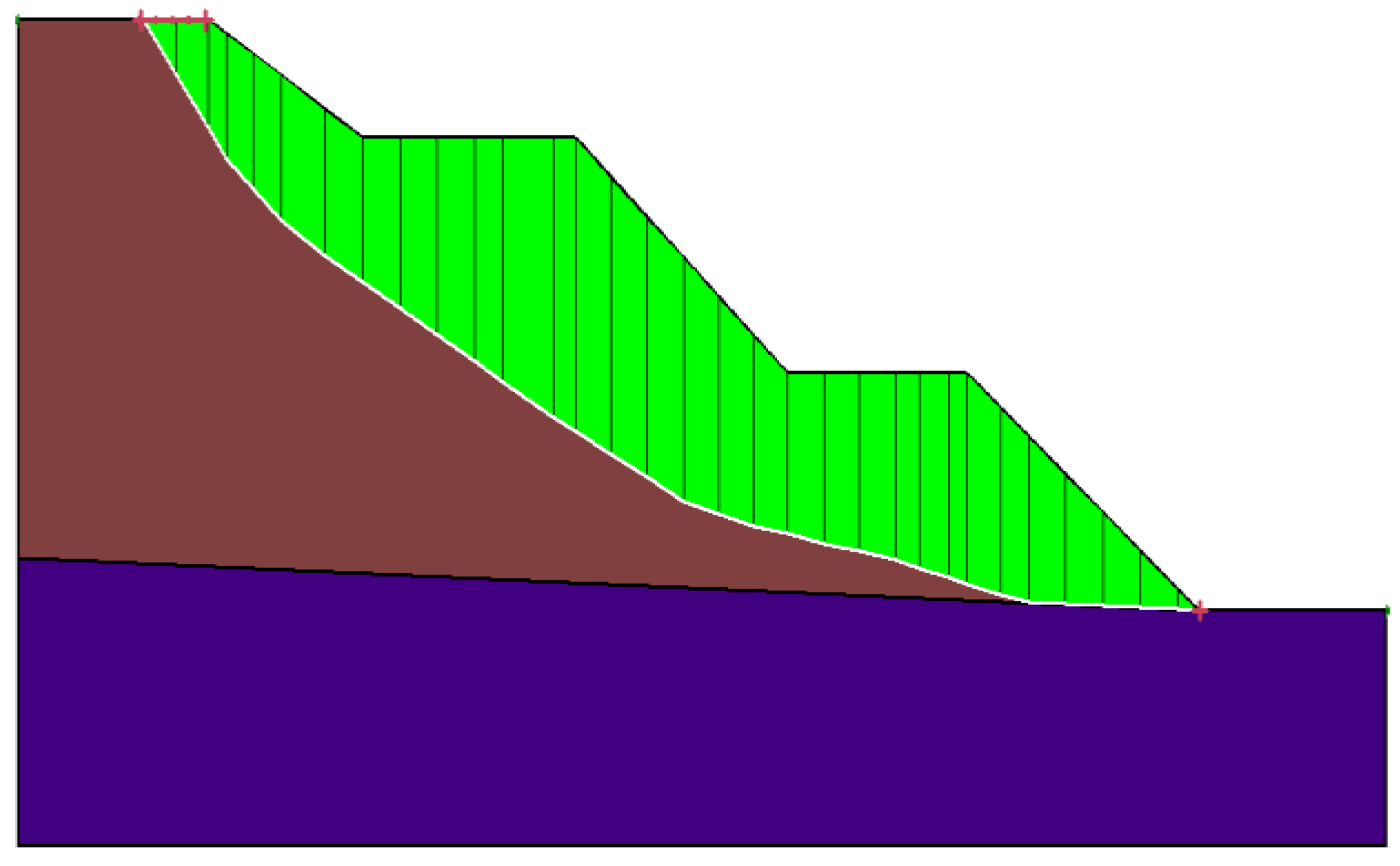
3.7. Checking Calculation of Slope Stability of Waste Dump after Disaster Control
3.8. Checking Calculation of Slope Stability of Open-Pit Waste Dump
- (1)
- Main factors affecting the stability of the waste dump
- (2)
- Checking the calculation of the slope stability of the waste dump
- (3)
- Checking section 1 of the inner waste slope
- (4)
- Checking section 2 of the inner waste dump slope
- (5)
- Checking section 3 of the inner waste dump slope
3.9. Evaluation Results
4. Conclusions and Recommendations
4.1. Conclusions
- (1)
- The geotechnical physical and mechanical indexes of the stope and the waste dump were collected and analyzed, and the geotechnical mechanical indexes in this report were determined, which basically meet the requirements of slope stability analysis.
- (2)
- The limit equilibrium method and finite element method were used to analyze and evaluate the current slope stability of the Nayuan open-pit coal mine. It was concluded that the foundation of the waste dump is basically stable, and the potential landslide modes of the slope are arc-shaped sliding surface and arc-shaped linear sliding surface. The numerical simulation and checking results show that the current stope and waste dump slope are stable.
- (3)
- Checking section 1 of the stope slope after disaster control is 30 m high, the slope angle is 38°, and the stability coefficients calculated by the Bishop method and the Morgenstern–Price method are 1.343 > 1.1 and 1.328 > 1.1, respectively. Checking section 2 of the stope slope after disaster control is 30 m high, the slope angle is 34°, and the stability coefficients calculated by the Bishop method and the Morgenstern–Price method are 1.362 > 1.1 and 1.356 > 1.1, respectively. Checking section 1 of the stope slope is 15 m high, the slope angle is 37°, and the stability coefficient calculated by the Bishop method and the Morgenstern–Price method are 1.423 > 1.1 and 1.419 > 1.1, respectively. Checking section 2 of the stope slope is 30 m high, and the slope angle is 25°. The stability coefficients calculated by the Bishop method and the Morgenstern–Price method are 1.378 > 1.1 and 1.374 > 1.1, respectively. Checking section 3 of the stope slope is 40 m high, and the slope angle is 47°. The stability coefficients calculated by the Bishop method and the Morgenstern–Price method are 1.161 > 1.1 and 1.157 > 1.1, respectively. The slope stability checking section of the waste dump after disaster control is 55 m high, and the slope angle is 25°. The stability coefficient calculated by the Bishop method is 1.263 > 1.2, and that calculated by the Morgenstern–Price method is 1.257 > 1.2. Checking calculation profile 1 of the waste dump slope is 60 m high, the slope angle is 32°, and the stability coefficients calculated by the Bishop method and the Morgenstern–Price method are 1.203 > 1.2 and 1.201 > 1.2, respectively. Checking calculation profile 2 of the waste dump slope is 60 m high, the slope angle is 16°, and the stability coefficients calculated by the Bishop method and the Morgenstern–Price method are 1.313 > 1.2 and 1.312 > 1.2, respectively. Checking section 1 of the internal waste dump slope is 50 m high, and the slope angle is 30°. The stability coefficient calculated by the Bishop method is 1.218 > 1.2, and that calculated by the Morgenstern–Price method is 1.214 > 1.2. Slope checking section 2 of the inner waste dump is 50 m high, and the slope angle is 25°. The stability coefficient calculated by the Bishop method is 1.227 > 1.2, and that calculated by the Morgenstern–Price method is 1.226 > 1.2. Slope checking section 3 of the inner waste dump is 60 m high, and the slope angle is 24°. The stability coefficient calculated by the Bishop method is 1.231 > 1.2, and that calculated by the Morgenstern–Price method is 1.229 > 1.2.
4.2. Recommendations
- (1)
- Attach great importance to the slope management of the stope and the waste dump, and establish and improve the slope safety management organization system.
- (2)
- Strengthen slope deformation monitoring and rapidly assess the dynamic situation and the law of slope deformation, conduct real-time monitoring of the stope and waste dump slopes, and assign special personnel for supervision. Once signs of a landslide are found, evacuate personnel and equipment immediately and carry out a special investigation, evaluation, and treatment engineering design for any local and small-scale slope collapses and slides.
- (3)
- This paper checked and analyzed the slope according to the actual situation of the coal mine, which is only applicable to the current stage. It is suggested that the coal mine should carry out mining and stripping operations in strict accordance with the design and specification requirements in the future production process, strengthen slope monitoring, take prevention measures immediately when there are signs of a landslide on the slope, and organize the evacuation of personnel and equipment as soon as possible to ensure the safety of personnel and equipment.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Zhu, X. Application of Gravity Increase Method in Slope Stability Analysis; Xi’an University of Science and Technology: Xi’an, China, 2010. [Google Scholar]
- Kong, D. Analysis Theory and Practice of Slope Stability; Hefei University of Technol: Hefei, China, 2009. [Google Scholar]
- Cundall, P.A. Formulation of a three-dimensional distinct element model—Part I: A scheme to detect and represent contacts in a system composed of many polyhedral blocks. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1988, 25, 107–116. [Google Scholar] [CrossRef]
- Zienkiewicz, O.C.; Humpheson, C.; Lewis, R.W. Associated and non—Associated viseo-plasticity and plasticity in soil mechanics. Geotechnique 1975, 25, 671–689. [Google Scholar] [CrossRef]
- Ghaboussi, J.R.; Barboosa, R. Three-dimensional and discrete element methods for granular material. Int. J. Numer. Anal. Methods Geomech. 1990, 14, 451–472. [Google Scholar] [CrossRef]
- Shi, G.H. Numerical Manifold Method and Discontinuous Deformation Analysis; Pei, J.M., Translator; Tsinghua University Press: Beijing, China, 1997. [Google Scholar]
- Zhuo, J.S.; Zhang, Q. Interface Element Method for Mechanical Problems of Discontinuous Media; Pei, J.M., Translator; Science Press: Beijing, China, 2000. [Google Scholar]
- Wu, S.C.; Jin, A.B.; Liu, Y. Slope Engineering; Metallurgical Industry Press: Beijing, China, 2020. [Google Scholar]
- Jin, F.C. Research status and Prospect of slope stability methods. West-China Explor. Eng. 2007, 4, 5–6. [Google Scholar]
- Wang, Y.P. Summary of slope stability analysis methods. J. Xihua Univ. 2012, 31, 101–102. [Google Scholar]
- Xiang, J. Study on Stability Analysis and Prevention of Cutting Slope of Tongping Expressway; Central South University: Changsha, China, 2011. [Google Scholar]
- Zhu, C.B. Analysis and Evaluation of Slope Stability—Taking the Slope of Yuanjiang-Mohei Expressway as an Example; Kunming University of Technology: Kunming, China, 2005. [Google Scholar]
- Wang, J.C. Stochastic Analysis Principle of Slope Engineering; Coal Industry Press: Beijing, China, 1996. [Google Scholar]
- Chen, X.M.; Luo, G.H. Grey system analysis and evaluation of slope stability based on experience. J. Geotech. Eng. 1999, 21, 638–641. [Google Scholar]
- Wang, Y.X. Application of fuzzy mathematics in slope stability analysis. J. Geotech. Eng. 2010, 31, 30. [Google Scholar]
- Xiong, H.F. Study on Slope Stability Evaluation Based on Artificial Neural Network Technology; Wuhan University of Technology: Wuhan, China, 2003. [Google Scholar]
- Bishop, A.W. The use of the slip circle in the stability analysis of slopes. Geothenique 1955, 5, 7–17. [Google Scholar] [CrossRef]
- Morgenstern, N.R.; Price, V.E. The analysis of the stability of general slip surface. Geotechnique 1965, 15, 79–93. [Google Scholar] [CrossRef]
- Cheng, J. Residential land leasing and price under public land ownership. J. Urban Plan. Dev. 2021, 147, 05021009. [Google Scholar] [CrossRef]
- Cheng, J. Analysis of commercial land leasing of the district governments of Beijing in China. Land Use Policy 2021, 100, 104881. [Google Scholar] [CrossRef]
- Cheng, J. Analyzing the factors influencing the choice of the government on leasing different types of land uses: Evidence from Shanghai of China. Land Use Policy 2020, 90, 104303. [Google Scholar] [CrossRef]
- Cheng, J. Data analysis of the factors influencing the industrial land leasing in Shanghai based on mathematical models. Math. Probl. Eng. 2020, 2020, 9346863. [Google Scholar] [CrossRef]
- Cheng, J. Mathematical models and data analysis of residential land leasing behavior of district governments of Beijing in China. Mathematics 2021, 9, 2314. [Google Scholar] [CrossRef]
- Zheng, G.D.; Cheng, Y.M. The improved element-free Galerkin method for diffusional drug release problems. Int. J. Appl. Mech. 2020, 12, 2050096. [Google Scholar] [CrossRef]
- Wu, Q.; Peng, P.P.; Cheng, Y.M. The interpolating element-free Galerkin method for elastic large deformation problems. Sci. China Technol. Sci. 2021, 64, 364–374. [Google Scholar] [CrossRef]
- Cheng, H.; Peng, M.J.; Cheng, Y.M. The hybrid complex variable element-free Galerkin method for 3D elasticity problems. Eng. Struct. 2020, 219, 110835. [Google Scholar] [CrossRef]
- Li, J.; Wu, J. Study on elastoplastic damage constitutive model of concrete I:Basic formula. J. Civ. Eng. 2005, 38, 14–20. [Google Scholar]
- Wu, J. The elastoplastic Damage Constitutive Model of Concrete Based on the Damage Energy Release Rate and the Application in Structural Nonlinear Analysis. Ph.D. Thesis, Tongji University, Shanghai, China, 2004. [Google Scholar]
- Ma, W.B.; Chai, J.F.; Li, Z.F.; Han, Z.L.; Ma, C.F.; Li, Y.J.; Zhu, Z.Y.; Liu, Z.Y.; Niu, Y.B.; Ma, Z.G.; et al. Research on vibration Law of railway tunnel substructure under different axle loads and health conditions. Shock Vib. 2021, 2021, 9954098. [Google Scholar] [CrossRef]
- Han, Z.L.; Ma, W.B.; Chai, J.F.; Zhu, Z.Y.; Lin, C.N.; An, Z.L.; Ma, C.F.; Xu, X.L.; Xu, T.Y. A Treatment Technology for Optimizing the Stress State of Railway Tunnel Bottom Structure. Shock Vib. 2021, 2021, 9191232. [Google Scholar] [CrossRef]
- Ma, W.B.; Chai, J.F.; Han, Z.L.; Ma, Z.G.; Guo, X.X.; Zou, W.H.; An, Z.L.; Li, T.F.; Niu, Y.B. Research on Design Parameters and Fatigue Life of Tunnel Bottom Structure of Single-Track Ballasted Heavy-Haul Railway Tunnel with 40-Ton Axle Load. Math. Probl. Eng. 2020, 2020, 3181480. [Google Scholar] [CrossRef]
- Chai, J.F. Research on Multijoint Rock Failure Mechanism Based on Moment Tensor Theory. Math. Probl. Eng. 2020, 2020, 6816934. [Google Scholar] [CrossRef]







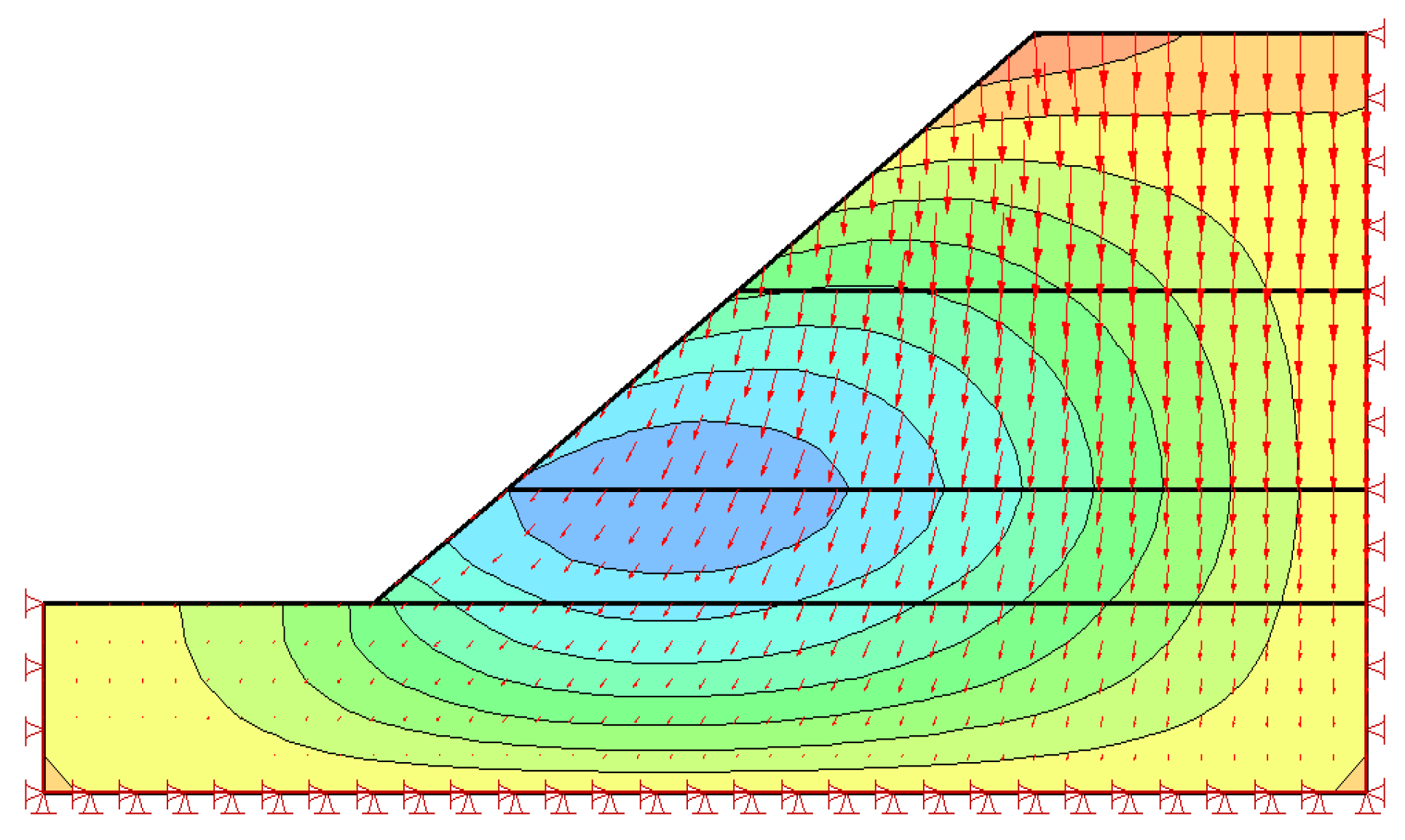
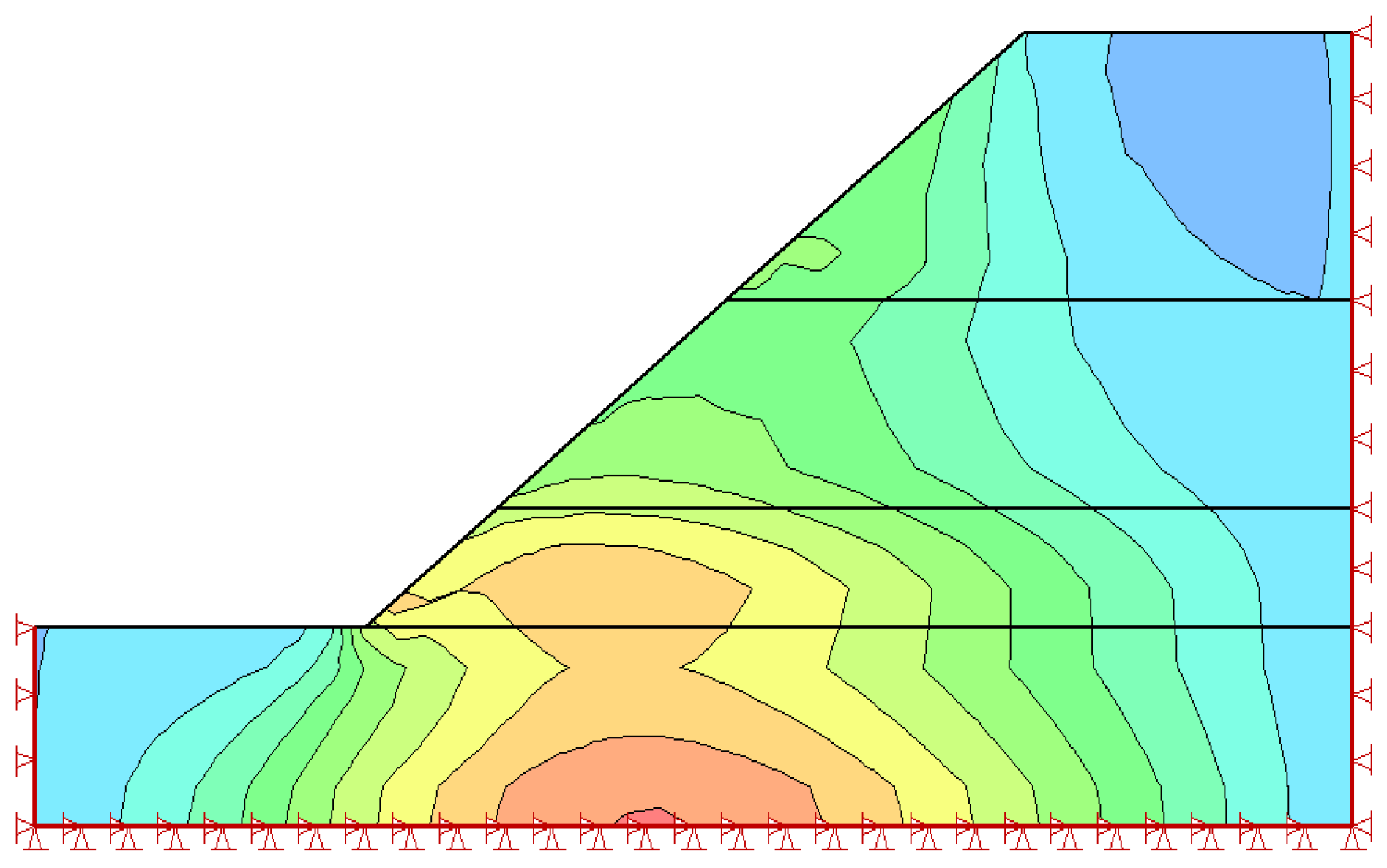
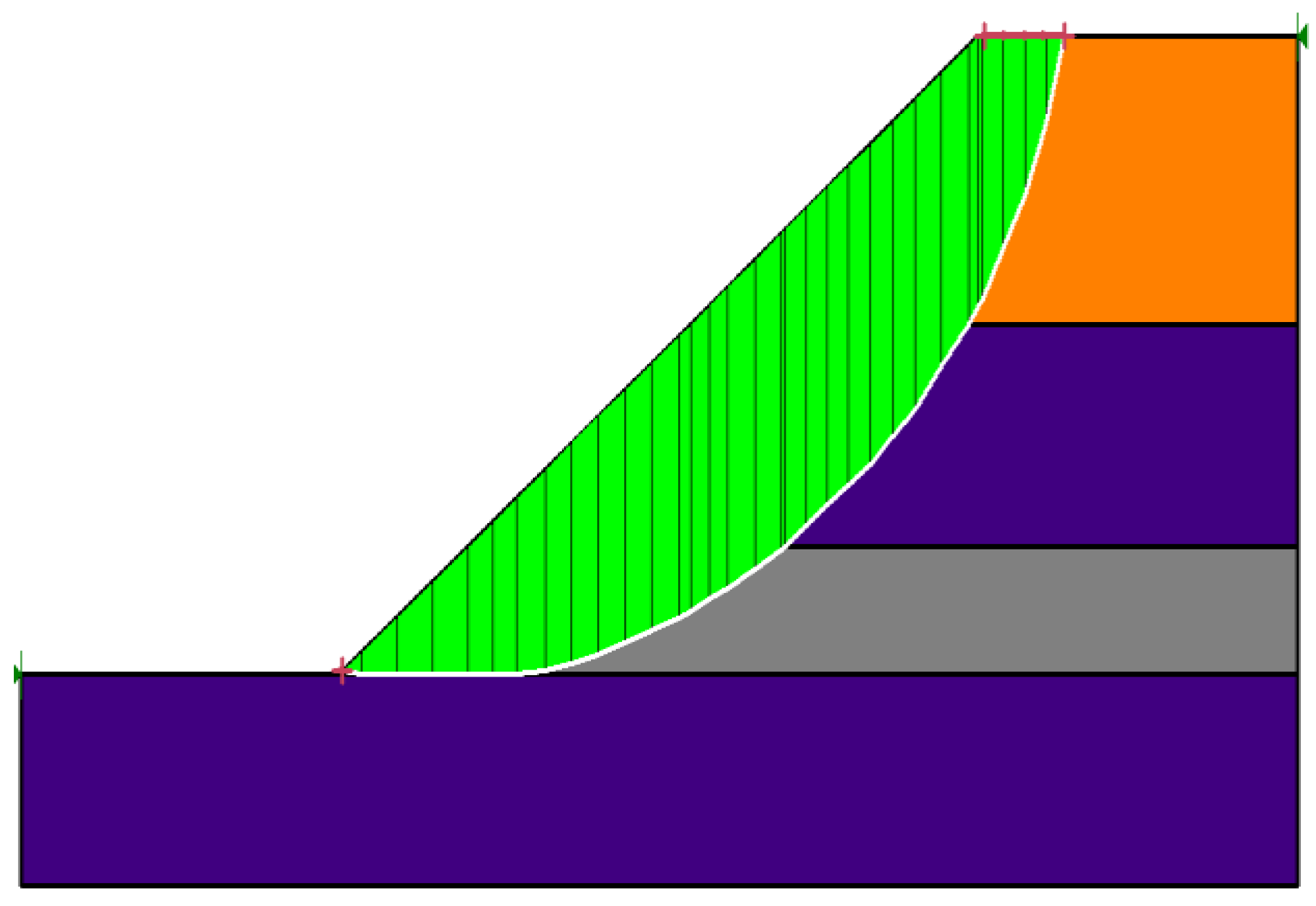

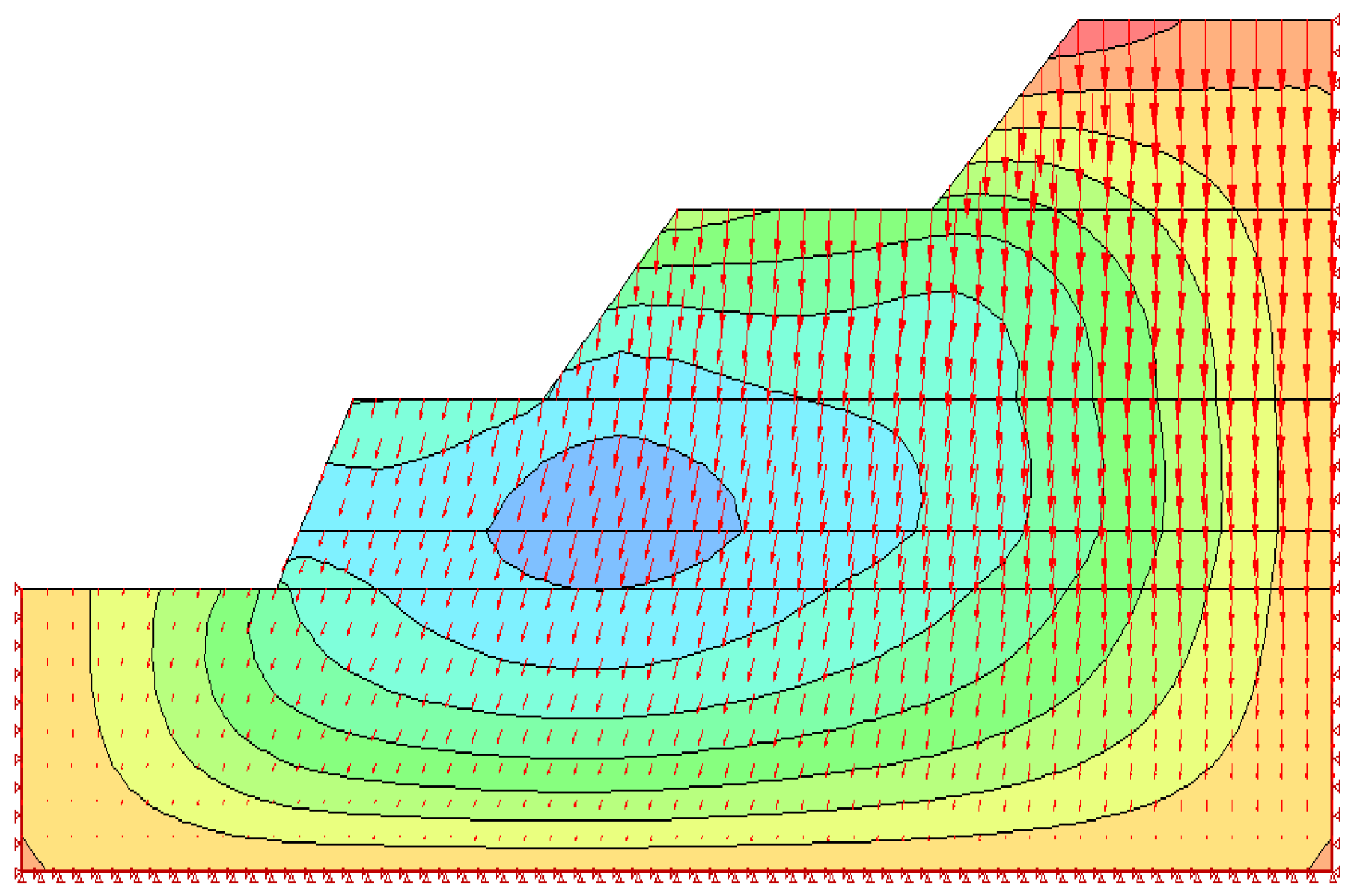
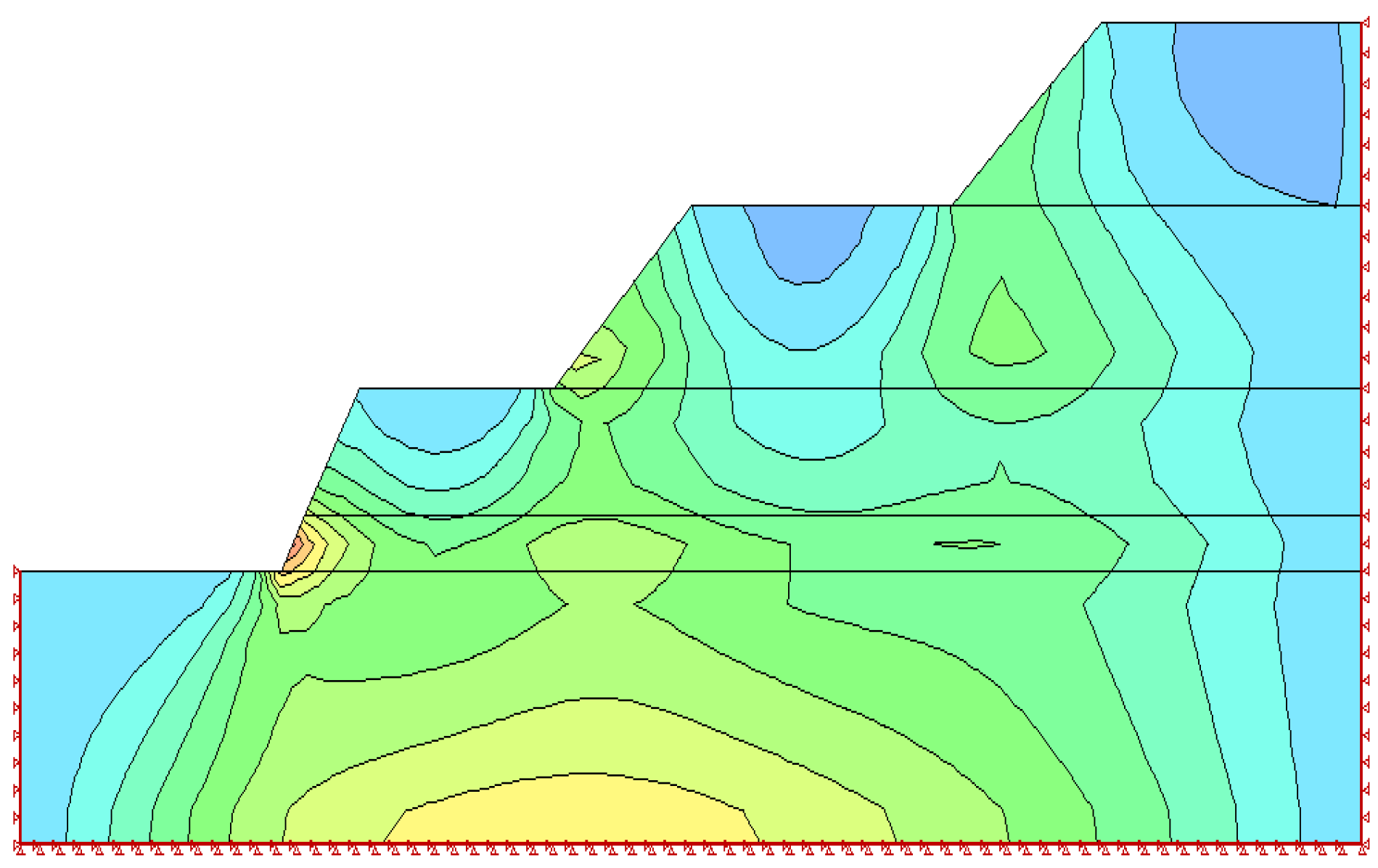
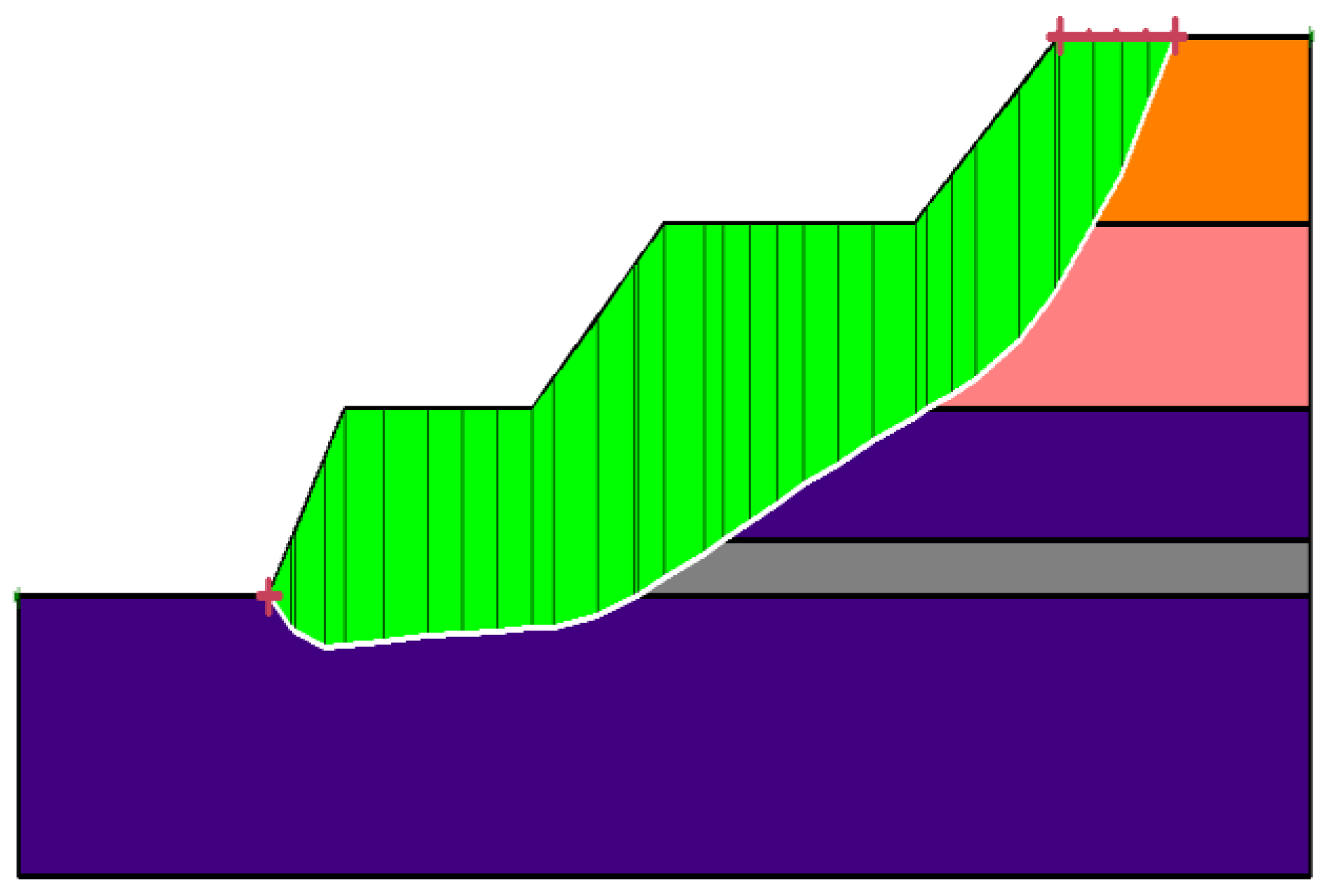
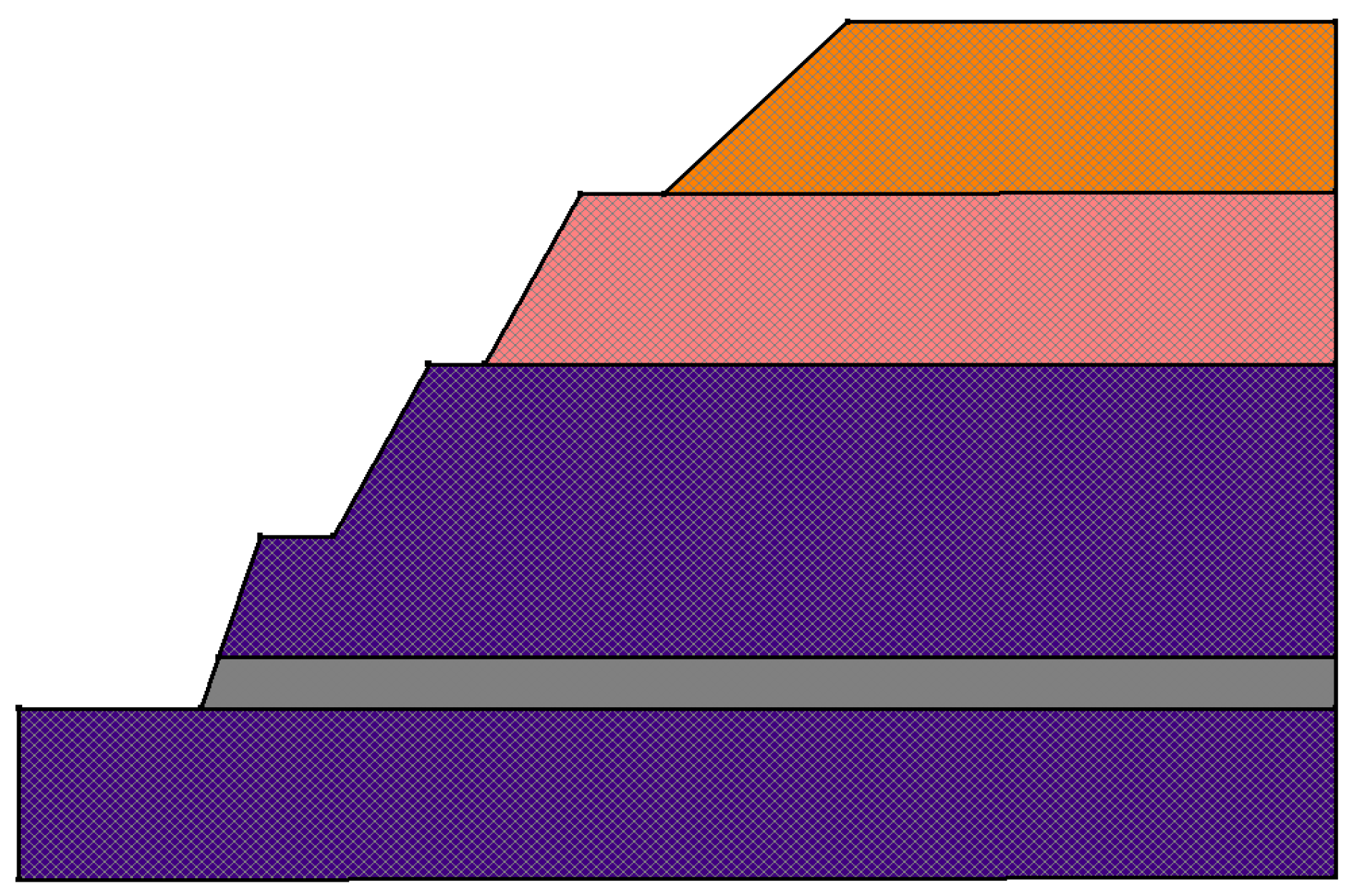










| Rock Name | Shear Strength (MPA) | ||||||
|---|---|---|---|---|---|---|---|
| 45° | 55° | 65° | Cohesive Force (MPa) | ||||
| Normal Stress | Shear Stress | Normal Stress | Shear Stress | Normal Stress | Shear Stress | ||
| Sandy mudstone | 4.52–4.64 | 4.52–4.64 | 2.29–2.52 | 3.28–3.60 | 0.81–0.88 | 1.74–1.88 | 1.3–1.4 |
| Medium coarse-grained sandstone | 27.15 | 27.15 | 9.41 | 13.43 | 4.73 | 10.15 | 6.4 |
| Siltstone | 4.64 | 4.64 | 1.84 | 2.62 | 0.54 | 1.16 | 0.9 |
| Rock | Unit Weight (kN/m³) | Cohesion (kPa) | Internal Friction Angle (°) | Modulus of Elasticity (GPA) | Poisson’s Ratio v |
|---|---|---|---|---|---|
| Quaternary | 18.45 | 37 | 25 | 0.043 | 0.33 |
| Mudstone | 23.13 | 170 | 29 | 5.7 | 0.24 |
| Sandy mudstone | 23.11 | 124 | 31 | 2.4 | 0.3 |
| Coal | 13.14 | 132 | 29 | 5.4 | 0.31 |
| Waste disposal | 19.22 | 16 | 23 | 0.019 | 0.43 |
| Slope Type | Years of Service (a) | Safety Factor, Fs |
|---|---|---|
| There are particularly important buildings on the slope or the slope slides Causing heavy loss of life and property | >20 | >1.5 |
| Final slope of excavation site | >20 | 1.3~1.5 |
| Nonworking slope | <10 | 1.1~1.2 |
| 10~20 | 1.2~1.3 | |
| >20 | 1.3~1.5 | |
| Working slope | Temporary | 1.05~1.2 |
| Waste dump slope | >20 | 1.2~1.5 |
| Inner waste dump slope | <10 | 1.2 |
| ≥10 | 1.3 |
| Name | Slope Area | Side Slope Height | Slope Composition | Slope Angle | Safety Factor | |
|---|---|---|---|---|---|---|
| Bishop Method | M–P Method | |||||
| Stope | Checking section 1 of stope slope after disaster control | 30 m | Quaternary, mudstone, sandy mudstone, coal seam, etc. | 38° | 1.343 | 1.328 |
| Checking section 2 of stope slope after disaster control | 30 m | Quaternary, mudstone, sandy mudstone, coal seam, etc. | 34° | 1.362 | 1.356 | |
| Checking section 1 of stope slope | 15 m | Quaternary, mudstone, sandy mudstone, coal seam, etc. | 37° | 1.423 | 1.419 | |
| Checking section 2 of stope slope | 30 m | Quaternary, mudstone, sandy mudstone, coal seam, etc. | 25° | 1.378 | 1.374 | |
| Checking section 3 of stope slope | 40 m | Quaternary, mudstone, sandy mudstone, coal seam, etc. | 47° | 1.161 | 1.157 | |
| Waste dump | Checking section of waste dump slope after disaster control | 55 m | Waste disposal | 25° | 1.263 | 1.257 |
| Checking section 1 of waste dump slope | 60 m | Waste disposal | 32° | 1.203 | 1.201 | |
| Checking section 2 of waste dump slope | 60 m | Waste disposal | 16° | 1.313 | 1.312 | |
| Checking section 1 of inner waste dump slope | 50 m | Waste disposal | 30° | 1.218 | 1.214 | |
| Checking section 2 of inner waste dump slope | 50 m | Waste disposal | 25° | 1.227 | 1.226 | |
| Checking section 3 of inner waste dump slope | 60 m | Waste disposal | 24° | 1.231 | 1.229 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Du, X.; Chai, J. Stability Evaluation of Medium Soft Soil Pile Slope Based on Limit Equilibrium Method and Finite Element Method. Mathematics 2022, 10, 3709. https://doi.org/10.3390/math10193709
Du X, Chai J. Stability Evaluation of Medium Soft Soil Pile Slope Based on Limit Equilibrium Method and Finite Element Method. Mathematics. 2022; 10(19):3709. https://doi.org/10.3390/math10193709
Chicago/Turabian StyleDu, Xiaoyan, and Jinfei Chai. 2022. "Stability Evaluation of Medium Soft Soil Pile Slope Based on Limit Equilibrium Method and Finite Element Method" Mathematics 10, no. 19: 3709. https://doi.org/10.3390/math10193709
APA StyleDu, X., & Chai, J. (2022). Stability Evaluation of Medium Soft Soil Pile Slope Based on Limit Equilibrium Method and Finite Element Method. Mathematics, 10(19), 3709. https://doi.org/10.3390/math10193709






