A New Series Representation and the Laplace Transform for the Lognormal Distribution
Abstract
:1. Introduction
- 1.
- Let It corresponds to a simple variable change For simplicity, we set , and ;
- 2.
- We use the bilateral Laplace transform (BLT):where is any real or complex function defined on , and is its transform, provided it has a non void region of convergence (ROC). The BLT has several advantages [12], namely, it does not introduce any initial conditions and has the FT as a particular case.
2. Attempts to Compute the Laplace Transform
2.1. First Attempt
- that gives, by application of the LT,
- and
- which leads towhere A is a constant such that
- Then,with the region of convergence as the whole complex plane.
2.2. Second Attempt
3. A Different Approach
3.1. A New Series Representation for the Gaussian of the Logarithm
3.2. The Laplace Transform of the Bilinear Function
3.3. A New Series for the Lognormal Distribution
3.4. The LT of the Lognormal Distribution
4. Conclusions
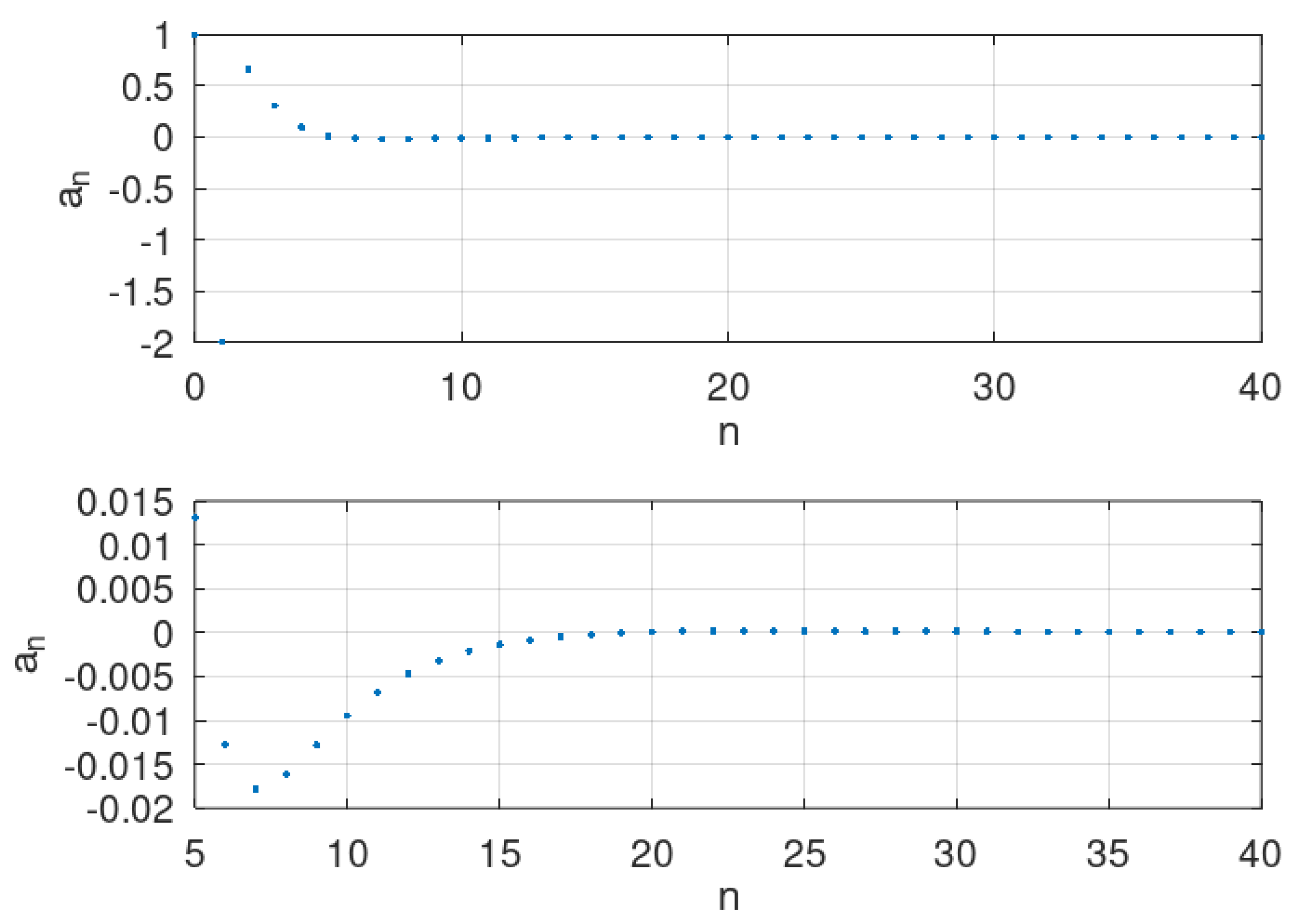
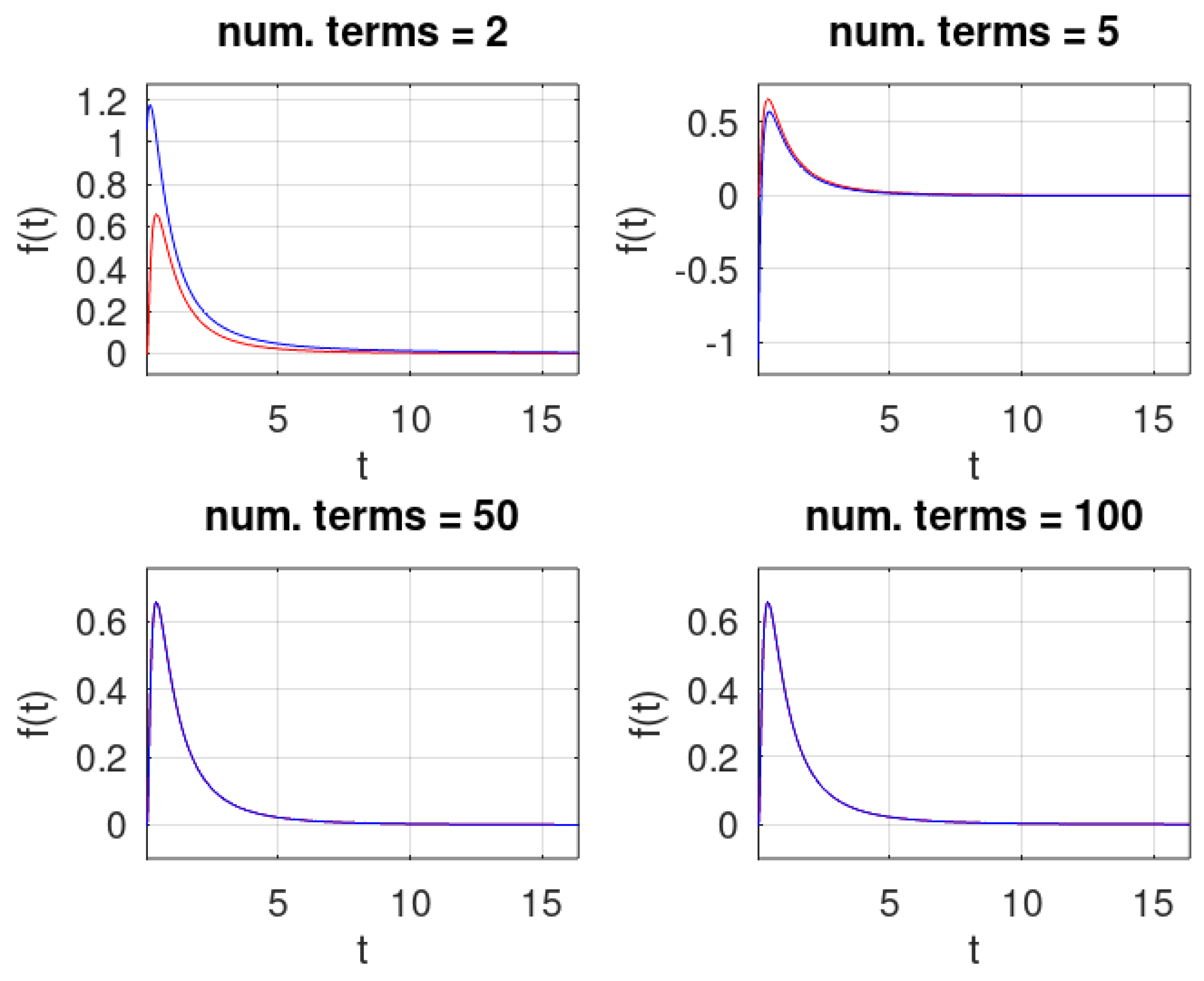
- With (25) we can approximate with a finite number of terms.
- The series in (45) has simpler terms that have LT. Thus, the series can be transformed term by term.
- It is very curious, since it expresses the LT of the LGN as the difference of two functions: one is analytic in the right half complex plane, while the other is holomorphic.
- The LT initial value theorem tells us that the two terms must tend mutually asymptotically on the real axis, since they have polynomial behavior and the initial value is zero.
- Although interesting from a theoretical point of view, it is not very useful for numerical implementations due to the presence of the factorial function that causes the appearance of numerical overflows.
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| LGN | lognormal |
| LT | bilateral Laplace transform |
| FT | Fourier transform |
References
- Cramér, H. The Elements of Probability Theory and Some of Its Applications; John Wiley & Sons: New York, NY, USA; Chapman & Hall Ltd.: London, UK, 1955. [Google Scholar]
- Pratesi, M.; Santucci, F.; Graziosi, F.; Ruggieri, M. Outage analysis in mobile radio systems with generically correlated log-normal interferers. IEEE Trans. Commun. 2000, 48, 381–385. [Google Scholar] [CrossRef]
- Laourine, A.; Stephenne, A.; Affes, S. Estimating the Ergodic Capacity of Log-Normal Channels. IEEE Commun. Lett. 2007, 11, 568–570. [Google Scholar] [CrossRef]
- Ai, J.; Qi, X.; Yu, W.; Deng, Y.; Liu, F.; Shi, L. A New CFAR Ship Detection Algorithm Based on 2-D Joint Log-Normal Distribution in SAR Images. IEEE Geosci. Remote Sens. Lett. 2010, 7, 806–810. [Google Scholar] [CrossRef]
- Li, J.; Zhou, Q.; Yeh, W.W.G. A Bayesian hierarchical model for estimating the statistical parameters in a three-parameter log-normal distribution for monthly average streamflows. J. Hydrol. 2020, 591, 125265. [Google Scholar] [CrossRef]
- Zuo, R.; Wang, J.; Xiong, Y.; Wang, Z. The processing methods of geochemical exploration data: Past, present, and future. Appl. Geochem. 2021, 132, 105072. [Google Scholar] [CrossRef]
- Limpert, E.; Stahel, W.A.; Abbt, M. Log-normal Distributions across the Sciences: Keys and Clues. BioScience 2001, 51, 341–352. Available online: https://academic.oup.com/bioscience/article-pdf/51/5/341/26891292/51-5-341.pdf (accessed on 15 July 2022). [CrossRef]
- Leipnik, R.B. On lognormal random variables: I-the characteristic function. ANZIAM J. 1991, 32, 327–347. [Google Scholar] [CrossRef]
- Beaulieu, N.C. A power series expansion for the truncated lognormal characteristic function. In Proceedings of the 2010 25th Biennial Symposium on Communications, Kingston, ON, Canada, 12–14 May 2010; pp. 284–287. [Google Scholar]
- Asmussen, S.; Jensen, J.L.; Rojas-Nandayapa, L. On the Laplace Transform of the Lognormal Distribution. Methodol. Comput. Appl. Probab. 2016, 18, 441–458. [Google Scholar] [CrossRef]
- Miles, J. On the Laplace transform of the lognormal distribution: Analytic continuation and series approximations. J. Comput. Appl. Math. 2022, 404, 113904. [Google Scholar] [CrossRef]
- Ortigueira, M.D.; Machado, J.T. Revisiting the 1D and 2D Laplace transforms. Mathematics 2020, 8, 1330. [Google Scholar] [CrossRef]
- Deakin, M.A. The Laplace transform of exp (et). ANZIAM J. 1996, 37, 279–287. [Google Scholar] [CrossRef]
- Boyadzhiev, K.N. A series transformation formula and related polynomials. Int. J. Math. Math. Sci. 2005, 2005, 3849–3866. [Google Scholar] [CrossRef] [Green Version]
- Papoulis, A. The Fourier Integral and Its Applications; Polytechnic Institute of Brooklyn, McCraw-Hill Book Company Inc.: New York, NY, USA, 1962. [Google Scholar]
- Abramowitz, M.; Stegun, I.A. Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables; US Government Printing Office: Washington, DC, USA, 1964; Volume 55.
- Oppenheim, A.V.; Willsky, A.S.; Hamid, S. Signals and Systems, 2nd ed.; Prentice-Hall: Upper Saddle River, NJ, USA, 1997. [Google Scholar]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ortigueira, M.D. A New Series Representation and the Laplace Transform for the Lognormal Distribution. Mathematics 2022, 10, 3474. https://doi.org/10.3390/math10193474
Ortigueira MD. A New Series Representation and the Laplace Transform for the Lognormal Distribution. Mathematics. 2022; 10(19):3474. https://doi.org/10.3390/math10193474
Chicago/Turabian StyleOrtigueira, Manuel D. 2022. "A New Series Representation and the Laplace Transform for the Lognormal Distribution" Mathematics 10, no. 19: 3474. https://doi.org/10.3390/math10193474
APA StyleOrtigueira, M. D. (2022). A New Series Representation and the Laplace Transform for the Lognormal Distribution. Mathematics, 10(19), 3474. https://doi.org/10.3390/math10193474





