The Generalized Exponential Extended Exponentiated Family of Distributions: Theory, Properties, and Applications
Abstract
:1. Introduction
- 1.
- , is differentiable and increases monotonically.
- 2.
1.1. Reliability Analysis
1.2. Useful Expansion of the NGE3-G Family
2. Statistical Properties of NGE3-G Family (NGE3GF)
2.1. Quantile Function (QF)
2.2. Skewness and Kurtosis
2.3. Probability Weighted Moments (PWMs)
2.4. Moments
2.5. Moment Generating Function (mgf)
2.6. Mean Deviation (MD)
2.7. Order Statistics (OS)
2.8. Renyi Entropy (RE)
3. Maximum Likelihood (ML) Method
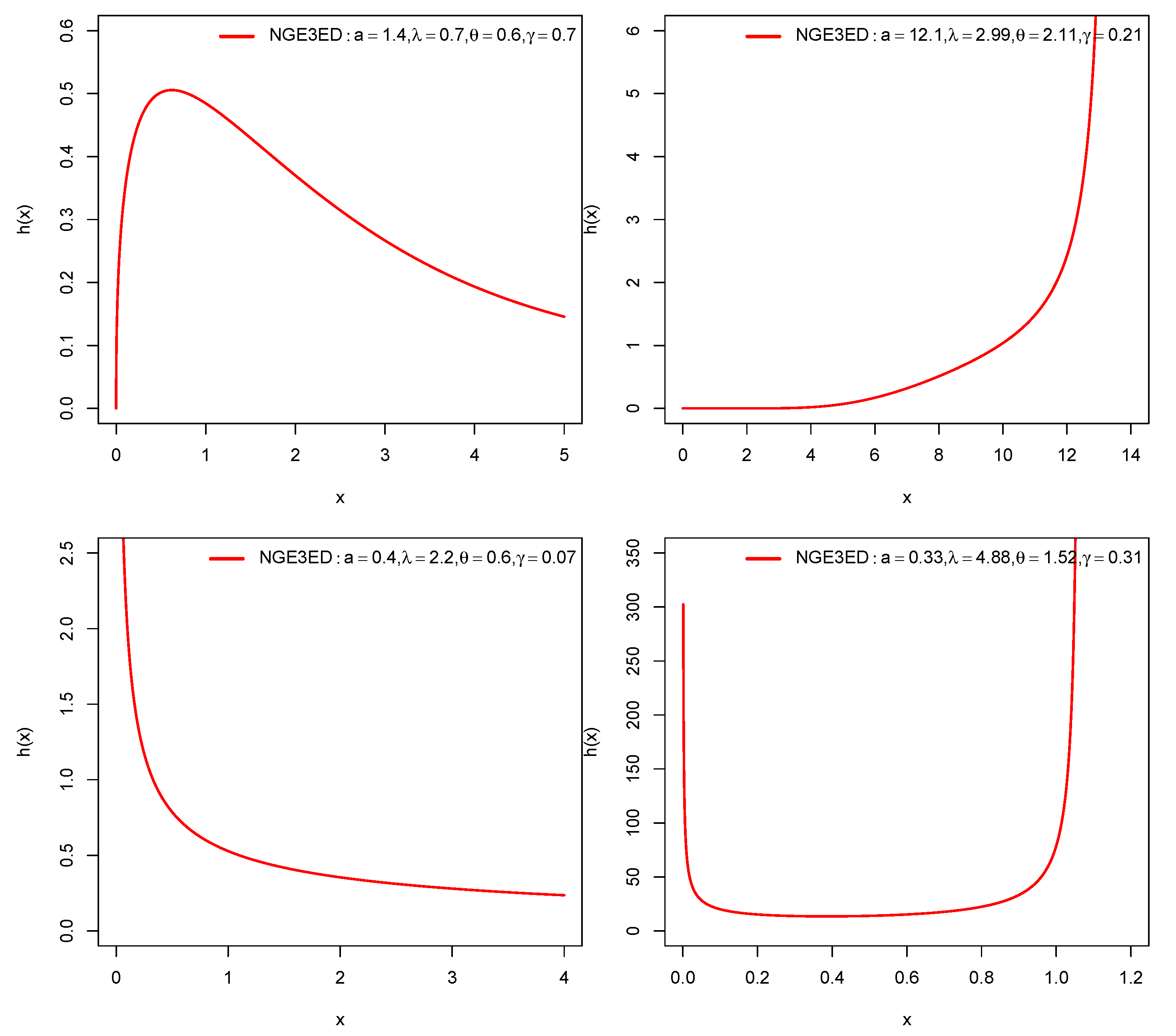
4. NGE3-Exponential Distribution (NGE3ED)
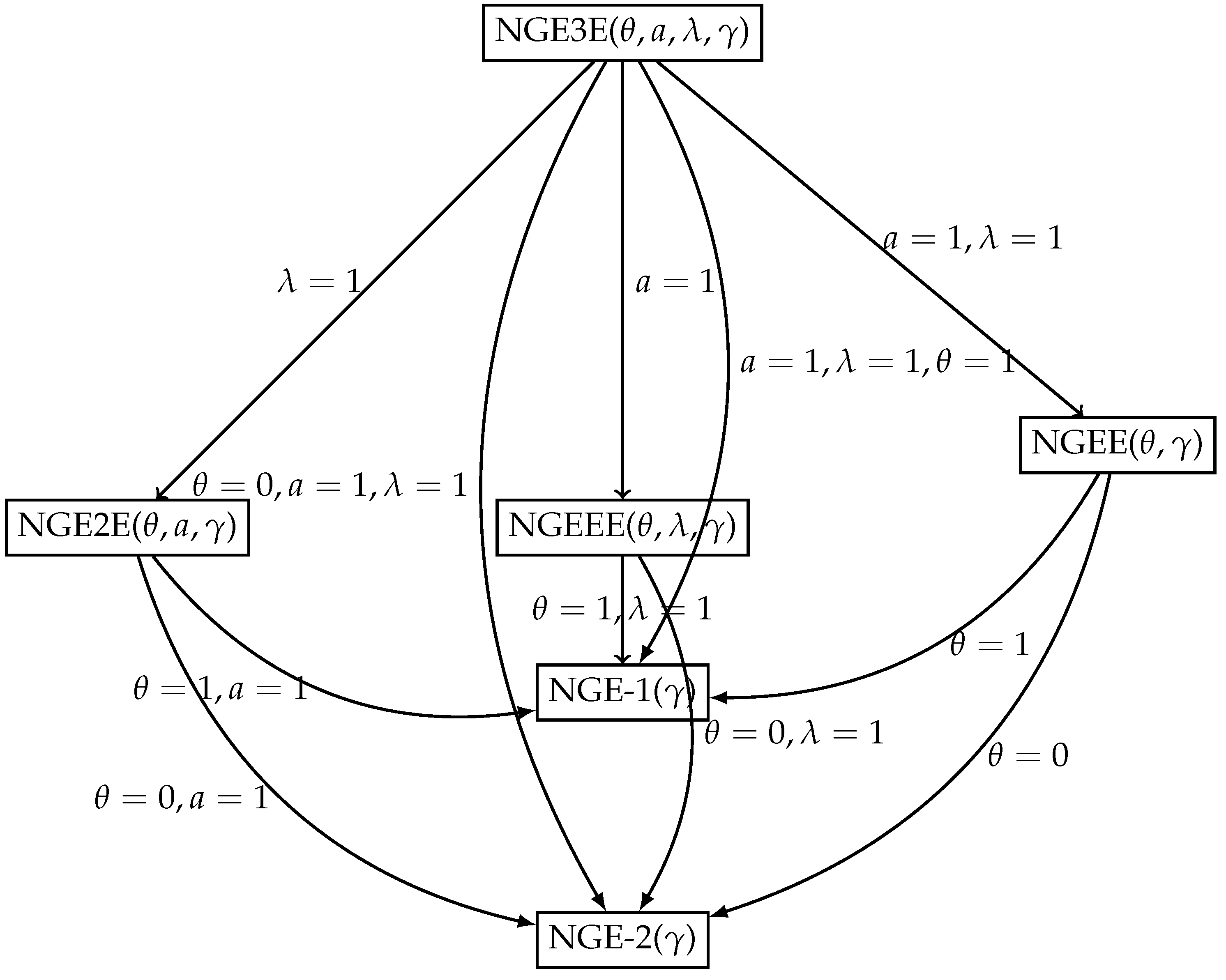
4.1. Relationship among Submodels of NGE3ED
- 1.
- For , the NGE3E () model reduces to new generalized extended exponentiated exponential NGE2E () model.
- 2.
- For , the NGE3E () model reduces to new generalized exponential extended exponential model NGEEE ().
- 3.
- For , the NGE3E () model reduces to new generalized extended exponential model NGEE ().
- 4.
- For , the NGE3E () model reduces to new generalized exponential model NGE-1 ().
- 5.
- For , the NGE3E () model reduces to the NGE-2 model ().
4.2. Quantile Function and Simulation Study
4.3. Estimation
4.4. Empirical Illustrations of the NGE3E Model
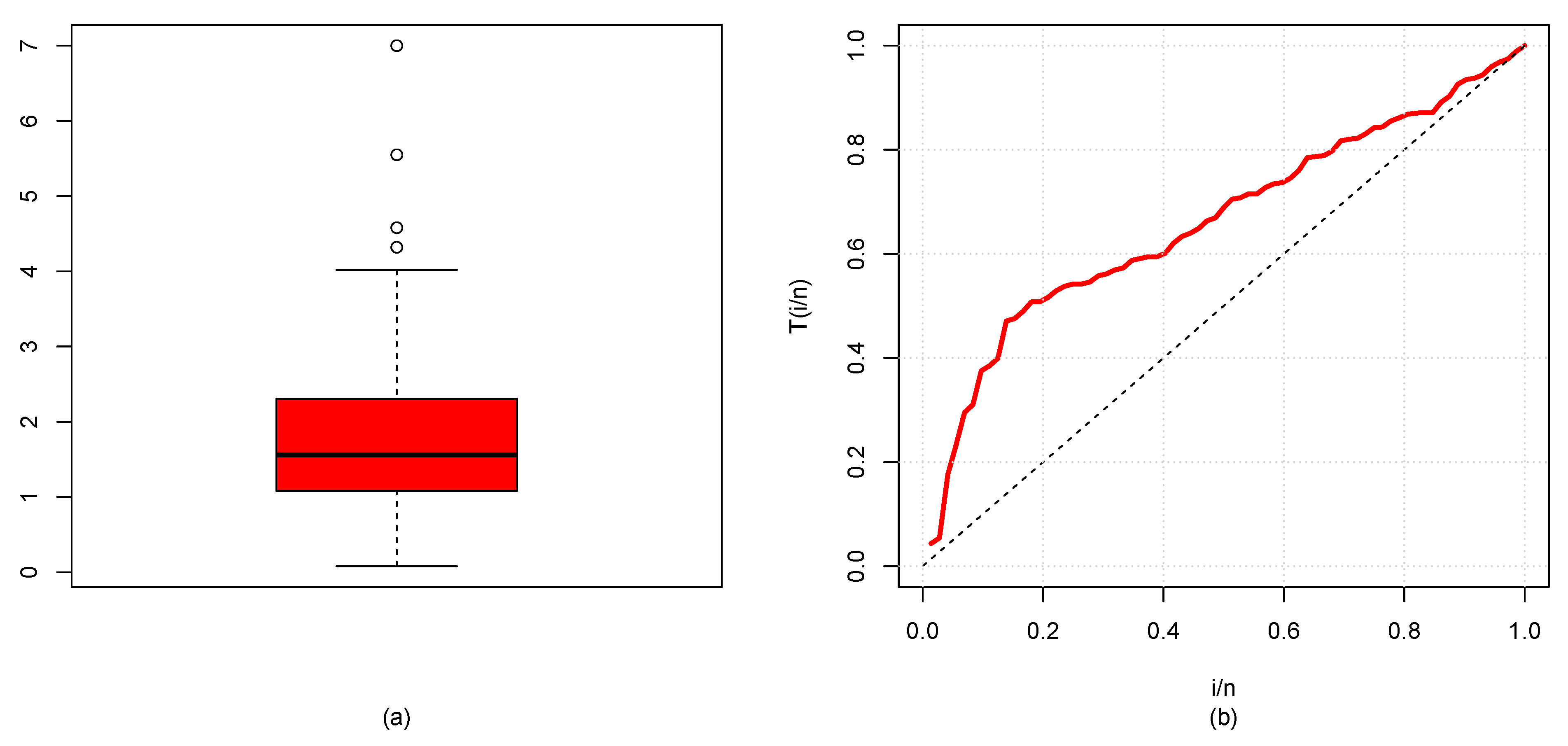
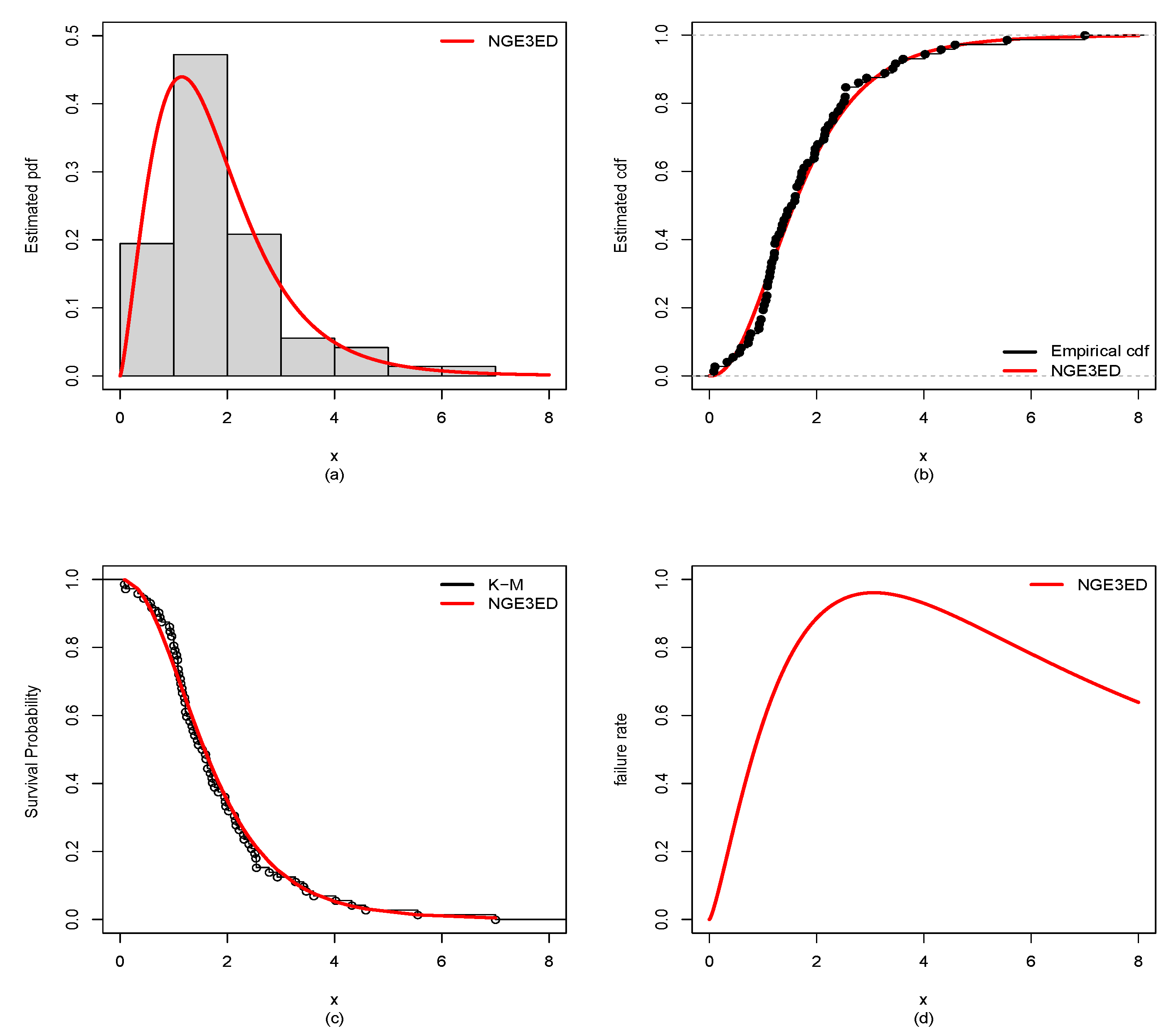
4.4.1. Dataset 1: Guinea Pig (GP) Data
| 0.10 | 0.33 | 0.44 | 0.56 | 0.59 | 0.72 | 0.74 | 0.77 | 0.92 | 0.93 | |
| 0.96 | 1.0 | 1.0 | 1.02 | 1.05 | 1.07 | 7.0 | 0.08 | 1.08 | 1.08 | 1.09 |
| 1.12 | 1.13 | 1.15 | 1.16 | 1.20 | 1.21 | 1.22 | 1.22 | 1.24 | 1.30 | 1.34 |
| 1.36 | 1.39 | 1.44 | 1.46 | 1.53 | 1.59 | 1.60 | 1.63 | 1.63 | 1.68 | 1.71 |
| 1.72 | 1.76 | 1.83 | 1.95 | 1.96 | 1.97 | 2.02 | 2.13 | 2.15 | 2.16 | 2.22 |
| 2.30 | 2.31 | 2.40 | 2.45 | 2.51 | 2.53 | 2.54 | 2.54 | 2.78 | 2.93 | 3.27 |
| 3.42 | 3.47 | 3.61 | 4.02 | 4.32 | 4.58 | 5.55 |
4.4.2. Dataset 2: Bladder Cancer (BC) Data
| 0.08 | 2.09 | 22.69 | 12.63 | 3.48 | 4.87 | 8.65 | 6.93 | 6.94 | 8.66 | |
| 3.36 | 2.07 | 13.11 | 23.63 | 21.73 | 12.07 | 0.20 | 2.23 | 6.76 | 3.36 | 3.52 |
| 4.98 | 2.02 | 20.28 | 6.97 | 9.02 | 12.03 | 8.53 | 13.29 | 0.40 | 6.54 | 4.51 |
| 2.26 | 3.57 | 3.31 | 2.02 | 5.06 | 7.09 | 9.22 | 12.02 | 13.80 | 8.37 | 25.74 |
| 6.25 | 0.50 | 4.50 | 2.46 | 3.25 | 3.46 | 1.76 | 5.09 | 19.13 | 7.26 | 11.98 |
| 9.47 | 8.26 | 14.24 | 5.85 | 25.82 | 4.40 | 0.51 | 1.46 | 2.54 | 18.10 | 3.70 |
| 11.79 | 5.17 | 7.93 | 7.28 | 5.71 | 9.74 | 4.34 | 14.76 | 3.02 | 26.31 | 1.40 |
| 0.81 | 4.33 | 17.36 | 2.83 | 11.64 | 1.26 | 7.87 | 46.12 | 5.62 | 17.12 | 2.87 |
| 7.63 | 1.35 | 79.05 | 5.41 | 17.14 | 4.26 | 11.25 | 2.75 | 7.66 | 1.19 | 5.49 |
| 43.01 | 10.66 | 16.62 | 7.59 | 5.34 | 4.18 | 10.75 | 7.62 | 2.69 | 5.41 | 0.90 |
| 4.23 | 34.26 | 2.69 | 14.83 | 1.05 | 10.34 | 36.66 | 7.39 | 15.96 | 5.32 | 3.88 |
| 2.64 | 32.15 | 14.77 | 10.06 | 7.32 | 5.32 | 3.82 | 2.62 |
- The EWE distribution
- WE distribution:
- EEW distribution:
- BEF distribution:
- OGEW distribution:
- OGEFr distribution:
- EKE distribution:
- KwW distribution:
- WL distribution:
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Lemonte, A.J. A new exponential-type distribution with constant, decreasing, increasing, upside-down bathtub and bathtub-shaped failure rate function. Comput. Stat. Data Anal. 2013, 62, 149–170. [Google Scholar] [CrossRef]
- Adamidis, K.; Loukas, S. A lifetime distribution with decreasing failure rate. Stat. Probab. Lett. 1998, 39, 35–42. [Google Scholar] [CrossRef]
- Gupta, R.D.; Kundu, D. Exponentiated exponential family: An alternative to gamma and Weibull distribution. Biom. J. 2001, 43, 117–130. [Google Scholar] [CrossRef]
- Gupta, R.D.; Kundu, D. Generalized exponential distribution. Aust. N. Z. J. Stat. 1999, 41, 173–188. [Google Scholar] [CrossRef]
- Gupta, R.D.; Kundu, D. Generalized exponential distribution: Existing results and some recent developments. J. Stat. Plan. Inference 2007, 137, 3537–3547. [Google Scholar] [CrossRef]
- Balakrishnan, K. Exponential Distribution: Theory, Methods and Applications; CRC Press: Boca Raton, FL, USA, 2019. [Google Scholar]
- Johnson, N.L.; Kotz, S.; Balakrishnan, N. Related distributions and some generalizations. In Exponential Distribution: Theory, Methods and Applications; Balakrsihnan, K., Baus, A.P., Eds.; CRC Press: Boca Raton, FL, USA, 2019; pp. 297–306. [Google Scholar]
- Hussain, S.; Rashid, M.S.; Ul Hassan, M.; Ahmed, R. The Generalized Alpha Exponent Power Family of Distributions: Properties and Applications. Mathematics 2022, 10, 1421. [Google Scholar] [CrossRef]
- Martinez-Florez, G.; Bolfarine, H.; Gómez, H.W. A Unification of Families of Birnbaum-Saunders Distributions with Applications. Revstat-Stat. J. 2020, 18, 637–660. [Google Scholar] [CrossRef]
- Alzaatreh, A.; Lee, C.; Famoye, F. A new method for generating families of continuous distributions. Metron 2013, 71, 63–79. [Google Scholar] [CrossRef]
- Flajonet, P.; Odlyzko, A. Singularity analysis of generating function. SIAM J. Discr. Math. 1990, 3, 216–240. [Google Scholar] [CrossRef]
- Flajonet, P.; Sedgewich, R. Analytic Combinatorics; Cambridge University: Cambridge, MA, USA, 2009; ISBN 978-0-521-89806-5. [Google Scholar]
- Nielson, N. Handbuch der Theorie der Gamma Funktion; Chelsea Publishing Co.: New York, NY, USA, 1906. [Google Scholar]
- Ward, M. The representation of Stirling’s numbers and Stirling’s polynomials as sums of factorials. Am. J. Math. 1934, 56, 87–95. [Google Scholar] [CrossRef]
- Maple. Maplesoft, a Division of Waterloo Maple Incorporated, Ontario. 2016. Available online: https://www.maplesoft.com (accessed on 7 August 2022).
- Moors, J.J.A. A quantile alternative for kurtosis. Statistician 1998, 37, 25–32. [Google Scholar] [CrossRef]
- Kenney, J.; Keeping, E. Mathematics of Statistics, 3rd ed.; D. Van Nostrand: Princeton, NJ, USA, 1962; Volume 1. [Google Scholar]
- Smith, J.L.; Paul, D.; Paul, R. No place for a women: Evidence for gender bias in evaluations of presidential candidates. Basic Appl. Soc. Psych. 2007, 29, 225–233. [Google Scholar] [CrossRef]
- Renyi, A. On measures of entropy and information. Hung Acad. Sci. 1961, 1, 547–561. [Google Scholar]
- R Development Core Team. R: A Language and Environment for Statistical Computing; Foundation for Statistical Computing: Vienna, Austria, 2009. [Google Scholar]
- Oguntunde, P.E.; Balogon, O.S.; Okagbue, H.I.; Bishop, S.A. The Weibull-Exponential Distribution: Its Properties and Applications. J. Appl. Sci. 2015, 15, 1305–1311. [Google Scholar] [CrossRef]
- Elgarhy, M.; Shakil, M.; Golam Kibria, B.M. Exponentiated Weibull-Exponential Distribution with Applications. AAM Intern. J. 2017, 12, 710–725. [Google Scholar]
- Al Sulami, D. Exponentiated Exponential Weibull Distribution: Mathematical Properties and Application. Am. J. Appl. Sci. 2020, 17, 188–195. [Google Scholar] [CrossRef]
- Mead, M.E.; Afity, A.Z.; Hamedani, G.G.; Ghosh, I. The Beta Exponential Frechet Distribution with Applications. Austrian J. Stat. 2017, 46, 41–63. [Google Scholar] [CrossRef]
- Tahir, M.H.; Cordeiro, G.M.; Alizadeh, M.; Mansoor, M.; Zubair, M.; Hamedani, G.G. The odd generalized exponential family of distributions with applications. J. Stat. Distrib. Appl. 2015, 2, 1–28. [Google Scholar] [CrossRef]
- Rodrigues, J.A.; Silva, A.P.C.M. The Exponentiated Kumaraswamy Exponential Distribution. Br. J. Appl. Sci. Technol. 2015, 10, 1–12. [Google Scholar] [CrossRef]
- Cordeiro, G.M.; Ortega, E.M.M.; Nadarajah, S. The Kumaraswamy Weibull distribution with application to failure data. J. Frankl. Inst. 2010, 347, 1399–1429. [Google Scholar] [CrossRef]
- Tahir, M.H.; Cordeiro, G.M.; Mansoor, M.; Zubair, M. The Weibull-Lomax distribution: Properties and Applications. Hacet. J. Math. Stat. 2014, 44, 455–474. [Google Scholar] [CrossRef]
- Bjerkedal, T. Acquisition of resistance in Guinea Pies infected with different doses of Virulent Tubercle Bacilli. Am. J. Hyg. 1960, 72, 130–148. [Google Scholar]
- Ademola, A.A. Transmuted Sushila Distribution and its Application to Lifetime Data. J. Math. Anal. Model. 2021, 1, 1–14. [Google Scholar]

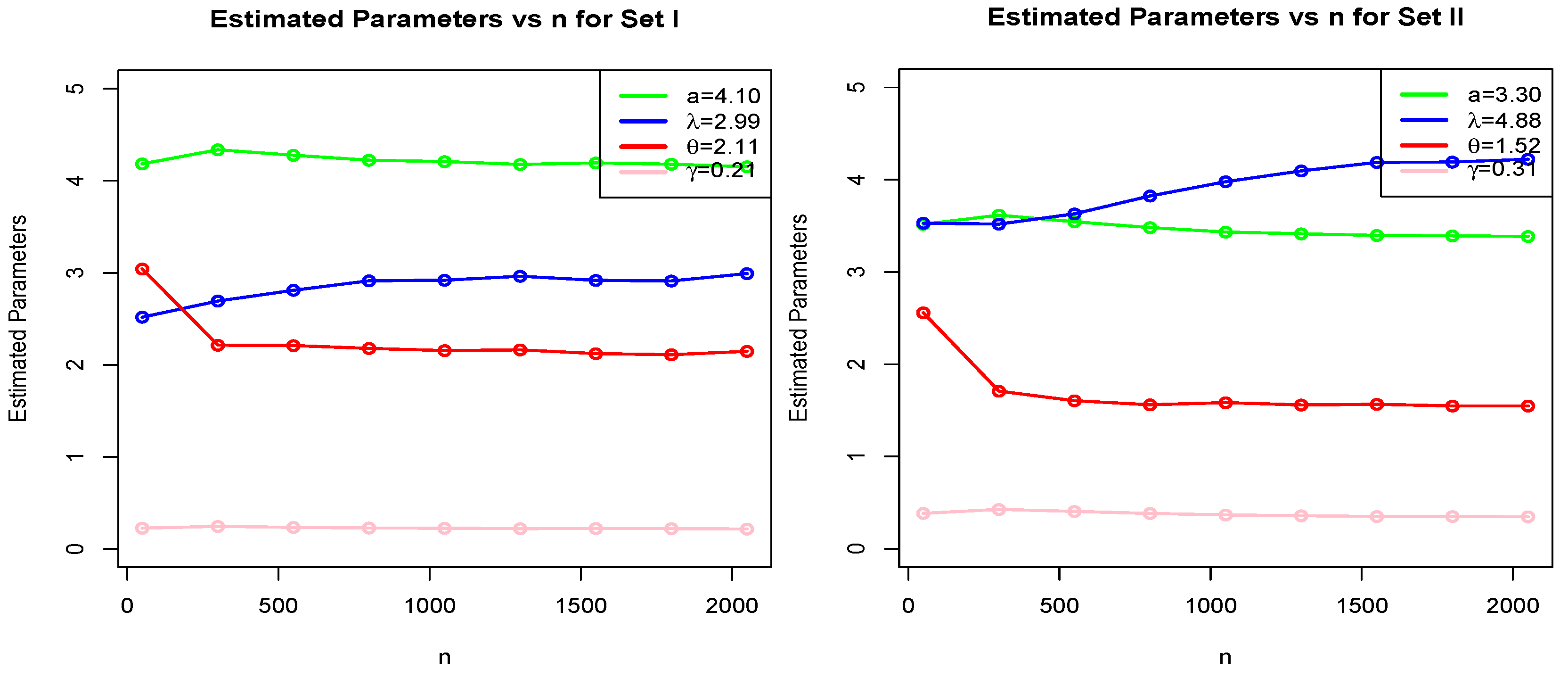




| Set I: | Set II: | ||||||
|---|---|---|---|---|---|---|---|
| n | Parameter | MLE | MSE | AB | MLE | MSE | AB |
| 50 | 4.178052 | 0.713267 | 0.078052 | 3.566745 | 1.041872 | 0.266745 | |
| 2.639318 | 2.241914 | 0.350682 | 3.607051 | 4.074313 | 1.272949 | ||
| 2.996142 | 2.892954 | 0.886142 | 2.542916 | 4.662988 | 1.022916 | ||
| 0.222999 | 0.007290 | 0.012999 | 0.383463 | 0.042189 | 0.073464 | ||
| 300 | 4.322833 | 0.380854 | 0.222833 | 3.625264 | 0.460963 | 0.325264 | |
| 2.709852 | 1.547025 | 0.280148 | 3.434403 | 4.584252 | 1.445597 | ||
| 2.243113 | 0.918195 | 0.133113 | 1.703476 | 0.713168 | 0.183476 | ||
| 0.243876 | 0.006906 | 0.033876 | 0.432456 | 0.047154 | 0.122456 | ||
| 550 | 4.265227 | 0.273629 | 0.165227 | 3.526255 | 0.245441 | 0.226255 | |
| 2.852406 | 1.260532 | 0.137594 | 3.694286 | 3.433749 | 1.185714 | ||
| 2.194856 | 0.586033 | 0.084856 | 1.610212 | 0.311643 | 0.090212 | ||
| 0.233397 | 0.004786 | 0.023397 | 0.398196 | 0.026787 | 0.088196 | ||
| 800 | 4.238538 | 0.217878 | 0.138538 | 3.471447 | 0.156626 | 0.171447 | |
| 2.892669 | 1.054043 | 0.097331 | 3.875455 | 2.720822 | 1.004546 | ||
| 2.168020 | 0.431818 | 0.058020 | 1.581829 | 0.180927 | 0.061829 | ||
| 0.228880 | 0.003738 | 0.018880 | 0.378363 | 0.017620 | 0.068363 | ||
| 1050 | 4.211064 | 0.170239 | 0.111064 | 3.446462 | 0.113253 | 0.146462 | |
| 2.919116 | 0.895349 | 0.070884 | 3.971339 | 2.321943 | 0.908661 | ||
| 2.157062 | 0.334494 | 0.047062 | 1.558690 | 0.119622 | 0.038690 | ||
| 0.225160 | 0.002968 | 0.015160 | 0.368524 | 0.012958 | 0.058524 | ||
| 1300 | 4.197459 | 0.140223 | 0.097459 | 3.416337 | 0.084250 | 0.116337 | |
| 2.926014 | 0.764960 | 0.063986 | 4.078104 | 1.970276 | 0.801896 | ||
| 2.141350 | 0.257725 | 0.031350 | 1.564415 | 0.101034 | 0.044415 | ||
| 0.223226 | 0.002444 | 0.013226 | 0.358211 | 0.009942 | 0.048211 | ||
| 1550 | 4.172582 | 0.116356 | 0.072582 | 3.407995 | 0.071701 | 0.107995 | |
| 2.938682 | 0.651132 | 0.051318 | 4.132392 | 1.763364 | 0.747608 | ||
| 2.150551 | 0.227299 | 0.040551 | 1.553020 | 0.080565 | 0.033020 | ||
| 0.220265 | 0.002023 | 0.010265 | 0.354000 | 0.008316 | 0.044000 | ||
| 1800 | 4.174525 | 0.104986 | 0.074525 | 3.391980 | 0.056767 | 0.091980 | |
| 2.938528 | 0.594311 | 0.051472 | 4.200579 | 1.543466 | 0.679421 | ||
| 2.136263 | 0.198713 | 0.026263 | 1.547978 | 0.065708 | 0.027978 | ||
| 0.220122 | 0.001831 | 0.010122 | 0.348373 | 0.006657 | 0.038373 | ||
| 2050 | 4.164815 | 0.092521 | 0.064815 | 3.382171 | 0.049089 | 0.082171 | |
| 2.940650 | 0.523406 | 0.049350 | 4.242065 | 1.409728 | 0.637934 | ||
| 2.138791 | 0.175037 | 0.028791 | 1.550037 | 0.059096 | 0.030037 | ||
| 0.218764 | 0.001610 | 0.008764 | 0.344838 | 0.005818 | 0.034838 | ||
| Dataset | Min. | Median | Max. | Mean | Variance | Skewness | Kurtosis | ||
|---|---|---|---|---|---|---|---|---|---|
| 1 | 0.080 | 1.080 | 1.560 | 2.303 | 7.000 | 1.837 | 1.478 | 1.755 | 4.152 |
| 2 | 0.080 | 3.348 | 6.395 | 11.838 | 79.050 | 9.366 | 110.425 | 3.287 | 15.483 |
| Dist. | ||||||
|---|---|---|---|---|---|---|
| NGE3E | 2.32272 | 5.56385 | 0.10187 | 0.31452 | – | – |
| (0.49245) | (7.00899) | (1.27805) | (0.18822) | |||
| EWE | 2.29855 | 0.04199 | – | – | 21.89576 | 1.04688 |
| (1.21005) | (0.02975) | (19.11364) | (0.25627) | |||
| WE | – | 0.03282 | – | – | 59.89789 | 1.54046 |
| (0.04996) | (162.26158) | (0.16146) | ||||
| EEW | – | 1.00906 | – | 1.15666 | 2.03844 | 1.33014 |
| (31.52095) | (0.27759) | (0.99803) | (35.93074) | |||
| BEF | – | 0.65072 | 91.12759 | 0.40903 | 13.59632 | 5.60806 |
| (0.36349) | (41.07691) | (0.06542) | (52.52073) | (21.90342) | ||
| OGEW | – | 21.13824 | 0.03953 | – | 2.42230 | 1.02483 |
| (38.08836) | (0.08007) | (1.41950) | (0.32531) | |||
| OGEFr | – | 43.95769 | 45.57000 | – | 0.68169 | 0.44654 |
| (31.45097) | (32.38208) | (0.35684) | (0.08105) | |||
| EKE | – | 16.65656 | 1.76429 | – | 0.08030 | 1.29005 |
| (65.36980) | (1.20989) | (0.28969) | (0.58900) | |||
| KwW | 0.86432 | – | – | 0.53177 | 2.73100 | 3.21403 |
| (0.82088) | (0.46183) | (3.05683) | (9.65113) | |||
| WL | 21.27763 | – | – | 0.70534 | 0.25353 | 2.19835 |
| (55.95618) | (0.84875) | (0.22020) | (0.54623) |
| Dist. | ||||||
|---|---|---|---|---|---|---|
| NGE3E | 1.46988 | 3.17280 | 0.04142 | 0.06681 | – | – |
| (0.22276) | (1.65586) | (0.76421) | (0.04233) | |||
| EWE | 4.03707 | 0.01171 | – | – | 7.07212 | 0.52346 |
| (2.10918) | (0.00599) | (2.37210) | (0.11822) | |||
| WE | – | 0.00691 | – | – | 13.77130 | 0.98836 |
| (0.00218) | (5.32372) | (0.06271) | ||||
| EEW | – | 0.75644 | – | 0.65442 | 2.79598 | 2.18408 |
| (41.90063) | (0.13484) | (1.26511) | (184.93870) | |||
| BEF | – | 154.72736 | 92.01702 | 0.05378 | 153.63292 | 1.85484 |
| (256.89845) | (426.07475) | (0.03714) | (249.12744) | (2.52944) | ||
| OGEW | – | 12.71015 | 0.04898 | – | 3.74616 | 0.53393 |
| (16.73725) | (0.07842) | (1.99784) | (0.15390) | |||
| OGEFr | – | 0.03261 | 0.02360 | – | 2.53262 | 0.67782 |
| (0.03068) | (0.02448) | (1.01072) | (0.12675) | |||
| EKE | – | 5.10315 | 3.89071 | – | 0.01387 | 0.48930 |
| (3.05943) | (3.16005) | (0.00977) | (0.23850) | |||
| KwW | 0.46255 | – | – | 0.49332 | 4.08136 | 2.87041 |
| (0.39433) | (0.42793) | (4.52386) | (6.00747) | |||
| WL | 14.27567 | – | – | 0.17142 | 0.17497 | 1.52178 |
| (26.47401) | (0.16655) | (0.15495) | (0.28860) |
| Dist. | -L | AIC | CAIC | BIC | KS | p-Value | CM | AD |
|---|---|---|---|---|---|---|---|---|
| NGE3E | 102.134 | 212.267 | 212.864 | 221.374 | 0.095 | 0.54 | 0.08360 | 0.57473 |
| EWE | 102.976 | 213.952 | 214.548 | 223.058 | 0.101 | 0.4569 | 0.11519 | 0.75801 |
| WE | 104.486 | 214.972 | 215.325 | 221.802 | 0.119 | 0.2632 | 0.17274 | 1.04466 |
| EEW | 102.810 | 213.620 | 214.217 | 222.726 | 0.101 | 0.4499 | 0.11008 | 0.72722 |
| BEF | 105.026 | 220.052 | 220.962 | 231.436 | 0.521 | 0.0000 | 0.12084 | 0.76687 |
| OGEW | 102.979 | 213.959 | 214.556 | 223.065 | 0.102 | 0.4421 | 0.11361 | 0.75265 |
| OGEFr | 105.679 | 219.353 | 219.950 | 228.460 | 0.131 | 0.1674 | 0.15307 | 1.07003 |
| EKE | 102.764 | 213.528 | 214.125 | 222.635 | 0.100 | 0.4704 | 0.11084 | 0.72641 |
| KwW | 102.710 | 213.420 | 214.017 | 222.526 | 0.101 | 0.4559 | 0.10771 | 0.71078 |
| WL | 102.781 | 213.562 | 214.159 | 222.668 | 0.105 | 0.4045 | 0.11242 | 0.73235 |
| Dist. | -L | AIC | CAIC | BIC | KS | p-Value | CM | AD |
|---|---|---|---|---|---|---|---|---|
| NGE3E | 409.823 | 827.645 | 827.971 | 839.053 | 0.037 | 0.9954 | 0.02147 | 0.14416 |
| EWE | 411.320 | 830.641 | 830.966 | 842.049 | 0.048 | 0.9244 | 0.05658 | 0.37454 |
| WE | 415.905 | 837.811 | 838.004 | 846.367 | 0.080 | 0.3840 | 0.16541 | 0.98962 |
| EEW | 410.680 | 829.360 | 829.685 | 840.768 | 0.045 | 0.9576 | 0.04367 | 0.28848 |
| BEF | 415.946 | 841.892 | 842.384 | 856.152 | 0.987 | 0.0000 | 0.20020 | 1.48189 |
| OGEW | 410.922 | 829.844 | 830.169 | 841.252 | 0.047 | 0.9427 | 0.04845 | 0.32110 |
| OGEFr | 410.838 | 829.676 | 830.001 | 841.084 | 0.044 | 0.9613 | 0.04767 | 0.31411 |
| EKE | 411.000 | 830.000 | 830.325 | 841.408 | 0.046 | 0.9459 | 0.05222 | 0.33891 |
| KwW | 410.569 | 829.138 | 829.464 | 840.546 | 0.045 | 0.9600 | 0.04145 | 0.27310 |
| WL | 410.165 | 828.329 | 828.655 | 839.737 | 0.043 | 0.9721 | 0.03296 | 0.21647 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hussain, S.; Sajid Rashid, M.; Ul Hassan, M.; Ahmed, R. The Generalized Exponential Extended Exponentiated Family of Distributions: Theory, Properties, and Applications. Mathematics 2022, 10, 3419. https://doi.org/10.3390/math10193419
Hussain S, Sajid Rashid M, Ul Hassan M, Ahmed R. The Generalized Exponential Extended Exponentiated Family of Distributions: Theory, Properties, and Applications. Mathematics. 2022; 10(19):3419. https://doi.org/10.3390/math10193419
Chicago/Turabian StyleHussain, Sajid, Muhammad Sajid Rashid, Mahmood Ul Hassan, and Rashid Ahmed. 2022. "The Generalized Exponential Extended Exponentiated Family of Distributions: Theory, Properties, and Applications" Mathematics 10, no. 19: 3419. https://doi.org/10.3390/math10193419
APA StyleHussain, S., Sajid Rashid, M., Ul Hassan, M., & Ahmed, R. (2022). The Generalized Exponential Extended Exponentiated Family of Distributions: Theory, Properties, and Applications. Mathematics, 10(19), 3419. https://doi.org/10.3390/math10193419






