Magnetorheological Fluid of High-Speed Unsteady Flow in a Narrow-Long Gap: An Unsteady Numerical Model and Analysis
Abstract
:1. Introduction
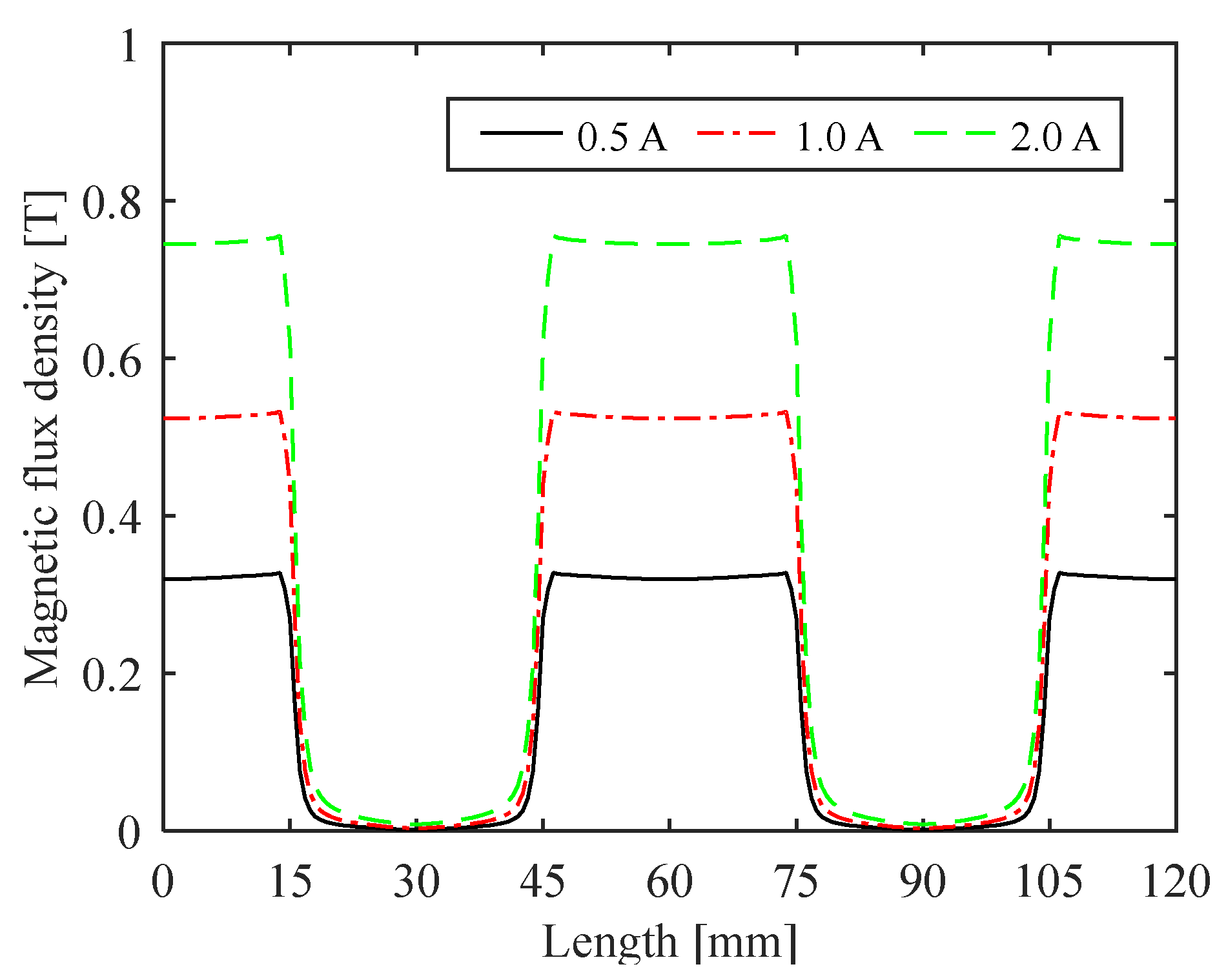
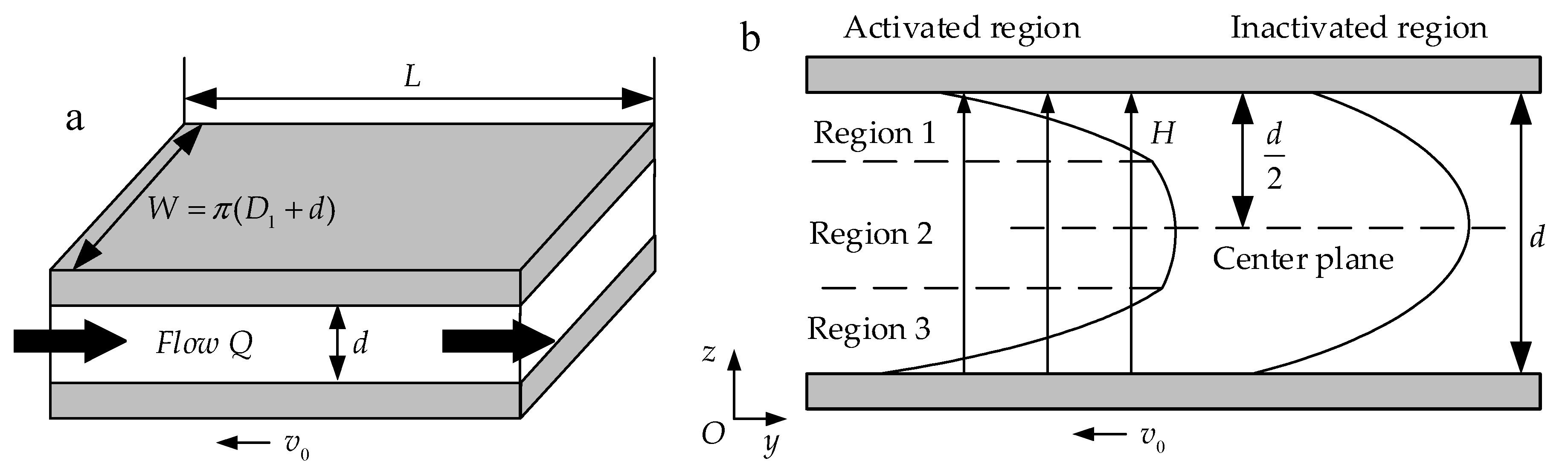
2. Structure and Magnetic Field Analysis of the MRA
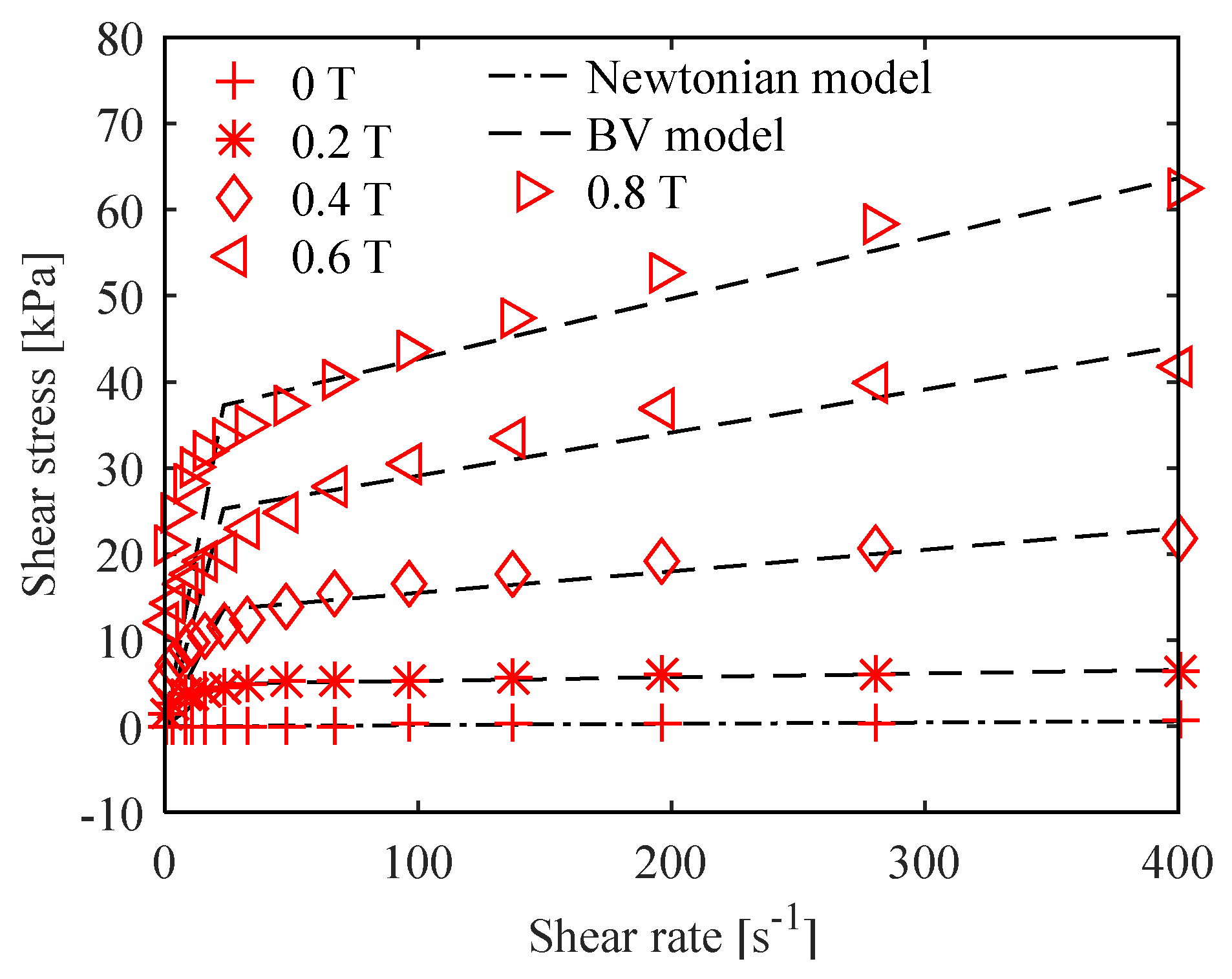
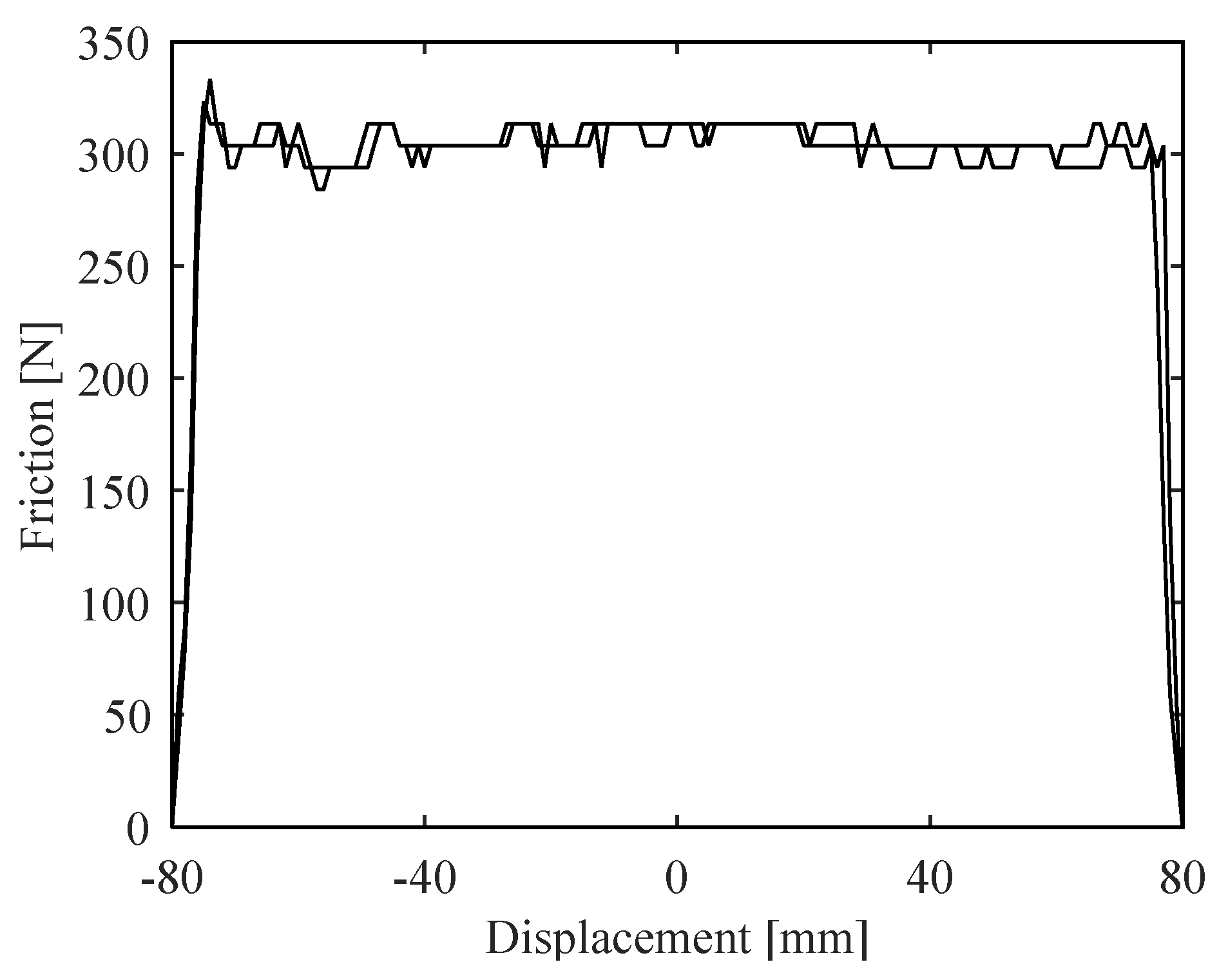
3. MR Fluid Characteristics
4. Unsteady Numerical Model
- In the post-yield region,where , is the pressure gradient of the activated region at time .
- In the pre-yield region,where .
- 3.
- For
- 4.
- For
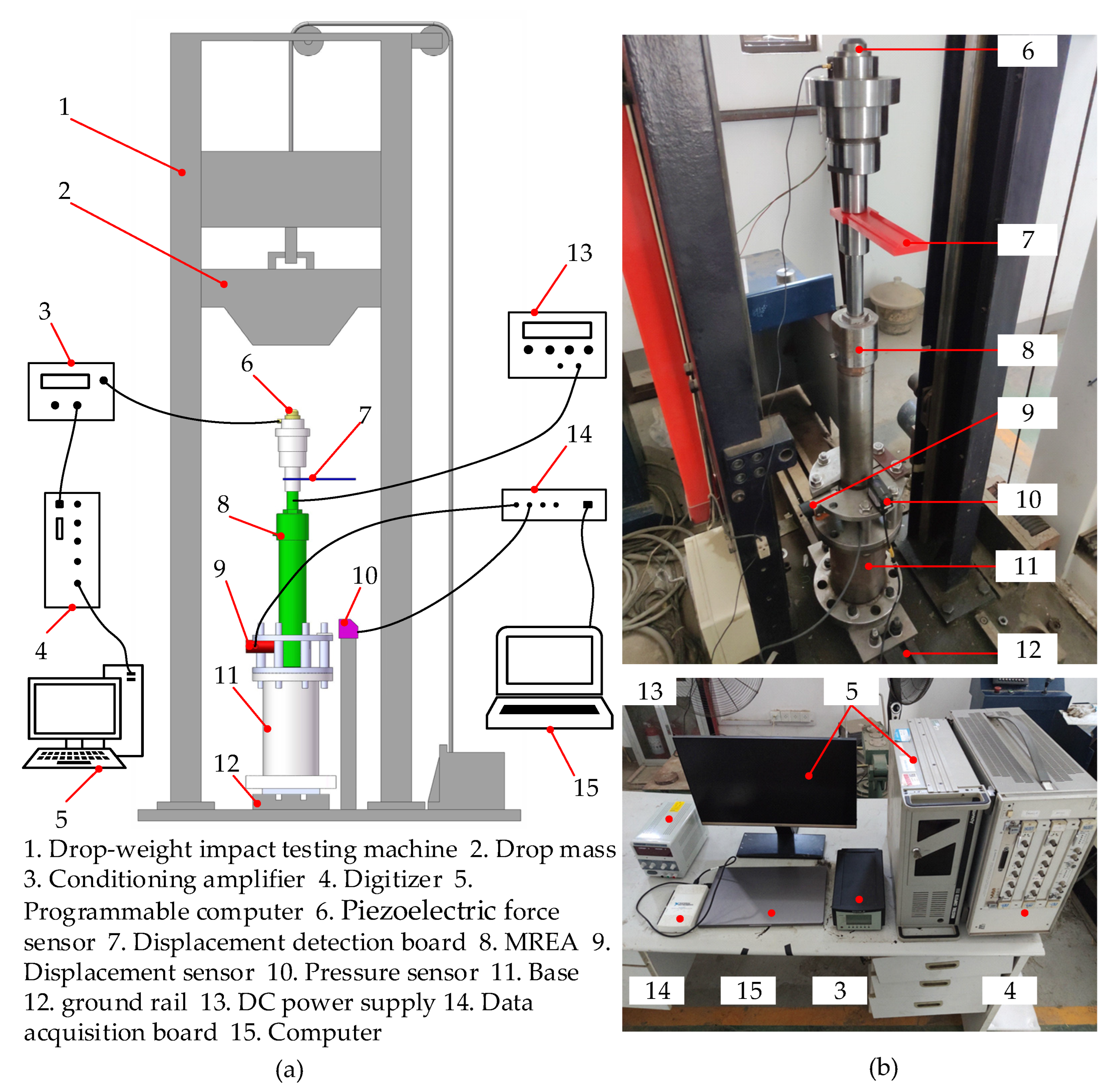
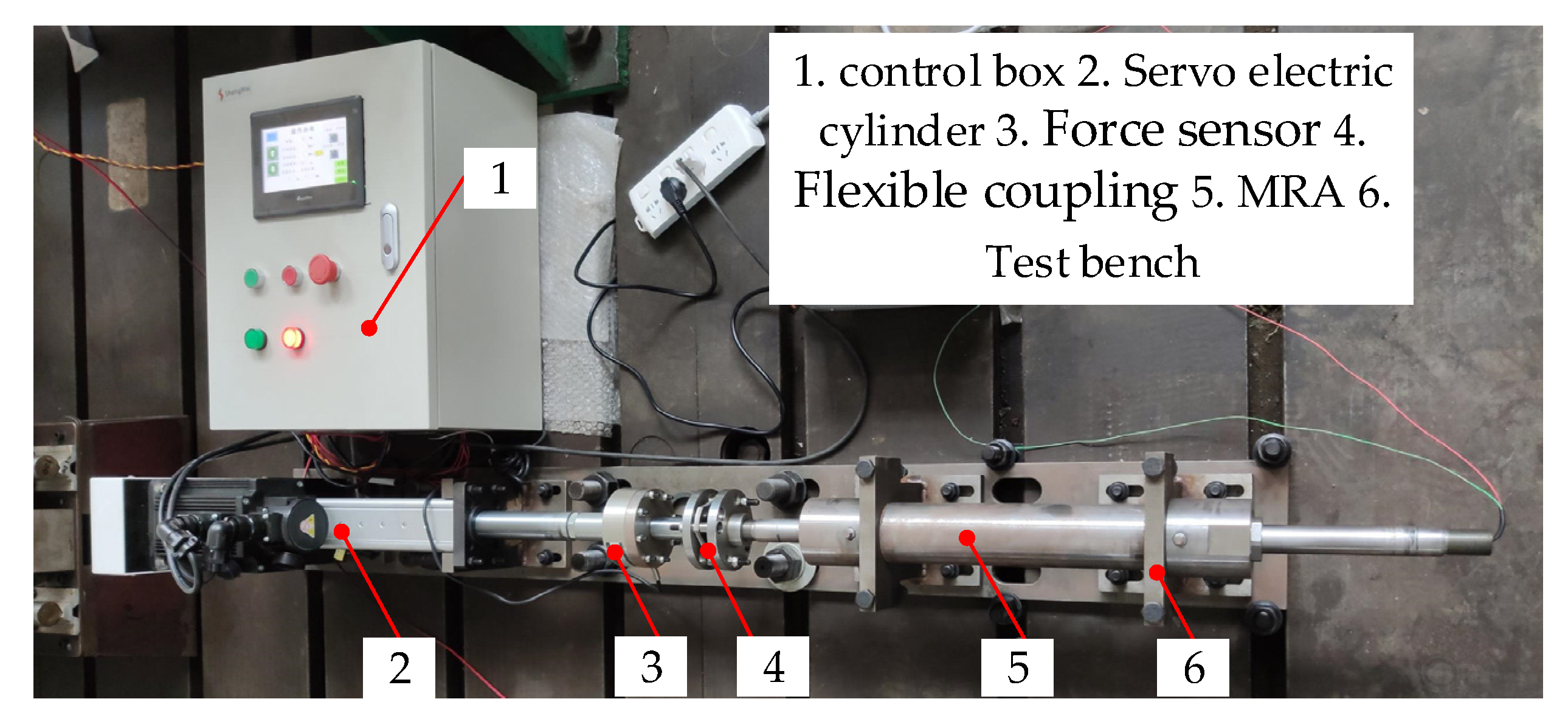
5. Experimental Setup
6. Numerical Simulation Calculation
- At the initial time , the unsteady governing PDE is established, and the initial-boundary conditions are determined.
- The space-time 2D solution domain is discretized. The governing PDE is translated into implicit partial difference equations according to the finite difference method, and the initial-boundary conditions are transformed into the discrete scheme.
- The moving boundary velocity is calculated from Equations (23)–(26), and the volume flow rate is calculated from Equations (18) and (19).
- The starting values of and are used to define the interval , which contains the numerical solution of the pressure gradient. Substitute into Equations (12)–(16) and consider Equations (20)–(22) for the whole activated region. Similarly, substitute into Equations (11) and (16) and consider Equations (20)–(22) for the whole inactivated region. The judgment condition is used to determine if the pressure gradient solution (or ) is within the stated interval. If (or ) does not fall inside the interval, increase the interval range and judge again.
- Iterative calculation yields the numerical solution (or ) of pressure gradient based on the mass conservation, continuity equation, and bisection method. Substituting and the interval midpoint into Equations (12)–(16) and considering Equations (20)–(22) for the whole activated region deduces the corresponding volume flow rate in the gap and , respectively. Similarly, substituting and the interval midpoint into Equations (11) and (16) and considering Equations (20)–(22) for the whole inactivated region deduces the corresponding volume flow rate in the gap and , respectively. Tolerance is defined. If , set ; if , set , then repeat the procedure. The iteration continues until the length of the interval is smaller than the tolerance, i.e., , and the numerical solution of the pressure gradient (or ) is found.
- Substitute the numerical solution of the pressure gradient into the Equations (12)–(15) to calculate the mesh points velocity for the whole activated region. Similarly, substitute the numerical solution of the pressure gradient into the Equation (11) to calculate the mesh points velocity for the total inactivated region.
- Output the kinematic parameters of the moving boundary (or piston rod) as well as the mesh points velocity and pressure gradient in the gap.
- ; repeat steps 3–7.
7. Results and Discussion
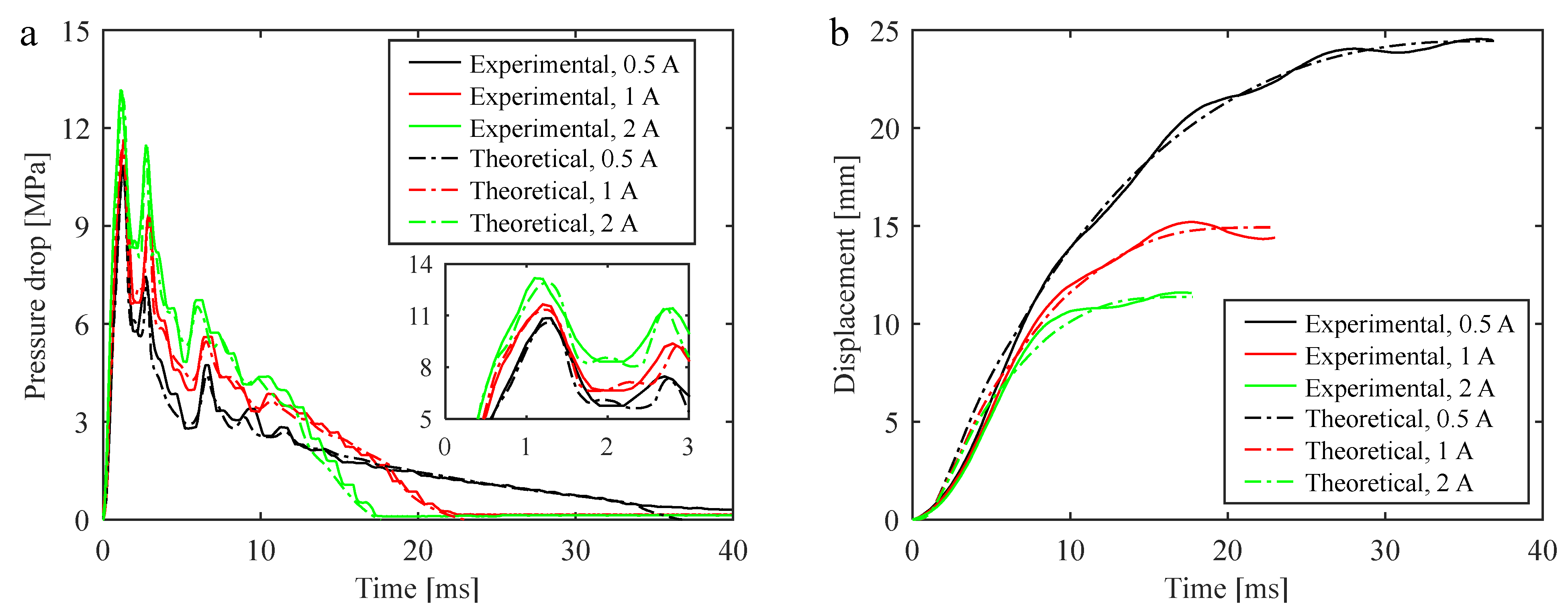
7.1. Model Results and Analysis
7.2. Velocity Profiles and Acceleration Profiles of the MR Fluid in the Gap
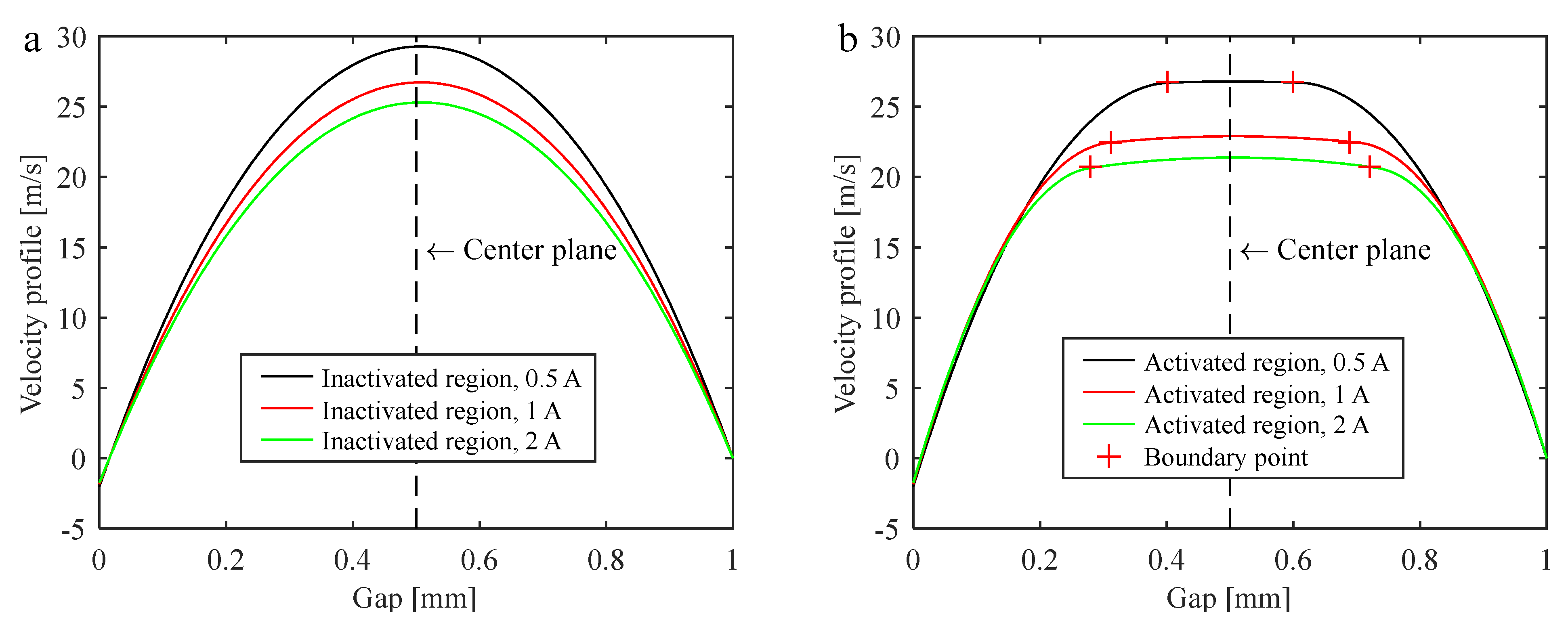
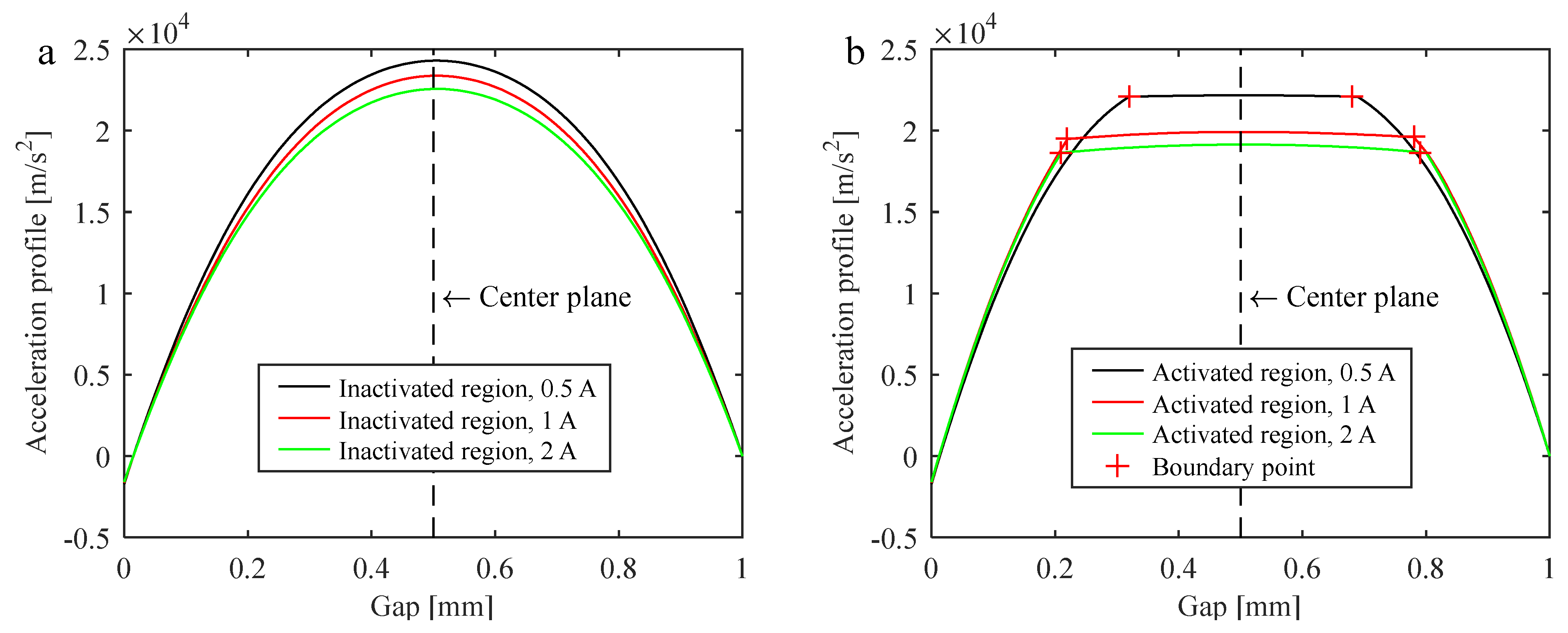
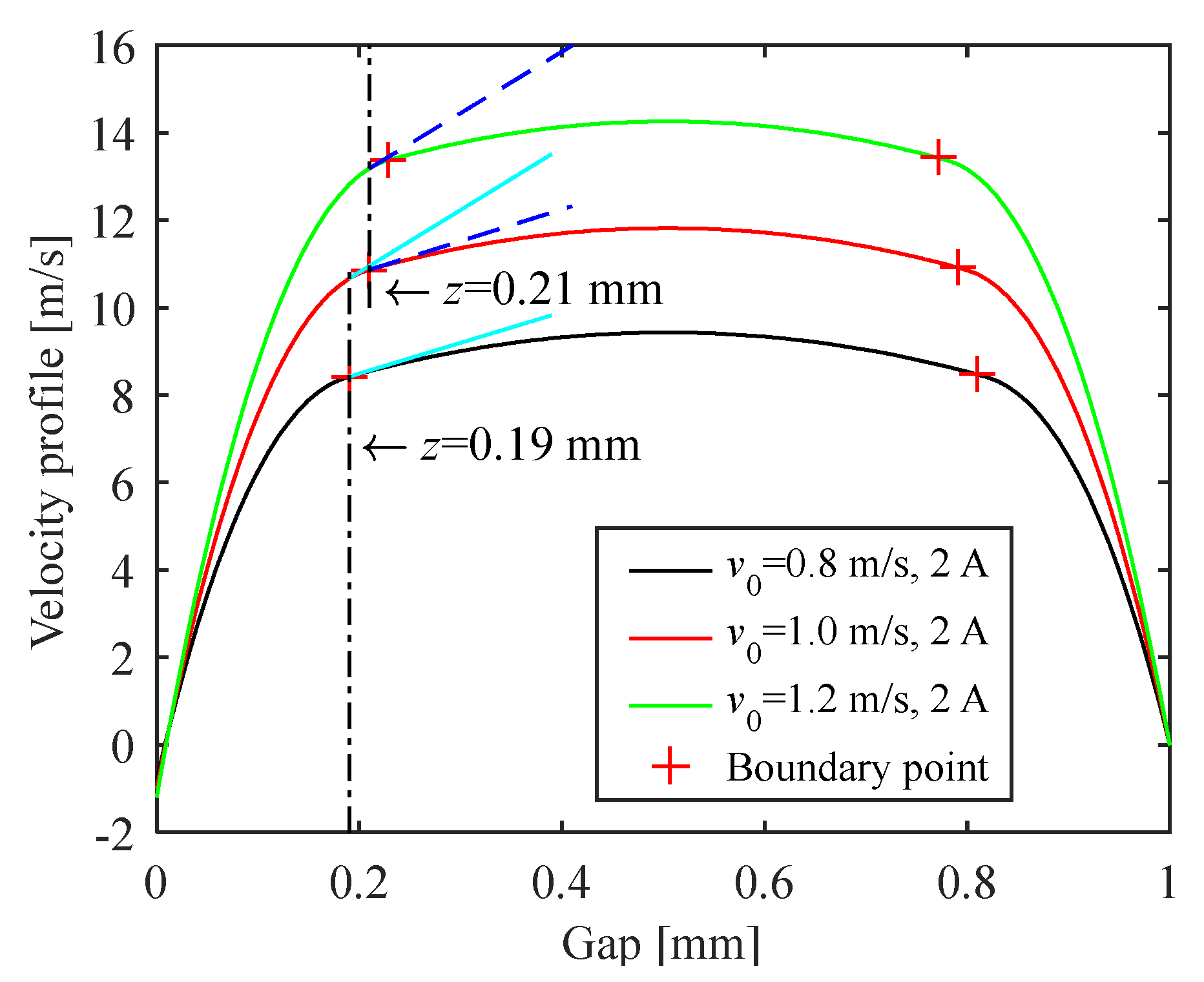
7.2.1. Peak Velocity Profiles and Peak Acceleration Profiles
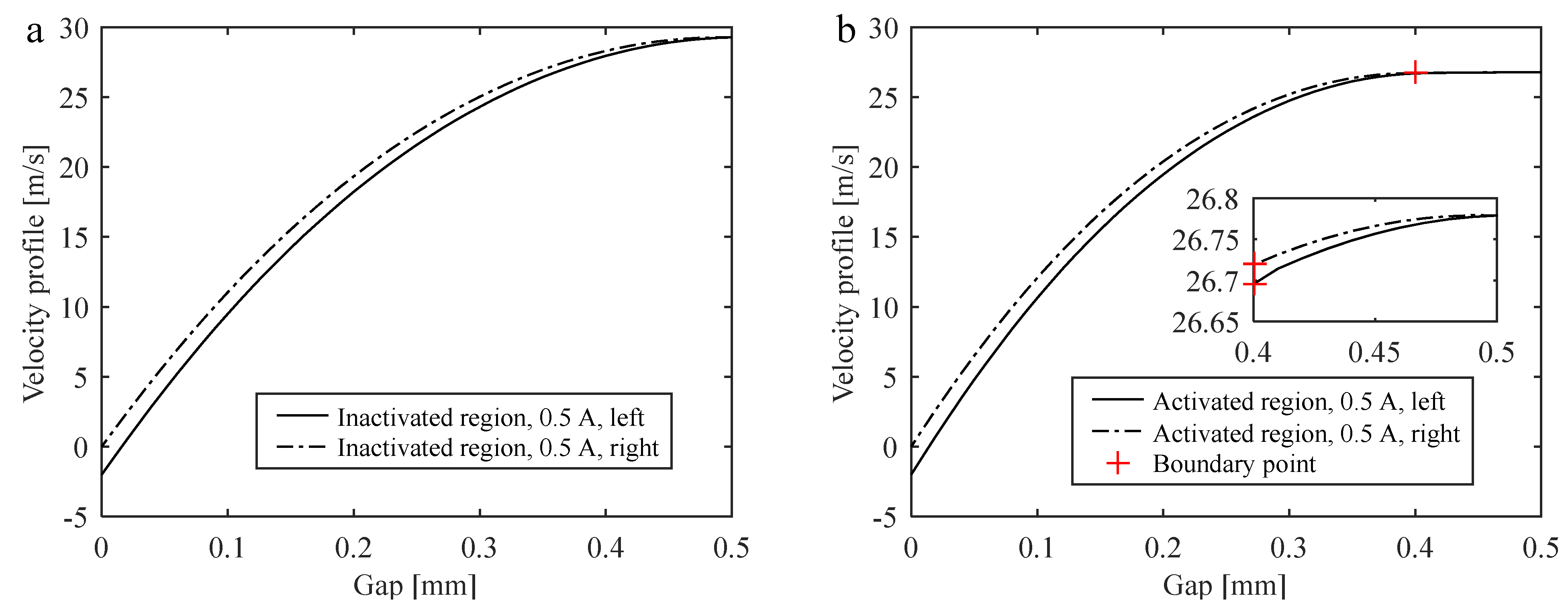
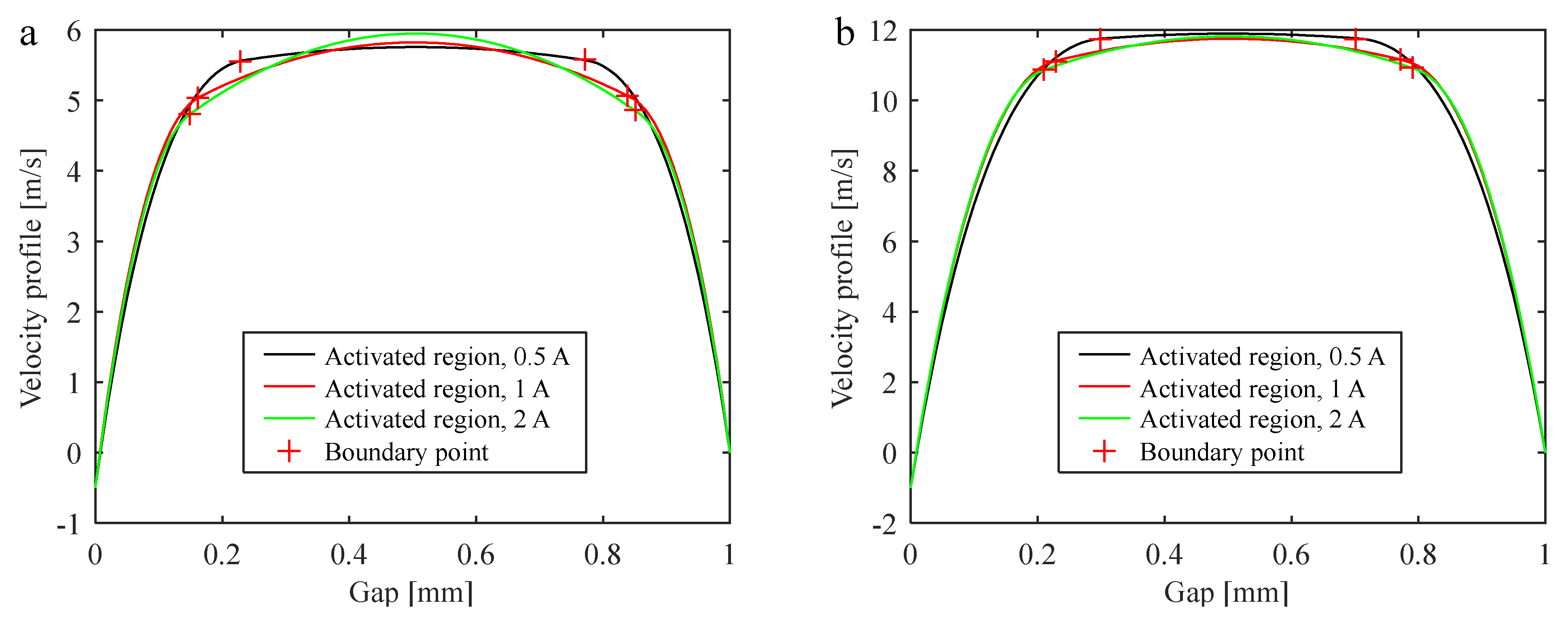
7.2.2. Asymmetric Distribution of the Flow Field
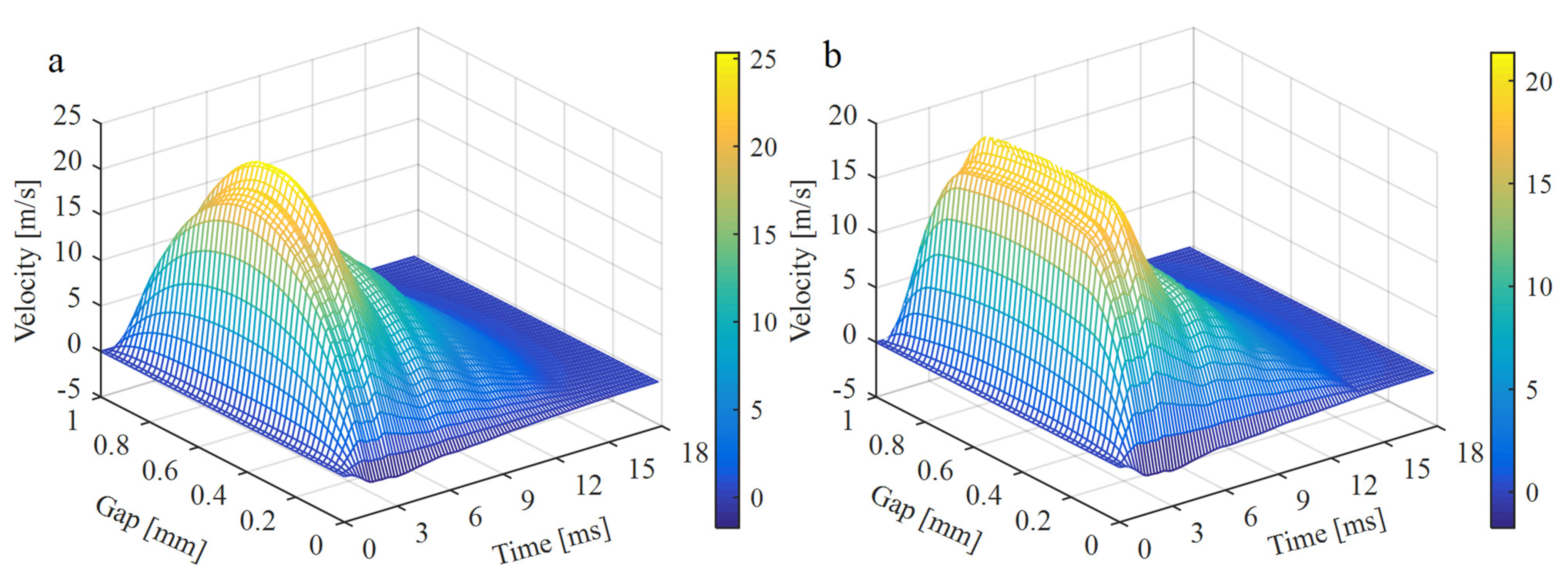
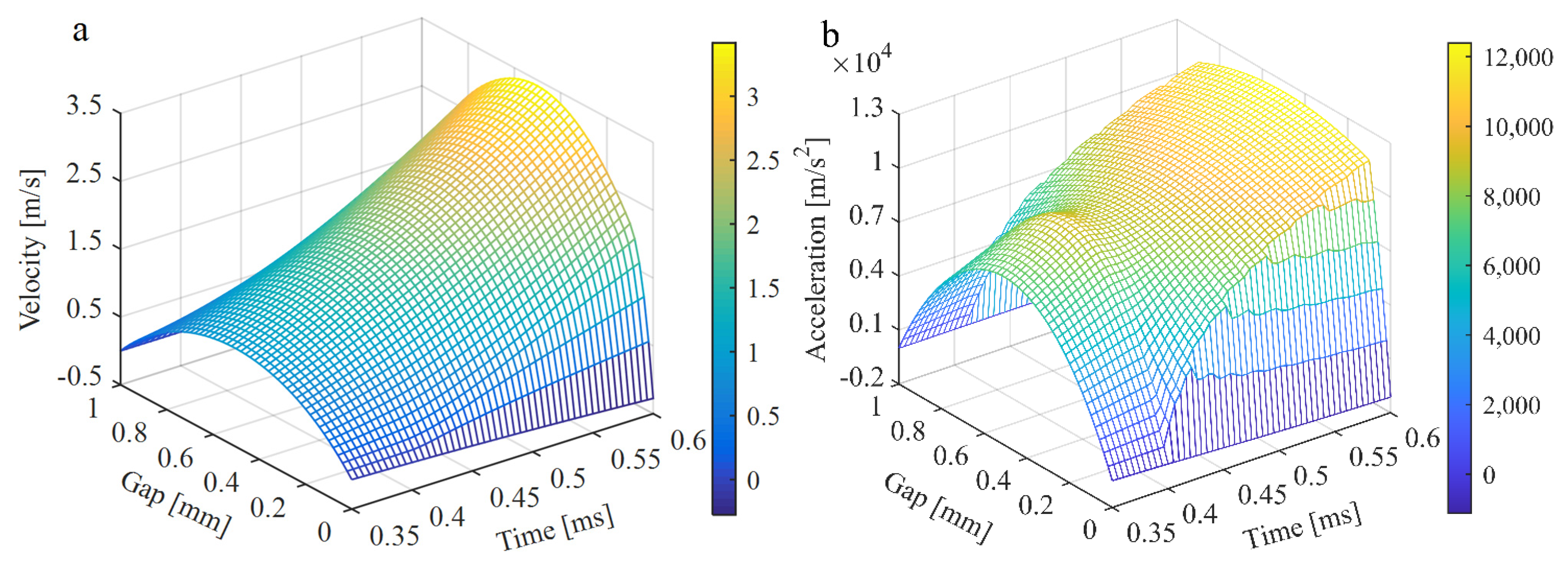
7.2.3. Space-Time Dynamic Distribution of the MR Fluid
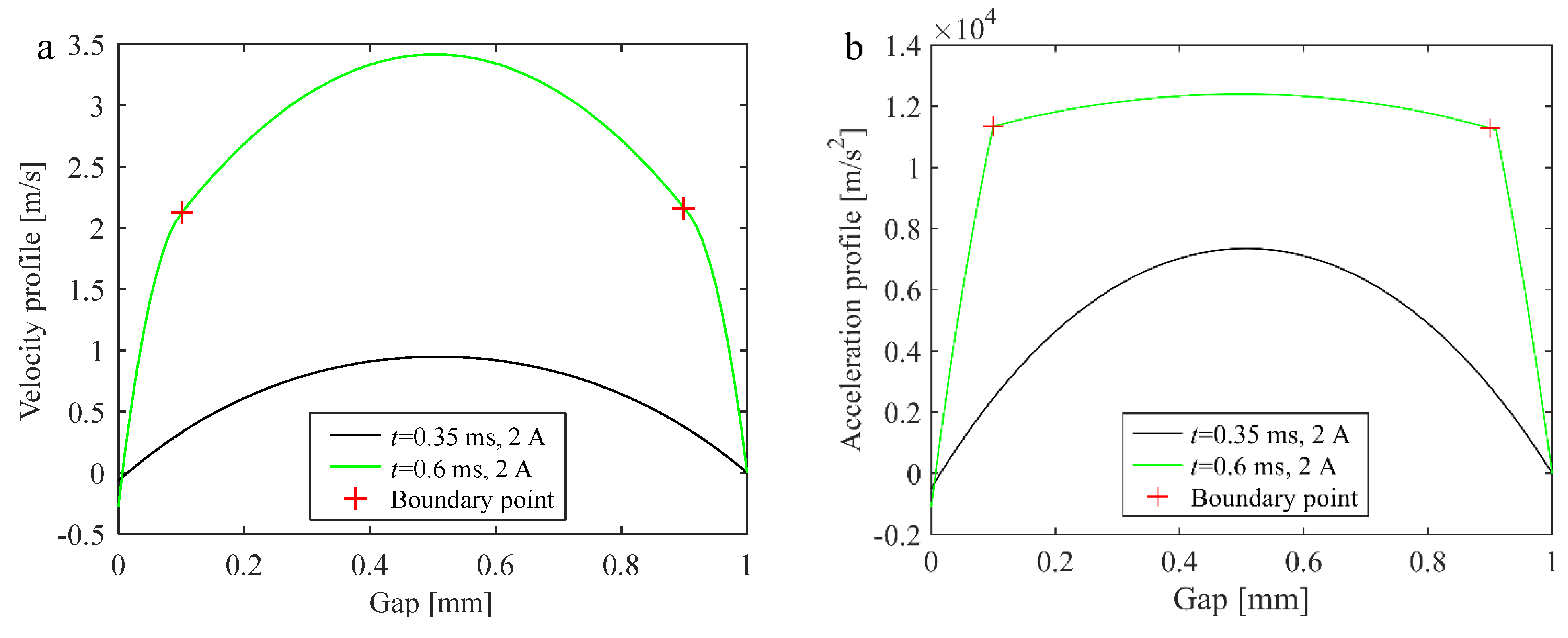
7.2.4. Transition Flow Phenomenon in the Activated Region
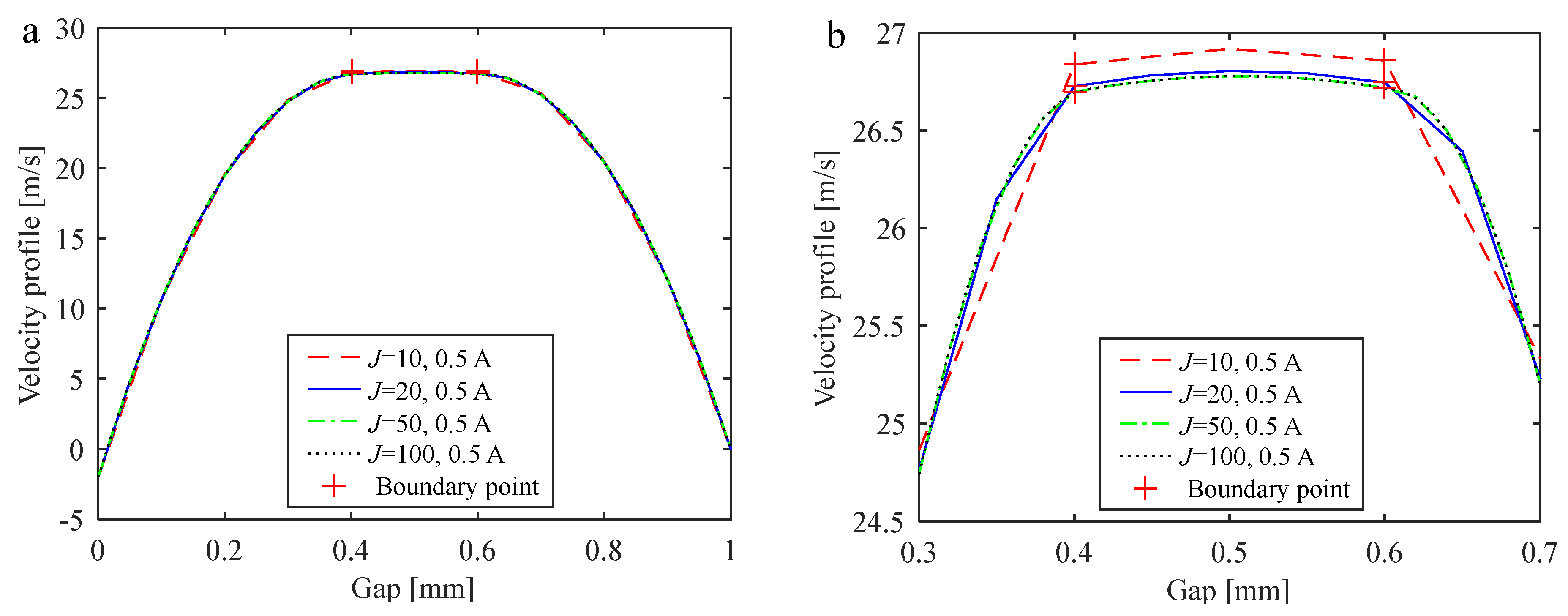
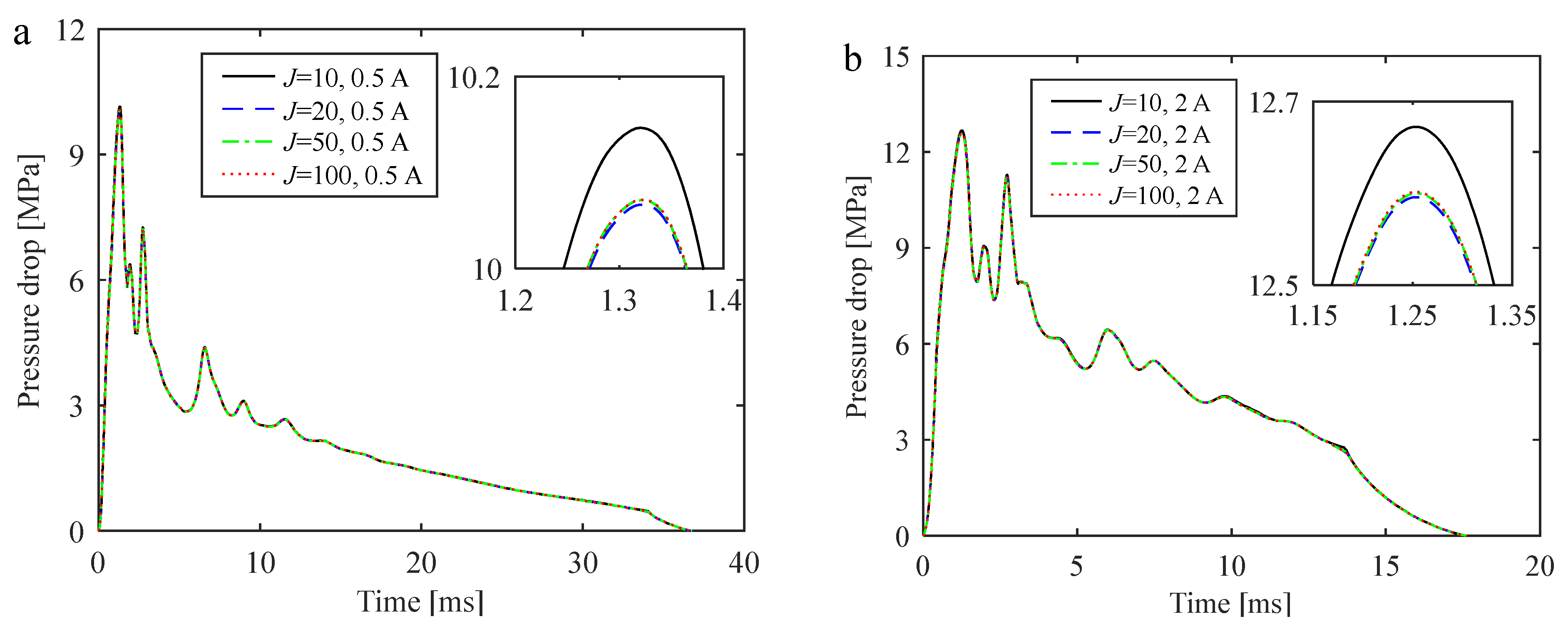
7.3. Influence of Mesh Density on Calculation Results of Unsteady State Model
7.3.1. Influence of Mesh Density on the Velocity Profiles
7.3.2. Influence of Mesh Density on the Pressure Drop
8. Conclusions
- The acceleration profiles are in a non-uniform distribution along the gap’s height when MR fluid unsteady flow is in the gap. The pressure drop of the unsteady flow field in the gap is generated by the dynamic coupling of fluid viscosity and non-uniform flow inertia.
- The asymmetry of the unsteady flow field in the gap is caused by the moving boundary, and the maxima of the velocity and acceleration profiles occur in the center plane. The velocity and acceleration profiles on one side of the moving boundary are less than those on the other side of the fixed boundary with relation to the gap’s central plane, and the thickness of the pre-yield region is symmetrical about the center plane.
- When the excitation current is determined, the thickness of the pre-yield region in the activated region gradually decreases as the volume flow rate is increased. When the volume flow rate is determined, increasing the excitation current increases the transition stress of the MR fluid in the activated region, which increases the thickness of the pre-yield region.
- There is a transition flow phenomenon during the change of the MR fluid volume flow rate in the activated region. When the volume flow rate is small, the activated region only has the pre-yield region. However, as the volume flow rate increases, the post-yield region will appear on both sides of the boundary. Meanwhile, the distribution of velocity and acceleration profiles changes noticeably before and after the transition.
- When the mesh number J along the gap’s height is 10–100, the pressure drop of the gap solved by the unsteady numerical model has good convergence under the constraint of the numerical scheme stability.
- The proposed unsteady numerical model helps to better understand the properties of the unsteady laminar boundary layer flow of MR fluid in the gap, which may also be used as a theoretical reference for solving Newtonian and bi-viscous fluid unsteady laminar boundary layer flow problems.
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Alam, M.K.; Bibi, K.; Khan, A.; Noeiaghdam, S. Dufour and Soret Effect on Viscous Fluid Flow between Squeezing Plates under the Influence of Variable Magnetic Field. Mathematics 2021, 9, 2404. [Google Scholar] [CrossRef]
- Abderrahmane, A.; Qasem, N.A.A.; Younis, O.; Marzouki, R.; Mourad, A.; Shah, N.A.; Chung, J.D. MHD Hybrid Nanofluid Mixed Convection Heat Transfer and Entropy Generation in a 3-D Triangular Porous Cavity with Zigzag Wall and Rotating Cylinder. Mathematics 2022, 10, 769. [Google Scholar] [CrossRef]
- Murillo-García, O.F.; Jurado, F. Adaptive Boundary Control for a Certain Class of Reaction–Advection–Diffusion System. Mathematics 2021, 9, 2224. [Google Scholar] [CrossRef]
- Wilson, S. Squeezing flow of a Bingham material. J. Non-Newton Fluid Mech. 1993, 47, 211–219. [Google Scholar] [CrossRef]
- Lin, C.J.; Yau, H.T.; Lee, C.Y.; Tung, K.H. System Identification and Semiactive Control of a Squeeze-Mode Magnetorheological Damper. IEEE ASME Trans. Mechatron. 2013, 18, 1691–1701. [Google Scholar] [CrossRef]
- Kim, P.; Seok, J. Viscoplastic flow in slightly varying channels with wall slip pertaining to a magnetorheological (MR) polishing process. J. Non-Newton Fluid Mech. 2011, 166, 972–992. [Google Scholar] [CrossRef]
- Jiang, R.L.; Rui, X.T.; Yang, F.F.; Zhu, W.; Zhu, H.T.; Jiang, M. Simulation and experiment of the magnetorheological seat suspension with a seated occupant in both shock and vibration occasions. Smart Mater. Struct. 2020, 29, 105008. [Google Scholar] [CrossRef]
- Liu, X.H.; Wang, N.N.; Wang, K.; Chen, S.M.; Sun, S.S.; Li, Z.X.; Li, W.H. A new AI-surrogate model for dynamics analysis of a magnetorheological damper in the semi-active seat suspension. Smart Mater. Struct. 2020, 29, 037001. [Google Scholar] [CrossRef]
- Saleh, M.; Sedaghati, R.; Bhat, R. Dynamic analysis of an SDOF helicopter model featuring skid landing gear and an MR damper by considering the rotor lift factor and a Bingham number. Smart Mater. Struct. 2018, 27, 065013. [Google Scholar] [CrossRef]
- Yoon, J.Y.; Kang, B.H.; Kin, J.H.; Choi, S.B. New control logic based on mechanical energy conservation for aircraft landing gear system with magnetorheological dampers. Smart Mater. Struct. 2020, 29, 084003. [Google Scholar] [CrossRef]
- Liu, S.C.; Chen, Z.Q.; Wang, X.Y.; Ko, J.M.; Ni, Y.Q.; Spencer, B.F., Jr.; Yang, G. MR damping system on Dongting Lake cable-stayed bridge. In Proceedings of the Smart Structures and Materials 2003: Smart Systems and Nondestructive Evaluation for Civil Infrastructures, San Diego, CA, USA, 18 August 2003; pp. 229–235. [Google Scholar] [CrossRef]
- Kim, Y.; Langari, R.; Hurlebaus, S. Semiactive nonlinear control of a building with a magnetorheological damper system. Mech. Syst. Signal. Process. 2009, 23, 300–315. [Google Scholar] [CrossRef]
- Yang, G.; Spencer, B.F., Jr.; Carlson, J.D.; Sain, M.K. Large-scale MR fluid dampers: Modeling and dynamic performance considerations. Eng. Struct. 2002, 24, 309–323. [Google Scholar] [CrossRef]
- Ai, H.X.; Wang, D.H.; Liao, W.H. Design and modeling of a magnetorheological valve with both annular and radial flow paths. J. Intell. Mater. Syst. Struct. 2006, 17, 327–334. [Google Scholar] [CrossRef]
- Hong, S.R.; John, S.; Wereley, N.M.; Choi, Y.T.; Choi, S.B. A Unifying Perspective on the Quasi-steady Analysis of Magnetorheological Dampers. J. Intell. Mater. Syst. Struct. 2007, 19, 959–976. [Google Scholar] [CrossRef]
- Zhang, X.J.; Wu, R.C.; Guo, K.H.; Zu, P.Y.; Ahmadian, M. Dynamic characteristics of magnetorheological fluid squeeze flow considering wall slip and inertia. J. Intell. Mater. Syst. Struct. 2020, 31, 229–242. [Google Scholar] [CrossRef]
- Chen, P.; Bai, X.X.; Qian, L.J. Magnetorheological fluid behavior in high-frequency oscillatory squeeze mode: Experimental tests and modelling. J. Appl. Phys. 2016, 119, 105101. [Google Scholar] [CrossRef]
- Nguyen, Q.H.; Choi, S.B. Dynamic modeling of an electrorheological damper considering the unsteady behavior of electrorheological fluid flow. Smart Mater. Struct. 2009, 18, 055016. [Google Scholar] [CrossRef]
- Zolfagharian, M.M.; Kayhani, M.H.; Norouzi, M.; Norouzi, M.; Jalali, A. Parametric investigation of twin tube magnetorheological dampers using a new unsteady theoretical analysis. J. Intell. Mater. Syst. Struct. 2019, 30, 878–895. [Google Scholar] [CrossRef]
- Wang, J.; Li, Y.C. Dynamic Simulation and Test Verification of MR Shock Absorber under Impact Load. J. Intell. Mater. Syst. Struct. 2006, 17, 309–314. [Google Scholar] [CrossRef]
- Zhang, X.W.; Yu, T.X.; Wen, W.J. Electro-rheological Cylinders used as Impact Energy Absorbers. J. Intell. Mater. Syst. Struct. 2010, 21, 729–745. [Google Scholar] [CrossRef]
- Mao, M. Adaptive Magnetorheological Sliding Seat System for Ground Vehicles. Ph.D. Thesis, University of Maryland, College Park, MD, USA, 2011. [Google Scholar]
- Mao, M.; Hu, W.; Choi, Y.T.; Wereley, N.M.; Browne, A.L.; Ulicny, J.; Johnson, N. Nonlinear modeling of magnetorheological energy absorbers under impact conditions. Smart Mater. Struct. 2013, 22, 115015. [Google Scholar] [CrossRef]
- Haberman, R. Applied Partial Differential Equations with Fourier Series and Boundary Value Problems, 5th ed.; Pearson Education: Upper Saddle River, NJ, USA, 2012. [Google Scholar]
- Woodford, C.; Phillips, C. Numerical Methods with Worked Examples: Matlab Edition, 2nd ed.; Springer: New York, NY, USA, 2012. [Google Scholar]


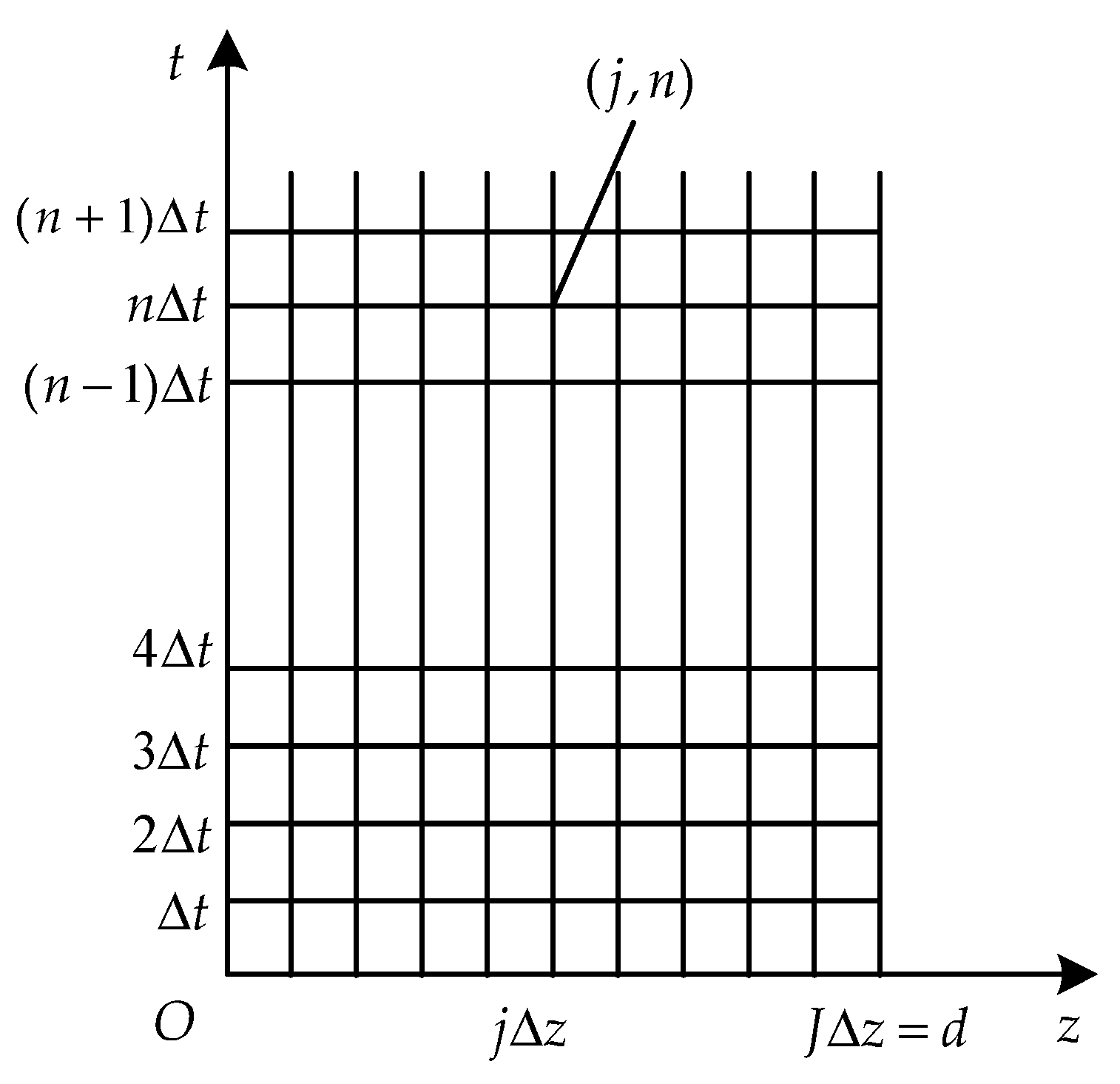






| Parameters | Value |
|---|---|
| Piston rod diameter, D0 | 30 mm |
| Piston head diameter, D1 | 54 mm |
| Inner diameter of hydraulic cylinder, D2 | 56 mm |
| Height of the gap, d | 1 mm |
| Total length of the gap, L | 120 mm |
| Total length of the activated region, La | 60 mm |
| MR fluid density, ρ | 2650 kg/m3 |
| 0.5 A | 1 A | 2 A | |
|---|---|---|---|
| J = 10 | 7.479 × 10−2 | 7.960 × 10−2 | 7.099 × 10−2 |
| J = 20 | 5.155 × 10−3 | 5.499 × 10−3 | 5.699 × 10−3 |
| J = 50 | 5.611 × 10−4 | 1.993 × 10−4 | 1.592 × 10−3 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zheng, P.; Hou, B.; Zou, M. Magnetorheological Fluid of High-Speed Unsteady Flow in a Narrow-Long Gap: An Unsteady Numerical Model and Analysis. Mathematics 2022, 10, 2493. https://doi.org/10.3390/math10142493
Zheng P, Hou B, Zou M. Magnetorheological Fluid of High-Speed Unsteady Flow in a Narrow-Long Gap: An Unsteady Numerical Model and Analysis. Mathematics. 2022; 10(14):2493. https://doi.org/10.3390/math10142493
Chicago/Turabian StyleZheng, Pengfei, Baolin Hou, and Mingsong Zou. 2022. "Magnetorheological Fluid of High-Speed Unsteady Flow in a Narrow-Long Gap: An Unsteady Numerical Model and Analysis" Mathematics 10, no. 14: 2493. https://doi.org/10.3390/math10142493
APA StyleZheng, P., Hou, B., & Zou, M. (2022). Magnetorheological Fluid of High-Speed Unsteady Flow in a Narrow-Long Gap: An Unsteady Numerical Model and Analysis. Mathematics, 10(14), 2493. https://doi.org/10.3390/math10142493






