Time-Dependent Analytic Solutions for Water Waves above Sea of Varying Depths
Abstract
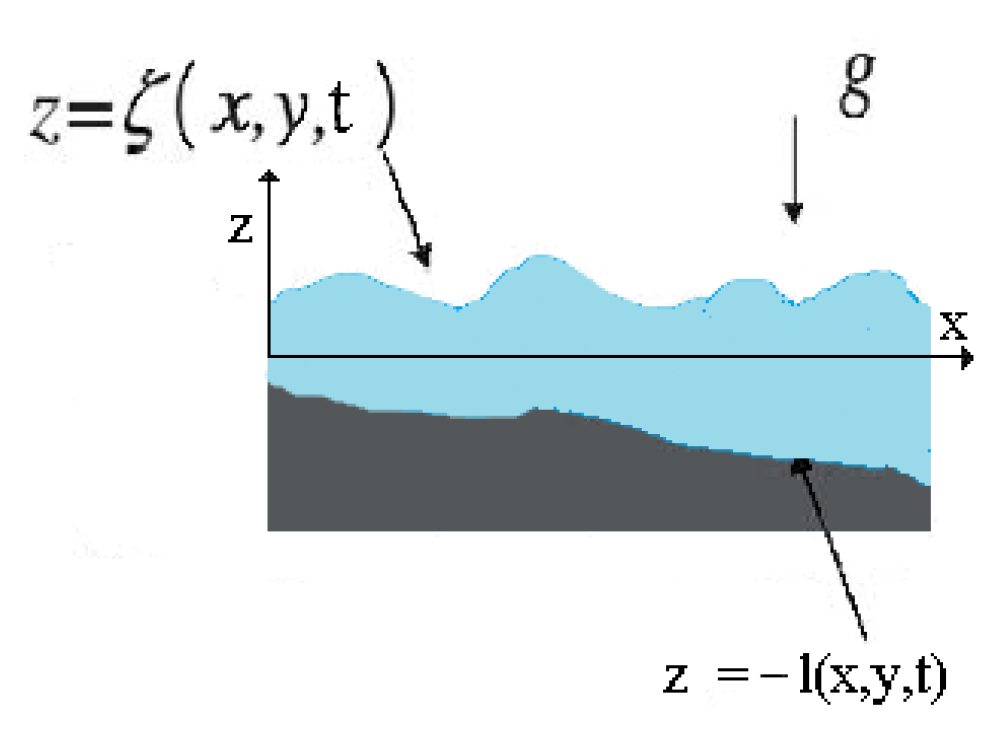
1. Introduction
2. Theory and Results
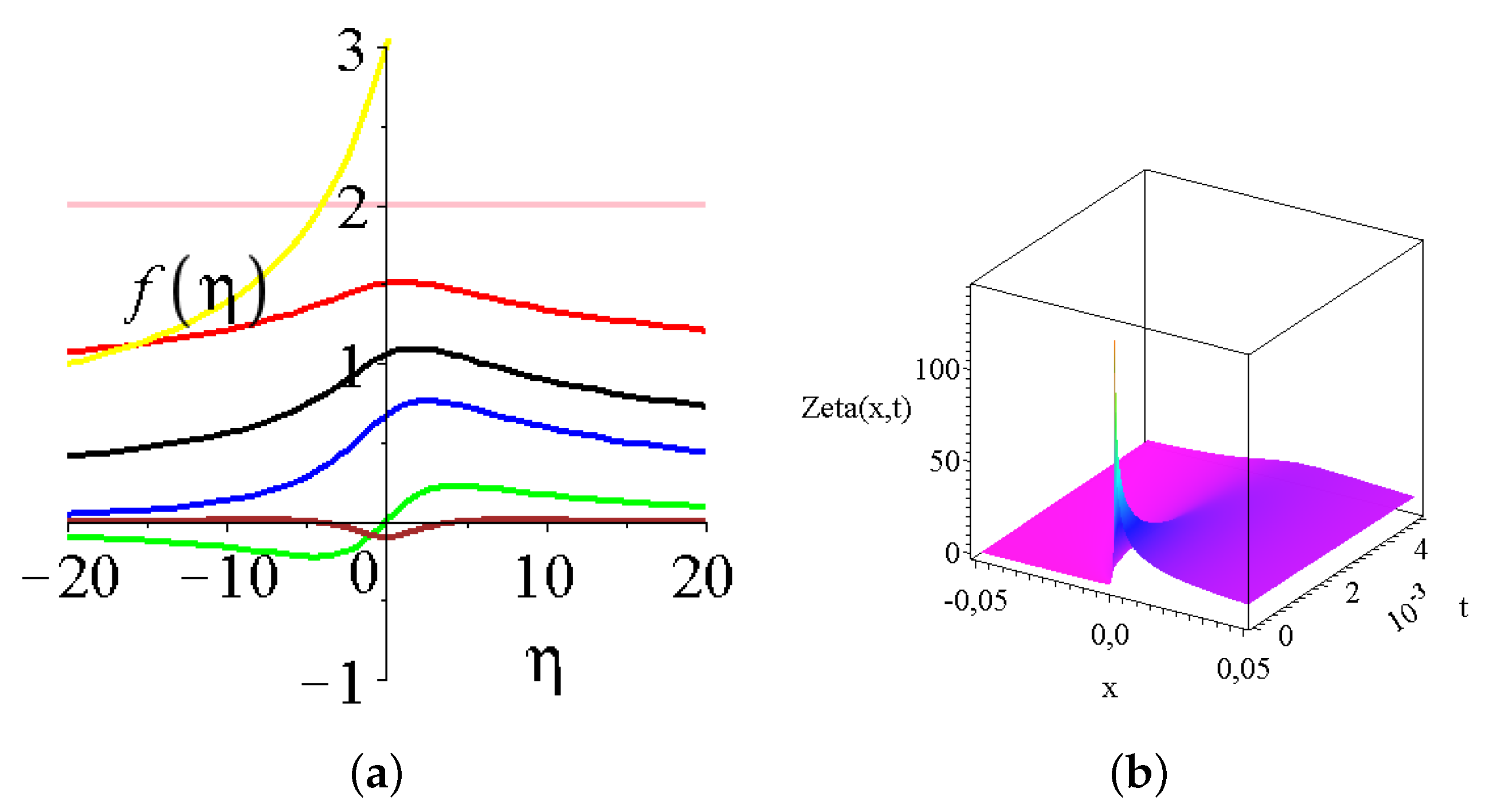
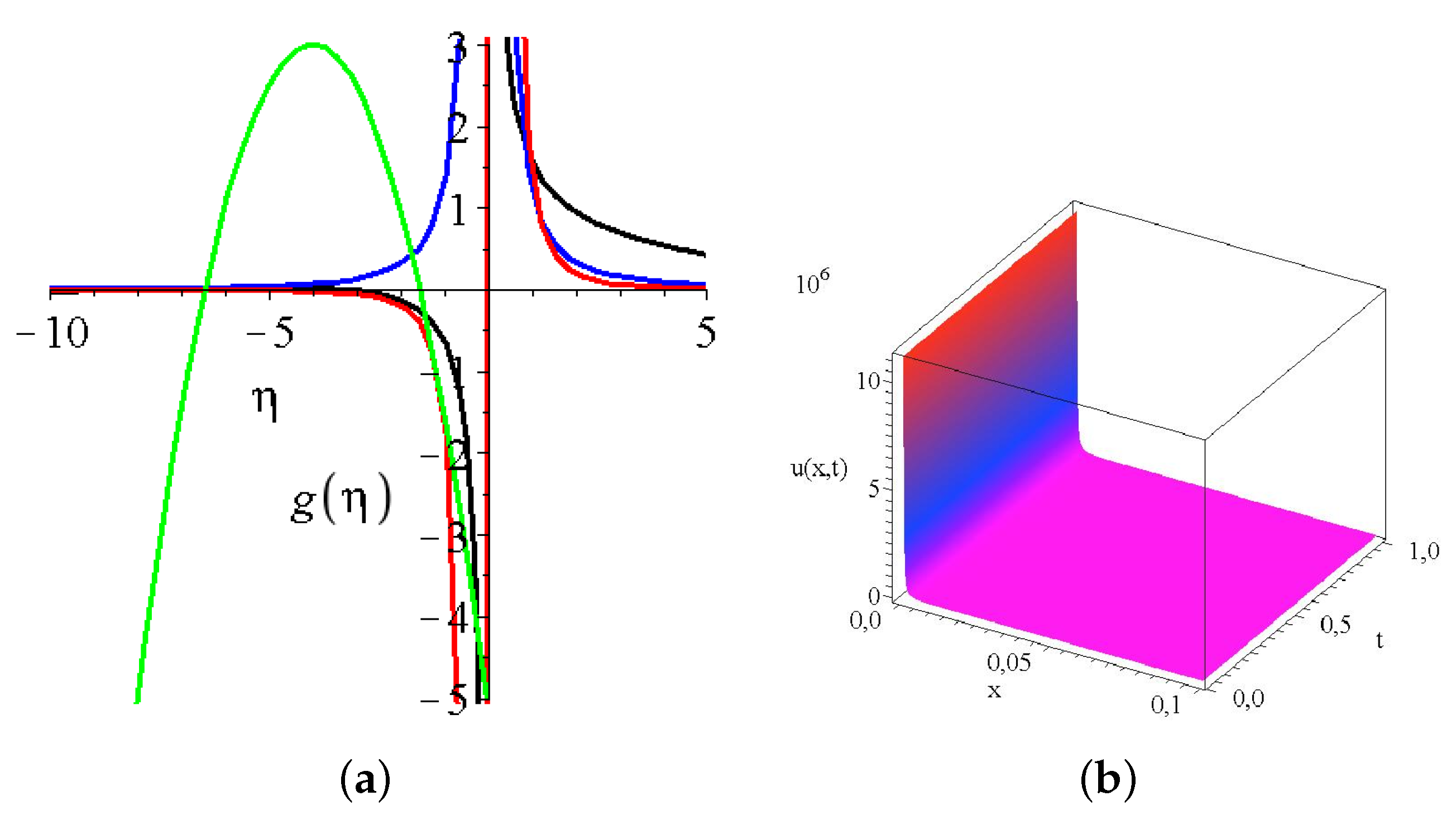
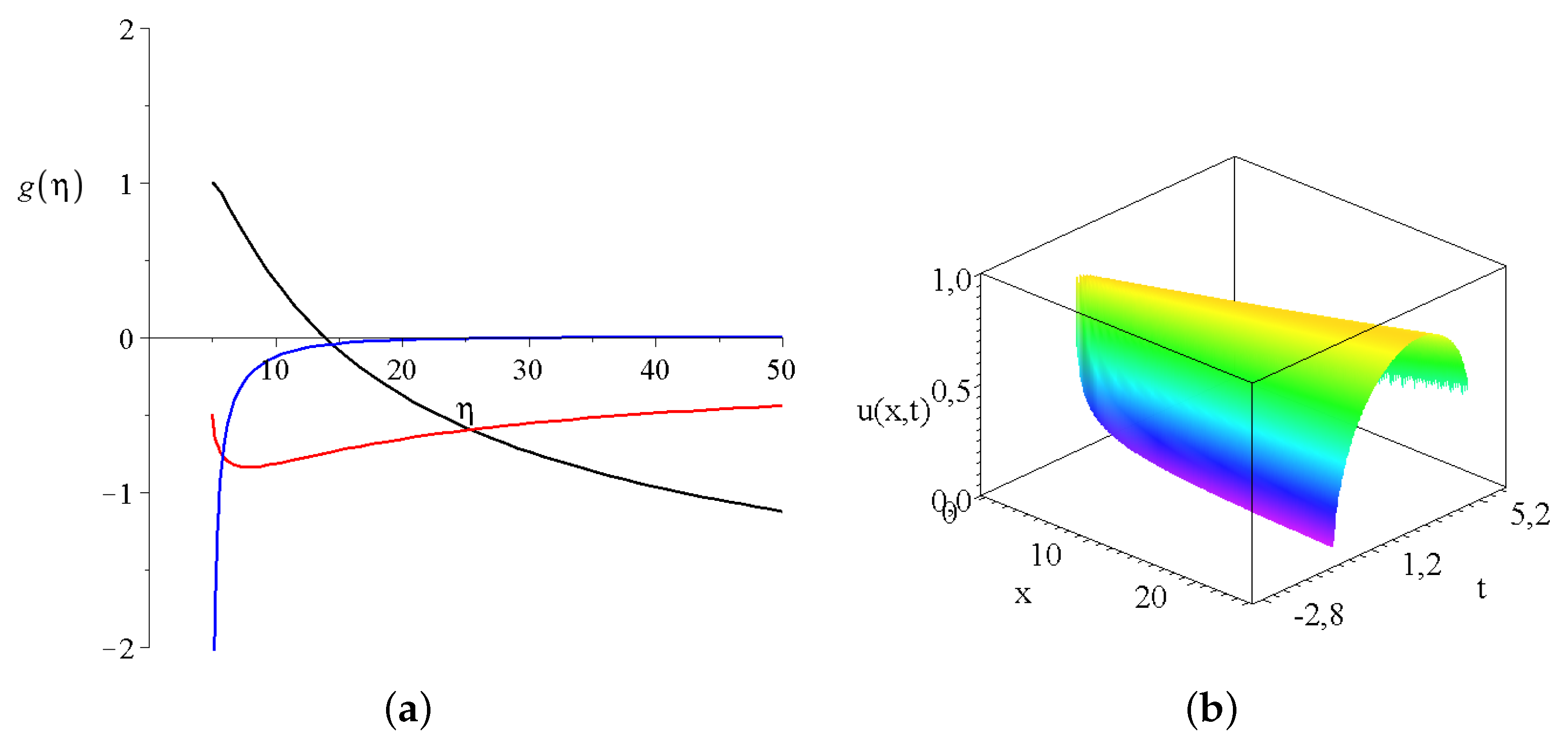
2.1. The Constant Seabed Function
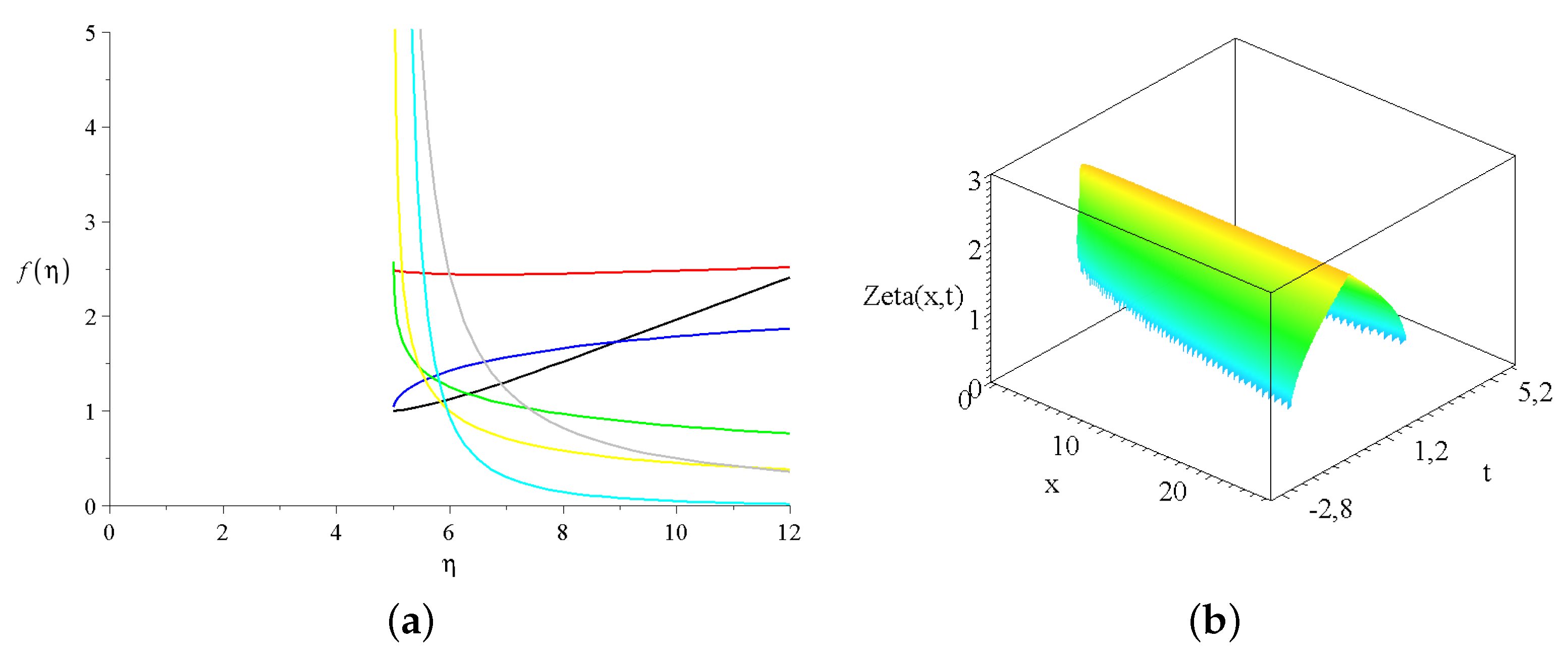
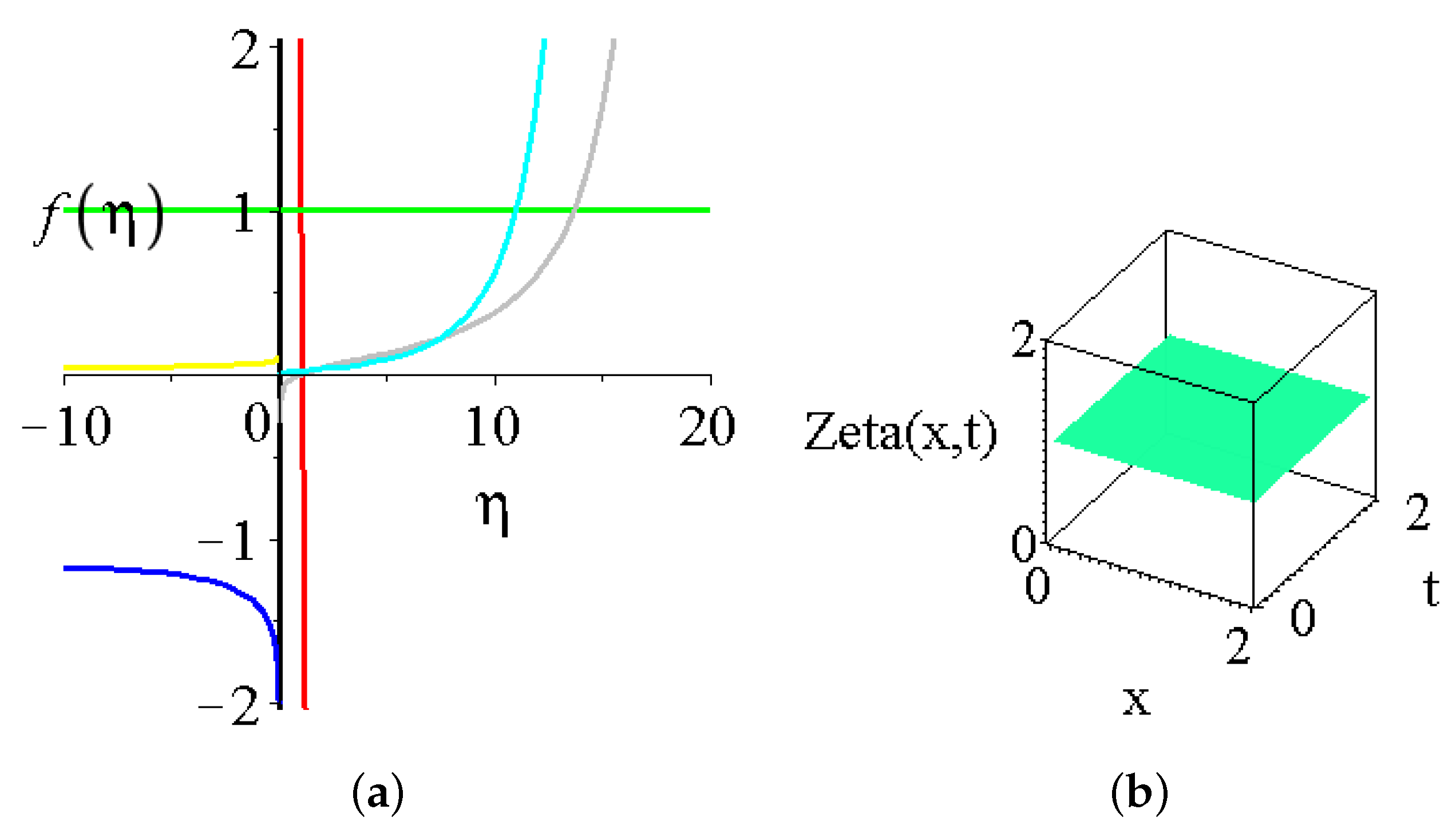
2.2. The Linear Seabed Function
2.3. Traveling Wave Analysis
3. Conclusions and Outlook
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Crawford, F.S., Jr. Waves; Berkeley Physics Course; McGraw-Hill: New York, NY, USA, 1965; Volume 3. [Google Scholar]
- Coulson, C.A.; Jeffrey, A. Waves: A Mathematical Approach to the Common Types of Wave Motion, 2nd ed.; Longman: London, UK, 1977. [Google Scholar]
- King, G.C. Vibration and Waves; The Manchester Physics Series; John Wiley & Sons: Hoboken, NJ, USA, 2009. [Google Scholar]
- Whitham, G.B. Linear and Nonlinear Waves; John Wiley & Sons: Hoboken, NJ, USA, 1974. [Google Scholar]
- Brillouine, L. Wave Propagation and Group Velocity, 1st ed.; Academic Press: New York, NY, USA, 1960. [Google Scholar]
- Fritz, J. Nonlinear Wave Equations, Formation of Singularities; University Lecture Series; American Mathematical Society: Providence, RI, USA, 1990; Volume 2. [Google Scholar]
- Ablowitz, M.J. Nonlinear Dispersive Waves: Asymptotic Analysis and Solitons; Cambridge University Press: Cambridge, UK, 2011. [Google Scholar]
- Debnath, L. Nonlinear Dispersive Waves, 1st ed.; Cambridge University Press: New York, NY, USA, 1994. [Google Scholar]
- Kundu, A. (Ed.) Tsunami and Nonlinear Waves; Springer: Berlin/Heidelberg, Germany, 2007. [Google Scholar]
- Pedlosky, J. (Ed.) Waves in the Ocean and Atmosphere; Springer: Berlin/Heidelberg, Germany, 2003. [Google Scholar]
- Holthuijsen, L.H. (Ed.) Waves in Oceanic and Coastal Waters; Cambridge University Press: Cambridge, UK, 2007. [Google Scholar]
- Johnson, R.S. (Ed.) A Modern Introduction to the Mathematical Theory of Water Waves; Cambridge University Press: Cambridge, UK, 1997. [Google Scholar]
- Pelinovsky, E.; Kharif, C. (Eds.) Extreme Ocean Waves; Springer: Dordrecht, The Netherlands, 2008. [Google Scholar]
- Komen, G.J.; Cavaleri, L.; Donel, M.; Hasselmann, K.; Hasselmann, S.; Janssen, P.A.E.M. Dynamics and Modelling of Ocean Waves; Cambridge University Press: Cambridge, UK, 1994. [Google Scholar]
- Young, I.R. Wind Generated Ocean Waves; Elsevier Ocean Engineering Series; Elsevier: Amsterdam, The Netherlands, 1999; Volume 2. [Google Scholar]
- Huang, H. Dynamics of Surface Waves in Coastal Waters, 1st ed.; Springer: Berlin/Heidelberg, Germany, 2009. [Google Scholar]
- Barber, N.F.; Ghey, G. Water Waves; The Wykeham Science Series for Schools and Universities, Wykeham Publications and London & Winchester: London, UK, 1969; Volume 5. [Google Scholar]
- Airy, G.B. Tides and Waves. In Encyclopedia Metropolitana; Smedley, E., Rose, H.J., Rose, H.J., Eds.; Part Publication: London, UK, 1841. [Google Scholar]
- Timman, R.; Hermans, A.J.; Hsiao, G.C. Water Waves and Ship Hydrodynamics, 1st ed.; Mechanics of Fluids and Transport Processes; Springer: Dodrecht, The Netherlands, 1985; Volume IX. [Google Scholar]
- Madsen, P.A.; Fuhrman, D.R.; Wang, B. A Boussinesq-type method for fully nonlinear waves interacting with a rapidly varying bathymetry. Coast. Eng. 2006, 53, 487–504. [Google Scholar] [CrossRef]
- Wazwaz, A.M. The variational iteration method for rational solutions for KdV, K(2,2), Burgers, and cubic Boussinesq equations. J. Comput. Appl. Math. 2007, 207, 18–23. [Google Scholar] [CrossRef]
- Wazwaz, A.M. New travelling wave solutions to the Boussinesq and the Klein–Gordon equations. Commun. Nonlinear Sci. Numer. Simul. 2008, 13, 889–901. [Google Scholar] [CrossRef]
- Roeber, V.; Cheung, K.F. Boussinesq-type model for energetic breaking waves in fringing reef environments. Coast. Eng. 2012, 70, 1–20. [Google Scholar] [CrossRef]
- Shi, F.; Kirby, J.T.; Harris, J.C.; Geiman, J.D.; Grilli, S.T. A high-order adaptive time-stepping TVD solver for Boussinesq modeling of breaking waves and coastal inundation. Ocean Model. 2012, 43–44, 36–51. [Google Scholar] [CrossRef]
- Wazwaz, A.M. A Variety of Soliton Solutions for the Boussinesq–Burgers Equation and the Higher-Order Boussinesq–Burgers Equation. Filomat 2017, 31, 831–840. [Google Scholar] [CrossRef]
- Helal, M.; Seadawy, A.; Zekry, M. Stability analysis of solitary wave solutions for the fourth-order nonlinear Boussinesq water wave equation. Appl. Math. Comput. 2014, 232, 1094–1103. [Google Scholar] [CrossRef]
- Kazolea, M.; Delis, A.I. Irregular wave propagation with a 2DH Boussinesq-type model and an unstructured finite volume scheme. Eur. J. Mech. B Fluids 2018, 72, 432–448. [Google Scholar] [CrossRef]
- Vucheva, V.; Kolkovska, N. High order symplectic finite difference scheme for double dispersion equations. AIP Conf. Proc. 2021, 2321, 030037. [Google Scholar] [CrossRef]
- Yan, X.J.; Machado, J.A.T.; Baleanu, D. Exact Traveling-Wave Solution for Local Fractional Boussinesq Equation in Fractal Domain. Fractals 2017, 25, 1740006. [Google Scholar] [CrossRef]
- Danchin, R.; Paicu, M. Global Existence Results for the Anisotropic Boussinesq System in Dimension Two. Math. Model. Methods Appl. Sci. 2011, 21, 421–457. [Google Scholar] [CrossRef]
- Gastine, T.; Wicht, J.; Aurnou, J.M. Turbulent Rayleigh–Bénard convection in spherical shells. J. Fluid Mech. 2015, 778, 721–764. [Google Scholar] [CrossRef]
- Animasaun, I.L. Double diffusive unsteady convective micropolar flow past a vertical porous plate moving through binary mixture using modified Boussinesq approximation. Ain Shams Eng. J. 2016, 7, 755–765. [Google Scholar] [CrossRef]
- Weiss, S.; He, X.; Ahlers, G.; Bodenschatz, E.; Shishkina, O. Bulk temperature and heat transport in turbulent Rayleigh–Bénard convection of fluids with temperature-dependent properties. J. Fluid Mech. 2018, 851, 374–390. [Google Scholar] [CrossRef]
- Xi, H.D.; Zhou, Q.; Xia, K.Q. Azimuthal motion of the mean wind in turbulent thermal convection. Phys. Rev. E 2006, 73, 056312. [Google Scholar] [CrossRef]
- Lappa, M.; Gradinscak, T. On the oscillatory modes of compressible thermal convection in inclined differentially heated cavities. Int. J. Heat Mass Transf. 2018, 121, 412–436. [Google Scholar] [CrossRef]
- Ahlers, G.; He, X.; Funfschilling, D.; Bodenschatz, E. Heat transport by turbulent Rayleigh–Bénard convection for Pr≃0.8 and 3·1012≲Ra≲1015: Aspect ratio Γ = 0.50. New J. Phys. 2012, 14, 103012. [Google Scholar] [CrossRef]
- Ahlers, G.; Bodenschatz, E.; He, X. Logarithmic temperature profiles of turbulent Rayleigh–Bénard convection in the classical and ultimate state for a Prandtl number of 0.8. J. Fluid Mech. 2014, 758, 436–467. [Google Scholar] [CrossRef][Green Version]
- Parodi, A.; Emanuel, K.A.; Provenzale, A. Plume patterns in radiative–convective flows. New J. Phys. 2003, 5, 106. [Google Scholar] [CrossRef]
- Cavaleri, L.; Alves, J.H.; Ardhuin, F.; Babanin, A.; Banner, M.; Belibassakis, K.; Benoit, M.; Donelan, M.; Groeneweg, J.; Herbers, T.; et al. Wave modelling – The state of the art. Prog. Oceanogr. 2007, 75, 603–674. [Google Scholar] [CrossRef]
- Li, T.H. An analysis to a model of tornado. Z. Angew. Math. Phys. 2021, 73, 17. [Google Scholar] [CrossRef]
- Xiao-Jun, Y.; Baleanu, D.; Khan, Y.; Mohyud-Din, S.T. Local Fractional Variational Iteration Method for Diffusion and Wave Equations on Cantor Sets. Rom. J. Phys. 2014, 59, 36–48. [Google Scholar]
- Abu-Irwaq, I.; Alquran, M.; Jaradat, I.; Noorani, M.; Momani, S.; Baleanu, D. Numerical investigations on the physical dynamics of the coupled fractional boussinesq-burgers system. Rom. J. Phys. 2020, 65, 111. [Google Scholar]
- Sedov, L.I. Similarity and Dimensional Methods in Mechanics; CRC Press: Boca Raton, FL, USA, 1993. [Google Scholar]
- Zel’dovich, Y.B.; Raizer, Y.P. Physics of Shock Waves and High Temperature Hydrodynamic Phenomena; Academic Press: New York, NY, USA, 1966. [Google Scholar]
- Bluman, G.W.; Cole, J.D. The General Similarity Solution of the Heat Equation. J. Math. Mech. 1969, 18, 1025–1042. [Google Scholar]
- Barna, I.F. Self-Similar Solutions of Three-Dimensional Navier–Stokes Equation. Commun. Theor. Phys. 2011, 56, 745. [Google Scholar] [CrossRef]
- Barna, I.F.; Mátyás, L. Analytic solutions for the three-dimensional compressible Navier–Stokes equation. Fluid Dyn. Res. 2014, 46, 055508. [Google Scholar] [CrossRef]
- Barna, I.F.; Mátyás, L. Analytic self-similar solutions of the Oberbeck–Boussinesq equations. Chaos Solitons Fractals 2015, 78, 249–255. [Google Scholar] [CrossRef]
- Barna, I.F.; Mátyás, L.; Pocsai, M.A. Self-similar analysis of a viscous heated Oberbeck–Boussinesq flow system. Fluid Dyn. Res. 2020, 52, 015515. [Google Scholar] [CrossRef]
- Campos, D. (Ed.) Handbook on Navier–Stokes Equations; Nova Publishers: New York, NY, USA, 2017; Chapter 16; pp. 275–304. [Google Scholar]
- Barna, I.F.; Mátyás, L. Analytic Solutions of the Rotating and Stratified Hydrodynamical Equations. Asian J. Res. Rev. Phys. 2021, 4, 1426. [Google Scholar] [CrossRef]
- Barna, I.F.; Mátyás, L. Analytic solutions of a two-fluid hydrodynamic model. Math. Model. Anal. 2021, 26, 582–590. [Google Scholar] [CrossRef]
- Barna, I.F.; Bognár, G.; Guedda, M.; Hriczó, K.; Mátyás, L. Analytic Self-Similar Solutions of the Kardar–Parisi–Zhang Interface Growing Equation with Various Noise Terms. Math. Model. Anal. 2020, 25, 241–256. [Google Scholar] [CrossRef]
- Barna, I.F.; Bognár, G.; Mátyás, L.; Guedda, M.; Hriczó, K. Travelling-wave solutions of the Kardar–Parisi–Zhang interface growing equation with different kind of noise terms. AIP Conf. Proc. 2020, 2293, 280005. [Google Scholar] [CrossRef]
- Provis, D.G.; Radok, R. (Eds.) Waves on Water of Variable Depth; Lecture Notes in Physics; Springer: Berlin/Heidelberg, Germany, 1977; Volume 64. [Google Scholar]
- Staziker, D.J.; Porter, D.; Stirling, D.S.G. The scattering of surface waves by local bed elevations. Appl. Ocean. Res. 1996, 18, 283–291. [Google Scholar] [CrossRef]
- Porter, R.; Porter, D. Water wave scattering by a step of arbitrary profile. J. Fluid Mech. 2000, 411, 131–164. [Google Scholar] [CrossRef]
- Liggett, J.A.; Liu, P.L.F. Basic Principles and Applications; Topics in Boundary Element Research, Chapter Applications of Boundary Element Methods to Fluid Mechanics; Springer: Berlin/Heidelberg, Germany, 1984; Volume 1, pp. 78–96. [Google Scholar]
- Wang, C.D.; Meylan, M.H. The linear wave response of a floating thin plate on water of variable depth. Appl. Ocean. Res. 2002, 24, 163–174. [Google Scholar] [CrossRef]
- Chowdhury, D.; Debsarma, S. Nonlinear Evolution Equations of Co-propagating Waves over Finite Depth Fluid. Water Waves 2019, 1, 259–273. [Google Scholar] [CrossRef]
- Manafian, J.; Mohammed, S.A.; Alizadeh, A.; Baskonus, H.M.; Gao, W. Investigating lump and its interaction for the third-order evolution equation arising propagation of long waves over shallow water. Eur. J. Mech. B/Fluids 2020, 84, 289–301. [Google Scholar] [CrossRef]
- Ilhan, O.A.; Manafian, J.; Baskonus, H.M.; Lakestani, M. Solitary wave solitons to one model in the shallow water waves. Eur. Phys. J. Plus 2021, 136, 337. [Google Scholar] [CrossRef]
- Lakestani, M.; Manafian, J. Application of the ITEM for the modified dispersive water-wave system. Opt. Quantum Electron. 2017, 49, 128. [Google Scholar] [CrossRef]
- Shen, G.; Manafian, J.; Huy, D.T.N.; Nisar, K.S.; Abotaleb, M.; Trung, N.D. Abundant soliton wave solutions and the linear superposition principle for generalized (3+1)-D nonlinear wave equation in liquid with gas bubbles by bilinear analysis. Results Phys. 2022, 32, 105066. [Google Scholar] [CrossRef]
- Qian, Y.; Manafian, J.; Mohyaldeen, S.Y.; Esmail, L.S.; Gorovoy, S.A.; Singh, G. Multiple-order line rogue wave, lump and its interaction, periodic, and cross-kink solutions for the generalized CHKP equation. Propuls. Power Res. 2021, 10, 277–293. [Google Scholar] [CrossRef]
- Khakimzyanov, G.; Dutykh, D.; Fedotova, Z.; Gusev, O. Dispersive Shallow Water Waves; Lecture Notes in Geosystems Mathematics and Computing; Springer: Berlin/Heidelberg, Germany, 2020. [Google Scholar]
- Barna, I.F.; Kersner, R. Heat conduction: A telegraph-type model with self-similar behavior of solutions. J. Phys. A Math. Theor. 2010, 2010. 43, 375210. [Google Scholar] [CrossRef]
- Olver, F.W.J.; Lozier, D.W.; Boisvert, R.F.; Clark, C.W. (Eds.) NIST Handbook of Mathematical Functions; Cambridge University Press: Cambridge, UK, 2010. [Google Scholar]
- Trichtchenko, O.; Deconinck, B.; Wilkening, J. The instability of Wilton ripples. Wave Motion 2016, 66, 147–155. [Google Scholar] [CrossRef]
- Paldor, N. Shallow Water Waves on the Rotating Earth; SpringerBriefs in Earth System Sciences; Springer: Berlin/Heidelberg, Germany, 2015. [Google Scholar]
- Bognár, G.; Hriczó, K. Ferrofluid Flow in Magnetic Field Above Stretching Sheet with Suction and Injection. Math. Model. Anal. 2020, 25, 461–472. [Google Scholar] [CrossRef]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Barna, I.F.; Pocsai, M.A.; Mátyás, L. Time-Dependent Analytic Solutions for Water Waves above Sea of Varying Depths. Mathematics 2022, 10, 2311. https://doi.org/10.3390/math10132311
Barna IF, Pocsai MA, Mátyás L. Time-Dependent Analytic Solutions for Water Waves above Sea of Varying Depths. Mathematics. 2022; 10(13):2311. https://doi.org/10.3390/math10132311
Chicago/Turabian StyleBarna, Imre Ferenc, Mihály András Pocsai, and László Mátyás. 2022. "Time-Dependent Analytic Solutions for Water Waves above Sea of Varying Depths" Mathematics 10, no. 13: 2311. https://doi.org/10.3390/math10132311
APA StyleBarna, I. F., Pocsai, M. A., & Mátyás, L. (2022). Time-Dependent Analytic Solutions for Water Waves above Sea of Varying Depths. Mathematics, 10(13), 2311. https://doi.org/10.3390/math10132311






