Novel Recurrence Relations for Volumes and Surfaces of n-Balls, Regular n-Simplices, and n-Orthoplices in Real Dimensions

Abstract

This study examines n-balls, n-simplices, and n-orthoplices in real dimensions using novel recurrence relations that remove the indefiniteness present in known formulas. They show that in the negative, integer dimensions, the volumes of n-balls are zero if n is even, positive if n = −4k − 1, and negative if n = −4k − 3, for natural k. The volumes and surfaces of n-cubes inscribed in n-balls in negative dimensions are complex, wherein for negative, integer dimensions they are associated with integral powers of the imaginary unit. The relations are continuous for n ∈ ℝ and show that the constant of π is absent for 0 ≤ n < 2. For n < −1, self-dual n-simplices are undefined in the negative, integer dimensions, and their volumes and surfaces are imaginary in the negative, fractional ones and divergent with decreasing n. In the negative, integer dimensions, n-orthoplices reduce to the empty set, and their real volumes and imaginary surfaces are divergent in negative, fractional ones with decreasing n. Out of three regular, convex polytopes present in all natural dimensions, only n-orthoplices and n-cubes (and n-balls) are defined in the negative, integer dimensions.

1. Introduction

The notion of dimension n of a set has various definitions [1,2]. Natural dimensions define a minimum number of independent parameters (coordinates) needed to specify a point within Euclidean space ℝⁿ, where n = −1 is the dimension of the empty set, the void, having zero volume and undefined surface. Negatively dimensional spaces can be defined by analytic continuations from positive dimensions [3]. A spectrum, a topological generalization of the notion of space, allows for negative dimensions [2,4,5,6] that refer to densities, rather than to sizes as in the natural ones.

Fractional (or fractal) dimensions extend the notion of dimension to real, including negative [7], numbers. Negative dimensions are considered in probabilistic fractal measures [8]. Fractal dimension and lacunarity [9,10] allow for an investigation of the fractal nature of prime sequences [11]. Fractal dimensions have been verified to be consistent with experimental observations and allow for the analysis of the transport properties, such as permeability, thermal dispersion, and conductivities (both thermal and electrical) in multiphase fractal media [12]. The probability models for pore distribution and for permeability of porous media can also be expressed as a function of fractal dimensions [13]. Interestingly, the dimension of the boundary of the Mandelbrot set equals 2 [14], and the generalized Mandelbrot set in higher-dimensional hypercomplex number spaces, when the power α of the iterated complex variable z tends to infinity, is convergent to the unit (α − 1)-sphere [15].

Complex dimensions can also be considered [2]. Furthermore, geometric concepts (such as lengths, volumes, and surfaces) can be related to negative, fractional, and complex numbers. Complex geodesic paths emerge in the presence of black hole singularities [16] and when studying entropic dynamics on curved statistical manifolds [17]. Fractional derivatives of complex functions could be able to describe different physical phenomena [18].

In ℝ², there is a countably infinite number of regular, convex polygons; in ℝ³, there are five regular, convex Platonic solids; in ℝ⁴, there are six regular, convex polytopes. For n > 4, there are only three: self-dual n-simplex and n-cube dual to n-orthoplex [19]. Furthermore, ℝⁿ is also equipped with a perfectly regular, convex n-ball. The properties of these three regular, convex polytopes in natural dimensions are well known [20,21,22]. Fractal dimensions of hyperfractals based on these polytopes in natural dimensions were disclosed in [23].

This study examines n-balls, regular n-simplices, and n-orthoplices in real dimensions using novel recurrence relations that remove the indefiniteness present in known formulas.

The paper is structured as follows. Section 2 presents known formulas for volumes and surfaces of n-balls, regular n-simplices, and n-orthoplices in natural dimensions. Section 3 defines novel recurrence relations for these geometric objects in real dimensions and presents their algebraic forms in integer dimensions. Section 4 refers to n-balls circumscribed about and inscribed in n-cubes in real dimensions. Section 5 summarizes the findings of this paper. Their possible applications are discussed in Section 6.

2. Known Formulas

The volume of an n-ball (B) is known to be

$$V_n{\left(R\right)}_B=\frac{{\pi }^{n/2}}{\mathsf{\Gamma }\left(n/2+1\right)}{R}^n$$

(1)

where Γ is the Euler’s gamma function and R is the n-ball radius. This becomes

$$V_{2k}{\left(R\right)}_B=\frac{{\pi }^k{R}^{2k}}{k!}$$

(2)

if n is even (n = 2k, k ∈ ℕ₀) and

$$V_{2k-1}{\left(R\right)}_B=\frac{2^{2k}{\pi }^{k-1}k!}{\left(2k\right)!}{R}^{2k-1}$$

(3)

if n is odd (n = 2k − 1, k ∈ ℕ). Expressed in terms of n-ball diameter (1) is the rescaling factor between the n-dimensional Lebesgue measure and Hausdorff measure for n ∈ ℝ⁺ [2,24].

Another known [21] recurrence relation expresses the volume of an n-ball in terms of the volume of an (n − 2)-ball of the same radius

$$V_n{\left(R\right)}_B=\frac{2\pi R^2}{n}{V}_{n-2}{\left(R\right)}_B$$

(4)

where V₀(R)_B = 1 and V₁(R)_B = 2R. It is also known [21] that the (n − 1)-dimensional surface of an n-ball can be expressed as

$$S_n{\left(R\right)}_B=\frac{n}{R}{V}_n{\left(R\right)}_B$$

(5)

Furthermore, it is known [25] that the sequence

$$f_n=\frac{2\pi }{n}{f}_{n-2}$$

(6)

satisfies the same recursion formula as (4) for unit radius.

The volume of a regular n-simplex (S) is known [20,26] to be

$$V_n{\left(A\right)}_S=\frac{\sqrt{n+1}}{n!\sqrt{2^n}}{A}^n$$

(7)

where A is the edge length. A regular n-simplex has n + 1 (n − 1)-facets [21], so its surface is

$$S_n{\left(A\right)}_S=\left(n+1\right)V_{n-1}{\left(A\right)}_S$$

(8)

The volume of n-orthoplex (O) is known [22] to be

$$V_n{\left(A\right)}_O=\frac{\sqrt{2^n}}{n!}{A}^n$$

(9)

As n-orthoplex has 2ⁿ facets [21], being regular (n − 1)-simplices, its surface is

$$S_n{\left(A\right)}_O=2^n{V}_{n-1}{\left(A\right)}_S$$

(10)

Formulas (1)–(3) and (7)–(10) are undefined in negative dimensions since the factorial is defined only for non-negative integers, while the gamma function is undefined for non-positive integers. Relations (4)–(6) are undefined if n = 0.

3. Novel Recurrence Relations

A radius recurrence relation

$$f_n\doteq \frac{2}{n}{f}_{n-2},$$

(11)

for n ∈ ℕ₀, where f₀ := 1 and f₁ := 2, allows for expressing the volumes and, using (5), surfaces of n-balls as

$$V_n{\left(R\right)}_B\doteq f_n{\pi }^{\lfloor n/2\rfloor }{R}^n,$$

(12)

$$S_n{\left(R\right)}_B\doteq n{f}_n{\pi }^{\lfloor n/2\rfloor }{R}^{n-1}=\frac{d}{dR}{V}_n{\left(R\right)}_B,$$

(13)

where “⌊x⌋” denotes the floor function giving the greatest integer less than or equal to its argument x.

Proof. 

If n = 2k for k ∈ ℕ₀, then by equating (2) with (12)

$$\frac{{\pi }^k{R}^{2k}}{k!}=f_{2k}{\pi }^k{R}^{2k}\iff f_{2k}=\frac{1}{k!}=\frac{1}{\left(n/2\right)!}.$$

(14)

Then, with (11), e.g., for k = 3

$$\begin{array}{l}{f}_6=\frac{2}{6}{f}_4,\hspace{0.17em}{f}_4=\frac{2}{4}{f}_2,\hspace{0.17em}{f}_2=\frac{2}{2}{f}_0\\ f_6=\frac{2}{6}\frac{2}{4}\frac{2}{2}1=\frac{2^3}{6!!}\iff \hspace{0.17em}{f}_{2k}=\frac{2^k}{\left(2k\right)!!}=\frac{2^k}{2^{k}k!}\end{array}.$$

For even n ≥ 0, n!! = 2^kk!

If n = 2k − 1, k ∈ ℕ, then by equating (3) with (12), we have

$$\begin{array}{l}\frac{2^{2k}{\pi }^{k-1}k!}{\left(2k\right)!}{R}^{2k-1}=f_{2k-1}{\pi }^{\lfloor \left(2k-1\right)/2\rfloor }{R}^{2k-1}\\ \frac{2^{2k}{\pi }^{k-1}k!}{\left(2k\right)!}=f_{2k-1}{\pi }^{k-1}\iff \\ f_{2k-1}=\frac{2^{2k}k!}{\left(2k\right)!}=\frac{2^{2k-1}\left(k-1\right)!}{\left(2k-1\right)!}=\frac{2^n\left(\frac{n-1}{2}\right)!}{n!}\end{array}$$

(15)

Then, with (11), e.g., for k = 4

$$\begin{array}{l}{f}_7=\frac{2}{7}{f}_5,\hspace{0.17em}{f}_5=\frac{2}{5}{f}_3,\hspace{0.17em}{f}_3=\frac{2}{3}{f}_1,\hspace{0.17em}{f}_1=\frac{2}{1}{f}_{-1}\\ f_7=\frac{2}{7}\frac{2}{5}\frac{2}{3}\frac{2}{1}1=\frac{2^4}{7!!}\iff f_{2k-1}=\frac{2^k}{\left(2k-1\right)!!}\end{array}.$$

For odd n ≥ 1, n!! = (2k − 1)!/(2^k−1(k − 1)!), which completes the proof. □

The sequence (11) allows for presenting an n-ball’s volume and surface recurrence relations (12), (13) as a product of the rational factor f_n or nf_n, the irrational factor π^⌊n/2⌋, and the metric (radius) factor Rⁿ or Rⁿ⁻¹. The relation (11) can be extended into negative dimensions as

$$f_n=\frac{n+2}{2}{f}_{n+2}$$

(16)

solving (11) for f_n−2 and assigning new n ∈ ℤ as old n − 2. Thus, it is sufficient to define f₋₁ := 1, f₀ := 1 (for the empty set and point dimension) to initiate (11) and (16).

The same assignment of new n ∈ ℤ as old n − 2 can be made in (4) solved for V_n−2(R)_B, yielding

$$V_n{\left(R\right)}_B=\frac{n+2}{2\pi R^2}{V}_{n+2}{\left(R\right)}_B$$

(17)

which enables us to avoid the indefiniteness of factorial and gamma function in negative dimensions present in Formulas (1)–(3) and removing the singularity present in relation (4).

If n ≤ −3 and odd

$$f_n=i^{n+1}\frac{2^{n+2}\left(-n-2\right)!}{\left(\frac{-n-3}{2}\right)!}$$

(18)

Proof. 

Set n = −2k − 1, k ∈ ℕ. Then, with (16), e.g., for k = 3

$$\begin{array}{l}{f}_{-7}=-\frac{5}{2}{f}_{-5},\hspace{0.17em}{f}_{-5}=-\frac{3}{2}{f}_{-3},\hspace{0.17em}{f}_{-3}=-\frac{1}{2}{f}_{-1}\\ f_{-7}={\left(-1\right)}^3\frac{5}{2}\frac{3}{2}\frac{1}{2}1={\left(-1\right)}^3\frac{5!!}{2^3}\iff \hspace{0.17em}\\ f_{-2k-1}=\frac{{\left(-1\right)}^k\left(2k-1\right)!!}{2^k}=\frac{{\left(-1\right)}^k\left(2k-1\right)!}{2^{2k-1}\left(k-1\right)!}\end{array}$$

Also

$$\begin{array}{l}{\left(-1\right)}^k={\left(-1\right)}^{\left(-n-1\right)/2}={\left[{\left(-1\right)}^{\frac{1}{2}}\right]}^{-n-1}=i^{-\left(n+1\right)}\\ =-i^{n+1}={\left(-1\right)}^{n+1}{i}^{n+1}=i^{n+1}\end{array}$$

since n is odd. □

The factorial can be expressed by the gamma function. Thus, for n = 2k, k ∈ ℕ, (14) becomes

$$f_{2k}=\frac{1}{\left(n/2\right)!}=\frac{1}{\mathsf{\Gamma }\left(n/2+1\right)}$$

(19)

while for n = 2k − 1, k ∈ ℕ, (15) becomes

$$f_{2k-1}=\frac{2^n}{\mathsf{\Gamma }\left(n+1\right)}\frac{n!\sqrt{\pi }}{2^n\left(n/2\right)!}=\frac{\sqrt{\pi }}{\mathsf{\Gamma }\left(n/2+1\right)}$$

(20)

the forms which are, similarly to the gamma function, defined for all complex numbers except the non-positive, even integers.

The radius recurrence relation f_n (16) is listed in

Table 1. Volumes and surfaces of n-balls for −11 ≤ n ≤ 9.

n	fn	gn	Vn (R = 1)B	Sn (R = 1)B	Vn (D = 1)B	Sn (D = 1)B
−11	−945/32	−60,480	−0.031	0.338	−62.909	1383.997
−9	105/16	3360	0.021	−0.193	10.980	−197.634
−7	−15/8	−240	−0.019	0.135	−2.464	34.494
−5	3/4	24	0.024	−0.121	0.774	−7.7404
−3	−1/2	−4	−0.051	0.152	−0.405	2.432
−1	1	2	0.318	−0.318	0.637	−1.273
0	1	1	1	0	1	0
1	2/1	1	2	2	1	2
2	1/1	1/4	3.142	6.283	0.785	3.142
3	4/3	1/6	4.189	12.566	0.524	3.142
4	½	1/32	4.935	19.739	0.308	2.467
5	8/15	1/60	5.264	26.319	0.164	1.645
6	1/6	1/384	5.168	31.006	0.081	0.969
7	16/105	1/840	4.725	33.073	0.037	0.517
8	1/24	1/6144	4.059	32.470	0.016	0.254
9	32/945	1/15,120	3.299	29.687	0.006	0.116

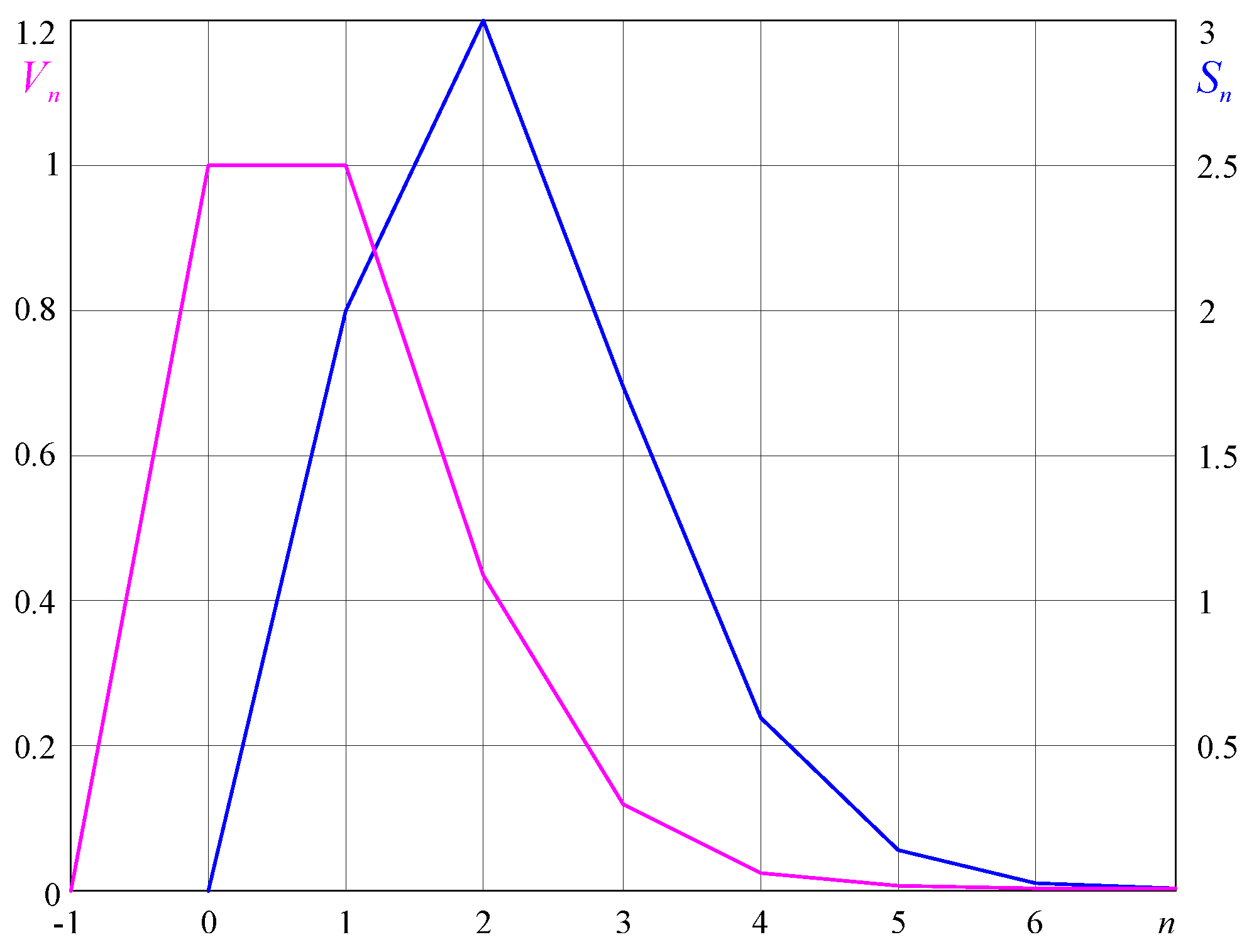
for n ∈ ℤ, and shown in Figure 1 along with the even algebraic form of f_n (19), odd algebraic form of f_n, (20) and the π^⌊n/2⌋ factor for n ∈ ℂ (for complex numbers ⌊a + bi⌋ = ⌊a⌋ + ⌊b⌋i). As shown, (19) and (20) bound the relation (16) for Re(n). Volumes and surfaces of n-balls calculated with (12) and (13) are shown in Figure 2.

Furthermore, for n ∈ ℤ

$$f_n{f}_{-n-2}=\mathrm{Re}\left(i^{n+1}\right)=\cos \left(\frac{\pi }{2}\left(n+1\right)\right)$$

(21)

where

$$\begin{array}{l}{i}^{n+1}=e^{i\pi \left(n+1\right)/2}\\ =\cos \left(\frac{\pi }{2}\left(n+1\right)\right)+i\sin \left(\frac{\pi }{2}\left(n+1\right)\right)\end{array}$$

(22)

Proof. 

If n = 2k, then

$$f_n{f}_{-n-2}=f_{2k}{f}_{-2k-2}=0=\mathrm{Re}\left(i^{n+1}\right)$$

since f_n = 0 for negative, even n.

If n = 2k − 1 then, using (15) and (18)

$$\begin{array}{l}{f}_n{f}_{-n-2}=f_{2k-1}{f}_{-2k-1}\\ =\frac{2^{2k-1}\left(k-1\right)!}{\left(2k-1\right)!}{\left(-1\right)}^k\frac{\left(2k-1\right)!}{2^{2k-1}\left(k-1\right)!}\\ ={\left(-1\right)}^k={\left(-1\right)}^{\left(n+1\right)/2}=i^{n+1}=\mathrm{Re}\left(i^{n+1}\right)\end{array}$$

since n is odd. □

Furthermore, for n ∈ ℝ, k ∈ ℤ

$${\pi }^{\lfloor n/2\rfloor }{\pi }^{\lfloor \left(-n-2\right)/2\rfloor }=\{\begin{array}{cc}{\pi }^{-1}& n=2k\\ {\pi }^{-2}& n\ne 2k\end{array}$$

(23)

Proof. 

If n = 2k, then π^kπ^−k−1 = π⁻¹. Otherwise, set n = 2k ± ε, where 0 < ε ≤ 1, ε ∈ ℝ. For n = 2k + ε

$${\pi }^{\lfloor k+\epsilon /2\rfloor }{\pi }^{\lfloor -k-\epsilon /2-1\rfloor }={\pi }^k{\pi }^{-k-2}={\pi }^{-2}$$

while for n = 2k − ε

$${\pi }^{\lfloor k-\epsilon /2\rfloor }{\pi }^{\lfloor -k+\epsilon /2-1\rfloor }={\pi }^{k-1}{\pi }^{-k-1}={\pi }^{-2}$$

□

Furthermore, the following holds for n-balls surfaces (13)

$$S_n{}_B{S}_{\left(2-n\right)B}=n\left(2-n\right)f_n{f}_{2-n}=4\mathrm{Re}\left(i^{n-1}\right)$$

(24)

for n ∈ ℤ, where

$$\begin{array}{l}{i}^{n-1}=e^{i\pi \left(n-1\right)/2}\\ =\cos \left(\frac{\pi }{2}\left(n-1\right)\right)+i\sin \left(\frac{\pi }{2}\left(n-1\right)\right)=-i^{n+1}\end{array}$$

(25)

Proof. 

If n = 2k then

$$\begin{array}{l}{S}_{\left(2k\right)}{}_B{S}_{\left(2-2k\right)B}\\ =2k{f}_{2k}{\pi }^k{R}^{2k-1}\left(2-2k\right)f_{2-2k}{\pi }^{1-k}{R}^{1-2k}\\ =4k\left(1-k\right)f_{2k}{f}_{2-2k}\pi =0=4\mathrm{Re}\left(i^{n-1}\right)\end{array}$$

for k = {0, 1} and for the remaining k’s, as f_−2k = 0 for k ∈ ℕ. Also Re(iⁿ⁻¹) = 0 and Im(iⁿ⁻¹) = ±1, as n is even.

If n = 2k − 1 then

$$\begin{array}{l}{S}_{\left(2k-1\right)}{}_B{S}_{\left(3-2k\right)B}\\ =\left(2k-1\right)\left(3-2k\right)f_{2k-1}{f}_{3-2k}{\pi }^{\lfloor \left(2k-1\right)/2\rfloor }{\pi }^{\lfloor \left(3-2k\right)/2\rfloor }\\ =\left(2k-1\right)\left(3-2k\right)f_{2k-1}{f}_{3-2k}{\pi }^{k-1}{\pi }^{-k+1}\\ =\left(2k-1\right)\left(3-2k\right)f_{2k-1}{f}_{3-2k}\end{array}.$$

For k = 1, using (15)

$$S_{\left(1\right)}{}_B{S}_{\left(1\right)B}=f_1^2=4=4\mathrm{Re}\left(i^0\right)$$

wherein for the remaining k’s, we shall use both (15) and (18) (and f₋₁ = 1). For instance, for k = {0, 2}

$$S_{\left(3\right)B}{S}_{\left(-1\right)}{}_B=3\left(-1\right)f_3{f}_{-1}=-3\frac{4}{3}1=-4=4\mathrm{Re}\left(i^2\right),$$

and further, for k ≤ −1 or k ≥ 3

$$\begin{array}{l}{S}_{\left(2k-1\right)}{}_B{S}_{\left(3-2k\right)B}\\ =\left(2k-1\right)\left(3-2k\right)\frac{2^{2k-1}\left(k-1\right)!}{\left(2k-1\right)!}\frac{{\left(-1\right)}^{k-2}\left(2k-5\right)!}{2^{2k-5}\left(k-3\right)!}\\ =\left(2k-1\right)\left(3-2k\right){\left(-1\right)}^{k-2}{2}^4\frac{\left(k-1\right)!\left(2k-5\right)!}{\left(2k-1\right)!\left(k-3\right)!}\\ =4{\left(-1\right)}^{k-1}=4i^{n-1}=4\mathrm{Re}\left(i^{n-1}\right)\end{array},$$

since n is odd and thus, n − 1 is even. □

Furthermore, for n ∈ ℝ, k ∈ ℤ

$${\pi }^{\lfloor n/2\rfloor }{\pi }^{\lfloor \left(2-n\right)/2\rfloor }=\{\begin{array}{cc}\pi & n=2k\\ 1& n\ne 2k\end{array}$$

(26)

Proof. 

If n = 2k, then π^kπ^1−k = π. Otherwise, set n = 2k ± ε, where 0 < ε ≤ 1, ε ∈ ℝ. For n = 2k + ε

$${\pi }^{\lfloor k+\epsilon /2\rfloor }{\pi }^{\lfloor 1-k-\epsilon /2\rfloor }={\pi }^k{\pi }^{-k}={\pi }^0=1$$

while for n = 2k − ε

$${\pi }^{\lfloor k-\epsilon /2\rfloor }{\pi }^{\lfloor 1-k+\epsilon /2\rfloor }={\pi }^{k-1}{\pi }^{-k+1}={\pi }^0=1$$

□

Moreover, the following holds for n-ball volumes (12)

$$\frac{n\pi }{2}{V}_n{}_B{V}_{\left(-n\right)B}=\mathrm{Re}\left(i^{n-1}\right)$$

(27)

for n ∈ ℤ.

Proof. 

If n = 2k, k ∈ ℕ, then

$$\begin{array}{l}{V}_{\left(2k\right)}{}_B{V}_{\left(-2k\right)B}=f_{2k}{\pi }^k{R}^{2k}{f}_{-2k}{\pi }^{-k}{R}^{-2k}\\ =f_{2k}{f}_{-2k}=0=\frac{2}{2k\pi }\mathrm{Re}\left(i^{2k-1}\right)\end{array}$$

If n = 2k − 1, k ∈ ℕ, then

$$V_{\left(2k-1\right)}{}_B{V}_{\left(1-2k\right)B}=f_{2k-1}{\pi }^{k-1}{f}_{1-2k}{\pi }^{-k}=f_{2k-1}{f}_{1-2k}{\pi }^{-1}.$$

For k = 1, using (15) and f₋₁ = 1

$$V_{\left(1\right)}{}_B{V}_{\left(-1\right)B}=f_1{f}_{-1}{\pi }^{-1}=\frac{2}{1\pi }=\frac{2}{1\pi }\mathrm{Re}\left(i^0\right).$$

For the remaining k’s, we shall use both (15) and (18)

$$\begin{array}{l}{V}_{\left(2k-1\right)}{}_B{V}_{\left(-2k+1\right)B}=f_{2k-1}{f}_{1-2k}{\pi }^{-1}=\\ \frac{2^{2k-1}\left(k-1\right)!}{\left(2k-1\right)!}\frac{{\left(-1\right)}^{k-1}\left(2k-3\right)!}{2^{2k-3}\left(k-2\right)!}{\pi }^{-1}\\ ={\left(-1\right)}^{k-1}\frac{2}{n\pi }=\frac{2}{n\pi }{i}^{n-1}=\frac{2}{n\pi }\mathrm{Re}\left(i^{n-1}\right)\end{array},$$

since n is odd and, thus n − 1 is even. □

Furthermore, for n ∈ ℝ, k ∈ ℤ

$${\pi }^{\lfloor n/2\rfloor }{\pi }^{\lfloor -n/2\rfloor }=\{\begin{array}{cc}1& n=2k\\ {\pi }^{-1}& n\ne 2k\end{array}$$

(28)

Proof. 

If n = 2k, then π^kπ^−k = 1. Otherwise, set n = 2k ± ε, where 0 < ε ≤ 1, ε ∈ ℝ. For n = 2k + ε

$${\pi }^{\lfloor k+\epsilon /2\rfloor }{\pi }^{\lfloor -k-\epsilon /2\rfloor }={\pi }^k{\pi }^{-k-1}={\pi }^{-1}$$

while for n = 2k − ε

$${\pi }^{\lfloor k-\epsilon /2\rfloor }{\pi }^{\lfloor -k+\epsilon /2\rfloor }={\pi }^{k-1}{\pi }^{-k}={\pi }^{-1}$$

□

One can also express the volumes and, using (5), surfaces of n-balls in terms of their diameters D as

$$V_n{\left(D\right)}_B\doteq g_n{\pi }^{\lfloor n/2\rfloor }{D}^n$$

(29)

$$S_n{\left(D\right)}_B\doteq 2n{g}_n{\pi }^{\lfloor n/2\rfloor }{D}^{n-1}=2\frac{d}{dD}{V}_n{\left(D\right)}_B$$

(30)

defining diameter recurrence relation

$$g_n\doteq \frac{1}{2n}{g}_{n-2}$$

(31)

having inverse

$$g_n=2\left(n+2\right)g_{n+2}$$

(32)

for n ∈ ℤ, where g₋₁ := 2 and g₀ := 1. The diameter recurrence relation (31), (32) is related to radius recurrence relation (11), (16) by

$$f_n=2^n{g}_n$$

(33)

Proof. 

By equating (12) with (29), we have

$$f_n{\pi }^{\lfloor n/2\rfloor }{R}^n=g_n{\pi }^{\lfloor n/2\rfloor }{2}^n{R}^n$$

which completes the proof. □

Furthermore (proof follows from (21) and (33))

$$g_n{g}_{-n-2}=4\mathrm{Re}\left(i^{n+1}\right)$$

(34)

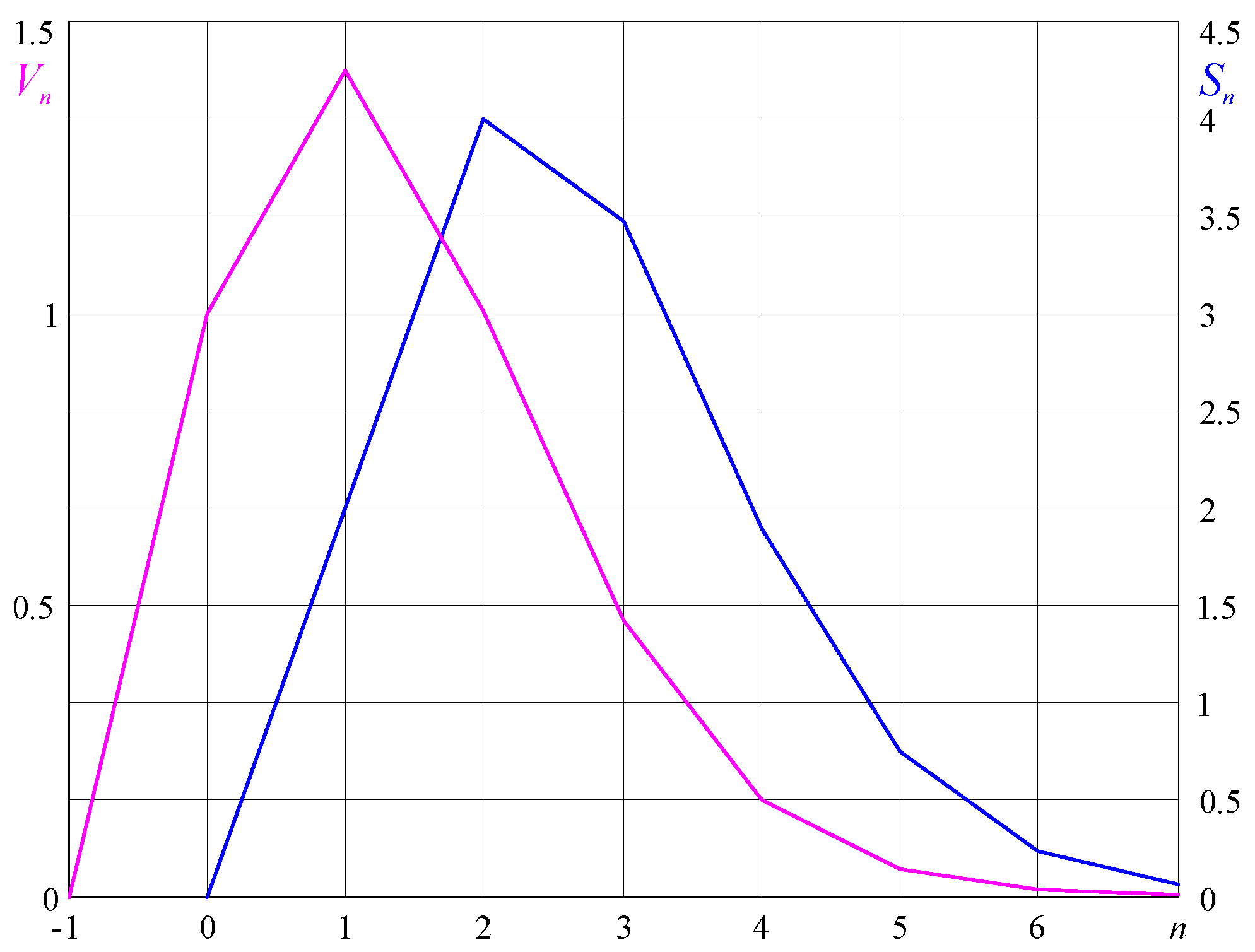
The diameter recurrence relation g_n (32) is listed in Table 1 for n ∈ ℤ, and shown in Figure 3 along with the even algebraic form of g_n ((19) with (33)) the odd algebraic form of g_n ((20) with (33)), and the π^⌊n/2⌋ factor for n ∈ ℂ. Volumes and surfaces of n-balls calculated with relations (29) and (30) are shown in Figure 4.

In the case of regular n-simplices, Equation (7) can be written as a recurrence relation, with V₀(A)_S := 1

$$V_n{\left(A\right)}_S\doteq A{V}_{n-1}{\left(A\right)}_S\sqrt{\frac{n+1}{2n^3}}$$

(35)

Proof. 

By equating (7) with (35), we have

$$\begin{array}{l}\frac{\sqrt{n+1}}{n!2^{n/2}}{A}^n=A{V}_{n-1}{\left(A\right)}_S\frac{\sqrt{n+1}}{2^{1/2}{n}^{3/2}}\\ V_{n-1}{\left(A\right)}_S=\frac{2^{\left(1-n\right)/2}{n}^{3/2}}{n!}{A}^{n-1}\\ V_n{\left(A\right)}_S=\frac{{\left(n+1\right)}^{3/2}}{2^{n/2}\left(n+1\right)!}{A}^n=\frac{\sqrt{n+1}}{n!\sqrt{2^n}}{A}^n\end{array}$$

which recovers (7) and completes the proof. □

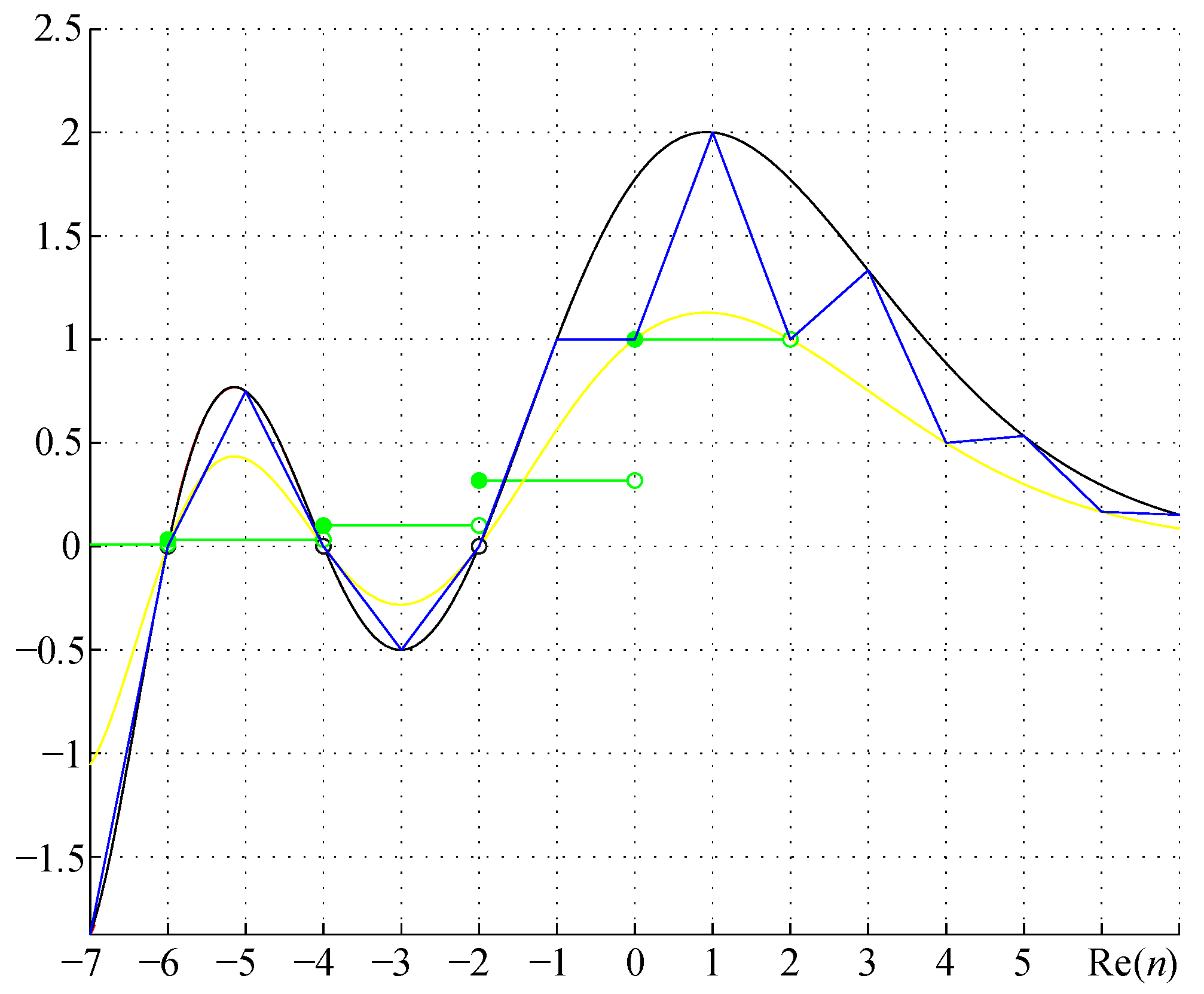
The relation (35) removes the indefiniteness of the factorial for n < 0 and singularity for n = −1 present in (7). Solving (35) for V_n−1 and assigning new n ∈ ℤ as old n − 1, yields

$$V_n{\left(A\right)}_S=\frac{V_{n+1}\left(A\right)}{A}\sqrt{\frac{2{\left(n+1\right)}^3}{n+2}}$$

(36)

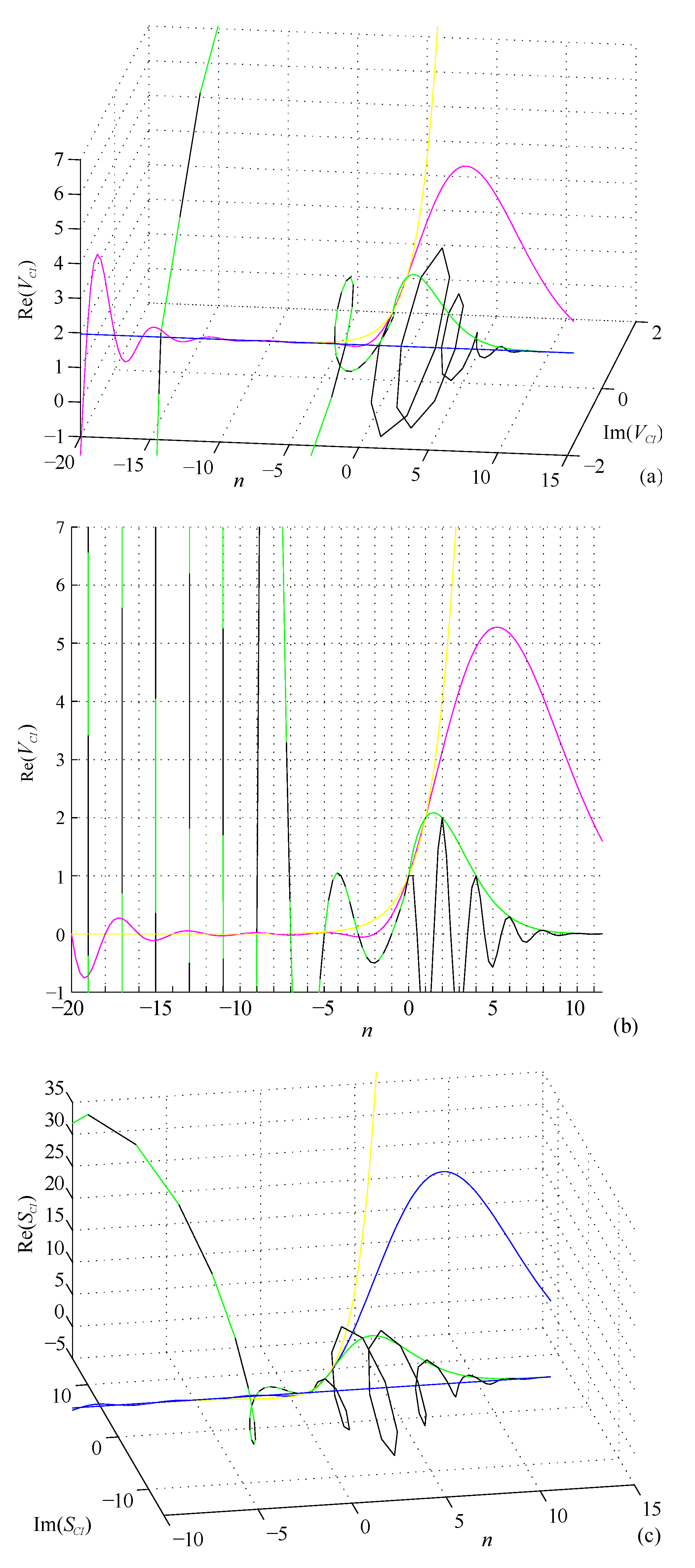
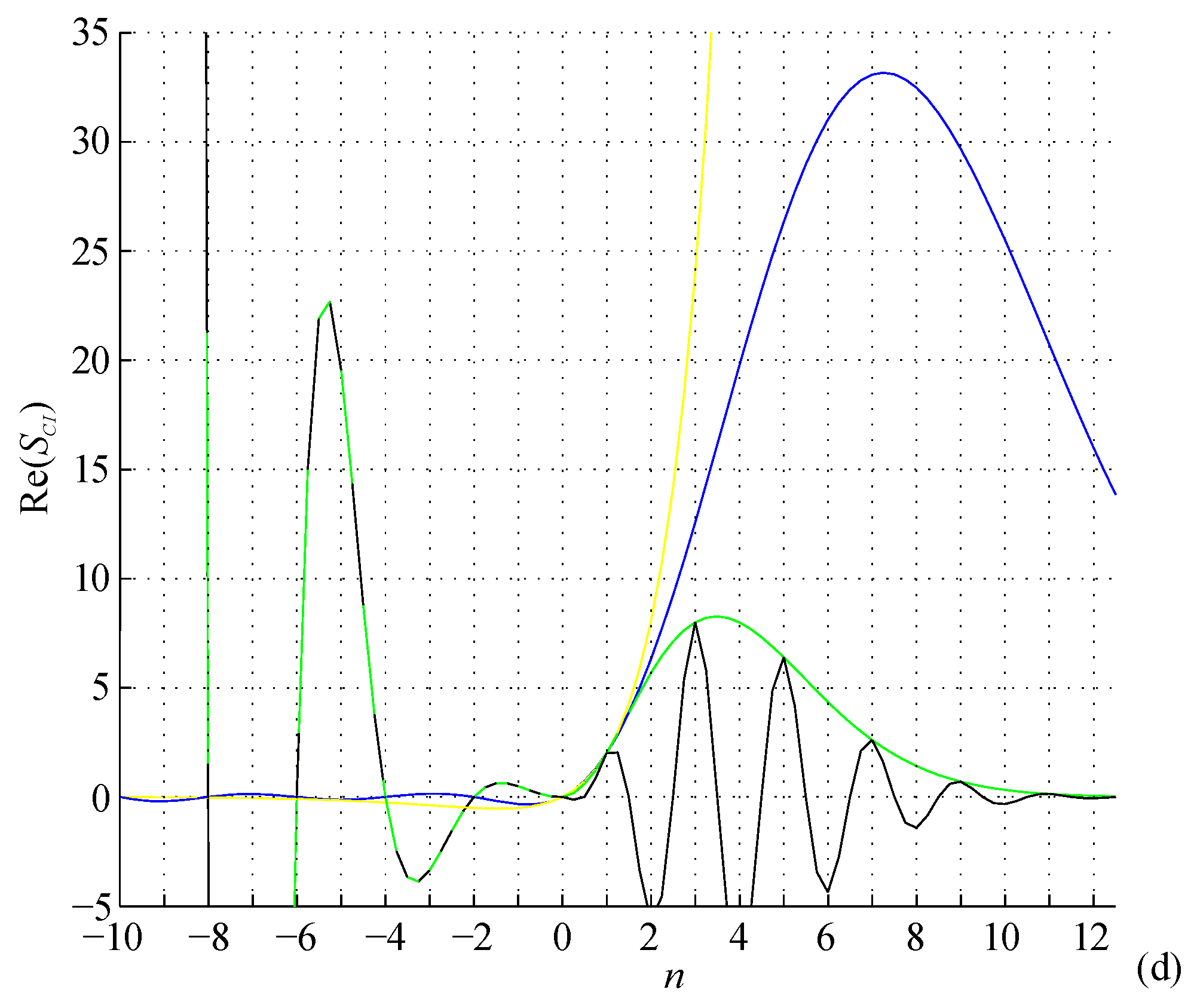
which shows that n-simplices are indefinite only for integer n < −1, as shown in Figure 5. The volume of an empty or void (−1)-simplex is V₋₁(A)_S = 0, while its surface S₋₁(A)_S (8) is undefined, as for the void itself.

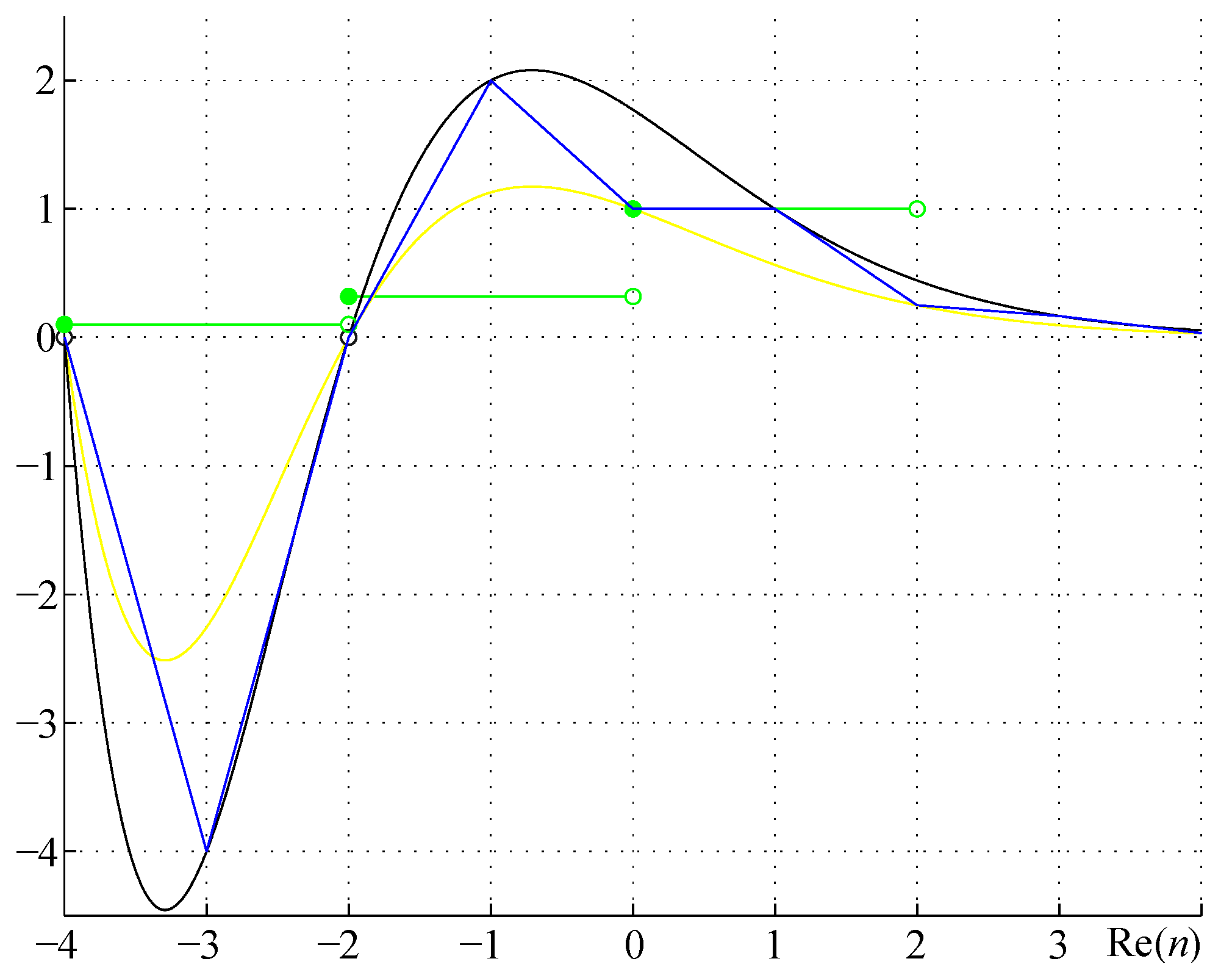
In the case of n-orthoplices, Equation (9) can be written as a recurrence relation

$$V_n{\left(A\right)}_O\doteq A{V}_{n-1}{\left(A\right)}_O\frac{\sqrt{2}}{n}$$

(37)

with V₀(A)_O := 1.

Proof. 

By equating (9) with (37), we have

$$\begin{array}{l}\frac{\sqrt{2^n}}{n!}{A}^n=A{V}_{n-1}{\left(A\right)}_O\frac{\sqrt{2}}{n}\\ V_{n-1}{\left(A\right)}_O=\frac{n}{n!}{A}^{n-1}{2}^{\left(n-1\right)/2}\\ V_n{\left(A\right)}_O=\frac{n+1}{\left(n+1\right)!}{A}^n{2}^{n/2}=\frac{\sqrt{2^n}}{n!}{A}^n\end{array}$$

which recovers (9) and completes the proof. □

The relation (37) removes the indefiniteness of the factorial for n < 0 present in (9). Solving (37) for V_n−1 and assigning new n ∈ ℤ as old n − 1, yields

$$V_n{\left(A\right)}_O=V_{n+1}{\left(A\right)}_O\frac{n+1}{A\sqrt{2}}$$

(38)

which removes singularity from (37) and is zero for integer n ≤ −1, showing that for negative, integer dimensions, the volumes of n-orthoplices are zero, while their surfaces (10) are undefined, as shown in Figure 6.

4. n-Balls Circumscribed about and Inscribed in n-Cubes

The edge length A_CC of an n-cube circumscribed (CC) about an n-ball corresponds to the diameter D of this n-ball. Thus, the volume of this cube is simply V_n(D)_CC = Dⁿ, and the surface is S_n(D)_CC = 2nDⁿ⁻¹.

However, the edge length A_CI of an n-cube inscribed (CI) inside an n-ball of diameter D is A_CI = D/√n, which is singular for n = 0 and complex for n < 0. Thus, the volume of an n-cube inscribed in an n-ball is

$$V_n{\left(D\right)}_{CI}=A_{CI}^n=D^n{n}^{-n/2}$$

(39)

and the surface is

$$\begin{array}{ll}{S}_n{\left(D\right)}_{CI}& =2n{A}_{CI}^{n-1}=2D^{n-1}{n}^{\left(3-n\right)/2}\\ & =2V_n{\left(D\right)}_{CI}{D}^{-1}n\sqrt{n}\end{array}$$

(40)

The volumes (39) and surfaces (40) are real if n ≥ 0 (by convention 0⁰ := 1), and complex if n < 0, n ∈ ℝ. To examine reflection relations we set m = −n in (39) and (40). This yields volume

$$V_m{\left(D\right)}_{CI}=i^m{D}^{-m}{m}^{m/2}$$

(41)

and surface

$$\begin{array}{ll}{S}_m{\left(D\right)}_{CI}& =-2i^{m+1}{D}^{-\left(m+1\right)}{m}^{\left(3+m\right)/2}\\ & =-2i{V}_m{\left(D\right)}_{CI}{D}^{-1}m\sqrt{m}\end{array}$$

(42)

which are complex for all m ∈ ℝ.

Volume formulas (39) and (41) correspond to each other for n ≤ 0, n ∈ ℝ and for n = 2k, k ∈ ℤ.

Proof. 

By equating (39) with (41), we have

$$D^n{n}^{-n/2}=i^m{D}^{-m}{m}^{m/2}$$

Setting n = −m, that is, reflecting (39) around zero, while leaving (41) intact, yields

$$\begin{array}{l}{D}^{-m}{\left(-m\right)}^{m/2}=i^m{D}^{-m}{m}^{m/2}\\ {\left[{\left(-1\right)}^{1/2}\right]}^m{m}^{m/2}=i^m{m}^{m/2}\iff i^m=i^m\hspace{0.17em}\forall m\in ℝ\end{array}$$

On the other hand, setting m = −n

$$\begin{array}{l}{D}^n{n}^{-n/2}=i^{-n}{D}^n{\left(-n\right)}^{-n/2}\\ n^{-n/2}=i^{-n}{\left[{\left(-1\right)}^{1/2}\right]}^{-n}{n}^{-n/2}\\ i^{2n}=1\iff n=2k,\hspace{0.17em}k\in \mathbb{Z}\end{array}$$

□

Thus, the volumes (39), (41) are real if n is negative and even and imaginary if n is negative and odd.

Surface Formulas (40) and (42) correspond to each other for n ≤ 0, n ∈ ℝ, and for n = 2k − 1, k ∈ ℤ.

Proof. 

By equating (40) with (42), we have

$$2D^{n-1}{n}^{\left(3-n\right)/2}=-2i^{m+1}{D}^{-\left(m+1\right)}{m}^{\left(3+m\right)/2}$$

Setting n = −m yields

$$\begin{array}{l}2D^{-m-1}{\left(-m\right)}^{\left(3+m\right)/2}=-2i^{m+1}{D}^{-m-1}{m}^{\left(3+m\right)/2}\\ {\left[{\left(-1\right)}^{1/2}\right]}^{3+m}{m}^{\left(3+m\right)/2}=-i^{m+1}{m}^{\left(3+m\right)/2}\\ i^{3+m}=-i^{1+m}\iff i^{1+m}=i^{1+m}\hspace{0.17em}\forall m\in ℝ\end{array}$$

On the other hand, setting m = −n, that is, reflecting (42) around zero, while leaving (40) intact, yields

$$\begin{array}{l}2D^{n-1}{n}^{\left(3-n\right)/2}=-2i^{1-n}{D}^{n-1}{\left(-n\right)}^{\left(3-n\right)/2}\\ n^{\left(3-n\right)/2}=-i^{1-n}{\left[{\left(-1\right)}^{1/2}\right]}^{3-n}{n}^{\left(3-n\right)/2}\\ 1=-i^{1-n}{i}^{3-n}\iff i^{-2n}=-1\iff n=2k-1,\hspace{0.17em}k\in \mathbb{Z}\end{array}$$

□

Thus, the surfaces (40), (42) are real if n is negative and odd and imaginary if n is negative and even.

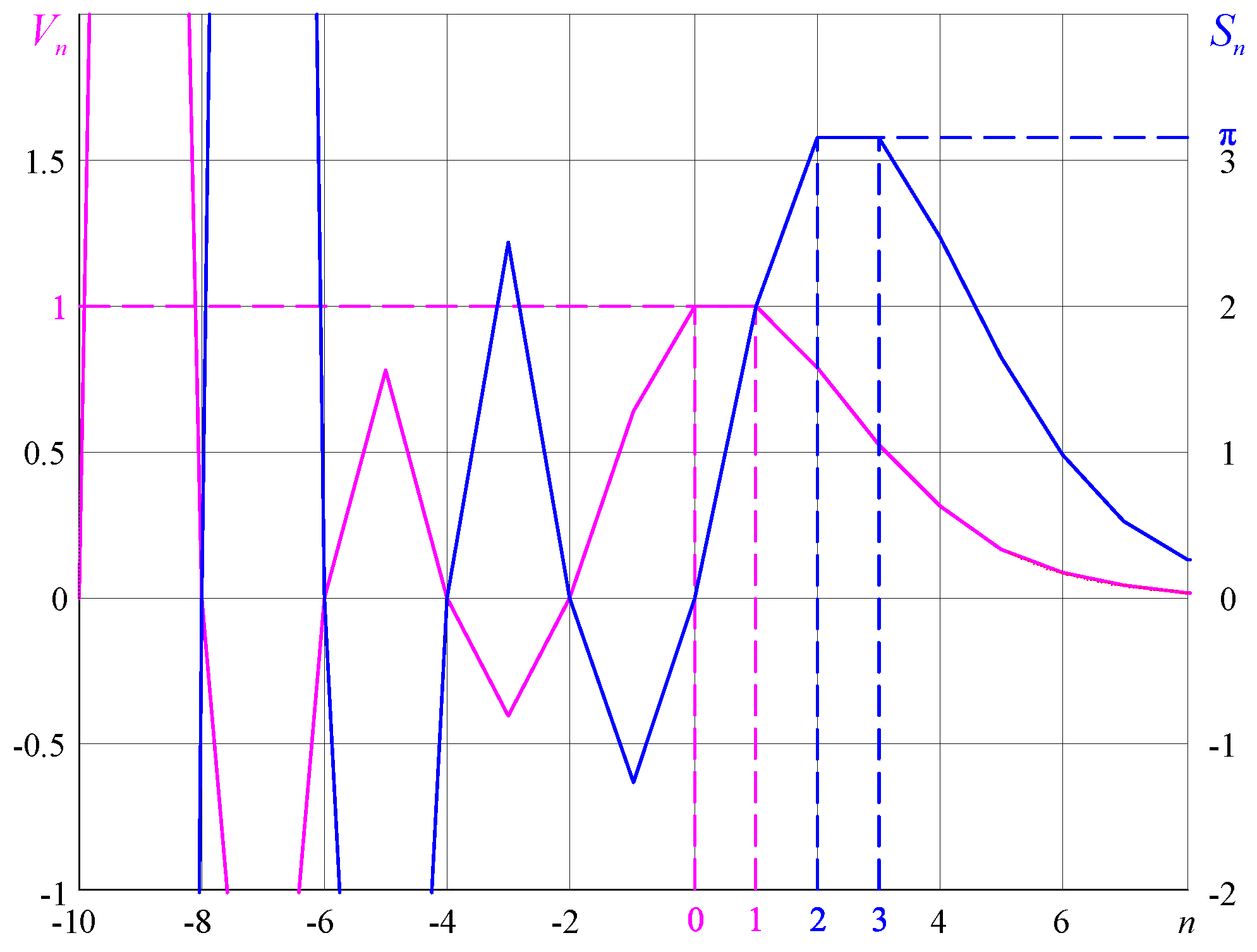
Volumes and surfaces of n-cubes given by Formulas (39)–(42) are shown in Figure 7 and listed in

Table 2. Volumes and surfaces of n-cubes inscribed in n-balls of unit radius and diameter for −8 ≤ n ≤ 3 (rational fraction approximation using Matlab rats function).

n	Vn(R = 1)CI	Sn (R = 1)CI	Vn (D = 1)CI	Sn (D = 1)CI
−8	16	−362.0387i	4096	−185,363.8i
−7	−7.0898i	−16,807/128	−907.4927i	−33,614
−6	−27/8	49.6022i	−216	6349.077i
−5	1.7469i	625/32	55.9017i	1250
−4	1	−8i	16	−256i
−3	−0.6495i	−27/8	−5.1961i	−54
−2	−1/2	i√2	−2	8i√2
−1	i/2	1/2	i	2
0	1	0	1	0
1	2	2	1	2
2	2	4√2	½	2√2
3	8 × 3−3/2	8	3−3/2	2

. This peculiar mixture of integer, rational, and irrational coefficients requires further research.

The ratio of the volume or surface of an n-ball to the volume or surface of an n-cube circumscribing this n-ball can be expressed using diameter recurrence relations (29), (30) as

$$\frac{V_{nB}}{V_{nCC}}=\frac{S_{nB}}{S_{nCC}}=g_n{\pi }^{\lfloor n/2\rfloor }$$

(43)

and similarly, the ratio of volume and surface of an n-ball to volume (39) and surface (40) of an n-cube inscribed in this n-ball can be expressed as

$$\frac{V_{nB}}{V_{nCI}}=g_n{\pi }^{\lfloor n/2\rfloor }{n}^{n/2}$$

(44)

$$\frac{S_{nB}}{S_{nCI}}=g_n{\pi }^{\lfloor n/2\rfloor }{n}^{\left(n-1\right)/2}=\frac{V_{nB}}{V_{nCI}\sqrt{n}}$$

(45)

We conjecture that for 0 < n < 1 volumes of n-cubes inscribed inside n-balls are larger than volumes of those n-balls.

Furthermore, the following holds for (39) and (41) with m = n

$$V_n{\left(D\right)}_{CI}{V}_m{\left(D\right)}_{CI}\stackrel{m=n}{=}{D}^n{n}^{-n/2}{i}^n{D}^{-n}{n}^{n/2}=i^n$$

(46)

5. Summary

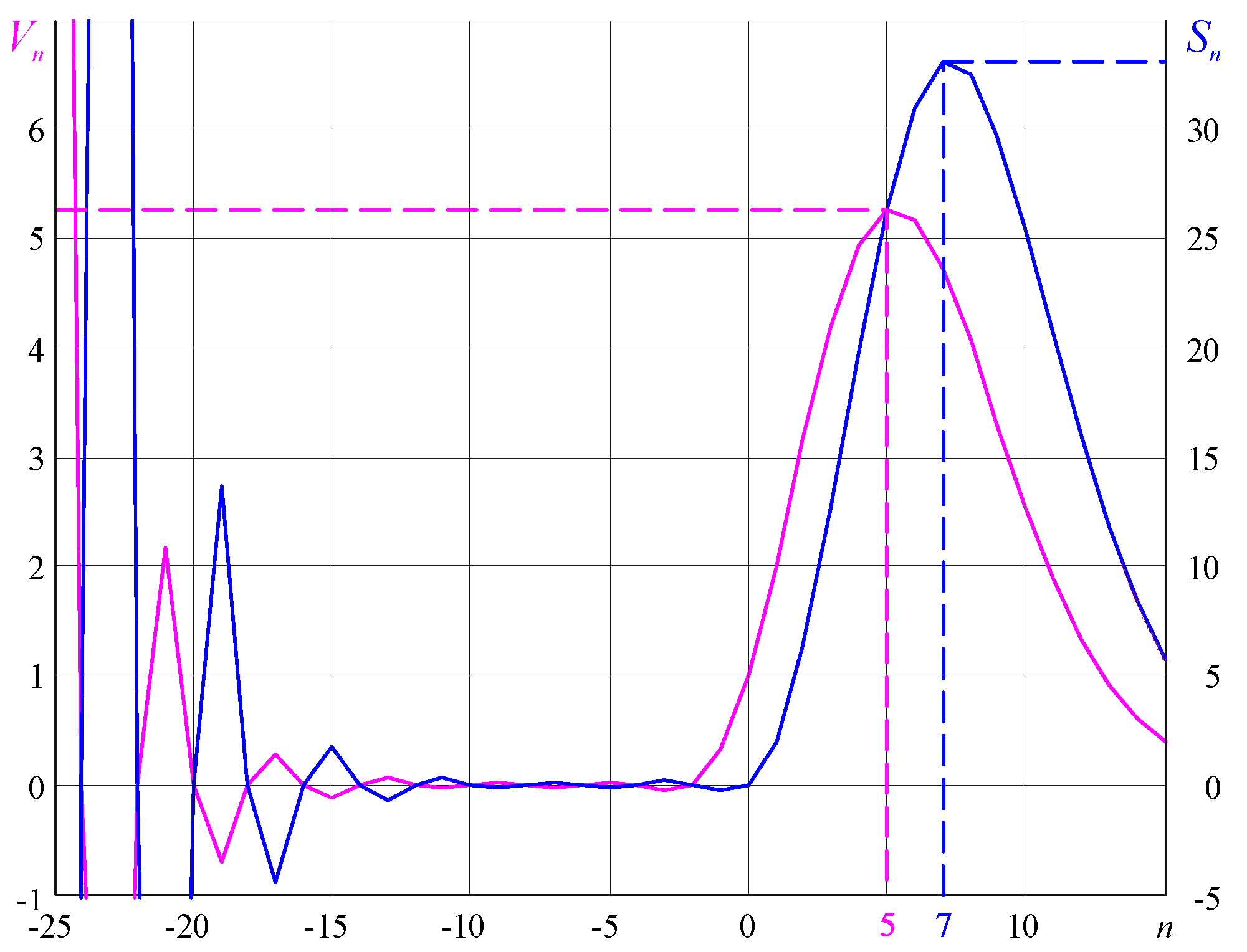
The novel radius recurrence relation f_n (11) enables us to express the known recurrence relation (4) for n-ball volume and the known relation (5) for n-ball surface as a function of π^⌊n/2⌋, showing that the value of π as n-ball volume and surface irrational factor appears only for n < 0 and n ≥ 2 (π^⌊n/2⌋ = 1 for 0 ≤ n < 2).

Sequence (16), inverse to sequence (11), enables the examination of n-ball volumes and surfaces in the negative dimensions. Since f₋₂ = 0, in negative, even dimensions, n-balls have zero (void-like) volumes and zero (point-like) surfaces and become divergent with decreasing n. Curiously, the double factorial n‼ can be extended to negative, odd integers by inverting its recurrence relation and is not defined for negative even integers.

For positive dimensions, n = 5 (the largest unit radius n-ball volume) is the largest odd n where f_n > f_n−1, while n = 7 (the largest unit radius n-ball surface) is the smallest odd n where f_n < f_n−1. The diameter recurrence relation g_n (32) is related with (16) by f_n = 2ⁿg_n.

Algebraic forms (14), (15), (18)–(20) of the relation (16) were presented for even and odd dimensions. Algebraic forms (19), (20) for n ∈ ℂ, expressed in terms of the gamma function, bound the relation (16) for n ∈ ℤ.

Constant (21) of products of pairs of these sequence values for integer n and −n−2 reveal symmetry that is the additive inverse of the symmetry {n, n−2} or equivalence of an ordinary (n − 2)-dimensional space to the n-dimensional superspace [3]. Furthermore sequence (16) reveals symmetry {n, 2 − n} (24) and {n, −n} (27), respectively, between n-ball surfaces and volumes in integer dimensions.

Sequence (16) comprises rational numbers, while all π^⌊n/2⌋ (for n < 0 and n ≥ 2) are most likely transcendental numbers.

It was shown that the known formula (7) for the volume of a regular n-simplex can be expressed as a recurrence relation (35) to remove the indefiniteness of the factorial, and further expressed as (36) to remove singularity for n = 0. Thus, n-simplices are undefined in the negative, integer dimensions if n < −1. This is congruent with the fact that every simplicial n-manifold inherits a natural topology from Euclidean space ℝⁿ [27], and by researching Euclidean space ℝⁿ as a simplicial n-manifold, topological (metric-independent) and geometrical (metric-dependent) content of the modeled quantities are disentangled [27]. Therefore, the lack of n-simplices in the negative, integer dimensions excludes the notion of negatively dimensional Euclidean space ℝⁿ for n < −1. Volumes and surfaces of regular n-simplices are imaginary in negative, fractional dimensions for n < −1 (surfaces also for n < 0) and are divergent with decreasing n.

It was shown that the known formula (9) for the volume of n-orthoplex can be expressed as a recurrence relation (37) to remove indefiniteness of factorial and further expressed as (38) to remove singularity for n = 0. Thus, the volumes of n-orthoplices are zero in the negative, integer dimensions and divergent in the negative, fractional ones with decreasing n. Moreover, the surfaces of n-orthoplices are undefined for integer n < −1 (n-orthoplex has facets that are regular simplices of the previous dimension (10), and these are undefined for integer n ≤ −1), imaginary for fractional n < 0, and also divergent with decreasing n. Peculiarly, in 1 dimension the volume V₁(A)_O = A√2 not A, as in the case of 1-simplex and 1-cube.

Relations (4), (5), (8), (10), (12), (13), (17), (19)–(22), (24), (25), (27), (29), (30), (34)–(46) are continuous on their domains of definitions for n ∈ ℝ. The starting points for fractional dimensions can be provided, e.g., using spline interpolation between two (or three in the case of n-balls) subsequent integer dimensions.

In the negative dimensions, n-simplices, n-orthoplices, and n-balls have different properties than their positively dimensional counterparts, with the n-cube being an exception. The volume V_n(A)_C = Aⁿ and surface S_n(A)_C = 2nAⁿ⁻¹ = 2dV_n(A)_C/dA of an n-cube are defined for any n ∈ ℝ, and are real if A ∈ ℝ. Interestingly, in ℝ³, the fractal dimension of the Sierpiński 3-simplex is 2, of the Sierpiński 3-orthoplex is 2.585, while only the Sierpiński 3-cube retains its regular dimension [28].

Out of three regular, convex polytopes (and n-balls) present in all non-negative dimensions [19], only n-cubes, n-orthoplices, and n-balls are defined in the negative, integer dimensions, with n-cubes being dual to the void. This should not be surprising. There are no 0-dimensional points in negative dimensions.

6. Discussion

Once upon a time, there was a (−1)-dimensional void of volume zero and undefined surface. Then, a 0-dimensional point of unit volume and null surface somehow appeared in this void. This first point is now called the primordial Big Bang singularity. The existence of the first point implied a countably infinite number of other labeled points forming various relations among each other. And thus, the void expanded into real and imaginary dimensionalities.

The presented recurrence relations remove indefiniteness and singularities present in known formulas, revealing the properties of the relevant geometric objects in negative and real dimensions.

The results of this study could perhaps be applied in linguistic statistics, where the dimension in the distribution for frequency dictionaries is chosen to be negative [4], and in fog computing, where n-simplex is related to a full mesh pattern, n-orthoplex is linked to a quasi-full mesh structure, and n-cube is referred to as a certain type of partial mesh layout [29].

Another possible application of the results of this study could be molecular physics and crystallography. There are countably infinitely many spherical harmonics, but nature uses only the first four as subshells of s, p, d, and f electron shells that can hold 2, 6, 10, and 14 electrons, respectively. Further subshells are not populated in the ground states of all the observed elements. The first element that would require a g subshell (18 electrons) would have an atomic number of 121, while the heaviest element synthesized is Oganesson, with an atomic number of 118 and a half-life of about 1/1000 of a second. Perhaps this is linked with properties of the unit radius n-balls in negative dimensions, as illustrated in Figure 2. The “flattening” occurring between dimensions −14 and −2 is intriguing. Dimensions −2, −6, −10, and −14 are bounded from both sides, with −14, which would represent the f subshell, already at the onset of divergence. In nature, the f subshell occurs essentially only in lanthanides and actinides. A simple and approximate formula for a spherical nuclear radius that generates very precise results in quantum and nuclear techniques is R = r₀A^1/3, where A is the atomic number and r₀ = 1.25 ± 0.2 fm.