Some Categorical Properties of Linear Systems
Abstract
:1. Introduction
2. Some Preliminaries
3. Kernels and Cokernels of Feedback Morphisms
Kernels and Cokernels

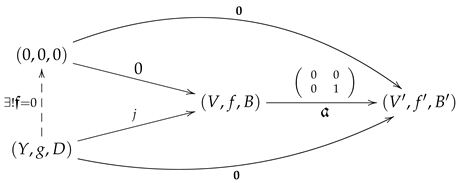
- (i)
- (ii)
- (iii)
- (iv)
- A morphism must be on the block form in order to assure
- (v)
- and because is a feedback morphism
- (vi)
- (by iv) yields
- (vii)
- by (v).
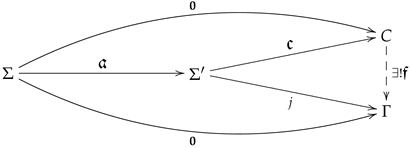
 Then, is a well defined feedback morphism and it is unique, making the left triangle commutative □
Then, is a well defined feedback morphism and it is unique, making the left triangle commutative □- (i)
- (ii)
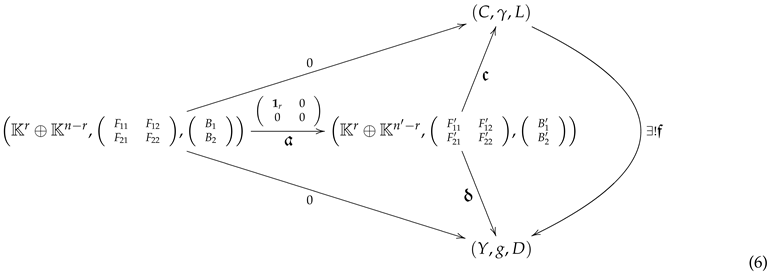
- (i)
- (trivial).
- (ii)
- is not f-invariant because
- (iii)
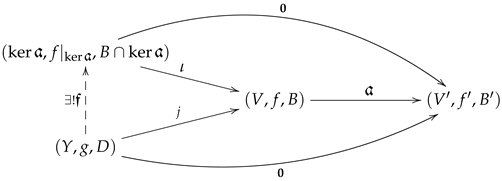
- However, the morphism has a kernel in . In fact, in because of any feedback morphism j in the below diagram
needs to be .
4. Feedback Morphisms, Monomorphisms, and Epimorphisms
- (i)
- If is a monomorphism in -Vect (i.e., injective), then is a monomorphism in .
- (ii)
- If is an epimorphism in -Vect (i.e., surjective), then is an epimorphism in .
5. Sections, Retracts, and Feedback Decompositions
- (i)
- (ii)
- (iii)
- (iv)
- (see [1])
6. Split Exact Sequences of Linear Systems
- (i)
- (ii)
- The following is a split short exact sequence of linear systemswhere ι is the standard inclusion, and π is quotient modulo subspace .
7. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Carriegos, M.V. Morphisms of linear control systems. Open Math. 2022. submitted. [Google Scholar]
- Carriegos, M.V. Enumeration of classes of linear systems via equations and via partitions in a ordered abelian monoid. Linear Algebra Its Appl. 2013, 438, 1132–1148. [Google Scholar] [CrossRef]
- Brunovsky, P.A. A classification of linear controllable systems. Kibernetika 1970, 3, 173–188. [Google Scholar]
- Brewer, J.W.; Klingler, L. On feedback invariants for linear dynamical systems. Linear Algebra Its Appl. 2001, 325, 209–220. [Google Scholar] [CrossRef] [Green Version]
- Kalman, R.E. Kronecker invariants and Feedback. In Ordinary Differential Equations; Academic: Cambridge, MA, USA, 1972; pp. 459–471. [Google Scholar]
- Carriegos, M.V.; Muñoz Castañeda, A.L. On the K-theory of feedback actions on linear systems. Linear Algebra Its Appl. 2014, 440, 233–242. [Google Scholar] [CrossRef]
- Adámek, J.; Herrlich, H.; Strecker, G.E. Abstract and Concrete Categories. The Joy of Cats, TAC Reprints No. 17. 2006. Available online: http://www.tac.mta.ca/tac/reprints/articles/17/tr17.pdf (accessed on 19 April 2022).
- Bühler, T. Exact categories. Expo. Math. 2010, 28, 1–69. [Google Scholar] [CrossRef] [Green Version]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Carriegos, M.V. Some Categorical Properties of Linear Systems. Mathematics 2022, 10, 2088. https://doi.org/10.3390/math10122088
Carriegos MV. Some Categorical Properties of Linear Systems. Mathematics. 2022; 10(12):2088. https://doi.org/10.3390/math10122088
Chicago/Turabian StyleCarriegos, Miguel V. 2022. "Some Categorical Properties of Linear Systems" Mathematics 10, no. 12: 2088. https://doi.org/10.3390/math10122088
APA StyleCarriegos, M. V. (2022). Some Categorical Properties of Linear Systems. Mathematics, 10(12), 2088. https://doi.org/10.3390/math10122088