Techno-Mathematical Discourse: A Conceptual Framework for Analyzing Classroom Discussions
Abstract
:1. Introduction
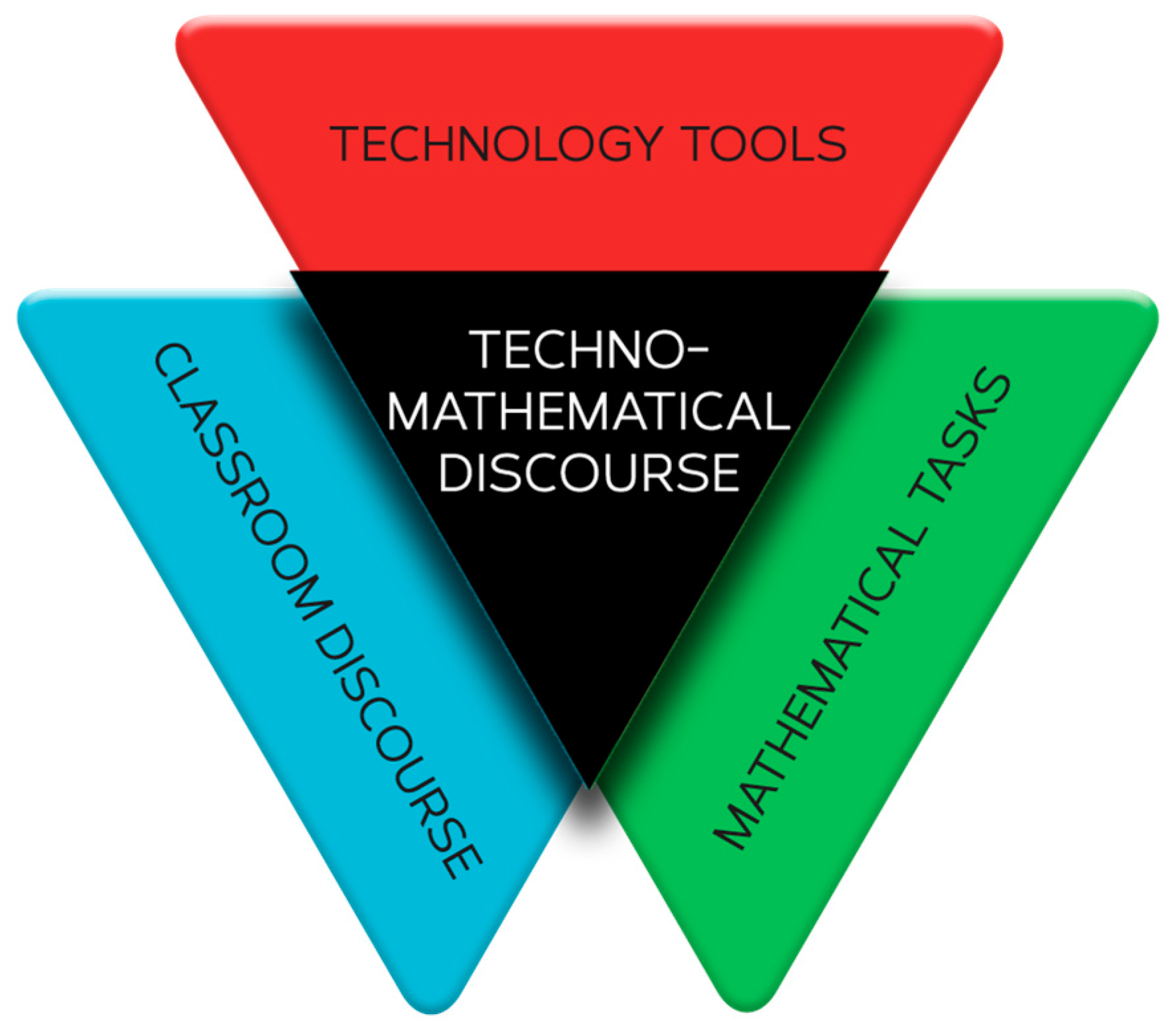
2. Techno-Mathematical Discourse Conceptual Framework
2.1. Classroom Discourse
Impact of Classroom Discourse on TMD
2.2. Technology Tools
Impact of Technology Tools on TMD
2.3. Mathematical Tasks
Impact of Mathematical Tasks on TMD
2.4. Links Among Mathematical Discourse, Technology Tools, and Mathematical Tasks
2.4.1. Impact of Dynamic Representations on Classroom Discourse
2.4.2. Impact of Computer Feedback on Student Collaborations
3. Applying the TMD Framework
3.1. Examples of TMD in Action
3.1.1. Filling Boxes
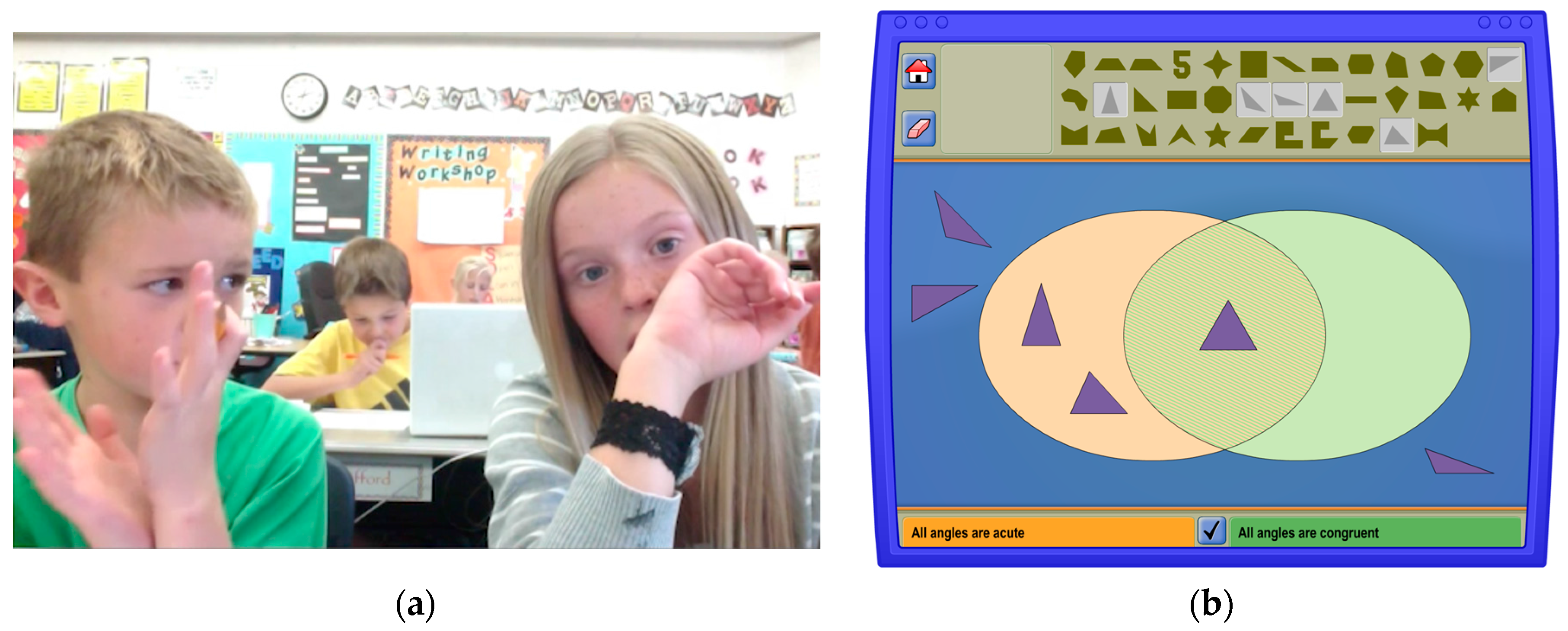
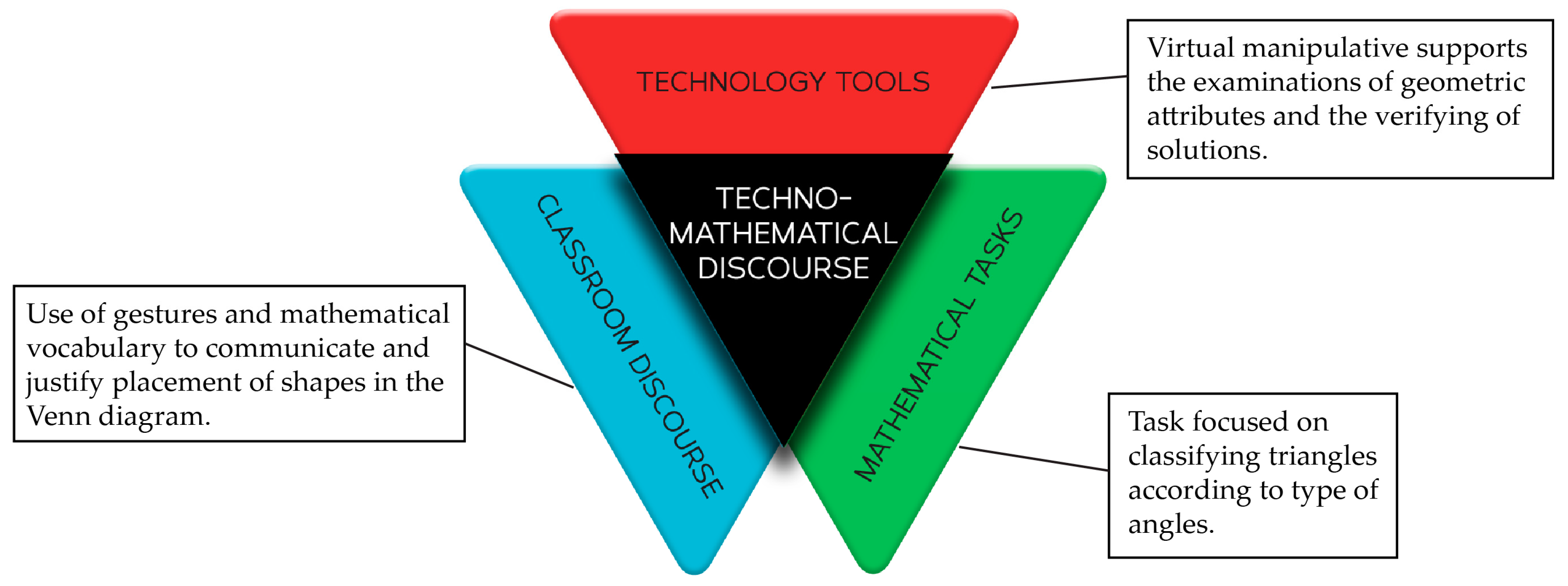
3.1.2. Sorting Triangles
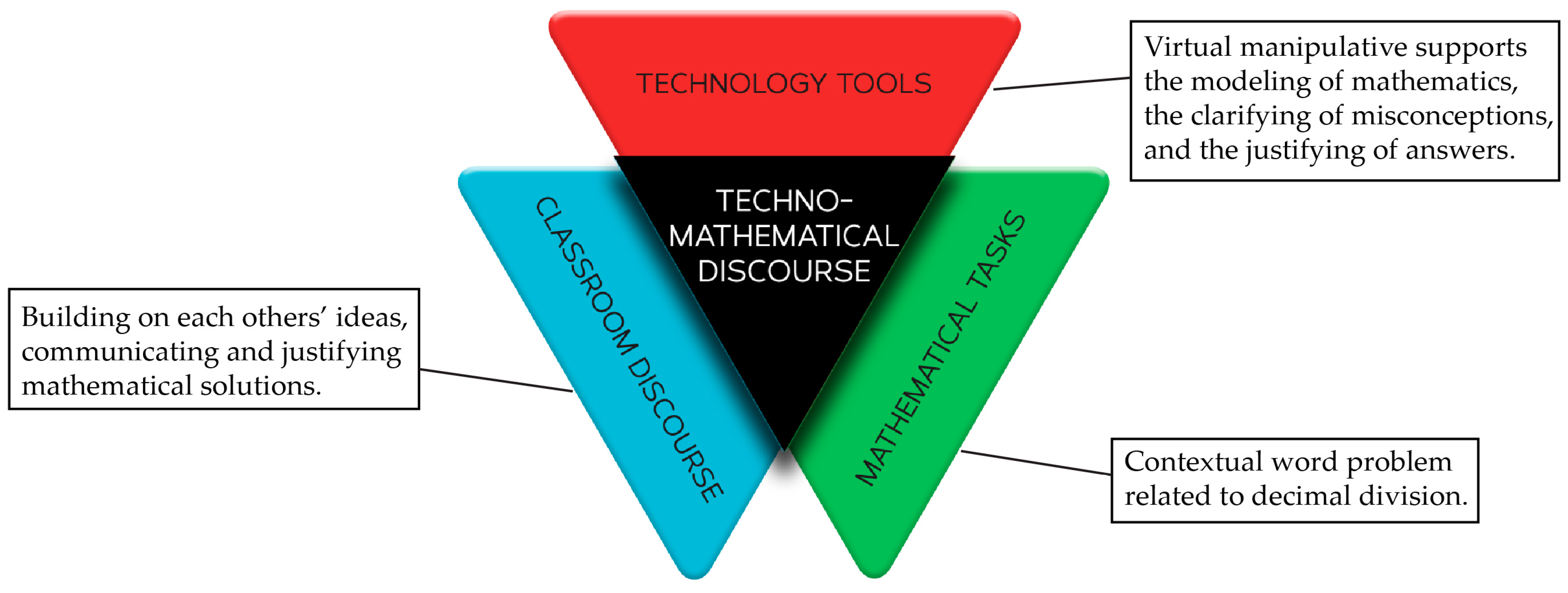
3.1.3. Decimal Division
Nancy’s poster for the school council election covers a space of 6 point 4 square meters. She wants to divide the poster into 4 equal sections for her slogan. How much space will be in each section? Hint: one 10 by 10 square represents 1 square meter. Talk with your partner about how to solve this problem. Write down your answer and explain your thinking.
4. TMD as a Framework for Classroom Implementation
5. TMD as a Framework for Research
6. Conclusions
Acknowledgments
Conflicts of Interest
References
- National Council of Teachers of Mathematics. Mathematics Teaching Today; Martin, T.S., Spear, W.R., Eds.; National Council of Teachers of Mathematics: Reston, VA, USA, 2007. [Google Scholar]
- Evans, M.A.; Feenstra, E.; Ryon, E.; McNeill, D. A multimodal approach to coding discourse: Collaboration, distributed cognition, and geometric reasoning. Int. J. Comput.-Support. Collab. Learn. 2011, 6, 253–278. [Google Scholar] [CrossRef]
- Gresalfi, M.; Martin, T.; Hand, V.; Greeno, J. Constructing competence: An analysis of student participation in the activity systems of mathematics classrooms. Educ. Stud. Math. 2008, 70, 49–70. [Google Scholar] [CrossRef]
- Herbel-Eisenmann, B.; Wagner, D. Appraising lexical bundles in mathematics classroom discourse: Obligation and choice. Educ. Stud. Math. 2010, 75, 43–63. [Google Scholar] [CrossRef]
- Hufferd-Ackles, K.; Fuson, K.C.; Sherin, M.G. Describing levels and components of a math-talk learning community. J. Res. Math. Educ. 2004, 35, 81–116. [Google Scholar] [CrossRef]
- Iiskala, T.; Vauras, M.; Lehtinen, E.; Salonen, P. Socially shared metacognition of dyads of pupils in collaborative mathematical problem-solving processes. Learn. Instr. 2011, 21, 379–393. [Google Scholar] [CrossRef]
- Imm, K.; Stylianou, D.A. Talking mathematically: An analysis of discourse communities. J. Math. Behav. 2012, 31, 130–148. [Google Scholar] [CrossRef]
- Mendez, E.P.; Sherin, M.G.; Louis, D.A. Multiple perspectives on the development of an eighth-grade mathematical discourse community. Elem. Sch. J. 2007, 108, 41–61. [Google Scholar] [CrossRef]
- Nathan, M.J.; Eilam, B.; Kim, S. To disagree, we must also agree: How intersubjectivity structures and perpetuates discourse in a mathematics classroom. J. Learn. Sci. 2007, 16, 523–563. [Google Scholar] [CrossRef]
- Nathan, M.J.; Knuth, E.J. A study of whole classroom mathematical discourse and teacher change. Cognit. Instr. 2003, 21, 175–207. [Google Scholar] [CrossRef]
- Piccolo, D.L.; Harbaugh, A.P.; Carter, T.A.; Capraro, M.M.; Capraro, R.M. Quality of instruction: Examining discourse in middle school mathematics instruction. J. Adv. Acad. 2008, 19, 376–410. [Google Scholar]
- Sherin, M.G. A balancing act: Developing a discourse community in a mathematics classroom. J. Math. Teach. Educ. 2002, 5, 205–233. [Google Scholar] [CrossRef]
- Sinclair, M.P. Peer interactions in a computer lab: Reflections on results of a case study involving web-based dynamic geometry sketches. J. Math. Behav. 2005, 24, 89–107. [Google Scholar] [CrossRef]
- Truxaw, M.P.; DeFranco, T.C. Mathematics in the making: Mapping verbal discourse in Pólya’s “Let Us Teach Guessing” lesson. J. Math. Behav. 2007, 26, 96–114. [Google Scholar] [CrossRef]
- Truxaw, M.P.; DeFranco, T.C. Mapping mathematics classroom discourse and its implications for models of teaching. J. Res. Math. Educ. 2008, 39, 489–525. [Google Scholar]
- Webb, N.M.; Nemer, K.M.; Ing, M. Small-group reflections: Parallels between teacher discourse and student behavior in peer-directed groups. J. Learn. Sci. 2006, 15, 63–119. [Google Scholar] [CrossRef]
- Wood, M.B.; Kalinec, C.A. Student talk and opportunities for mathematical learning in small group interactions. Int. J. Educ. Res. 2012, 51–52, 109–127. [Google Scholar] [CrossRef]
- Zolkower, B.; Shreyar, S. A teacher’s mediation of a thinking-aloud discussion in a 6th grade mathematics classroom. Educ. Stud. Math. 2007, 65, 177–202. [Google Scholar] [CrossRef]
- Pea, R.D. Beyond amplification: Using the computer to reorganize mental functioning. Educ. Psychol. 1985, 20, 167–182. [Google Scholar] [CrossRef]
- Moyer-Packenham, P.S.; Bolyard, J.J. Revisitng the Definition of a Virtual Manipulative. In International Perspectives on Teaching and Learning Mathematics with Virtual Manipulatives; Moyer-Packenham, P.S., Ed.; Springer: Cham, Switzerland, 2016; pp. 3–23. [Google Scholar]
- Moyer-Packenham, P.S.; Westenskow, A. Effects of virtual manipulatives on student achievement and mathematics learning. Int. J. Virtual Pers. Learn. Environ. 2013, 4, 35–50. [Google Scholar] [CrossRef]
- Manches, A.; O’Malley, C.; Benford, S. The role of physical representations in solving number problems: A comparison of young children’s use of physical and virtual materials. Comput. Educ. 2010, 54, 622–640. [Google Scholar] [CrossRef]
- Elstad, E. (Ed.) Why is there a wedge between the promise of educational technology and the experiences in a technology-rich pioneer school? In Digital Expectations and Experiences in Education; Sense Publishers: Rotterdam, The Netherlands, 2016; pp. 77–96.
- Mishra, P.; Koehler, M.J. Technological pedagogical content knowledge: A framework for teacher knowledge. Teach. Coll. Rec. 2006, 108, 1017–1054. [Google Scholar] [CrossRef]
- Anderson-Pence, K.L. Examining the impact of different virtual manipulative types on the nature of students’ small-group discussions: An exploratory mixed methods case study of techno-mathematical discourse. Ph.D. Thesis, Utah State University, Logan, UT, USA, 2014. [Google Scholar]
- Kane, T.J.; Staiger, D.O. Gathering Feedback for Teaching: Combining High-Quality Observations with Student Surveys and Achievement Gains; MET Project; Bill and Melinda Gates Foundation: Seattle, WA, USA, 2012. [Google Scholar]
- Vygotsky, L.S. Mind in Society: The Development of Higher Psychological Processes; Harvard University Press: Cambridge, MA, USA, 1978. [Google Scholar]
- Sfard, A. When the rules of discourse change, but nobody tells you: Making sense of mathematics learning from a commognitive standpoint. J. Learn. Sci. 2007, 16, 565–613. [Google Scholar] [CrossRef]
- Yackel, E.; Cobb, P. Sociomathematical norms, argumentation, and autonomy in mathematics. J. Res. Math. Educ. 1996, 27, 458–477. [Google Scholar] [CrossRef]
- Chapin, S.H.; O’Connor, C.; Anderson, N.C. Classroom Discussions: Using Math Talk to Help Students Learn, 2nd ed.; Math Solutions: Sausalito, CA, USA, 2009. [Google Scholar]
- Cobb, P.; Wood, T.; Yackel, E. Discourse, mathematical thinking, and classroom practice. In Contexts for Learning: Sociocultural Dynamics in Children’s Development; Forman, E.A., Minick, N., Stone, C.A., Eds.; Oxford University Press: New York, NY, USA, 1993; pp. 91–119. [Google Scholar]
- Huang, J.; Normandia, B.; Greer, S. Communicating mathematically: Comparison of knowledge structures in teacher and student discourse in a secondary math classroom. Commun. Educ. 2005, 54, 34–51. [Google Scholar] [CrossRef]
- Brodie, K. Working with learners’ mathematical thinking: Towards a language of description for changing pedagogy. Teach. Teach. Educ. 2011, 27, 174–186. [Google Scholar] [CrossRef]
- Hunter, R. Changing roles and identities in the construction of a community of mathematical inquiry. J. Math. Teach. Educ. 2010, 13, 397–409. [Google Scholar] [CrossRef]
- Mueller, M.F. The co-construction of arguments by middle-school students. J. Math. Behav. 2009, 28, 138–149. [Google Scholar] [CrossRef]
- Wagganer, E.L. Creating math talk communities. Teach. Child. Math. 2015, 22, 248–254. [Google Scholar] [CrossRef]
- White, D.Y. Promoting productive mathematical classroom discourse with diverse students. J. Math. Behav. 2003, 22, 37–53. [Google Scholar] [CrossRef]
- Gibbs, M.J. Patterns of interactions as affected by graphing software: Developing a theoretical framework. Int. J. Technol. Math. Educ. 2006, 13, 139–149. [Google Scholar]
- Moreno-Armella, L.; Hegedus, S.; Kaput, J. From static to dynamic mathematics: Historical and representational perspectives. Educ. Stud. Math. 2008, 68, 99–111. [Google Scholar] [CrossRef]
- Suh, J. Leveraging cognitive technology tools to expand opportunities for critical thinking in elementary mathematics. J. Comput. Math. Sci. Teach. 2010, 29, 289–302. [Google Scholar]
- Lesh, R.; Post, T.; Behr, M. Representations and translations among representations in mathematics learning and problem solving. In Problems of Representation in the Teaching and Learning of Mathematics; Janvier, C., Ed.; Lawrence Erlbaum Associates: Hillsdale, NJ, USA, 1987; pp. 33–40. [Google Scholar]
- Dorward, J. Intuition and research: Are they compatible? Teach. Child. Math. 2002, 8, 329–332. [Google Scholar]
- Anderson-Pence, K.L.; Moyer-Packenham, P.S.; Westenskow, A.; Shumway, J.; Jordan, K. Relationships between visual static models and students’ written solutions to fraction tasks. Int. J. Math. Teach. Learn. 2014, 15, 1–18. [Google Scholar]
- Hensberry, K.K.R.; Moore, E.B.; Perkins, K.K. Effective student learning of fractions with an interactive simulation. J. Comput. Math. Sci. Teach. 2015, 34, 273–298. [Google Scholar]
- Lee, N.H.; Tan, B.L.J. The role of virtual manipulatives on the concrete-pictorial-abstract approach in teaching primary mathematics. Electron. J. Math. Technol. 2014, 8, 102–121. [Google Scholar]
- Moyer-Packenham, P.; Baker, J.; Westenskow, A.; Anderson, K.; Shumway, J.; Jordan, K. Predictors of achievement when virtual manipulatives are used for mathematics instruction. J. Res. Math. Educ. 2014, 3, 121–150. [Google Scholar]
- Westenskow, A.; Moyer-Packenham, P.S.; Anderson-Pence, K.; Shumway, J.F.; Jordan, K. Cute drawings? The disconnect between students’ pictorial representations and their mathematics responses to fraction questions. Int. J. Res. Math. Edu. 2014, 1, 81–105. [Google Scholar]
- Baccaglini-Frank, A.; Maracci, M. Multi-touch technology and preschoolers’ development of number-sense. Digit. Exp. Math. Educ. 2015, 1, 7–27. [Google Scholar] [CrossRef]
- Chiu, T.K. Effects of Prior Knowledge on Mathematics Different Order Thinking Skills in Mobile Multimedia Environments. In Mobile Learning Design; Churchill, D., Lu, J., Chiu, T.K.F., Fox, B., Eds.; Springer: Singapore, 2016; pp. 373–386. [Google Scholar]
- Khoo, K.Y. Enacting app-based learning activities with viewing and representing skills in preschool mathematics lessons. In Mobile Learning Design; Churchill, D., Lu, J., Chiu, T.K.F., Fox, B., Eds.; Springer: Singapore, 2016; pp. 351–372. [Google Scholar]
- Moyer-Packenham, P.S.; Shumway, J.F.; Bullock, E.; Tucker, S.I.; Anderson-Pence, K.L.; Westenskow, A.; Boyer-Thurgood, J.; Maahs-Fladung, C.; Symanzik, J.; Mahamane, S.; et al. Young children’s learning performance and efficiency when using virtual manipulative mathematics iPad apps. J. Comput. Math. Sci. Teach. 2015, 34, 41–69. [Google Scholar]
- Zbiek, R.M.; Heid, M.K.; Blume, G.W.; Dick, T.P. Research on technology in mathematics education: A perspective of constructs. In Second Handbook of Research on Mathematics Teaching and Learning; Lester, F.K., Ed.; Information Age Publishing Incorporated: Charlotte, NC, USA, 2007; Volume 2, pp. 1169–1207. [Google Scholar]
- Ares, N.; Stroup, W.M.; Schademan, A.R. The power of mediating artifacts in group-level development of mathematical discourses. Cognit. Instr. 2008, 27, 1–24. [Google Scholar] [CrossRef]
- González, G.; Herbst, P.G. Students’ conceptions of congruency through the use of dynamic geometry software. Int. J. Comput. Math. Learn. 2009, 14, 153–182. [Google Scholar] [CrossRef]
- Clark, J.M.; Paivio, A. Dual coding theory and education. Educ. Psychol. Rev. 1991, 3, 149–210. [Google Scholar] [CrossRef]
- Smith, M.S.; Stein, M.K. Selecting and creating mathematical tasks: From research to practice. Math. Teach. Middle Sch. 1998, 3, 344–350. [Google Scholar]
- Piaget, J. The Origins of Intelligence; International Universities Press: Madison, CT, USA, 1952. [Google Scholar]
- Stein, M.K.; Lane, S. Instructional tasks and the development of student capacity to think and reason: An analysis of the relationship between teaching and learning in a reform mathematics project. Educ. Res. Eval. 1996, 2, 50–80. [Google Scholar] [CrossRef]
- Lack, B. Student Participation in Mathematics Discourse in a Standards-Based Middle Grades Classroom. Ph.D. Thesis, Georgia State University, Atlanta, GA, USA, 2010. [Google Scholar]
- Cubes. Available online: http://illuminations.nctm.org/Activity.aspx?id=4095 (accessed on 13 January 2017).
- Shape Sorter. Available online: http://illuminations.nctm.org/Activity.aspx?id=3581 (accessed on 13 January 2017).
- Base Ten Blocks. Available online: http://www.glencoe.com/sites/common_assets/mathematics/ebook_assets/vmf/VMF-Interface.html (accessed on 13 January 2017).
- Carpenter, T.P.; Fennema, E.; Franke, M.L.; Levi, L.; Empson, S.B. Children’s Mathematics: Cognitively Guided Instruction, 2nd ed.; Heinemann: Portsmouth, NH, USA, 2014. [Google Scholar]
- Equivalent Fractions. Available online: https://illuminations.nctm.org/activity.aspx?id=3510 (accessed on 13 January 2017).
- National Library of Virtual Manipulatives. Available online: http://nlvm.usu.edu/ (accessed on 13 January 2017).
- Motion Math: Fractions! Available online: http://motionmathgames.com/motion-math-game/ (accessed on 13 January 2017).
- Mind Research Institute: ST Math. Available online: http://www.mindresearch.org/stmath (accessed on 13 January 2017).



| Line | Student | Speaking Turn |
|---|---|---|
| 65 | Callie | Ok, so how many unit blocks to fill the box? |
| 66 | Colton | 20… |
| 67 | Callie | …So 6, no that’s 5 blocks… |
| 68 | Colton | (using the mouse pointer to count the “blue” height of the box) 1, 2, 3, 4, 5, 6, 7. 20 times 7 is 140. So it should be 140 blocks. |
| 69 | Callie | …For the whole thing, yeah. 140 blocks. |
| 70 | Colton | 20 times 7 is 140. I’ll just write 140 blocks… So change it to 5. What’s the next number? |
| 71 | Callie | Width 5, depth 7, height 3. (Colton types second set of dimensions into the virtual manipulatives (VM)) So 3 times… |
| 72 | Colton | Wait. Hold on. Just a second. Let’s go back to that one. It didn’t have 20 on the bottom. We need to go back (Colton types previous set of dimensions into the VM). |
| 73 | Callie | Yeah it did. |
| 74 | Colton | 3…5…7. Look, (using mouse pointer to count the dimensions of the base of the first box) 1, 2, 3, 4, 5 times 3. So it’s … |
| 75 | Both | …15… |
| 76 | Colton | …times 7 … Not 20. |
| 77 | Callie | 15…105. |
| 78 | Colton | Ok. All right. Now we do this… (Types second set of dimensions into the VM). |
| 79 | Callie | So the next one is width 5, depth 7, height 3. So… |
| 80 | Both | (Colton uses mouse pointer as both count aloud the dimensions of the base of the second box) 1, 2, 3, 4, 5, 6, 7… |
| 81 | Callie | …times 5. |
| 82 | Colton | …times (using mouse pointer to count) 1, 2, 3, 4, 5. Yep. |
| 83 | Callie | So 35 times… |
| 84 | Colton | (makes a sweeping motion with the mouse across the “blue” height of the box) …3. I got… |
| 85 | Both | …105. |
| 86 | Callie | Again! …Ok (reading task) “What is the volume of a box with width 7, depth 3,…” |
| 87 | Colton | (types third set of dimensions into the VM) It’s just changing the numbers up. So I think it will be 105. |
| 88 | Callie | (reading task) “…height, 5.” Let’s double check to see if it is 105 blocks. So… |
| 89 | Colton | (Colton makes sweeping motion with the mouse pointer over the dimensions of the base of the third box) 1, 2, 3. |
| 90 | Callie | So 7 times 3. 21 times… |
| 91 | Colton | (examining image without counting with the mouse pointer) …5? |
| 92 | Callie | 5…. So…105. |
| 93 | Colton | Yep … Ok. |
| Line | Student | Speaking Turn |
|---|---|---|
| 90 | Callie | So all angles are acute (selects “All angles are acute” on VM). All angles are congruent (selects “All angles are congruent” on VM). Ok. So all 7 triangles… So this triangle... (hovers mouse pointer over the first triangle in the set). |
| 91 | Colton | …has 2 acute. |
| 92 | Callie | All angles are congruent—that’s not true. All of the angles are not the same length. |
| 93 | Colton | Well it doesn’t have all angles acute. So…it wouldn’t be… |
| 94 | Callie | So it wouldn’t go in either. (moves the first triangle into the space outside of the Venn diagram) This one. (hovers mouse pointer over the next triangle in the set) It definitely has all acute angles. (makes a sweeping pointing motion toward the computer screen) But… |
| 95 | Colton | Are all the angles congruent? |
| 96 | Callie | No. Wait. |
| 97 | Colton | No, they’re not. Like the acute angles. Like one of them is like bigger and the other ones are smaller (demonstrating larger and smaller angles with hands and forearms). |
| 98 | Callie | Oh, yeah. ‘Cause on this shape… (circles mouse pointer over the triangle in question). |
| 99 | Colton | That would just be in the red. |
| 100 | Callie | (moves the triangle into the left-hand section of the Venn diagram) This one? |
| 101 | Colton | Yeah. |
| 102 | Callie | Then next shape. This one… (hovers mouse over the next triangle in the set). |
| 103 | Colton | That’s a right angle, (pointing to triangle on screen with finger) so… |
| 104 | Callie | Yeah, so it’s not all acute. And it’s not congruent. (moves the triangle to the space outside of the Venn diagram) So…ok. (hovers mouse pointer over the next triangle in the set) This one. It definitely does not have all acute angles… |
| 105 | Colton | Not all acute angles. |
| 106 | Callie | (pointing to screen) and it’s a huge line. It doesn’t match up with anything else. (Colton moves the triangle into the space outside of the Venn diagram) So it’s neither. Next one. (Colton hovers mouse pointer over the next triangle in the set) That’s an obtuse angle, (pointing to triangle on screen with finger) so it’s not all acute and it’s not all congruent. (Colton moves the triangle into the space outside of the Venn diagram). |
| 107 | Colton | This one… (hovers mouse pointer over the next triangle in the set). |
| 108 | Callie | All acute, right? And then it’s all… |
| 109 | Colton | …congruent. |
| 110 | Callie | It’s congruent, so… (Colton moves triangle into the center of the Venn diagram) and then… (Colton hovers mouse pointer over the last triangle in the set) This one. (points to next triangle with finger) All angles are acute. |
| 111 | Colton | And… I don’t think they’re all congruent. (moves triangle into the left-hand section of the Venn diagram) Check. (clicks on the checkmark to check solution. VM feedback: all correct). |
| 112 | Callie | Yep. We got them all right. |
| Line | Student | Speaking Turn |
|---|---|---|
| 45 | Colton | So 64 divided by 4 equals what? |
| 46 | Callie | Yeah, so we know that we have 6 point 4 square meters. And a 10 by 10 equals one square meter. So if we have six, then we need six 10 by 10s. (Colton moves 6 hundreds-flats onto the workspace section with mouse) Ok, then it says, (reading task) “Talk with your partner about…” So we know that we have 6 whole pieces. But then what about the other 4…? |
| 47 | Colton | We need point 4. So that would be the tens. |
| 48 | Callie | Yeah. (Colton moves 2 tens-rods onto the workspace section with the mouse) |
| 49 | Colton | So… |
| 50 | Callie | 4 (points to the tens-rods with finger. Colton moves two more tens-rods on the workspace section with the mouse) So ok, then it says, (reading task) “Write down your answer and explain your thinking.” |
| 51 | Colton | So we need to split it into 4 equal sections. So that’s… |
| 52 | Callie | You can do… |
| 53 | Colton | (counting hundreds-flats while pointing with the mouse) 1, 2, 3… One and a half… Wait. One and a half [10 by 10 blocks]. Then add one of these (points to a 1x10 block with finger). That’s 1 point 6 meters. And then… Yeah. It would be 1… Wait. |
| 54 | Callie | We need 1 point 1 if we take those two. (pointing to blocks on screen with finger) |
| 55 | Colton | (moves the triangle into the left-hand section of the Venn diagram) This one? |
| 56 | Callie | Yeah. |
| 57 | Colton | And then 1 divided by 4 would be… No, 2 divided by 4 would be a half. So you would add another point 5. |
| 58 | Callie | So… |
| 59 | Colton | That would be 1 point…6. |
| 60 | Callie | 6. Yeah. |
| 61 | Colton | So it would be 1 point 6 square meters. |
© 2017 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license ( http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Anderson-Pence, K.L. Techno-Mathematical Discourse: A Conceptual Framework for Analyzing Classroom Discussions. Educ. Sci. 2017, 7, 40. https://doi.org/10.3390/educsci7010040
Anderson-Pence KL. Techno-Mathematical Discourse: A Conceptual Framework for Analyzing Classroom Discussions. Education Sciences. 2017; 7(1):40. https://doi.org/10.3390/educsci7010040
Chicago/Turabian StyleAnderson-Pence, Katie L. 2017. "Techno-Mathematical Discourse: A Conceptual Framework for Analyzing Classroom Discussions" Education Sciences 7, no. 1: 40. https://doi.org/10.3390/educsci7010040
APA StyleAnderson-Pence, K. L. (2017). Techno-Mathematical Discourse: A Conceptual Framework for Analyzing Classroom Discussions. Education Sciences, 7(1), 40. https://doi.org/10.3390/educsci7010040





