Teacher Moves for Building a Mathematical Modeling Classroom Community
Abstract
1. Introduction
2. Literature Review
2.1. Classroom Norms as a Foundation for Student Participation in Mathematical Modeling
2.2. Teacher Moves in Facilitating Mathematical Modeling Classroom Discussions
2.3. The Framework
3. Methods and Materials
3.1. Research Setting, Participants, and Classroom Activities
3.2. Data Collection, Coding, and Analysis
3.3. Findings and Discussion
3.4. Phase of Making Assumptions and Building a Model
- Alyse:
- So far, our key components are how long employees have worked there, their job positions, and salaries.
- Ann:
- And job experience.
- T:
- May I ask how you thought about job experience?
- Alyse:
- I think that higher-level jobs already mean more experience. You cannot be a manager without having experience. It is part of the job position.
- Cora
- We made a list. The five employees work in a fast-food place; they all do the same job, work for the same amount of money, and the same hours. We would like to keep things simple.
- T:
- So, you are saying you made some assumptions.
- Cole:
- Yeah, we are thinking of building a rating system, so that whoever gets more rating points could receive the highest raise.
- T:
- What I see is that you listed your key components and thought about what is essential for you. I am curious how you will convince anyone that your method works?
- Cora:
- I will show examples from the graph once we create it.
- Cole:
- Yes, it is different from the first method, which simply divides USD 10,000 by 5, thereby giving an equal share of USD 2000 to each employee.
- Cora:
- Agree. It would be easier to explain why our method makes sense using our graph.
3.5. Phase of Working Mathematically and Determining Results
- T:
- How would you describe the relationship between your equation and graph?
- Cole:
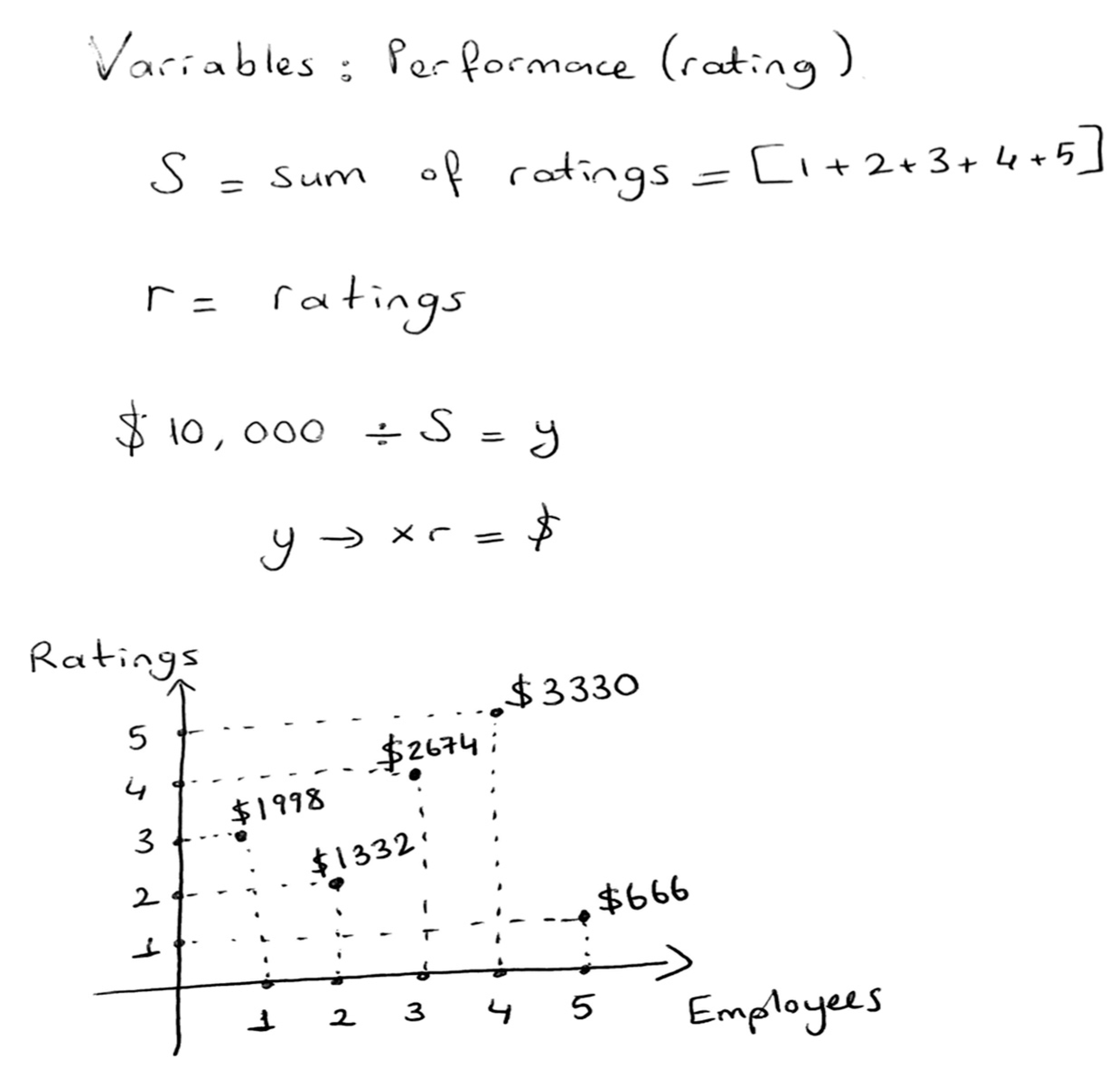
- We first imagined this graph, and the equation came up later. The points on the x-axis represent workers, and the points on the y-axis represent the rating points. For example, Worker 2 receives two stars, and Worker 1 receives one star; thus, the little points on the coordinate graph represent each employee’s money.
- Cora:
- The equation we created there helped us find out unit money, which we then multiplied by each employee’s rating points.
- Cora:
- USD 10,000 is what we want to distribute. S is the sum of the ratings. Here, S is 15 [indicating 1 + 2 + 3 + 4 + 5]. We divide USD 10,000 by S to calculate the unit money.
- T:
- I see two variables in this equation: the independent variable S, which is the sum of the rating points you chose freely, and the dependent variable y, which depends on S.
- Alyse:
- Yes, unit money y is dependent on the sum of the rating points, which represent a worker’s performance, ensuring that we distribute USD10,000 using this equation.
- T:
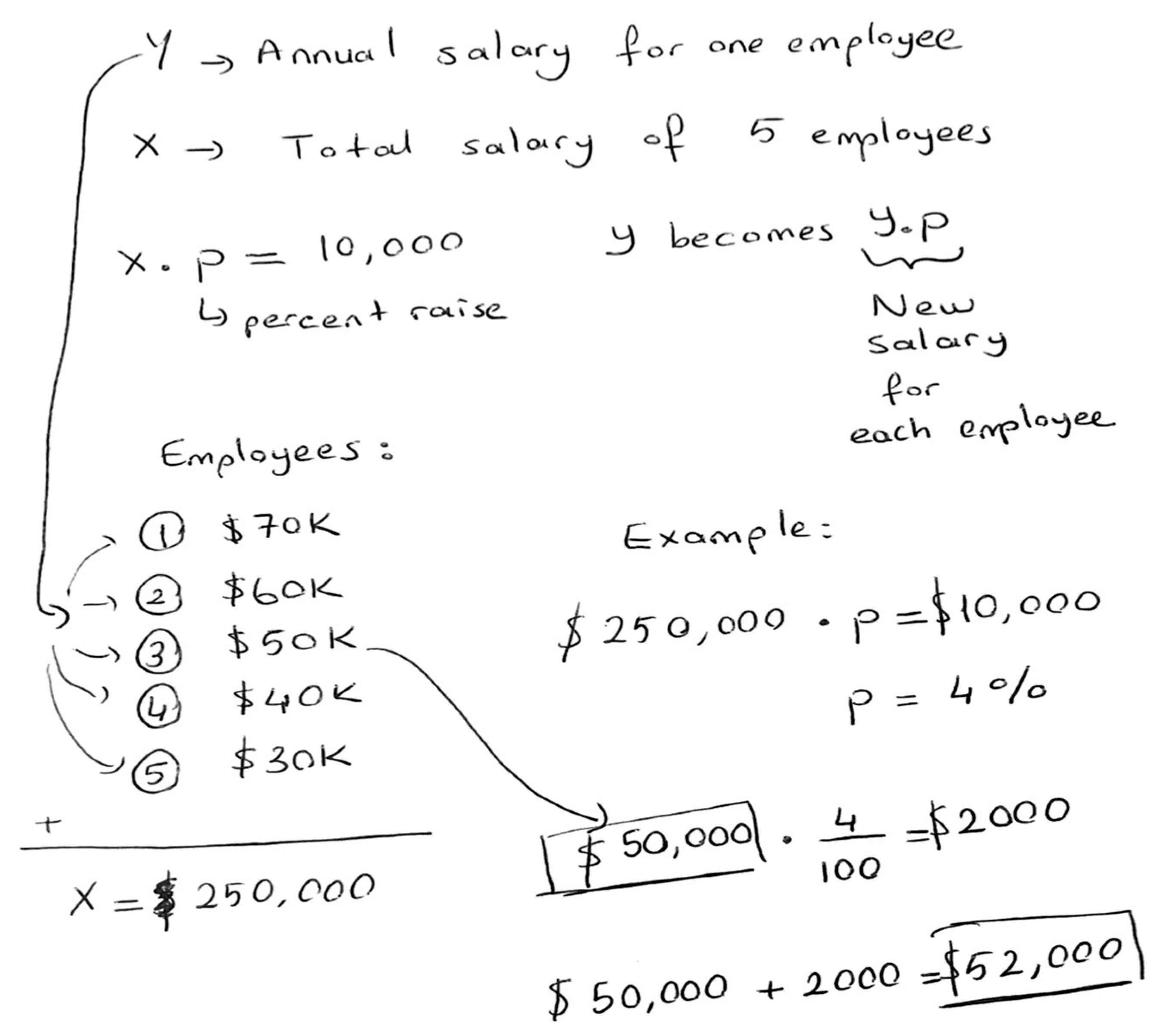
- We have two methods for using percentages. For those who find this method [pointing out Figure 3] more convenient, convince us why.
- Burcin:
- This makes sense because our group randomly assigned percentages and multiplied them by salaries to determine the raises. However, we were not sure whether we had distributed exactly USD 10,000; we ended up with a total of USD 8000.
- Ann:
- Our method focuses on creating an equation to determine percent raise. Our variables are X, total salary of 5 employees; Y, annual salary for each employee.
- Burcin:
- Why do you divide 10,000 by X?
- Ann:
- To calculate the percent raise for each employee.
- Cora:
- Y is important to find each employee’s raise based on the salaries.
3.6. Phase of Interpreting
- Alyse:
- One advantage of our method is that it can be applied to other jobs.
- T:
- Could you explain what you mean by “applied to other jobs”?
- Ann:
- Let us say it is the office of a company that includes a manager, assistant, full-time and part-time workers, etc.
- Alyse:
- We could distribute raises for all types of workers.
- T:
- Regarding the applicability of Alyse’s group method, they concluded that their model is realistic because it can benefit any type of company and different workers through pay raises.
- Cora:
- So, are we looking at how realistic our models are?
- T:
- Yes, how practical and realistic your model is!
- Beth:
- I now think that the more variables are included in a model, the more realistic the results become.
- Cora:
- However, we cannot include all variables, even if they are important.
- T:
- Exactly! That is why we are choosing key variables. Models might have their own limitations, but we will determine which one seems the most realistic and applicable.
- Beth:
- The title on the y-axis [see Figure 2] should be “average rating points” if we want it to be more realistic.
- T:
- Can someone who agrees with Beth explain why “average rating points” are important?
- Cora:
- It’s because, currently, we do not know if each employee was rated by the same customers.
- Burcin:
- It is like—50 customers rated those five employees, and each employee received average points from those 50 customers.
3.7. Phase of Checking Results and Repeating the Process
- T:
- How do you know that your results are accurate and reliable? Are there any parts of your model that could be improved to make more sense?
- Cole:
- Compared to other groups, I would say that mathematically our solution [see Figure 2] is correct. We successfully distributed USD 10,000 among five employees using their rating points. However, we only considered job performance when deciding on the raises. We could multiply the years of experience by the rating points to help divide the USD 10,000 and determine the amount that each person should receive.
- T:
- Cole’s group decided to verify the accuracy of the calculations and determine whether the model needed refinement. We are doing this collectively to enhance our shared understanding.
- Ann:
- I think our model [see Figure 3]—the equation used to calculate percentages by considering years of work, annual salary, and the total salary of five different employees—results in correct calculations. We were able to distribute USD 10,000. With this system, we can distribute any amount of money.
- T:
- Okay, that is a strength of your model. It produces an accurate calculation.
- Ann:
- Our model considers more variables. However, we are missing the years of experience. This could have been another variable and made a part of the equation as a multiplier.
- T:
- We have two suggestions for revising this model [see Figure 2]. One idea is to give raises to employees who get three stars or more. The other is to multiply years of experience by the rating points. Who supports the first idea? Convince us.
- Pera:
- I do. Just because someone has more experience, it does not mean they are doing a better job. The three-star system rewards performance and gives employees a clear goal to reach.
- Yang:
- I disagree! Experience matters, too. Someone with ten years at the company might not always get the highest ratings, but their experience still benefits the company in ways that ratings do not show. We could combine them.
- Beth:
- I agree with Yang. The three-star system is not clear on how it would work mathematically, but using experience with ratings is doable and USD 10,000 can be distributed.
- Cole:
- Yeah, adding experience as a multiplier makes the system more complex but also more reliable.
4. Conclusions
Implications and Limitations
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Abassian, A., Safi, F., Bush, S., & Bostic, J. (2020). Five different perspectives on mathematical modeling in mathematics education. Investigations in Mathematics Learning, 12(1), 53–65. [Google Scholar] [CrossRef]
- Anhalt, C. O., & Cortez, R. (2015). Mathematical modeling: A structured process. The Mathematics Teacher, 108(6), 446–452. [Google Scholar] [CrossRef]
- Aroeira, A. J., Carreira, S., & da Ponte, J. P. (2024). Teacher strategic interventions to support students in constructing the model of the situation in a modelling task. In H.-S. Siller, V. Geiger, & G. Kaiser (Eds.), Researching mathematical modelling education in disruptive times (pp. 161–171). Springer Nature. [Google Scholar]
- Blum, W. (2015). Quality teaching of mathematical modelling: What do we know, what can we do? In S. J. Cho (Ed.), The proceedings of the 12th international congress on mathematical education (pp. 73–96). Springer. [Google Scholar]
- Borromeo Ferri, R. (2018). Learning how to teach mathematical modeling in school and teacher education. Springer. [Google Scholar]
- Brady, C., & Jung, H. (2022). Modeling presentations: Toward an assessment of emerging classroom cultures of modeling. Educational Studies in Mathematics, 109(2), 237–261. [Google Scholar] [CrossRef]
- Brady, C., Ramírez, P., & Lesh, R. (2024). Problem posing and modeling: Confronting the dilemma of rigor or relevance. In T. L. Toh, M. Santos-Trigo, P. H. Chua, N. A. Abdullah, & D. Zhang (Eds.), Problem posing and problem solving in mathematics education: International research and practice trends (pp. 33–50). Springer Nature. [Google Scholar]
- Campbell, T. (2023). Examining how middle grade mathematics students seize learning opportunities through conflict in small groups. Mathematical Thinking and Learning, 25(2), 208–231. [Google Scholar] [CrossRef]
- Campbell, T. G., & Yeo, S. (2021). Exploring in-the-moment teaching moves that support sociomathematical and general social norms in dialogic instruction. International Journal of Science and Mathematics Education, 21, 1–23. [Google Scholar] [CrossRef]
- Chapin, S. H., O’Connor, C., O’Connor, M. C., & Anderson, N. C. (2009). Classroom discussions: Using math talk to help students learn, grades K–6. Math Solutions. [Google Scholar]
- Cobb, P., Boufi, A., McClain, K., & Whitenack, J. (1997). Reflective discourse and collective reflection. Journal for Research in Mathematics Education, 28(3), 258–277. [Google Scholar] [CrossRef]
- de Oliveira, A. M. P., & Barbosa, J. C. (2013). Mathematical modelling, mathematical content and tensions in discourses. In G. A. Stillman, G. Kaiser, W. Blum, & J. Brown (Eds.), Teaching mathematical modelling: Connecting to research and practice (pp. 67–76). Springer. [Google Scholar]
- Doerr, H. M. (2006). Examining the tasks of teaching when using students’ mathematical thinking. Educational Studies in Mathematics, 62(1), 3–24. [Google Scholar] [CrossRef]
- Doerr, H. M., & English, L. D. (2006). Middle grade teachers’ learning through students’ engagement with modeling tasks. Journal of Mathematics Teacher Education, 9(1), 5–32. [Google Scholar] [CrossRef]
- Elia, I., Gagatsis, A., Panaoura, A., Zachariades, T., & Zoulinaki, F. (2009). Geometric and algebraic approaches in the concept of “limit” and the impact of the “didactic contract”. International Journal of Science and Mathematics Education, 7(4), 765–790. [Google Scholar] [CrossRef]
- English, L. D. (2003). Reconciling theory, research, and practice: A models and modelling perspective. Educational Studies in Mathematics, 54(2), 225–248. [Google Scholar] [CrossRef]
- English, L. D., & Doerr, H. M. (2004). Listening and responding to students’ ways of thinking. In I. Putt, R. Farragher, & M. McLean (Eds.), Mathematics education for the third millennium: Towards 2010 (pp. 215–222). MERGA. [Google Scholar]
- Galbraith, P., & Stillman, G. (2006). A framework for identifying student blockages during transitions in the modelling process. ZDM—Mathematics Education, 38(2), 143–162. [Google Scholar] [CrossRef]
- Garfunkel, S. A., Montgomery, M., Bliss, K., Fowler, K., Galluzzo, B., Giordano, F., Godbold, L., Gould, H., Levy, R., Libertini, J., & Long, M. (2016). GAIMME: Guidelines for assessment & instruction in mathematical modeling education. Consortium for Mathematics and Its Applications. [Google Scholar]
- Geiger, V., Galbraith, P., Niss, M., & Delzoppo, C. (2022). Developing a task design and implementation framework for fostering mathematical modelling competencies. Educational Studies in Mathematics, 109, 313–336. [Google Scholar] [CrossRef]
- Greefrath, G., Siller, H. S., Klock, H., & Wess, R. (2022). Pre-service secondary teachers’ pedagogical content knowledge for the teaching of mathematical modelling. Educational Studies in Mathematics, 109(2), 383–407. [Google Scholar] [CrossRef]
- Hofmann, R., & Ruthven, K. (2018). Operational, interpersonal, discussional and ideational dimensions of classroom norms for dialogic practice in school mathematics. British Educational Research Journal, 44(3), 496–514. [Google Scholar] [CrossRef]
- Illustrative Mathematics. (2016, May 4). HSN-Q.A. Reason quantitively and use units to solve problems. Available online: http://tasks.illustrativemathematics.org/content-standards/HSN/Q/A (accessed on 28 September 2024).
- Jung, H., & Brady, C. (2023). Modeling actions foregrounded in whole-class modeling discourse: A case study of a model-eliciting activity and a three-act task. Mathematical Thinking and Learning, 27, 1–24. [Google Scholar] [CrossRef]
- Jung, H., & Brady, C. (2024). Teachers posing model-eliciting activities to connect socially-relevant perspective with closely connected mathematical ideas. In H.-S. Siller, V. Geiger, & G. Kaiser (Eds.), Researching mathematical modelling education in disruptive times (pp. 317–327). Springer Nature Switzerland. [Google Scholar]
- Kaiser, G., Schwarz, B., & Tiedemann, S. (2010). Future teachers’ professional knowledge on modeling. In R. Lesh, P. L. Galbraith, C. R. Haines, & A. Hurford (Eds.), Modeling students’ mathematical modeling competencies (pp. 433–444). Springer. [Google Scholar]
- Kaiser, G., & Sriraman, B. (2006). A global survey of international perspectives on modelling in mathematics education. ZDM, 38, 302–310. [Google Scholar] [CrossRef]
- Kang, S. M., & Kim, M. K. (2016). Sociomathematical norms and the teacher’s mathematical belief: A case study from a Korean in-service elementary teacher. Eurasia Journal of Mathematics, Science and Technology Education, 12(10), 2733–2751. [Google Scholar]
- Langer-Osuna, J. M. (2016). The social construction of authority among peers and its implications for collaborative mathematics problem solving. Mathematical Thinking and Learning, 18(2), 107–124. [Google Scholar] [CrossRef]
- Lesh, R., Hoover, M., Hole, B., Kelly, A., & Post, T. (2000). Principles for developing thought-revealing activities for students and teachers. In A. Kelly, & R. Lesh (Eds.), Handbook of research design in mathematics and science education (pp. 591–646). Lawrence Erlbaum Associates. [Google Scholar]
- Lesh, R., & Lehrer, R. (2003). Models and modeling perspectives on the development of students and teachers. Mathematical Thinking and Learning, 5(2–3), 109–129. [Google Scholar] [CrossRef]
- Lesh, R., & Sriraman, B. (2005). Mathematics education as a design science. ZDM—Mathematics Education, 37(6), 490–505. [Google Scholar] [CrossRef]
- Lesh, R. A., & Doerr, H. M. (Eds.). (2003). Beyond constructivism: Models and modeling perspectives on mathematics problem solving, learning, and teaching. Lawrence Erlbaum Associates. [Google Scholar]
- Manouchehri, A., Bekdemir, M., & Yao, X. (2020). Facilitating modelling activities in a grade 5 classroom. In G. A. Stillman, G. Kaiser, & C. E. Lampen (Eds.), Mathematical modelling education and sense-making (pp. 187–197). Springer. [Google Scholar]
- Mayring, P. (2015). Qualitative content analysis: Theoretical background and procedures. In A. Bikner-Ahsbahs, C. Knipping, & N. Presmeg (Eds.), Approaches to qualitative research in mathematics education: Examples of methodology and methods (pp. 365–380). Springer. [Google Scholar]
- National Council of Teachers of Mathematics. (2011). Cash or gas? NCTM. [Google Scholar]
- Ozturk, A. (2021). Examining the relationship between instructional practices and students’ mathematical modeling competencies [Doctoral dissertation, Ohio State University]. Available online: http://rave.ohiolink.edu/etdc/view?acc_num=osu1625748925881711 (accessed on 5 January 2025).
- Ozturk, A. (2023). Fostering quantitative reasoning through a question of fairness. Mathematics Teacher: Learning and Teaching PK-12, 116(4), 265–272. [Google Scholar] [CrossRef]
- Ozturk, A. (2024). Examining translanguaging practices in mathematical modelling. In H. S. Siller, V. Geiger, & G. Kaiser (Eds.), Researching mathematical modelling education in disruptive times. International perspectives on the teaching and learning of mathematical modelling. Springer. [Google Scholar] [CrossRef]
- Partanen, A. M., & Kaasila, R. (2015). Sociomathematical norms negotiated in the discussions of two small groups investigating calculus. International Journal of Science and Mathematics Education, 13(4), 927–946. [Google Scholar] [CrossRef]
- Savuran, R., & Akkoç, H. (2021). Examining pre-service mathematics teachers’ use of technology from a sociomathematical norm perspective. International Journal of Mathematical Education in Science and Technology, 54(1), 74–98. [Google Scholar] [CrossRef]
- Schukajlow, S., Kolter, J., & Blum, W. (2015). Scaffolding mathematical modelling with a solution plan. ZDM—Mathematics Education, 47(7), 1241–1254. [Google Scholar] [CrossRef]
- Schukajlow, S., Krawitz, J., Kanefke, J., Blum, W., & Rakoczy, K. (2023). Open modelling problems: Cognitive barriers and instructional prompts. Educational Studies in Mathematics, 114(3), 417–438. [Google Scholar] [CrossRef]
- Siller, H. S., Geiger, V., & Kaiser, G. (2024). Researching mathematical modelling education in disruptive times—An introduction. In Researching mathematical modelling education in disruptive times (pp. 3–11). Springer Nature Switzerland. [Google Scholar] [CrossRef]
- Siller, H. S., Nitzan-Tamar, O., & Kohen, Z. (2023). Scaffolding practices for modelling instruction in STEM-related contexts: Insights from expert and novice teachers. ZDM—Mathematics Education, 55(7), 1351–1364. [Google Scholar] [CrossRef]
- Steffe, L. P., & Ulrich, C. (2020). Constructivist teaching experiment. In S. Lerman (Ed.), Encyclopedia of mathematics education (pp. 134–141). Springer. [Google Scholar]
- Stein, M. K., Engle, R. A., Smith, M. S., & Hughes, E. K. (2008). Orchestrating productive mathematical discussions: Five practices for helping teachers move beyond show and tell. Mathematical Thinking and Learning, 10(4), 313–340. [Google Scholar] [CrossRef]
- Stender, P. (2019). Heuristic strategies as a toolbox in complex modelling problems. In G. A. Stillman, & J. P. Brown (Eds.), Lines of inquiry in mathematical modelling research in education (pp. 197–212). Springer. [Google Scholar]
- Stephan, M. (2020). Sociomathematical norms in mathematics education. In S. Lerman (Ed.), Encyclopedia of mathematics education (pp. 802–805). Springer. [Google Scholar]
- Tropper, N., Leiss, D., & Hänze, M. (2015). Teachers’ temporary support and worked-out examples as elements of scaffolding in mathematical modeling. ZDM—Mathematics Education, 47(7), 1225–1240. [Google Scholar] [CrossRef]
- Wickstrom, M. H., & Jung, H. (2024). Understanding preservice elementary teachers as mathematical modelers and their perceptions of the process. Journal for Research in Mathematics Education, 55(4), 182–209. [Google Scholar] [CrossRef]
- Wilhelm, A. G. (2014). Mathematics teachers’ enactment of cognitively demanding tasks: Investigating links to teachers’ knowledge and conceptions. Journal for Research in Mathematics Education, 45(5), 637–675. [Google Scholar] [CrossRef]
- Yackel, E., & Cobb, P. (1996). Sociomathematical norms, argumentation, and autonomy in mathematics. Journal for Research in Mathematics Education, 27(4), 458–477. [Google Scholar] [CrossRef]
- Yackel, E., Rasmussen, C., & King, K. (2000). Social and sociomathematical norms in an advanced undergraduate mathematics course. The Journal of Mathematical Behavior, 19(3), 275–287. [Google Scholar] [CrossRef]
- Yenmez, A. A., & Erbaş, A. K. (2023). Facilitating a sustainable transformation of sociomathematical norms through mathematical modeling activities. International Journal of Science and Mathematics Education, 21(3), 761–785. [Google Scholar] [CrossRef]
- Zahner, W., & Moschkovich, J. (2010). Talking while computing in groups: The not-so-private functions of computational private speech in mathematical discussions. Mind, Culture, and Activity, 17(3), 265–283. [Google Scholar] [CrossRef]

| Weeks | Mathematical Goal | Sample Task |
|---|---|---|
| 1 | Identifying and relating quantities | Cash or Gas Problem (Adapted from National Council of Teachers of Mathematics, 2011): Suppose you have two job options: One pays you USD 8.50 an hour, and the other one gives a fixed amount of USD 300 a week. Which option would you take? |
| 2 | Defining appropriate quantities for a descriptive model | Giving Raises Problem (Adapted from Illustrative Mathematics, 2016): A small company wants to give raises to their 5 employees. They have USD 10,000 available to distribute. Imagine you are in charge of deciding how the raises should be determined. |
| 3 | Estimating and relating quantities | Traffic Jam Problem (Adapted from Illustrative Mathematics, 2016): Last Sunday, an accident caused a traffic jam 15 miles long on a straight stretch of a two-lane freeway. How can we figure out the number of people in the traffic jam? |
| 4 | Defining appropriate quantities for a descriptive model | Choosing College Problem (Adapted from Garfunkel et al., 2016): Choose 5 colleges that you are interested in and indicate how well they match or meet your chosen criteria? How can you use your responses to create a mathematical system that will help you choose the most suitable school for you? |
| Categories of Teacher Talk Moves | Descriptions | Examples |
|---|---|---|
| Eliciting students’ ideas | Teacher prompts students to share their strategies and solutions, using questions and restatements to help clarify their ideas. |
|
| Introducing terms and ideas used in mathematical modeling | Teacher introduces terms while summarizing ideas, ensuring the whole class can follow the students’ explanations to build a shared understanding. |
|
| Encouraging students to take a position | Teacher interacts with students by acknowledging their perspectives on solutions, prompting them to argue for or against specific approaches to model development. |
|
| Weeks | Eliciting Ideas | Introducing Terms and Ideas | Encouraging Students to Take a Position | Total (Moves per Week) |
|---|---|---|---|---|
| Week 1 | 73% | 12% | 15% | 52 |
| Week 2 | 50% | 21% | 29% | 48 |
| Week 3 | 25% | 38% | 37% | 68 |
| Week 4 | 26% | 30% | 44% | 32 |
| Teacher Talk Moves | Students’ Participation in Modeling Phases | Total Codes | |||
|---|---|---|---|---|---|
| Make Assumptions; Build a Model | Work Mathematically; Determine Results | Interpret | Check Results; Repeat Process | ||
| Eliciting ideas | 34% | 14% | 27% | 25% | 86 |
| Introducing terms and ideas | 7% | 30% | 41% | 22% | 51 |
| Encouraging students to take a position | 12% | 24% | 29% | 35% | 63 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ozturk, A. Teacher Moves for Building a Mathematical Modeling Classroom Community. Educ. Sci. 2025, 15, 376. https://doi.org/10.3390/educsci15030376
Ozturk A. Teacher Moves for Building a Mathematical Modeling Classroom Community. Education Sciences. 2025; 15(3):376. https://doi.org/10.3390/educsci15030376
Chicago/Turabian StyleOzturk, Ayse. 2025. "Teacher Moves for Building a Mathematical Modeling Classroom Community" Education Sciences 15, no. 3: 376. https://doi.org/10.3390/educsci15030376
APA StyleOzturk, A. (2025). Teacher Moves for Building a Mathematical Modeling Classroom Community. Education Sciences, 15(3), 376. https://doi.org/10.3390/educsci15030376






