Exploring Learning Difficulties in Abstract Algebra: The Case of Group Theory
Abstract
:1. Introduction
1.1. Literature Review
- How should instructional elements be designed when teaching group theory?
- Do learning difficulties found with qualitative methods also present themselves in a quantitative setting? If so, which difficulties can be observed and how pronounced are they?
1.2. The Hildesheim Teaching Concept
- ⋯examines the impact of the curriculum on students’ development of conceptual understanding of group theory, and;
- ⋯that explores possible learning difficulties that appear regarding introductory group theory.
2. Research Questions
- RQ1:
- Do learners achieve an adequate conceptual understanding of introductory group theory when instructed with the Hildesheim Teaching Concept and which concepts post the most hurdles for learners?
- RQ2:
- Which learning difficulties regarding introductory group theory can be identified?
3. Methods
3.1. Study Design and Samples
- An expert survey with experts from mathematics and mathematics education.
- A quantitative evaluation of the Hildesheim Teaching Concept with pre-service teachers.
3.2. Instruments
- D1: Naive Set Theory, Binary operations, associativity, commutativity;
- D2: Neutral element and inverses;
- D3: Cayley Tables;
- D4: Cyclic groups and dihedral groups;
- D5: Subgroups;
- D6: Isomorphism.
- Domain 1—Definitional Fundamentals: Naive set theory, binary operations, associativity, commutativity;
- Domain 2—Beginner Concepts: Neutral element, inverses, Cayley Tables;
- Domain 3—Intermediate Concepts: Dihedral groups, cyclic groups, isomorphisms.
3.3. Data Analysis
3.3.1. Analysis Carried Out to Answer RQ1
3.3.2. Analysis Carried Out to Answer RQ2
- Calculate the average CRI for each wrong answer option and investigate options with .
- Calculate for each wrong answer option the number of responses that were given confident (CRI = 4) or very confident (CRI = 5).
4. Results and Discussion
4.1. Results Regarding RQ1
4.2. Discussion of RQ1
4.3. Results Regarding RQ2
4.4. Discussion of RQ2
4.4.1. Problems with Associativity and Commutativity
4.4.2. Problems with Inverses and the Neutral Element
4.4.3. Problems with Visualizations of Abstract Notions
5. Limitations of This Study
“The associativity property is required because we do not want the order of composition to matter.”
“The notion isomorphic means that the Cayley tables are identical.”
6. Conclusions and Outlook
- Does a revision of items 1 and 9 lead to a disappearance of the observed learning difficulties?
- How and to what extent do the expounded learning difficulties impede learning gain?
- Can these systematic learning obstacles also be observed in qualitative settings with individual learners? If so, can they be characterized in greater detail?
- Can the cognitive structure of gestalt and functionality be extracted empirically to enrich the understanding of learning processes in introductory group theory?
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A
| Item 1: The associativity property is required because ⋯ | |||||
| □ | ⋯ otherwise it is not clear how to compose 3 or more elements. | ||||
| □ | ⋯ we do not want the order of composition to matter. | ||||
| □ | ⋯ along with distributivity and commutativity it is a fundamental rule of mathematics. | ||||
| □ Very sure | □ Sure | □ Undecided | □ Unsure | □ Guessed | |
| Item 2: A binary operation on a set M is ⋯ | |||||
| □ | ⋯ a map . | ||||
| □ | ⋯ a map . | ||||
| □ | ⋯ a map . | ||||
| □ Very sure | □ Sure | □ Undecided | □ Unsure | □ Guessed | |
| Item 3: An example for a group is ⋯ | |||||
| □ | ⋯ | ||||
| □ | ⋯ | ||||
| □ | ⋯ | ||||
| □ Very sure | □ Sure | □ Undecided | □ Unsure | □ Guessed | |
| Item 4: Let be non-abelian and . The inverse of is ⋯ | |||||
| □ | ⋯ | ||||
| □ | ⋯ | ||||
| □ | ⋯ | ||||
| □ Very sure | □ Sure | □ Undecided | □ Unsure | □ Guessed | |
| Item 5: One can show that defines an operation on such that is a group. The neutral element of this operation is ⋯ | |||||
| □ | ⋯ 5 | ||||
| □ | ⋯ 0 | ||||
| □ | ⋯ | ||||
| □ Very sure | □ Sure | □ Undecided | □ Unsure | □ Guessed | |
| Item 6: One can show that defines an operation on such that is a group. The inverse of is given by ⋯ | |||||
| □ | ⋯ | ||||
| □ | ⋯ | ||||
| □ | ⋯ | ||||
| □ Very sure | □ Sure | □ Undecided | □ Unsure | □ Guessed | |
| Item 7: Let and , then ⋯ | |||||
| □ | ⋯ | ||||
| □ | ⋯ | ||||
| □ | ⋯ | ||||
| □ Very sure | □ Sure | □ Undecided | □ Unsure | □ Guessed | |
| Item 8: In the group the equation is solved by ⋯ | |||||
| □ | ⋯ | ||||
| □ | ⋯ | ||||
| □ | ⋯ | ||||
| □ Very sure | □ Sure | □ Undecided | □ Unsure | □ Guessed | |
| Item 9: The notion isomorphic means that ⋯ | |||||
| □ | ⋯ the groups are indifferentiable from a mathematical point of view. | ||||
| □ | ⋯ the Cayley tables are identical. | ||||
| □ | ⋯ the groups are identical. | ||||
| □ Very sure | □ Sure | □ Undecided | □ Unsure | □ Guessed | |
| Item 10: The operation ⊕ within the group has been altered to ∘ such that is no longer necessarily the neutral element. Find the neutral element with the help of the Cayley table. | |||||
| □ | |||||
| □ | |||||
| □ | |||||
| □ Very sure | □ Sure | □ Undecided | □ Unsure | □ Guessed | |
| Item 11: A group structure is to be established on the set where the following Cayley table is given. Which element must be at ⋆? | |||||
| □ | |||||
| □ | |||||
| □ | |||||
| □ Very sure | □ Sure | □ Undecided | □ Unsure | □ Guessed | |
| Item 12: The set has been equipped with a group structure by the following Cayley table. What is the inverse of z? | |||||
| □ | |||||
| □ | |||||
| □ | |||||
| □ Very sure | □ Sure | □ Undecided | □ Unsure | □ Guessed | |
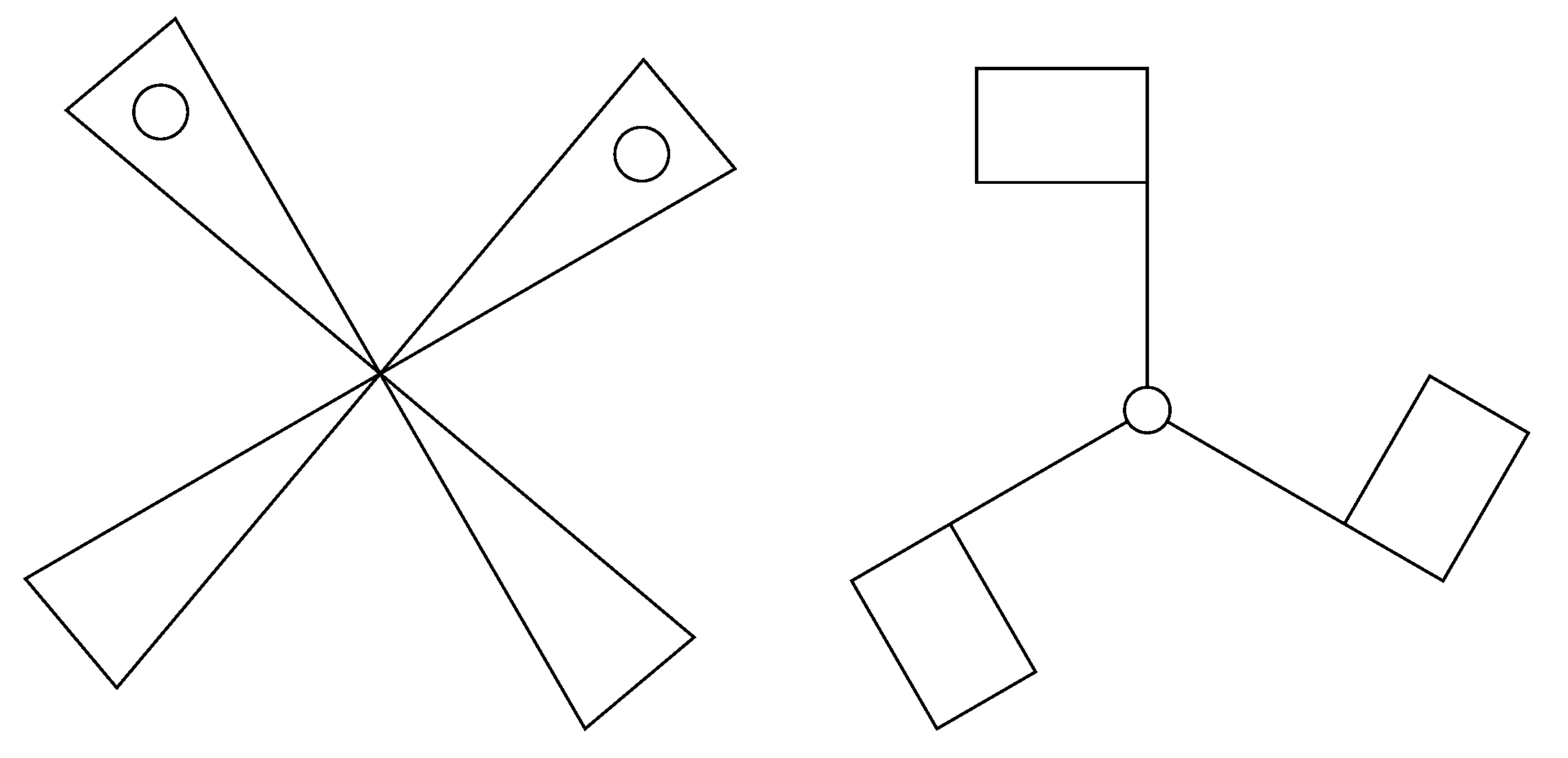
| Item 13: What is the symmetry group of the figure? | |||||
 | |||||
| □ | |||||
| □ | |||||
| □ | |||||
| □ Very sure | □ Sure | □ Undecided | □ Unsure | □ Guessed | |
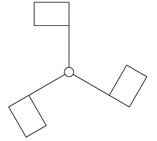
| Item 14: What is the symmetry group of the figure? | |||||
 | |||||
| □ | |||||
| □ | |||||
| □ | |||||
| □ Very sure | □ Sure | □ Undecided | □ Unsure | □ Guessed | |
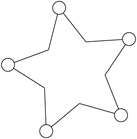
| Item 15: What is the symmetry group of the figure? | |||||
 | |||||
| □ | |||||
| □ | |||||
| □ | |||||
| □ Very sure | □ Sure | □ Undecided | □ Unsure | □ Guessed | |
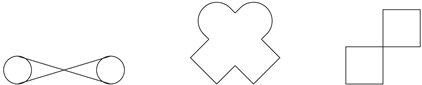
| Item 16: Which two of the following figures have an isomorphic symmetry group? | |||||
 | |||||
| □ | The first and the third. | ||||
| □ | The first and the second. | ||||
| □ | The second and the third. | ||||
| □ Very sure | □ Sure | □ Undecided | □ Unsure | □ Guessed | |
| Item 17: If a Group is commutative the Cayley tyble is ⋯ | |||||
| □ | ⋯ axially symmetric to the diagonal. | ||||
| □ | ⋯ point symmetric to the entry in the middle. | ||||
| □ | ⋯ axially symmetric to the anti diagonal (top left to bottom right). | ||||
| □ Very sure | □ Sure | □ Undecided | □ Unsure | □ Guessed | |
| Item 18: Which of the following sets is a subgroup of if equipped with ⊕? | |||||
| □ | |||||
| □ | |||||
| □ | |||||
| □ Very sure | □ Sure | □ Undecided | □ Unsure | □ Guessed | |
| Item 19: Which of the following permutations does not describe an isometry of the square? | |||||
| □ | |||||
| □ | |||||
| □ | |||||
| □ Very sure | □ Sure | □ Undecided | □ Unsure | □ Guessed | |
| Item 20: Which of the following tables is a Cayley table? | |||||
| □ | The third. | ||||
| □ | The first. | ||||
| □ | The second. | ||||
| □ Very sure | □ Sure | □ Undecided | □ Unsure | □ Guessed | |
References
- Wasserman, N.H. Introducing Algebraic Structures through Solving Equations: Vertical Content Knowledge for K-12 Mathematics Teachers. Primus 2014, 24, 191–214. [Google Scholar] [CrossRef]
- Wasserman, N.H. Abstract Algebra for Algebra Teaching: Influencing School Mathematics Instruction. Can. J. Sci. Math. Technol. Educ. 2016, 16, 28–47. [Google Scholar] [CrossRef]
- Wasserman, N.H. Making Sense of Abstract Algebra: Exploring Secondary Teachers’ Understandings of Inverse Functions in Relation to Its Group Structure. Math. Think. Learn. 2017, 19, 181–201. [Google Scholar] [CrossRef]
- Wasserman, H.H. Connecting Abstract Algebra to Secondary Mathematics, for Secondary Mathematics Teachers, 1st ed.; Springer: Basel, Switzerland, 2018. [Google Scholar]
- Melhuish, K. The Design and Validation of a Group Theory Concept Inventory. Ph.D. Thesis, Portland State University, Portland, OR, USA, 2015. [Google Scholar]
- Melhuish, K.; Fagan, J. Connecting the Group Theory Concept Assessment to Core Concepts at the Secondary Level. In Connecting Abstract Algebra to Secondary Mathematics, for Secondary Mathematics Teachers; Springer: Basel, Switzerland, 2018; pp. 19–45. [Google Scholar]
- Melhuish, K. The Group Theory Concept Assessment: A Tool for Measuring Conceptual Understanding in Introductory Group Theory. Int. J. Res. Undergrad. Math. Educ. 2019, 5, 359–393. [Google Scholar] [CrossRef]
- Veith, J.M.; Bitzenbauer, P. What Group Theory Can Do for You: From Magmas to Abstract Thinking in School Mathematics. Mathematics 2022, 10, 703. [Google Scholar] [CrossRef]
- Larsen, S. Struggling to Disentangle the Associative and Commutative Properties. Learn. Math. 2010, 30, 37–42. [Google Scholar]
- Zaslavsky, O.; Peled, I. Inhibiting Factors in Generating Examples by Mathematics Teachers and Student Teachers: The Case of Binary Operation. J. Res. Math. Educ. 1996, 27, 67–78. [Google Scholar] [CrossRef]
- Veith, J.M.; Bitzenbauer, P.; Girnat, B. Towards Describing Student Learning of Abstract Algebra: Insights into Learners’ Cognitive Processes from an Acceptance Survey. Mathematics 2022, 10, 1138. [Google Scholar] [CrossRef]
- Even, R. The relevance of advanced mathematics studies to expertise in secondary school mathematics teaching: Practitioners’ views. ZDM Math. Educ. 2011, 43, 941–950. [Google Scholar] [CrossRef]
- Veith, J.M.; Bitzenbauer, P.; Girnat, B. Assessing Learners’ Conceptual Understanding of Introductory Group Theory Using the CI2GT: Development and Analysis of a Concept Inventory. Educ. Sci. 2022, 12, 376. [Google Scholar] [CrossRef]
- Chick, H.L.; Harris, K. Grade 5/6 Teachers’ Perceptions of Algebra in the Primary School Curriculum. In Proceedings of the 31st Conference of the International Group for the Psychology of Mathematics Education, Seoul, Korea, 8–13 July 2007; pp. 127–134. [Google Scholar]
- Common Core State Standards in Mathematics (CCSS-M). 2010. Available online: http://www.corestandards.org/Math/Content/mathematics-glossary (accessed on 4 July 2022).
- Branco, N.; Ponte, J.P. Analysis of Teaching and Learning Situations in Algebra in Prospective Teacher Education. J. Educ. 2013, 1, 182–213. [Google Scholar]
- Shamash, J.; Barabash, M.; Even, R. From Equations to Structures: Modes of Relevance of Abstract Algebra to School Mathematics as Viewed by Teacher Educators and Teachers. In Connecting Abstract Algebra to Secondary Mathematics, for Secondary Mathematics Teachers; Springer: Basel, Switzerland, 2018; pp. 241–262. [Google Scholar]
- Burn, R. What Are the Fundamental Concepts of Group Theory? Educ. Stud. Math. 1996, 31, 371–377. [Google Scholar] [CrossRef]
- Baldinger, E.E. Learning Mathematical Practices to Connect Abstract Algebra to High School Algebra. In Connecting Abstract Algebra to Secondary Mathematics, for Secondary Mathematics Teachers; Springer: Cham, Switzerland, 2018; pp. 211–239. [Google Scholar]
- Shimizu, J.K. The Nature of Secondary Mathematics Teachers’ Efforts to Make Ideas of School Algebra Accessible. Ph.D. Thesis, The Pennsylvania State University, University Park, PA, USA, 2013. [Google Scholar]
- Zbiek, R.M.; Heid, M.K. Making Connections from the Secondary Classroom to the Abstract Algebra Course: A Mathematical Activity Approach. In Connecting Abstract Algebra to Secondary Mathematics, for Secondary Mathematics Teachers; Springer: Cham, Switzerland, 2018; pp. 189–209. [Google Scholar]
- Leron, U.; Dubinsky, E. An abstract algebra story. Am. Math. Mon. 1995, 102, 227–242. [Google Scholar] [CrossRef]
- Hake, R.R. Interactive-engagement versus traditional methods: A six-thousand-student survey of mechanics test data for introductory physics courses. Am. J. Phys. 1998, 66, 64. [Google Scholar] [CrossRef]
- Hasan, S.; Bagayoko, D.; Kelley, E.L. Misconceptions and the certainty of response index. Phys. Educ. 1999, 34, 294–299. [Google Scholar] [CrossRef]
- Rappaport, D. The New Math and Its Aftermath. Sch. Sci. Math. 1976, 7, 563–570. [Google Scholar] [CrossRef]
- Larsen, S.P. A local instructional theory for the guided reinvention of the group and isomorphism concepts. J. Math. Behav. 2013, 32, 712–725. [Google Scholar] [CrossRef]
- Larsen, S.P.; Lockwood, E. A local instructional theory for the guided reinvention of the quotient group concept. J. Math. Behav. 2013, 32, 726–742. [Google Scholar] [CrossRef]
- Leppig, M. Beispiele zum Rechnen in endlichen Gruppen. Der Mathematikunterricht 1966, 2, 39–49. [Google Scholar]
- Machi, A. Groups. An Instruction to Ideas and Methods of the Theory of Groups, 1st ed.; Springer: Milano, Italy, 2012. [Google Scholar]
- Lienert, G.A.; Raatz, U. Testaufbau und Testanalyse, 6th ed.; Verlagsgruppe Beltz: Weinheim, Germany, 1998. [Google Scholar]
- Bauer, A. Cronbachs α im Kontext des Grundmodells der Klassischen Testtheorie und darüber Hinaus. Master’s Thesis, Ludwig-Maximilian-University, Munich, Germany, 2015. [Google Scholar]
- Coletta, V.P.; Steinert, J.J. Why normalized gain should continue to be used in analyzing preinstruction and postinstruction scores on concept inventories. Phys. Rev. Phys. Educ. Res. 2020, 16, 010108. [Google Scholar] [CrossRef]
- Finkenberg, F. Flipped Classroom im Physikunterricht; Logos Verlag Berlin: Berlin, Germany, 2018. [Google Scholar]
- Bitzenbauer, P. Quantenoptik an Schulen. Studie im Mixed-Methods Design zur Evaluation des Erlanger Unterrichtskonzepts zur Quantenoptik, 1st ed.; Logos Verlag Berlin: Berlin, Germany, 2020. [Google Scholar]
- Wilcoxon, F. Individual comparisons by ranking methods. Biometrics 1945, 1, 80–83. [Google Scholar] [CrossRef]
- Kruskal, W.H.; Wallis, W.A. Use of Ranks in One-Criterion Variance Analysis. J. Am. Stat. Assoc. 1952, 47, 260. [Google Scholar] [CrossRef]
- Douglas, C.E.; Michael, F.A. On Distribution-Free Multiple Comparisons in the One-Way Analysis of Variance. Commun. Stat.—Theory Methods 1991, 20, 127–139. [Google Scholar] [CrossRef]
- Rost, J. Allgemeine Standards für die Evaluationsforschung. In Handbuch Evaluation Psychologischer Interventionsmaßnahmen; Verlag Hans Huber: Bern, Switzerland, 2000; pp. 129–140. [Google Scholar]
- Zenger, T.; Bitzenbauer, P. Exploring German Secondary School Students’ Conceptual Knowledge of Density. Sci. Educ. Int. 2022, 33, 86–92. [Google Scholar] [CrossRef]
- Tirosh, D.; Hadass, R.; Movshovitz-Hadar, N. Overcoming overgeneralizations: The case of commutativity and associativity. In Proceedings of the Fifteenth Annual Conference of the International Group for Psychology of Mathematics Education, Assisi, Italy, 29 June–4 July 1991; pp. 310–315. [Google Scholar]
- Ubben, M.S.; Heusler, S. Gestalt and Functionality as Independent Dimensions of Mental Models in Science. Res. Sci. Educ. 2021, 51, 1349–1363. [Google Scholar] [CrossRef]
- Ubben, M.S.; Bitzenbauer, P. Two Cognitive Dimensions of Students’ Mental Models in Science: Fidelity of Gestalt and Functional Fidelty. Educ. Sci. 2022, 12, 163. [Google Scholar] [CrossRef]
- Rupnow, R.; Sassman, P. Sameness in algebra: Views of isomorphism and homomorphism. Educ. Stud. Math. 2022. [Google Scholar] [CrossRef]
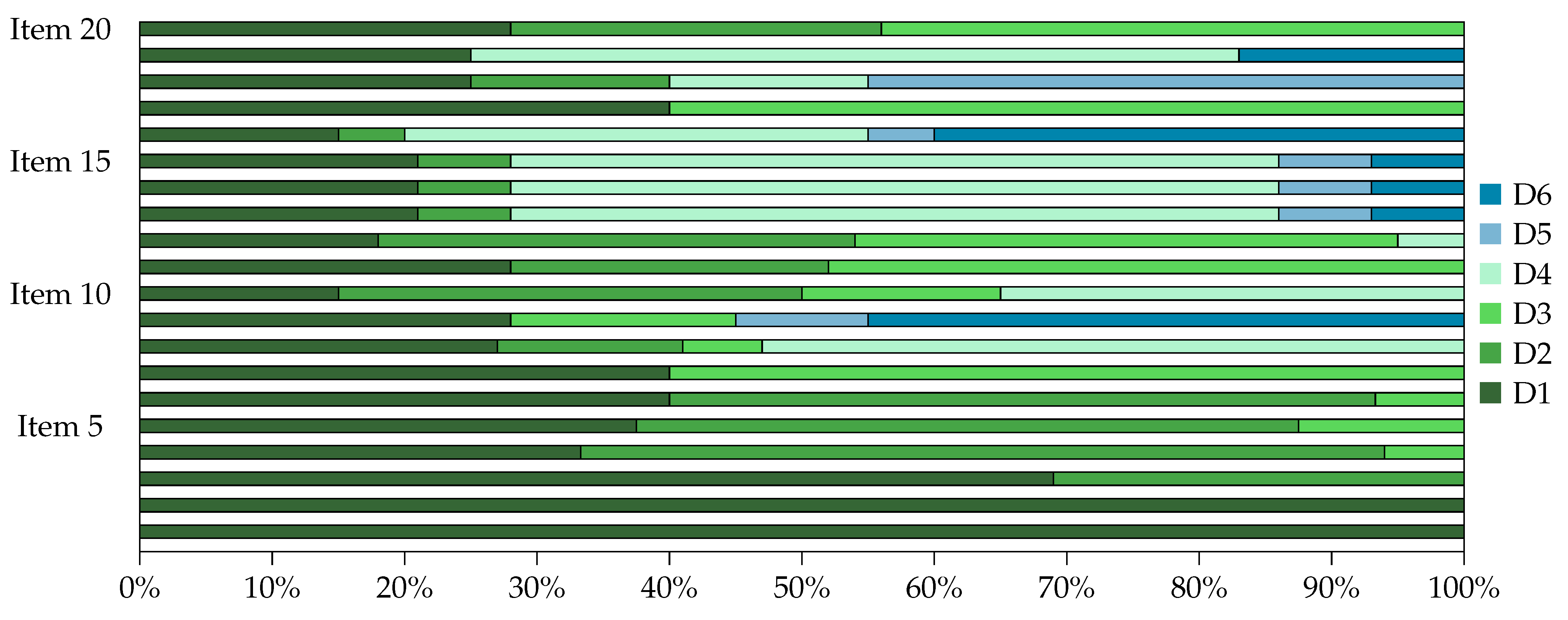

| Domain | Items | Length | Description | ||
|---|---|---|---|---|---|
| 1 | 1,2,3,7 | 4 | Definitional Fundamentals | 0.41 | 0.28 |
| 2 | 4,5,6,10,11,12,17,20 | 8 | Beginner Concepts | 0.48 | 0.54 |
| 3 | 8,9,13,14,15,16,18,19 | 8 | Intermediate Concepts | 0.48 | 0.50 |
| Low CRI () | High CRI () | |
|---|---|---|
| Correct Answer | Correct answer and low CRI Uncertainty of Knowledge | Correct answer and high CRI Knowledge of scientific concept |
| Wrong Answer | Wrong answer and low CRI Lack of knowledge | Wrong answer and high CRI Misconceptions |
| Wilcoxon Signed-Rank Test | |||
|---|---|---|---|
| pretest | , ; | ||
| post-test |
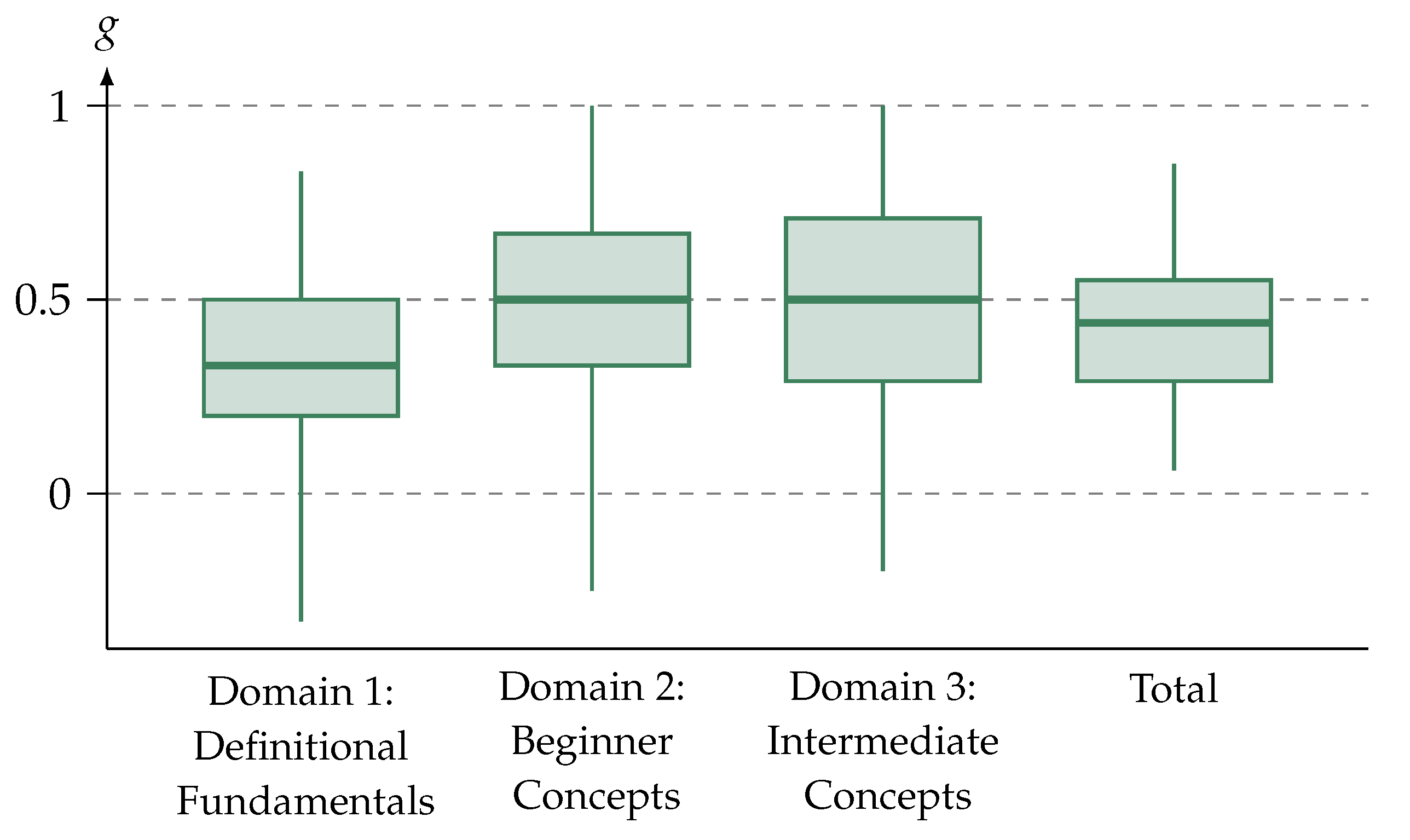
| Domain | Normalized Gain | ||
|---|---|---|---|
| Domain 1: Definitional Fundamentals | 0.37 | 0.26 | |
| (i.e., Naive set theory, binary operations, associativity, commutativity) | |||
| Domain 2: Beginner Concepts | 0.44 | 0.26 | |
| (i.e., Neutral element, inverses, Cayley Tables) | |||
| Domain 3: Intermediate Concepts | 0.47 | 0.26 | |
| (i.e., Dihedral groups, cyclical groups, isomorphisms) | |||
| Overall CIGT | 0.40 | 0.21 | |
| (i.e., all domains) |
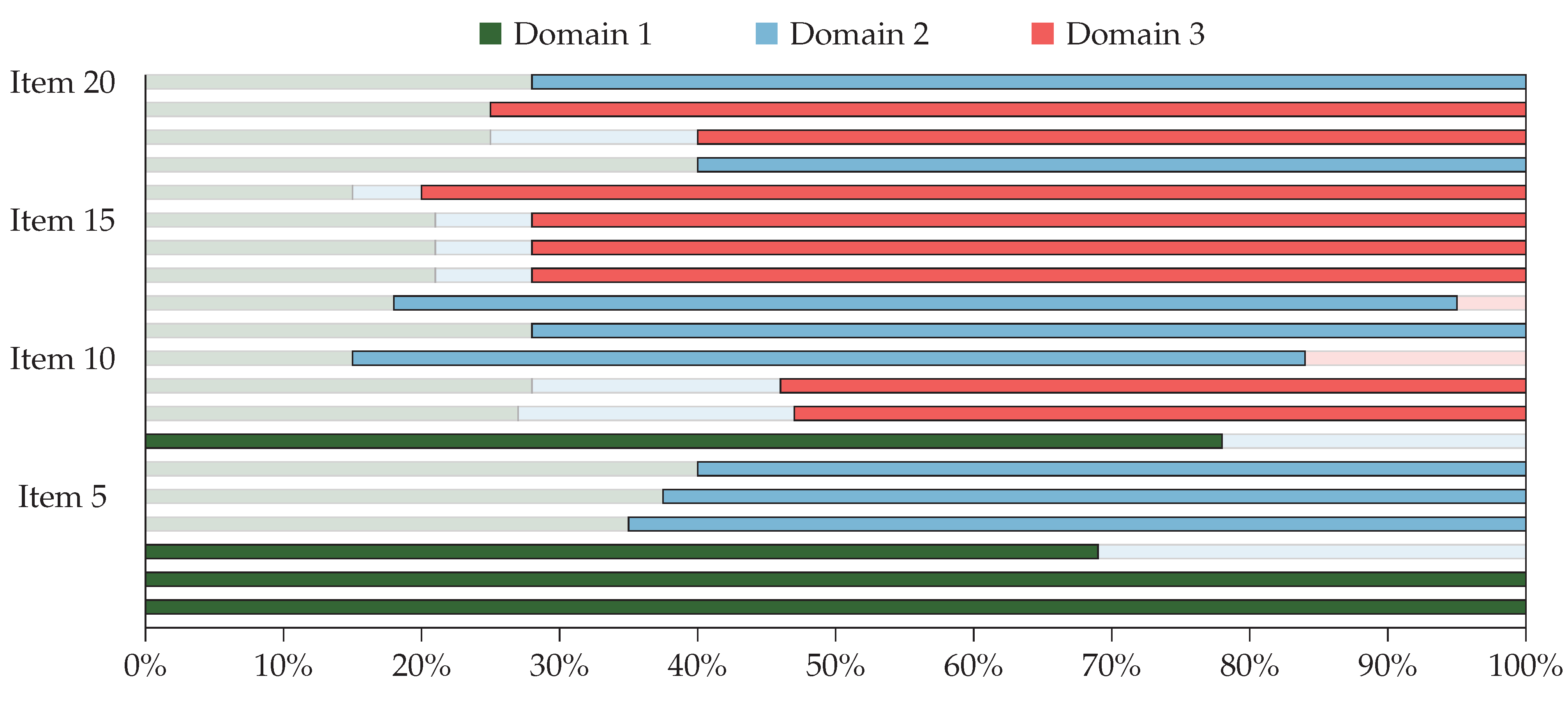
| Option 2 | Option 3 | |||||
|---|---|---|---|---|---|---|
| Item | tot. # | rel. # | tot. # | rel. # | ||
| 1 | 71 | 50% | 3.86 | 4 | 3% | 3.50 |
| 2 | 10 | 7% | 3.48 | 0 | 0% | 2.83 |
| 3 | 19 | 13% | 3.77 | 15 | 10% | 3.48 |
| 4 | 7 | 5% | 3.50 | 54 | 38% | 3.44 |
| 5 | 15 | 10% | 3.15 | 7 | 5% | 3.10 |
| 6 | 13 | 9% | 2.00 | 1 | 0% | 2.54 |
| 7 | 25 | 17% | 3.37 | 15 | 10% | 3.02 |
| 8 | 7 | 5% | 2.58 | 2 | 1% | 3.00 |
| 9 | 25 | 17% | 4.13 | 39 | 27% | 4.15 |
| 10 | 13 | 10% | 3.13 | 2 | 1% | 2.75 |
| 11 | 17 | 12% | 3.74 | 15 | 10% | 3.56 |
| 12 | 5 | 3% | 3.00 | 36 | 25% | 3.48 |
| 13 | 25 | 17% | 2.90 | 13 | 9% | 3.25 |
| 14 | 26 | 18% | 3.11 | 0 | 0% | 1.86 |
| 15 | 8 | 6% | 2.66 | 5 | 3% | 3.27 |
| 16 | 10 | 7% | 3.50 | 5 | 3% | 3.27 |
| 17 | 3 | 2% | 2.92 | 5 | 3% | 2.69 |
| 18 | 13 | 9% | 3.00 | 13 | 9% | 2.82 |
| 19 | 15 | 10% | 3.58 | 15 | 10% | 3.67 |
| 20 | 9 | 6% | 3.46 | 20 | 14% | 3.56 |
| Domain | Domain Description | Learning Difficulty | Item | Option 2 | Option 3 |
|---|---|---|---|---|---|
| 1 | Definitional Fundamentals (Naive set theory, associativity, commutativity, etc.) | Problems with associativity | 1 | 50% | – |
| 3 | 13% | 10% | |||
| 4 | – | 38% | |||
| 7 | 17% | 10% | |||
| 2 | Beginner Concepts (Neutral element, inverses, etc.) | Problems with inverses and the neutral element | 5 | 10% | – |
| 10 | 10% | – | |||
| 12 | – | 25% | |||
| 20 | – | 14% | |||
| 3 | Intermediate Concepts (Dihedral and cyclic groups, isomorphism, etc.) | Problems with visualizing abstract notions | 9 | 17% | 27% |
| 13 | 17% | – | |||
| 14 | 18% | – |
| ∘ | a | t | w | z |
| a | z | w | a | t |
| t | w | z | t | a |
| w | a | t | w | z |
| z | t | a | z | w |
| ∘ | a | b | c | ∘ | a | b | c | ∘ | a | b | c | ||
| a | a | c | b | a | c | a | b | a | c | a | b | ||
| b | c | a | b | b | b | c | a | b | a | b | c | ||
| c | b | b | a | c | a | b | c | c | b | c | a |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Veith, J.M.; Bitzenbauer, P.; Girnat, B. Exploring Learning Difficulties in Abstract Algebra: The Case of Group Theory. Educ. Sci. 2022, 12, 516. https://doi.org/10.3390/educsci12080516
Veith JM, Bitzenbauer P, Girnat B. Exploring Learning Difficulties in Abstract Algebra: The Case of Group Theory. Education Sciences. 2022; 12(8):516. https://doi.org/10.3390/educsci12080516
Chicago/Turabian StyleVeith, Joaquin M., Philipp Bitzenbauer, and Boris Girnat. 2022. "Exploring Learning Difficulties in Abstract Algebra: The Case of Group Theory" Education Sciences 12, no. 8: 516. https://doi.org/10.3390/educsci12080516
APA StyleVeith, J. M., Bitzenbauer, P., & Girnat, B. (2022). Exploring Learning Difficulties in Abstract Algebra: The Case of Group Theory. Education Sciences, 12(8), 516. https://doi.org/10.3390/educsci12080516






