Radiation Efficiency Enhancement of Graphene Plasmonic Devices Using Matching Circuits
Abstract
1. Introduction
2. Materials and Methods
2.1. Surface Conductivity
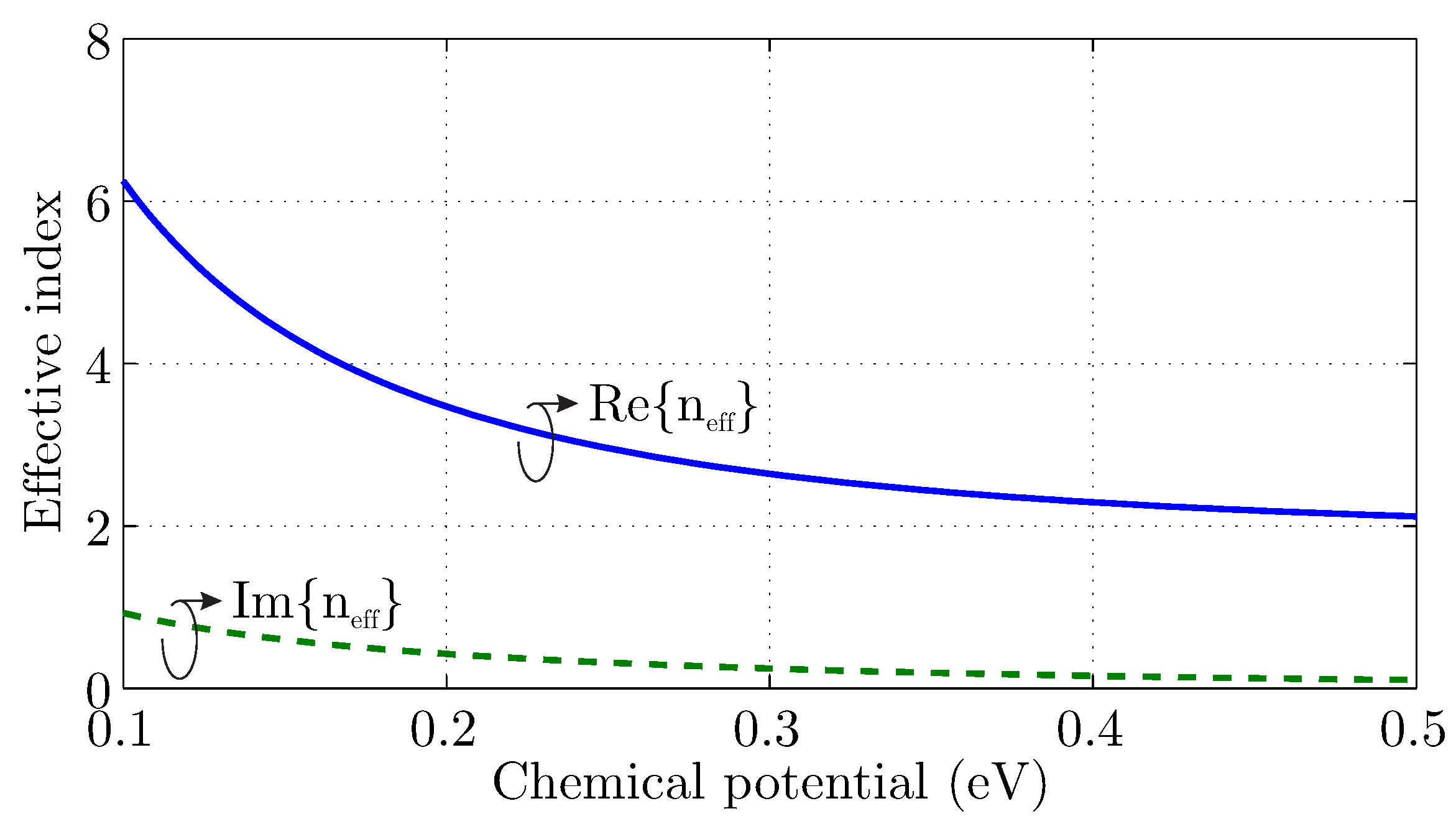
2.2. Surface Wave Propagation Properties
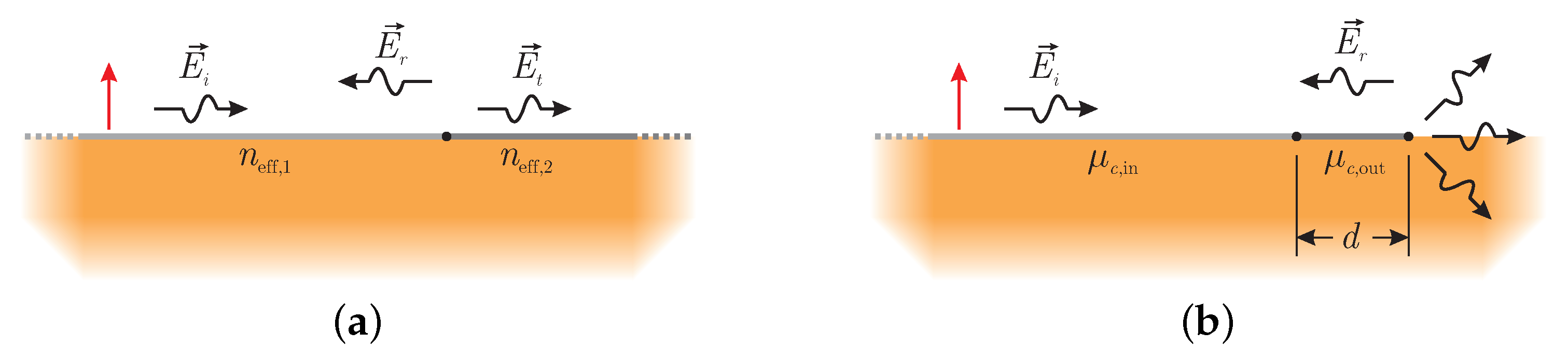
2.3. Reflection Due to Graphene Discontinuities
3. Results and Discussion
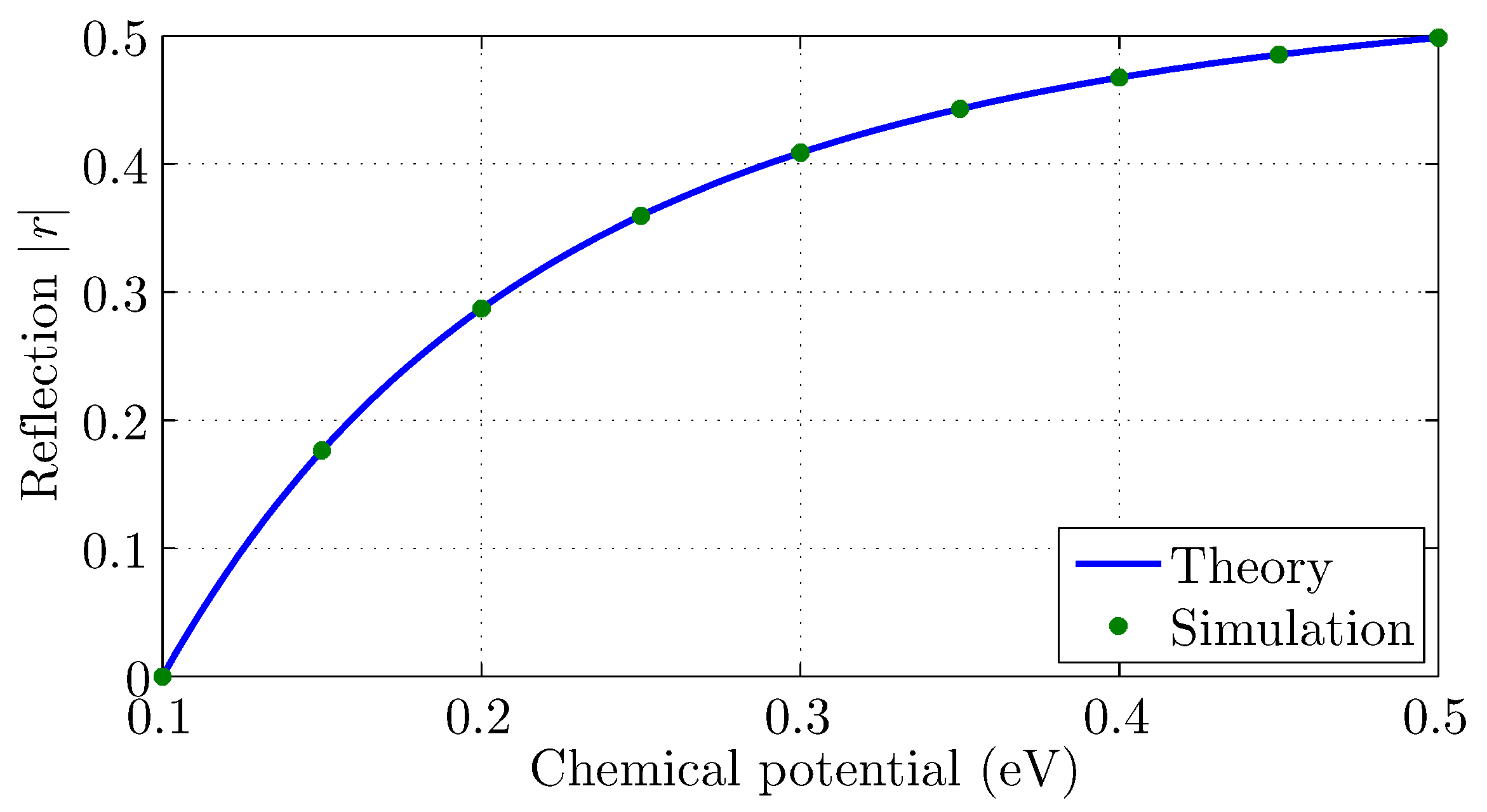
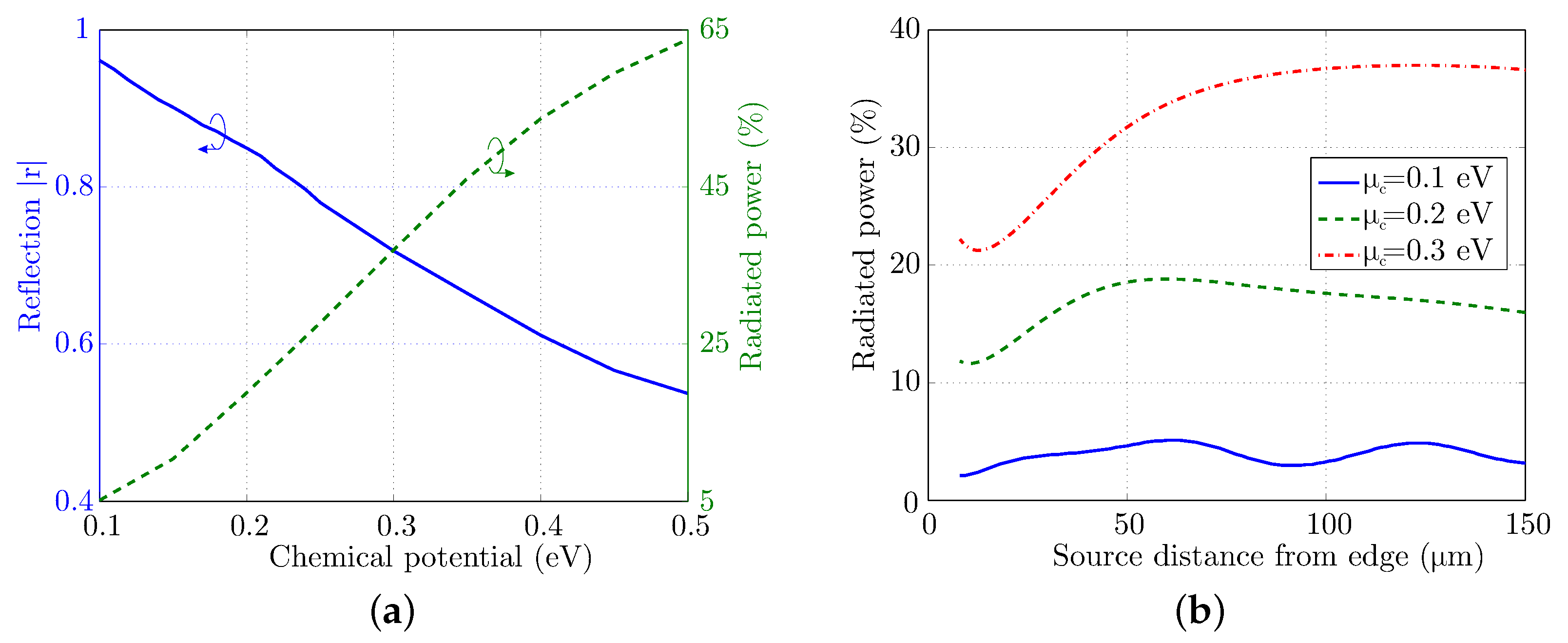
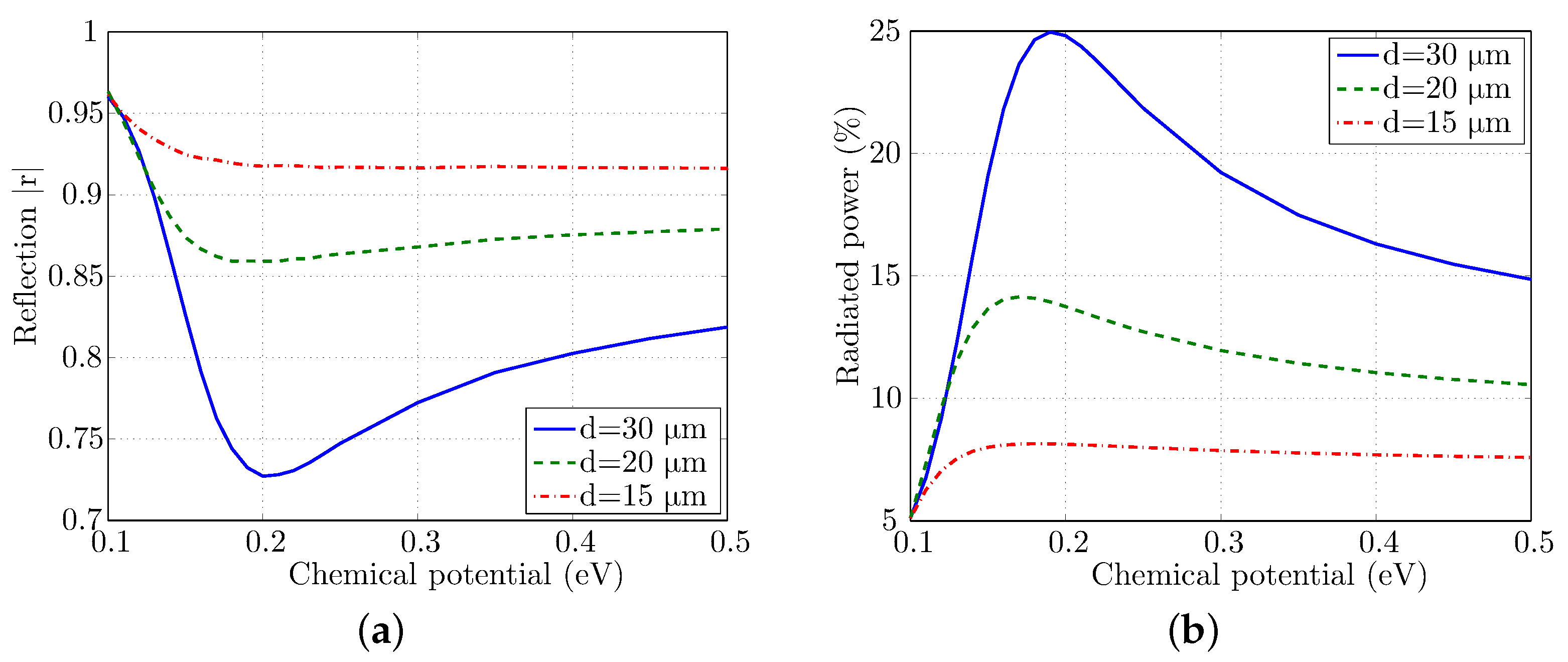
3.1. Surface Wave Reflection and Power Flow towards Free-Space
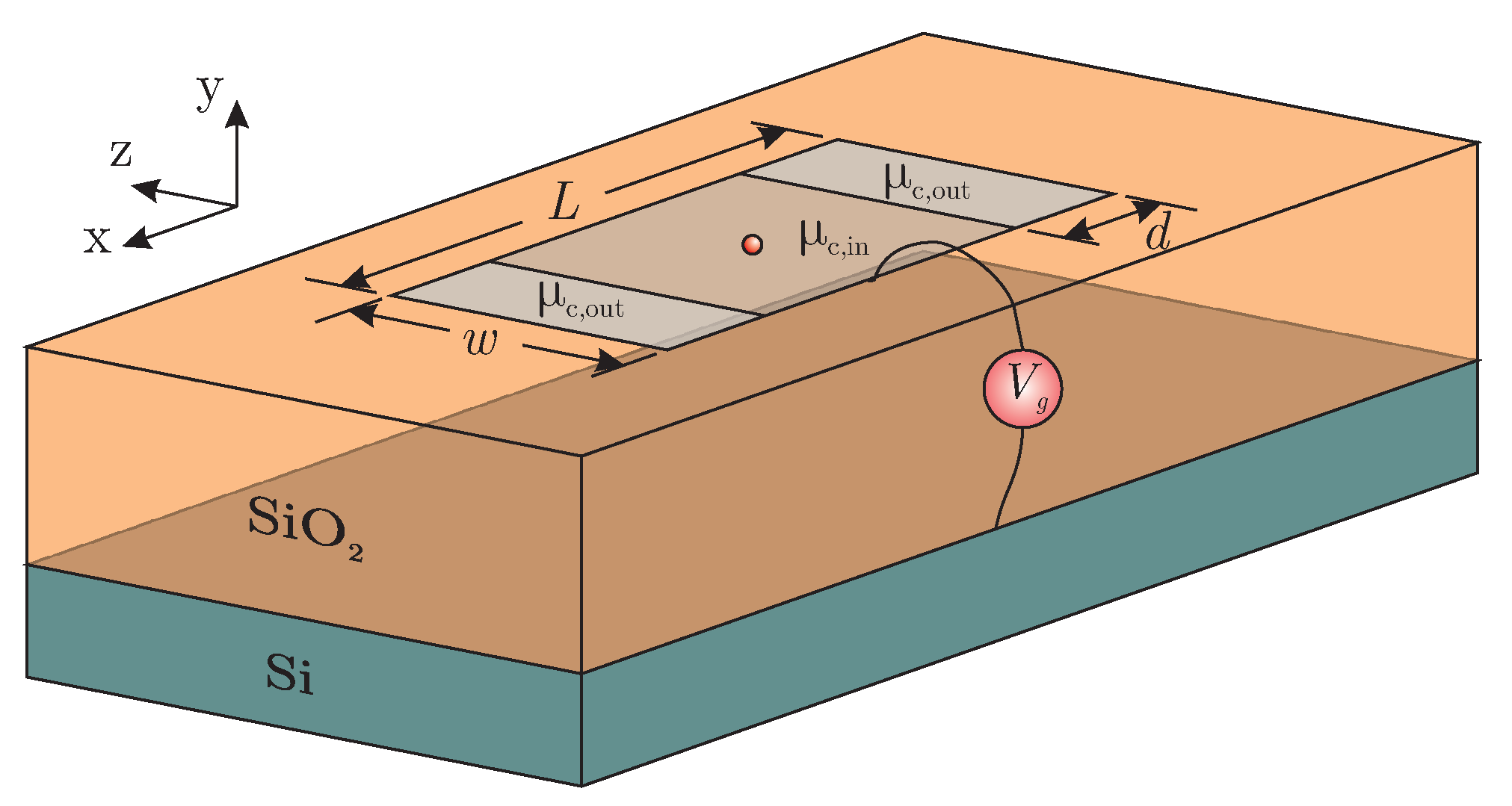
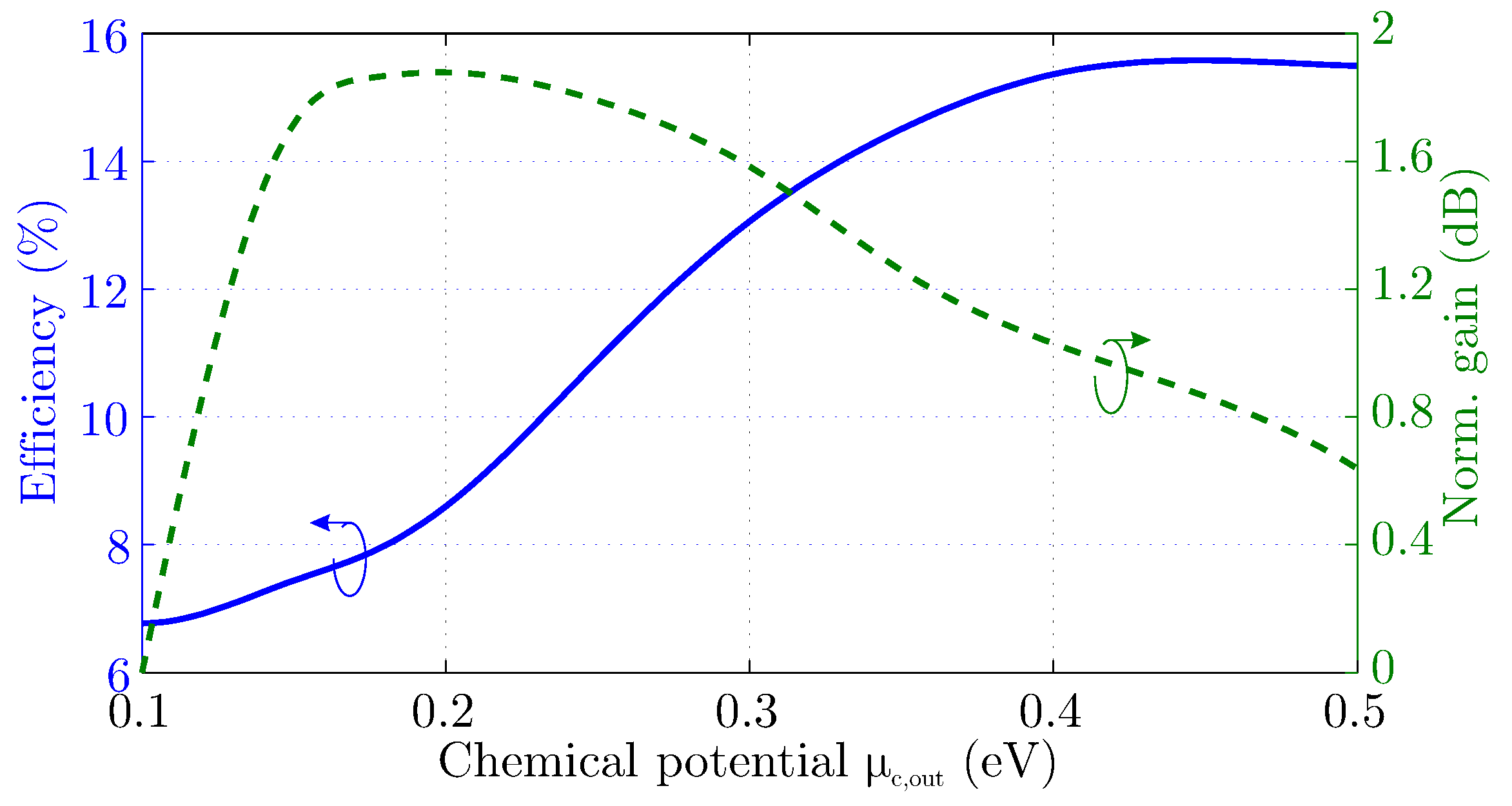
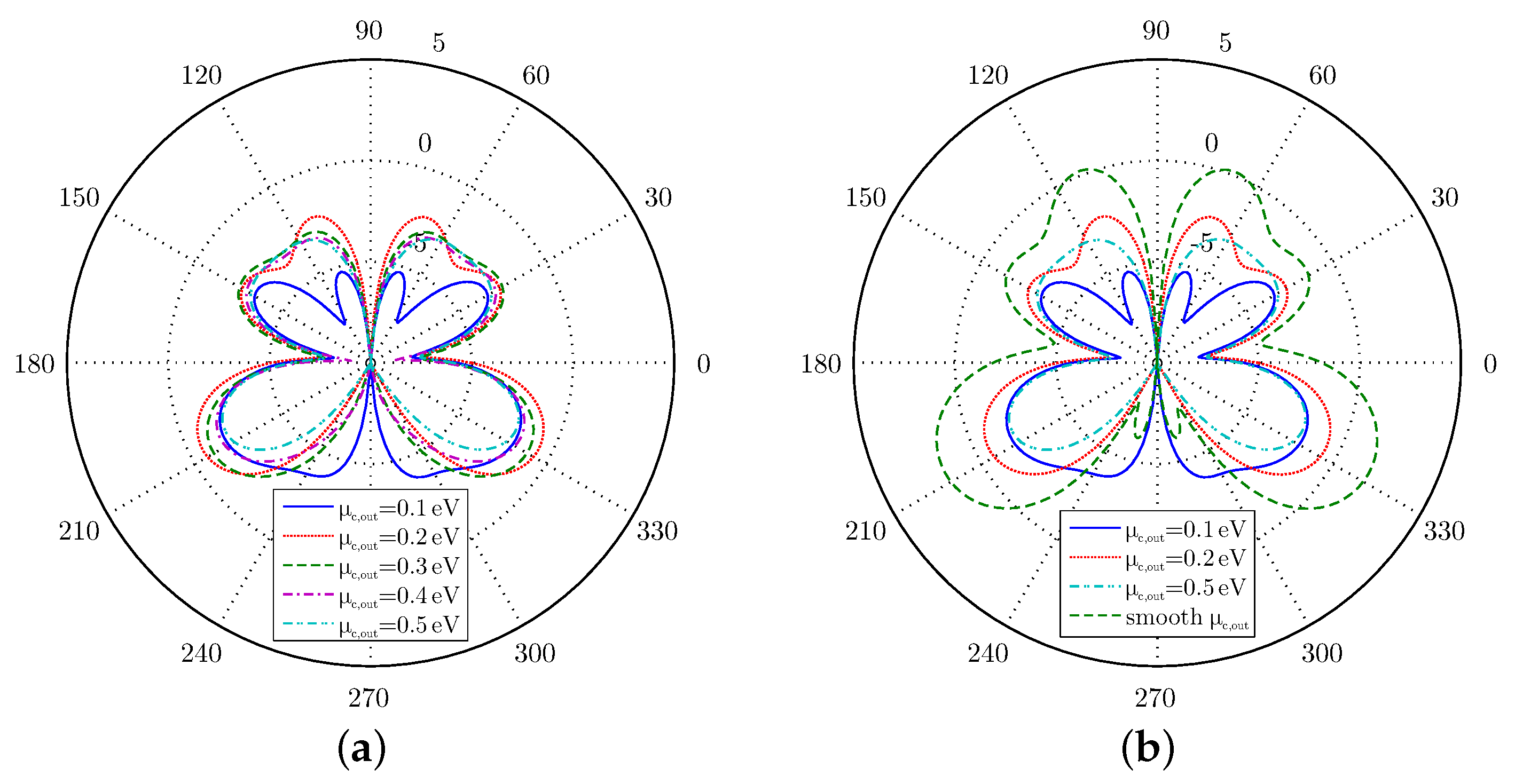
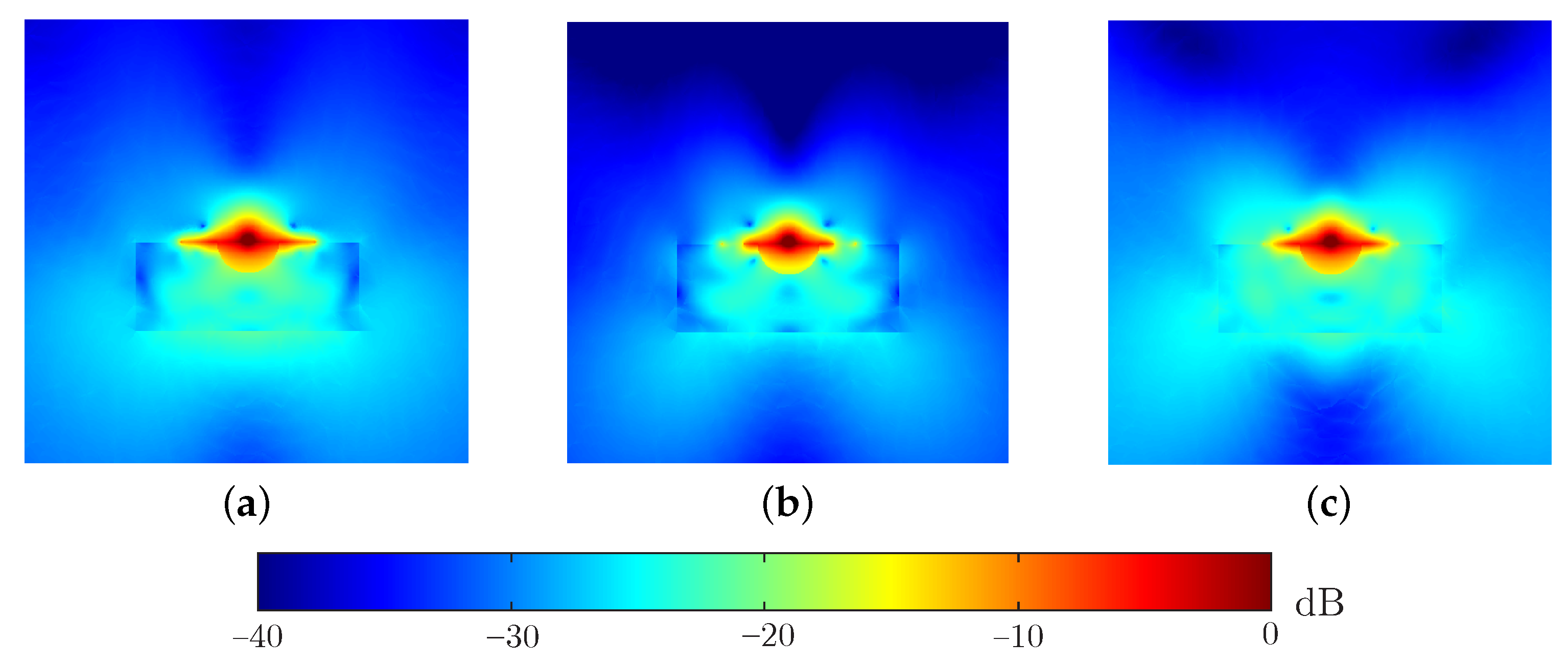
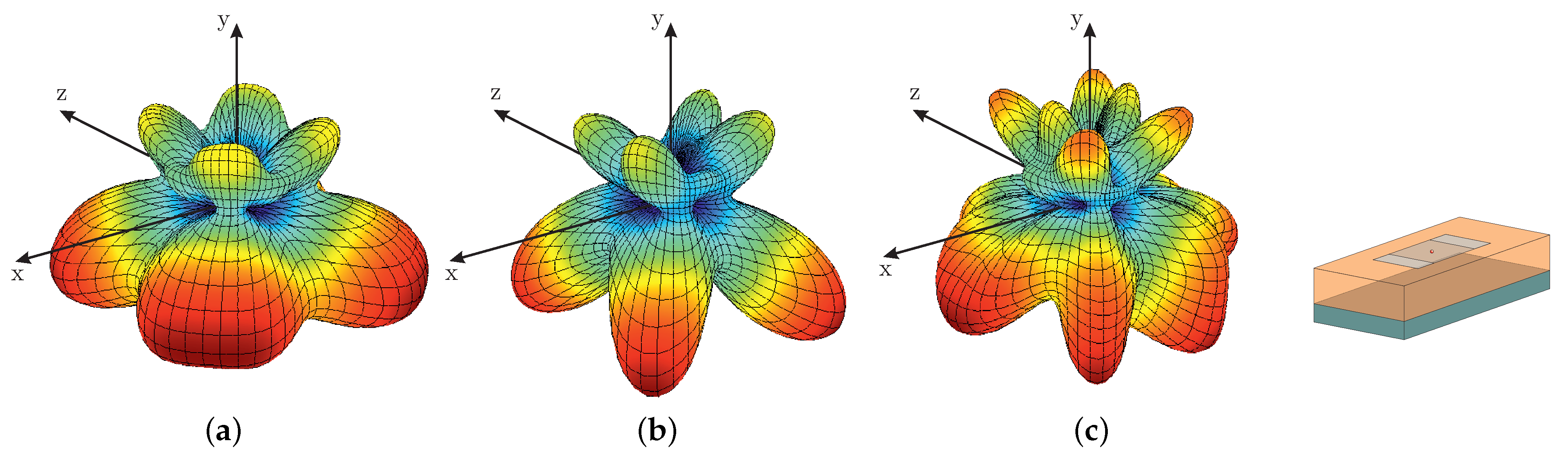
3.2. Performance Enhancement of Graphene Antenna
4. Conclusions
Author Contributions
Funding

Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Samaddar, P.; Son, Y.S.; Tsang, D.C.; Kim, K.H.; Kumar, S. Progress in graphene-based materials as superior media for sensing, sorption, and separation of gaseous pollutants. Coord. Chem. Rev. 2018, 368, 93–114. [Google Scholar] [CrossRef]
- Perreault, F.; De Faria, A.F.; Elimelech, M. Environmental applications of graphene-based nanomaterials. Chem. Soc. Rev. 2015, 44, 5861–5896. [Google Scholar] [CrossRef]
- Zhang, Y.; Nayak, T.R.; Hong, H.; Cai, W. Graphene: A versatile nanoplatform for biomedical applications. Nanoscale 2012, 4, 3833–3842. [Google Scholar] [CrossRef] [PubMed]
- Akyildiz, I.F.; Jornet, J.M. Electromagnetic wireless nanosensor networks. Nano Commun. Netw. 2010, 1, 3–19. [Google Scholar] [CrossRef]
- Geyi, W. Physical limitations of antenna. IEEE Trans. Antennas Propag. 2003, 51, 2116–2123. [Google Scholar] [CrossRef]
- Shahpari, M.; Thiel, D.V. The impact of reduced conductivity on the performance of wire antennas. IEEE Trans. Antennas Propag. 2015, 63, 4686–4692. [Google Scholar] [CrossRef]
- Novoselov, K.; Geim, A.K.; Morozov, S.; Jiang, D.; Zhang, Y.; Dubonos, S.; Grigorieva, I.; Firsov, A. Electric field effect in atomically thin carbon films. Science 2004, 306, 666–669. [Google Scholar] [CrossRef] [PubMed]
- Geim, A.; Novoselov, K. The rise of graphene. Nat. Mater. 2007, 6, 183–191. [Google Scholar] [CrossRef]
- Vakil, A.; Engheta, N. Transformation optics using graphene. Science 2011, 332, 1291–1294. [Google Scholar] [CrossRef]
- Mikhailov, S.A.; Ziegler, K. New electromagnetic mode in graphene. Phys. Rev. Lett. 2007, 99, 016803. [Google Scholar] [CrossRef]
- Hanson, G.W. Dyadic Green’s functions and guided surface waves for a surface conductivity model of graphene. J. Appl. Phys. 2008, 103, 064302. [Google Scholar] [CrossRef]
- Hwang, E.; Sarma, S.D. Dielectric function, screening, and plasmons in two-dimensional graphene. Phys. Rev. B 2007, 75, 205418. [Google Scholar] [CrossRef]
- Cen, C.; Zhang, Y.; Chen, X.; Yang, H.; Yi, Z.; Yao, W.; Tang, Y.; Yi, Y.; Wang, J.; Wu, P. A dual-band metamaterial absorber for graphene surface plasmon resonance at terahertz frequency. Phys.-Low-Dimens. Syst. Nanostruct. 2020, 117, 113840. [Google Scholar] [CrossRef]
- Wang, X.; Duan, J.; Chen, W.; Zhou, C.; Liu, T.; Xiao, S. Controlling light absorption of graphene at critical coupling through magnetic dipole quasi-bound states in the continuum resonance. Phys. Rev. B 2020, 102, 155432. [Google Scholar] [CrossRef]
- Xiao, S.; Liu, T.; Wang, X.; Liu, X.; Zhou, C. Tailoring the absorption bandwidth of graphene at critical coupling. Phys. Rev. B 2020, 102, 085410. [Google Scholar] [CrossRef]
- Cen, C.; Chen, Z.; Xu, D.; Jiang, L.; Chen, X.; Yi, Z.; Wu, P.; Li, G.; Yi, Y. High quality factor, high sensitivity metamaterial graphene—perfect absorber based on critical coupling theory and impedance matching. Nanomaterials 2020, 10, 95. [Google Scholar] [CrossRef]
- Liao, B.; Guo, X.; Hu, H.; Liu, N.; Chen, K.; Yang, X.; Dai, Q. Ultra-compact graphene plasmonic filter integrated in a waveguide. Chin. Phys. B 2018, 27, 094101. [Google Scholar] [CrossRef]
- Moazami, A.; Hashemi, M.; Shirazi, N.C. High efficiency tunable graphene-based plasmonic filter in the THz frequency range. Plasmonics 2019, 14, 359–363. [Google Scholar] [CrossRef]
- Bao, Q.; Zhang, H.; Wang, B.; Ni, Z.; Lim, C.H.Y.X.; Wang, Y.; Tang, D.Y.; Loh, K.P. Broadband graphene polarizer. Nat. Photonics 2011, 5, 411–415. [Google Scholar] [CrossRef]
- Zhang, X.; Liu, Z.; Zhang, Z.; Gao, E.; Luo, X.; Zhou, F.; Li, H.; Yi, Z. Polarization-sensitive triple plasmon-induced transparency with synchronous and asynchronous switching based on monolayer graphene metamaterials. Opt. Express 2020, 28, 36771–36783. [Google Scholar] [CrossRef]
- Luan, J.; Zheng, P.; Yang, H.; Hu, G.; Zhang, R.; Yun, B.; Cui, Y. A compact graphene modulator based on localized surface plasmon resonance with a chain of metal disks. Plasmonics 2019, 14, 1949–1954. [Google Scholar] [CrossRef]
- Zheng, Q.; Xia, L.; Tang, L.; Du, C.; Cui, H. Low Voltage Graphene-Based Amplitude Modulator for High Efficiency Terahertz Modulation. Nanomaterials 2020, 10, 585. [Google Scholar] [CrossRef] [PubMed]
- Dragoman, M.; Muller, A.; Dragoman, D.; Coccetti, F.; Plana, R. Terahertz antenna based on graphene. J. Appl. Phys. 2010, 107, 104313. [Google Scholar] [CrossRef]
- Tamagnone, M.; Gómez-Díaz, J.; Mosig, J.R.; Perruisseau-Carrier, J. Analysis and design of terahertz antennas based on plasmonic resonant graphene sheets. J. Appl. Phys. 2012, 112, 114915. [Google Scholar] [CrossRef]
- Llatser, I.; Kremers, C.; Cabellos-Aparicio, A.; Jornet, J.M.; Alarcón, E.; Chigrin, D.N. Graphene-based nano-patch antenna for terahertz radiation. Photonics Nanostruct. 2012, 10, 353–358. [Google Scholar] [CrossRef]
- Cabellos-Aparicio, A.; Llatser, I.; Alarcon, E.; Hsu, A.; Palacios, T. Use of terahertz photoconductive sources to characterize tunable graphene RF plasmonic antennas. IEEE Trans. Nanotechnol. 2015, 14, 390–396. [Google Scholar] [CrossRef]
- Dash, S.; Patnaik, A.; Kaushik, B.K. Performance enhancement of graphene plasmonic nanoantennas for THz communication. IET Microw. Antennas Propag. 2018, 13, 71–75. [Google Scholar] [CrossRef]
- Chen, S.J.; Fumeaux, C.; Tung, T.T.; Losic, D. High-efficiency microwave graphene antenna. In Proceedings of the 2017 IEEE International Symposium on Antennas and Propagation & USNC/URSI National Radio Science Meeting, San Diego, CA, USA, 9–14 July 2017; pp. 317–318. [Google Scholar]
- Alvarez, C.N.; Cheung, R.; Thompson, J.S. Performance analysis of hybrid metal–graphene frequency reconfigurable antennas in the microwave regime. IEEE Trans. Antennas Propag. 2017, 65, 1558–1569. [Google Scholar] [CrossRef]
- Amanatiadis, S.A.; Karamanos, T.D.; Kantartzis, N.V. Radiation efficiency enhancement of graphene THz antennas utilizing metamaterial substrates. IEEE Antennas Wirel. Propag. Lett. 2017, 16, 2054–2057. [Google Scholar] [CrossRef]
- Tamagnone, M.; Gomez-Diaz, J.S.; Mosig, J.R.; Perruisseau-Carrier, J. Reconfigurable terahertz plasmonic antenna concept using a graphene stack. Appl. Phys. Lett. 2012, 101, 214102. [Google Scholar] [CrossRef]
- Bouzianas, G.D.; Kantartzis, N.V.; Yioultsis, T.V.; Tsiboukis, T.D. Consistent study of graphene structures through the direct incorporation of surface conductivity. IEEE Trans. Magn. 2014, 50, 161–164. [Google Scholar] [CrossRef]
- Amanatiadis, S.A.; Zygiridis, T.T.; Kantartzis, N.V. Combining standard with optimised split-step finite-difference time-domain methods for the study of graphene configurations. IET Sci. Meas. Technol. 2019, 13, 1150–1157. [Google Scholar] [CrossRef]
- Gusynin, V.; Sharapov, S.; Carbotte, J. Magneto-optical conductivity in graphene. J. Phys. Condens. Matter 2006, 19, 026222. [Google Scholar] [CrossRef]
- Nikitin, A.Y.; Guinea, F.; García-Vidal, F.; Martín-Moreno, L. Edge and waveguide terahertz surface plasmon modes in graphene microribbons. Phys. Rev. B 2011, 84, 161407. [Google Scholar] [CrossRef]
- Depine, R.A. Graphene Optics: Electromagnetic Solution of Canonical Problems; Morgan & Claypool Publishers: San Rafael, CA, USA, 2017. [Google Scholar]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Amanatiadis, S.; Zygiridis, T.; Kantartzis, N. Radiation Efficiency Enhancement of Graphene Plasmonic Devices Using Matching Circuits. Technologies 2021, 9, 4. https://doi.org/10.3390/technologies9010004
Amanatiadis S, Zygiridis T, Kantartzis N. Radiation Efficiency Enhancement of Graphene Plasmonic Devices Using Matching Circuits. Technologies. 2021; 9(1):4. https://doi.org/10.3390/technologies9010004
Chicago/Turabian StyleAmanatiadis, Stamatios, Theodoros Zygiridis, and Nikolaos Kantartzis. 2021. "Radiation Efficiency Enhancement of Graphene Plasmonic Devices Using Matching Circuits" Technologies 9, no. 1: 4. https://doi.org/10.3390/technologies9010004
APA StyleAmanatiadis, S., Zygiridis, T., & Kantartzis, N. (2021). Radiation Efficiency Enhancement of Graphene Plasmonic Devices Using Matching Circuits. Technologies, 9(1), 4. https://doi.org/10.3390/technologies9010004




