Design of an Integrated Near-Field Communication and Wireless Power Transfer Coupler for Mobile Device Applications

Abstract

In this study, we propose a model that integrates a near-field communication (NFC) coupler and a wireless power transfer (WPT) coupler for mobile device applications. The NFC and WPT couplers were independently designed and then combined into a four-port NFC–WPT coupler. The proposed practical equivalent circuit (PEC) introduces a novel multi-port network representation, where inductive and capacitive coupling structures are modeled using T-model and Pi-model configurations, respectively. Based on this circuit model, we present a detailed theoretical approach for deriving a 4 × 4 S-parameter matrix by converting the transmission matrices of the partitioned circuit networks into S-parameters. The comparison between the theoretical analysis and the simulation results shows an error of less than 2.4%, which demonstrates the high accuracy of the proposed method.

1. Introduction

Wireless power transfer (WPT) technology enables wireless energy transmission through various methods, including inductive coupling-based inductive wireless power transfer (IPT) and capacitive coupling-based capacitive wireless power transfer (CPT) [1]. This technology has been extensively studied for application in devices requiring a power supply [2,3,4].

The IPT method utilizes a coil structure for inductive coupling, whereas the CPT method employs a metal plate structure for capacitive coupling. The IPT achieves high power transfer efficiency through strong magnetic coupling at close range; however, when metal plates are nearby, eddy currents are induced, leading to magnetic field distortion and variations in electrical characteristics such as the magnetic coupling coefficient and self-inductance [5]. Additionally, its effects on other devices and human exposure cannot be ignored [6,7]. In contrast, CPT is free from these issues [8], but it has limitations in terms of frequency characteristics and restrictions on the density of the electric field, which is the primary medium for energy transfer [1].

On the other hand, there is a near-field communication (NFC) system that utilizes the principles of WPT. It is an essential technology incorporated into mobile devices for short-range communication. NFC employs a coil-based magnetic resonance method to transfer power and transmit data at 13.56 MHz. Therefore, its power transfer mechanism is fundamentally similar to that of IPT. Since both WPT and NFC technologies must be integrated into a single device, research is being conducted to design systems with efficient structures [9,10].

There are two main approaches to integrating WPT and NFC; one method involves using a single coil for both technologies [11], while the other approach designs a single coil to support both WPT and NFC operations simultaneously [12]. However, such methods are highly sensitive to impedance variations and struggle to meet the wide bandwidth required by NFC, making simultaneous implementation challenging. Additionally, a fundamental limitation is that WPT and NFC cannot operate simultaneously on a single coil. Therefore, an alternative approach is to design WPT and NFC couplers independently and then combine them [13,14,15].

Previous research has explored hybrid structures by integrating NFC antennas with metal covers for smartphone applications [15]. In this design, the metal cover is utilized as a CPT coupler, while the NFC coil functions as an IPT coupler, following the principle of mixed-coupling wireless power transfer. However, this method is also unable to achieve simultaneous activation of both NFC and WPT in practical applications.

Therefore, as illustrated in Figure 1, designing WPT and NFC separately so that the transmitter and receiver of each system have distinct ports can potentially overcome the limitations of simultaneous activation. However, analyzing the coupling effects of couplers, including this approach, is a crucial step. In multi-port analysis, impedance matching circuits are typically not applied, eliminating the need for complex equivalent circuit model analysis [16,17,18,19,20,21]. Nevertheless, when additional circuits are introduced, a partitional circuit network (PCN) becomes essential to enable precise theoretical design.

Conventional coupling methods are typically configured as two-port networks, making it challenging to analyze mutual coupling factors, as they primarily rely on evaluating the overall impedance of the coupler. However, by developing a PCN for theoretical design, it is possible to accurately analyze the coupling effects and losses between couplers.

In this study, we propose a theoretical design for an NFC with a WPT coupler, which combines an NFC coupler resonating at 13.56 MHz with a WPT coupler resonating at 6.78 MHz. The integrated WPT system incorporates both IPT and CPT couplers. A practical equivalent circuit (PEC) model, including coupler loss resistance, is developed and validated by comparing it with 3D full-wave simulation results.

The PEC is formulated using a T-model to represent the transmission matrix, while the NFC coupler incorporates a ferrite material to examine the effects of magnetic interference. A high-efficiency coupler is designed to demonstrate its suitability for mobile device applications.

The key contributions of this study can be summarized as follows:

• An equivalent circuit model is proposed, in which all self and mutual coupling elements in a multi-port network are represented using unified T-model and Pi-model structures. This contrasts with conventional methods that describe each port independently, without fully expressing inter-port coupling.
• A formalized theoretical formulation is presented for converting the transmission matrix of the PCN in the practical equivalent circuit model of the NFC–IPT and NFC–CPT couplers into S-parameters.

2. Models of NFC–WPT Coupler

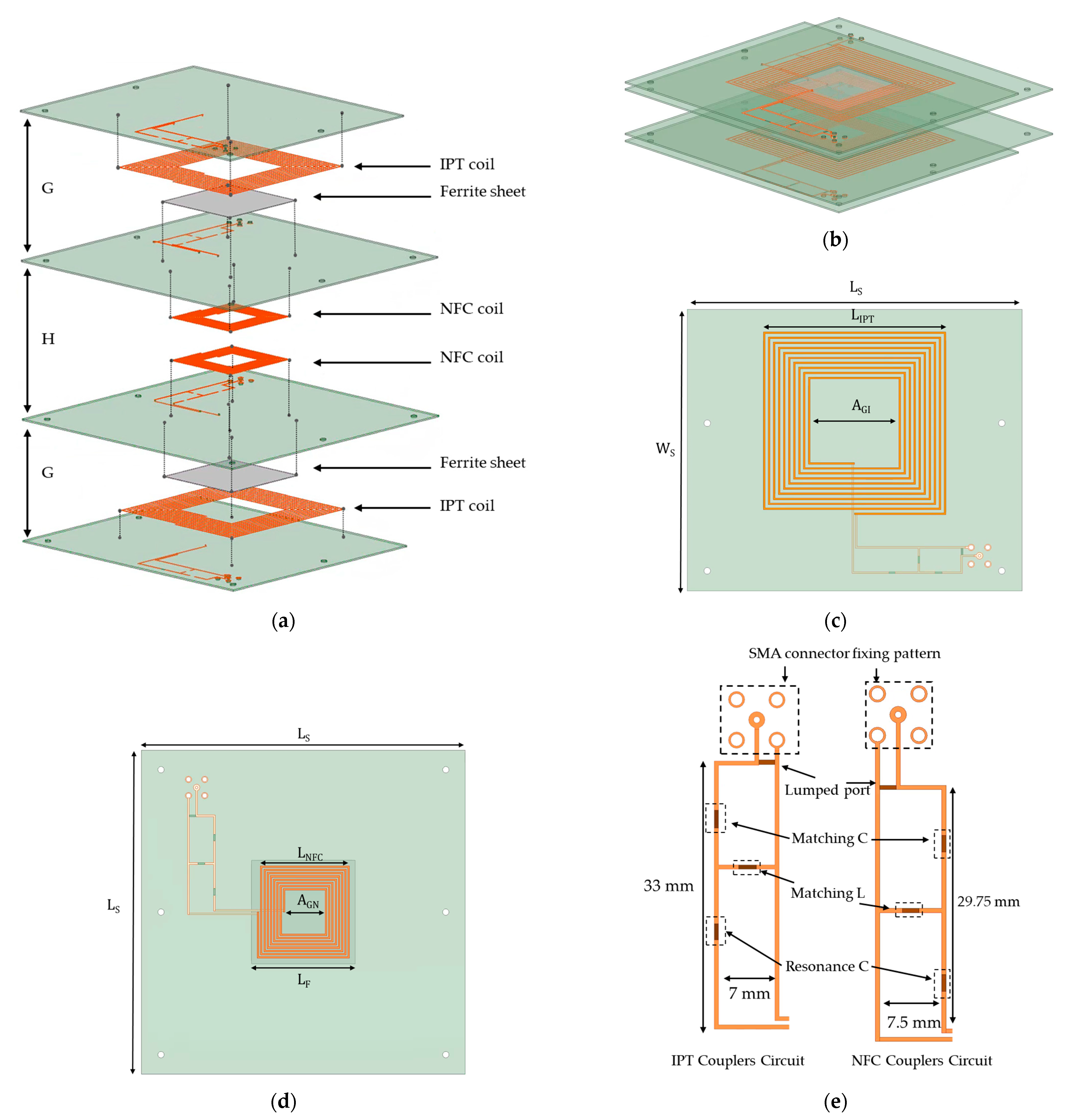
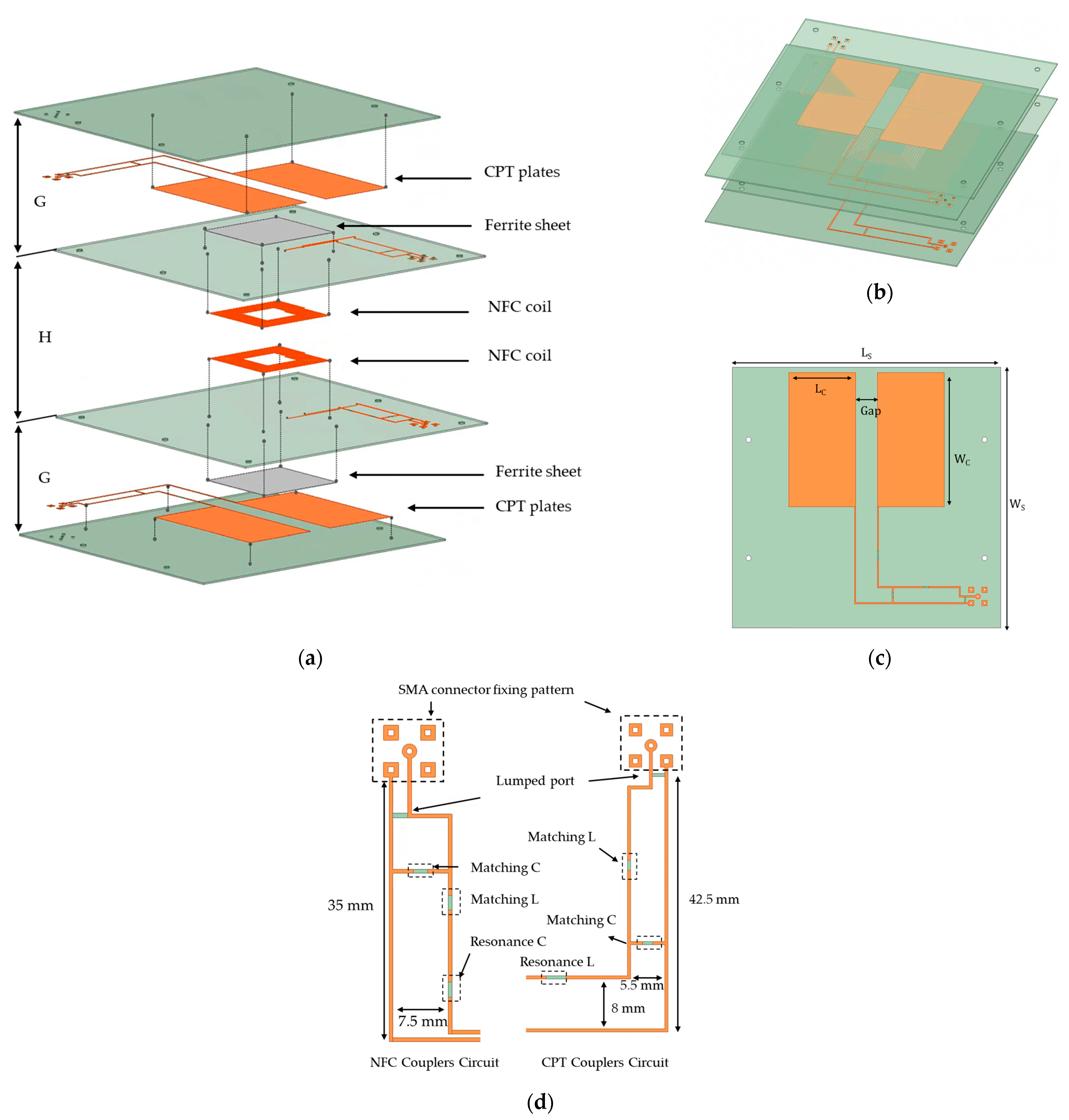
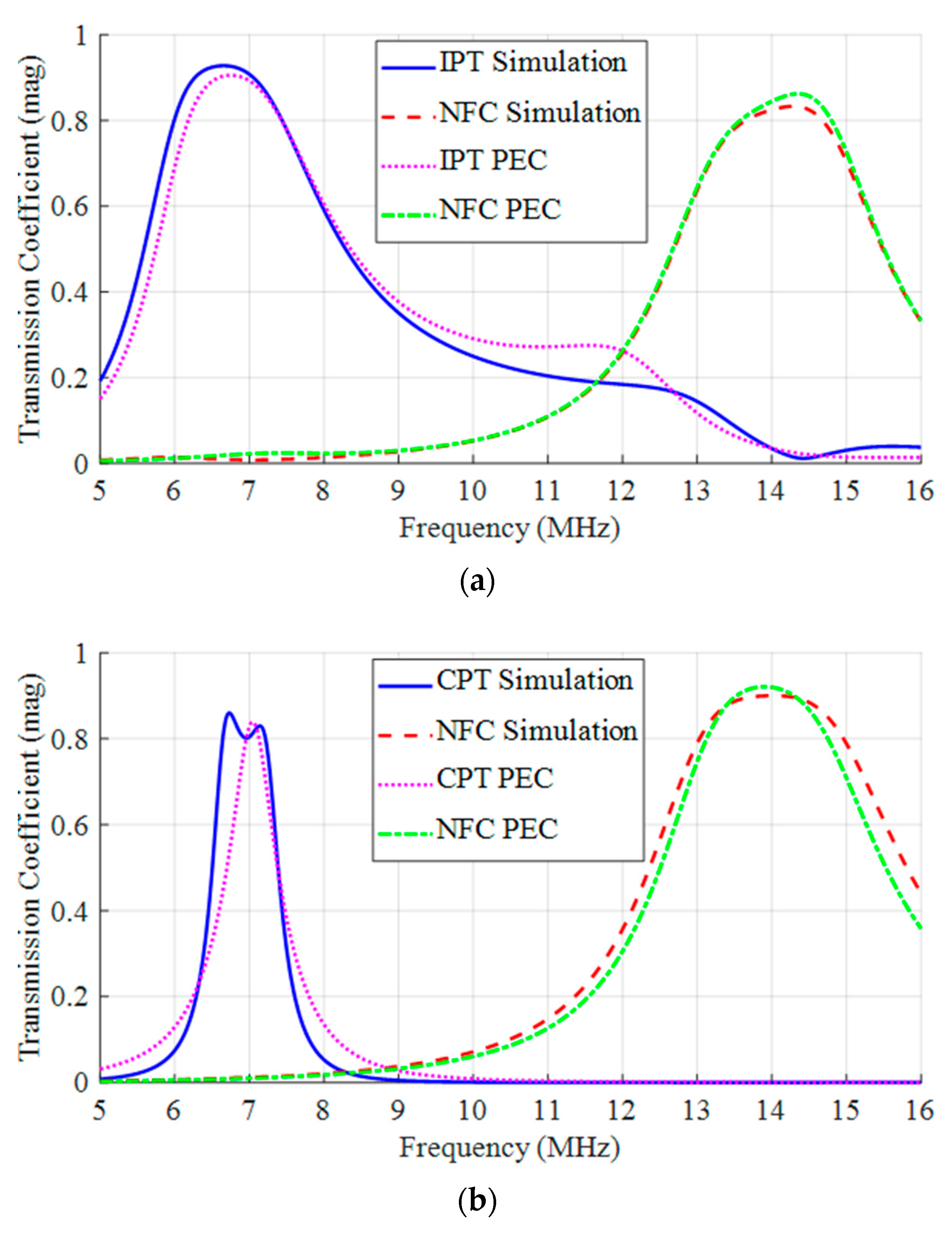
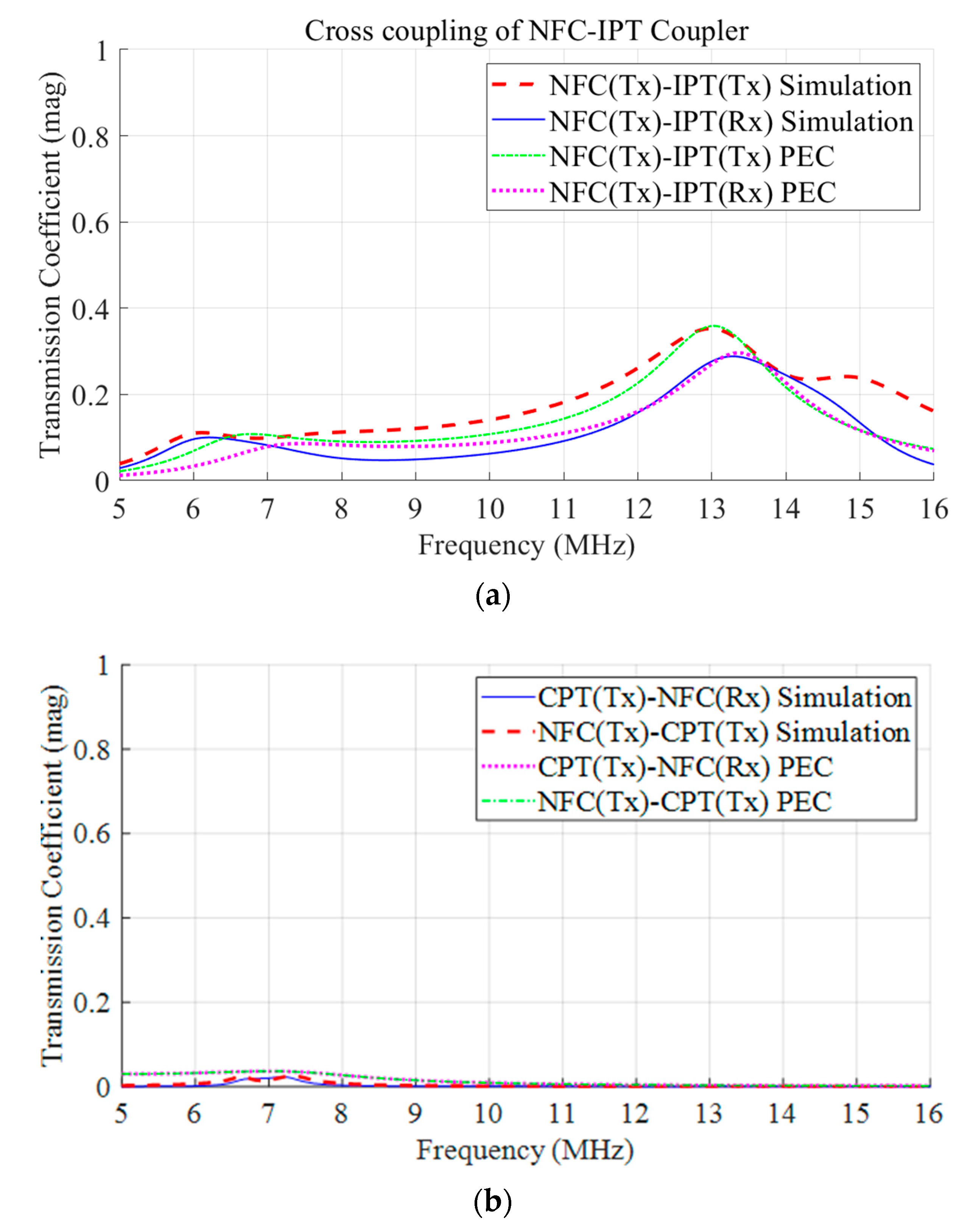
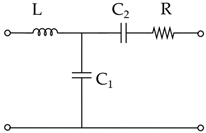
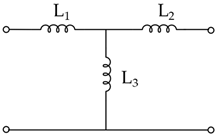
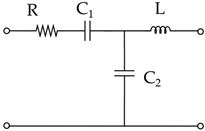
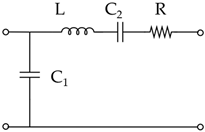
2.1. Theoretical Deisgn Method of PEC Circuit

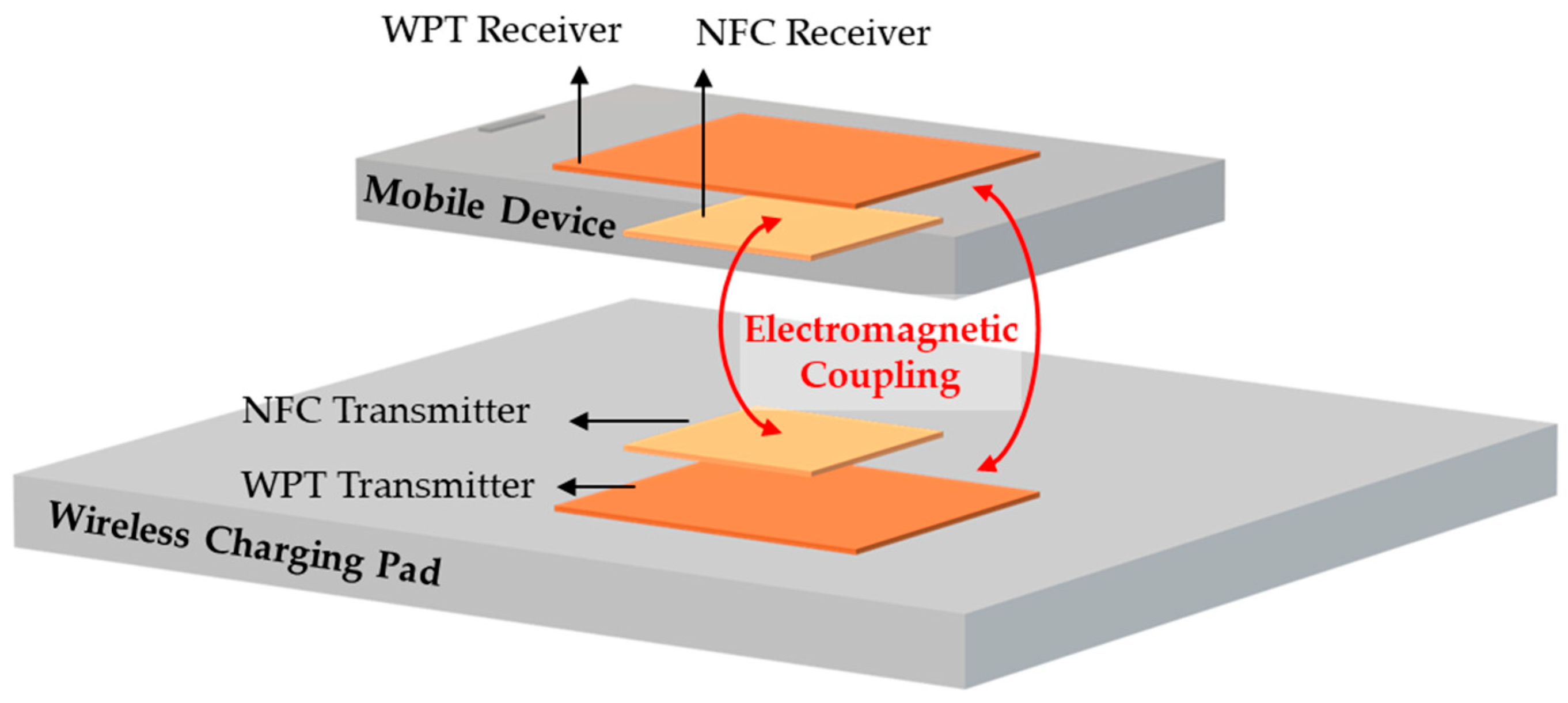
The configurations of the inductive power transfer (IPT) system, based on magnetic field coupling, and the capacitive power transfer (CPT) system, based on electric field coupling, are shown in Figure 2. In Figure 2a, an AC voltage source generates magnetic coupling between two coils, enabling power transfer in the IPT system. In contrast, in Figure 2b, an AC voltage source generates electric coupling between four metal plates, facilitating power transfer in the CPT system.

For these couplers, precise design of the impedance matching circuits for both the transmitter and receiver is essential to maximize coupling at the resonant frequency. In general, the term ‘coupler’ refers to a structure that includes the impedance matching circuit, resonant elements, and primary coupling components, such as coils or metal plates. Accurate equivalent circuit design and analysis at the coupler level are crucial, as they enable the quantification of interference and losses in system design through equivalent circuit parameters.

Therefore, a PEC for a four-port network incorporating loss resistance is designed for the NFC–IPT and NFC–CPT hybrid coupler model, and its S-parameters are calculated. Furthermore, previous studies have demonstrated that theoretical analysis based on the equivalent circuit model and electromagnetic simulation results exhibit high agreement with experimental validation [22]. Based on these findings, this study focuses on validating the PEC-based theoretical analysis by comparing it with electromagnetic simulation results obtained using ANSYS HFSS 2021R2, excluding experimental verification.

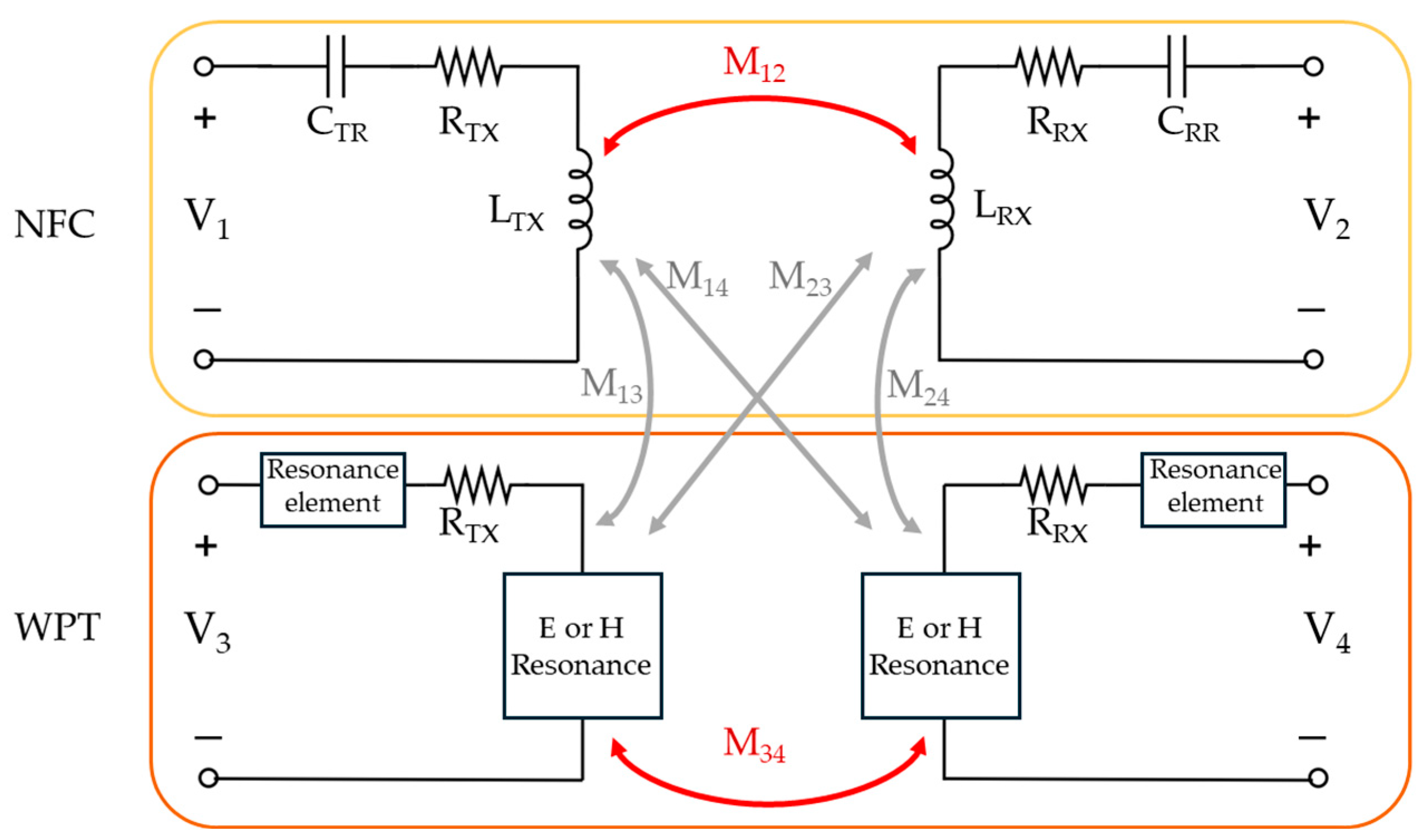
Figure 3 illustrates the link structure of the four-port coupler combining NFC and WPT. The WPT system can be implemented using either IPT or CPT and follows the MIMO WPT link principle [23]. In the NFC coupler, V1 and V3 represent the source voltages of NFC and WPT, respectively, while V2 and V4 correspond to the load voltages.

Since the four-port network has a total of six mutual terms, it consists of two main coupling terms and four cross-coupling terms. The main coupling in the NFC coupler is denoted as M12, while that in the WPT coupler is represented as M34. When the WPT system coupled with NFC operates in CPT mode, the resonant fields differ, meaning the cross-coupling terms M13, M14, M23, and M24 can be assumed to be nearly zero. Conversely, when the IPT coupler is applied, magnetic coupling occurs, potentially leading to cross-coupling with the NFC coupler. The equivalent circuit model proposed in this study explicitly represents this coupling effect.

In this study, the PEC of each coupler is theoretically designed and compared with 3D full-wave simulation results. Since the NFC and WPT couplers are combined, a 4 × 4 transmission matrix is derived for four-port network analysis, and the overall S-parameters are calculated. The PEC is structured by segmenting the PCN and defining the transmission matrix for each segment. By converting and multiplying the transmission matrices of each segment into S-parameters, the overall S-parameters of the coupler can be obtained.

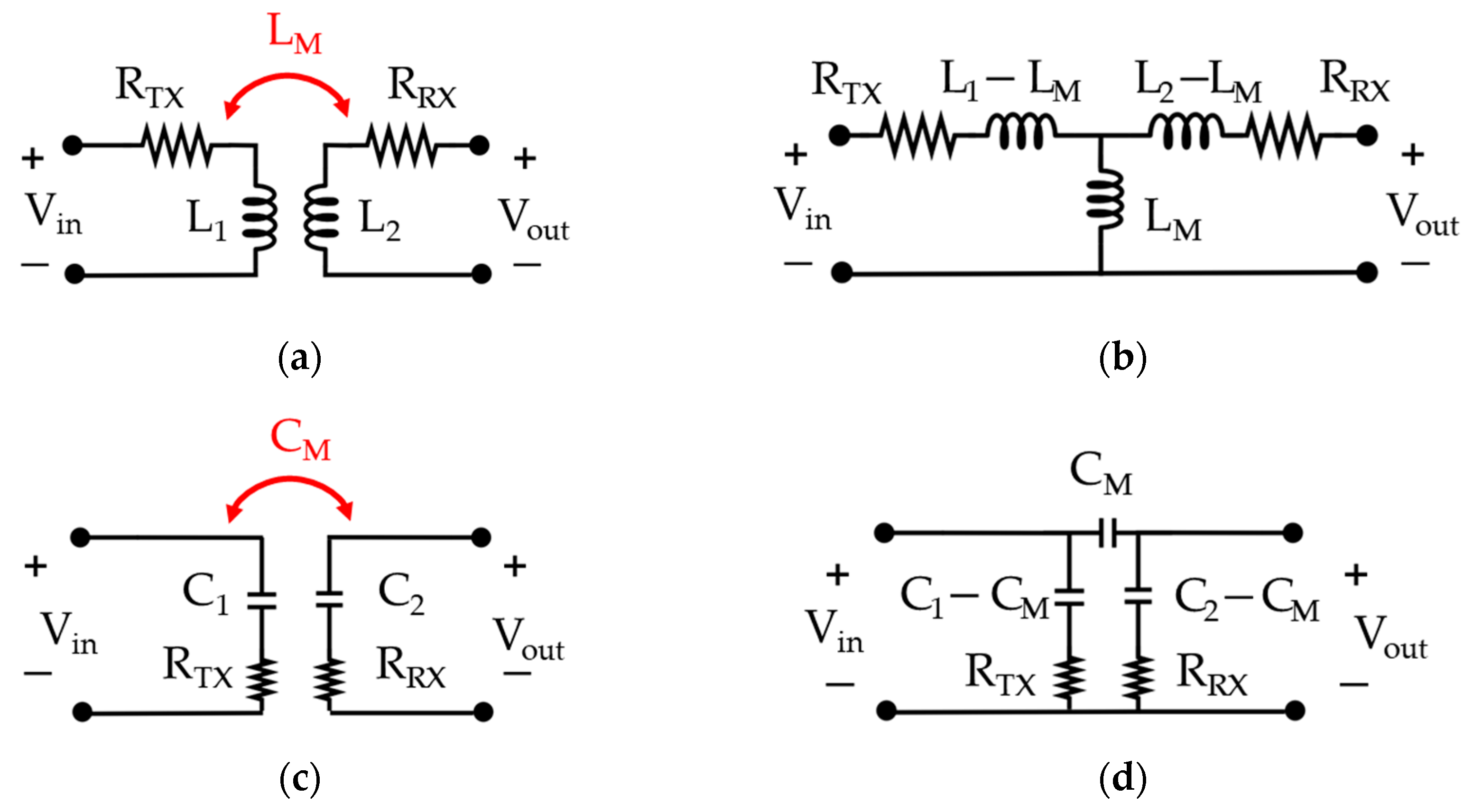
The structure of the PEC matrix varies depending on the coupling field and the matching circuit configuration of the coupler. In this study, the PEC matrix is classified into two types. Since IPT operates via magnetic coupling, the equivalent circuit of Figure 4a can be transformed into a T-model, as shown in Figure 4b, incorporating mutual terms. Meanwhile, the four-plate CPT coupler utilizes electric field coupling, and its equivalent circuit can be transformed from the structure in Figure 4c into a pi-model, as illustrated in Figure 4d. Additionally, all PEC models incorporate coupler loss resistance to ensure high accuracy in comparison with simulation results.

In the equivalent circuits of the two couplers proposed in this study, the PCNs exhibit several common structural patterns. Due to the redundancy in the transmission matrix formulations across all circuit networks, different types of networks are classified. The PCNs of the couplers are categorized accordingly, allowing each to be substituted into the corresponding transmission matrix equation for calculation.

2.2. PEC Model of NFC–IPT

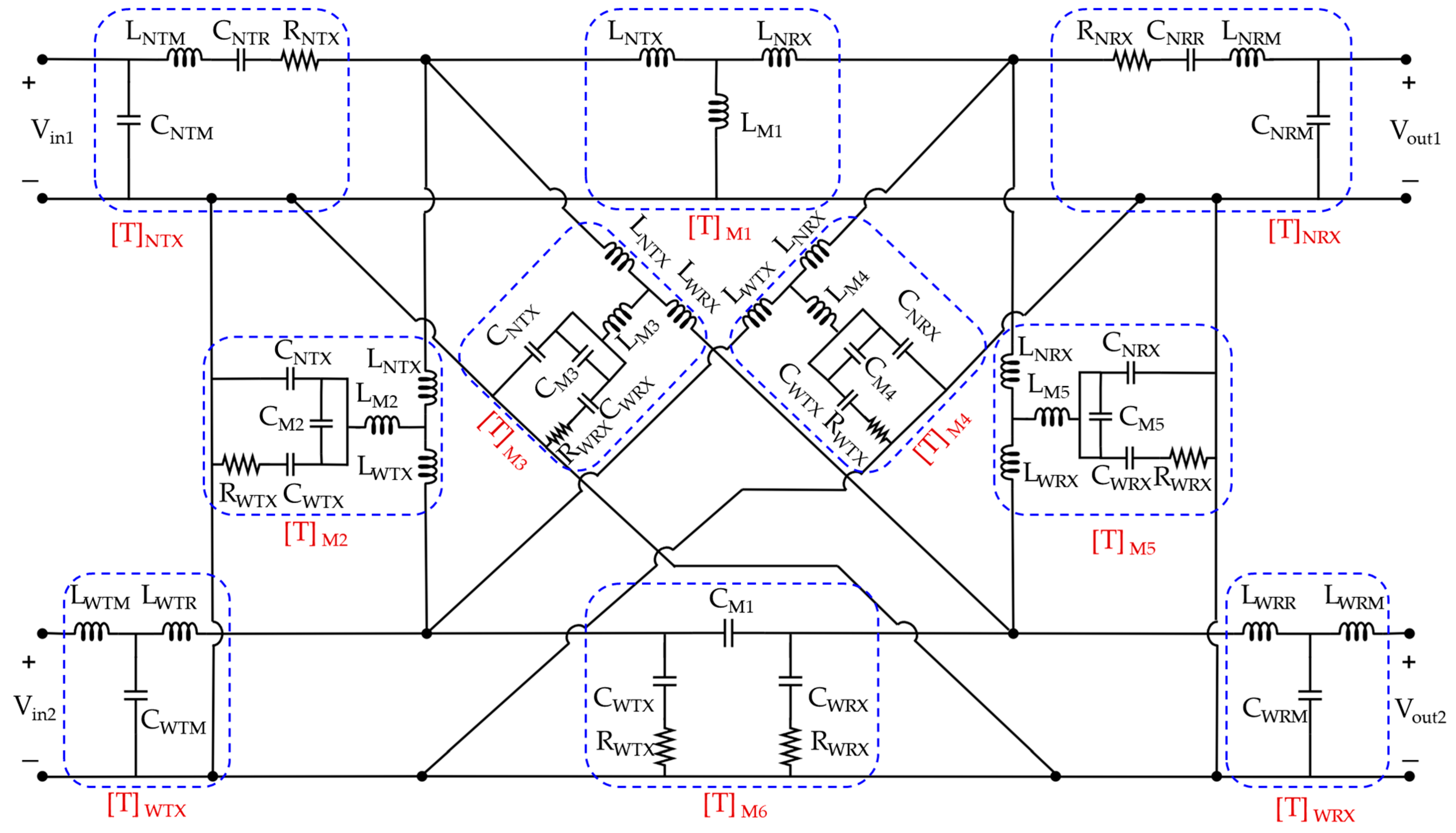
Figure 5 illustrates the four-port network equivalent circuit model combining NFC and IPT couplers. Since coupling occurs between coils, self-inductance and mutual inductance are represented using a T-model circuit. In the four-coil system, six mutual inductance components can be defined and are denoted as L_M1 to L_M6 in the equivalent circuit model. For example, the mutual inductance between the NFC transmitter and the IPT receiver is represented as L_M5.

The impedance matching circuits and resonant capacitors in the NFC transmitter and receiver, along with the inherent loss resistance of the coupler, are represented as [T]_NTX and [T]_NRX. The circuit network including the self-inductance and mutual inductance of the NFC transmitter and receiver is denoted as [T]_M1. Similarly, the circuit network representing the self-inductance and mutual inductance in the coupling between the NFC transmitter and the IPT coupler is expressed as [T]_M2.

The 4 × 4 S-parameters of the four-port network can be defined as a combination of 2 × 2 matrices obtained by decomposing Figure 5 into two-port network representations. The S-parameters of the PCNs calculated and multiplied to define the S-parameters of the two-port network as follows.

$$S_{TOTAL}=\left[\begin{array}{cc}\begin{array}{cc}{S}_{11}& S_{12}\\ S_{21}& S_{22}\end{array}& \begin{array}{cc}{S}_{13}& S_{14}\\ S_{23}& S_{24}\end{array}\\ \begin{array}{cc}{S}_{31}& S_{32}\\ S_{41}& S_{42}\end{array}& \begin{array}{cc}{S}_{33}& S_{34}\\ S_{43}& S_{44}\end{array}\end{array}\right]$$

(1)

$$\begin{gathered} \left[\begin{array}{cc}{S}_{11}& S_{12}\\ S_{21}& S_{22}\end{array}\right]={\left[S\right]}_{NTX}·{\left[S\right]}_{M1}·{\left[S\right]}_{NRX} \\ \left[\begin{array}{cc}{S}_{11}& S_{13}\\ S_{31}& S_{33}\end{array}\right]={\left[S\right]}_{NTX}·{\left[S\right]}_{M2}·{\left[S\right]}_{WTX} \\ \left[\begin{array}{cc}{S}_{11}& S_{14}\\ S_{41}& S_{44}\end{array}\right]={\left[S\right]}_{NTX}·{\left[S\right]}_{M5}·{\left[S\right]}_{WRX} \\ \left[\begin{array}{cc}{S}_{22}& S_{23}\\ S_{32}& S_{33}\end{array}\right]={\left[S\right]}_{NRX}·{\left[S\right]}_{M6}·{\left[S\right]}_{WRX} \\ \left[\begin{array}{cc}{S}_{22}& S_{24}\\ S_{42}& S_{44}\end{array}\right]={\left[S\right]}_{NRX}·{\left[S\right]}_{M4}·{\left[S\right]}_{WRX} \\ \left[\begin{array}{cc}{S}_{33}& S_{34}\\ S_{43}& S_{44}\end{array}\right]={\left[S\right]}_{WTX}·{\left[S\right]}_{M3}·{\left[S\right]}_{WRX} \end{gathered}$$

(2)

The parameters illustrated in Figure 5 are summarized in

Table 1. Equivalent parameters of NFC–IPT coupler.

Component	Notation	Value
Coupler self-inductance of NFC coupler	LNTX, LNRX	2.6 μH
Coupler self-inductance of IPT coupler	LWTX, LWRX	5.2 μH
Coupler intrinsic resistance	RNTX, RNRX, RNTX, RNRX	2.7 Ω
Mutual inductance of NFC coupler	LM1	0.2 μH
Mutual inductance of IPT coupler	LM3	1.2 μH
Mutual inductance of NFC IPT coupler	LM2, LM4, LM5, LM6	1 μH, 1 μH, 1.2 μH, 1.2 μH
Lumped C of NFC coupler	CNTR, CNRR,	50 pF
Lumped C of IPT coupler	CWTR, CWRR	96 pF
Impedance-matching L of NFC coupler	LNTM, LNRM,	100 nH
Impedance-matching L of IPT coupler	LWTM, LWRM	500 nH
Impedance-matching C of NFC coupler	CNTM, CNRM,	250 pF
Impedance-matching C of IPT coupler	CWTM, CWRM	200 pF

. To accurately represent self-inductance in the T-model circuit, it must be considered as the value obtained after subtracting mutual inductance, as shown in Figure 4b. In a four-port network, each self-inductance accounts for three mutual inductances.

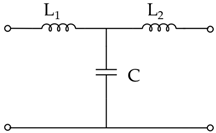
The PCNs of the NFC–IPT coupled system can be classified into Type A, Type B, and Type C, as summarized in

Table 2. PCN types and corresponding circuit labels of NFC–IPT coupler.

Circuit Type	Configuration of Circuit	PCN Types
Type A		[T]NTX, [T]WTX
Type B		[T]M1 to [T]M6
Type C		[T]NRX, [T]WRX

. Each classification corresponds to a specific circuit configuration, allowing for a systematic analysis of the coupler’s PCN.

For an N-port network’s T-model equivalent circuit, self-inductance (L_C) and mutual inductance (M_1j) can be conceptually defined as shown in Equation (3). The self-inductance values listed in the table represent ideal values that do not account for the mutual inductance between NFC and IPT. This relationship is incorporated into the S-parameter calculation process of the equivalent circuit model.

$$L_C=L_{self}-\sum _{j=N}{M}_{1j}$$

(3)

Using the transmission matrix conversion formulas for each circuit type in the table, the equation can be expressed as follows.

$$\left\{\begin{array}{c}\begin{array}{c}\begin{array}{c}\begin{array}{c}\begin{array}{l}{\left[T\right]}_{TypeA}=\left[\begin{array}{cc}1-{\omega }^{2}L{C}_1& \left({\displaystyle \frac{1}{j\omega C_2}}+R\right)\left(1-{\omega }^{2}L{C}_1\right)+j\omega L\\ j\omega C_1 & 1+{\displaystyle \frac{C_1}{C_2}}+j\omega C_{1}R\end{array}\right]\\ {\left[T\right]}_{TypeB}=\left[\begin{array}{cc}1-{\displaystyle \frac{L_1}{L_3}}& j\omega \left(L_1+L_2\left({\displaystyle \frac{1+L_1}{L_3}}\right)\right)\\ {\displaystyle \frac{1}{j\omega L_3}}& 1+{\displaystyle \frac{L_2}{L_3}}\end{array}\right]\\ {\left[T\right]}_{TypeC}=\left[\begin{array}{cc}1+{\displaystyle \frac{C_2}{C_1}}+j\omega C_{2}R& \left({\displaystyle \frac{1}{j\omega C_1}}+R\right)\left(1-{\omega }^{2}L{C}_2\right)+j\omega L\\ j\omega C_2 & 1-{\omega }^{2}L{C}_2\end{array}\right]\end{array}\end{array}\end{array}\end{array}\end{array}\right.$$

(4)

To convert the transmission matrix into S-parameters, ref. [24] proposed a method to simplify the complex equations through substitution. By defining the elements of the above matrix as t, the components of the S-parameters can be organized as shown in

Table 3. S-parameters of the NFC–IPT coupler for each PCN type.

Matrix	Parameter
${\left[S\right]}_{Type A}$	$\left\{\begin{array}{l}{\mathrm{t}}_{\mathrm{n}}=j\omega C_1\left(j\omega L-R\right)-{\displaystyle \frac{C_1}{C_2}}\\ {\mathrm{t}}_{\mathrm{m}}=2+j\omega C_1\left(j\omega L+R\right)+{\displaystyle \frac{C_1}{C_2}}\\ {\mathrm{t}}_{\mathrm{i}}={\displaystyle \frac{1}{Z_0}}\left(\left({\displaystyle \frac{1}{j\omega C_2}}+R\right)\left(1-{\omega }^{2}L{C}_1\right)+j\omega L\right)-Z_{0}j\omega C_1\\ {\mathrm{t}}_{\mathrm{j}}={\displaystyle \frac{1}{Z_0}}\left(\left({\displaystyle \frac{1}{j\omega C_2}}+R\right)\left(1-{\omega }^{2}L{C}_1\right)+j\omega L\right)+Z_{0}j\omega C_1\end{array}\right.$
${\left[S\right]}_{Type B}$	$\left\{\begin{array}{l}{\mathrm{t}}_{\mathrm{n}}=-{\displaystyle \frac{L_1+L_2}{L_3}}\\ {\mathrm{t}}_{\mathrm{m}}=2+{\displaystyle \frac{L_2-L_1}{L_3}}\\ {\mathrm{t}}_{\mathrm{i}}={\displaystyle \frac{1}{Z_0}}\left(j\omega \left(L_1+L_2\left({\displaystyle \frac{1+L_1}{L_3}}\right)\right)\right)-Z_0{\displaystyle \frac{1}{j\omega L_3}}\\ {\mathrm{t}}_{\mathrm{j}}={\displaystyle \frac{1}{Z_0}}\left(j\omega \left(L_1+L_2\left({\displaystyle \frac{1+L_1}{L_3}}\right)\right)\right)+Z_0{\displaystyle \frac{1}{j\omega L_3}}\end{array}\right.$
${\left[S\right]}_{Type C}$	$\left\{\begin{array}{l}{\mathrm{t}}_{\mathrm{n}}=j\omega C_2\left(R-j\omega L\right)+{\displaystyle \frac{C_2}{C_1}}\\ {\mathrm{t}}_{\mathrm{m}}=2+j\omega C_2\left(R+j\omega L\right)+{\displaystyle \frac{C_2}{C_1}}\\ {\mathrm{t}}_{\mathrm{i}}={\displaystyle \frac{1}{Z_0}}\left(\left({\displaystyle \frac{1}{j\omega C_1}}+R\right)\left(1-{\omega }^{2}L{C}_2\right)+j\omega L\right)-Z_{0}j\omega C_2\\ {\mathrm{t}}_{\mathrm{j}}={\displaystyle \frac{1}{Z_0}}\left(\left({\displaystyle \frac{1}{j\omega C_1}}+R\right)\left(1-{\omega }^{2}L{C}_2\right)+j\omega L\right)+Z_{0}j\omega C_2\end{array}\right.$

. Each term is derived from equations used for substitution, and the S-parameters can be obtained by referring to Equation (5) of the referenced study.

2.3. PEC Model of NFC–CPT

Figure 6 illustrates the four-port network equivalent circuit model combining NFC and CPT couplers. The detailed parameters are summarized in

Table 4. Equivalent parameters of NFC–CPT coupler.

Component	Notation	Value
Coupler self-inductance of NFC coupler	LNTX, LNRX	2.6 μH
Coupler self-capacitance of CPT coupler	CWTX, CWRX	1 pF
Coupler intrinsic resistance	RNTX, RNRX, RNTX, RNRX	1 Ω
Mutual inductance of NFC coupler	LM1	0.2
Mutual capacitance of CPT coupler	CM1	1.9 pF
Mutual inductance of NFC–CPT coupler	LM2, LM4, LM5, LM6	0.0005 nH
Mutual capacitance of NFC–CPT coupler	CM2, CM3, CM4, CM5	0.1 pF
Lumped C of NFC coupler	CNTR, CNRR,	47 pF
Lumped L of CPT coupler	LWTR, LWRR	180 μH
Impedance-matching L of NFC coupler	LNTM, LNRM,	150 nH
Impedance-matching L of CPT coupler	LWTM, LWRM	3.05 μH
Impedance-matching C of NFC coupler	CNTM, CNRM,	150 pF
Impedance-matching C of CPT coupler	CWTM, CWRM	160 pF

. Since the NFC transmitter and receiver are coupled through coils, self-inductance and mutual inductance can be represented using a T-model circuit. However, when the NFC transmitter is coupled with the CPT transmitter or receiver, the interaction between coils and metal plates must be considered. The coupling strength between magnetic and electric field components is generally close to zero.

Therefore, in the equivalent circuit model, the matrices for the circuit networks corresponding to [T]_M2 through [T]_M5 are not explicitly defined but are calculated accordingly. Additionally, the CPT coupler’s self-capacitance, mutual capacitance, and coupler loss resistance are included in a separate network, denoted as [T]_M6.

Similar to the NFC–IPT coupler, the 4 × 4 S-parameters can be derived by defining the S-parameters of the two-port networks as follows. While the equivalent circuit model and calculation equations remain the same as those for the NFC–IPT coupler, it is important to note that the numbering of the coupled networks differs.

$$\begin{gathered} \left[\begin{array}{cc}{S}_{11}& S_{12}\\ S_{21}& S_{22}\end{array}\right]={\left[S\right]}_{NTX}·{\left[S\right]}_{M1}·{\left[S\right]}_{NRX} \\ \left[\begin{array}{cc}{S}_{11}& S_{13}\\ S_{31}& S_{33}\end{array}\right]={\left[S\right]}_{NTX}·{\left[S\right]}_{M2}·{\left[S\right]}_{WTX} \\ \left[\begin{array}{cc}{S}_{11}& S_{14}\\ S_{41}& S_{44}\end{array}\right]={\left[S\right]}_{NTX}·{\left[S\right]}_{M3}·{\left[S\right]}_{WRX} \\ \left[\begin{array}{cc}{S}_{22}& S_{23}\\ S_{32}& S_{33}\end{array}\right]={\left[S\right]}_{NRX}·{\left[S\right]}_{M4}·{\left[S\right]}_{WRX} \\ \left[\begin{array}{cc}{S}_{22}& S_{24}\\ S_{42}& S_{44}\end{array}\right]={\left[S\right]}_{NRX}·{\left[S\right]}_{M5}·{\left[S\right]}_{WRX} \\ \left[\begin{array}{cc}{S}_{33}& S_{34}\\ S_{43}& S_{44}\end{array}\right]={\left[S\right]}_{WTX}·{\left[S\right]}_{M6}·{\left[S\right]}_{WRX} \end{gathered}$$

(5)

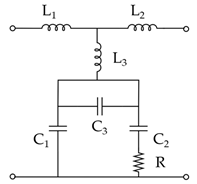
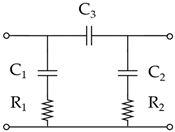
The PCNs of the NFC and CPT combined coupler can be classified into Type D, Type E, Type F, and Type G, as shown in

Table 5. PCN types and corresponding circuit labels of NFC–CPT coupler.

Circuit Type	Configuration of Circuit	PCN Types
Type D		[T]NTX, [T]NRX
Type E		[T]WTX, [T]WRX
Type F		[T]M2 to [T]M5
Type G		[T]M6

. Each classification corresponds to a specific circuit configuration, allowing for an analysis of the coupler’s PCN. Among them, Type F, which is an uncommon configuration, can be calculated as the sum of Type B and Type G. Type F represents a circuit designed to simultaneously express self-inductance and self-capacitance in the coupling between coils and metal plates. This circuit can be referenced in studies on hybrid wireless power transfer couplers that combine IPT and CPT designs [25].

Since the coupler’s loss resistance of the coil has already been considered in the impedance matching circuit network, only the loss resistance of the CPT coupler is included in Type F. Therefore, the self-capacitance and loss resistance, which are expressed in series, are represented only on the CPT side.

The transmission matrices of the partitioned circuit networks for each Type in Table 5 are as follows.

$$\left\{\begin{array}{c}\begin{array}{c}\begin{array}{c}\begin{array}{c}{\left[T\right]}_{TypeD}=\left[\begin{array}{cc}1& j\omega L+{\displaystyle \frac{1}{j\omega C_2}}+R\\ j\omega C_1 & C_1\left({\displaystyle \frac{1}{C_2}}\left(j\omega R-{\omega }^{2}L\right)\right)\end{array}\right]\end{array}\\ {\left[T\right]}_{TypeE}=\left[\begin{array}{cc}1-{\omega }^2{L}_{1}C& j\omega \left(L_1+L_2\left(1-{\omega }^2{L}_{1}C\right)\right)\\ j\omega C& 1-{\omega }^2{L}_{2}C\end{array}\right]\end{array}\\ {\left[T\right]}_{TypeF}=\left[\begin{array}{cc}2+{\displaystyle \frac{C_2}{C_3}}+{\displaystyle \frac{1}{j\omega C_{3}R}}-{\displaystyle \frac{L_1}{L_3}}& {\displaystyle \frac{1}{j\omega C_3}}+ j\omega \left(L_1+L_2\left({\displaystyle \frac{1+L_1}{L_3}}\right)\right)\\ \left(j\omega C_2+{\displaystyle \frac{1}{R}}\right)\left(1+{\displaystyle \frac{C_2}{C_3}}\right)+j\omega C_1+{\displaystyle \frac{1}{R}}+{\displaystyle \frac{1}{j\omega L_3}}& 2+{\displaystyle \frac{C_1}{C_3}}+{\displaystyle \frac{L_2}{L_3}}\end{array}\right]\end{array}\\ {\left[T\right]}_{TypeG}=\left[\begin{array}{cc}1+{\displaystyle \frac{C_2}{C_3}}+{\displaystyle \frac{1}{j\omega C_{3}R}}& {\displaystyle \frac{1}{j\omega C_3}}\\ \left(j\omega C_2+{\displaystyle \frac{1}{R_2}}\right)\left(1+{\displaystyle \frac{C_1}{C_3}}+{\displaystyle \frac{1}{j\omega C_3{R}_1}}\right)+j\omega C_1+{\displaystyle \frac{1}{R}}& 1+{\displaystyle \frac{C_1}{C_3}}+{\displaystyle \frac{1}{j\omega C_{3}R}}\end{array}\right]\end{array}\right.$$

(6)

Similar to the NFC–IPT coupler, the S-parameters of the partitioned circuit in the NFC–CPT coupler are shown in

Table 6. S-parameters of the NFC–CPT coupler for each PCN type.

Matrix	Parameter
${\left[S\right]}_{Type D}$	$\left\{\begin{array}{l}{\mathrm{t}}_{\mathrm{n}}=1-C_1\left({\displaystyle \frac{1}{C_2}}\left(j\omega R-{\omega }^{2}L\right)\right)\\ {\mathrm{t}}_{\mathrm{m}}=1+C_1\left({\displaystyle \frac{1}{C_2}}\left(j\omega R-{\omega }^{2}L\right)\right)\\ {\mathrm{t}}_{\mathrm{i}}={\displaystyle \frac{1}{Z_0}}\left(j\omega L+{\displaystyle \frac{1}{j\omega C_2}}+R\right)-Z_{0}j\omega C_1\\ {\mathrm{t}}_{\mathrm{j}}={\displaystyle \frac{1}{Z_0}}\left(j\omega L+{\displaystyle \frac{1}{j\omega C_2}}+R\right)+Z_{0}j\omega C_1\end{array}\right.$
${\left[S\right]}_{Type E}$	$\left\{\begin{array}{l}{\mathrm{t}}_{\mathrm{n}}={\omega }^{2}C\left(L_2-L_1\right)\\ {\mathrm{t}}_{\mathrm{m}}=2-{\omega }^{2}C\left(L_2+L_1\right)\\ {\mathrm{t}}_{\mathrm{i}}={\displaystyle \frac{1}{Z_0}}\left(j\omega \left(L_1+L_2\left(1-{\omega }^2{L}_{1}C\right)\right)\right)-Z_{0}j\omega C\\ {\mathrm{t}}_{\mathrm{j}}={\displaystyle \frac{1}{Z_0}}\left(j\omega \left(L_1+L_2\left(1-{\omega }^2{L}_{1}C\right)\right)\right)+Z_{0}j\omega C\end{array}\right.$
${\left[S\right]}_{Type F}$	$\left\{\begin{array}{l}{\mathrm{t}}_{\mathrm{n}}={\displaystyle \frac{1}{\omega L}}\left({\displaystyle \frac{1}{\omega }}\left({\displaystyle \frac{1}{C_2}}-{\displaystyle \frac{1}{C_1}}\right)-jR\right)\\ {\mathrm{t}}_{\mathrm{m}}=2-{\displaystyle \frac{1}{\omega L}}\left({\displaystyle \frac{1}{\omega }}\left({\displaystyle \frac{1}{C_2}}+{\displaystyle \frac{1}{C_1}}\right)+jR\right)\\ {\mathrm{t}}_{\mathrm{i}}={\displaystyle \frac{1}{Z_0}}\left({\displaystyle \frac{1}{j\omega C_3}}+j\omega \left(L_1+L_2\left({\displaystyle \frac{1+L_1}{L_3}}\right)\right)\right)-Z_0\left(\left(j\omega C_2+{\displaystyle \frac{1}{R}}\right)\left(1+{\displaystyle \frac{C_2}{C_3}}\right)+j\omega C_1+{\displaystyle \frac{1}{R}}+{\displaystyle \frac{1}{j\omega L_3}}\right)\\ {\mathrm{t}}_{\mathrm{j}}={\displaystyle \frac{1}{Z_0}}\left({\displaystyle \frac{1}{j\omega C_3}}+j\omega \left(L_1+L_2\left({\displaystyle \frac{1+L_1}{L_3}}\right)\right)\right)+Z_0\left(\left(j\omega C_2+{\displaystyle \frac{1}{R}}\right)\left(1+{\displaystyle \frac{C_2}{C_3}}\right)+j\omega C_1+{\displaystyle \frac{1}{R}}+{\displaystyle \frac{1}{j\omega L_3}}\right)\end{array}\right.$
${\left[S\right]}_{Type G}$	$\left\{\begin{array}{l}{\mathrm{t}}_{\mathrm{n}}={\displaystyle \frac{1}{C_3}}\left(C_2-C_1\right)\\ {\mathrm{t}}_{\mathrm{m}}=2+{\displaystyle \frac{1}{C_3}}\left(C_2+C_1+{\displaystyle \frac{2}{j\omega R}}\right)\\ {\mathrm{t}}_{\mathrm{i}}={\displaystyle \frac{1}{Z_0}}\left({\displaystyle \frac{1}{j\omega C_3}}\right)-Z_0\left(\left(j\omega C_2+{\displaystyle \frac{1}{R_2}}\right)\left(1+{\displaystyle \frac{C_1}{C_3}}+{\displaystyle \frac{1}{j\omega C_3{R}_1}}\right)+j\omega C_1+{\displaystyle \frac{1}{R}}\right)\\ {\mathrm{t}}_{\mathrm{j}}={\displaystyle \frac{1}{Z_0}}\left({\displaystyle \frac{1}{j\omega C_3}}\right)+Z_0\left(\left(j\omega C_2+{\displaystyle \frac{1}{R_2}}\right)\left(1+{\displaystyle \frac{C_1}{C_3}}+{\displaystyle \frac{1}{j\omega C_3{R}_1}}\right)+j\omega C_1+{\displaystyle \frac{1}{R}}\right)\end{array}\right.$

.

3. Simulation Models

3.1. NFC–IPT Coupler

In the simulation model, the substrate on which the copper sheets are placed is Taconic RF-35™ with a dielectric layer. The substrate has a thickness of 0.5 mm, the copper sheet thickness is 0.035 mm, the dielectric constant is 3.5 at 1.9 GHz, and the dielectric loss tangent is 0.0018.

Figure 7a illustrates the coupling structure of the NFC–IPT coupler, where each component is shown separately for clarity. The model replicates a configuration in which coil structures are printed on one side of four substrates. The NFC coupler is stacked between IPT couplers, which are also arranged in a stacked structure. Ferrite material is applied to minimize interference caused by the magnetic field of the coils.

Figure 7b presents the final assembled model based on the coupling method shown in Figure 7a. Figure 7c,d display the top views of the standalone IPT and NFC couplers, respectively. Additionally, the coupler includes circuit traces that incorporate resonant elements, impedance matching circuits, and ports. To implement the SMA connector interface for actual measurements, the model is designed as shown in Figure 7e. Detailed specifications of the coupler can be found in

Table 7. Specifications of NFC–IPT coupler.

Components	Notation	Value
Substrate length	LS	100 mm
Substrate width	WS	84 mm
IPT coil outer length	LIPT	54.5 mm
IPT coil inner length	AGI	26.5 mm
IPT coil conductor spacing and width	-	1 mm
IPT coil conductor width	-	0.5 mm
NFC coil conductor spacing and width	-	0.5 mm
NFC coil outer length	LNFC	28.5 mm
NFC coil inner width	AGN	12.5 mm
Ferrite side length	LF	32 mm
Spacing between NFC and IPT Tx or Rx couplers	G	2 mm
Spacing between NFC Tx and Rx	H	15 mm

, referencing the figures.

3.2. NFC–CPT Coupler

The NFC–CPT simulation model is designed using the same RF-35™ substrate as the NFC–IPT model. Figure 8a illustrates the coupling structure of the NFC–CPT coupler, where each component is shown separately for clarity. The model replicates a configuration in which coil structures are printed on one side of four substrates. The NFC coupler is stacked between the IPT couplers, which are also arranged in a stacked structure. Ferrite material is applied to minimize interference caused by the magnetic field of the coils.

Figure 8b presents the final assembled model based on the coupling method shown in Figure 8a. Figure 8c shows the top view of the CPT coupler. The NFC coupler combined with CPT has the same specifications as the NFC coupler combined with IPT, as described earlier. The circuit implementation for the NFC–CPT coupler follows the same approach as the NFC–IPT model and is designed as shown in Figure 8d. Detailed specifications of the coupler can be found in

Table 8. Specifications of NFC–CPT coupler.

Components	Notation	Value
Substrate length	LS	100 mm
Substrate width	WS	97 mm
Spacing between NFC and CPT Tx or Rx couplers	G	2 mm
Spacing between NFC Tx and Rx	H	15 mm
Copper plate width	WS	25 mm
Copper plate length	LC	50 mm
Copper plate spacing	Gap	10 mm

, referencing the figures.

4. Results and Discussion

A coupler structure with four ports was designed by combining NFC and WPT couplers. As a result, six mutual coupling components are generated. Among them, primary coupling occurs between couplers that exhibit frequency-selective characteristics corresponding to their respective resonant frequencies. The WPT system, which resonates at 6.78 MHz, and the NFC system, which resonates at 13.56 MHz, exhibit precise frequency-selective characteristics. Therefore, it is necessary to observe the transmission coefficients between the NFC transmitter/receiver and the WPT transmitter/receiver, as well as the effects of other coupling interactions.

Figure 9 presents the transmission coefficients of the NFC–IPT coupler and the NFC–CPT coupler. The maximum transmission coefficient of the IPT coupler is 0.93 in the simulation and 0.9 in the PEC model. The maximum transmission coefficient of the NFC coupler is 0.83 in the simulation and 0.86 in the PEC model. The maximum transmission coefficient of the CPT coupler is 0.86 in the simulation and 0.84 in the PEC model. The maximum transmission coefficient of the NFC coupler is 0.9 in the simulation and 0.92 in the PEC model.

When comparing the maximum values of the PEC model analysis and the simulation results, the error rate was within 2.4%. The overall trends in the graphs exhibit similar characteristics.

Figure 10 presents the transmission characteristics of cross-coupling components in NFC–IPT and NFC–CPT couplers. Figure 10a illustrates the coupling between the NFC transmitter and the IPT receiver, as well as between the NFC transmitter and the IPT transmitter. In the 6–7 MHz range, the transmission coefficient for the coupling between the NFC transmitter and the IPT receiver reached a maximum of 0.1 in the simulation and 0.07 in the PEC analysis. Additionally, harmonic components were observed in the cross-coupling. Since 13.56 MHz is an integer multiple of 6.78 MHz, coupling increases when the resonance conditions overlap. Because both NFC and IPT couplers transfer power through inductive coupling, the extent of cross-coupling was significantly high. In contrast, for the NFC–CPT coupler, the transmission coefficient for cross-coupling had a maximum value of 0.03 in the PEC analysis, which is negligible.

The reason why the cross-coupling between the CPT and NFC components is negligible is that the primary energy transfer mechanism in CPT is based on electric fields, whereas NFC operates using magnetic fields. In contrast, in the case of the NFC–IPT coupler, both systems rely on magnetic coupling for energy transfer, which can result in a non-negligible level of cross-coupling.

There are various methods for defining self-inductance in a four-port network to represent the T-model. The key aspect is to accurately determine the inherent self-inductance of the coupler and appropriately compute the mutual inductance according to the equivalent circuit modeling approach.

5. Conclusions

In this study, a hybrid coupler combining NFC and WPT couplers was proposed. Two types of hybrid couplers were designed: NFC–IPT, which combines NFC with IPT, and NFC–CPT, which combines NFC with CPT. A four-port network PEC model was provided for the coupled system, where each coupler has both a transmitter and a receiver.

To theoretically analyze the PEC model, the circuit structures of individual sub-networks were categorized, and the transmission matrices and S-parameters were systematically formulated. By converting the transmission matrices of the sub-networks into S-parameters, a 4 × 4 S-parameter matrix was constructed. The results obtained from this theoretical analysis were compared with 3D full-wave simulation results, demonstrating a high level of agreement.

As a result, both NFC–IPT and NFC–CPT couplers achieved high transmission coefficients. Furthermore, the self-inductance and mutual inductance in the T-model, which are difficult to define in a four-port network, were derived. These findings are expected to contribute to the development of wireless power transfer systems for future applications in mobile devices.