A Dynamic Mechanical Analysis Device for In Vivo Material Characterization of Plantar Soft Tissue
Abstract
1. Introduction
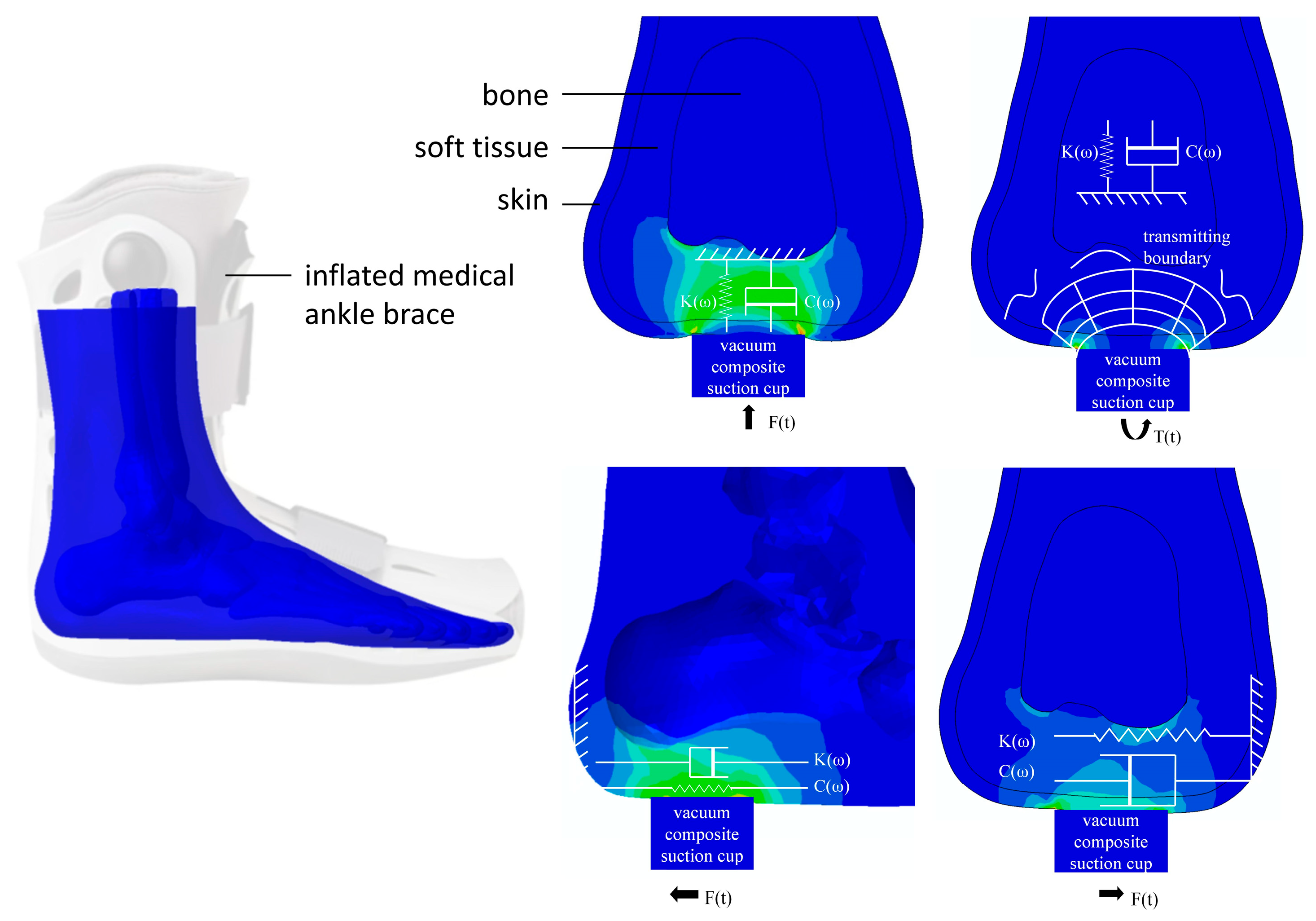
2. Materials and Methods
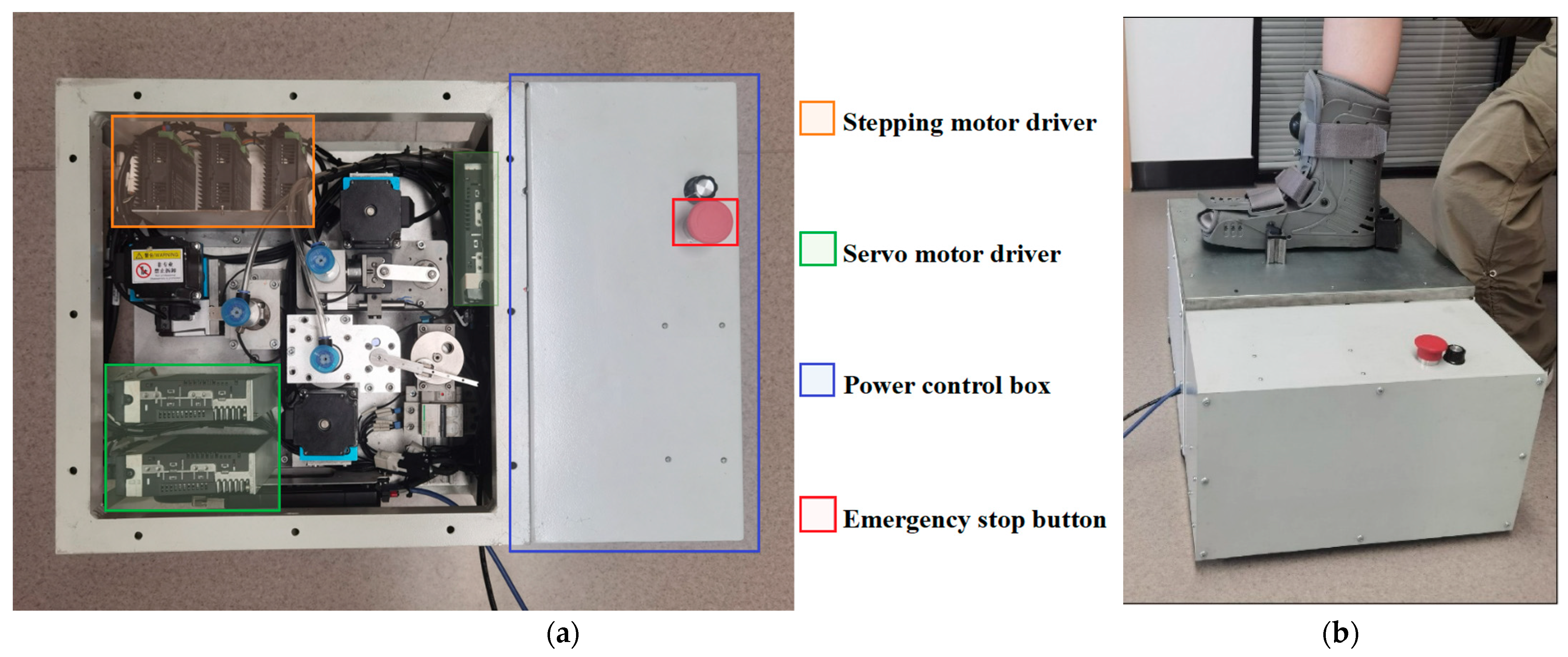
2.1. Build Instruction of Hardware
2.2. Operation Instructions
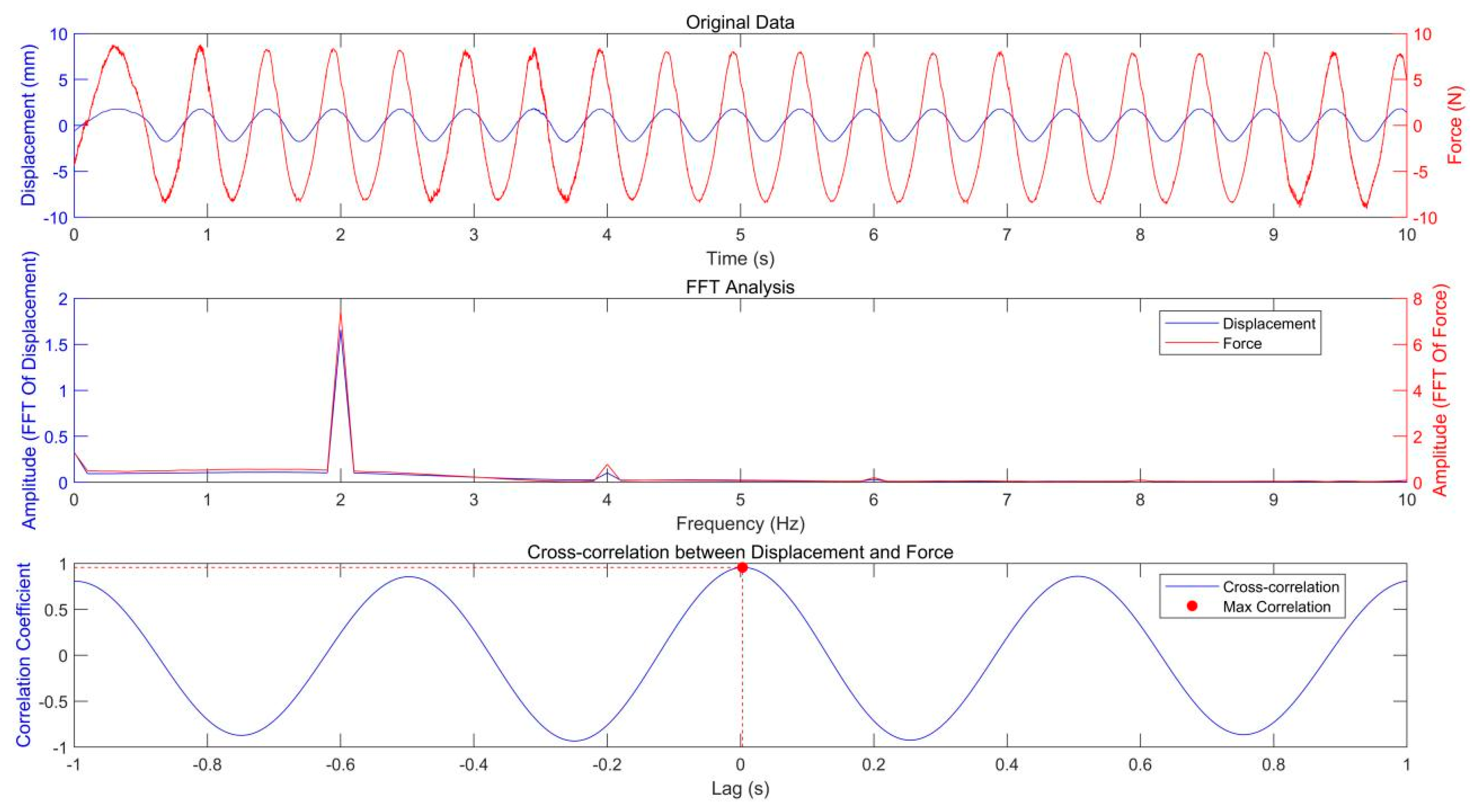
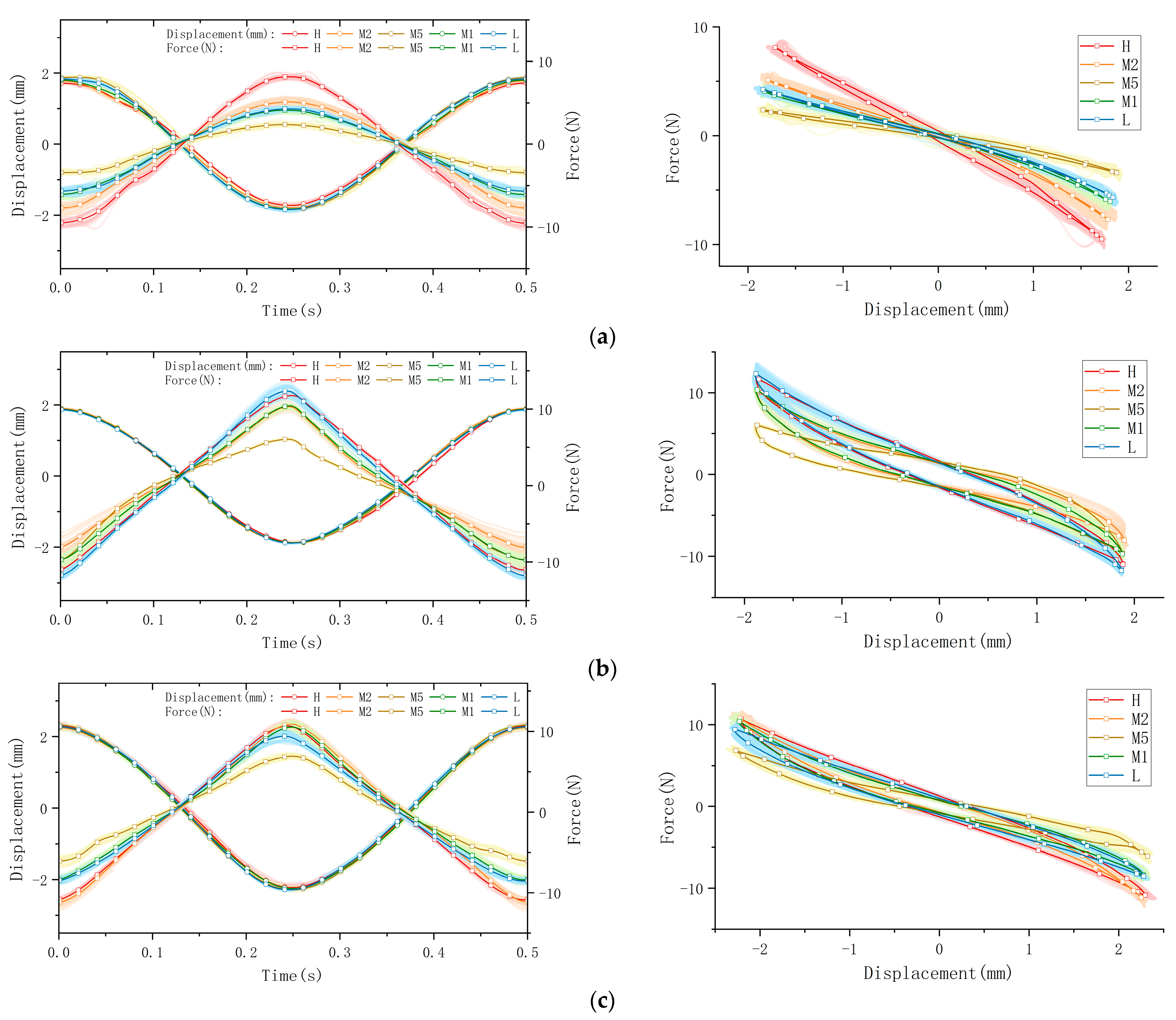
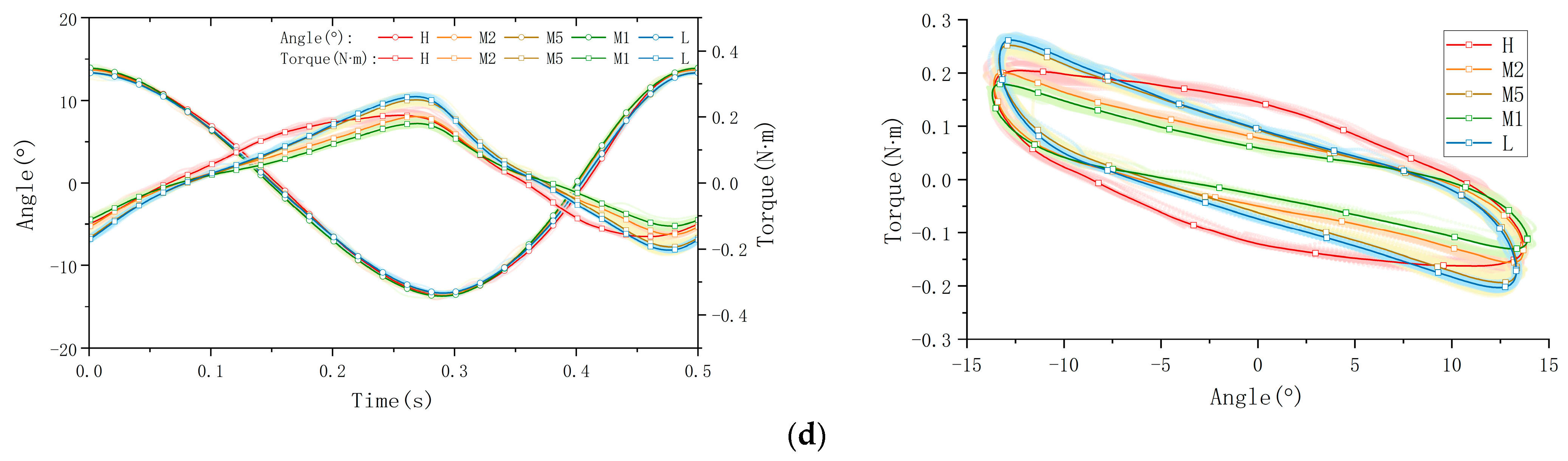
3. Results and Discussion
4. Conclusions
5. Patents
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A
| Hardware name | In Situ Plantar Dynamic Mechanical Analysis |
| Subject area | Medical |
| Hardware type | Measuring physical properties and in-lab sensors |
| Closest commercial analog | No commercial analog is available. |
| Cost of hardware | RMB¥21233–estimated for purchase part |
| Source file repository | https://data.mendeley.com/datasets/y6nm3pj39r/1 DOI:10.17632/y6nm3pj39r.1 |
| Design File Name | File Type | Location of the File |
|---|---|---|
| 3D model of the device | STEP file | 3D model of a novel in situ dynamic mechanical detection machine for human plantar soft tissue—Mendeley Data |
| CAD drawings of the device | CAD file | CAD drawing of a novel in situ dynamic mechanical detection machine for human plantar soft tissue—Mendeley Data |
| Component | Number | Cost per Unit-Currency | Total Cost- | Component |
|---|---|---|---|---|
| Force sensor (DYMH-103) | 4 | RMB¥171 | RMB¥684 | 1688.com (accessed on 28 August 2024) |
| LVDT (GA09) | 2 | RMB¥800 | RMB¥1600 | 1688.com (accessed on 28 August 2024) |
| Torque sensor (FYAH) | 1 | RMB¥5960 | RMB¥5960 | 1688.com (accessed on 28 August 2024) |
| Angle sensor (GT-D) | 1 | RMB¥202 | RMB¥202 | 1688.com (accessed on 28 August 2024) |
| Screw lifting module (CB1605-30) | 3 | RMB¥319 | RMB¥957 | 1688.com (accessed on 28 August 2024) |
| Stepper motor and driver (57 stepper and DM542) | 3 | RMB¥86 | RMB¥258 | 1688.com (accessed on 28 August 2024) |
| Servo motor and driver (Panasonic A6 and MADLT05SF) | 3 | RMB¥2000 | RMB¥6000 | 1688.com (accessed on 28 August 2024) |
| Solenoid valve (2V025-08) | 3 | RMB¥16 | RMB¥48 | 1688.com (accessed on 28 August 2024) |
| Vacuum pump (G7BL2485S) | 1 | RMB¥568 | RMB¥568 | 1688.com (accessed on 28 August 2024) |
| Slider and slide rail (MGN5C) | 1 | RMB¥42 | RMB¥42 | 1688.com (accessed on 28 August 2024) |
| Linear sliding table (CHTF-W40-L35) | 2 | RMB¥235 | RMB¥470 | 1688.com (accessed on 28 August 2024) |
| Timing belt and pulleys (HTD3M) | 1 | RMB¥45 | RMB¥45 | 1688.com (accessed on 28 August 2024) |
| DAQ (USB 3151) | 1 | RMB¥4399 | RMB¥4399 | 1688.com (accessed on 28 August 2024) |
References
- Natali, A.N.; Fontanella, C.G.; Carniel, E.L. Constitutive formulation and analysis of heel pad tissues mechanics. Med. Eng. Phys. 2010, 32, 516–522. [Google Scholar] [CrossRef] [PubMed]
- Hegde, M.; Karthik, K.; Managuli, V.; Bhat, S.K. A consistent transversely-isotropic hyper-viscoelastic model: Finite element implementation and mechanical characterization of biological tissues. Int. J. Non-Linear Mech. 2024, 160, 104663. [Google Scholar] [CrossRef]
- Haron, A.; Li, L.; Shuang, J.; Lin, C.; Mansoubi, M.; Shi, X.; Horn, D.; Reeves, N.; Bowling, F.; Bradbury, K.; et al. In-shoe plantar temperature, normal and shear stress relationships during gait and rest periods for people living with and without diabetes. Sci. Rep. 2025, 15, 8804. [Google Scholar] [CrossRef]
- Yang, X.; Peng, Z.; Liu, X.; Liu, X.-L.; Lu, S. A narrative review of the measurement methods for biomechanical properties of plantar soft tissue in patients with diabetic foot. Front. Endocrinol. 2024, 15, 1332032. [Google Scholar] [CrossRef] [PubMed]
- Kwan, R.L.C.; Zheng, Y.P.; Cheing, G.L.Y. The effect of aging on the biomechanical properties of plantar soft tissues. Clin. Biomech. 2010, 25, 601–605. [Google Scholar] [CrossRef]
- Cavanagh, P.R. Plantar soft tissue thickness during ground contact in walking. J. Biomech. 1999, 32, 623–628. [Google Scholar] [CrossRef]
- Scott, G.; Menz, H.B.; Newcombe, L. Age-related differences in foot structure and function. Gait Posture 2007, 26, 68–75. [Google Scholar] [CrossRef]
- Ledoux, W.R.; Blevins, J.J. The compressive material properties of the plantar soft tissue. J. Biomech. 2007, 40, 2975–2981. [Google Scholar] [CrossRef]
- Brady, L.; Pai, S.; Iaquinto, J.M.; Wang, Y.-N.; Ledoux, W.R. The compressive, shear, biochemical, and histological characteristics of diabetic and non-diabetic plantar skin are minimally different. J. Biomech. 2021, 129, 110797. [Google Scholar] [CrossRef]
- Tecse, A.; Romero, S.E.; Naemi, R.; Castaneda, B. Characterisation of the soft tissue viscous and elastic properties using ultrasound elastography and rheological models: Validation and applications in plantar soft tissue assessment. Phys. Med. Biol. 2023, 68, 105005. [Google Scholar] [CrossRef]
- Latorre-Ossa, H.; Gennisson, J.L.; De Brosses, E.; Tanter, M. Quantitative imaging of nonlinear shear modulus by combining static elastography and shear wave elastography. IEEE Trans. Ultrason. Ferroelectr. Freq. Control. 2012, 59, 833–839. [Google Scholar] [CrossRef] [PubMed]
- Tonna, R.; Chatzistergos, P.E.; Wyatt, O.; Chockalingam, N. Reliability and Validity of Shore Hardness in Plantar Soft Tissue Biomechanics. Sensors 2024, 24, 539. [Google Scholar] [CrossRef]
- Behforootan, S.; Chatzistergos, P.E.; Chockalingam, N.; Naemi, R. A clinically applicable non-invasive method to quantitatively assess the visco-hyperelastic properties of human heel pad, implications for assessing the risk of mechanical trauma. J. Mech. Behav. Biomed. Mater. 2017, 68, 287–295. [Google Scholar] [CrossRef] [PubMed]
- Macan, J. Application of thermal analysis methods in biology and medicine. Period. Biol. 2023, 125, 75–100. [Google Scholar] [CrossRef]
- Menard, K.P.; Menard, N. Dynamic Mechanical Analysis; CRC Press: Boca Raton, FL, USA, 2020. [Google Scholar]
- Ginic-Markovic, M.; Choudhury, N.R.; Dimopoulos, M.; Williams, D.R.; Matisons, J. Characterization of elastomer compounds by thermal analysis. Thermochim. Acta 1998, 316, 87–95. [Google Scholar] [CrossRef]
- Yang, G.; Pang, X.; Liu, A.; Li, C.; Zhu, J.; Huang, R.; Ma, X. Design and validation of a DMA-inspired device for in-vivo measurement of human skin mechanics: A finite element analysis approach. In Journal of Physics: Conference Series, Proceedings of the 7th International Conference on Physics, Mathematics and Statistics, Yichang, China, 23–25 May 2024; IOP Publishing: Bristol, UK, 2024; Volume 2851, p. 012019. [Google Scholar]
- Grigoriadis, G.; Newell, N.; Carpanen, D.; Christou, A.; Bull, A.M.; Masouros, S.D. Material properties of the heel fat pad across strain rates. J. Mech. Behav. Biomed. Mater. 2017, 65, 398–407. [Google Scholar] [CrossRef]
- Negishi, T.; Ito, K.; Kamono, A.; Lee, T.; Ogihara, N. Strain-rate dependence of viscous properties of the plantar soft tissue identified by a spherical indentation test. J. Mech. Behav. Biomed. Mater. 2020, 102, 103470. [Google Scholar] [CrossRef]
- Huang, R.; Ning, X.; Wu, L.; Zhu, J.; Tang, L.; Ma, X. An exploratory in-situ dynamic mechanical analysis on the shearing stress–strain mechanism of human plantar soft tissue. Sci. Rep. 2024, 14, 11953. [Google Scholar] [CrossRef]
- Boyer, G.; Laquièze, L.; Le Bot, A.; Laquièze, S.; Zahouani, H. Dynamic indentation on human skin in vivo: Ageing effects. Ski. Res. Technol. 2009, 15, 55–67. [Google Scholar] [CrossRef]
- Stief, T.; Peikenkamp, K. A new insole measurement system to detect bending and torsional moments at the human foot during footwear condition: A technical report. J. Foot Ankle Res. 2015, 8, 1–8. [Google Scholar] [CrossRef]
- Lewandowski, R.; Chorążyczewski, B. Identification of the parameters of the Kelvin–Voigt and the Maxwell fractional models, used to modeling of viscoelastic dampers. Comput. Struct. 2010, 88, 1–17. [Google Scholar] [CrossRef]
- Pai, S.; Ledoux, W.R. The compressive mechanical properties of diabetic and non-diabetic plantar soft tissue. J. Biomech. 2010, 43, 1754–1760. [Google Scholar] [CrossRef] [PubMed]
- Chavan, R.; Kamble, N.; Kuthe, C.; Sarnobat, S. On Mechanical Behavior and Characterization of Soft Tissues. Biomed. Eng. Comput. Biol. 2024, 15, 11795972241294115. [Google Scholar] [CrossRef] [PubMed]


| Tensile/Compress Displacement (mm) | Longitudinal Shear Displacement (mm) | Transverse Shear Displacement (mm) | Torsion Displacement (degree) | |
|---|---|---|---|---|
| Mean Value | 3.63506 | 3.78726 | 4.57726 | 27.27410 |
| Standard Deviation | 0.08795 | 0.00477 | 0.04109 | 0.40308 |
| Sensor | Specifications | Values |
|---|---|---|
| Force sensor (DYMH-103) | Capacity | 0–5 kg |
| Response frequency | 10 KHz | |
| Output sensitivity | 1.0–1.5 ± 10% mV/V | |
| Hysteresis | 0.1% F.S. | |
| Repeatability | 0.1% F.S. | |
| Temperature sensitivity drift | 0.1% F.S./10 °C | |
| Zero temperature drift | 0.1% F.S./10 °C | |
| LVDT (GA09) | Capacity | ±5 mm |
| Response frequency | 1–10 KHz | |
| Nonlinearity error | ±0.5% BFSL | |
| Torque sensor (FYAH) | Capacity | 0–1 NM |
| Response frequency | 200 Hz | |
| Nonlinearity | ±0.25% of R.O. | |
| Hysteresis | ±0.25% of R.O. | |
| Nonrepeatability | ±0.1% of R.O. | |
| Angle sensor (GT-D) | Capacity | 0–360° |
| Resolution | 4096, 12 bits | |
| independent linearity | 0.15% F.S. | |
| Response frequency | 5 KHz |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, L.; Huang, R.; Zhu, J.; Ma, X. A Dynamic Mechanical Analysis Device for In Vivo Material Characterization of Plantar Soft Tissue. Technologies 2025, 13, 191. https://doi.org/10.3390/technologies13050191
Wu L, Huang R, Zhu J, Ma X. A Dynamic Mechanical Analysis Device for In Vivo Material Characterization of Plantar Soft Tissue. Technologies. 2025; 13(5):191. https://doi.org/10.3390/technologies13050191
Chicago/Turabian StyleWu, Longyan, Ran Huang, Jun Zhu, and Xin Ma. 2025. "A Dynamic Mechanical Analysis Device for In Vivo Material Characterization of Plantar Soft Tissue" Technologies 13, no. 5: 191. https://doi.org/10.3390/technologies13050191
APA StyleWu, L., Huang, R., Zhu, J., & Ma, X. (2025). A Dynamic Mechanical Analysis Device for In Vivo Material Characterization of Plantar Soft Tissue. Technologies, 13(5), 191. https://doi.org/10.3390/technologies13050191






