Effect of Anti-Bending Bars on Vertical Vibrations of Passenger Carriage Body
Abstract
1. Introduction
2. Mechanical Model of the Passenger Carriage with an ABB System and Governing Equations
2.1. Mechanical Model
2.2. Equations of Motion
- -
- For the bounce motion,
- -
- For the pitch motion,
- -
- For the first bending mode,
- -
- For the bounce motion,
- -
- For the pitch motion,
- -
- For the bounce motion,
- -
- For the pitch motion,
- -
- For the first bending mode,
- -
- For the bounce motion,
- -
- For the pitch motion,
- -
- For the first bending mode,
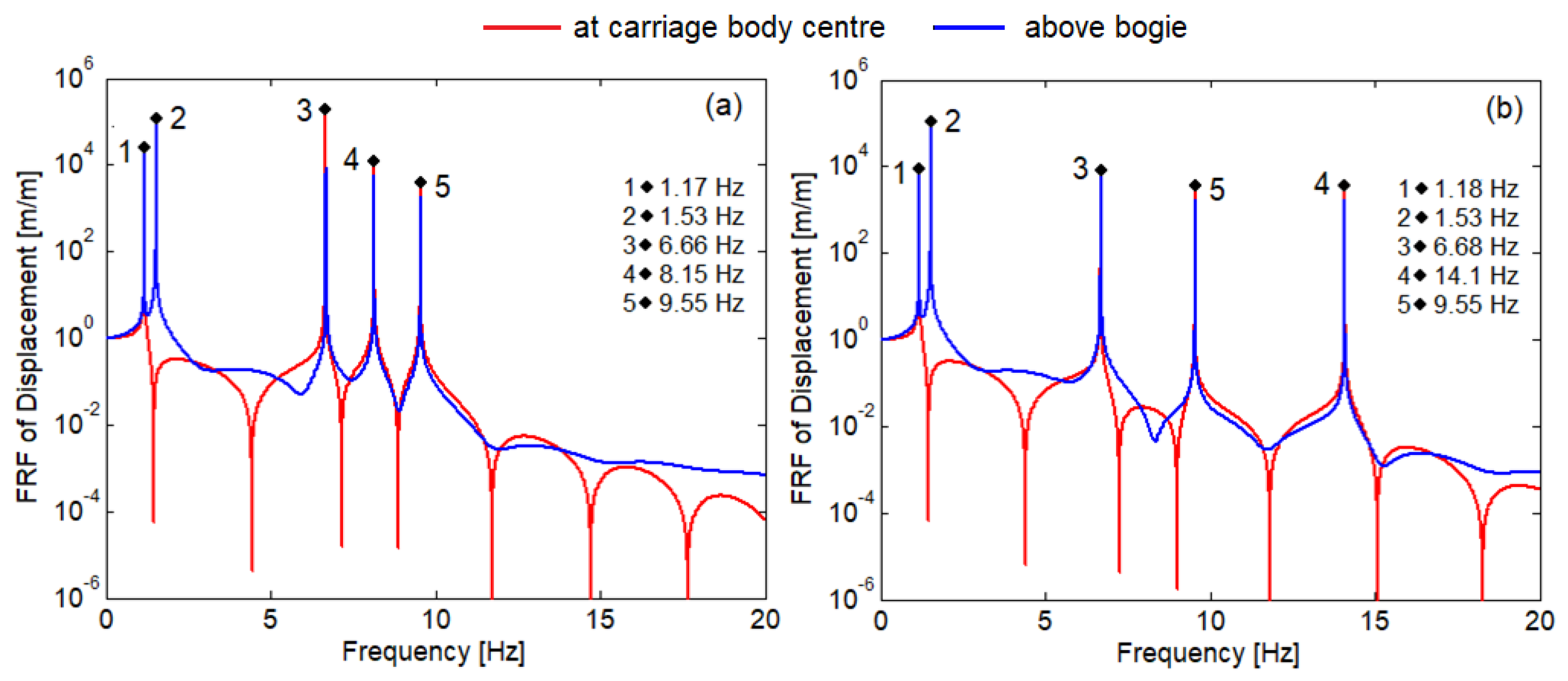
3. Frequency Response Functions
- -
- For the carriage body,
- -
- For the ABBs,
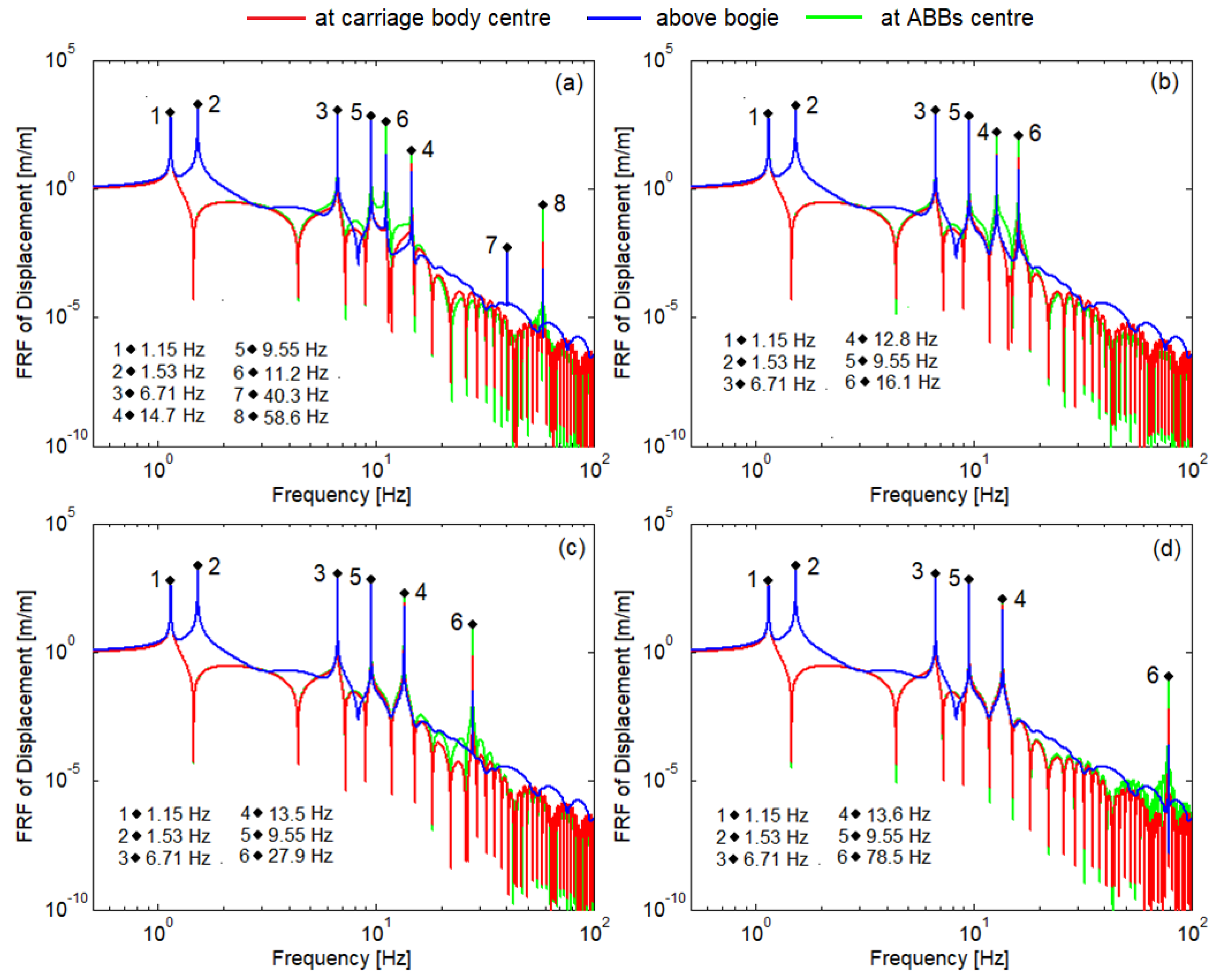
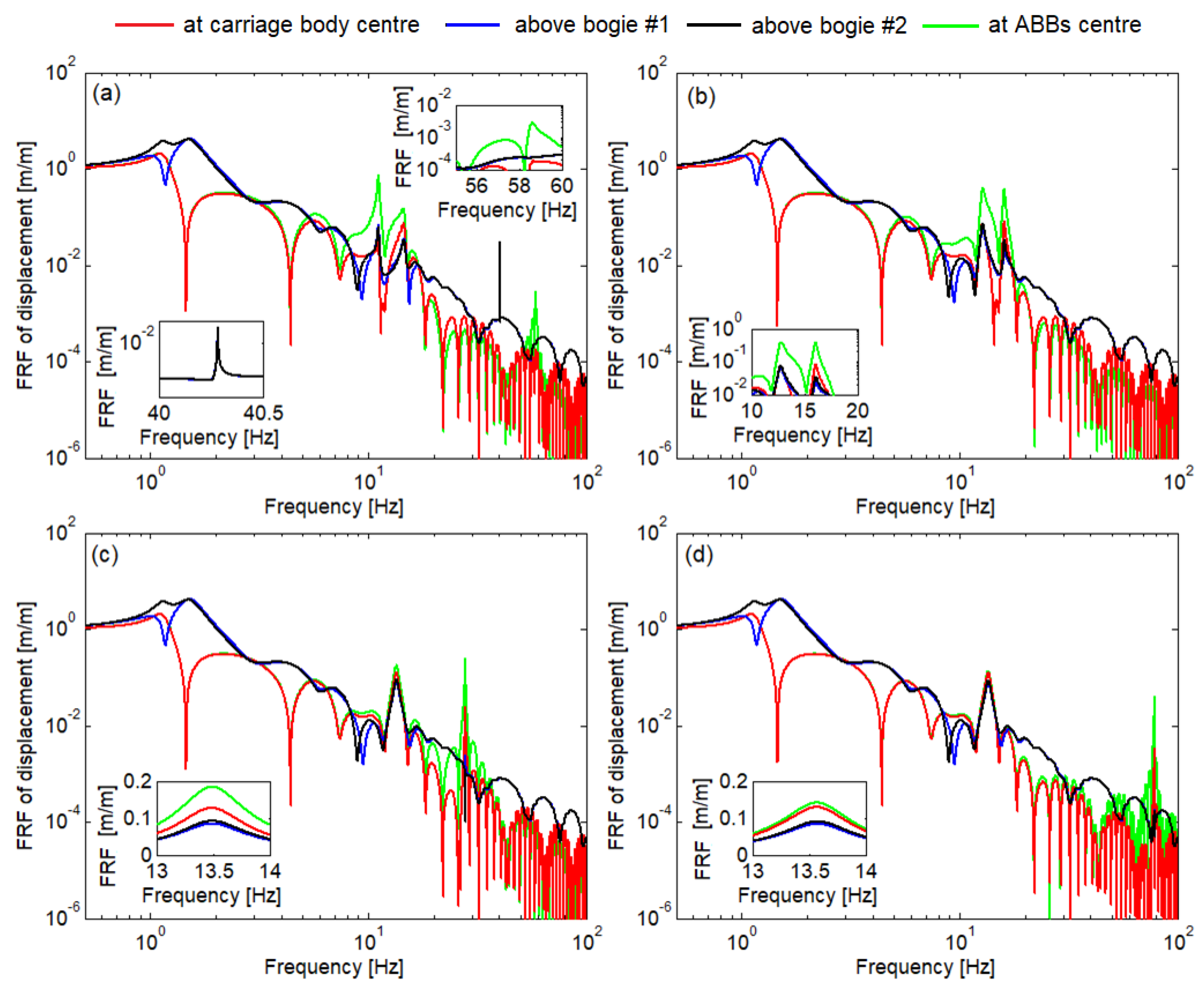
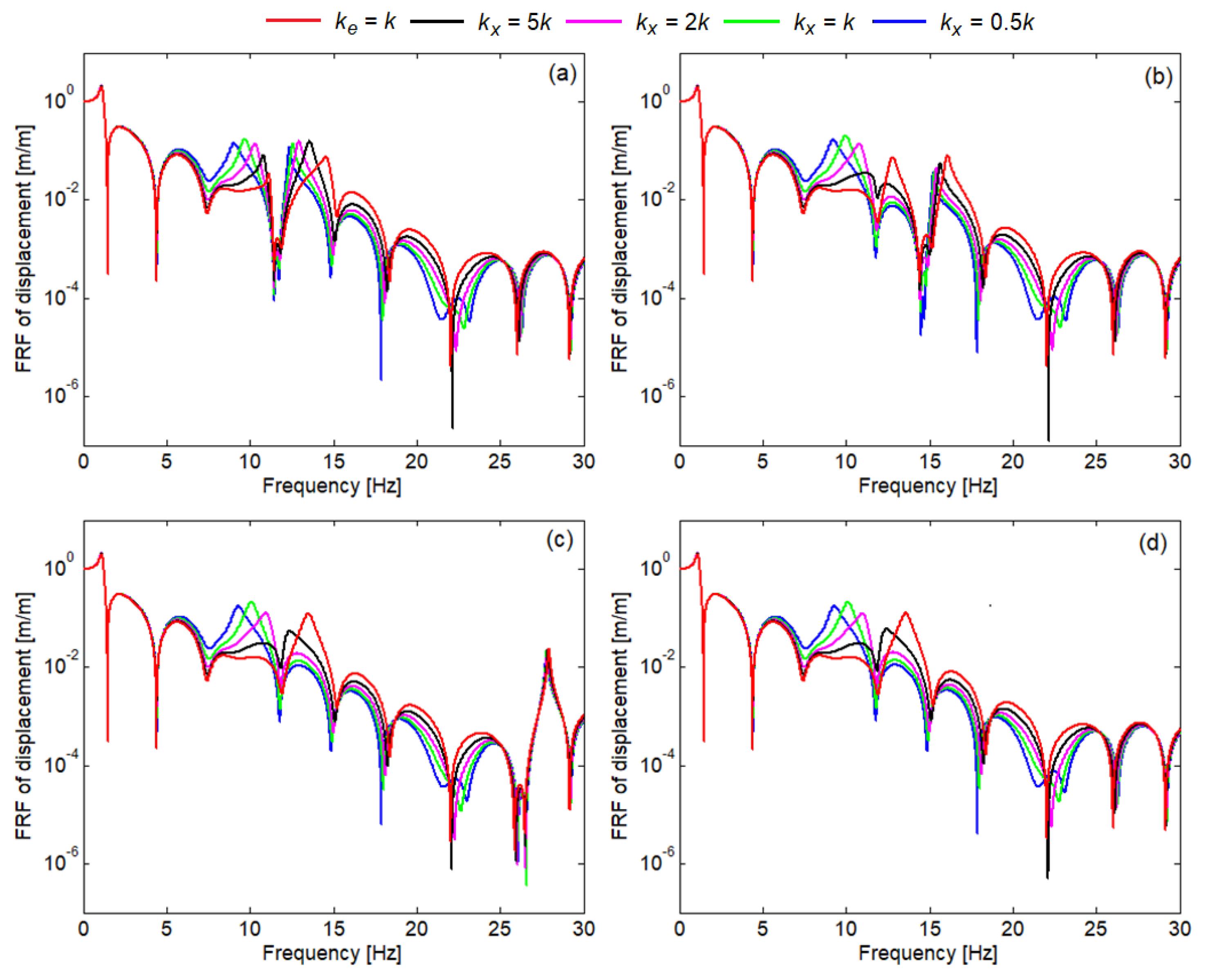
4. Study on ABBs Effect on Vertical Vibrations of the Carriage Body
4.1. Dynamic Response of the Carriage Body with ABB
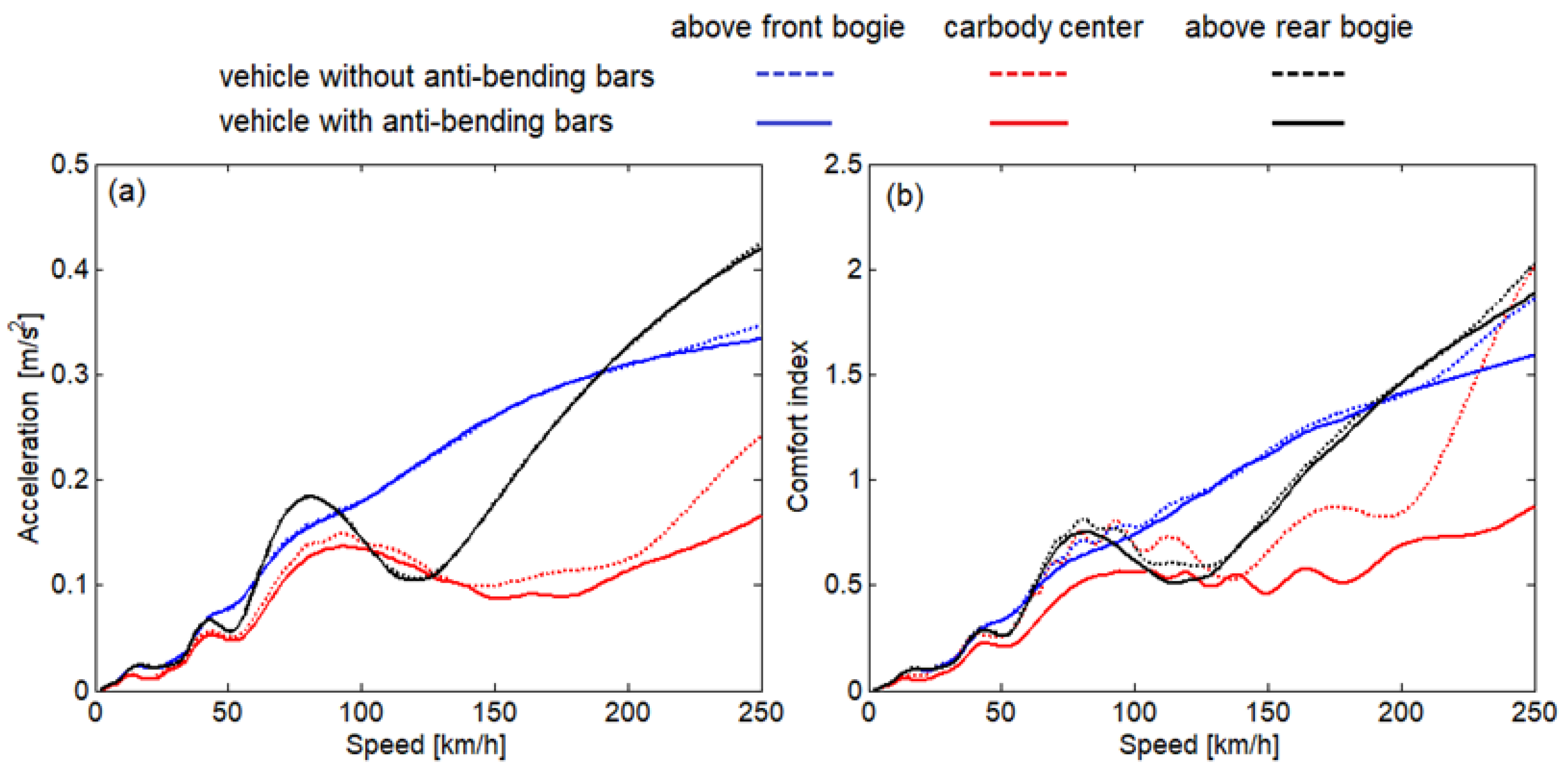
4.2. Evaluating the Running Behaviour and Comfort Index of the Passenger Carriage with ABBs
5. Conclusions
- (a)
- The parametric study of the FRF of the carriage body shows that the vibration behaviour of the carriage body is a little influenced by the vertical stiffness of the fastening;
- (b)
- The parametric study of the FRF of the ABBs shows that the resonance peaks move along the frequency axis as the fastening between the ABBs and the vertical supports is more rigid in the vertical direction;
- (c)
- The running behaviour reflected by the acceleration and the ride comfort quantified by the comfort index are not significantly influenced by the vertical stiffness of the fastening, if the fastening is perfectly rigid in the longitudinal direction;
- (d)
- When the fastening becomes elastic in the longitudinal direction, then both the running behaviour and ride comfort are affected;
- (e)
- To compensate the depreciation in terms of running behaviour and ride comfort caused by the above situation, longer ABBs with stiffer fastening in the vertical direction should be used.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- EN 12299; Railway Applications. Ride Comfort for Passengers. Measurement and Evaluation. European Committee for Standardization: Brussels, Belgium, 1999.
- Testing and Approval of Railway Vehicles from the Point of View of Their Dynamic Behaviour-Safety-Track Fatigue-Running Behaviour, 4th ed.; UIC 518 Leaflet: Paris, France, 2009.
- Zhang, C.; Kordestani, H.; Shadabfar, M. A combined review of vibration control strategies for high-speed trains and railway infrastructures: Challenges and solutions. J. Low Freq. Noise Vib. Act. Control 2023, 42, 272–291. [Google Scholar] [CrossRef]
- Tomioka, T.; Takigami, T.; Suzuki, Y. Numerical analysis of three-dimensional flexural vibration of railway vehicle car body. Veh. Syst. Dyn. 2006, 44 (Suppl. S1), 272–285. [Google Scholar] [CrossRef]
- Hui, C.; Weihua, Z.; Bingring, M. Vertical vibration analysis of the flexible carbody of high-speed train. Int. J. Veh. Struct. Syst. 2015, 7, 55–60. [Google Scholar] [CrossRef]
- Palomares, E.; Morales, A.L.; Nieto, A.J.; Chicharro, J.M.; Pintado, P. Comfort improvement in railway vehicles via optimal control of adaptive pneumatic suspensions. Veh. Syst. Dyn. 2022, 60, 1702–1721. [Google Scholar] [CrossRef]
- Lewis, T.D.; Jiang, J.Z.; Neild, S.A.; Gong, C.; Iwnicki, S.D. Using an inerter-based suspension to improve both passenger comfort and track wear in railway vehicles. Veh. Syst. Dyn. 2020, 58, 472–493. [Google Scholar] [CrossRef]
- Sugahara, Y.; Kojima, T. Suppression of vertical vibration in railway vehicle carbodies through control of damping force in primary suspension: Presentation of results from running tests with meter-gauge car on a secondary line. WIT Trans. Built Environ. 2018, 181, 329–337. [Google Scholar]
- Sugahara, Y.; Kazato, A.; Koganei, R.; Sampei, M.; Nakaura, S. Suppression of vertical bending and rigid-body-mode vibration in railway vehicle car body by primary and secondary suspension control: Results of simulations and running tests using Shinkansen vehicle. Proc. Inst. Mech. Eng. J. Rail Rapid Transit 2009, 223, 517–531. [Google Scholar] [CrossRef]
- Chen, J.; Wu, Y.; Zhang, L.; He, X.; Dong, S. Dynamic optimization design of the suspension parameters of car body mounted equipment via analytical target cascading. J. Mech. Sci. Technol. 2020, 34, 1957–1969. [Google Scholar] [CrossRef]
- Dumitriu, M. Study on improving the ride comfort in railway vehicles using anti-bending dampers. Appl. Mech. Mater. 2018, 880, 207–212. [Google Scholar] [CrossRef]
- Gong, D.; Wang, K.; Duan, Y.; Zhou, J. Car body floor vibration of high-speed railway vehicles and its reduction. J. Low Freq. Noise Vibr. Act. Control 2020, 39, 925–938. [Google Scholar] [CrossRef]
- Graa, M. Modeling and control for vertical rail vehicle dynamic vibration with comfort evaluation. Int. J. Math. Model. Methods Appl. Sci. 2017, 11, 240–245. [Google Scholar]
- Gong, D.; Zhou, J.; Sun, W. Passive control of railway vehicle car body flexural vibration by means of under frame dampers. J. Mech. Sci. Technol. 2017, 31, 555–564. [Google Scholar] [CrossRef]
- Huang, C.; Zeng, J.; Luo, G.; Shi, H. Numerical and experimental studies on the car body flexible vibration reduction due to the effect of car body-mounted equipment. Proc. Inst. Mech. Eng. J. Rail Rapid Transit 2016, 232, 103–120. [Google Scholar] [CrossRef]
- Isacchi, G.; Corsi, M.; Ripamonti, F. Ride comfort assessment of high-speed rail vehicles: Influence of yaw dampers installation angle. Vibroengineering Procedia 2023, 50, 98–104. [Google Scholar] [CrossRef]
- Johnssons, A.; Berbyuk, V.; Enelund, M. Pareto optimisation of railway bogie suspension damping to enhance safety and comfort. Veh. Syst. Dyn. 2012, 50, 1379–1407. [Google Scholar] [CrossRef]
- Nakajima, D.; Aida, K.I.; Akiyama, Y.; Tkigami, T.; Tomioka, T.; Nishiyama, Y.; Tanaka, T.; Miyamoto, T. Development of a new mounting structure of yaw damper to reduce railway carbody vibration. Trans. JSME 2017, 83, 17–00078. (In Japanese) [Google Scholar]
- Shi, H.L.; Luo, R.; Wu, P.B.; Zeng, J.; Guo, J.Y. Application of DVA theory in vibration reduction of carriage body with suspended equipment for high-speed EMU. Sci. China Technol. Sci. 2014, 57, 1425–1438. [Google Scholar] [CrossRef]
- Sharma, S.K.; Sharma, R.C.; Lee, J.; Jang, H.L. Numerical and experimental analysis of DVA on the flexible-rigid rail vehicle carriage body resonant vibration. Sensors 2022, 22, 1922. [Google Scholar] [CrossRef] [PubMed]
- Nitish; Singh, A.K. Active control of railway vehicle suspension using PID controller with pole placement technique. Mater. Today Proc. 2023, 80, 278–284. [Google Scholar] [CrossRef]
- Kozek, M.; Benatzky, C.; Schirrer, A.; Stribersky, A. Vibration Damping of a Flexible Car Body Structure Using Piezo-Stack Actuators. In Proceedings of the 17th World Congress The International Federation of Automatic Control, Seoul, Republic of Korea, 6–11 July 2008; pp. 8284–8299. [Google Scholar]
- Kamada, T.; Hiraizumi, T.; Nagai, M. Active vibration suppression of lightweight railway vehicle body by combined use of piezoelectric actuators and linear actuators. Veh. Syst. Dyn. 2010, 48, 73–87. [Google Scholar] [CrossRef]
- Takigami, T.; Tomioka, T. Bending vibration suppression of railway vehicle carbody with piezoelectric elements (Experimental results of excitation tests with a commuter car). J. Mech. Syst. Transp. Logist. 2008, 1, 111–121. [Google Scholar] [CrossRef]
- Zhou, J.; Goodall, R.; Ren, L.; Zhang, H. Influences of car body vertical flexibility on ride quality of passenger railway vehicles. Proc. Inst. Mech. Eng. J. Rail Rapid Transit 2009, 223, 461–471. [Google Scholar] [CrossRef]
- Cheli, F.; Corradi, R. On rail vehicle vibrations induced by track unevenness: Analysis of the excitation mechanism. J. Sound Vib. 2011, 330, 3744–3765. [Google Scholar] [CrossRef]
- You, T.; Zhou, J.; Thompson, D.J.; Gong, D.; Chen, J.; Sun, Y. Vibration reduction of a high-speed train floor using multiple dynamic vibration absorbers. Veh. Syst. Dyn. 2022, 60, 2919–2940. [Google Scholar] [CrossRef]
- Gong, D.; Zhao, K.; Liu, G.; Wang, Z.; You, T.; Zhou, J. Modal vibration decomposition method and its application on multi-mode vibration control of high-speed railway car bodies. J. Frankl. Inst. 2022, 359, 4699–4726. [Google Scholar] [CrossRef]
- Dumitriu, M. A new passive approach to reducing the carbody vertical bending vibration of railway vehicles. Veh. Syst. Dyn. 2017, 55, 1787–1806. [Google Scholar] [CrossRef]
- Fu, B.; Bruni, S. An examination of alternative schemes for active and semi-active control of vertical car-body vibration to improve ride comfort. Proc. Inst. Mech. Eng. J. Rail Rapid Transit 2022, 236, 386–405. [Google Scholar] [CrossRef]
- Fu, B.; Liu, B.; Di Gialleonardo, E.; Bruni, S. Semi-active control of primary suspensions to improve ride quality in a high-speed railway vehicle. Veh. Syst. Dyn. 2023, 61, 2664–2688. [Google Scholar] [CrossRef]
- Dumitriu, M.; Mazilu, T.; Apostol, I.I. Scale Models to Verify the Effectiveness of the Methods to Reducing the Vertical Bending Vibration of the Railway Vehicles Carbody: Applications and Design Elements. Appl. Sci. 2023, 13, 2368. [Google Scholar] [CrossRef]
- Mazilu, T.; Dumitriu, M.; Sorohan, Ș.; Gheți, M.A.; Apostol, I.I. Testing the Effectiveness of the Anti-Bending Bar System to Reduce the Vertical Bending Vibrations of the Railway Vehicle Carbody Using an Experimental Scale Demonstrator. Appl. Sci. 2024, 14, 4687. [Google Scholar] [CrossRef]
- Apostol, I.I.; Mazilu, T.; Dumitriu, M. Study on Dynamic Response of the Carbody–Anti-Bending Bar System. Technologies 2025, 13, 31. [Google Scholar] [CrossRef]
- Gong, D.; Gu, Y.J.; Song, Y.J.; Zhou, J. Study on geometry filtering phenomenon and flexible car body resonant vibration of articulated trains. Adv. Mater. Res. 2013, 787, 542–547. [Google Scholar] [CrossRef]
- Zhou, J.; Wenjing, S. Analysis on geometric filtering phenomenon and flexible car body resonant vibration of railway vehicles. J. Tongji Univ. 2009, 37, 1653–1657. [Google Scholar]
- Dumitriu, M.; Apostol, I.I. The Effect of the Traction Rod on the Vertical Vibration Behavior of the Railway Vehicle Carbody. Vehicles 2023, 5, 1482–1504. [Google Scholar] [CrossRef]

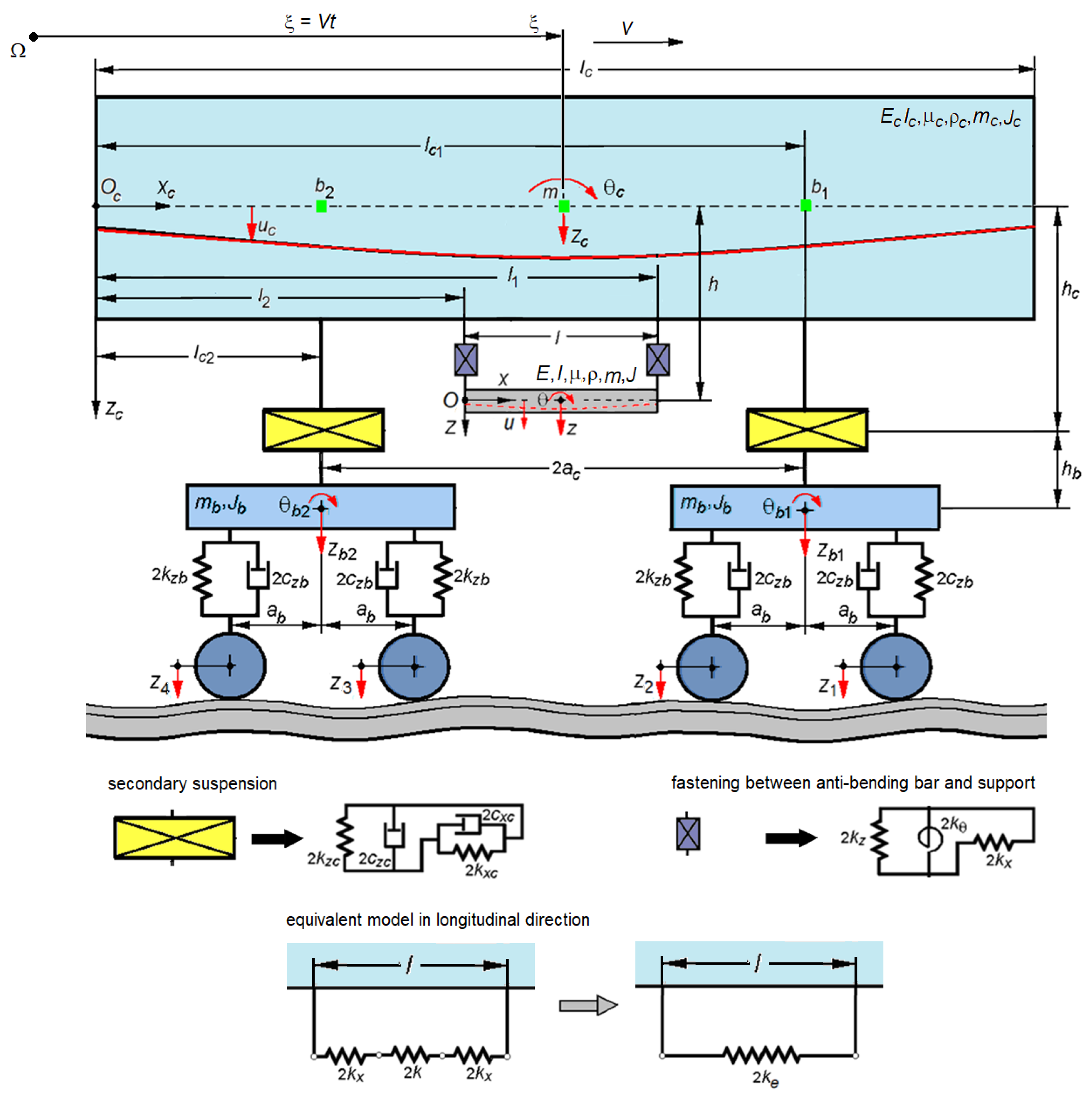





| mc = 34,000 kg | lc2 = 3.7 m |
| mb = 3200 kg | 2kzc = 1.2 MN/m |
| Jc = 1,963,840 kg m2 | 2kxc = 4 MN/m |
| Jb = 2048 kg m2 | 2czc = 34.28 kNs/m |
| EcIc = 3.158 × 109 Nm2 | 2cxc = 50 kNs/m |
| lc = 26.4 m | 4kzb = 4.4 MN/m |
| 2ac = 19 m | 4czb = 52.21 kNs/m |
| 2ab = 2.56 m | mmc = 35,224 kg |
| hc = 1.3 m | kmc = 88.998 MN/m |
| hb = 0.2 m | cmc = 53.117 kNs/m |
| lc1 = 22.7 m | h = 1.2 m; |
| m = 941 kg | k = 902.76 MN/m |
| ρ = 178.18 kg/m | mm = 975 kg |
| E = 210 GPa | km = 30.33 MN/m |
| l = 5.28 m | cm = 860 Ns/m |
| d = 0.17 m | l1 = 15.84 m |
| I = 4.0998 × 10−5 m4 | l2 = 10.56 m |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Apostol, I.-I.; Mazilu, T.; Dumitriu, M. Effect of Anti-Bending Bars on Vertical Vibrations of Passenger Carriage Body. Technologies 2025, 13, 73. https://doi.org/10.3390/technologies13020073
Apostol I-I, Mazilu T, Dumitriu M. Effect of Anti-Bending Bars on Vertical Vibrations of Passenger Carriage Body. Technologies. 2025; 13(2):73. https://doi.org/10.3390/technologies13020073
Chicago/Turabian StyleApostol, Ioana-Izabela, Traian Mazilu, and Mădălina Dumitriu. 2025. "Effect of Anti-Bending Bars on Vertical Vibrations of Passenger Carriage Body" Technologies 13, no. 2: 73. https://doi.org/10.3390/technologies13020073
APA StyleApostol, I.-I., Mazilu, T., & Dumitriu, M. (2025). Effect of Anti-Bending Bars on Vertical Vibrations of Passenger Carriage Body. Technologies, 13(2), 73. https://doi.org/10.3390/technologies13020073







