Numerical Simulation and Optimization of Microwave Heating Effect on Coal Seam Permeability Enhancement
Abstract
:1. Introduction
- The electric field profile must be homogeneous, either by using state stirrers or by rotating the reaction cavity itself.
- The geometry of the reactor must be well designed considering the depth of microwave penetration.
- The temperature and pressure inside the reaction chamber must be controlled for a continuous control of the process parameters.
- The costs of the reactor and spare parts must be considered.
- Safety and leakage of microwaves must be considered.
2. Theory
2.1. Symbols
2.2. Maxwell Equations
2.3. Heat Transfer
2.4. Mass Transfer
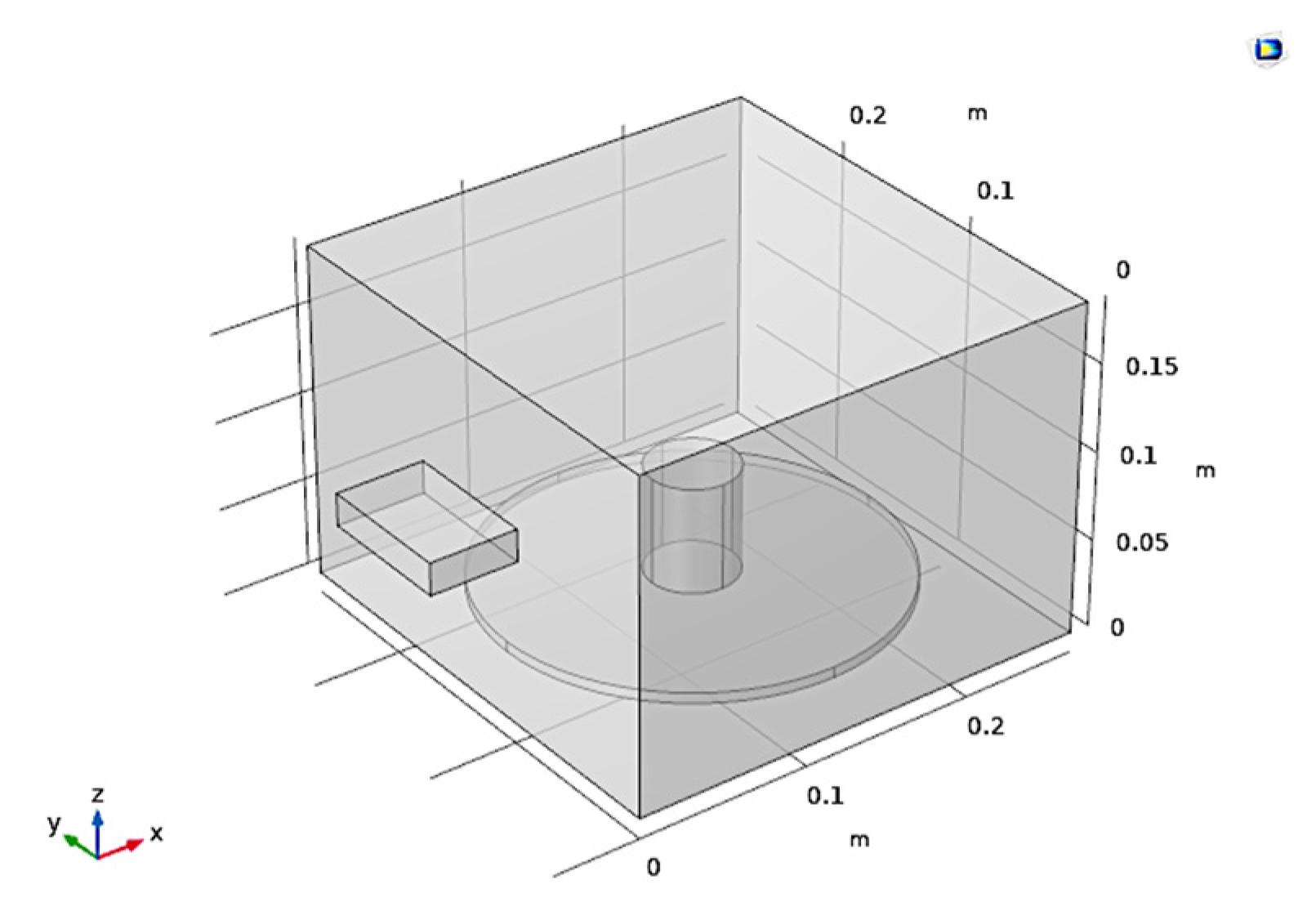
2.5. System Design
2.6. Initial Conditions
2.7. Boundary Conditions
2.8. Assumptions
- The microwave only heats the specimen placed inside it and has no effect on the air or the glass container on which the specimen is placed.
- The wall material is assumed to be copper.
- The specimens placed in the microwave oven are isotropic and homogeneous.
- The electrical, magnetic and thermal properties of coal are constant.
- The motion of water molecules in the magnetic and electric field is simplified as a fixed mass transfer.
- The chemical reaction of objects is ignored (the ignition temperature of coal is 360 °C [20]).
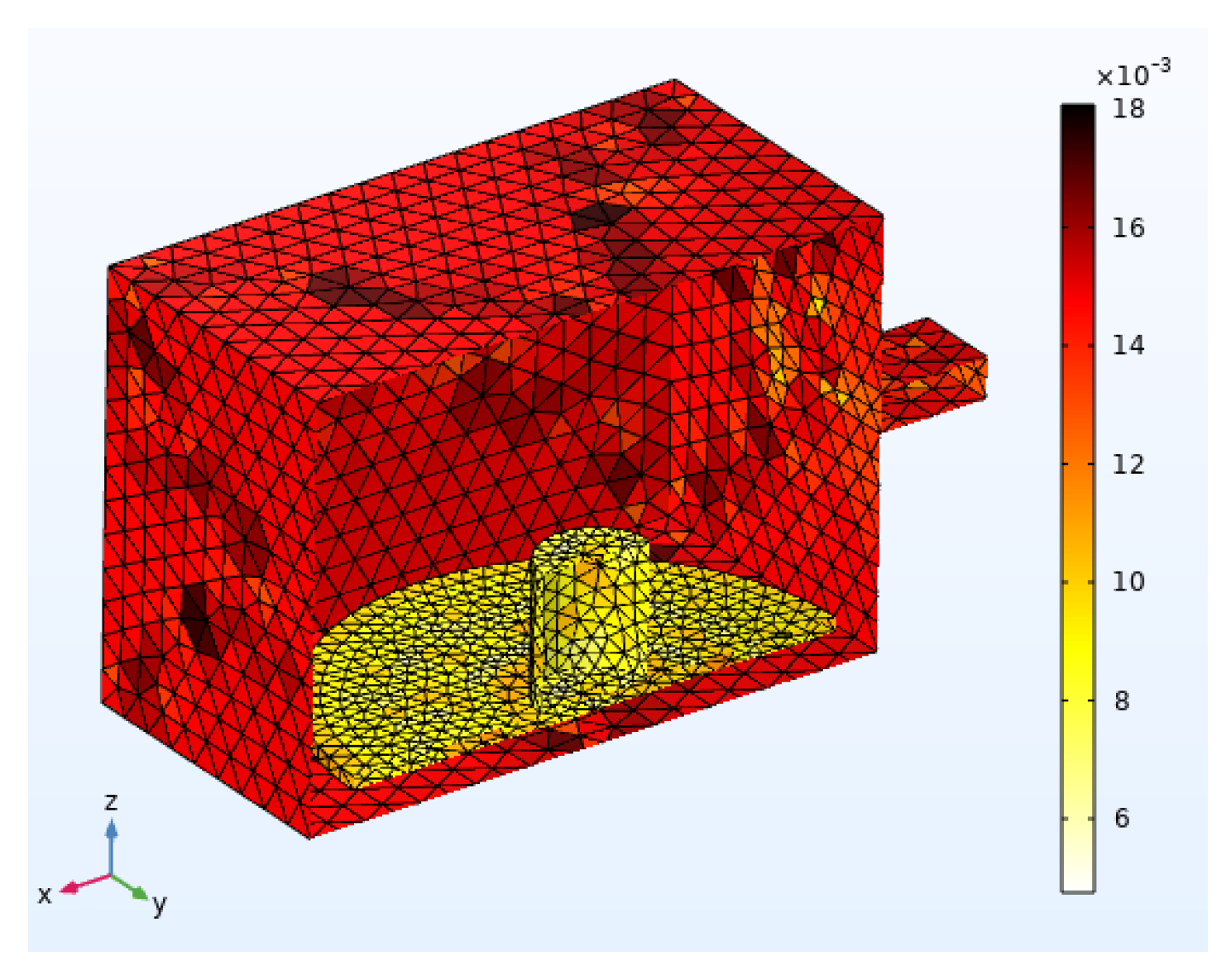
3. Methodology
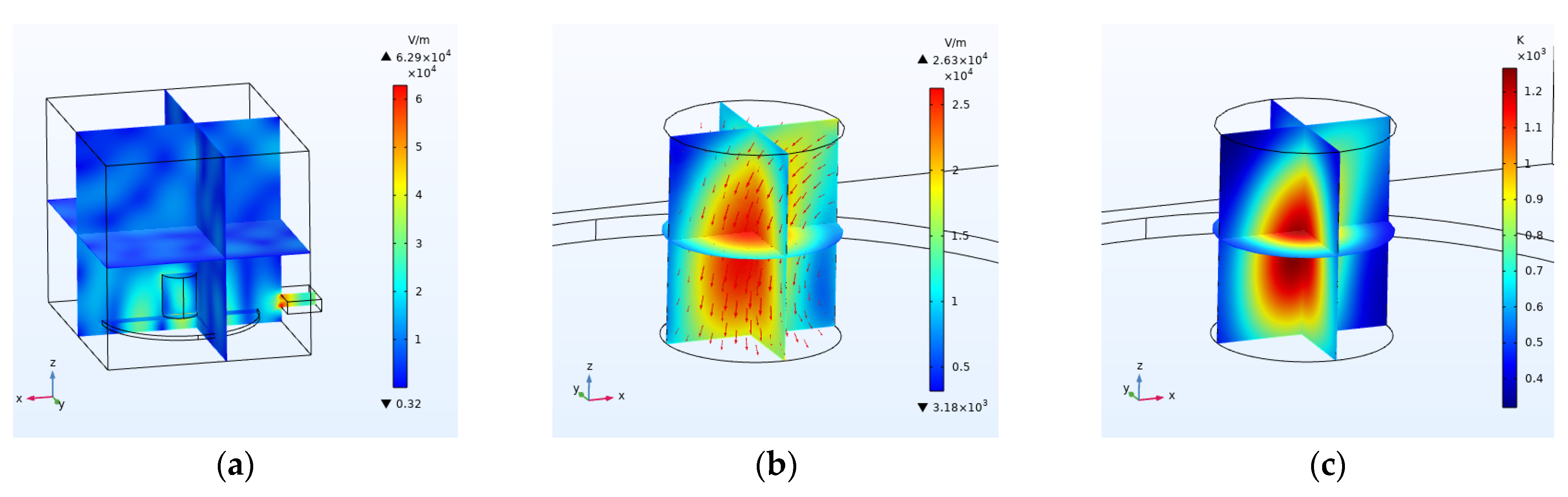
4. Tests and Simulations
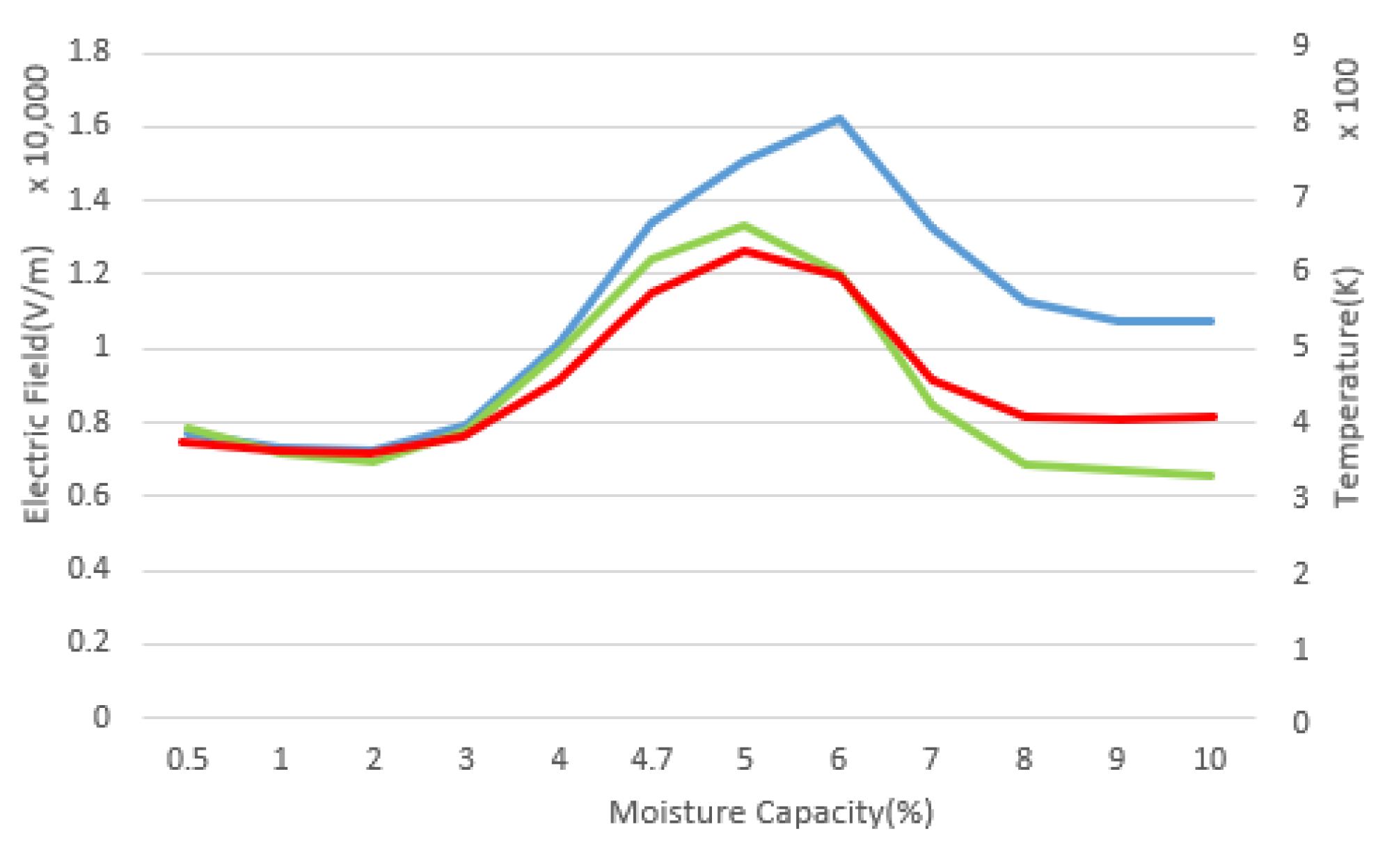
4.1. Coal Moisture Capacity
4.2. Effect of Microwave Operating Frequency
4.3. Effect of the Microwave Power and Heating Time
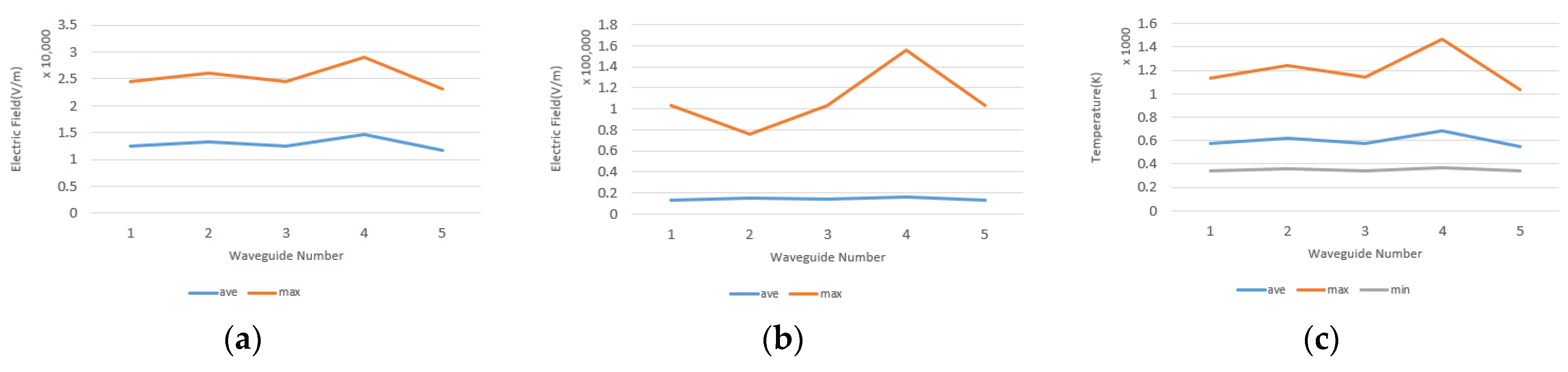
4.4. Effect of the Waveguide Port Location
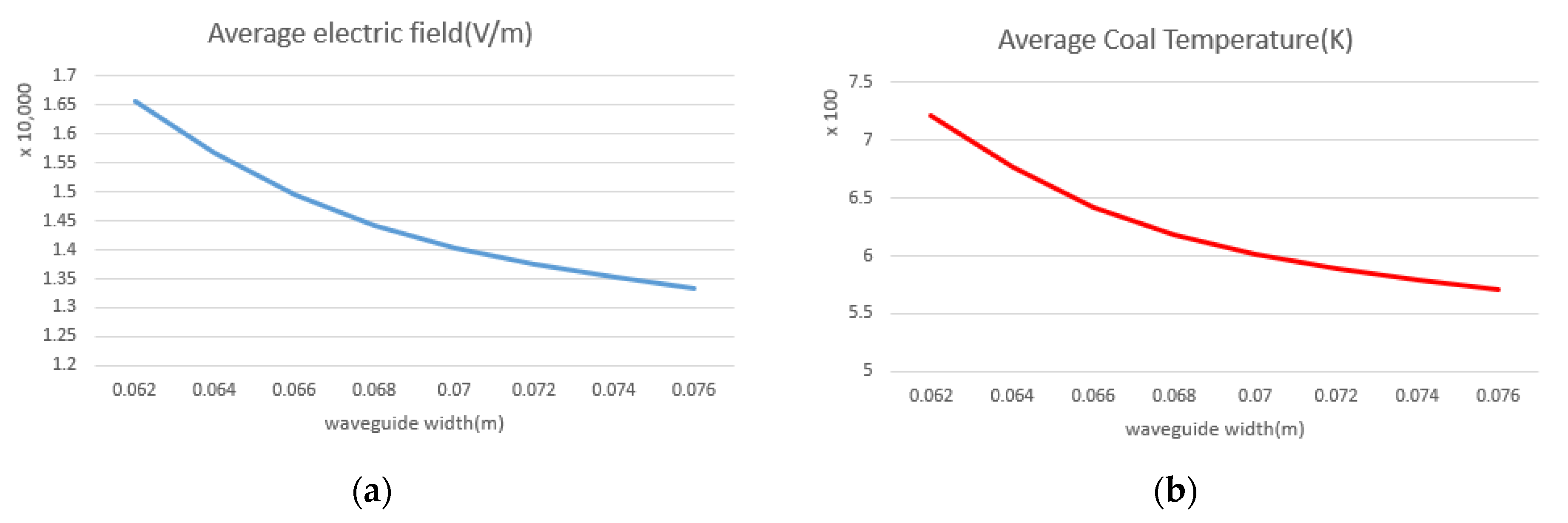
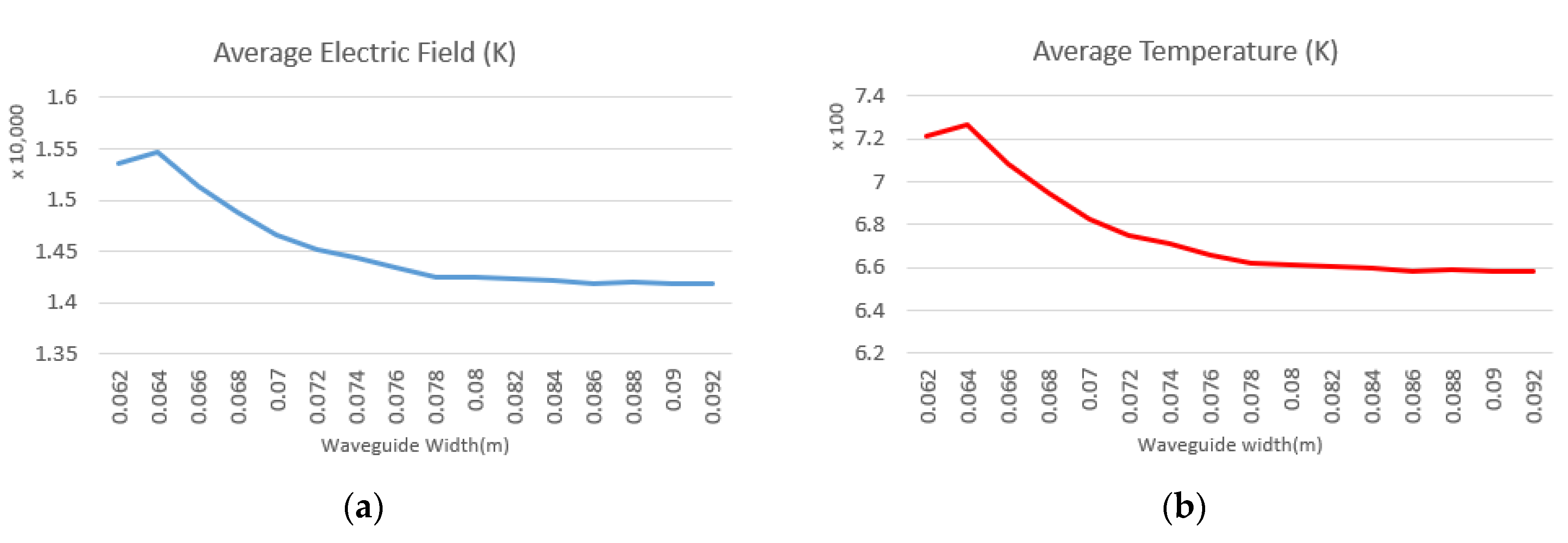
4.5. Effect of the Waveguide Chamber Size
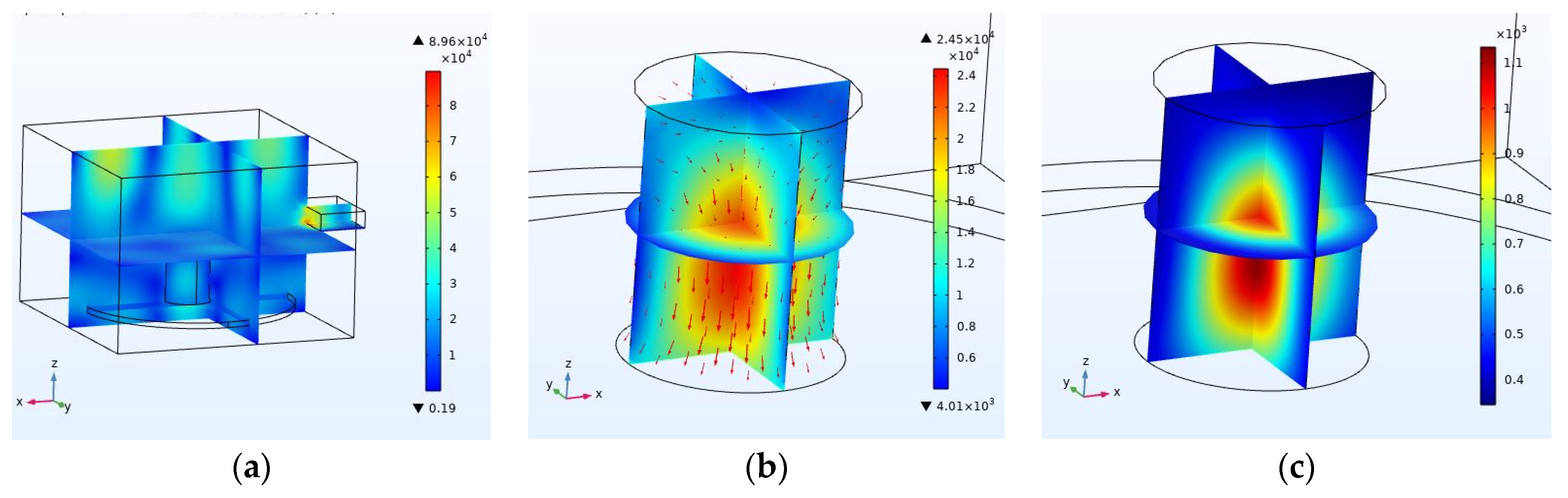
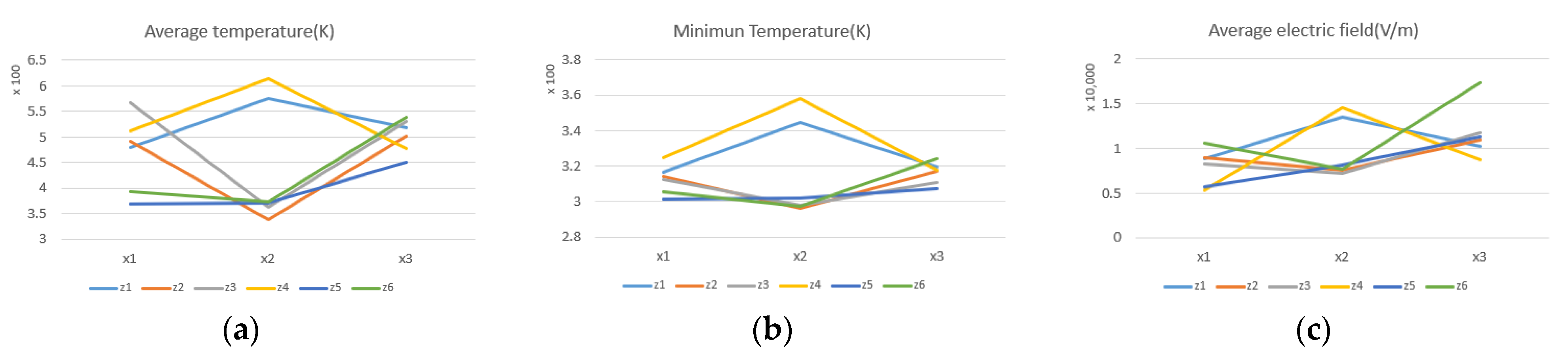
4.6. Place of Coal
5. Changing Cavity Size
5.1. Coal Moisture Capacity
5.2. Microwave Frequency
5.3. Microwave Time and Power
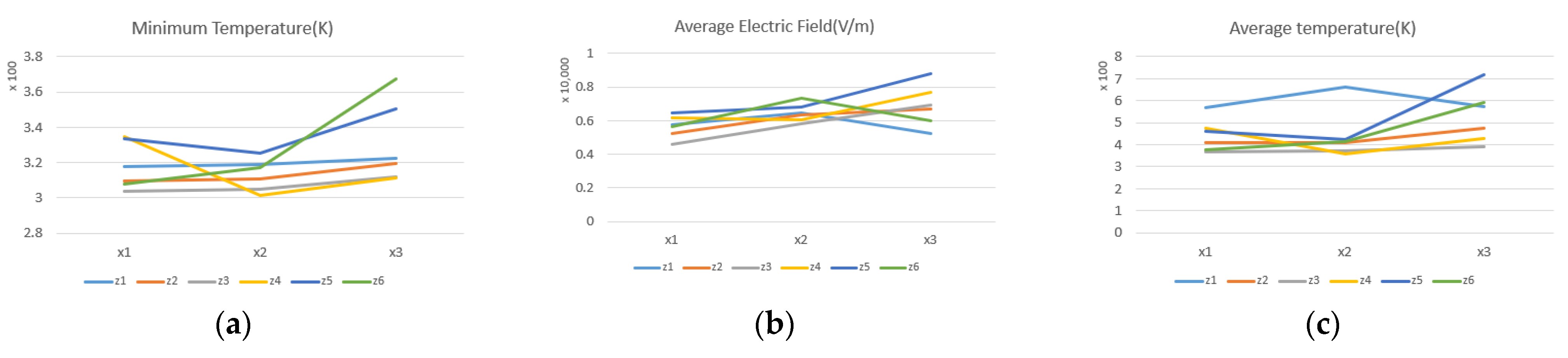
5.4. Waveguide Place
5.5. Waveguide Size
5.6. Place of Coal
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Moore, T.A. Coalbed methane: A review. Int. J. Coal Geol. 2012, 101, 36–81. [Google Scholar] [CrossRef]
- Binner, E.; Lester, E.; Kingman, S.; Dodds, C.; Robinson, J.; Wu, T.; Wardle, P.; Mathews, J.P. A Review of Microwave Coal Processing. J. Microw. Power Electromagn. Energy 2014, 48, 35–60. [Google Scholar] [CrossRef]
- Al-Jubor, A.; Johnsto, S.; Boyer, C.; Lambert, S.W.; Bustos, O.A.; Pashin, J.C.; Wray, A. Coalbed Methane: Clean Energy for the World. Oilfield Rev. 2009, 21, 4–13. [Google Scholar]
- Zhang, J.; Si, L.; Chen, J.; Kizil, M.; Wang, C.; Chen, Z. Stimulation Techniques of Coalbed Methane Reservoirs. Geofluids 2020, 2020, 5152646. [Google Scholar] [CrossRef]
- Coronel, P. Continuous Flow Processing of Foods Using Cylindrical Applicator Microwave Systems Operating at 915 Mhz. Ph.D. Thesis, North Carolina State University, Raleigh, NC, USA, 2006. [Google Scholar]
- Huang, J.; Guang, X.; Guozhong, H.; Kizil, M.; Chen, Z. A coupled electromagnetic irradiation, heat and mass transfer model for microwave heating and its numerical simulation on coal. Fuel Processing Technol. 2018, 177, 237–245. [Google Scholar] [CrossRef]
- Chemat, F. Microwave-Assisted Extraction for Bioactive Compounds; Springer: New York, NY, USA, 2012. [Google Scholar]
- Lin, B.; Li, H.; Chen, Z.; Zheng, C.; Hong, Y.; Wang, Z. Sensitivity analysis on the microwave heating of coal: A coupled electromagnetic and heat transfer model. Appl. Therm. Eng. 2017, 126, 949–962. [Google Scholar] [CrossRef]
- Peng, Z.; Lin, X.; Li, Z.; Hwang, J.-Y.; Kim, B.-G.; Zhang, Y.; Li, G.; Jiang, T. Dielectric characterization of Indonesian low-rank coal for microwave processing. Fuel Process. Technol. 2017, 156, 171–177. [Google Scholar] [CrossRef]
- Pozar, D.M. Microwave Engineering, 3rd ed.; Wiley: Hoboken, NJ, USA, 2009. [Google Scholar]
- Jebelli, A.; Mahabadi, A.; Yagoub MC, E.; Chaoui, H. Magnetic Force Calculation between Magnets and Coils. Int. J. Phys. 2020, 8, 71–80. [Google Scholar] [CrossRef]
- Jebelli, A.; Mahabadi, A.; Chaoui, H.; Yagoub, M.C.E. Simulation of Magnetic Force between Two Coaxial Coils with Air Core and Uniform Flow in MATLAB. Int. J. Phys. 2021, 9, 186–196. [Google Scholar]
- Vashisht, R.K.; Peng, Q. Efficient active chatter mitigation for boring operation by electromagnetic actuator using optimal fractional order PDλ controller. J. Mater. Processing Technol. 2020, 276, 116423. [Google Scholar] [CrossRef]
- Shao, Z.; Wang, Y.; Bai, H. A superhydrophobic textile inspired by polar bear hair for both in air and underwater thermal insulation. Chem. Eng. J. 2020, 397, 125441. [Google Scholar] [CrossRef]
- Ghalichia, F.; Behniab, S.; Sadigh Radb, E. Calculations for Ultrasonic Transducer Design and Temperature Tracking for Hyperthermia in Cervical Cancer. In Proceedings of the Iranian Conference on Biomedical Engineering, Tehran, Iran, 29 February–2 March 2004; Volume 11. [Google Scholar]
- Zhuang, C.; Xiong, Z.; Ding, H. Temperature-constrained topology optimization of nonlinear heat conduction problems. J. Comput. Des. Eng. 2021, 8, 1059–1081. [Google Scholar] [CrossRef]
- Mitrović, M.; Jokanović, B. Field tunneling and losses in narrow waveguide channel. Microw. Rev. 2010, 16, 8–13. [Google Scholar]
- Hauck, H.S. Design Considerations for Microwave Oven Cavities. IEEE Trans. Ind. Gen. Appl. 1970, IGA-6, 74–80. [Google Scholar] [CrossRef]
- Xia, Q.; Shi, T. Multiphysics Topology Optimization of Thermal Actuators by Using the Level Set-Based Multiple-Type Boundary Method. Int. J. Comput. Methods 2020, 17, 1950044. [Google Scholar] [CrossRef]
- Miroshnichenko, D.V.; Shulga, I.V.; Kaftan, Y.S.; Desna, N.A.; Nikolaichuk, Y.V. Ignition temperature of coal 3. Multicomponent coal mixtures. Coke Chem. 2017, 60, 343–347. [Google Scholar] [CrossRef]
- Wein, F.; Dunning, P.D.; Norato, J.A. A review on feature-mapping methods for structural optimization. Struct. Multidiscip. Optim. 2020, 62, 1597–1638. [Google Scholar] [CrossRef]
- Álvarez-Trejo, A.; Cuan-Urquizo, E.; Roman-Flores, A.; Trapaga-Martinez, L.G.; Alvarado-Orozco, J.M. Bézier-based metamaterials: Synthesis, mechanics and additive manufacturing. Mater. Des. 2021, 199, 109412. [Google Scholar] [CrossRef]
- Tombe, F.D. The Significance of the Poynting Vector. Gen. Sci. J. 2020, 1–9. [Google Scholar]
- Bratislav, M.; Jugoslav, J.; Tijana, D. Analysis of feed waveguide length influence on EM field in microwave applicator using TLM method AU. Facta Univ. Ser. Electron. Energetics 2008, 21, 65–72. [Google Scholar]

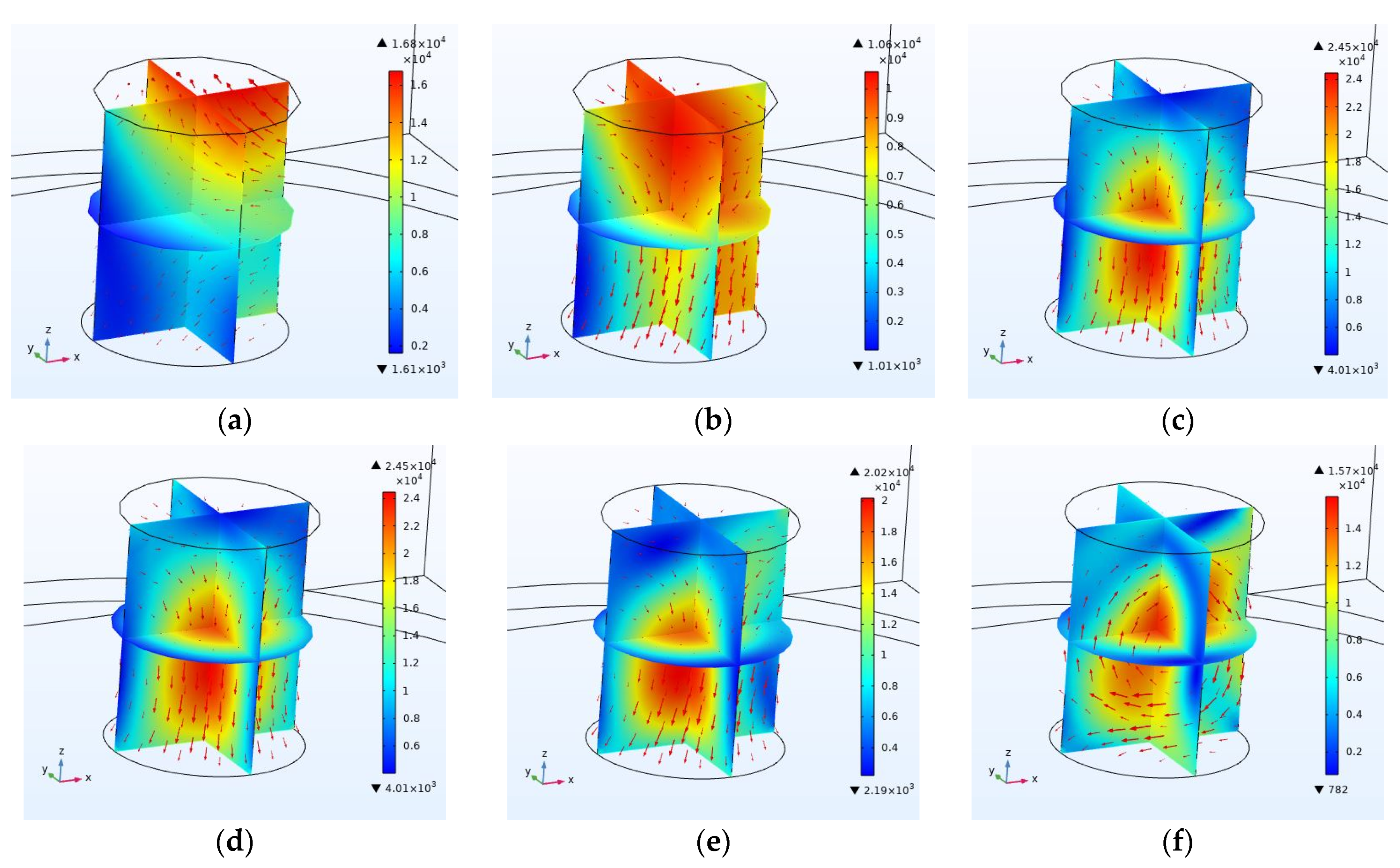

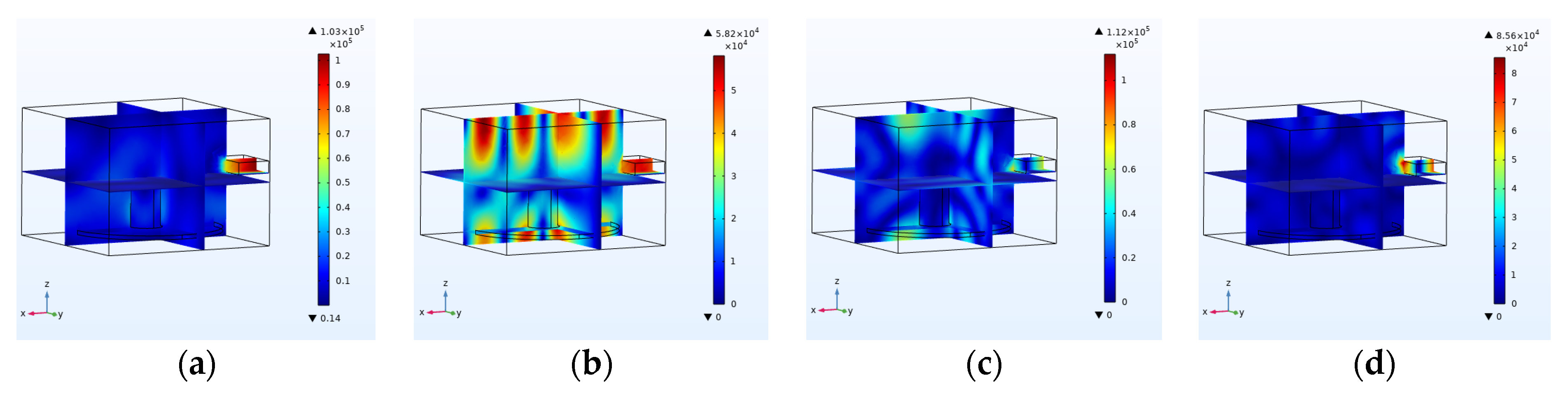
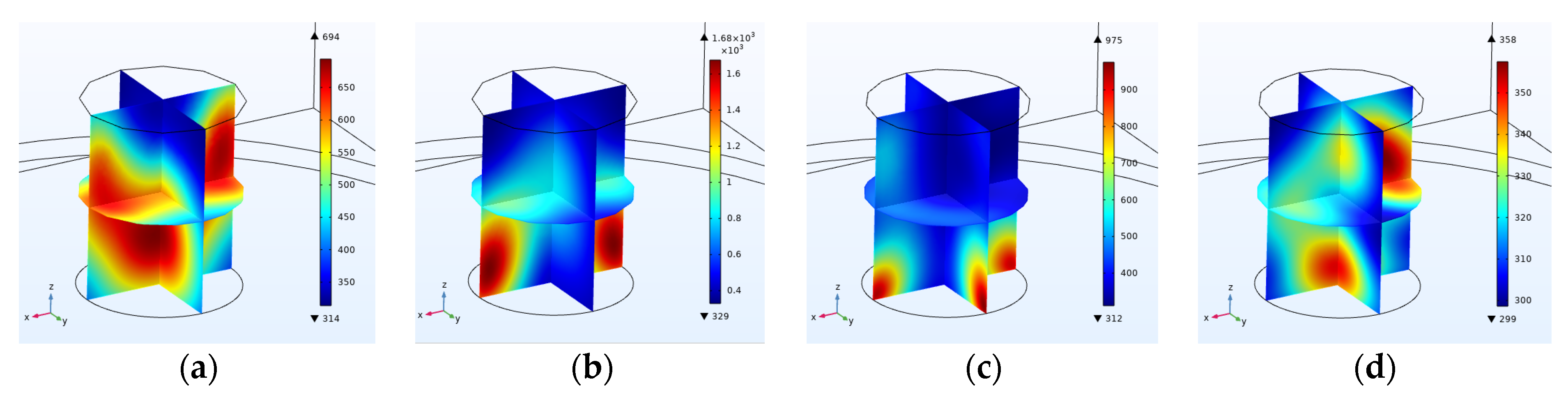

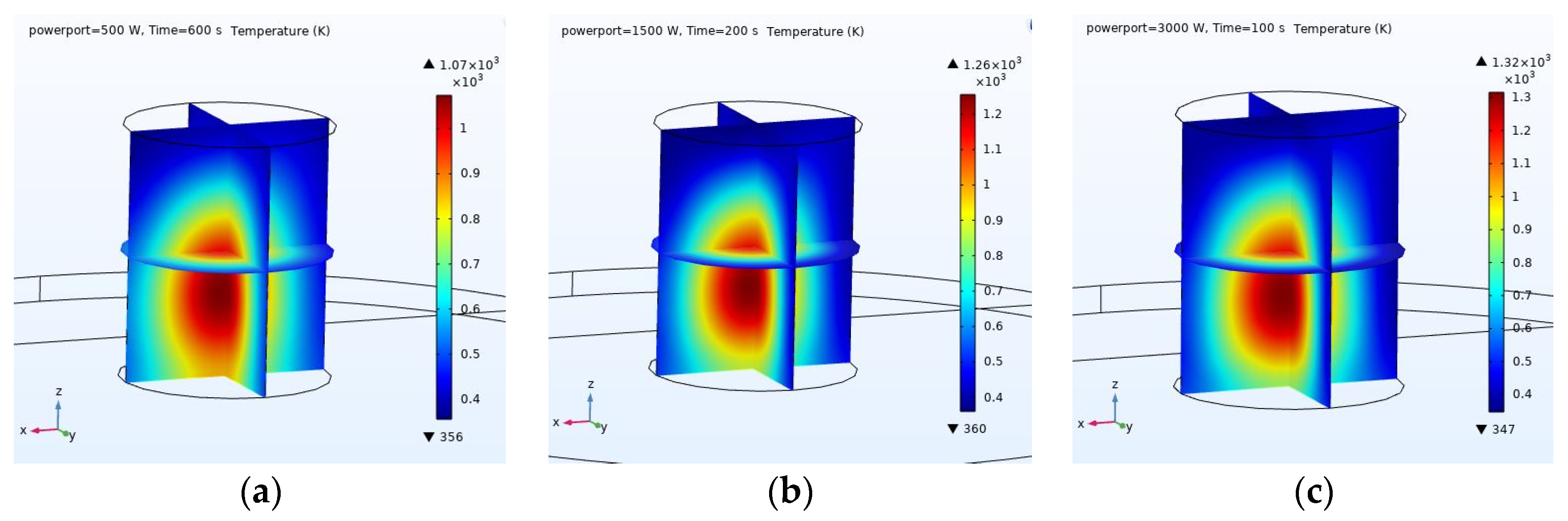





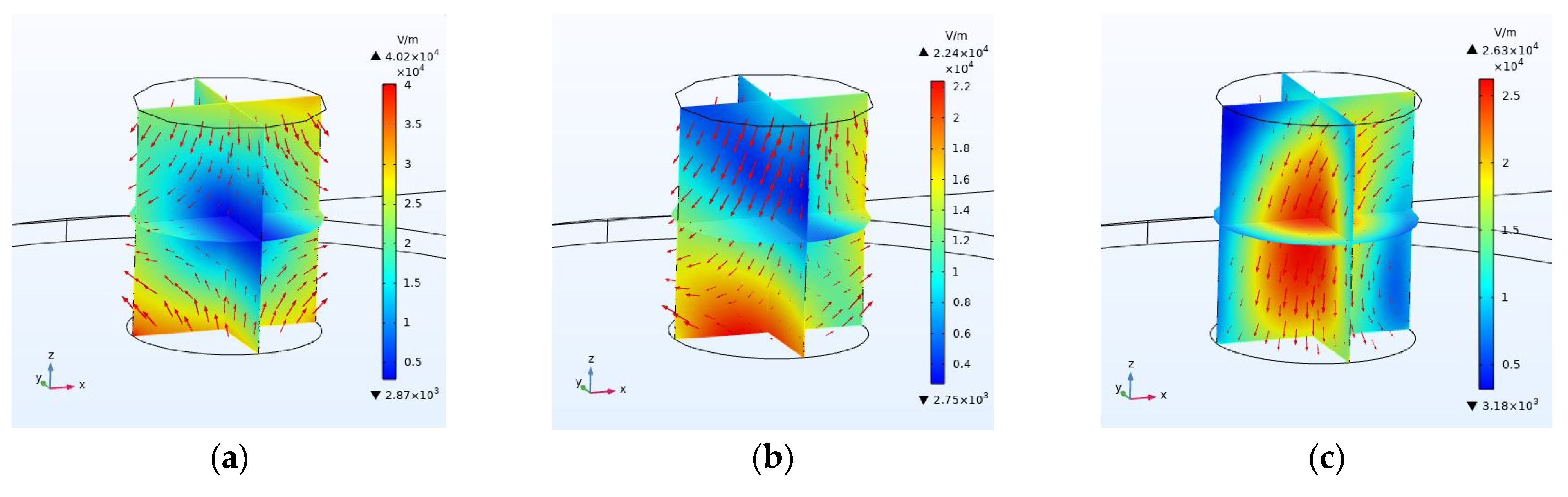
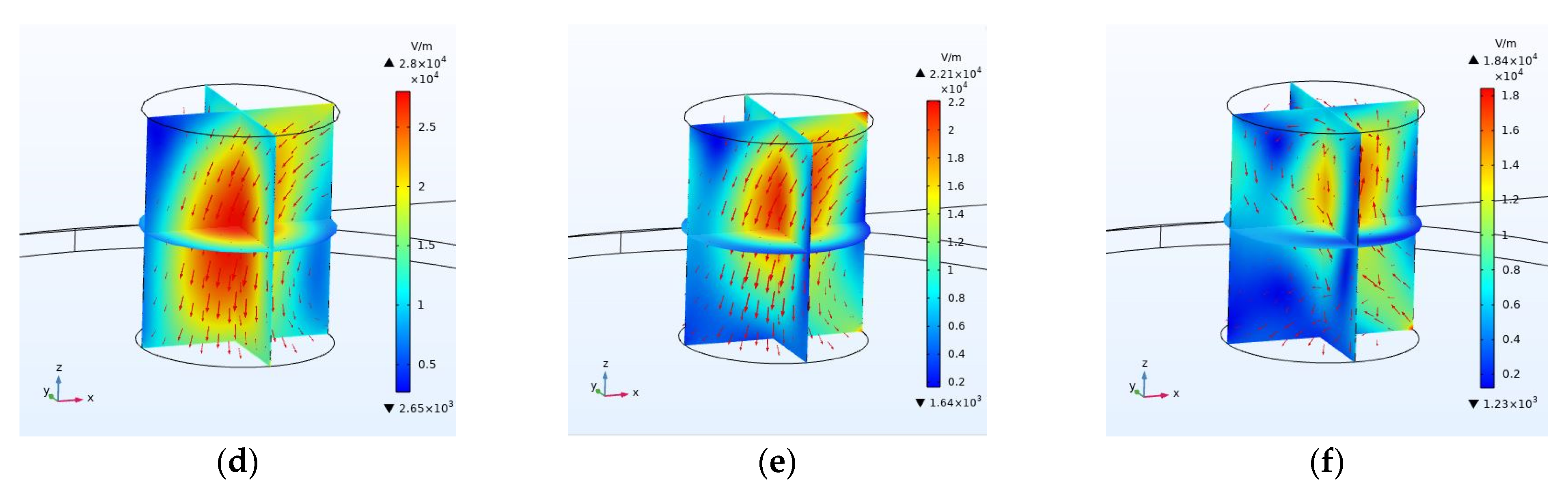
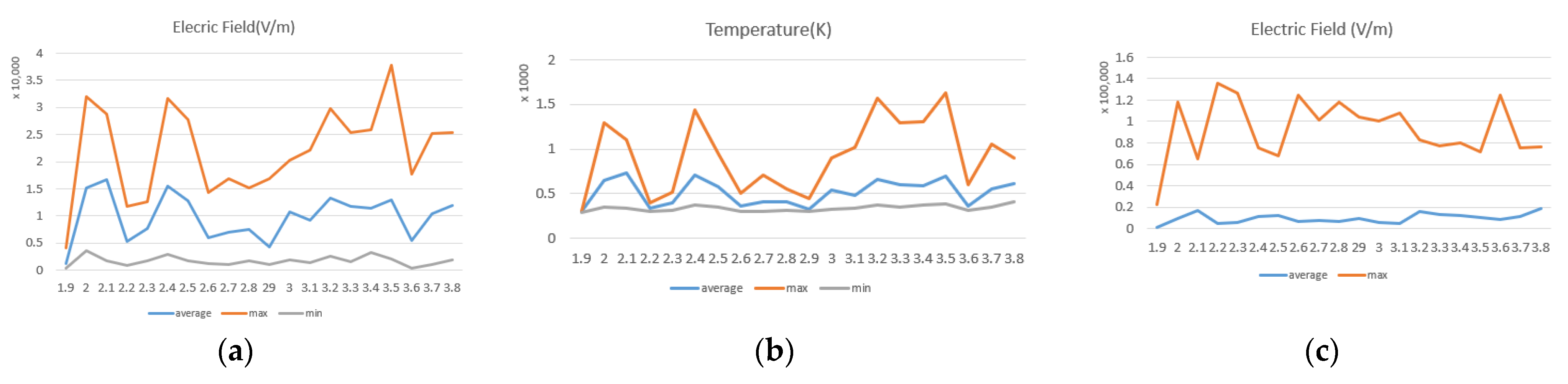



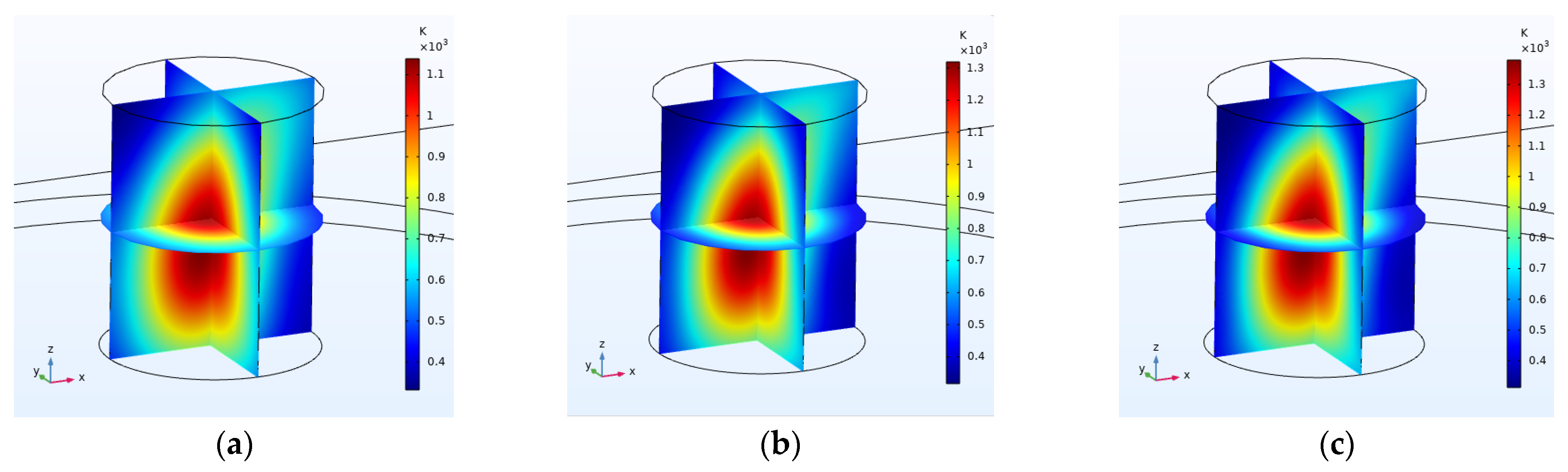




| Name | Symbol | Name | Symbol | Name | Symbol |
|---|---|---|---|---|---|
| electric field intensity (V/m) | E | electric current density (A/m2) | J | relative permittivity | εr |
| magnetic field intensity (A/m) | H | electric charge density (C/m3) | P | free space wave number | k0 |
| electric flux density (C/m) | D | permeability (H/m) | μ | conductivity | σ |
| magnetic flux density (Wb/m) | B | relative permeability | μr | angular frequency | ω |
| magnetic current density (V/m2) | M | permittivity (F/m) | ε | surface normal vector | N |
| density (kg/m3) | ρ | heat flux (W/m2) | q | mass averaged velocity vector (m/s) | u |
| specific heat capacity at constant stress (J/(kg·K)) | Cp | material’s conductivity | k | reaction rate for the species (mol/(m3·s)) | R |
| absolute temperature (K) | T | time | t | diffusive flux vector (mol/(m2·s) | Jm |
| heat source (W/m3) | Q | concentration of species (mol/m3) | c | Cutoff frequency | fc |
| velocity vector of translational motion (m/s) | U | diffusion coefficient (m2/s) | Dc | Speed of light | C0 |
| wavelength | λ | moisture conductivity | km | dynamic viscosity (kg/m·s) | v |
| Rayleigh number | RaL | specific moisture capacity | Cm | mass transfer coefficient | kc |
| length | L |
| Width (mm) | Depth (mm) | Height (mm) | Radius (mm) | |
|---|---|---|---|---|
| Microwave oven | 267 | 270 | 188 | - |
| Waveguide | 50 | 78 | 18 | - |
| Glass plate | - | - | 6 | 113.5 |
| Sample | - | - | 60 | 25 |
| Name | Units | Values | |||
|---|---|---|---|---|---|
| Material | - | Coal | Glass | Copper | Air |
| Relative permittivity (ε′-jε″) | - | Variable | 2.55 | 1 | 1 |
| Relative permeability (μ′-jμ″) | - | 1 | 1 | 1 | 1 |
| Electrical conductivity | S/m | 0.02 | 0 | 5.998 × 107 | 0 |
| Thermal conductivity | W/(m·k) | 0.478 | - | 400 | 0.0256 |
| Density | Kg/m3 | 1300 | - | 8960 | 1.204 |
| Heat capacity at constant pressure | J/(kg·K) | 4186.8 | - | 385 | 1015.1 |
| Specific Moisture Capacity (Cm %) | Real Permittivity (ε′) | Imaginary Permittivity (ε″) | Diffusion Coefficient (D m2/s) |
|---|---|---|---|
| 0.5 | 0.578 | 0.161 | 1.47 × 10−2 |
| 1 | 0.852 | 0.178 | 7.37 × 10−3 |
| 2 | 1.401 | 0.211 | 3.69 × 10−3 |
| 3 | 1.949 | 0.245 | 2.46 × 10−3 |
| 4 | 2.498 | 0.278 | 1.84 × 10−3 |
| 4.7 | 2.882 | 0.301 | 1.57 × 10−3 |
| 5 | 3.046 | 0.311 | 1.47 × 10−3 |
| 6 | 3.595 | 0.345 | 1.23 × 10−3 |
| 7 | 4.143 | 0.378 | 1.05 × 10−3 |
| 8 | 4.692 | 0.411 | 9.22 × 10−4 |
| 9 | 5.240 | 0.444 | 8.19 × 10−4 |
| 10 | 5.789 | 0.478 | 7.37 × 10−4 |
| Parameter | Unit | Value | |||||
|---|---|---|---|---|---|---|---|
| Power | W | 500 | 1000 | 1500 | 2000 | 2500 | 3000 |
| Time | s | 600 | 300 | 200 | 150 | 120 | 100 |
| Waveguide | Width (m) | Height (m) | Depth (m) |
|---|---|---|---|
| 1 | 0.078 | 0.018 | 0.05 |
| 2 | 0.078 | 0.039 | 0.05 |
| 3 | 0.078 | 0.018 | 0.1 |
| 4 | 0.064 | 0.018 | 0.05 |
| 5 | 0.091 | 0.018 | 0.05 |
| z1 = 0.022 | z2 = 0.042 | z3 = 0.062 | z4 = 0.082 | z5 = 0.102 | z6 = 0.122 | |
|---|---|---|---|---|---|---|
| x1 = 0.03 m | 8871.3 | 8972.2 | 8216.8 | 5301.3 | 5633 | 10,621 |
| x2 = 0.1335 m | 13,468 | 7576.1 | 7252.4 | 14,502 | 8077.2 | 7613 |
| x3 = 0.237 m | 10,248 | 10,938 | 11,740 | 8768.7 | 11,305 | 17,366 |
| Waveguide | Width (m) | Height (m) | Depth (m) |
|---|---|---|---|
| 1 | 0.078 | 0.018 | 0.05 |
| 2 | 0.078 | 0.039 | 0.05 |
| 3 | 0.078 | 0.018 | 0.1 |
| 4 | 0.064 | 0.018 | 0.05 |
| 5 | 0.091 | 0.018 | 0.05 |
| z1 = 0.022 | z2 = 0.042 | z3 = 0.062 | z4 = 0.082 | z5 = 0.102 | z6 = 0.122 | |
|---|---|---|---|---|---|---|
| x1 = 0.03 m | 48,921 | 17,035 | 12,489 | 17,755 | 18,440 | 12,992 |
| x2 = 0.1485 m | 25,998 | 15,330 | 16,072 | 17,902 | 14,317 | 14,453 |
| x3 = 0.267 m | 22,924 | 18,672 | 13,708 | 15,357 | 28,802 | 23,911 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jebelli, A.; Mahabadi, A.; Ahmad, R. Numerical Simulation and Optimization of Microwave Heating Effect on Coal Seam Permeability Enhancement. Technologies 2022, 10, 70. https://doi.org/10.3390/technologies10030070
Jebelli A, Mahabadi A, Ahmad R. Numerical Simulation and Optimization of Microwave Heating Effect on Coal Seam Permeability Enhancement. Technologies. 2022; 10(3):70. https://doi.org/10.3390/technologies10030070
Chicago/Turabian StyleJebelli, Ali, Arezoo Mahabadi, and Rafiq Ahmad. 2022. "Numerical Simulation and Optimization of Microwave Heating Effect on Coal Seam Permeability Enhancement" Technologies 10, no. 3: 70. https://doi.org/10.3390/technologies10030070
APA StyleJebelli, A., Mahabadi, A., & Ahmad, R. (2022). Numerical Simulation and Optimization of Microwave Heating Effect on Coal Seam Permeability Enhancement. Technologies, 10(3), 70. https://doi.org/10.3390/technologies10030070








