An a Priori Discussion of the Fill Front Stability in Semisolid Casting
Abstract
:1. Introduction
2. Methodology
3. Theoretical Framework
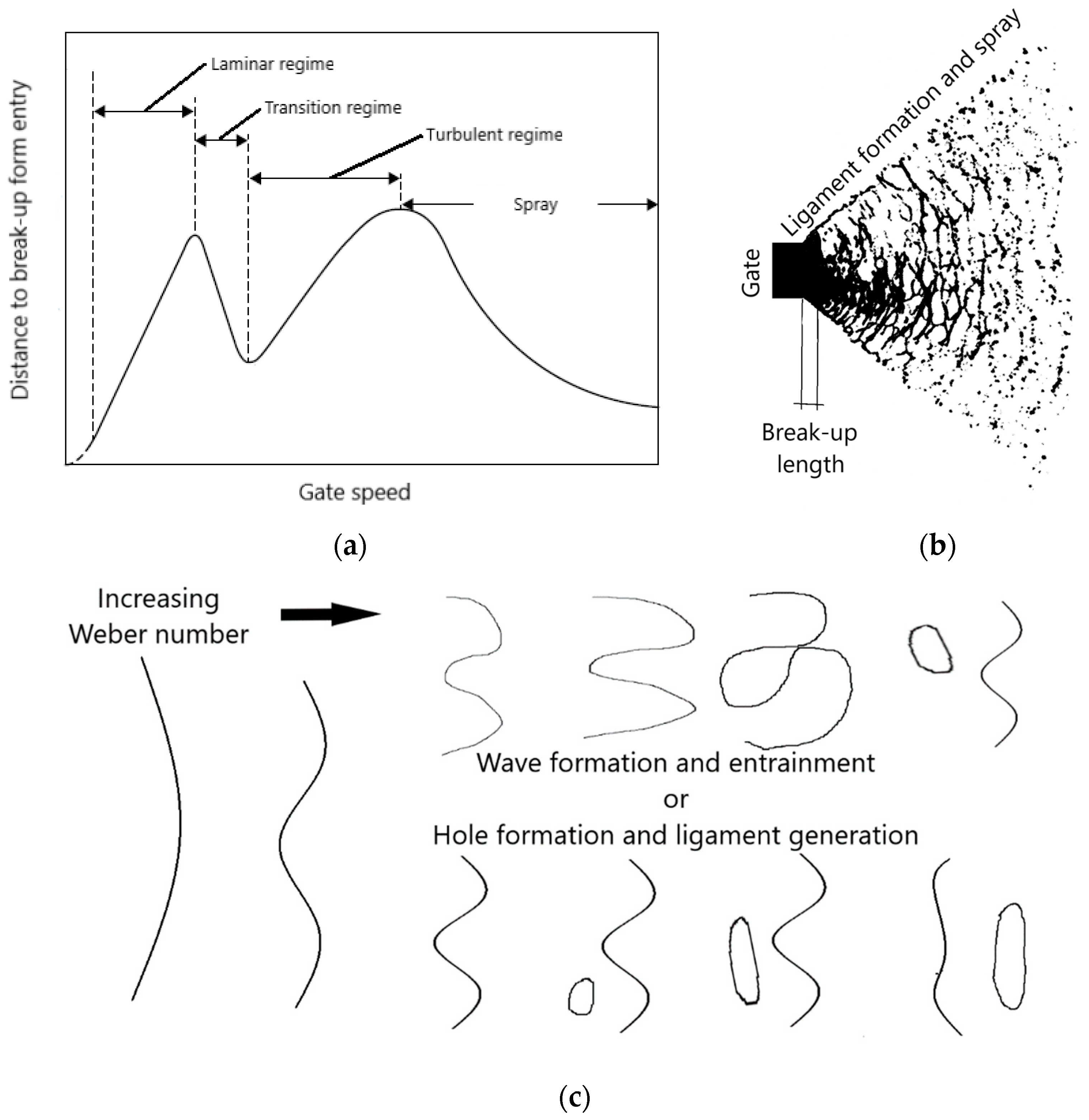
3.1. Turbulence, Surface Stability and Fill Front Break-Up
3.2. Surface Tension and Shear Strength Build-Up in the Mushy State
4. Discussion
4.1. Effect of a Solid Fraction Present on the Weber Number and Front Stability
4.2. Effect of the Solid Fraction of the Spray Behaviour
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Campbell, J. Complete Casting Handbook Metal Casting Processes, Metallurgy, Techniques and Design, 2nd ed; Butterworth-Heinemann: Oxford, UK, 2015; ISBN 978-0-444-63509-9. [Google Scholar]
- Jarfors, A.E.W.; Seifeddine, S. Metal Casting; Springer: Berlin, Germany, 2015; ISBN 9781447146704/9781447146698. [Google Scholar]
- Jarfors, A.E.W.; Zheng, J.C.; Chen, L.; Yang, J. Recent Advances in Commercial Application of the Rheometal Process in China and Europe. Solid State Phenom. 2019, 285, 405–410. [Google Scholar] [CrossRef]
- Li, D.Q.; Zhang, F.; Midson, S.P.; Liang, X.K.; Yao, H. Recent Developments of Rheo-Diecast Components for Transportation Markets. Solid State Phenom. 2019, 285, 417–422. [Google Scholar] [CrossRef]
- Jarfors, A.E.W. A Comparison between Semisolid Casting Methods for Aluminium Alloys. Metals 2020, 10, 1368. [Google Scholar] [CrossRef]
- Atkinson, H.V. Semisolid Processing of Metallic Materials. Mater. Sci. Technol. 2010, 26, 1401–1413. [Google Scholar] [CrossRef] [Green Version]
- Jarfors, A.E.W. Pressure Different Casting. Encycl. Mater. Met. Alloys 2022, 4, 117–128. [Google Scholar] [CrossRef]
- Jarfors, A.E.W. Semisolid Casting of Metallic Parts and Structures; Elsevier Ltd.: Amsterdam, The Netherlands, 2022; Volume 4, ISBN 9780128197264. [Google Scholar]
- Street, A.C. The Diecasting Handbook; Portcullis Press Ltd.: Redhill, UK, 1977. [Google Scholar]
- Janudom, S.; Wannasin, J.; Basem, J.; Wisutmethangoon, S. Characterization of Flow Behavior of Semi-Solid Slurries Containing Low Solid Fractions in High-Pressure Die Casting. Acta Mater. 2013, 61, 6267–6275. [Google Scholar] [CrossRef]
- Lefebvre, A.H.; Mcdonell, V.G. Atomization and Sprays, 2nd ed.; CRC Press: Boca Raton, FL, USA, 1989; ISBN 9781498736268. [Google Scholar]
- Hao, S.; Hu, B.; Pehlke, R. Atomization in High Pressure Die Casting—A Problem and a Challenge. Die Cast. Eng. 1998, 42, 42. [Google Scholar]
- Saeedipour, M.; Pirker, S.; Schneiderbauer, S. Numerical Study on Liquid Jet Breakup and Droplet-Wall Interaction in High Pressure Die Casting Process. In Proceedings of the ICLASS 2015—13th International Conference on Liquid Atomization and Spray Systems, Tainan, Taiwan, 23–27 August 2015. [Google Scholar]
- Saeedipour, M.; Schneiderbauer, S.; Pirker, S.; Bozorgi, S. Prediction of Surface Porosity Defects in High Pressure Die Casting. TMS Annu. Meet. 2015, 155–163. [Google Scholar] [CrossRef]
- Miller, R.A. Casting Solutions for Readiness, Thin Wall and High Strength Die Casting Alloys; The Ohio State University: Columbus, OH, USA, 2017; Available online: https://files.core.ac.uk/pdf/23/84591373.pdf (accessed on 26 May 2022).
- Pan, Q.Y.; Apelian, D.; Alexandrou, A.N. Yield Behavior of Commercial Al-Si Alloys in the Semisolid State. Metall. Mater. Trans. B 2004, 35, 1187–1202. [Google Scholar] [CrossRef]
- Dinsdale, A.T.; Quested, P.N. The Viscosity of Aluminium and Its Alloys—A Review of Data and Models. J. Mater. Sci. 2004, 39, 7221–7228. [Google Scholar] [CrossRef]
- Zhang, F.; Du, Y.; Liu, S.; Jie, W. Modeling of the Viscosity in the AL-Cu-Mg-Si System: Database Construction. Calphad 2015, 49, 79–86. [Google Scholar] [CrossRef]
- Ma, Z.; Zhang, H.; Fu, H.; Fonseca, J.; Yang, Y.; Du, M.; Zhang, H. Modelling Flow-Induced Microstructural Segregation in Semi-Solid Metals. Mater. Des. 2022, 213, 110364. [Google Scholar] [CrossRef]
- Das, P.; Samanta, S.K.; Dutta, P. Rheological Behavior of Al-7Si-0.3Mg Alloy at Mushy State. Metall. Mater. Trans. B Process Metall. Mater. Process. Sci. 2015, 46, 1302–1313. [Google Scholar] [CrossRef]

| Process | Gate Speed | v/fs | Gate Thickness | Weber Numbers for the Gate Widths 3 | ||
|---|---|---|---|---|---|---|
| (m/s) | (m/s) | (mm) | (mm) | |||
| 10 | 100 | 300 | ||||
| HPDC 1 fs = 0.0 | 45 | N/A | 2 | 6242.98 | 6732.63 | 6821.80 |
| 35 | N/A | 4 | 6923.80 | 7989.00 | 8199.24 | |
| 30 | N/A | 6 | 7043.37 | 8638.09 | 8976.84 | |
| SSM 2 fs = 0.0 | 8 | N/A | 2 | 197.31 | 212.78 | 215.60 |
| 4 | N/A | 4 | 90.43 | 104.35 | 107.09 | |
| 3.5 | N/A | 6 | 95.87 | 117.57 | 122.18 | |
| SSM 2 fs = 0.15 | 8 | 53 | 2 | 2.68 | 2.89 | 2.93 |
| 4 | 27 | 4 | 1.23 | 1.42 | 1.46 | |
| 3.5 | 23 | 6 | 1.30 | 1.60 | 1.66 | |
| SSM 2 fs = 0.25 | 8 | 32 | 2 | 0.79 | 0.85 | 0.86 |
| 4 | 16 | 4 | 0.36 | 0.42 | 0.43 | |
| 3.5 | 14 | 6 | 0.38 | 0.47 | 0.49 | |
| SSM 2 fs = 0.35 | 8 | 23 | 2 | 0.26 | 0.28 | 0.28 |
| 4 | 11 | 4 | 0.12 | 0.14 | 0.14 | |
| 3.5 | 10 | 6 | 0.13 | 0.15 | 0.16 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jarfors, A.E.W.; Zhang, Q.; Jonsson, S. An a Priori Discussion of the Fill Front Stability in Semisolid Casting. Technologies 2022, 10, 67. https://doi.org/10.3390/technologies10030067
Jarfors AEW, Zhang Q, Jonsson S. An a Priori Discussion of the Fill Front Stability in Semisolid Casting. Technologies. 2022; 10(3):67. https://doi.org/10.3390/technologies10030067
Chicago/Turabian StyleJarfors, Anders E. W., Qing Zhang, and Stefan Jonsson. 2022. "An a Priori Discussion of the Fill Front Stability in Semisolid Casting" Technologies 10, no. 3: 67. https://doi.org/10.3390/technologies10030067
APA StyleJarfors, A. E. W., Zhang, Q., & Jonsson, S. (2022). An a Priori Discussion of the Fill Front Stability in Semisolid Casting. Technologies, 10(3), 67. https://doi.org/10.3390/technologies10030067







