Assessing the Performance of Hypersonic Inlets by Applying a Heat Source with the Throttling Effect
Abstract
1. Introduction
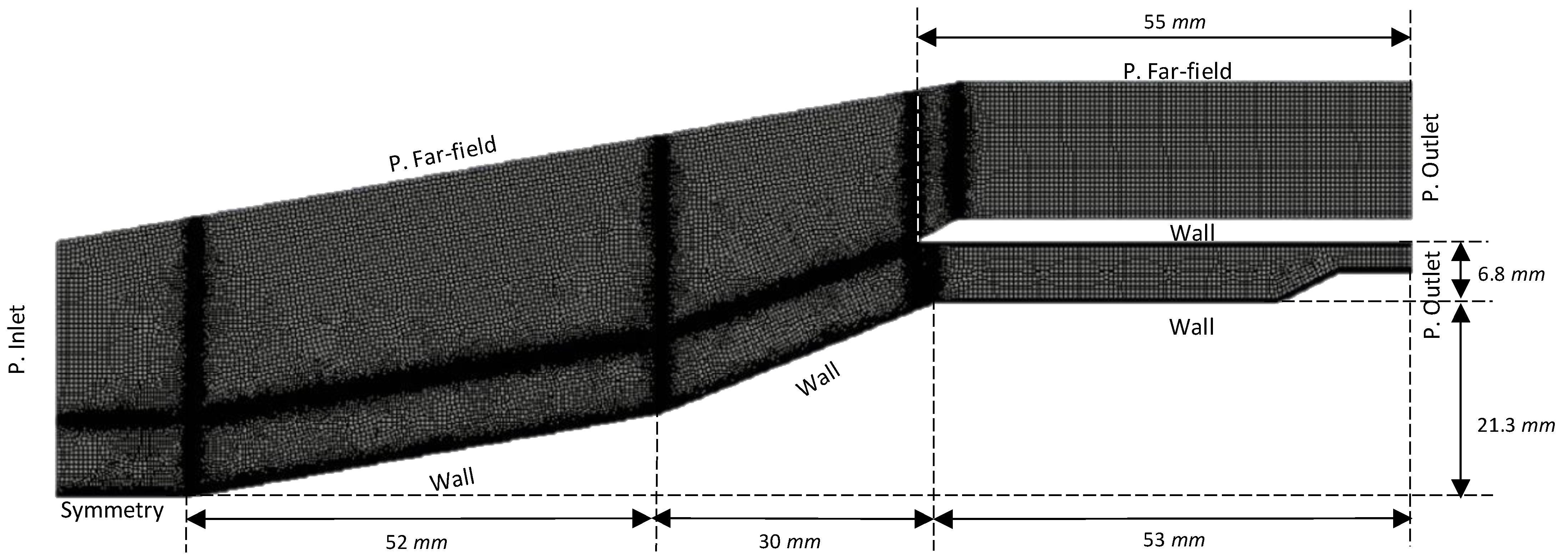
2. Numerical Work
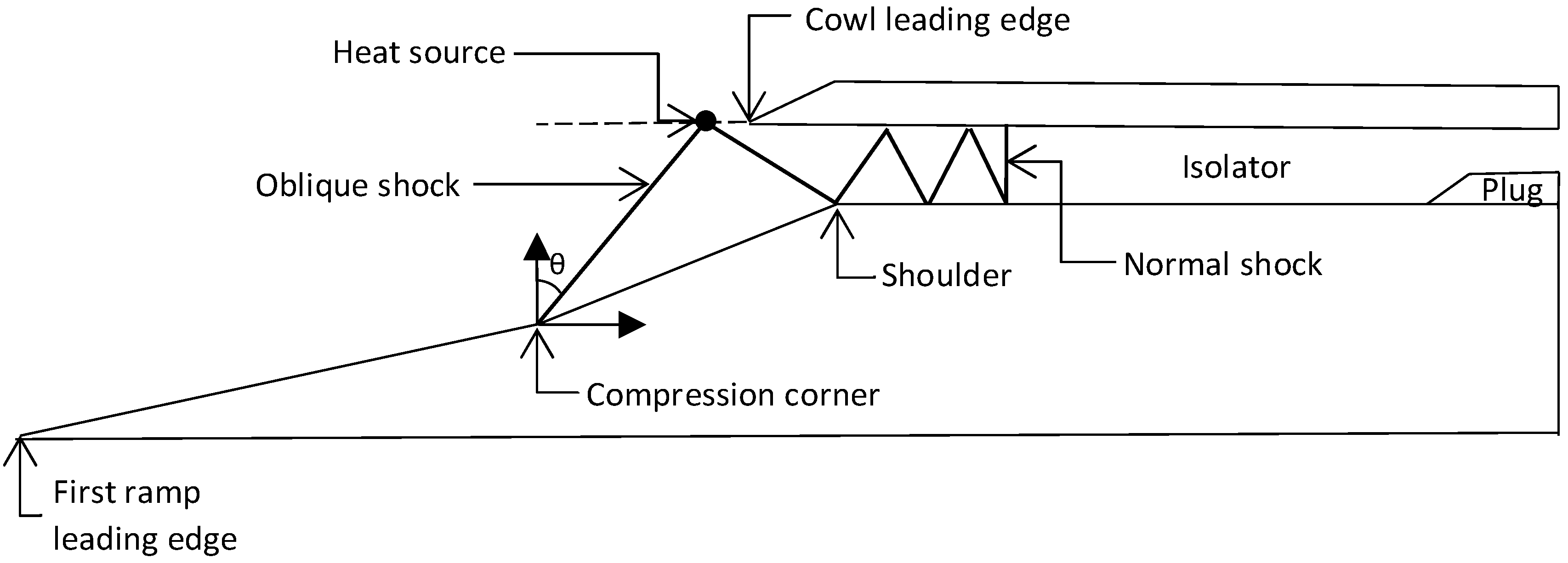
2.1. Position of the Heat Source
2.2. Performance Parameters and Operating Conditions
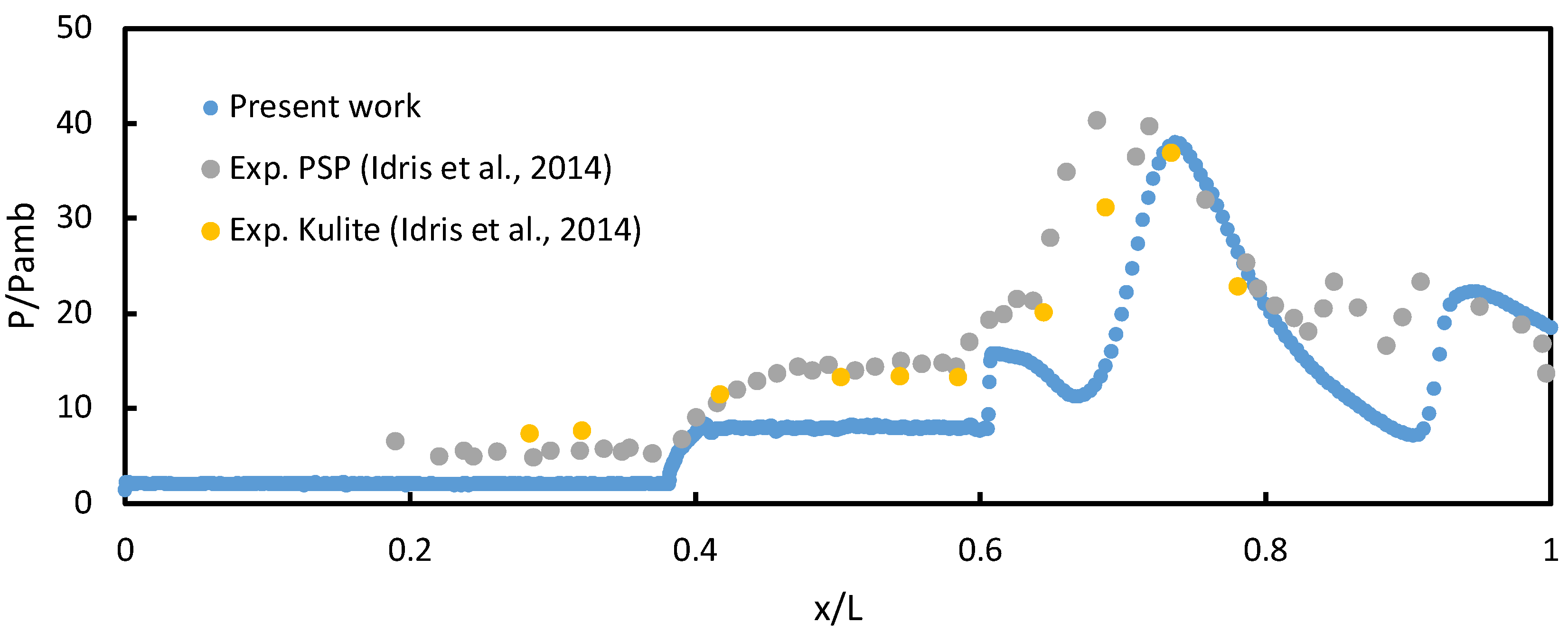
2.3. Validation
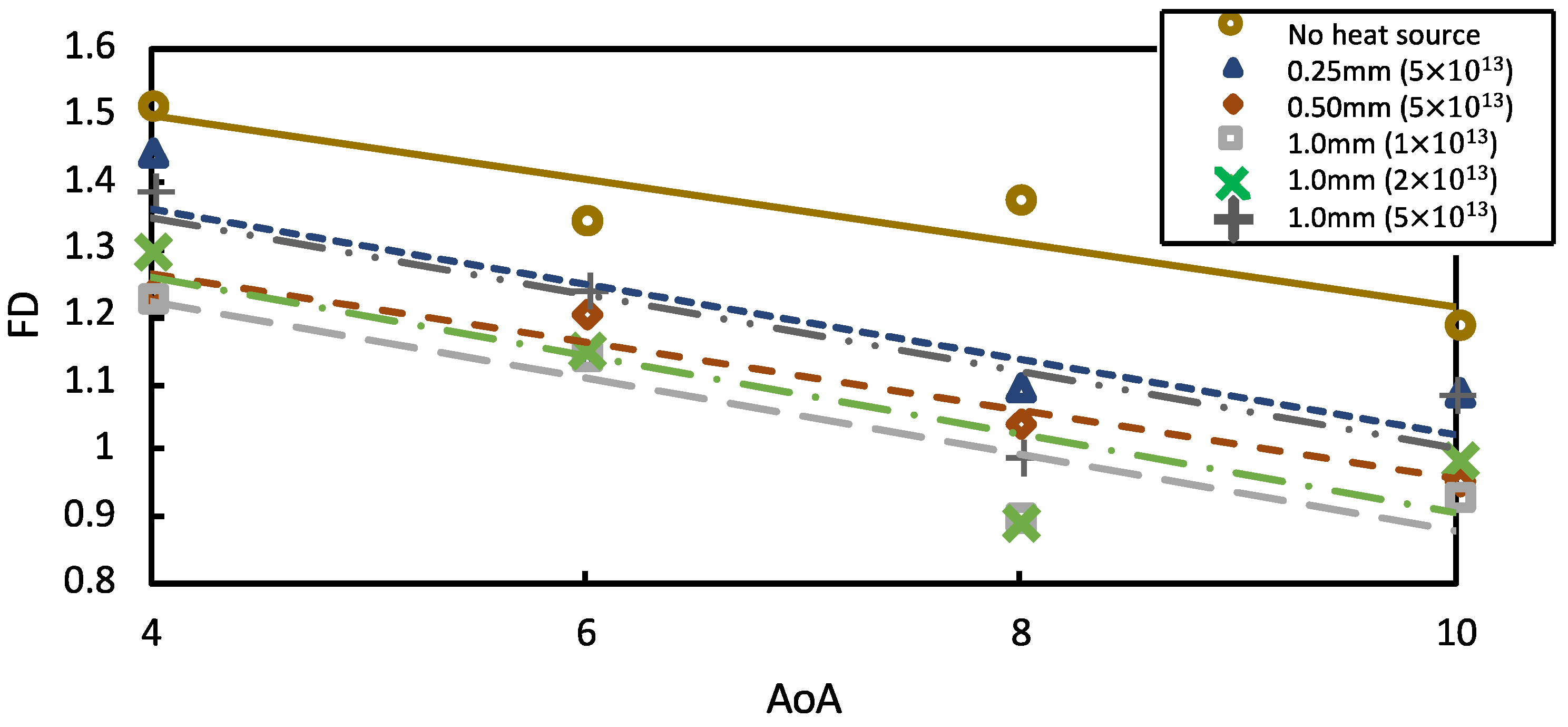
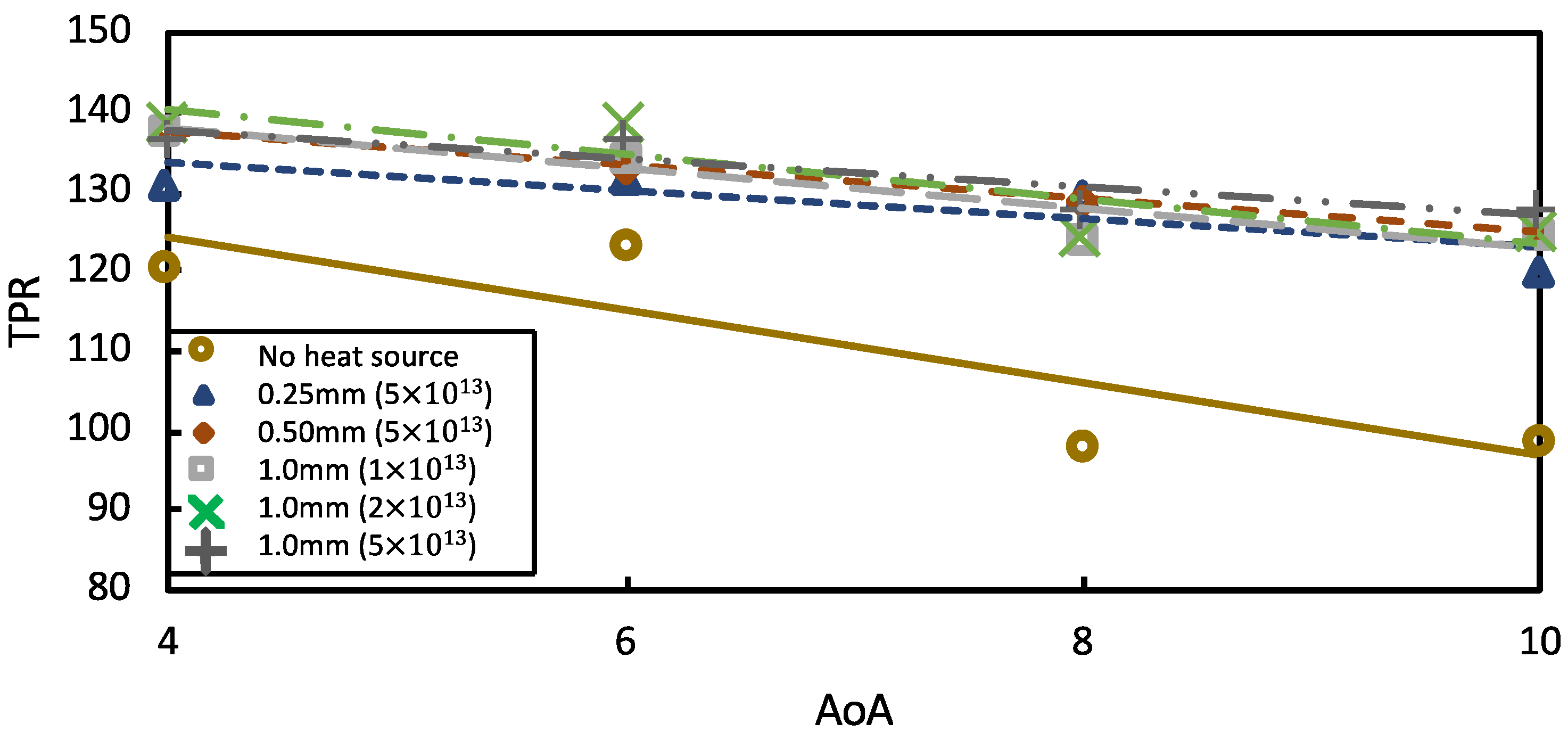
3. Results and Discussion
Internal Shock Structures
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| Mach number | |
| Stagnation pressure (Pa) | |
| Pressure at Station 0 (Pa) | |
| Pressure at Station 3 (Pa) | |
| FD | Flow distortion |
| TPR | Total pressure recovery |
| TR | Throttling ratio |
| Plug area (m3) | |
| Cross-sectional isolator area (m3) | |
| Total pressure at the inlet’s exit (Pa) | |
| Stagnation pressure at the inlet’s exit (Pa) | |
| Maximum total pressure at the inlet’s exit (Pa) | |
| Minimum total pressure at the inlet’s exit (Pa) | |
| Average total pressure at the inlet’s exit (Pa) |
References
- Van Wie, D.M.; Kwok, F.T.; Walsh, R.F. Starting characteristics of supersonic inlets. In Proceedings of the 32nd Joint Propulsion Conference and Exhibit, Lake Buena Vista, FL, USA, 1–3 July 1996. [Google Scholar] [CrossRef]
- Kantrowitz, A.; Donaldson, C.D. Prelminiary Investigation of Supersonic Diffusors; National Advisory Committee for Aeronautics: Washington, DC, USA, 1945. [Google Scholar]
- Heiser, W.H.; Pratt, D.T. Chapter 5: Compression Systems or Components. In Hypersonic Airbreathing Propulsion; AIAA Education Series; AIAA: Washington, DC, USA, 1994. [Google Scholar]
- Tan, H.J.; Guo, R.W. Experimental study of the unstable-unstarted condition of a hypersonic inlet at mach 6. J. Propuls. Power 2007, 23, 783–788. [Google Scholar] [CrossRef]
- Wagner, J.L.; Yuceil, K.B.; Valdivia, A.; Clemens, N.T.; Dolling, D.S. Experimental investigation of unstart in an inlet/isolator model in mach 5 flow. AIAA J. 2009, 47, 1528–1542. [Google Scholar] [CrossRef]
- Tan, H.J.; Sun, S.; Yin, Z.L. Oscillatory flows of rectangular hypersonic inlet unstart caused by downstream mass-flow choking. J. Propuls. Power 2009, 25, 138–147. [Google Scholar] [CrossRef]
- Li, Z.; Gao, W.; Jiang, H.; Yang, J. Unsteady behaviors of a hypersonic inlet caused by throttling in shock tunnel. AIAA J. 2013, 51, 2485–2492. [Google Scholar] [CrossRef]
- Tan, H.J.; Li, L.G.; Wen, Y.F.; Zhang, Q.F. Experimental investigation of the unstart process of a generic hypersonic inlet. AIAA J. 2011, 49, 279–288. [Google Scholar] [CrossRef]
- Tan, H.J.; Sun, S.; Huang, H.X. Behavior of shock trains in a hypersonic inlet/isolator model with complex background waves. Exp. Fluids 2012, 53, 1647–1661. [Google Scholar] [CrossRef]
- Sun, P.Z.; Shi, H.T.; Lu, X.Y. Numerical Investigation of the Unsteady Behavior of a Hypersonic Inlet under Throttling. Procedia Eng. 2015, 126, 179–183. [Google Scholar] [CrossRef][Green Version]
- Wang, C.; Xue, L.; Tian, X. Experimental characteristics of oblique shock train upstream propagation. Chin. J. Aeronaut. 2017, 30, 663–676. [Google Scholar] [CrossRef]
- Devaraj, M.K.K.; Jutur, P.; Rao, S.M.V.; Jagadeesh, G.; Anavardham, G.T.K. Experimental investigation of unstart dynamics driven by subsonic spillage in a hypersonic scramjet intake at Mach 6. Phys. Fluids 2020, 32, 026103. [Google Scholar] [CrossRef]
- Sekar, K.R.; Karthick, S.K.; Jegadheeswaran, S.; Kannan, R. On the unsteady throttling dynamics and scaling analysis in a typical hypersonic inlet-isolator flow. Phys. Fluids 2020, 32, 126104. [Google Scholar] [CrossRef]
- Saravanan, R.; Desikan, S.L.N.; Francise, K.J.; Kalimuthu, R. Experimental investigation of start/unstart process during hypersonic intake at Mach 6 and its control. Aerosp. Sci. Technol. 2021, 113, 106688. [Google Scholar] [CrossRef]
- Devaraj, M.K.K.; Jutur, P.; Rao, S.M.V.; Jagadeesh, G.; Anavardham, G.T.K. Investigation of local unstart in a hypersonic scramjet intake at a Mach number of 6. Aerosp. Sci. Technol. 2021, 115, 106789. [Google Scholar] [CrossRef]
- Devaraj, M.K.K.; Jutur, P.; Rao, S.M.V.; Jagadeesh, G.; Anavardham, G.T.K. Length scale for the estimation of buzz frequency in the limit of high mechanical blockage in mixed-compression intakes. J. Fluid Mech. 2021, 916, R3. [Google Scholar] [CrossRef]
- Gao, W.; LI, Z.; Yang, J.; Zeng, Y. Effects of trips on the oscillatory flow of an axisymmetric hypersonic inlet with downstream throttle. Chin. J. Aeronaut. 2018, 31, 225–236. [Google Scholar] [CrossRef]
- Chen, H.; Tan, H.J.; Zhang, Q.F.; Zhang, Y. Throttling process and buzz mechanism of a supersonic inlet at overspeed mode. AIAA J. 2018, 56, 1953–1964. [Google Scholar] [CrossRef]
- Wagner, J.L.; Yuceil, K.B.; Clemens, N.T. Velocimetry measurements of unstart in an inlet-isolator model in Mach 5 flow. AIAA J. 2010, 48, 1875–1888. [Google Scholar] [CrossRef]
- Zhang, Q.F.; Tan, H.J.; Chen, H.; Yuan, Y.Q.; Zhang, Y.C. Unstart process of a rectangular hypersonic inlet at different Mach numbers. AIAA J. 2016, 54, 3681–3691. [Google Scholar] [CrossRef]
- Chang, J.; Li, N.; Xu, K.; Bao, W.; Yu, D. Recent research progress on unstart mechanism, detection and control of hypersonic inlet. Prog. Aerosp. Sci. 2017, 89, 1–22. [Google Scholar] [CrossRef]
- Im, S.K.; Do, H. Unstart phenomena induced by flow choking in scramjet inlet-isolators. Prog. Aerosp. Sci. 2018, 97, 1–21. [Google Scholar] [CrossRef]
- Kang, K.; Wermer, L.; Im, S.; Song, S.J.; Do, H. Fast-acting boundary-layer suction to control unstarting and unstarted flows. AIAA J. 2020, 58, 2475–2485. [Google Scholar] [CrossRef]
- Newsome, R.W.W. Numerical simulation of near-critical and unsteady, subcritical inlet flow. AIAA J. 1984, 22, 1375–1379. [Google Scholar] [CrossRef]
- Yamamoto, J.; Kojima, Y.; Kameda, M.; Watanabe, Y.; Hashimoto, A.; Aoyama, T. Prediction of the onset of supersonic inlet buzz. Aerosp. Sci. Technol. 2020, 96, 105523. [Google Scholar] [CrossRef]
- De Vanna, F.; Picano, F.; Benini, E.; Quinn, M.K. Large-eddy simulations of the unsteady behavior of a hypersonic intake at mach 5. AIAA J. 2021, 59, 3859–3872. [Google Scholar] [CrossRef]
- Lu, P.-J.; Jain, L.-T. Numerical investigation of inlet buzz flow. J. Propuls. Power 1998, 14, 90–100. [Google Scholar] [CrossRef]
- Trapier, S.; Deck, S.; Duveau, P. Delayed detached-eddy simulation and analysis of supersonic inlet buzz. AIAA J. 2008, 46, 118–131. [Google Scholar] [CrossRef]
- Hong, W.; Kim, C. Numerical study on supersonic inlet buzz under various throttling conditions and fluid-structure interaction. In Proceedings of the 29th AIAA Applied Aerodynamics Conference, Honolulu, HI, USA, 27–30 June 2011; pp. 1–16. [Google Scholar] [CrossRef]
- Xie, W.Z.; Wu, Z.M.; Yu, A.Y.; Guo, S. Control of severe shock-wave/boundary-layer interactions in hypersonic inlets. J. Propuls. Power 2018, 34, 614–623. [Google Scholar] [CrossRef]
- Lee, J.; Kang, S.H. Numerical study on the start and unstart phenomena in a scramjet inlet-isolator model. PLoS ONE 2019, 14, e0224994. [Google Scholar] [CrossRef] [PubMed]
- Soltani, M.R.; Daliri, A.; Sepahi Younsi, J. Effects of shock wave/boundary-layer interaction on performance and stability of a mixed-compression inlet. Sci. Iran. 2016, 23, 1811–1825. [Google Scholar] [CrossRef]
- Yuan, H.; Liu, F.; Wang, X.; Zhou, Z. Design and analysis of a supersonic axisymmetric inlet based on controllable bleed slots. Aerosp. Sci. Technol. 2021, 118, 107008. [Google Scholar] [CrossRef]
- De Vanna, F.; Bof, D.; Benini, E. Multi-Objective RANS Aerodynamic Optimization of a Hypersonic Intake Ramp at Mach 5. Energies 2022, 15, 2811. [Google Scholar] [CrossRef]
- Askari, R.; Soltani, M.R.; Mostoufi, K.; Fard, A.K.; Abedi, M. Angle of attack investigations on the performance of a diverterless supersonic inlet. J. Appl. Fluid Mech. 2019, 12, 2017–2030. [Google Scholar] [CrossRef]
- Lee, H.-J.; Jeung, I.-S. Experimental and numerical investigation on the supersonic inlet buzz with angle of attack. In Shock Waves; Springer: Berlin/Heidelberg, Gertmany, 2009; pp. 1111–1116. [Google Scholar] [CrossRef]
- Macheret, S.O.; Shneider, M.N.; Miles, R.B. Scramjet inlet control by off-body energy addition: A virtual cowl. AIAA J. 2004, 42, 2294–2302. [Google Scholar] [CrossRef]
- Kremeyer, K.; Sebastian, K.; Shu, C.-W. Computational study of shock mitigation and drag reduction by pulsed energy lines. AIAA J. 2006, 44, 1720–1731. [Google Scholar] [CrossRef][Green Version]
- Russell, A.; Myokan, M.; Bottini, H.; Sasoh, A.; Zare-Behtash, H.; Kontis, K. Application of laser energy deposition to improve performance for high speed intakes. Propuls. Power Res. 2020, 9, 15–25. [Google Scholar] [CrossRef]
- Ambe Verma, K.; Murari Pandey, K.; Ray, M.; Kumar Sharma, K. Effect of transverse fuel injection system on combustion efficiency in scramjet combustor. Energy 2021, 218, 119511. [Google Scholar] [CrossRef]
- Krause, M.; Reinartz, B.; Behr, M. Numerical analysis of transition effects in 3d hypersonic intake flows. In High Performance Computing in Science and Engineering 2009; Transactions of the High Performance Computing Center (HLRS): Stuttgart, Germany, 2010; pp. 395–409. [Google Scholar]
- Bosco, A.; Brown, L.M.; Boyce, R.R. Investigation of a Compression Corner at Hypersonic Conditions using a Reynolds Stress Model. In Proceedings of the 17th AIAA International Space Planes and Hypersonic Systems and Technologies Conference, San Francisco, CA, USA, 11–14 April 2011. [Google Scholar]
- Gnoffo, P.A.; Berry, S.A.; Norman, J.W. Van Uncertainty Assessments of 2D and Axisymmetric Hypersonic Shock Wave-Turbulent Boundary Layer Interaction Simulations at Compression Corners. In Proceedings of the 42nd AIAA thermophysics conference, Honolulu, HI, USA, 27–30 June 2011. [Google Scholar]
- Reinartz, B. Parameter study for scramjet intake concerning wall temperatures and turbulence modeling. In High Performance Computing in Science and Engineering 2011; Transactions of the High Performance Computing Center (HLRS): Stuttgart, Germany, 2012; pp. 425–436. [Google Scholar]
- Knight, D.D. Energy Deposition for High-Speed Flow Control; Cambridge University Press: Cambridge, UK, 2019; ISBN 9781316389331. [Google Scholar]
- Sepahi-Younsi, J.; Esmaeili, S. Performance Enhancement of a Supersonic Air Intake by Applying a Heat Source. J. Aerosp. Eng. 2020, 33, 04020048. [Google Scholar] [CrossRef]
- Idris, A.C.; Saad, M.R.; Zare-Behtash, H.; Kontis, K. Luminescent measurement systems for the investigation of a scramjet inlet-isolator. Sensors 2014, 14, 6606–6632. [Google Scholar] [CrossRef] [PubMed]
- Gudmundsson, S. General Aviation Aircraft Design: Applied Methods and Procedures; Elsevier Inc.: Amsterdam, The Netherlands, 2013; pp. 1–1034. ISBN 9780123973085. [Google Scholar]






| Properties | Value |
|---|---|
| Mach number | 5 |
| Stagnation pressure (MPa) | 0.65 |
| Stagnation inlet temperature (K) | 375 |
| Reynolds number (m−1) | 13.2 × 106 |
| Angle of attack (°) | 0–10 |
| Throttling ratio | 0, 0.4, 0.5, 0.6 |
| Diameter of the laser (mm) | 0.25, 0.5, 1 |
| Laser energy (Wm−3) | 1 × 1013, 2 × 1013, 5 × 1013 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zahrolayali, N.; Saad, M.R.; Idris, A.C.; Rahman, M.R.A. Assessing the Performance of Hypersonic Inlets by Applying a Heat Source with the Throttling Effect. Aerospace 2022, 9, 449. https://doi.org/10.3390/aerospace9080449
Zahrolayali N, Saad MR, Idris AC, Rahman MRA. Assessing the Performance of Hypersonic Inlets by Applying a Heat Source with the Throttling Effect. Aerospace. 2022; 9(8):449. https://doi.org/10.3390/aerospace9080449
Chicago/Turabian StyleZahrolayali, Nurfathin, Mohd Rashdan Saad, Azam Che Idris, and Mohd Rosdzimin Abdul Rahman. 2022. "Assessing the Performance of Hypersonic Inlets by Applying a Heat Source with the Throttling Effect" Aerospace 9, no. 8: 449. https://doi.org/10.3390/aerospace9080449
APA StyleZahrolayali, N., Saad, M. R., Idris, A. C., & Rahman, M. R. A. (2022). Assessing the Performance of Hypersonic Inlets by Applying a Heat Source with the Throttling Effect. Aerospace, 9(8), 449. https://doi.org/10.3390/aerospace9080449






