A Preliminary Top-Down Parametric Design of Electromechanical Actuator Position Control
Abstract
:1. Introduction
- The sizing of EMAs is highly dependent on the mission profile (time history of position and force at actuator/load interface), which affects mechanical, magnetic and thermal stresses. It involves two sizing loops because the motor sizing depends on the motor design itself (rotor inertia and mean and maximal temperatures of the windings). A simple second-order representation model of the closed-loop performance is generally used to translate the mission profile from the load to the motor shaft levels. This method ignores how the controllers will solicit the EMA in practice.
- Although the power sizing ensures sufficient power capability, there is no early validation that the choices made are consistent with the specified closed-loop performance.
- Linking formally, in a noncausal manner, the control and digital implementation parameters to the EMA dynamic specification and top-level design parameters;
- Avoiding the use of unrealistic linear control models of phenomena by verifying a posteriori the control robustness to unmodelled dynamics and nonlinearities, with resort to high-fidelity virtual tests.
2. Top-Down Controller Design
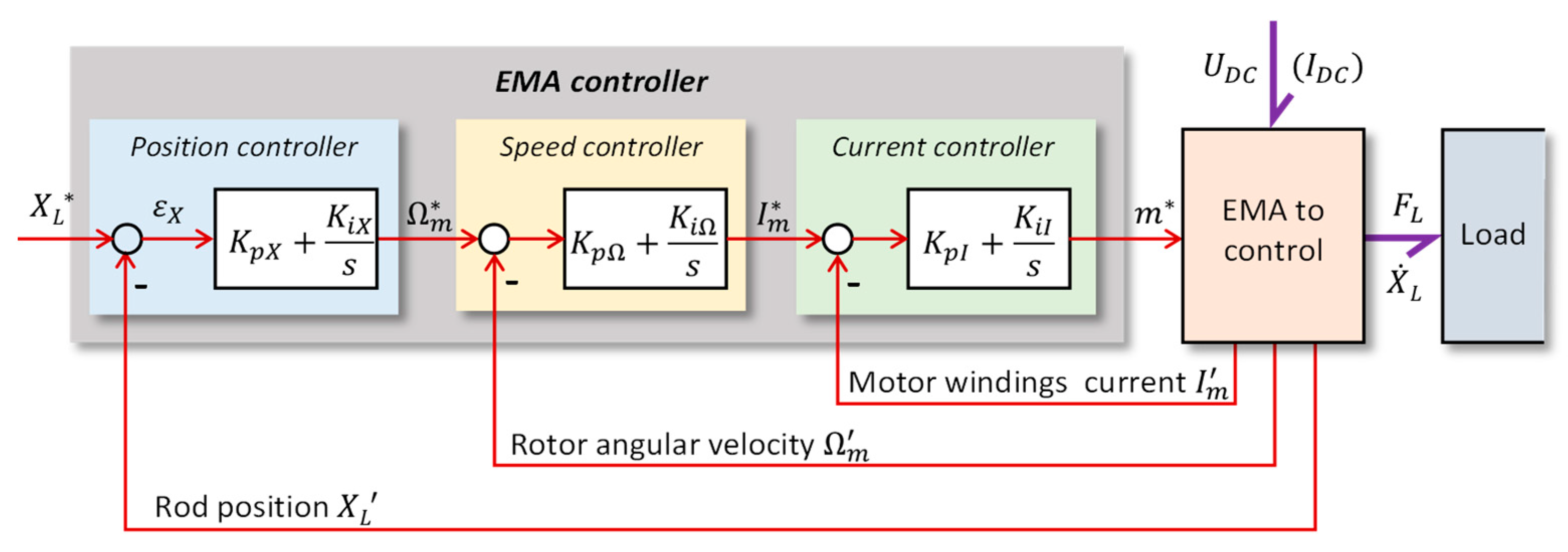
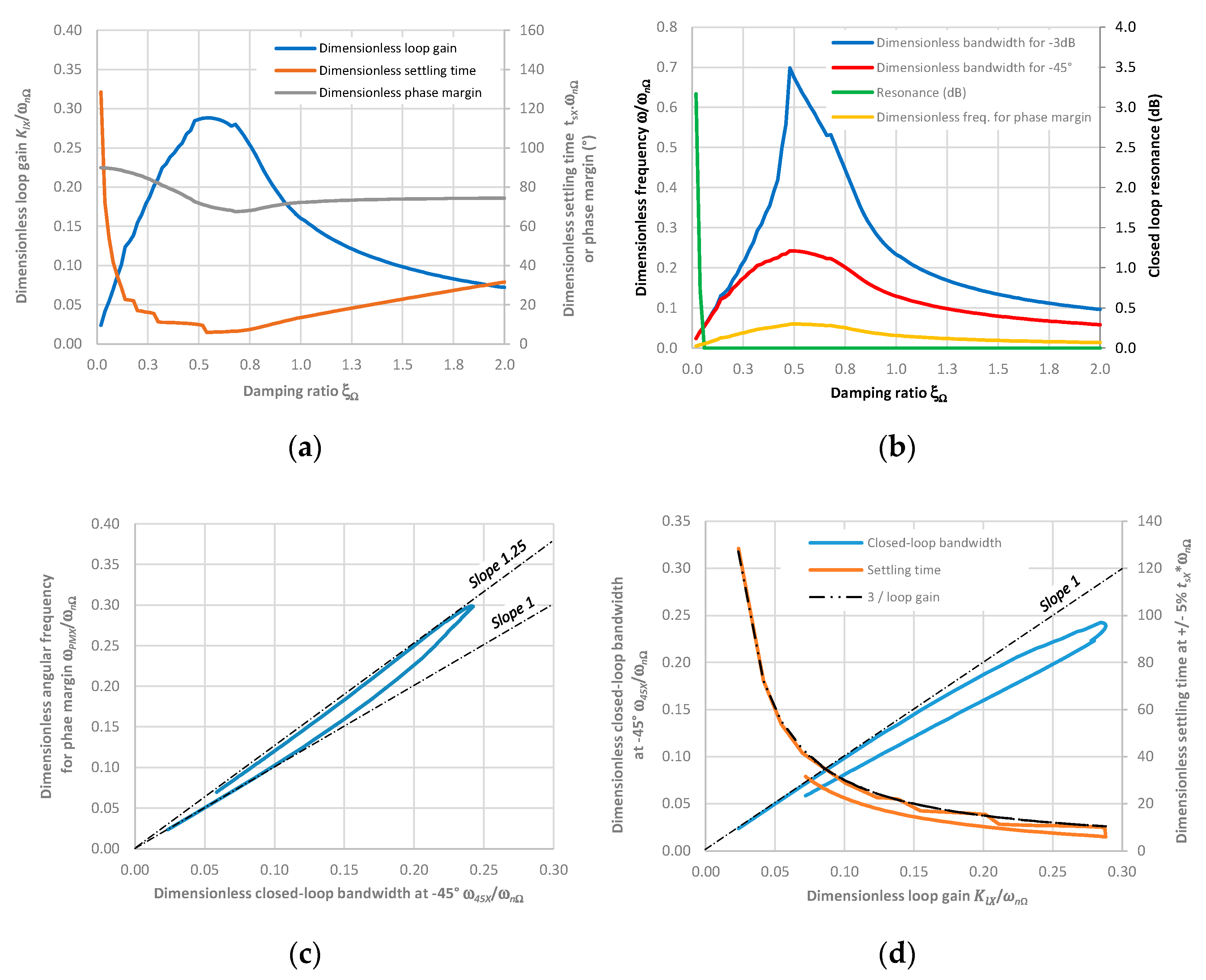
2.1. Step 1: Design of the Position Controller and Specification of the Speed Loop Dynamics
2.1.1. Performance of the Position Loop with I-P Speed Controller
2.1.2. Performance of the Position Loop with P-I Speed Controller
2.1.3. Digital Implementation of the Position Controller
- There must be at least 7 to 15 samples in the rise time of the system response to a step input; or
- The sampling frequency must be at least 15 to 25 times the closed-loop bandwidth.
- The phase lag introduced by the position measurement is not considered. Although it is generally negligible, this assumption must be verified (when the measurement chain is known), ensured by relevant specification (when the measurement chain is to be defined) or removed by adding the position measurement dynamics in Equation (13).
- For LVDTs position sensors, the demodulation filter is welcome to avoid any frequency aliasing.
2.2. Step 2: Design of the Speed Controller and Specification of the Current Loop Dynamics
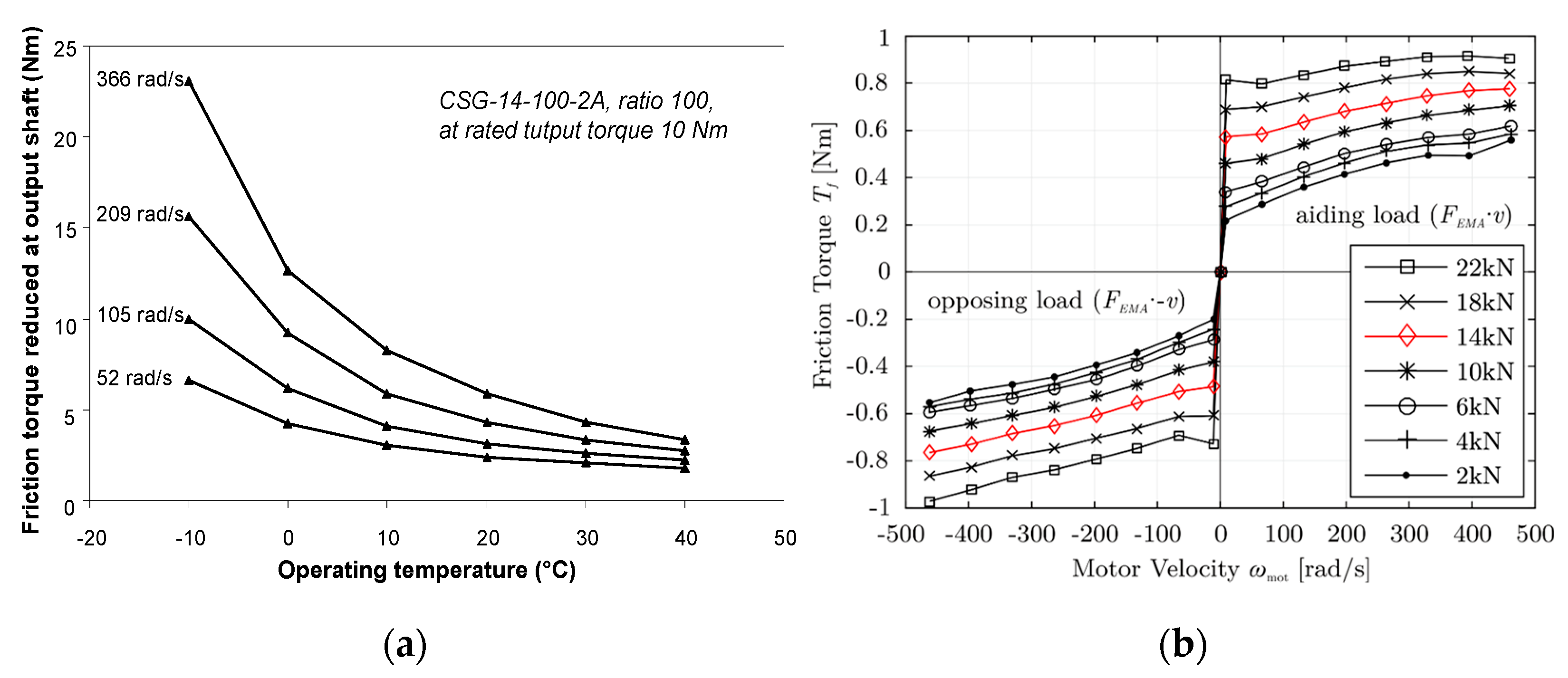
2.2.1. Viscous Friction vs. Real Friction
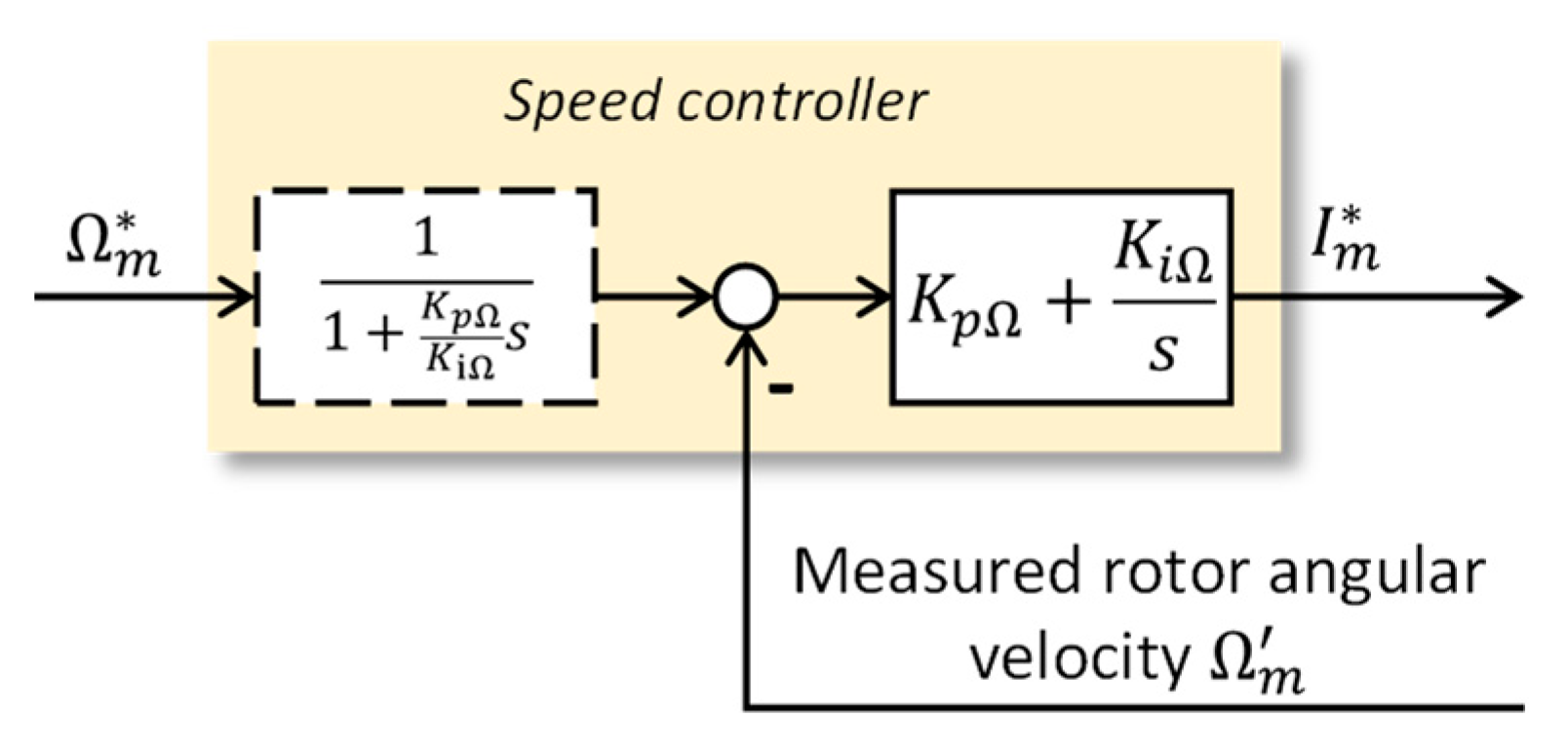
2.2.2. Setting the Speed Loop Controller
2.2.3. Digital Implementation of the Speed Controller
2.2.4. Specification of the Current Loop Dynamics
2.3. Step 3. Design of the Current Controller
2.3.1. Setting the P-I Controller of the Current Loop
2.3.2. Digital Implementation of the Current Controller
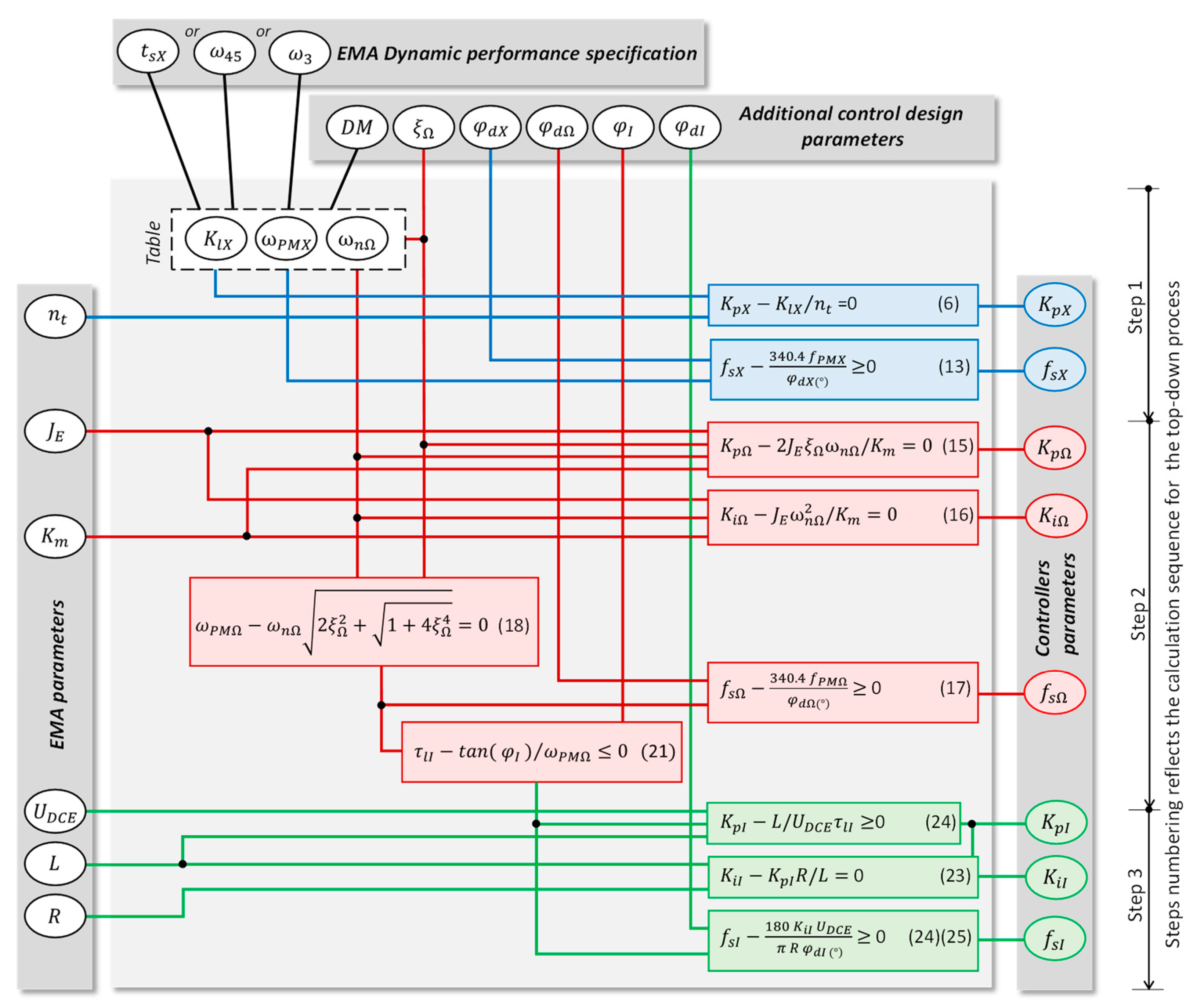
2.4. Synthesis of the Top-Down Process
3. Illustrative Example
3.1. Virtual Prototype
3.2. Virtual Validation
3.2.1. Current Loop

- The responses of the controlled virtual prototype globally agree well with the responses expected from the linear model and control strategy.
- As anticipated, stability is degraded by sampling and antialiasing but remains acceptable given the active management of this effect in the control design process and the 10° allocation.
- Figure 8a shows the influence of the current and speed measurement noises. Although the current demand is only twice the peak noise of the current measurement prior to the antialiasing filter, the current response remains globally stable.
- Under medium-magnitude excitations (Figure 8b), the relative importance of noises on response decreases.
- Under high-current and high-speed excitations (Figure 8c), the current response to the speed disturbance is affected by a significant ripple. As explained in [24], this effect comes from the tracking error of the rotor position measurement used by the dq0 transforms, although this is very fast, which generates an alias Id current proportionally to the Iq current demand.
3.2.2. Speed Loop
- Once again, the responses of the controlled virtual prototype globally agree well with the response expected from the linear model and control strategy.
- Even when the excitation magnitudes are only a few times the measurement noise before the antialiasing filter (Figure 9a), the expected dynamics and average response are still satisfactory.
- There is very little difference between the simulated and expected responses for medium-excitation magnitudes when they do not lead to saturation (Figure 9b).
- For high magnitudes of excitations (Figure 9c), the current and the voltage demand saturate for a long time during transients (Figure 9d). This makes the EMA operate temporarily in an open loop. At the end of the saturating phases, the normal control is recovered with high rapidity and stability. The absence of an excessive overshoot or limit cycle proves the correct setting and efficiency of the antiwindup function of the P-I controllers, implemented using the back calculation and tracking scheme [25].
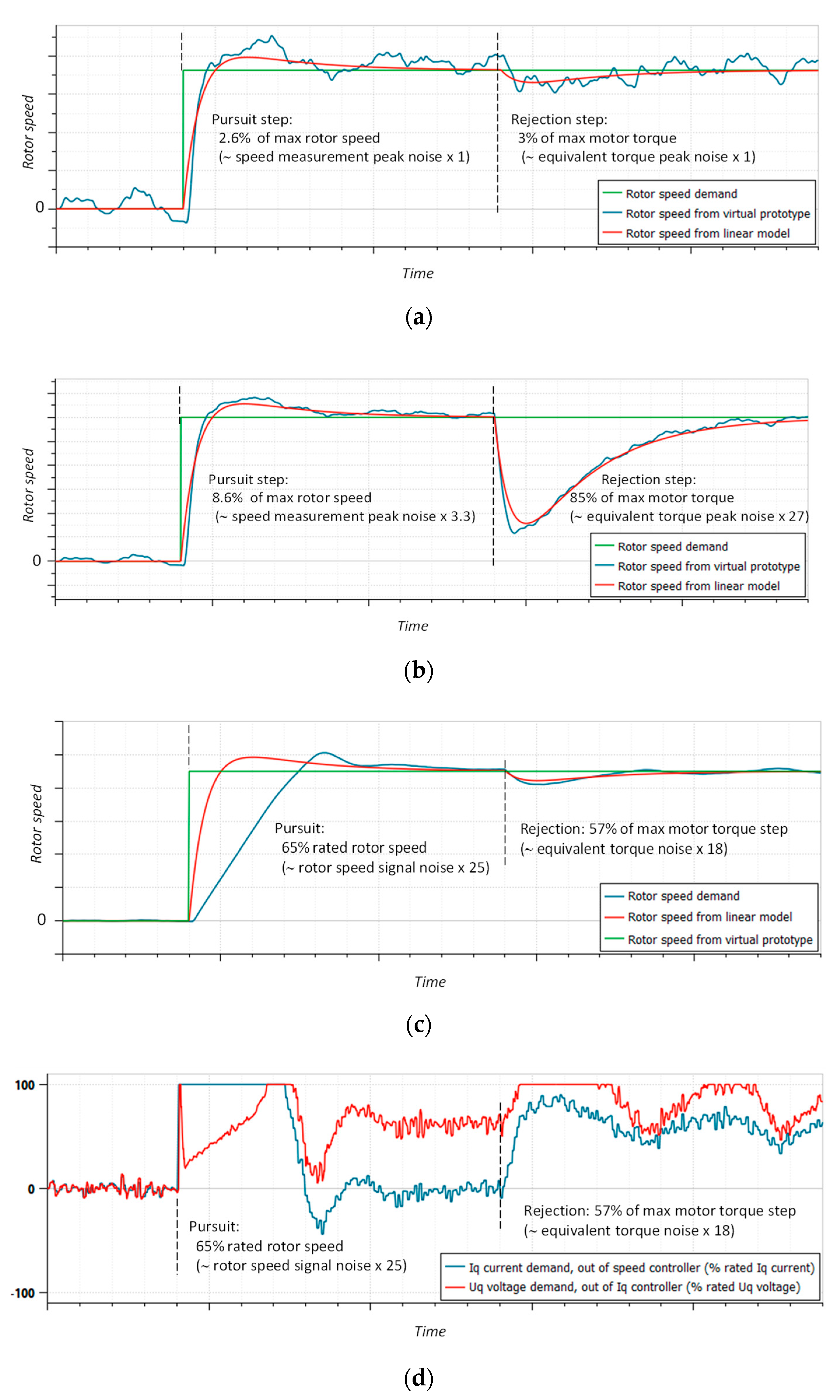
3.2.3. Position Loop
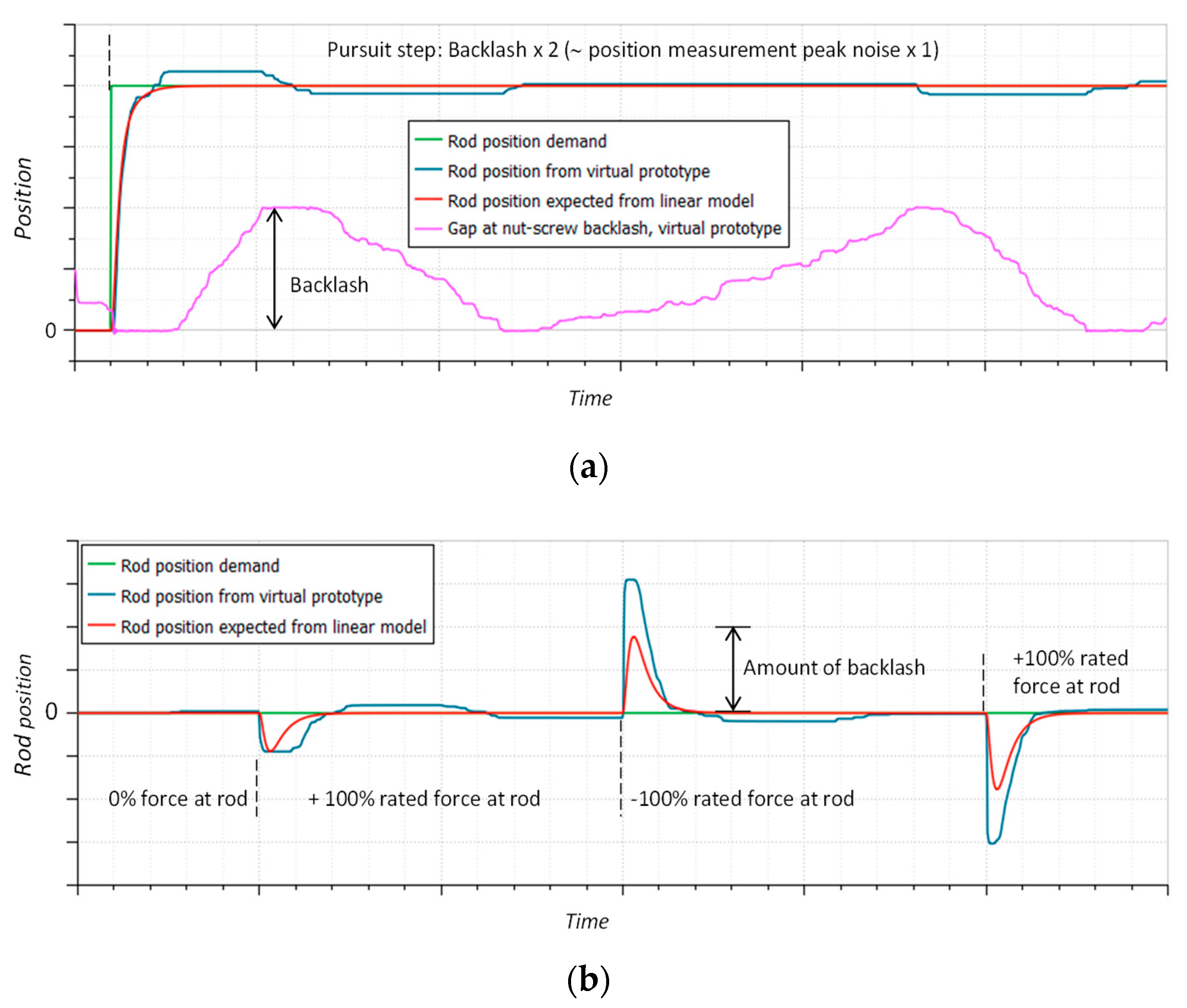
- At a very low magnitude of rod position demand (twice the EMA internal backlash), the response is still smooth and close to that expected from the linear model (Figure 10a). Logically, the combination of Coulomb friction, backlash and I control (speed and current loops) generates a limit cycle, albeit with a very low magnitude (<10% of the backlash).
- Even in the presence of backlash, the rod force step is rejected with the same dynamics as that of the linear model (Figure 10b). For 100% force, the transient position error does not exceed 155% of the backlash or 10% of the nut-screw lead. This excellent capability of external force disturbance confirms the soundness of the proposed approach, which is intended to maximise the position loop gain for a given .
- The position response under saturating excitations is illustrated by Figure 10c,d. The position demand is a pulse whose magnitude is just lower than the actuator stroke. A 100% rated rod force is applied to assess the position response under aiding and opposite loads. The influence of speed, current and voltage saturations clearly appears in Figure 10c, where the position response becomes unable to meet the expected dynamics. When the controllers leave the saturation domain, control is rapidly recovered in the linear domain with very few oscillations.

4. Discussion
- For very preliminary control design, it is advised to link the EMA internal parameters (e.g., inertia, nut-screw lead, motor windings resistance) to the EMA top-level specifications (maximal or rated speed and force at rod, along with reliability). This can, for example, be achieved using scaling laws, as proposed in [8]. Combining power and control preliminary designs would then enable more global and automated EMA design exploration and optimisation.
- Two examples have been provided in Section 2.1 to generate the performance charts. As these charts are precalculated once, other types of constraints can be applied and combined, without any need to change the process.
- Although the top-down process is driven by the overall control need, it is worth mentioning that it may output hardware and software specifications that cannot be met because of, for example, a lack of performance, maturity, availability or excessive cost. Therefore, precautions must be taken to check that each subspecification generated can be met in practice. If not, the concerned subspecification has to be replaced by a constraint, and the data flow of the design process has to be revised accordingly. This is enabled by the noncausal representation used in Figure 7.
5. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| Acronyms | |||
| BEMF | Back ElectroMotive Force | PMSM | Permanent Magnet Synchronous Machine |
| EMA | ElectroMechanical Actuator | PWM | Pulse Width Modulation |
| DAL | Design Assurance Level | RMS | Root Mean Square |
| FOC | Field-Oriented Control | RDC | Resolver To Digital Converter |
| I-P | Integral-Proportional | SE | Systems Engineering |
| LVDT | Linear Variometer Differential Transformer | TRL | Technology Readyness Level |
| P-I | Proportional-Integral | ||
| Notations | |||
| Viscous friction coefficient | Resistance | ||
| Design margin | t | Time | |
| Electromotive force | Torque | ||
| Frequency | Voltage | ||
| Force | Position | ||
| Current | θ | Angle | |
| Moment of inertia | Delay | ||
| Gain | Error | ||
| Nut-screw lead | Phase | ||
| Inductance | Dimensionless damping ratio | ||
| Modulation ratio | Time constant | ||
| Ratio | Angular frequency | ||
| N | Gear reduction ratio | Angular velocity | |
| Laplace variable | |||
| Subscript | |||
| Antialiasing | Proportional | ||
| Computation | Phase margin | ||
| Controller | Natural, undamped | ||
| Digital, direct (in-phase) | q | Quadrature | |
| Direct current supply | Settling | ||
| Electric | Sampling, or specified | ||
| Equivalent | Transmission | ||
| Integral | T | Torque | |
| Current | XF | Position–force | |
| Loop | Angular velocity | ||
| Load | 3 | At −3 dB magnitude | |
| Motor | 45 | At −45° phase | |
| Superscript | |||
| ′ | Modified or measured value | ||
| * | Setpoint | ||
| Dimensionless | |||
| ° | Angle expressed in degree | ||
Appendix A. Current Loop
- The modulation ratio is bounded to [−1; +1];
- Of course, the BEMF (disturbance) is correlated to the motor current (controlled variable) through the airgap torque and the motor shaft dynamics. However, this coupling can be neglected, except in very specific cases, for the current loop study. This is because this coupling appears at frequencies that are significantly lower than the current loop bandwidth.
| Constitutive Equations | ||
| Pulse width modulation | Variables: motor voltage, supply current, motor current, modulation ratio Parameters: equivalent line to line voltage | |
| Motor windings electrical circuit | Variables: motor shaft angular velocity, Laplace variable, motor BEMF Parameters: motor windings resistance, motor windings inductance | |
| P-I current controller | Parameters: current loop proportional gain, current loop integral gain Variables: motor current setpoint, measured current (equals the real current if the measurement is perfect) | |
| Current Open-Loop Transfer | ||
| Motor electric time constant Current controller time constant | ||
| Current Closed-Loop Transfer (P Control Only) | ||
| Apparent windings resistance Apparent electric time constant | ||
| Current Closed-Loop Transfer (P-I Control, ) | ||
| Static pursuit gain: | = 1 | |
| Tracking rejection gain: | ||
| Denominator time constant (pursuit): | ||
| Denominator time constant (rejection): | ||
| Controller setting for target pursuit dynamics: | ||
Appendix B. Speed Loop
- The dynamics of the current loop is neglected because in the very general case, it is much greater than the speed loop dynamics.
- At the speed loop level, the BEMF disturbance that applies to the current loop has no effect, either because the BEMF is compensated or because the integral action of the current controller removes its effect much faster than the speed loop dynamics.
- All mechanical effects are considered as their overall equivalent, expressed at the motor rotor level.
- The backlash and mechanical compliance of the actuator are not considered.
- Friction is assumed to be purely viscous, making it linearly dependent on relative speed only (see Section 2.2.1 for the discussion).
- As for the current loop, the digital implementation of the controller, the sensors and their conditioning, and thermal effects (in particular on through the magnet’s sensitivity to temperature) are not considered.
| Constitutive Equations | ||
| Perfect current control | Variables: motor actual current, current setpoint | |
| PI speed controller | Parameters: speed loop proportional gain, speed loop integral gain Variables: Laplace variable, rotor speed setpoint, measured rotor speed (equals real value if measurement perfect) | |
| Dynamics of the moving parts reflected at the rotor level | Parameters: equivalent inertia reflected at the rotor, equivalent viscous friction reflected at the rotor, motor torque constant Variables: EMA equivalent load reflected at the motor rotor | |
| Speed Open-Loop Transfer | ||
| Mechanical time constant | ||
| Speed Closed-Loop Transfer | ||
| Static pursuit gain: = 1 | Tracking rejection gain: | |
| Speed controller time constant: | is neglected) | |
| Denominator natural frequency: | Denominator damping factor: | |
| Controller gains for target second order: | ||
References
- Gonzalez, C.M. Engineering Refresher: The Basics and Benefits of Electromechanical Actuators. Available online: https://cdn.baseplatform.io/files/base/ebm/machinedesign/document/2019/04/machinedesign_15522_electromechanical.pdf (accessed on 8 February 2022).
- O’dwyer, A. Handbook of PI and PID Controller Tuning Rules, 3rd ed.; Imperial College Press: London, UK, 2009. [Google Scholar]
- Incose Systems Engineering Handbook: A Guide for System Life Cycle Processes and Activities, 4th ed.; Wiley-Blackwell: San Diego, CA, USA, 2015.
- Ali, Z.A.; Han, Z. Maneuvering Control of Hexrotor UAV Equipped With a Cable-Driven Gripper. IEEE Access 2021, 9, 65308–65318. [Google Scholar] [CrossRef]
- Fadel, M. Position Control for Laws for Electromechanical Actuator. In Proceedings of the 2005 International Conference on Electrical Machines and Systems, Nanjing, China, 27–29 September 2005; pp. 1708–1713. [Google Scholar] [CrossRef]
- Zhang, M.; Li, Q. A Compound Scheme Based on Improved ADRC and Nonlinear Compensation for Electromechanical Actuator. Actuators 2022, 11, 93. [Google Scholar] [CrossRef]
- Khanh, N.D.; Kuznetsov, V.E.; Lukichev, A.N.; Chung, P.T. Adaptive Control of Electromechanical Actuator Taking into Account Nonlinear Factors Based on Exo-model. In Proceedings of the 2022 Conference of Russian Young Researchers in Electrical and Electronic Engineering (ElConRus), St. Petersburg, Russia, 25–28 January 2022; pp. 803–807. [Google Scholar] [CrossRef]
- Budinger, M. Preliminary design and sizing of actuation systems. In Mechanical Engineering [physics.class-ph]; UPS Toulouse: Toulouse, France, 2014. [Google Scholar]
- Wu, S.; Bo, Y.; Jiao, Z.; Shang, X. Preliminary design and multi-objective optimization of electro-hydrostatic actuator. Proc. Inst. Mech. Eng. Part G J. Aerosp. Eng. 2010, 231, 1258–1268. [Google Scholar] [CrossRef]
- Vaculik, S.A. A Framework for Electromechanical Actuator Design. Ph.D. Thesis, The University of Texas at Austin, Austin, TX, USA, May 2008. Available online: https://repositories.lib.utexas.edu/bitstream/handle/2152/18161/vaculiks84501.pdf?sequence=2&isAllowed=y (accessed on 8 February 2022).
- Krishnan, R. Permanent Magnet Synchronous and Brushless DC Motor Drives; Taylor and Francis Group: Boca Raton, FL, USA, 2010. [Google Scholar] [CrossRef]
- Sang-Hoon, K. Electric Motor Control; Elsevier: Amsterdam, The Netherlands, 2017. [Google Scholar] [CrossRef]
- Dee, G.; Vanthuyne, T.; Alexandre, P. An electrical thrust vector control system with dynamic force feedback. In Proceedings of the 3rd International Conference on Recent Advances in Aerospace Actuation Systems and Components, Toulouse, France, 13–15 June 2007; pp. 75–79. [Google Scholar]
- Landau, I.D. Identification et Commande des Systèmes; Hermes: Paris, France, 1988. [Google Scholar]
- Krishnan, R. Electric Motor Drives: Modeling, Analysis, and Control; Prentice Hall: Upper Saddle River, NJ, USA, 2001. [Google Scholar]
- Crowder, R. Electric Drives and Electromechanical Systems Applications and Control, 2nd ed.; Elsevier: Oxford, UK, 2020. [Google Scholar]
- Maré, J.-C. Friction modelling and simulation at system level—Considerations to load and temperature effects. IMechE Part I J. Syst. Control. Eng. 2015, 229, 27–48. [Google Scholar] [CrossRef]
- Arriola, D. Model-based Design and Fault-tolerant Control of an Actively Redundant Electromechanical Flight Control Actuation System. Ph.D. Thesis, Technical University Hamburg, Hamburg, Germany, 2019. [Google Scholar]
- Dimino, I.; Gallorini, F.; Palmieri, M.; Pispola, G. Electromechanical Actuation for Morphing Winglets. Actuators 2019, 8, 42. [Google Scholar] [CrossRef] [Green Version]
- Wang, L. Force Equalization for Active/Active Redundant Actuation System Involving Servo-Hydraulic and Electro-Mechanical Technologies. Ph.D. Thesis, INSA, Toulouse, France, December 2012. Available online: http://www.theses.fr/2012ISAT0038/document (accessed on 8 February 2022).
- Karam, W. Générateurs de Forces Statiques et Dynamiques à Haute Puissance en Technologie Électromagnétique. Ph.D. Thesis, INSA, Toulouse, France, November 2007. Available online: http://eprint.insa-toulouse.fr/archive/00000182/http://www.theses.fr/2007ISAT0035/document (accessed on 8 February 2022).
- Fu, J.; Maré, J.-C.; Fu, Y. Modelling and simulation of flight control electromechanical actuators with special focus on model architecting, multidisciplinary effects and power flows. Chin. J. Aeronaut. 2017, 30, 47–65. [Google Scholar] [CrossRef] [Green Version]
- Fu, J.; Maré, J.-C.; Yu, L.; Fu, Y. Multi-level virtual prototyping of electromechanical actuation system for more electric aircraft. Chin. J. Aeronaut. 2018, 31, 889–913. [Google Scholar] [CrossRef]
- Maré, J.-C. Recent Experiences in Extending the Scope of EMA Simulation for Virtual V&V. In Proceedings of the SAE A-6B3 Panel, Spring Meeting, Virtual, 28 April 2021; Available online: https://www.sae.org/works/meetingminuteResources.do?comtID=TEAA6&resourceID=867983 (accessed on 8 February 2022).
- Åström, K.J. Advanced Control Methods: Survey and Assessment of Possibilities. In Advanced Control in Computer Integrated Manufacturing: A Control Engineer’s View; Morris, H.M., Kompass, E.J., Williams, T.J., Eds.; Purdue University: West Lafayette, IN, USA, 1987. [Google Scholar]
- Maré, J.-C. Practical Considerations in the Modelling and Simulation of Electromechanical Actuators. Actuators 2020, 9, 94. [Google Scholar] [CrossRef]
- Mohan, N.; Undeland, T.M.; Robbins, W.P. Power Electronics: Converters, Applications and Design, 1st ed.; Wiley: Hoboken, NJ, USA, 1989. [Google Scholar]


| Modelled | Not Modelled | |
|---|---|---|
| DC link * | Diode and capacitance | Parasitic serial and parallel resistances or capacitance |
| Braking resistance, chopper and its control | ||
| Three-phase inverter | 3 legs, 6 transistors | |
| Conduction and switching losses | ||
| Three-phase PMSM | Motor constant | Cyclic inductance Magnetic saturation Iron losses |
| Windings resistance and inductance | ||
| Temperature effects on motor constant and windings resistance | ||
| Mechanical transmission * | Mechanical transformation (nut-screw) | Moving body Side loads |
| Inertia of rotating and mass of translating assemblies | ||
| Rotational and translational friction with true sticking and effects of speed, load and temperature | ||
| Transmission compliance and backlash (in translational domain) | ||
| End stops | ||
| Kinematics to load | Three-bar mechanism (variable lever arm) | Friction and side loads at eye or hinge joints |
| Transmission compliance and backlash | ||
| Sensors * | Gain, range, dynamics, demodulation, antialiasing, sampling, quantisation, saturation and noise | Offset and thermal drift Hysteresis and nonlinearity |
| Controller | Discrete, with saturation and antiwindup, time for processing | |
| BEMF compensation if used, FOC using dq0 model | ||
| Limitation of speed, current and voltage demands (according to motor operating range) | ||
| PWM * | Symmetrical triangle carrier, sampling, saturation | |
| Timing and synchronisation with current loop | ||
| Thermal * | All energy losses made temperature-dependent and generating heat | Thermal transients |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Maré, J.-C. A Preliminary Top-Down Parametric Design of Electromechanical Actuator Position Control. Aerospace 2022, 9, 314. https://doi.org/10.3390/aerospace9060314
Maré J-C. A Preliminary Top-Down Parametric Design of Electromechanical Actuator Position Control. Aerospace. 2022; 9(6):314. https://doi.org/10.3390/aerospace9060314
Chicago/Turabian StyleMaré, Jean-Charles. 2022. "A Preliminary Top-Down Parametric Design of Electromechanical Actuator Position Control" Aerospace 9, no. 6: 314. https://doi.org/10.3390/aerospace9060314
APA StyleMaré, J.-C. (2022). A Preliminary Top-Down Parametric Design of Electromechanical Actuator Position Control. Aerospace, 9(6), 314. https://doi.org/10.3390/aerospace9060314






