Dynamic Response of a Composite Fan Blade Excited Instantaneously by Multiple MFC Actuators
Abstract
:1. Introduction
2. Investigated Composite Fan Blade
3. Vibration Test
4. Discussion
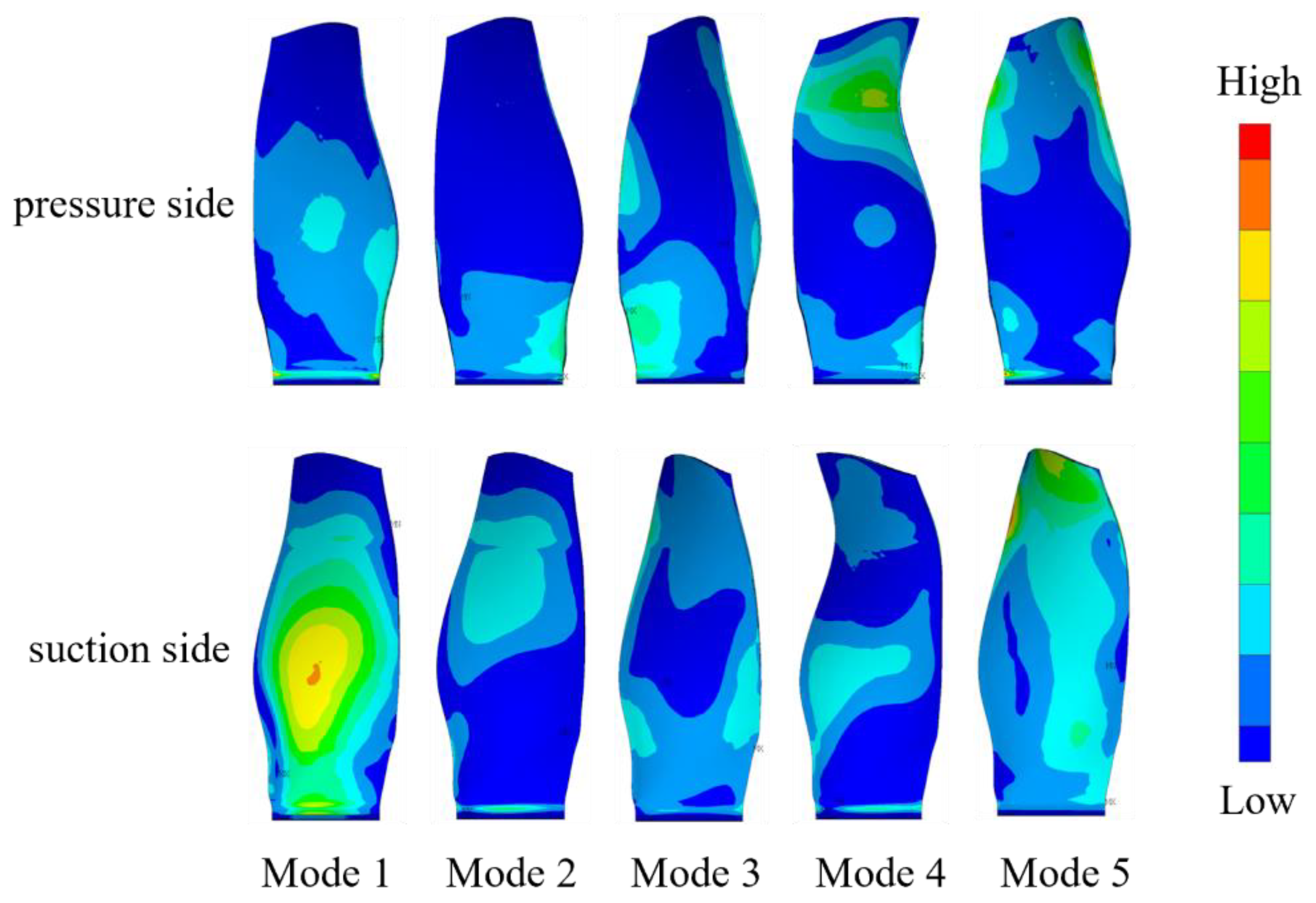
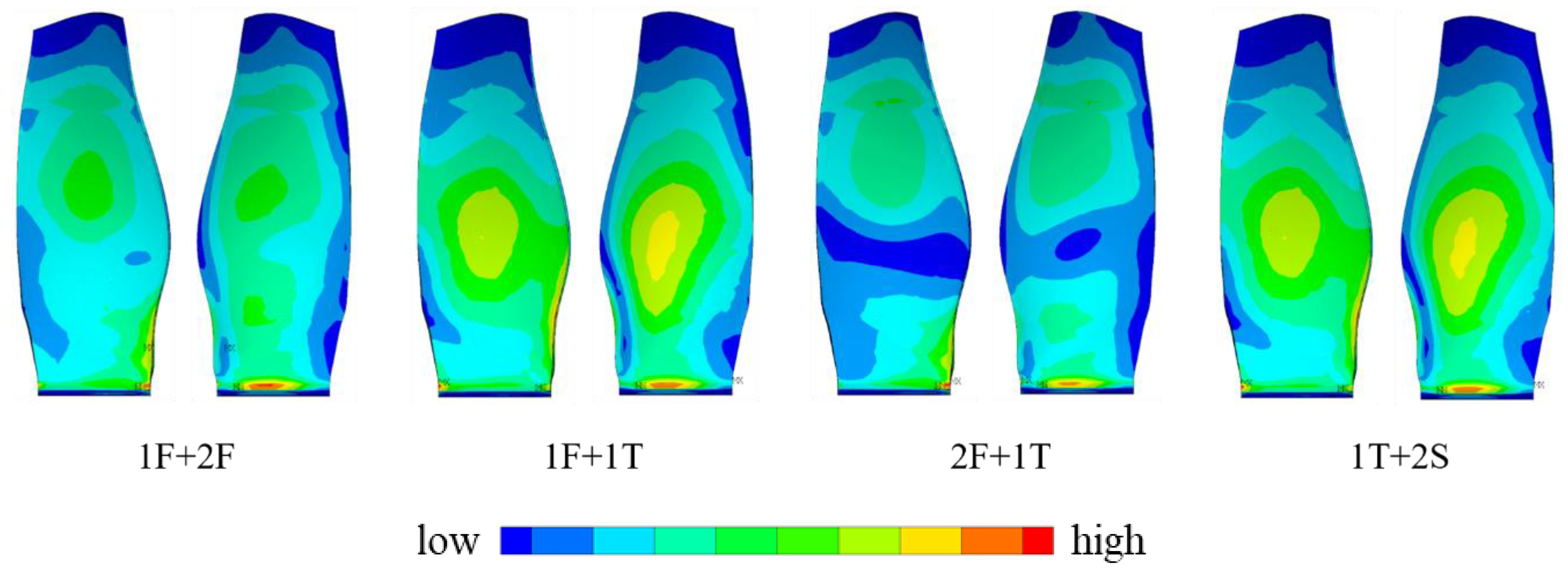
4.1. Natural Frequency and Mode Shape
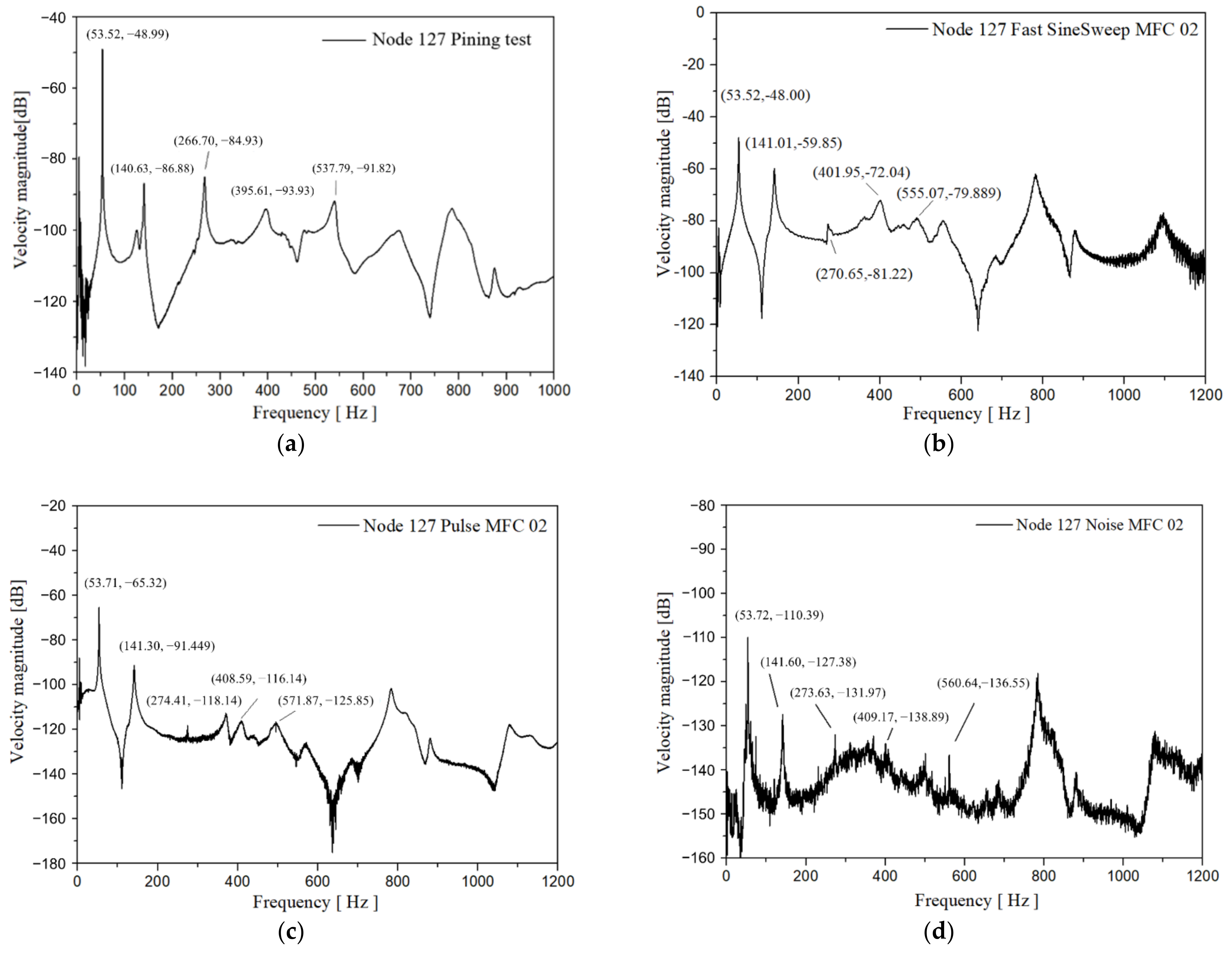
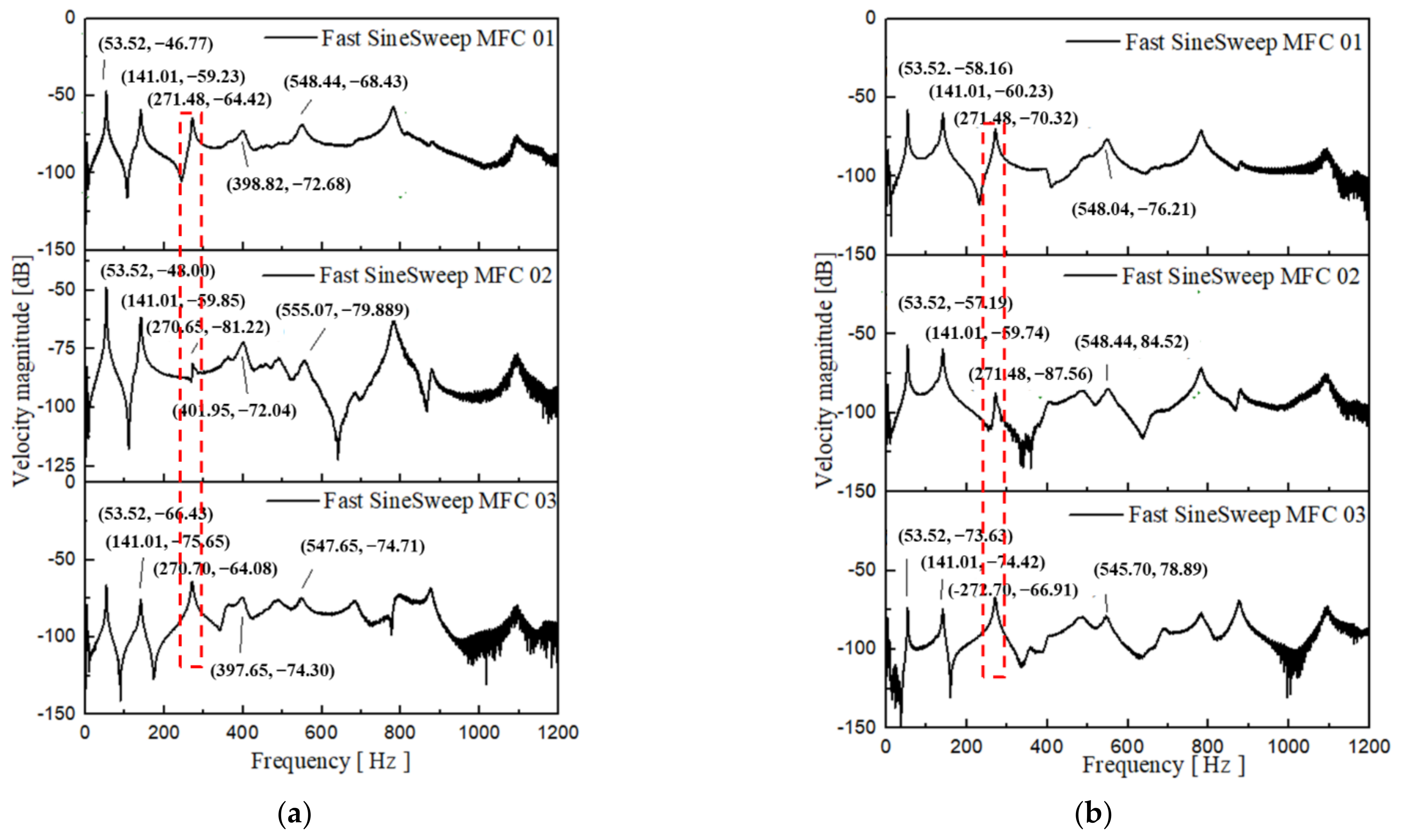
4.2. Blade Response Excited by Single MFC
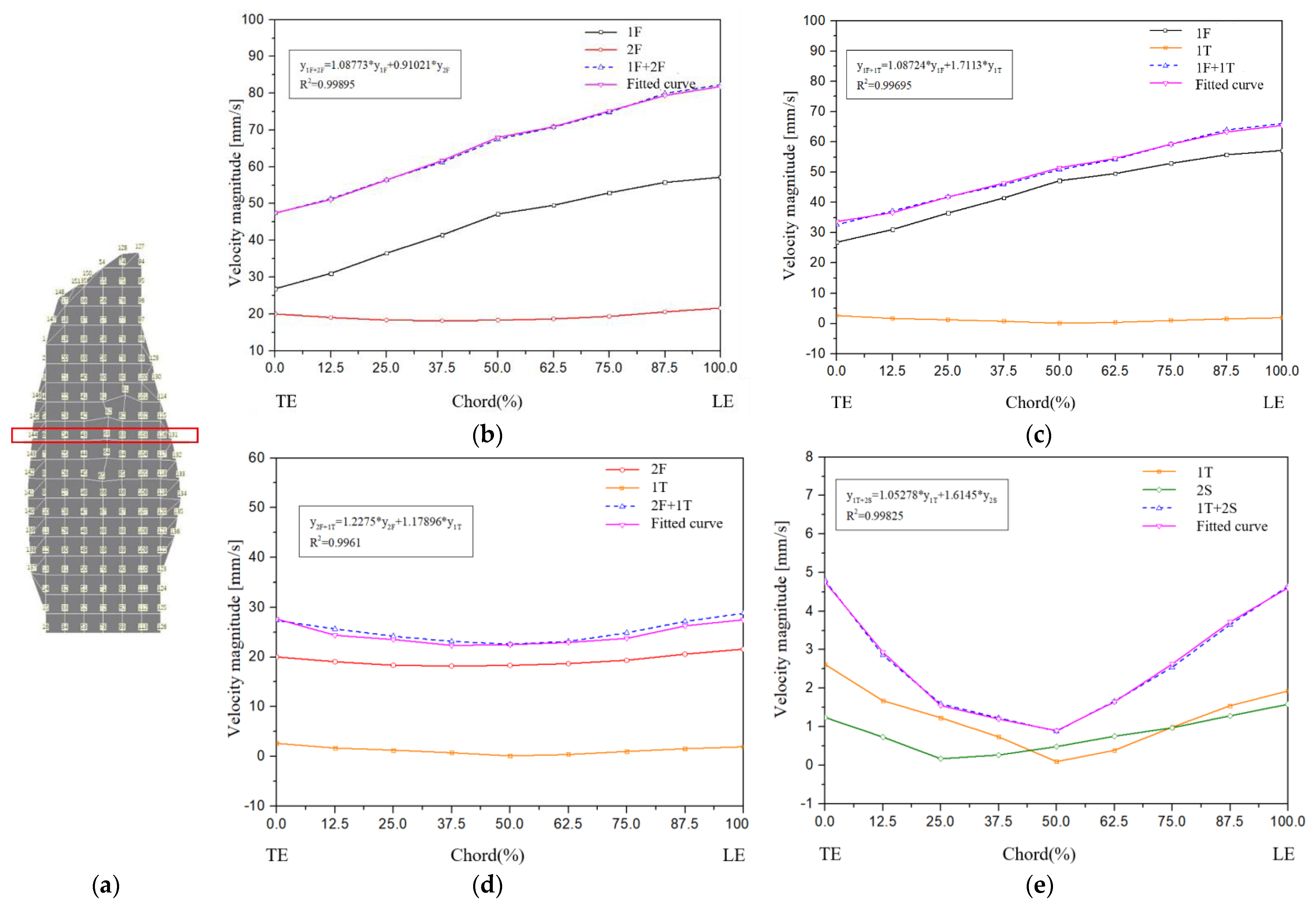
4.3. Blade Response Excited by Two MFCs with Same Frequency
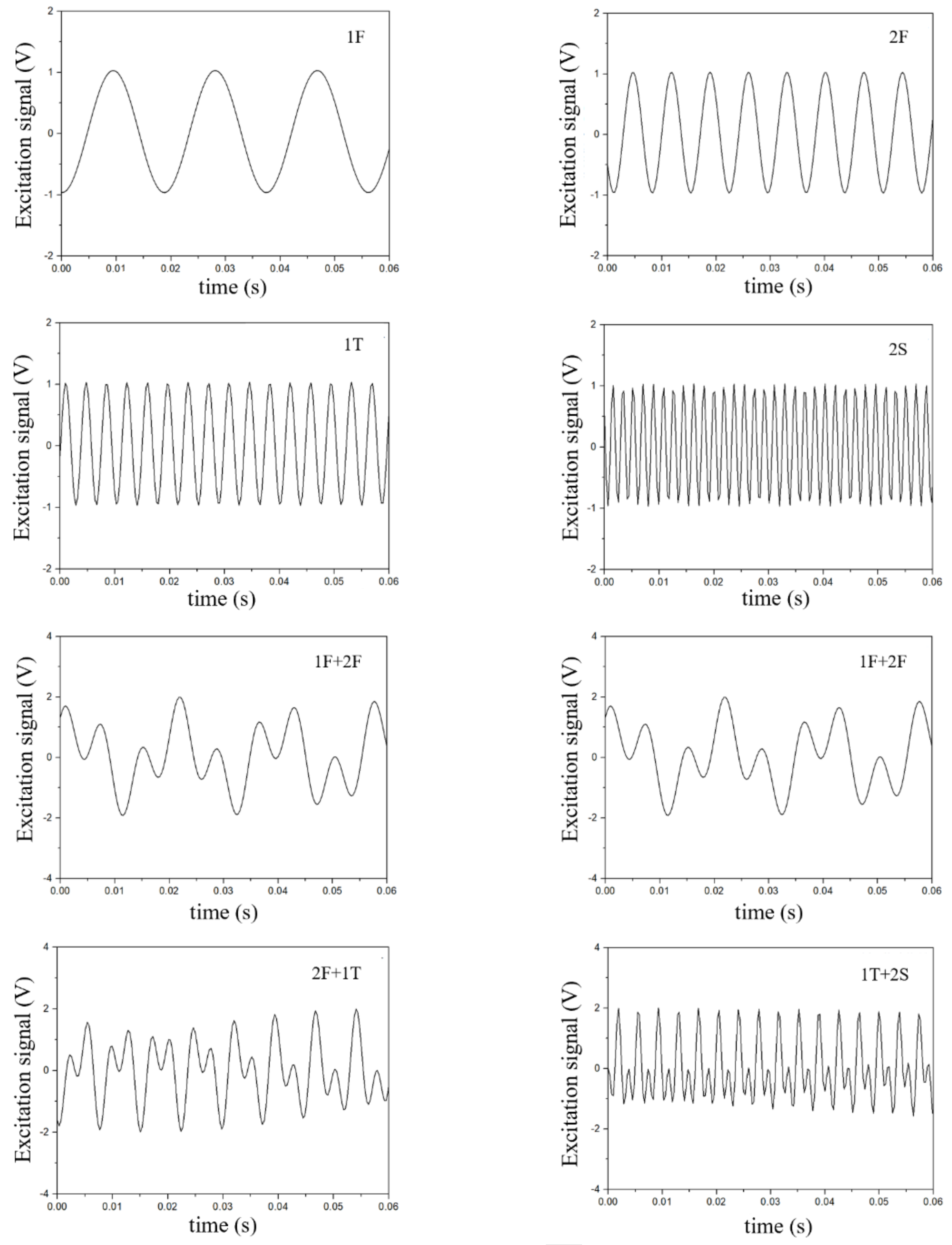
4.4. Blade Response Excited by Two MFCs with Different Frequencies
5. Conclusions
- (1)
- The first five natural frequencies and modal shapes of the composite fan blades obtained by FE simulation and modal testing using the MFC excitation method match very well, indicating that the MFC excitation method used in this paper and the high-fidelity finite element model have very good accuracy.
- (2)
- When using MFC to measure composite fan blade mode shape and natural frequency, the polarization direction and the paste area of the MFC must be carefully selected based on the FE results.
- (3)
- Blade response excited by two MFCs with same frequency is dependent on the position and direction of the MFC. The superimposed response amplitude of the bending mode is increased compared to that excited by a single MFC, and the superimposed response amplitude of the torsion mode is between the two response amplitudes excited by single MFC.
- (4)
- Blade response excited by two MFCs with different natural frequencies is dominated by the mode with a larger response excited by single MFC.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Amoo, L.M. On the design and structural analysis of jet engine fan blade structures. Prog. Aerosp. Sci. 2013, 60, 1–11. [Google Scholar] [CrossRef]
- Chinta, V.S.; Reddy, P.R.; Prasad, K.E. The effect of stacking sequence on the tensile properties of jute fibre reinforced hybrid composite material for axial flow fan blades: An experimental and finite element investigation. Mater. Today Proc. 2022, 59, 747–755. [Google Scholar] [CrossRef]
- Jin, H.; Liu, H.; Ma, L.; Gu, H.; Wang, J. Structural Response Analysis of Composite Fiber Blade of Small Wind Power Generator Turbine Blades. In Proceedings of the 2022 IEEE International Conference on Electrical Engineering, Big Data and Algorithms (EEBDA), Changchun, China, 25–27 February 2022; pp. 233–238. [Google Scholar]
- Morozov, A.V.; Kravchenko, I.F.; Torba, Y.I.; Lvov, G.I. Dynamics Numerical Prediction for Composite Wide-Chord Fan Blade. In Proceedings of the 2021 IEEE 2nd KhPI Week on Advanced Technology (KhPIWeek), Kharkiv, Ukraine, 13–17 September 2021; pp. 686–690. [Google Scholar]
- Sun, Y.; Zhang, Y.; Zhou, Y.; Zhang, H.; Zeng, H.; Yang, K. Evaluating Impact Damage of Flat Composite Plate for Surrogate Bird-Strike Testing of Aeroengine Fan Blade. J. Compos. Sci. 2021, 5, 171. [Google Scholar] [CrossRef]
- Jadhav, P. Design Methodologies for Composite Structures in Aircraft Engines. In Advanced Composites in Aerospace Engineering Applications; Springer: Cham, Switzerland, 2022; pp. 93–108. [Google Scholar]
- Federal Aviation Administration, FAR-33 Airworthiness Standards: Aircraft Engines; Federal Aviation: Washington, DC, USA, 2016.
- Wang, Z.; Chen, Y.; Ou, Y.; Wang, A. Vibration characteristics of titanium wide-chord fan blade. J. Aerosp. Power 2018, 33, 2593–2601. (In Chinese) [Google Scholar] [CrossRef]
- Kou, H. Research on Multi-Point Vibration Characteristics and Fatigue of Civil Aviation Engine High-Pressure Compressor Blade. Ph.D. Thesis, Tian Jin University, Tianjin, China, 2017. (In Chinese). [Google Scholar]
- Teter, A.; Gawryluk, J. Experimental modal analysis of a rotor with active composite blades. Compos. Struct. 2016, 153, 451–467. [Google Scholar] [CrossRef]
- Yang, S.; Allen, M.S. Output-only Modal Analysis using Continuous-Scan Laser Doppler Vibrometry and application to a 20 kW wind turbine. Mech. Syst. Signal Process. 2012, 31, 228–245. [Google Scholar] [CrossRef] [Green Version]
- Heylen, W. Modal Analysis Theory and Testing, 2nd ed.; Departement Werktuigkunde Press: Leuven, Belgium, 1998. [Google Scholar]
- Williams, R.B.; Park, G.; Inman, D.J.; Wilkie, W.K. An overview of composite actuators with piezoceramic fibers. Proc. IMAC XX 2002, 47, 421–427. [Google Scholar]
- Bent, A.A. Active Fiber Composites for Structural Actuation; Massachusetts Institute of Technology: Cambridge, MA, USA, 1997. [Google Scholar]
- Wilkie, W.K.; Bryant, R.G.; High, J.W.; Fox, R.L.; Hellbaum, R.F.; Jalink, A., Jr.; Little, B.D.; Mirick, P.H. Low-Cost Piezocomposite Actuator for Structural Control Applications. In Smart Structures and Materials 2000: Industrial and Commercial Applications of Smart Structures Technologies; International Society for Optics and Photonics: Bellingham, WA, USA, 2000; Volume 3991, pp. 323–335. [Google Scholar]
- Jemai, A.; Najar, F.; Chafra, M.; Ounaies, Z. Mathematical modeling of an active-fiber composite energy harvester with interdigitated electrodes. Shock. Vib. 2014, 2014, 971597. [Google Scholar] [CrossRef]
- Dong, Z.; Faria, C.; Hromčík, M.; Pluymers, B.; Šebek, M.; Desmet, W. Equivalent force modeling of macro fiber composite actuators integrated into non-homogeneous composite plates for dynamic applications. Smart Mater. Struct. 2017, 26, 095040. [Google Scholar] [CrossRef]
- Roosevel, A.F.D. Finite element modeling of piezoelectric structures. AIAA J. 1993, 31, 930–937. [Google Scholar]
- Guennam, A.E.; Luccioni, B.M. Piezoelectric shell FE for the static and dynamic analysis of piezoelectric fibre composite laminates. Smart Mater. Struct. 2009, 18, 095044. [Google Scholar] [CrossRef]
- Zhang, S.Q.; Li, Y.X.; Schmidt, R. Modeling and simulation of macro-fiber composite layered smart structures. Compos. Struct. 2015, 126, 89–100. [Google Scholar] [CrossRef]
- Prasath, S.S.; Arockiarajan, A. Influence of bonding layer on effective electromechanical properties of macro-fiber composites (MFCs). Smart Mater. Struct. 2014, 23, 095046. [Google Scholar] [CrossRef]
- Fagerholt, E.; Dørum, C.; Børvik, T.; Laukli, H.I.; Hopperstad, O.S. Experimental and numerical investigation of fracture in a cast aluminium alloy. Int. J. Solids Struct. 2010, 47, 3352–3365. [Google Scholar] [CrossRef] [Green Version]
- Smart Material MFC Overview. 2017. Available online: http://www.smart-material.com/MFC-product-main.html (accessed on 8 March 2021).
- Belz, J.; May, M.; Siemann, J.; Seume, J.R.; Voigt, C.; Böhmer, H.; Grüber, B. Excited Blade Vibration for Aeroelastic Investigations of a Rotating Blisk Using Piezo-Electric Macro Fiber Composites. In Proceedings of the ASME Turbo Expo 2013: Turbine Technical Conference and Exposition, San Antonio, TX, USA, 3–7 June 2013; American Society of Mechanical Engineers: New York, NY, USA, 2013. V07BT33A011. [Google Scholar]
- Deraemaeker, A.; Nasser, H. Numerical evaluation of the equivalent properties of Macro Fiber Composite (MFC) transducers using periodic homogenization. Int. J. Solids Struct. 2010, 47, 3272–3285. [Google Scholar] [CrossRef] [Green Version]
- Steiger, K.; Mokrý, P. Finite element analysis of the macro fiber composite actuator: Macroscopic elastic and piezoelectric properties and active control thereof by means of negative capacitance shunt circuit. Smart Mater. Struct. 2015, 24, 025026. [Google Scholar] [CrossRef]
- Di Rito, G.; Chiarelli, M.R.; Luciano, B. Dynamic Modelling and Experimental Characterization of a Self-Powered Structural Health-Monitoring System with MFC Piezoelectric Patches. Sensors 2020, 20, 950. [Google Scholar] [CrossRef] [Green Version]
- Baghaee, M.; Farrokhabadi, A.; Jafari-Talookolaei, R.A. A solution method based on Lagrange multipliers and Legendre polynomial series for free vibration analysis of laminated plates sandwiched by two MFC layers. J. Sound Vib. 2019, 447, 42–60. [Google Scholar] [CrossRef]
- Wang, X.; Zhou, W.; Zhang, Z.; Jiang, J.; Wu, Z. Theoretical and experimental investigations on modified LQ terminal control scheme of piezo-actuated compliant structures in finite time. J. Sound Vib. 2021, 491, 115762. [Google Scholar] [CrossRef]
- Baghaee, M.; Farrokhabadi, A.; Jafari-Talookolaei, R.A. Modeling, analysis, and control of MFC sandwiched laminate panel flutter with general layups and arbitrary boundary conditions. Compos. Struct. 2019, 223, 110940. [Google Scholar] [CrossRef]
- Chu, W.L.; Lin, C.J.; Li, M.J. Active multimode vibration control of a smart structure using macro fiber composite actuators based on ANFIS. J. Low Freq. Noise Vib. Act. Control 2020, 39, 1187–1203. [Google Scholar] [CrossRef]
- Zhang, J.; Tu, J.; Li, Z.; Gao, K.; Xie, H. Modeling on actuation behavior of Macro-Fiber Composite laminated structures based on sinusoidal shear deformation theory. Appl. Sci. 2019, 9, 2893. [Google Scholar] [CrossRef] [Green Version]
- Pastor, M.; Binda, M.; Harčarik, T. Modal assurance criterion. Procedia Eng. 2012, 48, 543–548. [Google Scholar] [CrossRef]
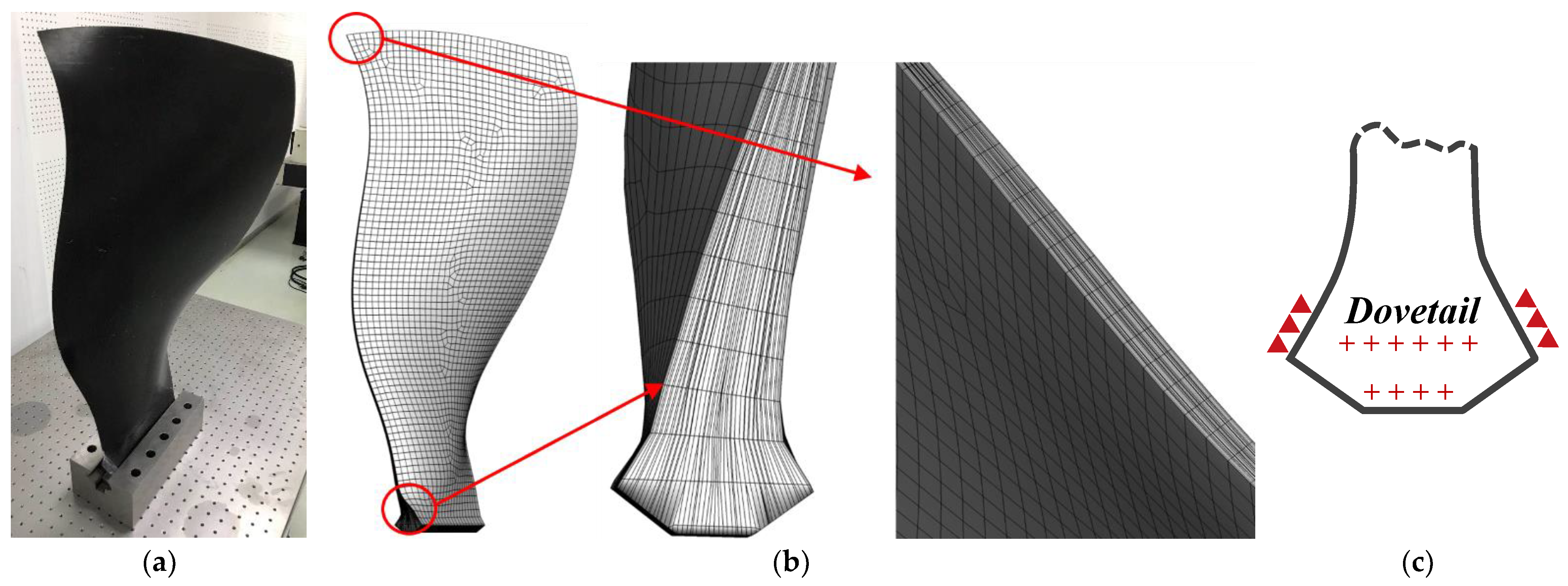
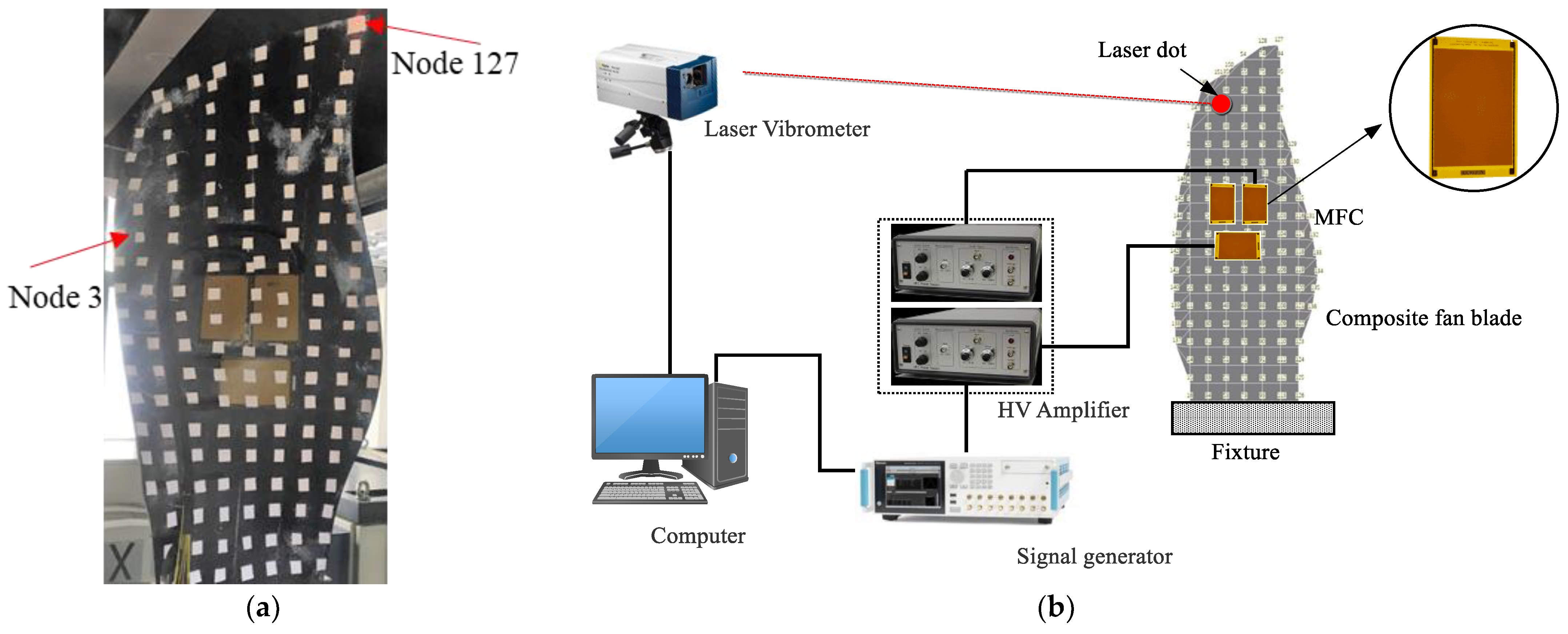


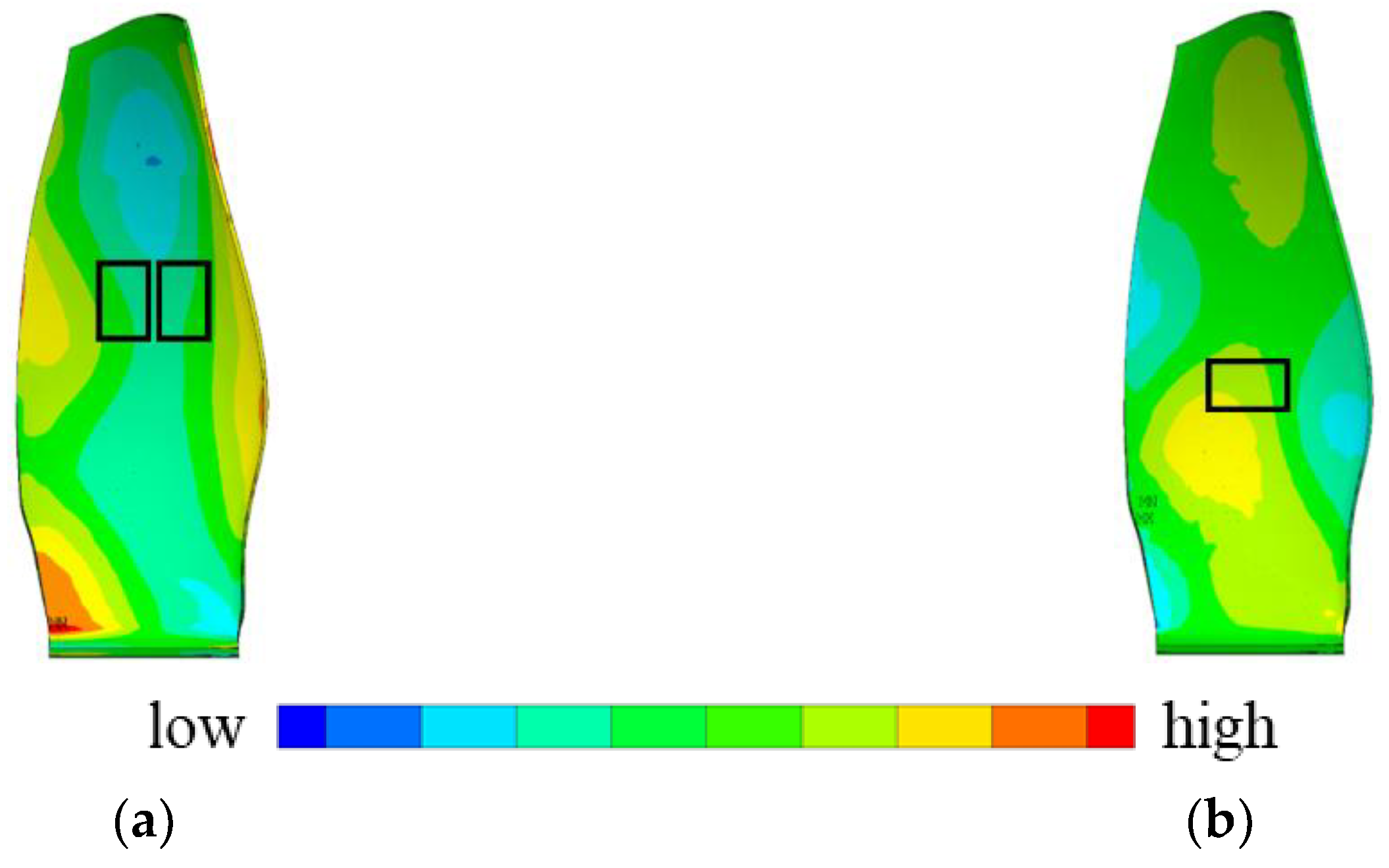

| Properties | Value |
| 130.4 GPa | |
| 8.41 GPa | |
| 3.12 GPa | |
| 3.11 GPa | |
| 0.322 | |
| 0.35 |
| Mode | Freq. (FE)/Hz | Pining Test | Excited by MFC 02 | ||
|---|---|---|---|---|---|
| Freq./Hz | Difference/% | Freq./Hz | Difference/% | ||
| 1 | 52.84 | 53.52 | 1.28 | 53.52 | 1.28 |
| 2 | 135.58 | 140.63 | 3.72 | 140.63 | 3.72 |
| 3 | 268.86 | 266.70 | −0.80 | 268.75 | −0.04 |
| 4 | 390.67 | 395.61 | 1.26 | 397.66 | 1.79 |
| 5 | 532.34 | 537.79 | 1.02 | 541.41 | 1.70 |
| Multiple MFC Excitation * | Single MFC Excitation ** | ||||||||
|---|---|---|---|---|---|---|---|---|---|
| Mode 1 | 1.66 | 1.66 | 0.81 | 7.75 | 0.72 | 6.97 | 1.00 | 9.62 | 9.62 |
| Mode 2 | 1.81 | 1.81 | 0.80 | 5.30 | 0.76 | 5.00 | 1.00 | 6.62 | 6.62 |
| Mode 3 | 0.12 | 6.40 | 0.04 | 0.27 | 5.99 | 0.83 | 54.95 | 7.64 | 0.14 |
| Mode 4 | 1.83 | 1.70 | 0.21 | 0.25 | 0.44 | 0.58 | 0.93 | 1.20 | 1.30 |
| Mode 5 | 0.31 | 1.17 | 0.75 | 1.55 | 0.84 | 0.46 | 3.74 | 2.06 | 0.55 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, Y.; Jin, L.; Tang, X.; Huang, D.; Zhang, J. Dynamic Response of a Composite Fan Blade Excited Instantaneously by Multiple MFC Actuators. Aerospace 2022, 9, 301. https://doi.org/10.3390/aerospace9060301
Chen Y, Jin L, Tang X, Huang D, Zhang J. Dynamic Response of a Composite Fan Blade Excited Instantaneously by Multiple MFC Actuators. Aerospace. 2022; 9(6):301. https://doi.org/10.3390/aerospace9060301
Chicago/Turabian StyleChen, Yong, Lu Jin, Xu Tang, Dandan Huang, and Jiguo Zhang. 2022. "Dynamic Response of a Composite Fan Blade Excited Instantaneously by Multiple MFC Actuators" Aerospace 9, no. 6: 301. https://doi.org/10.3390/aerospace9060301
APA StyleChen, Y., Jin, L., Tang, X., Huang, D., & Zhang, J. (2022). Dynamic Response of a Composite Fan Blade Excited Instantaneously by Multiple MFC Actuators. Aerospace, 9(6), 301. https://doi.org/10.3390/aerospace9060301






