Design and Fabrication of a Phase Change Material Heat Storage Device for the Thermal Control of Electronics Components of Space Applications
Abstract
:1. Introduction
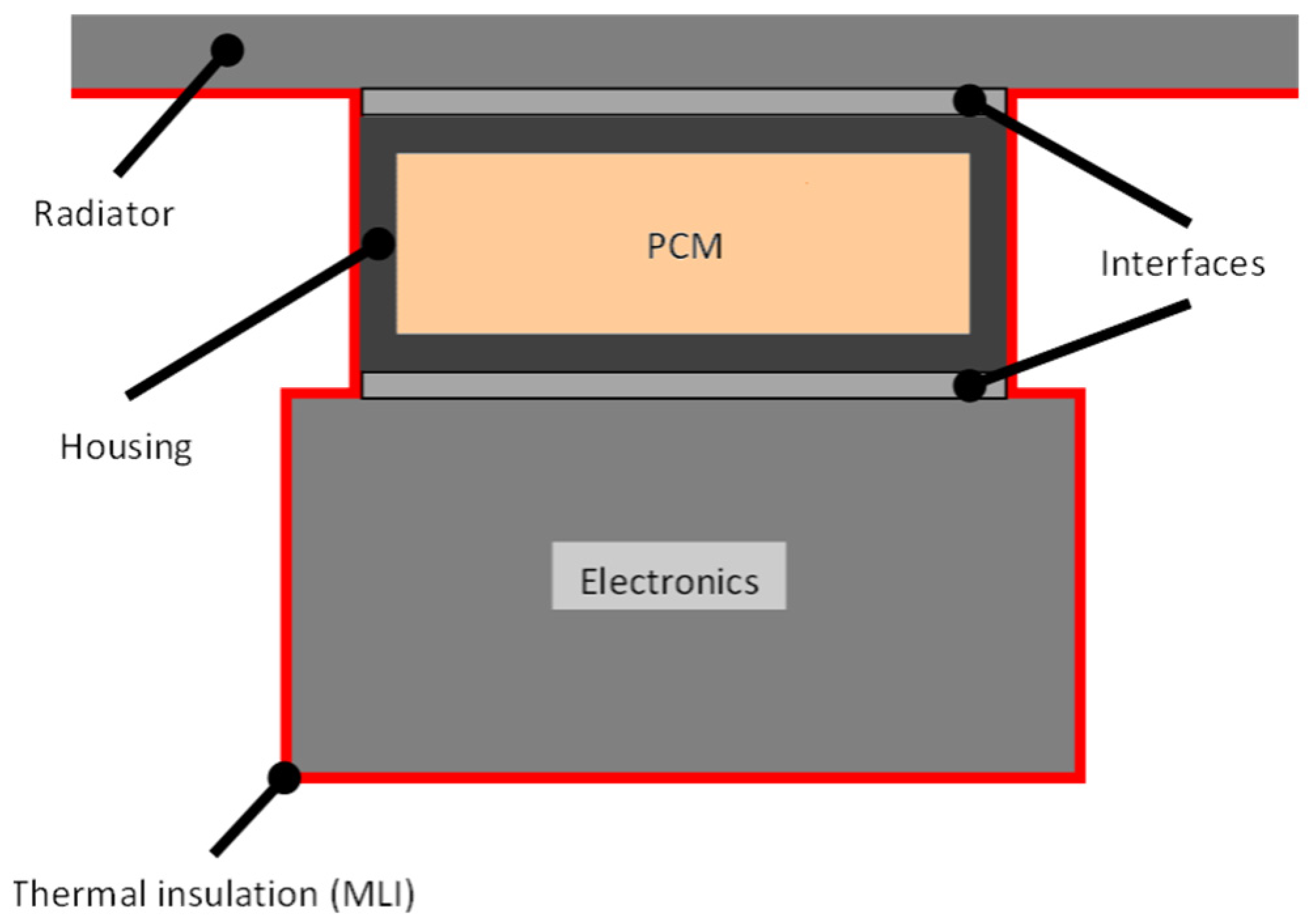
2. Phase Change Material Heat Storage Device (PCM-HSD)
2.1. Description
- The device must be capable of absorbing 30 W for 45 min;
- Operational temperature range must be (−20/+40 °C);
- The device’s mass shall be less than 0.50 kg;
- The device’s first resonance frequency must be higher than 140 Hz;
- The device shall sustain a mechanical environment characterized by dynamic loads.
2.2. Governing Equations and Numerical Methods
3. Design of the Phase Change Material Heat Storage Device (PCM-HSD)
3.1. Conceptual Design
3.1.1. PCM Selection
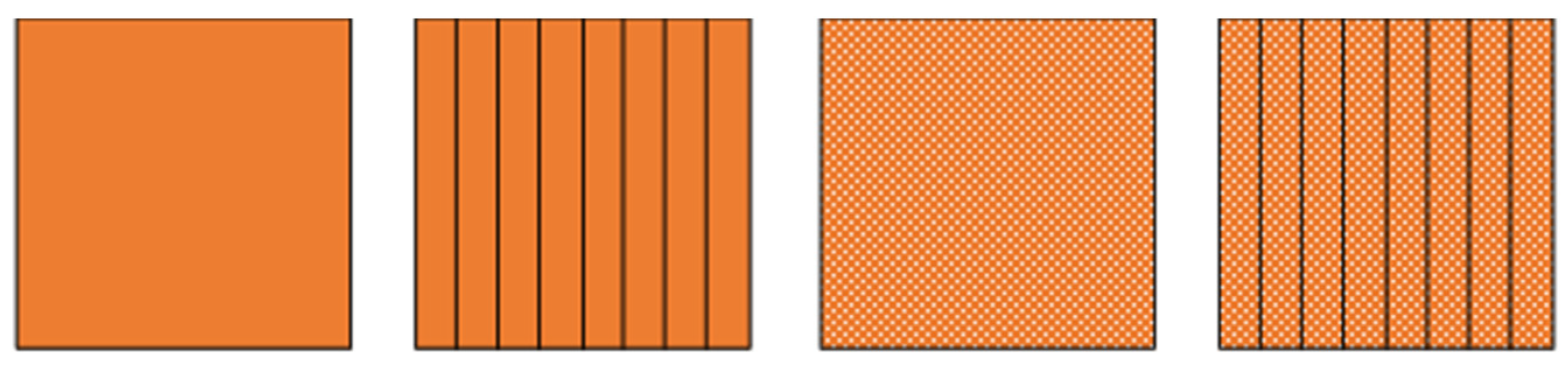
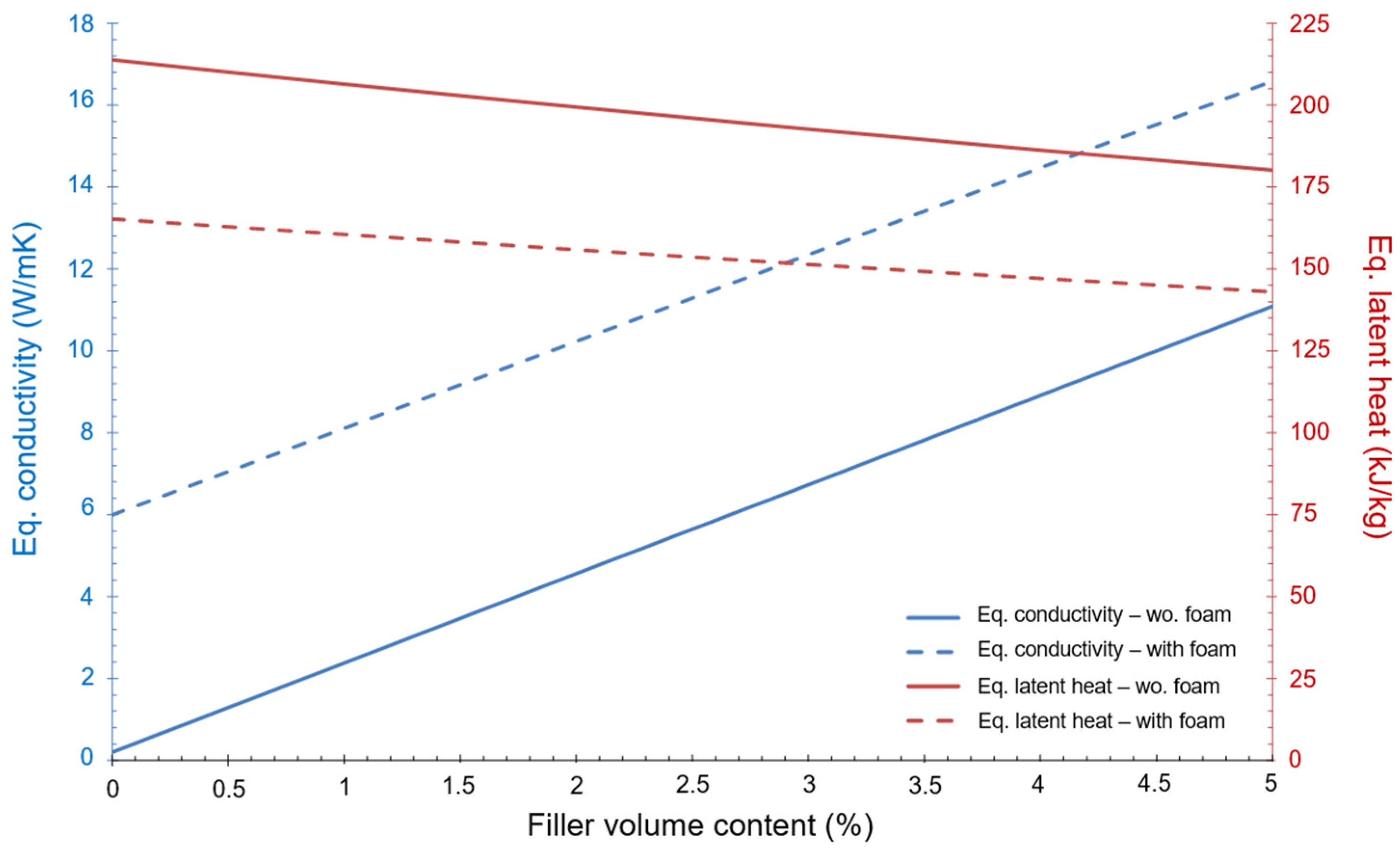
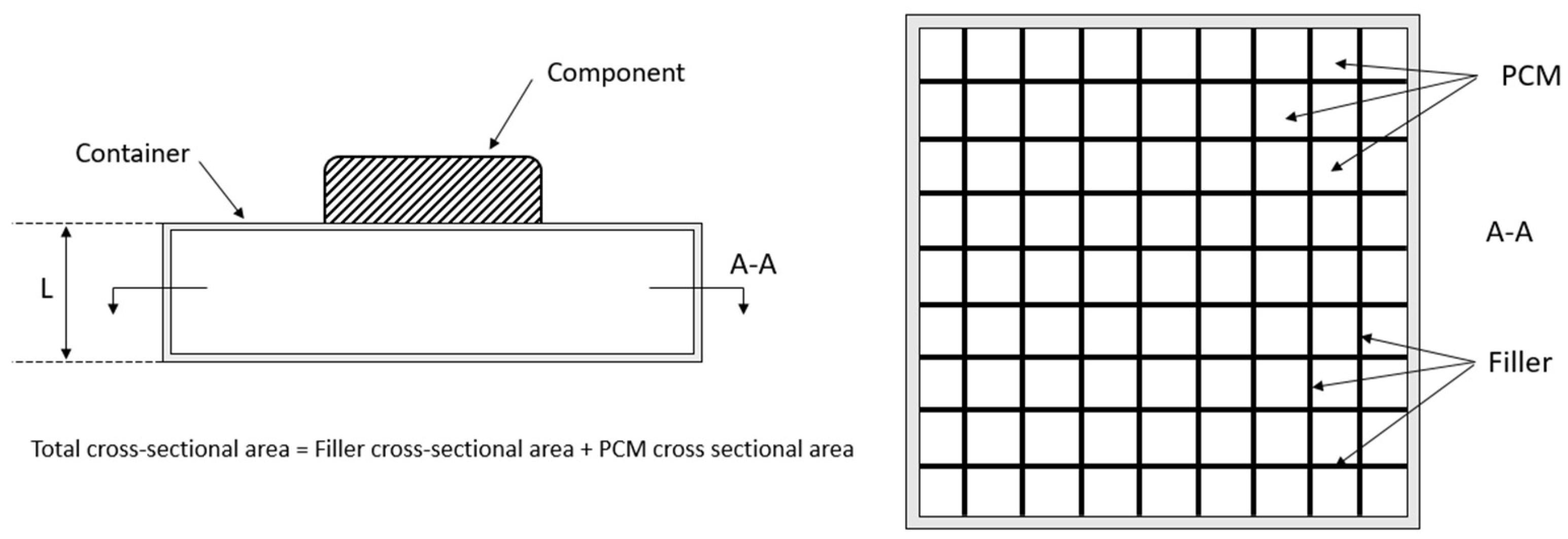
3.1.2. Enhancement of the Thermal Conductivity
3.1.3. Initial Conceptual Design
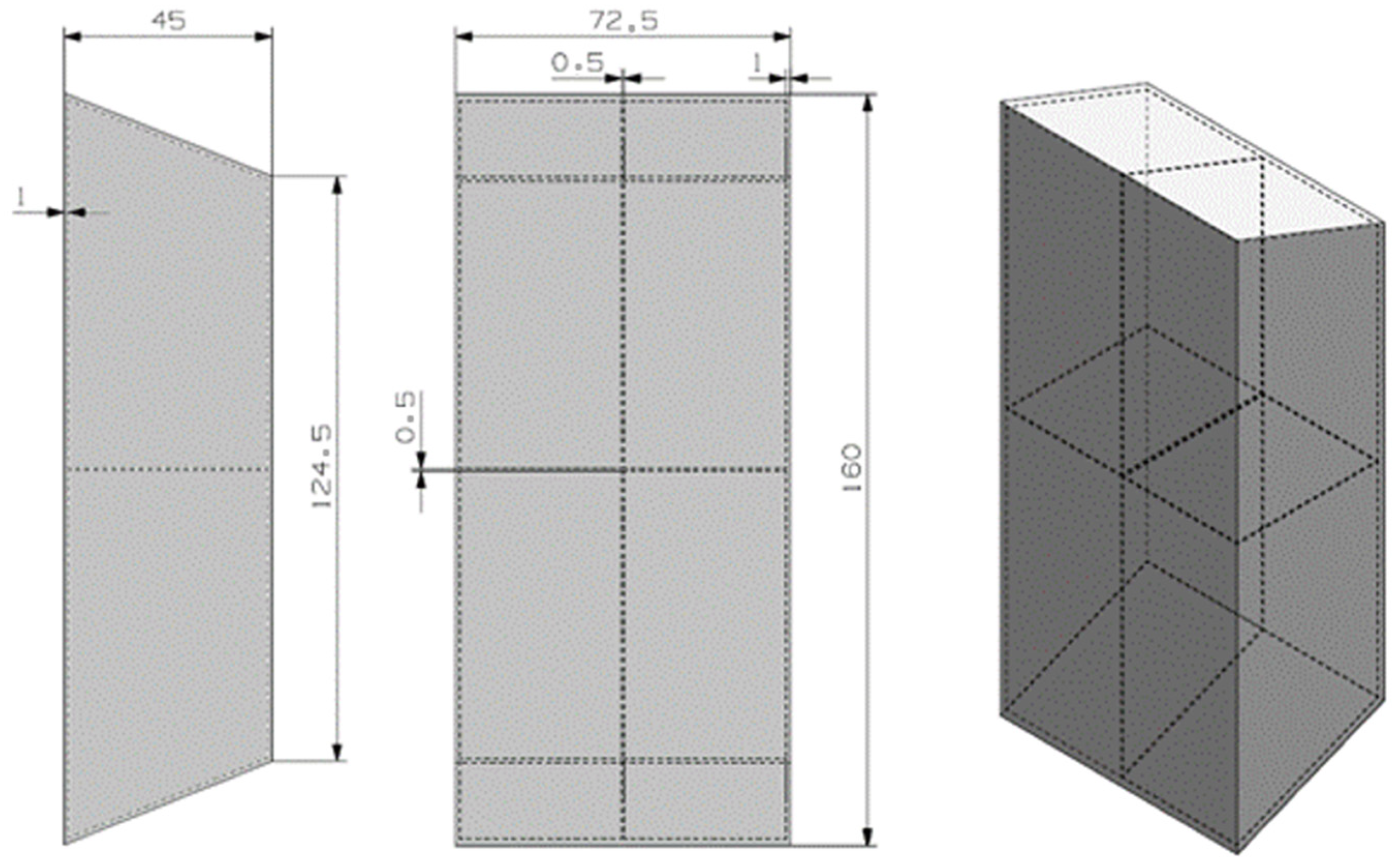
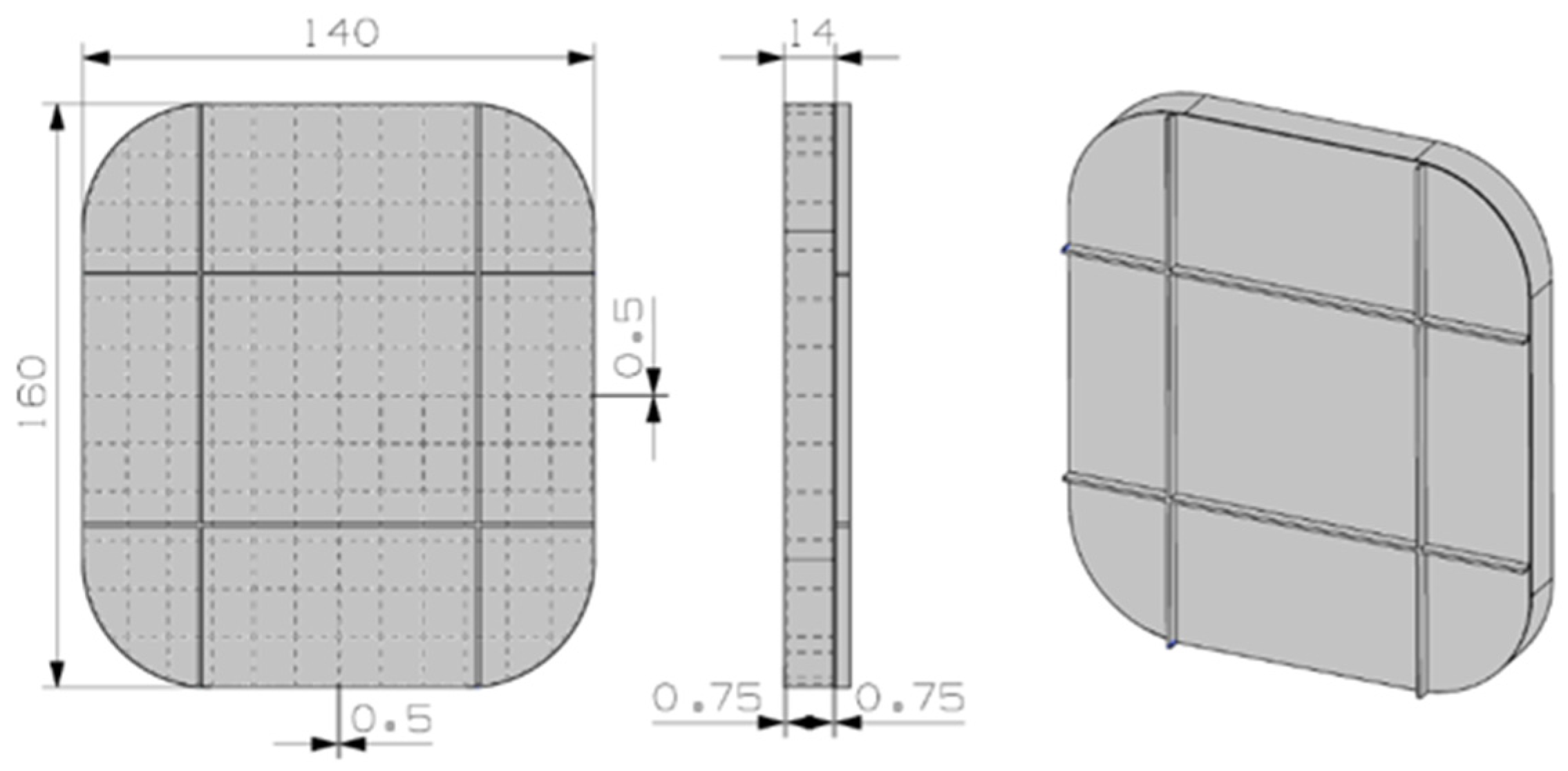
3.2. Preliminary Design of Two Possible Phase Change Material Heat Storage Devices
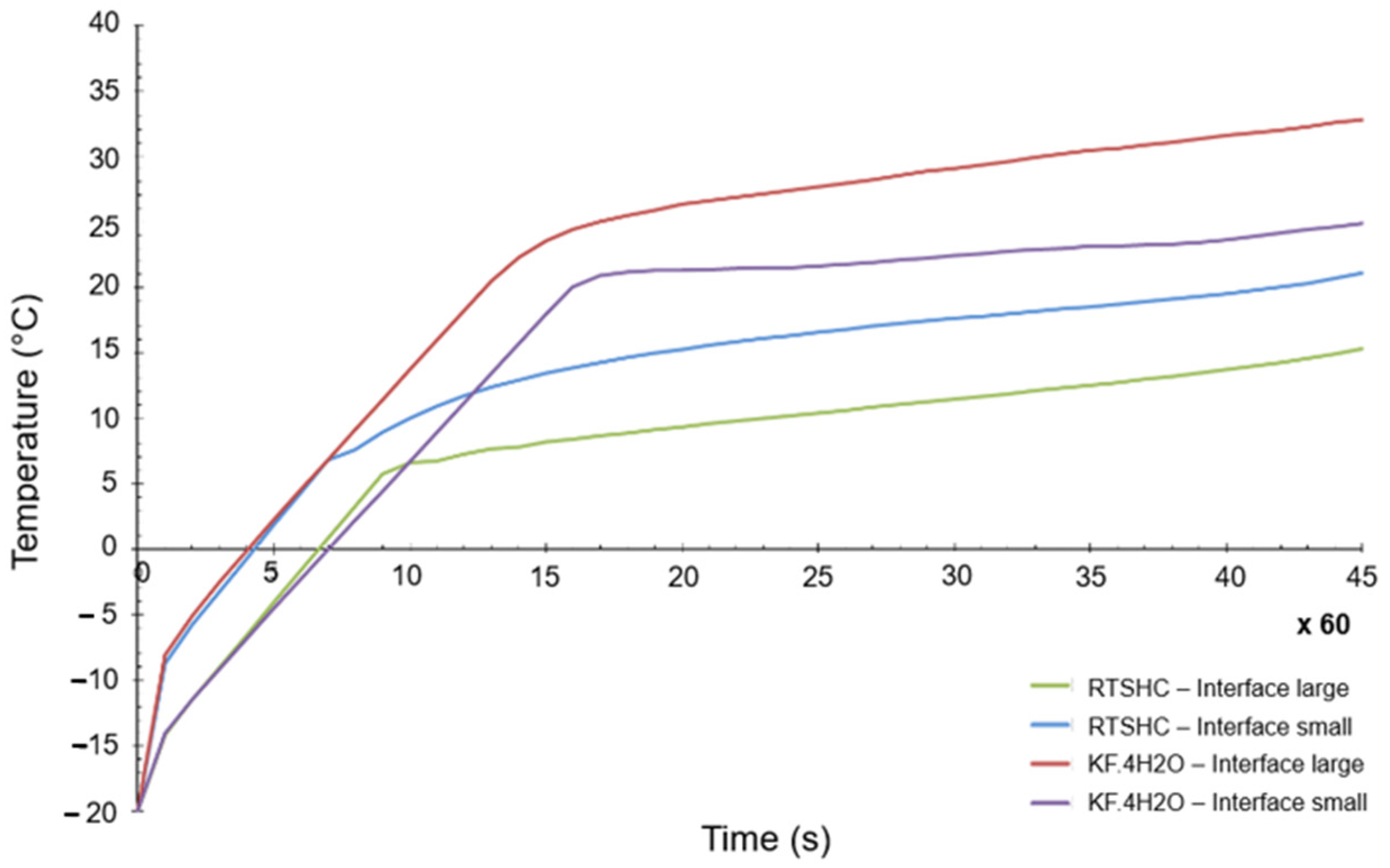
3.2.1. RT5HC Material
3.2.2. KF·4H2O Material
3.3. Detailed Design: Thermal and Mechanical Modelling of the Phase Change Material Heat Storage Devices
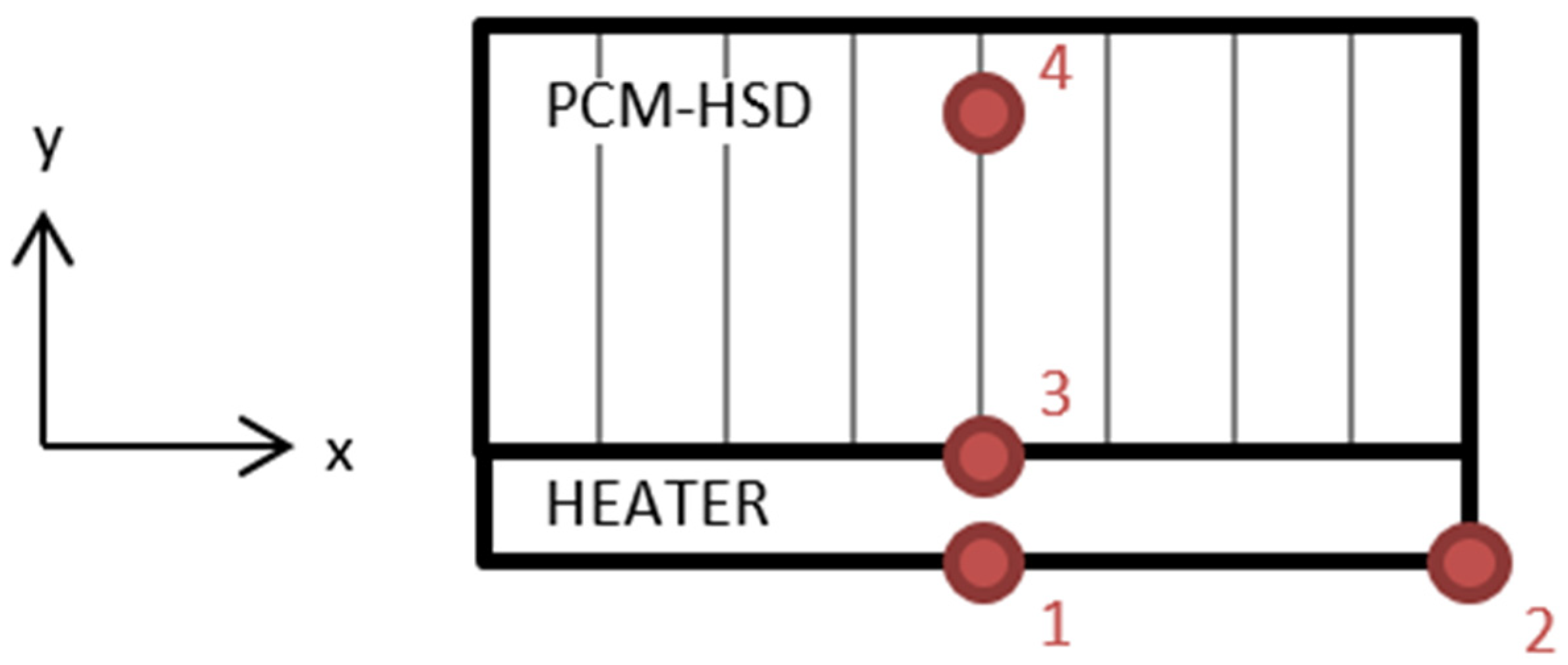
3.3.1. Thermal Behavior Modelling
3.3.2. Mechanical Behavior Modelling
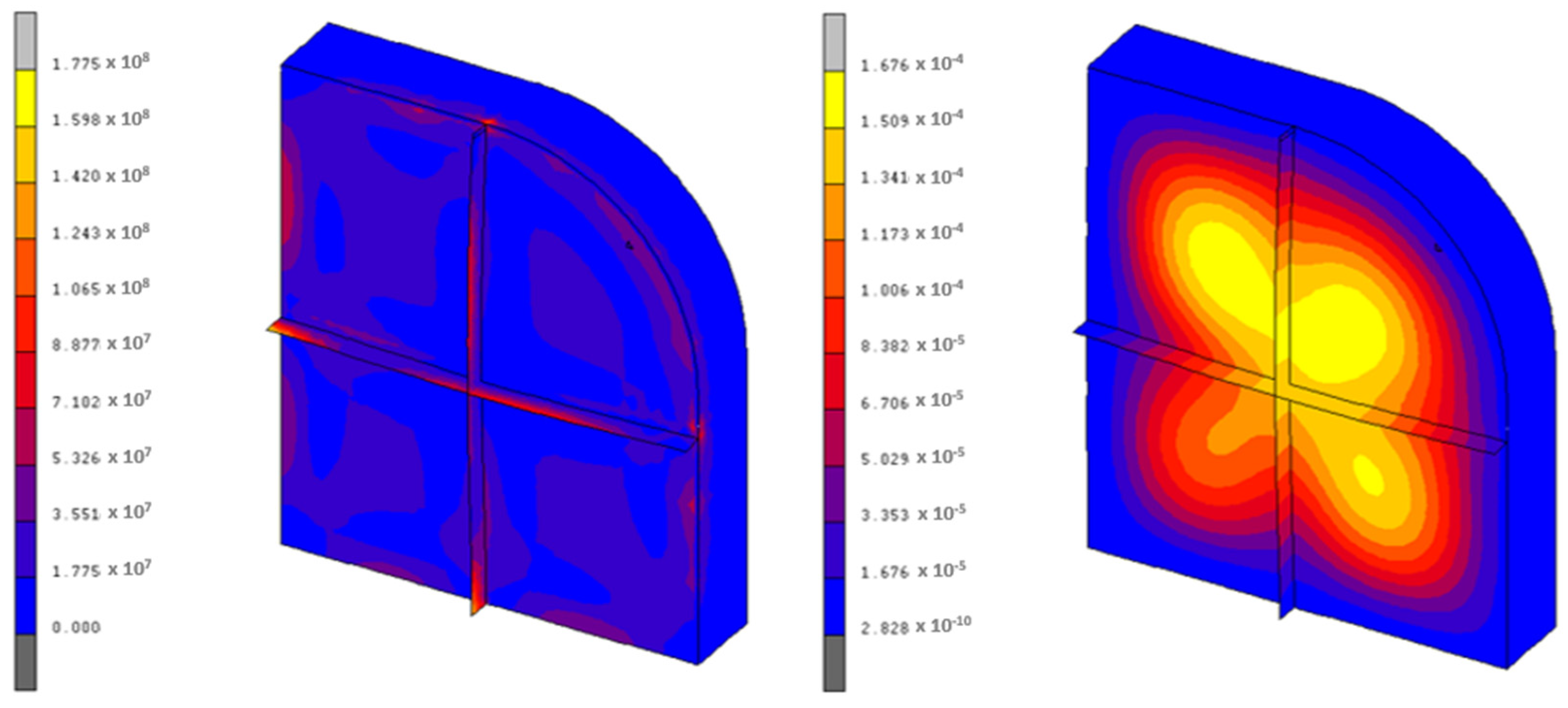
Volume Change during the Phase Change
Dynamic Response of the PCM Heat Storage Device
- First natural frequency must be higher than 140 Hz. This requirement was analyzed through an FEM calculation. The first frequency calculated is above 1200 Hz, so the requirement is fulfilled. It is interesting to note that as the PCM is considered in its liquid phase for this calculation, a non-geometrical mass homogenously applied on the inner walls of the container has been used.
- Random forced dynamic response. The requirements are collected in Table 5. Results show an RMS acceleration of 215 g, which produces a maximum of 18 MPa, well inside the allowed range.
- Sinus excitation. As stated in Table 5, the system must undergo a sinus excitation for frequencies between 5 and 1000 Hz of a 25 g level, with a sweep rate of 2 octaves/minute. As the first natural frequency is far enough from the excitation frequencies, the obtained von Mises stresses are quite low.
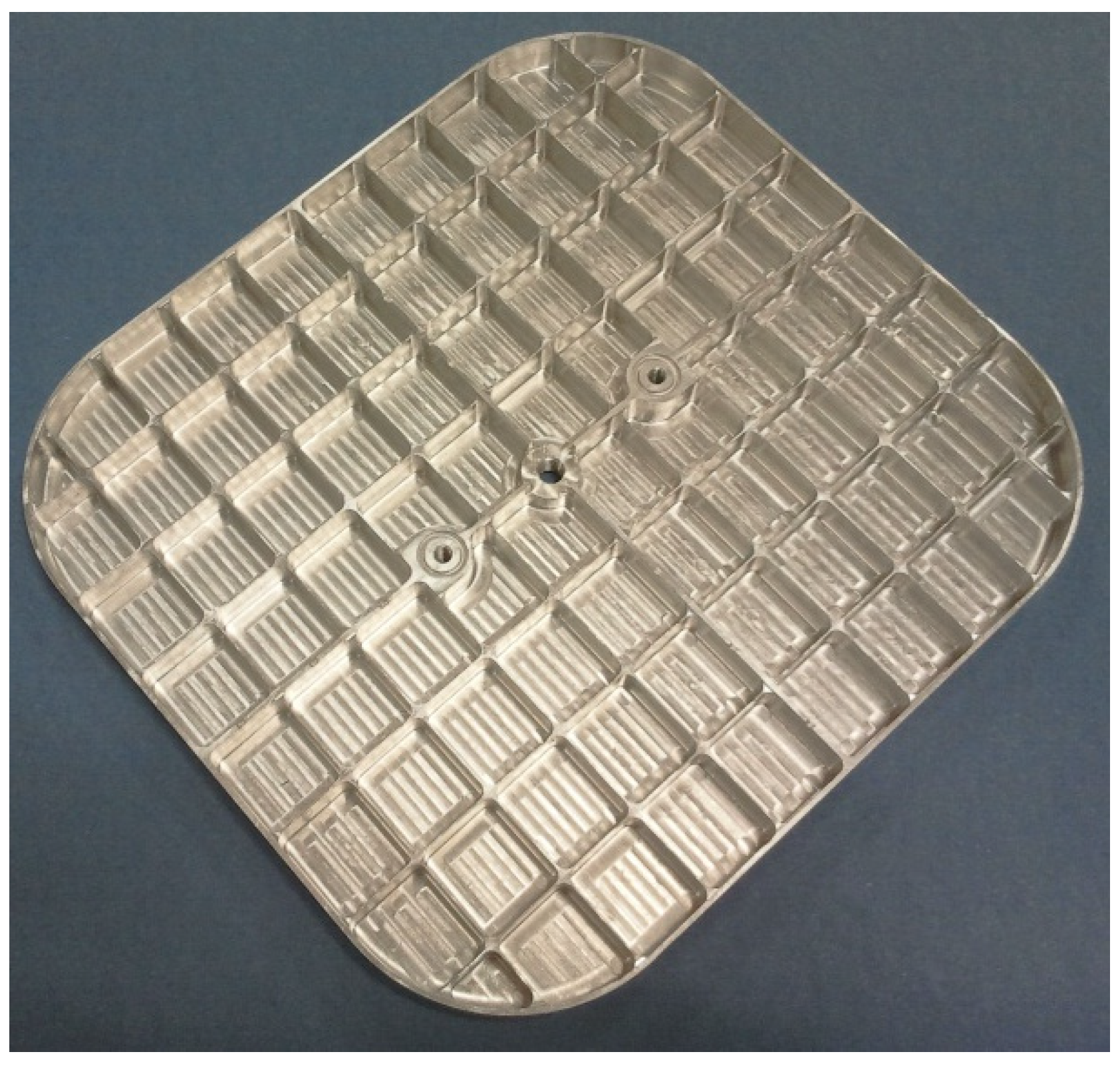
4. Fabrication of the Phase Change Material Heat Storage Devices
4.1. PCM Material
4.2. Container Material
4.3. Container Fabrication Process
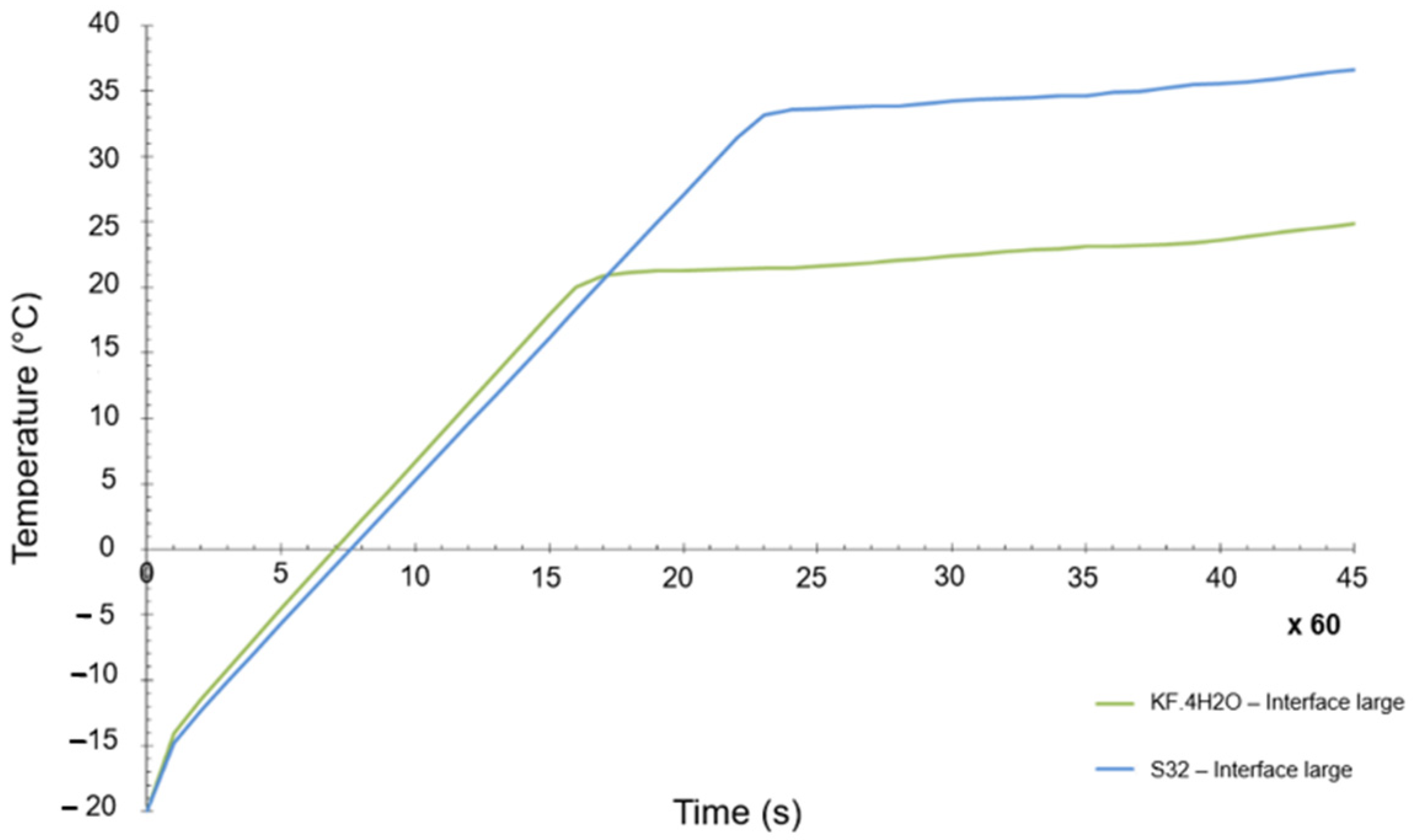
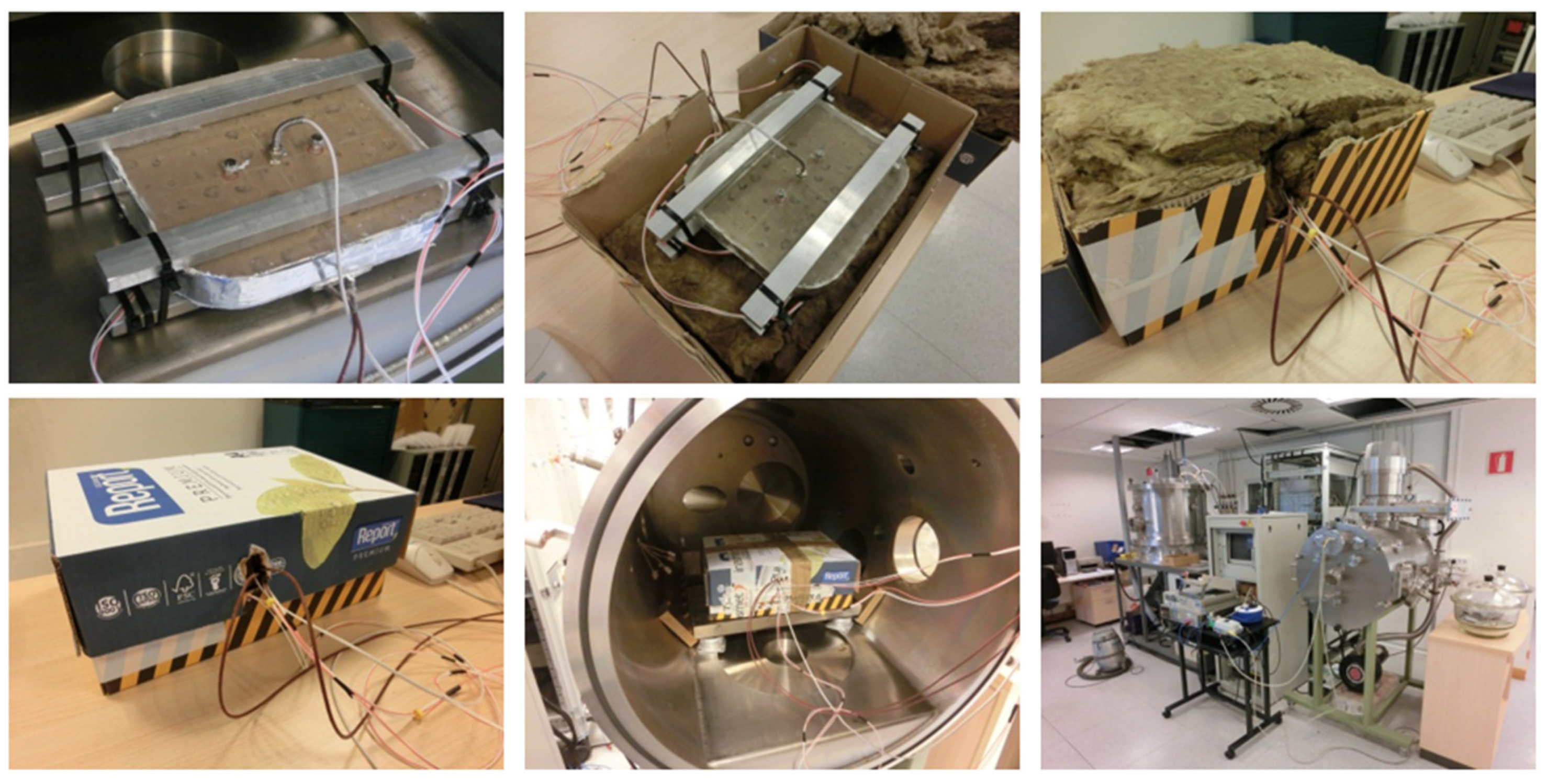
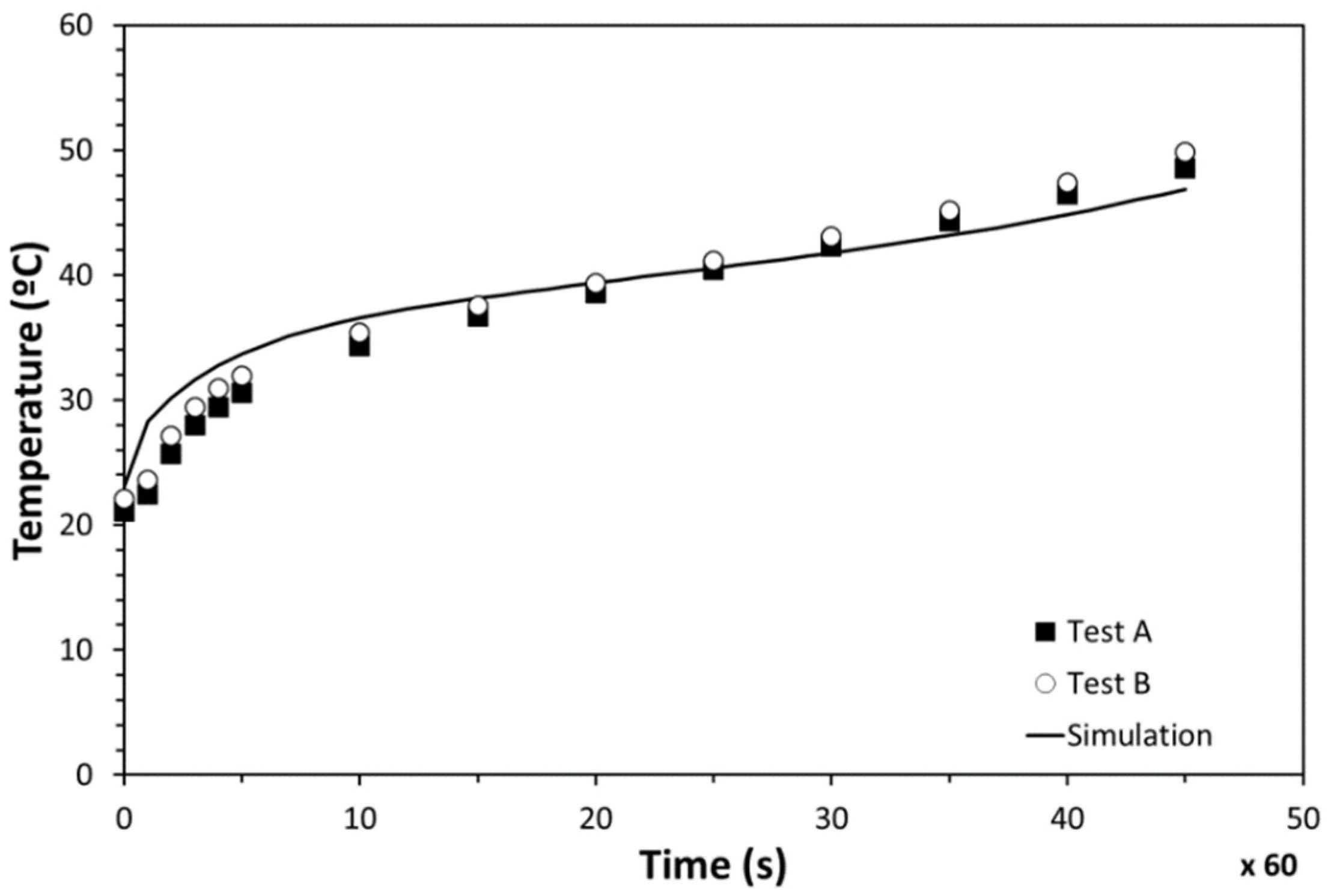
5. Testing and Experimental Validation
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Gilmore, D.G. Spacecraft Thermal Control Handbook, 2nd ed.; Gilmore, D.G., Ed.; American Institute of Aeronautics and Astronautics: El Segundo, CA, USA, 2002; Volume 1, ISBN 1-884989-11-X. [Google Scholar]
- Silk, E.A. Introduction to Spacecraft Thermal Design; Cambridge University Press: Cambridge, UK, 2020. [Google Scholar]
- Meseguer, J.; Pérez-Grande, I.; Sanz-Andrés, A. Spacecraft Thermal Control; Woodhead Publishing: Cambridge, UK, 2012; ISBN 978-0-84569-996-3. [Google Scholar]
- Miao, J.; Zhong, Q.; Zhao, Q.; Zhao, X. Spacecraft Thermal Control Technologies; Springer: Singapore, 2021; ISBN 978-981-15-4983-0. [Google Scholar]
- Karam, R.D. Satellite Thermal Control for Systems Engineers; Progress in Astronautics and Aeronautics; Zarchan, P., Ed.; American Institute of Aeronautics and Astronautics: Reston, VA, USA, 1998; Volume 181, ISBN 1-56347-276-7. [Google Scholar]
- Morea, S.F. The Lunar Roving Vehicle: Historical Perspective. In Proceedings of the The Second Conference on Lunar Bases and Space Activities of the 21st Century, Houston, TX, USA, 5–7 April 1988. [Google Scholar]
- Keville, J. Development of Phase Change Systems and Flight Experience on an Operational Satellite. In Proceedings of the 11th Thermophysics Conference, San Diego, CA, USA, 14–16 July 1976; American Institute of Aeronautics and Astronautics: Reston, Virigina, 1976. [Google Scholar]
- European Space Agency. Statement of Work, “Phase Change Materials for Space Thermal Applications”, TEC-MTT/2010/2721/Ln/RPL, AO6477-Ws00pe; European Space Agency: Paris, France, 2010. [Google Scholar]
- Zalba, B.; Marín, J.M.; Cabeza, L.F.; Mehling, H. Review on Thermal Energy Storage with Phase Change: Materials, Heat Transfer Analysis and Applications. Appl. Therm. Eng. 2003, 23, 251–283. [Google Scholar] [CrossRef]
- Lane, G.A. Solar Heat Storage: Latent Heat Materials, Volume 1: Background and Scientific Principles; CRC Press: Boca Raton, FL, USA, 1983. [Google Scholar]
- Abhat, A. Low Temperature Latent Heat Thermal Energy Storage: Heat Storage Materials. Sol. Energy 1983, 30, 313–332. [Google Scholar] [CrossRef]
- Humphries, W.R.; Griggs, E.I. A Design Handbook for Phase Change Thermal Control and Energy Storage Devices; National Aeronautics and Space Administration: Washington, DC, USA, 1977. [Google Scholar]
- Zhang, Y.; Zhou, G.; Lin, K.; Zhang, Q.; Di, H. Application of Latent Heat Thermal Energy Storage in Buildings: State-of-the-Art and Outlook. Build. Environ. 2007, 42, 2197–2209. [Google Scholar] [CrossRef]
- Ravikumar, M.; Srinivasan, P. Phase Change Materials as a Thermal Energy Storage Material for Cooling of Building. J. Theor. Appl. Inf. Technol. 2008, 4, 503–511. [Google Scholar]
- Nazari, A.; Emami, H. Thermal Control and Thermal Sensors of Observation Satellite. In Proceedings of the International Archives of the Photogrammetry, Remote Sensing and Spatial Information Sciences, Vol. XXXVII, Part B2, Beijing, China, 3–11 July 2008; pp. 949–952. [Google Scholar]
- Kürklü, A.; Wheldon, A.; Hadley, P. Mathematical Modelling of the Thermal Performance of a Phase-Change Material (PCM) Store: Cooling Cycle. Appl. Therm. Eng. 1996, 16, 613–623. [Google Scholar] [CrossRef]
- Stritih, U. An Experimental Study of Enhanced Heat Transfer in Rectangular PCM Thermal Storage. Int. J. Heat Mass Transf. 2004, 47, 2841–2847. [Google Scholar] [CrossRef]
- Fan, L.; Khodadadi, J.M. Thermal Conductivity Enhancement of Phase Change Materials for Thermal Energy Storage: A Review. Renew. Sustain. Energy Rev. 2011, 15, 24–46. [Google Scholar] [CrossRef]
- Nayak, K.C.; Saha, S.K.; Srinivasan, K.; Dutta, P. A Numerical Model for Heat Sinks with Phase Change Materials and Thermal Conductivity Enhancers. Int. J. Heat Mass Transf. 2006, 49, 1833–1844. [Google Scholar] [CrossRef]
- Wu, W.; Liu, N.; Cheng, W.; Liu, Y. Study on the Effect of Shape-Stabilized Phase Change Materials on Spacecraft Thermal Control in Extreme Thermal Environment. Energy Convers. Manag. 2013, 69, 174–180. [Google Scholar] [CrossRef]
- Baturkin, V. Micro-Satellites Thermal Control—Concepts and Components. Acta Astronaut. 2005, 56, 161–170. [Google Scholar] [CrossRef] [Green Version]
- K&K Associates. Thermal Network Modeling Handbook; K&K Associates, Ed.; K&K Associates: Westminster, CO, USA, 2002. [Google Scholar]
- Redor, J.F. Introduction to Spacecraft Thermal Control; ESA-EWP1599; ESA Publications Division: Noordwijk, The Netherlands, 1995. [Google Scholar]
- Garmendia, I.; Anglada, E.; Vallejo, H.; Seco, M. Accurate Calculation of Conductive Conductances in Complex Geometries for Spacecrafts Thermal Models. Adv. Space Res. 2016, 57, 1087–1097. [Google Scholar] [CrossRef]
- Incropera, F.P.; Dewitt, D.P.; Bergman, T.L.; Lavine, A.S. Fundamentals of Heat and Mass Transfer, 6th ed.; John Wiley & Sons: Hoboken, NJ, USA, 2006. [Google Scholar]
- García-Romero, A.; Delgado, A.; Urresti, A.; Martín, K.; Sala, J.M. Corrosion Behaviour of Several Aluminium Alloys in Contact with a Thermal Storage Phase Change Material Based on Glauber’s Salt. Corros. Sci. 2009, 51, 1263–1272. [Google Scholar] [CrossRef]



| PCM | Type | Tfusion (°C) | (kJ/kg) | (kg/m3) | (W/(m·K)) | Cp (J/(kg·K)) | EPCM (J) |
|---|---|---|---|---|---|---|---|
| RT5HC | Organic | 6 | 214 | 880/763 | 0.2 | 1800/2400 | 60,553 |
| Material | Cp (J/(kg·K)) | K (W/(m·K)) | ||
|---|---|---|---|---|
| Aluminum 6101 Duocel® foam | 207 | 2700 | 895 | 218 |
| Material | ρ (kg/m3) | Cp (J/(kg·K)) | K (W/(m·K)) |
|---|---|---|---|
| Aluminum | 2700 | 895 | 218 |
| Property | RT5HC | KF·4H2O |
|---|---|---|
| Melting point (°C) | 6 | 18.5 |
| Latent heat (kJ/kg) | 245 | 231 |
| Solid phase density (kg/m3) | 880 | 1455 |
| Liquid phase density (kg/m3) | 763 | 1447 |
| Specific heat solid phase (J/kg/°C) | 1800 | 1840 |
| Specific heat liquid phase (J/kg/°C) | 2400 | 1850 |
| Thermal conductivity (W/m/°C) | 0.2 | 0.2 |
| Excitation | Frequency (Hz) | Level |
|---|---|---|
| Sinusoidal | 5–1000 | 25 g |
| Random | 20–100 | +3 dB/oct |
| Random | 100–300 | 0.3 g2/Hz |
| Random | 300–2000 | −5 dB/oct |
| Property | PlusICE S32 |
|---|---|
| Melting point (°C) | 32 |
| Latent heat (kJ/kg) | 200 |
| Density (kg/m3) | 1460 |
| Specific heat (J/kg/°C) | 1910 |
| Thermal conductivity (W/m/°C) | 0.51 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Garmendia, I.; Vallejo, H.; Seco, M.; Anglada, E. Design and Fabrication of a Phase Change Material Heat Storage Device for the Thermal Control of Electronics Components of Space Applications. Aerospace 2022, 9, 126. https://doi.org/10.3390/aerospace9030126
Garmendia I, Vallejo H, Seco M, Anglada E. Design and Fabrication of a Phase Change Material Heat Storage Device for the Thermal Control of Electronics Components of Space Applications. Aerospace. 2022; 9(3):126. https://doi.org/10.3390/aerospace9030126
Chicago/Turabian StyleGarmendia, Iñaki, Haritz Vallejo, Miguel Seco, and Eva Anglada. 2022. "Design and Fabrication of a Phase Change Material Heat Storage Device for the Thermal Control of Electronics Components of Space Applications" Aerospace 9, no. 3: 126. https://doi.org/10.3390/aerospace9030126
APA StyleGarmendia, I., Vallejo, H., Seco, M., & Anglada, E. (2022). Design and Fabrication of a Phase Change Material Heat Storage Device for the Thermal Control of Electronics Components of Space Applications. Aerospace, 9(3), 126. https://doi.org/10.3390/aerospace9030126







