Multi-Scale Nonlinear Progressive Damage and Failure Analysis for Open-Hole Composite Laminates
Abstract
:1. Introduction
2. Multiscale Method of Progressive Damage and Failure
2.1. ST Model
2.2. Constitutive Relation
2.3. Calculating the Damage State
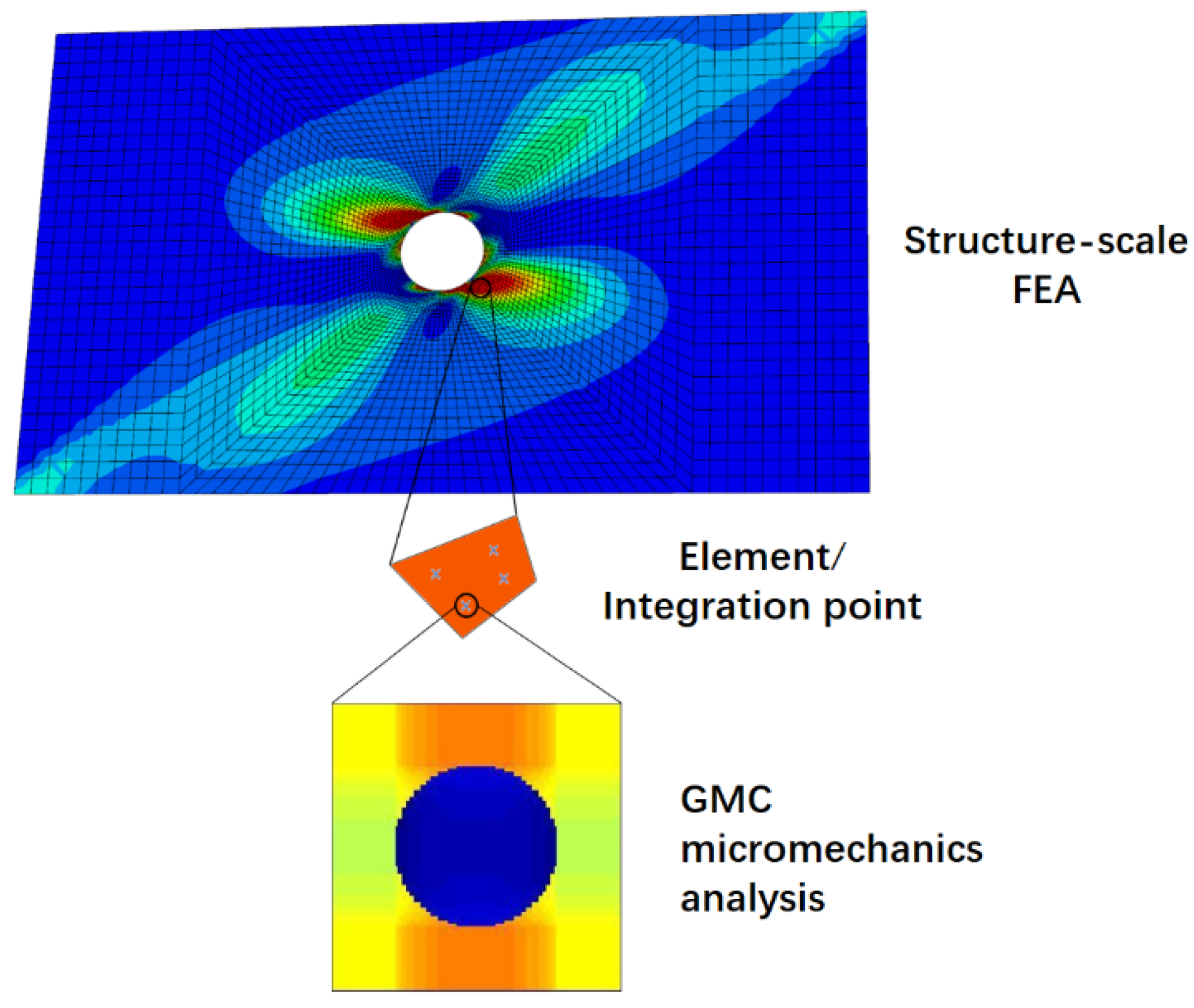
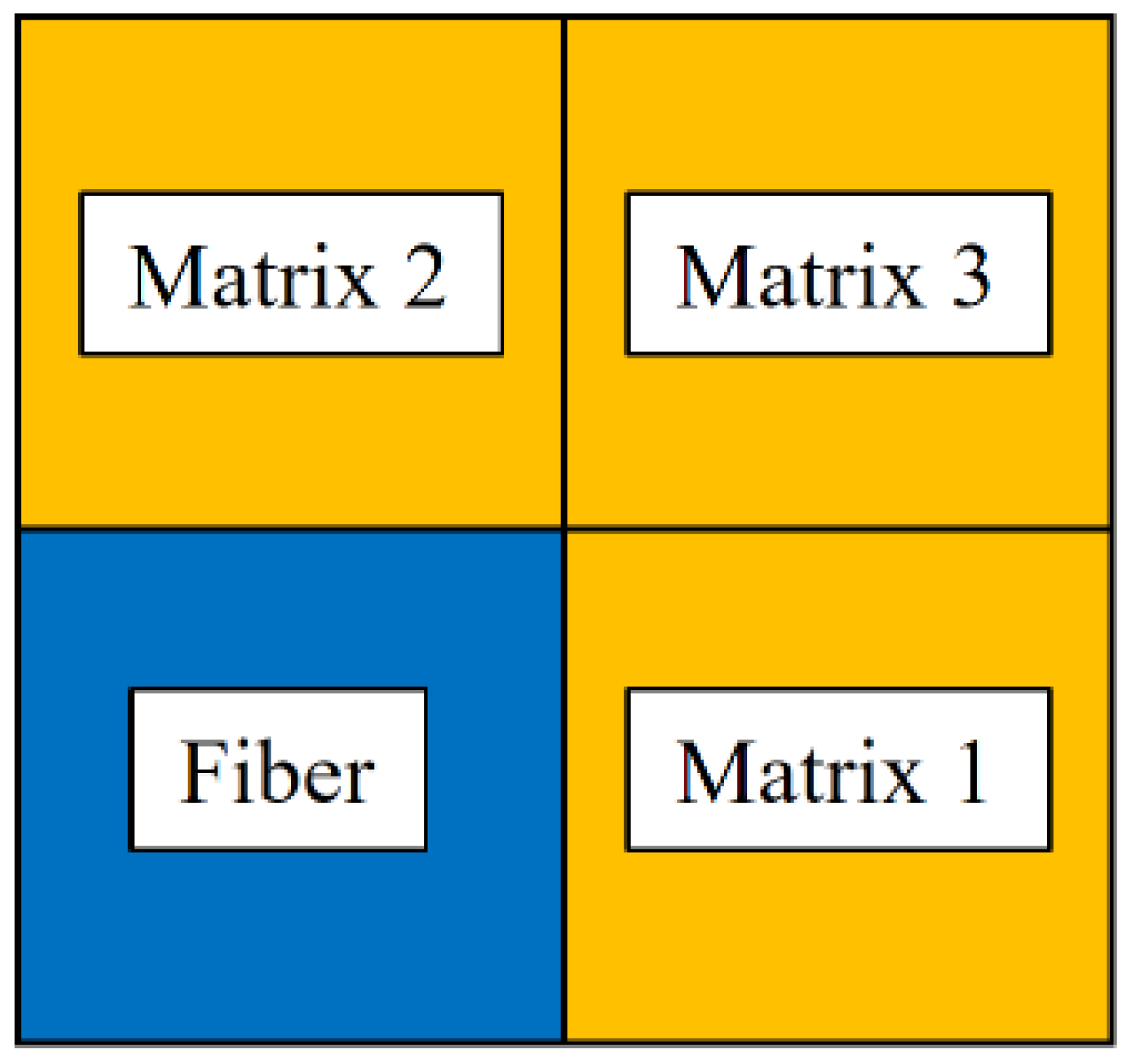
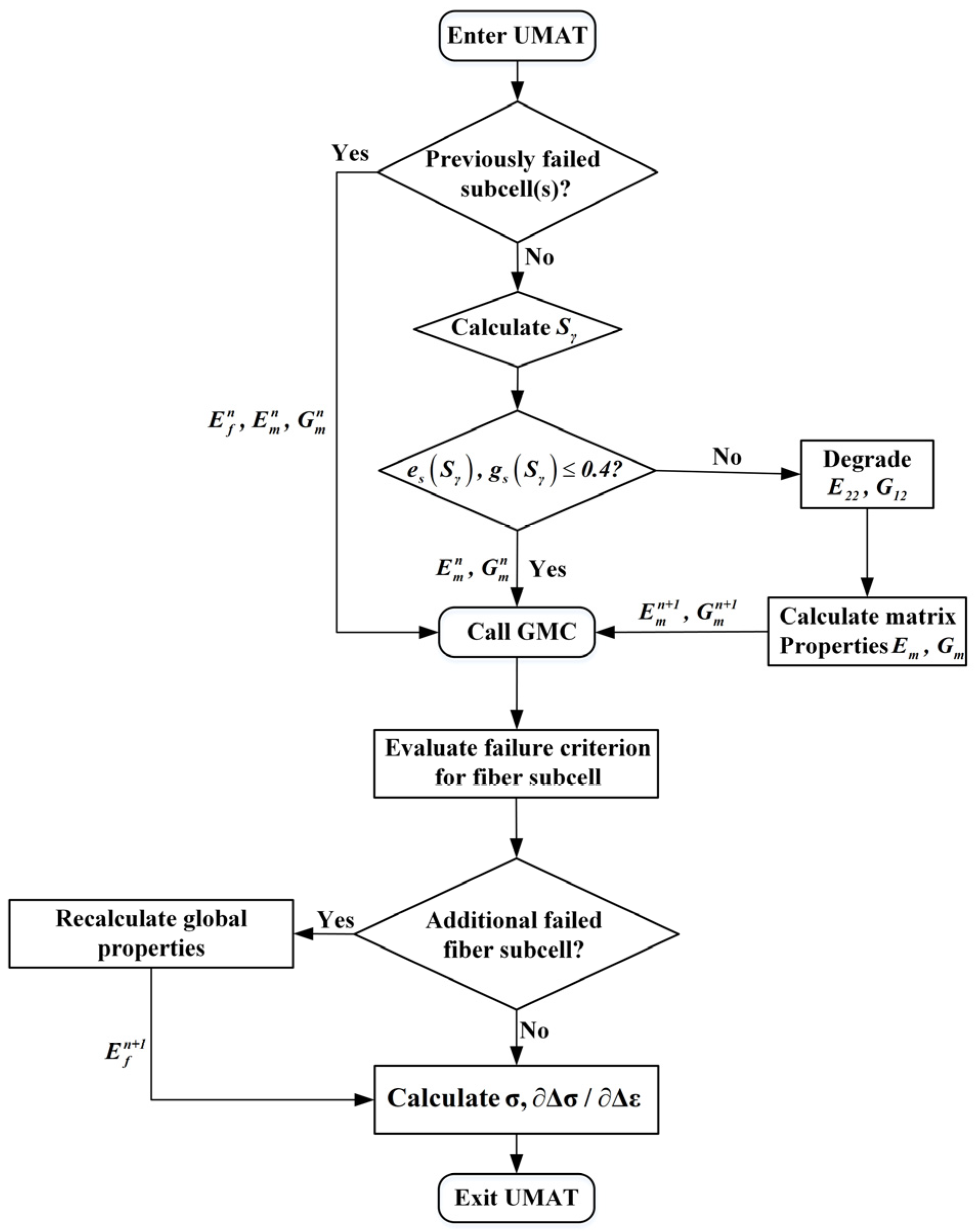
2.4. Generalized Method of Cells
3. Analysis Method
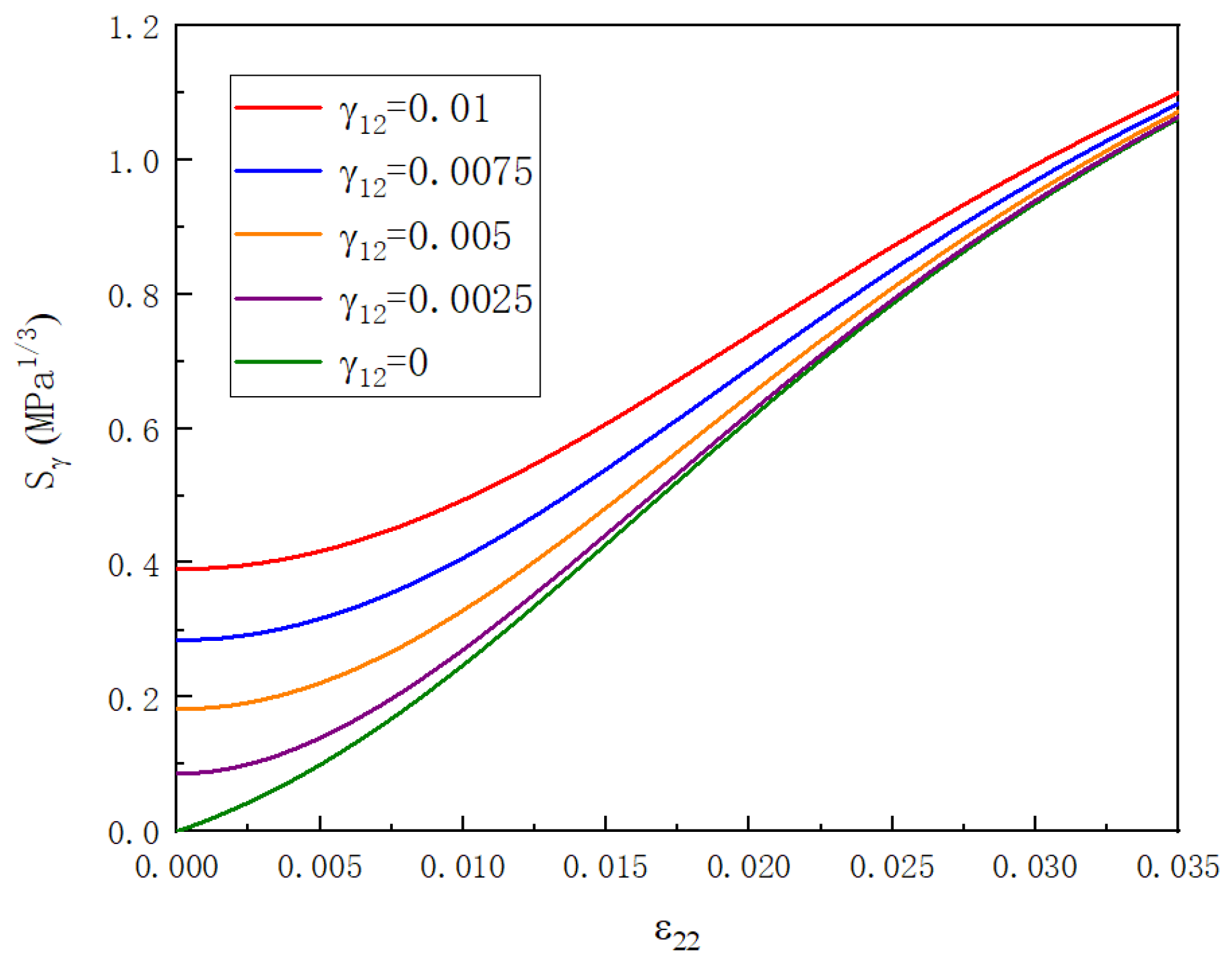
3.1. Lamina Level Damage and Failure Model Based on ST
3.2. Micro-Scale Damage and Failure Model
3.3. Lamina-Scale Failure Model-Based Hashin-Rotem Theory
4. Results and Discussion
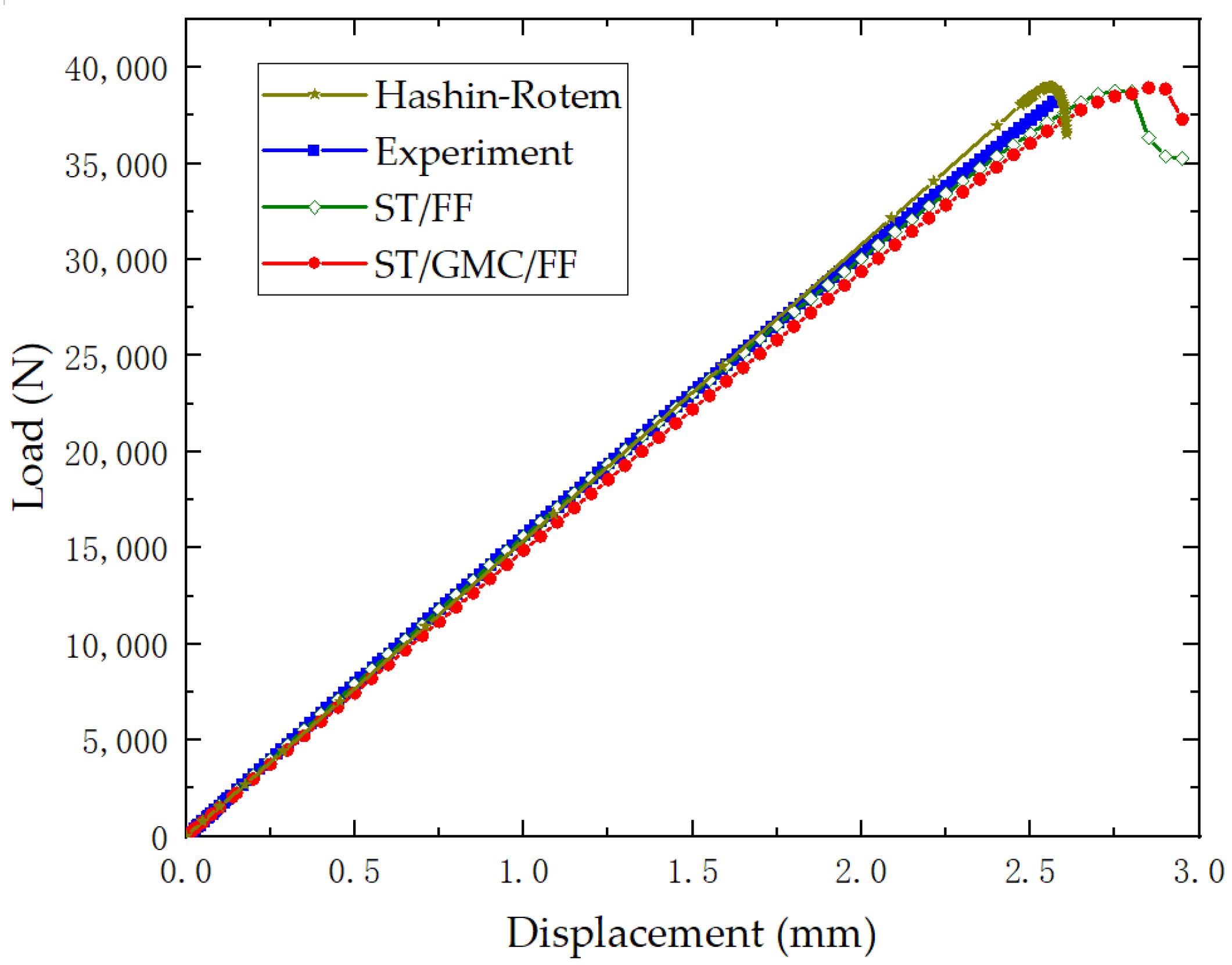
4.1. Experimental Result
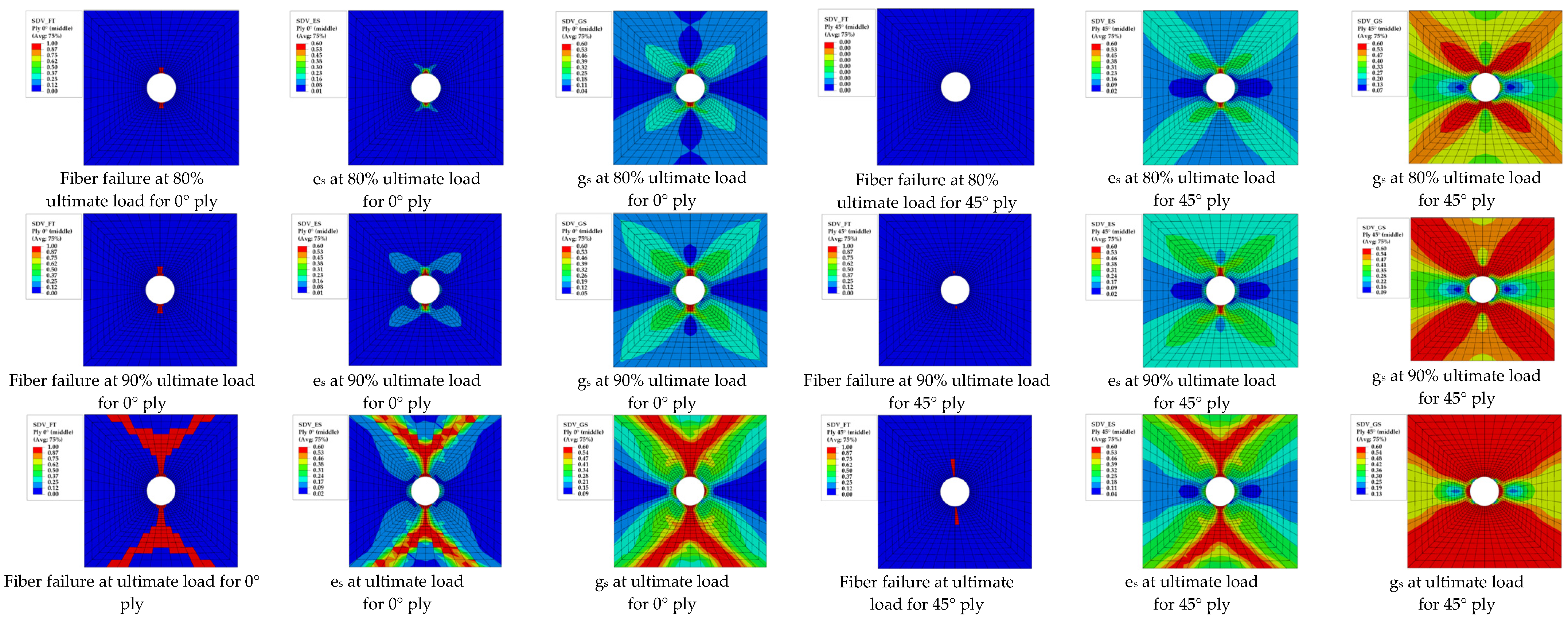
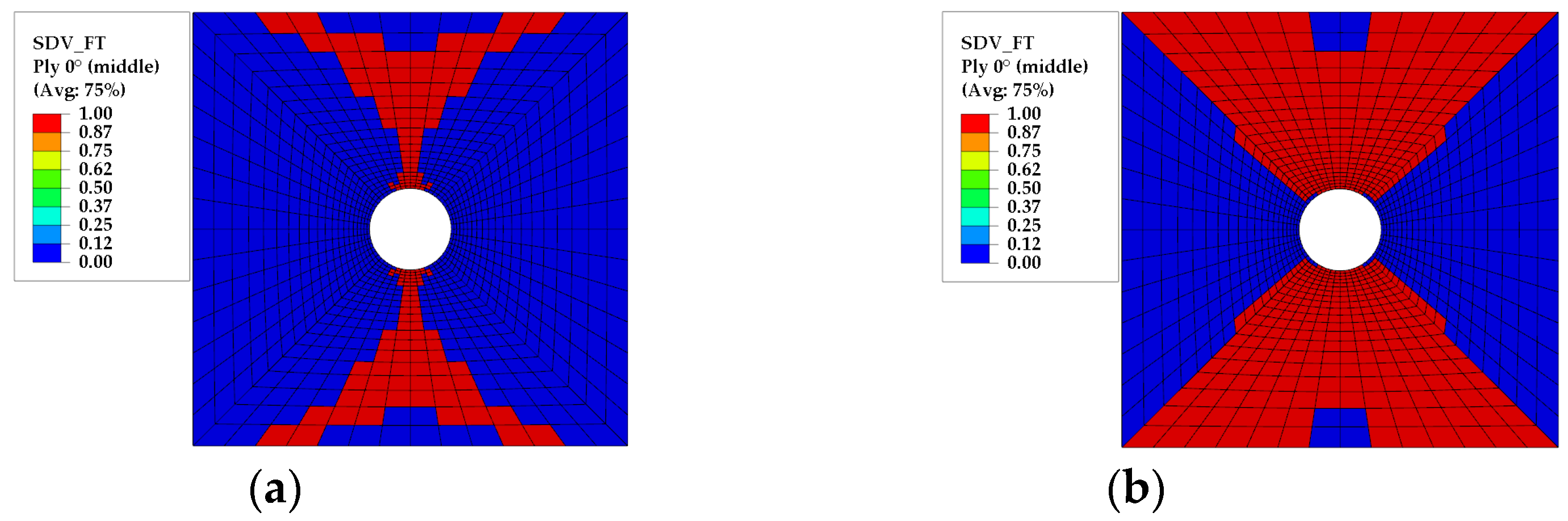
4.2. Results of Finite Element Analysis
5. Conclusions
- ST/FF nonlinear damage model, Hashin-Rotem progressive damage analysis method, and the ST/GMC/FF model based on the multi-scale progressive damage model are ideal to predict the damage state, failure pattern and the ultimate load, where the ST method well captured the nonlinear behavior of the laminates and reasonable failure mode prediction was obtained. The ST method makes the matrix damage become a natural nonlinear damage evolution and replaces the traditional matrix failure criterion model. Fiber tensile failure shows obvious brittleness behavior, so the failure criterion of fiber is still needed in both macroscopic and microscopic models.
- Based on the multi-scale progressive damage model of GMC model, ST/GMC/FF methods will resolve stresses or strains of a laminate to the repeating unit cell at the microscopic level, which make the matrix and fiber constituents to be distinguishable, and different matrix subcells also have independent damage evolvement and failure states. It shows that the multi-scale method based on the GMC model can better explain the crack path and failure mechanism for the matrix constituent of a laminate.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Soden, P.D.; Hinton, M.J.; Kaddour, A.S. Lamina properties, lay-up configurations and loading conditions for a range of fibre-reinforced composite laminates. Compos. Sci. Technol. 1998, 58, 1011–1022. [Google Scholar] [CrossRef]
- Soden, P.D.; Hinton, M.J.; Kaddour, A.S. A comparison of the predictive capabilities of current failure theories for composite laminates. Compos. Sci. Technol. 1998, 58, 1225–1254. [Google Scholar] [CrossRef]
- Hinton, M.J.; Kaddour, A.S.; Soden, P.D. A comparison of the predictive capabilities of current failure theories for composite laminates, judged against experimental evidence. Compos. Sci. Technol. 2002, 62, 1725–1797. [Google Scholar] [CrossRef]
- Puck, A.; Schürmann, H. Failure analysis of FRP laminates by means of physically based phenomenological models. Compos. Sci. Technol. 2002, 62, 1633–1662. [Google Scholar] [CrossRef]
- Pinho, S.; Iannucci, L.; Robinson, P. Physically-based failure models and criteria for laminated fibre reinforced composites with emphasis on fibre kinking: Part I: Development. Compos. Part A Appl. Sci. Manuf. 2006, 37, 63–73. [Google Scholar] [CrossRef] [Green Version]
- Icardi, U.; Locatto, S.; Longo, A. Assessment of recent theories for predicting failure of composite laminates. Appl. Mech. Rev. 2007, 60, 76–86. [Google Scholar] [CrossRef]
- Beaumont, P.; Dimant, R.A.; Shercliff, H.R. Failure processes in composite materials: Getting physical. J. Mater. Sci. 2006, 41, 6526–6546. [Google Scholar] [CrossRef]
- Mehrmashhadi, J.; Chen, Z.; Zhao, J.; Bobaru, F. A stochastically homogenized peridynamic model for intraply fracture in fiber-reinforced composites. Compos. Sci. Technol. 2019, 182, 107770. [Google Scholar] [CrossRef]
- Mehrmashhadi, J.; Tang, Y.; Zhao, X.; Xu, Z.; Pan, J.; Le, Q.V.; Bobaru, F. The effect of solder joint microstructure on the drop test failure—a peridynamic analysis. IEEE Trans. Compon. Packag. Manuf. Technol. 2018, 9, 58–71. [Google Scholar] [CrossRef]
- Tallia, R.; Morello, P.; Castellano, G. Damage and failure of composite materials. AIAA J. 2012, 52, 317–323. [Google Scholar]
- Hallett, S.R.; Green, B.G.; Jiang, W.G.; Wisnom, M.R. An experimental and numerical investigation into the damage mechanisms in notched composites. Compos. Part A Appl. Sci. Manuf. 2009, 40, 613–624. [Google Scholar] [CrossRef]
- Camanho, P.P.; Erçin, G.H.; Catalanotti, G.; Mahdi, S.; Linde, P. A finite fracture mechanics model for the prediction of the open-hole strength of composite laminates. Compos. A Appl. Sci. Manuf. 2012, 43, 1219–1225. [Google Scholar] [CrossRef] [Green Version]
- Pierron, F.; Green, B.; Wisnom, M.R.; Hallet, S.R. Full-field assessment of the damage process of laminated composite open-hole tensile specimens. Part II Exp. Results Compos. Part A Appl. Sci. Manuf. 2007, 38, 2321–2332. [Google Scholar] [CrossRef]
- Orifici, A.C.; Herszberg, I.; Thomson, R.S. Review of methodologies for composite material modelling incorporating failure. Compos. Struct. 2008, 86, 194–210. [Google Scholar] [CrossRef]
- Sadeghi, G.; Hosseini-Toudeshky, H.; Mohammadi, B. An investigation of matrix cracking damage evolution in composite laminates–development of an advanced numerical tool. Compos. Struct. 2014, 108, 937–950. [Google Scholar] [CrossRef]
- Iarve, E.V.; Mollenhauer, D.; Kim, R. Theoretical and experimental investigation of stress redistribution in open hole composite laminates due to damage accumulation. Compos. Part A Appl. Sci. Manuf. 2005, 36, 163–1717. [Google Scholar] [CrossRef]
- Talreja, R. Multi-scale modeling in damage mechanics of composite materials. J. Mater. Sci. 2006, 41, 6800–6812. [Google Scholar] [CrossRef]
- Paley, M.; Aboudi, J. Micromechanical analysis of composites by the generalized cells model. Mech. Mater. 1992, 14, 127–139. [Google Scholar] [CrossRef]
- Pindera, M.J.; Bednarcyk, B.A. An efficient implementation of the generalized method of cells for unidirectional, multi-phased composites with complex microstructures. Compos. Part B-Eng. 1999, 30, 87–105. [Google Scholar] [CrossRef]
- Naghipour, P.; Arnold, S.M.; Pineda, E.J.; Stier, B.; Hansen, L.; Bednarcyk, B.A.; Waas, A.M. Multiscale static analysis of notched and unnotched laminates using the generalized method of cells. J. Compos. Mater. 2016, 51, 1433–1454. [Google Scholar] [CrossRef] [Green Version]
- Shi, J.; Tong, M.; Zhou, C.; Ye, C.; Wang, X. Progressive Failure Analysis in Open-Hole Tensile Composite Laminates of Airplane Stringers Based on Tests and Simulations. Appl. Sci. 2020, 11, 185. [Google Scholar] [CrossRef]
- Schapery, R.A. A theory of mechanical behavior of elastic media with growing damage and other changes in structure. J. Mech. Phys. Solids 1990, 38, 215–253. [Google Scholar] [CrossRef]
- Schapery, R.A. Prediction of compressive strength and kink bands in composites using a work potential. Int. J. Solids. Struct. 1995, 32, 739–765. [Google Scholar] [CrossRef]
- Lamborn, M.J.; Schapery, R.A. An investigation of the existence of a work potential for fiber-reinforced plastic. J. Compos. Mater. 1993, 27, 352–382. [Google Scholar] [CrossRef]
- Sicking, D.L. Mechanical Characterization of Nonlinear Laminated Composites with Traverse Crack Growth; Texas AM University College: College Station, TX, USA, 1992. [Google Scholar]
- Pineda, E.J.; Waas, A.M. Numerical implementation of a multiple-ISV thermodynamically-based work potential theory for modeling progressive damage and failure in fiber-reinforced laminates. Int. J. Fract. 2013, 182, 93–122. [Google Scholar] [CrossRef] [Green Version]


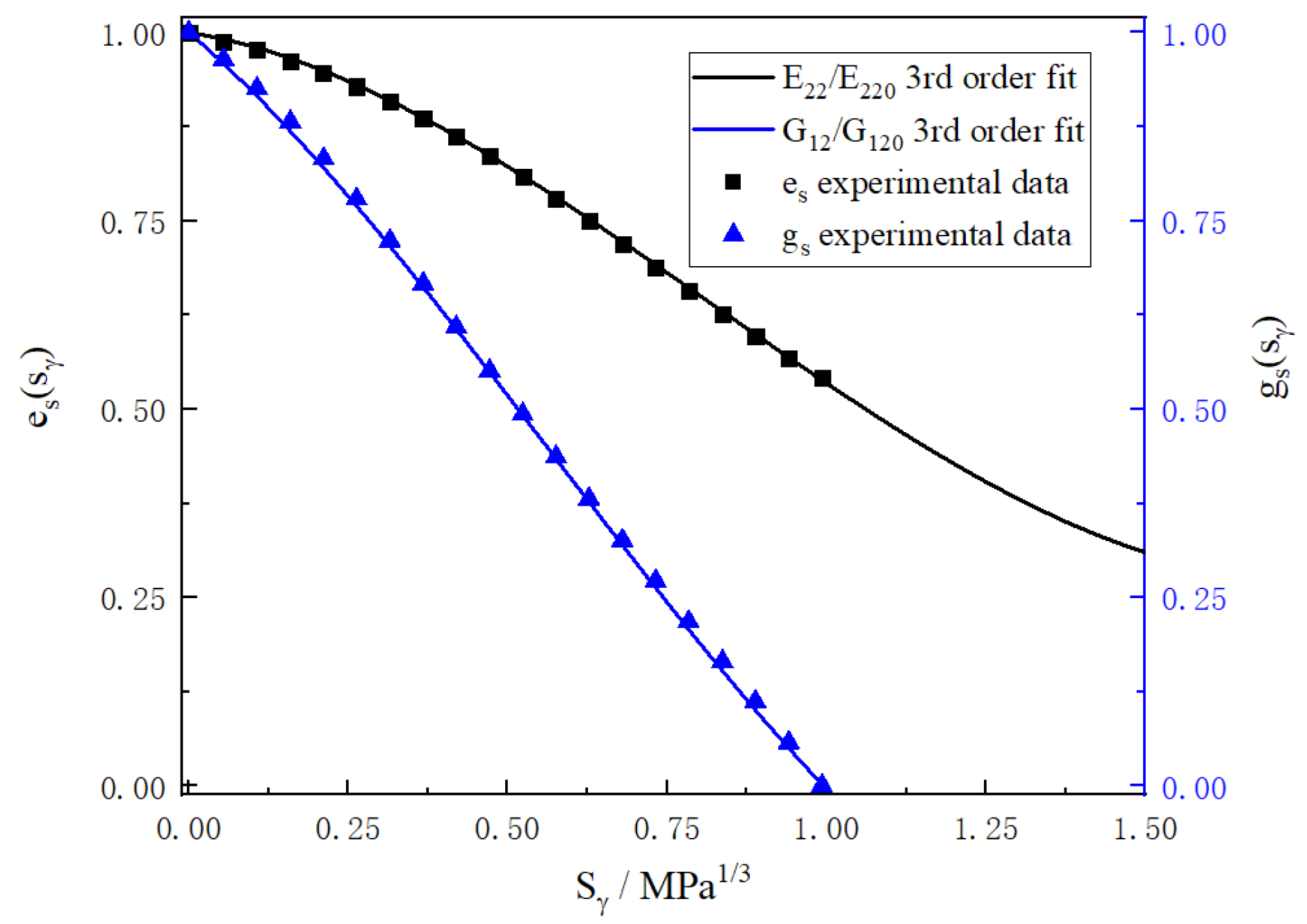



| E22 Coefficients | Values | G12 Coefficients | Values |
|---|---|---|---|
| eS0 | 1 | gS0 | 1 |
| eS1 | −0.1308 | gS1 | −0.7269 |
| eS2 | −0.5650 | gS2 | −0.6706 |
| eS3 | 0.2304 | gS3 | 0.3932 |
| Fiber Properties | Values/GPa | Matrix Properties | Values/GPa |
|---|---|---|---|
| Ef11 | 293 | Em11 | 2350 |
| Ef22 | 91 | Em22 | 2350 |
| υf12 | 0.23 | υm12 | 0.35 |
| υf23 | 0.45 | υm23 | 0.35 |
| Gf12 | 55 | Gm12 | 2300 |
| Properties | Values/MPa |
|---|---|
| Tensile fiber strength, Xft | 5200 |
| Compressive fiber strength, Xfc | 2855 |
| Matrix transverse tensile strength, Ymt | 49 |
| Matrix transverse compressive strength, Ymc | 124 |
| Matrix shear strength, S | 41 |
| Elastic Properties | Values | Elastic Properties | Values |
|---|---|---|---|
| E1/GPa | 154 | G23/MPa | 4340 |
| E2/GPa | 9 | Em12 | 0.29 |
| E3/GPa | 9 | vm13 | 0.29 |
| G12/MPa | 4340 | vm23 | 0.45 |
| G13/MPa | 4340 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shi, J.; Tong, M. Multi-Scale Nonlinear Progressive Damage and Failure Analysis for Open-Hole Composite Laminates. Aerospace 2022, 9, 59. https://doi.org/10.3390/aerospace9020059
Shi J, Tong M. Multi-Scale Nonlinear Progressive Damage and Failure Analysis for Open-Hole Composite Laminates. Aerospace. 2022; 9(2):59. https://doi.org/10.3390/aerospace9020059
Chicago/Turabian StyleShi, Jian, and Mingbo Tong. 2022. "Multi-Scale Nonlinear Progressive Damage and Failure Analysis for Open-Hole Composite Laminates" Aerospace 9, no. 2: 59. https://doi.org/10.3390/aerospace9020059
APA StyleShi, J., & Tong, M. (2022). Multi-Scale Nonlinear Progressive Damage and Failure Analysis for Open-Hole Composite Laminates. Aerospace, 9(2), 59. https://doi.org/10.3390/aerospace9020059





