Application of Fiber Optic Sensing System for Predicting Structural Displacement of a Joined-Wing Aircraft
Abstract
1. Introduction
2. Theoretical Background
2.1. Principle of Fiber Optic Sensing
2.2. Strain-to-Displacement Transformation (SDT)
3. System Development
3.1. Model Description: A Joined-Wing Aircraft
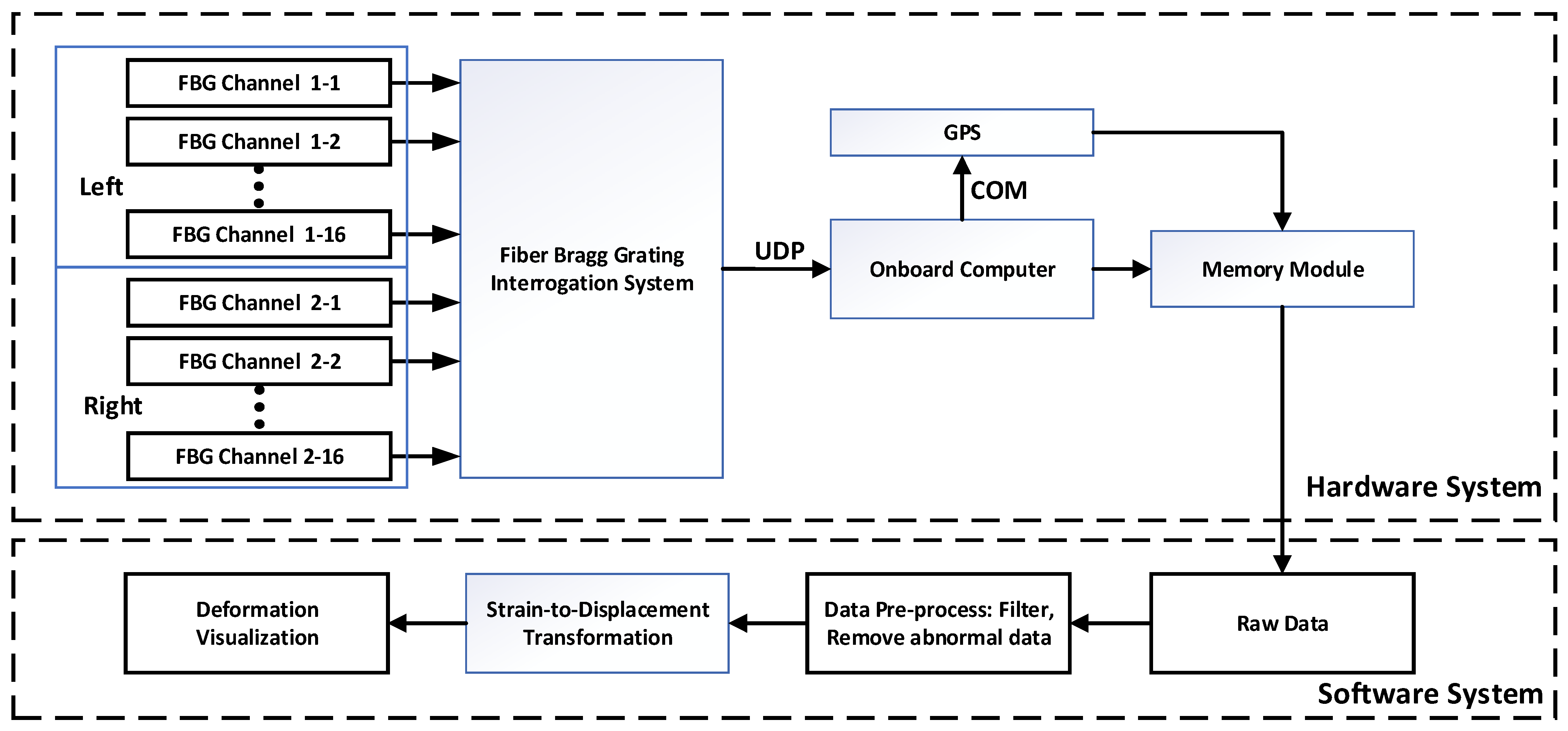
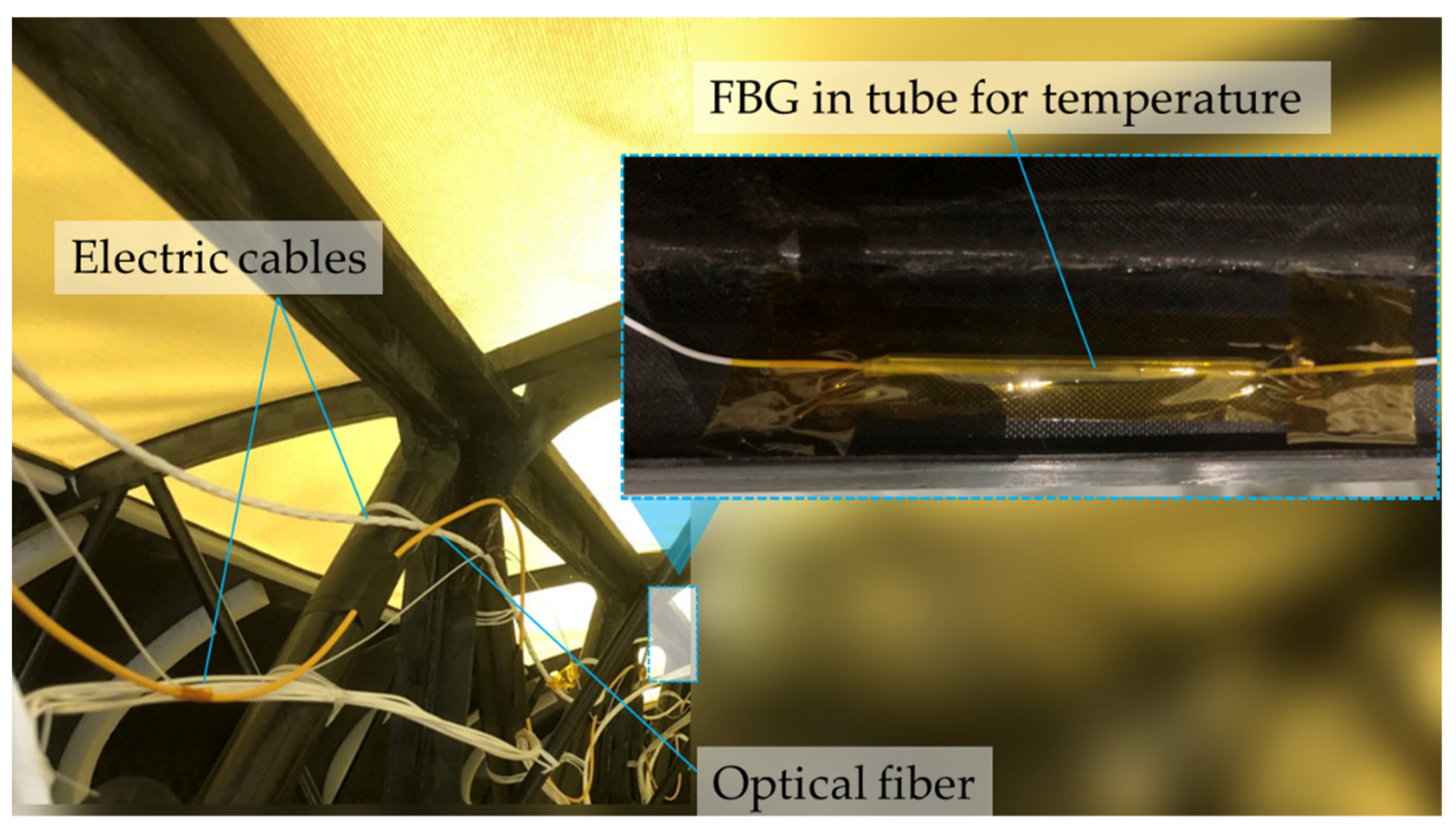
3.2. FOSS Design
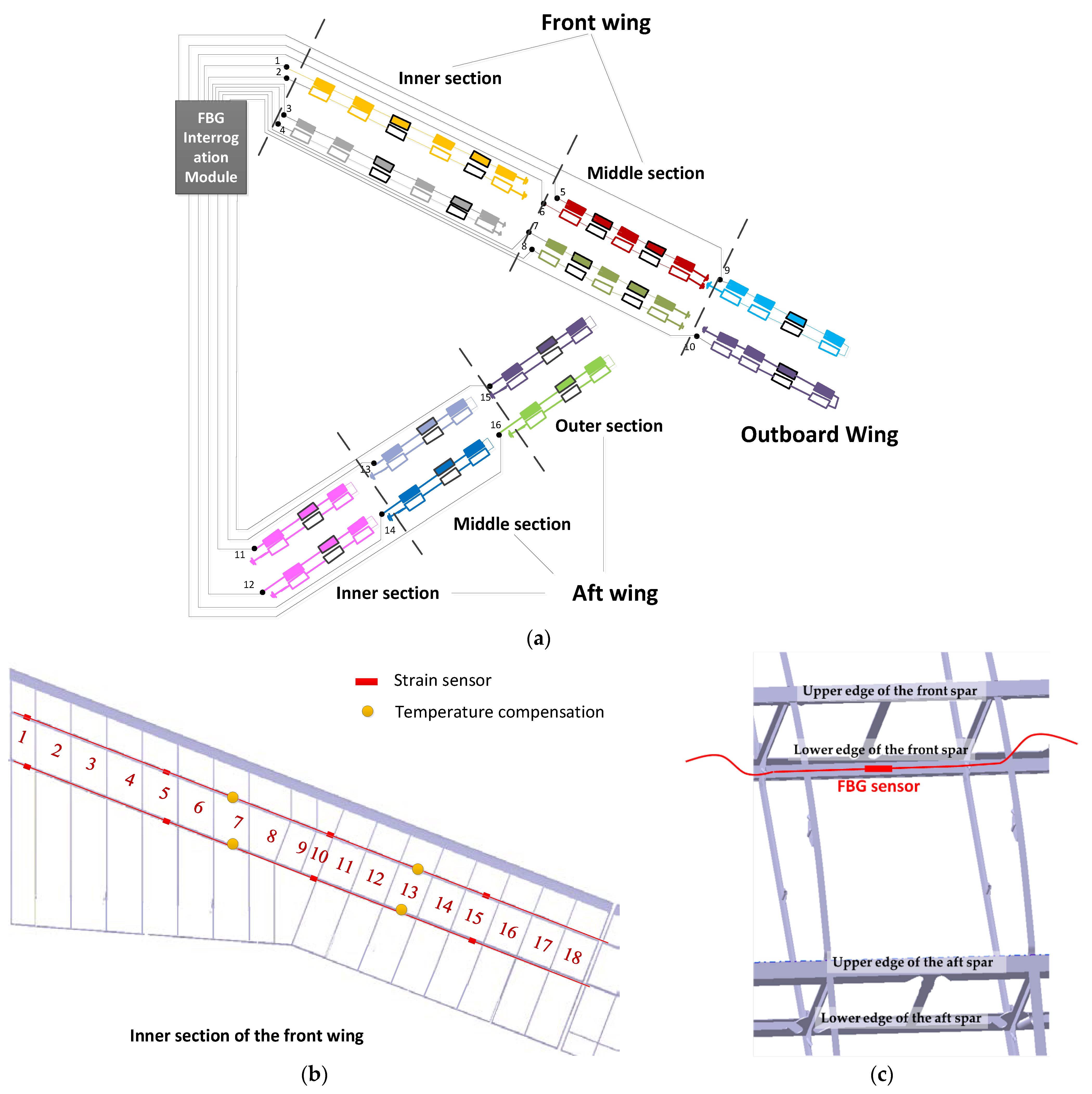
3.3. Sensor Arrangement
4. Results and Discussion
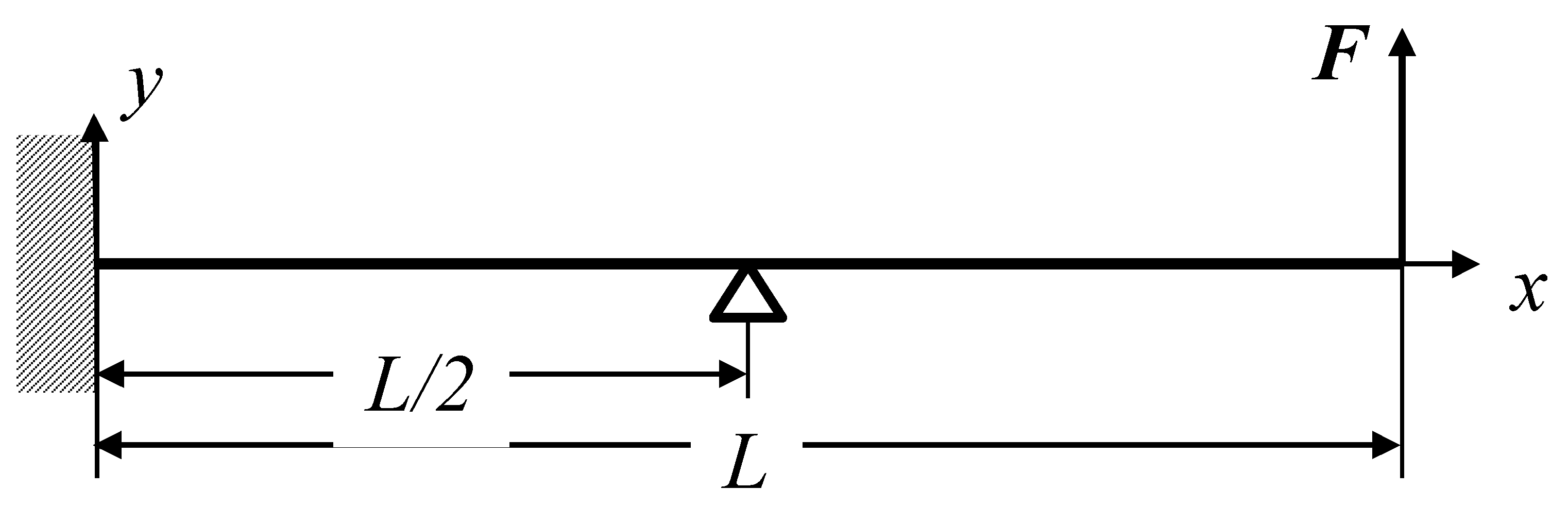
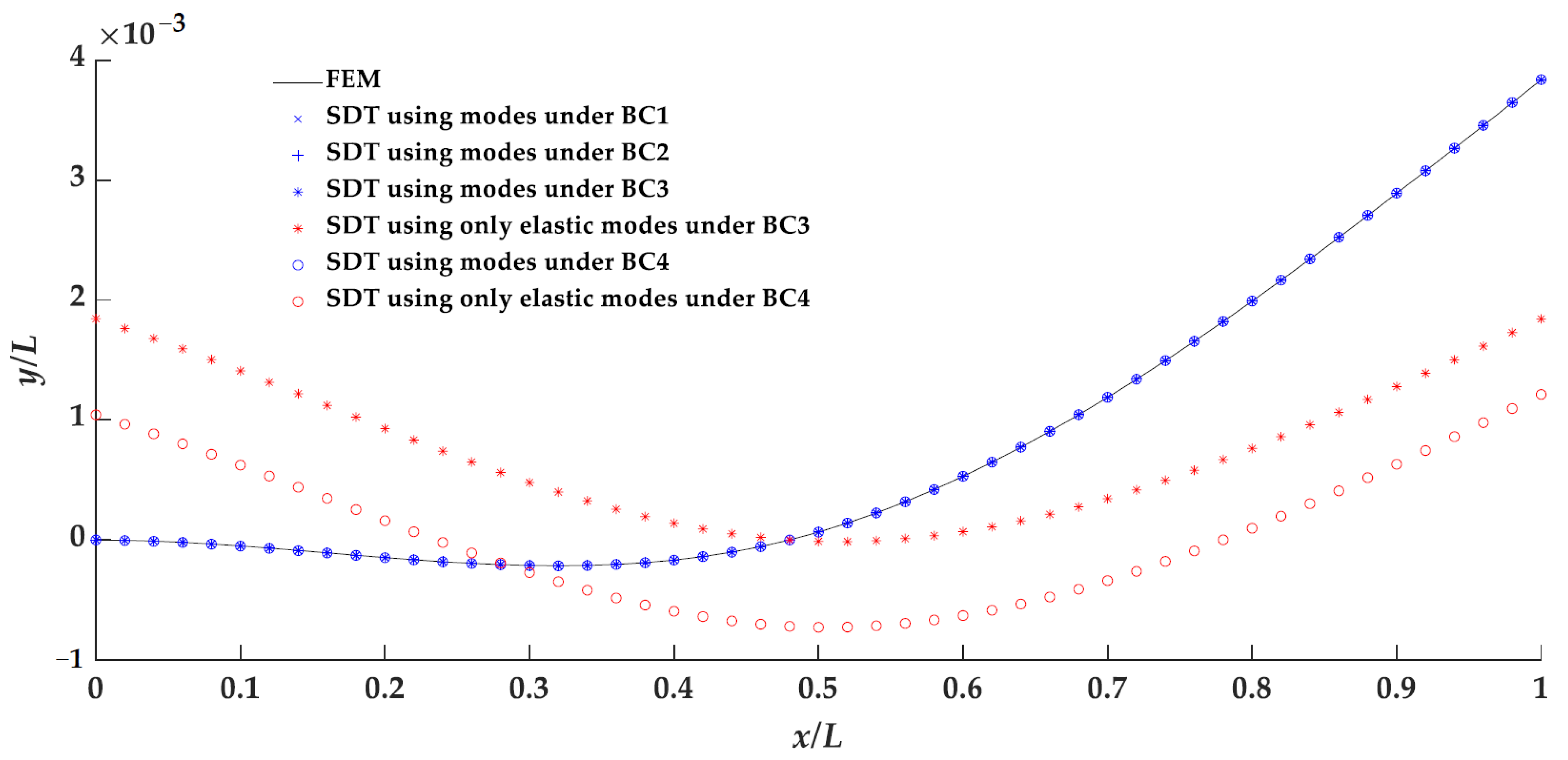
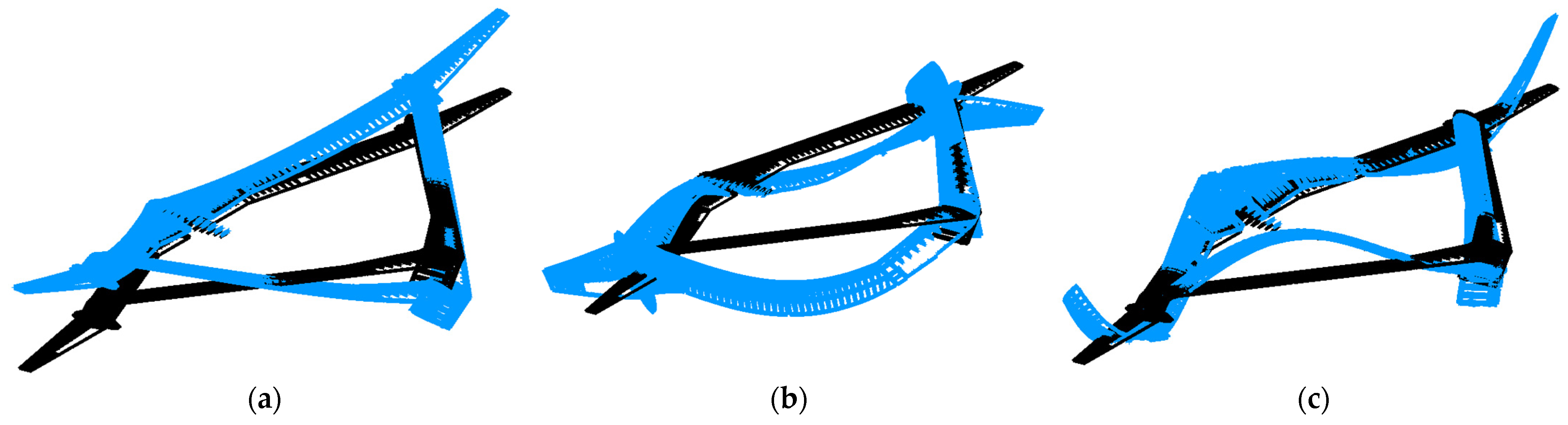
4.1. Validation on a Cantilever Beam Model
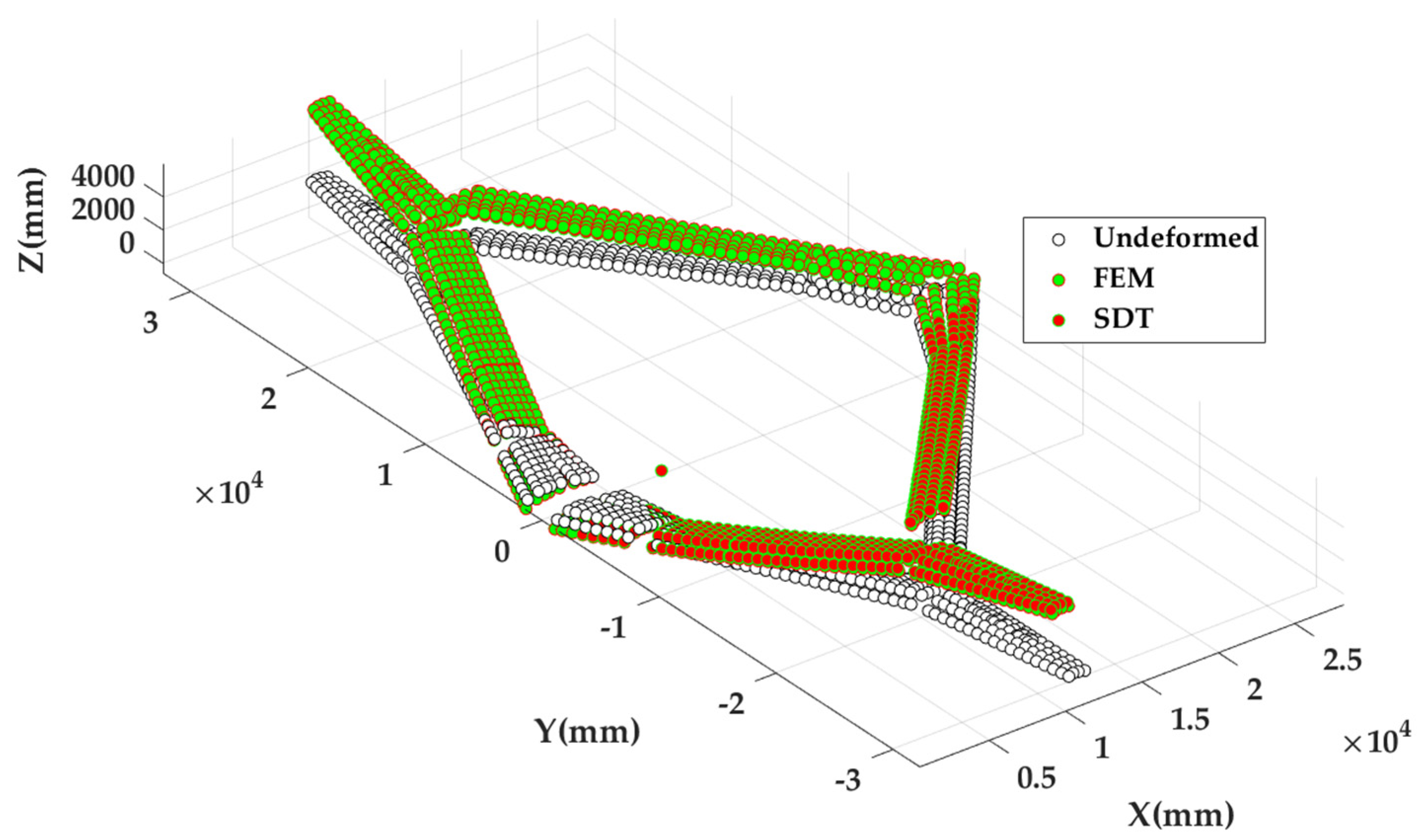
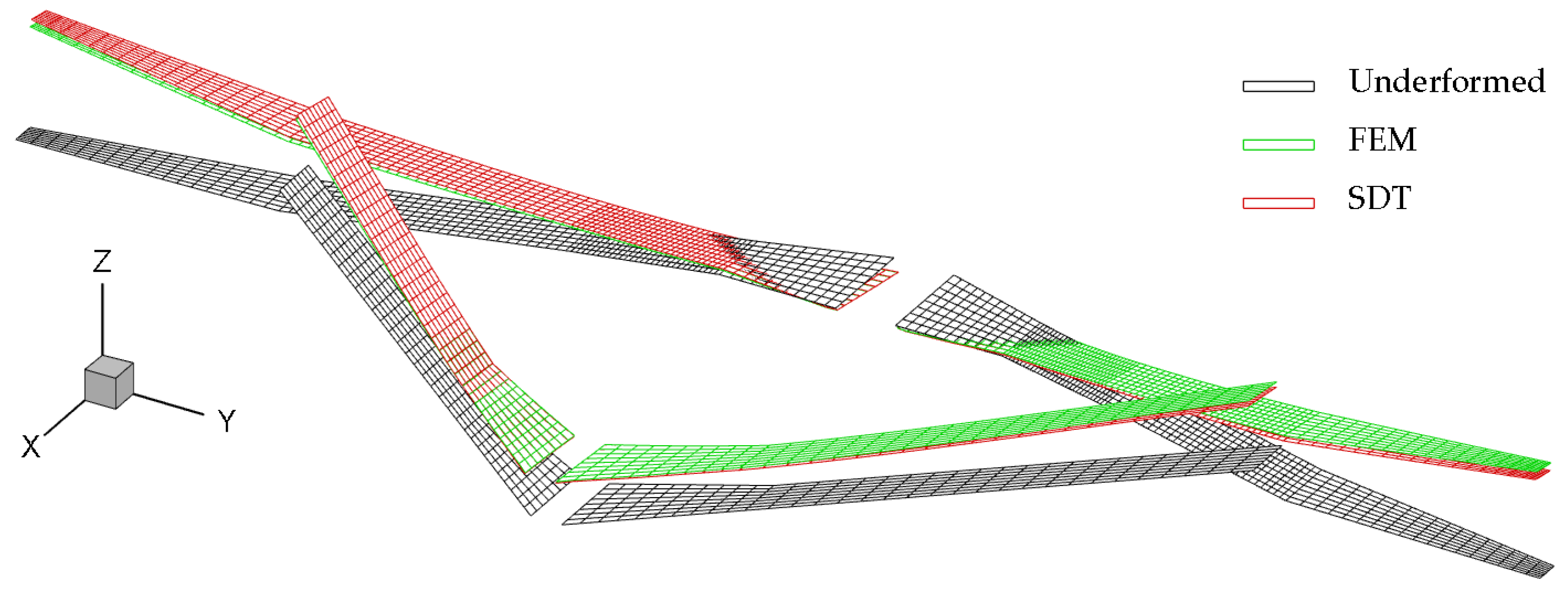
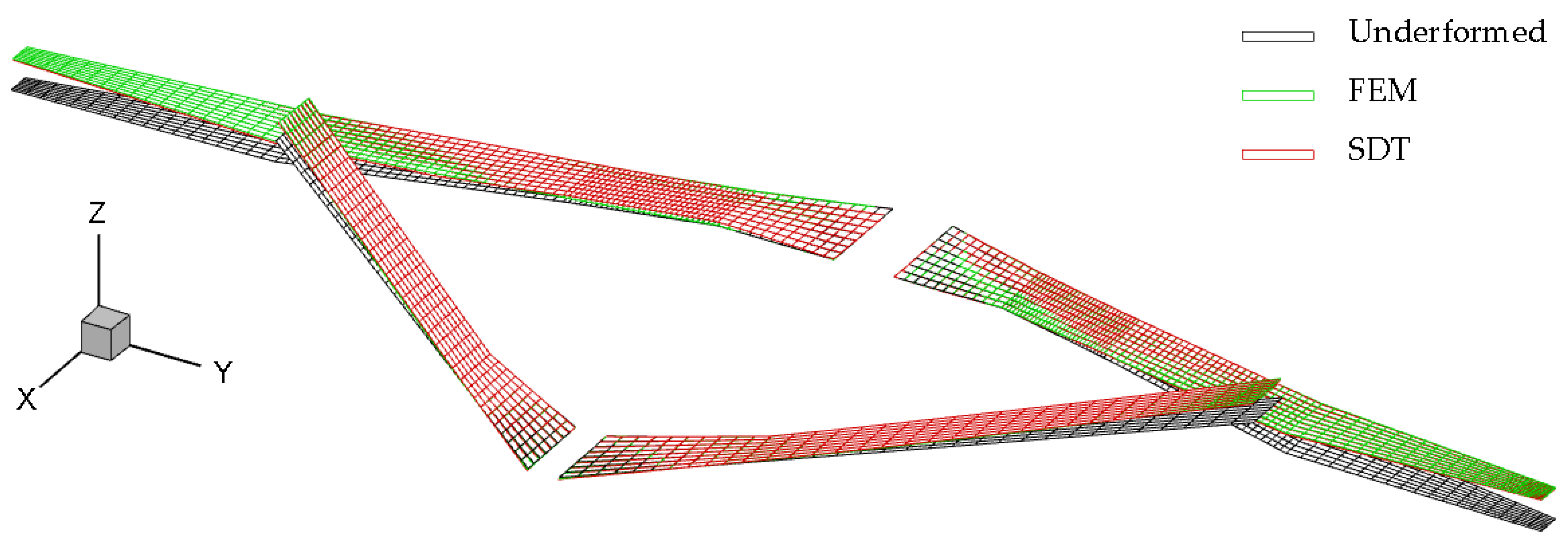
4.2. Numerical Studies on the Joined-Wing Aircraft
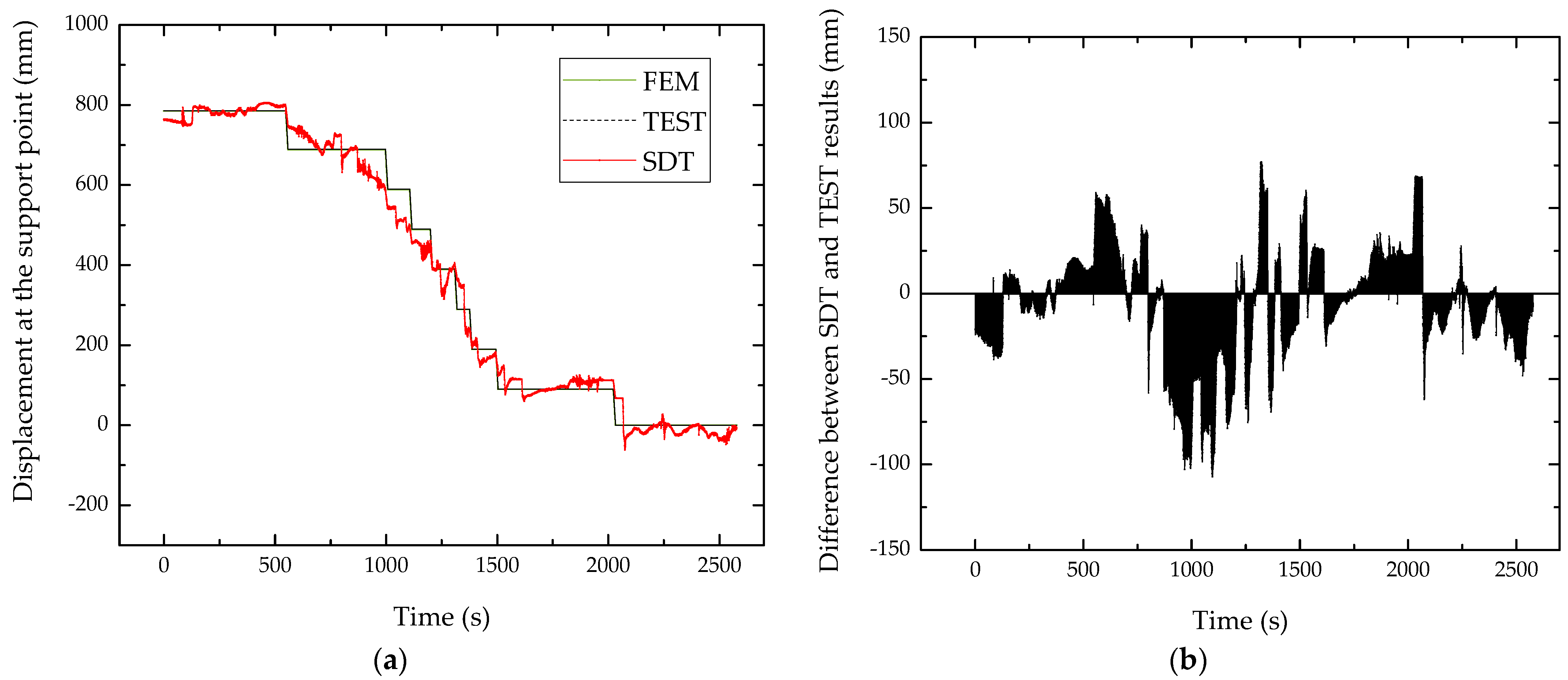
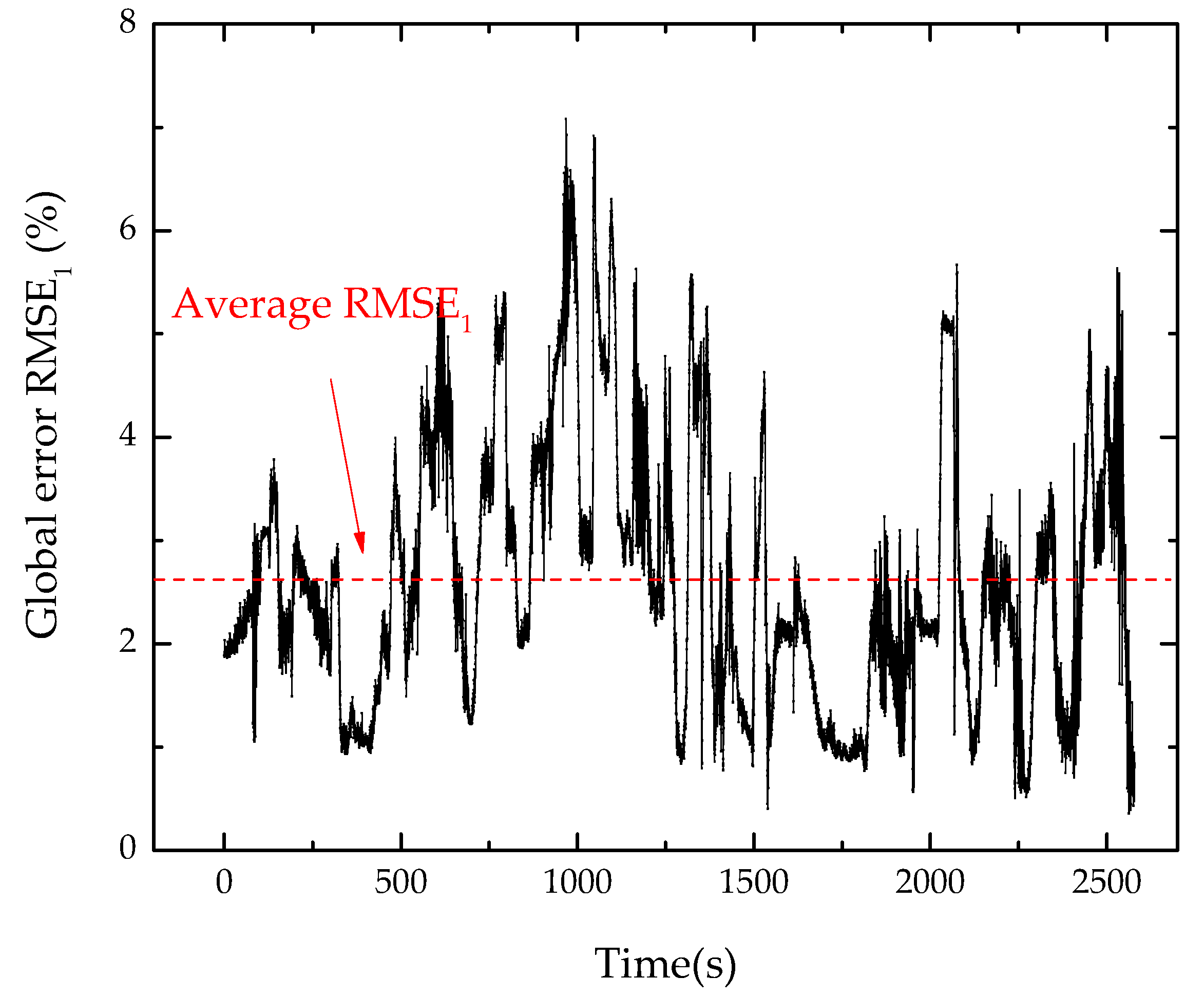
4.3. Ground Test on the Joined-Wing Aircraft
5. Conclusions
- (1)
- A FOSS with hardware and software subsystems is designed and installed on the target Joined-Wing aircraft. The system was then verified by the ground test.
- (2)
- The classical modal method is modified to adapt to various boundary conditions, which is common in practical applications. The improved SDT algorithm was then verified by numerical studies on a cantilever beam model and the Joined-Wing aircraft.
- (3)
- Both the numerical and experimental results show that the proposed SDT algorithm can accurately predict the overall configuration of the aircraft or deformations of a particular point. In the ground test, the relative error of the displacement at the support point is 6.6%. The global error of the overall deformation is less than 7%, and the average error is only 2.62%.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Mode Shapes of the Cantilever Beam Model with Different Boundary Conditions
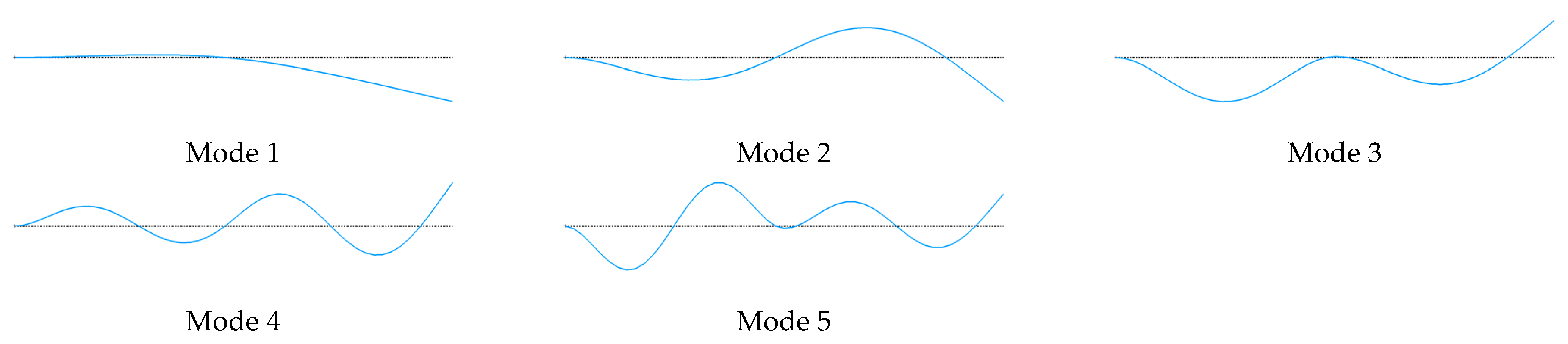
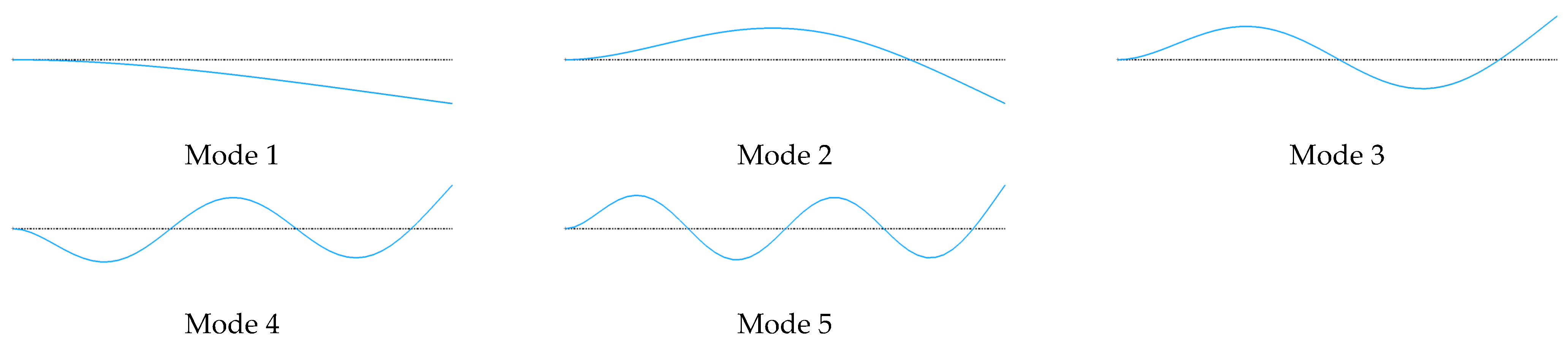
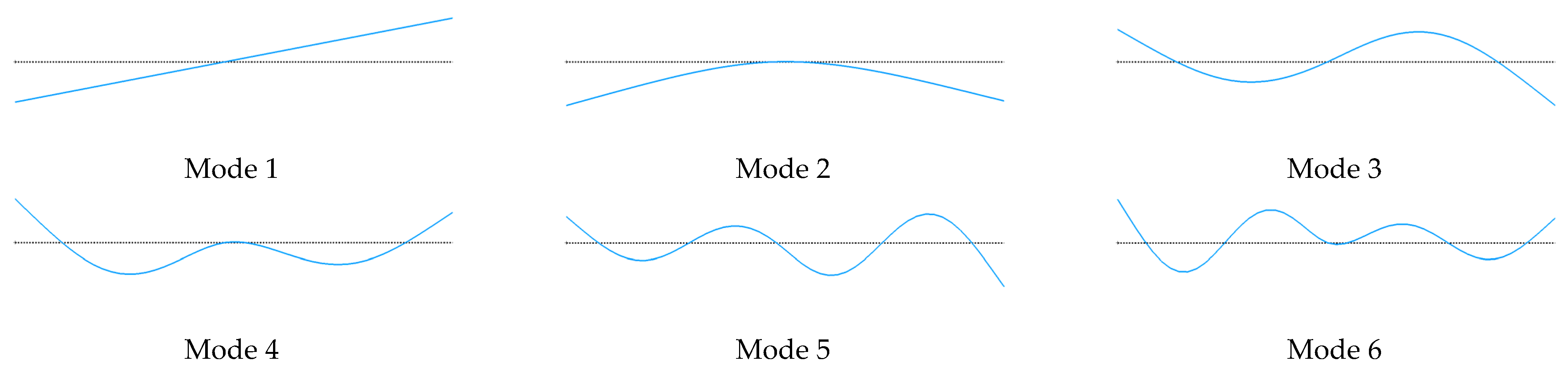

References
- Cavallaro, R.; Demasi, L. Challenges, Ideas, and Innovations of Joined-Wing Configurations: A Concept from the Past, an Opportunity for the Future. Prog. Aerosp. Sci. 2016, 87, 1–93. [Google Scholar] [CrossRef]
- Tilmann, C.P.; Flick, P.M.; Martin, C.A.; Love, M.H. High-Altitude Long Endurance Technologies for SensorCraft. In Proceedings of the RTO Paper MP-104-P-26, RTO AVT Symposium on Novel and Emerging Vehicle and Vehicle Technology Concepts, Brussels, Belgium, 7–11 April 2003. [Google Scholar]
- Su, W. Coupled Nonlinear Aeroelasticity and Flight Dynamics of Fully Flexible Aircraft; University of Michigan: Ann Arbor, MI, USA, 2008. [Google Scholar]
- Cavallaro, R.; Iannelli, A.; Demasi, L.; Razon, A.M. Phenomenology of nonlinear aeroelastic responses of highly deformable joined wings. Adv. Aircr. Spacecr. Sci. 2015, 2, 125–168. [Google Scholar] [CrossRef]
- Ma, Z.; Chen, X. Fiber Bragg Gratings Sensors for Aircraft Wing Shape Measurement: Recent Applications and Technical Analysis. Sensors 2018, 19, 55. [Google Scholar] [CrossRef] [PubMed]
- Floris, I.; Adam, J.M.; Calderón, P.A.; Sales, S. Fiber Optic Shape Sensors: A comprehensive review. Opt. Lasers Eng. 2021, 139, 106508. [Google Scholar] [CrossRef]
- Masoudi, A.; Newson, T.P. Contributed Review: Distributed optical fibre dynamic strain sensing. Rev. Sci. Instrum. 2016, 87, 011501. [Google Scholar] [CrossRef] [PubMed]
- Noll, T.E.; Brown, J.M.; Perez-Davis, M.E.; Ishmael, S.D.; Tiffany, G.C.; Gaier, M. Investigation of the Helios Prototype Aircraft Mishap; Volume I Mishap Report; NASA: Washington, DC, USA, 2004. [Google Scholar]
- Ko, W.L.; Richards, W.L.; Tran, V.T. Displacement Theories for In-Flight Deformed Shape Predictions of Aerospace Structures; NASA Dryden Flight Research Center: Edwards, CA, USA, 2007. [Google Scholar]
- Ko, W.L.; Fleischer, V.T. Further Development of Ko Displacement Theory for Deformed Shape Predictions of Nonuniform Aerospace Structures; NASA Dryden Flight Research Center: Edwards, CA, USA, 2009. [Google Scholar]
- Derkevorkian, A.; Masri, S.F.; Alvarenga, J.; Boussalis, H.; Bakalyar, J.; Richards, W.L. Strain-Based Deformation Shape-Estimation Algorithm for Control and Monitoring Applications. AIAA J. 2013, 51, 2231–2240. [Google Scholar] [CrossRef]
- KO, W.L.; Richards, W.L.; Fleischer, V.T. Applications of KO Displacement Theory to the Deformed Shape Predictions of the Doubly-Tapered Ikhana Wing; NASA Dryden Flight Research Center: Edwards, CA, USA, 2009. [Google Scholar]
- Nicolas, M.J.; Sullivan, R.W.; Richards, W.L. Large Scale Applications Using FBG Sensors: Determination of In-Flight Loads and Shape of a Composite Aircraft Wing. Aerospace 2016, 3, 18. [Google Scholar] [CrossRef]
- Klotz, T.; Pothier, R.; Walch, D.; Colombo, T. Prediction of the business jet Global 7500 wing deformed shape using fiber Bragg gratings and neural network. Results Eng. 2020, 9, 100190. [Google Scholar] [CrossRef]
- Meng, Y.; Xie, C.C.; Wan, Z.Q. Deformed Wing Shape Prediction using Fiber Optic Strain Data. In Proceedings of the International Forum on Aeroelasticity and Structural Dynamics, Como, Italy, 25–28 June 2017. [Google Scholar]
- Tessler, A.; Spangler, J.L. A least-squares variational method for full-field reconstruction of elastic deformations in shear-deformable plates and shells. Comput. Methods Appl. Mech. Eng. 2005, 194, 327–339. [Google Scholar] [CrossRef]
- Tessler, A. Structural Health Monitoring Using High-Density Fiber Optic Strain Sensor and Inverse Finite Element Methods; NASA Langley Research Center TM-214871: Hampton, VA, USA, 2007. [Google Scholar]
- Gherlone, M.; Cerracchio, P.; Mattone, M.; Di Sciuva, M.; Tessler, A. Shape sensing of 3D frame structures using an inverse Finite Element Method. Int. J. Solids Struct. 2012, 49, 3100–3112. [Google Scholar] [CrossRef]
- Gherlone, M.; Cerracchio, P.; Mattone, M.; Di Sciuva, M.; Tessler, A. An inverse finite element method for beam shape sensing: Theoretical framework and experimental validation. Smart Mater. Struct. 2014, 23, 045027. [Google Scholar] [CrossRef]
- Tessler, A.; Roy, R.; Esposito, M.; Surace, C.; Gherlone, M. Shape Sensing of Plate and Shell Structures Undergoing Large Displacements Using the Inverse Finite Element Method. Shock Vib. 2018, 2018, 8076085. [Google Scholar] [CrossRef]
- Gherlone, M.; Cerracchio, P.; Mattone, M. Shape sensing methods: Review and experimental comparison on a wing-shaped plate. Prog. Aerosp. Sci. 2018, 99, 14–26. [Google Scholar] [CrossRef]
- Esposito, M.; Gherlone, M. Composite wing box deformed-shape reconstruction based on measured strains: Optimization and comparison of existing approaches. Aerosp. Sci. Technol. 2020, 99, 105758. [Google Scholar] [CrossRef]
- Foss, G.C.; Haugse, E.D. Using Modal Test Results to Develop Strain to Displacement Transformations. In SPIE The International Society for Optical Engineering; SPIE: Bellingham, WA, USA, 1995; p. 112. [Google Scholar]
- A Davis, M.; Kersey, A.D.; Sirkis, J.S.; Friebele, E.J. Shape and vibration mode sensing using a fiber optic Bragg grating array. Smart Mater. Struct. 1996, 5, 759–765. [Google Scholar] [CrossRef]
- Kang, L.-H.; Kim, D.-K.; Han, J.-H. Estimation of dynamic structural displacements using fiber Bragg grating strain sensors. J. Sound Vib. 2007, 305, 534–542. [Google Scholar] [CrossRef]
- Kim, H.; Han, J.; Bang, H. Real-time deformed shape estimation of a wind turbine blade using distributed fiber Bragg grating sensors. Wind. Energy 2014, 17, 1455–1467. [Google Scholar] [CrossRef]
- Freydin, M.; Rattner, M.K.; Raveh, D.E.; Kressel, I.; Davidi, R.; Tur, M. Fiber-Optics-Based Aeroelastic Shape Sensing. AIAA J. 2019, 57, 5094–5103. [Google Scholar] [CrossRef]
- Martins, B.L.; Kosmatka, J.B. Health Monitoring of Aerospace Structures via Dynamic Strain Measurements: An Experimental Demonstration. In Proceedings of the AIAA Scitech 2020 Forum, Orlando, FL, USA, 6–10 January 2020. [Google Scholar] [CrossRef]
- Chang, C.X.; Chao, Y.; Xie, C.; Yang, C. Surface Splines Generalization and Large Deflection Interpolation. J. Aircr. 2007, 44, 1024–1026. [Google Scholar] [CrossRef]





| Parameter | Value |
|---|---|
| 3 dB bandwidth | ≤0.3 nm |
| Reflectivity | ≥90% |
| Side Lobe Suppression (SLS) | ≥15 dB |
| Grating length | 10 mm |
| Temperature sensitivity (temperature-optic coefficient) | 6.67 K−1·10−6 |
| Strain sensitivity (strain-optic coefficient) | 7.8 με−1·10−7 |
| Time (s) | Operation (H-Hold/D-Drop) | |
|---|---|---|
| 0 | 785 | H |
| 265 | 785 | D |
| 275 | 690 | H |
| 375 | 690 | D |
| 385 | 590 | H |
| 468 | 590 | D |
| 478 | 490 | H |
| 578 | 490 | D |
| 588 | 390 | H |
| 645 | 390 | D |
| 655 | 290 | H |
| 763 | 290 | D |
| 773 | 190 | H |
| 1294 | 190 | D |
| 1304 | 90 | H |
| 1854 | 90 | D |
| 1864 | 0 | H |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Meng, Y.; Bi, Y.; Xie, C.; Chen, Z.; Yang, C. Application of Fiber Optic Sensing System for Predicting Structural Displacement of a Joined-Wing Aircraft. Aerospace 2022, 9, 661. https://doi.org/10.3390/aerospace9110661
Meng Y, Bi Y, Xie C, Chen Z, Yang C. Application of Fiber Optic Sensing System for Predicting Structural Displacement of a Joined-Wing Aircraft. Aerospace. 2022; 9(11):661. https://doi.org/10.3390/aerospace9110661
Chicago/Turabian StyleMeng, Yang, Ying Bi, Changchuan Xie, Zhiying Chen, and Chao Yang. 2022. "Application of Fiber Optic Sensing System for Predicting Structural Displacement of a Joined-Wing Aircraft" Aerospace 9, no. 11: 661. https://doi.org/10.3390/aerospace9110661
APA StyleMeng, Y., Bi, Y., Xie, C., Chen, Z., & Yang, C. (2022). Application of Fiber Optic Sensing System for Predicting Structural Displacement of a Joined-Wing Aircraft. Aerospace, 9(11), 661. https://doi.org/10.3390/aerospace9110661






