Hopf Bifurcation Analysis of the Combustion Instability in a Liquid Rocket Engine
Abstract
1. Introduction
2. Simulation Models
2.1. Governing Equations and the Model of Flame Describing Function
2.2. Rijke Tube Model Combustor
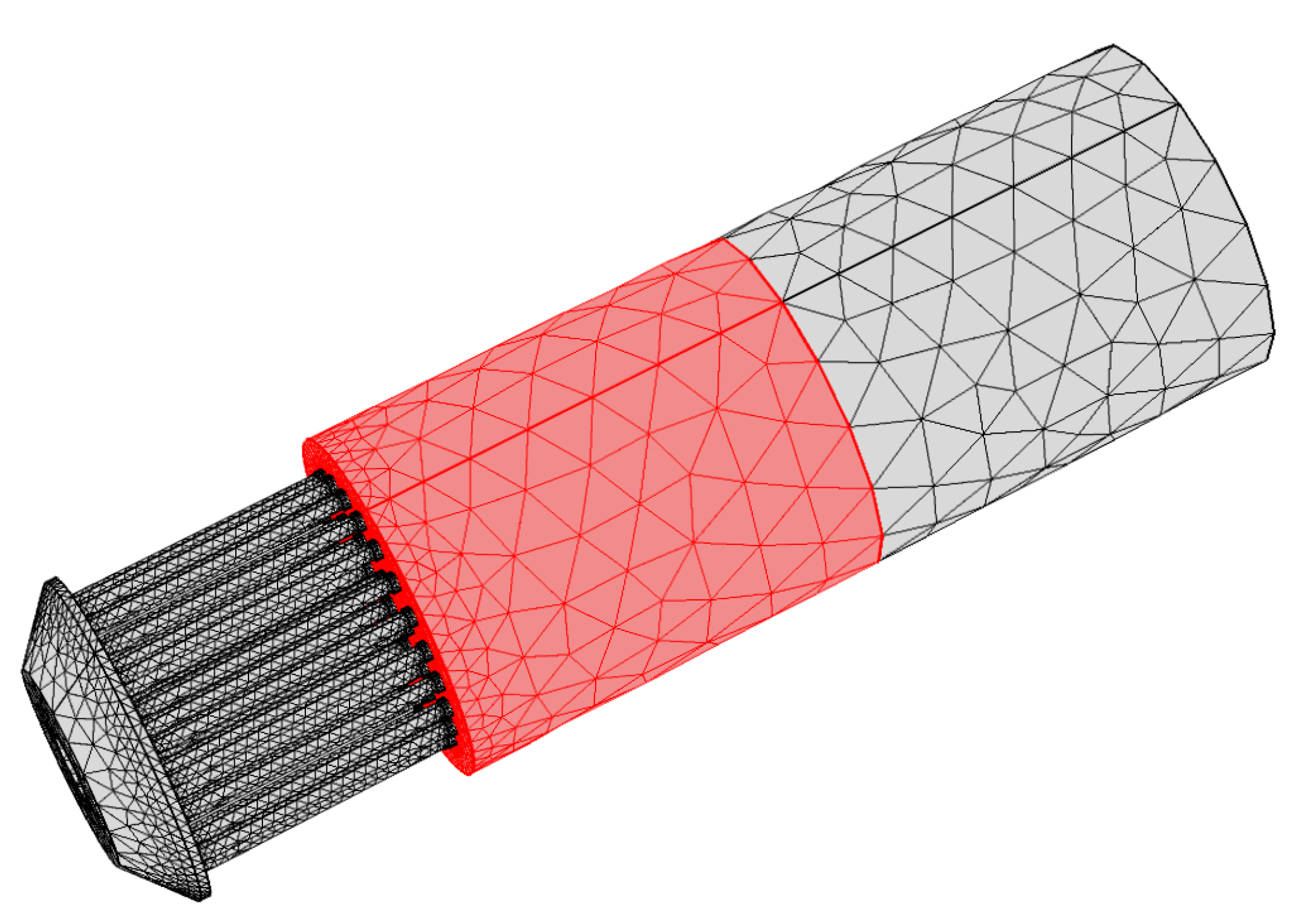
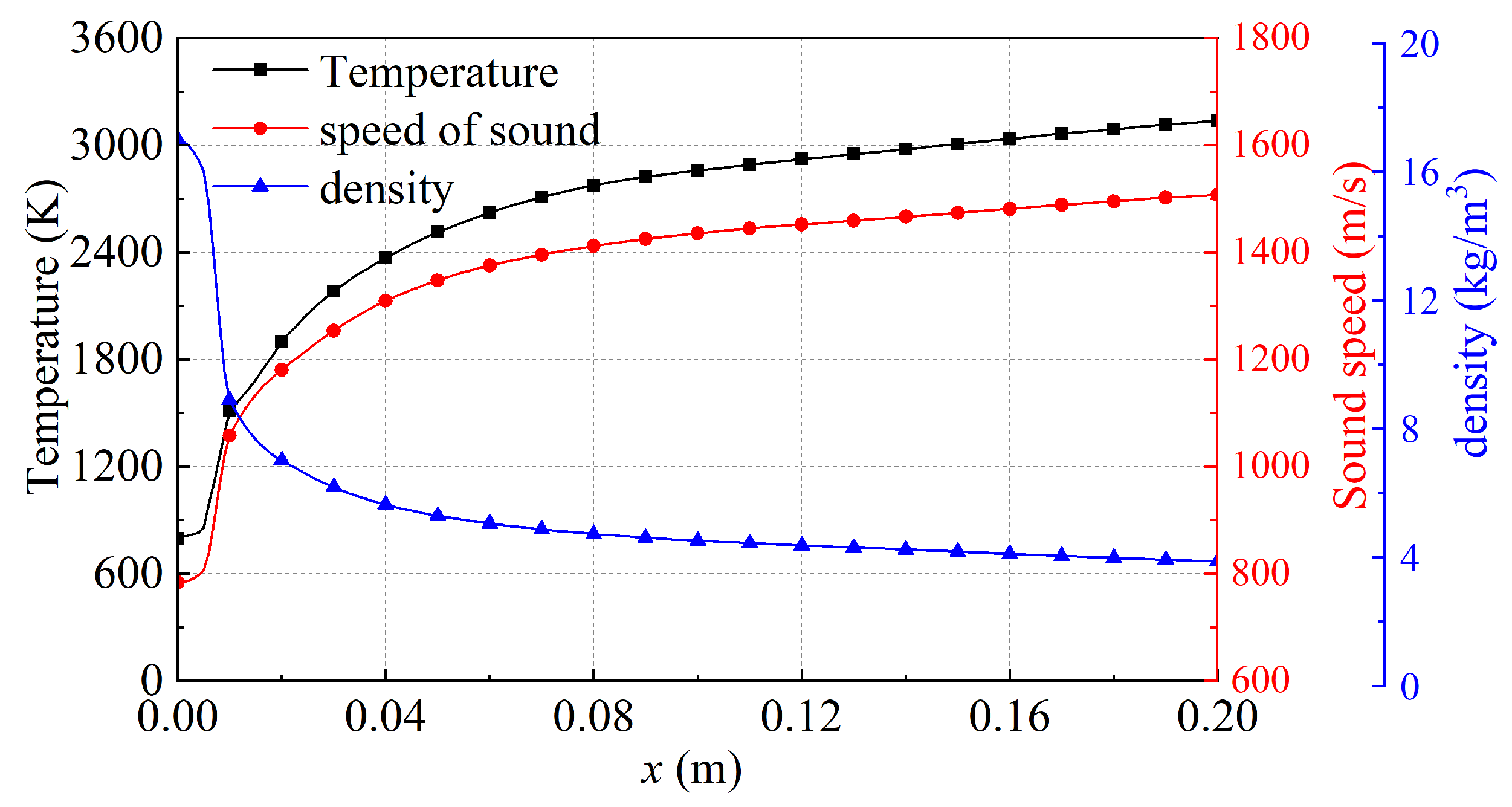
2.3. BKD Combustor Model
2.4. Models of Flame Distribution in the BKD Combustor
3. Nonlinear Analysis
4. Results and Discussion
4.1. Rijke Tube
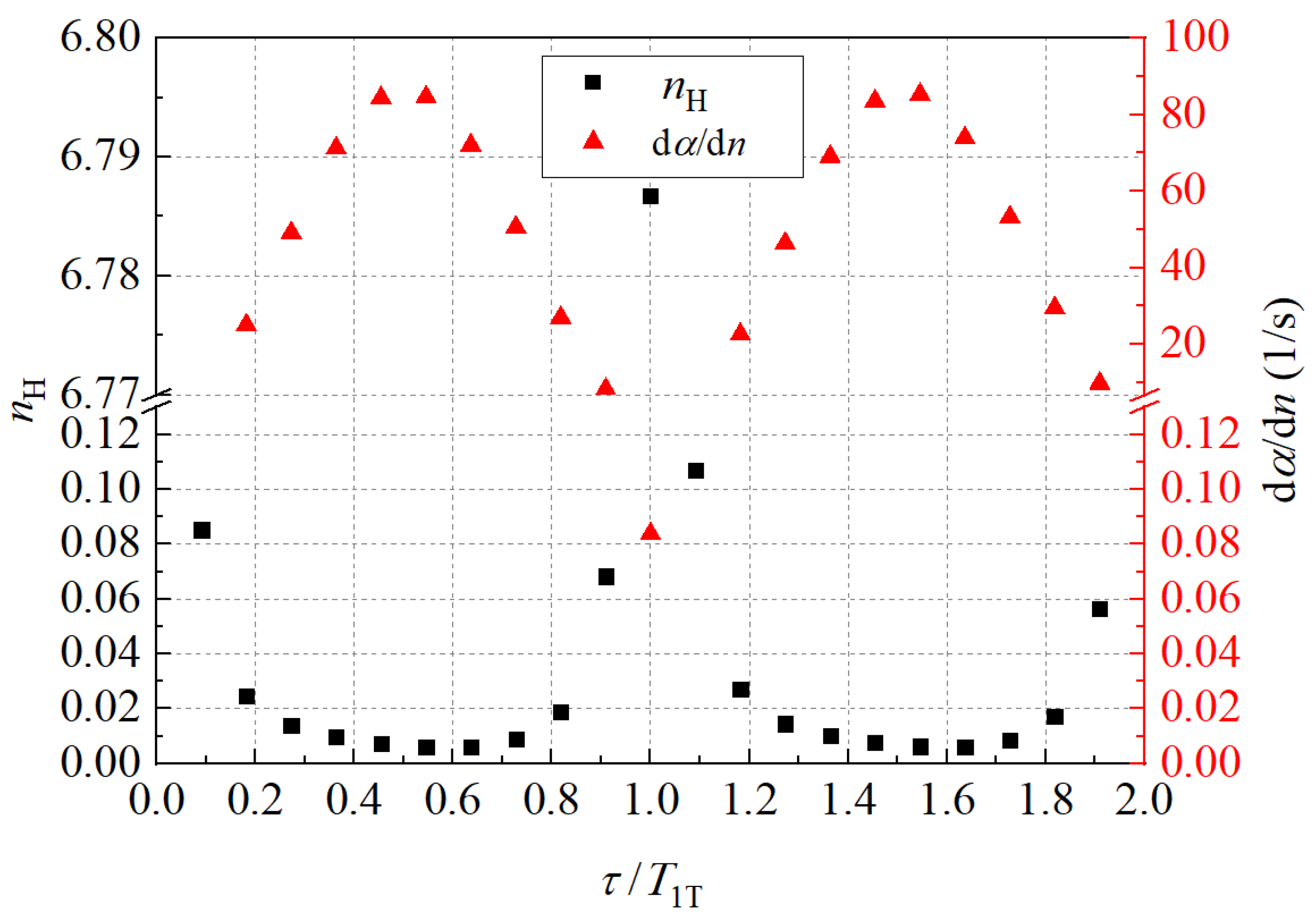
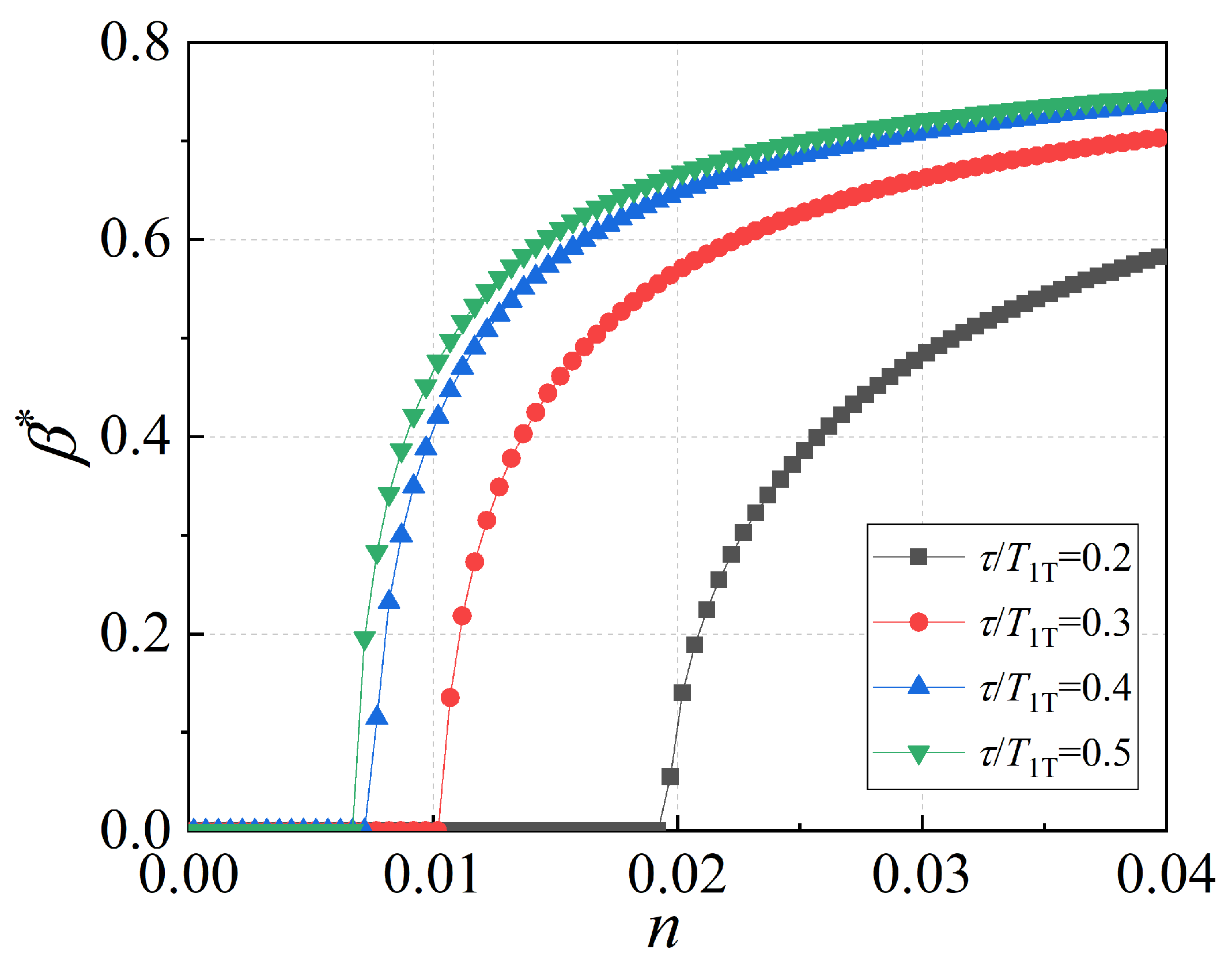
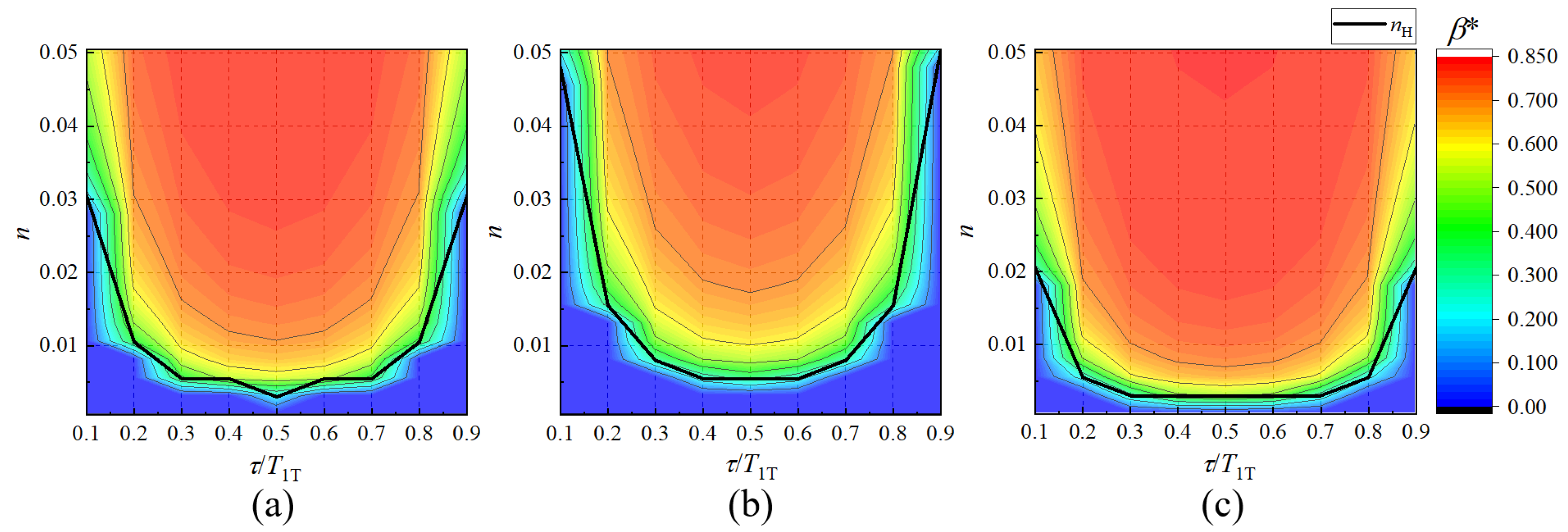
4.2. BKD Combustor with Uniform Flame 0 Distribution along the Radius
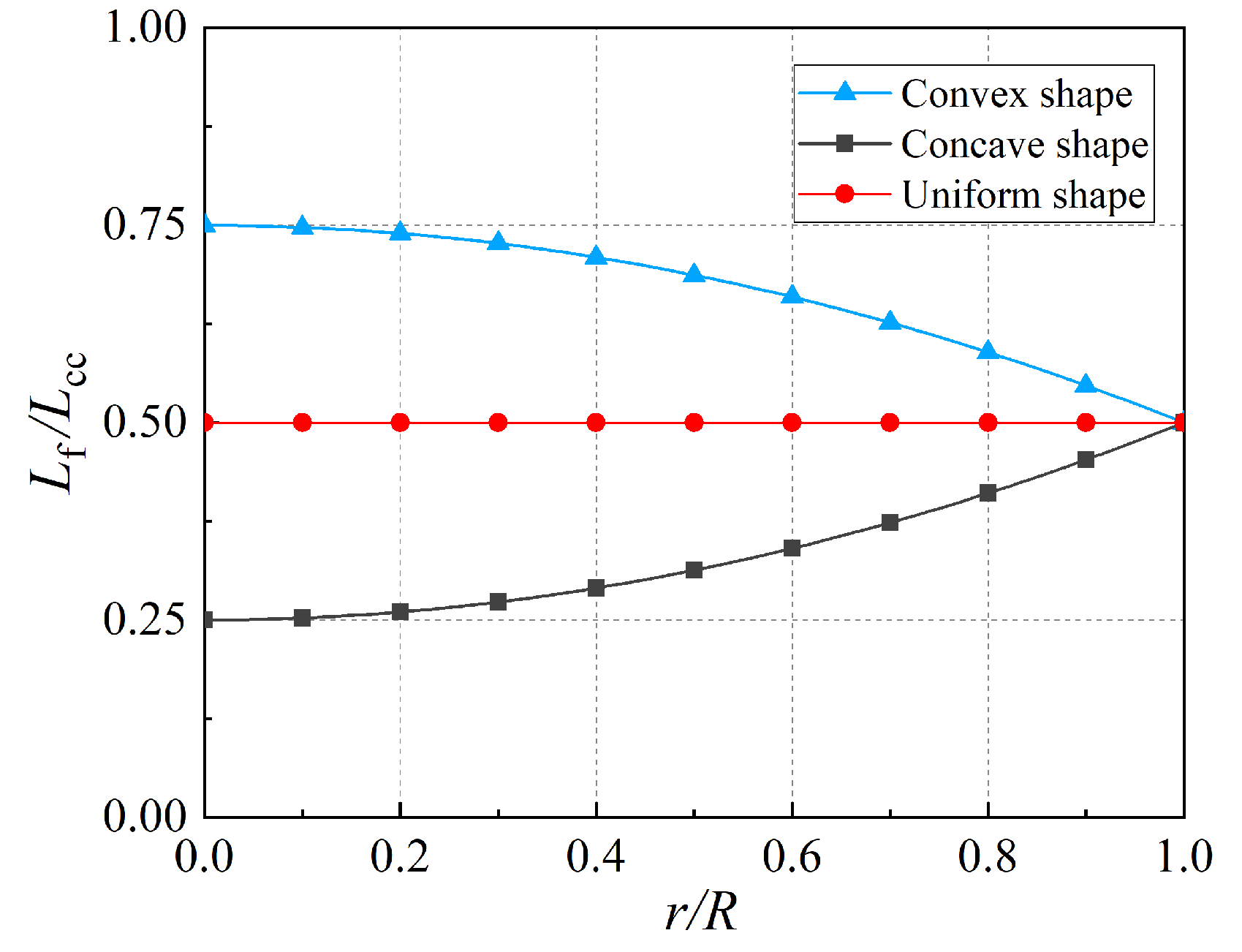
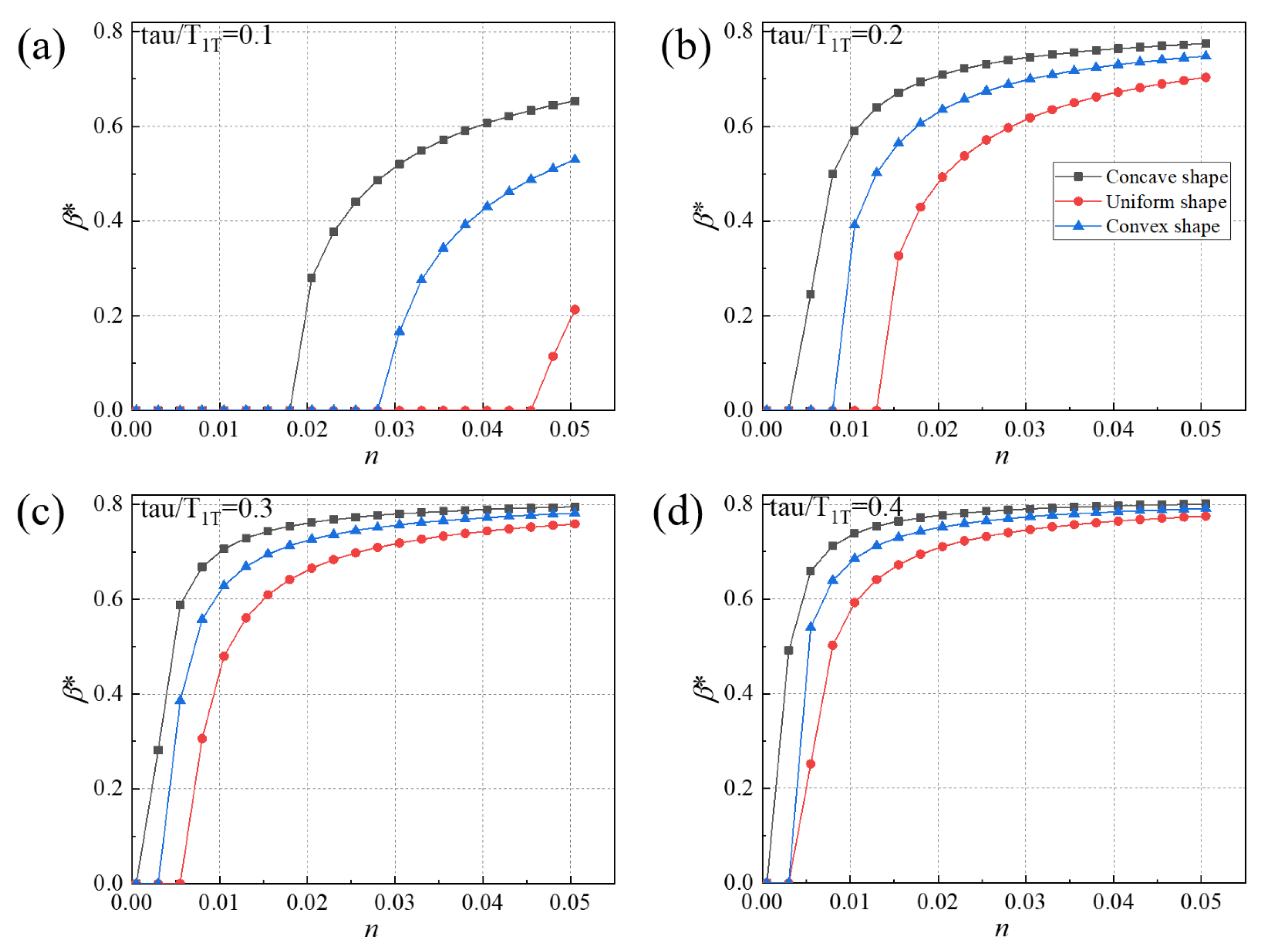
4.3. Effects of Flame 0 Distribution on the Bifurcation Diagram of the BKD Combustor
4.4. Bistable System
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| FDF | Flame describing function |
| CI | Combustion instability |
| RANS | Reynolds average Navier-Stokes |
| LRE | Liquid Rocket engine |
References
- Eckstein, J.; Sattelmayer, T. Low-Order Modeling of Low-Frequency Combustion Instabilities in AeroEngines. J. Propul. Power 2006, 22, 425–432. [Google Scholar] [CrossRef]
- Oefelein, J.C.; Yang, V. Comprehensive review of liquid-propellant combustion instabilities in F-1 engines. J. Propul. Power 1993, 9, 657–677. [Google Scholar] [CrossRef]
- Pieringer, J.; Sattelmayer, T.; Fassl, F. Simulation of Combustion Instabilities in Liquid Rocket Engines with Acoustic Perturbation Equations. J. Propul. Power 2009, 25, 1020–1031. [Google Scholar] [CrossRef]
- Lieuwen, T.C.; Yang, V. Combustion Instabilities in Gas Turbine Engines: Operational Experience, Fundamental Mechanisms, and Modeling; American Institute of Aeronautics and Astronautics: Reston, VA, USA, 2005. [Google Scholar]
- Candel, S. Combustion dynamics and control: Progress and challenges. P Combust. Inst. 2002, 29, 1–28. [Google Scholar] [CrossRef]
- Palies, P.; Schuller, T.; Durox, D.; Gicquel, L.; Candel, S. Acoustically perturbed turbulent premixed swirling flames. Phys. Fluids 2011, 23, 037101. [Google Scholar] [CrossRef]
- Zhou, S.; Nie, W.; Tian, Y. High frequency combustion instability control by discharge plasma in a model rocket engine combustor. Acta Astronaut. 2021, 179, 391–406. [Google Scholar] [CrossRef]
- Sujith, R.I.; Unni, V.R. Complex system approach to investigate and mitigate thermoacoustic instability in turbulent combustors. Phys. Fluids 2020, 32, 061401. [Google Scholar] [CrossRef]
- Richecoeur, F.; Ducruix, S.; Scouflaire, P.; Candel, S. Experimental investigation of high-frequency combustion instabilities in liquid rocket engine. Acta Astronaut. 2008, 62, 18–27. [Google Scholar] [CrossRef]
- Rijke, P.L. Notiz über eine neue Art, die in einer an beiden Enden offenen Röhre enthaltene Luft in Schwingungen zu versetzen. Ann. Der Phys. Und Chem. 1859, 183, 339–343. [Google Scholar] [CrossRef]
- Dowling, A.P. The calculation of thermoacoustic oscillations. J. Sound Vib. 1995, 180, 557–581. [Google Scholar] [CrossRef]
- Li, J.; Morgans, A.S. Time domain simulations of nonlinear thermoacoustic behaviour in a simple combustor using a wave-based approach. J. Sound Vib. 2015, 346, 345–360. [Google Scholar] [CrossRef]
- Juniper, M.P.; Sujith, R. Sensitivity and Nonlinearity of Thermoacoustic Oscillations. Annu. Rev. Fluid. Mech. 2018, 50, 661–689. [Google Scholar] [CrossRef]
- Dowling, A.P.; Stow, S.R. Acoustic Analysis of Gas Turbine Combustors. J. Propul. Power 2003, 19, 751–764. [Google Scholar] [CrossRef]
- Li, J.; Yang, D.; Luzzato, C.; Morgans, A.S. OSCILOS: The Open Sounce Combustion Instability Low Order Simulator Technical Report; Technical Report; Imperial College: London, UK, 2015. [Google Scholar]
- Balasubramanian, K.; Sujith, R.I. Thermoacoustic instability in a rijke tube: Non-normality and nonlinearity. Phys. Fluids 2008, 20, 044103. [Google Scholar] [CrossRef]
- Etikyala, S.; Sujith, R. Change of criticality in a prototypical thermoacoustic system. Chaos Interdiscip. J. Nonlinear Sci. 2017, 27, 023106. [Google Scholar] [CrossRef] [PubMed]
- Xi, Y.; Li, X.; Wang, Y.; Xu, B.; Wang, N.; Zhao, D. Experimental study of transition to instability in a Rijke tube with axially distributed heat source. Int. J. Heat Mass. Transf. 2022, 183, 122157. [Google Scholar] [CrossRef]
- Li, S.; Li, Q.; Tang, L.; Yang, B.; Fu, J.; Clarke, C.; Jin, X.; Ji, C.; Zhao, H. Theoretical and experimental demonstration of minimizing self-excited thermoacoustic oscillations by applying anti-sound technique. Appl. Energ. 2016, 181, 399–407. [Google Scholar] [CrossRef]
- Wu, G.; Lu, Z.; Pan, W.; Guan, Y.; Li, S.; Ji, C. Experimental demonstration of mitigating self-excited combustion oscillations using an electrical heater. Appl. Energ. 2019, 239, 331–342. [Google Scholar] [CrossRef]
- Mariappan, S.; Sujith, R.I.; Schmid, P.J. Non-normality of thermoacoustic interactions: An experimental investigation. In Proceedings of the 47th AIAA/ASME/SAE/ASEE Joint Propulsion Conference and Exhibit, San Diego, CA, USA, 31 July–3 August 2011. Number AIAA-2011-5555. [Google Scholar]
- Armbruster, W.; Hardi, J.S.; Suslov, D.; Oschwald, M. Experimental investigation of self-excited combustion instabilities with injection coupling in a cryogenic rocket combustor. Acta Astronaut. 2018, 151, 655–667. [Google Scholar] [CrossRef]
- Armbruster, W.; Hardi, J.S.; Miene, Y.; Suslov, D.; Oschwald, M. Damping device to reduce the risk of injection-coupled combustion instabilities in liquid propellant rocket engines. Acta Astronaut. 2020, 169, 170–179. [Google Scholar] [CrossRef]
- Hardi, J.S.; Traudt, T.; Bombardieri, C.; Börner, M.; Beinke, S.K.; Armbruster, W.; Nicolas Blanco, P.; Tonti, F.; Suslov, D.; Dally, B.; et al. Combustion dynamics in cryogenic rocket engines: Research programme at DLR Lampoldshausen. Acta Astronaut. 2018, 147, 251–258. [Google Scholar] [CrossRef]
- Schulze, M.; Urbano, A.; Zahn, M.; Schmid, M.; Sattelmayer, T.; Oschwald, M. Thermoacoustic feedback analysis of a cylindrical combustion chamber under supercritical conditions. In Proceedings of the 50th AIAA/ASME/SAE/ASEE Joint Propulsion Conference, Cleveland, OH, USA, 28–30 July 2014; p. 3776. [Google Scholar]
- Gröning, S.; Suslov, D.; Hardi, J.; Oschwald, M. Influence of hydrogen temperature on the acoustics of a rocket engine combustion chamber operated with LOX/H2 at representative conditions. In Proceedings of the Space Propulsion, Cologne, Germany, 19–22 May 2014. [Google Scholar]
- Urbano, A.; Selle, L.; Staffelbach, G.; Cuenot, B.; Schmitt, T.; Ducruix, S.; Candel, S. Exploration of combustion instability triggering using Large Eddy Simulation of a multiple injector liquid rocket engine. Combust. Flame 2016, 169, 129–140. [Google Scholar] [CrossRef]
- Urbano, A.; Douasbin, Q.; Selle, L.; Staffelbach, G.; Cuenot, B.; Schmitt, T.; Ducruix, S.; Candel, S. Study of flame response to transverse acoustic modes from the LES of a 42-injector rocket engine. P Combust. Inst. 2017, 36, 2633–2639. [Google Scholar] [CrossRef]
- Waxenegger-Wilfing, G.; Sengupta, U.; Martin, J.; Armbruster, W.; Oschwald, M. Early detection of thermoacoustic instabilities in a cryogenic rocket thrust chamber using combustion noise features and machine learning. Chaos 2021, 31, 063128. [Google Scholar] [CrossRef] [PubMed]
- Crocco, L. Aspects of Combustion Stability in Liquid Propellant Rocket Motors Part I: Fundamentals. Low Frequency Instability With Monopropellants. J. Am. Rocket Soc. 1951, 21, 163–178. [Google Scholar] [CrossRef]
- Dowling, A.P. Nonlinear self-excited oscillations of a ducted flame. J. Fluid Mech. 1997, 346, 271–290. [Google Scholar] [CrossRef]
- Schuermans, B.; Guethe, F.; Pennell, D.; Guyot, D.; Paschereit, C.O. Thermoacoustic Modeling of a Gas Turbine Using Transfer Functions Measured Under Full Engine Pressure. J. Eng. Gas Turbines Power 2010, 132, 111503. [Google Scholar] [CrossRef]
- Schuller, T.; Poinsot, T.; Candel, S. Dynamics and control of premixed combustion systems based on flame transfer and describing functions. J. Fluid Mech. 2020, 894, P1. [Google Scholar] [CrossRef]
- Lieuwen, T.C. Experimental investigation of limit-cycle oscillations in an unstable gas turbine combustor. J. Propul. Power 2002, 18, 61–67. [Google Scholar] [CrossRef]
- Sun, Y.; Rao, Z.; Zhao, D.; Wang, B.; Sun, D.; Sun, X. Characterizing nonlinear dynamic features of self-sustained thermoacoustic oscillations in a premixed swirling combustor. Appl. Energ. 2020, 264, 114698. [Google Scholar] [CrossRef]
- Flandro, G.A.; Fischbach, S.R.; Majdalani, J. Nonlinear rocket motor stability prediction: Limit amplitude, triggering, and mean pressure shift. Phys. Fluids 2007, 19, 094101. [Google Scholar] [CrossRef]
- Moeck, J.; Bothien, M.; Schimek, S.; Lacarelle, A.; Paschereit, C. Subcritical thermoacoustic instabilities in a premixed combustor. In Proceedings of the 14th AIAA/CEAS Aeroacoustics Conference (29th AIAA Aeroacoustics Conference), Vancouver, BC, Canada, 5–7 May 2008; American Institute of Aeronautics and Astronautics: Reston, VA, USA, 2008. [Google Scholar]
- Mariappan, S.; Sujith, R.I. Modelling nonlinear thermoacoustic instability in an electrically heated Rijke tube. J. Fluid Mech. 2011, 680, 511–533. [Google Scholar] [CrossRef]
- Wang, Z.; Liu, P.; Jin, B.; Ao, W. Nonlinear characteristics of the triggering combustion instabilities in solid rocket motors. Acta Astronaut. 2020, 176, 371–382. [Google Scholar] [CrossRef]
- Dowling, A.P. A kinematic model of a ducted flame. J. Fluid Mech. 1999, 394, 51–72. [Google Scholar] [CrossRef]
- Schuller, T.; Durox, D.; Candel, S. A unified model for the prediction of laminar flame transfer functions: Comparisons between conical and V-flame dynamics. Combust. Flame 2003, 134, 21–34. [Google Scholar] [CrossRef]
- Polifke, W. Modeling and analysis of premixed flame dynamics by means of distributed time delays. Prog. Energ. Combust. 2020, 79, 100845. [Google Scholar] [CrossRef]
- Li, J.; Liu, T.; Yang, L. An analytical model for the transversely forced flame transfer functions of conical and V-flame dynamics. Fuel 2020, 276, 117987. [Google Scholar] [CrossRef]
- Jiang, X.; Yang, L.; Liu, T.; Li, J. Nonlinear Models of Laminar Premixed Slit Flame Responses Subjected to Two-Way Perturbations. AIAA J. 2022, 60, 962–975. [Google Scholar] [CrossRef]
- Gopalakrishnan, E.A.; Sujith, R.I. Effect of external noise on the hysteresis characteristics of a thermoacoustic system. J. Fluid Mech. 2015, 776, 334–353. [Google Scholar] [CrossRef]
- Kabiraj, L.; Sujith, R.I.; Wahi, P. Bifurcations of self-excited ducted laminar premixed flames. J. Eng. Gas. Turb. Power 2012, 134, 031502. [Google Scholar] [CrossRef]
- Stow, S.R.; Dowling, A.P. A Time-Domain Network Model for Nonlinear Thermoacoustic Oscillations. J. Eng. Gas. Turb. Power 2009, 131, 031502. [Google Scholar] [CrossRef]
- Sayadi, T.; Le Chenadec, V.; Schmid, P.; Richecœur, F.; Massot, M. Time-domain analysis of thermo-acoustic instabilities in a ducted flame. P Combust. Inst. 2015, 35, 1079–1086. [Google Scholar] [CrossRef]
- Noiray, N.; Bothien, M.; Schuermans, B. Investigation of azimuthal staging concepts in annular gas turbines. Combust. Theor. Model 2011, 15, 585–606. [Google Scholar] [CrossRef]
- Yang, L.; Pang, B.; Li, J. Comparison of strongly and weakly nonlinear flame models applied to thermoacoustic instability. Phys. Fluids 2021, 33, 094108. [Google Scholar] [CrossRef]
- Campa, G.; Juniper, M.P. Obtaining bifurcation diagrams with a thermoacoustic network model. In Proceedings of the Volume 2: Combustion, Fuels and Emissions, Parts A and B; American Society of Mechanical Engineers: New York, NY, USA, 2012. [Google Scholar]
- Campa, G.; Cinquepalmi, M.; Camporeale, S.M. Influence of nonlinear flame models on sustained thermoacoustic oscillations in gas turbine combustion chambers. In Proceedings of the Volume 1A: Combustion, Fuels and Emissions; American Society of Mechanical Engineers: New York, NY, USA, 2013. [Google Scholar]
- Laera, D.; Campa, G.; Camporeale, S. A finite element method for a weakly nonlinear dynamic analysis and bifurcation tracking of thermo-acoustic instability in longitudinal and annular combustors. Appl. Energ. 2017, 187, 216–227. [Google Scholar] [CrossRef]
- Nicoud, F.; Benoit, L.; Sensiau, C.; Poinsot, T. Acoustic Modes in Combustors with Complex Impedances and Multidimensional Active Flames; American Institute of Aeronautics and Astronautics (AIAA): Reston, VA, USA, 2007. [Google Scholar]
- Lin, S.C.; Bomberg, S.; Polifke, W. Propagation and Generation of Acoustic and Entropy Waves Across a Moving Flame Front. Combust. Flame 2016, 166, 170–180. [Google Scholar]
- Lehoucq, R.B.; Sorensen, D.C.; Yang, C. ARPACK Users’ Guide; Society for Industrial and Applied Mathematics: Philadelphia, PA, USA, 1998. [Google Scholar]
- Anderson, W.E.; Yang, V. Liquid Rocket Engine Combustion Instability—Combustion Instability Analysis: Analytical Models for Combustion Instability; The American Institute of Aeronautics and Astronautics: Reston, VA, USA, 1995; pp. 403–430. [Google Scholar] [CrossRef]
- Noiray, N.; Durox, D.; Schuller, T.; Candel, S. A unified framework for nonlinear combustion instability analysis based on the flame describing function. J. Fluid Mech. 2008, 615, 139–167. [Google Scholar] [CrossRef]
- Gröning, S.; Hardi, J.S.; Suslov, D.; Oschwald, M. Injector-driven combustion instabilities in a hydrogen/oxygen rocket combustor. J. Propul. Power 2016, 32, 560–573. [Google Scholar] [CrossRef]
- Schulze, M.; Sattelmayer, T. Linear stability assessment of a cryogenic rocket engine. Int. J. Spray Combust. 2017, 9, 277–298. [Google Scholar] [CrossRef]
- Duran, I.; Moreau, S. Solution of the quasi-one-dimensional linearized Euler equations using flow invariants and the Magnus expansion. J. Fluid Mech. 2013, 723, 190–231. [Google Scholar] [CrossRef]
- Duran, I.; Morgans, A.S. On the reflection and transmission of circumferential waves through nozzles. J. Fluid Mech. 2015, 773, 137–153. [Google Scholar] [CrossRef]
- Duchaine, F.; Boudy, F.; Durox, D.; Poinsot, T. Sensitivity analysis of transfer functions of laminar flames. Combust. Flame 2011, 158, 2384–2394. [Google Scholar] [CrossRef]
- Hwang, W.S.; Sung, B.K.; Han, W.; Huh, K.Y.; Lee, B.J.; Han, H.S.; Sohn, C.H.; Choi, J.Y. Real-Gas-Flamelet-Model-Based Numerical Simulation and Combustion Instability Analysis of a GH2/LOX Rocket Combustor with Multiple Injectors. Energies 2021, 14, 419. [Google Scholar] [CrossRef]
- Armbruster, W.; Hardi, J.S.; Oschwald, M. Flame-acoustic response measurements in a high-pressure, 42-injector, cryogenic rocket thrust chamber. P Combust. Inst. 2021, 38, 5963–5970. [Google Scholar] [CrossRef]
- Silva, C.F.; Nicoud, F.; Schuller, T.; Durox, D.; Candel, S. Combining a Helmholtz solver with the flame describing function to assess combustion instability in a premixed swirled combustor. Combust. Flame 2013, 160, 1743–1754. [Google Scholar] [CrossRef]
- Subramanian, P.; Mariappan, S.; Sujith, R.I.; Wahi, P. Bifurcation analysis of thermoacoustic instability in a horizontal rijke tube. Int. J. Spray Combust. 2010, 2, 325–355. [Google Scholar] [CrossRef]

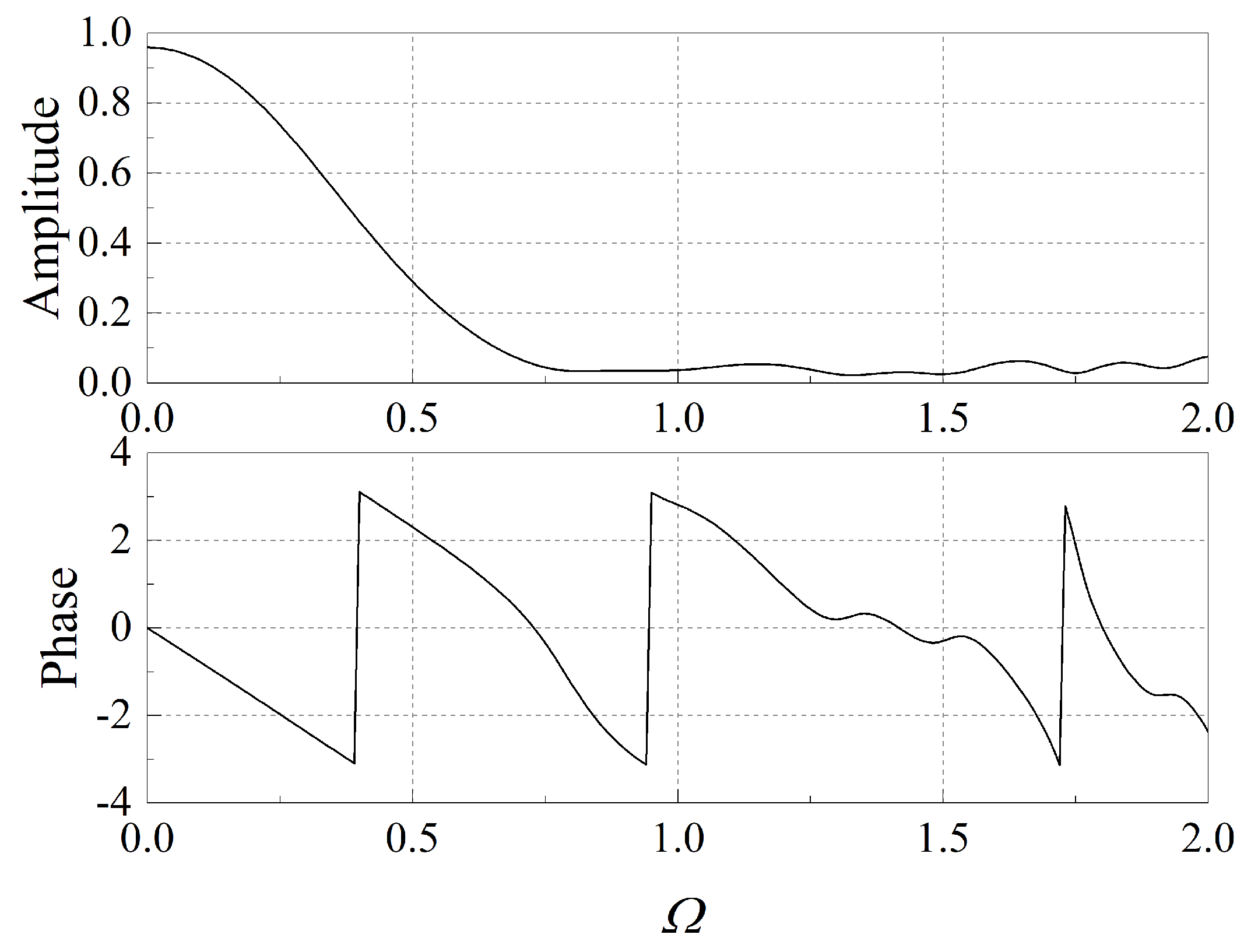




| (kg/s) | (kg/s) | ROF (-) | (m/s) | (m/s) | (bar) | |
|---|---|---|---|---|---|---|
| LP4 | 0.96 | 5.75 | 6 | 330 | 12.76 | 80.04 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liang, X.; Yang, L.; Wang, G.; Li, J. Hopf Bifurcation Analysis of the Combustion Instability in a Liquid Rocket Engine. Aerospace 2022, 9, 593. https://doi.org/10.3390/aerospace9100593
Liang X, Yang L, Wang G, Li J. Hopf Bifurcation Analysis of the Combustion Instability in a Liquid Rocket Engine. Aerospace. 2022; 9(10):593. https://doi.org/10.3390/aerospace9100593
Chicago/Turabian StyleLiang, Xuanye, Lijun Yang, Gaofeng Wang, and Jingxuan Li. 2022. "Hopf Bifurcation Analysis of the Combustion Instability in a Liquid Rocket Engine" Aerospace 9, no. 10: 593. https://doi.org/10.3390/aerospace9100593
APA StyleLiang, X., Yang, L., Wang, G., & Li, J. (2022). Hopf Bifurcation Analysis of the Combustion Instability in a Liquid Rocket Engine. Aerospace, 9(10), 593. https://doi.org/10.3390/aerospace9100593







