Influence of Ice Accretion on Stratospheric Airship in the Non-Forming Ascending Process
Abstract
:1. Introduction
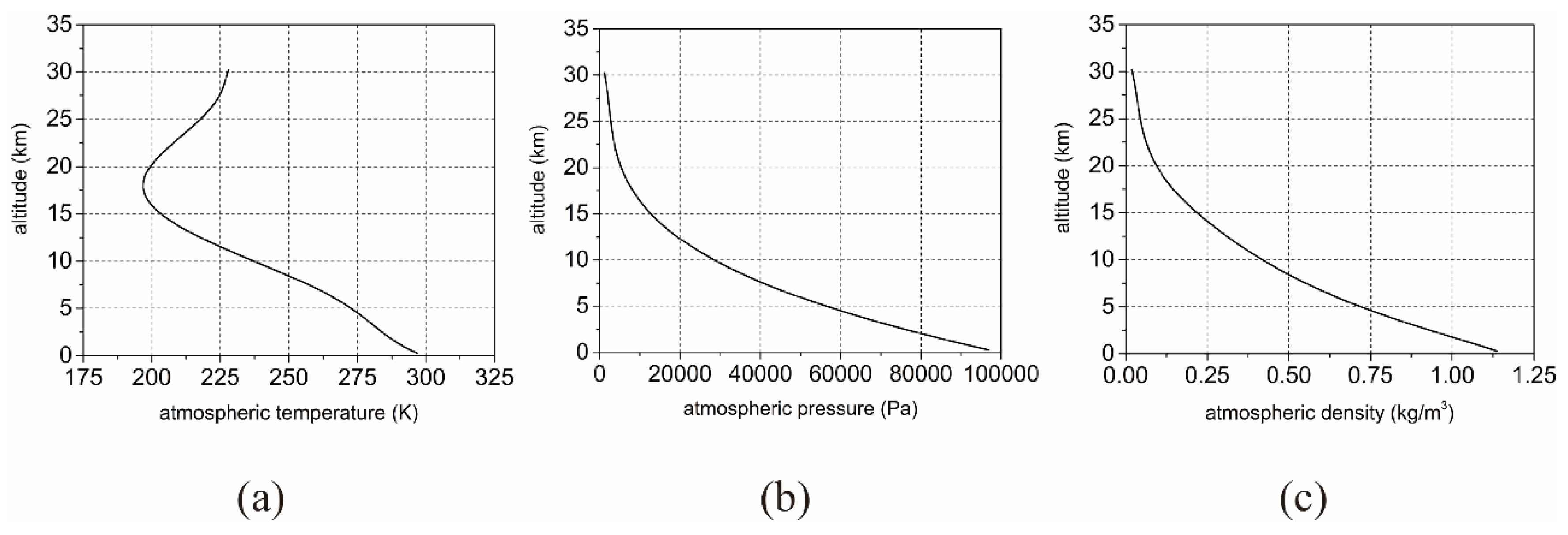
2. Methodology
2.1. Ice Accretion on Airship
2.2. Thermal and Dynamic Model of the Airship
2.3. Program Development Process
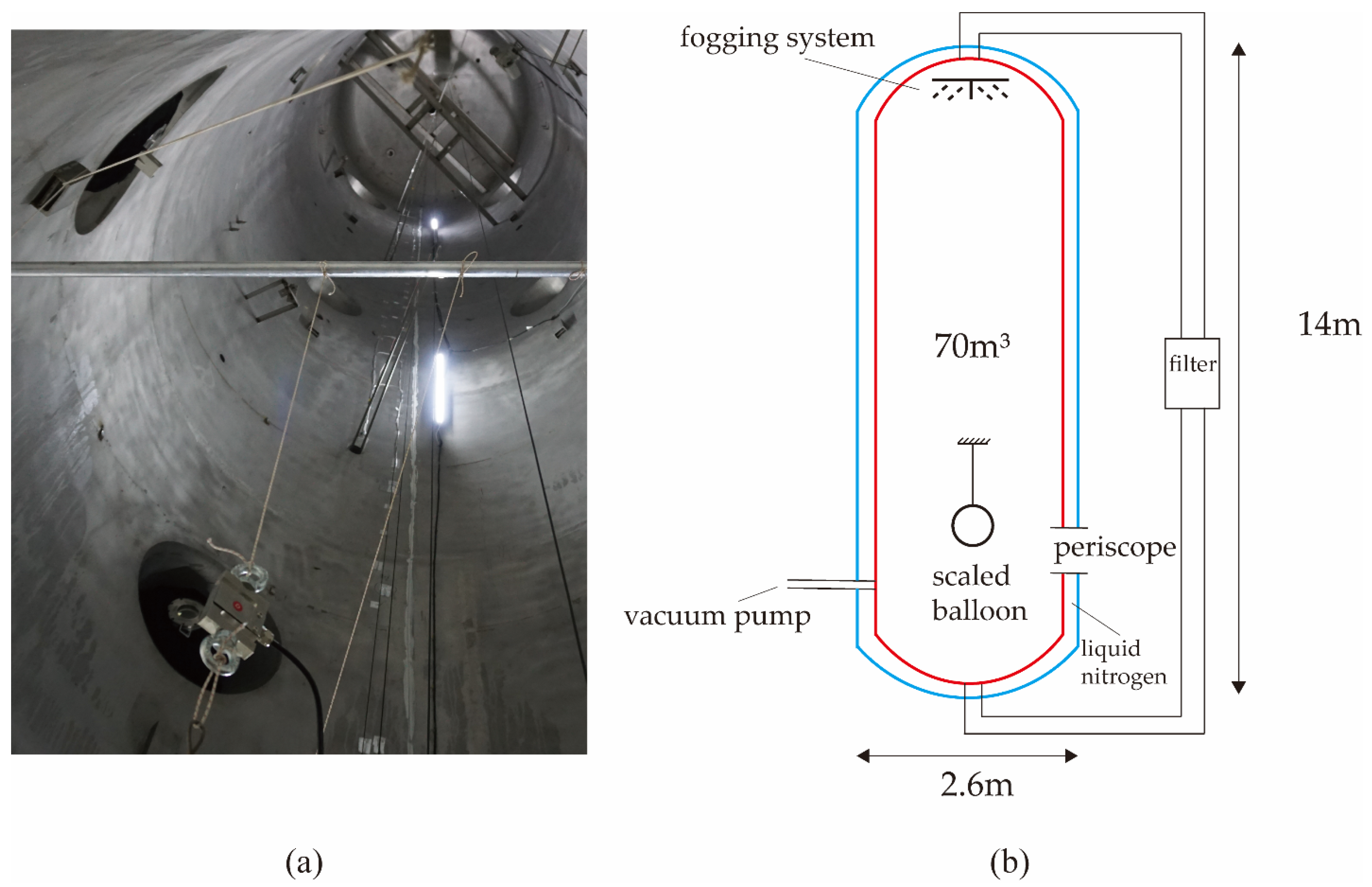
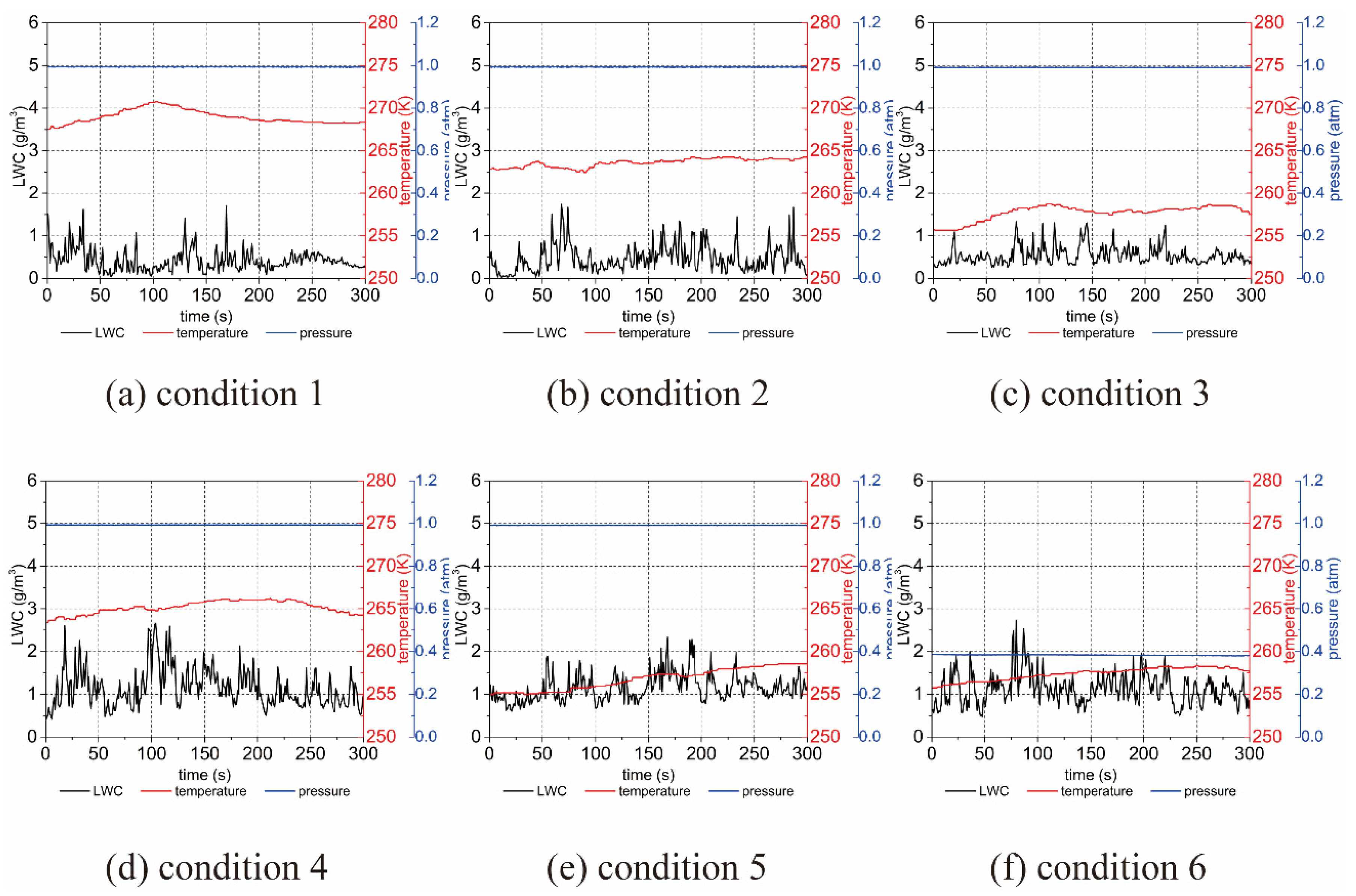
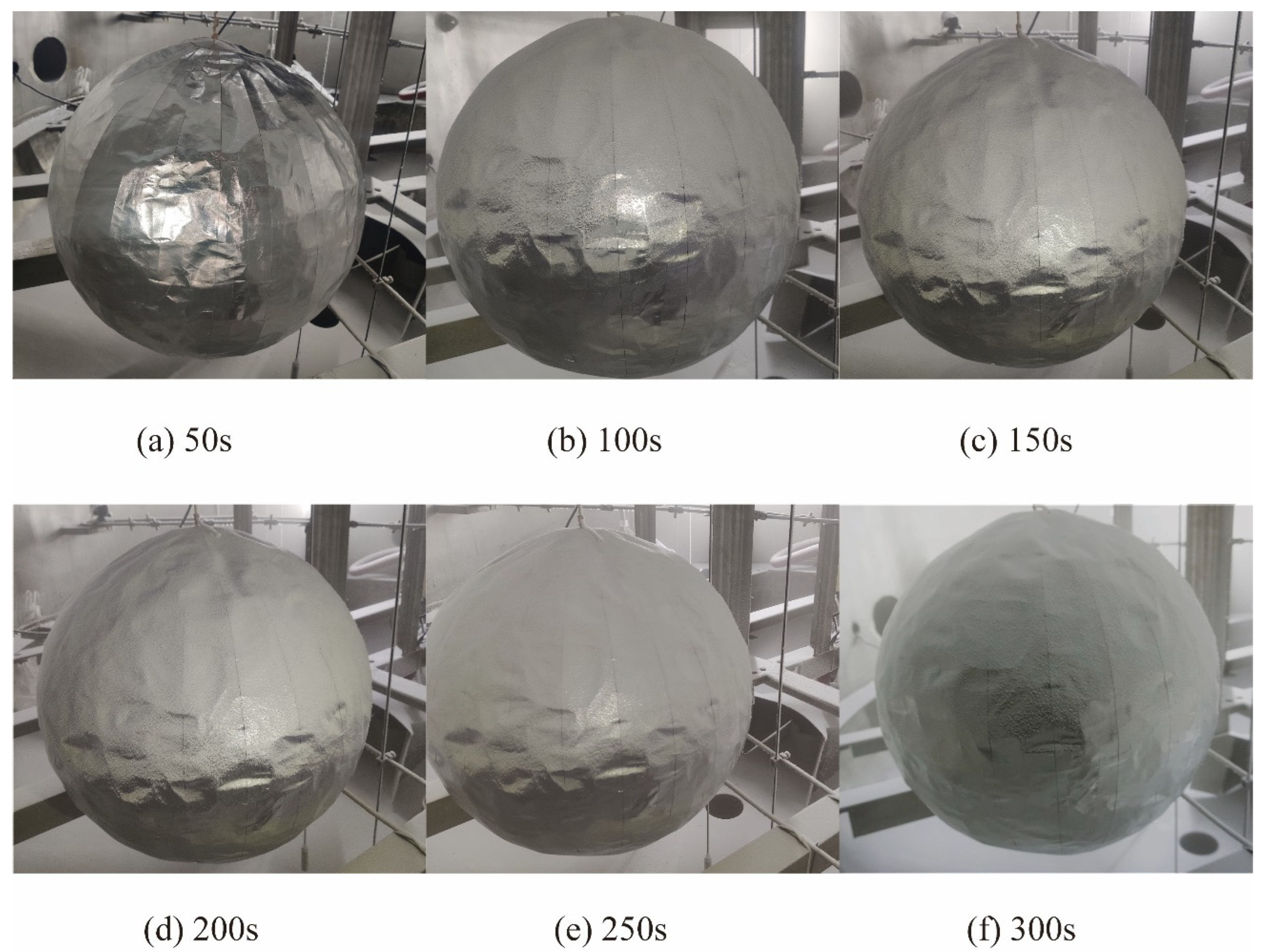
3. Experimental Validation
4. Results and Discussion
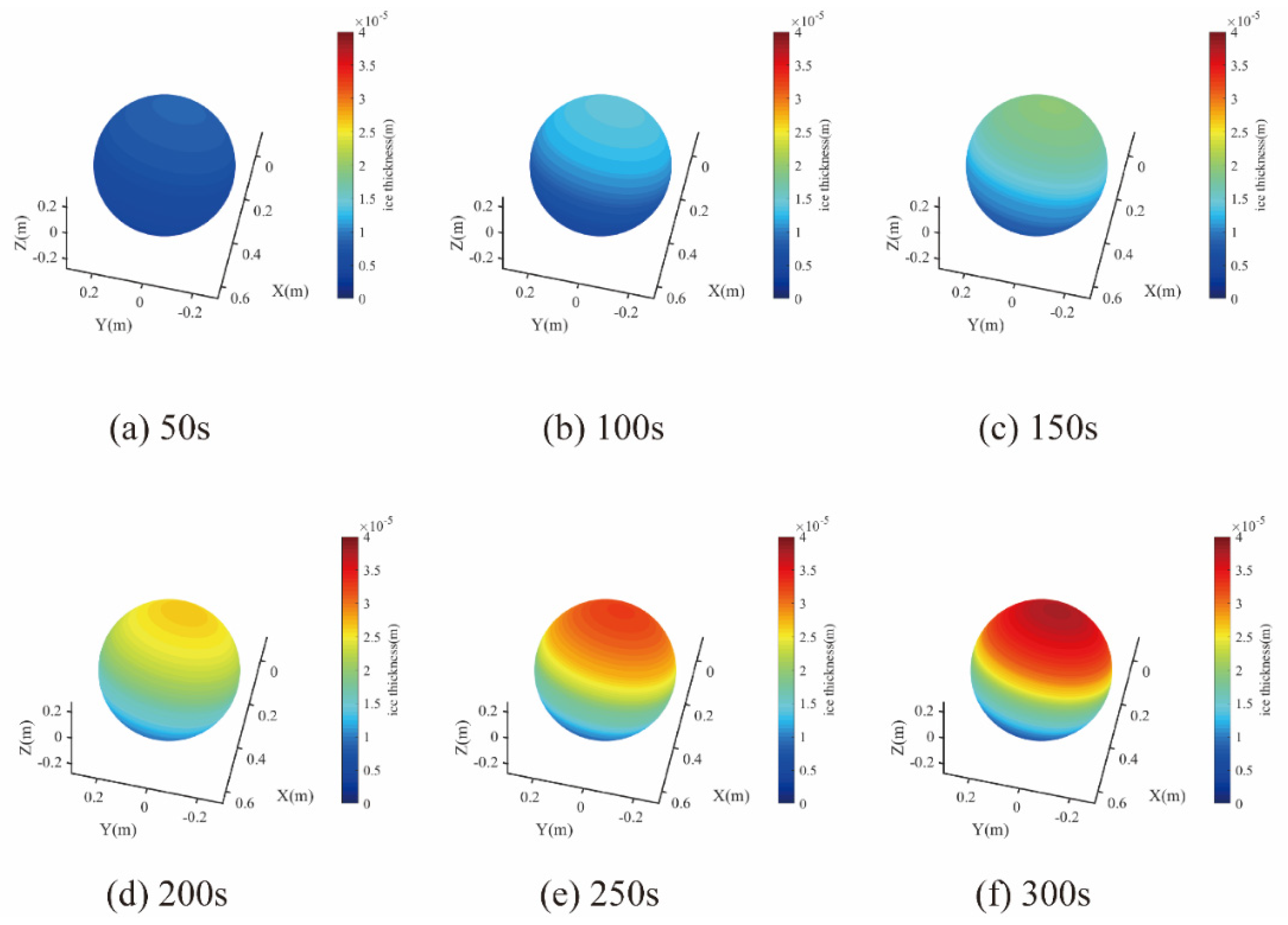
4.1. Model Establishment
4.2. Effect of Liquid Water Content
4.3. Effect of Helium Mass
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Zuo, Z.; Song, J.; Zheng, Z.; Han, Q.-L. A survey on modelling, control and challenges of stratospheric airships. Control Eng. Pract. 2021, 119, 104979. [Google Scholar]
- Li, J.; Lv, M.; Sun, K.; Zhu, W. Thermal insulation performance of lightweight substrate for solar array on stratospheric airships. Appl. Therm. Eng. 2016, 107, 1158–1165. [Google Scholar] [CrossRef]
- Li, J.; Lv, M.; Tan, D.; Zhu, W.; Sun, K.; Zhang, Y. Output performance analyses of solar array on stratospheric airship with thermal effect. Appl. Therm. Eng. 2016, 104, 743–750. [Google Scholar] [CrossRef]
- Liu, Q.; Cui, Y.; Yang, Y.; Cai, J.; Xu, G. Three dimensional simulation of stratospheric airship ice accretion in ascending process. In Proceedings of the AIAA SPACE 2016, Long Beach, CA, USA, 13–16 September 2016. [Google Scholar]
- Liu, Q.; Yang, Y.; Wang, Q.; Cui, Y.; Cai, J. Icing performance of stratospheric airship in ascending process. Adv. Space Res. 2019, 64, 2405–2416. [Google Scholar]
- Tran, P.; Brahimi, M.T.; Paraschivoiu, I.; Pueyo, A.; Tezok, F. Ice accretion on aircraft wings with thermodynamic effects. J. Aircr. 1995, 32, 444–446. [Google Scholar]
- Saeed, F.; Selig, M.S.; Bragg, M.B. Design of Subscale Airfoils with Full-Scale Leading Edges for Ice Accretion Testing. J. Aircr. 1997, 34, 94–100. [Google Scholar]
- Mingione, G.; Brandi, V. Ice Accretion Prediction on Multielement Airfoils. J. Aircr. 1998, 35, 240–246. [Google Scholar] [CrossRef]
- Cebeci, T.; Chen, H.H.; Alemdaroglu, N. Fortified LEWICE with viscous effects. J. Aircr. 1991, 28, 564–571. [Google Scholar]
- Zhao, S.; Liu, D.; Zhao, D.; Wu, G.; Yin, S.; Zhou, P. Change rules of a stratospheric airship’s envelope shape during ascent process. Chin. J. Aeronaut. 2017, 30, 752–758. [Google Scholar] [CrossRef]
- Smith, I.; Lee, M. The HiSentinel Airship. In Proceedings of the 7th AIAA ATIO Conf, 2nd CEIAT Int’l Conf on Innov and Integr in Aero Sciences, 17th LTA Systems Tech Conf; Followed by 2nd TEOS Forum, Belfast, UK, 18–20 September 2007. [Google Scholar]
- Androulakakis, S.P.; Judy, R. Status and Plans of High Altitude Airship (HAATM) program. In Proceedings of the AIAA Lighter-Than-Air Systems Technology (LTA) Conference, Daytona Beach, FL, USA, 25–28 March 2013. [Google Scholar]
- Lewis, W.; Perkins, P.J. A Flight Evaluation and Analysis of the Effect of Icing Conditions on the ZPG-2 Airship; technical report archive & image library; Lewis Flight Propulsion Laboratory: Cleveland, OH, USA, 1958. [Google Scholar]
- Bragg, M.; Hutchison, T.; Merret, J. Effect of ice accretion on aircraft flight dynamics. In Proceedings of the 38th Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 10–13 January 2000. [Google Scholar]
- Messinger, B.L. Equilibrium Temperature of an Unheated Icing Surface as a Function of Air Speed. J. Aeronaut. Sci. 1953, 20, 29–42. [Google Scholar] [CrossRef]
- Shen, X.; Lin, G.; Yu, J.; Bu, X.; Du, C. Three-Dimensional Numerical Simulation of Ice Accretion at the Engine Inlet. J. Aircr. 2013, 50, 635–642. [Google Scholar] [CrossRef]
- Cao, Y.; Huang, J.; Yin, J. Numerical simulation of three-dimensional ice accretion on an aircraft wing. Int. J. Heat Mass Transf. 2016, 92, 34–54. [Google Scholar] [CrossRef]
- Fortin, G.; Laforte, J.-L.; Ilinca, A. Heat and mass transfer during ice accretion on aircraft wings with an improved roughness model. Int. J. Therm. Sci. 2006, 45, 595–606. [Google Scholar] [CrossRef]
- Özgen, S.; Canıbek, M. Ice accretion simulation on multi-element airfoils using extended Messinger model. Heat Mass Transf. 2008, 45, 305–322. [Google Scholar] [CrossRef]
- Beaugendre, H.; Morency, F.; Habashi, W.G. FENSAP-ICE’s Three-Dimensional In-Flight Ice Accretion Module: ICE3D. J. Aircr. 2003, 40, 239–247. [Google Scholar] [CrossRef]
- Bu, X.; Huang, P.; Lin, G. Numerical simulation of mixed phase icing on two-dimensional airfoil. Acta Astronautica et Astronautica Sinica 2020, 41, 124085. [Google Scholar]
- Bu, X.; Lin, G.; Yu, J.; Shen, X.; Hou, P. Numerical analysis of a swept wing hot air ice protection system. Proc. Inst. Mech. Eng. Part G J. Aerosp. Eng. 2013, 228, 1507–1518. [Google Scholar] [CrossRef]
- Incropera, F.P. Fundamentals of Heat and Mass Transfer, 2nd ed.; John Wiley & Sons: Hoboken, NJ, USA, 2007. [Google Scholar]
- Bu, X.; Lin, G.; Shen, X.; Hu, Z.; Wen, D. Numerical simulation of aircraft thermal anti-icing system based on a tight-coupling method. Int. J. Heat Mass Transf. 2020, 148, 119061. [Google Scholar] [CrossRef]
- Zhao, D.; Liu, D.; Zhu, M. A surrogate model for thermal characteristics of stratospheric airship. Adv. Space Res. 2018, 61, 2989–3001. [Google Scholar] [CrossRef]
- Lv, M.; Yao, Z.; Zhang, L.; Du, H.; Meng, J.; Li, J. Effects of solar array on the thermal performance of stratospheric airship. Appl. Therm. Eng. 2017, 124, 22–33. [Google Scholar] [CrossRef]
- Farley, R. BalloonAscent: 3-D Simulation Tool for the Ascent and Float of High-Altitude Balloons. In Proceedings of the AIAA 16th Lighter-Than-Air and Balloon Systems Conference, Arlington, VA, USA, 26–28 September 2005. [Google Scholar]
- Morris, A. (Ed.) Scientific Ballooning Handbook—NCAR TN/1A-99; National Center for Atmospheric Research: Boulder CO, USA, 1975. [Google Scholar]
- Busch, G.T.; Broeren, A.P.; Bragg, M.B. Aerodynamic Simulation of a Horn-Ice Accretion on a Subscale Model. J. Aircr. 2008, 45, 604–613. [Google Scholar] [CrossRef]
- Su, Z.; Guo, X.; Zhuge, J.; Wang, P. Developing and Testing of an Expansion Cloud Chamber for Cloud Physics Research. J. Appl. Meteorol. Sci. 2019, 30, 722–730. [Google Scholar]
- Palumbo, R.; Russo, M.; Filippone, E.; Corraro, F. ACHAB: Analysis code for high-altitude balloons. In Proceedings of the AIAA Atmospheric Flight Mechanics Conference and Exhibit, Hilton Head, SC, USA, 20–23 August 2013; p. 6642. [Google Scholar]
- Garde, G. Comparison of Two Balloon Flight Simulation Programs. In Proceedings of the AIAA Atio & Lighter-Than-Air Sys Tech & Balloon Systems Conferences, Arlington, VA, USA, 26–28 September 2005. [Google Scholar]




| Heat | Expression |
| direct solar radiation | |
| atmospheric diffuse radiation | |
| ground reflected radiation | |
| atmospheric infrared radiation | |
| ground infrared radiation | |
| envelope infrared radiation | |
| external heat convection | |
| internal heat convection |
| Conditions | Pressure (Atm) | Liquid Water Content (g/m3) | Temperature (K) | Ice Mass(g) |
|---|---|---|---|---|
| 1 | 1.0 | 0.5 | 268 | 19 |
| 2 | 1.0 | 0.5 | 263 | 34 |
| 3 | 1.0 | 0.5 | 258 | 49 |
| 4 | 1.0 | 1.0 | 263 | 38 |
| 5 | 1.0 | 1.0 | 258 | 64 |
| 6 | 0.4 | 1.0 | 258 | 31 |
| Design Parameter | Values |
|---|---|
| Maximum volume/m3 | 113,000 |
| Cruising height/km | 30 |
| Balloon mass/kg | 1150 |
| Designed helium mass/kg | 335 |
| Load mass/kg | 600 |
| Launch time | 15 July, 8 a.m. |
| Visible spectrum absorption | 0.2 |
| Infrared spectrum emissivity | 0.7 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fu, C.; Zhu, M.; Ding, Y.; Tian, P.; Huang, M.; Liu, D.; Zhao, D. Influence of Ice Accretion on Stratospheric Airship in the Non-Forming Ascending Process. Aerospace 2022, 9, 536. https://doi.org/10.3390/aerospace9100536
Fu C, Zhu M, Ding Y, Tian P, Huang M, Liu D, Zhao D. Influence of Ice Accretion on Stratospheric Airship in the Non-Forming Ascending Process. Aerospace. 2022; 9(10):536. https://doi.org/10.3390/aerospace9100536
Chicago/Turabian StyleFu, Chenrui, Ming Zhu, Yang Ding, Ping Tian, Mengyu Huang, Dongxu Liu, and Da Zhao. 2022. "Influence of Ice Accretion on Stratospheric Airship in the Non-Forming Ascending Process" Aerospace 9, no. 10: 536. https://doi.org/10.3390/aerospace9100536
APA StyleFu, C., Zhu, M., Ding, Y., Tian, P., Huang, M., Liu, D., & Zhao, D. (2022). Influence of Ice Accretion on Stratospheric Airship in the Non-Forming Ascending Process. Aerospace, 9(10), 536. https://doi.org/10.3390/aerospace9100536






