Global Skewness and Coherence for Hypersonic Shock-Wave/Boundary-Layer Interactions with Pressure-Sensitive Paint
Abstract
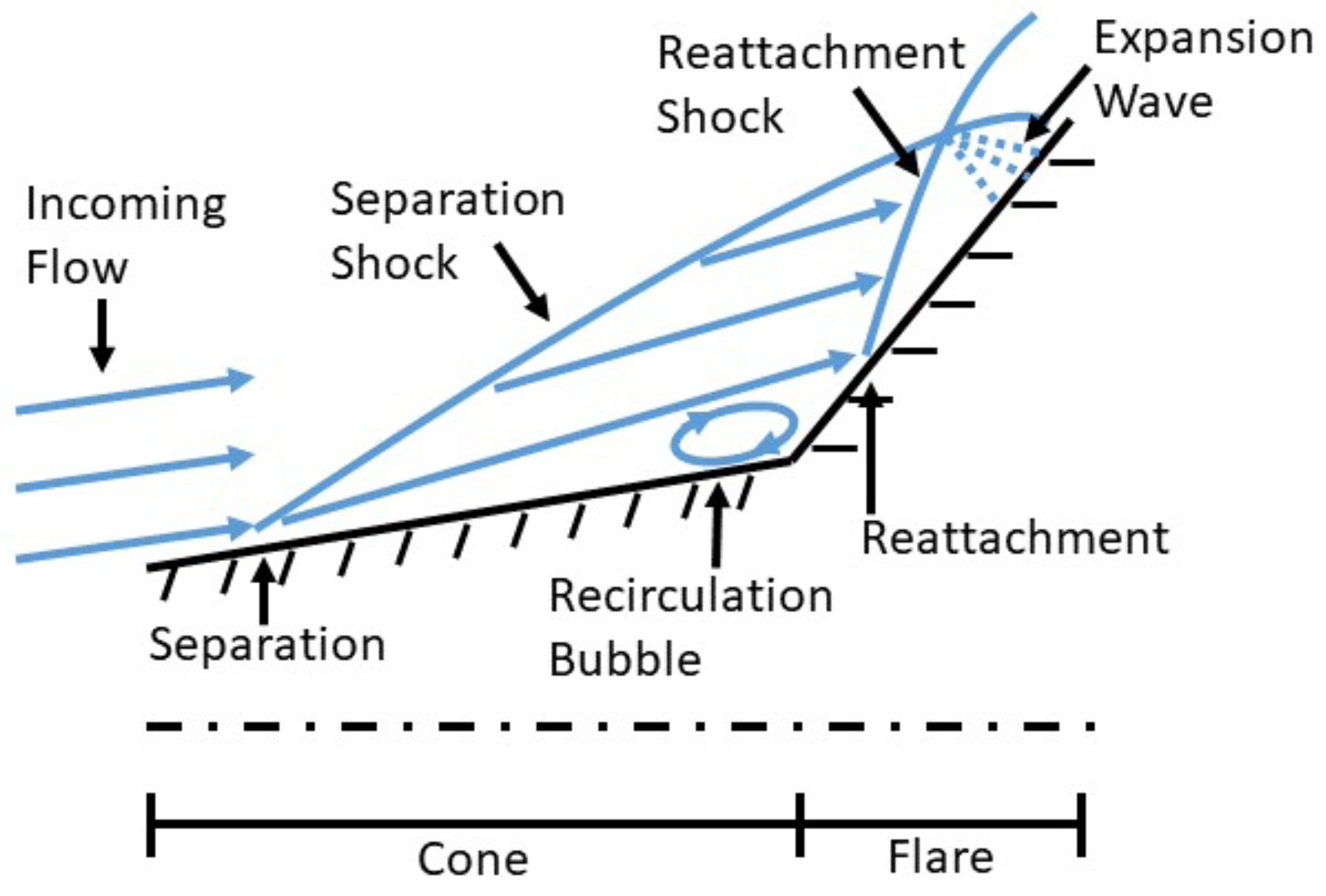
1. Introduction
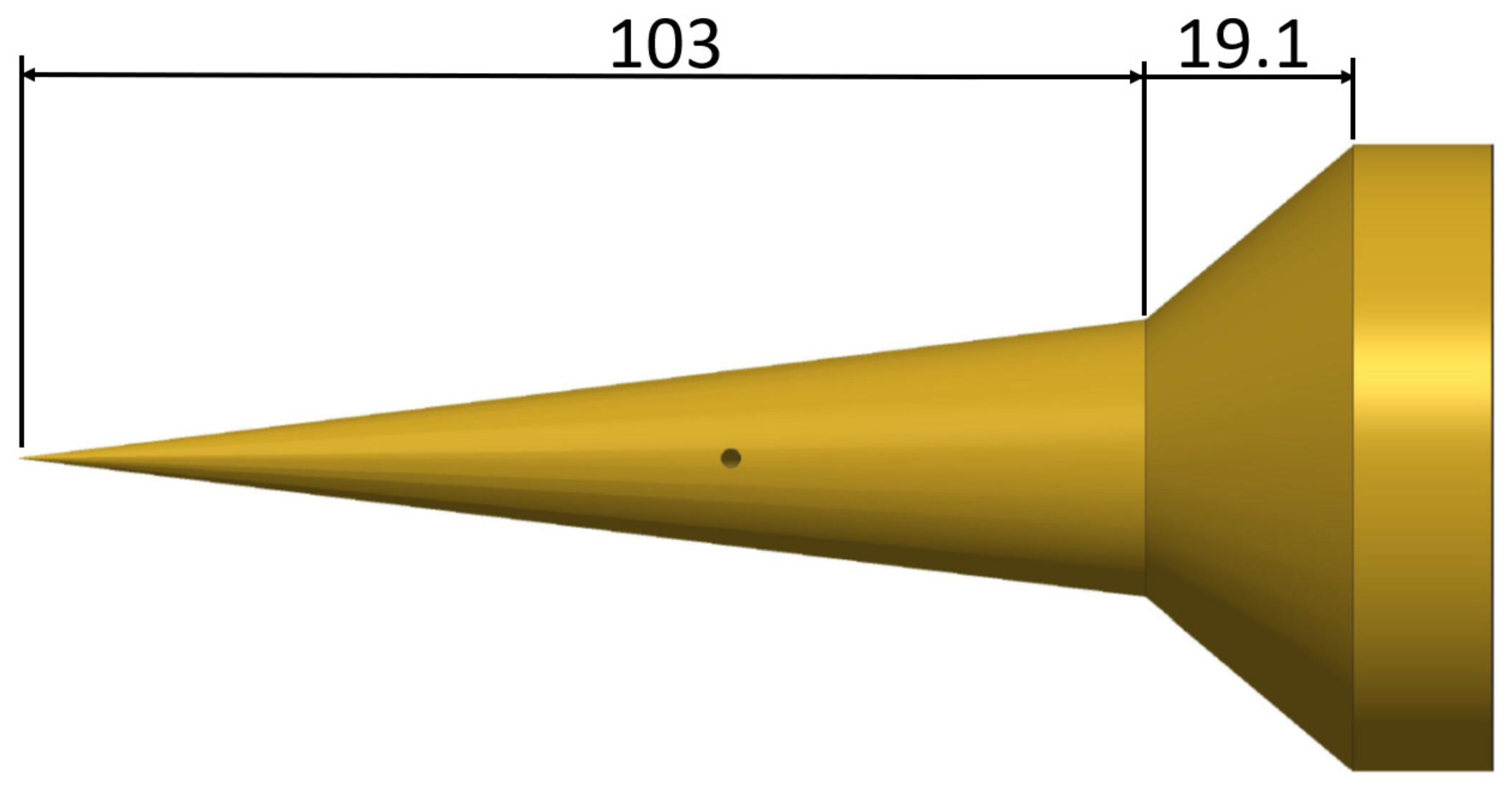
2. Materials and Methods
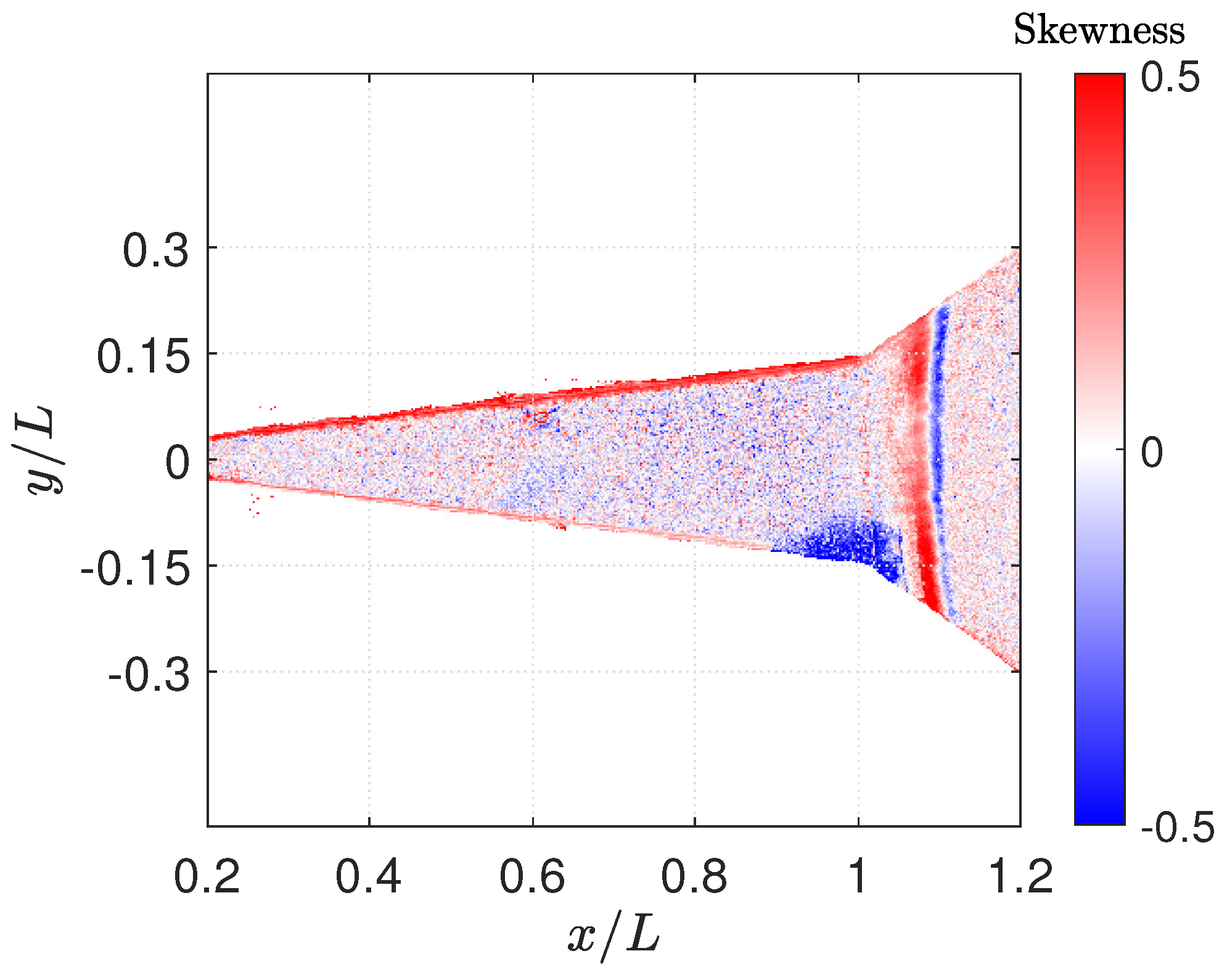
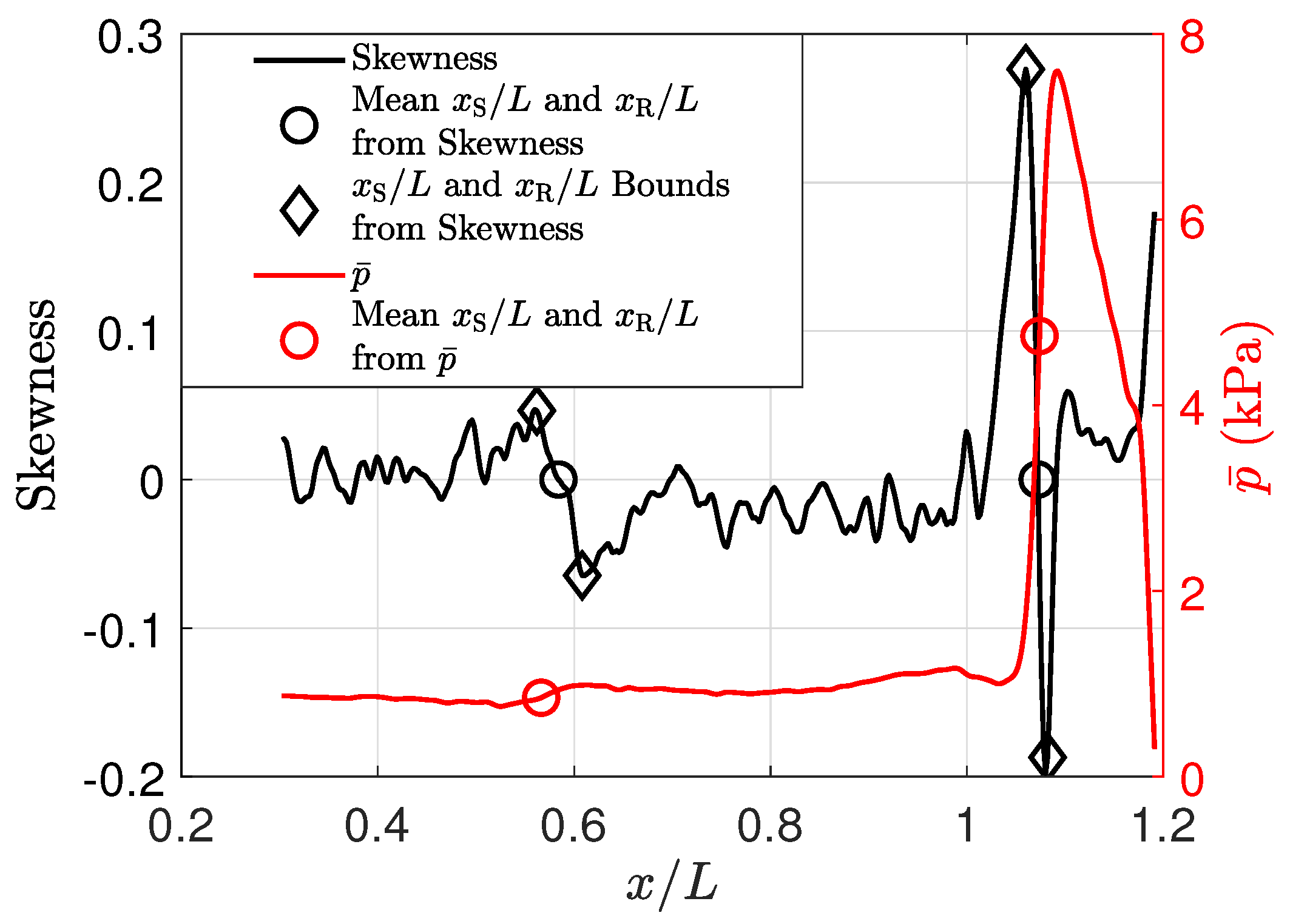
3. Results and Discussion
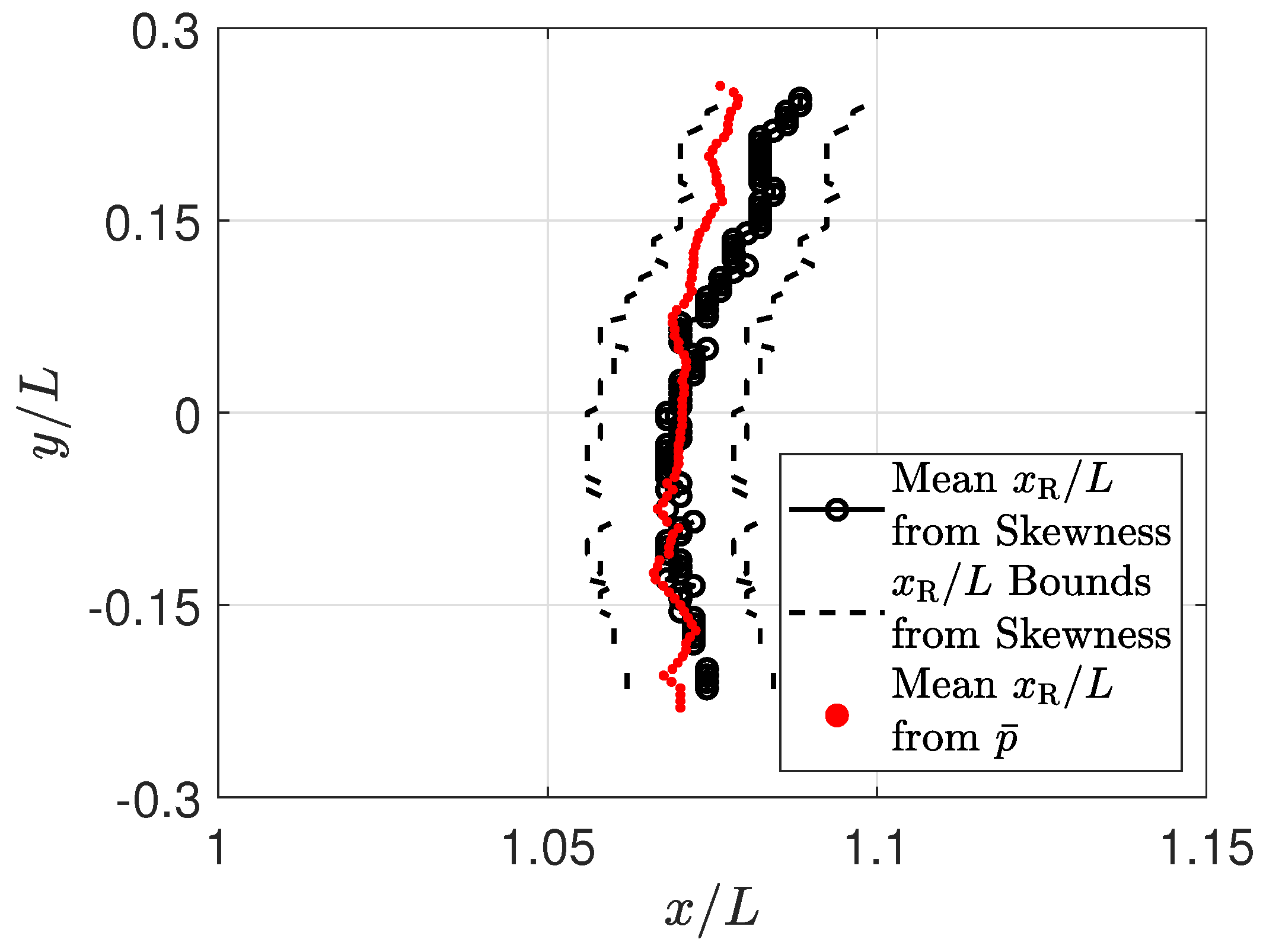
Skewness
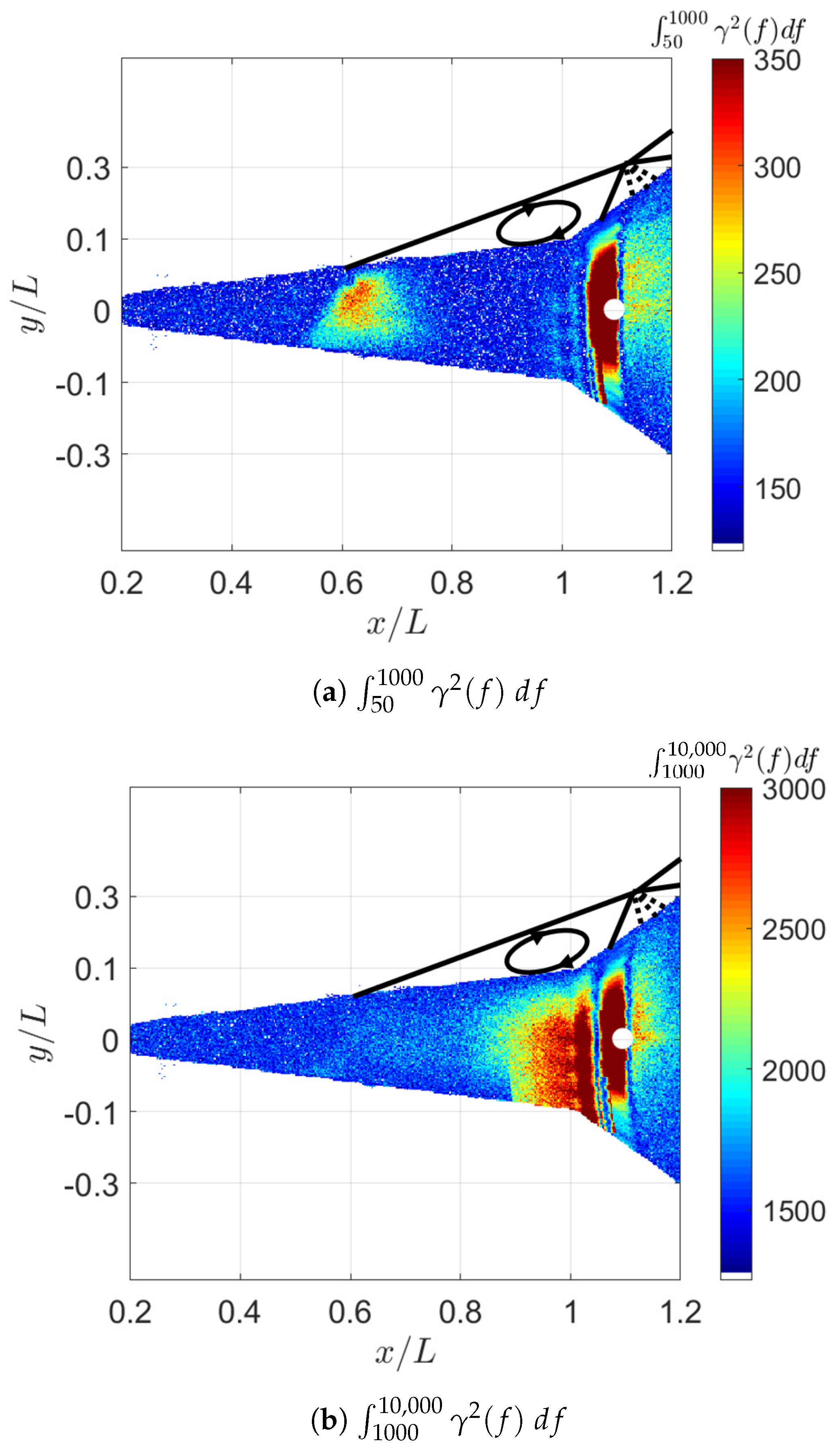
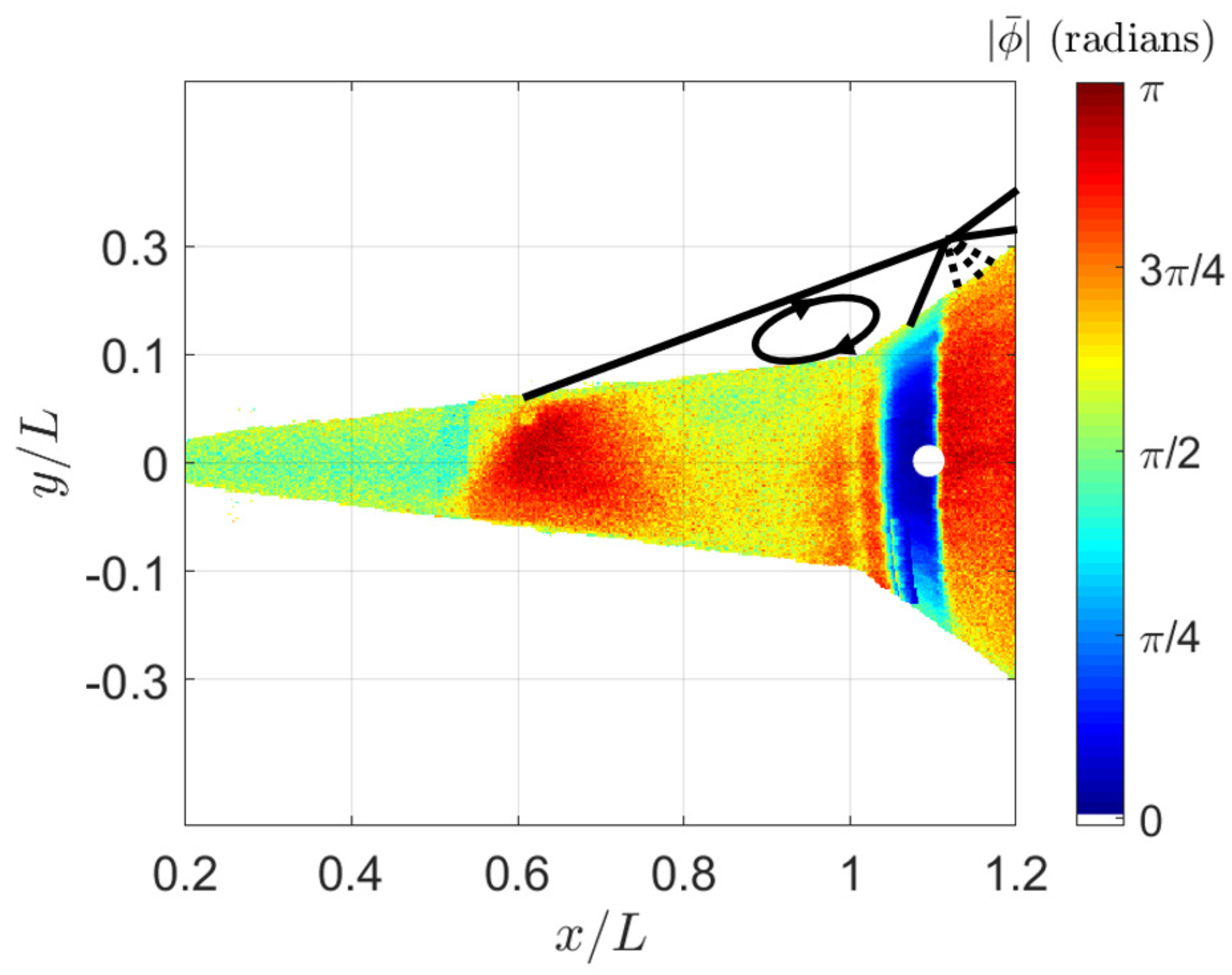
4. Coherence
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Babinsky, H.; Harvey, J. Shock Wave-Boundary-Layer Interactions; Cambridge: New York, NY, USA, 2011. [Google Scholar]
- Anderson, J. Hypersonic and High Temperature Gas Dynamics; American Institute of Aeronautics and Astronautics, Inc.: Reston, VA, USA, 2006; Chapter 7. [Google Scholar]
- Dupont, P.; Haddad, C.; Debiéve, J.F. Space and time organization in a shock-induced separated boundary layer. J. Fluid Mech. 2006, 559, 255–277. [Google Scholar] [CrossRef]
- Poggie, J.; Bisek, N.J.; Kimmel, R.L.; Stanfield, S.A. Spectral Characteristics of Separation Shock Unsteadiness. AIAA J. 2015, 53, 200–214. [Google Scholar] [CrossRef]
- Clemens, N.T.; Narayanaswamy, V. Low-Frequency Unsteadiness of Shock Wave/Turbulent Boundary Layer Interactions. Annu. Rev. Fluid Mech. 2014, 46, 469–492. [Google Scholar] [CrossRef]
- Casper, K.M.; Beresh, S.J.; Henfling, J.F.; Spillers, R.W.; Hunter, P.; Spitzer, S. Hypersonic Fluid-Structure Interactions on a Slender Cone. In Proceedings of the 2018 AIAA Aerospace Sciences Meeting, Kissimmee, FL, USA, 8–12 January 2018. [Google Scholar]
- Green, J. Interactions Between Shock Waves and Turbulent Boundary Layers. Prog. Aerosp. Sci. 1970, 11, 235–340. [Google Scholar] [CrossRef]
- Degrez, G.; Simeonides, G.; Delery, J.; Vandromme, D.; Dolling, D.; Knight, D. Shock-Wave/Boundary-Layer Interaction in Supersonic and Hypersonic Flows; Report 792; Advisory Group for Aerospace Research and Development: Rhode-Saint-Genése, Belgium, 1993. [Google Scholar]
- Dolling, D.S. Fifty Years of Shock-Wave/Boundary-Layer Interaction Research: What Next? AIAA J. 2001, 39, 1517–1531. [Google Scholar] [CrossRef]
- Gaitonde, D. Progress in Shock Wave/Boundary Layer Interactions. Prog. Aerosp. Sci. 2015, 72, 80–99. [Google Scholar] [CrossRef]
- Piponniau, S.; Dussauge, J.P.; Debiéve, J.F.; Dupont, P. A Simple Model for Low-Frequency Unsteadiness in Shock-Induced Separation. J. Fluid Mech. 2009, 629, 87–108. [Google Scholar] [CrossRef]
- Kimmel, R.L.; Adamczak, D.W.; Paull, A.; Shannon, J.; Pietsch, R.; Frost, M.; Alesi, H. HIFiRE-1 Preliminary Aerothermodynamic Measurements. In Proceedings of the 41st AIAA Fluid Dynamics Conference and Exhibit, Honolulu, HI, USA, 27 June–30 June 2011. [Google Scholar]
- Thiele, T.; Gülhan, A.; Olivier, H. Instrumentation and Aerothermal Postflight Analysis of the Rocket Technology Flight Experiment ROTEX-T. J. Spacecr. Rockets 2018, 55, 1050–1073. [Google Scholar] [CrossRef]
- Holden, M.; Carr, Z.; MacLean, M.; Wadhams, T. Measurements in Regions of Shock Wave/Turbulent Boundary Layer Interactions from Mach 5 to 6 at Flight Duplicated Velocities to Evaluate and Improve the Models of Turbulence in CFD Codes. In Proceedings of the 2018 Fluid Dynamics Conference, Atlanta, GA, USA, 25–29 June 2018. [Google Scholar]
- Heffner, K.; Chpoun, A.; Lengrand, J. Experimental Study of Transitional Axisymmetric Shock-Boundary Layer Interactions at Mach 5. In Proceedings of the 23rd Fluid Dynamics, Plasmadynamics, and Lasers Conference, Orlando, FL, USA, 6–9 July 1993. [Google Scholar]
- Vandomme, L.; Chanetz, B.; Benay, R.; Perraud, J. Transitional Shock Wave Boundary Layer Interactions in Hypersonic Flow at Mach 5. In Proceedings of the 12th AIAA International Space Planes and Hypersonic Systems and Technologies Conference, Norfolk, VA, USA, 15–19 December 2003. [Google Scholar]
- Benay, R.; Chanetz, B.; Mangin, B.; Vandomme, L.; Perraud, J. ShockWave/Transitional Boundary-Layer Interactions in Hypersonic Flow. AIAA J. 2006, 44, 1243–1254. [Google Scholar] [CrossRef]
- Wadhams, T.; Mundy, E.; Maclean, M.; Holden, M. Ground Test Studies of the HIFiRE-1 Transition Experiment Part 1: Experimental Results. J. Spacecr. Rockets 2008, 45, 1134–1148. [Google Scholar] [CrossRef]
- Maclean, M.; Wadhams, T.; Holden, M.; Johnson, H. Ground Test Studies of the HIFiRE-1 Transition Experiment Part 2: Computational Analysis. J. Spacecr. Rockets 2008, 45, 1149–1164. [Google Scholar] [CrossRef]
- Bur, R.; Chanetz, B. Experimental study on the PRE-X vehicle focusing on the transitional shock-wave/boundary-layer interactions. Aerosp. Sci. Technol. 2009, 13, 393–401. [Google Scholar] [CrossRef]
- Olivier, F.; Jacques, C.; Jean-Paul, C. Numerical analysis of a separated flow on a supersonic cone-flare model. In Proceedings of the 34th AIAA Applied Aerodynamics Conference, Washington, DC, USA, 13–17 June 2016. [Google Scholar]
- Holden, M. Studies of the Mean and Unsteady Structure of Turbulent Boundary Layer Separation in Hypersonic Flow. In Proceedings of the 22nd Fluid Dynamics, Plasma Dynamics and Lasers Conference, Honolulu, HI, USA, 24–26 June 1991. [Google Scholar]
- Holden, M. Shock Interaction Phenomena in Hypersonic Flows. In Proceedings of the 29th AIAA Plasmadynamics and Lasers Conference, Albuquerque, NM, USA, 15–18 June 1998. [Google Scholar]
- Dieudonne, W.; Boerrigter, H.; Charbonnier, J. Hypersonic Flow on a Blunted Cone-Flare Model and in the VKI-H3 Mach 6 Wind Tunnel; von Karman Institute Technical Note 193; Karman Institute in Rhode-Saint-Genése: Rhode-Saint-Genése, Belgium, 1997. [Google Scholar]
- Asma, C.; Paris, S.; Tapsoba, M. Transitional Shock-Wave Boundary Layer Interaction over a Cone-Flare Model AT Hypersonic Conditions. In Proceedings of the Fourth Symposium on Aerothermodynamics for Space Vehicles, Capua, Italy, 15–18 October 2002. [Google Scholar]
- Schrijer, F.; Scarano, F. Experiments on Hypersonic Boundary Layer Separation and Reattachment on a Blunted Cone-Flare using Quantitative InfraRed Thermography. In Proceedings of the 12th AIAA International Space Planes and Hypersonic Systems and Technologies, Norfolk, VA, USA, 15–19 December 2003. [Google Scholar]
- Running, C.L.; Juliano, T.J.; Jewell, J.S.; Borg, M.P.; Kimmel, R.L. Hypersonic Shock-Wave/Boundary-Layer Interactions on a Cone/Flare. Exp. Therm. Fluid Sci. 2019, 109, 109911. [Google Scholar] [CrossRef]
- Arnal, D.; Délery, J. Laminar-Turbulent Transition and Shock Wave/Boundary Layer Interaction; Technical Report 116; RTO-EN-AVT; von Karman Institute: Rhode-Saint-Gen, Belgium, 2005. [Google Scholar]
- Bogdonoff, S.M. Some Experimental Studies of the Separation of Supersonic Boundary Layers; Report 336; Department of Aeronautical Engineering, Princeton University: Princeton, NJ, USA, 1955. [Google Scholar]
- Price, A.E.; Stallings, R.L. Investigation of Turbulent Separated Flows in the Vicinity of Fin Type Protuberances at Supersonic Mach Numbers; NASA TN D-3840; NASA: Washington, DC, USA, 1967.
- Kaufman, L.G.; Korkegi, R.H.; Morton, L. Shock Impingement Caused by Boundary Layer Separation Ahead of Blunt Fins; ARL 72-0118; National Technical Information Service (NTIS) US Department of Commerce: Springfield, VA, USA, 1972.
- Winkelmann, A.E. Experimental Investigations of a Fin Protuberance Partially Immersed in a Turbulent Boundary Layer at Mach 5; NOLTR-72-33; Naval Air Systems Command: Washington, DC, USA, 1972.
- Kistler, A.L. Fluctuating Wall Pressure Under a Separated Supersonic Flow. J. Acoust. Soc. Am. 1964, 36, 543–550. [Google Scholar] [CrossRef]
- Erengil, M.E.; Dolling, D.S. Unsteady Wave Structure Near Separation in a Mach 5 Compression Ramp Interaction. AIAA J. 1990, 29, 728–735. [Google Scholar] [CrossRef]
- Willems, S.; Gülhan, A. Experiments on Shock Induced Laminar-Turbulent Transition on a Flat Plate at Mach 6. In Proceedings of the European Conference for Aeronautics and Space Sciences (EUCASS), Munchen, Germany, 1–5 July 2013. [Google Scholar]
- Schülein, E. Effects of Laminar-Turbulent Transition on the Shock-Wave/Boundary-Layer Interaction. In Proceedings of the 44th AIAA Fluid Dynamics Conference, Atlanta, GA, USA, 16–20 June 2014. [Google Scholar]
- Willems, S.; Gülhan, A.; Steelant, J. Experiments on Shock Induced Laminar-Turbulent Transition on the SWBLI in H2K at Mach 6. Exp. Fluids 2015, 56, 1–19. [Google Scholar] [CrossRef]
- Stanfield, S.A.; Kimmel, R.L.; Adamczak, D.W. HIFiRE-1 Flight Data Analysis: Turbulent Shock-Boundary-Layer Interaction Experiment During Ascent. In Proceedings of the 42nd AIAA Fluid Dynamics Conference and Exhibit, New Orleans, LA, USA, 25–28 June 2012. [Google Scholar]
- Liu, T.; Campbell, B.; Bruns, S.; Sullivan, J.P. Temperature- and Pressure-Sensitive Luminescent Paints in Aerodynamics. Appl. Mech. Rev. 1997, 50, 227–246. [Google Scholar] [CrossRef]
- Gregory, J.W.; Sakaue, H.; Liu, T.; Sullivan, J.P. Fast Pressure-Sensitive Paint for Flow and Acoustic Diagnostics. Annu. Rev. Fluid Mech. 2014, 46, 303–330. [Google Scholar] [CrossRef]
- Taira, K.; Brunton, S.L.; Dawson, S.T.M.; Rowley, C.W.; Colonius, T.; McKeon, B.J.; Schmidt, O.T.; Gordeyev, S.; Theofilis, V.; Ukeiley, L.S. Modal Analysis of Fluid Flows: An Overview. AIAA J. 2017, 55, 4013–4041. [Google Scholar] [CrossRef]
- Running, C.L.; Juliano, T.J. Global measurements of hypersonic shock-wave/boundary-layer interactions with pressure-sensitive paint. Exp. Fluids 2021. [Google Scholar] [CrossRef]
- Currao, G.M.D.; McQuellin, L.P.; Neely, A.J.; Zander, F.; Buttsworth, D.R.; McNamara, J.J.; Iahn, I. Oscillating Shock Impinging on a Flat Plate at Mach 6. In Proceedings of the AIAA Aviation 2019 Forum, Dallas, TX, USA, 17–21 June 2019. [Google Scholar]
- Lash, L.E.; Combs, C.S.; Kreth, P.A.; Schmisseur, J.D. Study of the Dynamics of Transitional Shock Wave-Boundary Layer Interactions using Optical Diagnostics. In Proceedings of the 47th AIAA Fluid Dynamics Conference, Denver, CO, USA, 5–9 June 2017. [Google Scholar]
- Vanstone, L.; Goller, T.; Clemens, N.T.; Mears, L.J. Separated Flow Unsteadiness in a Mach 2 Swept Compression-Ramp Interaction using High-Speed PSP. In Proceedings of the AIAA Aviation 2019 Forum, Dallas, TX, USA, 17–21 June 2019. [Google Scholar]
- Funderburk, M.L.; Narayanaswamy, V. Spectral Signal Quality of Fast Pressure Sensitive Paint Measurements in Turbulent Shock-Wave/Boundary Layer Interactions. Exp. Fluids 2019, 60, 1–20. [Google Scholar] [CrossRef]
- Varigonda, S.V.; Narayanaswamy, V.; Boxx, I. Investigations of FSI Generated By an Impinging SBLI on a Thin Panel Using Multivariate Imaging of Flow/Structural Properties. In Proceedings of the AIAA Aviation 2020 Forum, Online, 15–19 June 2020. [Google Scholar]
- Mears, L.J.; Baldwin, A.; Ali, M.Y.; Kumar, R.; Alvi, F.S. Spatially resolved mean and unsteady surface pressure in swept SBLI using PSP. Exp. Fluids 2020, 61, 1–14. [Google Scholar] [CrossRef]
- Baccarella, D.; Liu, Q.; Passaro, A.; Lee, T.; Do, H. Development and testing of the ACT-1 experimental facility for hypersonic combustion research. Meas. Sci. Technol. 2016, 27, 045902. [Google Scholar] [CrossRef]
- Hoberg, E.M.; Huffman, C.; Sanchez-Plesha, N.; Running, C.L.; Kato, N.; Im, S.; Juliano, T.J. Characterization of Test Conditions in the Notre Dame Arc-Heated Wind Tunnel. In Proceedings of the AIAA Aviation 2019 Forum, Dallas, TX, USA, 17–21 June 2019. [Google Scholar]
- Running, C.L.; Sakaue, H.; Juliano, T.J. Hypersonic Boundary-Layer Separation Detection with Pressure-Sensitive Paint for a Cone at High Angle of Attack. Exp. Fluids 2019, 60, 1–13. [Google Scholar] [CrossRef]
- Juliano, T.J.; Peng, D.; Jensen, C.; Gregory, J.W.; Liu, T.; Montefort, J.; Palluconi, S.; Crafton, J.; Fonov, S. PSP Measurements on an Oscillating NACA 0012 Airfoil in Compressible Flow. In Proceedings of the 41st AIAA Fluid Dynamics Conference and Exhibit, Honolulu, HI, USA, 27–30 June 2011. [Google Scholar]
- Running, C.L.; Thompson, M.J.; Juliano, T.J.; Sakaue, H. Boundary-layer Separation Detection for a Cone at High Angle of Attack in Mach 4.5 Flow with Pressure-Sensitive Paint. In Proceedings of the 47th AIAA Fluid Dynamics Conference, Denver, CO, USA, 5–9 June 2017. [Google Scholar]
- Running, C.L. Global Measurements of Axisymmetric Hypersonic Shock-Wave/Boundary-Layer Interactions. Ph.D. Thesis, University of Notre Dame, Notre Dame, IN, USA, 2020. [Google Scholar]
- Pandey, A.; Casper, K.M.; Spillers, R.; Soehnel, M.; Spitzer, S. Hypersonic Shock Wave-Boundary-Layer Interaction on the Control Surface of a Slender Cone. In Proceedings of the AIAA Scitech 2020 Forum, Orlando, FL, USA, 6–10 January 2020. [Google Scholar]
- Sansica, A.; Sandham, N.D.; Hu, Z. Instability and Low-Frequency Unsteadiness in a Shock-Induced Laminar Separation Bubble. J. Fluid Mech. 2016, 798, 5–26. [Google Scholar] [CrossRef]
- Whalen, T.; Kennedy, R.; Laurence, S.; Sullivan, B.; Bodony, D.; Buck, G. Unsteady Surface and Flowfield Measurements in Ramp-Induced Turbulent and Transitional Shock-Wave Boundary-Layer Interactions at Mach 6. In Proceedings of the AIAA Scitech 2019 Forum, San Diego, CA, USA, 7–11 January 2019. [Google Scholar]
- Vermeulen, J.P.; Simeonides, G. Parametric Studies of Shock Wave/Boundary Layer Interactions Over 2D Compression Corners at Mach 6; Technical Report 181; von Karman Institute for Fluid Dynamics: Rhode-Saint-Gen, Belgium, 1992. [Google Scholar]
- Souverein, L.J.; Bakker, P.G.; Dupont, P. A scaling analysis for turbulent shock-wave/boundary-layer interactions. J. Fluid Mech. 2013, 714, 505–535. [Google Scholar] [CrossRef]
- Bendat, J.S.; Piersol, A.G. Random Data: Analysis and Measurement Procedures; Wiley: New York, NY, USA, 2010. [Google Scholar]
- Mears, L.J.; Arora, N.; Alvi, F.S. Flowfield Response to Controlled Perturbations in Swept Shock/Boundary-Layer Interaction Using Unsteady Pressure-Sensitive Paint. In Proceedings of the AIAA Scitech 2019 Forum, San Diego, CA, USA, 7–11 January 2019. [Google Scholar]
- Benitez, E.K.; Jewell, J.S.; Schneider, S.P. Separation Bubble Variation Due to Small Angles of Attack for an Axisymmetric Model at Mach 6. In Proceedings of the AIAA Scitech 2021 Forum, Online, 11–15 and 19–21 January 2021. [Google Scholar]
- Priebe, S.; Tu, J.H.; Rowley, C.W.; Martin, M.P. Low-Frequency Dynamics in a Shock-Induced Separated Flow. J. Fluid Mech. 2016, 807, 441–477. [Google Scholar] [CrossRef]
- Thomas, F.O.; Putnam, C.M.; Chu, H.C. On the Mechanism of Unsteady Shock Oscillation in Shock Wave/Turbulent Boundary Layer Interactions. Exp. Fluids 1994, 18, 69–81. [Google Scholar] [CrossRef]
- Dupont, P.; Haddad, C.; Ardissone, J.P.; Debiéve, J.F. Space and time organization of a shock wave/turbulent boundary layer interaction. Aerosp. Sci. Technol. 2005, 9, 561–572. [Google Scholar] [CrossRef]
- Stoica, P.; Moses, R. Spectral Analysis of Signals; Prentice Hall: Hoboken, NJ, USA, 2005. [Google Scholar]
- Welch, P.D. The use of Fast Fourier Transform for the estimation of power spectra: A method based on time averaging over short, modified periodograms. IEEE Trans. Audio Electroacoust. 1967, 15, 70–73. [Google Scholar] [CrossRef]
| (kPa) | (K) | (×10/m) | () | |
|---|---|---|---|---|
| 6.25 | 440 | 296 | 8.3 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Running, C.L.; Juliano, T.J. Global Skewness and Coherence for Hypersonic Shock-Wave/Boundary-Layer Interactions with Pressure-Sensitive Paint. Aerospace 2021, 8, 123. https://doi.org/10.3390/aerospace8050123
Running CL, Juliano TJ. Global Skewness and Coherence for Hypersonic Shock-Wave/Boundary-Layer Interactions with Pressure-Sensitive Paint. Aerospace. 2021; 8(5):123. https://doi.org/10.3390/aerospace8050123
Chicago/Turabian StyleRunning, Carson L., and Thomas J. Juliano. 2021. "Global Skewness and Coherence for Hypersonic Shock-Wave/Boundary-Layer Interactions with Pressure-Sensitive Paint" Aerospace 8, no. 5: 123. https://doi.org/10.3390/aerospace8050123
APA StyleRunning, C. L., & Juliano, T. J. (2021). Global Skewness and Coherence for Hypersonic Shock-Wave/Boundary-Layer Interactions with Pressure-Sensitive Paint. Aerospace, 8(5), 123. https://doi.org/10.3390/aerospace8050123






