Rope-Hook Recovery Controller Designed for a Flying-Wing UAV
Abstract
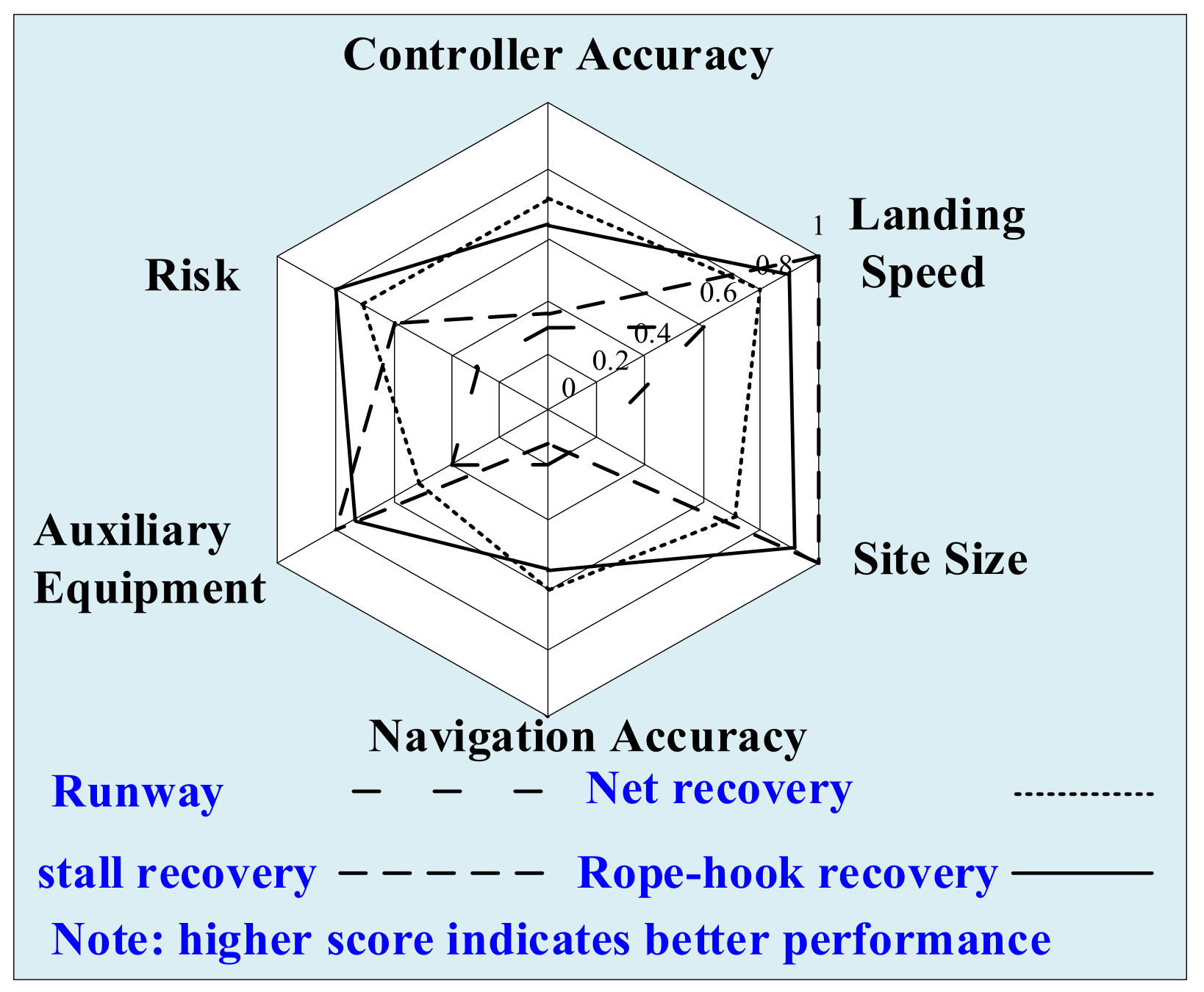
:1. Introduction
2. Navigation and Guidance
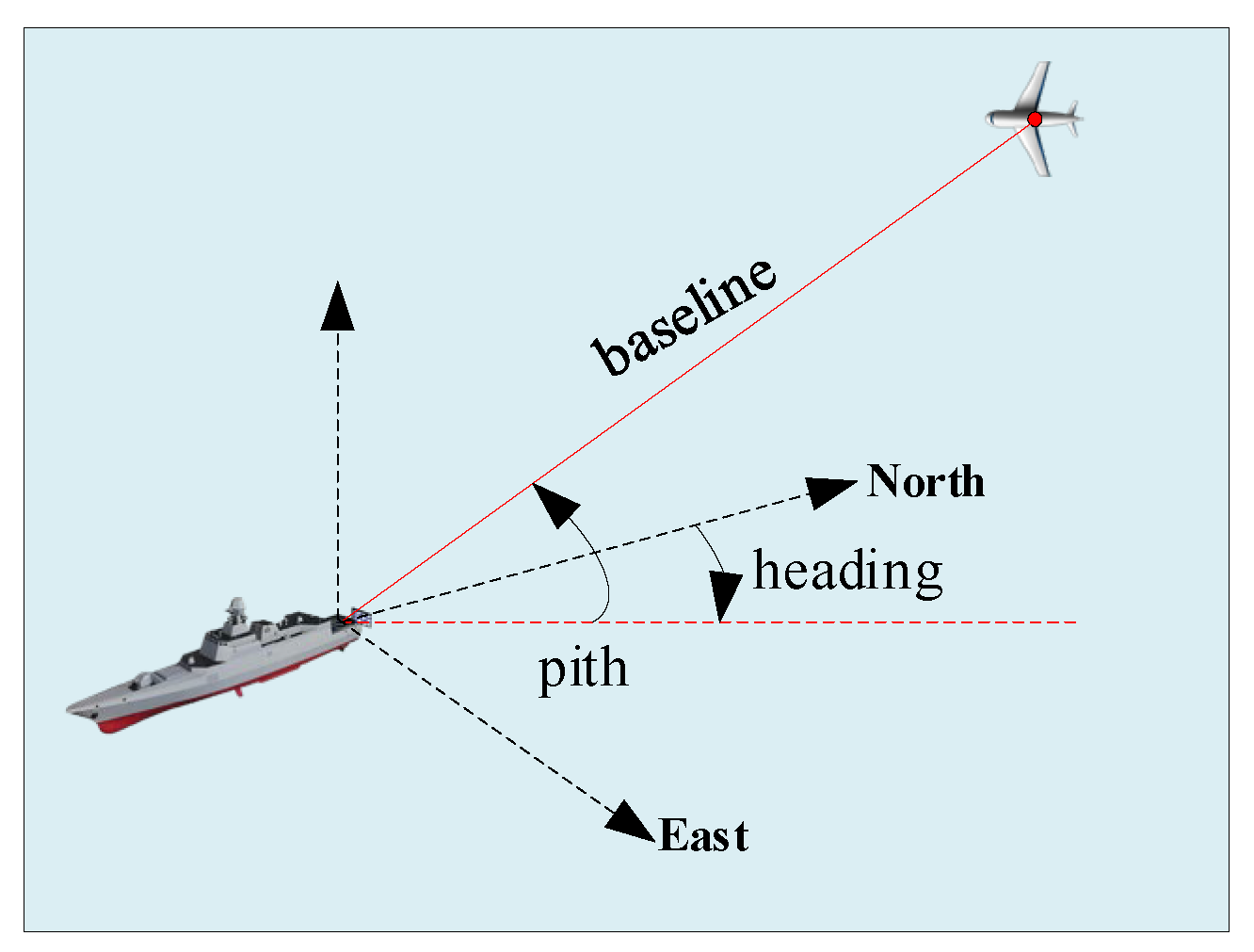
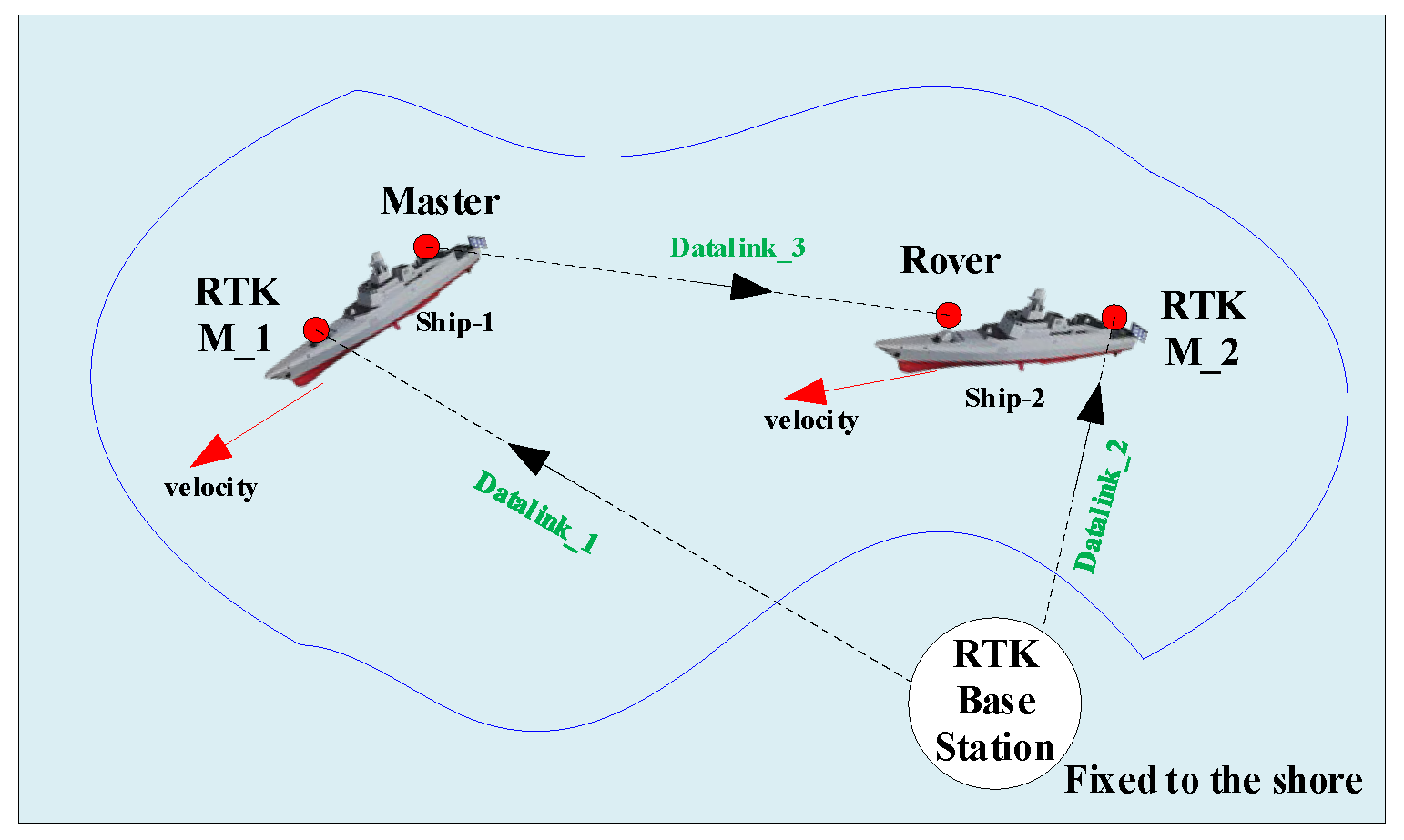
2.1. Navigation
- (1)
- Single-point positioning
- (2)
- Differential GPS
- (3)
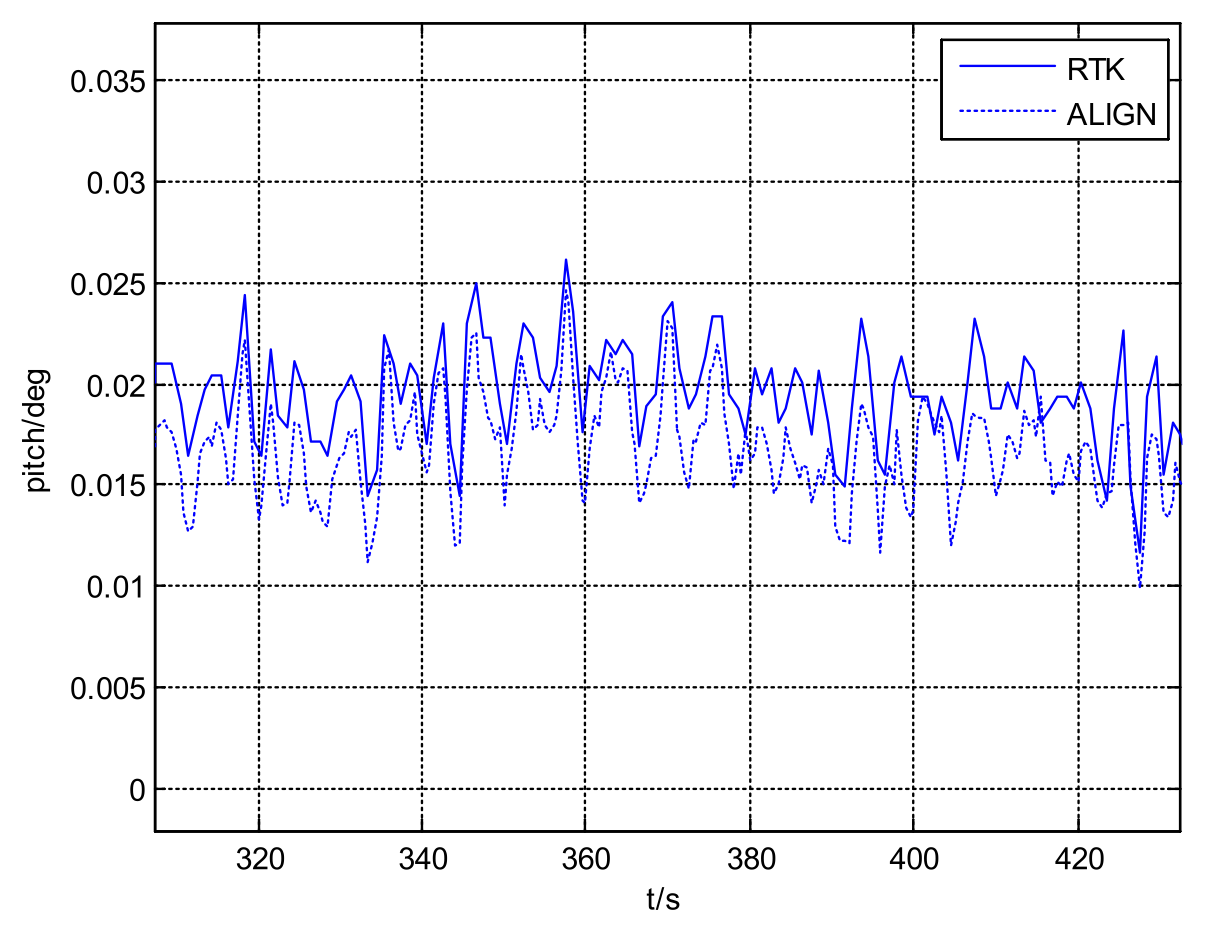
- ALIGN Precision test
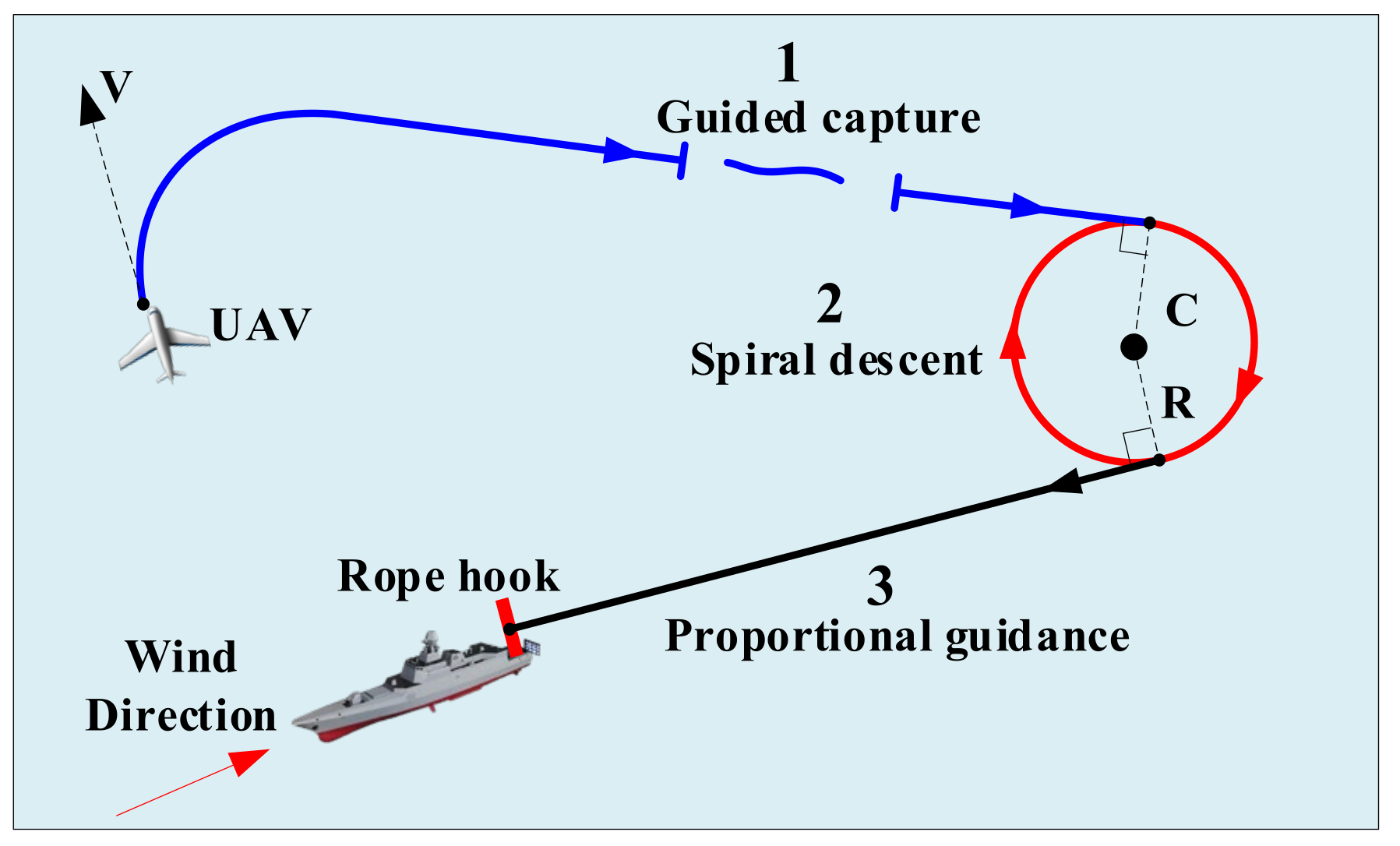
2.2. Guidance
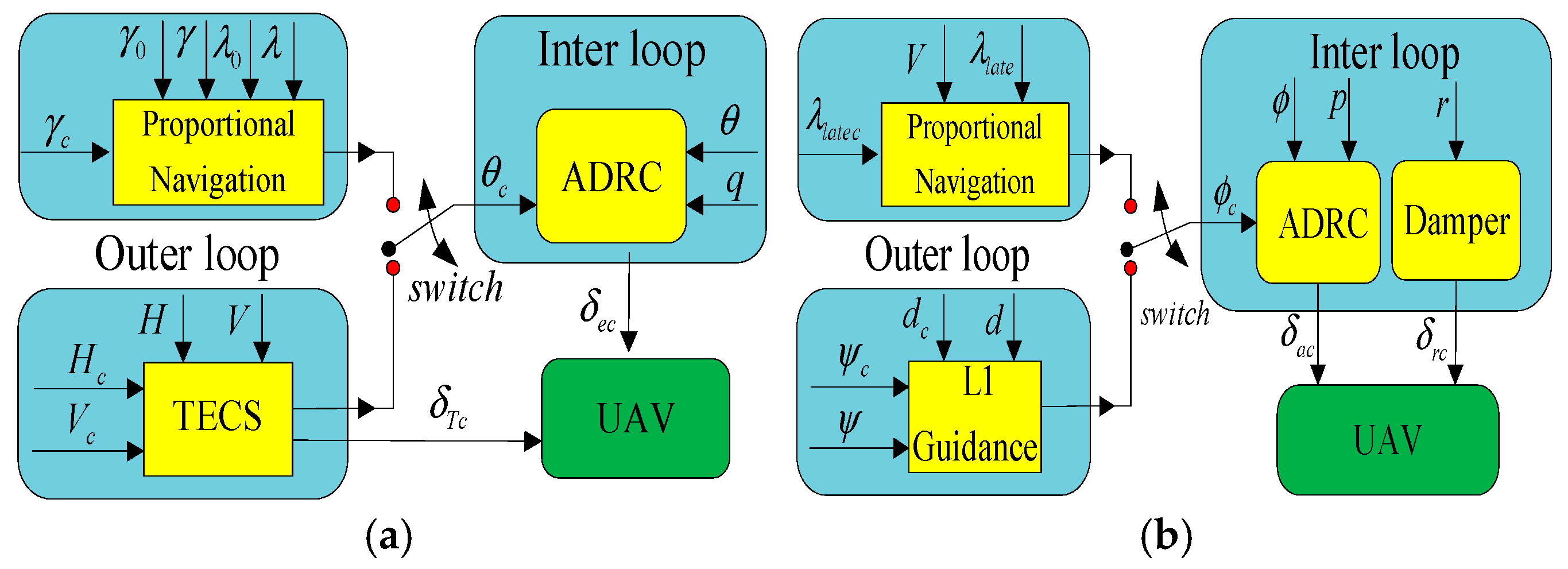
3. Controller Design
- (1)
- The UAV is rigid and its mass is constant;
- (2)
- The earth-surface inertial reference frame is an inertial coordinate system;
- (3)
- The curvature of the earth is ignored and the earth is flat;
- (4)
- The acceleration of gravity does not change with flight altitude;
- (5)
- The UAV is not only symmetrical in geometry, but also in internal mass distribution, that is, the product of inertia Ixy = Izy = 0;
- (6)
- Ignoring the longitudinal and lateral motion coupling, the motion of the UAV is divided into independent longitudinal motion and lateral motion.
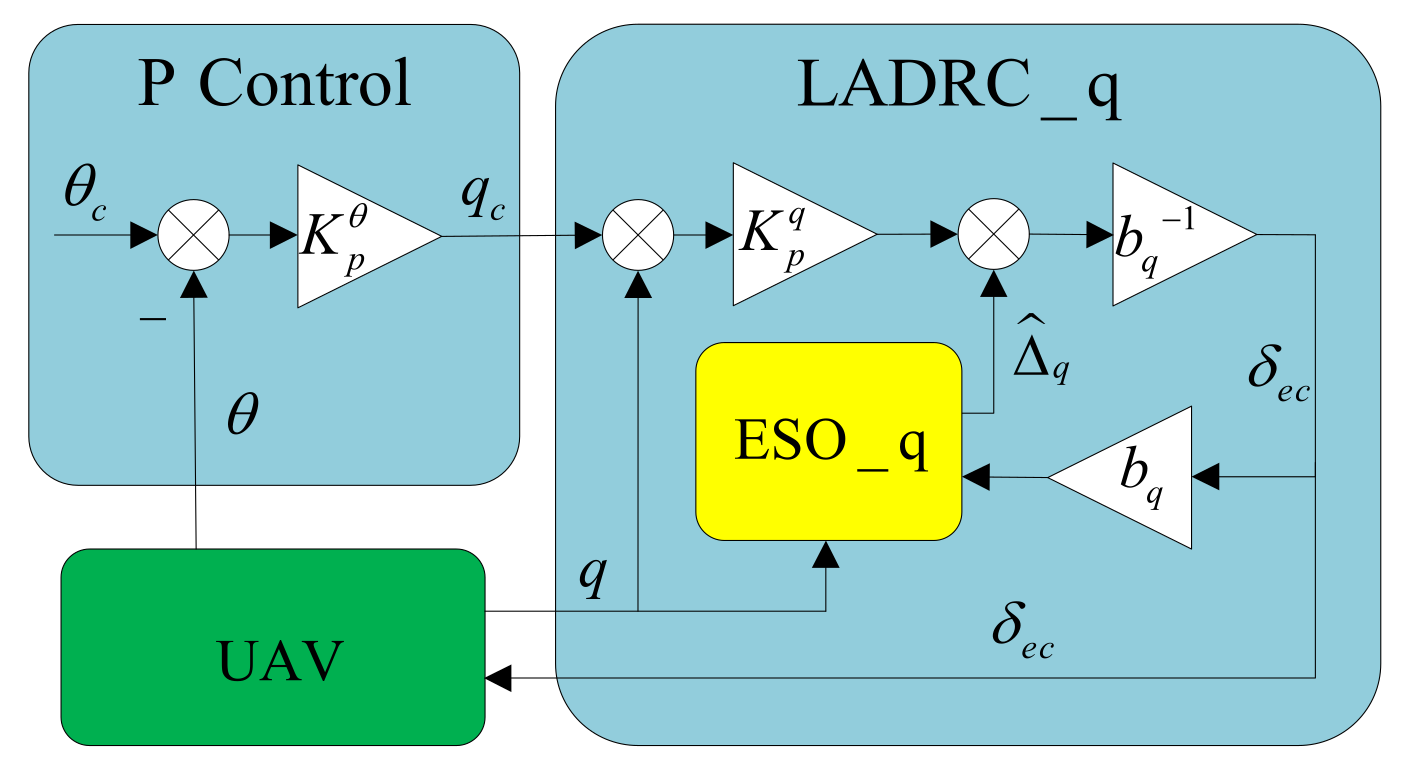
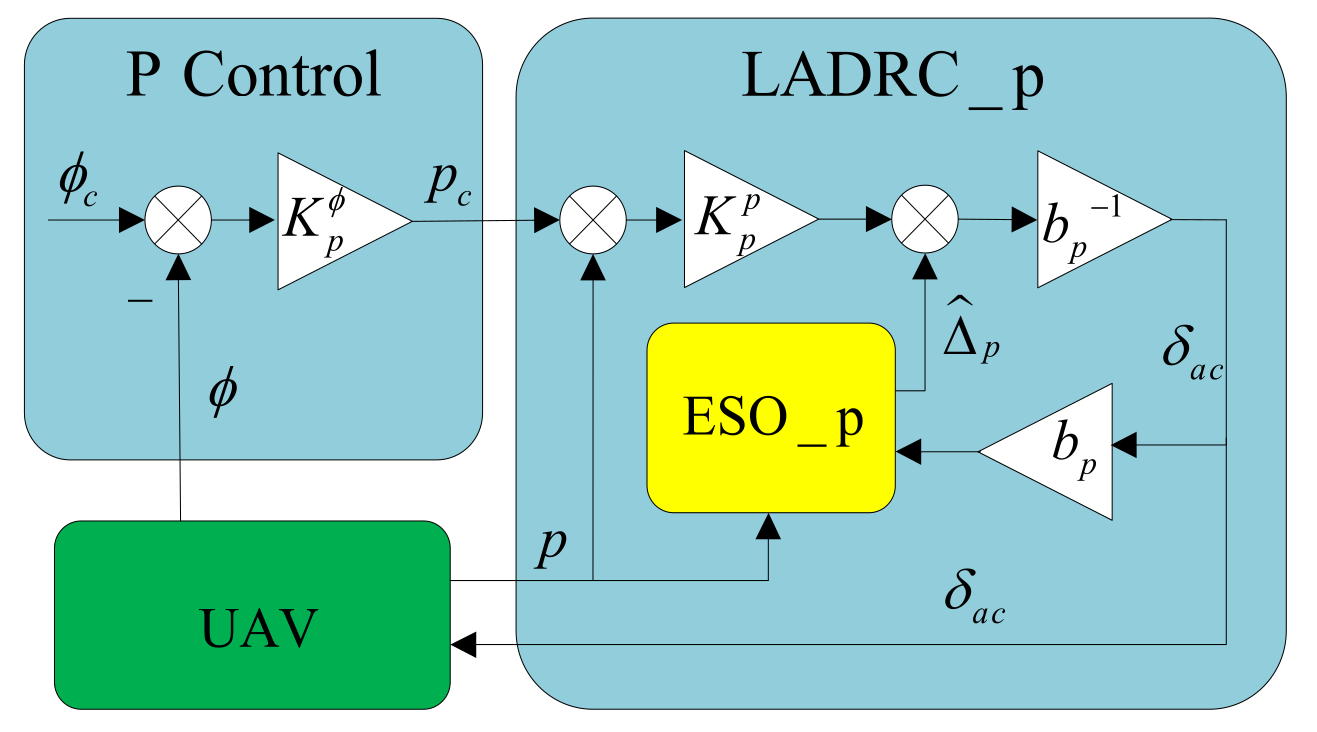
3.1. Attitude Controller Design
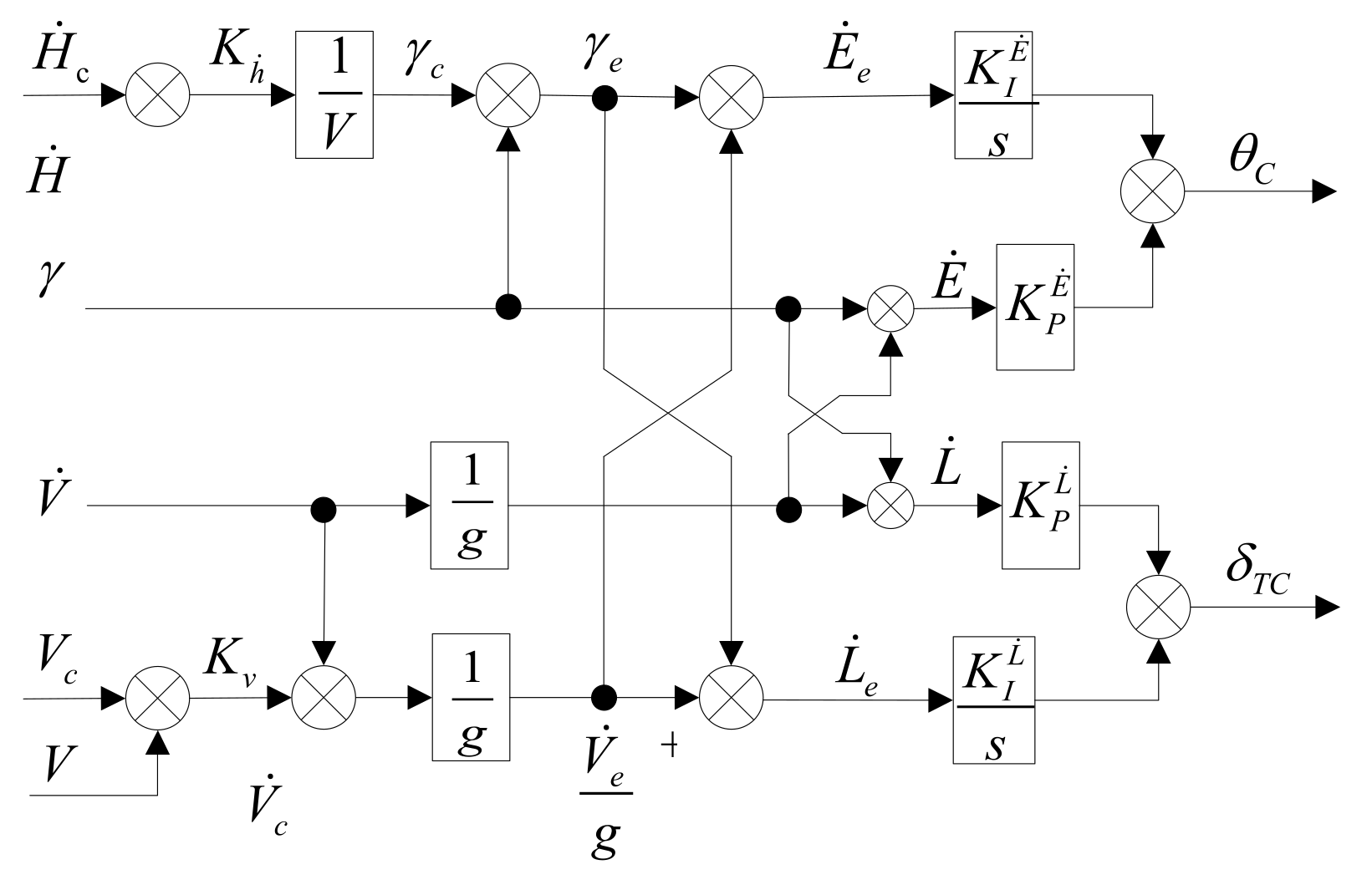
3.2. Height Controller Based on TECS
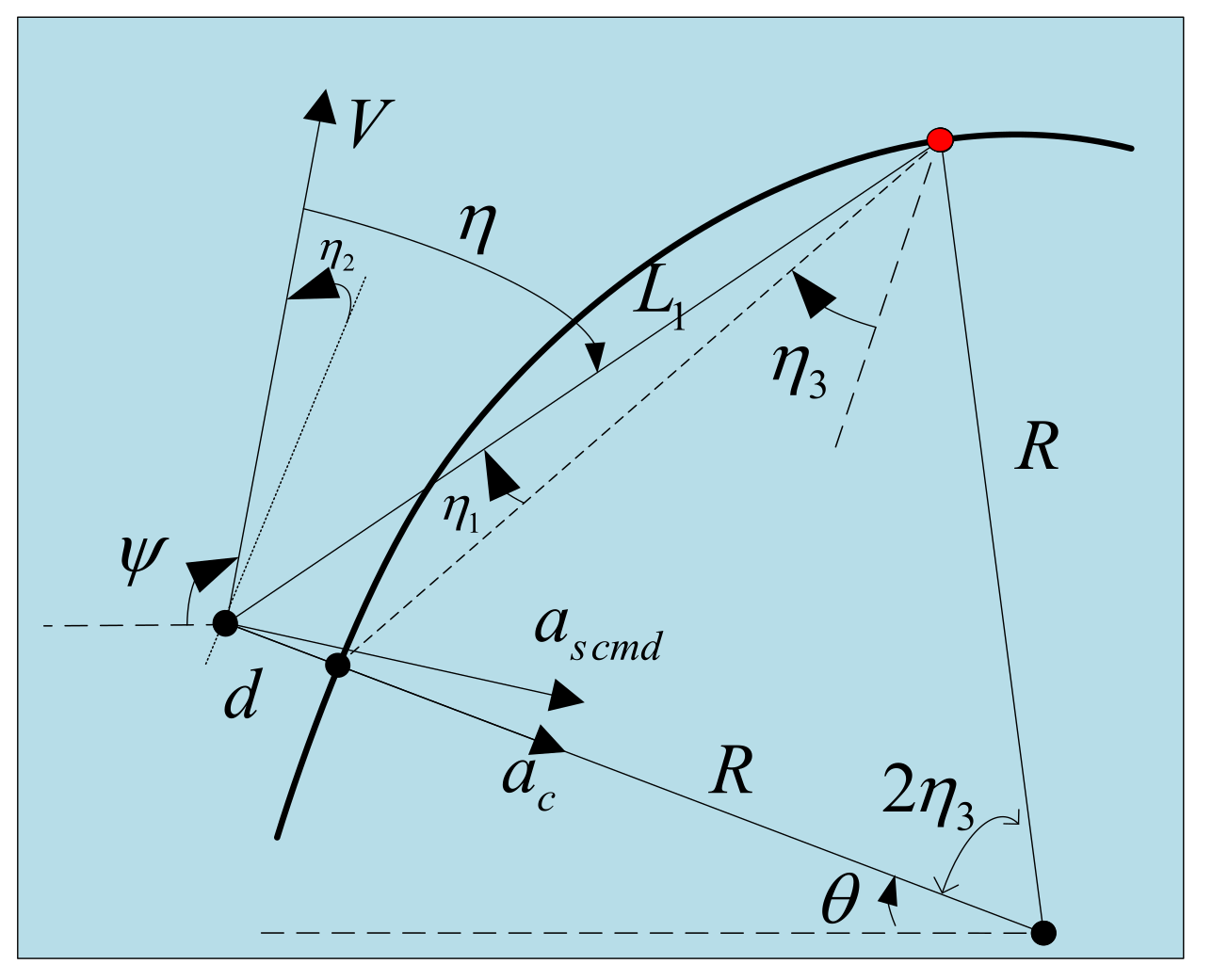
3.3. L1 Guidance Law for Lateral
3.4. Terminal PN Law
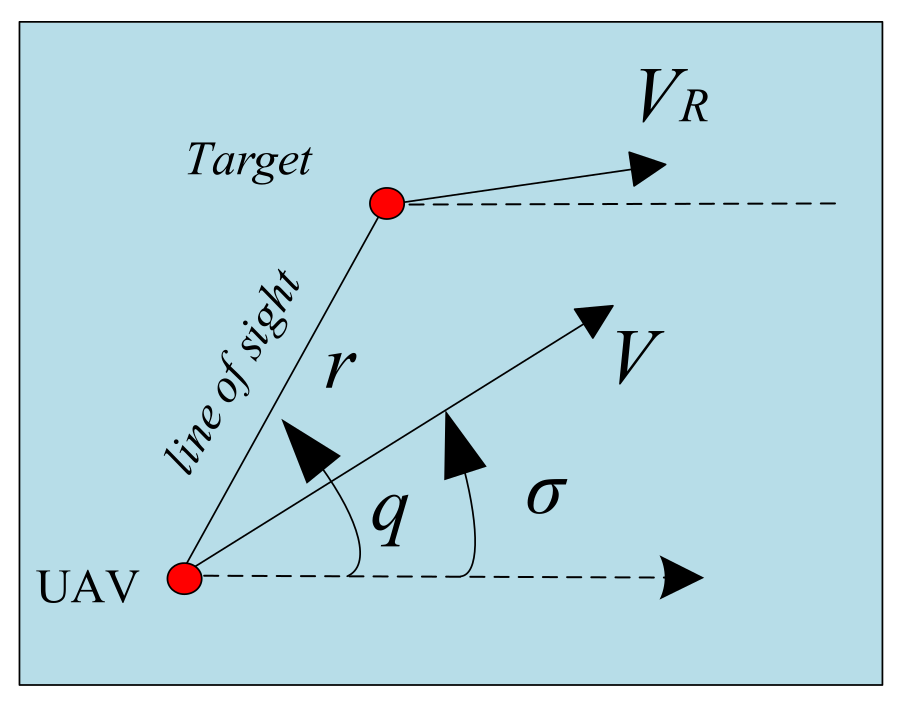
3.4.1. PN Law Based on Overload Control
- (1)
- The guidance law is based on the basic assumption of biplane decoupling. After heading correction, the UAV and the ship are in the same longitudinal motion plane. When conducting lateral guidance, the UAV and the ship are in the same lateral motion plane;
- (2)
- The motion of the UAV and the ship are particle motion in a two-dimensional plane;
- (3)
- Both the UAV and the ship move at a constant speed, and the ship moves in a straight line;
- (4)
- The response delay of the flight control system and the actuator is ignored.
3.4.2. Longitudinal PN Law
3.4.3. Lateral PN Law
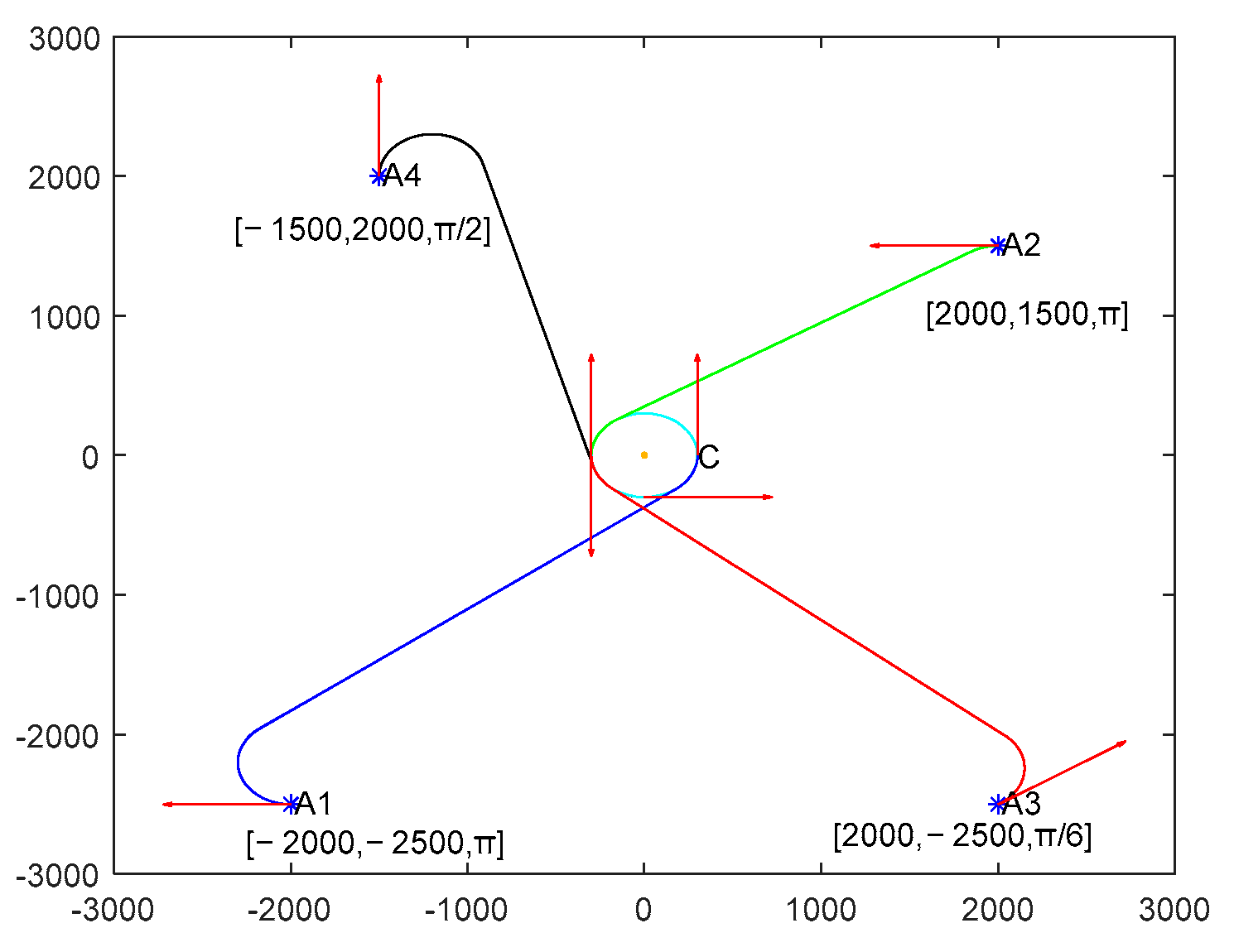
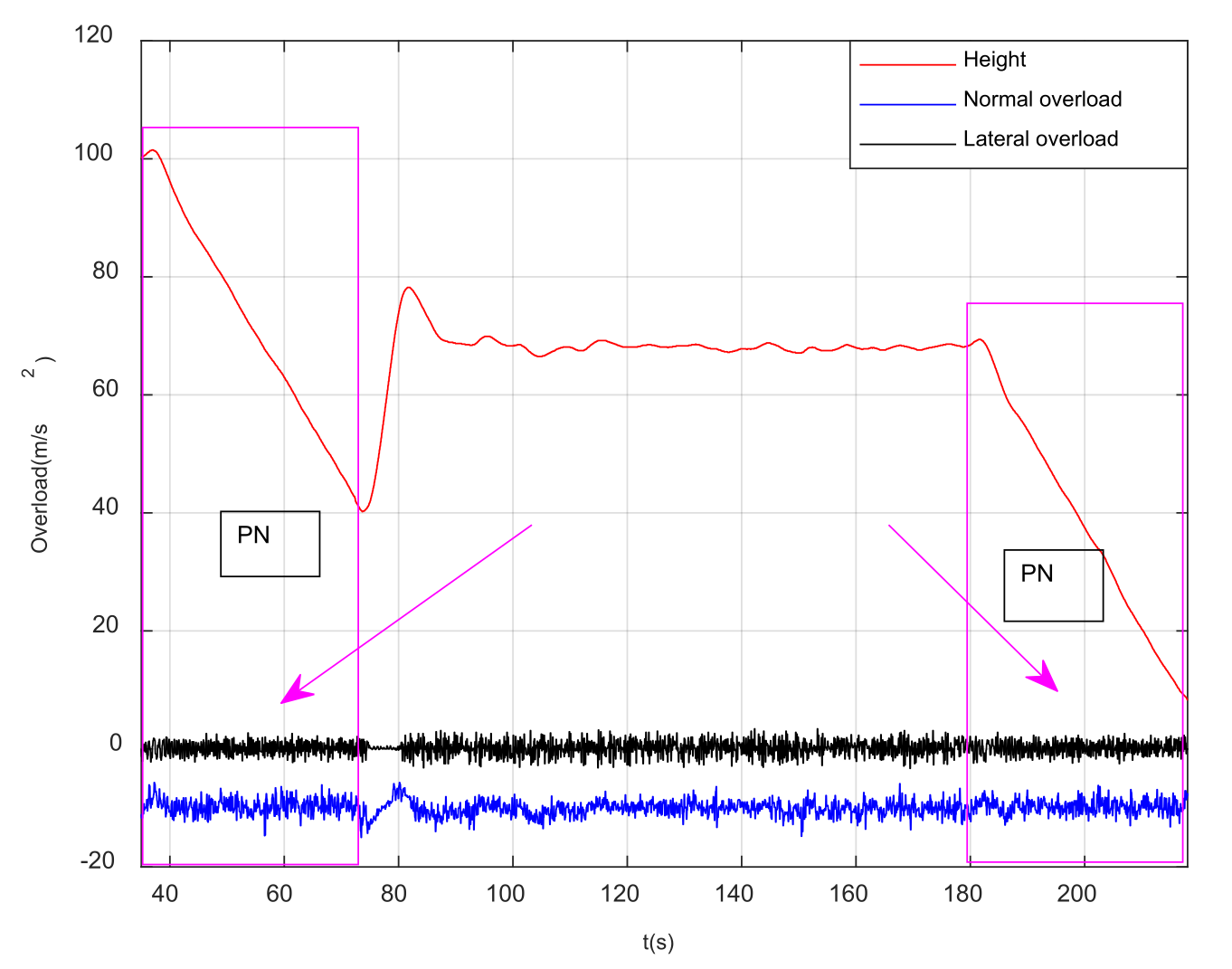
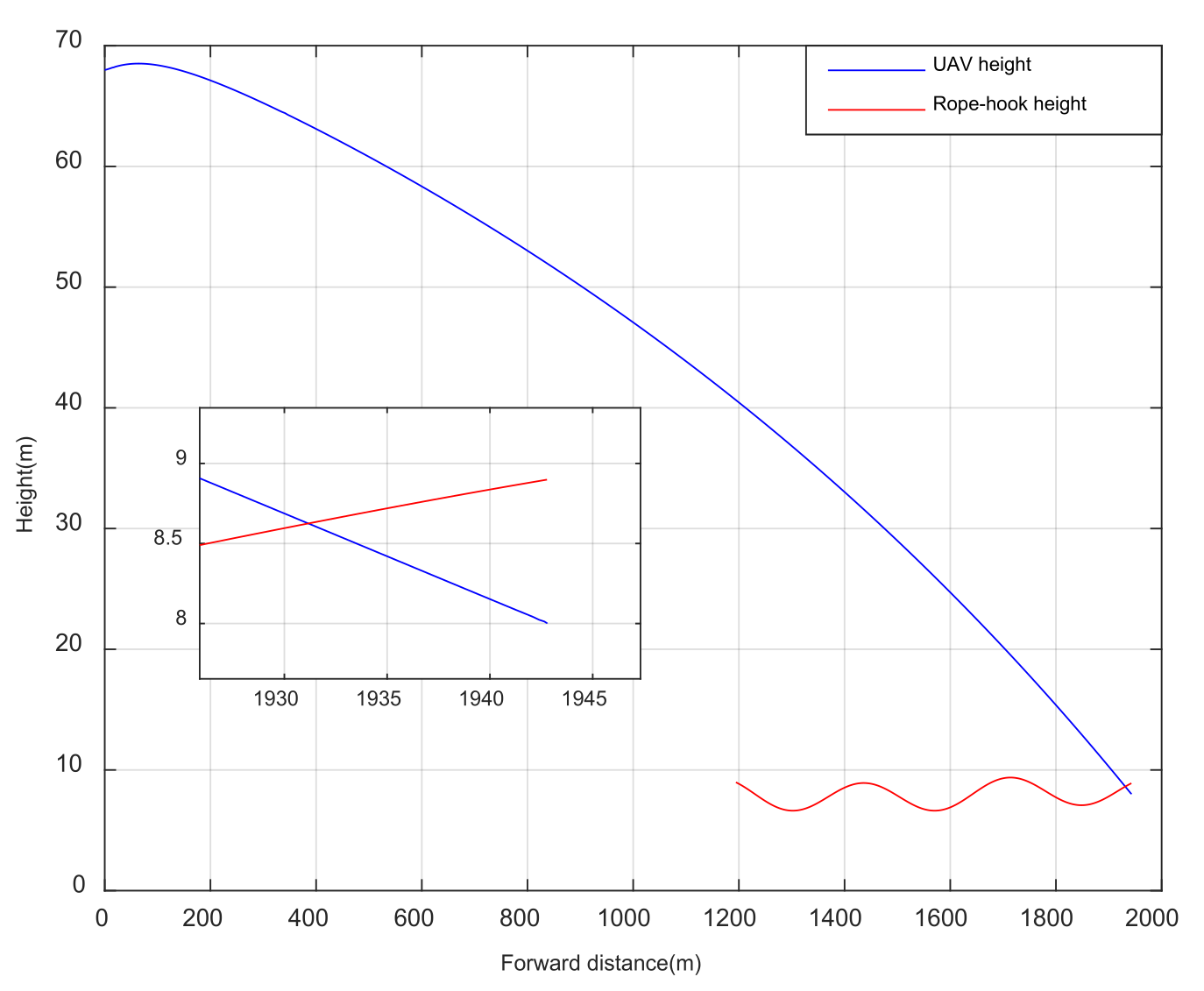
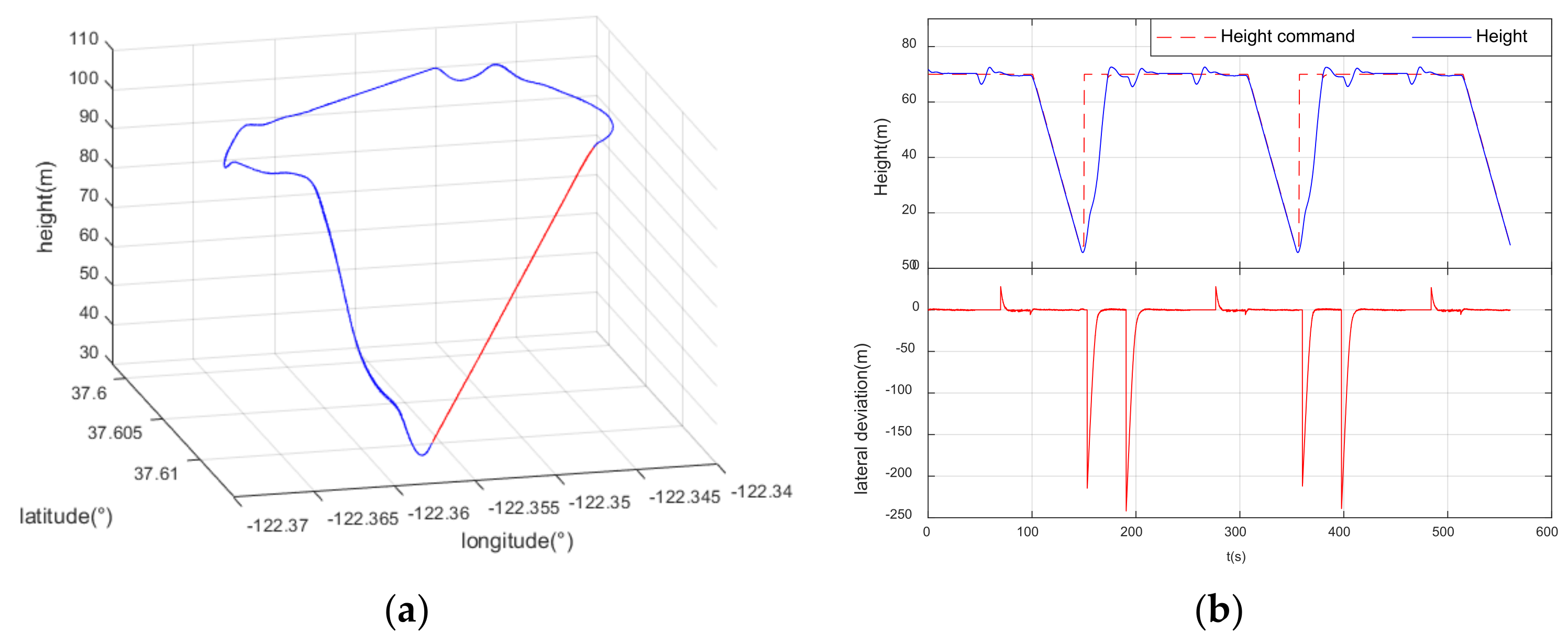
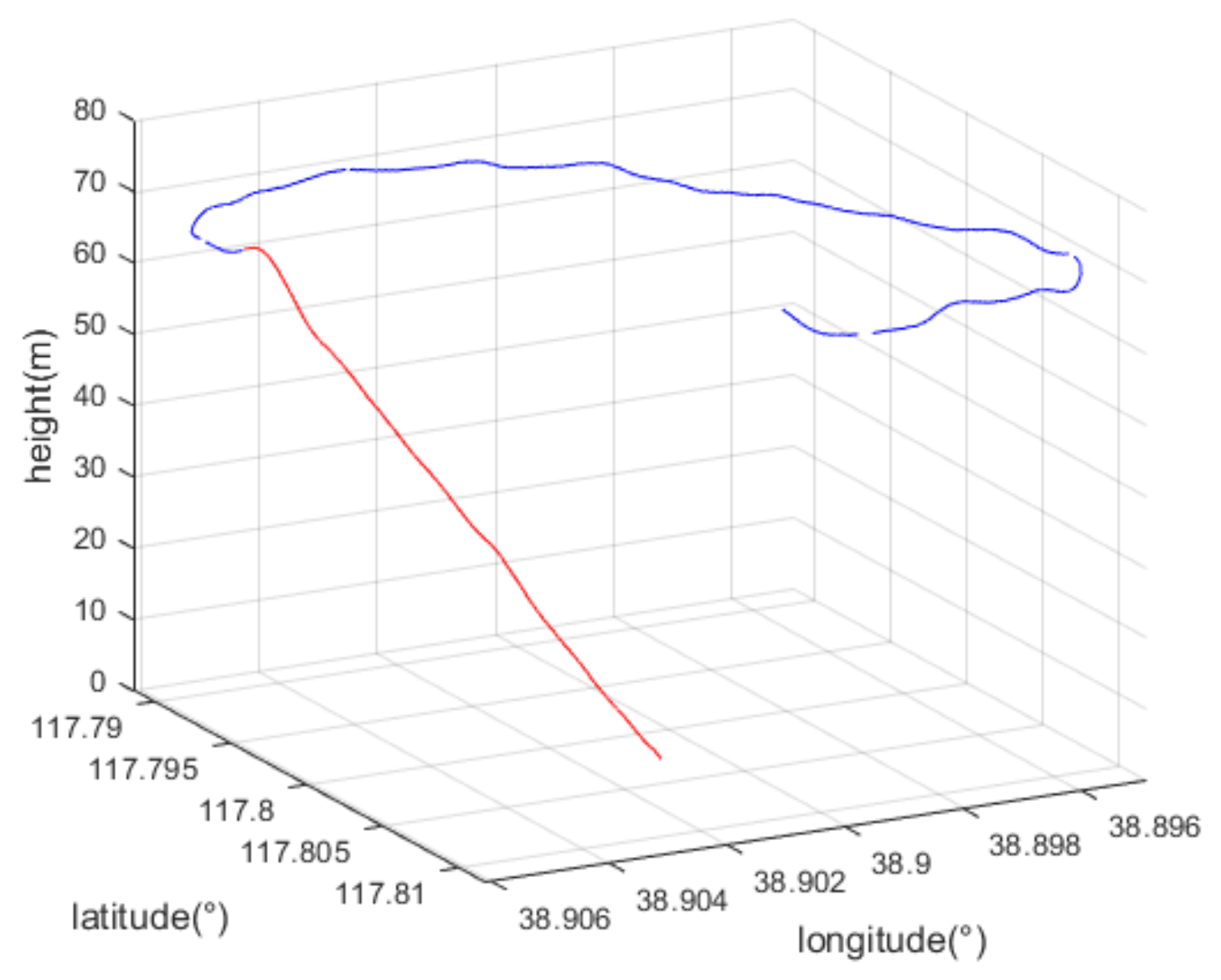
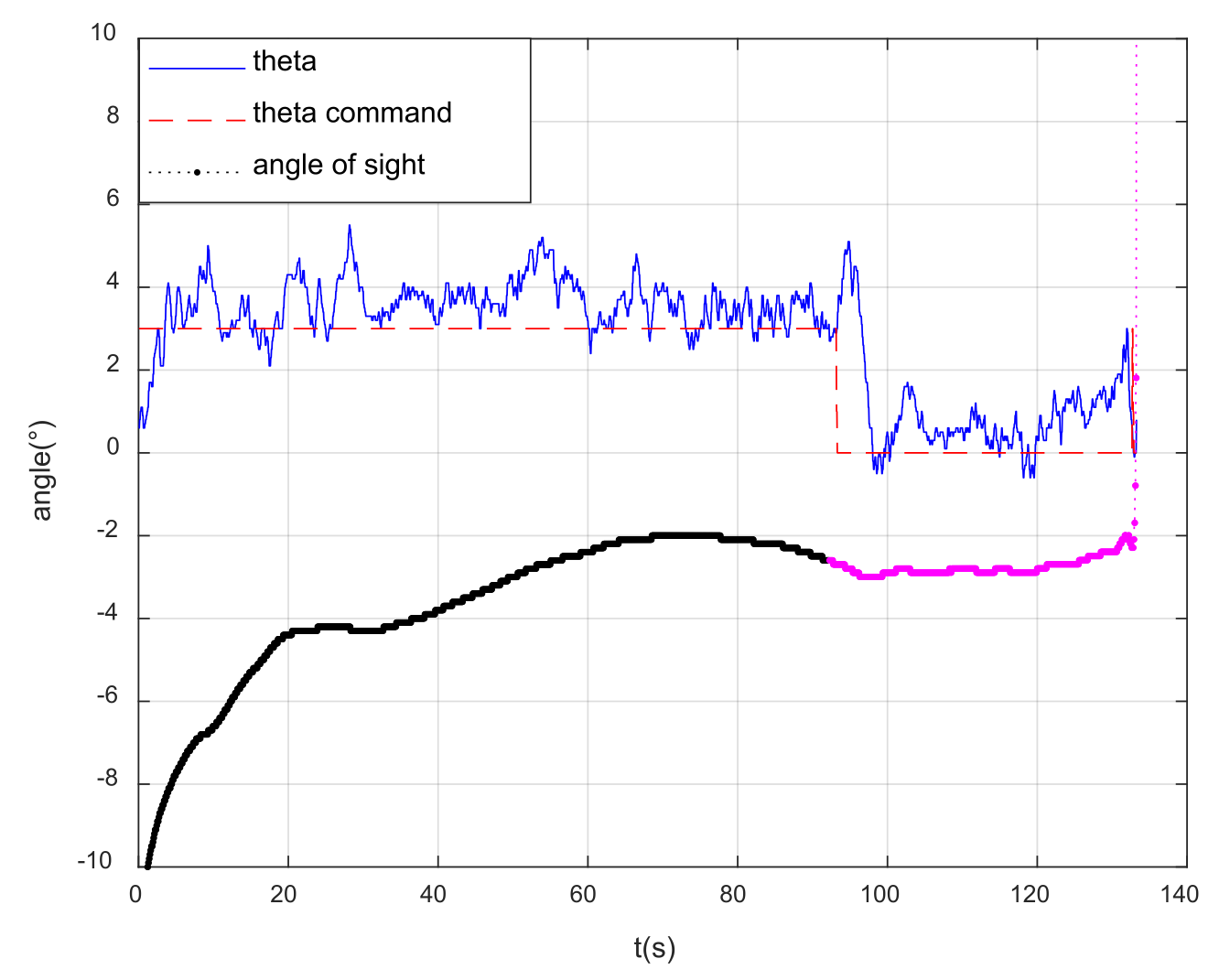
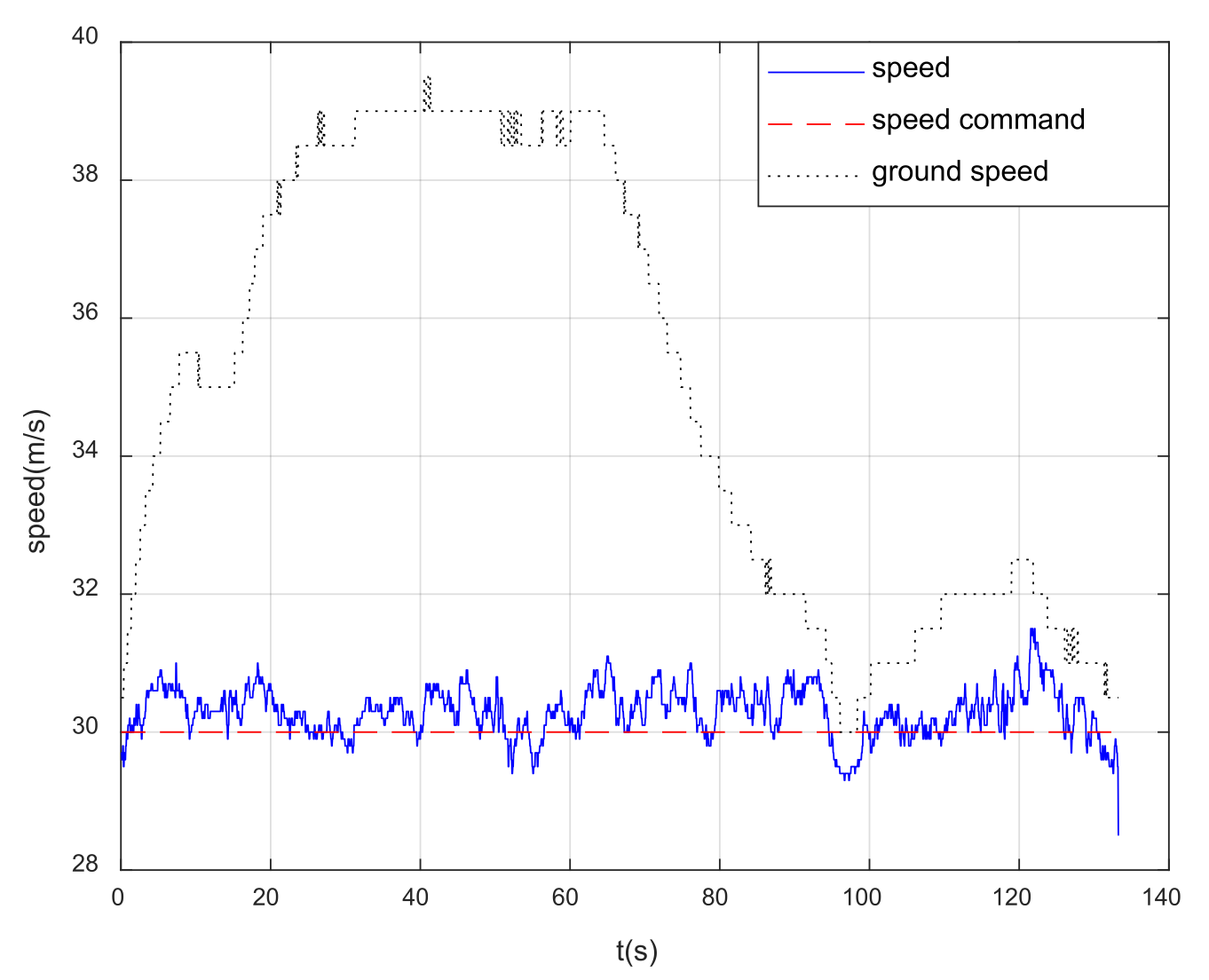
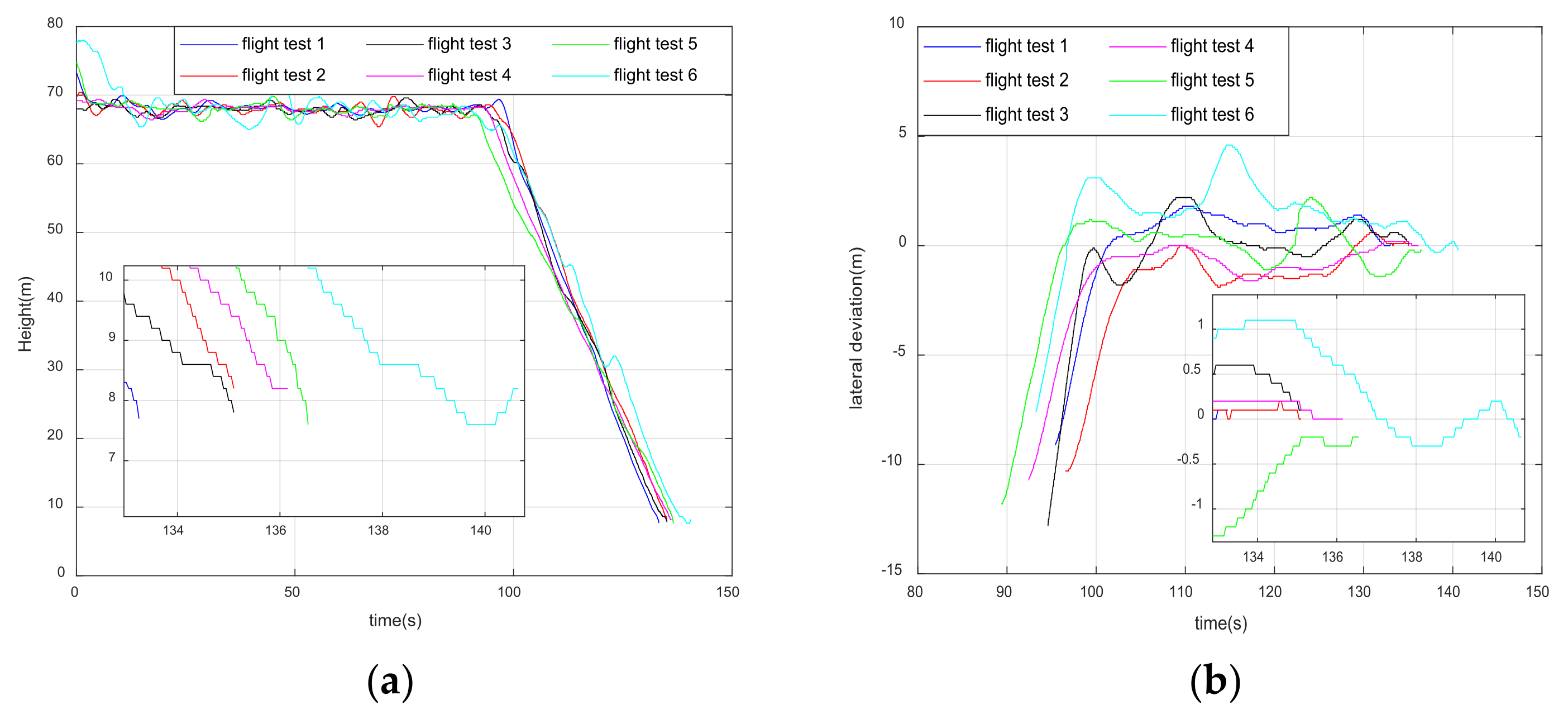
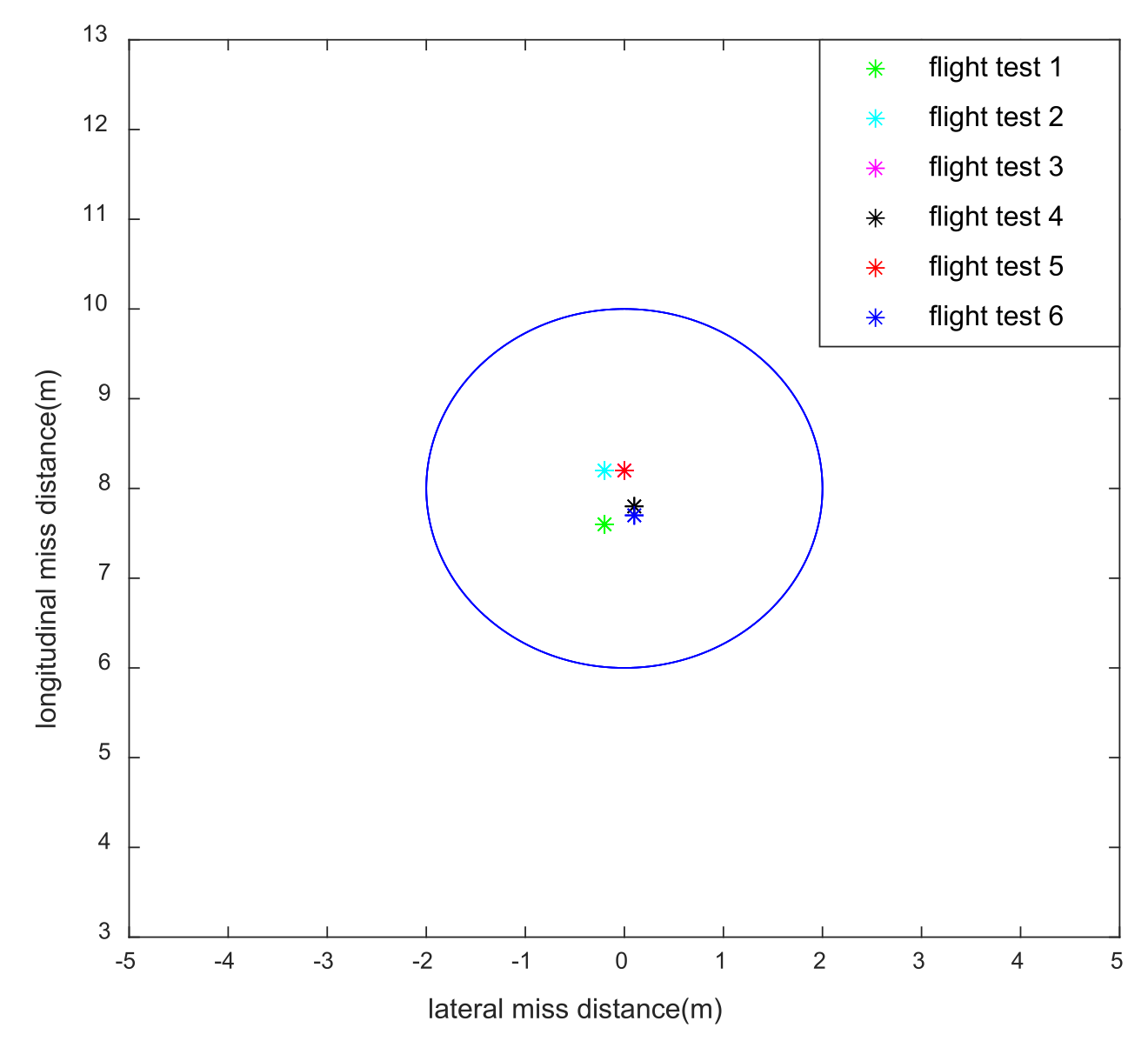
4. Results: Simulation and Flight Test
5. Discussion
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
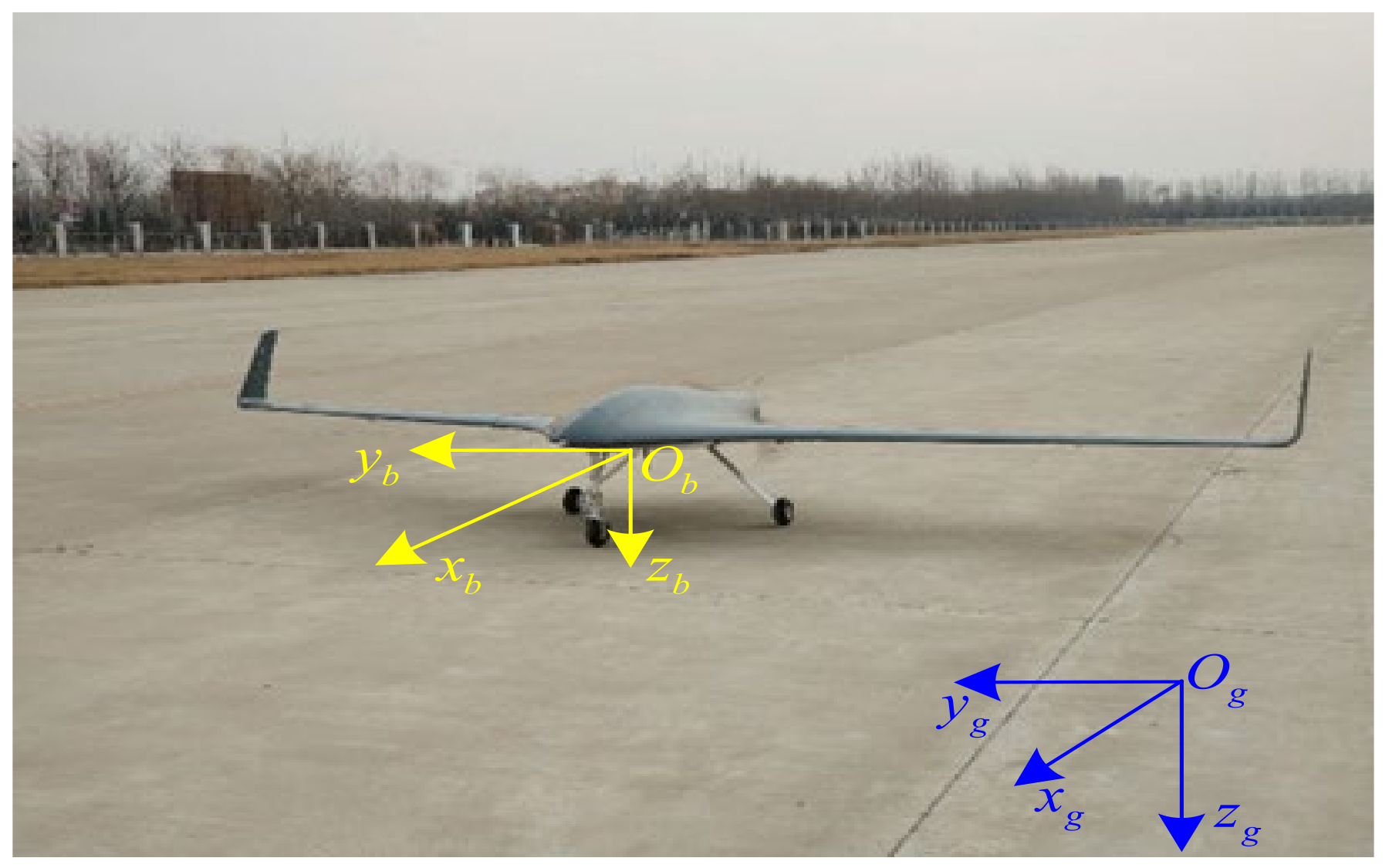
| aircraft-body coordinate frame, attached to the center of gravity of UVA | |
| earth-surface inertial reference frame | |
| position of rope-hook in frame | |
| position of the center of the circle in frame | |
| angle between rope-hook heading and the line of the rope-hook and the center of the spiral circle | |
| angle between rope-hook velocity vector and the x axis | |
| spiral radius | |
| distance between the cutting point of the spiral circle and the rope-hook | |
| distance to go | |
| pitch angle, roll angle, yaw angle | |
| pitch angle command, roll angle command, yaw angle command | |
| pitch angle rate, roll angle rate, yaw angle rate | |
| pitch angle rate command, roll angle rate command | |
| the deflection angle command of elevator, aileron and rudder, respectively | |
| disturbance estimation of the pitch rate loop and roll rate loop, respectively | |
| height of UAV and height command | |
| speed and speed command | |
| throttle opening command | |
| longitudinal line-of-sight angle, initial longitudinal line-of-sight angle | |
| lateral line-of-sight angle, lateral line-of-sight angle command | |
| flight path angle, initial flight path angle, flight path angle command | |
| cross-track error and cross-track error command | |
| proportional gain of pitch angle rate loop | |
| proportional gain of roll angle rate loop | |
| proportional gain of yaw angle rate loop | |
| control input gain from the elevator to pitch angle rate | |
| control input gain from the aileron to roll angle rate | |
| moment of inertia about the x axis and the y axis | |
| dynamic pressure | |
| effective elevator surface area | |
| coefficient of pitching moment and rolling moment | |
| observer bandwidth of roll and pitch angle rate loop, respectively | |
| energy rate distribution and energy rate distribution error | |
| total energy rate and total energy rate error | |
| proportional gain and integral gain of the rate of change in height | |
| throttle proportional and integral gains, respectively. | |
| pitch angle distribution proportional and integral gains, respectively | |
| acceleration command sideways | |
| distance from UAV position to a reference point on a desired trajectory | |
| lateral deviation adjustment time | |
| damping ratio and natural frequency of the lateral deviation system | |
| angular rate of UAV velocity vector | |
| guidance ratio | |
| target velocity vector | |
| proportional gain and integral gain of the flight path angle loop | |
| lateral overload (lateral acceleration) | |
| proportional gain and integral gain of the line-of-sight angle loop |
References
- Wang, X.; Chen, X.; Li, J.G. Summary on control technology of automatic carrier landing for flying wing unmanned aerial vehicle. Inf. Technol. Netw. Secur. 2017, 36, 7–9. [Google Scholar]
- Li, Q.; Zhang, S.L.; Meng, W.G. Surveys of Carrier Landing Techniques for UAVs. Unmanned Syst. Technol. 2018, 1, 49–54. [Google Scholar]
- Zhen, Z.Y. Research Development in Autonomous Carrier-Landing/Ship-Recovery Guidance and Control of Unmanned Aerial Vehicles. Acta Autom. Sin. 2019, 45, 669–681. [Google Scholar]
- Liang, L.; Xiao, J.; Deng, Y.C. Research and Development Trend of Carrier Landing Technology of UAV. J. Xi’an Univ. Aeronaut. 2020, 38, 23–28. [Google Scholar]
- Hong, D.; Zhou, L.; Zheng, Z.S. Research on equipment recovery technology development of foreign small shipborne fixed wing UAV. J. Aerodyn. Missile 2014, 4, 50–54. [Google Scholar]
- Bornebusch, M.F.; Johansen, T.A. Autonomous recovery of a Fixed-wing UAV Using a Line Suspended Between Two Multirotor UAVs. IEEE Trans. Aerosp. Electron. Syst. 2020, 57, 90–104. [Google Scholar] [CrossRef]
- Pei, J.H.; He, C.; Wang, T. Dynamics Analysis of Rope Hook Recovery System for Fixed Wing UAV. J. Nanjing Univ. Aeronaut. Astronaut. 2017, 49, 693–698. [Google Scholar]
- Hu, T. Research on Simulation Technology of UAV SideArm Fixed Track Arresting. Master’s Thesis, Nanjing University of Aeronautics and Astronautics, Nanjing, China, 2020. [Google Scholar]
- Yang, L.Q.; Zhen, Z.Y.; Xing, D.J. Automatic carrier landing adaptive control system design of carrier-based UAV. Flight Dyn. 2018, 36, 36–39. [Google Scholar]
- Zhen, Z.Y.; Tao, G.; Yu, C.H. A multivariable adaptive control scheme for automatic carrier landing of UAV. Aerosp. Sci. Technol. 2019, 92, 714–721. [Google Scholar] [CrossRef]
- Li, K. The Research of Ejection Takeoff and Skyhook Technology of Small UAVs. Master’s Thesis, Nanjing University of Aeronautics and Astronautics, Nanjing, China, 2019. [Google Scholar]
- Xi, J.C. Sky Hook Recycling Strategy Design and Software Development of a Type of UAVs. Master’s Thesis, Nanjing University of Aeronautics and Astronautics, Nanjing, China, 2020. [Google Scholar]
- Liu, C.G.; Chen, X.; Li, C.T. Controller Design of Dynamic Net Recovery for UAV. Electron. Opt. Control 2016, 23, 64–69. [Google Scholar]
- Zhang, M.X.; Li, Q.; Chu, L.L.; Du, X.T.; He, Y.Q. Rope-hook recovery system of fixed wing UAV based on FFT prediction algorithm and adaptive fuzzy PID control. In Proceedings of the 2020 IEEE International Conference on Mechatronics and Automation (ICMA), Beijing, China, 13–16 October 2020. [Google Scholar]
- Yoon, S.; Kim, H.J.; Kim, Y. Spiral Landing Trajectory and Pursuit Guidance Law Design for Vision-Based Net-Recovery UAV. In Proceedings of the AIAA Guidance, Navigation, and Control Conference, Chicago, IL, USA, 10–13 August 2009. [Google Scholar]
- Wang, K.; Sun, C.Z.; Jiang, Y. Research on Adaptive Guidance Technology of UAV Ship Landing System Based on Net Recovery. Proc. Eng. 2015, 99, 1027–1034. [Google Scholar]
- Yang, N.; Zhang, D.J.; Wu, L.N. A kind of moving net recovery technology for unmanned aerial vehicle. In Proceedings of the International Conference on Information and Communications Technologies, Xi’an, China, 24–26 April 2016. [Google Scholar]
- Liu, Q.; Yuan, S.Z. Research on total energy and H∞control in longitudinal carrier landing for unmanned aerial vehicle. J. Yanshan Univ. 2010, 34, 269–273. [Google Scholar]
- Yu, Y.; Wang, H.L.; Li, N. Automatic carrier landing system based on active disturbance rejection control with a novel parameters optimizer. Aerosp. Sci. Technol. 2017, 69, 149–160. [Google Scholar] [CrossRef]
- Zhao, D.H.; Li, C.H.; Zhang, X.W. Longitudinal control law design of carrier UAV during carrier landing. Electron. Opt. Control 2018, 25, 43–48. [Google Scholar]
- Sashank, M.; Satadal, G.; Sujit, P.B. Sliding Mode-Based Guidance for UAV Landing on a Stationary or Moving Ground Vehicle. IFAC-Papers OnLine 2020, 53, 453–458. [Google Scholar]
- Liu, H.Y.; Tian, Z.G.; Xu, C. Research on Autonomous Landing Technology of Ship-Borne Drones in Flying Wing Layout. Comput. Simul. 2020, 37, 38–43. [Google Scholar]
- Wang, S.S.; Li, C.T.; Wang, Z. Design of carrier landing controller based on adaptive dynamic inversion. Syst. Eng. Electron. 2021, 39, 55–63. [Google Scholar]
- Attar, M.; Wahnon, E.; Chaimovitz, D. Advanced flight control technologies for UAVs. In Proceedings of the 2nd AIAA “Unmanned Unlimited” Conference and Workshop & Exhibit, San Diego, CA, USA, 15–18 September 2003; p. 6537. [Google Scholar]
- Greg, L.; Steve, H. Landing Dispersion Results—Global Hawk Auto-land System. In Proceedings of the AIAA’s 1st Technical Conference and Workshop on Unmanned Aerospace Vehicles, Portsmouth, VA, USA, 23 May 2002. [Google Scholar]
- Wang, Y.X. Take-Off and Landing Control for Flying-Wing Unmanned Aerial Vehicle. Ph.D. Thesis, Northwestern Polytechnical University, Xi’an, China, 2017. [Google Scholar]
- Bruce, K.R. NASA B737 flight test results of the Total Energy Control System. In Proceedings of the AIAA Guidance, Navigation and Control Conference, Williamsburg, VA, USA, 17–19 August 1987. [Google Scholar]
- Faleiro, L.F.; Lambregts, A.A. Analysis and tuning of a ‘Total Energy Control System’ control law using eigenstructure assignment. Aerosp. Sci. Technol. 1999, 3, 127–140. [Google Scholar] [CrossRef]
- Lu, B.; Gao, B.; Wang, L.F. Research on the Total Energy Control System of Composite VTOL UAV Based on Px4. Dyn. Syst. Control 2020, 32, 99–108. [Google Scholar] [CrossRef]
- Park, S.; Deyst, J.; How, J. A New Nonlinear Guidance Logic for Trajectory Tracking. In Proceedings of the AIAA Guidance, Navigation and Control Conference and Exhibit, Providence, RI, USA, 16–19 August 2004. [Google Scholar]
- Chen, X.F.; Dong, Y.F. UAV Autonomous Landing Simulation Based on Matlab/Simulink. Fire Control Command Control 2014, 39, 55–63. [Google Scholar]
- Yang, Y.D. Guidance and Control of Unmanned Helicopter Ship Landing, 1st ed.; National Defense Industry Press: Beijing, China, 2013; pp. 154–196. [Google Scholar]








| Specification | Value |
|---|---|
| Wingspan | 4.9 m |
| Wing area Maximum takeoff weight Mean aerodynamic chord | 2.9 m2 |
| 60 kg | |
| 0.49 m | |
| Cruising speed | 30 m/s |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Deng, Z.; Bing, F.; Guo, Z.; Wu, L. Rope-Hook Recovery Controller Designed for a Flying-Wing UAV. Aerospace 2021, 8, 384. https://doi.org/10.3390/aerospace8120384
Deng Z, Bing F, Guo Z, Wu L. Rope-Hook Recovery Controller Designed for a Flying-Wing UAV. Aerospace. 2021; 8(12):384. https://doi.org/10.3390/aerospace8120384
Chicago/Turabian StyleDeng, Zhao, Fuqiang Bing, Zhiming Guo, and Liaoni Wu. 2021. "Rope-Hook Recovery Controller Designed for a Flying-Wing UAV" Aerospace 8, no. 12: 384. https://doi.org/10.3390/aerospace8120384
APA StyleDeng, Z., Bing, F., Guo, Z., & Wu, L. (2021). Rope-Hook Recovery Controller Designed for a Flying-Wing UAV. Aerospace, 8(12), 384. https://doi.org/10.3390/aerospace8120384






