Simulation of a GOx-GCH4 Rocket Combustor and the Effect of the GEKO Turbulence Model Coefficients
Abstract
:1. Introduction
2. Modelling Object and Methods
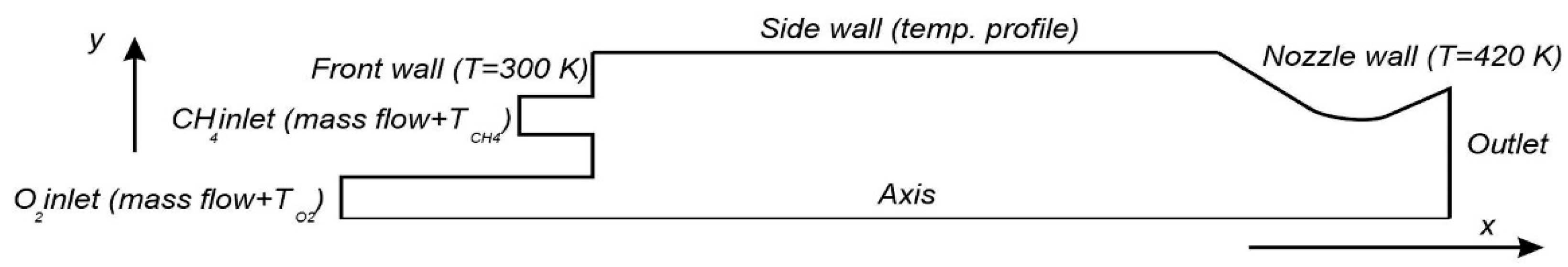
2.1. Object of Modelling
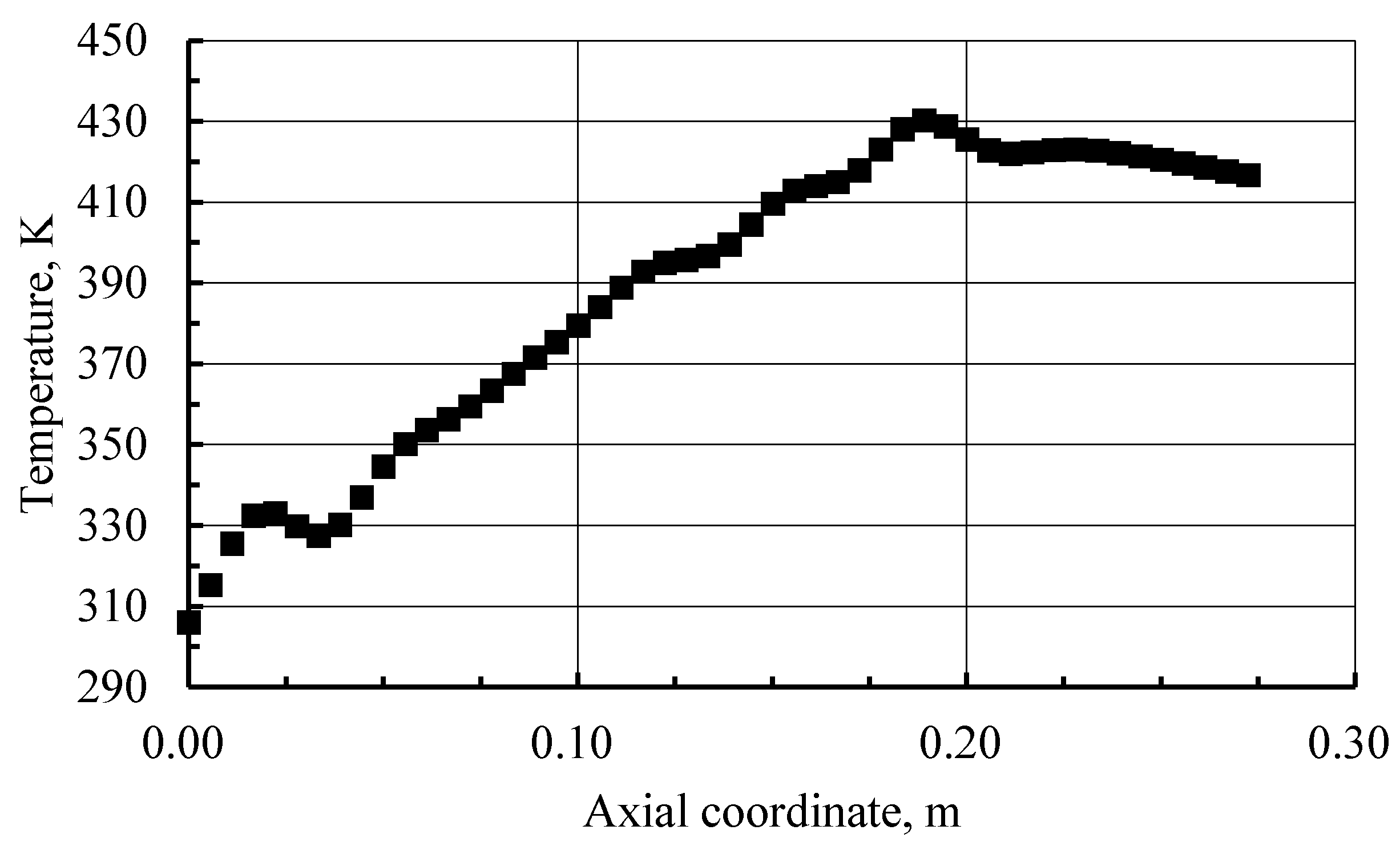
2.2. Boundary Conditions
2.3. Numerical Setup
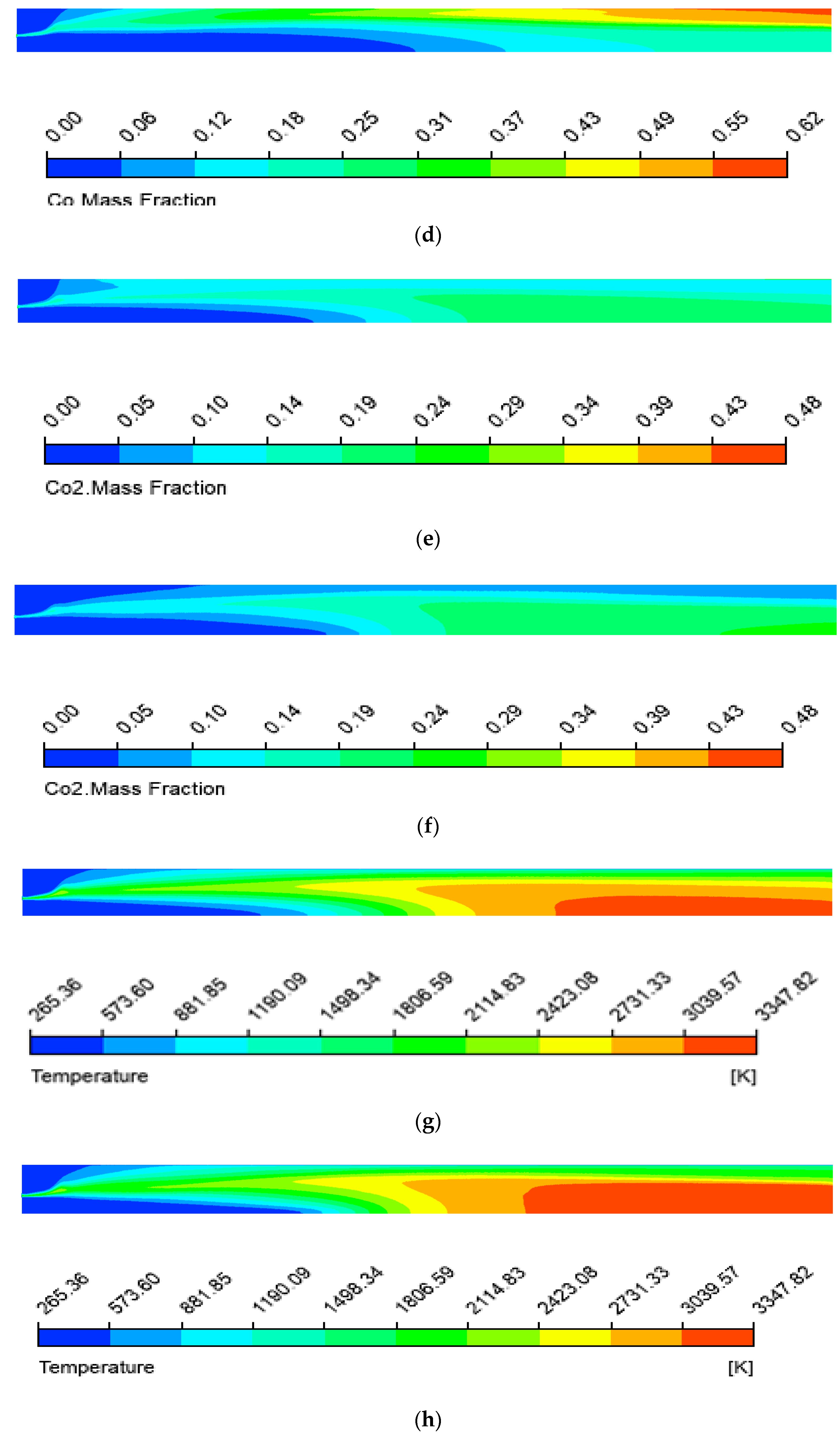
3. Discussion and Results
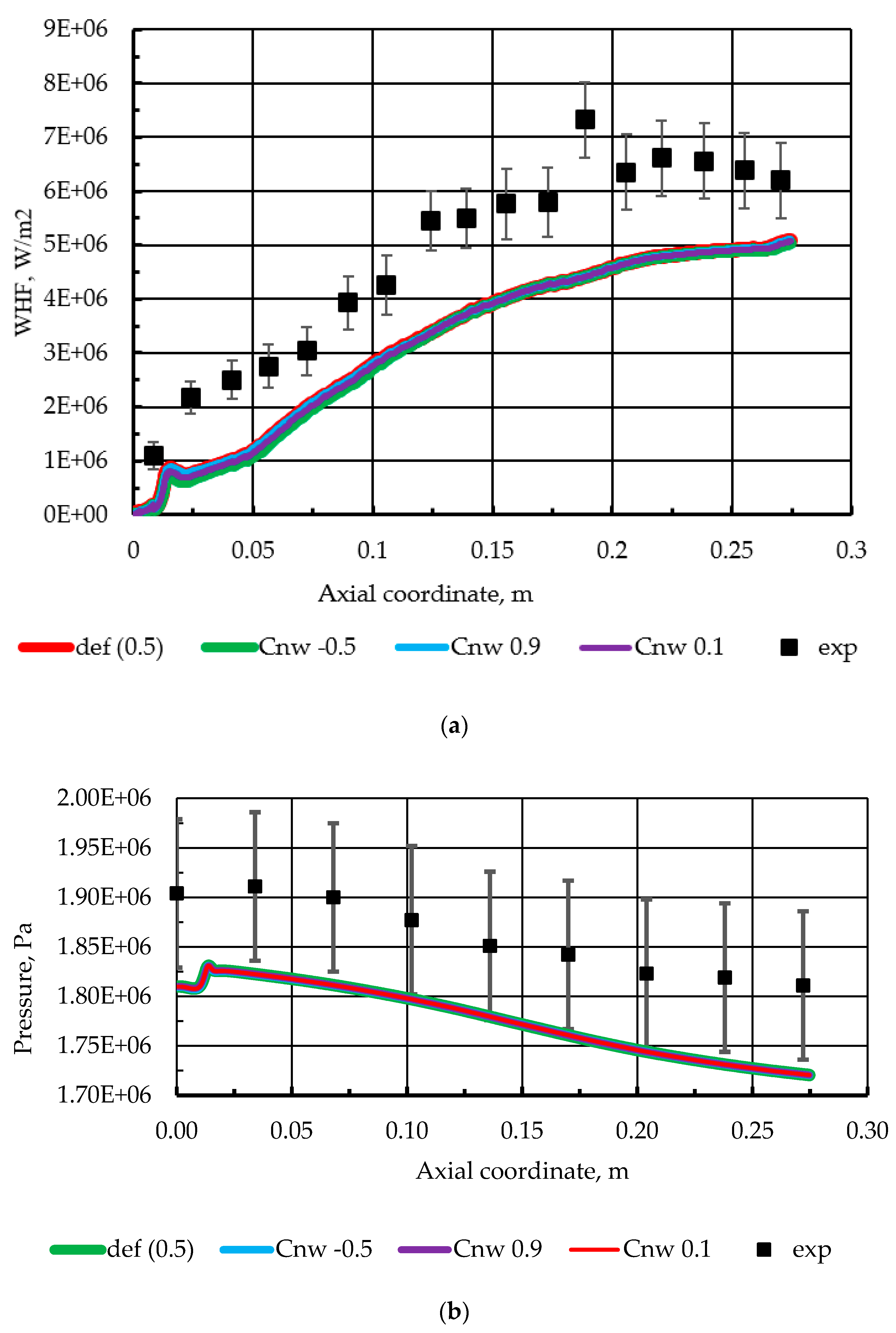
3.1. Effect of the “Near Wall” Parameter
3.2. Effect of the “Jet” Parameter
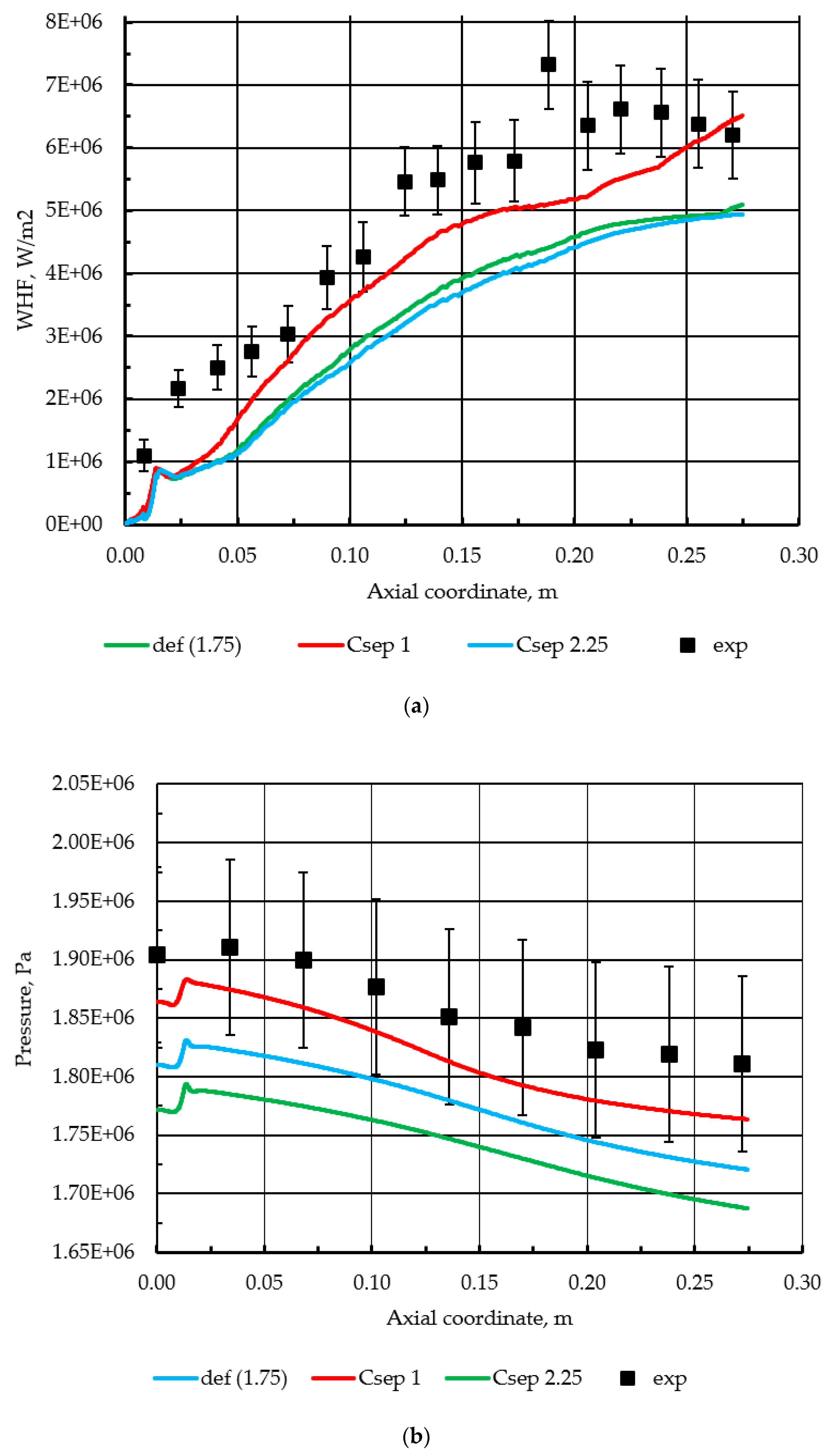
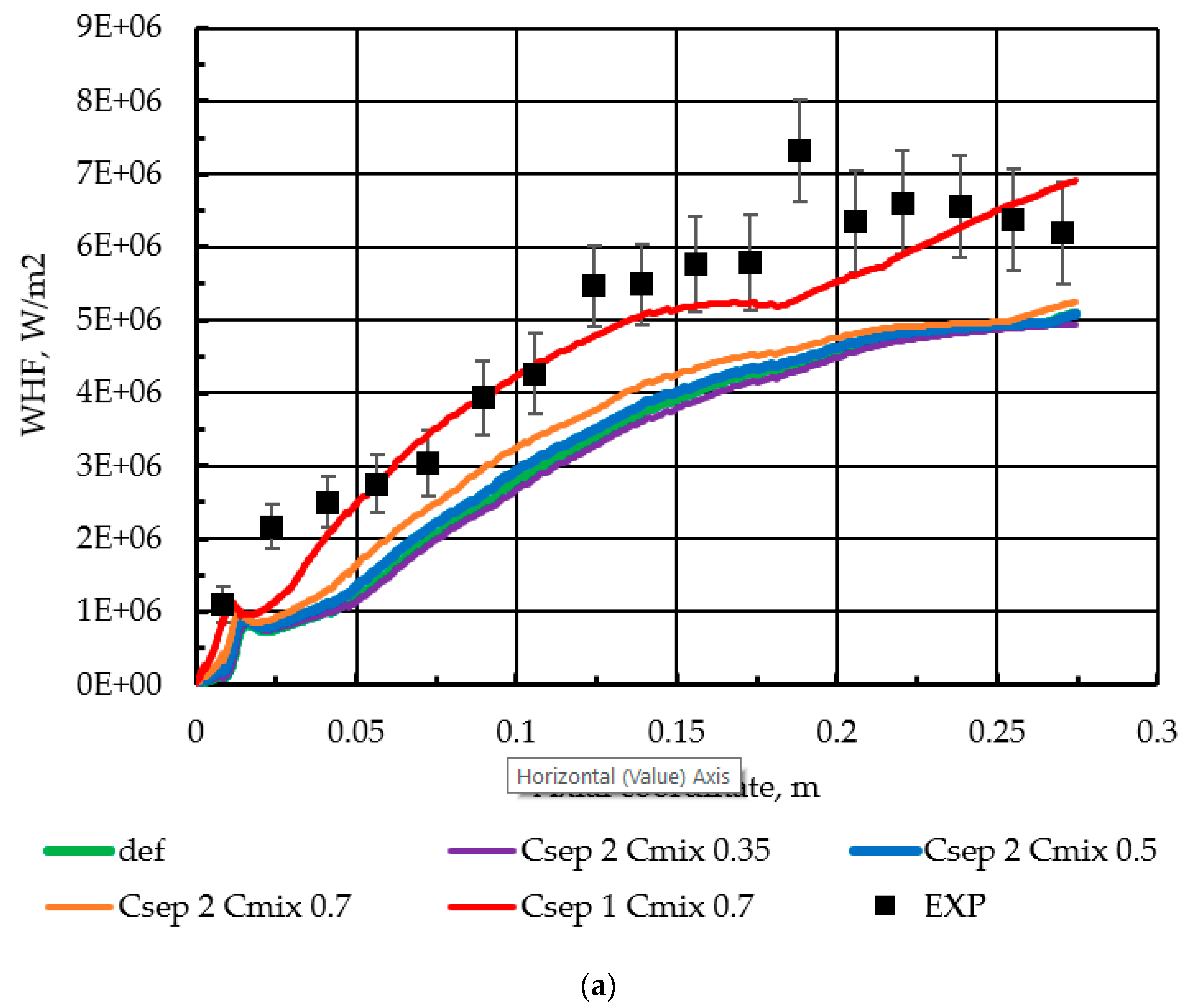
3.3. Effect of the “Separation” Parameter
3.4. Effect of the “Mixing” Parameter
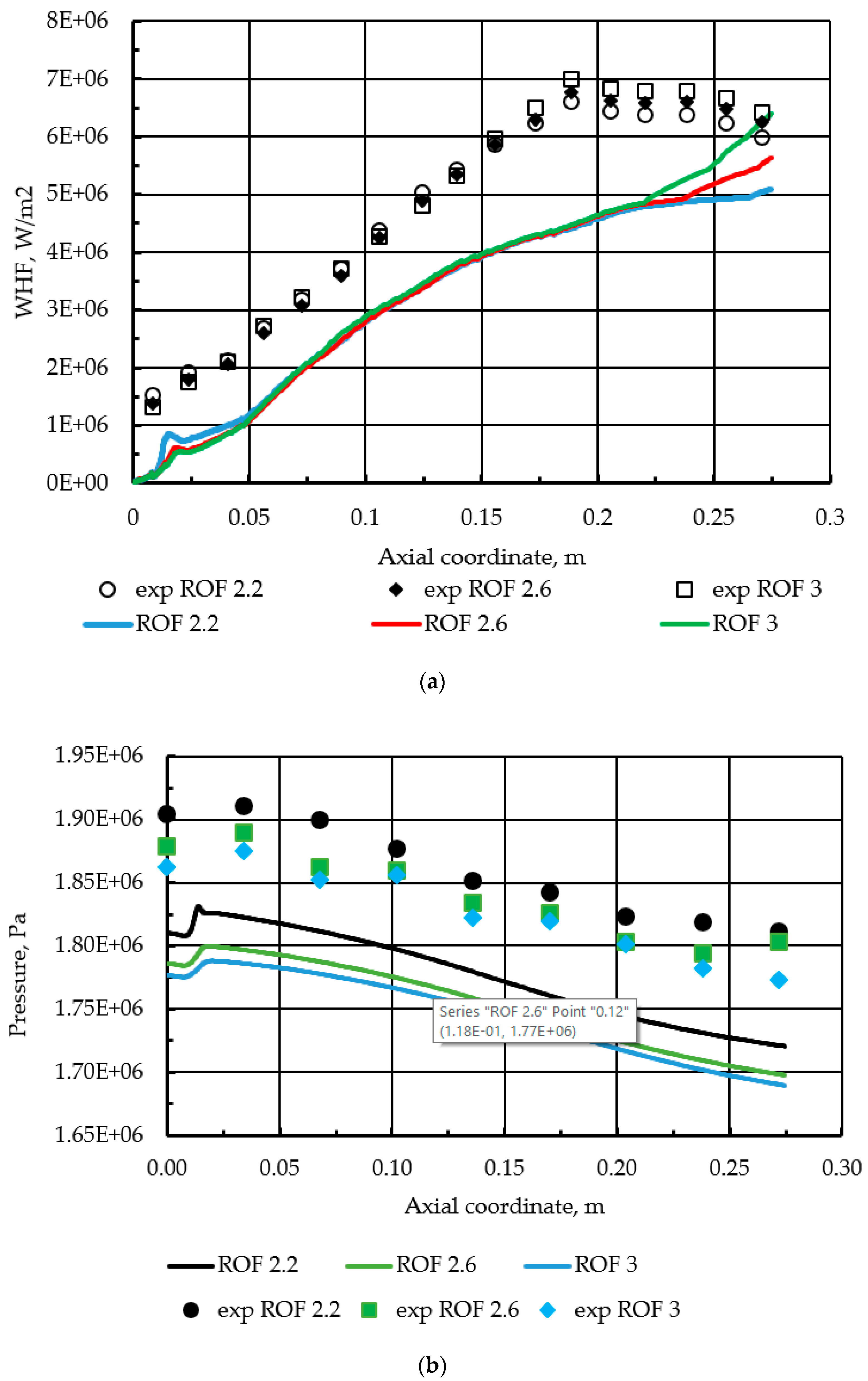
3.5. Performance of the GEKO Model at Different ROF
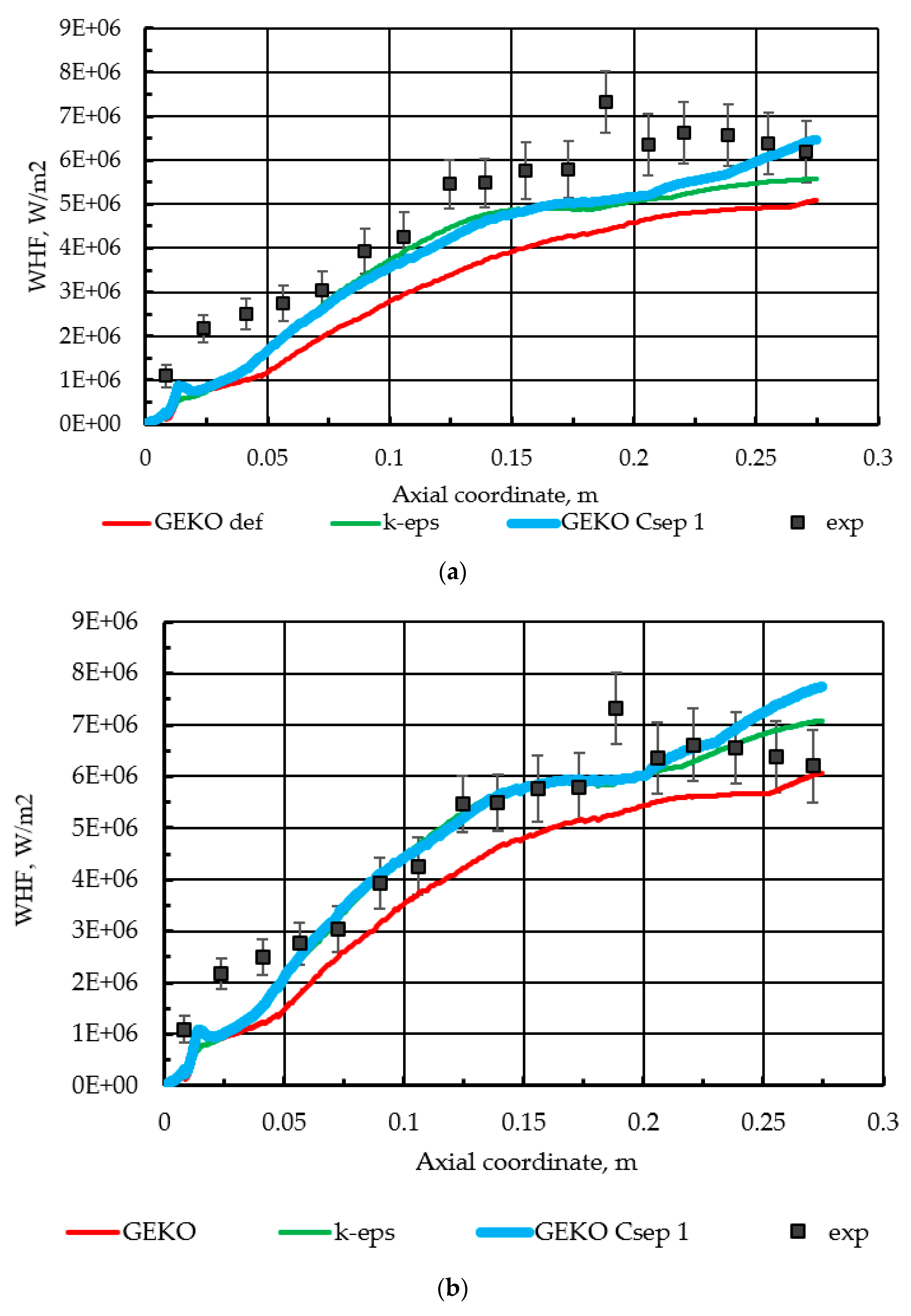
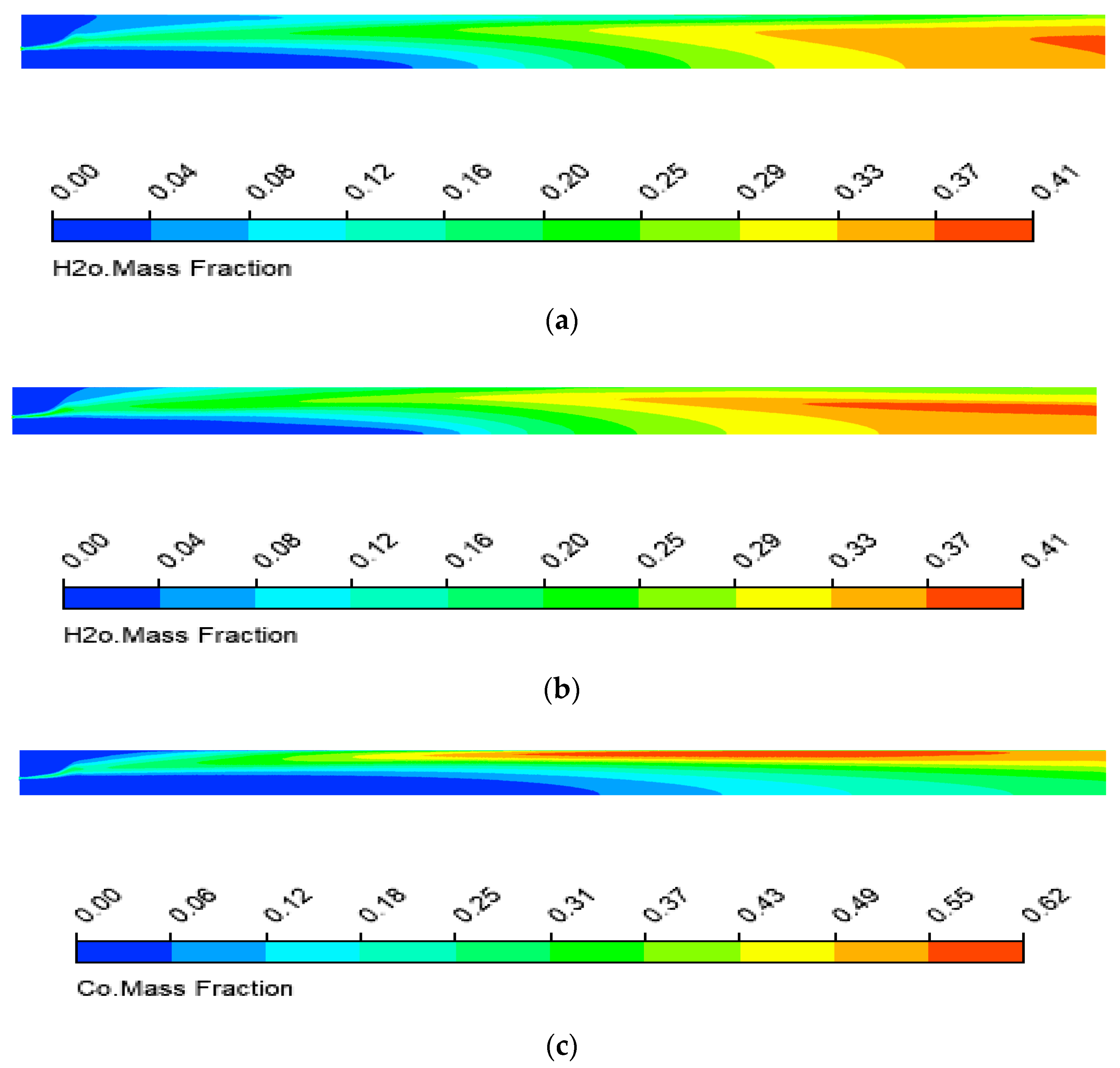
3.6. Effect of Detailed Kinetic Mechanism
4. Conclusions and Outlook
Author Contributions
Funding
Conflicts of Interest
References
- Perakis, N.; Rahn, D.; Haidn, O.J.; Eiringhaus, D. Heat Transfer and Combustion Simulation of Seven-Element O2/CH4 Rocket Combustor. J. Propuls. Power 2019, 35, 1080–1097. [Google Scholar] [CrossRef]
- Perakis, N.; Strauss, J.; Haidn, O. Heat flux evaluation in a multi-element CH4/O2 rocket combustor using an inverse heat transfer method. Int. J. Heat Mass Transf. 2019, 142, 118425. [Google Scholar] [CrossRef]
- Perakis, N.; Haidn, O. Wall heat transfer prediction in CH4/O2 and H2/O2 rocket thrust chambers using a non-adiabatic flamelet model. Acta Astronaut. 2020, 174, 254–269. [Google Scholar] [CrossRef]
- Zhukov, V.; van Schyndel, J.; Saulo, G. Simulation of Single-Injector Methane Rocket Combustor Using Different Numerical Codes. In Proceedings of the 32nd International Symposium on Space Technology and Science, Fukui, Japan, 15–21 June 2019. [Google Scholar]
- Zhukov, V.; Kong, A. A Compact Reaction Mechanism of Methane Oxidation at High Pressures. Prog. React. Kinet. Mech. 2018, 43, 62–78. [Google Scholar] [CrossRef] [Green Version]
- Roth, C.; Perakis, N.; Haidn, O.J. Modeling combustion and heat transfer in a single-element GCH4/GOX rocket combustor. In Proceedings of the 7th International Conference on Fluid Flow, Heat and Mass Transfer (FFHMT’20), Virtual Conference, 15–17 November 2020; pp. 178-1–178-8. [Google Scholar] [CrossRef]
- Maestro, D.; Cuenot, B.; Chemnitz, A.; Sattelmayer, T.; Roth, C.; Haidn, O.J.; Daimon, Y.; Keller, R.; Gerlinger, P.M.; Frank, G.; et al. Numerical Investigation of Flow and Combustion in a Single-Element GCH4/GOX Rocket Combustor: Chemistry Modeling and Turbulence-Combustion Interaction. In Proceedings of the 52nd AIAA/SAE/ASEE Joint Propulsion Conference, Salt Lake City, UT, USA, 25–27 July 2016. [Google Scholar]
- Perakis, N.; Haidn, O.; Eiringhaus, D.; Rahn, D.; Zhang, S.; Daimon, Y.; Karl, S.; Horchler, T. Qualitative and Quantitative Comparison of RANS Simulation Results for a 7-Element GOX/GCH4 Rocket Combustor. In Proceedings of the 54th AIAA/SAE/ASEE Joint Propulsion Conference, Cincinnati, OH, USA, 9–11 July 2018; p. 54. [Google Scholar]
- Chemnitz, A.; Sattelmayer, T.; Roth, C.; Haidn, O.; Daimon, Y.; Keller, R.; Gerlinger, P.; Zips, J.; Pfitzner, M. Numerical Investigation of Reacting Flow in a Methane Rocket Combustor: Turbulence Modeling. J. Propuls. Power 2018, 34, 864–877. [Google Scholar] [CrossRef]
- Sternin, A.; Perakis, N.; Celano, M.; Tajmar, M.; Haidn, O. CFD-analysis of the effect of a cooling film on flow and heat transfer characteristics in a GCH4/GOX rocket combustion chamber. In Proceedings of the Space Propulsion Conference, Seville, Spain, 14–18 May 2018. [Google Scholar]
- Poschner, M.; Pfitzner, M. Real Gas CFD Simulation of Supercritical H2-LOX in the MASCOTTE Single Injector Combustor Using a Commercial CFD Code. In Proceedings of the 46th AIAA Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 7–10 January 2008. [Google Scholar]
- Zhukov, V. Computational Fluid Dynamics Simulations of a GO2/GH2 Single Element Combustor. J. Propuls. Power 2015, 31, 1707–1714. [Google Scholar] [CrossRef] [Green Version]
- De Giorgi, M.G.; Sciolti, A.; Ficarella, A. Application and Comparison of Different Combustion Models of High Pressure LOX/CH4 Jet Flames. Energies 2014, 7, 477–497. [Google Scholar] [CrossRef] [Green Version]
- Kirubakaran, V.; Bhatt, D. Effect of different turbulence model on prediction of lean blowout limit for micro gas turbine combustor. Adv. Mater. Process. Technol. 2020. [Google Scholar] [CrossRef]
- Yilmaz, H.; Cam, O.; Tangoz, S.; Yilmaz, L. Effect of different turbulence models on combustion and emission characteristics of hydrogen/air flames. Int. J. Hydrog. Energy 2017, 42, 25744–25755. [Google Scholar] [CrossRef]
- Jiang, L. A Critical Evaluation of Turbulence Modeling in a Model Combustor. In Proceedings of the ASME Turbo Expo 2012: Turbine Technical Conference and Exposition, Volume 1: Aircraft Engine; Ceramics; Coal, Biomass and Alternative Fuels; Controls, Diagnostics and Instrumentation, Copenhagen, Denmark, 11–15 June 2012; pp. 535–545. [Google Scholar] [CrossRef]
- He, D.; Yu, Y.; Kuang, Y.; Wang, C. Model Comparisons of Flow and Chemical Kinetic Mechanisms for Methane–Air Combustion for Engineering Applications. Appl. Sci. 2021, 11, 4107. [Google Scholar] [CrossRef]
- Jiang, L.Y. RANS Modeling of Turbulence in Combustors. In Turbulence Modelling Approaches—Current State, Development Prospects, Applications; InTech: London, UK, 2017; Chapter 7. [Google Scholar]
- Zips, J.; Traxinger, C.; Breda, P.; Pfitzner, M. Assessment of presumed/transported probability density function methods for rocket combustion simulations. J. Propuls. Power 2019, 35, 1–19. [Google Scholar] [CrossRef]
- Zips, J.; Traxinger, C.; Pfitzner, M. Time-resolved flow field and thermal loads in a single-element GOx/GCH4 rocket combustor. Int. J. Heat Mass Transf. 2019, 143, 118474. [Google Scholar] [CrossRef]
- Perakis, N.; Haidn, O.J.; Ihme, M. Investigation of CO recombination in the boundary layer of CH4/O2 rocket engines. Proc. Combust. Inst. 2021, 38, 6403–6411. [Google Scholar] [CrossRef]
- Breda, P.; Pfitzner, M. Delayed detached eddy simulations with tabulated chemistry for thermal loads predictions. J. Propuls. Power 2021, 37, 29–46. [Google Scholar] [CrossRef]
- Indelicato, G.; Lapenna, P.E.; Remiddi, A.; Creta, F. An efficient modeling framework for wall heat flux prediction in rocket combustion chambers using non adiabatic flamelets and wall-functions. Int. J. Heat Mass Transf. 2021, 169, 120913. [Google Scholar] [CrossRef]
- Chung, W.T.; Mishra, A.A.; Perakis, N.; Ihme, M. Data-assisted combustion simulations with dynamic submodel assignment using random forests. Combust. Flame 2021, 227, 172–185. [Google Scholar] [CrossRef]
- Chung, W.T.; Mishra, A.A.; Perakis, N.; Ihme, M. Random forests for Accelerating Turbulent Combustion Simulations. In Proceedings of the Third Workshop on Machine Learning and the Physical Sciences (NeurIPS 2020), Vancouver, BC, Canada, 11 December 2020. [Google Scholar]
- Petersen, E.L.; Davidson, D.F.; Hanson, R.K. Combust. Flame 1999, 117, 272–290. [Google Scholar] [CrossRef]
- Roth, C.; Silvestri, S.; Perakis, N.; Haidn, O. Experimental and Numerical Investigation of Flow and Combustion in a Single Element Rocket Combustor using GH2/GOX and GCH4/GOX as Propellants. In Proceedings of the 31st International Symposium on Space Technology and Science, Matsuyama, Japan, 3–9 June 2017. [Google Scholar]
- Negishi, H.; Nakajima, T.; Keller, R.; Gerlinger, P. Heat Flux Estimation on a Chamber Wall of GH2/GOx and GCH4/GOx Single Element Rocket Combustors. In Proceedings of the 31st International Symposium on Space Technology and Science, Matsuyama, Japan, 3–9 June 2017. [Google Scholar]
- Daimon, Y.; Terashima, H.; Tani, H. Numerical Investigation on Effects of Recess Variation upon a GCH4/GOX Shear Coaxial Combustion Chamber. In Proceedings of the 31st International Symposium on Space Technology and Science, Matsuyama, Japan, 3–9 June 2017. [Google Scholar]
- Celano, M.; Silvestri, S.; Schlieben, G.; Kirchberger, C.; Knab, O.; Haidn, O. Transregio SFB-TR40—Test Case 1. Single Element Combustion Chamber GCH4/GOX. In Proceedings of the Space Propulsion, Cologne, Germany, 19–22 May 2014. [Google Scholar]
- Wilke, C. A Viscosity Equation for Gas Mixtures. J. Chem. Phys. 1950, 18, 517–519. [Google Scholar] [CrossRef]
- Mason, E.; Saxena, S. Approximate Formula for the Thermal Conductivity of Gas Mixtures. Phys. Fluids 1958, 1, 361–369. [Google Scholar] [CrossRef]
- Warnatz, J.; Maas, U.; Dibble, R. Combustion Physical and Chemical Fundamentals, Modeling and Simulations, Experiments, Pollutant Formation; Springer: Berlin/Heidelberg, Germany, 2001; pp. 1–350. [Google Scholar]
- Mcbride, B.; Gordon, S.; Reno, M. Coefficients for Calculating Thermodynamic and Transport Properties of Individual Species; NASA Technical Memorandum 4513; NASA: Wachington, DC, USA, 1993. [Google Scholar]
- Zhukov, V.; Borovik, I.; Strokach, E. Numerical Study of the Influence of Turbulent Diffusion Coefficients and Turbulent Prandtl Number on the Reactive Flow Simulation in a Combustor. Russ. Aeronaut. 2020, 63, 713–720. [Google Scholar] [CrossRef]
- Strokach, E.; Borovik, I.; Haidn, O. Simulation of the GOx/GCH4 Multi-Element Combustor Including the Effects of Radiation and Algebraic Variable Turbulent Prandtl Approaches. Energies 2020, 13, 5009. [Google Scholar] [CrossRef]
- Menter, F.; Lechner, R.; Matyushenko, A. Best Practice: Generalized K-Ω Two-Equation Turbulence Model in ANSYS CFD (GEKO); Technical Report ANSYS; ANSYS Inc.: Canonsburg, PA, USA, 2019. [Google Scholar]
- ANYS. ANYS Fluent Theory Guide; ANSYS Inc.: Canonsburg, PA, USA, 2019; Volume 15317, p. R2. [Google Scholar]
- Menter, F.R.; Matyushenko, A.; Lechner, R. Development of a Generalized K-ω Two-Equation Turbulence Model. In New Results in Numerical and Experimental Fluid Mechanics XII: DGLR 2018; Notes on Numerical Fluid Mechanics and Multidisciplinary Design; Dillmann, A., Heller, G., Krämer, E., Wagner, C., Tropea, C., Jakirlić, S., Eds.; Springer: Cham, Switzerland, 2020; Volume 142. [Google Scholar] [CrossRef]
- Zhukov, V. Extended Eddy-Dissipation Model for Modeling Hydrogen Rocket Combustors. Combust. Sci. Technol. 2020, 192, 531–546. [Google Scholar] [CrossRef] [Green Version]
- Leccese, G.; Bianchi, D.; Nasuti, F. Numerical Investigation on Radiative Heat Loads in Liquid Rocket Thrust Chambers. J. Propuls. Power 2019, 35, 930–943. [Google Scholar] [CrossRef]
- Thellmann, A. Impact of Gas Radiation on Viscous Flows, in Particular on Wall Heat Loads, in Hydrogen-Oxygen vs. Methane-Oxygen Systems, Based on the SSME Main Combustion Chamber. Ph.D. Thesis, Universitaet der Bundeswehr, Muenchen, Germany, 2010. [Google Scholar]
- Mazzei, L.; Puggelli, S.; Bertini, D.; Pampaloni, D.; Andreini, A. Modelling soot production and thermal radiation for turbulent diffusion flames. Energy Procedia 2017, 126, 826–833. [Google Scholar] [CrossRef]
- Poitou, D.; El Hafi, M.; Cuenot, B. Analysis of Radiation Modeling for Turbulent Combustion: Development of a Methodology to Couple Turbulent Combustion and Radiative Heat Transfer in LES. J. Heat Transf. 2011, 133, 062701. [Google Scholar] [CrossRef] [Green Version]
- Sternin, A.; Hao, M.; Liu, J.; Haidn, O.; Tajmar, M. Turbulence and Combustion and Film Prediction in Rocket Application via Parameter Adjustment, Model Variation and Deep Learning Method; Sonderforschungsbereich/Transregio 40–Summer Program Report: 2019; Technical University of Munich: Munich, Germany, 2019. [Google Scholar] [CrossRef]






| Criterion | Coarse (1st) | 2nd | 3rd | Fine (4th) |
|---|---|---|---|---|
| Num. of cells, mio. | 0.051 | 0.074 | 0.117 | 0.235 |
| Max. pressure, bar | 16.4 | 17.9 | 18.3 | 18.35 |
| Relative error, max pressure % | ---/--- | 8.379 | 2.19 | 0.28 |
| GCH4 Mass Flow, g/s | GO2 Mass Flow, kg/s | GCH4 Temperature, K | GO2 Temperature, K | |
|---|---|---|---|---|
| ROF 2.2 | 15.3 | 33.9 | 268 | 274 |
| ROF 2.6 | 13.3 | 35.6 | 269 | 275 |
| ROF 3.0 | 12.1 | 36.27 | 270 | 273 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Strokach, E.; Zhukov, V.; Borovik, I.; Sternin, A.; Haidn, O.J. Simulation of a GOx-GCH4 Rocket Combustor and the Effect of the GEKO Turbulence Model Coefficients. Aerospace 2021, 8, 341. https://doi.org/10.3390/aerospace8110341
Strokach E, Zhukov V, Borovik I, Sternin A, Haidn OJ. Simulation of a GOx-GCH4 Rocket Combustor and the Effect of the GEKO Turbulence Model Coefficients. Aerospace. 2021; 8(11):341. https://doi.org/10.3390/aerospace8110341
Chicago/Turabian StyleStrokach, Evgeny, Victor Zhukov, Igor Borovik, Andrej Sternin, and Oscar J. Haidn. 2021. "Simulation of a GOx-GCH4 Rocket Combustor and the Effect of the GEKO Turbulence Model Coefficients" Aerospace 8, no. 11: 341. https://doi.org/10.3390/aerospace8110341
APA StyleStrokach, E., Zhukov, V., Borovik, I., Sternin, A., & Haidn, O. J. (2021). Simulation of a GOx-GCH4 Rocket Combustor and the Effect of the GEKO Turbulence Model Coefficients. Aerospace, 8(11), 341. https://doi.org/10.3390/aerospace8110341






