Active Control of Laminar Separation: Simulations, Wind Tunnel, and Free-Flight Experiments
Abstract
1. Introduction
2. Investigative Methods
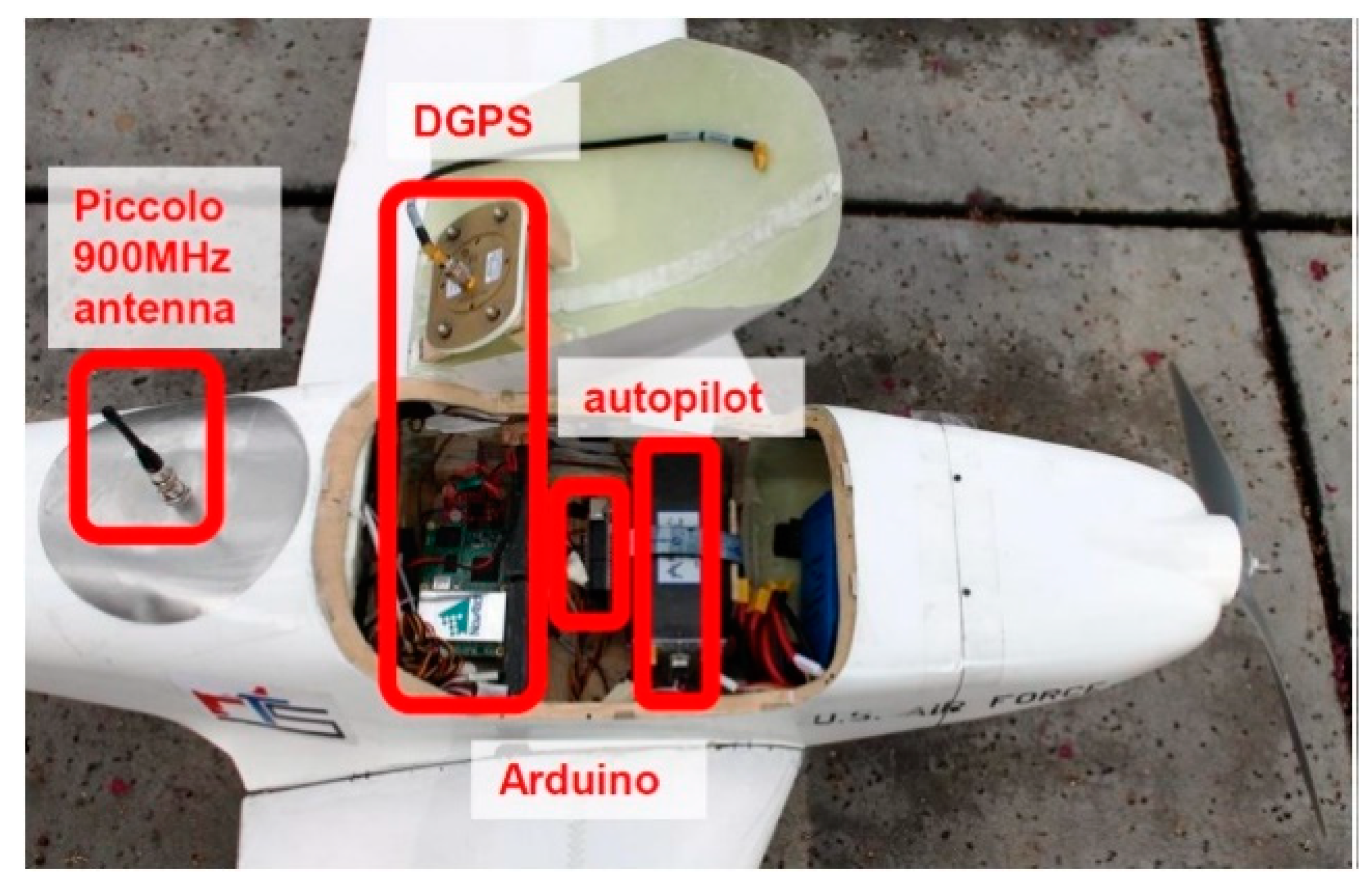
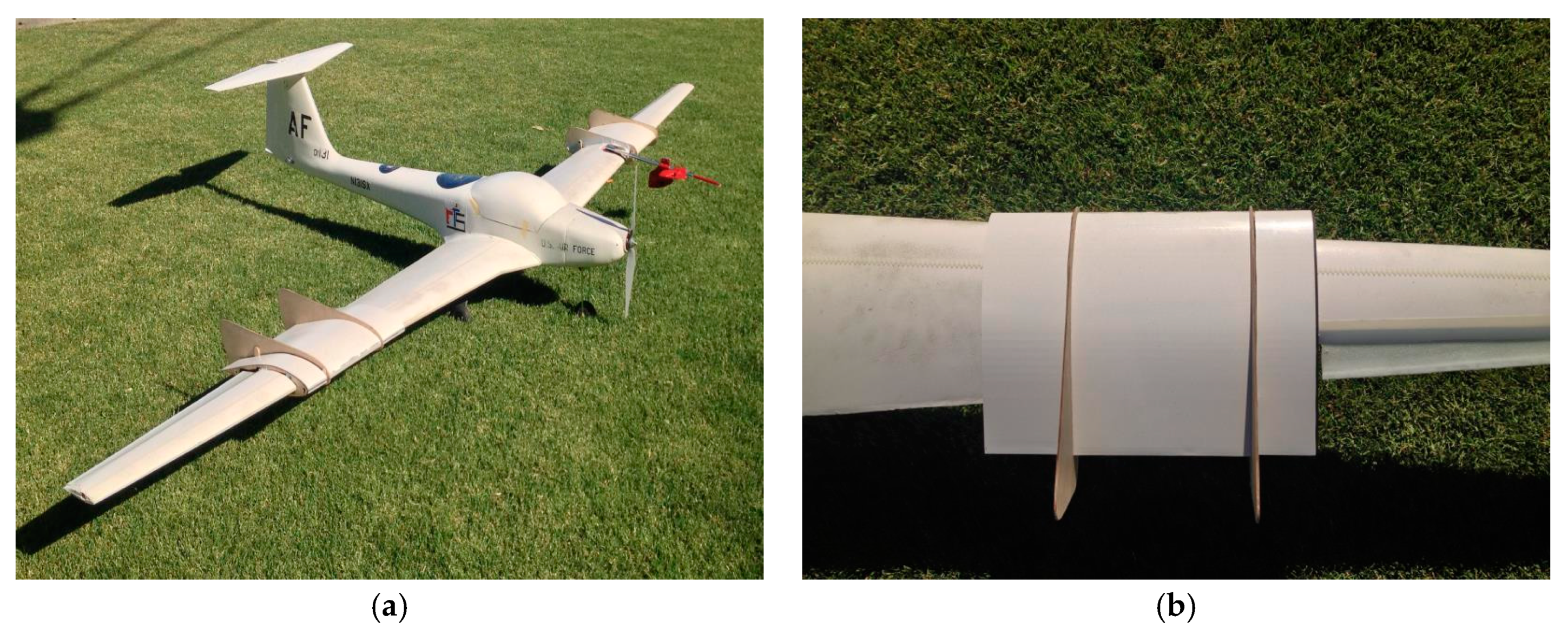
2.1. Flight Experiments
2.2. Wind Tunnel Experiments
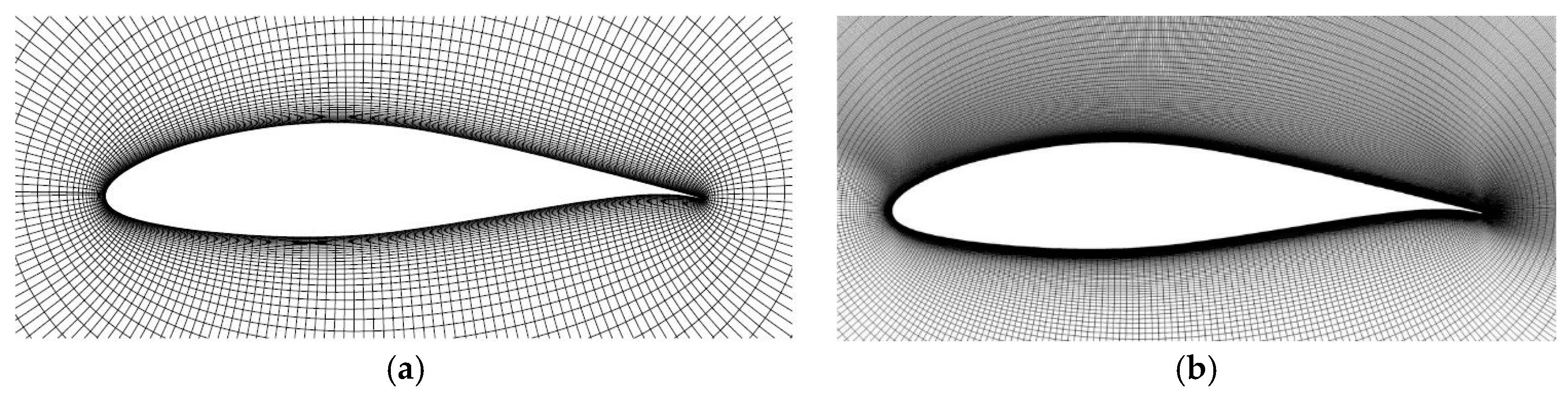
2.3. Computational Fluid Dynamics
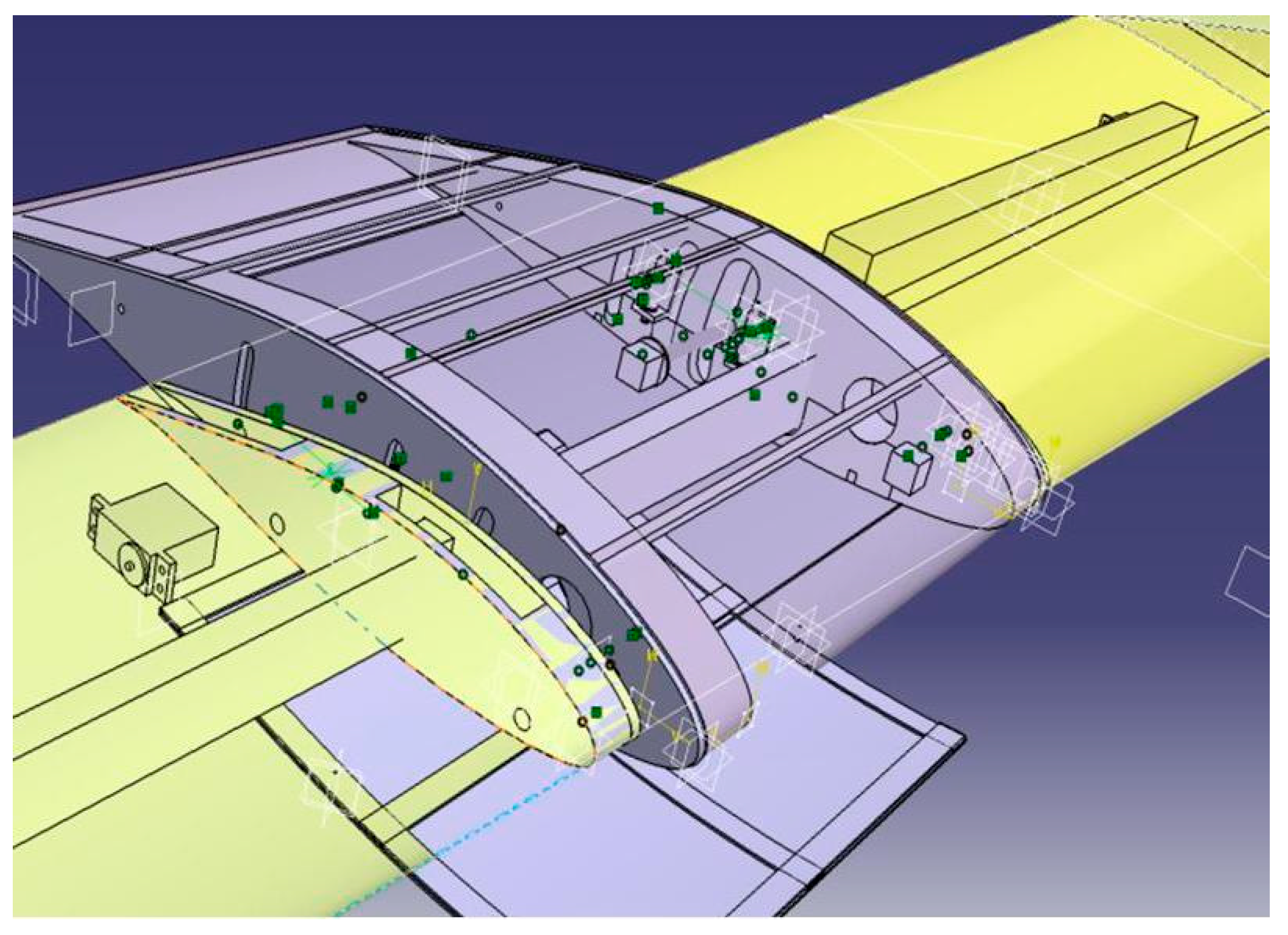
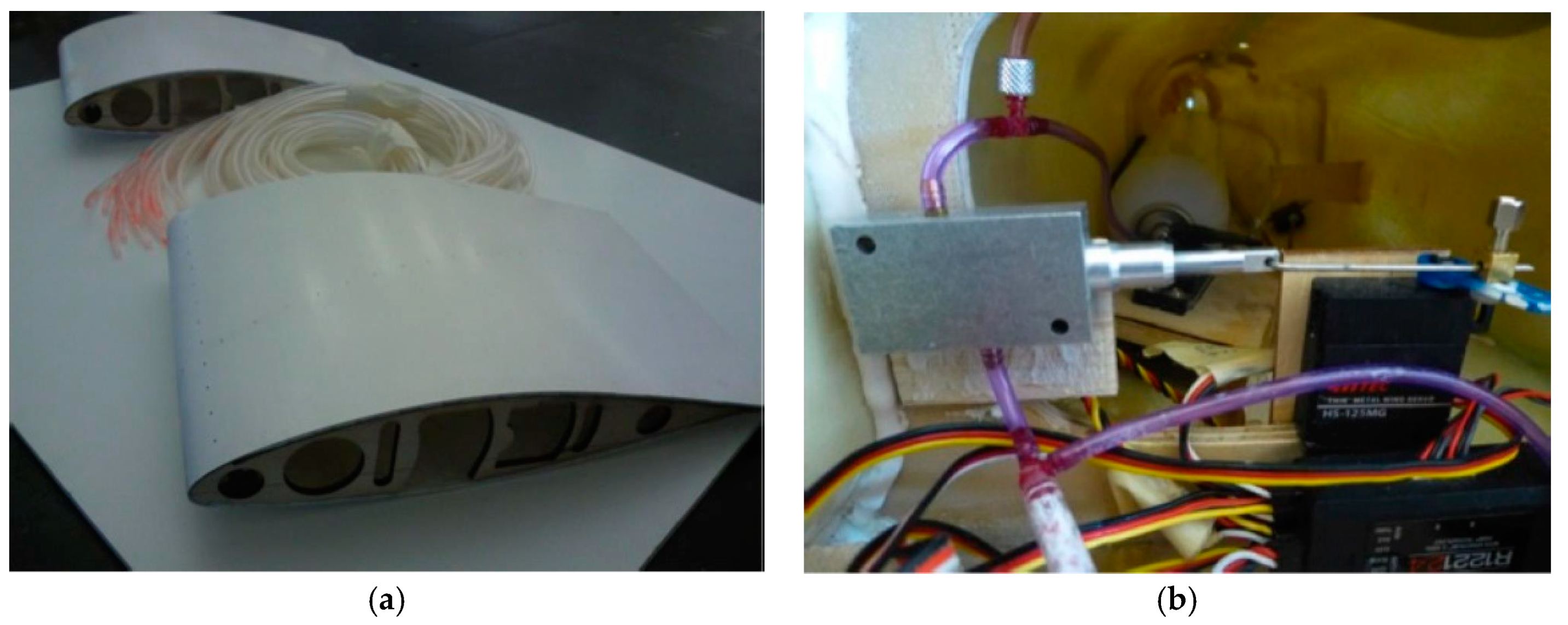
2.4. Flight Experiments with Wing Gloves
3. Results
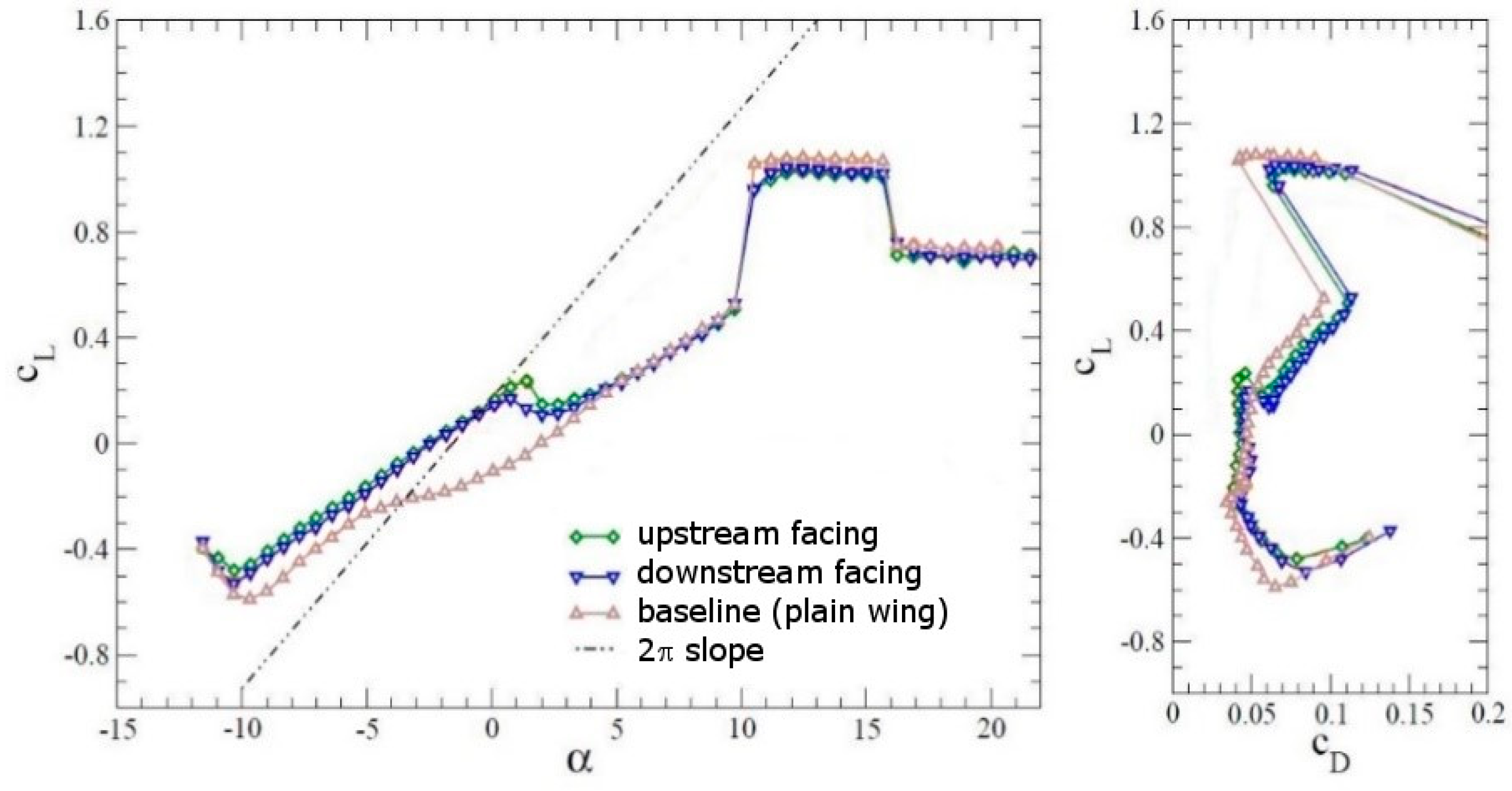
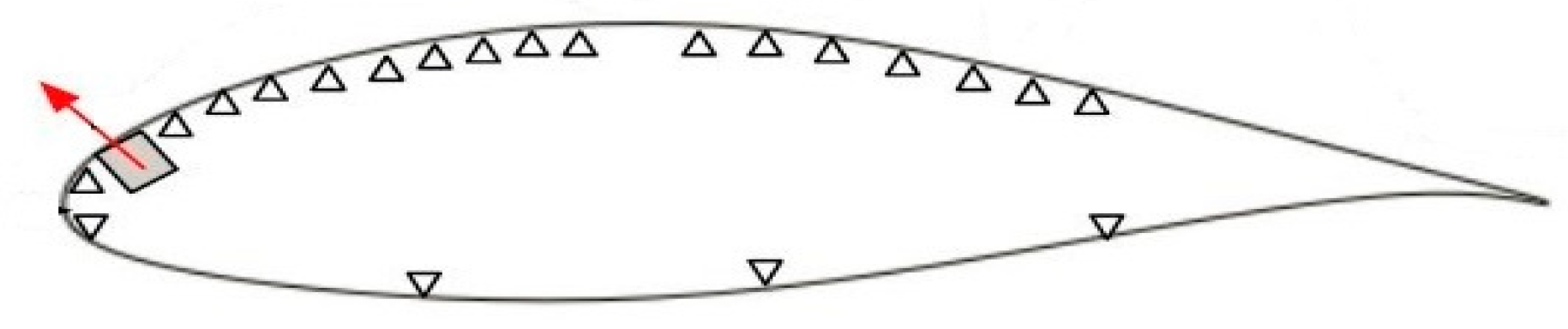
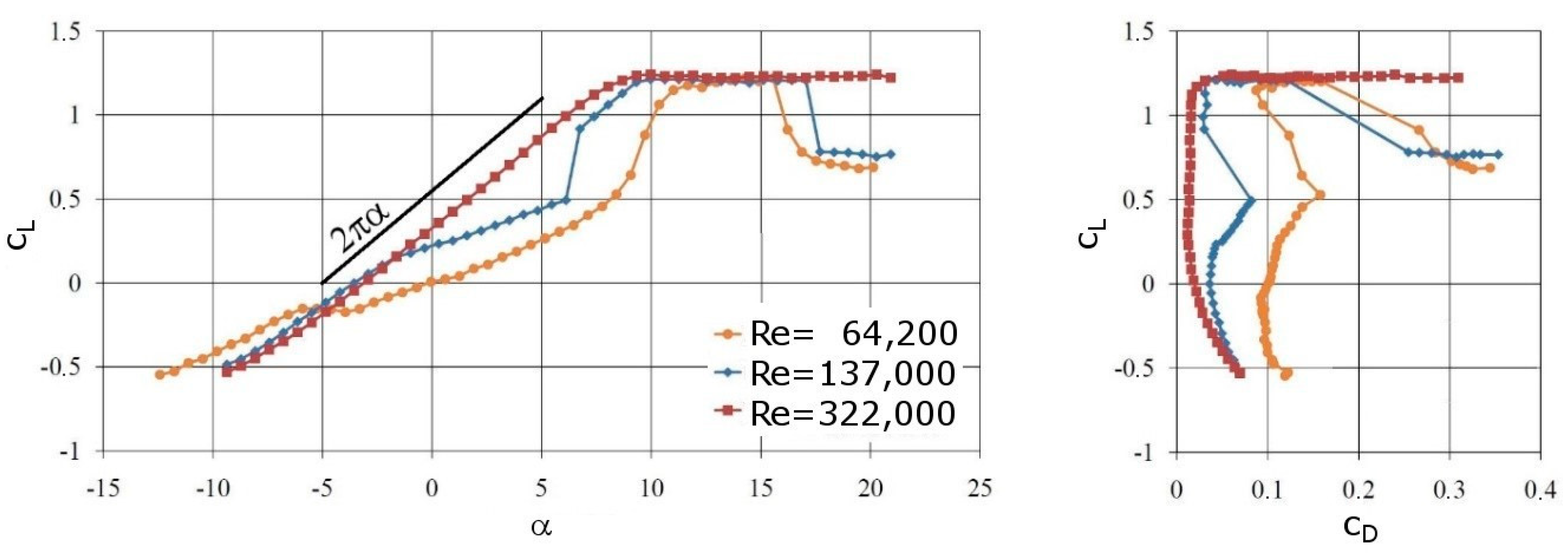
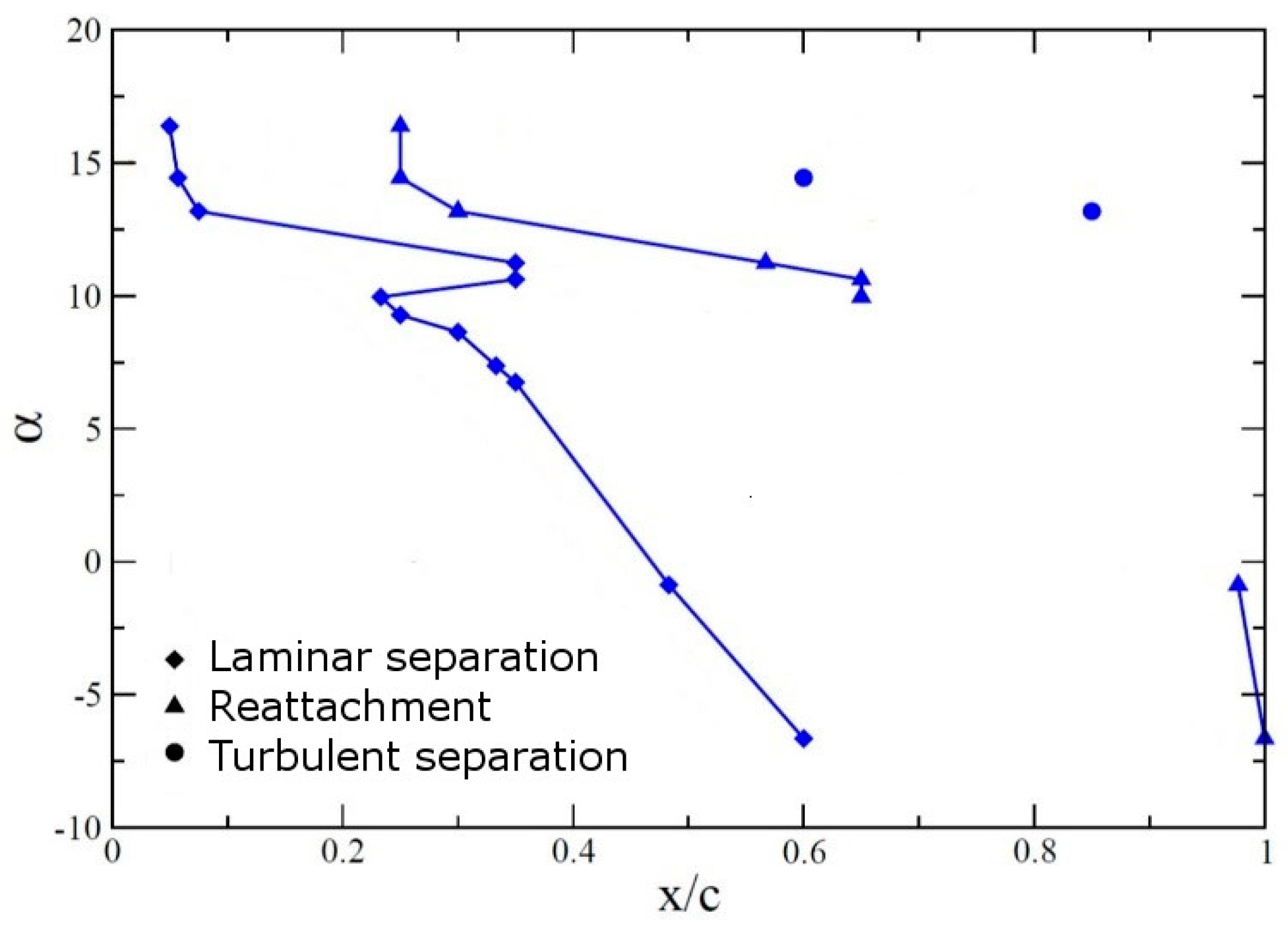
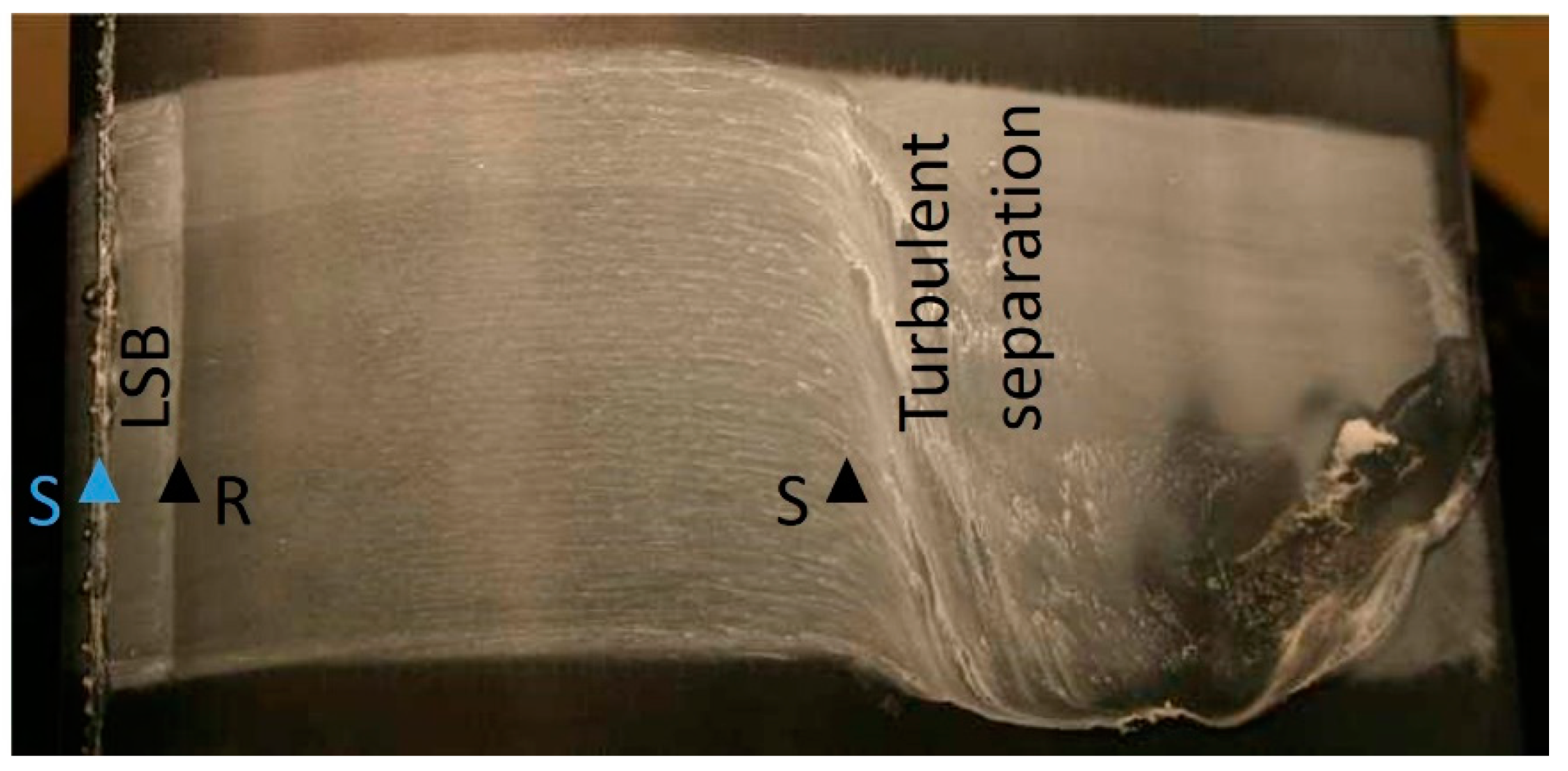
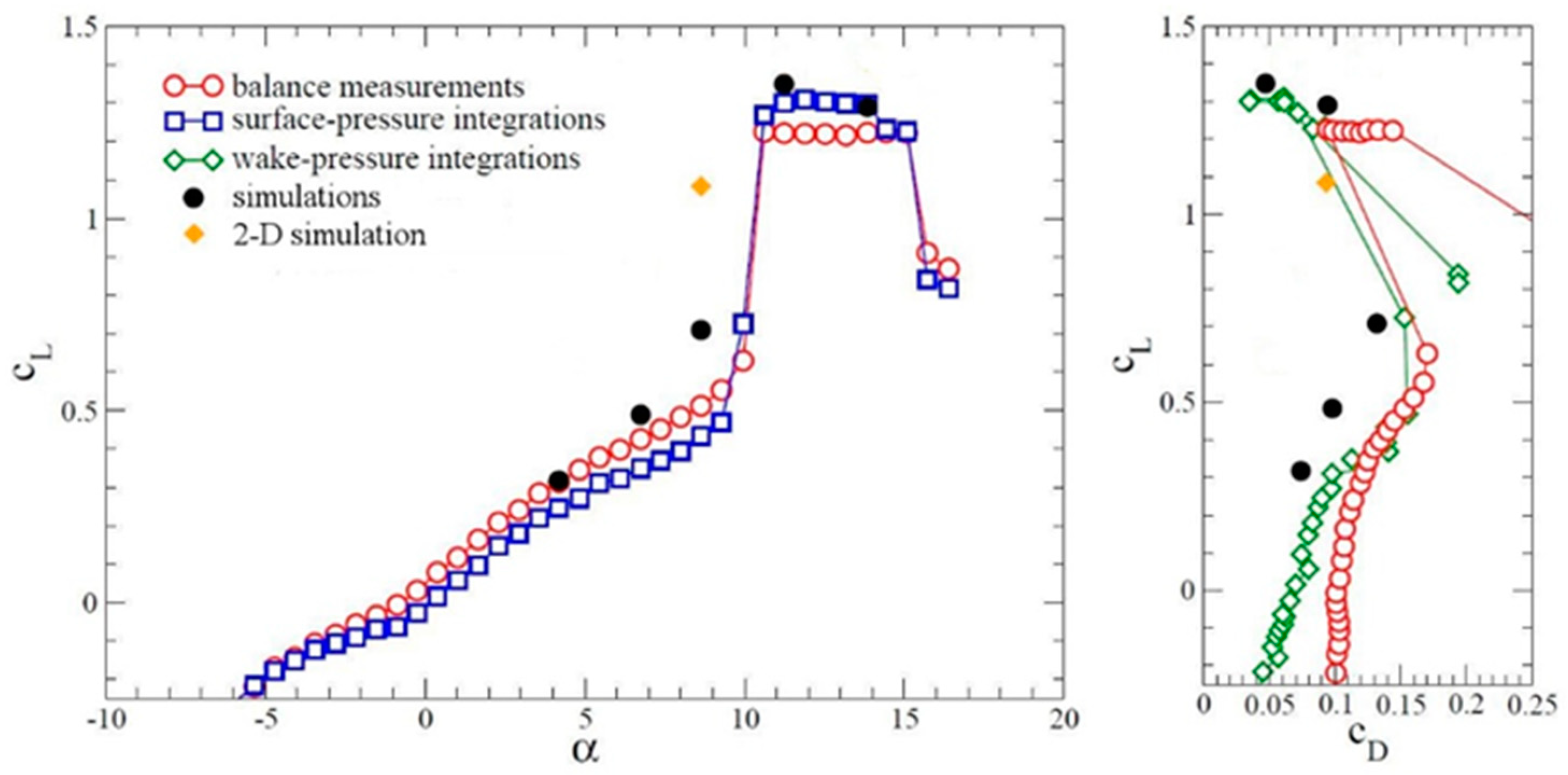
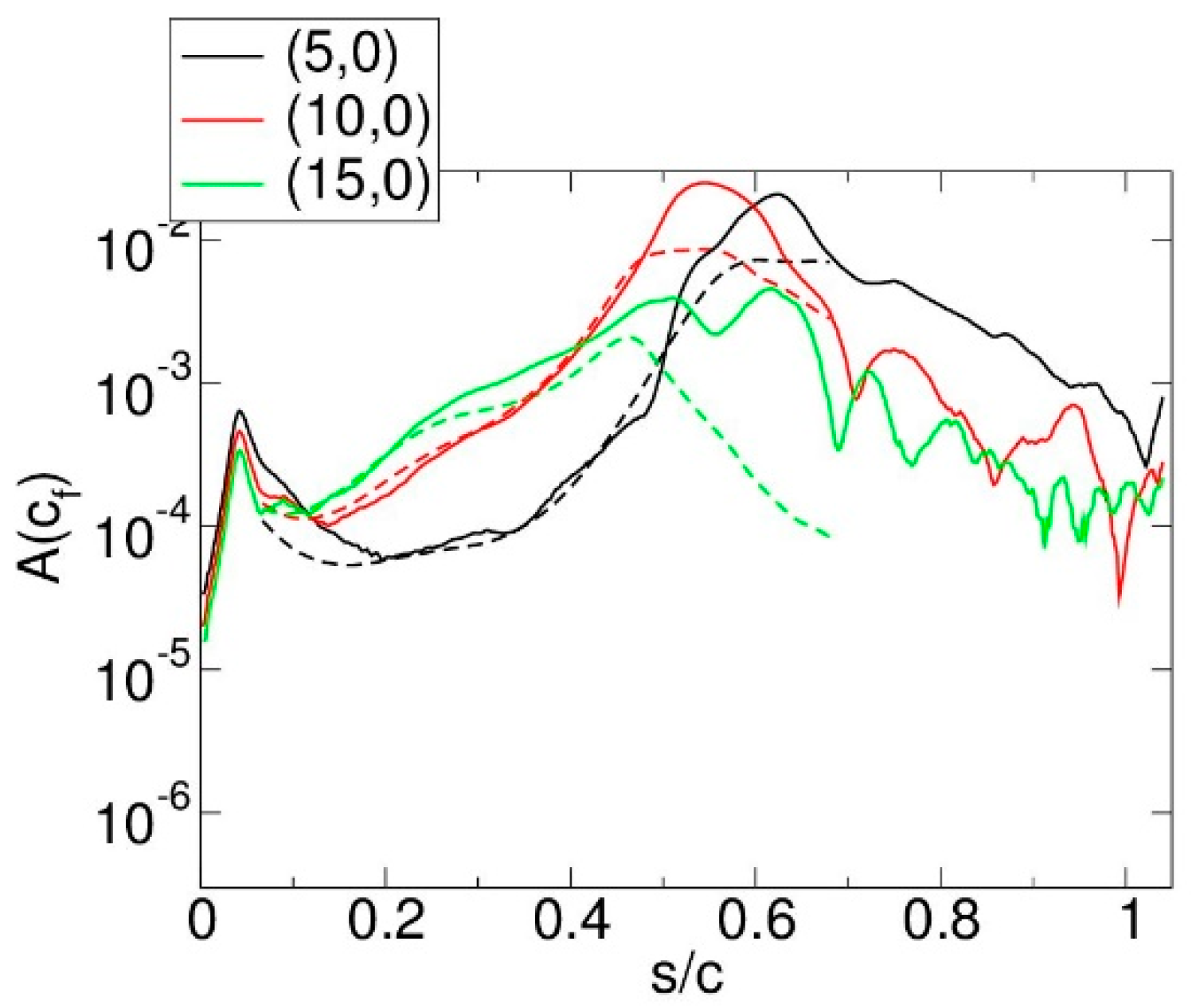
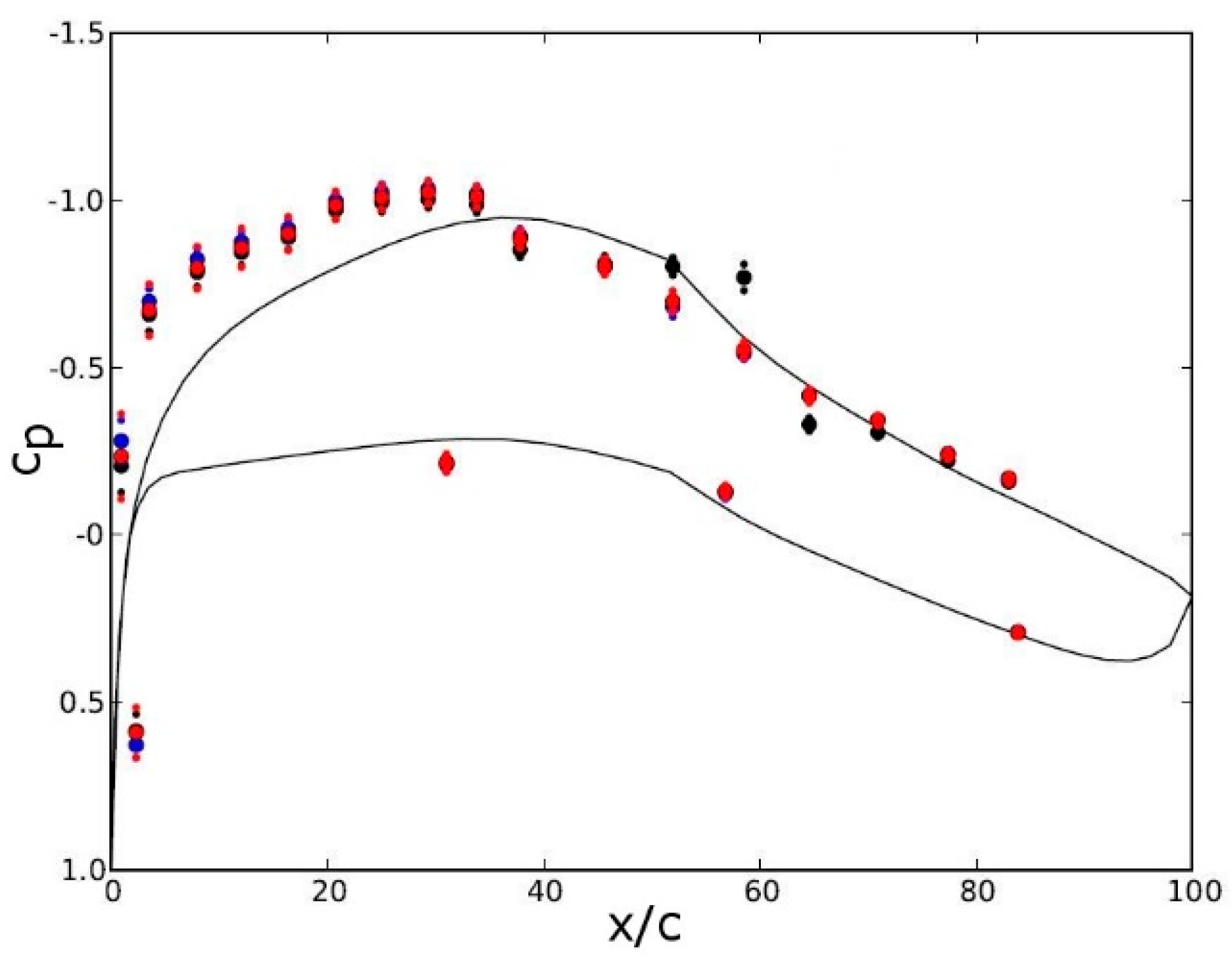
3.1. Wind Tunnel Investigation of Uncontrolled Flow
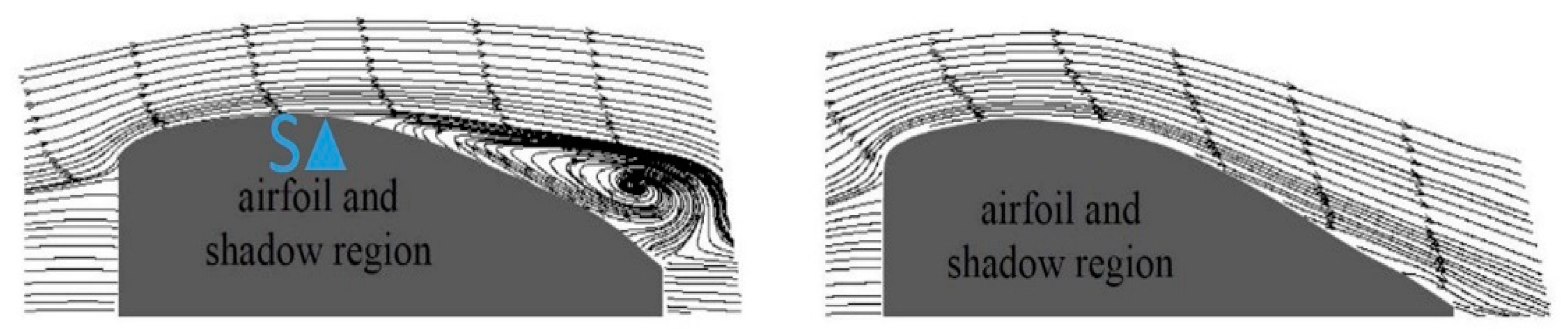
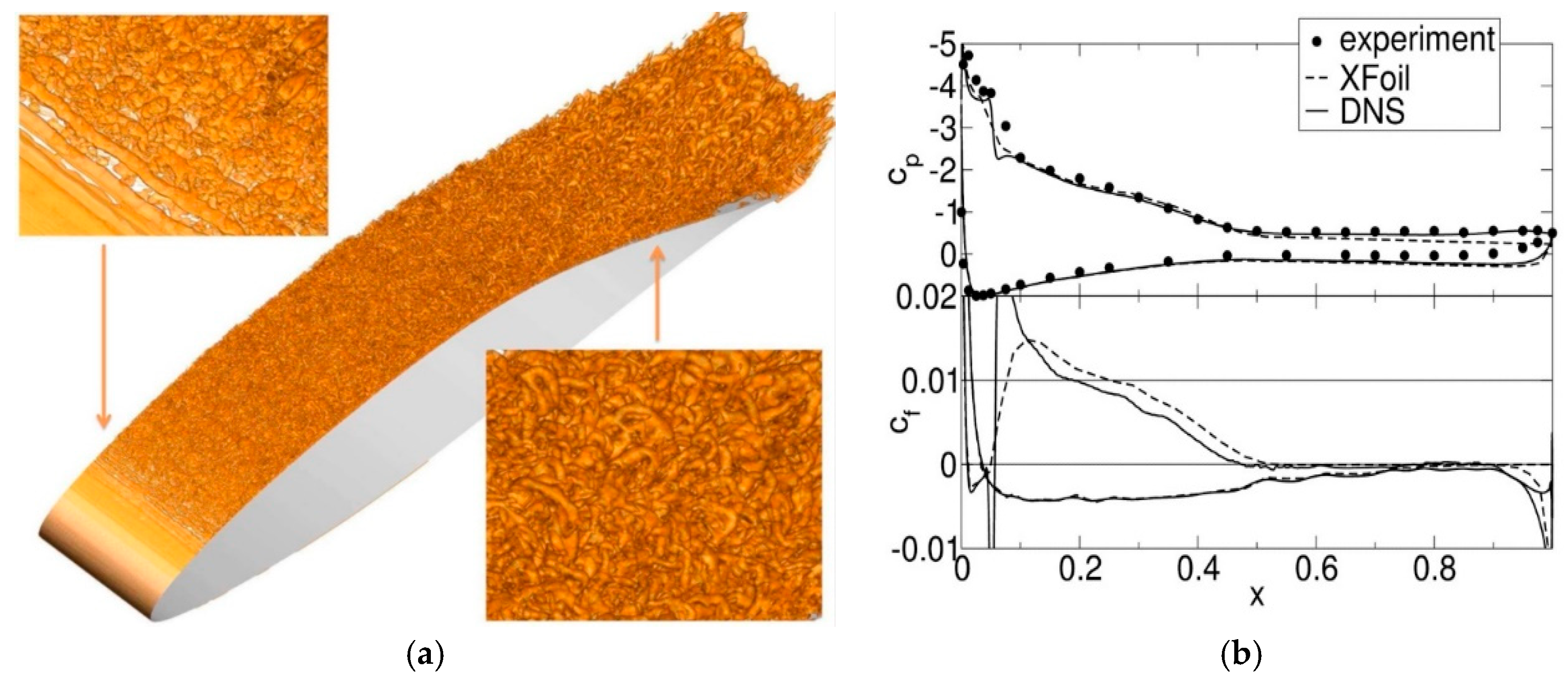
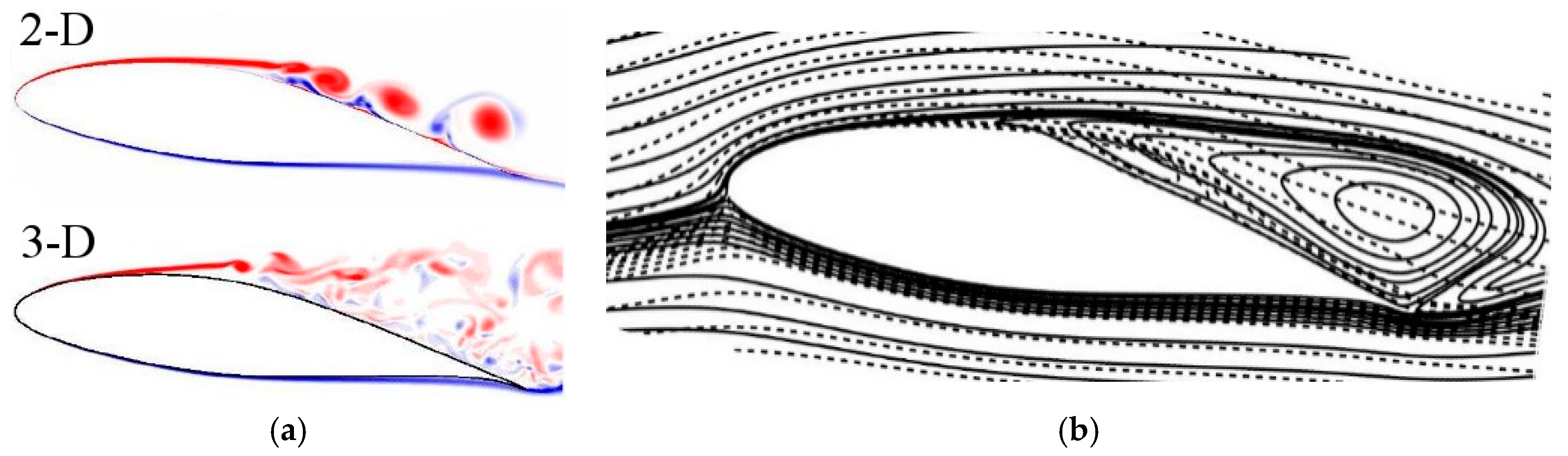
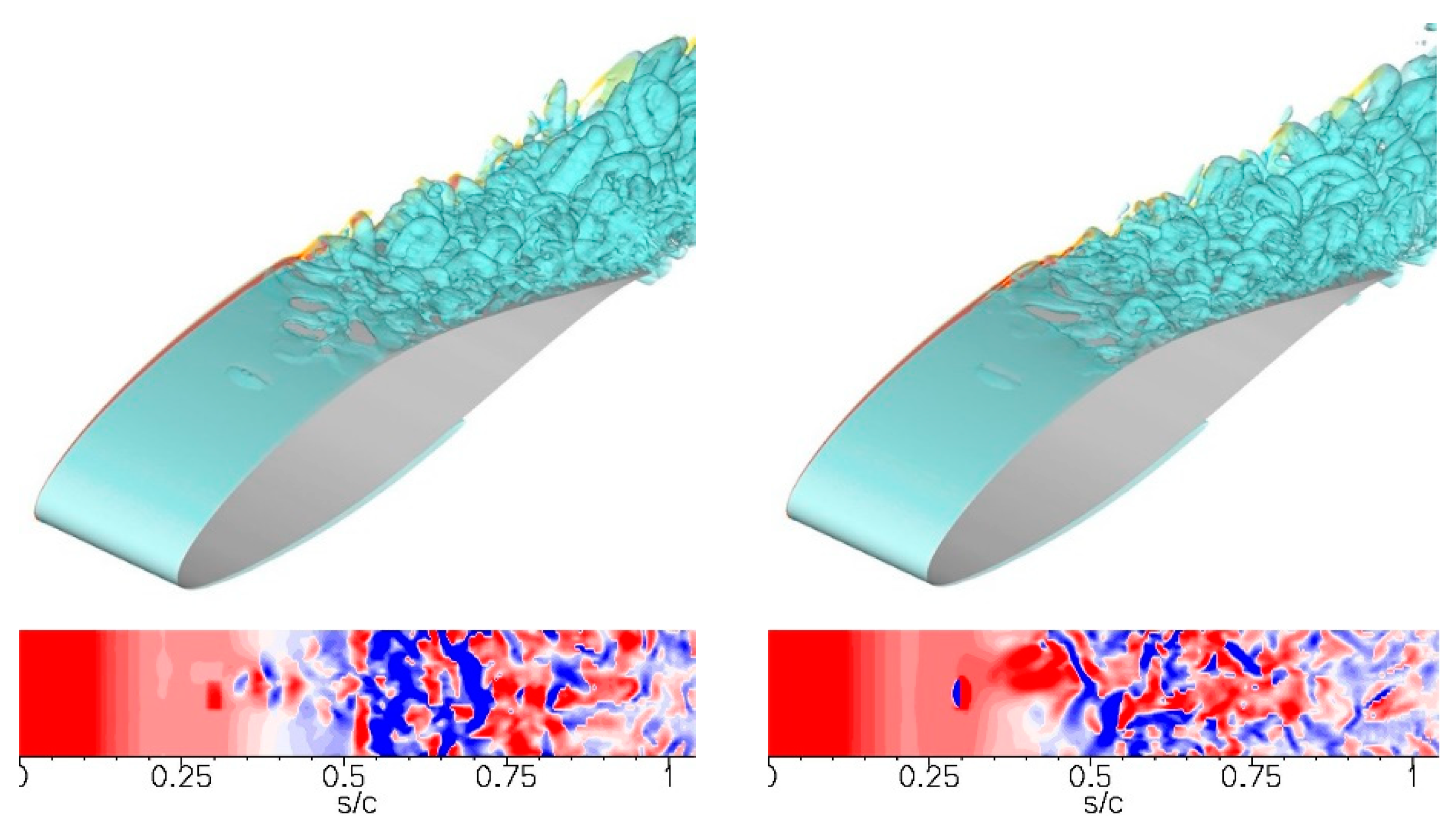
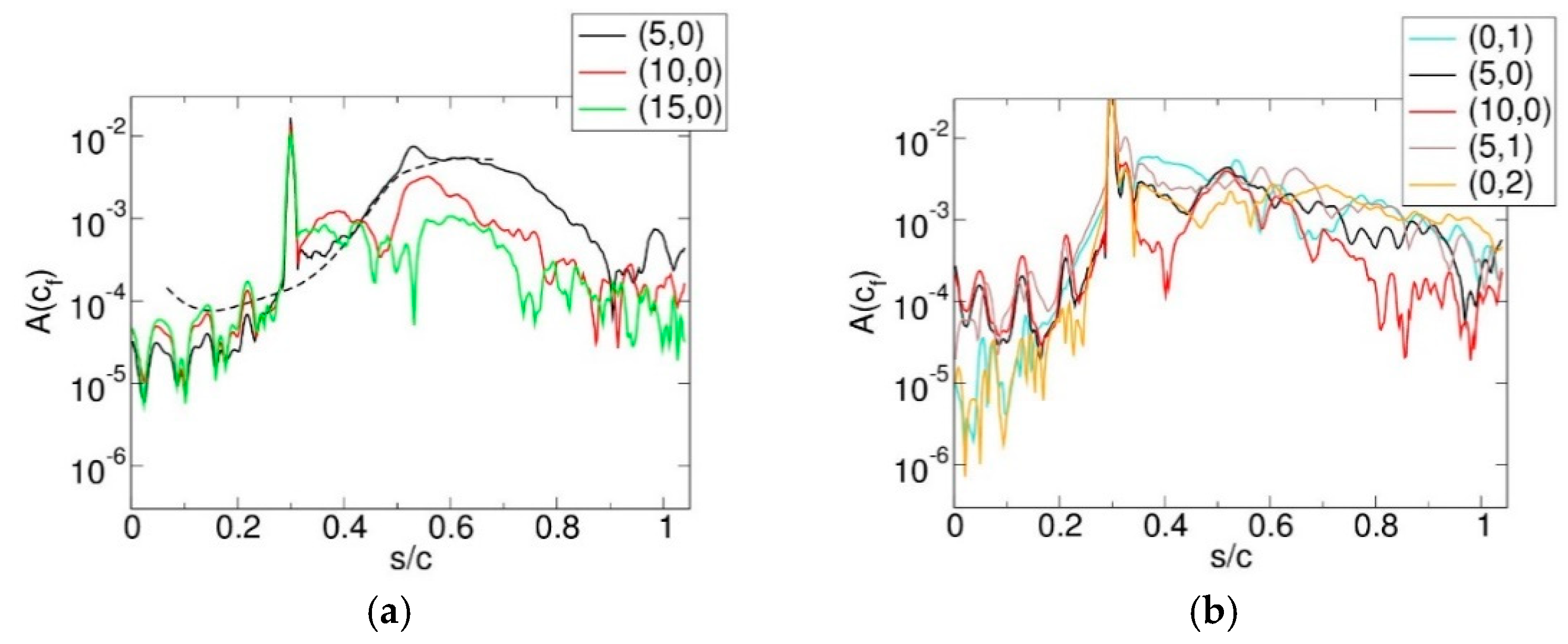
3.2. Simulations of Uncontrolled Flow
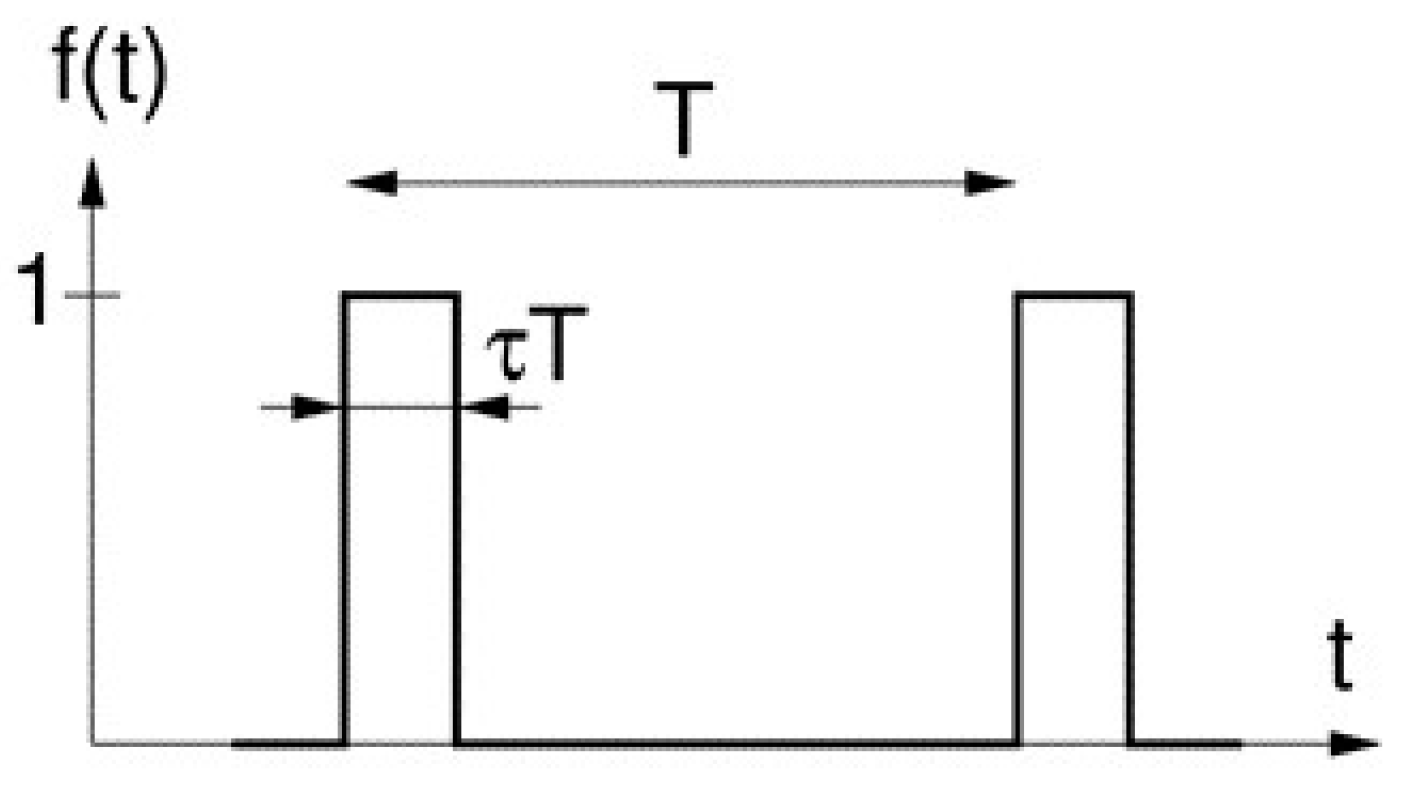
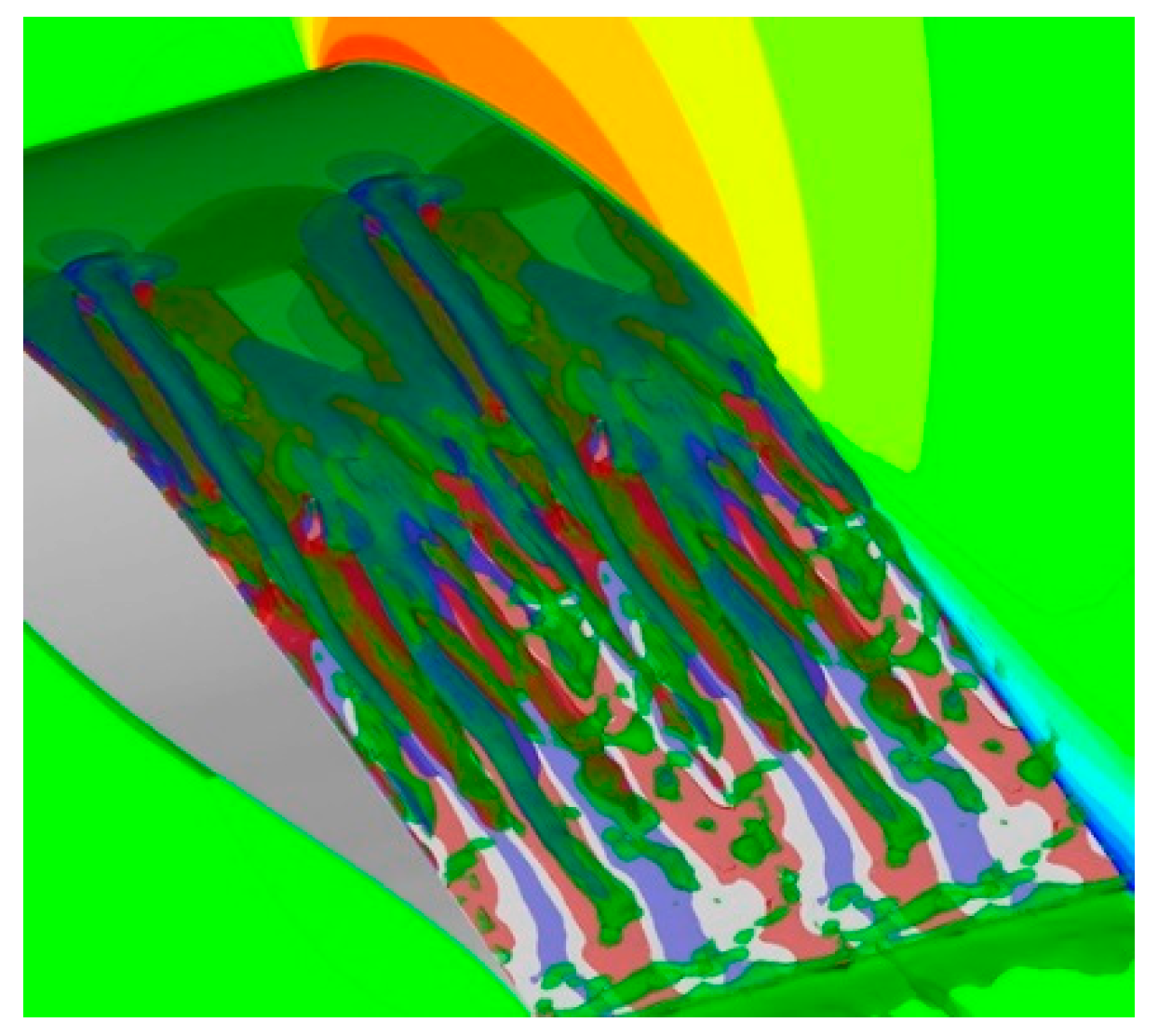
3.3. Numerical Simulations of Controlled Flow
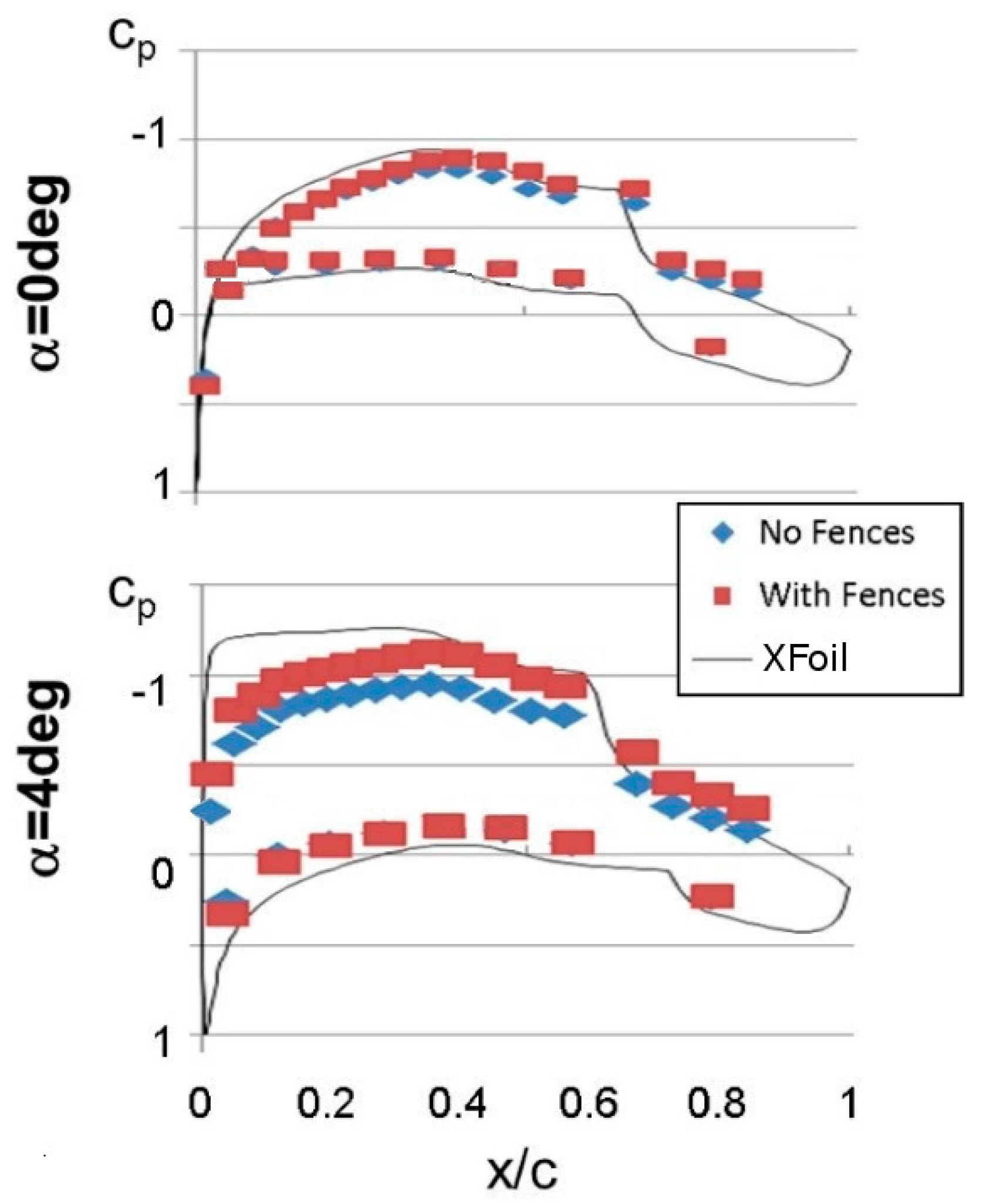
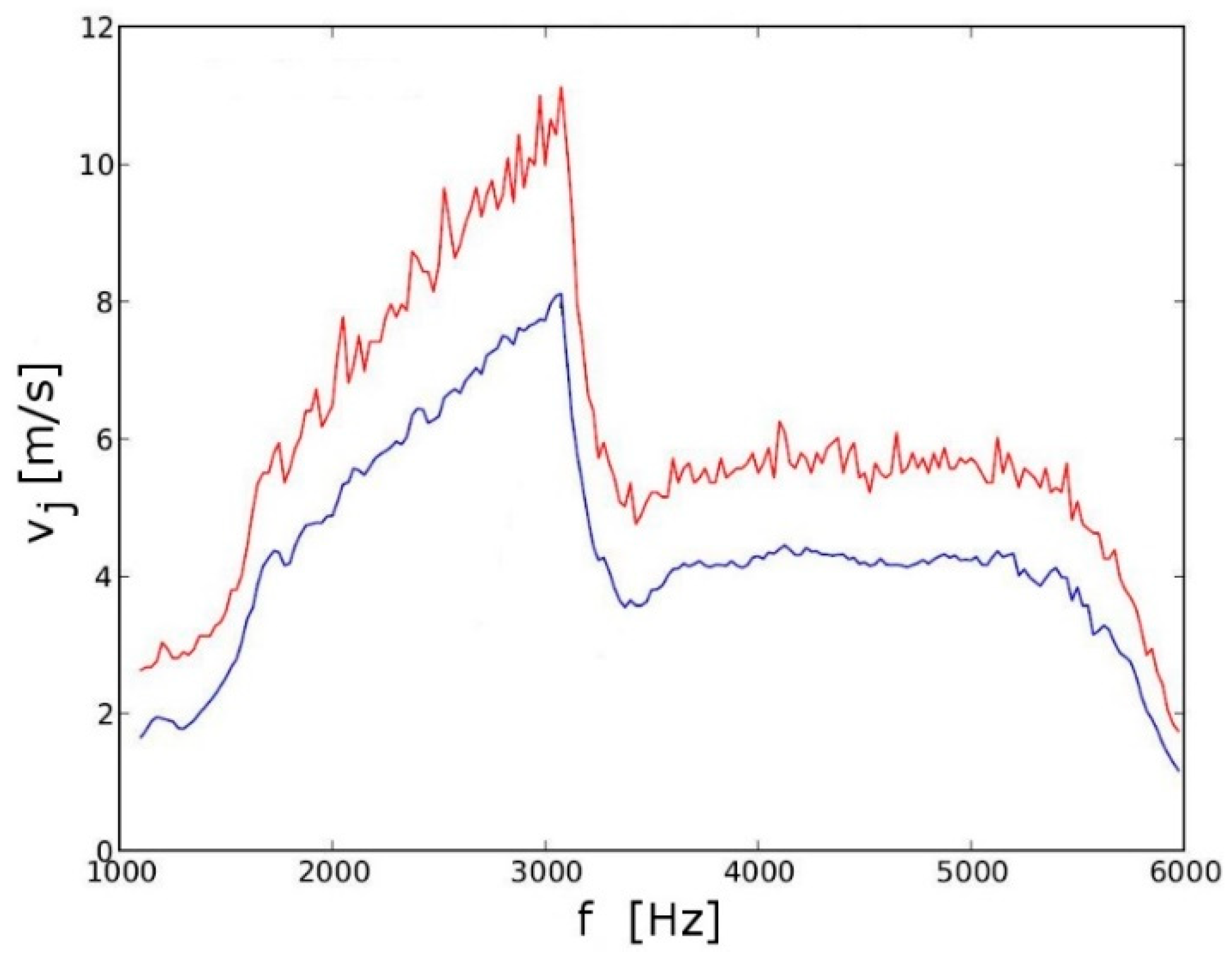
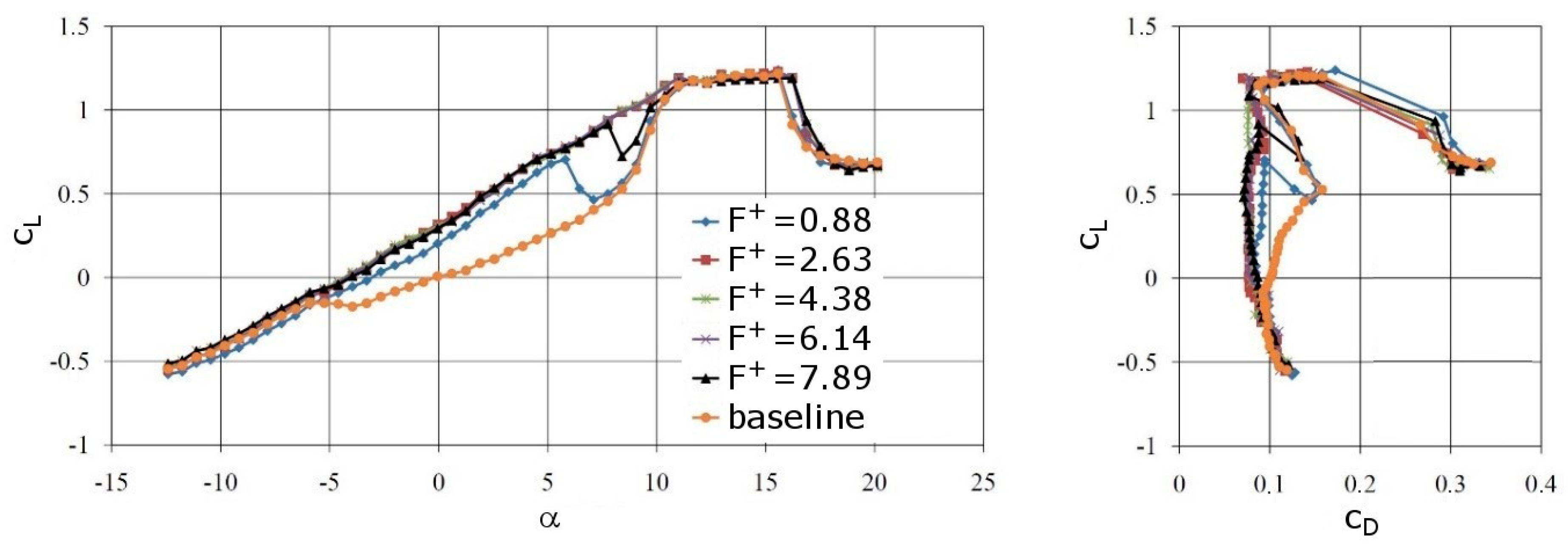
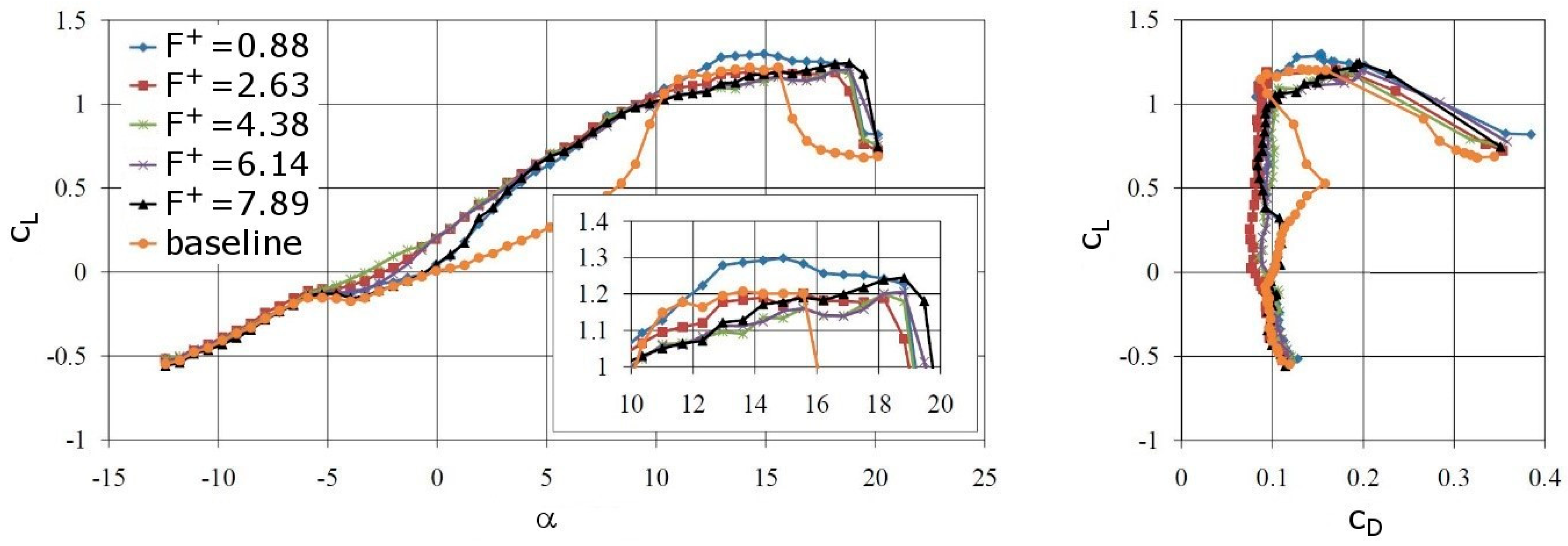
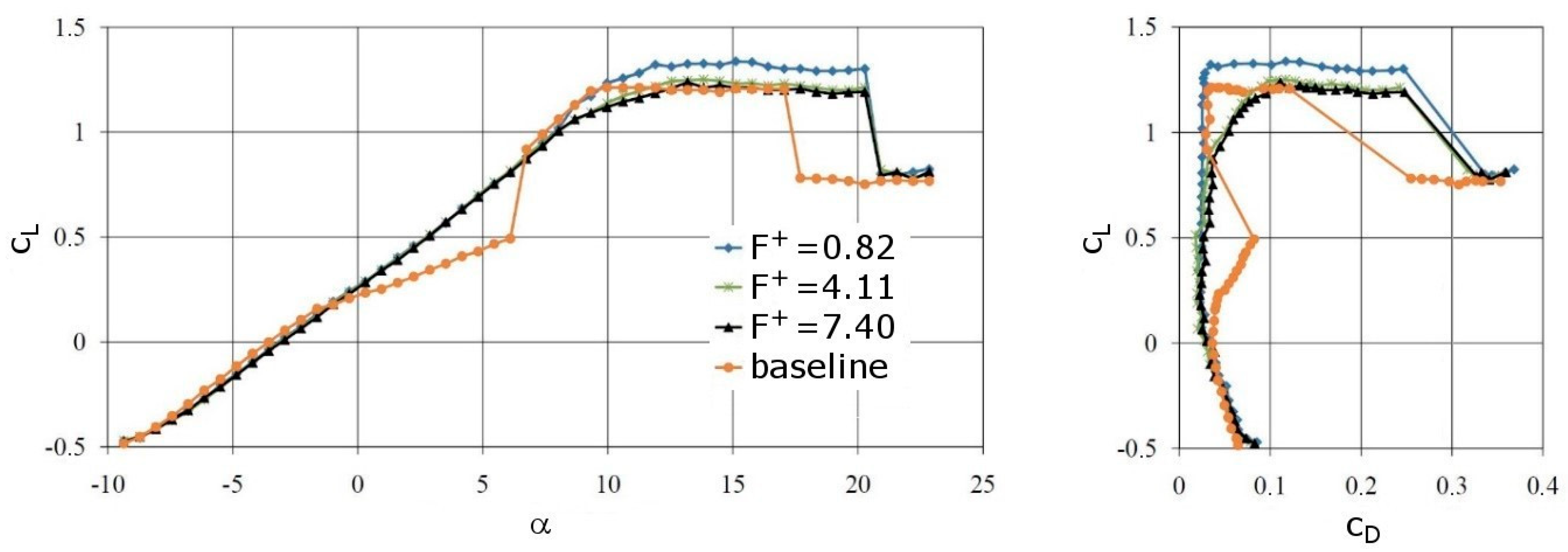
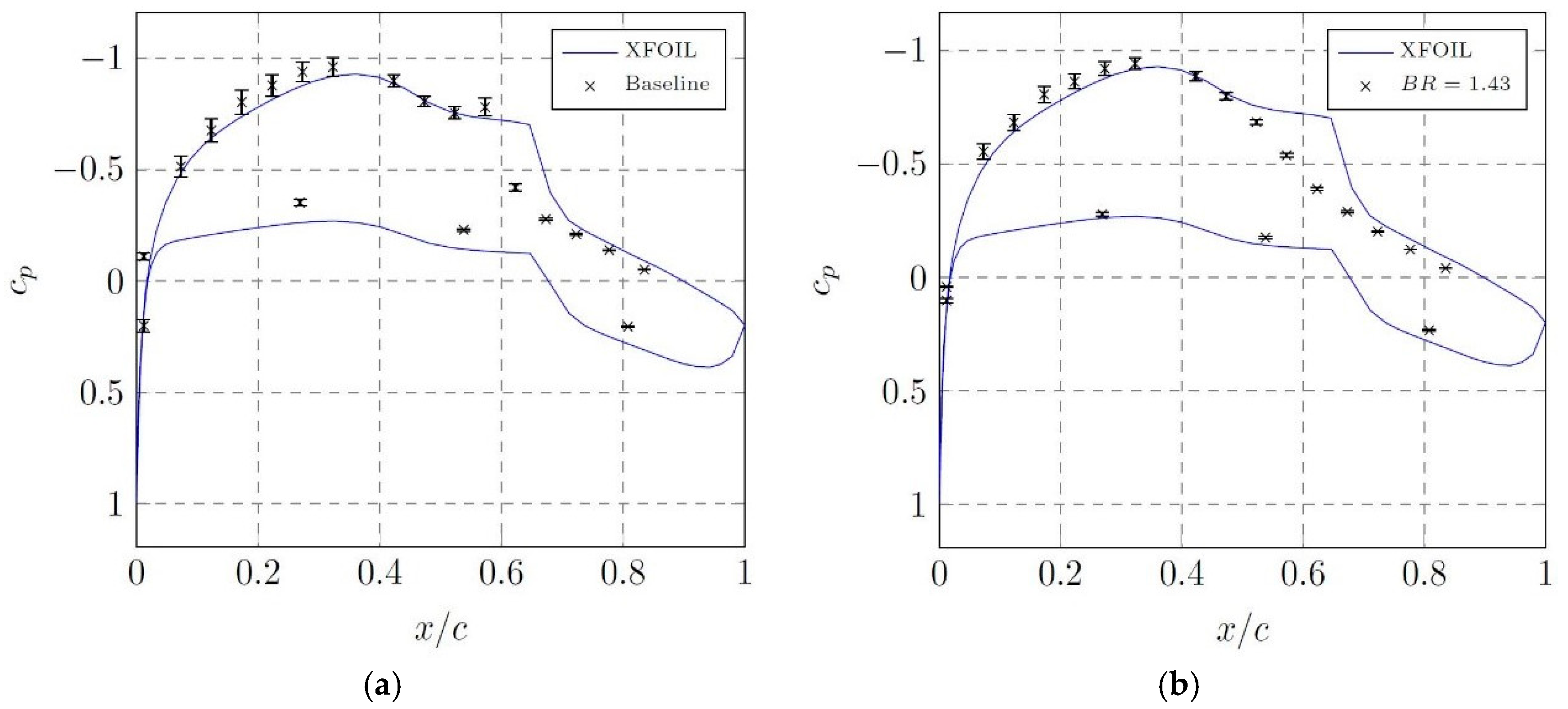
3.4. Wind Tunnel Investigations of Controlled Flow
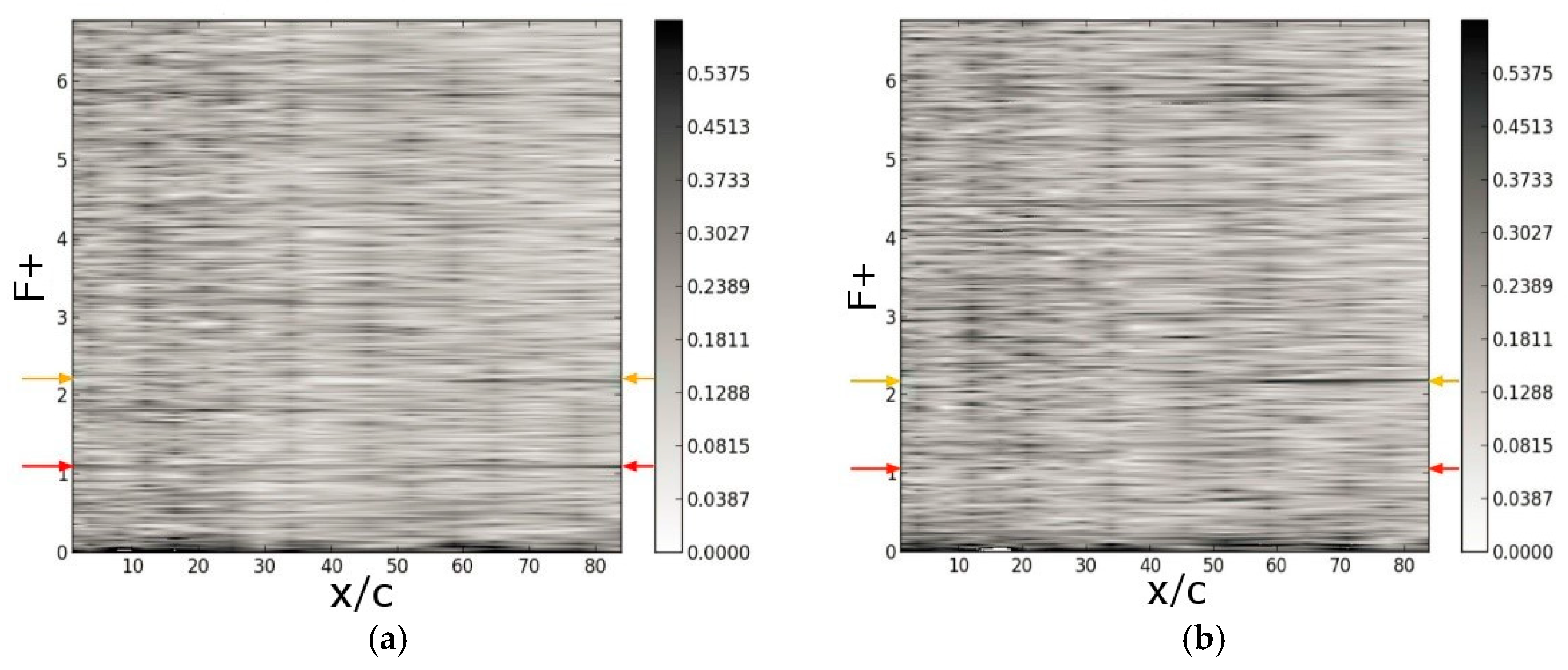
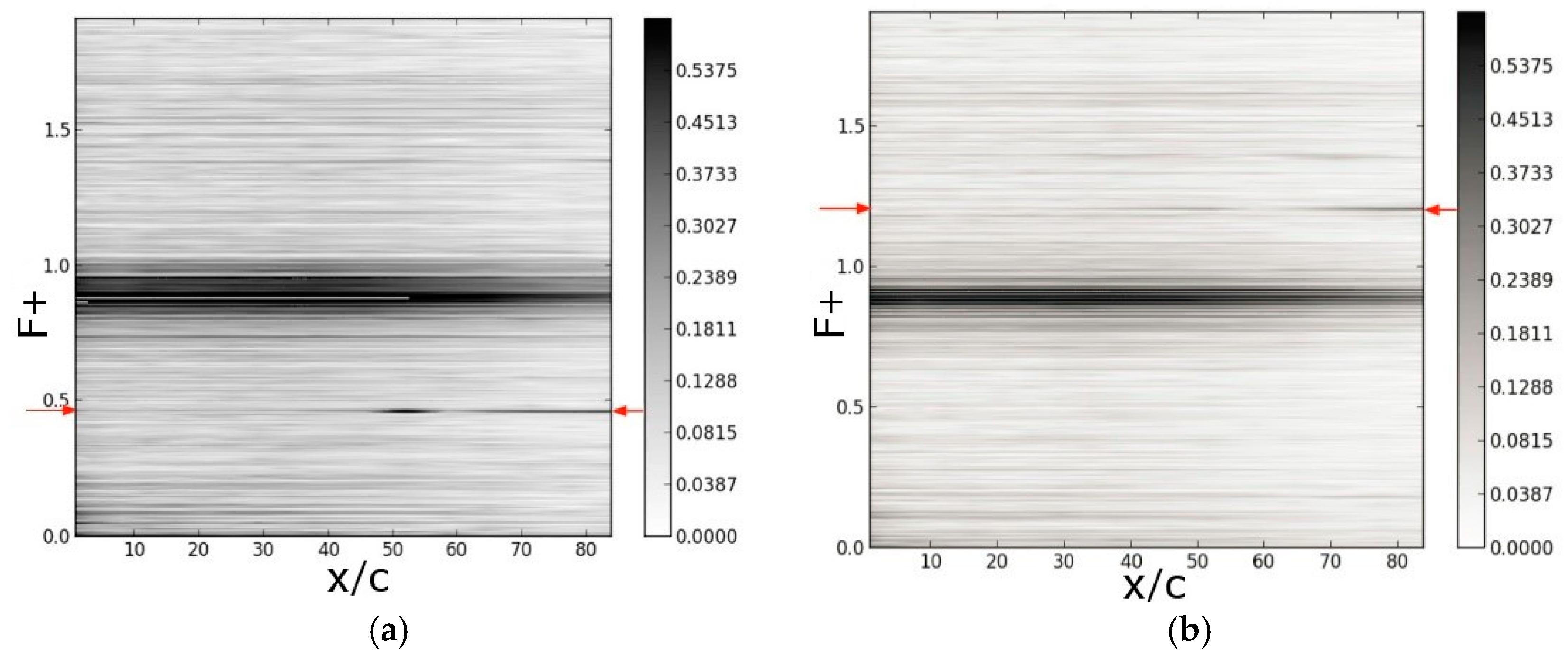
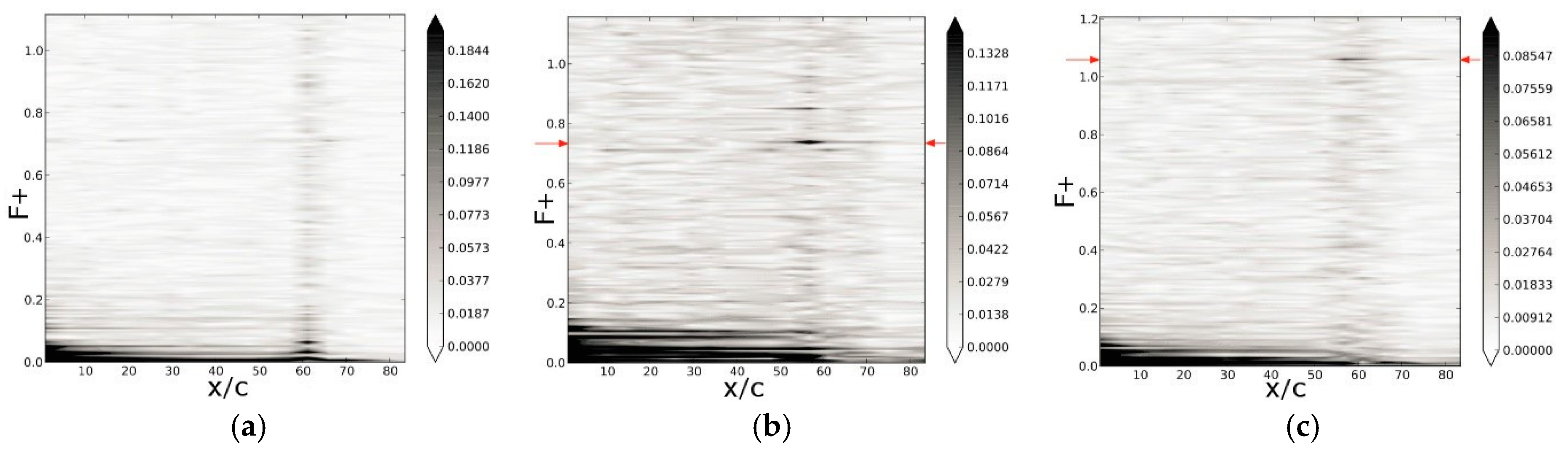
3.5. Wind Tunnel Experiments—Wing Glove
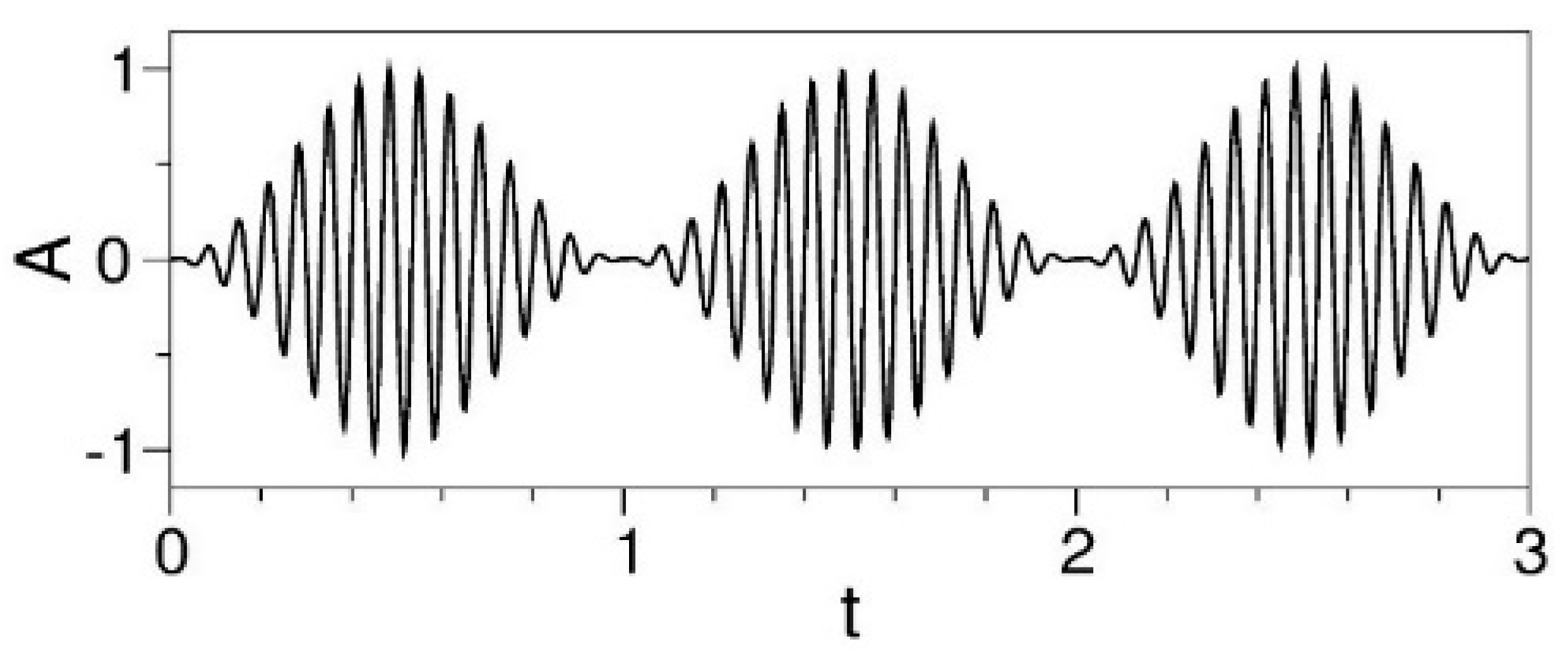
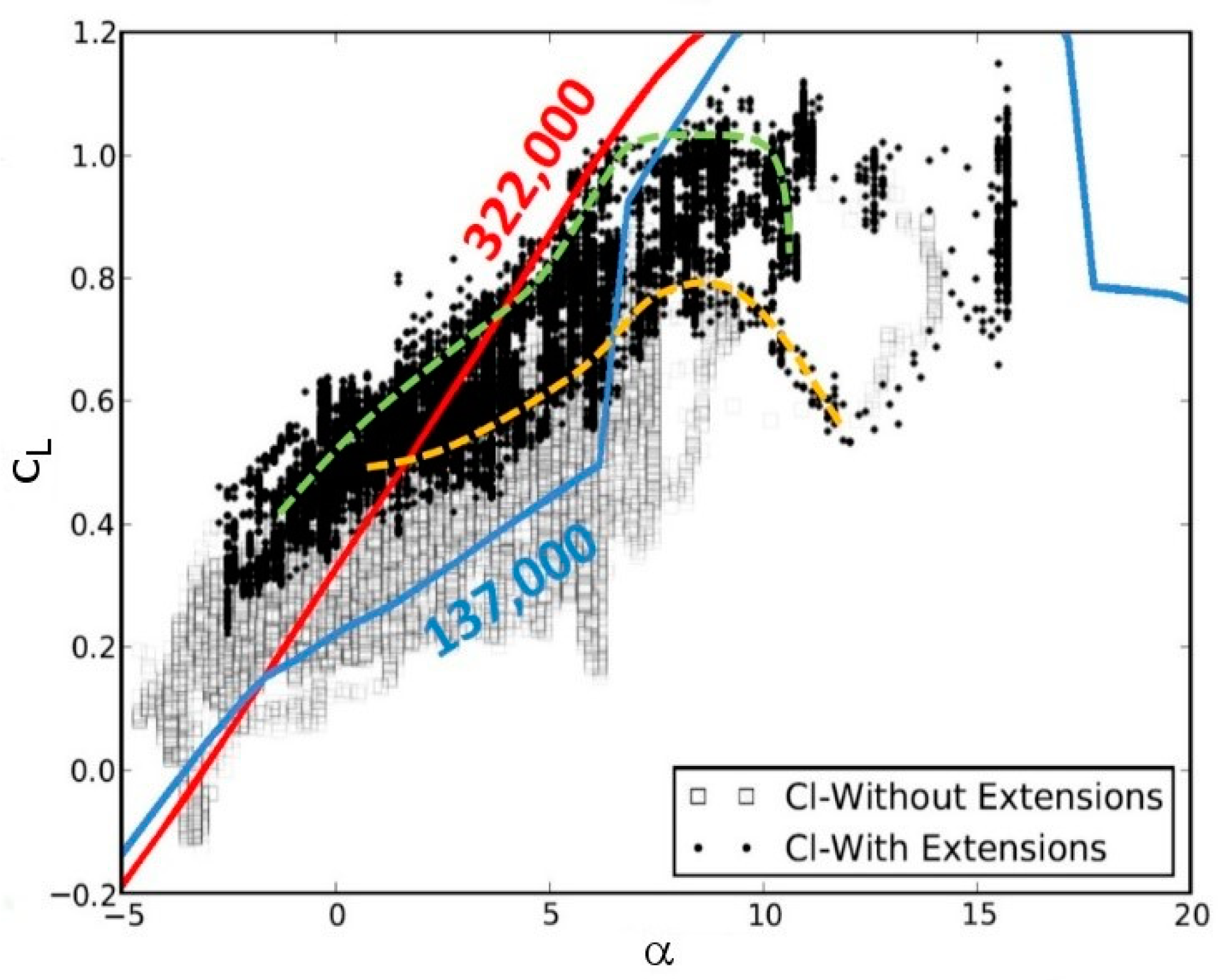
3.6. Free-Flight Experiments—Wing Glove
4. Conclusions and Outlook
Author Contributions
Funding
Conflicts of Interest
References
- Benton, S.I.; Visbal, M.R. Investigation of High-Frequency Separation Control Mechanisms for Delay of Unsteady Separation. In Proceedings of the 8th AIAA Flow Control Conference, Washington, DC, USA, 13–17 June 2016. [Google Scholar] [CrossRef]
- Benton, S.I.; Visbal, M.R. High-Frequency Forcing to Delay Dynamic Stall at Relevant Reynolds Number. In Proceedings of the 47th AIAA Fluid Dynamics Conference, Denver, CO, USA, 5–9 June 2017. [Google Scholar] [CrossRef]
- Gaster, M. The Structure and Behavior of Laminar Separation Bubbles; AGARD CP 4; AGARD: Neuilly-sur-Seine, France, 1966; pp. 819–854. [Google Scholar]
- Hain, R.; Kähler, C.J.; Radespiel, R. Dynamics of Laminar Separation Bubbles at Low-Reynolds-Number Aerofoils. J. Fluid Mech. 2009, 630, 129–153. [Google Scholar] [CrossRef]
- Jones, L.E.; Sandberg, R.D.; Sandham, N.D. Direct Numerical Simulations of Forced and Unforced Separation Bubbles on an Airfoil at Incidence. J. Fluid Mech. 2008, 602, 175–207. [Google Scholar] [CrossRef]
- Seifert, A.; Darabi, A.; Wygnanski, I. Delay of Airfoil Stall by Periodic Excitation. J. Aircr. 1996, 33, 691–698. [Google Scholar] [CrossRef]
- Bons, J.P.; Sondergaard, R.; Rivir, R.B. Turbine Separation Control Using Pulsed Vortex Generator Jets. J. Turbomach. 2001, 123, 198–206. [Google Scholar] [CrossRef]
- Postl, D.; Balzer, W.; Fasel, H.F. Control of laminar separation using pulsed vortex generator jets: Direct numerical simulations. J. Fluid Mech. 2011, 676, 81–109. [Google Scholar] [CrossRef]
- Gross, A.; Balzer, W.; Fasel, H. Numerical Investigation of Low-Pressure Turbine Flow Control (Invited). In Proceedings of the 38th Fluid Dynamics Conference and Exhibit, Fluid Dynamics and Co-located Conferences, Seattle, WA, USA, 23–26 June 2008. [Google Scholar] [CrossRef]
- Huang, J.; Corke, T.C.; Thomas, F.O. Plasma Actuators for Separation Control of Low-Pressure Turbine Blades. AIAA J. 2006, 44, 51–57. [Google Scholar] [CrossRef]
- Huang, J.; Corke, T.C.; Thomas, F.O. Unsteady Plasma Actuators for Separation Control of Low-Pressure Turbine Blades. AIAA J. 2006, 44, 1477–1487. [Google Scholar] [CrossRef]
- Gaitonde, D.V.; Visbal, M.R.; Roy, S. Control of Flow Past a Wing Section with Plasma-Based Body Forces. In Proceedings of the 36th AIAA Plasmadynamics and Lasers Conference, Toronto, ON, Canada, 6–9 June 2005. [Google Scholar] [CrossRef]
- Rizzetta, D.P.; Visbal, M.R. Numerical Investigation of Plasma-Based Flow Control for a Transitional Highly-Loaded Low-Pressure Turbine. AIAA J. 2007, 45, 2554–2564. [Google Scholar] [CrossRef]
- Visbal, M.R.; Gaitonde, D.V.; Roy, S. Control of Transitional and Turbulent Flows Using Plasma-Based Actuators. In Proceedings of the 36th AIAA Fluid Dynamics Conference and Exhibit, San Francisco, CA, USA, 5–8 June 2006. [Google Scholar] [CrossRef]
- Cain, A.B.; Ng, T.T.; Kerschen, E.J.; Fasel, H.F.; Fasse, E.D.; Israel, D.M. Flight Demonstration of Stealthy Closed-Loop Attitude Control; AFOSR STTR Final Report Contract FA49620-01-C-0044; Defense Technical Information Center: Fort Belvoir, VA, USA, 2002. [Google Scholar]
- Ciuryla, M.; Liu, Y.; Farnsworth, J.; Kwan, C.; Amitay, M. Flight Control Using Synthetic Jets on a Cessna 182 Model. J. Aircr. 2007, 44, 642–653. [Google Scholar] [CrossRef]
- Seifert, A.; David, S.; Fono, I.; Stalnov, O.; Dayan, I. Roll Control via Active Flow Control: From Concept to Flight. J. Aircr. 2010, 47, 864–874. [Google Scholar] [CrossRef]
- Braslow, A.L. A History of Suction-Type Laminar-Flow Control with Empahsis on Flight Research; NASA History Division, Monographs in Aerospace History: Washington, DC, USA, 1999. [Google Scholar]
- Nitsche, W.; Suttan, J.; Becker, S.; Erb, P.; Kloker, M.; Stemmer, C. Experimental and numerical investigations of controlled transition in low-speed free flight. Aerosp. Sci. Technol. 2001, 5, 245–255. [Google Scholar] [CrossRef]
- Duchmann, A.; Simon, B.; Tropea, C.; Grundmann, S. Dielectric Barrier Discharge Plasma Actuators for In-Flight Transition Delay. AIAA J. 2014, 52, 358–367. [Google Scholar] [CrossRef]
- Peltzer, I.; Pätzold, A.; Nitsche, W. In-Flight Experiments on Delaying Laminar-Turbulent Transition on a Laminar Wing Glove. J. Aerosp. Eng. 2009, 223, 619–626. [Google Scholar] [CrossRef]
- Peltzer, I.; Wicke, K.; Pätzold, A.; Nitsche, W. In-flight experiments on active TS-wave control on a 2D-laminar wing glove. In Seventh IUTAM Symposium on Laminar-Turbulent Transition; IUTAM Bookseries; Schlatter, P., Henningson, D., Eds.; Springer: Dordrecht, The Netherland, 2010; Volume 18. [Google Scholar]
- Belisle, M.; Roberts, M.; Williams, T.; Tufts, M.; Tucker, A.; Saric, W.; Reed, H. A Transonic Laminar-Flow Wing Glove Flight Experiment: Overview and Design Optimization. In Proceedings of the 30th AIAA Applied Aerodynamics Conference, New Orleans, LA, USA, 25–28 June 2012. [Google Scholar] [CrossRef]
- Gross, A.; Pearman, C.; Kremer, R.; Napier, B.; Gosla, C.; Kurz, A.; Mack, S.; Brehm, C.; Heine, B.; Radi, A.; et al. 1/5 Scale Model of Aeromot 200S SuperXimango for Scaled Flight Research. In Proceedings of the 26th AIAA Applied Aerodynamics Conference, Honolulu, HI, USA, 18–21 August 2008. [Google Scholar] [CrossRef]
- Wolowicz, C.H.; Bowman, J.S.; Gilbert, W.P. Similitude Requirements and Scaling Relationships as Applied to Model Testing; NASA TP-1435; NASA Langley Research Center: Hampton, VA, USA, 1979.
- Gainer, T.G.; Hoffman, S. Summary of Transformation Equations and Equations of Motion Used in Free-Flight and Wind Tunnel Data Reduction and Analysis; NASA SP-3070; NASA Langley Research Center: Hampton, VA, USA, 1972.
- Jordan, T.; Foster, J.; Bailey, R.; Belcastro, C. AirSTAR: A UAV Platform for Flight Dynamics and Control System Testing. In Proceedings of the 25th AIAA Aerodynamic Measurement Technology and Ground Testing Conference, San Francisco, CA, USA, 5–8 June2006. [Google Scholar] [CrossRef]
- Mack, S.; Brehm, C.; Heine, B.; Fasel, H. Experimental Investigation of Separation and Separation Control of a Laminar Airfoil. In Proceedings of the 4th Flow Control Conference, Seattle, WA, USA, 23–26 June 2008. [Google Scholar] [CrossRef]
- Plogmann, B.; Mack, S.; Fasel, H.F. Experimental Investigation of Open- and Closed-Loop Control for Airfoil under Low Reynolds Number Conditions. In Proceedings of the 39th AIAA Fluid Dynamics Conference, San Antonio, TX, USA, 22–25 June 2009. [Google Scholar] [CrossRef]
- Gross, A.; Fasel, H.F. High-Order-Accurate Numerical Method for Complex Flows. AIAA J. 2008, 46, 204–214. [Google Scholar] [CrossRef]
- Gross, A.; Fasel, H.F. Active Flow Control for NACA 6-Series Airfoil at Re = 64,200. AIAA J. 2010, 48, 1889–1902. [Google Scholar] [CrossRef]
- Brehm, C.; Mack, S.; Gross, A.; Fasel, H.F. Investigations of an Airfoil at Low Reynolds Number Conditions. In Proceedings of the 4th Flow Control Conference, Seattle, WA, USA, 23–26 June 2008. [Google Scholar] [CrossRef]
- Gross, A.; Fasel, H.F. Hybrid Turbulence Model Simulations of Partially Stalled Airfoil Flow. AIAA J. 2016, 54, 1220–1234. [Google Scholar] [CrossRef]
- Margolin, L.G.; Rider, W.J. A rationale for implicit turbulence modeling. Int. J. Num. Meth. Fluids 2002, 39, 821–841. [Google Scholar] [CrossRef]
- Visbal, M.R.; Garmann, D.J. Numerical Investigation of Spanwise End Effects on Dynamic Stall of a Pitching NACA 0012 Wing. In Proceedings of the 55th AIAA Aerospace Sciences Meeting, Grapevine, TX, USA, 9–13 January 2017. [Google Scholar] [CrossRef]
- Broeren, A.P.; Bragg, M.B. Spanwise Variation in the Unsteady Stalling Flowfields of Two-Dimensional Airfoil Models. AIAA J. 2001, 39, 1641–1651. [Google Scholar] [CrossRef]
- Dianics, J.V.; Balthazar, M.A.; Gross, A.; Fasel, H.F. Wind Tunnel and Free-Flight Testing of Active Flow Control for Modified NACA 643-618 Airfoil. In Proceedings of the 31st AIAA Applied Aerodynamics Conference, San Diego, CA, USA, 24–27 June 2013. [Google Scholar] [CrossRef]
- Dianics, J.V.; Ohno, D.M.; Fuggmann, S.D.; Lay, J.; Heim, D.; Fasel, H.F. Numerical and Experimental Wind Tunnel and Flight Testing of Active Flow Control for Modified NACA 643-618 Airfoil. In Proceedings of the 53rd AIAA Aerospace Sciences Meeting, Kissimmee, FL, USA, 5–9 January 2015. [Google Scholar] [CrossRef]
- Sinclair, A.R.; Robins, A.W. A Method for the Determination of the Time Lag in Pressure Measuring Systems Incorporating Capillaries; NACA TN 2793; NASA Langley Research Center: Hampton, VA, USA, 1952.
- Drela, M.; Giles, M.B. Viscous-inviscid analysis of transonic and low Reynolds number airfoils. AIAA J. 1987, 25, 1347–1355. [Google Scholar] [CrossRef]
- Hunt, J.C.R.; Wray, A.A.; Moin, P. Eddies, Stream, and Convergence Zones in Turbulent Flows; Report CTR-S88; Center for Turbulence Research: Stanford, CA, USA, 1988. [Google Scholar]
- Balzer, W.; Fasel, H.F. Direct Numerical Simulation of Laminar Boundary-Layer Separation and Separation Control on the Suction Side of an Airfoil at Low Reynolds Number Conditions. In Proceedings of the 40th Fluid Dynamics Conference and Exhibit, Chicago, IL, USA, 28 June–1 July 2010. [Google Scholar] [CrossRef]
- Embacher, M.; Fasel, H.F. Direct numerical simulations of laminar separation bubbles: Investigation of absolute instability and active flow control of transition to turbulence. J. Fluid Mech. 2014, 747, 141–185. [Google Scholar] [CrossRef]
- Hosseinverdi, S.; Fasel, H.F. Numerical Investigation of the Interaction of Active Flow Control and Klebanoff Modes. In Proceedings of the 47th AIAA Fluid Dynamics Conference, Denver, CO, USA, 5–9 June 2017. [Google Scholar] [CrossRef]
- Hansen, L.; Bons, J. Flow Measurements of Vortex Generator Jets in Separating Boundary Layer. J. Prop. Power 2006, 22, 558–566. [Google Scholar] [CrossRef]
- Postl, D. Numerical Investigation of Laminar Separation Control Using Vortex Generator Jets. Ph.D. Dissertation, University of Arizona, Tucson, AZ, USA, 2005. [Google Scholar]











| Aircraft and Airspeed | Reference Length | Reynolds Number |
|---|---|---|
| 1:5 scale takeoff | Wing tip chord | 64,200 |
| 1:5 scale takeoff | MAC | 137,000 |
| 1:5 scale cruise | MAC | 320,000 |
| Full-size slow flight (26.8 m/s) | MAC | 1,500,000 |
| Full-size cruise (56.6 m/s) | MAC | 3,200,000 |
| cl | cd | |
|---|---|---|
| Uncontrolled | 0.654 | 0.121 |
| 2D volume forcing | 1.26 | 0.0473 |
| VGJs, B = 1 | 1.24 | 0.0465 |
| VGJs, B = 4 | 1.12 | 0.0485 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gross, A.; Fasel, H.F. Active Control of Laminar Separation: Simulations, Wind Tunnel, and Free-Flight Experiments. Aerospace 2018, 5, 114. https://doi.org/10.3390/aerospace5040114
Gross A, Fasel HF. Active Control of Laminar Separation: Simulations, Wind Tunnel, and Free-Flight Experiments. Aerospace. 2018; 5(4):114. https://doi.org/10.3390/aerospace5040114
Chicago/Turabian StyleGross, Andreas, and Hermann F. Fasel. 2018. "Active Control of Laminar Separation: Simulations, Wind Tunnel, and Free-Flight Experiments" Aerospace 5, no. 4: 114. https://doi.org/10.3390/aerospace5040114
APA StyleGross, A., & Fasel, H. F. (2018). Active Control of Laminar Separation: Simulations, Wind Tunnel, and Free-Flight Experiments. Aerospace, 5(4), 114. https://doi.org/10.3390/aerospace5040114






