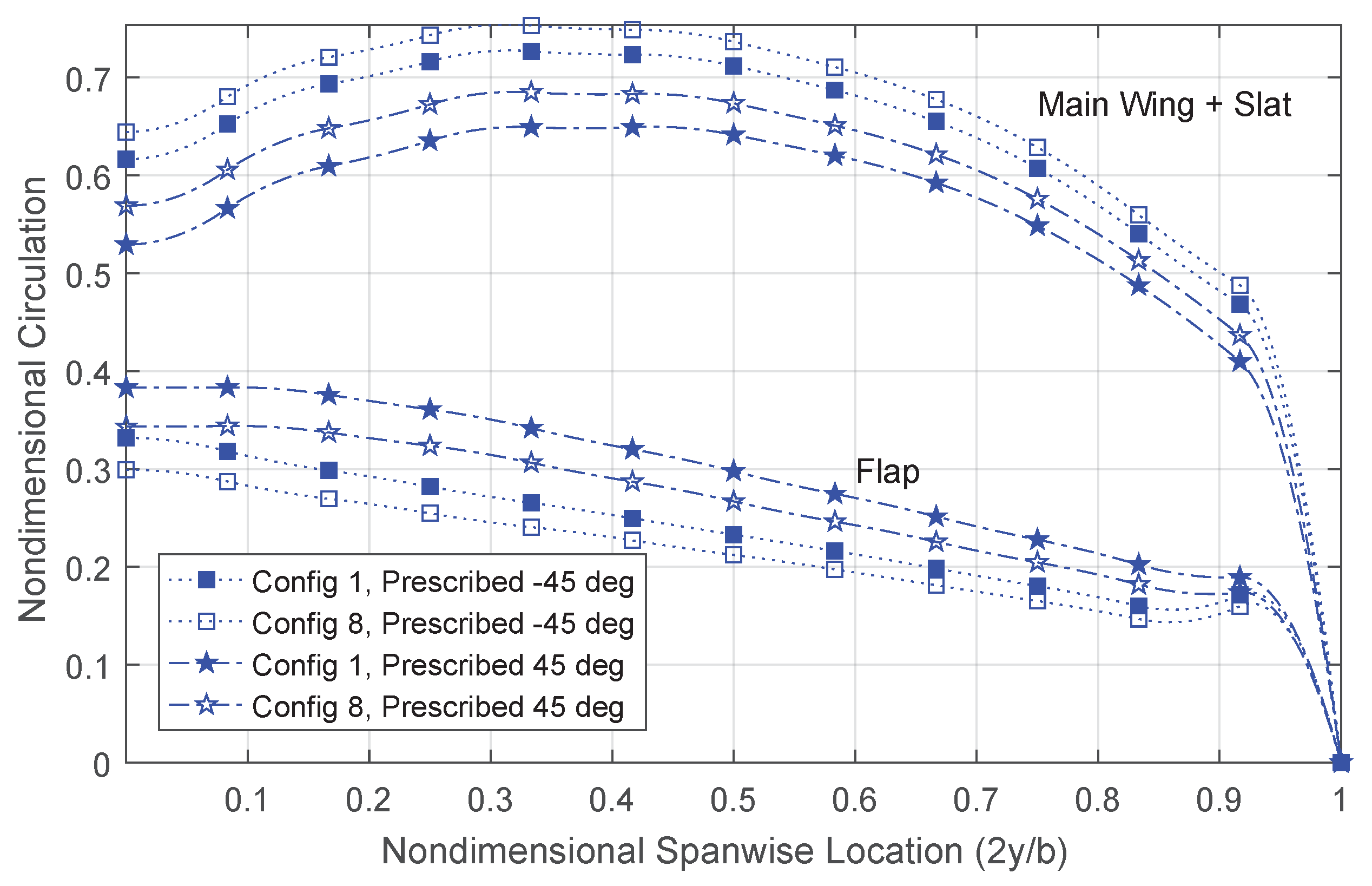
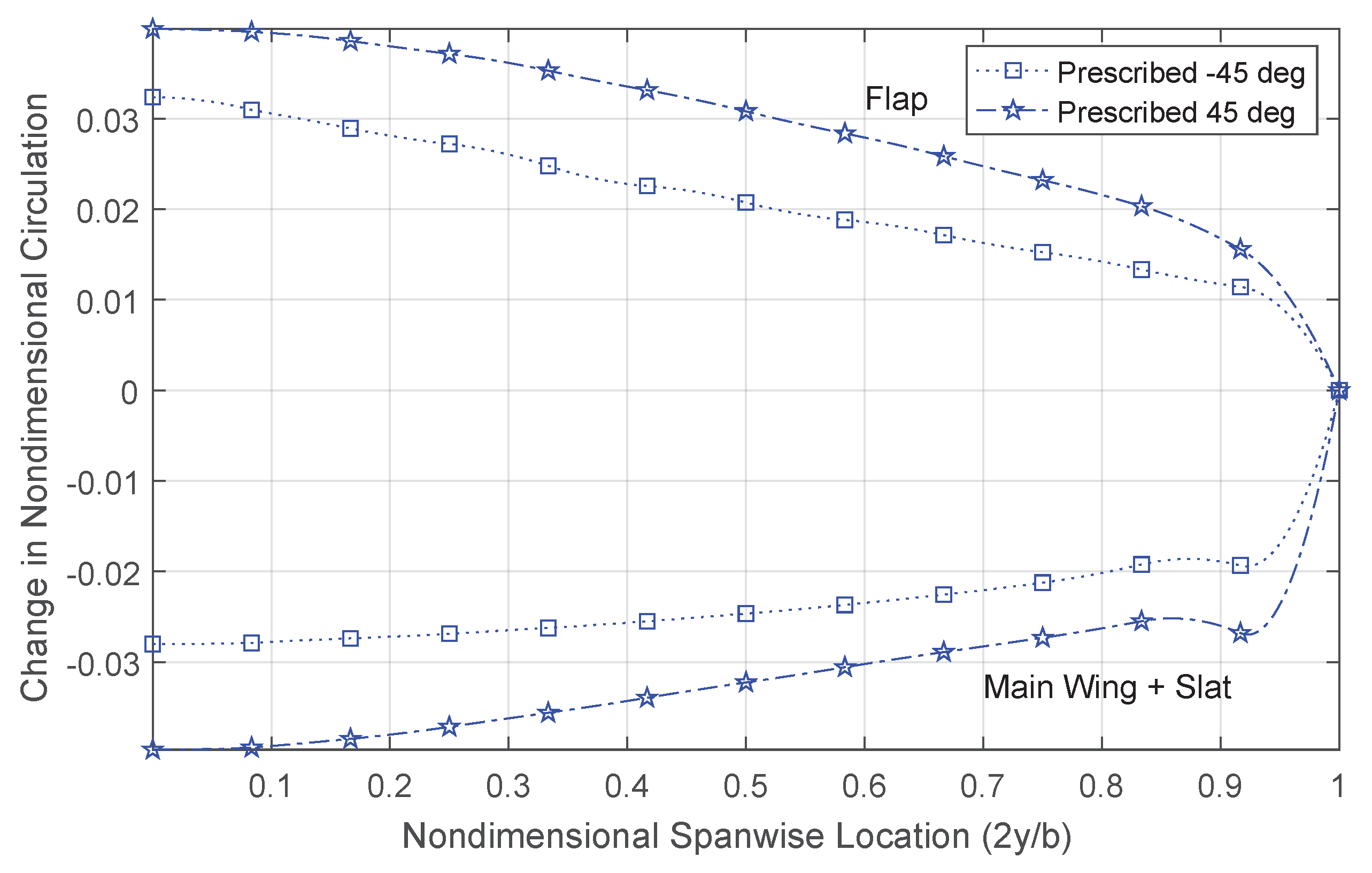
The study of the impact of various wakes on the predicted changes in aerodynamic performance is approached in three steps. First, the impact of various wake shapes is explored using a simple rectangular wing. In the next step, this exploration is extended to a complex high-lift system that consists of a slat and a slotted flap in addition to the main wing. The prediction results are directly compared with experimental data in order to determine the quality of the predictions. In a third step, the relative aerodynamic performance changes due to changes in configuration of the high-lift system are investigated using predictions based on the different wake models. As in the second step, experimental results provide a baseline.
4.1. Single Rectangular Wing
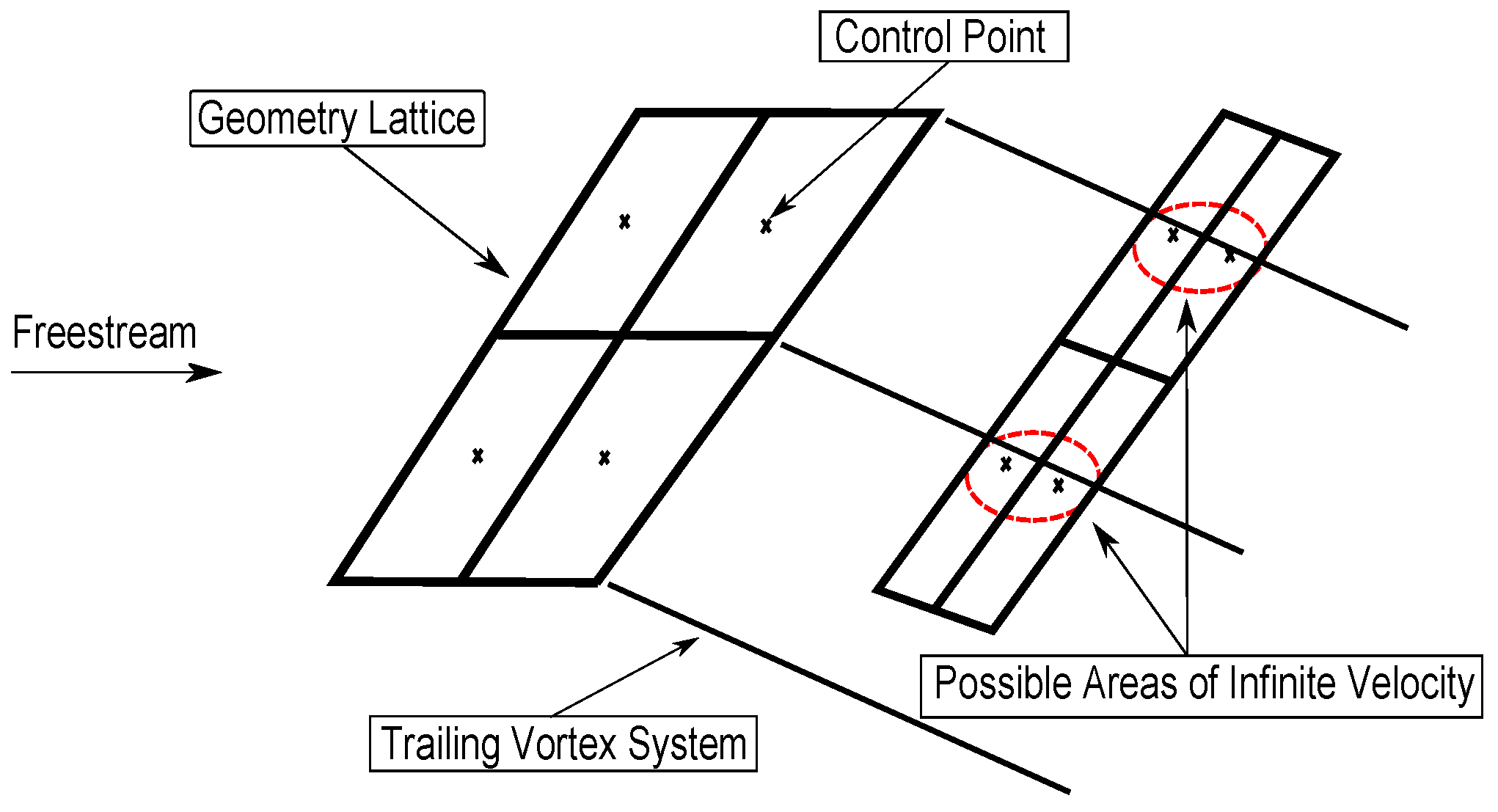
To analyse the effects of wake shapes on a single lifting surface, a rectangular wing with a symmetrical airfoil shape and an aspect ratio of 10 was analysed using the higher-order potential flow method. A convergence study was completed on the number of elements used to model the lifting surface for each type of wake used. The minimum number of elements in both the chordwise direction and the spanwise direction that produced converged results was then used for the remainder of the study on the rectangular wing.
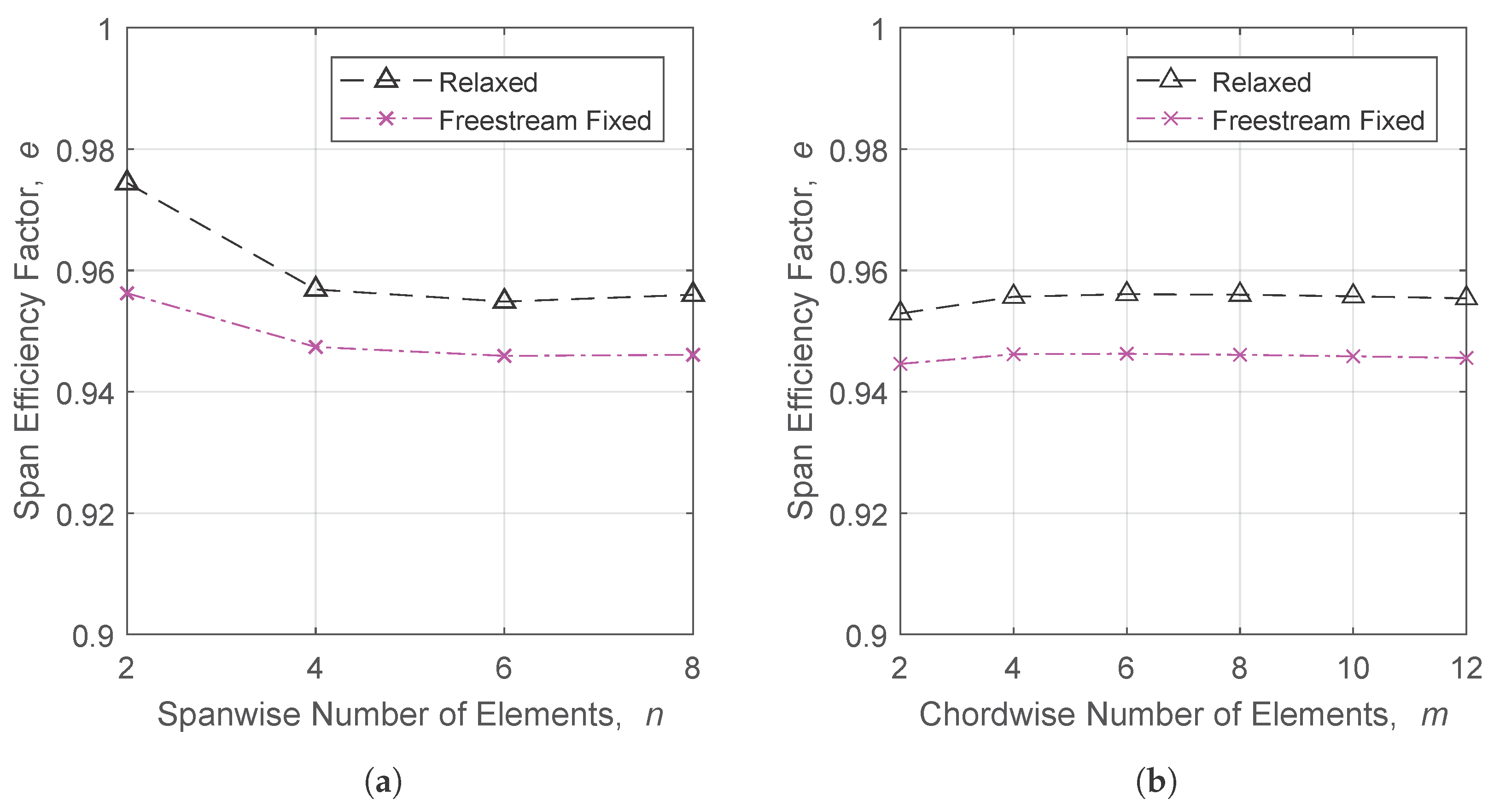
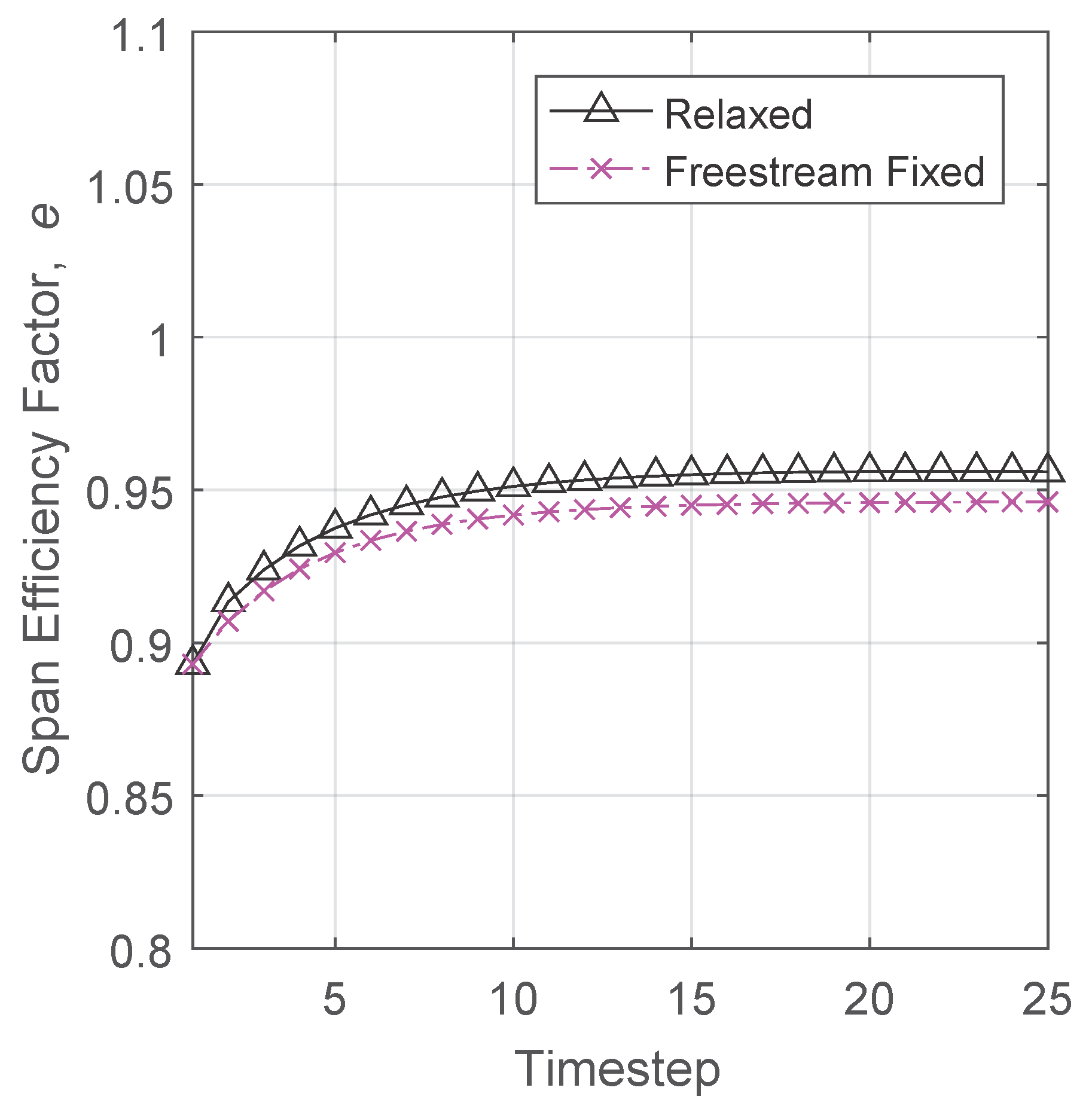
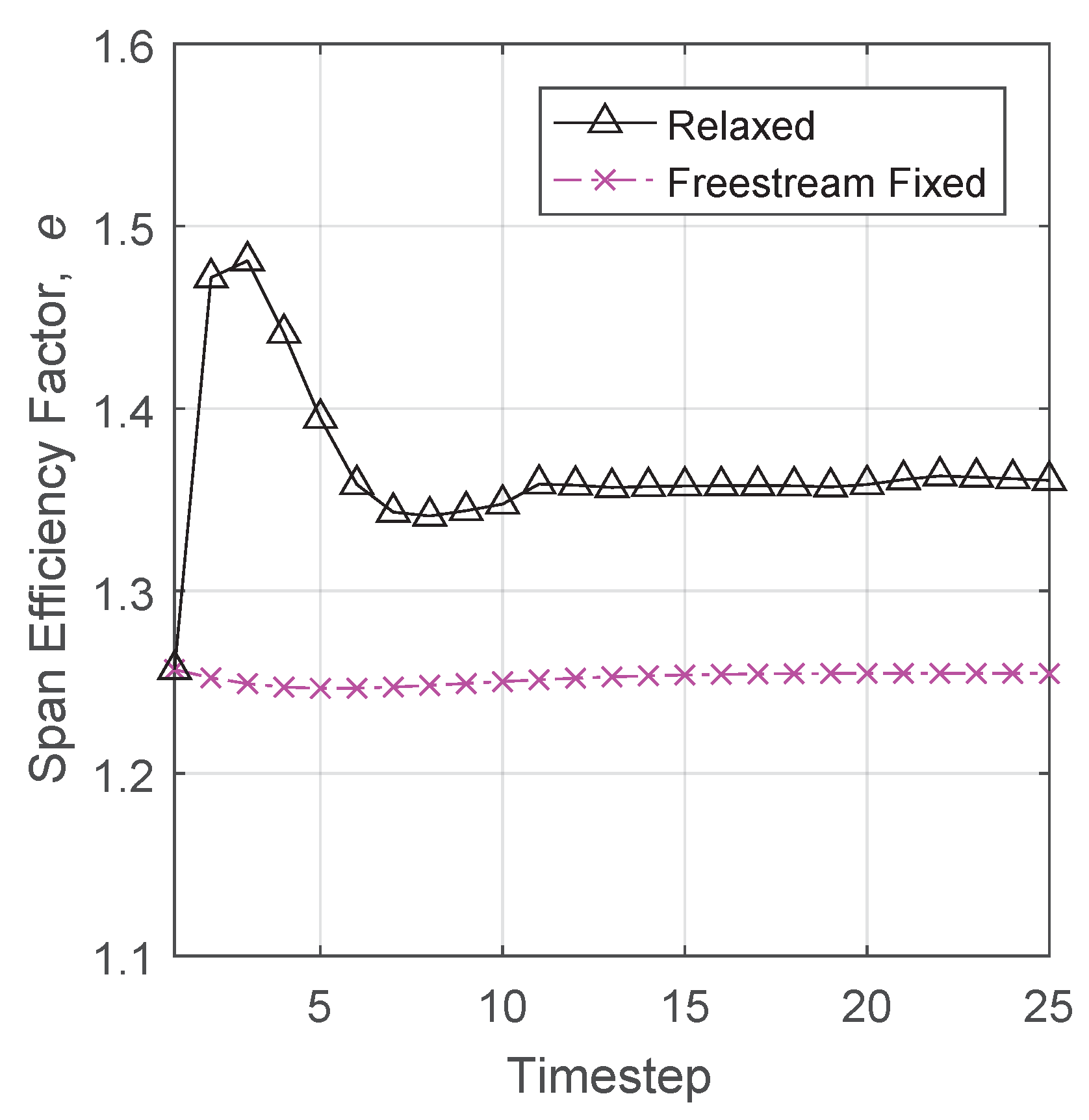
Figure 5a shows the convergence of the span efficiency factor at an angle of attack of 10 degrees, using eight elements in the chordwise direction while increasing the number of spanwise elements, for three of the wake types tested. Convergence appears to be reached when using eight or more spanwise elements, regardless of the wake shape. Similar trends were found when using wakes prescribed to other angles. This study was repeated while holding the number of spanwise elements constant at eight and increasing the number of chordwise elements until the span efficiency factor converged. The results are shown in
Figure 5b. Again, convergence for each wake type is reached when using eight or more elements in the chordwise direction. Based on these results, eight chordwise elements and eight spanwise elements were used to model the rectangular wing half-span for the remainder of the rectangular wing study.
In general, it can be concluded for single, high aspect ratio wings that the wake shape has negligible influence on the convergence with changing element density. As a result, the aerodynamic properties of simple configurations can be found without having to perform multiple convergence studies if multiple wake types are to be used. This means that, for this study, the same configuration can be modelled with multiple wake types without having to change the discretization of the surface to achieve the convergence of the desired parameters.
The size of the wake to be used for the study was selected based on the convergence of span efficiency, as the wake grew in length with increasing numbers of time step iterations.
Figure 6 shows the convergence of these parameters as the wake grows using eight spanwise and eight chordwise elements to model the half-span. As can be seen, the results have converged by 20 time steps, or after the wing has advanced a distance equal to five wing root chords. This was similar for all wake types used and therefore was used for the remainder of the study on the rectangular wing. Since convergence of the span efficiency as the wake grows is independent of the wake type for this single rectangular wing, the same configuration can be modelled with multiple wake types for this study, without having to change the size of the wake to achieve convergence.
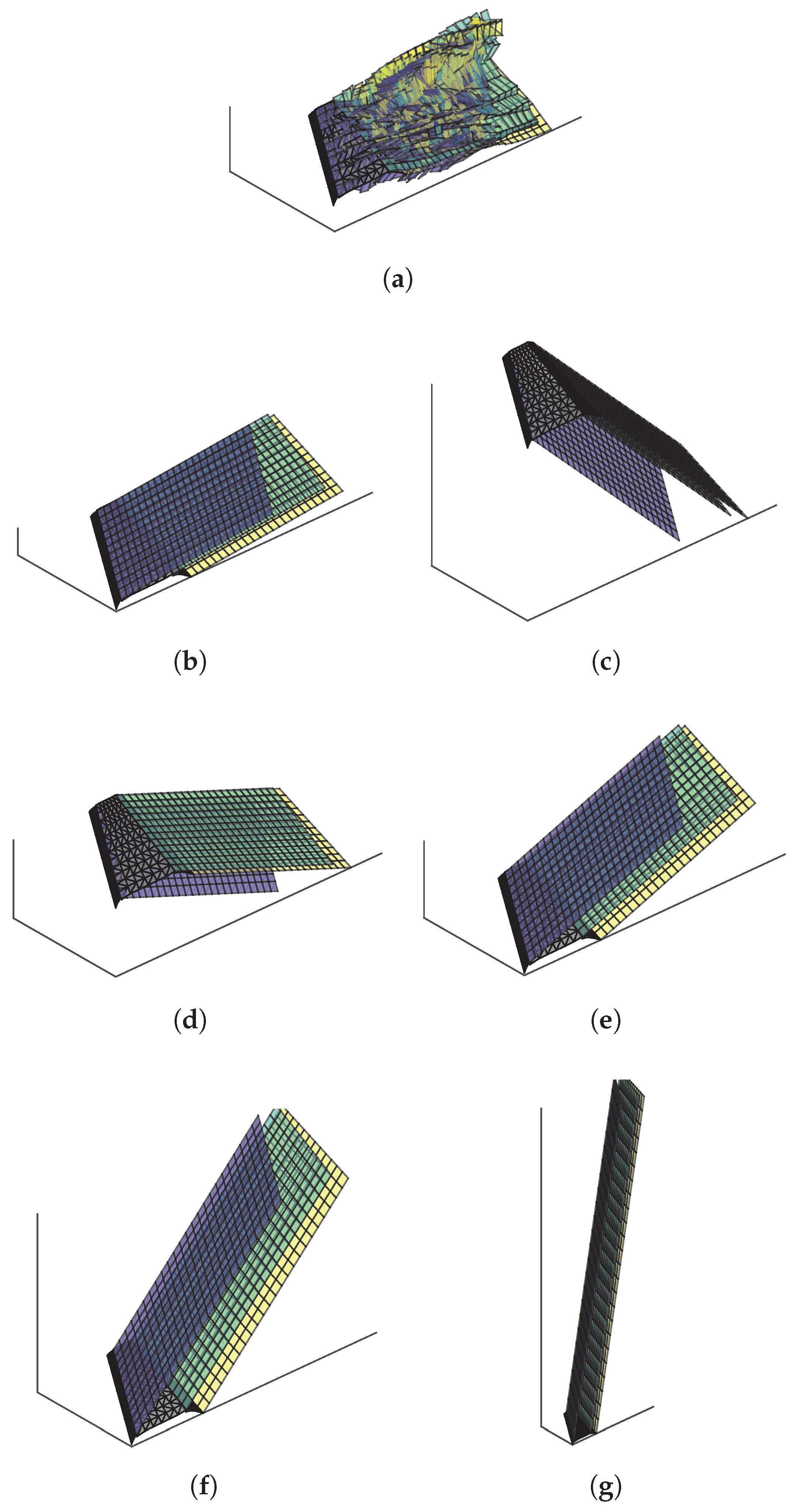
Plots of the five wake shapes tested are shown on the rectangular wing half-span in
Figure 7, for an analysis at an angle of attack of six degrees. Note that the prescribed angles and freestream direction are with respect to the global X–Y plane, shown in
Figure 3. As such, an analysis performed using a freestream fixed, drag-free wake at an angle of attack of 10 degrees, for example, would produce the same result as an analysis with a wake prescribed to +10 degrees.
The lift and drag polars for results obtained using a relaxed wake, a drag-free wake and wakes prescribed to 10, 45 and 80 degrees above the zero-lift line are plotted in
Figure 8. The results predict very little differences in the lift and induced drag for all wake shapes except the wakes prescribed to extreme angles. As shown in
Figure 9, with high positive wake angles, the velocity induced by the wake on the wing has a large component in the freestream direction. This results in an increase in the predicted lift when comparing high wake angles to drag-free wakes. Simultaneously, the component of the wake-induced velocity normal to the freestream direction is reduced, resulting in a smaller angle of attack reduction when compared to a freestream fixed wake. Thus, the predicted induced drag is decreased as the wake angle increases. An interesting side note is that a wake prescribed at positive mto the freestream will produce zero induced drag with the method. Additionally, although not tested in this case, a wake prescribed below the freestream direction will decrease lift, since the incoming flow velocity is reduced by the wake-induced velocity. This will also produce less induced drag than a freestream fixed wake. Therefore, a freestream fixed wake will produce the most induced drag for a given lift coefficient, for a single wing configuration. These correlations between prescribed wake shapes and aerodynamic predictions are also applicable to the relaxed, force-free shapes. In such cases, the effects are applied to each element in the wake, rather than the plane of the entire wake.
4.2. NASA Trap Wing
To study the effects of wake shapes on high-lift system predictions, the NASA Trap Wing was used as a source of experimental data to compare predictions to [
19].
Table 1 provides geometrical data for the stowed configuration, which is used for non-dimensionalization of forces when applicable.
The specific NASA Trap Wing experimental configurations under consideration are from the LaRC 14 × 22 ft low Reynolds number entry and are outlined in
Table 2. This experiment took place at a Reynolds number of 4.3 million and at a freestream Mach number of 0.2. The corresponding force and pressure data are provided in [
19] with wind tunnel boundary corrections applied.
Using this model allows for comparison to a multi-element system, which has been analysed using several modern CFD solvers [
20,
21,
22,
23,
24].
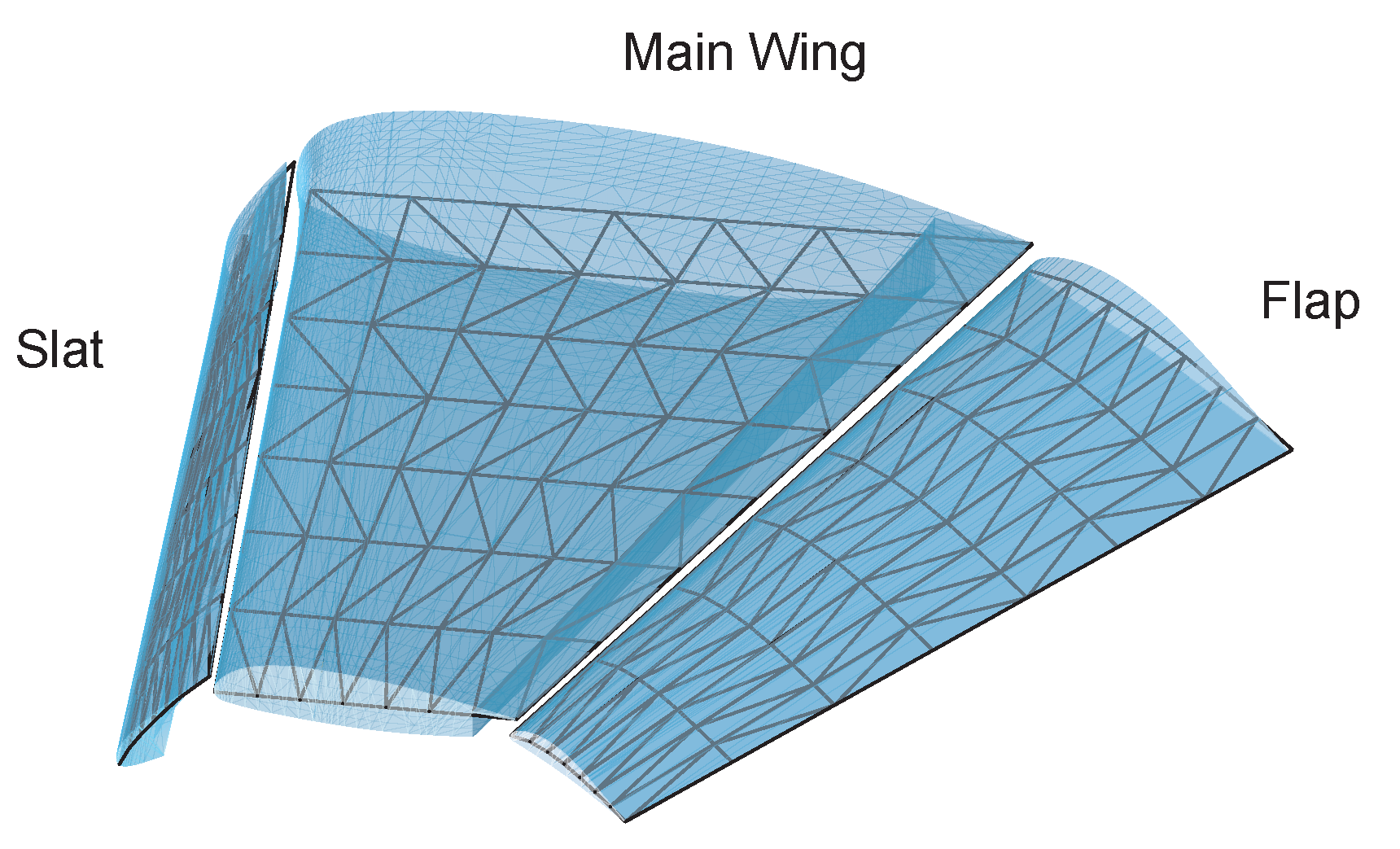
Figure 10 shows the three element system with the slat and flap in the LaRC Configuration 1 position, modelled using higher-order elements as described in [
10]. A preliminary study showed that lift and induced drag predictions using this inviscid method are relatively insensitive to the specific positioning of the camber line of the slat and main wing. Note that the slat and flap are sealed at the root and that the model does not include the main body cove that was used in the experiment.
Although not shown here, this study has found that predicted performance changes of prescribed wakes exhibited very little sensitivity to panel-density changes. However, results found when using a relaxed wake do differ, and therefore, a convergence study was completed on the NASA Trap Wing LaRC Configuration 1 at an angle of attack of 20 degrees to determine the minimum number of elements needed to model the wing while achieving converged results.
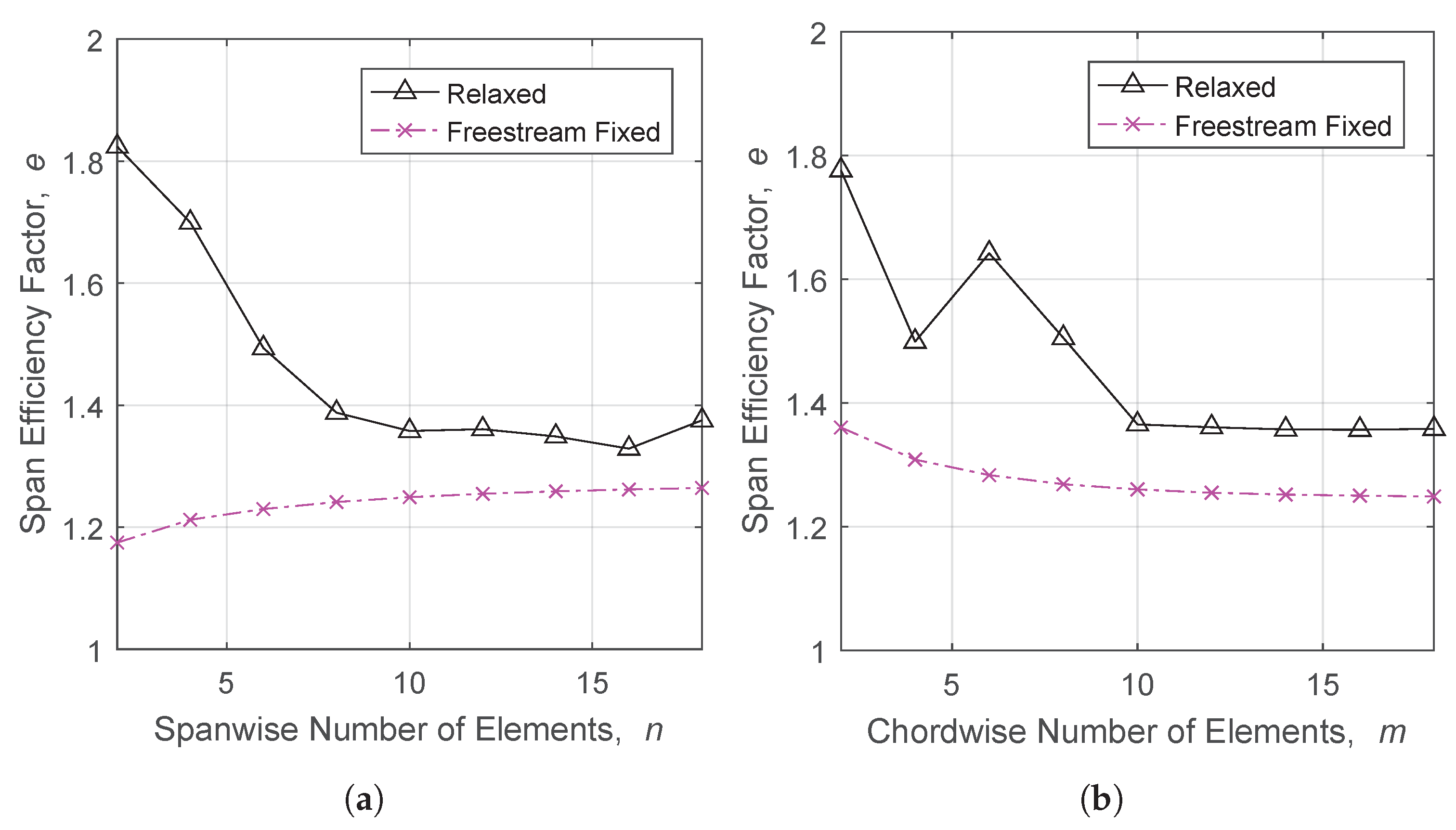
Figure 11a shows the convergence of the span efficiency factor as the number of spanwise elements is increased. For results found when using a freestream fixed wake model, good convergence can be seen when 12 elements are used in the spanwise direction. Similar results were found for all prescribed wake models, as well. In contrast to the results of the rectangular wing study, the span efficiency convergence when using a relaxed wake is more erratic when modelling multiple lifting surfaces with multiple wakes. This is due to the changing relaxed wake shape as the number of spanwise elements is increased and the strong interaction between wakes and surfaces. Nonetheless, the results attained with more than 12 spanwise elements produced results that were deemed accurate enough for this study. This number was then used to determine the number of elements needed in the chordwise direction.
Figure 11b shows the convergence in span efficiency as the number of chordwise elements is increased. Since the model includes camber, increasing the number of elements in the chordwise direction generates a geometry closer to the physical configuration. Based on these figures, 12 elements in the chordwise direction were chosen to be suitable for the subsequent study of the NASA Trap Wing.
The size of the wake used for the study was based on the convergence of span efficiency as the wake grew in length. In
Figure 12, convergence for both relaxed and fixed wake models can be seen when the number of time steps is equal to 20. This corresponds to a wake length that is two-times the root chord length in the streamwise direction. Since this trend continued for all wake shapes used, this wake size was used for this wake shape study on the NASA Trap Wing.
The seven wake positions corresponding to a relaxed wake, a freestream fixed (drag-free) wake and wakes prescribed to −45, −20, +20, +45 and +80 degrees are shown in
Figure 13. Similar to the single rectangular wing study, the global X–Y plane is used as the reference plane for the freestream direction and prescribed wake angles. This means that an analysis at an angle of attack of 10 degrees with a freestream fixed wake would produce a wake that is identical to a wake prescribed to 10 degrees.
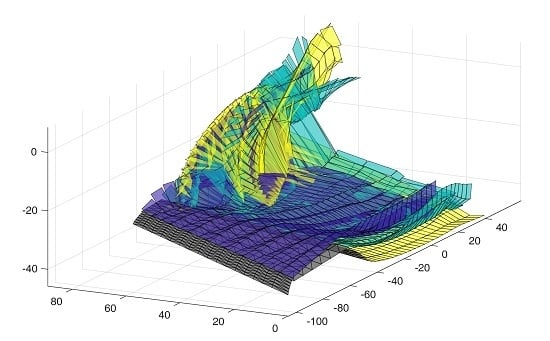
Note that each of the three lifting surfaces sheds a wake from its respective trailing edge. The wake of each lifting surface has been represented in
Figure 13 using different colors. Blue elements represent the wake shed from the slat, green elements are shed from the main wing, and yellow elements represent the wake shed from the flap. For a select number of cases, it can be seen that a wake passes through a downstream element. Although this is non-physical, the method can handle it without numerical issues. In terms of performance, it has been shown that the velocities induced by a body-piercing wake are almost identical to that of a properly-treated body-conforming wake [
25].
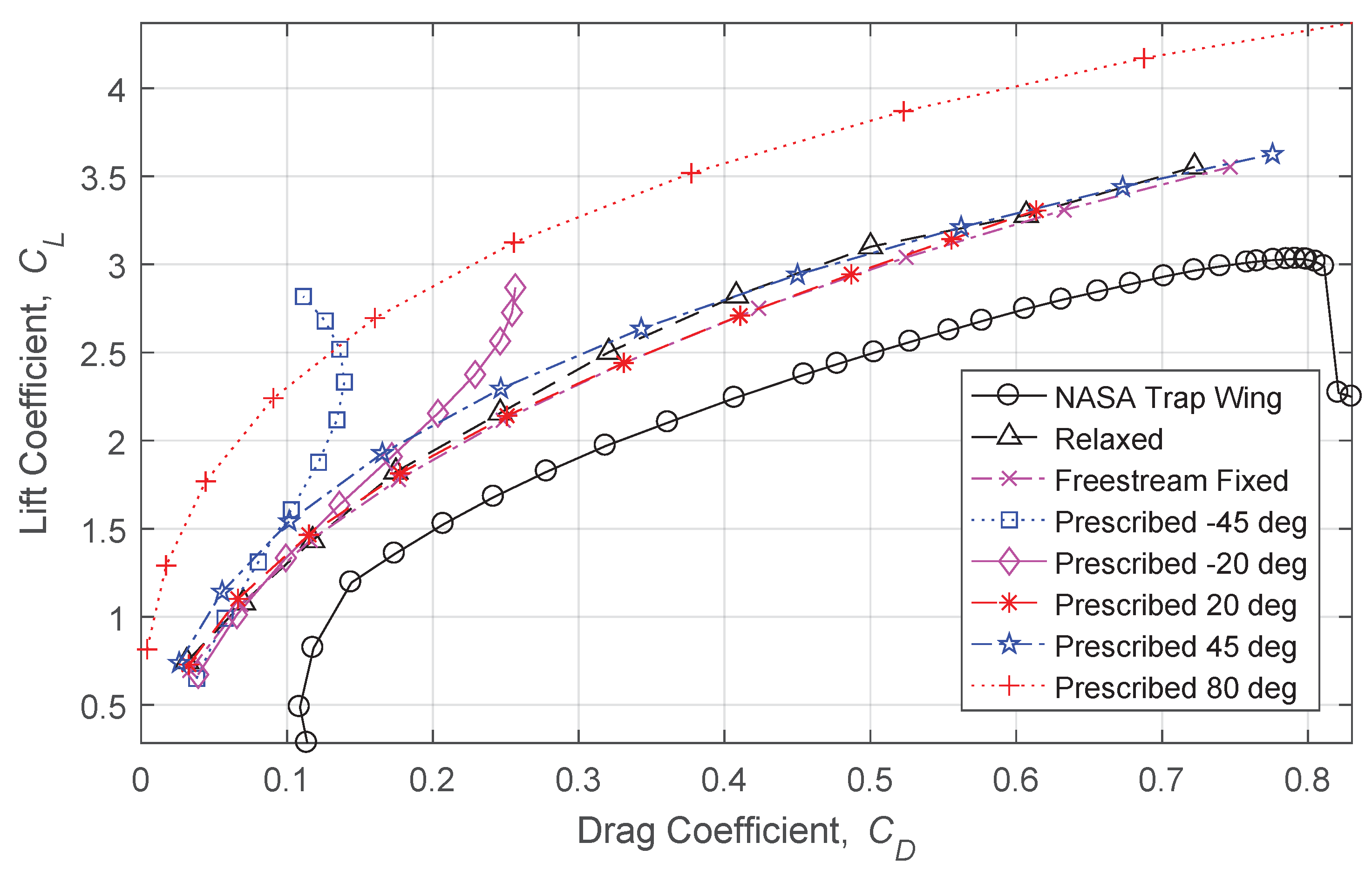
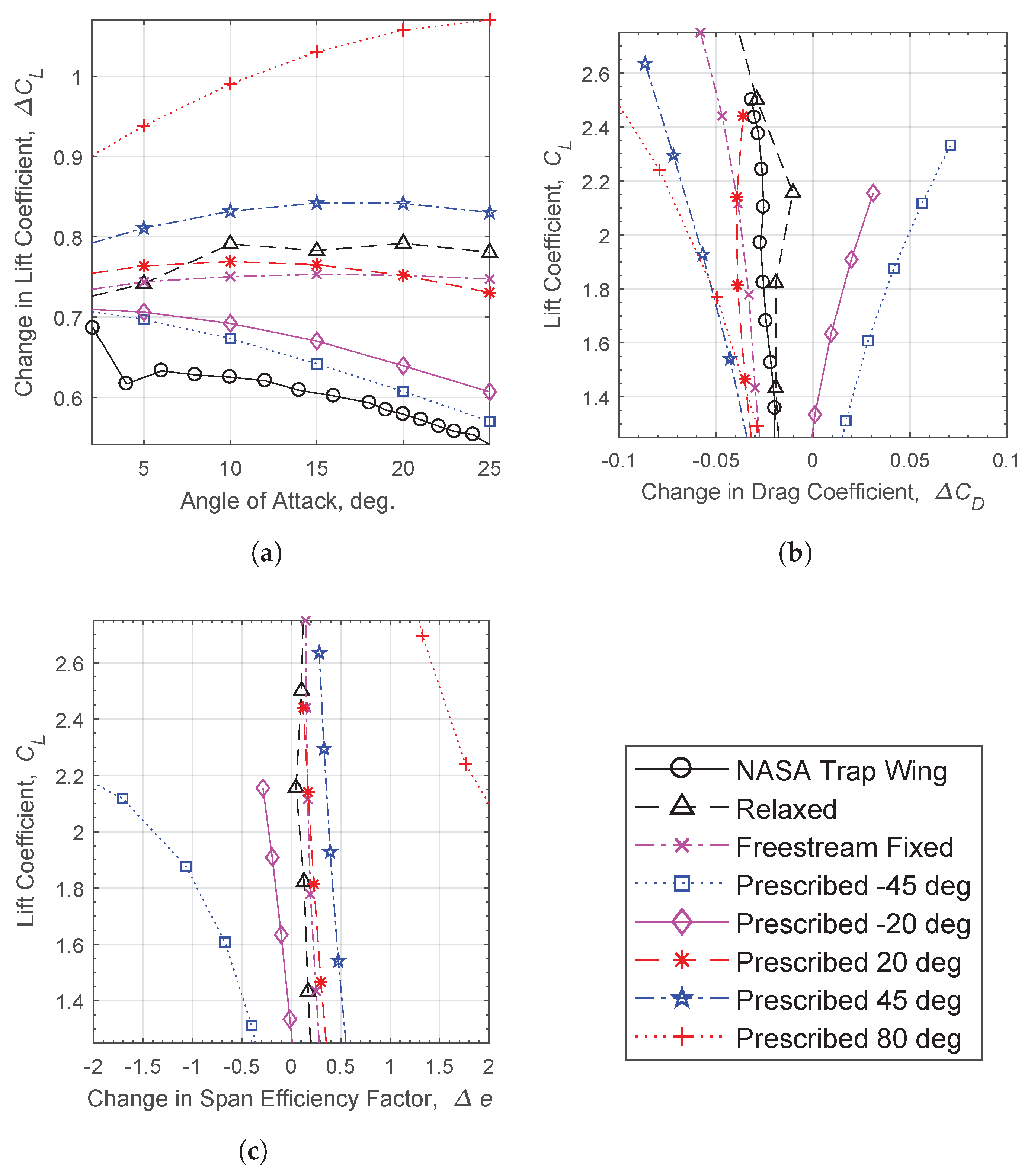
Figure 14 and
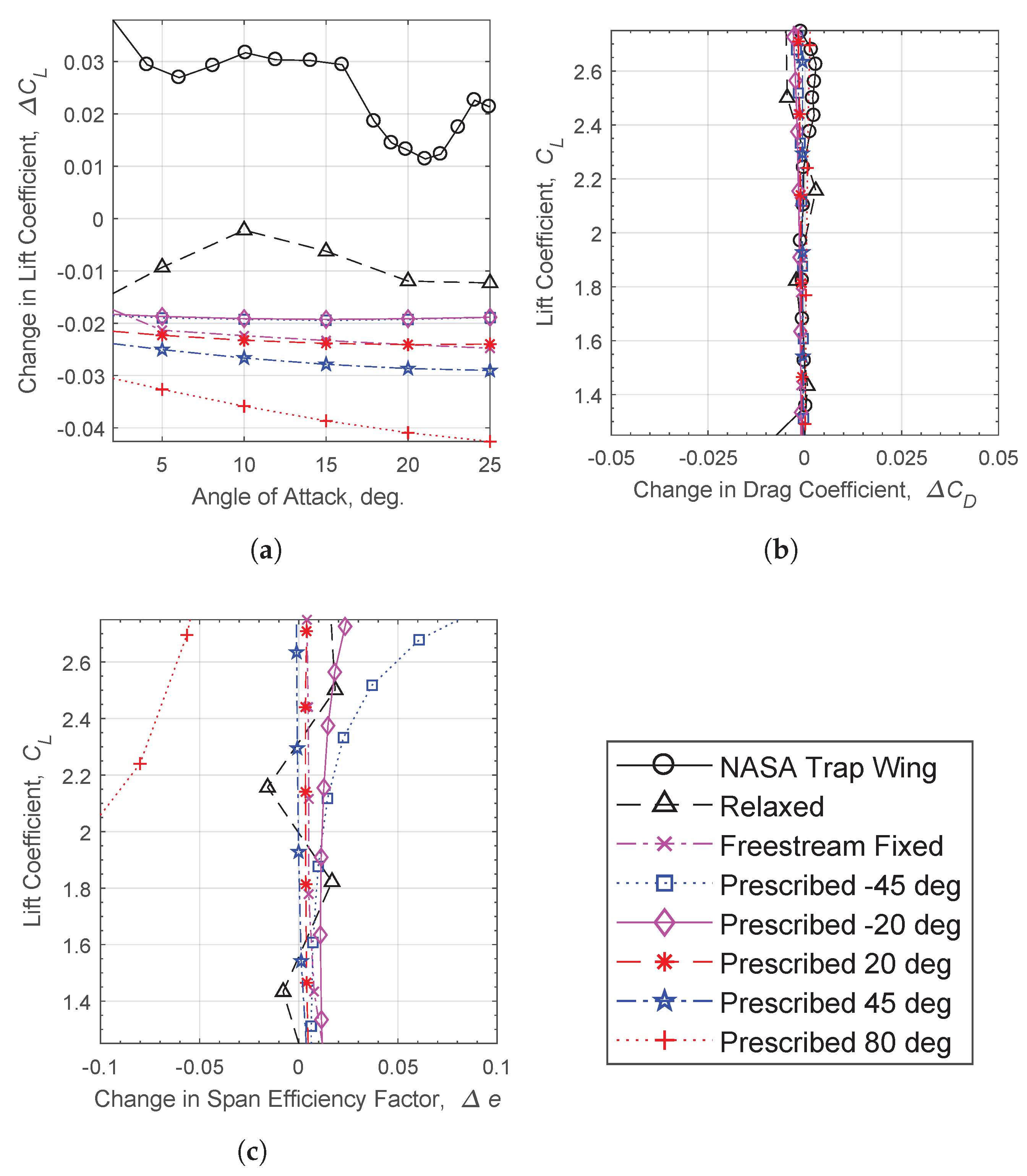
Figure 15 compare the results of the study to the experimentally-obtained NASA Trap Wing LaRC Configuration 1 data. It must be noted that the regions above an angle of attack of 30 degrees and below an angle of attack of five degrees are not compared in this study. It is assumed that viscous effects, in the form of significant flow separation, dominate in these regions. The current analysis method uses no viscous model and therefore cannot predict such effects. Hence, the predicted drag values are that of only induced drag, whereas the experimental drag values are total drag. Therefore, an offset in drag is expected, and only the general shape of the polars is being compared.
As was found with the rectangular wing study, the freestream fixed wake produces the most induced drag for a given lift coefficient. This value then decreases as the wake angle is tilted away from the freestream angle. The limit of zero predicted induced drag occurs when a wake is prescribed perpendicular to the freestream direction. This explains why, when analysing with negative prescribed wake angles, the induced drag predictions begin to decrease above a specific lift coefficient. The intersection points between the predictions of two prescribed wake angles represent two different wake shapes that predict the same induced drag at the same lift coefficient.
As was previously mentioned, a wake prescribed to the freestream angle will produce the same aerodynamic predictions as a freestream fixed wake. At lower and higher freestream angles, the prescribed wake will predict less induced drag. Therefore, the predicted drag polars will not be of the same shape, as shown in
Figure 14. As a result, for a given lift coefficient, wakes prescribed to angles less than 10 degrees from the freestream angle of attack produced very similar results to the freestream fixed wake.
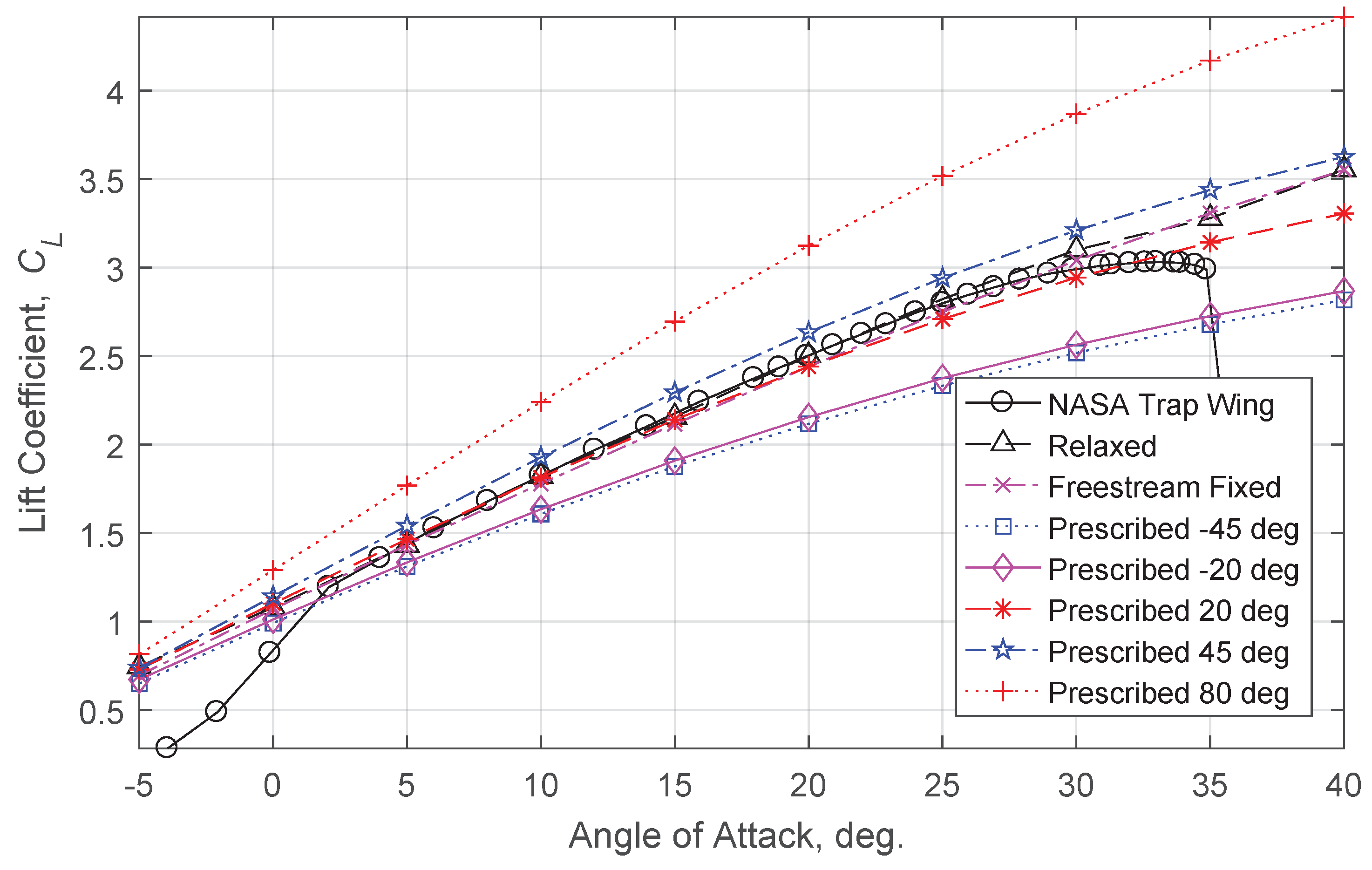
As can be seen in
Figure 15, the major difference between the predicted lift coefficients using different wake types is the lift curve slope. The predictions follow the rule that at a given angle of attack, lift coefficients deviate approximately 3.3% for every 10 degree difference between the prescribed wake angle and the freestream direction. Overall, predictions using relaxed wakes, freestream fixed wakes or wakes that are prescribed above the freestream direction, at angles of less than 45 degrees, agree relatively well with the experimental results. The latter is true since the angle of attack range in question is positive and less than 45 degrees. This also means that predictions using wake models that are prescribed below the freestream direction differ significantly from the experimental results and underpredict the lift curve slope.
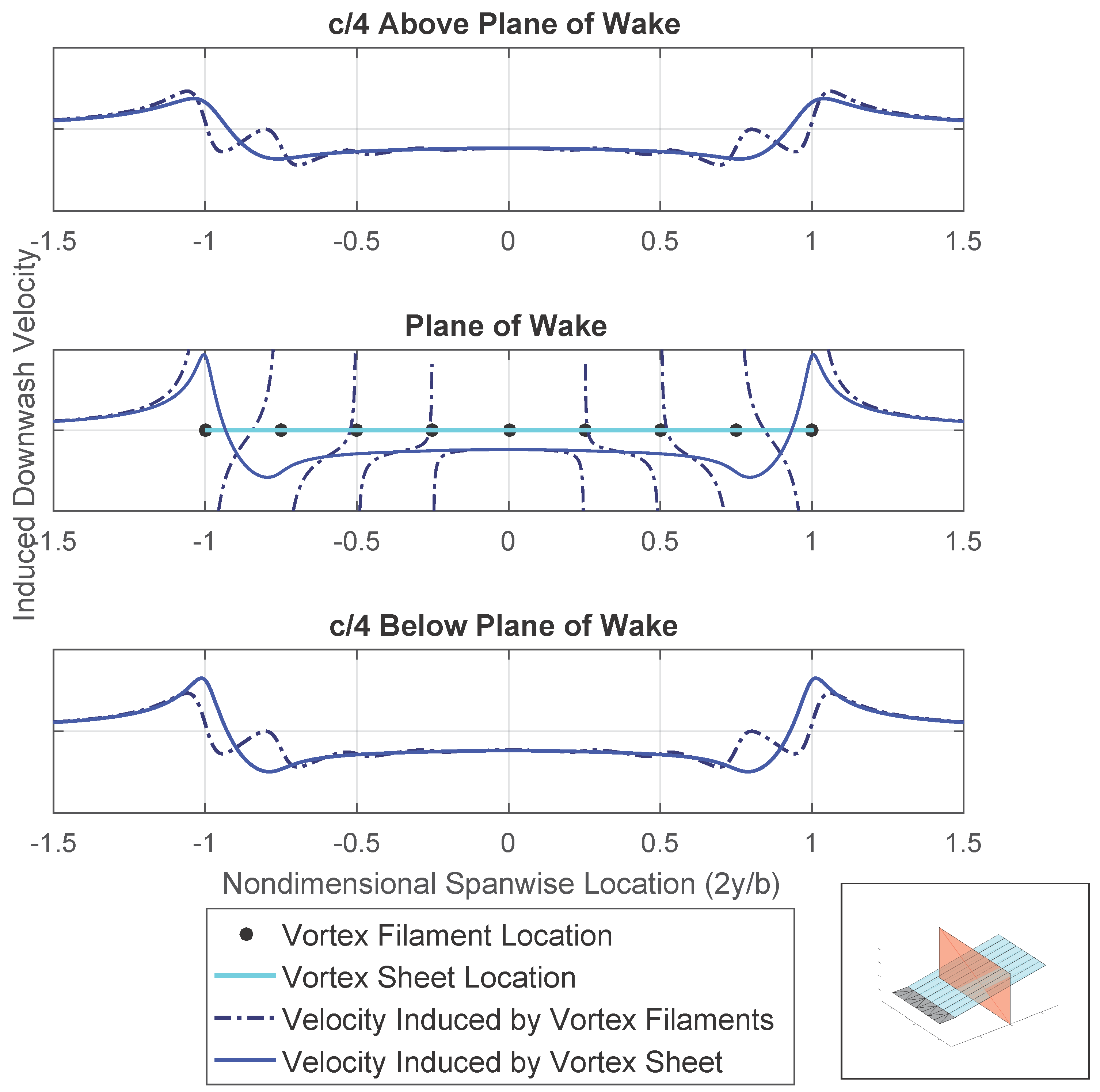
The different impact that the wake models have on the predicted lift curve slope is due to the combination of two effects. The first effect is that the wake of a lifting surface affects the incoming flow vector. This is in line with observations that were made with the single rectangular wing analysis and is depicted in
Figure 9. The second effect is unique to cases with multiple lifting elements. Since the downwash velocities are highest in the plane of the wake itself, prescribing a wake to a defined angle will affect the strength of the downwash field that a subsequent lifting surface passes through. The resulting spanwise circulation distributions will be affected, as shown for multiple wake types in
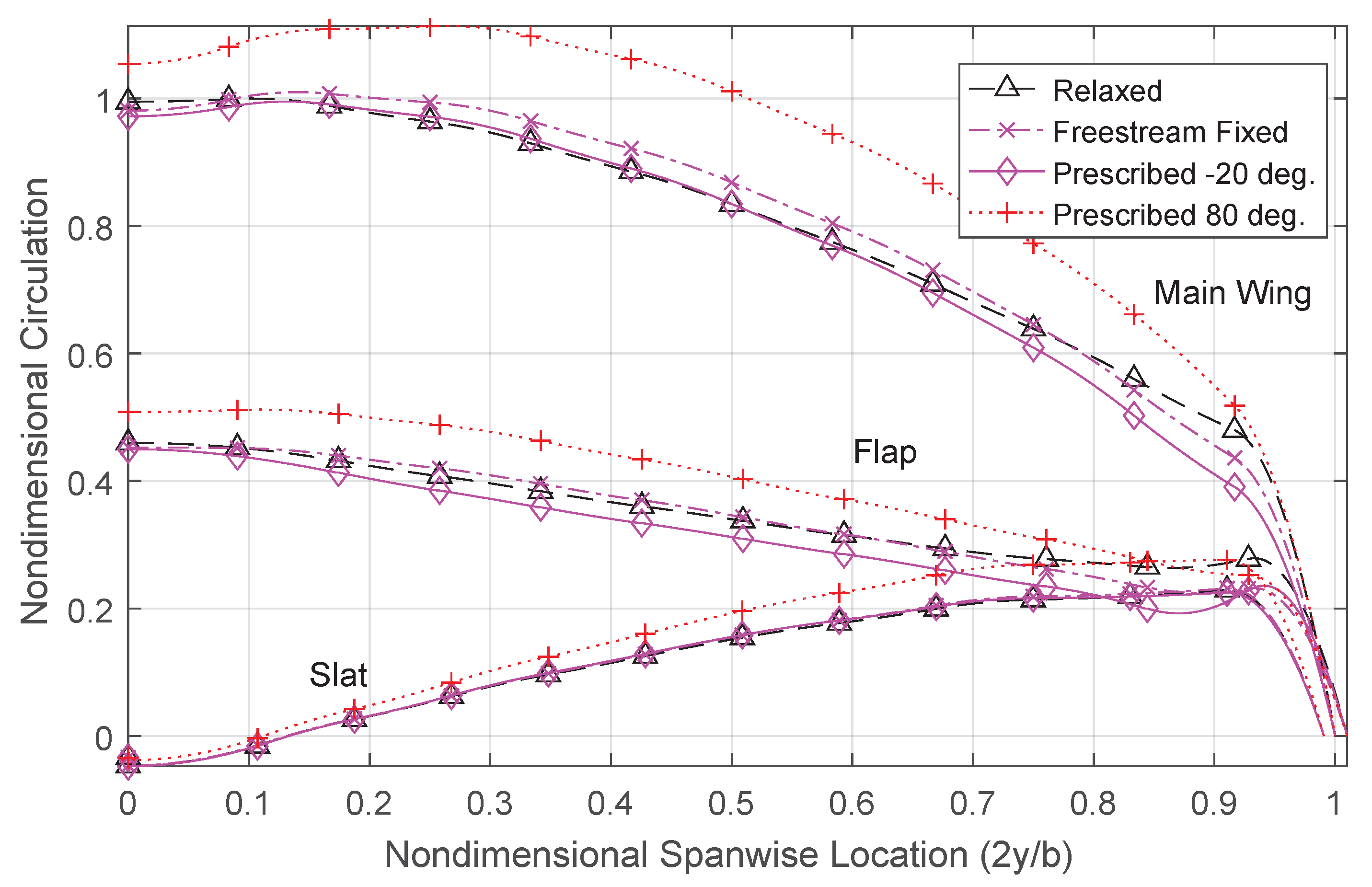
Figure 16. Since the trailing edge of the slat and main wing shed their wakes on top of the subsequent lifting surfaces, prescribing a wake angle to any angle above or below the subsequent lifting surface decreases the downwash at that location. Alternatively, prescribing a wake that passes through a downstream lifting surface results in large downwash velocities at the downstream location.
The results find the same conclusions that were made during the canard wing studies by Miller and Youngblood [
13] and further explored by Madson and Erickson [
15].
Figure 15 shows that a relaxed wake provides results closest to experimental data, and a freestream fixed wake will provide more lift at a given angle of attack when compared to a wake aligned close to the body.
The spanwise circulation distributions shown below are normalized to the circulation at the root of the main wing, as found when using a relaxed wake shape. Negative circulation at the inboard section of the slat produces a net downwards force at that location. This may be physical, due to the nature of the slat; however, it is likely due to the simplifications required to model a complex, three-dimensional surface with an infinitely thin wing. These problems, and the solution taken, are described in [
10].
As expected, the circulation values produced when using a wake prescribed to positive angles are higher than those predicted with a relaxed wake, except at the tip of the flap. This is a result of the relaxed wakes from the slat and main wing being rolled-up to angles higher than 80 degrees as they pass over the flap. This reduces the downwash and increases the velocities in the freestream direction at the location of the flap more than the wakes prescribed to high angles. The opposite effect happens with wakes prescribed to angles below the freestream direction.