Numerical Study of Transition of an Annular Lift Fan Aircraft
Abstract
:1. Introduction
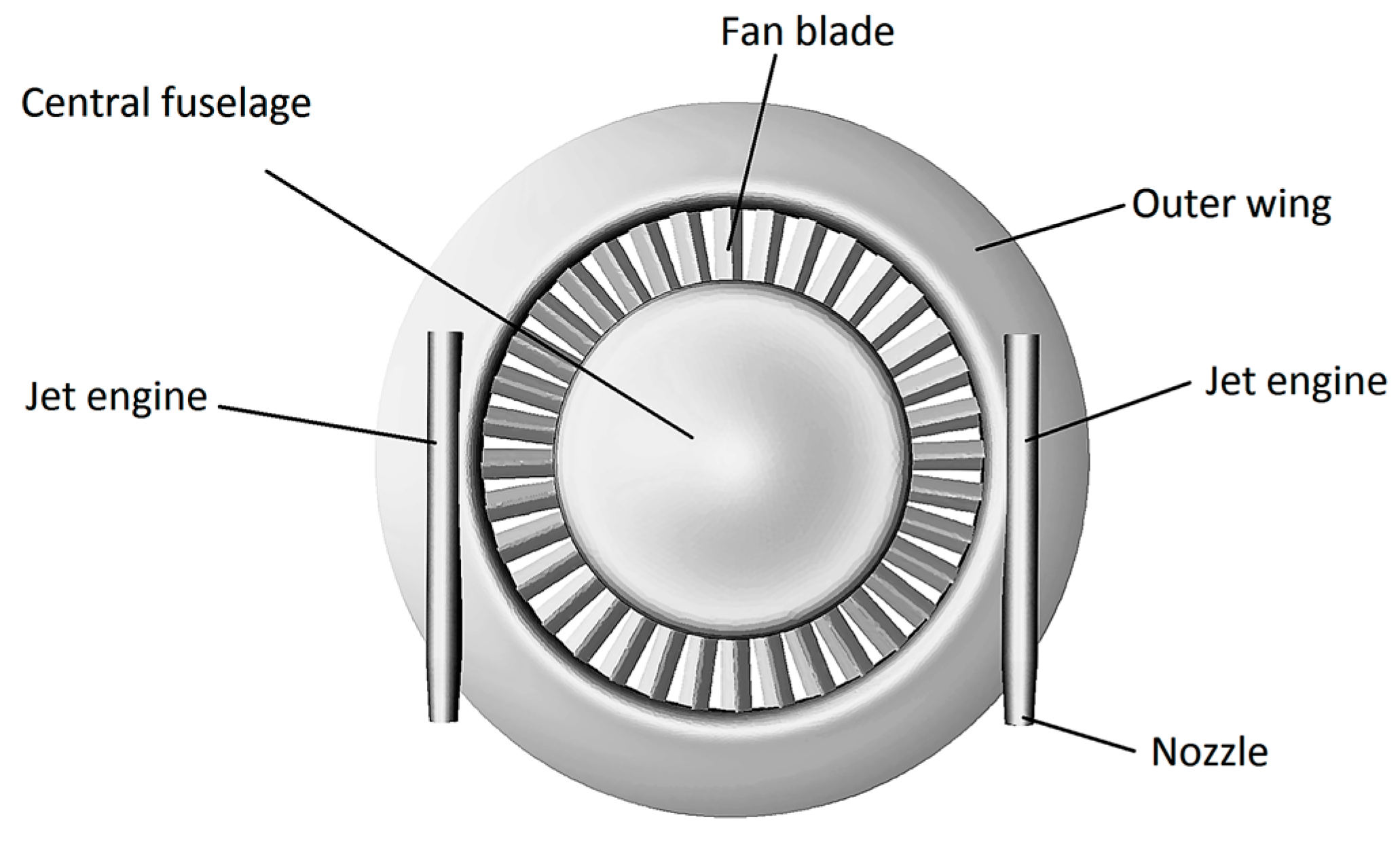
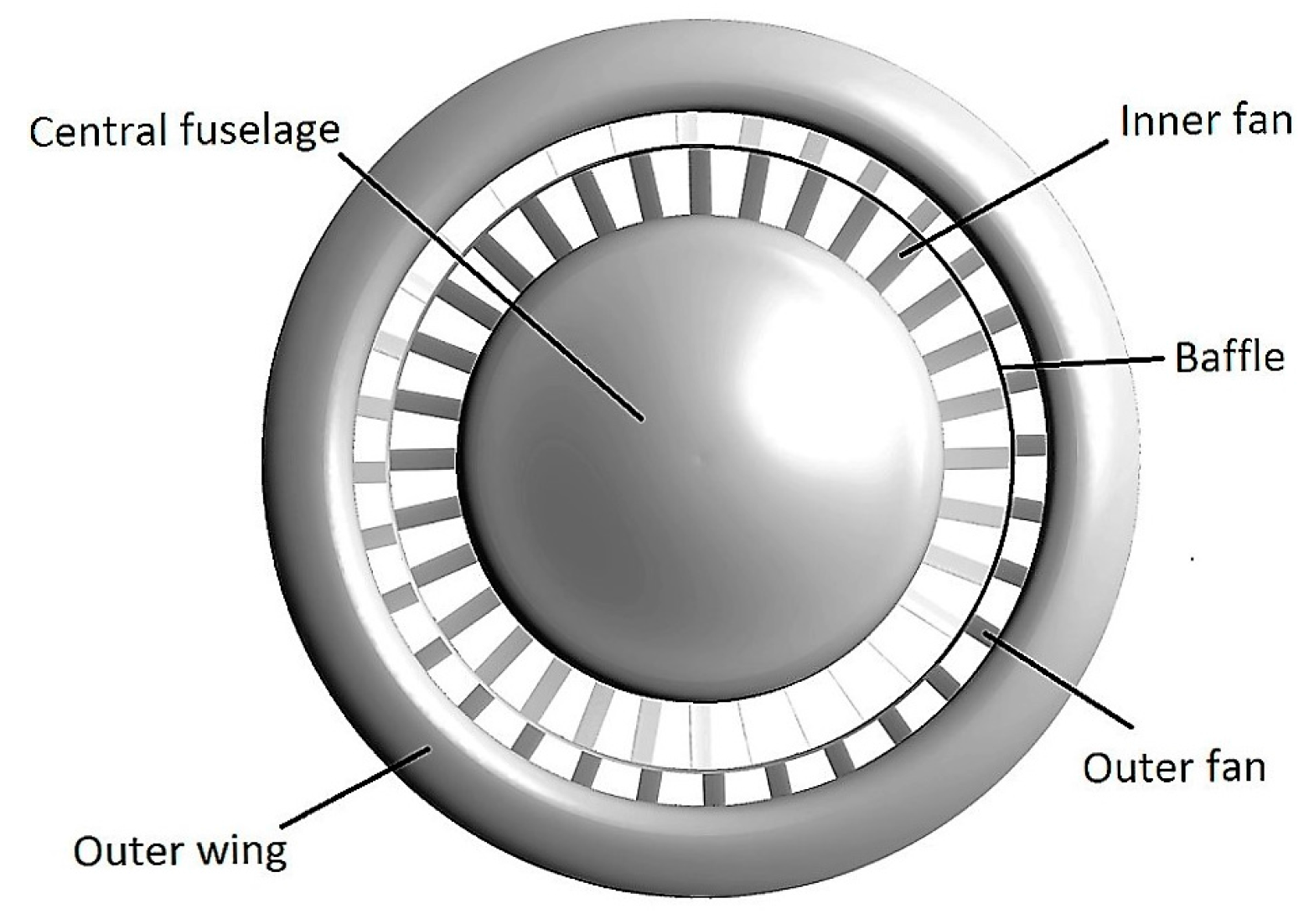
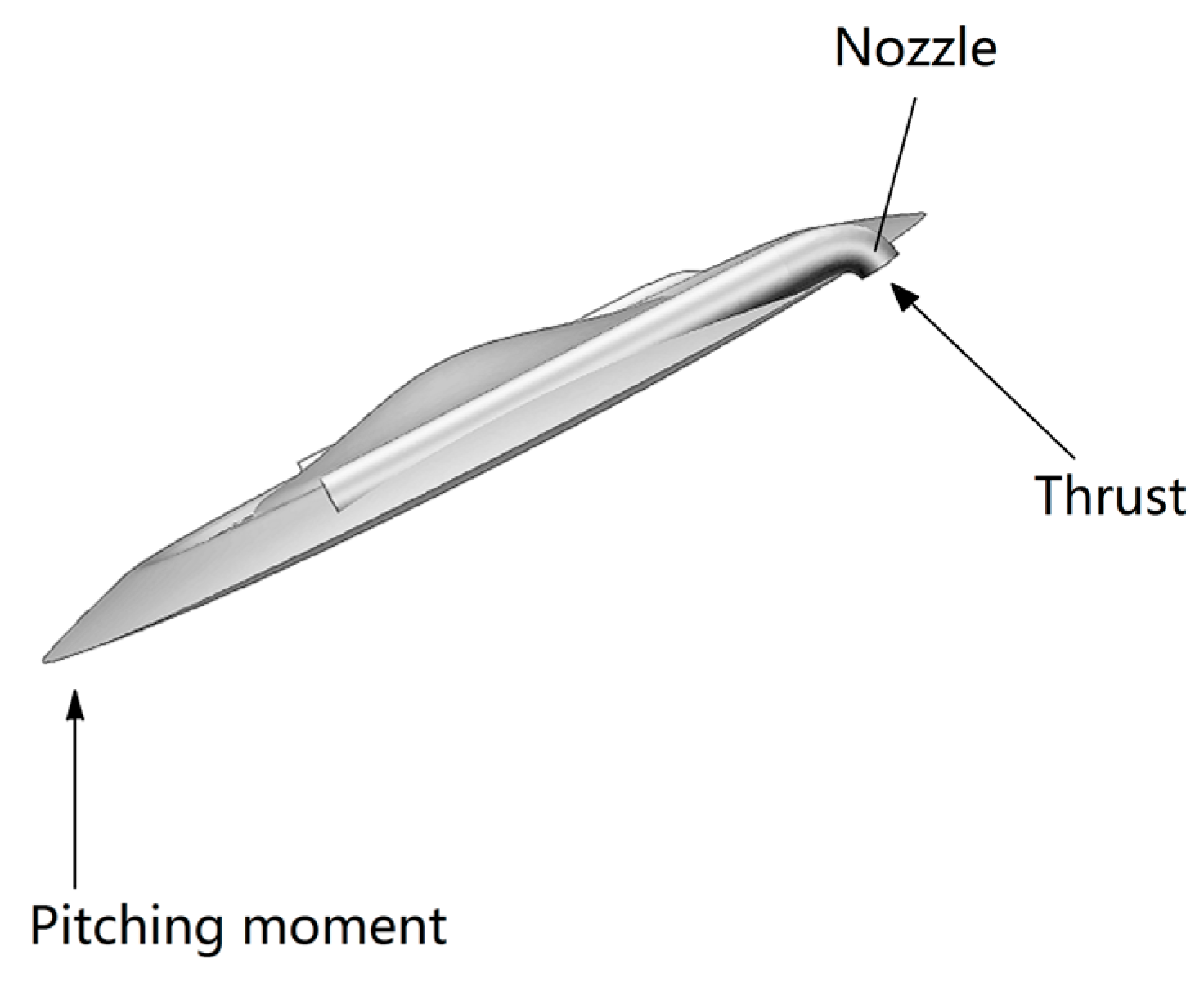
2. Geometry Definition
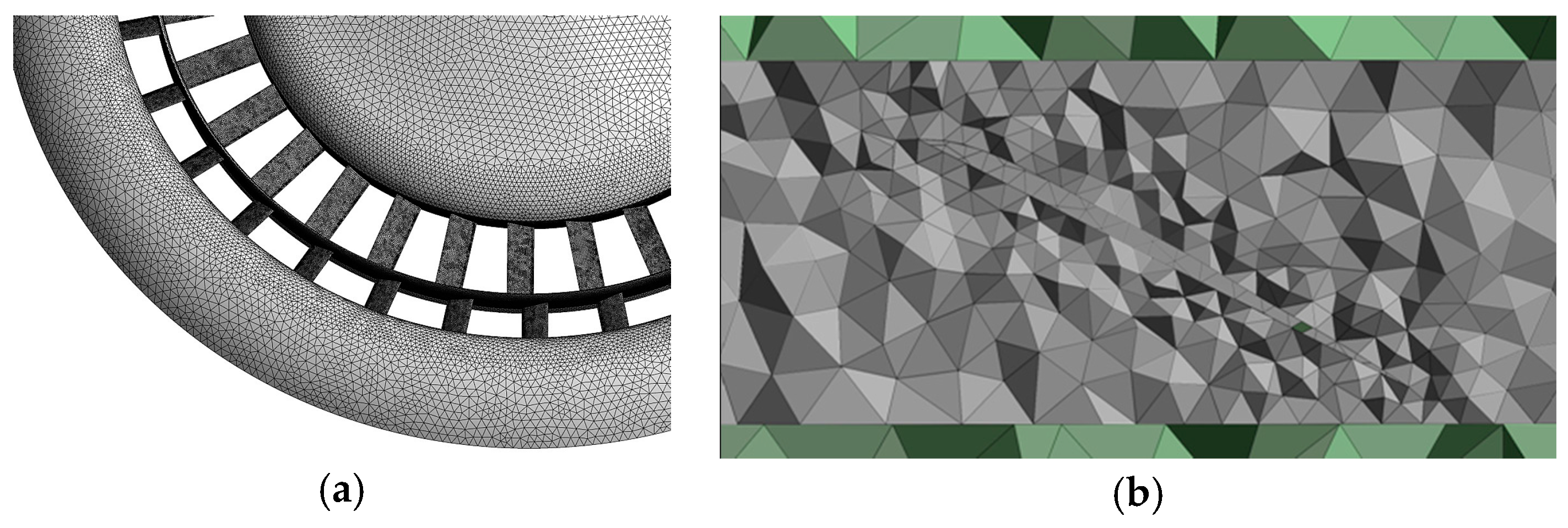
3. Computational Mesh
4. Boundary Condition and Simulation Setup
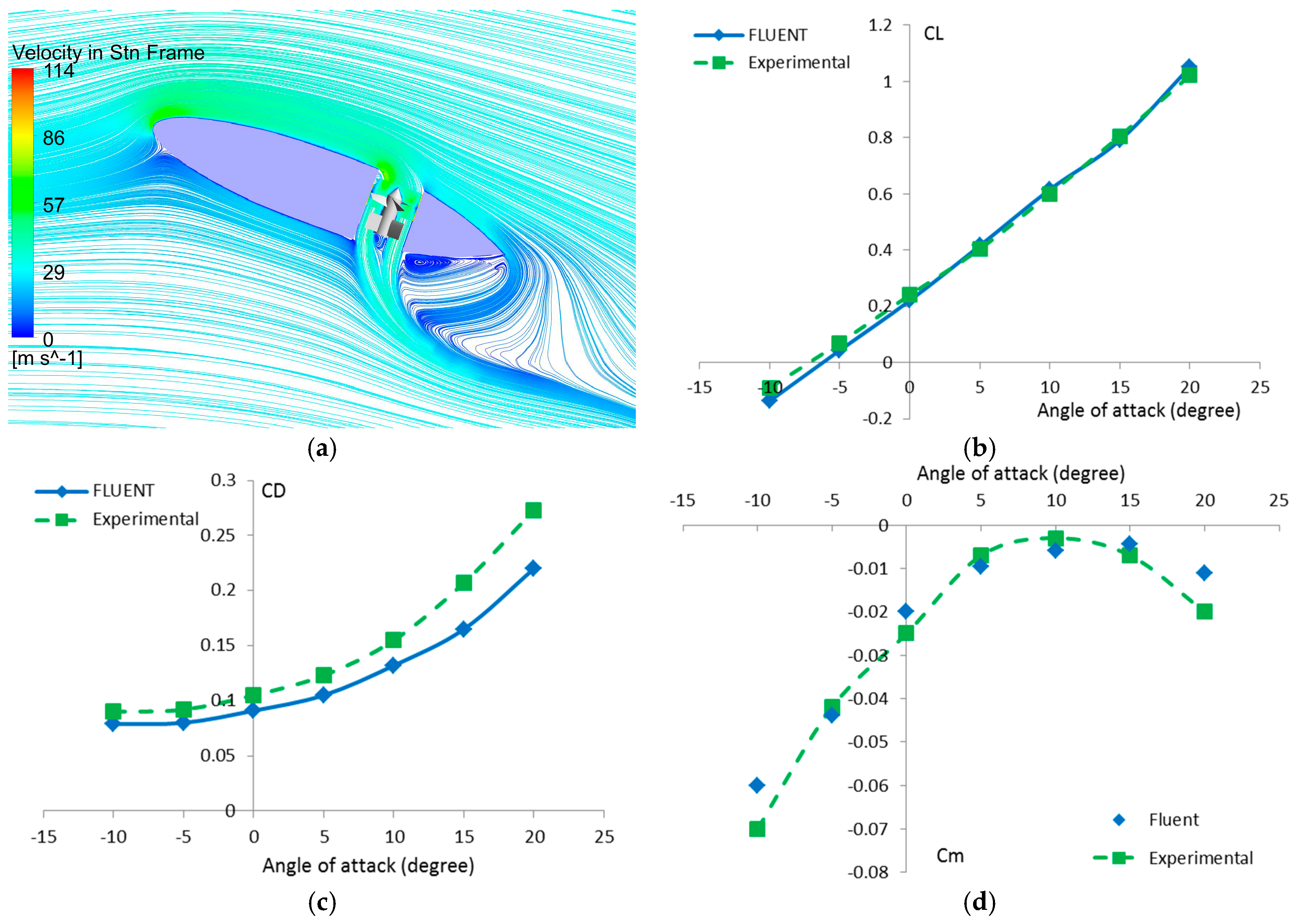
5. Numerical Model Validation
6. Results
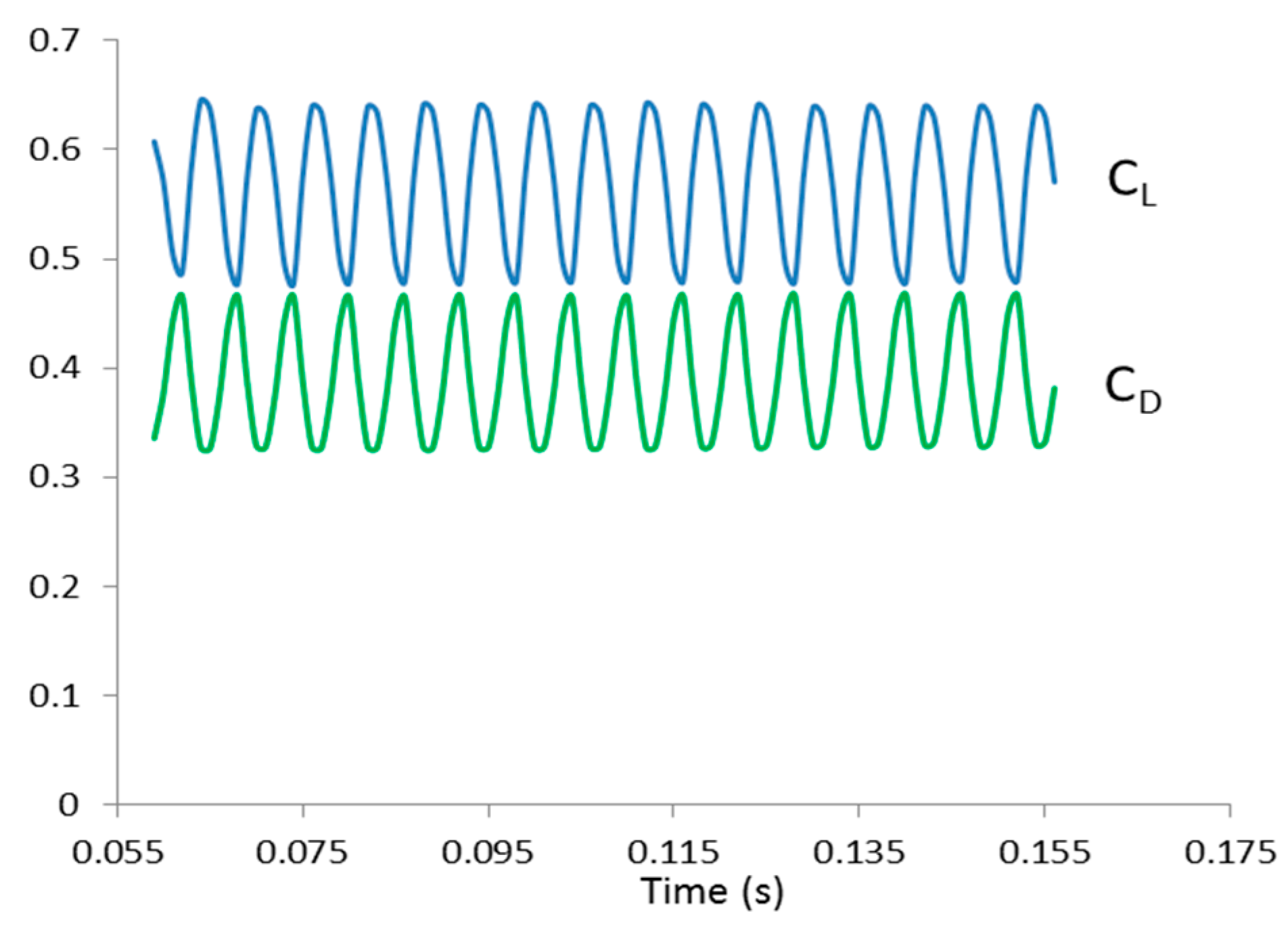
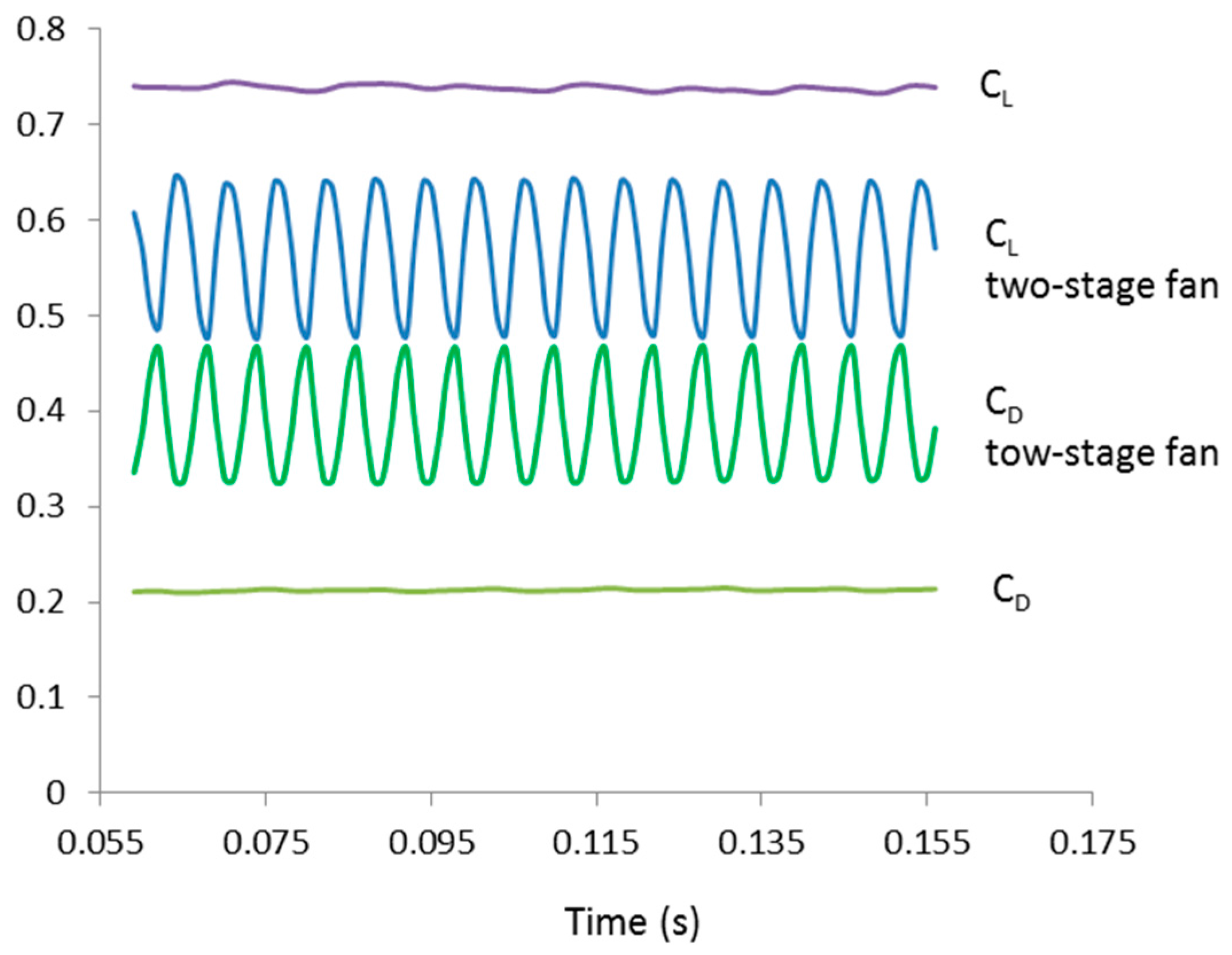
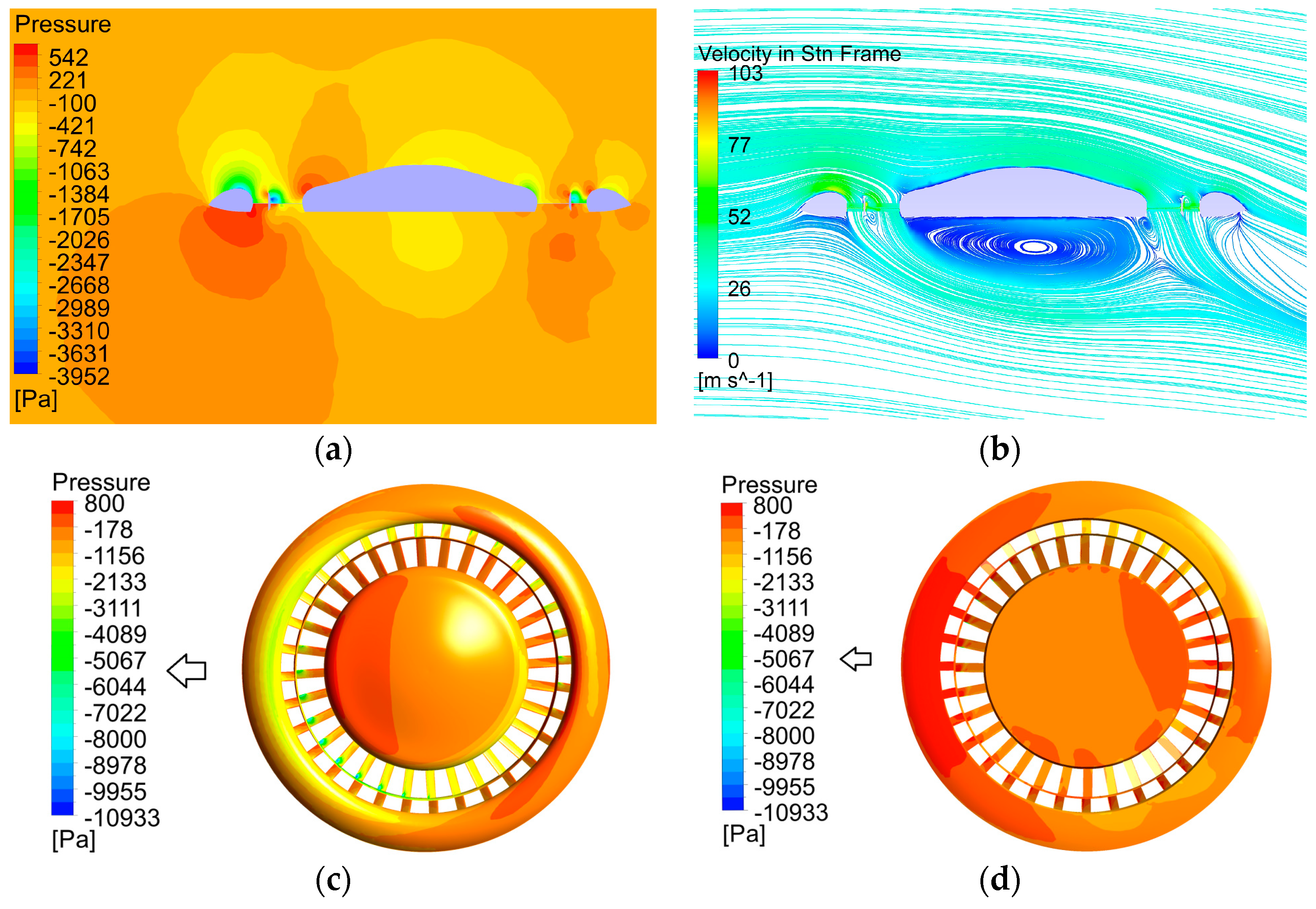
6.1. Oscillations and Elimination
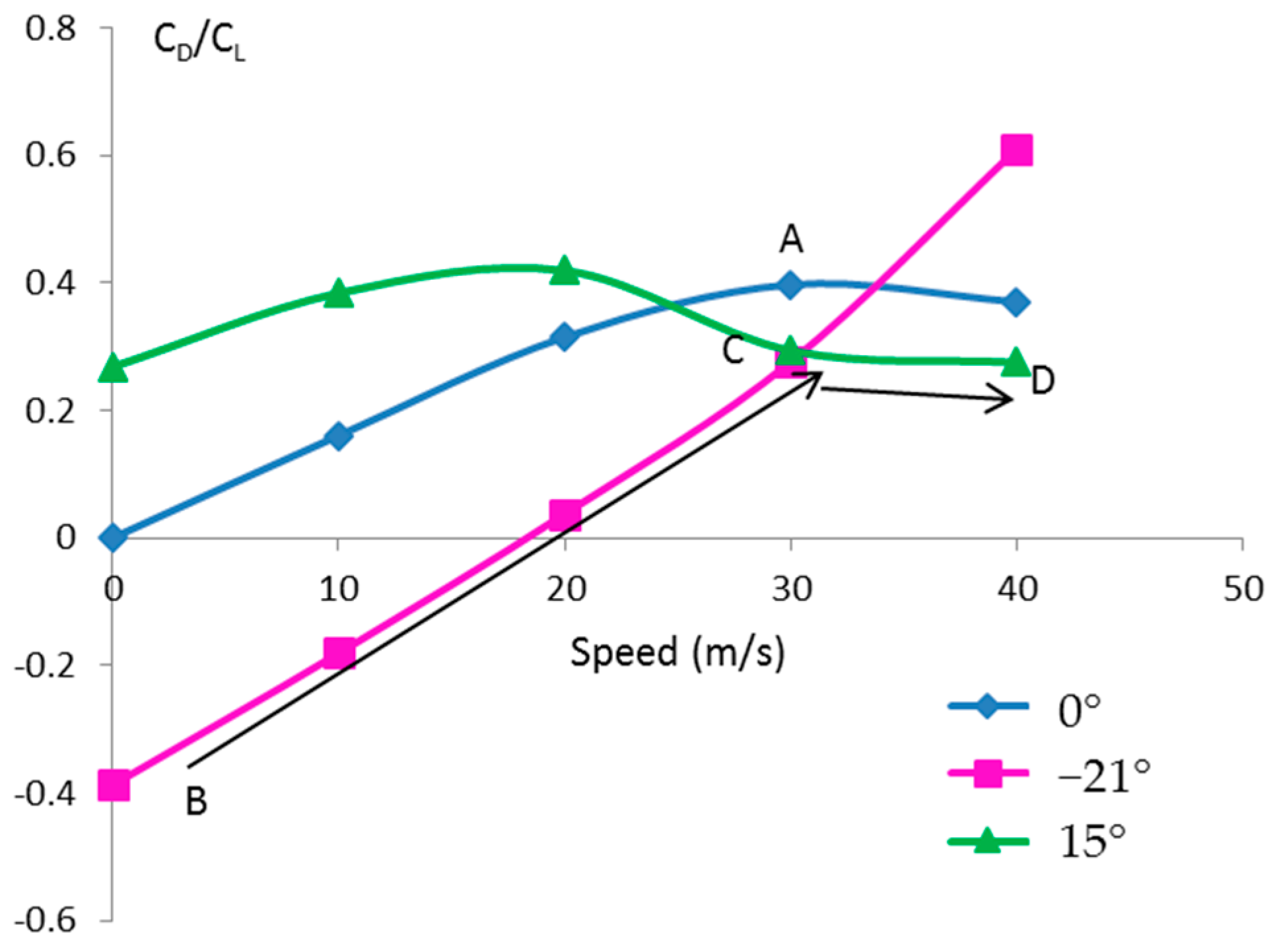
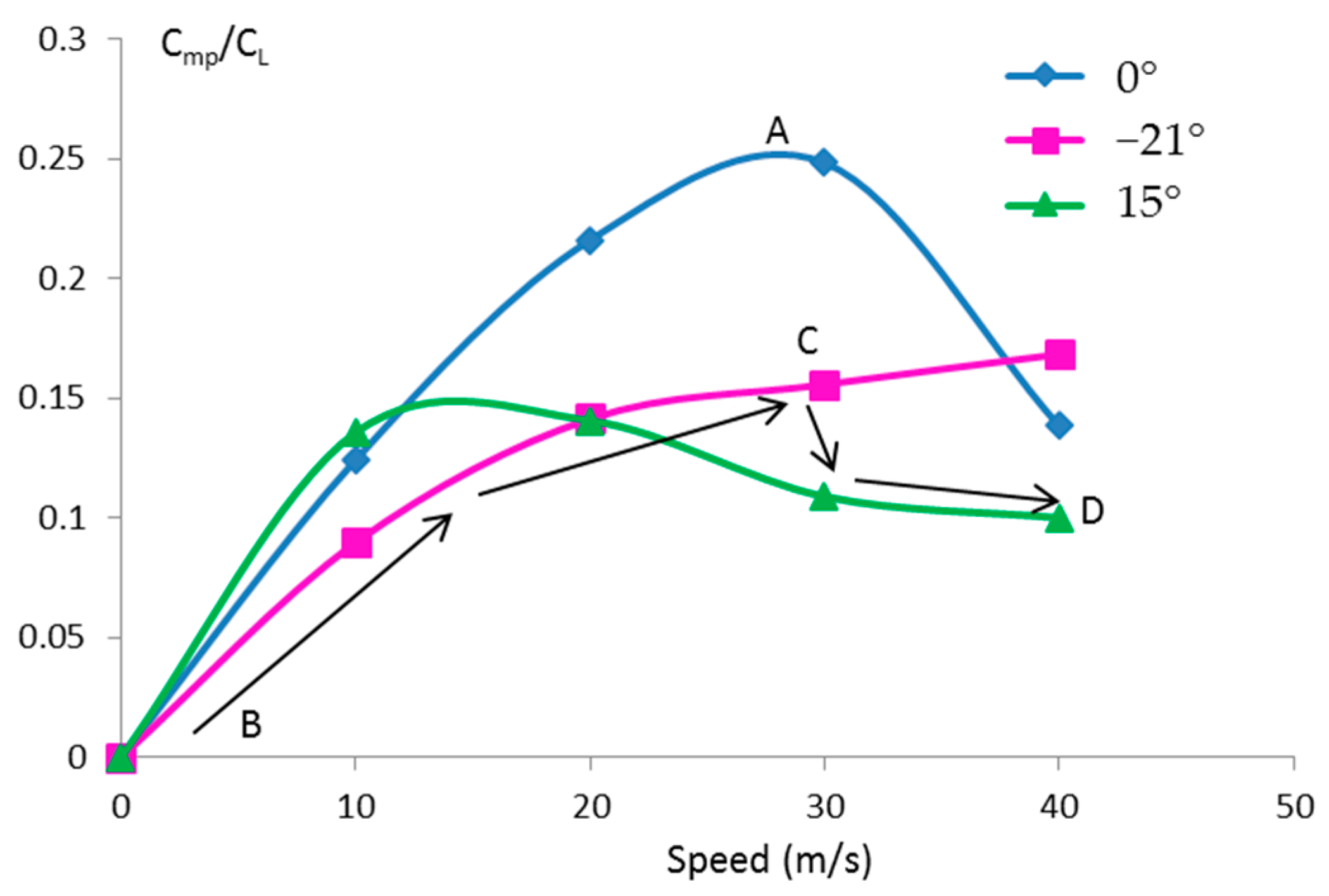
6.2. Transition Characteristics from Takeoff to Forward Flight
6.2.1. Momentum Drag
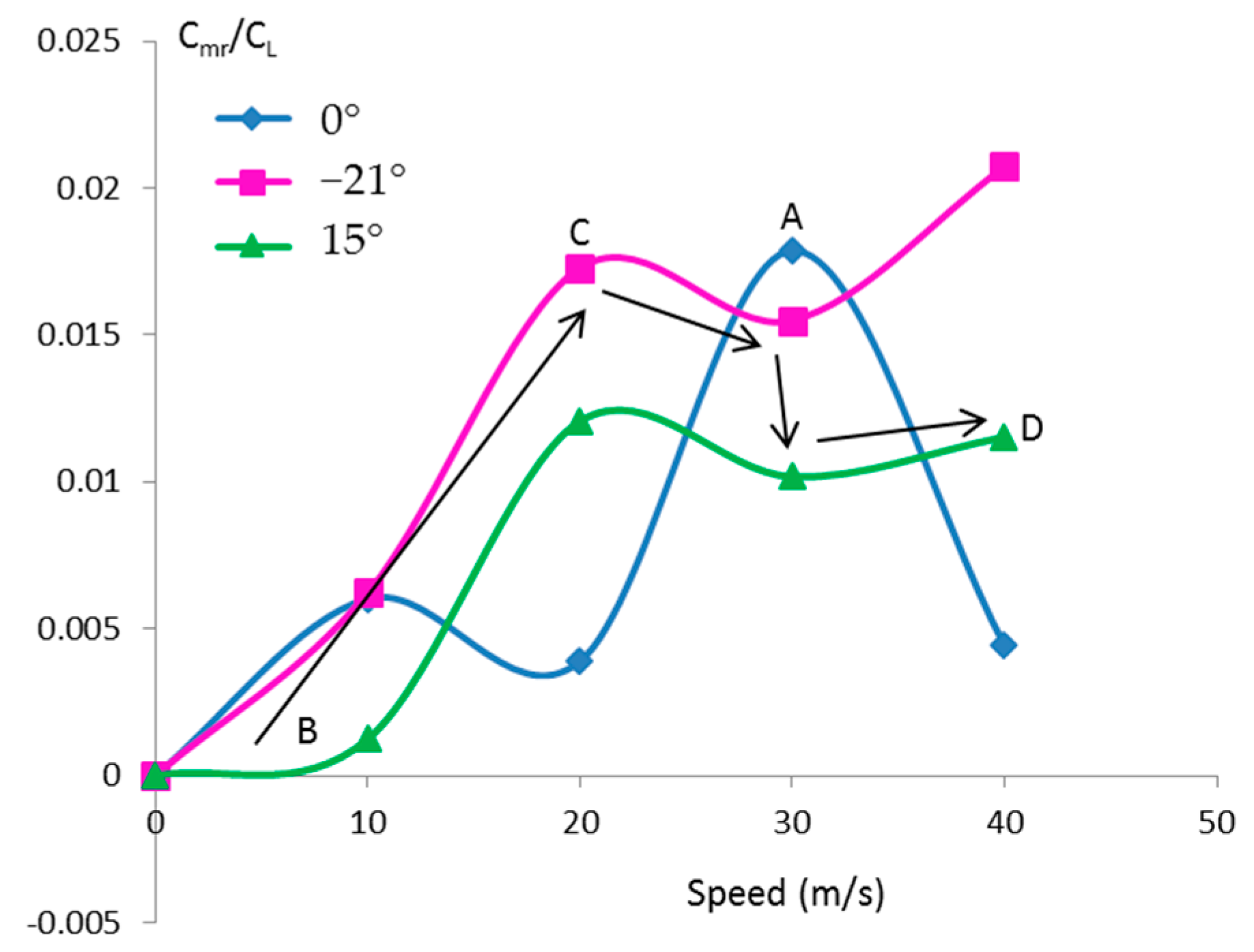
6.2.2. Pitching Moment
6.2.3. Rolling Moment
6.2.4. Transition from Cruise Flight to Landing
7. Conclusions
Author Contributions
Conflicts of Interest
Nomenclature
| T | thrust | N |
| ρ | density of air | kg·m−3 |
| A | area of lift fan | m2 |
| σ | duct diffusion ratio | |
| η | efficiency | |
| P | power | kw |
| F | drag | N |
| v | forward speed | m·s−1 |
| n | rotational speed | rpm |
References
- VTOL X-Plane. Available online: https://en.wikipedia.org/wiki/VTOL_X-Plane (accessed on 20 December 2015).
- Jiang, Y.; Zhang, B.; Huang, T. CFD study of an annular-ducted fan lift system for VTOL aircraft. Aerospace 2015, 2, 555–580. [Google Scholar] [CrossRef]
- Thouault, N.; Breitsamter, C.; Adams, N.A. Numerical and experimental analysis of a generic Fan-in-wing configuration. J. Aircr. 2009, 46, 656–666. [Google Scholar] [CrossRef]
- Asmus, F.J. Design and development of the tip turbine lift fan. Ann. N. Y. Acad. Sci. 1963, 107, 147–176. [Google Scholar] [CrossRef]
- Bevilaqua, P.M. Genesis of the F-35 joint strike fighter. J. Aircr. 2009, 46, 1825–1836. [Google Scholar] [CrossRef]
- Muraoka, K.; Odaka, N.; Kubo, D.; Sato, M. Transition flight of quad tilt wing VTOL UAV. In Proceedings of the 28th International Congress of the Aeronautical Sciences, Brisbane, Australia, 23–28 September 2012.
- Jiang, Y.; Zhang, B. Numerical optimization of hovering efficiency of an annular lift fan aircraft. Aerospace 2016. under review. [Google Scholar]
- Xu, H.Y.; Ye, Z.Y.; Shi, A.M. Numerical study of propeller slipstream based on unstructured overset grids. J. Aircr. 2012, 49, 384–389. [Google Scholar] [CrossRef]
- Blocken, B.; Defraeye, T.; Koninckx, E.; Carmekiet, J.; Hespel, P. CFD simulations of the aerodynamic drag of two drafting cyclists. Comput. Fluids 2013, 71, 435–445. [Google Scholar] [CrossRef]
- Simioni, N.; Ponza, R.; Benini, E. Numerical assessment of pneumatic devices on the wing/fuselage junction of a tiltrotor. J. Aircr. 2013, 50, 752–763. [Google Scholar] [CrossRef]
- Malipeddi, A.K.; Mahmoudnejad, N.; Hoffmann, K.A. Numerical analysis of effects of leading-edge protuberances on aircraft wing performance. J. Aircr. 2012, 49, 1336–1344. [Google Scholar] [CrossRef]
- Qu, Q.; Lu, Z.; Liu, P.; Agarwal, R.K. Numerical study of aerodynamics of a Wing-in-Ground-Effect craft. J. Aircr. 2014, 51, 913–924. [Google Scholar] [CrossRef]
- Al-Garni, A.Z.; Saeed, F.; Al-Garni, A.M. Experimental and numerical investigation of 65 degree Delta and 65/40 degree double-delta wings. J. Aircr. 2008, 45, 71–75. [Google Scholar] [CrossRef]
- Menter, F.R. Zonal two equation k–ω turbulence models for aerodynamic flows. In Proceedings of the 24th AIAA Fluid Dynamics Conference, Orlando, FL, USA, 6–9 July 1993.
- Menter, F.R. Two-equation eddy viscosity turbulence models for engineering applications. AIAA J. 1994, 32, 1598–1605. [Google Scholar] [CrossRef]
| Parameters | Coarse Grid | Medium Grid | Fine Grid |
|---|---|---|---|
| Element number (M) | 2.3 | 4.2 | 8.1 |
| CL | 6.48 | 6.56 | 6.58 |
| CD | 1.042 | 1.058 | 1.063 |
| Cmp | 0.805 | 0.803 | 0.802 |
| AOA\Forward Speed | 0 m/s | 10 m/s | 20 m/s | 30 m/s | 40 m/s |
|---|---|---|---|---|---|
| 0° | 137 rpm | 131 rpm | 115 rpm | 95 rpm | 65 rpm |
| −21° | 148 rpm | 142 pm | 138 rpm | 136 rpm | 135 rpm |
| 15° | 142 rpm | 130 rpm | 115 rpm | 65 rpm | 25 rpm |
© 2016 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jiang, Y.; Zhang, B. Numerical Study of Transition of an Annular Lift Fan Aircraft. Aerospace 2016, 3, 30. https://doi.org/10.3390/aerospace3040030
Jiang Y, Zhang B. Numerical Study of Transition of an Annular Lift Fan Aircraft. Aerospace. 2016; 3(4):30. https://doi.org/10.3390/aerospace3040030
Chicago/Turabian StyleJiang, Yun, and Bo Zhang. 2016. "Numerical Study of Transition of an Annular Lift Fan Aircraft" Aerospace 3, no. 4: 30. https://doi.org/10.3390/aerospace3040030
APA StyleJiang, Y., & Zhang, B. (2016). Numerical Study of Transition of an Annular Lift Fan Aircraft. Aerospace, 3(4), 30. https://doi.org/10.3390/aerospace3040030







