Flow and Flame Mechanisms for Swirl-Stabilized Combustors
Abstract
1. Introduction
1.1. Context, Applications, and Challenges
1.2. Previous Reviews, Motivation and Scope of This Article
| Title | Authors/Reference | Year | Journal/Publisher | Subject/Focus | Scope |
|---|---|---|---|---|---|
| Combustion aerodynamics | Beer and Chigier [4] | 1972 | Elsevier Science | Flowfield characterization Turbulence effect Measurements and theories | Review on theoretical and experimental studies on combustion aerodynamics |
| Vortex breakdown | Hall [8] | 1972 | Annual Review of Fluid Mechanics | Vortex core characterization Reversal flows | Review on vortex breakdown applications |
| Combustion in swirling flows: A review | Syred and Beer [6] | 1974 | Combustion and Flame | Reacting swirling flows Flame stabilization by swirl Three-dimensional flow | Comprehensive review on reacting swirling flows prior to 1974 |
| Swirl Flows in Combustion: A Review | Lilley [7] | 1977 | AIAA Journal | Combustion aerodynamics | Brief review on reacting swirling flows prior to 1977 |
| The structure of vortex breakdown | Leibovich [9] | 1978 | Annual Review of Fluid Mechanics | Vortex breakdown flowfield structure | Review on vortex breakdown |
| Swirl Flows | Gupta et al. [5] | 1984 | Abacus Press | Combustor swirling flows | Review on experimental and modeling work on swirling flows |
| Modeling of swirl in turbulent flow systems | Sloan et al. [11] | 1986 | Progress in Energy and Combustion Science | Computational modeling of swirling flows | Comprehensive review on modeling work on swirling flows |
| Vortex breakdown: Observations and explanations | Escudier [10] | 1988 | Progress in Aerospace Sciences | Understanding/assessment of existing theories for vortex breakdown | Overview of vortex- breakdown research |
| A review of active control of combustion instabilities | McManus et al. [12] | 1993 | Progress in Energy and Combustion Science | Combustion instability active control methods, theoretical basis | Comprehensive review on active control |
| Vortex breakdown: a review | Lucca-Negro and O’Doherty [13] | 2001 | Progress in Energy and Combustion Science | Phenomena of vortex breakdown Reversal flow | Comprehensive review on experimental, numerical, and theoretical works |
| Combustion instabilities in gas turbines, Operational experience, Fundamental mechanisms, and Modeling | Lieuwen and Yang [14] | 2005 | AIAA Progress in Astronautics and Aeronautics | Combustion instabilities | From fundamentals to applications |
| A review of oscillation mechanisms and the role of the precessing vortex core (PVC) in swirl combustion systems | Syred [15] | 2006 | Progress in Energy and Combustion Science | Precessing vortex core in non-reacting and reacting swirling flows Combustion/acoustics interactions | Comprehensive review on PVC |
| Dynamics and stability of lean-premixed swirl-stabilized combustion | Huang and Yang [16] | 2009 | Progress in Energy and Combustion Science | Injectors, fuel atomization, flow structures Active/passive control Combustion instability mechanisms/modeling | Comprehensive review on swirl-stabilized combustion systems with emphasis between 1989 and 2009 |
| Progress and challenges in swirling flame dynamics | Candel et al. [20] | 2012 | Compte Rendus Mécanique | Swirling flame dynamics | Brief summary of recent progress on swirling flames dynamics |
| Unsteady Combustor Physics | Lieuwen [17] | 2012 | Cambridge University Press | Combustion dynamics/instabilities of combustion systems | Dedicated sections on swirling flows and instabilities |
| Large-eddy simulations of gaseous flames in gas turbine combustion chambers | Gicquel et al. [19] | 2012 | Progress in Energy and Combustion Science | Coherent structures Modeling approaches | Review of LES of swirl-stabilized combustors |
| Dynamics of swirling flames | Candel et al. [21] | 2014 | Annual Review of Fluid Mechanics | Swirling flame dynamics | Summary of studies on swirling flame dynamics |
| Transverse combustion instabilities: Acoustic, fluid mechanic, and flame processes | O’Connor et al. [18] | 2015 | Progress in Energy and Combustion Science | Swirling flame dynamics | Transverse instabilities of swirling flames |
| Progress in analytical methods to predict and control azimuthal combustion instability modes in annular chambers | Bauerheim et al. [22] | 2016 | Physics of Fluids | Azimuthal modes Analytical analysis Combustion instability | Recent progress on theoretical tools for azimuthal combustion instabilities |
| Prediction and control of combustion instabilities in real engines | Poinsot [23] | 2017 | Proceedings of the Combustion Institute | Combustor LES Combustion instabilities modeling | Summary of recent progress on instabilities in propulsion systems |
| Modeling of flame response on canonical flames | Polifke [25] | 2020 | Progress in Energy and Combustion Science | Impulse response | Method of modeling flame transfer function in time domain |
| Modeling of ITA on canonical flames | Silva [26] | 2023 | Progress in Energy and Combustion Science | Intrinsic mode | Method of modeling flame transfer function in time domain |
2. Non-Reacting Swirling Flows and Mode Conversion
2.1. Introduction
2.2. Characterization of Swirling Flows
2.2.1. The Swirl Number and the Inner Recirculation Zone
Discussion
2.2.2. Overall Flowfield Observations and Characterization
Discussion
2.3. Theoretical Analyses for Non-Reacting Swirling Flows
Discussion
2.4. Acoustics to Convective Mode Conversion Processes in Swirler
Discussion
2.5. Numerical Simulations Methodologies
Remark
2.6. Swirling Flows Versus Swirling Flames
Discussion
2.7. Conclusions
3. Swirl-Stabilized Partially and Fully Premixed Flames
3.1. Introduction
3.2. Swirling Flames Complexities Due to Chemical Reactions
3.3. Laboratory-Scale Experiments
| Configurations | Mode | Fuel | Swirler | Diagnostic | P [atm] | T [K] | Power [kW] | S | Re | ||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Single injector | |||||||||||
| Komarek | [84] | pre. | NG | ax. swirler | FTF | 1 | 300 | 0.77 | 70 | ||
| Dawson | [165] | ||||||||||
| Richecoeur | [166] | p-pre. | C3H8 | tang. entries | FI + Ac | 1 | 300 | 0.75–0.80 | 33–35 | 0.7 | |
| Weigand | [150] | p-pre. | CH4 | 8/12 blades rad. dual-swirler | Phase-locked | 1 | 300 | 0.55, 0.75 0.65 | 7.6–10.3–34.9 | 0.55 0.9 | 15,000 58,000 |
| Hermeth | [167] | p-pre. | CH4 | ax./diag. 8/24 vanes | 17 | 700 | 100,000 | ||||
| Cochet | [168] | pre./liq. | CH4-Jet A | Preccinsta swirler | Time-averaged + HS | 1–5 | 300–600 | 0.6–1.8 | |||
| Biagioli | [169] | p-pre. | 2 half cones | Time-averaged + PL | 1 | ||||||
| Chterev | [170] | pre. | NG | FI + PIV + Co | 1 | 366–563 | 0.58–0.8 | 0.6 0.80 | 10,000 | ||
| Bellows | [171] | pre. | NG | 40° ax. blades | HS + PL | 1.7 | 300 | 0.83–1 | <100 kW | 1 | 22,500 |
| Schuermans | [172] | p-pre./liq. | 2 half cones | FTF | full | ||||||
| Dhanuka | [173] | p-pre. | Jet-A | twin annular prem. swirler | PLIF + PIV | 4.5 | 505 | 0.16-0.44 | |||
| Williams | [174] | pre. | CH4 | 6 45° vanes ax. swirler | 1 | 300 | 0.56–0.9 | 0.87 | 13,800–48,300 | ||
| Bellows | [158] | pre. | NG | 40° ax. vanes | HS + PL | 1 | 300 | 0.8 | <100 kW | 1 | 21,000–43,000 |
| DiSabatino | [175] | pre. | CH4 and C3H8 | - | FTF + PL | 1–5 | |||||
| Taamallah | [157] | pre. | CH4-H2 | 8 ax. blades | HS + Time-averaged | 1 | 300 | 0.5–0.75 | kW | 0.7 | 20,000 |
| Schimek | [176] | p-pre. | NG | 16 passages movable block | PL | 1 | 300–773 | 0.65 | 0–2 | 37,000 | |
| Terhaar | [177] | pre. | NG | movable block | HS PIV, OH | 1 | 300 | 0.7 | 0.6, 0.8, 1 | 35,000 | |
| Kim | [178] | pre | NG | 8 ax. vanes | PL, FTF | 1–4 | 373–573 | 0.55–0.75 | 0.7 | 10,000 | |
| Seo | [179] | pre. | NG | ax. 45° vane | AC + FI | 4.6 | 570–660 | 0.5–0.7 | 96 | 0.76 | 35,000 |
| Kim | [180] | pre. | NG | FI + PIV | |||||||
| Huang | [181] | liq. | Jet-A | ax. swirler 6 60° vane | 10 | 650–800 | 0.37–0.7 | ||||
| Apeloig | [182] | liq. | keros. | ax. + rad. | PL | 1–50 | 300–900 | 0.4–1.05 | |||
| Tachibana | [183] | p-pre. | ax. swirler | PL + Ac | 700 | 0.5 | |||||
| Tachibana | [184] | ||||||||||
| Providakis | [185] | p-pre. | Jet-A | pilot/main co-swirlers | PDA + PIV + PLIF + HS | 1 | 473 | 0.6 | 85 | 1 | |
| Anacleto | [186] | pre./liq. | C3H8/ | adjust. blade angle swirler | 1 | 573 | 0.5 | 0.5–1.05 | 80,000 | ||
| Rofi | [187] | pre. | CH4 | ax. swirler Ansaldo Energia | 1 | 600 | 0.51 | 2000 | |||
| Cheng | [152] | pre. | CH4-H2 | ax. blades + center holes | Phase-locked | 1 | 300 | 0.58–0.95 | 15–560 | <0.12 | |
| Lückoff | [188] | pre. | NG | ax. swirler | FTF + PL | 1 | 293 | 0.65 | 67 | 0.7 | 30,000 |
| Kim | [189] | pre. | CH4 | 2 ax. swirlers 6 45° vanes | FTF + PL | 1 | 300 | 0.6 | 10 | 0.55 | 8000 |
| Palies | [190] | pre. | CH4 | 8 twisted ax. vanes | PL | 1 | 300 | 0.7 | 2.5 4 | 0.55 | 3900 6000 |
| Palies | [191] | pre. | CH4 | 18 rad. vanes | Phase-locked | 1 | 300 | 0.7 | 2.5 | 0.55 0.65 | 3900 |
| Lartigue | [148] | pre. | CH4 | 12 rad. passages Turbomeca | Phase-locked | 1 | 300 | 0.7 0.83 | 25 30 | 0.6 | 35,000 |
| Stopper | [163] | pre. | NG | 12 passages rad. swirler | 3 6 | 673 | 0.6 | 335–1000 | 0.46/1.02 | 39,000–120,000 | |
| Kraus | [192] | p-pre. | NG | in./out. rad. swirlers | PL | 1 | 300 | 0.6 0.85 | 15–30 | 0.46/1.02 | 34,000–36,000 |
| Arndt | [193] | p-pre. | CH4 | in./out. rad. swirlers | FI + PIV + RS + PL | 1 | 366–563 | 0.7 | 25 | 0.73/1.06 | |
| Han | [194] | p-pre. | RP-3 | main ax. + 2 pilots rad. | Ac | 5–16 | 500–680 | 0.34 | 0.6/0.6, 0.8 | ||
| O’Connor | [160] | pre. | NG | 12 ax. 45° blades | PL | 1 | 300 | 0.85 | 0.85 | 21,500 | |
| Saurabh | [195] | pre. | NG | movable block | PL | 1 | 300 | 0.7 0.8 | 0.7 0.9 | 37,000 | |
| Zellhuber | [196] | pre. | NG | MS+RI | 1 | 673 | |||||
| Idahosa | [197] | n-pre. | 6 tang. entries | FI + PIV + PL | 1 | 300 | 0.09–0.34 | 2662–3043 | |||
| Ranalli | [198] | pre. | CH4-NG | ax. swirler 30° | FTF | 1 | 300 | 0.48–0.7 | |||
| Kim | [199] | pre. | CH4-H2 | ax. swirler 30, 45, 60° | IRZ + Flame shape | 1 | 300 | 0.7 | 5.8 | ||
| Guiberti | [200] | pre. | CH4-H2 | rad. swirler 35° | FDF | 1 | 293 | 0.6–1 | 4 | 0.4 | 13,000 |
| Schuller | [201] | pre. | CH4-H2 | rad. swirler 15° | FDF | 1 | 293 | 0.8 | 3.9 | 0.35 | 12,000 |
| Shoji | [202] | pre. | H2 | ax. swirler 37° | spectra/OH-PLIF | 1 | 293 | 0.25–0.55 | 0.39 | 32,000 | |
| Wicksall | [203] | pre. | H2 | ax. swirler 37° | spectra/OH-PLIF | 1 | 293 | 0.25–0.55 | 0.39 | 32,000 | |
| Multi-injectors | |||||||||||
| Barré | [204] | p-pre. | CH4 | radial swirler | 1 | 300 | 0.66 | 0.76 | |||
| Kwong | [205] | pre. | CH4 | radial swirler | 1 | 500 | 0.5 | ||||
| Santavicca | [206] | pre. | NG | axial swirler | FTF + PL | 20 | 323–523 | 0.6–0.75 | 250 | 0.7 | |
| Kraus | [192] | p-pre. | NG | inner/outer radial swirlers | PL | 1 | 300 | 0.6 0.85 | 60–120 | 0.46/1.02 | 34,000–36,000 |
| Annular chamber | |||||||||||
| Bourgouin | [161] | pre. | CH4 | 16 swirl injectors | Phase-locked | 1 | 300 | 0.76 | 40 | ||
| Worth | [207] | pre. | C2H4 | 12–15–18 swirl injectors | Phase-locked | 1 | 300 | 0.64–1 | 1.22 | 15,000 | |
| Betz | [208] | pre. | CH4 | 12 axial swirlers | 1 | 300 | 0.55 | 515–620 |
Remark
3.4. Turbulent Swirled Combustion
3.5. Swirling Flame Stabilization
Discussion
3.6. Swirling Flame Flashback
3.7. Swirling Flame Lean Blowout and Blowoff (LBO)
3.8. Conclusions
4. Swirling Flames and Combustion Instabilities
4.1. Introduction
4.2. Coupling and Stability Criteria
4.3. Transient Regimes
4.4. Intermittency Regime
4.5. Entropy and Compositional Modes Mechanisms
4.6. Tangential Instabilities Mechanisms
Remark
4.7. Conclusions
5. Dynamics of Modulated Reacting Swirling Flows
5.1. Introduction
5.2. Experimental Flame Responses
5.3. Computed Flame Responses
Remark
5.4. Combustion Dynamics Mechanisms
5.5. Conclusions
6. Perspectives
7. Conclusions
Funding
Conflicts of Interest
Abbreviations
| AIAA | American Institute of Aeronautics and Astronautics |
| APS | American Physical Society |
| ASME | American Society of Mechanical Engineers |
| CFD | Computational Fluid Dynamics |
| CPU | Central Processing Unit |
| DES | Detached Eddy Simulation |
| DNS | Direct Numerical Simulation |
| DMD | Dynamic Mode Decomposition |
| FTF | Flame Transfer Function |
| FDF | Flame Describing Function |
| IRZ | Inner Recirculation Zone |
| LDV | Laser Doppler Velocimetry |
| LES | Large-Eddy Simulation |
| LFP | Lean Fully Premixed |
| LIF | Laser Induced Fluorescence |
| LPP | Lean Premixed Pre-vaporized |
| NASA | National Aeronautics and Space Administration |
| PIV | Particle Imaging Velocimetry |
| PLIF | Planar Laser Induced Fluorescence |
| POD | Proper Orthogonal Decomposition |
| PTV | Particle Tracking Velocimetry |
| PVC | Precessing Vortex Core |
| RANS | Reynolds Averaged Navier–Stokes |
| RMS | Root Mean Square |
| VBB | Vortex Breakdown Bubble |
References
- UN. United Nations Paris Agreement. In Proceedings of the Report of the Conference of the Parties to the United Nations Framework Convention on Climate Change (21st Session, 2015: Paris), Paris, France, 12 December 2015; Volume 4. [Google Scholar]
- NASEM. Commercial Aircraft Propulsion and Energy Systems Research: Reducing Global Carbon Emissions; National Academies of Sciences, Engineering, and Medicine; National Academies Press: Washington, DC, USA, 2016. [Google Scholar]
- International Civil Aviation Organization (ICAO). Consolidated Statement of Continuing ICAO Policies and Practices Related to Environmental Protection-Carbon Offsetting and Reduction Scheme for International Aviation, 2022. Resolution A41-22 CORSIA.
- Beer, J.; Chigier, N. Combustion Aerodynamics; Elsevier Science: Amsterdam, The Netherlands, 1972. [Google Scholar]
- Gupta, A.; Lilley, D.; Syred, N. Swirl Flows; Abaqus Press: Hachette, UK, 1984. [Google Scholar]
- Syred, N.; Beer, J. Combustion in swirling flows: A review. Combust. Flame 1974, 23, 143–201. [Google Scholar] [CrossRef]
- Lilley, D. Swirl flows in combustion: A review. AIAA J. 1977, 15, 1065–1078. [Google Scholar] [CrossRef]
- Hall, M.G. Vortex Breakdown. Annu. Rev. Fluid Mech. 1972, 4, 195–218. [Google Scholar] [CrossRef]
- Leibovich, S. The Structure of Vortex Breakdown. Annu. Rev. Fluid Mech. 1978, 10, 221–246. [Google Scholar] [CrossRef]
- Escudier, M. Vortex breakdown: Observations and explanations. Prog. Aerosp. Sci. 1988, 25, 189–229. [Google Scholar] [CrossRef]
- Sloan, D.; Smith, P.; Smoot, L. Modeling of swirl in turbulent flow systems. Prog. Energy Combust. Sci. 1986, 12, 163–250. [Google Scholar] [CrossRef]
- McManus, K.R.; Poinsot, T.; Candel, S. A Review of Active Control of Combustion Instabilities. Prog. Energy Combust. Sci. 1993, 19, 1–29. [Google Scholar] [CrossRef]
- Lucca-Negro, O.; O’Doherty, T. Vortex breakdown: A review. Prog. Energy Combust. Sci. 2001, 27, 431–481. [Google Scholar] [CrossRef]
- Lieuwen, T.C.; Yang, V. (Eds.) Combustion instabilities in gas turbines, Operational experience, Fundamental mechanisms, and Modeling. In Progress in Astronautics and Aeronautics; American Institute of Aeronautics and Astronautics, Inc.: Reston, VA, USA, 2005; Volume 210. [Google Scholar]
- Syred, N. A review of oscillation mechanisms and the role of the precessing vortex core (PVC) in swirl combustion systems. Prog. Energy Combust. Sci. 2006, 32, 93–161. [Google Scholar] [CrossRef]
- Huang, Y.; Yang, V. Dynamics and stability of lean-premixed swirl-stabilized combustion. Prog. Energy Combust. Sci. 2009, 35, 293–384. [Google Scholar] [CrossRef]
- Lieuwen, T.C. Unsteady Combustor Physics; Cambridge University Press: Cambridge, UK, 2012. [Google Scholar]
- O’Connor, J.; Acharya, V.; Lieuwen, T. Transverse combustion instabilities: Acoustic, fluid mechanic, and flame processes. Prog. Energy Combust. Sci. 2015, 49, 1–39. [Google Scholar] [CrossRef]
- Gicquel, L.Y.; Staffelbach, G.; Poinsot, T. Large eddy simulations of gaseous flames in gas turbine combustion chambers. Prog. Energy Combust. Sci. 2012, 38, 782–817. [Google Scholar] [CrossRef]
- Candel, S.; Durox, D.; Schuller, T.; Palies, P.; Bourgouin, J.F.; Moeck, J.P. Progress and challenges in swirling flame dynamics. Comptes Rendus Mec. 2012, 340, 758–768. [Google Scholar] [CrossRef]
- Candel, S.; Durox, D.; Schuller, T.; Bourgouin, J.; Moeck, J. Dynamics of swirling flames. Annu. Rev. Fluid Mech. 2014, 46, 147–173. [Google Scholar] [CrossRef]
- Bauerheim, M.; Nicoud, F.; Poinsot, T. Progress in analytical methods to predict and control azimuthal combustion instability modes in annular chambers. Phys. Fluids 2016, 28, 021303. [Google Scholar] [CrossRef]
- Poinsot, T. Prediction and control of combustion instabilities in real engines. Proc. Combust. Inst. 2017, 36, 1–28. [Google Scholar] [CrossRef]
- Schuller, T.; Poinsot, T.; Candel, S. Dynamics and control of premixed combustion systems based on flame transfer and describing functions. J. Fluid Mech. 2020, 894, 1. [Google Scholar] [CrossRef]
- Polifke, W. Modeling and analysis of premixed flame dynamics by means of distributed time delays. Prog. Energy Combust. Sci. 2020, 79, 100845. [Google Scholar] [CrossRef]
- Silva, C.F. Intrinsic thermoacoustic instabilities. Prog. Energy Combust. Sci. 2023, 95, 101065. [Google Scholar] [CrossRef]
- Palies, P. Stabilization and Dynamic of Premixed Swirling Flames: Prevaporized, Stratified, Partially, and Fully Premixed Regimes; Academic Press: Cambridge, MA, USA, 2020. [Google Scholar]
- Palies, P.; Ilak, M.; Cheng, R. Transient and limit cycle combustion dynamics analysis of turbulent premixed swirling flame. J. Fluid Mech. 2017, 830, 681–707. [Google Scholar] [CrossRef]
- Vignat, G.; Durox, D.; Candel, S. The suitability of different swirl number definitions for describing swirl flows: Accurate, common and (over-) simplified formulations. Prog. Energy Combust. Sci. 2022, 89, 100969. [Google Scholar] [CrossRef]
- Billant, P.; Chomaz, J.; Huerre, P. Experimental study of vortex breakdown in swirling jets. J. Fluid Mech. 1998, 376, 183–219. [Google Scholar] [CrossRef]
- Sarpkaya, T. An Experimental Investigation of the Vortex-Breakdown Phenomenon; Technical Report NPS-59SL007IA; United States Naval Postgraduate School: Monterey, CA, USA, 1970. [Google Scholar]
- Chigier, N.; Beer, J. Velocity and static-pressure distributions in swirling air jets issuing from annular and divergent nozzles. J. Basic Eng. 1964, 86, 788–796. [Google Scholar] [CrossRef]
- Oberleithner, K.; Sieber, M.; Nayeri, C.; Paschereit, C.; Petz, C.; Hege, H.C.; Noack, B.; Wygnanski, I. Three-dimensional coherent structures in a swirling jet undergoing vortex breakdown: Stability analysis and empirical mode construction. J. Fluid Mech. 2011, 679, 383–414. [Google Scholar] [CrossRef]
- Benjamin, T.B. Theory of the vortex breakdown phenomenon. J. Fluid Mech. 1962, 14, 593–629. [Google Scholar] [CrossRef]
- Harvey, J.K. Some observations of the vortex breakdown phenomenon. J. Fluid Mech. 1962, 14, 585–592. [Google Scholar] [CrossRef]
- Delery, J.M. Aspects of vortex breakdown. Prog. Aerosp. Sci. 1994, 30, 1–59. [Google Scholar] [CrossRef]
- Shtern, V.; Hussain, F. Collapse, symmetry breaking, and hysteresis in swirling flows. Annu. Rev. Fluid Mech. 1999, 31, 537–566. [Google Scholar] [CrossRef]
- Sarpkaya, T. On stationary and travelling vortex breakdowns. J. Fluid Mech. 1971, 45, 545–559. [Google Scholar] [CrossRef]
- Faler, J.H.; Leibovich, S. Disrupted states of vortex flow and vortex breakdown. Phys. Fluids 1977, 20, 1385–1400. [Google Scholar] [CrossRef]
- Gelfgat, A.Y.; Bar-Yoseph, P.; Solan, A. Stability of confined swirling flow with and without vortex breakdown. J. Fluid Mech. 1996, 311, 1–36. [Google Scholar] [CrossRef]
- Faler, J.H.; Leibovich, S. An experimental map of the internal structure of a vortex breakdown. J. Fluid Mech. 1978, 86, 313–335. [Google Scholar] [CrossRef]
- Brücker, C.; Althaus, W. Study of vortex breakdown by particle tracking velocimetry (PTV). Part 1: Bubble-type vortex breakdown. Exp. Fluids 1992, 13, 339–349. [Google Scholar] [CrossRef]
- Brücker, C.; Althaus, W. Study of vortex breakdown by particle tracking velocimetry (PTV). Part 2: Spiral-type vortex breakdown. Exp. Fluids 1993, 14, 133–139. [Google Scholar] [CrossRef]
- Brücker, C.; Althaus, W. Study of vortex breakdown by particle tracking velocimetry (PTV). Part 3: Time-dependent structure and development of breakdown-modes. Exp. Fluids 1995, 18, 174–186. [Google Scholar] [CrossRef]
- Panda, J.; McLaughlin, D. Experiments on the instabilities of a swirling jet. Phys. Fluids 1994, 6, 263–276. [Google Scholar] [CrossRef]
- Vanierschot, M.; Van den Bulck, E. Influence of swirl on the initial merging zone of a turbulent annular jet. Phys. Fluids 2008, 20, 105104. [Google Scholar] [CrossRef]
- Vanierschot, M.; Van den Bulck, E. Experimental study of low precessing frequencies in the wake of a turbulent annular jet. Exp. Fluids 2011, 50, 189–200. [Google Scholar] [CrossRef]
- Vanierschot, M.; Van Dyck, K.; Sas, P.; Van den Bulck, E. Symmetry breaking and vortex precession in low-swirling annular jets. Phys. Fluids 2014, 26, 105110. [Google Scholar] [CrossRef]
- Santhosh, R.; Miglani, A.; Basu, S. Transition in vortex breakdown modes in a coaxial isothermal unconfined swirling jet. Phys. Fluids 2014, 26, 043601. [Google Scholar] [CrossRef]
- Percin, M.; Vanierschot, M.; Oudheusden, B.W.v. Analysis of the pressure fields in a swirling annular jet flow. Exp. Fluids 2017, 58, 166. [Google Scholar] [CrossRef]
- Xi, Z.; Liu, Z.; Shi, X.; Lian, T.; Li, Y. Numerical investigation on flow characteristics and emissions under varying swirler vane angle in a lean premixed combustor. Case Stud. Therm. Eng. 2022, 31, 101800. [Google Scholar] [CrossRef]
- Ramos, J.; Somer, H. Swirling flow in a research combustor. AIAA J. 1985, 23, 241–248. [Google Scholar] [CrossRef]
- Dellenback, P.; Metzger, D.; Neitzel, G. Measurements in turbulent swirling flow through an abrupt axisymmetric expansion. AIAA J. 1988, 26, 669–681. [Google Scholar] [CrossRef]
- Wang, P.; Bai, X.; Wessman, M.; Klingmann, J. Large eddy simulation and experimental studies of a confined turbulent swirling flow. Phys. Fluids 2004, 16, 3306–3324. [Google Scholar] [CrossRef]
- Escudier, M.; Keller, J. Recirculation in swirling flow—A manifestation of vortex breakdown. AIAA J. 1985, 23, 111–116. [Google Scholar] [CrossRef]
- Lilley, D. Swirling flows in typical combustor geometries. J. Propuls. Power 1986, 2, 64–72. [Google Scholar] [CrossRef]
- Graftieaux, L.; Michard, M.; Grosjean, N. Combining PIV, POD and vortex identification algorithms for the study of unsteady turbulent swirling flows. Meas. Sci. Technol. 2001, 12, 1422. [Google Scholar] [CrossRef]
- Mak, H.; Balabani, S. Near field characteristics of swirling flow past a sudden expansion. Chem. Eng. Sci. 2007, 62, 6726–6746. [Google Scholar] [CrossRef]
- Cala, C.E.; Fernandes, E.C.; Heitor, M.V.; Shtork, S.I. Coherent structures in unsteady swirling jet flow. Exp. Fluids 2006, 40, 267–276. [Google Scholar] [CrossRef]
- Liang, H.; Maxworthy, T. An experiment investigation of swirling jets. J. Fluid Mech. 2005, 525, 115–159. [Google Scholar] [CrossRef]
- Loiseleux, T.; Chomaz, J.M. Breaking of rotational symmetry in a swirling jet experiment. Phys. Fluids 2003, 15, 511–523. [Google Scholar] [CrossRef]
- Squire, H. Analysis of the Vortex Breakdown Phenomenon; Imperial College of Science and Technology, Aeronautics Department: London, UK, 1960. [Google Scholar]
- Benjamin, T.B. Some developments in the theory of vortex breakdown. J. Fluid Mech. 1967, 28, 65–84. [Google Scholar] [CrossRef]
- Wang, S.; Rusak, Z. The dynamics of a swirling flow in a pipe and transition to axisymmetric vortex breakdown. J. Fluid Mech. 1997, 340, 177–223. [Google Scholar] [CrossRef]
- Oberleithner, K.; Paschereit, C.; Seele, R.; Wygnanski, I. Formation of turbulent vortex breakdown: Intermittency, criticality, and global instability. AIAA J. 2012, 50, 1437–1452. [Google Scholar] [CrossRef]
- Lessen, M.; Singh, P.; Paillet, F. The stability of a trailing line vortex. Part 1. Inviscid theory. J. Fluid Mech. 1974, 63, 753–763. [Google Scholar] [CrossRef]
- Lessen, M.; Paillet, F. The stability of a trailing line vortex. Part 2. Viscous theory. J. Fluid Mech. 1974, 65, 769–779. [Google Scholar] [CrossRef]
- Martin, J.; Meiburg, E. On the stability of the swirling jet shear layer. Phys. Fluids 1994, 6, 424–426. [Google Scholar] [CrossRef]
- Loiseleux, T.; Chomaz, J.; Huerre, P. The effect of swirl on jets and wakes: Linear instability of the Rankine vortex with axial flow. Phys. Fluids 1998, 10, 1120–1134. [Google Scholar] [CrossRef]
- Loiseleux, T.; Delbende, I.; Huerre, P. Absolute and convective instabilities of a swirling jet/wake shear layer. Phys. Fluids 2000, 12, 375–380. [Google Scholar] [CrossRef]
- Gallaire, F.; Chomaz, J.M. Instability mechanisms in swirling flows. Phys. Fluids 2003, 15, 2622–2639. [Google Scholar] [CrossRef]
- Oberleithner, K.; Paschereit, C.; Wygnanski, I. On the impact of swirl on the growth of coherent structures. J. Fluid Mech. 2014, 741, 156–199. [Google Scholar] [CrossRef]
- Tammisola, O.; Juniper, M.P. Coherent structures in a swirl injector at Re = 4800 by nonlinear simulations and linear global modes. J. Fluid Mech. 2016, 792, 620–657. [Google Scholar] [CrossRef]
- Vyas, A.B.; Majdalani, J. Exact solution of the bidirectional vortex. AIAA J. 2006, 44, 2208–2216. [Google Scholar] [CrossRef]
- Smith, F.T. On the non-parallel flow stability of the Blasius boundary layer. Proc. R. Soc. Lond. A Math. Phys. Sci. 1979, 366, 91–109. [Google Scholar]
- Moise, P. Bistability of bubble and conical forms of vortex breakdown in laminar swirling jets. J. Fluid Mech. 2020, 889. [Google Scholar] [CrossRef]
- Rusak, Z.; Wang, S.; Xu, L.; Taylor, S. On the global nonlinear stability of a near-critical swirling flow in a long finite-length pipe and the path to vortex breakdown. J. Fluid Mech. 2012, 712, 295–326. [Google Scholar] [CrossRef]
- Rusak, Z.; Choi, J.J.; Bourquard, N.; Wang, S. Vortex breakdown of compressible subsonic swirling flows in a finite-length straight circular pipe. J. Fluid Mech. 2015, 781, 3. [Google Scholar] [CrossRef]
- Palies, P. The Flame Surface Speed Budget for Turbulent Premixed Flame Stabilization Studies. In Proceedings of the AIAA Scitech 2020 Forum, Orlando, FL, USA, 6–10 January 2020. [Google Scholar]
- Palies, P. The flame displacement speed: A key quantity for turbulent combustion and combustion instability. Int. J. Spray Combust. Dyn. 2022, 14, 4–16. [Google Scholar] [CrossRef]
- Palies, P.; Premchand, C. Hydrogen-air lean premixed turbulent highly swirled flames stabilization: Experimental demonstration and mechanistic-kinematic description. Aeronaut. J. 2025, 1–24. [Google Scholar] [CrossRef]
- Chu, B.T.; Kovásznay, L.S. Non-linear interactions in a viscous heat-conducting compressible gas. J. Fluid Mech. 1958, 3, 494–514. [Google Scholar] [CrossRef]
- Noiray, N.; Durox, D.; Schuller, T.; Candel, S. Mode conversion in acoustically modulated confined jets. AIAA J. 2009, 47, 2053–2062. [Google Scholar] [CrossRef]
- Komarek, T.; Polifke, W. Impact of swirl fluctuations on the flame response of a perfectly premixed swirl burner. J. Eng. Gas Turbine Power 2010, 132, 061503. [Google Scholar] [CrossRef]
- Palies, P.; Durox, D.; Schuller, T.; Candel, S. Acoustic-convective mode conversion in an aerofoil cascade. J. Fluid Mech. 2011, 672, 545–569. [Google Scholar] [CrossRef]
- Richards, G.; Yip, M. Oscillation combustion from a premix fuel nozzle. In Proceedings of the Combustion Institute/American Flame Research Committee Meeting, San Antonio, TX, USA, 23–26 April 1995. [Google Scholar]
- Straub, D.L.; Richards, G.A. Effect of fuel nozzle configuration on premix combustion dynamics. In Proceedings of the International Gas Turbine & Aeroengine Congress & Exhibition, Stockholm, Sweden, 2–5 June 1998; American Society of Mechanical Engineers: New York, NY, USA, 1998; Volume 78644, p. V003T06A044. [Google Scholar]
- Straub, D.; Richards, G. Effect of axial swirl vane location on combustion dynamics. In Proceedings of the ASME 1999 International Gas Turbine and Aeroengine Congress and Exhibition, Indianapolis, IN, USA, 7–10 June 1999; Volume 99-GT-109. [Google Scholar]
- Palies, P. Dynamique et Instabilités de Combustion des Flammes Swirlées. Ph.D. Thesis, Ecole Centrale Paris, Gif-sur-Yvette, France, 2010. [Google Scholar]
- Bunce, N.A. Flame Transfer Function Measurements and Mechanisms in a Single-Nozzle Combustor. Ph.D. Thesis, Penn State University, University Park, PA, USA, 2013. [Google Scholar]
- Albayrak, A. Time Scales of Equivalence Ratio and Inertial Waves in Unsteady Combustion Dynamics. Ph.D. Thesis, Technische Universität München, München, Germany, 2019. [Google Scholar]
- Albayrak, A.; Juniper, M.P.; Polifke, W. Propagation speed of inertial waves in cylindrical swirling flows. J. Fluid Mech. 2019, 879, 85–120. [Google Scholar] [CrossRef]
- Markstein, G.H. Nonsteady Flame Propagation; Elsevier: Amsterdam, The Netherlands, 1964; Volume 75. [Google Scholar]
- Darmofal, D.L. The role of vorticity dynamics in vortex breakdown. In Proceedings of the 23rd Fluid Dynamics, Plasmadynamics, and Lasers Conference, Orlando, FL, USA, 6–9 July 1993. AIAA-93-3036. [Google Scholar]
- Martin, J.; Meiburg, E. The growth and nonlinear evolution of helical perturbations in a swirling jet model. Eur. J. Mech.-B/Fluids 1998, 17, 639–651. [Google Scholar] [CrossRef]
- Grinstein, F.F.; Young, T.R.; Li, G.; Gutmark, E.; Hsiao, G.; Mongia, H.C. Flow Dynamics in a Swirling Jet Combustor; Begel House Inc.: Danbury, CT, USA, 2001. [Google Scholar]
- Kilik, E. The Influence of Swirler Design Parameters on the Aerodynamics of Downstream Recirculation Region. Ph.D. Thesis, Cranfield University, Bedford, UK, 1976. [Google Scholar]
- Raj, R.T.K.; Ganesan, V. Study on the effect of various parameters on flow development behind vane swirlers. Int. J. Therm. Sci. 2008, 47, 1204–1225. [Google Scholar]
- He, C.; Gan, L.; Liu, Y. The formation and evolution of turbulent swirling vortex rings generated by axial swirlers. Flow Turbul. Combust. 2020, 104, 795–816. [Google Scholar] [CrossRef]
- Nikjooy, M.; Mongia, H. A second-order modeling study of confined swirling flow. Int. J. Heat Fluid Flow 1991, 12, 12–19. [Google Scholar] [CrossRef]
- Kubo, I.; Gouldin, F. Numerical calculations of turbulent swirling flow. J. Fluids Eng. 1975, 97, 310–315. [Google Scholar] [CrossRef]
- Sharif, M.; Wong, Y. Evaluation of the performance of three turbulence closure models in the prediction of confined swirling flows. Comput. Fluids 1995, 24, 81–100. [Google Scholar] [CrossRef]
- Jakirlic, S.; Hanjalic, K.; Tropea, C. Modeling rotating and swirling turbulent flows: A perpetual challenge. AIAA J. 2002, 40, 1984–1996. [Google Scholar] [CrossRef]
- Favaloro, S.; Nejad, A.; Ahmed, S. Experimental and computational investigation of isothermal swirling flow in an axisymmetric dump combustor. J. Propuls. Power 1991, 7, 348–356. [Google Scholar] [CrossRef]
- Leschziner, M.A.; Hogg, S. Computation of highly swirling confined flow with a Reynolds stress turbulence model. AIAA J. 1989, 27, 57–63. [Google Scholar] [CrossRef]
- Dong, M.; Lilley, D.G. Inlet velocity profile effects on turbulent swirling flow predictions. J. Propuls. Power 1994, 10, 155–160. [Google Scholar] [CrossRef]
- Xia, J.; Smith, B.; Benim, A.; Schmidli, J.; Yadigaroglu, G. Effect of inlet and outlet boundary conditions on swirling flows. Comput. Fluids 1997, 26, 811–823. [Google Scholar] [CrossRef]
- Ahmed, S.A. Velocity measurements and turbulence statistics of a confined isothermal swirling flow. Exp. Therm. Fluid Sci. 1998, 17, 256–264. [Google Scholar] [CrossRef]
- Palies, P.; Acharya, R. Flame-resolved transient simulation with swirler-induced turbulence applied to lean blowoff premixed flame experiment. Combust. Flame 2021, 226, 14–30. [Google Scholar] [CrossRef]
- Lu, X.; Wang, S.; Sung, H.; Hsieh, S.; Yang, V. Large-eddy simulations of turbulent swirling flows injected into a dump chamber. J. Fluid Mech. 2005, 527, 171–195. [Google Scholar] [CrossRef]
- Wang, S.; Hsieh, S.; Yang, V. Unsteady flow evolution in swirl injector with radial entry. I. Stationnary conditions. Phys. Fluids 2005, 17, 045106. [Google Scholar] [CrossRef]
- Wang, S.; Yang, V. Unsteady flow evolution in swirl injector with radial entry. II. External excitations. Phys. Fluids 2005, 17, 045107. [Google Scholar] [CrossRef]
- Wang, S.; Yang, V.; Hsiao, G.; Hsieh, S.Y.; Mongia, H. Large-eddy simulations of gas-turbine swirl injector flow dynamics. J. Fluid Mech. 2007, 583, 99–122. [Google Scholar] [CrossRef]
- Duwig, C.; Fuchs, L.; Lacarelle, A.; Beutke, M.; Paschereit, C.O. Study of the vortex breakdown in a conical swirler using LDV, LES and POD. In Proceedings of the ASME Turbo Expo 2007: Power for Land, Sea, and Air, Montreal, QC, Canada, 14–17 May 2007; American Society of Mechanical Engineers: New York, NY, USA, 2007; pp. 1–10. [Google Scholar]
- Stone, C.; Menon, S. Swirl control of combustion instabilities in a gas turbine combustor. Proc. Combust. Inst. 2002, 29, 155–160. [Google Scholar] [CrossRef]
- Javadi, A.; Nilsson, H. LES and DES of strongly swirling turbulent flow through a suddenly expanding circular pipe. Comput. Fluids 2015, 107, 301–313. [Google Scholar] [CrossRef]
- Wang, Y.; Wang, X.; Yang, V. Evolution and transition mechanisms of internal swirling flows with tangential entry. Phys. Fluids 2018, 30, 013601. [Google Scholar] [CrossRef]
- Ruith, M.; Chen, P.; Meiburg, E.; Maxworthy, T. Three-dimensional vortex breakdown in swirling jets and wakes: Direct numerical simulation. J. Fluid Mech. 2003, 486, 331–378. [Google Scholar] [CrossRef]
- Freitag, M.; Klein, M. Direct Numerical Simulation of a Recirculating, Swirling Flow. Flow Turbul. Combust. 2005, 75, 51–66. [Google Scholar] [CrossRef]
- Shamami, K.K.; Birouk, M. Assessment of the performances of RANS models for simulating swirling flows in a can-combustor. Open Aerosp. Eng. J. 2008, 1, 8–27. [Google Scholar] [CrossRef]
- Gouldin, F.; Depsky, J.; Lee, S. Velocity field characteristics of a swirling flow combustor. AIAA J. 1983, 23, 95–102. [Google Scholar] [CrossRef]
- Gouldin, F.; Halthore, R.; Vu, B. Periodic oscillations observed in swirling flows with and without combustion. Symp. (Int.) Combust. 1985, 20, 269–276. [Google Scholar] [CrossRef]
- Brum, R.D.; Samuelsen, G.S. Two-component laser anemometry measurements of non-reacting and reacting complex flows in a swirl-stabilized model combustor. Exp. Fluids 1987, 5, 95–102. [Google Scholar] [CrossRef]
- Roux, S.; Lartigue, G.; Poinsot, T.; Berat, T. Studies of mean and unsteady flow in a swirled combustor using experiments, acoustic analysis and large eddy simulations. Combust. Flame 2005, 141, 40–54. [Google Scholar] [CrossRef]
- Schneider, C.; Dreizler, A.; Janicka, J. Fluid Dynamical Analysis of Atmospheric Reacting and Isothermal Swirling Flows. Flow Turbul. Combust. 2005, 74, 103–127. [Google Scholar] [CrossRef]
- Beneddine, S.; Sipp, D.; Arnault, A.; Dandois, J.; Lesshafft, L. Conditions for validity of mean flow stability analysis. J. Fluid Mech. 2016, 798, 485–504. [Google Scholar] [CrossRef]
- Karban, U.; Bugeat, B.; Martini, E.; Towne, A.; Cavalieri, A.; Lesshafft, L.; Agarwal, A.; Jordan, P.; Colonius, T. Ambiguity in mean-flow-based linear analysis. J. Fluid Mech. 2020, 900, R5. [Google Scholar] [CrossRef]
- Rusak, Z.; Kapila, A.; Choi, J.J. Effect of combustion on near-critical swirling flow. Combust. Theory Model. 2002, 6, 625–645. [Google Scholar] [CrossRef]
- Oberleithner, K.; Schimek, S.; Paschereit, C.O. Shear flow instabilities in swirl-stabilized combustors and their impact on the amplitude dependent flame response: A linear stability analysis. Combust. Flame 2015, 162, 86–99. [Google Scholar] [CrossRef]
- Umeh, C.O.; Rusak, Z.; Gutmark, E. Vortex breakdown in a swirl-stabilized combustor. J. Propuls. Power 2012, 28, 1037–1051. [Google Scholar] [CrossRef]
- Taamallah, S.; LaBry, Z.; Shanbhogue, S.; Habib, M.; Ghoniem, A. Correspondence Between Stable Flame Macrostructure and Thermo-acoustic Instability in Premixed Swirl-Stabilized Turbulent Combustion. J. Eng. Gas Turbines Power 2015, 137, 071505. [Google Scholar] [CrossRef]
- Meier, W.; Weigand, P.; Duan, X.R.; Giezendanner-Thoben, R. Detailed characterization of the dynamics of thermoacoustic pulsations in a lean premixed swirl flame. Combust. Flame 2007, 150, 2–26. [Google Scholar] [CrossRef]
- Steinberg, A.M.; Arndt, C.M.; Meier, W. Parametric study of vortex structures and their dynamics in swirl-stabilized combustion. Proc. Combust. Inst. 2013, 34, 3117–3125. [Google Scholar] [CrossRef]
- O’Connor, J. Visualization of shear layer dynamics in a transversely forced flow and flame. J. Propuls. Power 2015, 31, 1127–1136. [Google Scholar] [CrossRef]
- Steinberg, A.M.; Boxx, I.; Stohr, M.; Meier, W.; Carter, C.D. Effects of flow structure dynamics on thermoacoustic instabilities in swirl-stabilized combustion. AIAA J. 2012, 50, 952–967. [Google Scholar] [CrossRef]
- Moeck, J.P.; Bourgouin, J.F.; Durox, D.; Schuller, T.; Candel, S. Nonlinear interaction between a precessing vortex core and acoustic oscillations in a turbulent swirling flame. Combust. Flame 2012, 159, 2650–2668. [Google Scholar] [CrossRef]
- Oberleithner, K.; Stöhr, M.; Im, S.H.; Arndt, C.M.; Steinberg, A.M. Formation and flame-induced suppression of the precessing vortex core in a swirl combustor: Experiments and linear stability analysis. Combust. Flame 2015, 162, 3100–3114. [Google Scholar] [CrossRef]
- An, Q.; Kwong, W.Y.; Geraedts, B.D.; Steinberg, A.M. Coupled dynamics of lift-off and precessing vortex core formation in swirl flames. Combust. Flame 2016, 168, 228–239. [Google Scholar] [CrossRef]
- Taamallah, S.; Dagan, Y.; Chakroun, N.; Shanbhogue, S.; Vogiatzaki, K.; Ghoniem, A.F. Helical vortex core dynamics and flame interaction in turbulent premixed swirl combustion: A combined experimental and large eddy simulation investigation. Phys. Fluids 2019, 31, 025108. [Google Scholar] [CrossRef]
- Zhang, R.; Boxx, I.; Meier, W.; Slabaugh, C.D. Coupled interactions of a helical precessing vortex core and the central recirculation bubble in a swirl flame at elevated power density. Combust. Flame 2019, 202, 119–131. [Google Scholar] [CrossRef]
- Chterev, I.; Emerson, B.; Lieuwen, T. Velocity and stretch characteristics at the leading edge of an aerodynamically stabilized flame. Combust. Flame 2018, 193, 92–111. [Google Scholar] [CrossRef]
- Roy, S.; Yi, T.; Jiang, N.; Gunaratne, G.; Chterev, I.; Emerson, B.; Lieuwen, T.; Caswell, A.; Gord, J. Dynamics of robust structures in turbulent swirling reacting flows. J. Fluid Mech. 2017, 816, 554–585. [Google Scholar] [CrossRef]
- Douglas, C.; Lim, J.; Smith, T.; Emerson, B.; Lieuwen, T.; Jiang, N.; Fugger, C.; Yi, T.; Felver, J.; Roy, S.; et al. Measurements of periodic reynolds stress oscillations in a forced turbulent premixed swirling flame. J. Eng. Gas Turbines Power 2019, 141, 011001. [Google Scholar] [CrossRef]
- Kazbekov, A.; Kumashiro, K.; Steinberg, A.M. Enstrophy transport in swirl combustion. J. Fluid Mech. 2019, 876, 715–732. [Google Scholar] [CrossRef]
- Kazbekov, A.; Steinberg, A.M. Physical space analysis of cross-scale turbulent kinetic energy transfer in premixed swirl flames. Combust. Flame 2021, 229, 111403. [Google Scholar] [CrossRef]
- Luckoff, F.; Sieber, M.; Paschereit, C.O.; Oberleithner, K. Impact of the Precessing Vortex Core on NOx Emissions in Premixed Swirl Stabilized Flames: An Experimental Study. J. Eng. Gas Turbines Power 2020, 142, 111010. [Google Scholar] [CrossRef]
- Garcia, A.M.; Le Bras, S.; Prager, J.; Boxx, I.; Polifke, W. Impact of H2-enrichment on the response of a partially premixed CH4–air flame to velocity and equivalence ratio fluctuations. Combust. Flame 2024, 268, 113595. [Google Scholar] [CrossRef]
- Lartigue, G.; Meier, U.; Berat, C. Experimental and numerical investigation of self-excited combustion oscillations in a scaled gas turbine combustor. Appl. Therm. Eng. 2004, 24, 1583–1592. [Google Scholar] [CrossRef]
- Salaün, E.; Malbois, P.; Vandel, A.; Godard, G.; Grisch, F.; Renou, B.; Cabot, G.; Boukhalfa, A. Experimental investigation of a spray swirled flame in gas turbine model combustor. In Proceedings of the 18th International Symposium on the Application of Laser and Imaging Techniques to Fluid Mechanics, Lisbon, Portugal, 4–7 July 2016. [Google Scholar]
- Weigand, P.; Meier, W.; Duan, X.; Stricker, W.; Aigner, M. Investigations of swirl flames in a gas turbine model combustor I. Flow field, structures, temperature, and species distributions. Combust. Flame 2006, 144, 205–224. [Google Scholar] [CrossRef]
- Chterev, I.; Rock, N.; Ek, H.; Emerson, B.; Seitzman, J.; Jiang, N.; Roy, S.; Lee, T.; Gord, J.; Lieuwen, T. Simultaneous imaging of fuel, OH, and three component velocity fields in high pressure, liquid fueled, swirl stabilized flames at 5 kHz. Combust. Flame 2017, 186, 150–165. [Google Scholar] [CrossRef]
- Cheng, R.; Yegian, D.; Miyasato, M.; Samuelsen, G.; Benson, C.; Pellizzari, R.; Loftus, P. Scaling and development of low-swirl burners for low-emission furnaces and boilers. Proc. Combust. Inst. 2000, 28, 1305–1313. [Google Scholar] [CrossRef]
- Cheng, R.; Littlejohn, D.; Nazeer, W.; Smith, K. Laboratory Studies of the Flow Field Characteristics of Low-Swirl Injectors for Application to Fuel-Flexible Turbines. J. Eng. Gas Turbines Power 2008, 130, 21501–21511. [Google Scholar] [CrossRef]
- Chan, C.; Lau, K.; Chin, W.; Cheng, R. Freely Propagating Open Premixed Turbulent Flames Stabilized by Swirl. Proc. Combust. Inst. 2005, 24, 511–518. [Google Scholar] [CrossRef]
- Cheng, R.K. Velocity and scalar characteristics of premixed turbulent flames stabilized by weak swirl. Combust. Flame 2005, 101, 1–14. [Google Scholar] [CrossRef]
- Therkelsen, P.; Enrique Portillo, J.; Littlejohn, D.; Martin, S.; Cheng, R. Self-induced unstable behaviors of CH4 and H2/CH4 flames in a model combustor with a low-swirl injector. Combust. Flame 2013, 160, 307–321. [Google Scholar] [CrossRef]
- Taamallah, S.; LaBry, Z.A.; Shanbhogue, S.J.; Ghoniem, A.F. Thermo-acoustic instabilities in lean premixed swirl-stabilized combustion and their link to acoustically coupled and decoupled flame macrostructures. Proc. Combust. Inst. 2015, 35, 3273–3282. [Google Scholar] [CrossRef]
- Bellows, B.D.; Bobba, M.K.; Forte, A.; Seitzman, J.M.; Lieuwen, T. Flame transfer function saturation mechanisms in a swirl-stabilized combustor. Proc. Combust. Inst. 2007, 31, 3181–3188. [Google Scholar] [CrossRef]
- Thumuluru, S.; Lieuwen, T. Characterization of acoustically forced swirl flame dynamics. Proc. Combust. Inst. 2009, 32, 2893–2900. [Google Scholar] [CrossRef]
- O’Connor, J.; Lieuwen, T. Disturbance Field Characteristics of a Transversely Excited Burner. Combust. Sci. Technol. 2011, 183, 427–443. [Google Scholar] [CrossRef]
- Bourgouin, J.F.; Durox, D.; Moeck, J.; Schuller, T.; Candel, S. Self-Sustained Instabilities in an Annular Combustor Coupled by Azimuthal and Longitudinal Acoustic Modes. In Proceedings of the ASME Turbo Expo GT2013-95010, San Antonio, TX, USA, 3–7 June 2013. [Google Scholar]
- Vignat, G. Injection and Combustion Dynamics in Swirled Spray Flames and Azimuthal Coupling in Annular Combustors. Ph.D. Thesis, Université Paris-Saclay, Gif-sur-Yvette, France, 2020. [Google Scholar]
- Stopper, U.; Meier, W.; Sadanandan, R.; Stöhr, M.; Aigner, M.; Bulat, G. Experimental study of industrial gas turbine flames including quantification of pressure influence on flow field, fuel/air premixing and flame shape. Combust. Flame 2013, 160, 2103–2118. [Google Scholar] [CrossRef]
- Bulat, G.; Fedina, E.; Fureby, C.; Meier, W.; Stopper, U. Reacting flow in an industrial gas turbine combustor: LES and experimental analysis. Proc. Combust. Inst. 2015, 35, 3175–3183. [Google Scholar] [CrossRef]
- Dawson, J.; Rodriguez-Martinez, V.; Syred, N.; O’Doherty, T. The effect of combustion instability on the structure of recirculation zones in confined swirling flames. Combust. Sci. Technol. 2005, 177, 2349–2371. [Google Scholar] [CrossRef]
- Richecoeur, F.; Schuller, T.; Lamraoui, A.; Ducruix, S. Analytical and experimental investigations of gas turbine model combustor acoustics operated at atmospheric pressure. Comptes Rendus Mécanique 2013, 341, 141–151. [Google Scholar] [CrossRef]
- Hermeth, S. Mechanisms Affecting the Dynamic Response of Swirled Flames in Gas Turbines. Ph.D. Thesis, INPT, Rabat, Morocco, 2012. [Google Scholar]
- Cochet, A.; Bodoc, V.; Brossard, C.; Dessornes, O.; Guin, C.; Lecourt, R.; Orain, M.; Vincent-Randonnier, A. ONERA test Facilities for Combustion in Aero Gas Turbine Engines, and Associated Optical Diagnostics. J. Aerosp. Lab 2016, 11, 16. [Google Scholar]
- Biagioli, F.; Gösthe, F.; Schuermans, B. Combustion dynamics linked to flame behaviour in a partially premixed swirled industrial burner. Exp. Therm. Fluid Sci. 2008, 32, 1344–1353. [Google Scholar] [CrossRef]
- Chterev, I.; Foley, C.W.; Foti, D.; Kostka, S.; Caswell, A.W.; Jiang, N.; Lynch, A.; Noble, D.R.; Menon, S.; Seitzman, J.M.; et al. Flame and Flow Topologies in an Annular Swirling Flow. Combust. Sci. Technol. 2014, 186, 1041–1074. [Google Scholar] [CrossRef]
- Bellows, B.D.; Neumeier, Y.; Lieuwen, T. Forced response of a swirling, premixed flame to flow disturbances. J. Propuls. Power 2006, 22, 1075–1084. [Google Scholar] [CrossRef]
- Schuermans, B.; Guethe, F.; Pennell, D.; Guyot, D.; Paschereit, C. Thermoacoustic modeling of a gas turbine using transfer functions measured under full engine pressure. J. Eng. Gas Turbines Power 2010, 132, 111503. [Google Scholar] [CrossRef]
- Dhanuka, S.; Temme, J.; Driscoll, J. Unsteady Aspects of Lean Premixed Prevaporized Gas Turbine Combustors: Flame-Flame Interactions. J. Propuls. Power 2011, 27, 631–641. [Google Scholar] [CrossRef]
- Williams, T.C.; Schefer, R.W.; Oefelein, J.C.; Shaddix, C.R. Idealized gas turbine combustor for performance research and validation of large eddy simulations. Rev. Sci. Instrum. 2007, 78, 035114. [Google Scholar] [CrossRef]
- Di Sabatino, F.; Guiberti, T.; Boyette, W.; Roberts, W.; Moeck, J.; Lacoste, D. Effect of pressure on the transfer functions of premixed methane and propane swirl flames. Combust. Flame 2018, 193, 272–282. [Google Scholar] [CrossRef]
- Schimek, S.; Moeck, J.P.; Paschereit, C.O. An experimental investigation of the nonlinear response of an atmospheric swirl-stabilized premixed flame. J. Eng. Gas Turbines Power 2011, 133, 101502. [Google Scholar] [CrossRef]
- Terhaar, S.; Cosic, B.; Pashereit, C.; Oberleithner, K. Impact of Shear Flow Instabilities on the Magnitude and Saturation of the Flame Response. J. Eng. Gas Turbines Power 2014, 136, 071502. [Google Scholar] [CrossRef]
- Kim, K.; Santavicca, D. Interference mechanisms of acoustic-convective disturbances in a swirl-stabilized lean premixed combustor. Combust. Flame 2013, 160, 1441–1457. [Google Scholar] [CrossRef]
- Seo, S. Parametric Study of Lean-Premixed Combustion Instability in a Pressurized Model Gas Turbine Combustor. Ph.D. Thesis, The Pennsylvania State University, University Park, PA, USA, 1999. [Google Scholar]
- Kim, M.; Yoon, J.; Park, S.; Lee, M.; Yoon, Y. Effects of unstable flame structure and recirculation zones in a swirl-stabilized dump combustor. Appl. Therm. Eng. 2013, 58, 125–135. [Google Scholar] [CrossRef]
- Huang, C.; Gejji, R.; Anderson, W.; Yoon, C.; Sankaran, V. Combustion Dynamics Behavior in a Single-Element Lean Direct Injection (LDI) Gas Turbine Combustor. AIAA Propuls. Energy 2014. [Google Scholar]
- Apeloig, J.; d’Herbigny, F.; Simon, F.; Gajan, P.; Orain, M.; Roux, S. Liquid-Fuel Behavior in an Aeronautical Injector Submitted to Thermoacoustic Instabilities. J. Propuls. Power 2014, 31, 309–319. [Google Scholar] [CrossRef]
- Tachibana, S.; Zimmer, L.; Kurosawa, Y.; Suzuki, K. Active control of combustion oscillations in a lean premixed combustor by secondary fuel injection coupling with chemiluminescence imaging technique. Proc. Combust. Inst. 2007, 31, 3225–3233. [Google Scholar] [CrossRef]
- Tachibana, S.; Saito, K.; Yamamoto, T.; Makida, M.; Kitano, T.; Kurose, R. Experimental and numerical investigation of thermo-acoustic instability in a liquid-fuel aero-engine combustor at elevated pressure: Validity of large-eddy simulation of spray combustion. Combust. Flame 2015, 162, 2621–2637. [Google Scholar] [CrossRef]
- Providakis, T.; Zimmer, L.; Scouflaire, P.; Ducruix, D. Characterization of the Acoustic Interactions in a Two Stage Multi Injection Combustor Fed with Liquid Fuel. J. Eng. Gas Turbines Power 2012, 134, 111503. [Google Scholar] [CrossRef]
- Anacleto, P.; Fernandes, E.; Heitor, M.; Shtork, S. Swirl flow structure and flame characteristics in a model lean premixed combustor. Combust. Sci. Technol. 2003, 175, 1369–1388. [Google Scholar] [CrossRef]
- Laera, D.; Camporeale, S. A Weakly Nonlinear Approach Based on a Distributed Flame Describing Function to Study the Combustion Dynamics of a Full-Scale Lean-Premixed Swirled Burner. J. Eng. Gas Turbines Power 2017, 139, 091501. [Google Scholar] [CrossRef]
- Lückoff, F.; Kaiser, T.L.; Paschereit, C.O.; Oberleithner, K. Mean field coupling mechanisms explaining the impact of the precessing vortex core on the flame transfer function. Combust. Flame 2020, 223, 254–266. [Google Scholar] [CrossRef]
- Kim, K.; Hochgreb, S. Measurements of triggering and transient growth in a model lean-premixed gas turbine combustor. Combust. Flame 2012, 159, 1215–1227. [Google Scholar] [CrossRef]
- Palies, P.; Durox, D.; Schuller, T.; Candel, S. The combined dynamics of swirler and turbulent premixed swirling flames. Combust. Flame 2010, 157, 1698–1717. [Google Scholar] [CrossRef]
- Palies, P.; Durox, D.; Schuller, T.; Candel, S. Experimental study on effects of swirler geometry and swirl number on flame describing functions. Combust. Sci. Technol. 2011, 183, 704–717. [Google Scholar] [CrossRef]
- Kraus, C.; Harth, S.; Bockhorn, H. Experimental investigation of combustion instabilities in lean swirl-stabilized partially-premixed flames in single- and multiple-burner setup. Int. J. Spray Combust. Dyn. 2016, 8, 4–26. [Google Scholar] [CrossRef]
- Arndt, C.M.; Severin, M.; Dem, C.; Stöhr, M.; Steinberg, A.M.; Meier, W. Experimental analysis of thermo-acoustic instabilities in a generic gas turbine combustor by phase-correlated PIV, chemiluminescence, and laser Raman scattering measurements. Exp. Fluids 2015, 56, 69. [Google Scholar] [CrossRef]
- Han, X.; Hui, X.; Zhang, C.; Lin, Y.; He, P.; Sung, C. Combustion Instabilities in a Lean Premixed Pre-Vaporized Combustor at High-Pressure High-Temperature. ASME Proc. 2017, 177, 2349–2371. [Google Scholar]
- Saurabh, A.; Paschereit, C. Dynamics of premixed swirl flames under the influence of transverse acoustic fluctuations. Combust. Flame 2017, 182, 298–312. [Google Scholar] [CrossRef]
- Zellhuber, M.; Schwing, J.; Schuermans, B.; Sattelmayer, T.; Polifke, W. Experimental and Numerical Investigation of Thermoacoustic Sources Related to High-Frequency Instabilities. Int. J. Spray Combust. Dyn. 2014, 6, 1–34. [Google Scholar] [CrossRef]
- Idahosa, U.; Saha, A.; Xu, C.; Basu, S. Non-premixed acoustically perturbed swirling flame dynamics. Combust. Flame 2010, 157, 1800–1814. [Google Scholar] [CrossRef]
- Ranalli, J.A.; Martin, C.R.; Black, P.R.; Vandsburger, U.; West, R. Measurement of Flame Transfer Functions in Swirl-Stabilized, Lean-Premixed Combustion. J. Propuls. Power 2009, 25, 1350–1354. [Google Scholar] [CrossRef]
- Kim, H.S.; Arghode, V.K.; Gupta, A.K. Flame characteristics of hydrogen-enriched methane–air premixed swirling flames. Int. J. Hydrogen Energy 2009, 34, 1063–1073. [Google Scholar] [CrossRef]
- Guiberti, T.F.; Durox, D.; Scouflaire, P.; Schuller, T. Impact of heat loss and hydrogen enrichment on the shape of confined swirling flames. Proc. Combust. Inst. 2015, 35, 1385–1392. [Google Scholar] [CrossRef]
- Schuller, T.; Marragou, S.; Oztarlik, G.; Poinsot, T.; Selle, L. Influence of hydrogen content and injection scheme on the describing function of swirled flames. Combust. Flame 2022, 240, 111974. [Google Scholar] [CrossRef]
- Shoji, T.; Iwasaki, Y.; Kodai, K.; Yoshida, S.; Tachibana, S.; Yokomori, T. Effects of flame behaviors on combustion noise from lean-premixed hydrogen low-swirl flames. AIAA J. 2020, 58, 4505–4521. [Google Scholar] [CrossRef]
- Wicksall, D.M.; Agrawal, A.K.; Schefer, R.W.; Keller, J.O. Influence of hydrogen addition on flow structure in confined swirling methane flame. J. Propuls. Power 2005, 21, 16–24. [Google Scholar] [CrossRef]
- Barré, D.; Esclapez, L.; Cordier, M.; Riber, E.; Cuenot, B.; Staffelbach, G.; Renou, B.; Vandel, A.; Gicquel, L.Y.; Cabot, G. Flame propagation in aeronautical swirled multi-burners: Experimental and numerical investigation. Combust. Flame 2014, 161, 2387–2405. [Google Scholar] [CrossRef]
- Kwong, W.Y.; Steinberg, A.M. Blowoff and reattachment dynamics of a linear multinozzle combustor. J. Eng. Gas Turbines Power 2019, 141, 011015. [Google Scholar] [CrossRef]
- Santavicca, D.; Lieuwen, T. Combustion Dynamics in Multi-Nozzle Combustors Operating on High-Hydrogen Fuels; Technical Report Project DE-FC26-08NT05054; DOE: Washington, DC, USA, 2014.
- Worth, N.; Dawson, J. Modal dynamics of self-excited azimuthal instabilities in an annular combustion chamber. Combust. Flame 2013, 160, 2476–2489. [Google Scholar] [CrossRef]
- Betz, M.; Zahn, M.; Sattelmayer, T. Influence of dampers on the damping rates of a lab-scale annular combustor. In Proceedings of the 24th International Congress on Sound and Vibration, London, UK, 23–27 July 2017. [Google Scholar]
- Giusti, A.; Mastorakos, E. Turbulent combustion modelling and experiments: Recent trends and developments. Flow Turbul. Combust. 2019, 103, 847–869. [Google Scholar] [CrossRef]
- Driscoll, J.F.; Chen, J.H.; Skiba, A.W.; Carter, C.D.; Hawkes, E.R.; Wang, H. Premixed flames subjected to extreme turbulence: Some questions and recent answers. Prog. Energy Combust. Sci. 2020, 76, 100802. [Google Scholar] [CrossRef]
- Steinberg, A.M.; Hamlington, P.E.; Zhao, X. Structure and dynamics of highly turbulent premixed combustion. Prog. Energy Combust. Sci. 2021, 85, 100900. [Google Scholar] [CrossRef]
- Palies, P.P. Hydrogen Thermal-Powered Aircraft Combustion and Propulsion System. J. Eng. Gas Turbines Power 2022, 144, 101007. [Google Scholar] [CrossRef]
- Moureau, V.; Domingo, D.; Vervisch, L. From Large-Eddy Simulation to Direct Numerical Simulation of a lean premixed swirl flame: Filtered laminar flame-PDF modeling. Combust. Flame 2011, 158, 1340–1357. [Google Scholar] [CrossRef]
- Bell, J.; Day, M.; Lijewski, M. Simulation of nitrogen emissions in a premixed hydrogen flame stabilized on a low swirl burner. Proc. Combust. Inst. 2013, 34, 1173–1182. [Google Scholar] [CrossRef]
- Schmid, P.J. Dynamic mode decomposition of numerical and experimental data. J. Fluid Mech. 2010, 656, 5–28. [Google Scholar] [CrossRef]
- Xu, C.; Park, J.W.; Yoo, C.S.; Chen, J.H.; Lu, T. Identification of premixed flame propagation modes using chemical explosive mode analysis. Proc. Combust. Inst. 2019, 37, 2407–2415. [Google Scholar] [CrossRef]
- Benard, P.; Lartigue, G.; Moureau, V.; Mercier, R. Large-Eddy Simulation of the lean-premixed PRECCINSTA burner with wall heat loss. Proc. Combust. Inst. 2019, 37, 5233–5243. [Google Scholar] [CrossRef]
- Mercier, R.; Moureau, V.; Veynante, D.; Fiorina, B. LES of turbulent combustion: On the consistency between flame and flow filter scales. Proc. Combust. Inst. 2015, 35, 1359–1366. [Google Scholar] [CrossRef]
- Franzelli, B.; Riber, E.; Cuenot, B. Impact of the chemical description on a Large Eddy Simulation of a lean partially premixed swirled flame. Comptes Rendus Mécanique 2013, 341, 247–256. [Google Scholar] [CrossRef]
- Wang, P.; Platova, N.; Fröhlich, J.; Maas, U. Large eddy simulation of the PRECCINSTA burner. Int. J. Heat Mass Transf. 2014, 70, 486–495. [Google Scholar] [CrossRef]
- Ansari, N.; Strakey, P.; Goldin, G.; Givi, P. Filtered density function simulation of a realistic swirled combustor. Proc. Combust. Inst. 2015, 35, 1433–1442. [Google Scholar] [CrossRef]
- Bell, J.; Day, M.; Almgren, A.; Cheng, R.; Shepherd, I. Numerical simulation of premixed turbulent methane combustion. In Computational Fluid and Solid Mechanics 2003; Elsevier: Amsterdam, The Netherlands, 2003; pp. 1247–1250. [Google Scholar]
- Cheng, R.; Littlejohn, D.; Strakey, P.; Sidwell, T. Laboratory investigations of a low-swirl injector with H2 and CH4 at gas turbine conditions. Proc. Combust. Inst. 2009, 32, 3001–3009. [Google Scholar] [CrossRef]
- Day, M.; Tachibana, S.; Bell, J.; Lijewski, M.; Beckner, V.; Cheng, R. A combined computational and experimental characterization of lean premixed turbulent low swirl laboratory flames: I. Methane flames. Combust. Flame 2012, 159, 275–290. [Google Scholar] [CrossRef]
- Day, M.; Tachibana, S.; Bell, J.; Lijewski, M.; Beckner, V.; Cheng, R.K. A combined computational and experimental characterization of lean premixed turbulent low swirl laboratory flames II. Hydrogen flames. Combust. Flame 2015, 162, 2148–2165. [Google Scholar] [CrossRef]
- Nogenmyr, K.J.; Fureby, C.; Bai, X.S.; Petersson, P.; Collin, R.; Linne, M. Large eddy simulation and laser diagnostic studies on a low swirl stratified premixed flame. Combust. Flame 2009, 156, 25–36. [Google Scholar] [CrossRef]
- Mao, R.; Wang, J.; Zhang, W.; An, Z.; Lin, W.; Zhang, M.; Huang, Z. Effect of high hydrogen enrichment on the outer-shear-layer flame of confined lean premixed CH4/H2/air swirl flames. Int. J. Hydrogen Energy 2021, 46, 17969–17981. [Google Scholar] [CrossRef]
- Dupuy, F.; Gatti, M.; Mirat, C.; Gicquel, L.; Nicoud, F.; Schuller, T. Combining analytical models and LES data to determine the transfer function from swirled premixed flames. Combust. Flame 2020, 217, 222–236. [Google Scholar] [CrossRef]
- Chong, L.; Komarek, T.; Kaess, R.; Foller, S.; Polifke, W. Identification of flame transfer functions from LES of a premixed swirl burner. In Proceedings of the ASME Turbo Expo 2010: Power for Land, Sea, and Air, Glasgow, UK, 14–18 June 2010. Number GT2010-22769. [Google Scholar]
- Tay-Wo-Chong, L.; Polifke, W. Large eddy simulation-based study of the influence of thermal boundary condition and combustor confinement on premix flame transfer functions. J. Eng. Gas Turbines Power 2013, 135, 021502. [Google Scholar] [CrossRef]
- Nogenmyr, K.J.; Cao, H.; Chan, C.; Cheng, R. Effects of confinement on premixed turbulent swirling flame using large eddy simulation. Combust. Theory Model. 2013, 17, 1003–1019. [Google Scholar] [CrossRef]
- Karlis, E.; Liu, Y.; Hardalupas, Y.; Taylor, A.M. Extinction strain rate suppression of the precessing vortex core in a swirl stabilised combustor and consequences for thermoacoustic oscillations. Combust. Flame 2020, 211, 229–252. [Google Scholar] [CrossRef]
- Guiberti, T.; Zimmer, L.; Durox, D.; Schuller, T. Experimental analysis of V-to M-shape transition of premixed CH4/H2/air swirling flames. In Proceedings of the ASME Turbo Expo 2013: Turbine Technical Conference and Exposition, San Antonio, TX, USA, 3–7 June 2013; American Society of Mechanical Engineers: New York, NY, USA, 2013; Volume 55102, p. V01AT04A063. [Google Scholar]
- Zhang, J.; Ratner, A. Experimental study on the excitation of thermoacoustic instability of hydrogen-methane/air premixed flames under atmospheric and elevated pressure conditions. Int. J. Hydrogen Energy 2019, 44, 21324–21335. [Google Scholar] [CrossRef]
- Mansouri, Z.; Aouissi, M.; Boushaki, T. Numerical computations of premixed propane flame in a swirl-stabilized burner: Effects of hydrogen enrichment, swirl number and equivalence ratio on flame characteristics. Int. J. Hydrogen Energy 2016, 41, 9664–9678. [Google Scholar] [CrossRef]
- Burguburu, J.; Cabot, G.; Renou, B.; Boukhalfa, A.M.; Cazalens, M. Effects of H2 enrichment on flame stability and pollutant emissions for a kerosene/air swirled flame with an aeronautical fuel injector. Proc. Combust. Inst. 2011, 33, 2927–2935. [Google Scholar] [CrossRef]
- Malanoski, M.; Aguilar, M.; Shin, D.H.; Lieuwen, T. Flame leading edge and flow dynamics in a swirling, lifted flame. Combust. Sci. Technol. 2014, 186, 1816–1843. [Google Scholar] [CrossRef]
- Laera, D.; Agostinelli, P.W.; Selle, L.; Cazères, Q.; Oztarlik, G.; Schuller, T.; Gicquel, L.; Poinsot, T. Stabilization mechanisms of CH4 premixed swirled flame enriched with a non-premixed hydrogen injection. Proc. Combust. Inst. 2021, 38, 6355–6363. [Google Scholar] [CrossRef]
- Taamallah, S.; Shanbhogue, S.J.; Ghoniem, A.F. Turbulent flame stabilization modes in premixed swirl combustion: Physical mechanism and Karlovitz number-based criterion. Combust. Flame 2016, 166, 19–33. [Google Scholar] [CrossRef]
- Bertsch, J.; Poinsot, T.; Bertier, N.; Ruan, J.L. Stabilization regimes and flame structure at the flame base of a swirled lean premixed hydrogen–air injector with a pure hydrogen pilot injection. Proc. Combust. Inst. 2024, 40, 105660. [Google Scholar] [CrossRef]
- Foley, C.; Chterev, I.; Noble, B.; Seitzman, J.; Lieuwen, T. Shear layer flame stabilization sensitivities in a swirling flow. Int. J. Spray Combust. Dyn. 2017, 9, 3–18. [Google Scholar] [CrossRef]
- Iudiciani, P.; Duwig, C. Large eddy simulation of the sensitivity of vortex breakdown and flame stabilisation to axial forcing. Flow Turbul. Combust. 2011, 86, 639–666. [Google Scholar] [CrossRef]
- Lacoste, D.; Moeck, J.; Durox, D.; Laux, C.; Schuller, T. Effect of nanosecond repetitively pulsed discharges on the dynamics of a swirl-stabilized lean premixed flame. J. Eng. Gas Turbines Power 2013, 135, 101501. [Google Scholar] [CrossRef]
- Barbosa, S.; Pilla, G.; Lacoste, D.; Scouflaire, P.; Ducruix, S.; Laux, C.O.; Veynante, D. Influence of nanosecond repetitively pulsed discharges on the stability of a swirled propane/air burner representative of an aeronautical combustor. Phil. Trans. R. Soc. A 2015, 373, 20140335. [Google Scholar] [CrossRef] [PubMed]
- Stöhr, M.; Arndt, C.; Meier, W. Transient effects of fuel–air mixing in a partially-premixed turbulent swirl flame. Proc. Combust. Inst. 2015, 35, 3327–3335. [Google Scholar] [CrossRef]
- De Rosa, A.J.; Peluso, S.J.; Quay, B.D.; Santavicca, D.A. Lean-Premixed, Swirl-Stabilized Flame Response: Flame Structure and Response as a Function of Confinement. J. Eng. Gas Turbines Power 2018, 140, 031504. [Google Scholar] [CrossRef]
- Kalantari, A.; McDonell, V. Boundary layer flashback of non-swirling premixed flames: Mechanisms, fundamental research, and recent advances. Prog. Energy Combust. Sci. 2017, 61, 249–292. [Google Scholar] [CrossRef]
- Benim, A.C.; Syed, K.J. Flashback Mechanisms in Lean Premixed Gas Turbine Combustion; Academic Press: Cambridge, MA, USA, 2014. [Google Scholar]
- Plee, S.; Mellor, A. Review of flashback reported in prevaporizing/premixing combustors. Combust. Flame 1978, 32, 193–203. [Google Scholar] [CrossRef]
- Nauert, A.; Petersson, P.; Linne, M.; Dreizler, A. Experimental analysis of flashback in lean premixed swirling flames: Conditions close to flashback. Exp. Fluids 2007, 43, 89–100. [Google Scholar] [CrossRef]
- Ebi, D.; Clemens, N.T. Experimental investigation of upstream flame propagation during boundary layer flashback of swirl flames. Combust. Flame 2016, 168, 39–52. [Google Scholar] [CrossRef]
- Ebi, D.; Ranjan, R.; Clemens, N.T. Coupling between premixed flame propagation and swirl flow during boundary layer flashback. Exp. Fluids 2018, 59, 1–16. [Google Scholar] [CrossRef]
- Hoferichter, V.; Hirsch, C.; Sattelmayer, T.; Kalantari, A.; Sullivan-Lewis, E.; McDonell, V. Comparison of two methods to predict boundary layer flashback limits of turbulent hydrogen-air jet flames. Flow Turbul. Combust. 2018, 100, 849–873. [Google Scholar] [CrossRef]
- Ebi, D.; Bombach, R.; Jansohn, P. Swirl flame boundary layer flashback at elevated pressure: Modes of propagation and effect of hydrogen addition. Proc. Combust. Inst. 2021, 38, 6345–6353. [Google Scholar] [CrossRef]
- Heeger, C.; Gordon, R.; Tummers, M.; Sattelmayer, T.; Dreizler, A. Experimental analysis of flashback in lean premixed swirling flames: Upstream flame propagation. Exp. Fluids 2010, 49, 853–863. [Google Scholar] [CrossRef]
- Syred, N.; Giles, A.; Lewis, J.; Abdulsada, M.; Medina, A.V.; Marsh, R.; Bowen, P.J.; Griffiths, A.J. Effect of inlet and outlet configurations on blow-off and flashback with premixed combustion for methane and a high hydrogen content fuel in a generic swirl burner. Appl. Energy 2014, 116, 288–296. [Google Scholar] [CrossRef]
- Sullivan Lewis, E.; McDonell, V.G.; Kalantari, A.; Saxena, P. Evaluation of a Turbulent Jet Flame Flashback Correlation Applied to Annular Flow Configurations With and Without Swirl. J. Eng. Gas Turbines Power 2020, 144, 091018. [Google Scholar] [CrossRef]
- Reichel, T.G.; Paschereit, C.O. Interaction mechanisms of fuel momentum with flashback limits in lean-premixed combustion of hydrogen. Int. J. Hydrogen Energy 2017, 42, 4518–4529. [Google Scholar] [CrossRef]
- Reichel, T.G.; Terhaar, S.; Paschereit, O. Increasing flashback resistance in lean premixed swirl-stabilized hydrogen combustion by axial air injection. J. Eng. Gas Turbines Power 2015, 137, 071503. [Google Scholar] [CrossRef]
- Al-Fahham, M.; Hatem, F.A.; Alsaegh, A.S.; Valera Medina, A.; Bigot, S.; Marsh, R. Experimental study to enhance resistance for boundary layer flashback in swirl burners using microsurfaces. In Proceedings of the ASME Turbo Expo 2017: Turbomachinery Technical Conference and Exposition, Charlotte, NC, USA, 26–30 June 2017; American Society of Mechanical Engineers: New York, NY, USA, 2017; Volume 50848, p. V04AT04A030. [Google Scholar]
- Hoferichter, V.; Hirsch, C.; Sattelmayer, T. Analytic prediction of unconfined boundary layer flashback limits in premixed hydrogen-air flames. Combust. Theory Model. 2017, 21, 382–418. [Google Scholar] [CrossRef]
- Burmberger, S.; Sattelmayer, T. Optimization of the aerodynamic flame stabilization for fuel flexible gas turbine premix burners. J. Eng. Gas Turbines Power 2011, 133. [Google Scholar] [CrossRef]
- Burmberger, S.; Hirsch, C.; Sattelmayer, T. Design rules for the velocity field of vortex breakdown swirl burners. In Proceedings of the Turbo Expo: Power for Land, Sea, and Air, Barcelona, Spain, 8–11 May 2006; Volume 42363, pp. 413–421. [Google Scholar]
- Eichler, C.; Baumgartner, G.; Sattelmayer, T. Experimental investigation of turbulent boundary layer flashback limits for premixed hydrogen-air flames confined in ducts. J. Eng. Gas Turbines Power 2012, 134, 011502. [Google Scholar] [CrossRef]
- Kiesewetter, F.; Konle, M.; Sattelmayer, T. Analysis of combustion induced vortex breakdown driven flame flashback in a premix burner with cylindrical mixing zone. J. Eng. Gas Turbines Power 2007, 129, 929–936. [Google Scholar] [CrossRef]
- Stöhr, M.; Boxx, I.; Carter, C.; Meier, W. Dynamics of lean blowout of a swirl-stabilized flame in a gas turbine model combustor. Proc. Combust. Inst. 2011, 33, 2953–2960. [Google Scholar] [CrossRef]
- Muruganandam, T.; Nair, S.; Olsen, R.; Neumeier, Y.; Meyers, A.; Lieuwen, T.; Seitzman, J.; Jagoda, J.; Zinn, B. Blowout control in turbine engine combustors. In Proceedings of the 42nd AIAA Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 5–8 January 2004. [Google Scholar]
- Muruganandam, T.; Seitzman, J. Origin of Lean Blow out Precursors in Swirled Gas Turbine Combustors. In Proceedings of the 43rd AIAA Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 10–13 January 2005; p. 1163. [Google Scholar]
- Cavaliere, D.E.; James, K.; Mastorakos, E. A Comparison of the Blow-Off Behaviour of Swirl-Stabilized Premixed, Non-Premixed and Spray Flames. Flow Turbul. Combust. 2013, 91, 347–372. [Google Scholar] [CrossRef]
- Tyliszczak, A.; Cavaliere, D.E.; Mastorakos, E. LES/CMC of Blow-off in a Liquid Fueled Swirl Burner. Flow Turbul. Combust. 2014, 92, 237–267. [Google Scholar] [CrossRef]
- Zhang, H.; Mastorakos, E. Prediction of Global Extinction Conditions and Dynamics in Swirling Non-premixed Flames Using LES/CMC Modelling. Flow Turbul. Combust. 2016, 96, 863–889. [Google Scholar] [CrossRef]
- Giusti, A.; Kotzagianni, M.; Mastorakos, E. LES/CMC simulations of swirl-stabilised ethanol spray flames approaching blow-off. Flow Turbul. Combust. 2016, 97, 1165–1184. [Google Scholar] [CrossRef] [PubMed]
- Giusti, A.; Mastorakos, E. Detailed chemistry LES/CMC simulation of a swirling ethanol spray flame approaching blow-off. Proc. Combust. Inst. 2017, 36, 2625–2632. [Google Scholar] [CrossRef]
- Guiberti, T.F.; Durox, D.; Zimmer, L.; Schuller, T. Analysis of topology transitions of swirl flames interacting with the combustor side wall. Combust. Flame 2015, 162, 4342–4357. [Google Scholar] [CrossRef]
- Degenève, A.; Jourdaine, P.; Mirat, C.; Caudal, J.; Vicquelin, R.; Schuller, T. Effects of a Diverging Cup on Swirl Number, Flow Pattern, and Topology of Premixed Flames. J. Eng. Gas Turbines Power 2019, 141, 031022. [Google Scholar] [CrossRef]
- Crocco, L. Research on combustion instability in liquid propellant rockets. Proc. Combust. Inst. 1968, 12, 85–99. [Google Scholar] [CrossRef]
- Putnam, A. Combustion Driven Oscillations in Industry; Elsevier: New York, NY, USA, 1971. [Google Scholar]
- Culick, F. Dynamics of combustion systems: Fundamentals, acoustics and control. In Proceedings of the RTO AVT Course on “Active Control of Engine Dynamics”, Brussels, Belgium, 14–18 May 2001. [Google Scholar]
- Candel, S. Combustion Dynamics and Control: Progress and Challenges. Proc. Combust. Inst. 2002, 29, 1–28. [Google Scholar] [CrossRef]
- Lawn, C.J.; Penelet, G. Common features in the thermoacoustics of flames and engines. Int. J. Spray Combust. Dyn. 2017, 10, 3–37. [Google Scholar] [CrossRef]
- Juniper, M.P.; Sujith, R. Sensitivity and nonlinearity of thermoacoustic oscillations. Annu. Rev. Fluid Mech. 2017, 50, 661–689. [Google Scholar] [CrossRef]
- Silva, C.; Magri, L.; Runte, T.; Polifke, W. Uncertainty quantification of growth rates of thermoacoustic instability by an adjoint Helmholtz solver. J. Eng. Gas Turbines Power 2017, 139, 011901. [Google Scholar] [CrossRef]
- Juniper, M.P. Sensitivity analysis of thermoacoustic instability with adjoint Helmholtz solvers. Phys. Rev. Fluids 2018, 3, 110509. [Google Scholar] [CrossRef]
- Magri, L. Adjoint methods as design tools in thermoacoustics. Appl. Mech. Rev. 2019, 71, 020801. [Google Scholar] [CrossRef]
- Aguilar, J.G.; Juniper, M.P. Thermoacoustic stabilization of a longitudinal combustor using adjoint methods. Phys. Rev. Fluids 2020, 5, 083902. [Google Scholar] [CrossRef]
- Rayleigh, L. The explanation of certain acoustical phenomena. Roy. Inst. Proc. 1878, 8, 536–542. [Google Scholar] [CrossRef]
- Chu, B.T. Stability of Systems Containing a Heat Source-The Rayleigh Criterion; National Advisory Committee for Aeronautics: Washington, DC, USA, 1956; Volume 70.
- Nicoud, F.; Poinsot, T. Thermoacoustic instabilities: Should the Rayliegh criterion be extended to include entropy changes. Combust. Flame 2005, 142, 153–159. [Google Scholar] [CrossRef]
- Culick, F.E.C. Unsteady Motions in Combustion Chambers for Propulsion Systems; AGARDograph; NATO/RTO-AG-AVT-039; North Atlantic Treaty Organisation Research and Technology Organisation: Neuilly-sur-Seine, France, 2006. [Google Scholar]
- Huang, Y.; Ratner, A. Experimental investigation of thermoacoustic coupling for low-swirl lean premixed flames. J. Propuls. Power 2009, 25, 365–373. [Google Scholar] [CrossRef]
- Kang, D.M.; Culick, F.E.C.; Ratner, A. Combustion dynamics of a low-swirl combustor. Combust. Flame 2007, 151, 412–425. [Google Scholar] [CrossRef]
- Lieuwen, T. Modeling premixed combustion-acoustic wave interactions: A review. J. Propuls. Power 2003, 19, 765–781. [Google Scholar] [CrossRef]
- Ducruix, S.; Schuller, T.; Durox, D.; Candel, S. Combustion dynamics and instabilities: Elementary coupling and driving mechanisms. J. Propuls. Power 2003, 19, 722–734. [Google Scholar] [CrossRef]
- Broda, J.C.; Seo, S.; Santoro, R.J.; Shirhattikar, G.; Yang, V. An experimental study of combustion dynamics of a premixed swirl injector. Proc. Combust. Inst. 1998, 27, 1849–1856. [Google Scholar] [CrossRef]
- Huang, Y.; Yang, V. Bifurcation of flame structure in a lean-premixed swirl-stabilized combustor: Transition from stable to unstable flame. Combust. Flame 2004, 136, 383–389. [Google Scholar] [CrossRef]
- Gotoda, H.; Nikimoto, H.; Miyano, T.; Tachibana, S. Dynamic properties of combustion instability in a lean premixed gas-turbine combustor. Chaos 2011, 21, 013124. [Google Scholar] [CrossRef]
- Nagaraja, S.; Kedia, K.; Sujith, R. Characterizing energy growth during combustion instabilities: Singularvalues or eigenvalues? Proc. Combust. Inst. 2009, 32, 2933–2940. [Google Scholar] [CrossRef]
- Palies, P.; Davis, D.; Cheng, R.; Ilak, M. Dynamic Mode Decomposition (DMD) Application to Premixed Low Swirl Injector Flames. In Proceedings of the 68th Annual Meeting of the APS Division of Fluid Dynamics, Boston, MA, USA, 22–24 November 2015; Volume 60. [Google Scholar]
- Sayadi, T.; Schmid, P.; Richecoeur, F.; Durox, D. Parametrized data-driven decomposition for bifurcation analysis, with application to thermo-acoustically unstable systems. Phys. Fluids 2015, 27, 037102. [Google Scholar] [CrossRef]
- Motheau, E.; Nicoud, F.; Poinsot, T. Mixed acoustic-entropy combustion instabilities in gas turbines. J. Fluid Mech. 2014, 749, 542–576. [Google Scholar] [CrossRef]
- LaBry, Z.A.; Taamallah, S.; Kewlani, G.; Shanbhogue, S.J.; Ghoniem, A.F. Mode transition and intermittency in an acoustically uncoupled lean premixed swirl-stabilized combustor. In Proceedings of the ASME Turbo Expo 2014: Turbine Technical Conference and Exposition, Düsseldorf, Germany, 16–20 June 2014; American Society of Mechanical Engineers: New York, NY, USA, 2014; Volume 45691, p. V04BT04A061. [Google Scholar]
- Bonciolini, G.; Ebi, D.; Doll, U.; Weilenmann, M.; Noiray, N. Effect of wall thermal inertia upon transient thermoacoustic dynamics of a swirl-stabilized flame. Proc. Combust. Inst. 2019, 37, 5351–5358. [Google Scholar] [CrossRef]
- Shoji, T.; Tachibana, S.; Nakazumi, Y.; Fujii, R.; Masugi, J.; Yokomori, T. Detailed unsteady dynamics of flame-flow interactions during combustion instability and its transition scenario for lean-premixed low-swirl hydrogen turbulent flames. Proc. Combust. Inst. 2023, 39, 4741–4750. [Google Scholar] [CrossRef]
- Gong, Y.; Fredrich, D.; Marquis, A.J.; Jones, W.P. Numerical investigation of combustion instabilities in swirling flames with hydrogen enrichment. Flow, Turbul. Combust. 2023, 111, 953–993. [Google Scholar] [CrossRef]
- Kabiraj, L.; Saurabh, A.; Wahi, P.; Sujith, R. Route to chaos for combustion instability in ducted laminar premixed flames. Chaos Interdiscip. J. Nonlinear Sci. 2012, 22, 023129. [Google Scholar] [CrossRef]
- Nair, V.; Thampi, G.; Sujith, R. Intermittency route to thermoacoustic instability in turbulent combustors. J. Fluid Mech. 2014, 756, 470–487. [Google Scholar] [CrossRef]
- Nair, V.; Sujith, R. A reduced-order model for the onset of combustion instability: Physical mechanisms for intermittency and precursors. Proc. Combust. Inst. 2015, 35, 3193–3200. [Google Scholar] [CrossRef]
- Seshadri, A.; Nair, V.; Sujith, R. A reduced-order deterministic model describing an intermittency route to combustion instability. Combust. Theory Model. 2016, 20, 441–456. [Google Scholar] [CrossRef]
- Gotoda, H.; Shinoda, Y.; Kobayashi, M.; Okuno, Y.; Tachibana, S. Detection and control of combustion instability based on the concept of dynamical system theory. Phys. Rev. E 2014, 89, 022910. [Google Scholar] [CrossRef] [PubMed]
- Gotoda, H.; Okuno, Y.; Hayashi, K.; Tachibana, S. Characterization of degeneration process in combustion instability based on dynamical systems theory. Phys. Rev. E 2015, 92, 052906. [Google Scholar] [CrossRef]
- Kheirkhah, S.; Cirtwill, J.M.; Saini, P.; Venkatesan, K.; Steinberg, A.M. Dynamics and mechanisms of pressure, heat release rate, and fuel spray coupling during intermittent thermoacoustic oscillations in a model aeronautical combustor at elevated pressure. Combust. Flame 2017, 185, 319–334. [Google Scholar] [CrossRef]
- Kushwaha, A.; Kasthuri, P.; Pawar, S.A.; Sujith, R.; Chterev, I.; Boxx, I. Dynamical Characterization of Thermoacoustic Oscillations in a Hydrogen-Enriched Partially Premixed Swirl-Stabilized Methane/Air Combustor. J. Eng. Gas Turbines Power 2021, 143, 121022. [Google Scholar] [CrossRef]
- Magri, L.; O’Brien, J.; Ihme, M. Compositional inhomogeneities as a source of indirect combustion noise. J. Fluid Mech. 2016, 799. [Google Scholar] [CrossRef]
- Giusti, A.; Magri, L.; Zedda, M. Flow inhomogeneities in a realistic aeronautical gas-turbine combustor: Formation, evolution, and indirect noise. J. Eng. Gas Turbines Power 2019, 141, 011502. [Google Scholar] [CrossRef]
- De Domenico, F.; Rolland, E.O.; Rodrigues, J.; Magri, L.; Hochgreb, S. Compositional and entropy indirect noise generated in subsonic non-isentropic nozzles. J. Fluid Mech. 2021, 910, A5. [Google Scholar] [CrossRef]
- Zhao, D.; Xia, Y.; Ge, H.; Lin, Q.; Wang, G. Large eddy simulation of flame propagation during the ignition process in an annular multiple-injector combustor. Fuel 2020, 263, 116402. [Google Scholar] [CrossRef]
- Zhong, L.; Yang, Y.; Jin, T.; Xia, Y.; Fang, Y.; Zheng, Y.; Wang, G. Local flame and flow properties of propagating premixed turbulent flames during light-round process in a MICCA-type annular combustor. Combust. Flame 2021, 231, 111494. [Google Scholar] [CrossRef]
- Wang, G.; Zhong, L.; Yang, Y.; Zheng, Y.; Fang, Y.; Xia, Y.; Ye, C. Experimental investigation of the ignition dynamics in an annular premixed combustor with oblique-injecting swirling burners. Fuel 2021, 287, 119494. [Google Scholar] [CrossRef]
- Worth, N.A.; Dawson, J.R. Self-excited circumferential instabilities in a model annular gas turbine combustor: Global flame dynamics. Proc. Combust. Inst. 2013, 34, 3127–3134. [Google Scholar] [CrossRef]
- Palies, P.; Durox, D.; Schuller, T.; Morenton, P.; Candel, S. Dynamics of premixed confined swirling flames. Comptes Rendus Mecanique 2009, 337, 395–405. [Google Scholar] [CrossRef]
- Baillot, F.; Patat, C.; Cáceres, M.; Blaisot, J.B.; Domingues, E. Saturation phenomenon of swirling spray flames at pressure antinodes of a transverse acoustic field. Proc. Combust. Inst. 2021, 38, 5987–5995. [Google Scholar] [CrossRef]
- Worth, N.A.; Dawson, J.R.; Sidey, J.A.; Mastorakos, E. Azimuthally forced flames in an annular combustor. Proc. Combust. Inst. 2017, 36, 3783–3790. [Google Scholar] [CrossRef]
- Nygård, H.T.; Mazur, M.; Dawson, J.R.; Worth, N.A. Flame dynamics of azimuthal forced spinning and standing modes in an annular combustor. Proc. Combust. Inst. 2019, 37, 5113–5120. [Google Scholar] [CrossRef]
- Liu, W.; Xue, R.; Zhang, L.; Yang, Q.; Wang, H. Nonlinear response of a premixed low-swirl flame to acoustic excitation with large amplitude. Combust. Flame 2021, 235, 111733. [Google Scholar] [CrossRef]
- Yilmaz, I.; Ratner, A.; Ilbas, M.; Huang, Y. Experimental investigation of thermoacoustic coupling using blended hydrogen-methane fuels in a low swirl burner. Int. J. Hydrogen Energy 2010, 35, 329–336. [Google Scholar] [CrossRef]
- Æsøy, E.; Indlekofer, T.; Gant, F.; Cuquel, A.; Bothien, M.R.; Dawson, J.R. The effect of hydrogen enrichment, flame-flame interaction, confinement, and asymmetry on the acoustic response of a model can combustor. Combust. Flame 2022, 242, 112176. [Google Scholar] [CrossRef]
- Freitag, E.; Konle, H.; Lauer, M.; Hirsch, C.; Sattelmayer, T. Pressure influence on the flame transfer function of a premixed swirling flame. In Proceedings of the ASME Turbo Expo 2006: Power for Land, Sea, and Air, Barcelona, Spain, 8–11 May 2006; Volume 42363, pp. 477–486. [Google Scholar]
- Freitag, E. On the Measurement and Modelling of Flame Transfer Functions at Elevated Pressure. Ph.D. Thesis, Technische Universität München, Munich, Germany, 2009. [Google Scholar]
- Di Sabatino, F.; Guiberti, T.; Moeck, J.; Roberts, W.; Lacoste, D. Influence of the laminar burning velocity on the transfer function of premixed methane and propane swirl flames. In Proceedings of the ASME Turbo Expo 2019: Turbomachinery Technical Conference and Exposition, Phoenix, AZ, USA, 17–21 June 2019. GT2019-90870. [Google Scholar]
- Schuermans, B.B.; Polifke, W.; Paschereit, C.O. Modeling transfer matrices of premixed flames and comparison with experimental results. In Proceedings of the ASME 1999 International Gas Turbine and Aeroengine Congress and Exhibition, Indianapolis, IN, USA, 7–10 June 1999; American Society of Mechanical Engineers: New York, NY, USA, 1999; Volume 78590, p. V002T02A024. [Google Scholar]
- Schuermans, B.; Bellucci, V.; Guethe, F.; Meili, F.o.; Flohr, P.; Paschereit, C.O. A detailed analysis of thermoacoustic interaction mechanisms in a turbulent premixed flame. In Proceedings of the ASME Turbo Expo 2004: Power for Land, Sea, and Air, Vienna, Austria, 14–17 June 2004; Volume 41669, pp. 539–551. [Google Scholar]
- Ćosić, B.; Terhaar, S.; Moeck, J.; Paschereit, C. Response of a swirl-stabilized flame to simultaneous perturbations in equivalence ratio and velocity at high oscillation amplitudes. Combust. Flame 2015, 162, 1046–1062. [Google Scholar] [CrossRef]
- Kather, V.; Löckoff, F.; Paschereit, C.O.; Oberleithner, K. Interaction of equivalence ratio fluctuations and flow fluctuations in acoustically forced swirl flames. Int. J. Spray Combust. Dyn. 2021, 13, 72–95. [Google Scholar] [CrossRef]
- Nygård, H.T.; Ghirardo, G.; Worth, N.A. Azimuthal flame response and symmetry breaking in a forced annular combustor. Combust. Flame 2021, 233, 111565. [Google Scholar] [CrossRef]
- Mirat, C.; Durox, D.; Schuller, T. Analysis of the spray and transfer function of swirling spray flames from a multi-jet steam assisted liquid fuel injector. In Proceedings of the ASME Turbo Expo 2014: Turbine Technical Conference and Exposition, Düsseldorf, Germany, 16–20 June 2014; American Society of Mechanical Engineers: New York, NY, USA, 2014. [Google Scholar]
- Biagioli, F.; Scarpato, A.; Syed, K. Dynamic response of swirl stabilized turbulent premixed flames based on the Helmholtz-Hodge velocity decomposition. Flow Turbul. Combust. 2016, 96, 1005–1022. [Google Scholar] [CrossRef]
- Xia, Y.; Laera, D.; Jones, W.P.; Morgans, A.S. Numerical prediction of the flame describing function and thermoacoustic limit cycle for a pressurized gas turbine combustor. Combust. Sci. Technol. 2019, 191, 979–1002. [Google Scholar] [CrossRef]
- Hermeth, S.; Staffelbach, G.; Gicquel, L.; Poinsot, T. LES evaluation of the effects of equivalence ratio fluctuations on the dynamic flame response in a real gas turbine combustion chamber. Proc. Combust. Inst. 2013, 34, 3165–3173. [Google Scholar] [CrossRef]
- Palies, P.; Schuller, T.; Durox, D.; Candel, S. Modeling of premixed swirling flames transfer functions. Proc. Combust. Inst. 2011, 33, 2967–2974. [Google Scholar] [CrossRef]
- Xia, Y.; Duran, I.; Morgans, A.S.; Han, X. Dispersion of entropy perturbations transporting through an industrial gas turbine combustor. Flow Turbul. Combust. 2018, 100, 481–502. [Google Scholar] [CrossRef] [PubMed]
- Palies, P.; Acharya, R.; Hoffie, A.; Thomas, M. Lean Fully Premixed Injection for Commercial Jet Engines: An Initial Design Study. In Proceedings of the ASME Turbo Expo 2019: Turbomachinery Technical Conference and Exposition, Phoenix, AZ, USA, 17–21 June 2019. GT2019-91653. [Google Scholar]
- Yahou, T.; Dawson, J.R.; Poinsot, T.; Selle, L.; Schuller, T. Flame front dynamics during ignition of lean premixed H2/Air and CH4/Air flames. Combust. Flame 2024, 269, 113708. [Google Scholar] [CrossRef]
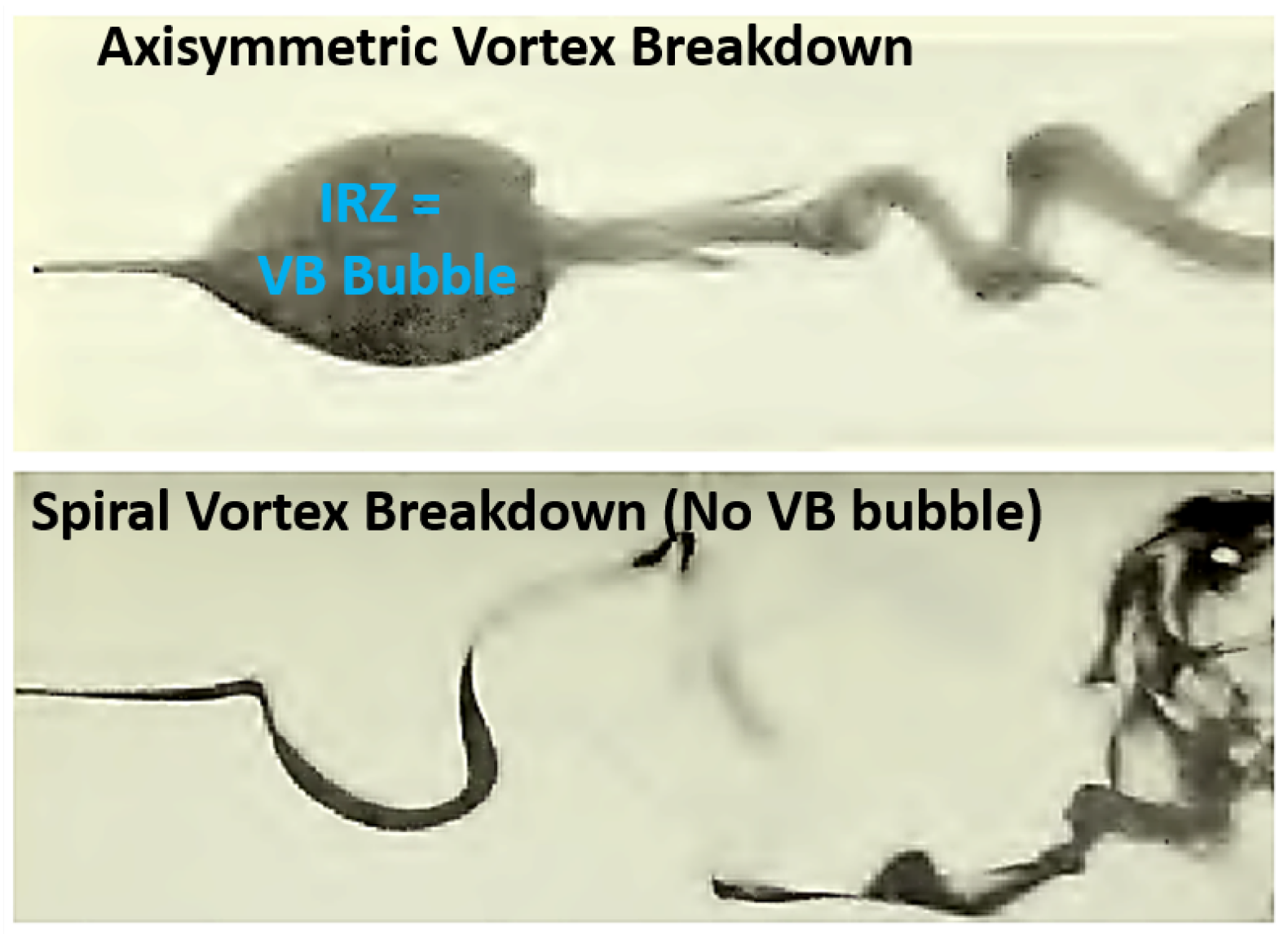
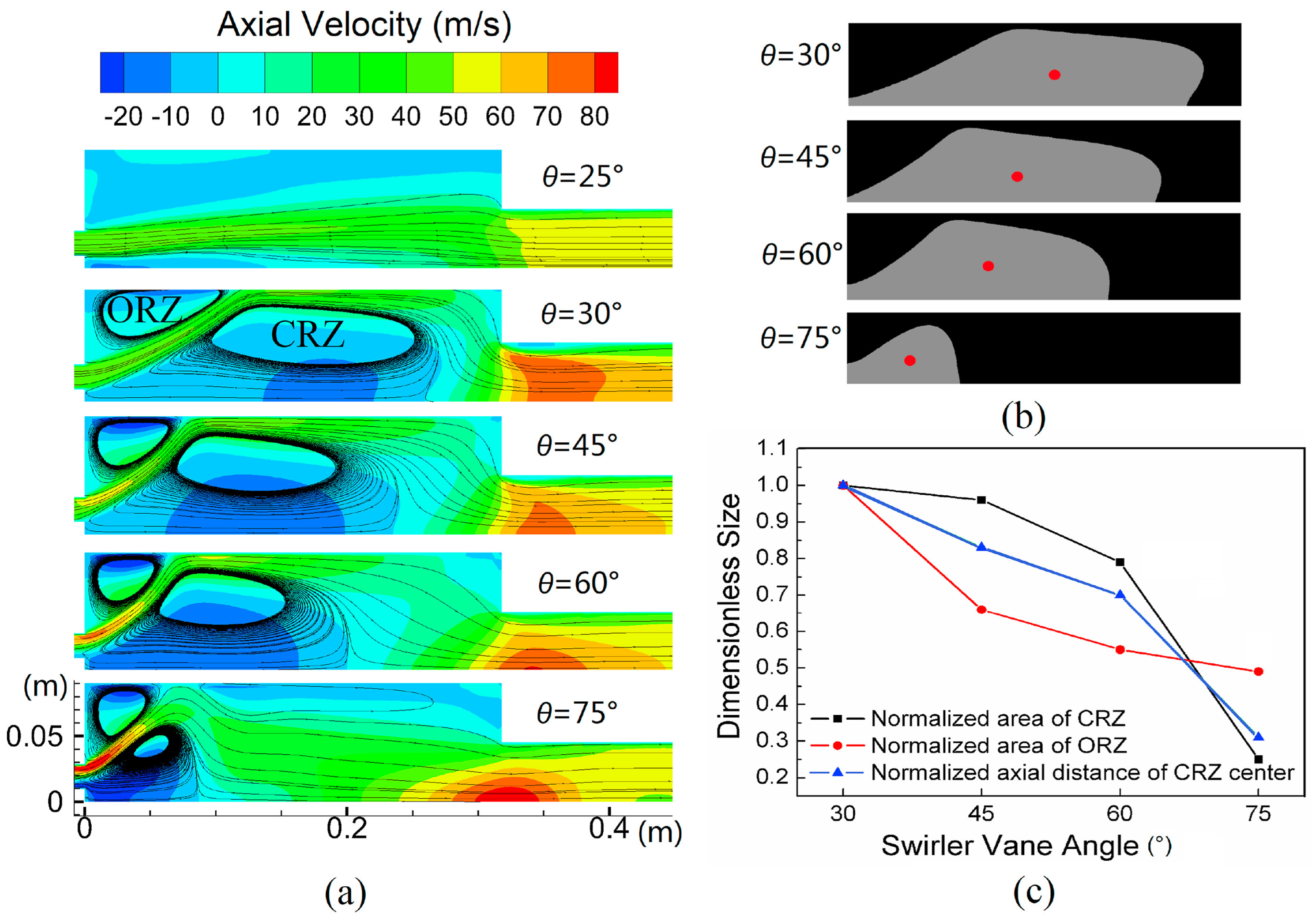
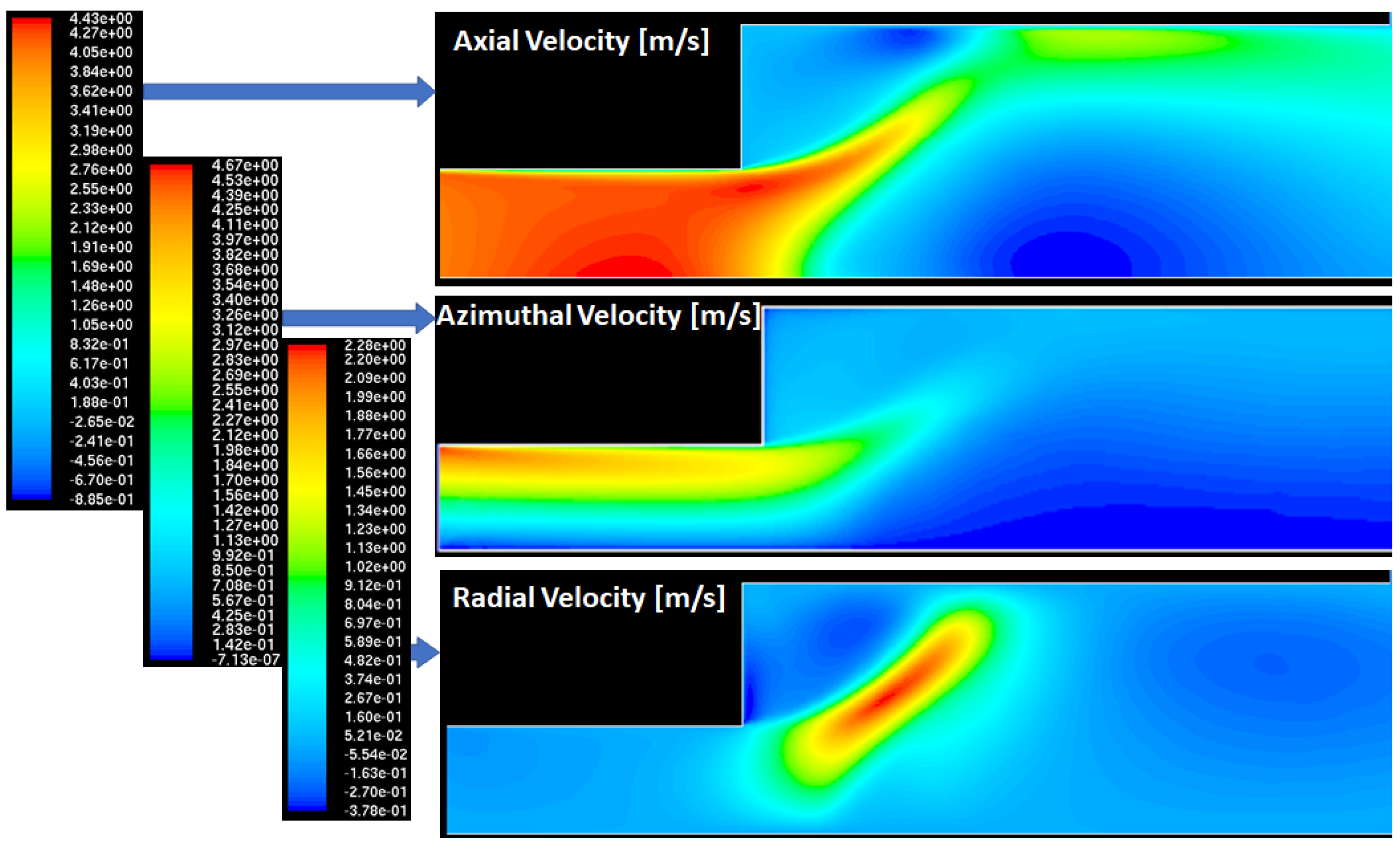
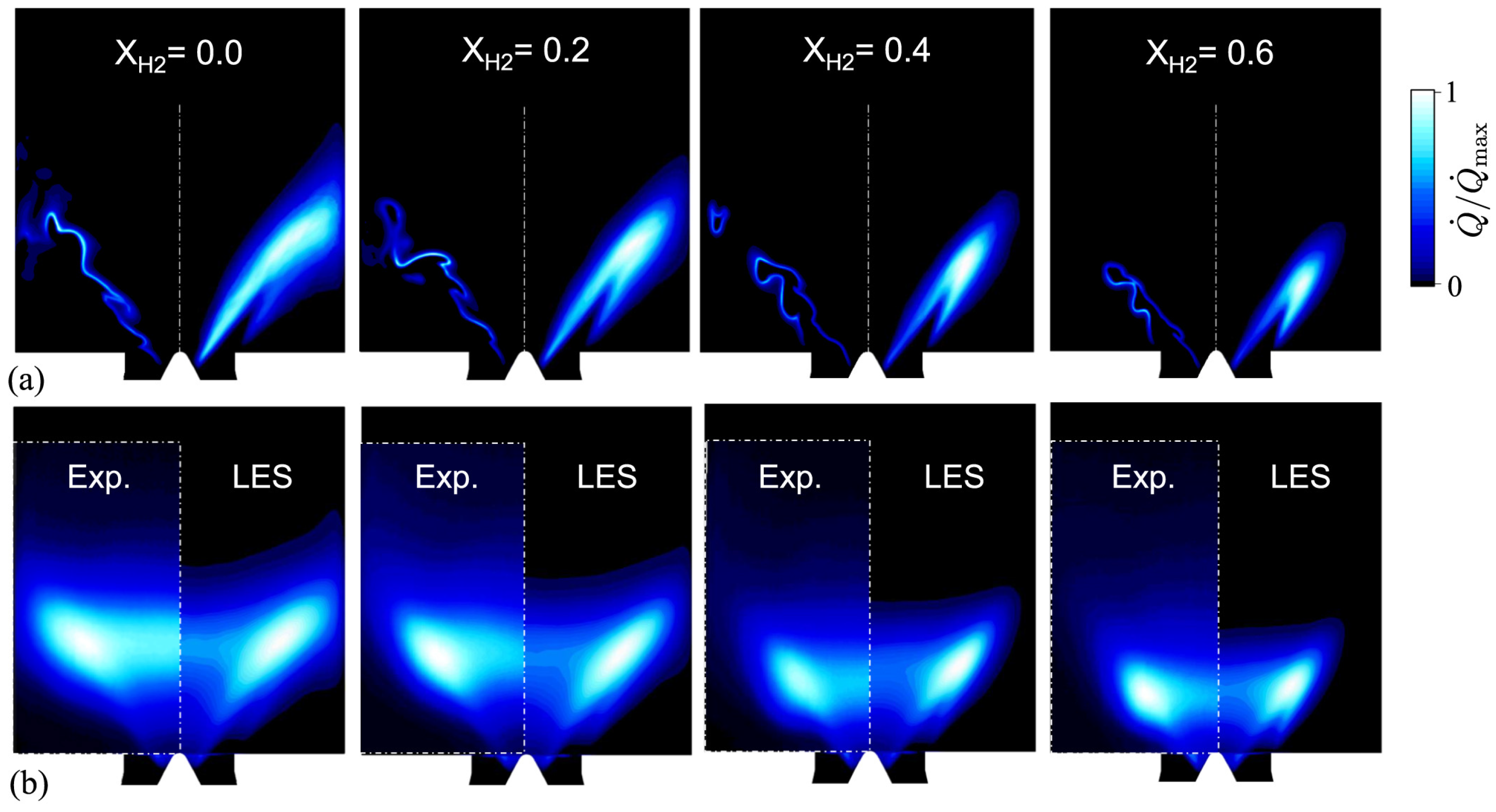

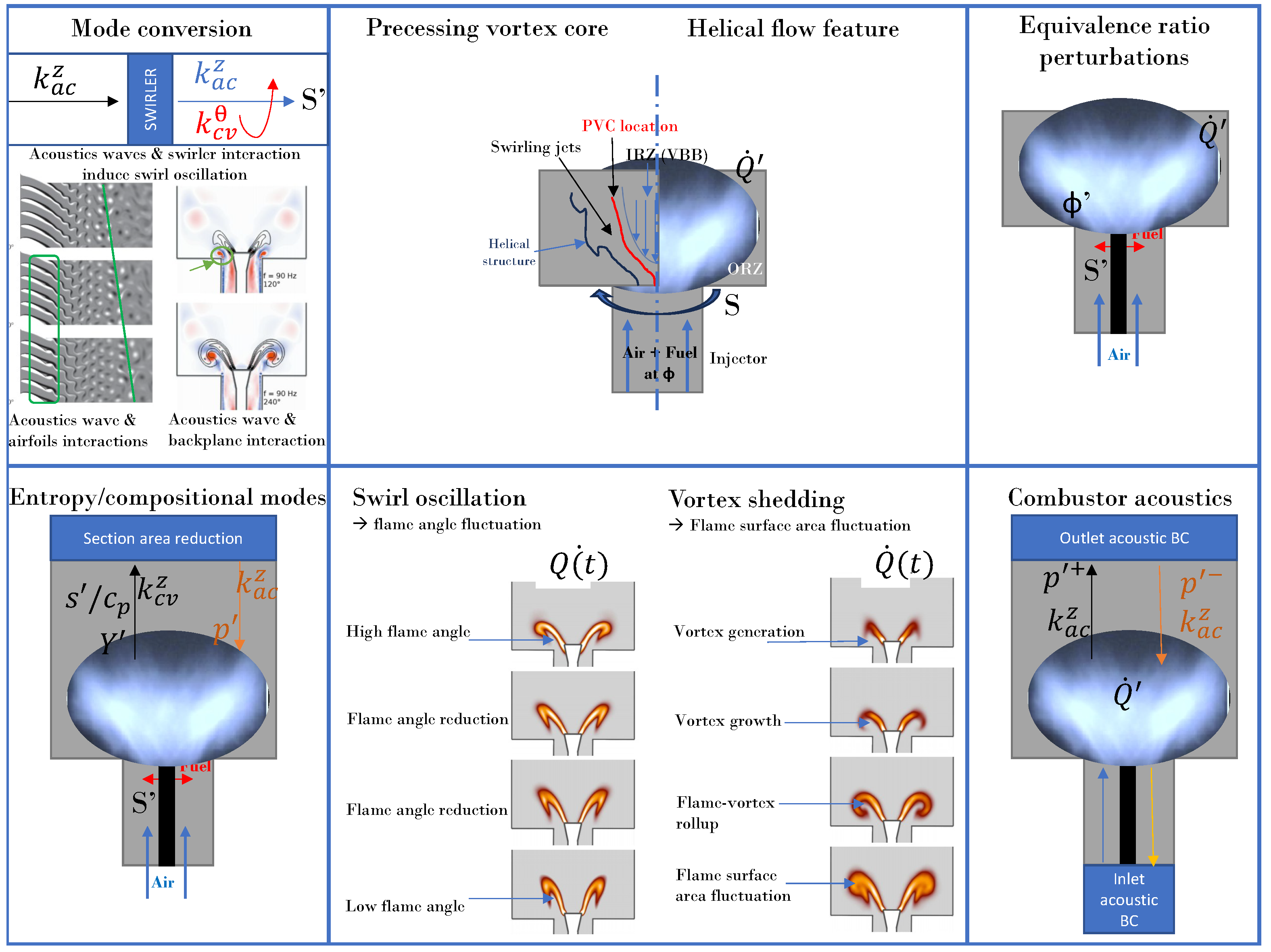
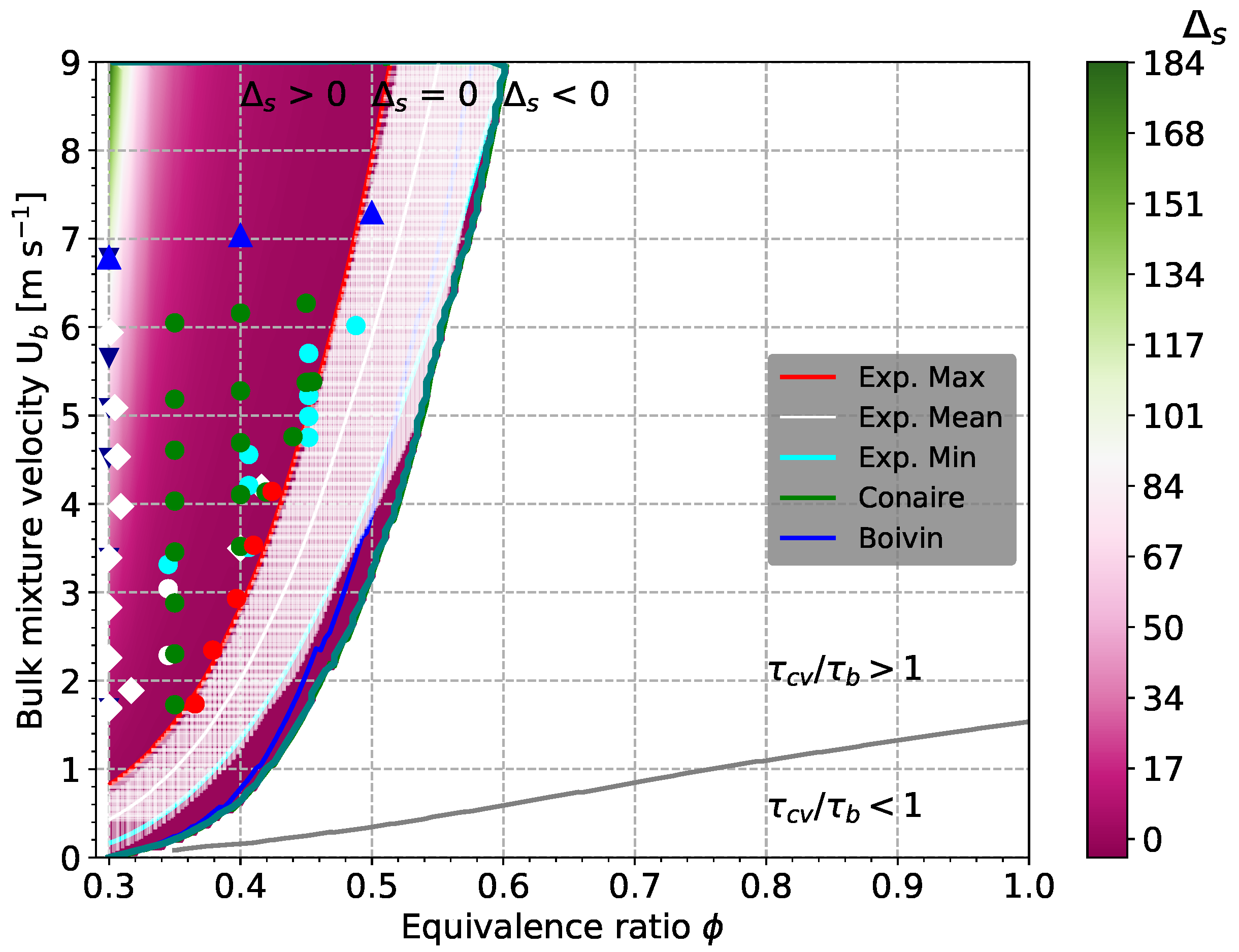
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Palies, P. Flow and Flame Mechanisms for Swirl-Stabilized Combustors. Aerospace 2025, 12, 430. https://doi.org/10.3390/aerospace12050430
Palies P. Flow and Flame Mechanisms for Swirl-Stabilized Combustors. Aerospace. 2025; 12(5):430. https://doi.org/10.3390/aerospace12050430
Chicago/Turabian StylePalies, Paul. 2025. "Flow and Flame Mechanisms for Swirl-Stabilized Combustors" Aerospace 12, no. 5: 430. https://doi.org/10.3390/aerospace12050430
APA StylePalies, P. (2025). Flow and Flame Mechanisms for Swirl-Stabilized Combustors. Aerospace, 12(5), 430. https://doi.org/10.3390/aerospace12050430






