Permeation Investigation of Carbon Fibre Reinforced Polymer Material for LH2 Storage Thermally Shocked and Mechanically Cycled at Cryogenic Temperature

Abstract

To achieve the sustainability goals set for the European aviation sector, hydrogen-powered solutions are currently being investigated. Storage solutions are of particular interest, with liquid hydrogen tanks posing numerous challenges with regard to the structural integrity of materials at cryogenic temperatures, as well as safety issues because of the high flammability of hydrogen. In this context and in the scope of the Horizon 2020 Clean Aviation Joint Undertaking (CAJU) project H2ELIOS, the gas permeability behavior of prepreg tape carbon fibre reinforced polymer (CFRP) material was studied. Investigations were performed after thermal shock to 20 K (liquid hydrogen immersion) as well as after a uniaxial stress application at 77 K to identify the shift from Fickian behavior after diverse aging conditions. Helium gas permeation was tested at room temperature (RT), and its representativeness to hydrogen permeation in a range of temperatures was considered in the study. The material’s permeation behavior was compared to ideal Fickian diffusion as a means of identifying related permeation barrier function degradation. Finally, it was possible to identify Fickian, near-Fickian, and non-Fickian behaviors and correlate them with the material’s preconditioning.

1. Introduction

In 2022, it was reported that the aviation sector in Europe was directly responsible for about 4% of total European Union Green House Gas emissions in that year and about 14% of total European transport emissions. Also, the International Civil Aviation Organization (ICAO) forecasted that by 2050, emissions could triple, which was based on air travel demand forecasts [1,2]. To counteract this trend, sustainable aviation fuels, improvements in airframes and engines, operational optimizations, and new propulsion technologies are being developed, and they play a key role in helping to reach the sustainability goals set. One of the promising technologies currently being investigated are fuel cells powered by hydrogen stored in liquid form. The choice of storing hydrogen in liquid form comes from the possible benefit in terms of the gravimetric and volumetric energy densities that are achieved when cooling down this fuel to 20 K, its liquefaction temperature. The volumetric energy density of LH2 is about two times higher than hydrogen stored at 700 bar, and the gravimetric energy density is higher than any other hydrocarbon fuel solution [3]. However, the downside of storing hydrogen in liquid phase comes with the need for the insulation materials and technologies for maintaining the liquid hydrogen (LH2) at 20 K and avoiding its “boil-off”, gas evaporation induced from heat ingress, which causes unwanted losses. The upside is that, even with the added weight penalty coming from the additional system, the gravimetric energy density remains the highest among other fuel options. Therefore, the European Union has been supporting research activities and projects such as H2ELIOS [4], which funds this research work and that specifically aim to investigate the feasibility and manufacturing of a polymer matrix composite (PMC) tank and conformal LH2 storage tank. Composite storage solutions are in the interest of the aviation sector because of the low weight attainable for the structure itself, possibly reaching a gravimetric index (GI, the ratio between the weight of the stored fuel and the weight of the storage system plus fuel) higher than 35%.

To satisfy the stringent requirements of this application, great attention must be placed on the choice and selection of the structural materials, which need to have properties such as low micro-cracking, low permeability, suitable coefficient of thermal expansion (CTE), and sufficient strength and toughness over the whole range of operating temperatures of the storage solution. The low permeation requirement is of particular importance because hydrogen gas possesses a very wide flammability range when in an air mixture (4–75% [5]). Therefore, safety concerns regarding gaseous hydrogen leaking from tank walls and mixing with air volumes nearby must be considered thoroughly. Epoxy matrices with carbon fiber reinforcements are promising materials and seem to posses the required properties for an LH2 storage solution [6]. When evaluating the suitability of CFRPs as materials for LH2 storage solutions, it is essential to investigate their permeation under conditions representative of the final envisioned application. However, cryogenic permeation testing is well known to be cost intensive; it is, therefore, avoided when possible and substituted by property extrapolation and modeling. In the literature, the term permeability has been used to refer to leak rate, permeability as defined by Fick’s law, and permeability as defined by Darcy’s law [7,8,9,10,11,12,13]. Importantly, it needs to be emphasized that permeability and diffusion calculated through Fick’s laws are only meaningful under the condition that the investigated material is homogeneous or that the diffusion behavior is Fickian, while materials that show non-Fickian diffusion behavior cannot be evaluated using the same criteria. Because of this, it is of necessary importance to verify how closely the diffusion behavior of the tested material resembles the Fickian one.

Scheerer reported results on composites showing a diffusion-like behavior at room temperature, which decreased at 77 K in agreement with Arrhenius law, however, no Fickian investigation was performed. He also reported composites with connected matrix crack networks that showed a pressure-dependent rapid increase in permeability [13]. Condé-Wolter reported a good agreement with Fickian-like diffusion behavior for pristine thermoplastic PA6 carbon fiber-reinforced specimens and contrastingly different, immediate leakage results after 5J impact load [14]. Flanagan reported Fickian diffusion behavior for pristine CF-PEEK specimens as well as a shift to non-Fickian behavior for both damaged specimens and specimens where manufacturing defects were identified [12]. He also found the permeability of pristine specimens to be several order of magnitudes lower at 77 K when compared to 293 K, in agreement with Arrhenius’ law. Liu found that thermoset epoxy-based, carbon fiber-reinforced material prepreg with a fiber volume fraction similar to the one from the P1 material investigated in the current study agreed well with the assumptions of Fickian diffusion theory or leak rate being proportional to pressure and inversely proportional to thickness. A numerical model was built and successfully implemented to predict the leak rate increase following a step-wise pressure increase [15].

Hosseini investigated a semi-crystalline low-melt polyaryletherketone resin with carbon fiber reinforcements (CF/LMPAEK) before and after thermal cycling to liquid nitrogen temperature (293–77–293 K). He found no crack networks developed by micrography and computed tomography investigations. He also reported that no observable change in permeation between pristine and cycled specimens could be found [16]. Choi found that graphite/epoxy laminates, when subject to few cryogenic thermal cycles, showed an increased micro-crack density, and he correlated that with higher permeability readings. He additionally reported the improved resistance to this particular conditioning method of fabric materials [10]. However, Bechel and Gates found that the micro-cracking density increased starting after a couple hundred cycles [7,17]. Rauh investigated gas leakage in carbon composite laminates subject to cryogenic thermal cycling and found that cycled samples showed higher leak rates compared to uncycled specimens. He also found higher resistance to damage in cross-ply specimens compared to unidirectional ones. The variability in leak rate was assumed to be likely due to internal flaws in the laminate [18]. Schulteiss investigated metal-coated and uncoated CFRP materials with the aim of finding a viable liner solution for cryogenic storage and found mostly near-Fickian diffusion behavior results for pristine uncoated CFRP specimens at room temperature [9].

Katsivalis investigated hydrogen’s permeation in thin ply composites and concluded that mechanically pre-loading specimens at room temperature up to 1.4% did not generate micro-cracks or damage that would accelerate the diffusion mechanism. He also demonstrated that manufacturing defects such as voids and pinholes lead to unsuccessful permeation measurements, highlighting the importance of implementing high-quality manufacturing and developing methods able to detect the effect of defects in permeation [19]. Stokes investigated the hydrogen permeability of polymer matrix graphite fiber composite panels manufactured from tape raw material after tetra-axial cyclic loading at liquid hydrogen temperatures and reported significant, widespread micro-cracking onset at 2000 to 3000 $\mu ϵ$ (micro-strains). He reported a contiguous through-thickness crack system beginning to form between 3500 and 4000 micro-strain (0.45% strain) at room temperature under uniform tetra-axial in-plane strain and he also reported significantly higher permeation from cryogenic temperature leak testing [20]. Gates also investigated hydrogen through thickness permeation simultaneous to strain application and was able to correlate the damage state and leak rate value, demonstrating leak rate variability on the IM7/977-2 material from as low as 1700 $\mu ϵ$ (0.17% strain) [17]. Hamori investigated the effectiveness of thin plies’ inclusion in laminates as permeation barrier and found that leak values were lower and initiated at higher mechanical strains when thin plies were included in the layup [21]. Grenoble studied the permeability ofIM-7/977-2 composite material after cyclic room temperature strain application and found increased permeability at cryogenic-temperature testing when compared to room-temperature testing [22]. Saha highlighted inconsistencies in Fickian behavior assessment for composite materials, some being reported following Fickian behavior and some non-Fickian. He also recommended verifying the diffusion behavior when investigating permeation testing as well as using permeance instead of permeability (as determined by standard ASTM D1434) [23] when investigating the diffusion performance of materials subject to cryogenic conditions [24].

The present study acknowledges the inconsistencies found in the literature on the topic of thermoset CFRP Fickian diffusion behavior and identifies the need for deeper insights into the Fickian behavior of CFRP epoxy-based materials, currently lacking in the available literature. Additionally, this research work aimed to complement Flanagan’s work by investigating the diffusion behavior of thermoset material systems in more depth than explored by previous studies. It aimed to achieve this objective by investigating the CFRPs homogeneous diffusion behavior as well as investigating the effect of different types of preconditioning by analyzing the difference between experimental and theoretical diffusion curves.

2. Materials

The combination of stresses forecasted in the H2ELIOS storage solution concept makes selecting structural materials for manufacturing challenging. Because of the specific insulation concept, materials are faced with considerable thermal stresses (about 200 °C differential, from 293 K to 80 K and back to 293 K in the worst-case scenario) as well as structural stresses from the inherent storage tank loads. The material considered in this study was selected after careful evaluation of thirteen materials from three different suppliers. The best candidate material was identified as the one able to reach the stringent requirements of an H2ELIOS storage design, performing with the lowest amount of micro-cracking behavior. Carbon fiber-reinforced plastic and epoxy-based tape prepreg material were considered for the manufacturing of most parts of the tank. Its layup, considered as a specimen configuration specifically oriented for coupon testing in initial H2ELIOS stages and not representing any specific LH2 storage demonstrator area, as well as the fiber fraction, is reported in

Table 1. Layup and fiber content of laminate plates from the P1, and P2 materials.

Material ID	Layup	Diameter [mm]	Thickness [mm]	Fiber Content [%]
P1	${\left[45,-45,90,0,90,0\right]}_{s-1}$	50 ± 0.5	2.09 ± 0.14	64.5
P2	-	50 ± 0.5	4 ± 0.10	20

. Tape prepreg is referred to in this paper as P1. Laminate plates of the P1 material were manufactured by the automated tape laying method. At least 3 specimens per testing condition were considered so as to establish a statistical significance for the results. Additionally, a thermoplastic matrix with a short fiber reinforcement material system (hereinafter named P2) was also considered in this study, which was manufactured by 3D printing and included short carbon fiber reinforcements. This material was included in the study to verify the Fickian behavior of a more homogeneous material system. The thickness of the specimens was measured over at least 5 different locations on the specimen surface with a resolution of 10⁻⁵ m. The average thickness was used for the calculation of the permeability of the material. All the available material details are provided in Table 1.

Samples Preconditioning

Previous works have indicated that thermal stresses alone provide only partial insights into material suitability in selecting an appropriate material for cryogenic pressurized hydrogen applications. Mechanical stresses, combined with the application’s normal-operation environmental parameters, are crucial in inducing significant stress and micro-cracks in the material [17,22,25,26]. For this reason, thermal stresses and mechanical stresses were considered in this study as methods for preconditioning the materials to evaluate their permeation behavior after preconditioning as representative as possible to what is required for similar works. Combinations of the two stresses were applied to the different materials prior to testing, and a summary of this is reported in

Table 2. Preconditioning considered for each material system. Capital “N” stands for immersions in liquid nitrogen; capital “H” stands for immersions in liquid hydrogen.

Material System	Preconditioning ID	Cryogenic Cycles	Mechanical Cycles to 4500 $\mathit{\mu }\mathit{\epsilon }$ @77 K
P1	N0-M0	0	0
P1	N1-M0	1	0
P1	N5-M0	5	0
P1	H0-M50	0	50
P1	H5-M50	5	50

. A total of 5 immersions in liquid hydrogen (liquid nitrogen was implemented as a substitute at the early stages of the study because of the challenge in securing LH2 availability) were performed based on the works of Choi et al. [10] who reported a significant permeation increase after the first thermal cycle and subsequent stabilization shortly after, as well as the work of Timmermann et al. [26] who reported a substantial increase in crack density occurring after the first thermal cycle and subsequent plateauing shortly after and, therefore, concluded that the probability and severity of micro-cracking could be determined just after a few cycles. A total of 50 cycles of mechanical strain applied at 77 K up to 0.45% strain (4500 $\mu \epsilon$, micro-strain) were performed. The number of immersions and mechanical cycles were derived from H2ELIOS proprietary studies that considered this amount sufficient for the scope of screening cryogenic material properties, taking into account previous proprietary validation tests of such materials as well. H2ELIOS proprietary studies have also considered 77 K to be a representative temperature to condition the material with mechanical strains given its environmental envelope. Samples considered for preconditioning and leak testing were 5 cm in diameter. Samples that required mechanical stress preconditioning were first cut in rectangles for uniaxial cryogenic stress application; then, 5 cm diameter discs were cut out of the stressed rectangles and tested at a later stage. A representative sketch of such coupon manufacturing is presented in Figure 1.

The liquid hydrogen (LH2) immersions were performed in a pressurized LH2 cryostat, ensuring adequate time for both the cooling and heating of the samples. The duration required for this procedure was determined based on the existing literature. Numerous research articles and laboratory reports have demonstrated that 3 min is sufficient to cool specimens of approximately 2 mm thickness from 293 K to 77 K [10,12,16,18]. In this study, once the measured temperature reached 20 K (the temperature of liquid hydrogen in this study), a hold time of 20 min was selected to ensure proper cooling. Figure 2 presents the temperature readings from thermocouples inside the cryostat.

The mechanical stress preconditioning was performed by an experienced testing entity, a partner of the H2ELIOS project. The coupons were subjected to a 0.45% strain at 77 K according to standard ISO 527/2/1A/5 [27] with a standard tensile testing machine. The loads to reach the necessary strain were calibrated before the conditioning procedure. The strain application was then repeated 50 times.

3. Methods

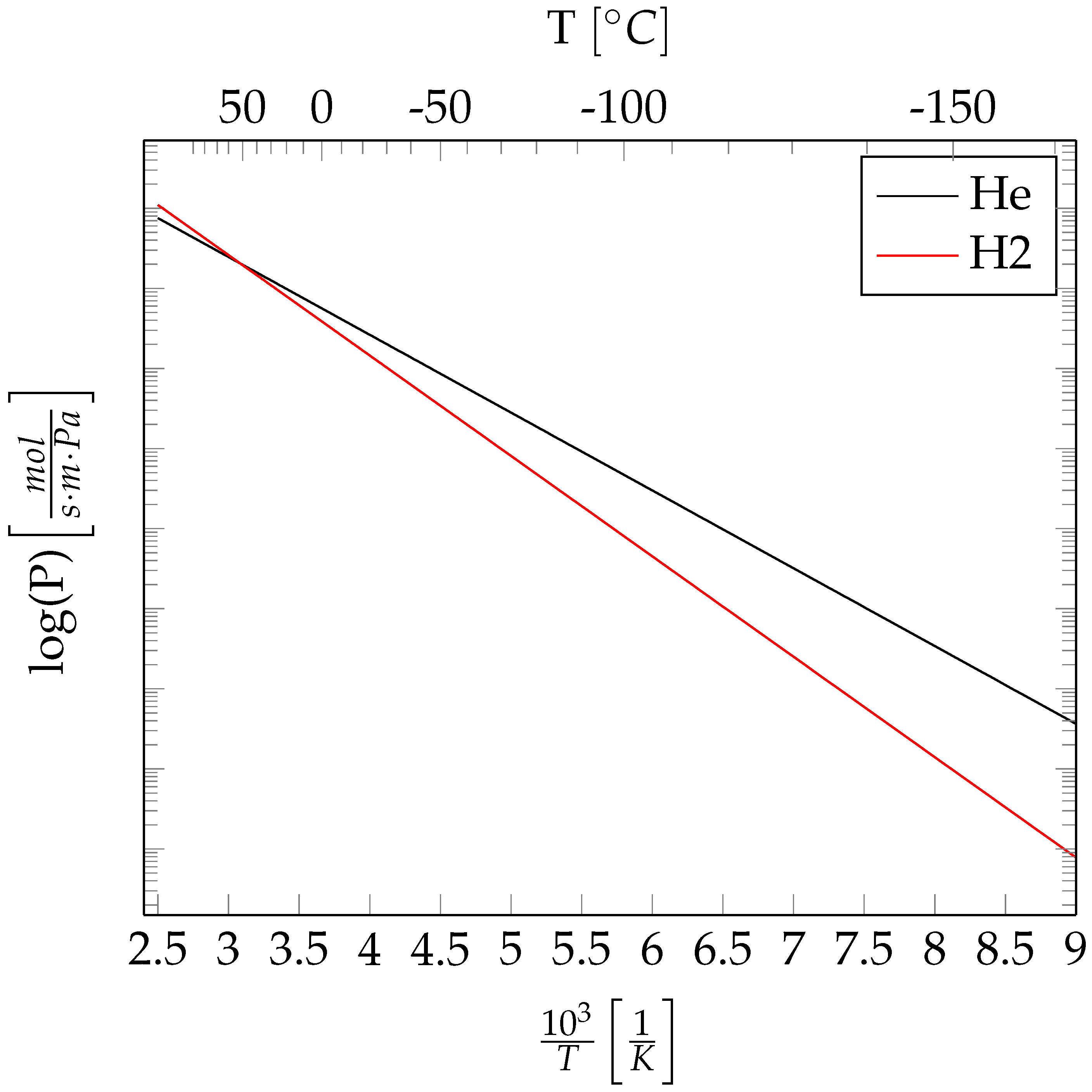
3.1. Helium and Hydrogen

The tracer gas considered for leak testing in this study was helium gas instead of hydrogen gas, and there are several reasons for this substitution, which are also found in similar experimental works: safety concerns due to hydrogen’s wide flammability range in air, instruments’ sensitivity to the specific tracer gas (hydrogen sensitivity being about 3 to 4 decades worse sensitivity), and the proven similarity in diffusion behaviors [9,12,18,25]. The understanding of hydrogen and helium diffusion in plastic materials as similar and comparable is unilaterally reported in the literature. In fact, Humpenöder reported a direct comparison of permeation vs. temperature between helium and hydrogen, which was tested on CFRP materials from room temperature to cryogenic temperatures [8]. The findings were both a linear relation between the logarithm of permeation and temperature, and a steeper slope of this relation for hydrogen with respect to helium. This means that when testing diffusion properties of carbon fiber-reinforced thermosets, adopting helium instead of hydrogen provides a conservative estimation of the permeation coefficient. The same was found by Schulteiß, where the experimental results showed a steeper slope for hydrogen with respect to helium on CFRP materials [9]. A graphical sketch of these results is given in Figure 3.

There are two principal explanations for this: the first is that the kinetic diameter of a hydrogen gas molecule is bigger than that of helium (289 pm and 260 pm, respectively [28]), and the second is that the permeation of gases in polymers, being a product of diffusivity D and solubility S, is mainly dependent on the gas size. Therefore, since diffusivity increases when the gas size decreases with exponential dependency [8], helium is able to permeate more easily within the free volume of the polymeric chains. The Arrhenius equation is used to describe the temperature dependency of the diffusion coefficient and the solubility of the gas in the material. The permeation coefficient is then calculated as the product of the diffusion coefficient and the solubility of the gas in the material.

$$P=D·S$$

(1)

The following are the equations that define diffusivity, solubility, and permeability, as well as their relation [29]:

$$D=D_{0}exp\left(-{\displaystyle \frac{E_D}{RT}}\right)$$

(2)

$$S=S_{0}exp\left(-{\displaystyle \frac{E_S}{RT}}\right)$$

(3)

$$E_P=E_D+E_S$$

(4)

$$P=D_0·S_{0}exp\left(-{\displaystyle \frac{E_D·E_S}{RT}}\right)=P_{0}exp\left(-{\displaystyle \frac{E_P}{RT}}\right)$$

(5)

where $E_D$ and $E_S$ are the activation energies for both diffusion and solution processes, respectively. It has also been proven that the solubility of helium and hydrogen is generally stable with respect to temperature variations [30]; $E_D$ is found to be always positive; therefore, the diffusion coefficient always increases with temperature. From this, permeation can be expected to increase with increasing temperatures and conversely decrease with decreasing temperatures. Using available data in the literature, it is possible to prove with simulations that helium provides a conservative, valid estimation of hydrogen permeation, and that is owing to both the higher hydrogen permeation activation energy as well as hydrogen’s higher pre-exponential factor of the Arrhenius-type equation [8,30].

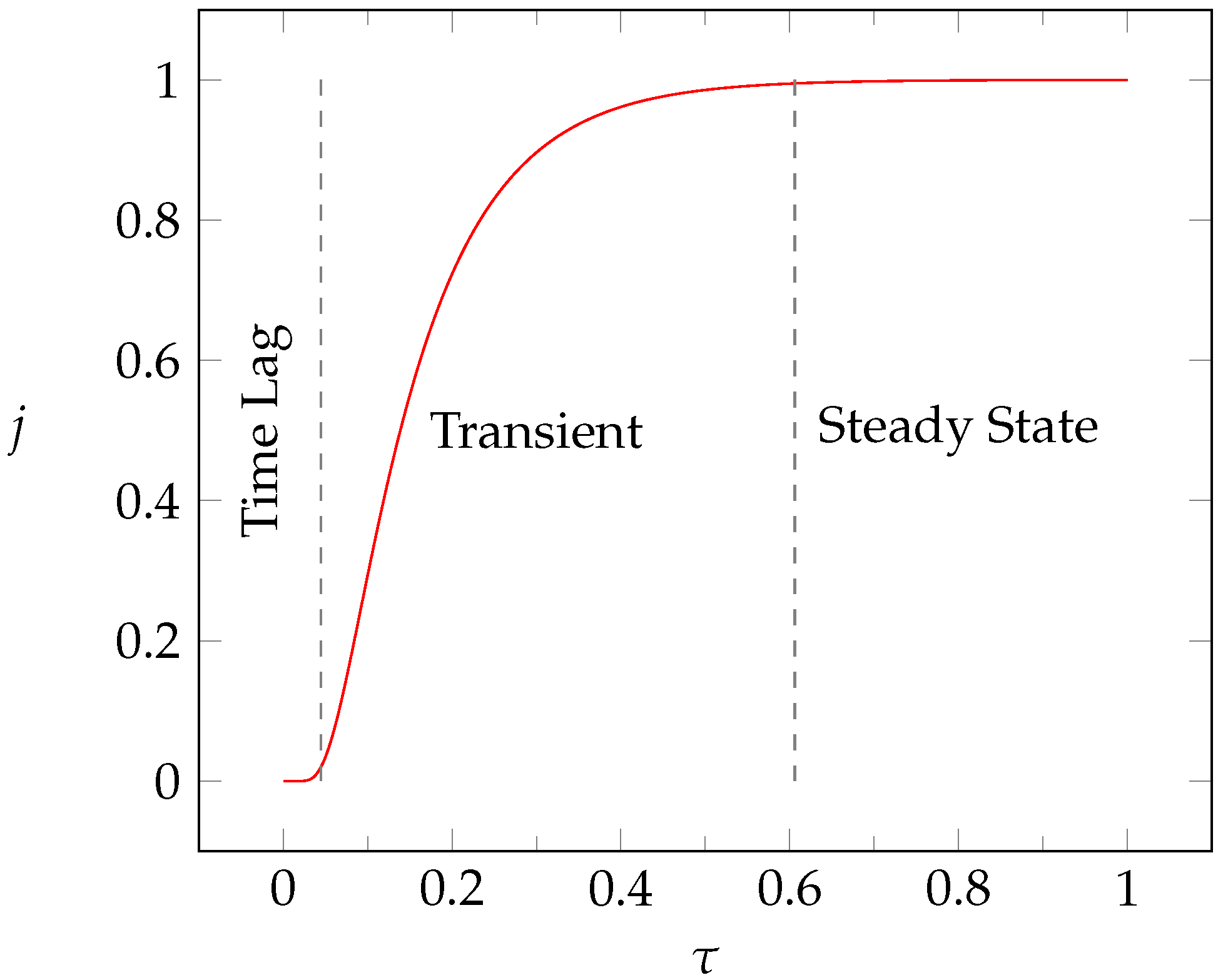
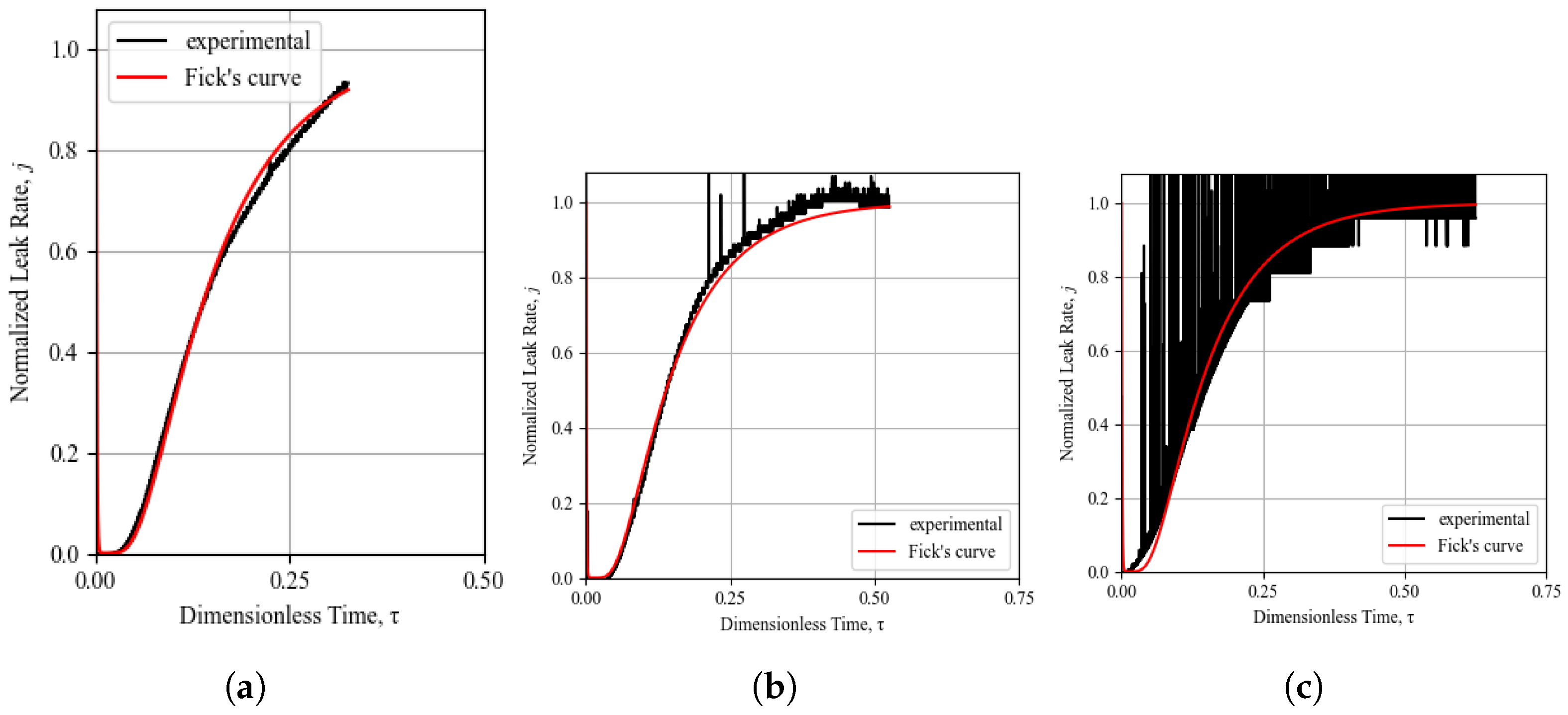
3.2. Theoretical Fickian Diffusion

Diffusion in homogeneous materials is a phenomenon governed by Fick’s laws of diffusion, namely

$$J=-D\nabla C$$

(6)

Fick’s first law of diffusion, where J is the diffused molecule’s flux, D is the diffusion coefficient, and $\nabla C$ is the concentration, states that the diffusion flux is proportional to the concentration gradient. And

$${\displaystyle \frac{\partial C}{\partial t}}=\nabla ·(D\nabla C)$$

(7)

Fick’s second law of diffusion, where ${\displaystyle \frac{\partial C}{\partial t}}$ is the time derivative of the concentration and D is the diffusion coefficient that might be dependent on the spatial coordinate system, describes the transient state of the diffusion phenomenon. It is important to consider the steady state for this equation, which is represented by the condition ${\displaystyle \frac{\partial C}{\partial t}}=0$. When substituting this condition in Equation (7), the equation simplifies to

$$\nabla ·(D\nabla C)=0$$

(8)

At this point, considering the diffusion coefficient independently of spatial coordinates, the equation can be simplified to

$$D{\nabla }^{2}C=0$$

(9)

If at this point the equation is integrated for the steady state, the mono-dimensional steady state flux can be calculated.

$$J\left(x\right)=J_{SS}=-D{\displaystyle \frac{\Delta C}{\Delta x}}$$

(10)

These equations can be solved mono-dimensionally under the assumption of a homogeneous material and the permeation area being much greater than the permeation thickness (if l is the thickness and A is the area, this relationship should stand: $l<<A$). In this case, Fick’s equations become

$$J\left(x\right)=-D{\displaystyle \frac{\partial C}{\partial x}}$$

(11)

$${\displaystyle \frac{\partial C}{\partial t}}={\displaystyle \frac{D{\partial }^{2}C}{\partial x^2}}$$

(12)

And under the following assumptions

• The concentration at the feed side $(x=0)$ is referenced as $C_f$;
• The concentration at the permeate side $(x=l)$ is referenced as $C_p$;
• At $t=0$, the concentration $C_f^0$ is applied as a constant: $C_f(t\ge 0)=C_f^0$;
• For every t, $C_p\ll C_f$ and $C_p\approx 0$.

Crank [31] calculated the flux at the permeate side to be

$$J\left(t\right)={\displaystyle \frac{D{C}_f^0}{L}}\left[1+2\sum _{n=1}^{\infty }{(-1)}^{n}exp{\displaystyle \frac{-D{n}^2{\pi }^2}{l^2}}t\right]$$

(13)

This equation is valid for the transient state of the diffusion phenomenon. In the steady state, the flux is calculated as

$$J_{SS}=\underset{t\to \infty }{lim}J\left(t\right)={\displaystyle \frac{D{C}_f^0}{L}}$$

(14)

To be able to compare the experimental results to the theoretical Fick’s curve, the dimensionless form of this equation is required. This is calculated by introducing the normalized flux

$$j={\displaystyle \frac{J}{J_{SS}}}$$

(15)

and the dimensionless time

$$\tau ={\displaystyle \frac{tD}{l^2}}$$

(16)

With these definitions, the normalized flux can be calculated from Equation (13) as

$$j\left(\tau \right)=1+2\sum _{n=1}^{\infty }{(-1)}^{n}exp{\displaystyle \frac{-n^2{\pi }^2}{\tau }}$$

(17)

which is presented in Figure 4.

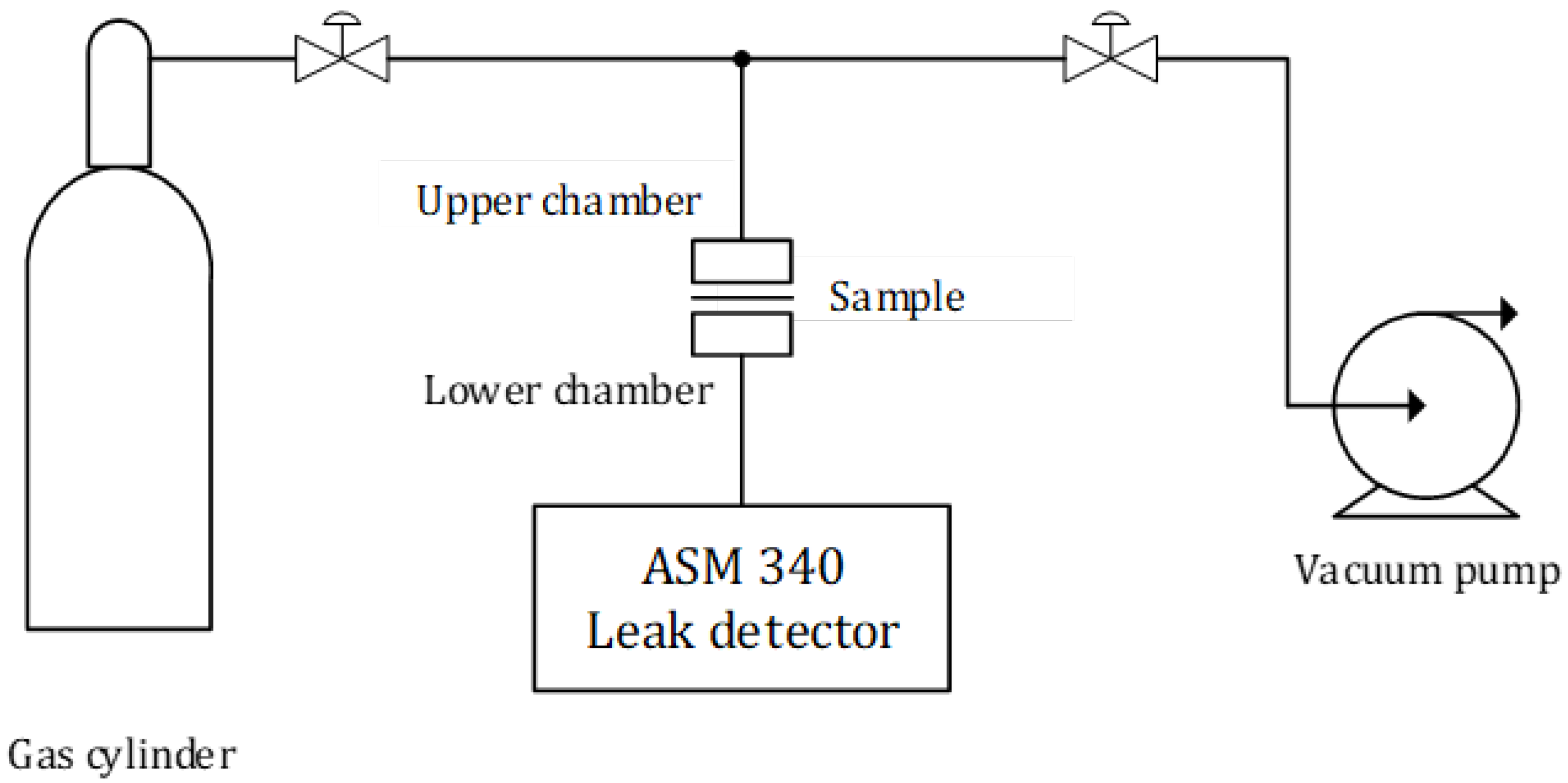
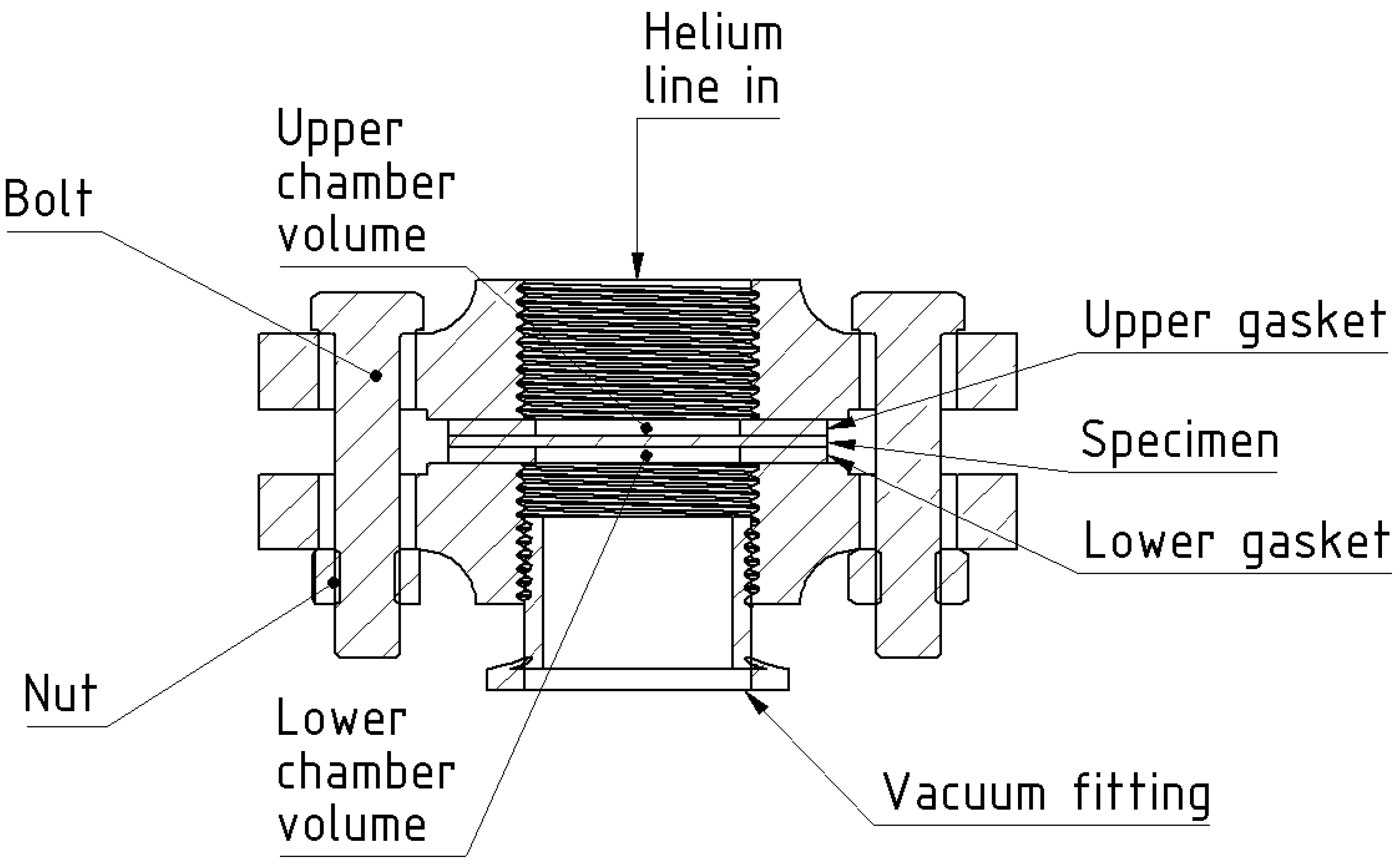
3.3. Test Setup

The test apparatus is schematically represented in Figure 5, where the principal components of the system are depicted. A gas cylinder is connected to the sample holder assembly (Figure 6) where the CFRP specimen is secured with fluoro-polymer gaskets in between stainless steel flanges. The same section of instrumentation tubing is also connected to an evacuation system that allows for performing several evacuation cycles. These evacuation cycles ensure as little air molecules as possible are left within the tubing assembly prior to tracer gas pressure application. With the evacuation cycles, a near-100% concentration of the tracer gas is ensured inside the upper chamber of the sample holder at the start of the testing procedure. Once the tracer gas permeates through the CFRP specimen, it is detected in the evacuated bottom chamber by the ASM340 Leak Detector instrument sourced from Pfeiffer-Vacuum’s Slovenian redistributor, connected to it. The instrument records the rate at which tracer gas molecules reach its measuring chamber through a mass spectrometer inner cell, and it outputs a leak rate value in ${\displaystyle \frac{mbar·L}{s}}$ units.

3.4. Permeation Testing Methodology

The test procedure can be outlined as follows:

• Assemble gaskets and the specimen concentrically in the sample holder.
• Tighten the sample holder assembly and connect the upper and lower chambers to the respective instrumentation tubing.
• Run preliminary checks. Apply vacuum to both the upper and lower chambers and make sure the required pressure is reached (in this case, selected at $4\times {10}^{-2}$ mbar), so as to make sure that all connections are sufficiently vacuum-tight. Then, run pressure tightness checks in the upper chamber by increasing the pressure within the tubing, closing necessary valves and verifying that the pressure inside the tubing assembly does not drop rapidly. This ensures that no gross leaks are present.
• Evacuate the upper chamber tubing assembly at least 5 times if all previous preliminary checks are successful.
• Start pulling vacuum and testing on the lower chamber with ASM340.
• Increase the tracer gas pressure in the upper chamber assembly to the desired amount, making sure that the same pressure will be delivered for the whole duration of the test.
• Start recording on ASM340.

This testing procedure ensures that a reliable leak rate value for the specimen tested is measured. The leak rate value can then be used to directly compare different specimens or to calculate the permeation coefficient for each of them. The specific ASTM D1434 standard for the gas permeation of plastic materials was followed in terms of general principles, definitions, and recommendations [23]. However, the volumetric and manometric testing procedures described in the document were not suitable for a test setup that included a mass spectrometer such as the Pfeiffer ASM340. In the current investigation, the permeation coefficient can be calculated directly from the output value of the Pfeiffer instrument (in ${\displaystyle \frac{mbar·l}{s}}$) through the following actions:

• Convert the leak rate value from $L{R}_{mbar}={\displaystyle \frac{mbar·l}{s}}$ to $L{R}_{Pa}={\displaystyle \frac{Pa·m^3}{s}}$ by dividing if by a factor of 10 [32].
• Convert in $m^2$ the surface area (A) of the specimen exposed to the tracer gas.
• Convert in K the temperature (T) at which the test was carried out.
• Calculate the thickness of the specimen (l) in m.
• Calculate the gas transmission rate (GTR) with the following equation:

  $$GTR={\displaystyle \frac{L{R}_{Pa}}{ART}}=\left[{\displaystyle \frac{Pa·m^3}{s·m^2·\frac{m^3·Pa}{Kmol}K}}\right]=\left[{\displaystyle \frac{mol}{s·m^2}}\right]$$

  (18)

  where R is the ideal gas constant in ${\displaystyle \frac{m^3·Pa}{Kmol}}$.
• Calculate the difference in partial pressure of the permeating gas between the upper and lower chambers ($\mathrm{\Delta }p$ in $Pa$).
• Calculate permeance ($Pr$) by dividing the GTR by ($\mathrm{\Delta }p$).

  $$Pr={\displaystyle \frac{GTR}{\mathrm{\Delta }P}}=\left[{\displaystyle \frac{mol}{s·m^2·Pa}}\right]$$

  (19)
• Calculate the permeation coefficient by multiplying the permeance by the thickness of the specimen.

  $$P=Pr·l=\left[{\displaystyle \frac{mol}{s·m^2·Pa}}\right]·m=\left[{\displaystyle \frac{mol}{s·m·Pa}}\right]$$

  (20)

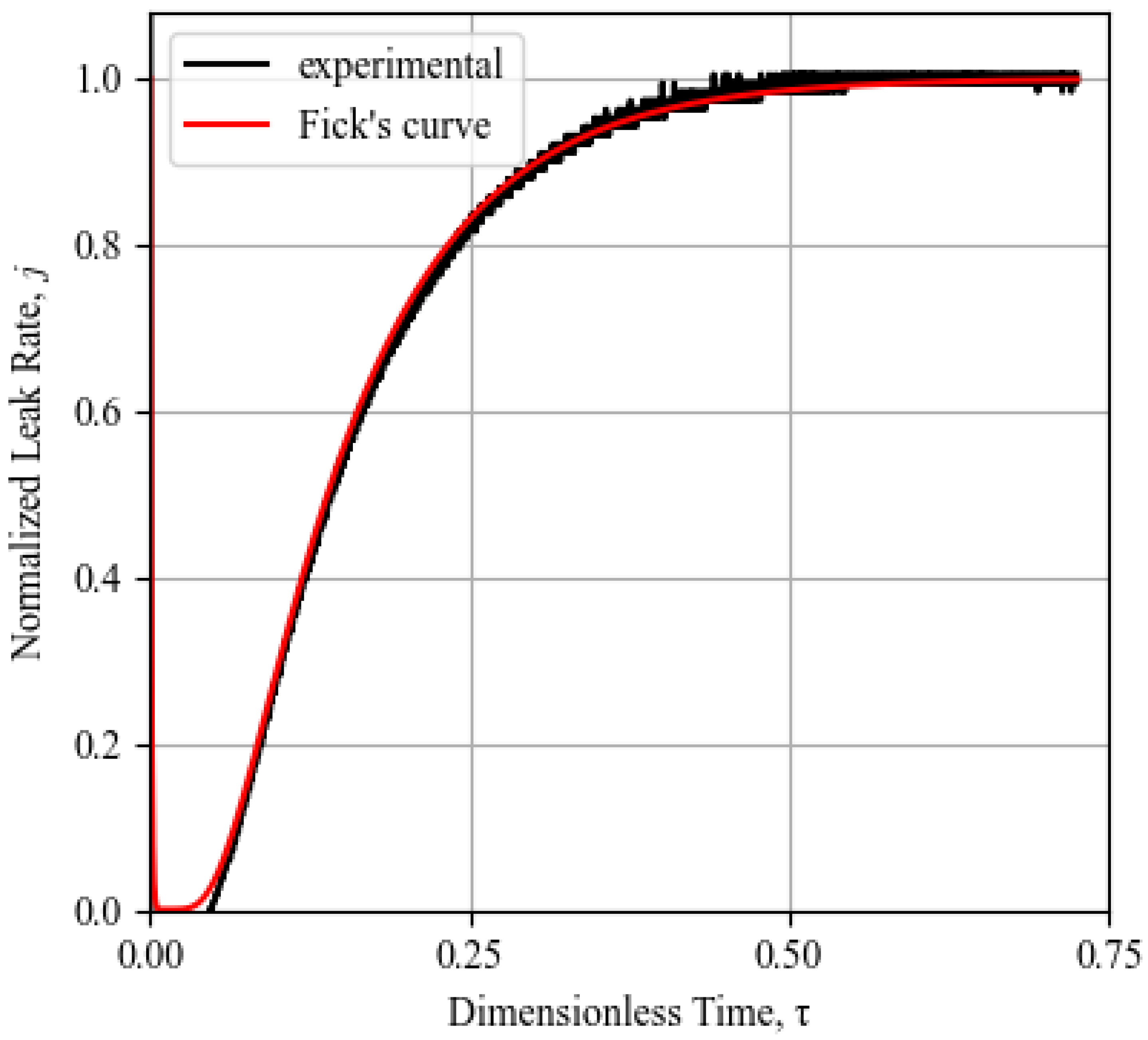
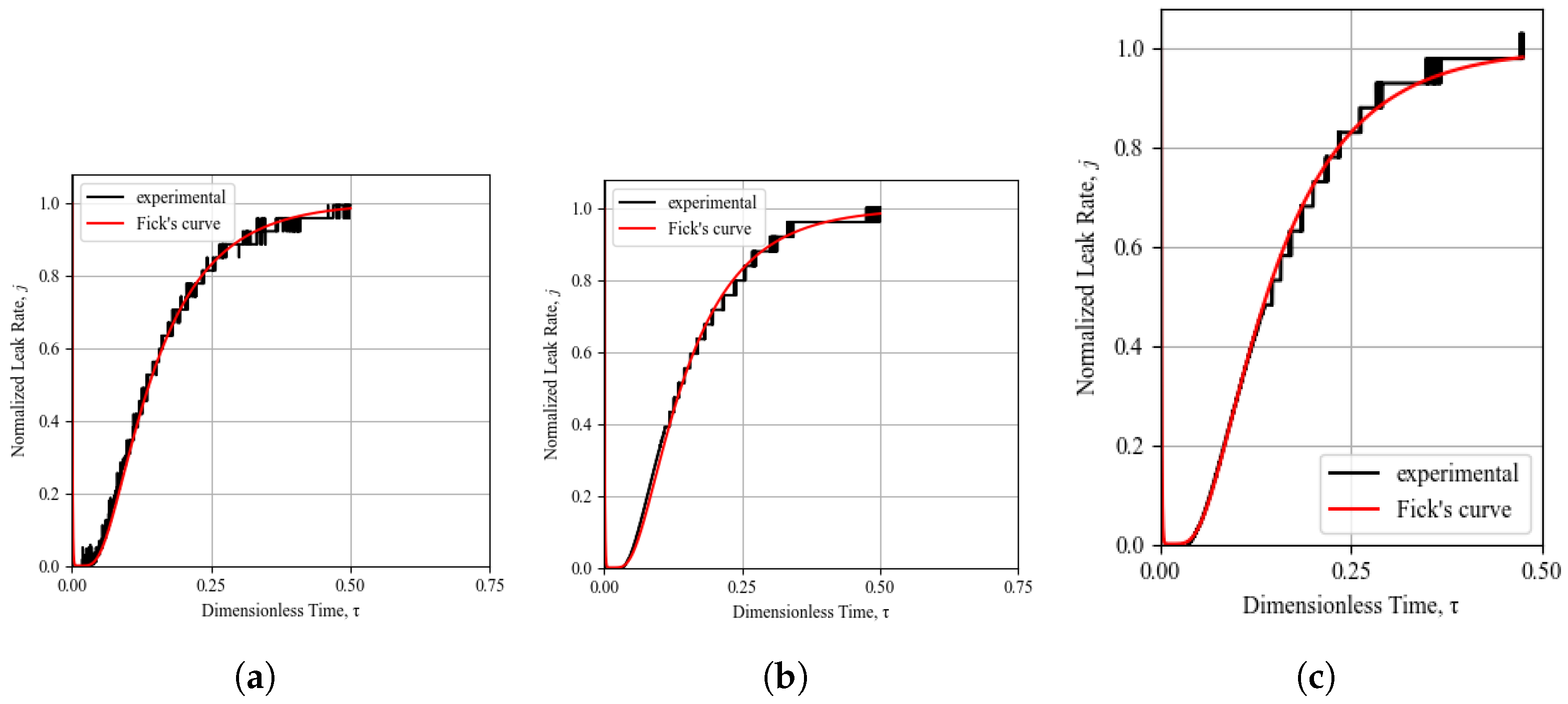
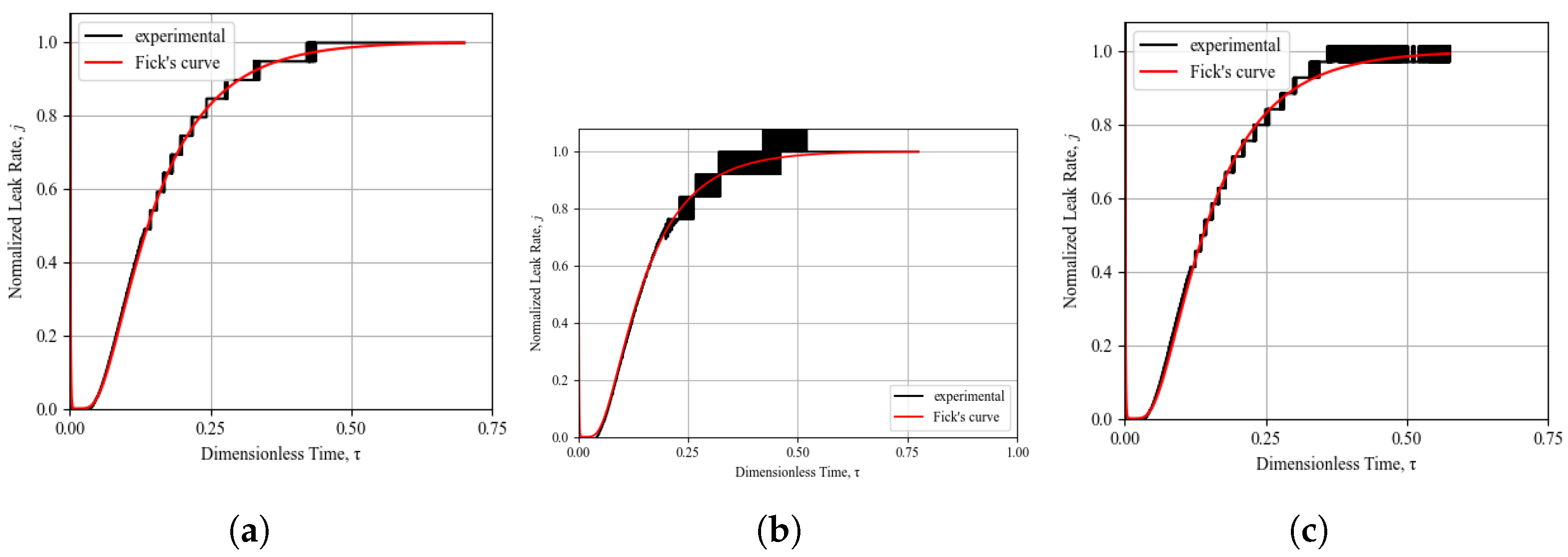
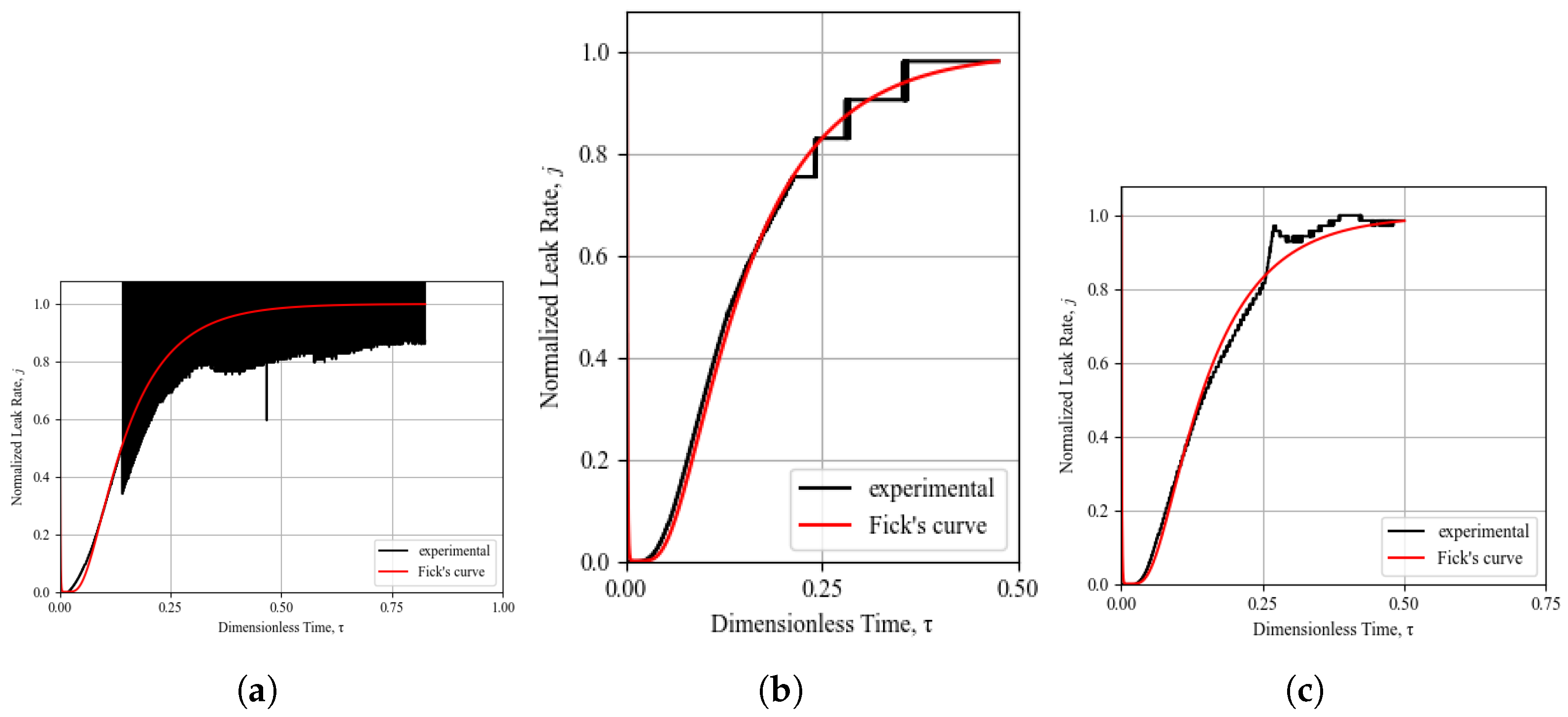
4. Results

In this study, the leak rate of every sample was measured and its Fickian behavior was identified by fitting Fick’s normalized diffusion curve (calculated by as outlined in Section 3.2, Equation (17)) to normalized experimental result curves. A further quantification of the offset between theoretical Fickian behavior and the experimental result was investigated by means of the coefficient of determination $R^2$. However, because of the frequent inconsistencies found in the recorded experimental leak tests, it was apparent that this coefficient was not easily implemented to compare results, and subjective judgment was always required to integrate the coefficient of determination indication. The subjective judgment of the Fickian curve fitting is based on the observation of good agreement or deviations in the three separate sections of the diffusion curve shown in Figure 4, namely the time lag, transient state, and steady state sections of the curve. It was identified that on multiple occasions, the subjective judgment of the curve fitting led to a Fickian behavior assessment while the calculated $R^2$ coefficient was in disagreement with the subjective conclusion. An example of this phenomenon is demonstrated by the experimental result shown in Figure 7, where the coefficient of determination is calculated to be 0.86 while the subjective assessment of the curve fitting to Fickian theory assigns a perfect Fickian behavior.The most easily detectable disagreement between the experiments and the theoretical diffusion is observed as the lack or reduction in the time lag, an identification method similarly used by [18]. The most common deviation identified in this study was a reduction in the time lag, coupled with a slower rise in the leak rate in the transient section of the curve, which can be verified by an analysis of the curves in Figure 8, Figure 9, Figure 10, Figure 11, Figure 12 and Figure 13.

Therefore, as the outcome of the investigation of the applicability of the coefficient of determination to the Fickian behavior or permeation tests, it is possible to recommend an indicative range of $R^2$ values, listed in

Table 3. $R^2$ ranges recommended for the identification of Fickian permeation behavior.

Behavior	Non-Fickian	Near-Fickian	Fickian
Range	$0.00\le x<0.95$	$0.95\le x\le 0.98$	$0.98<x\le 1$

, which could possibly indicate the respective Fickian behavior. However, subjective and objective evaluations of the curve fitting need to be performed in parallel to achieve a reliable understanding and results.

The curve fitting performed on the P1 material specimens can be seen in Figure 8, Figure 9, Figure 10, Figure 11 and Figure 12 while curve fittings performed on the P2 material specimens can be seen in Figure 13. The tests performed on the thermoplastic P2 material confirmed the findings of [12] who also identified that PEEK, a thermoplastic material in its pristine condition, follows Fickian permeation behavior.

Table 4. Collection of diffusion behaviors for tested specimens, compared to the ideal Fickian diffusion behavior.

Material System	Preconditioning ID	Sample ID	Behavior
P1	N0-M0	S1	Fickian
		S2	Near-Fickian
		S3	Fickian
P1	N1-M0	S1	Fickian
		S2	Fickian
		S3	Fickian
P1	N5-M0	S1	Fickian
		S2	Fickian
		S3	Fickian
P1	H0-M50	S1	Non-Fickian
		S2	Fickian
		S3	Near-Fickian
P1	H5-M50	S1	Near-Fickian
		S2	Near-Fickian
		S3	Non-Fickian
P2	H0-M0	S1	Fickian
		S2	Fickian

presents the summary of the Fickian behavior of every sample tested in this study in relation to its deviation from diffusion theory.

The diffusion coefficient of tested specimens can be calculated with the methodology proposed in Section 3.2 following the algorithm shown in Equation (21) to perform the curve fitting between theoretical and experimental curves:

$${\left[\tau ,j\right]}_{\mathrm{Fick}}°{\left[\begin{array}{cc}{l}^2/D& 0\\ 0& J_{SS}\end{array}\right]}_{\mathrm{fit}\mathrm{parameter}}\to {\left[t,J\right]}_{\mathrm{Experimental}\mathrm{data}}$$

(21)

Since $J_{SS}$ and l are known parameters from experiments, only the diffusivity must be varied to fit the experimental data. With this algorithm, it was then possible to obtain the diffusivity values reported in

Table 5. Calculated diffusivity coefficients for the tested preconditioning IDs.

Material System	Preconditioning ID	Diffusivity Avg. $\left[\frac{{\mathit{m}}^2}{\mathit{s}}\right]$	Diffusivity St. Dev. $\left[\frac{{\mathit{m}}^2}{\mathit{s}}\right]$
P1	N0-M0	2.72$·{10}^{-11}$	3.97$·{10}^{-12}$
P1	N1-M0	3.08$·{10}^{-11}$	1.03$·{10}^{-12}$
P1	N5-M0	1.85$·{10}^{-11}$	6.6$·{10}^{-13}$
P1	H0-M50	3.24$·{10}^{-11}$	9.74$·{10}^{-13}$
P1	H5-M50	4.12$·{10}^{-11}$	1.49$·{10}^{-12}$
P2	H0-M0	2.36$·{10}^{-10}$	8$·{10}^{-12}$

. Preconditioning H0-M50 and H5-M50 present the highest calculated diffusion coefficients. The thermoplastic P2 material, included here for a comparison of diffusion values, shows diffusion values one order of magnitude greater.

By a thorough observation of the curve fitting graphs, it is apparent how the experimental results present in many cases, significant, abrupt deviations from the ideal behavior. This phenomenon might be attributed to sudden changes within the material. It is also noticeable how the experimental curves in many instances present significantly bigger steps than usual. This is due to the specific recording apparatus used in this study, which was limited in resolution to units and their first decimal places for each decade. The occurrence of these bigger steps in the experimental recordings sometimes prevented an accurate evaluation of the $R^2$ coefficient. The permeation coefficient could be used as a comparison criterion for the Fickian specimens; however, since there are deviations from the ideal behavior, the leak rate independent of the testing area ($L{R}_{SP}={\displaystyle \frac{mbar·L}{s·m^2}}$) was used as a comparison value for all the specimens for consistency reasons. The steady-state leak rate for the P1 material was averaged for each preconditioning group and is presented in Figure 14. A statistical analysis was performed considering all P1 material specimens (three at the minimum, tested within one specific preconditioning condition, and the results are presented in

Table 6. Summary of statistical data for tested specimens of the P1 material, grouped by preconditioning type. Capital letter N stands for liquid nitrogen immersions instead of liquid hydrogen ones.

${\mathit{LR}}_{\mathit{SP}}$$\left[\frac{\mathit{mbar}·\mathit{L}}{\mathit{s}·{\mathit{m}}^2}\right]$	N0-M0	N1-M0	N5-M0	H0-M50	H5-M50
Minimum	1.49$·{10}^{-10}$	1.83$·{10}^{-10}$	1.49$·{10}^{-10}$	1.01 $·{10}^{-10}$	8.74$·{10}^{-11}$
Maximum	2.28$·{10}^{-10}$	2.89$·{10}^{-10}$	2.07$·{10}^{-10}$	1.87$·{10}^{-10}$	1.91$·{10}^{-10}$
Average	1.79$·{10}^{-10}$	2.44$·{10}^{-10}$	1.80$·{10}^{-10}$	1.41$·{10}^{-10}$	1.43$·{10}^{-10}$
Standard Deviation	2.96$·{10}^{-11}$	4.42$·{10}^{-11}$	2.41$·{10}^{-11}$	3.55$·{10}^{-11}$	4.26$·{10}^{-11}$
Coefficient of Variation [%]	16.6	18	13.4	25.2	29.8

). The high coefficient of variation in these results comes from the unavoidable uncertainty associated with the appropriate testing time caused by the unknown material behavior. This factor, coupled with statistical differences in the specimen thickness and pressure applied, led to significant variations in the time to reach steady state, and as a consequence, some tests were stopped before they reached the steady-state leak rate. To account for this, an estimated steady-state leak rate was calculated using the solution to Fick’s equations. This procedure allowed us to account for an imprecise timing evaluation of the testing time, which could have caused an imprecise understanding and recording of the leak rate.

The results show how there is no significant change in leak rate between all tested specimens of the P1 material. A minor reduction in specific leak rate was identified for preconditioning types H0-M50 and H5-M50 when compared to N0-M0. A minor increase in specific leak rate was identified for preconditioning type N1-M0 when compared to N0-M0.

5. Discussion

A Fickian behavior investigation was performed, and deviations from the ideal behavior were assessed for every specimen considered by simultaneously evaluating both subjective and objective comparison criteria. This practice is recommended for future Fickian behavior investigations, even though it is acknowledged that the implementation methodology for the coefficient of determination needs to be further refined.

No substantial changes in permeation were observed throughout the testing of the P1 material system. However, the Fickian investigation showed a clear difference between the non-preconditioned P1 material and increasing levels of preconditioning. This study highlights the possibility of evaluating the formation of inhomogeneities within the material without the aid of additional evaluation tools or technology. This was corroborated by evidences of statistically diverse (Fickian and non-Fickian) diffusion behaviors over the same material, depending on the preconditioning it was subject to.

The Fickian behavior of specimens only thermally conditioned further reinforces the understanding previously found in the literature, which is that thermal cycles alone are not sufficient to properly investigate the resistance or suitability of a material for its use in cryogenic storage solutions. The results of Fickian curve fitting on the experiments also showed the importance of including mechanical stress application in the preconditioning studies of materials envisioned for cryogenic storage solutions, reinforcing the understanding introduced in the literature [17,24] as well as highlighting the need for Fickian behavior investigations on top of permeation/leak rate measurements. This was particularly evident in the results for preconditions H0-M50 and H5-M50, where a lower value for average leak rate alone could not suggest any modifications in the material’s behavior.

However, even though this study proves and identified changes in material behavior due to thermal and mechanical cycling, deviations from ideal diffusion are not as incisive as Just [33] found, showing a complete lack of time lag and immediate leak behavior. The experimental results showed that the main features of the diffusion were still visible for every specimen and that the offset from the ideal behavior must be judged by evaluating them against theory.

The slight increase in leak rate for N1-M0 compared to N0-M0 and the slight decrease for H0-M50 and H5-M50 compared to N0-M0 are difficult to explain. The moderate increase could be linked to releasing internal thermal stresses originally present in the material, induced by the manufacturing method, which reinforces conclusions already drawn by Choi [10] or Timmerman who identified a high crack density increase after the first cryogenic cycles [26].

Identifying the correct testing time for every experiment was difficult, and such uncertainty led to identifying the need to estimate the actual steady-state leak rate instead of just averaging a selected time frame at the end of the permeation recording. This was necessary in order to provide reliable results, avoiding unexpected underestimations of the leak rate. This practice is recommended for permeation testing both for understanding the proper testing time and for validating the final leak rate in the case of near-Fickian behavior.

Because of the estimation performed, an additional error factor needs to be considered in the final results presented. However, at the moment, it is not possible to quantify this introduced error. It is anyway understood that the error induced by estimating the steady state is less than that induced by utilizing results from experiments terminated ahead of time. In the current investigation, the average normalized time of the experiment, considering $\tau$ values in Figure 4, was found to be 0.59 over every test performed. The normalized flux j value of $0.995$ corresponds to the normalized time of $0.606$, which means that the at this normalized time, the steady-state leak rate or flux can be assumed. In light of this, considering that the experimental normalized time of the experiment averaged over every test was found to be $0.59$, the evaluation of the experimental steady-state leak rate can be considered reliable.

Diffusion coefficient calculations were possible and were found to be in good agreement within a few units of the same order of magnitude for ever specimen tested for the P1 material. The soundness of the measurement was validated by a comparison with the diffusion coefficient of the thermoplastic P2 material, manufactured by additive manufacturing, which was expected to have a higher diffusion coefficient.

6. Conclusions

Here, the most relevant conclusions from this work are listed:

• A Fickian behavior investigation was performed on carbon fiber-reinforced thermoset and thermoplastic materials with specimens subjected to different preconditioning scenarios. Diffusion coefficients were calculated for every preconditioning scenario.
• The Fickian investigation on permeation measurements allowed us to identify material inhomogeneities undetectable by permeation coefficient or leak rate measurements alone and link them to specific preconditioning. This result could aid further research on similar investigations, where other methods to evaluate the material’s homogeneity cannot be implemented.
• The use of coefficient of determination as an objective method to evaluate and categorize Fickian, near-Fickian, and non-Fickian permeation behaviors needs to always be performed in conjunction with subjective evaluation of the main experimental permeation diffusion features (time lag, transient state, steady state) and compared to their respective theoretical ones.
• A methodology to improve the evaluation of correct testing time and steady-state leak rate determination is suggested.
• Cryogenic mechanical loading was demonstrated to be a more effective preconditioning method with respect to cryogenic thermal cycles alone.

Future Work

Some recommendations and directions for future work can be drawn. It is recommended to increase the scope, length, and effect of preconditioning performed, aiming for conditions that are as best as possible representative of the full life cycle of the application subject of the study. Performing Fickian investigations under such conditions could lead to an improved understanding of the actual permeation behavior of the final application. The implementation of the coefficient of determination $R^2$ must be improved with further studies, with the aim of making it reliable in evaluating Fickian, near-Fickian, and non-Fickian behaviors even in cases of experimental curve recording anomalies. Structural investigation and damage analysis should be performed on the same specimens following the results of this study to evaluate types of inhomogeneities present and quantify them, leading to possibilities for further correlations with permeation/diffusion behavior. Permeation studies should be performed at cryogenic temperatures when possible, aiming to perform permeation testing as close as possible to operating conditions for cryogenic LH2 storage solutions. Possibly, simultaneous strain application and leak rate measurements at cryogenic temperatures should be considered. Finally, sub-component or system permeation investigations should be performed with the aim of obtaining more representative results that consider edge effects and stress concentration locations.