Finite Element Analysis of Structural Parameter Effects on Stiffness Nonlinearity Behavior in Aero-Engine Elastic Rings
Abstract
1. Introduction
2. Theoretical Model of Elastic Ring Calculation
3. Establishment and Validation of Finite Element Model
3.1. Finite Element Model of Elastic Ring
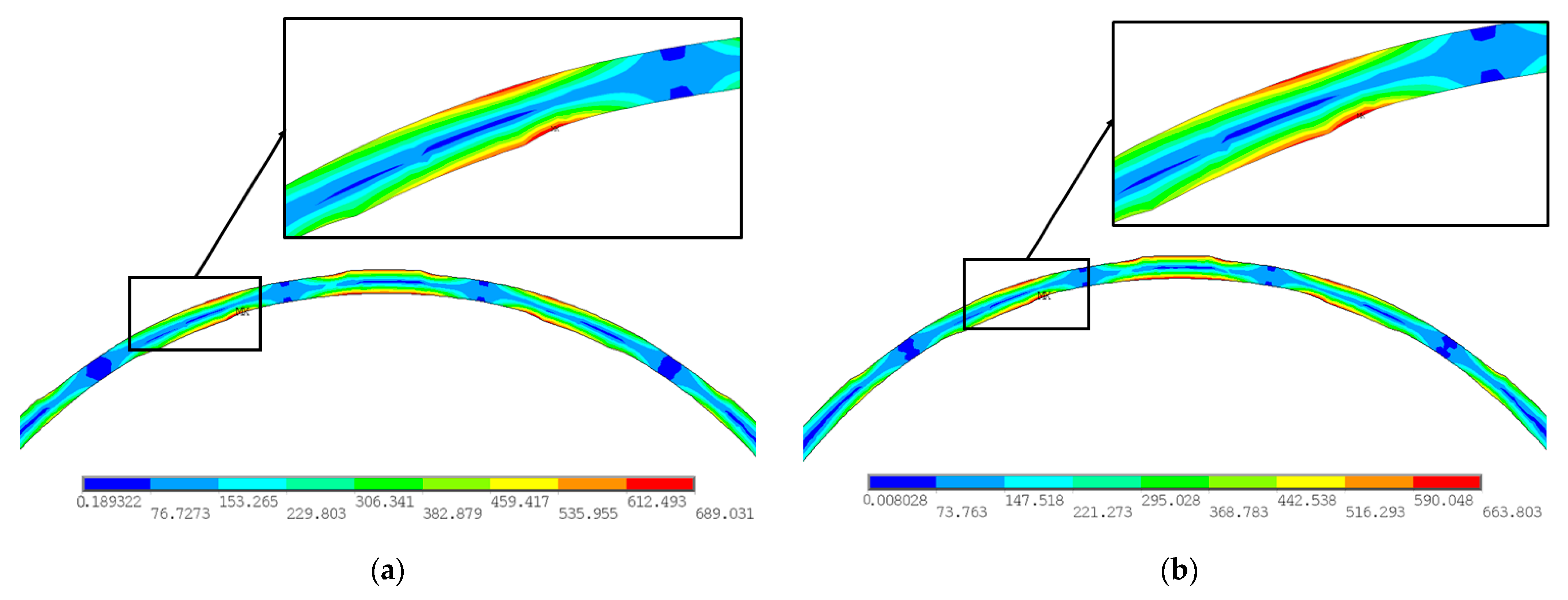
3.2. Validation of the Finite Element Model
4. Results and Analysis
4.1. Whirl Radius
4.2. Boss Width
4.3. Boss Height
4.4. Number of Bosses
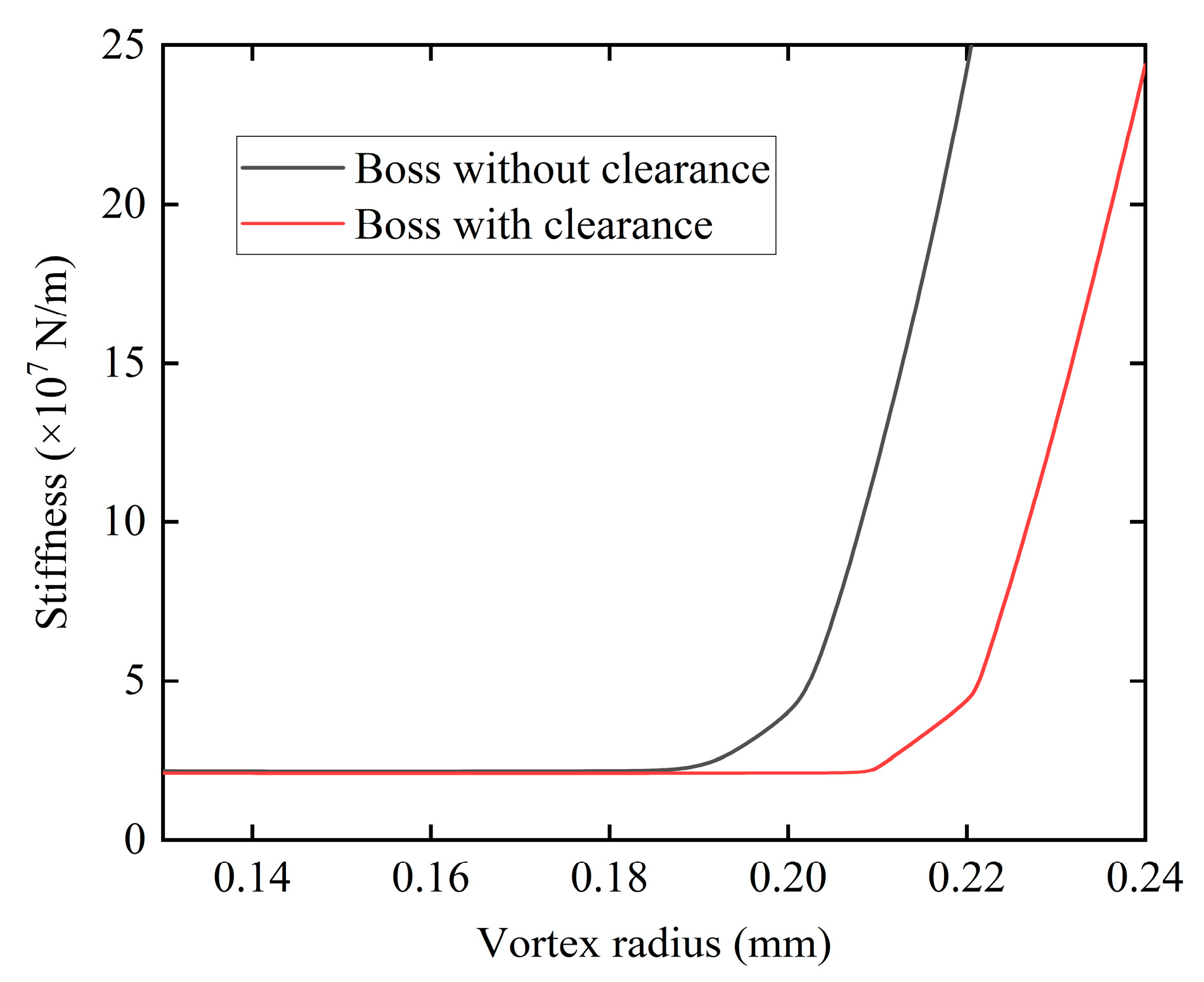
4.5. Clearance Fit
5. Conclusions
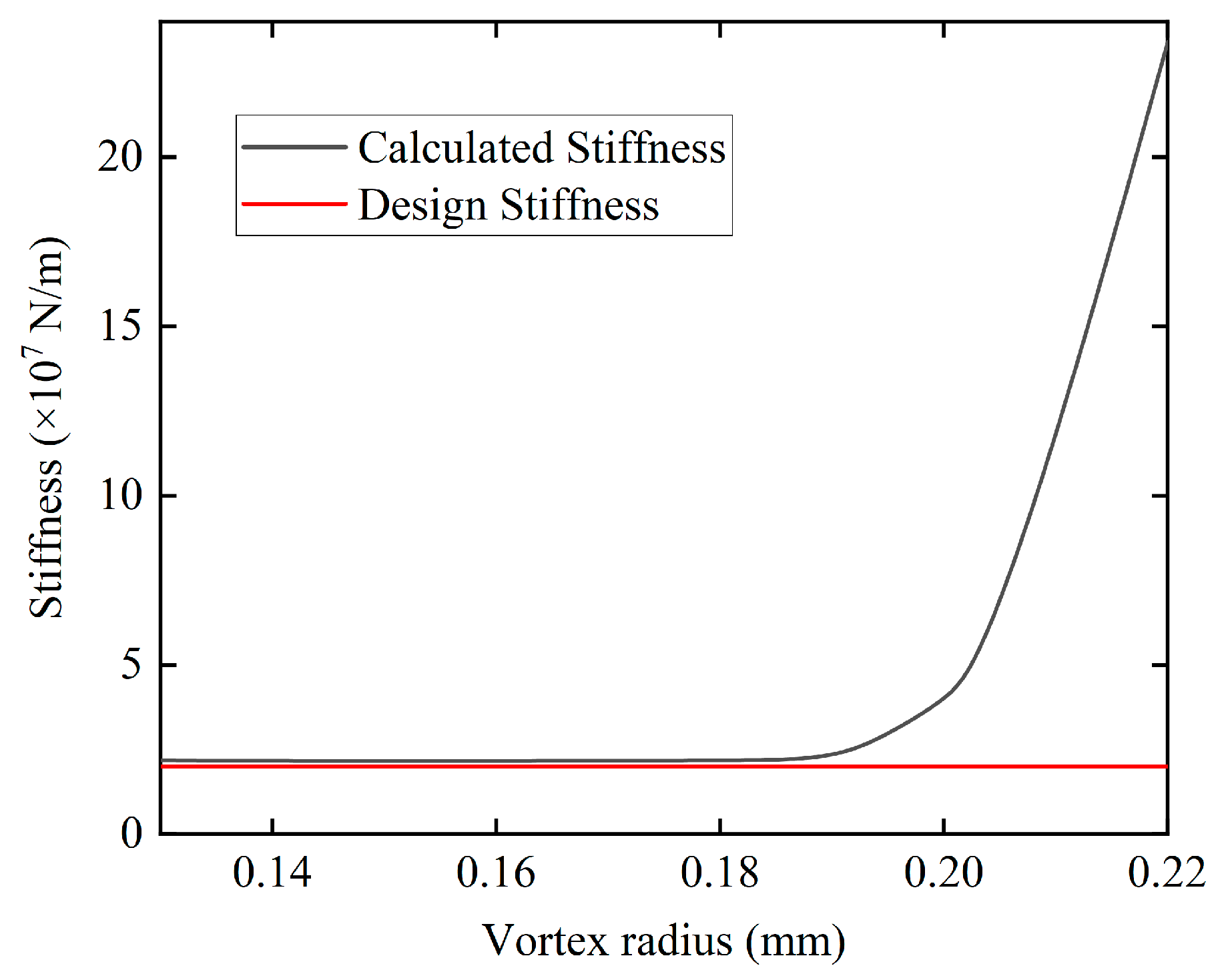
- Finite element analysis of the elastic ring provides a more accurate representation of its stiffness characteristics. Fitting stiffness formulas, which consider only geometric parameters of the elastic ring, fail to capture nonlinear behavior. As the rotor whirl radius increases, the elastic ring exhibits nonlinear stiffness due to plastic deformation at the bosses, where partial flattening occurs. At larger whirl radii, regions with higher equivalent stress should be selected as critical points for fatigue life assessment.
- Geometric parameters of the elastic ring, such as boss width, the ratio of boss height to ring thickness, and the number of bosses, significantly influence the onset of stiffness nonlinearity. Reducing the boss width or increasing the ratio of boss height to ring thickness mitigates stiffness nonlinearity at the same whirl radius, preventing significant shifts in the rotor’s critical speed.
- We provided design recommendations for optimizing the elastic ring in rotor–elastic support systems based on our findings. Maintaining the boss width between 2 mm and 6 mm can reduce the nonlinear behavior of the elastic ring while ensuring the stability of the rotor support system’s dynamic characteristics. Furthermore, the ratio of boss height to ring thickness should be maintained between 20% and 30%, which helps prevent the onset of stiffness nonlinearity without imposing overly strict requirements on the rotor system’s whirl radius. Given that variations in the number of bosses significantly affect stiffness and considering that nonlinear stiffness behavior is not particularly sensitive to changes in boss number, it is not recommended to suppress nonlinear stiffness by altering the number of bosses.
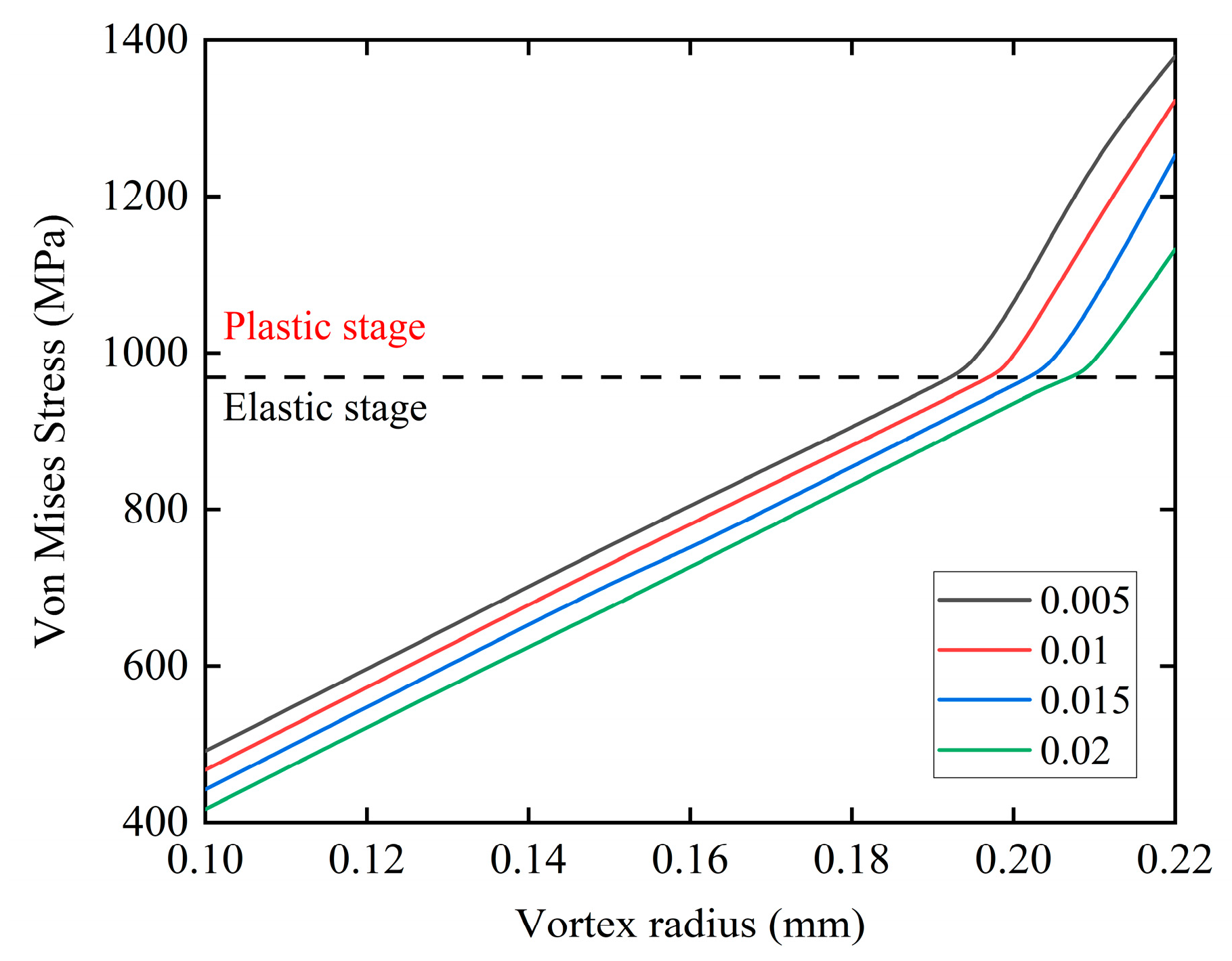
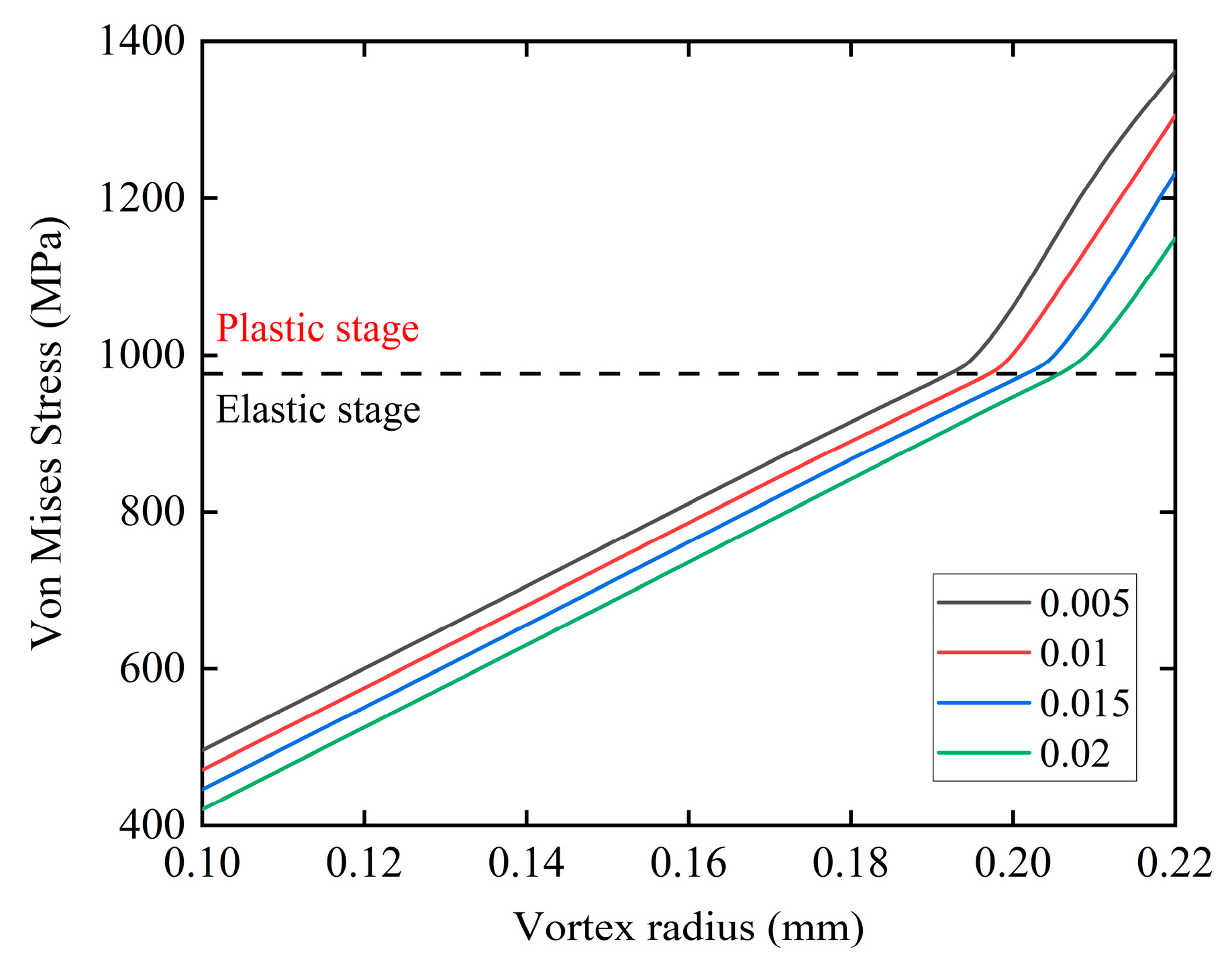
- Increasing the clearance fit between the elastic ring bosses and the mating components (inner and outer interfaces) suppresses stiffness nonlinearity. For the same clearance value, the outer clearance demonstrates a stronger inhibitory effect than the inner clearance. Compared to zero-clearance assemblies, small clearance fits are recommended in practical engineering to delay the onset of stiffness nonlinearity.
- While the finite element model has been verified by simulation and experiments in other studies and provides valuable insights, this study still has certain limitations in experimental validation. In future studies, establishing a rotor system test rig with an elastic ring support would be highly beneficial for substantiating our simulation results. Such a test rig would offer a clearer insight into the dynamic behavior changes of the elastic ring throughout the variation process and further enhance our understanding of the underlying mechanisms in practical applications.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| FEA | Finite Element Analysis |
| ERSFD | Elastic Ring Squeeze Film Damper |
| FEM | Finite Element Method |
References
- Lei, L.; Zhong, L.; Kaining, L.; Jilai, Z. Dynamic stiffness characteristics of aero-engine elastic support structure and its effects on rotor systems: Mechanism and numerical and experimental studies. Appl. Math. Mech. 2023, 44, 221–236. [Google Scholar] [CrossRef]
- Yongfeng, W.; Yanhong, M.; Jie, H. Study on dynamic stiffness of supporting structure and its influence on vibration of rotors. Chin. J. Aeronaut. 2022, 35, 252–263. [Google Scholar]
- Zhong, L.; Lei, L.; Yang, Y.; Xiaojie, H.; Jiaxi, L.; Zhe, D. Experimental and numerical investigations on novel models for mechanical behaviors of the elastic ring in aero-engine. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2021, 235, 6257–6267. [Google Scholar]
- Xing, Z.; Wang, X. Critical speed calculation and modal analysis of rotor for high-speed machine. In Proceedings of the 2017 IEEE Transportation Electrification Conference and Expo, Asia-Pacific (ITEC Asia-Pacific), Harbin, China, 7–10 August 2017; pp. 1–6. [Google Scholar]
- Zapoměl, J.; Ferfecki, P. A computational investigation on the reducing lateral vibration of rotors with rolling-element bearings passing through critical speeds by means of tuning the stiffness of the system supports. Mech. Mach. Theory 2011, 46, 707–724. [Google Scholar] [CrossRef]
- Alexander, B.; Robert, G. Damping and Stiffness Coefficients of Elastomer Rings and Their Optimised Application in Rotor Dynamics. Aust. J. Mech. Eng. 2004, 1, 91–101. [Google Scholar]
- Diligenskiy, D.S.; Novikov, D.K. Studying of Manufacturing Tolerance Influence on the Performance of GTE Rotor Elastic Rings. Procedia Eng. 2017, 176, 483–497. [Google Scholar] [CrossRef]
- Liu, Z.; Ju, J. Developing a scalable analytical framework for predicting the contact behavior of elastic rings against rigid surfaces. Int. J. Solids Struct. 2024, 301, 112967. [Google Scholar] [CrossRef]
- Ding, H.; Zhu, M.; Zhang, Z.; Zhang, Y.-W.; Chen, L.-Q. Free Vibration of a Rotating Ring on an Elastic Foundation. Int. J. Appl. Mech. 2017, 9, 1750051. [Google Scholar] [CrossRef]
- Paridie, A.M.; Ene, N.M. Theoretical study of effect of the geometrical parameters on the dynamic properties of the elastic rings of an air journal bearing. Heliyon 2023, 9, e16129. [Google Scholar] [CrossRef]
- Liu, C.; Cooley, C.G.; Parker, R.G. Parametric instability of spinning elastic rings excited by fluctuating space-fixed stiffnesses. J. Sound Vib. 2017, 400, 533–549. [Google Scholar] [CrossRef]
- Forbes, G.L.; Randall, R.B. Resonance phenomena of an elastic ring under a moving load. J. Sound Vib. 2008, 318, 991–1004. [Google Scholar] [CrossRef][Green Version]
- Stelian, A.; FlorinaCarmen, C.; IonutCristian, R. Stress State in an Eccentric Elastic Ring Loaded Symmetrically by Concentrated Forces. Mathematics 2022, 10, 1314. [Google Scholar] [CrossRef]
- Navazi, H.M.; Hojjati, M. Nonlinear vibrations and stability analysis of a rotor on high-static-low-dynamic-stiffness supports using method of multiple scales. Aerosp. Sci. Technol. 2017, 63, 259–265. [Google Scholar] [CrossRef]
- Zharilkassin, I.; Kuatbay, B.; Nutpulla, J.; Azizbek, A. Modeling the Dynamics of a Gyroscopic Rigid Rotor with Linear and Nonlinear Damping and Nonlinear Stiffness of the Elastic Support. Machines 2021, 9, 276. [Google Scholar] [CrossRef]
- Zhou, J.; Luo, Z.; Li, L.; Ma, T.; Li, H. Numerical and experimental analysis of the influence of elastic supports on bearing-rotor systems. Mech. Syst. Signal Process. 2025, 224, 112235. [Google Scholar] [CrossRef]
- Kai, S.; Zhong, L.; Lei, L.; Jiaxi, L.; Fayong, W. Dynamic analysis of the variable stiffness support rotor system with elastic rings. Nonlinear Dyn. 2022, 110, 201–217. [Google Scholar]
- Zhang, W.; Ding, Q. Elastic ring deformation and pedestal contact status analysis of elastic ring squeeze film damper. J. Sound Vib. 2015, 346, 314–327. [Google Scholar] [CrossRef]
- Wang, Z.; Liu, Z.; Zhang, G. Dynamic characteristics of elastic ring squeeze film damper. Ind. Lubr. Tribol. 2019, 71, 1144–1151. [Google Scholar] [CrossRef]
- Chen, W.; Chen, S.; Hu, Z.; Tang, J.; Li, H. A novel dynamic model for the spiral bevel gear drive with elastic ring squeeze film dampers. Nonlinear Dyn. 2019, 98, 1081–1105. [Google Scholar] [CrossRef]
- Yongxiang, X.; Xiao, C.; Jibin, Z.; Wenjuan, Q.; Yong, L. Influence of Orifice Distribution on the Characteristics of Elastic Ring-Squeeze Film Dampers for Flywheel Energy-Storage System. IEEE Trans. Plasma Sci. 2013, 41, 1272–1279. [Google Scholar]
- Yang, X.; Jiang, B.; Li, Y.; Zhao, Q.; Deng, S.; Zhang, W.; Cui, Y. Dynamic characteristics of elastic ring squeeze film damper coupled high-speed ball bearings. J. Sound Vib. 2022, 537, 117186. [Google Scholar] [CrossRef]
- Zhang, Y.; Wang, S.; Zhao, K.; Xu, L.; Yang, F.; Li, Q. Vibration characteristics of dual-rotor system with staggered-type double elastic ring squeeze film dampers under maneuvering flight. Aerosp. Sci. Technol. 2024, 152, 109351. [Google Scholar] [CrossRef]
- Yang, X.; Jiang, B.; Li, Y.; Zhao, Q.; Deng, S. Dynamic Characteristics of Elastic Ring Squeeze Film Damper Oscillated by Bearing Contact Force. J. Vib. Eng. Technol. 2024, 12, 433–455. [Google Scholar] [CrossRef]
- Sun, K.; Luo, Z.; Li, L.; Wu, F.; Hao, H. Mechanical and vibration characteristics investigation of the elastic ring squeeze film damper-rotor considering oil film sealing and leakage. Int. J. Non-Linear Mech. 2024, 161, 104701. [Google Scholar] [CrossRef]
- Li, Y.; Yang, H.; Deng, S. Dynamic characteristics study of elastic ring squeeze film damper with rigid–elastic–oil coupled model. Lubricants 2023, 11, 491. [Google Scholar] [CrossRef]
- Wang, Y.X.; Yan, J.; Wang, S.Z. An Effective Method for Determining Finite Element Model Parameters of Rotor Elastic Support System. Appl. Mech. Mater. 2012, 141, 191–197. [Google Scholar]
- Chiang, H.-W.D.; Hsu, C.-N.; Tu, S.-H. Rotor-bearing analysis for turbomachinery single-and dual-rotor systems. J. Propuls. Power 2004, 20, 1096–1104. [Google Scholar] [CrossRef]
- Zhang, R.-H.; Zhu, Y.-F.; Xiong, Z.-W.; Ye, Y.-H. Dynamical characteristics research on the squeezed film damper stiffness rotor. In Proceedings of the 2012 Symposium on Piezoelectricity, Acoustic Waves, and Device Applications (SPAWDA), Shanghai, China, 23–25 November 2012; pp. 438–442. [Google Scholar]
- Wang, Z.; Xu, N.; Yu, X.; Liu, Z.; Zhang, G. The dynamic characteristic analysis of elastic ring squeeze film damper by fluid-structure interaction approach. In Proceedings of the Turbo Expo: Power for Land, Sea, and Air, Charlotte, NC, USA, 26–30 June 2017; p. V07AT34A005. [Google Scholar]
- Long, X.Y.; Hong, J.; Zhang, D.Y.; Lin, H.Y. Study of the methods for analyzing the rigidity and strength of an elastic ring and its mechanics characteristics. J. Eng. Therm. Energy Power 2010, 25, 145–149. [Google Scholar]
- Jialiu, G. Rotor Dynamics; National Defence Industry Press: Beijing, China, 1985. [Google Scholar]
- Agarwal, A.; Mthembu, L. CFD analysis of conical diffuser under swirl flow inlet conditions using turbulence models. Mater. Today Proc. 2020, 27, 1350–1355. [Google Scholar] [CrossRef]
- Agarwal, A.; Mthembu, L. Finite element investigation of the vibration characteristics of francis turbine vanes. In Emerging Trends in Mechanical and Industrial Engineering: Select Proceedings of ICETMIE 2022; Springer: Berlin/Heidelberg, Germany, 2023; pp. 945–960. [Google Scholar]
- Haishen, J.; Jiancheng, S.; Wencui, L.; Xiangbin, Y. Rheological behaviours and constitutive models of 9Cr18Mo stainless steel at high temperature and high strain rate. Iron Steel Vanadium Titan. 2023, 44, 158–166+175. [Google Scholar]
- Simo, J.C.; Hughes, T.J. Computational Inelasticity; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2006; Volume 7. [Google Scholar]
- Bathe, K.-J. Finite Element Procedures; Prentice Hall: Englewood Cliffs, NJ, USA, 2006. [Google Scholar]
- Zhifei, H.; Qian, D.; Wei, Z. Dynamical analysis of an elastic ring squeeze film damper-rotor system. Mech. Mach. Theory 2018, 131, 406–419. [Google Scholar]
- Sun, X.; Deng, S.; Chen, G.; Zhang, W. Calculation and Analysis of Groove Elastic Support’s Radial Stiffness (RESEARCH NOTE). Int. J. Eng. 2017, 30, 1066–1073. [Google Scholar]
- Zhou, H.; Yu, J.; Wang, C.; Ke, A. Study on the influence of contact behavior on nonlinear stiffness characteristics of elastic rings. Int. J. Non-Linear Mech. 2025, 170, 104972. [Google Scholar] [CrossRef]

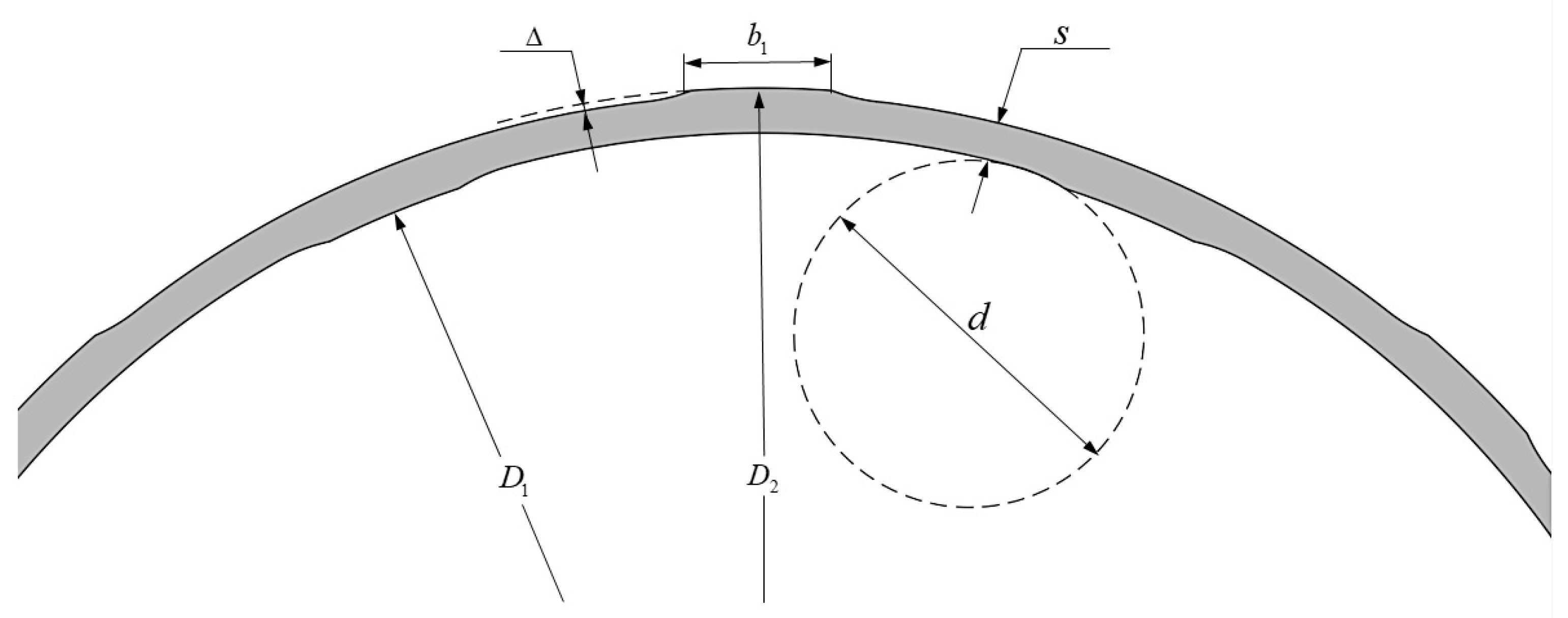
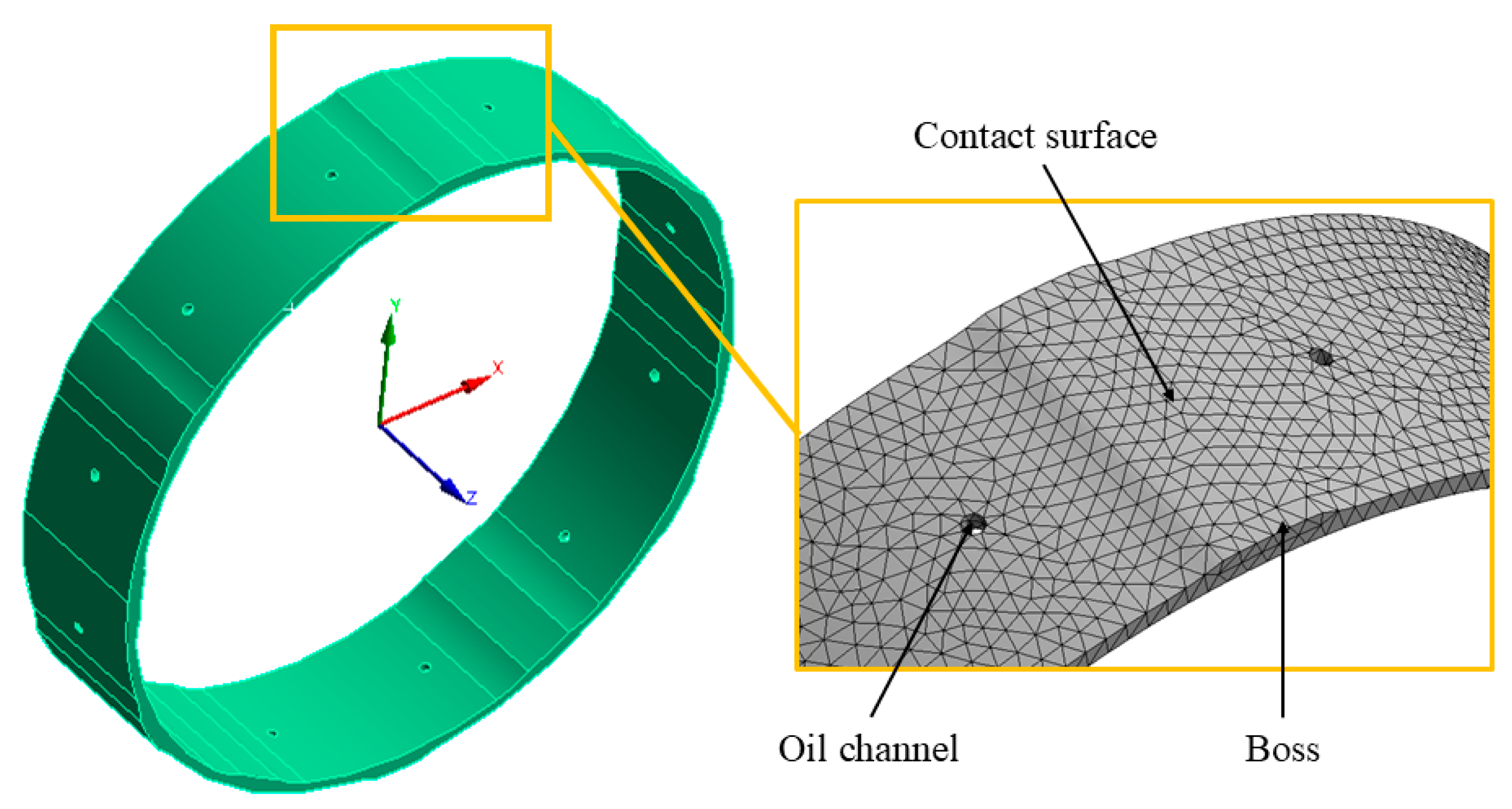



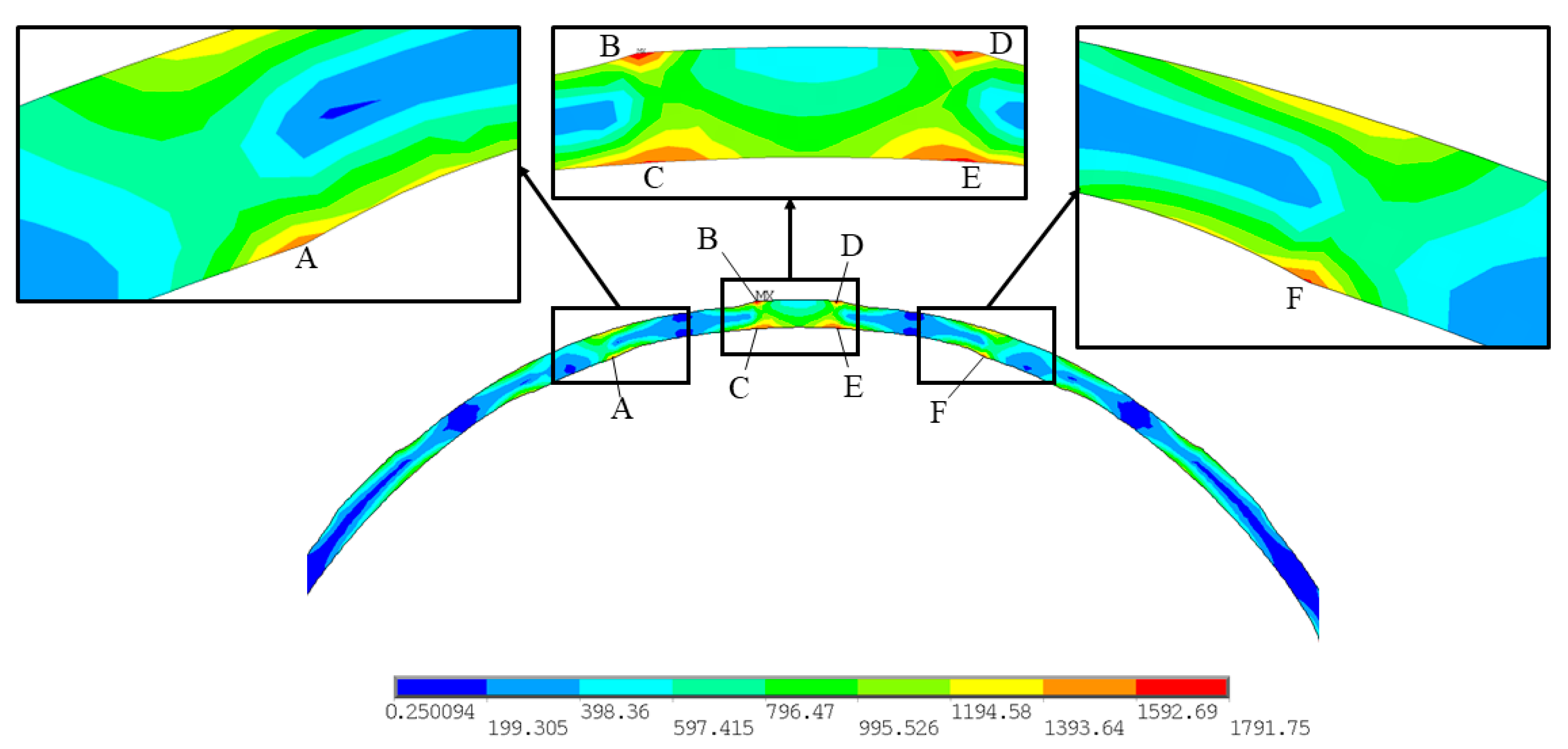
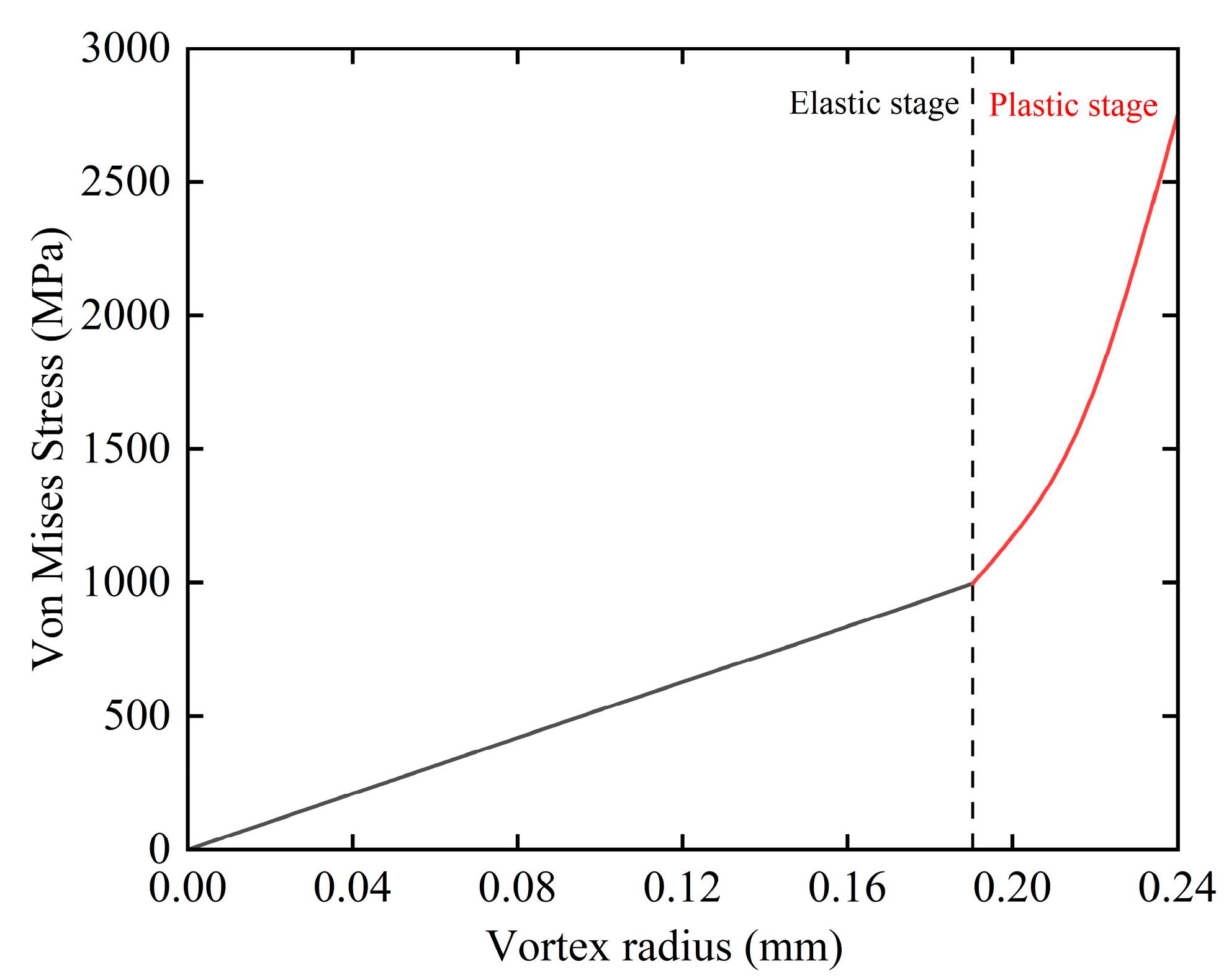



| Number of Bosses n | Inner Diameter D1 | Outer Diameter D2 | Boss Width b1 | Boss Height Δ | Axial Length L | Chamfer Diameter d |
|---|---|---|---|---|---|---|
| 8 | 55 mm | 58 mm | 4 mm | 0.2 mm | 13.5 mm | 5 mm |
| Thickness (mm) | Han’s Stiffness (N/m) | Fitting Stiffness (N/m) | Fitting Error (%) | Proposed FEM Stiffness (N/m) | Proposed FEM Error (%) |
|---|---|---|---|---|---|
| 0.5 | 9.8 × 105 | 1.2 × 106 | 22.45 | 1.04 × 106 | 6.12 |
| 0.7 | 2.68 × 106 | 3.16 × 106 | 17.91 | 2.89 × 106 | 7.84 |
| 0.95 | 6.7 × 106 | 7.26 × 106 | 8.36 | 6.98 × 106 | 4.18 |
| Outer Clearance (mm) | Transition Point (mm) | Maximum Equivalent Stress * (MPa) |
|---|---|---|
| 0.005 | 0.1939 | 979.97 |
| 0.01 | 0.1981 | 976.24 |
| 0.015 | 0.2039 | 981.93 |
| 0.02 | 0.2083 | 975.08 |
| Inner Clearance (mm) | Transition Point (mm) | Maximum Equivalent Stress * (MPa) |
|---|---|---|
| 0.005 | 0.1932 | 981.71 |
| 0.01 | 0.1973 | 976.92 |
| 0.015 | 0.2032 | 984.34 |
| 0.02 | 0.2048 | 970.46 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shi, Y.; Li, J.; Yang, Z.; Feng, Y. Finite Element Analysis of Structural Parameter Effects on Stiffness Nonlinearity Behavior in Aero-Engine Elastic Rings. Aerospace 2025, 12, 338. https://doi.org/10.3390/aerospace12040338
Shi Y, Li J, Yang Z, Feng Y. Finite Element Analysis of Structural Parameter Effects on Stiffness Nonlinearity Behavior in Aero-Engine Elastic Rings. Aerospace. 2025; 12(4):338. https://doi.org/10.3390/aerospace12040338
Chicago/Turabian StyleShi, Yihang, Jiaqi Li, Zhongyu Yang, and Yinli Feng. 2025. "Finite Element Analysis of Structural Parameter Effects on Stiffness Nonlinearity Behavior in Aero-Engine Elastic Rings" Aerospace 12, no. 4: 338. https://doi.org/10.3390/aerospace12040338
APA StyleShi, Y., Li, J., Yang, Z., & Feng, Y. (2025). Finite Element Analysis of Structural Parameter Effects on Stiffness Nonlinearity Behavior in Aero-Engine Elastic Rings. Aerospace, 12(4), 338. https://doi.org/10.3390/aerospace12040338






