A Preliminary Exploratory Study of the Flow and Heat Transfer Characteristics of Fuel Elements in Low-Enriched Uranium Cores
Abstract
1. Introduction
2. Fuel Element Description
2.1. Fuel Element Coolant
2.2. Fuel Element Geometric Model
3. Numerical Model
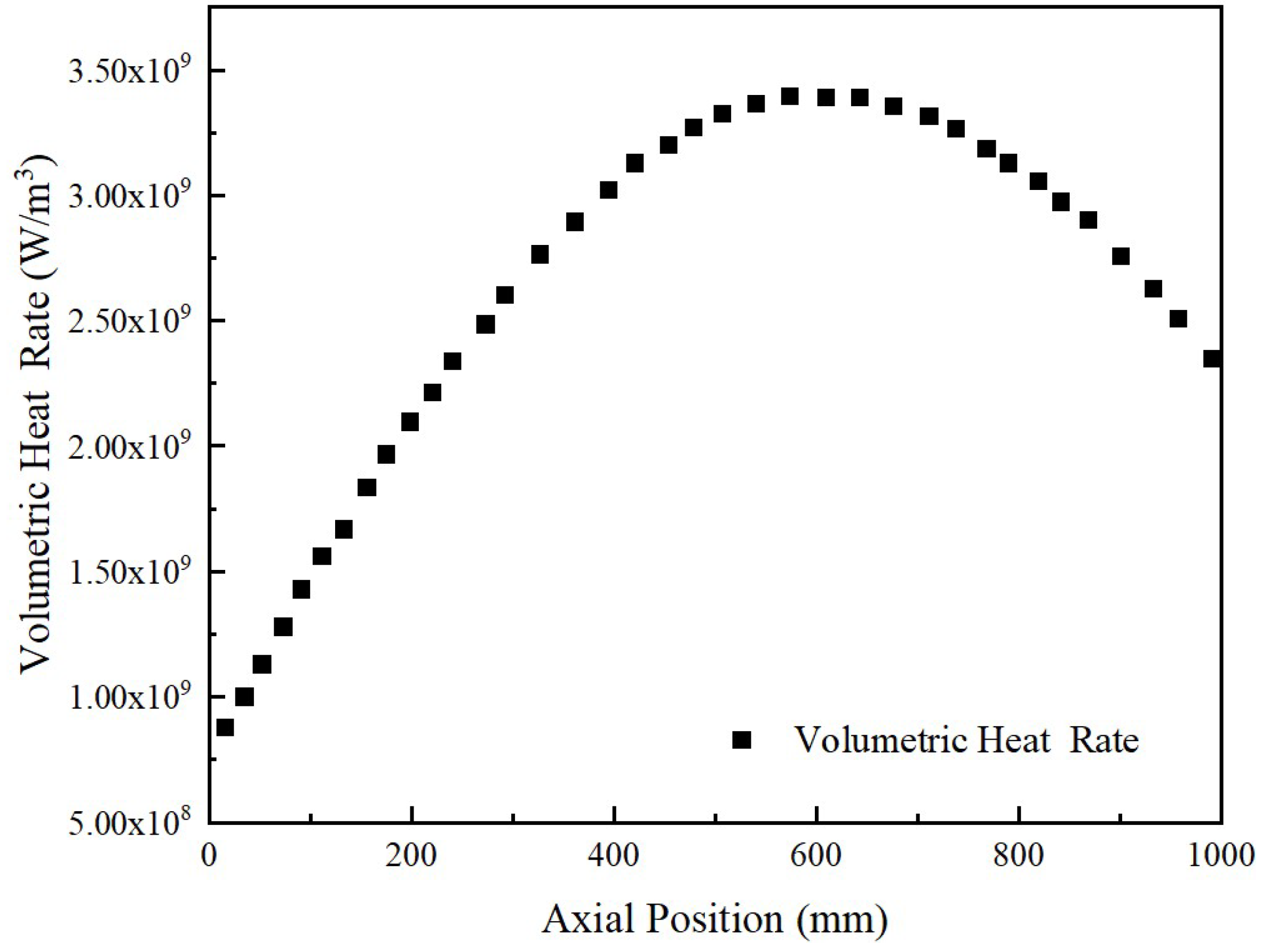
3.1. Boundary Condition Setting
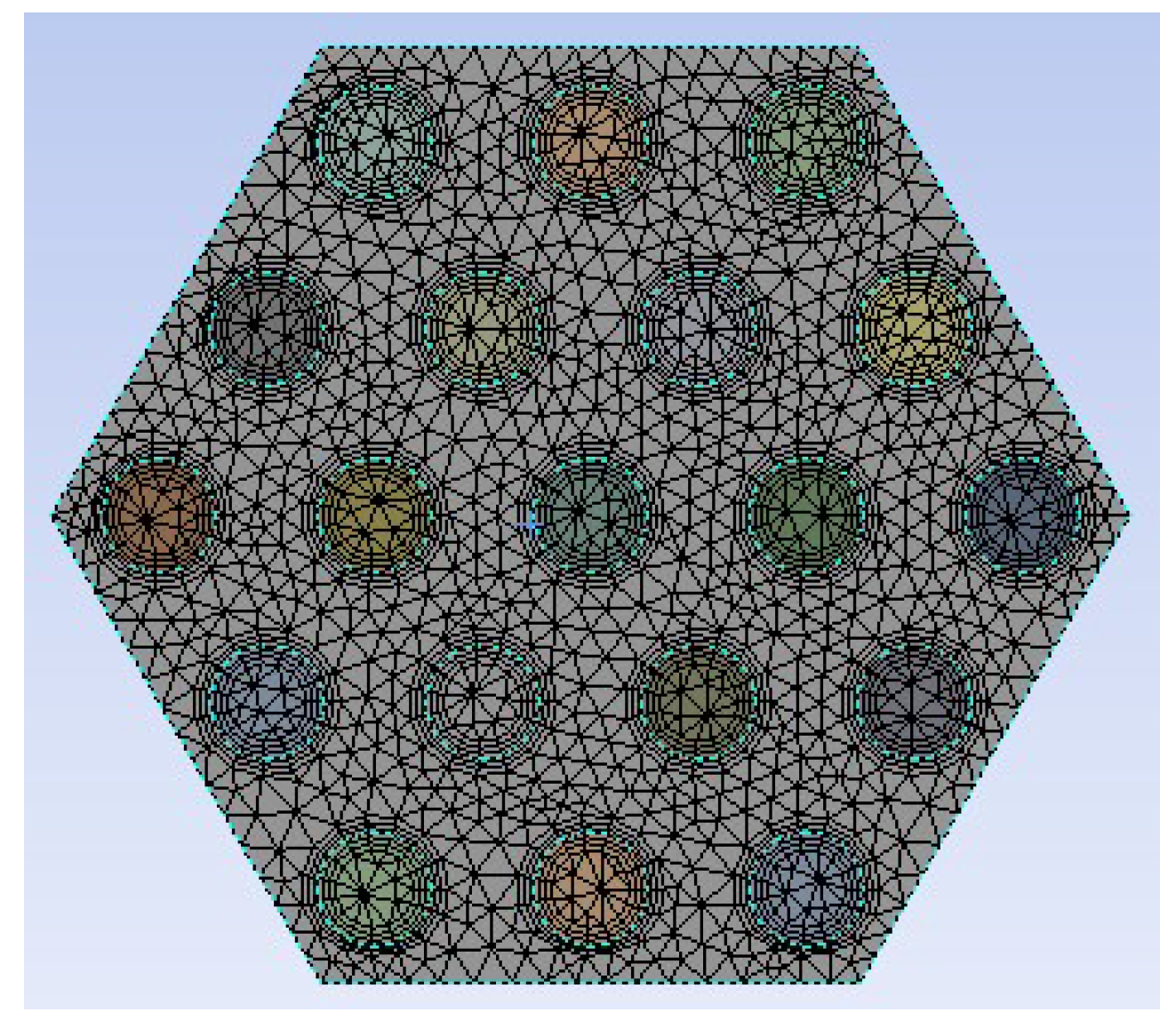
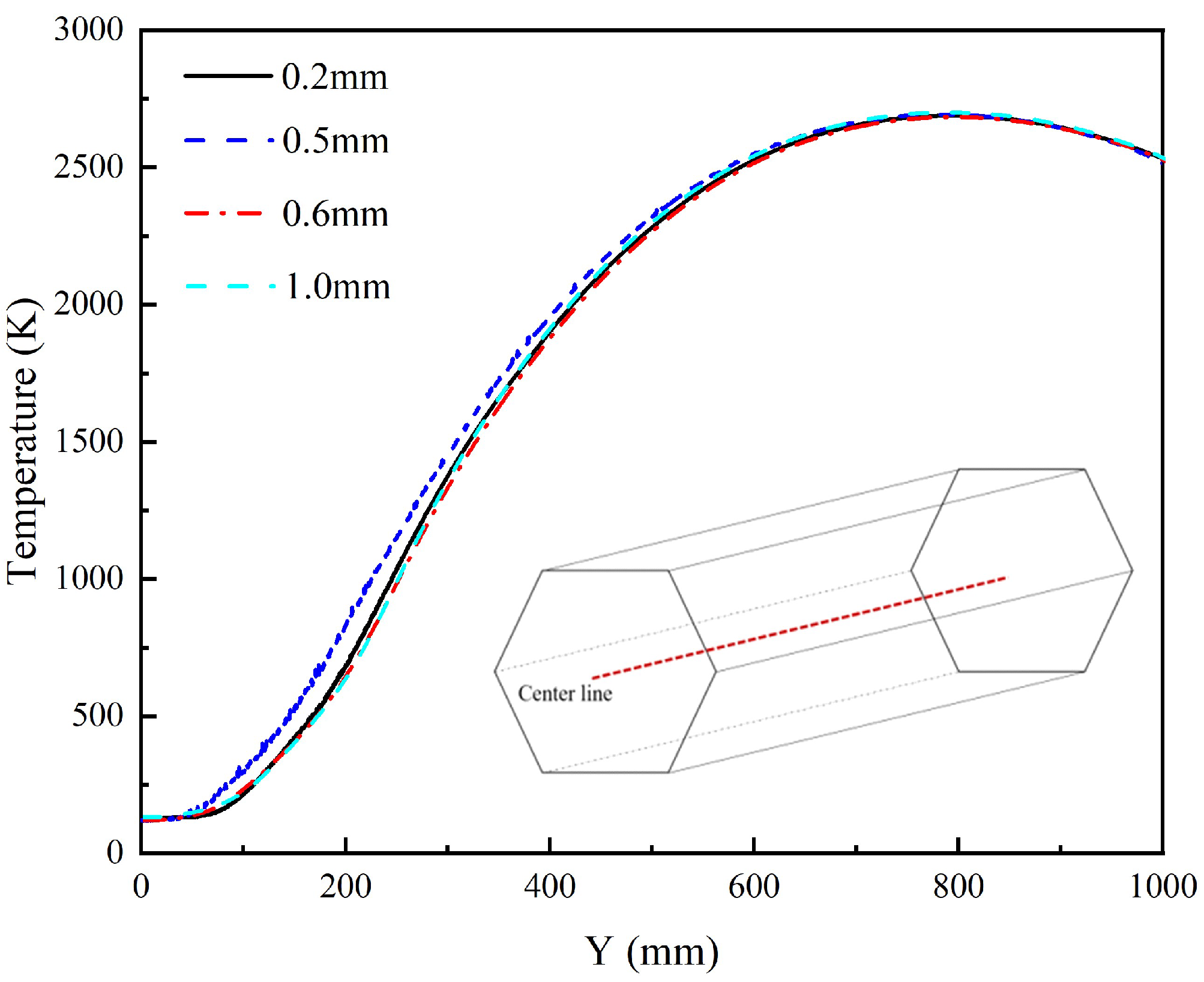
3.2. Mesh-Independent Verification
3.3. Numerical Modeling
3.4. Entrance Condition Design
4. Results and Discussion
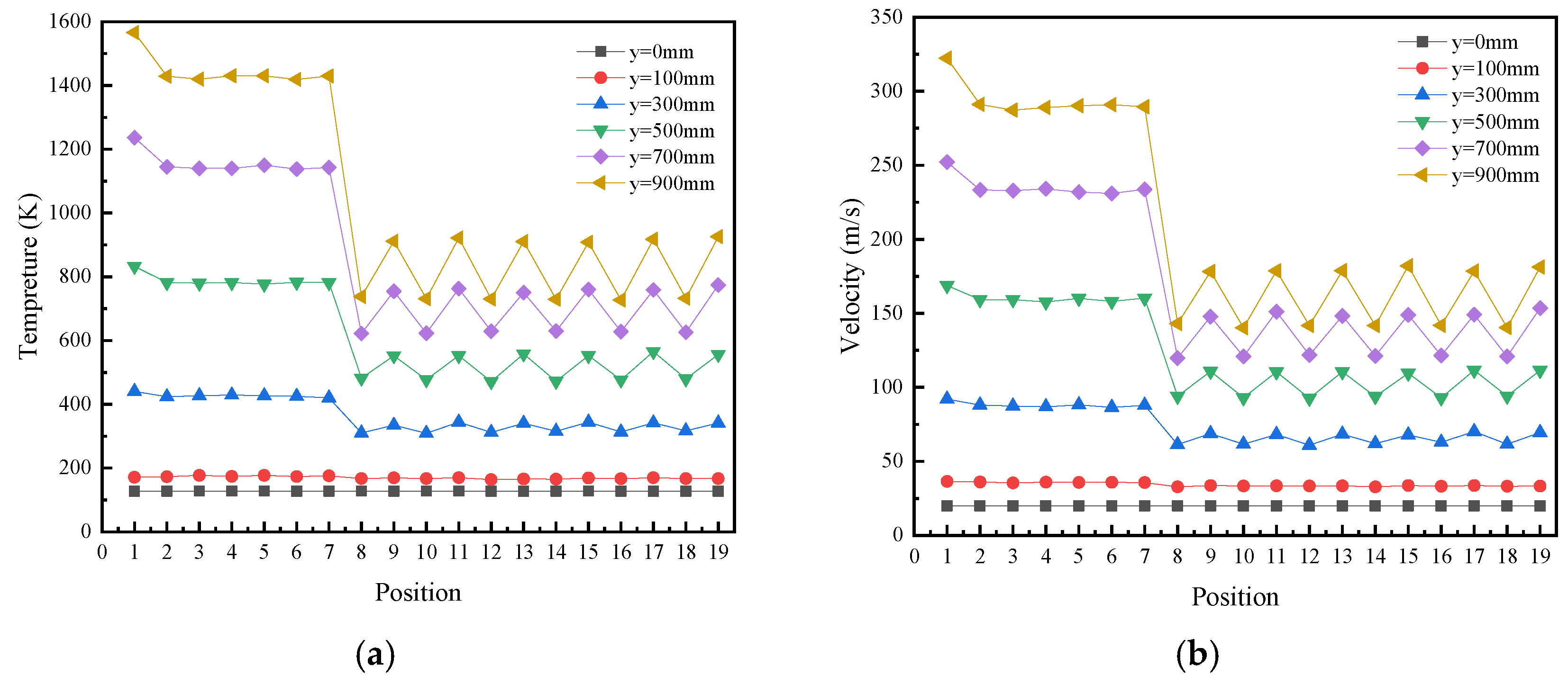
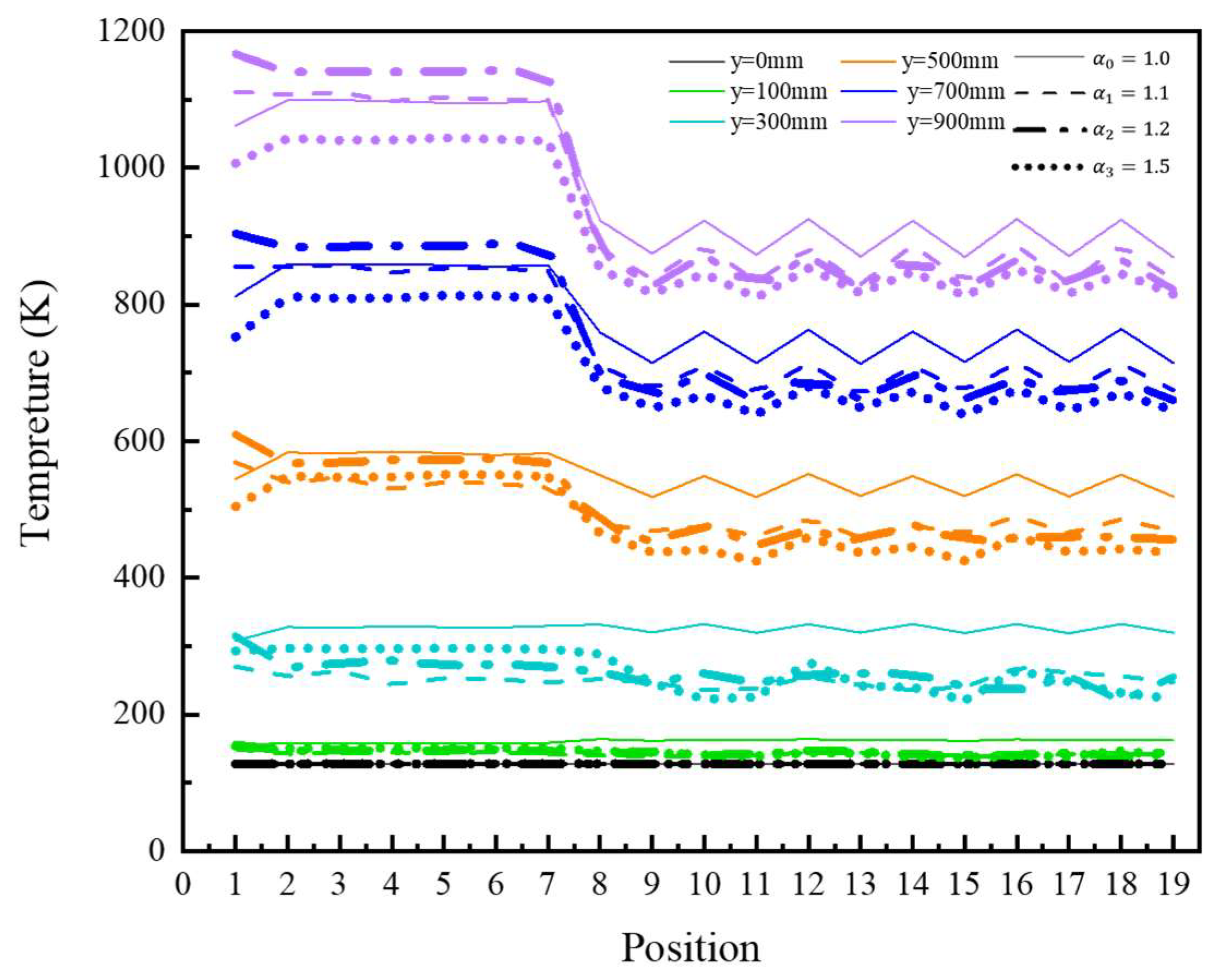
4.1. Temperature Distributions of the Radial Extraction Lines at Different Axial Positions
4.1.1. Conventional Fuel Elements
4.1.2. New Fuel Elements
4.2. Temperature Distribution at the Center of the Coolant Channel
4.2.1. Conventional Fuel Elements
4.2.2. New Fuel Elements
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| LEU | Low-enriched Uranium. |
| FE | Fuel element. |
| ZrC | Zirconium carbide. |
| NERVA | Nuclear engine for rocket engine application. |
| RNG | Renormalization group theory. |
| NRX | National research experimental reactor. |
| SST | Shear stress transport. |
References
- Menglong, Z.; Yue, Z.; Baohe, W. Review and prospect of space nuclear propulsion system. J. Ordnance Equip. Eng. 2018, 39, 96–100. [Google Scholar]
- Vadim, Z.; Vladimir, P. Russian nuclear rocket engine design for mars exploration. Tsinghua Sci. Technol. 2007, 12, 256–260. [Google Scholar] [CrossRef]
- Clark, J.S.; Mcdaniel, P.; Howe, S.; Helms, I.; Stanley, M. Nuclear Thermal Propulsion Technology: Results of an Interagency Panel in FY 1991; Technical Report; NASA: Washington, DC, USA, 1993. [Google Scholar]
- Walton, L.; Sapyta, J. SNTP program reactor design. In Proceedings of the Joint Propulsion Conference and Exhibit, San Jose, CA, USA, 15–17 July 2013. [Google Scholar] [CrossRef]
- Powell, J.; Maise, G.; Paniagua, J. Mini-MITEE: Ultra Small, Ultra-Light NTP Engines for Robotic Science and Manned Exploration Missions. AIP Conf. Proc. 2006, 813, 879–888. [Google Scholar] [CrossRef]
- Furman, E.M.; Anghaie, S. Thermal hydraulic design analysis of ternary carbide fueled square-lattice honeycomb nuclear rocket engine. Am. Inst. Phys. 1999, 458, 1501–1506. [Google Scholar] [CrossRef]
- Donders, N.; Emrich, B.; Taylor, B.; Tucker, D.; Barnes, M.; Benensky, K. Study of a Tri carbide Grooved Ring Fuel Element for Nuclear Thermal Propulsion. In Proceedings of the 2018 ANS/ENS Nuclear and Emerging Technologies for Space Conference, Las Vegas, NV, USA, 26 February–1 March 2018. [Google Scholar]
- Yuliang, F.; Lin, L.; Hailiang, S.; Zhiqiang, W.; Guohua, Z.; Yu, W. Overview of fuel element development for nuclear thermal propulsion reactors. Aerosp. Gen. Technol. 2020, 4, 63–70. [Google Scholar]
- Belair, M.L.; Sarmiento, C.; Lavelle, T. Nuclear Thermal Rocket Simulation in NPSS. In Proceedings of the 49th AIAA/ASME/SAE/ASEE Joint Propulsion Conference, San Jose, CA, USA, 14–17 July 2013. [Google Scholar] [CrossRef]
- Joyner, C.R.; Jennings, T.; Hanks, D.E.; Levack, D.J. NTP Engine System Design and Modeling. In Proceedings of the AIAA 2022-4373, Las Vegas, NV, USA, 24–26 October 2022. [Google Scholar] [CrossRef]
- Duan, Z.; Zhang, J.; Han, Z.; Wang, M.; Wu, Y.; He, Y.; Tian, W.; Qiu, S.; Su, G. Nuclear thermal coupling optimization of baffled NTP reactor. Ann. Nucl. Energy 2023, 180, 109496. [Google Scholar] [CrossRef]
- Xianbao, Y.; Chao, L.; Wei, T.; Yonghong, Z.; Binhang, Z.; Zhangliang, M.; Jianjun, Z.; Haibo, T.; Furong, L. COMSOL-based study of flow channel blockage accidents in plate fuel assemblies. J. Three Gorges Univ. Nat. Sci. Ed. 2021, 43, 6. [Google Scholar] [CrossRef]
- Dong, H.; Fan, W.; Guo, Y. CFD investigation and optimization on flow distribution of plate-type assembly. Nucl. Tech. 2016, 39, 60–64. [Google Scholar] [CrossRef]
- Hess, H.L.; Kunz, H.R. A Study of Forced Convection Heat Transfer to Supercritical Hydrogen. J. Heat Transf. 1965, 87, 371. [Google Scholar] [CrossRef]
- Fang, Y.; Yu, Q.; Wang, C.; Tian, W.; Su, G.; Qiu, S. Heat transfer of hydrogen with variable properties in a heated tube. Int. J. Heat Mass Transf. 2023, 209, 124128. [Google Scholar] [CrossRef]
- Wang, J.C.; Kotlyar, D. High-resolution thermal analysis of nuclear thermal propulsion fuel element using Open FOAM. Nucl. Eng. Des. 2021, 372, 110957. [Google Scholar] [CrossRef]
- AI, Q.; Shuai, W.; Jia-Huan, W.; Haochun, Z.; Xinlin, X. Study on influencing factors of thermal characteristics of nuclear thermal propulsion cooling channel. J. Eng. Thermophys. 2018, 39, 2745–2748. [Google Scholar]
- Li, W.; Guan, C.; Song, H.; Chai, X.; Liu, X. Numerical investigation of heat transfer characteristics of moderator assembly employed in a low-enriched uranium nuclear thermal propulsion reactor. Front. Energy Res. 2022, 10, 875371. [Google Scholar] [CrossRef]
- Ji, Y.; Li, Z.G.; Sun, J.; You, E.; Minggang, L.; Shi, L. Numerical investigation and parametric study on thermal-hydraulic characteristics of particle bed reactors for nuclear thermal propulsion. Nucl. Technol. 2020, 206, 1155–1170. [Google Scholar] [CrossRef]
- Nam, S.H.; Venneri, P.; Kim, Y.H.; Sang, C.H.; Jeong, Y.H. Preliminary conceptual design of a new moderated reactor utilizing an LEU fuel for space nuclear thermal propulsion. Prog. Nucl. Energy 2016, 91, 183–207. [Google Scholar] [CrossRef]
- Zhang, J.; Wang, C.; Zhang, J.; Zhang, D.; Tian, W.; Qiu, S.; Su, G. Design research for the porous hexagonal prism shaped fuel element adopted in nuclear thermal propulsion reactor based on neutronics and thermal hydraulics coupled method. Ann. Nucl. Energy 2021, 150, 107847. [Google Scholar] [CrossRef]
- Altman, D. Thermodynamic Properties and Calculated Rocket Performance of Hydrogen at 20,000 K. Available online: https://www.osti.gov/biblio/4216735 (accessed on 18 March 2025).
- Krecicki, M.; Kotlyar, D. Low enriched nuclear thermal propulsion neutronic, thermal hydraulic, and system design space analysis. Nucl. Eng. Des. 2020, 363, 110605. [Google Scholar] [CrossRef]
- Ju, W.; Dong, X.; Lu, R.; Shen, H.; Zhao, F.; Tan, S. Heat transfer performance of high temperature and high velocity hydrogen flow and analysis of blockage characteristics. Int. J. Adv. Nucl. React. Des. Technol. 2022, 4, 205–216. [Google Scholar]
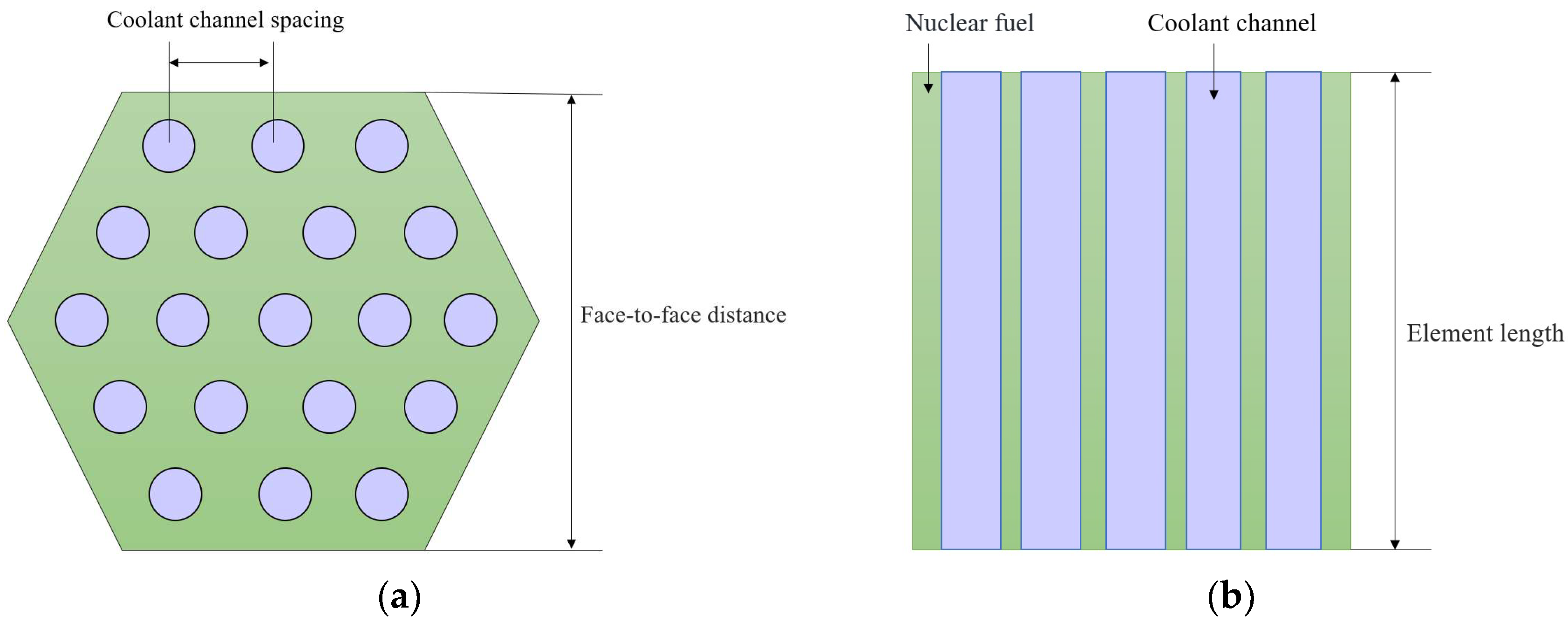

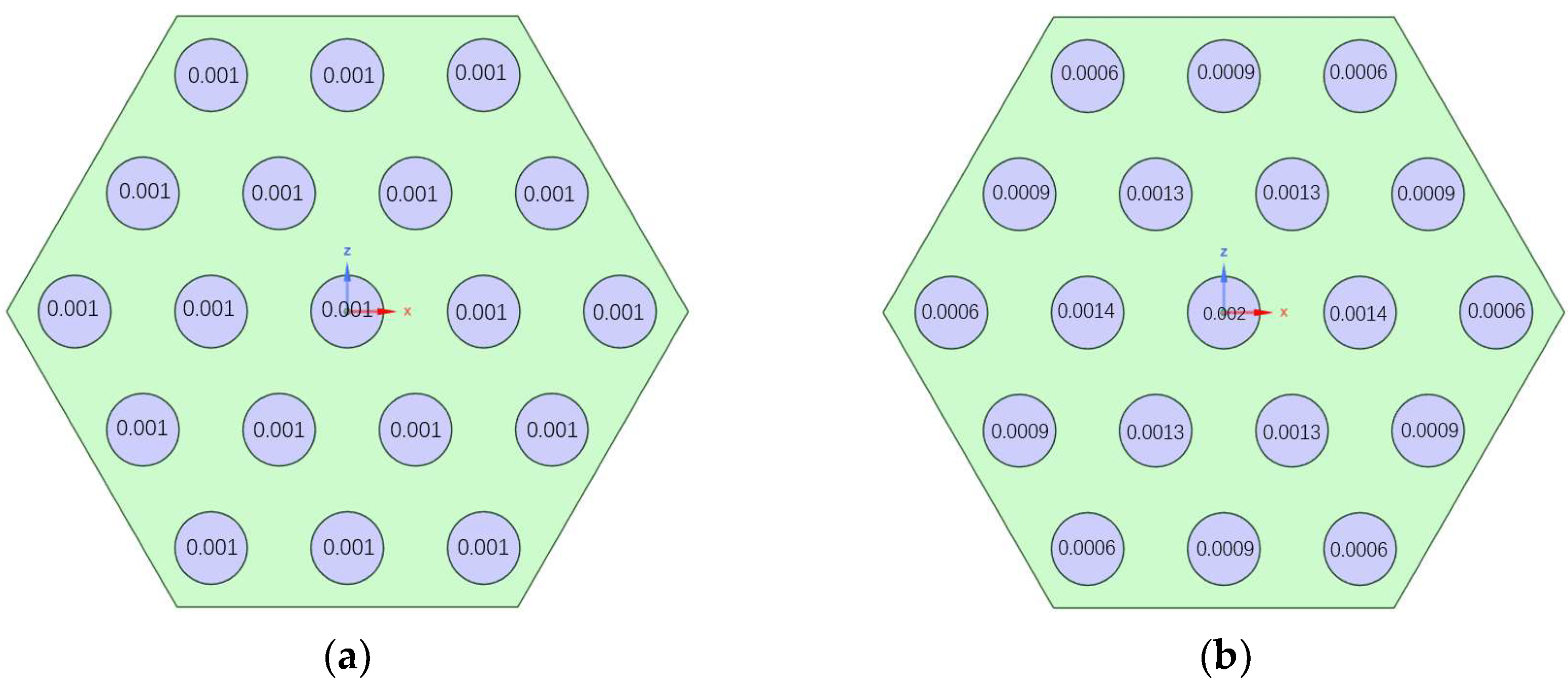
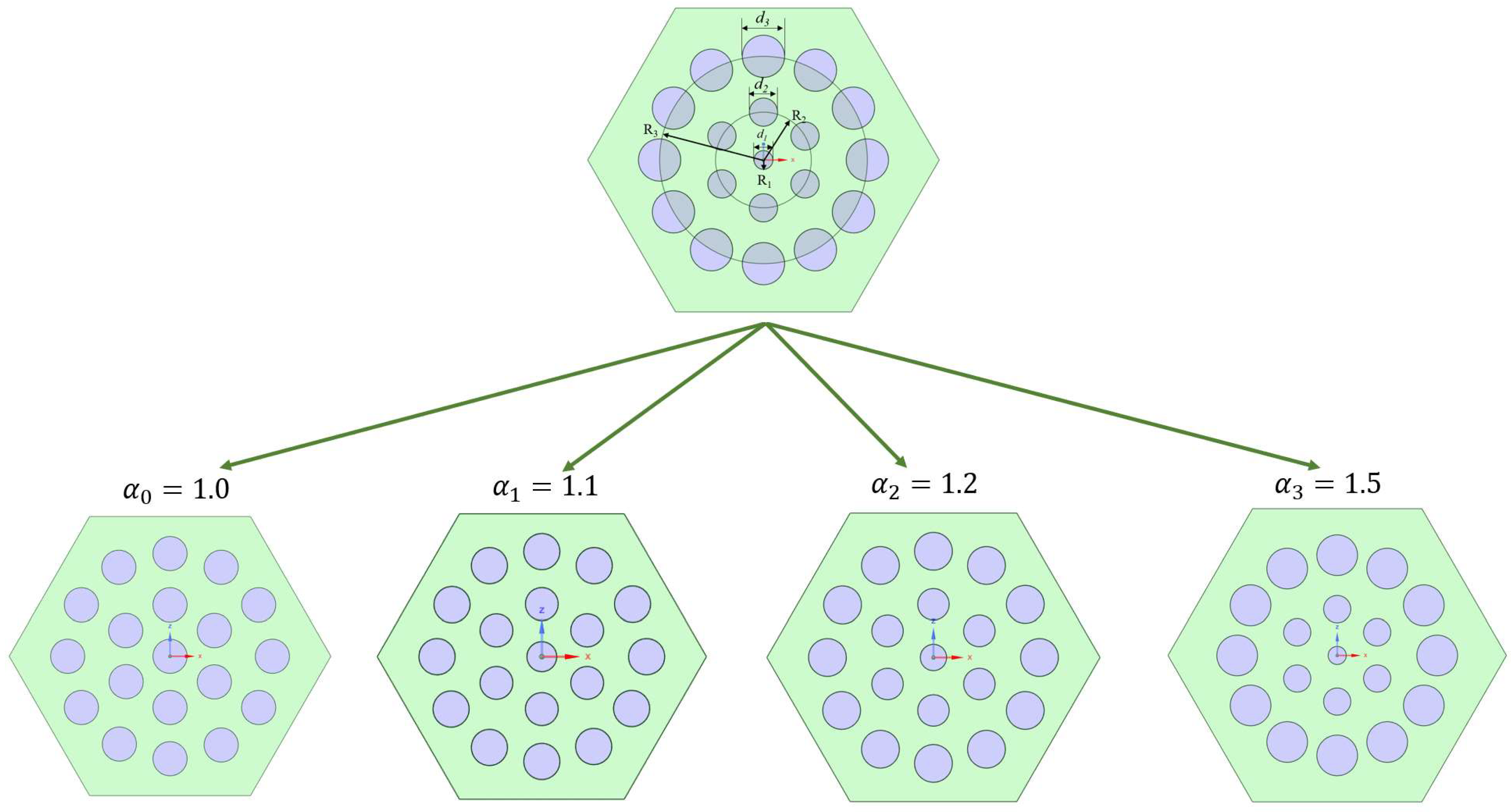




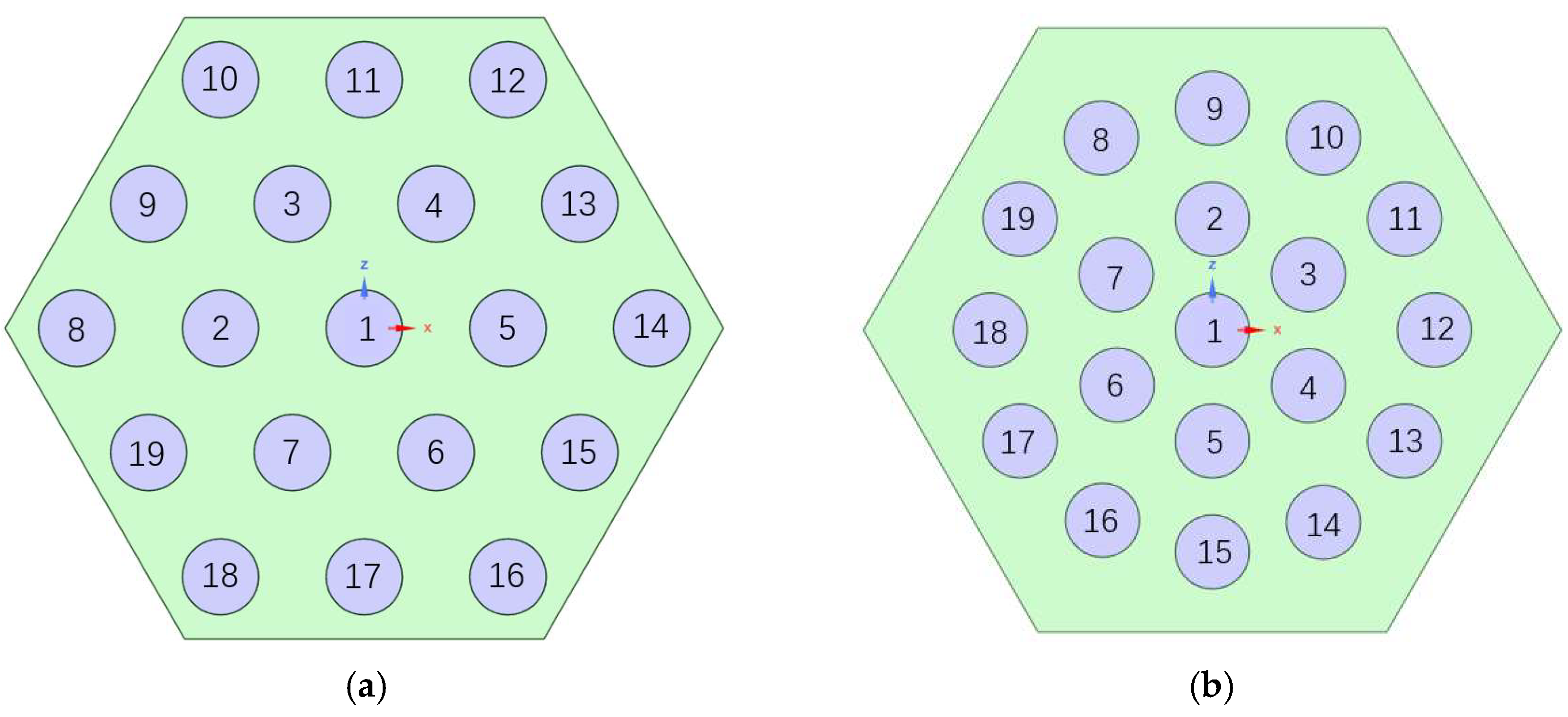

| Name | Structural Parameters | Value (mm) |
|---|---|---|
| Fuel element | Face-to-face distance | 19.05 |
| Coolant channel diameter | 2.34 | |
| Coolant channel spacing | 4.4 | |
| Element length | 1000 |
| Case | Number of Boundary Layers in Fluid Region | Surface Mesh Size of the Fluid Region\mm | Mesh Size\mm | Skewness | |
|---|---|---|---|---|---|
| 1 | 3 | 0.5 | 1.0 | 0.301 | 1.781 |
| 2 | 3 | 0.6 | 1.5 | 0.153 | 0.921 |
| 3 | 3 | 1.0 | 5.0 | 0.254 | 0.267 |
| 4 | 5 | 0.2 | 2.0 | 0.239 | 4.350 |
| d1/mm | d2/mm | d3/mm | ||
|---|---|---|---|---|
| 1.0 | 2.34 | 2.34 | 2.34 | R1 = d1; R2 = 3.5 mm; R3 = 7.0 mm |
| 1.1 | 2.00 | 2.20 | 2.42 | R1 = d1; R2 = 3.5 mm; R3 = 7.0 mm |
| 1.2 | 1.74 | 2.08 | 2.50 | R1 = d1; R2 = 3.5 mm; R3 = 7.0 mm |
| 1.5 | 1.18 | 1.77 | 2.65 | R1 = d1; R2 = 3.0 mm; R3 = 6.5 mm |
| Fuel Elements | Uniform Entrance Conditions | Nonuniform Entrance Conditions | |
|---|---|---|---|
| Conventional fuel elements | √ | √ | |
| New fuel element | √ | × | |
| √ | × | ||
| √ | × | ||
| √ | × | ||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shao, M.; Feng, S.; Guo, K.; Tong, Y.; Lin, W. A Preliminary Exploratory Study of the Flow and Heat Transfer Characteristics of Fuel Elements in Low-Enriched Uranium Cores. Aerospace 2025, 12, 290. https://doi.org/10.3390/aerospace12040290
Shao M, Feng S, Guo K, Tong Y, Lin W. A Preliminary Exploratory Study of the Flow and Heat Transfer Characteristics of Fuel Elements in Low-Enriched Uranium Cores. Aerospace. 2025; 12(4):290. https://doi.org/10.3390/aerospace12040290
Chicago/Turabian StyleShao, Mingxue, Songjiang Feng, Kangkang Guo, Yiheng Tong, and Wei Lin. 2025. "A Preliminary Exploratory Study of the Flow and Heat Transfer Characteristics of Fuel Elements in Low-Enriched Uranium Cores" Aerospace 12, no. 4: 290. https://doi.org/10.3390/aerospace12040290
APA StyleShao, M., Feng, S., Guo, K., Tong, Y., & Lin, W. (2025). A Preliminary Exploratory Study of the Flow and Heat Transfer Characteristics of Fuel Elements in Low-Enriched Uranium Cores. Aerospace, 12(4), 290. https://doi.org/10.3390/aerospace12040290






