The Optimization Design of Dynamic Similarity for the Ground Experimental System of an Aircraft Launch Unit
Abstract
1. Introduction
2. Structure of the Ground Launcher System
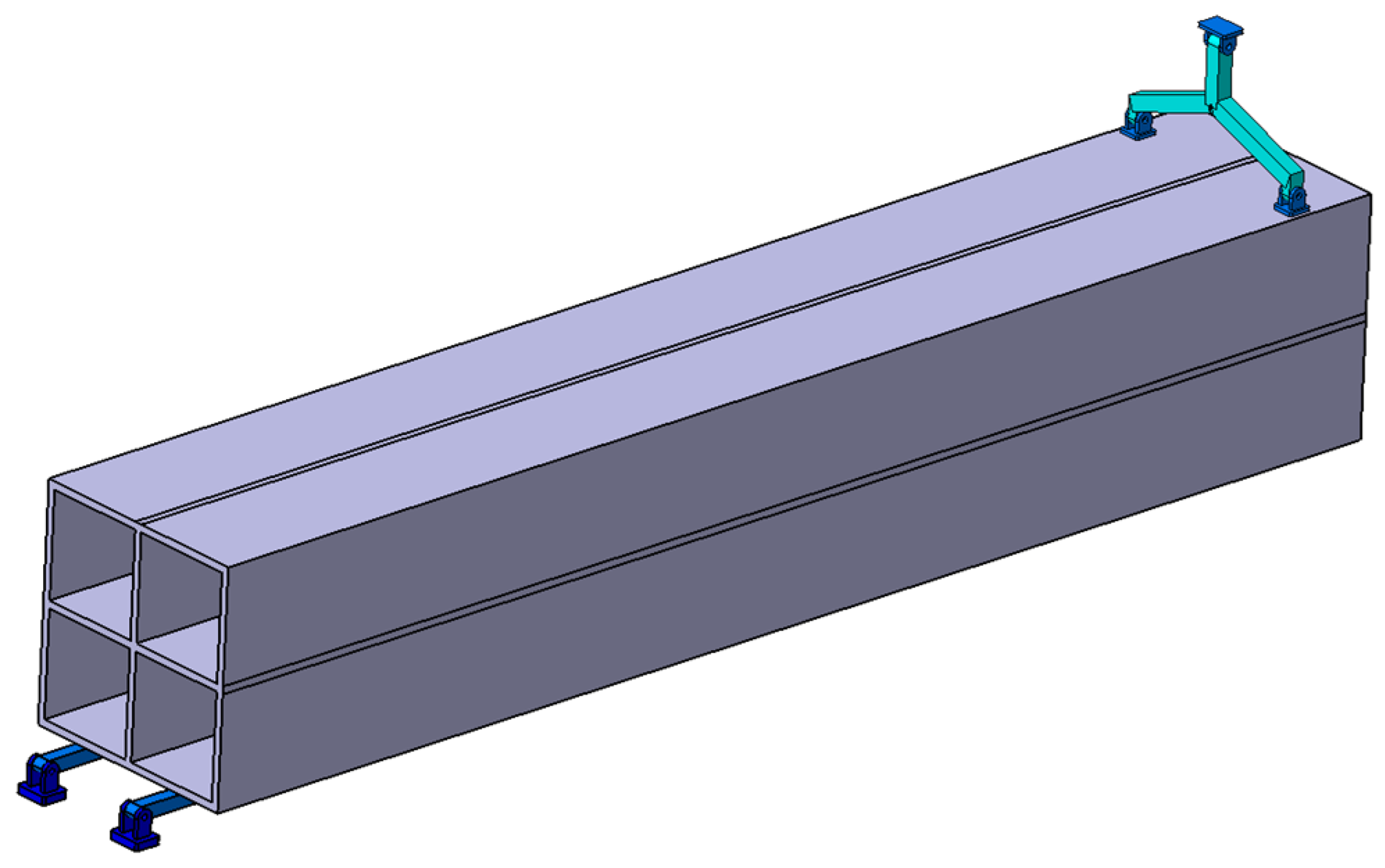
2.1. The Launcher Model (Without the Launch Unit)
2.2. The Launcher Model (with the Launch Unit)
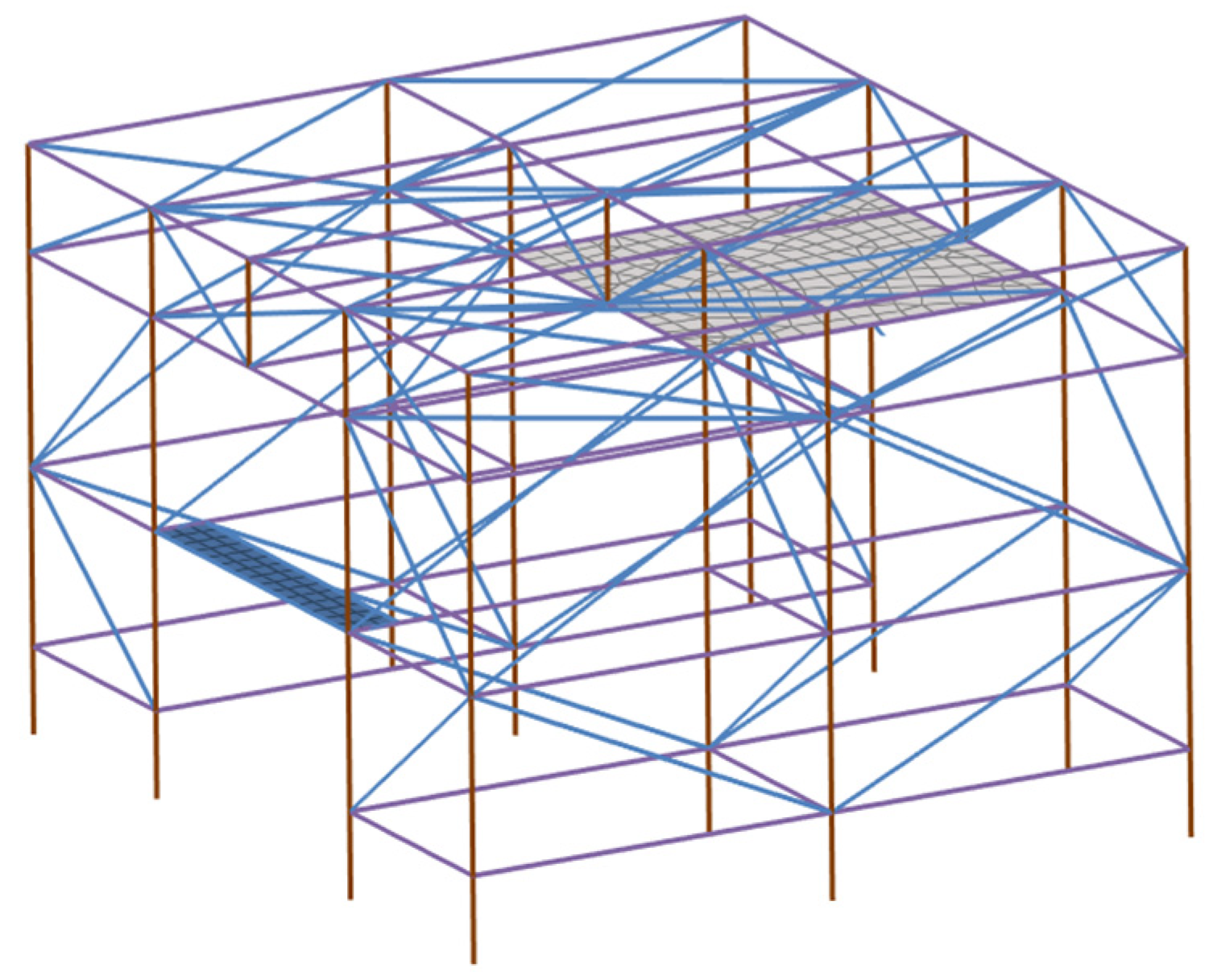
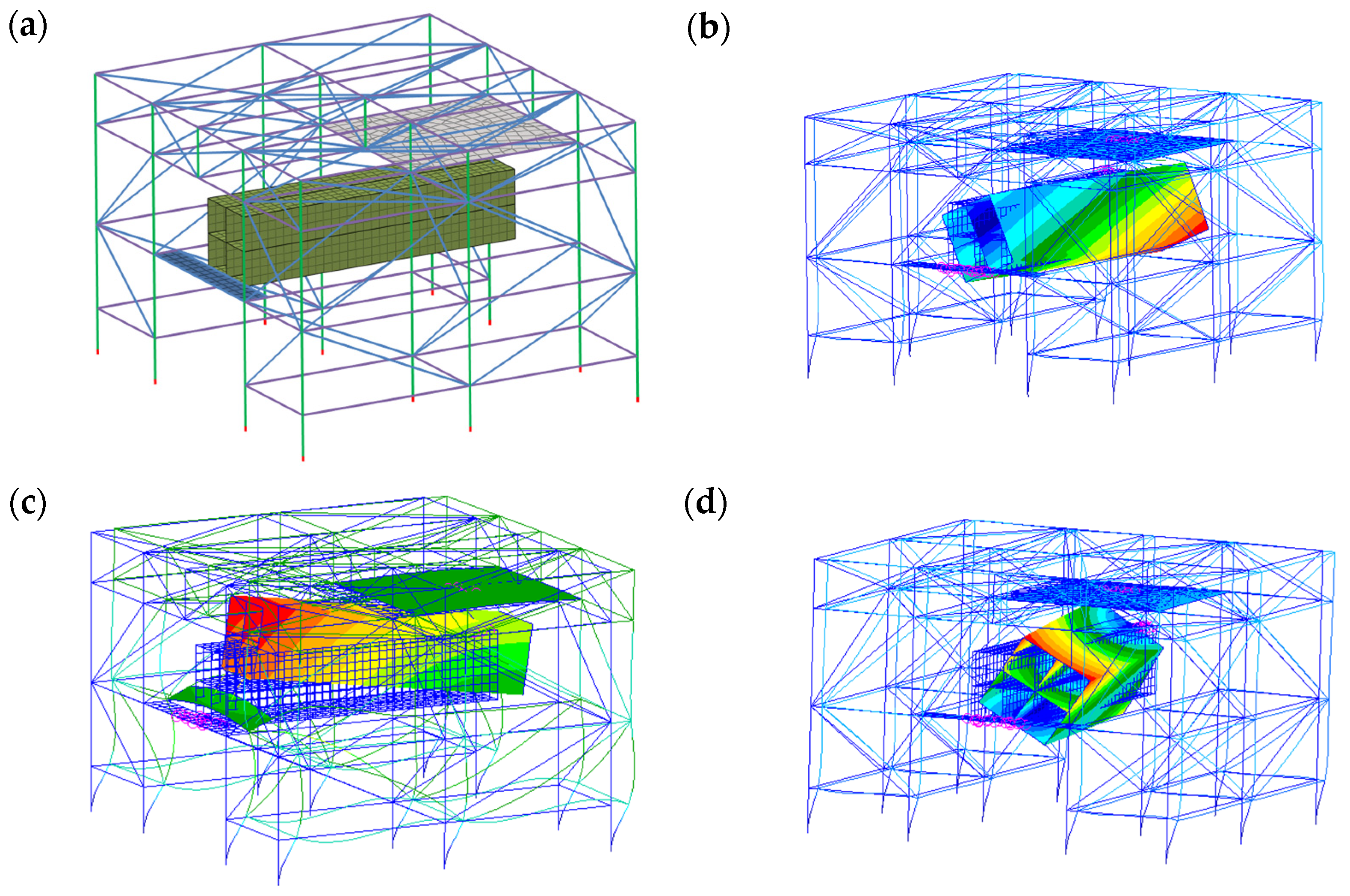
3. Structural Dynamic Characteristics of the Launcher Test System
3.1. Structural Dynamic Models
3.2. Effect of Different Ground Boundary Conditions
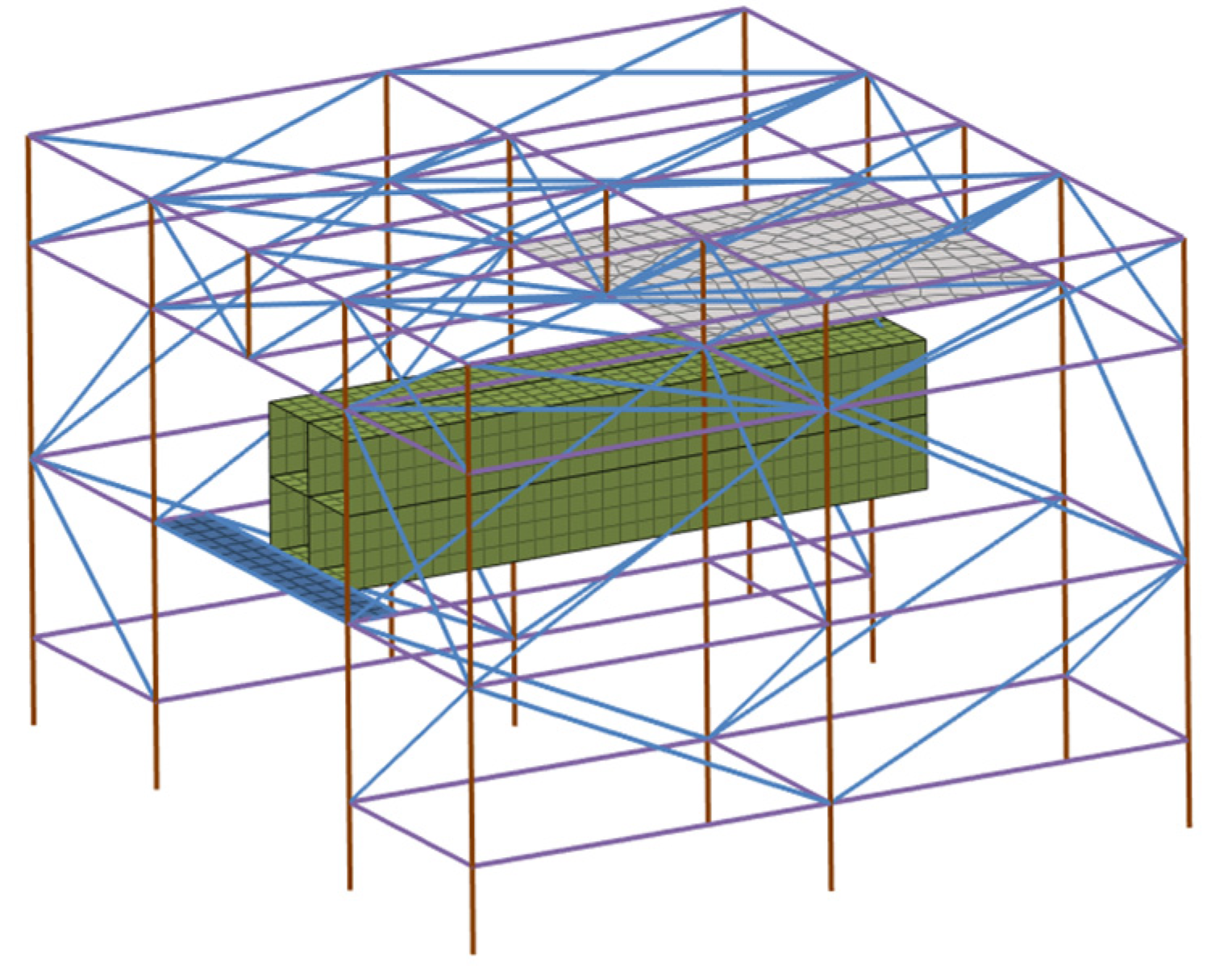
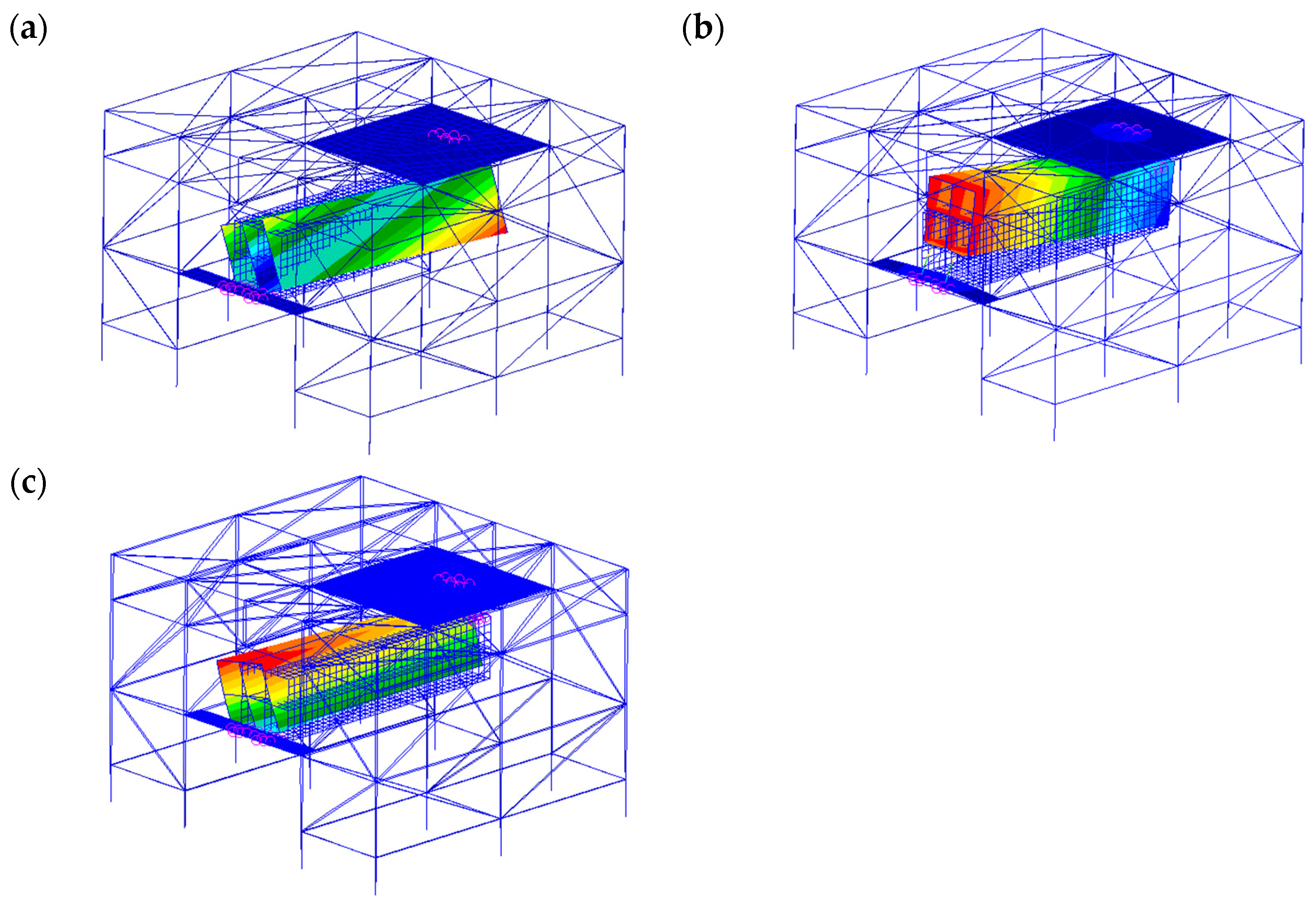
3.3. A Simulation of the Overall Structural Dynamic Characteristics of the Launcher Test System
4. Optimization Design Method for Dynamic Characteristics of Launcher Test System
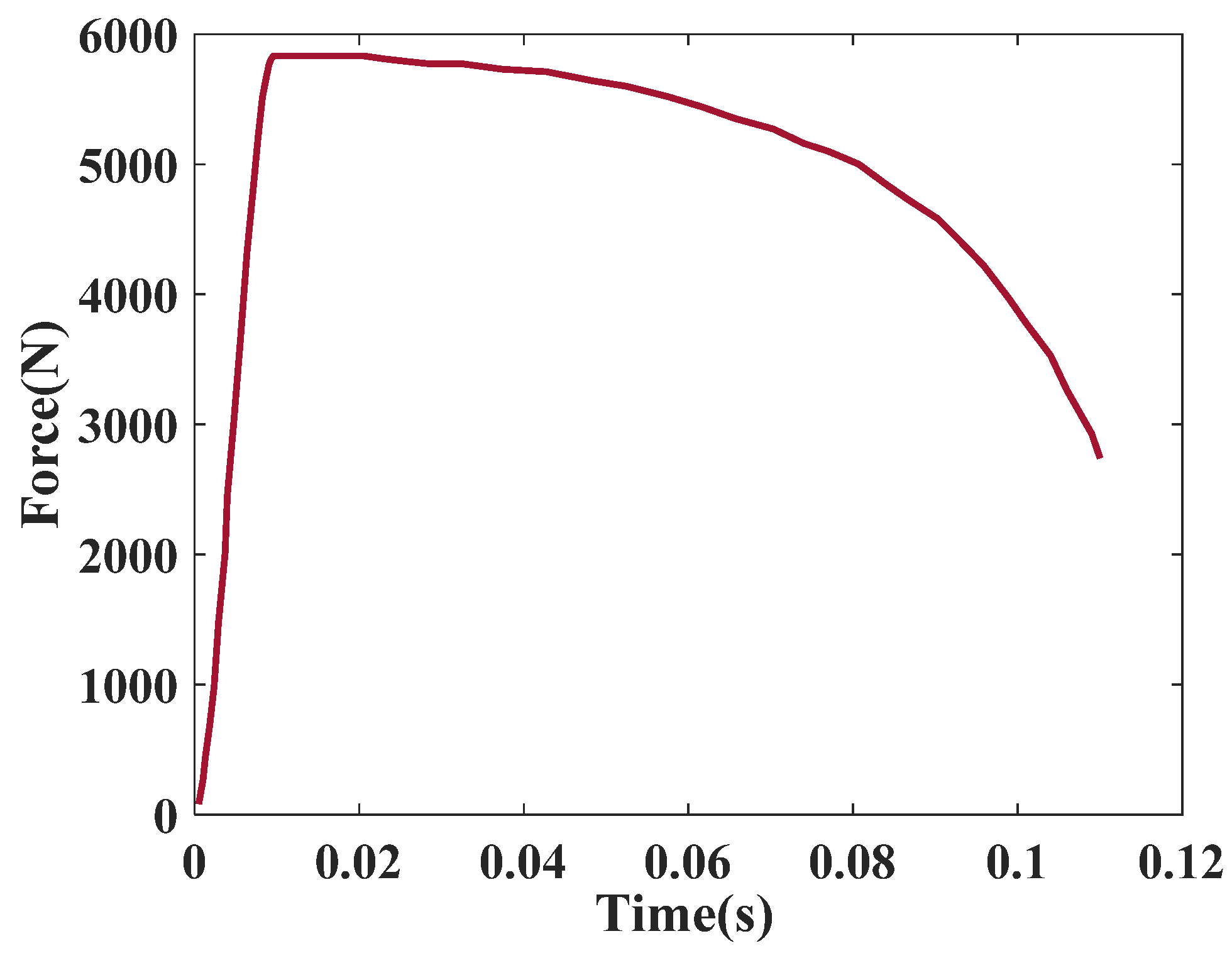
4.1. Structural Dynamics of the Launch Unit in the Airborne State
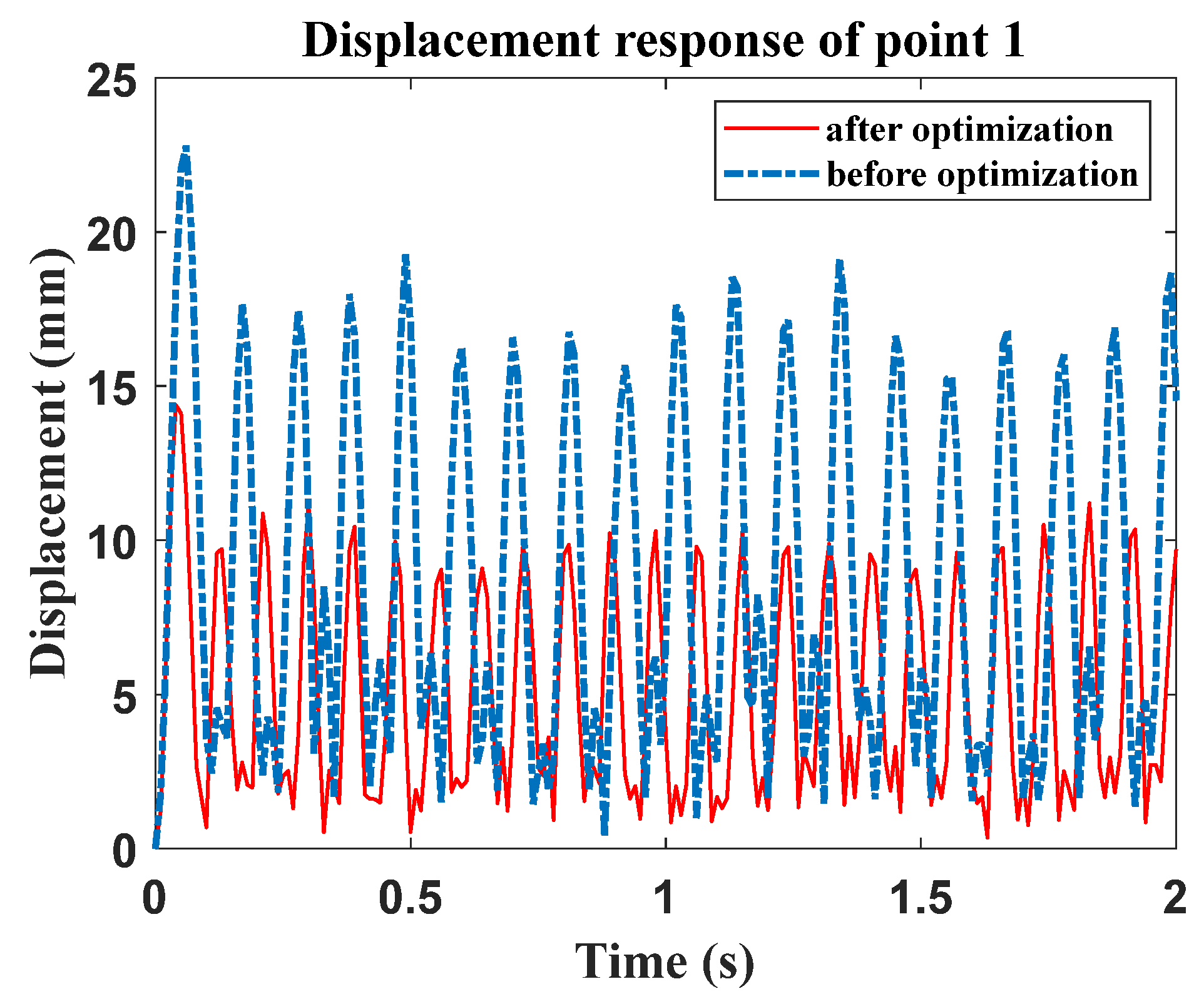
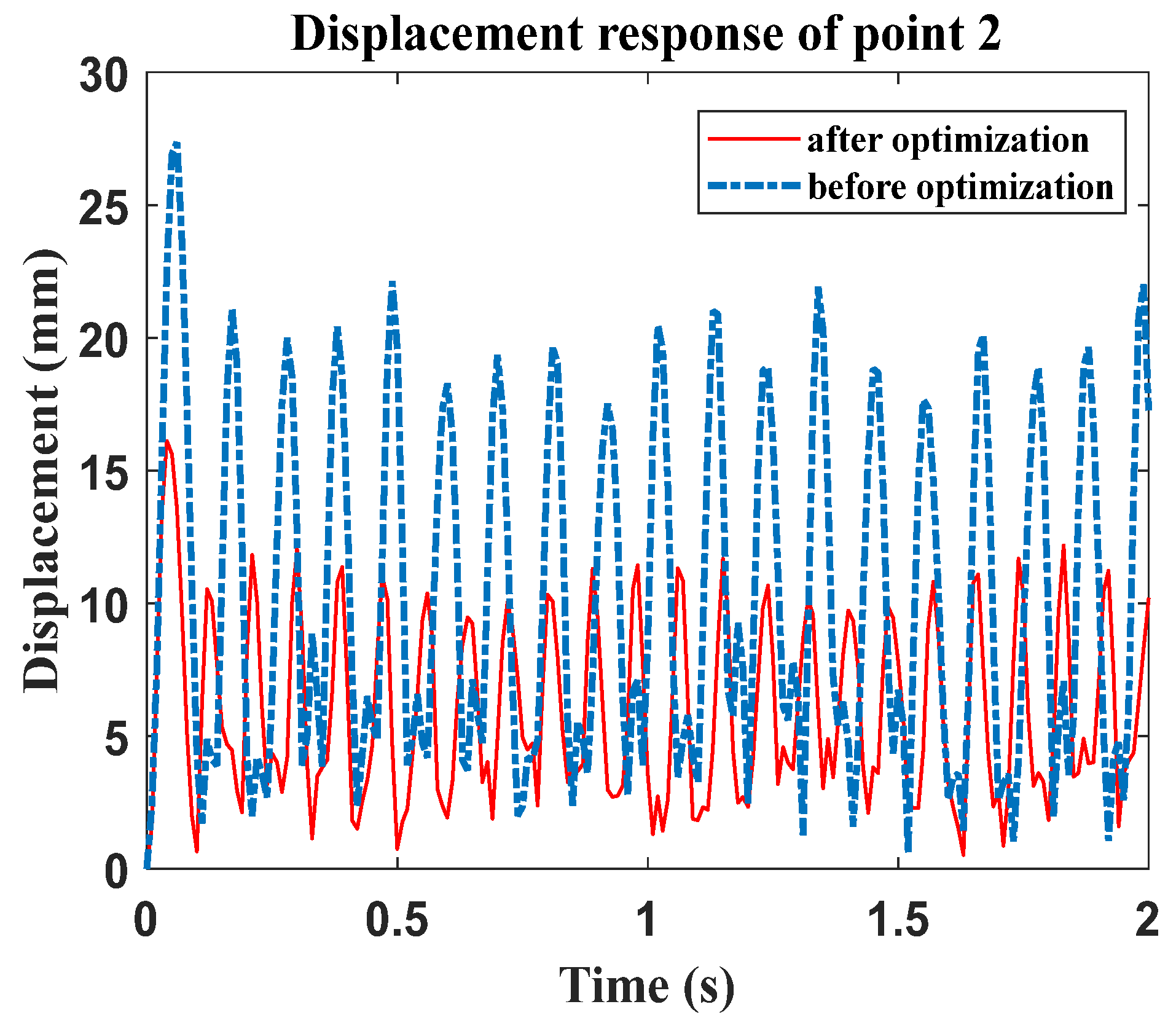
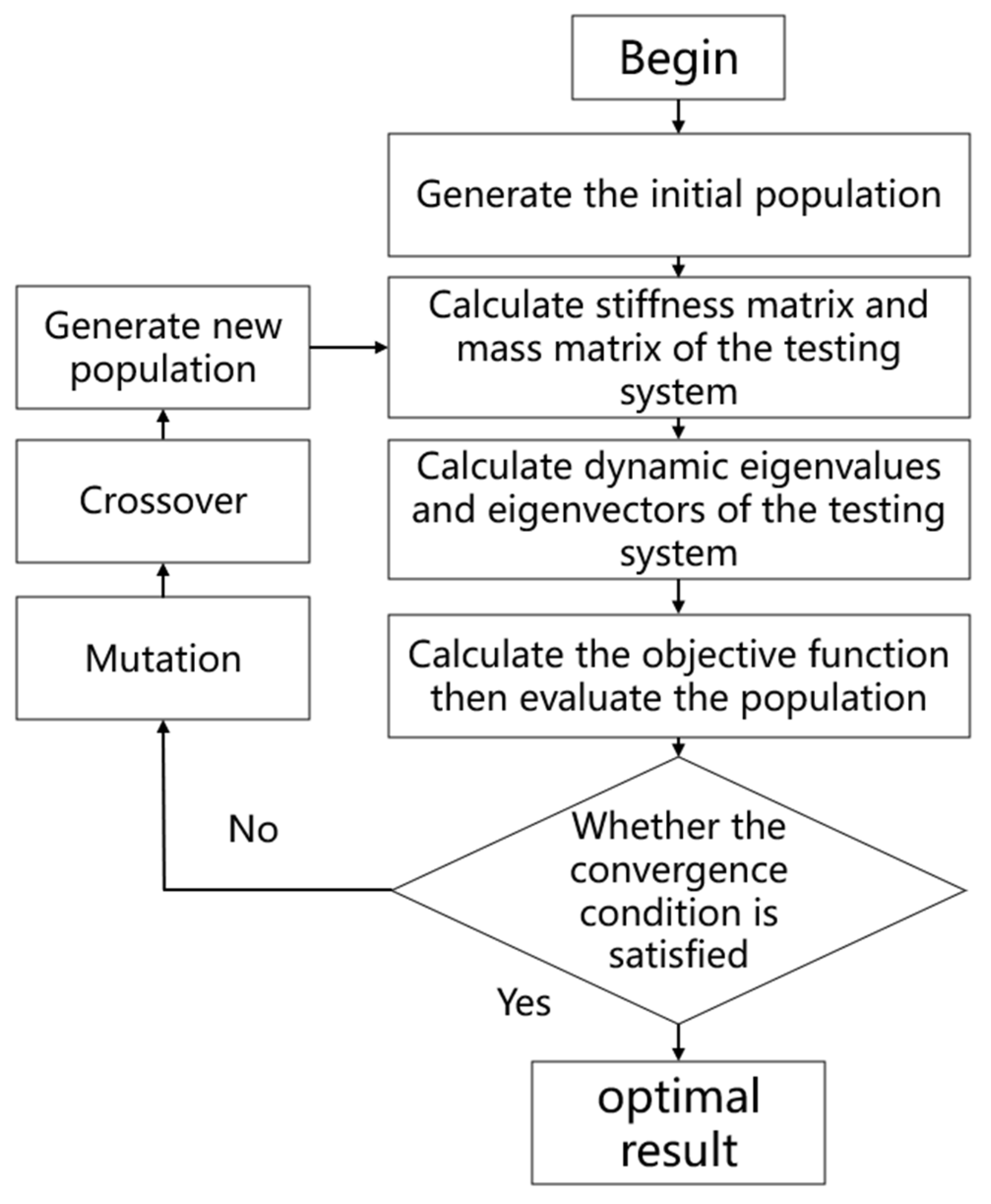
4.2. Optimization Design for the Launcher Test System
- (1)
- Randomized generation of initial populations:
- (2)
- Population variation:
- (3)
- Crossover
5. Conclusions
- (1)
- Dynamic characteristics of the ground launcher test system relies on an accurate simulation model. Different ground boundary conditions are investigated in this paper in order to find an accurate simulation model to obtain useful dynamic characteristics for the ground launcher test system.
- (2)
- An optimization design method for the ground launcher test system is proposed in this paper based on the consideration of the overall structural dynamic characteristics. Natural frequencies of the present launcher structures increase with the optimized design, meaning that they do not participate in the main vibration response of the launching unit. This does not influence the design effectiveness of testing missile launcher units when they are employed for ground launcher vibration experiments.
- (3)
- For ground experiments of airborne missile launches, an effective structural dynamic optimization method can be used to obtain structural dynamic similarity via the airborne state; thus, it is necessary to verify the airborne launch conditions through ground launching tests.
Author Contributions
Funding
Data Availability Statement
DURC Statement
Conflicts of Interest
References
- Coutinho, C.P.; Baptista, A.J.; Rodrigues, J.D. Reduced scale models based on similitude theory: A review up to 2015. Eng. Struct. 2016, 119, 81–94. [Google Scholar] [CrossRef]
- Filippou, E.; Kilimtzidis, S.; Kotzakolios, A.; Kostopoulos, V. Towards Structural and Aeroelastic Similarity in Scaled Wing Models: Development of an Aeroelastic Optimization Framework. Aerospace 2024, 11, 180. [Google Scholar] [CrossRef]
- Bantscheff, K.; Breitsamter, C. Dynamic Structural Scaling Concept for a Delta Wing Wind Tunnel Configuration Using Additive Manufacturing. Aerospace 2023, 10, 581. [Google Scholar] [CrossRef]
- Gao, G.; Shu, H.; Yi, Z.; Yang, S.; Dai, J.; Zhang, F. A Scaled Numerical Simulation Model for Structural Analysis of Large Wind Turbine Blade. Energies 2024, 17, 4849. [Google Scholar] [CrossRef]
- Cao, X.; Wang, Z.; Wang, W.; Dong, X. Application of Similarity Theory for Dynamic Characteristics Prediction of Crane Flexible Truss Boom. Math. Probl. Eng. 2020, 2020, 4273810. [Google Scholar] [CrossRef]
- Zhang, K.; Lu, F.; Peng, Y.; Li, X. Study on dynamic response of gravity dam under air blast load based on similarity law. Eng. Fail. Anal. 2022, 138, 106225. [Google Scholar] [CrossRef]
- Zhou, H.; Okada, T.; Kawamura, Y.; Wang, D.; Hayakawa, G. Improved Similarity Law for Scaling Dynamic Responses of Stiffened Plates with Distorted Stiffener Configurations. Appl. Sci. 2024, 14, 6265. [Google Scholar] [CrossRef]
- De Rosa, S.; Franco, F.; Polito, T. Structural similitudes for the dynamic response of plates and assemblies of plates. Mech. Syst. Signal Process. 2011, 25, 969–980. [Google Scholar] [CrossRef]
- Zhang, H.; Wang, L.; Wang, A. Vibration response analysis of gas generator rotor system with squeeze film damper based on dynamic similarity. Results Eng. 2023, 20, 101618. [Google Scholar] [CrossRef]
- Zhao, R.; Jiao, Y.; Qu, X. Scaling design strategy for experimental rotor systems subjected to restricted support stiffness. Appl. Math. Model. 2022, 109, 265–282. [Google Scholar] [CrossRef]
- Fan, X.; Liang, J.; Wang, K.; Zhou, H. Parallel Calculation of Random Vibration for Reentry Vehicles in Fluctuating Pressure Environment. Shock Vib. 2021, 2021, 6864973. [Google Scholar] [CrossRef]
- Guo, L.; Ge, J.; Liu, S. Analysis of Vibration and Acoustic Characteristics of a Simply Supported Double-Panel Partition under Thermal Environment. Shock Vib. 2020, 2020, 5613232. [Google Scholar] [CrossRef]
- Liu, J.; Sun, X.; Zhang, X.; Hou, X. Research on torsional vibration characteristics of reciprocating compressor shafting and dynamics modification. Mech. Adv. Mater. Struct. 2020, 27, 687–696. [Google Scholar] [CrossRef]
- Yang, Z.; Yang, C.; Zhao, J.; Wu, Z. Fluid–Structure Interaction Dynamic Response of Rocket Fairing in Falling Phase. Aerospace 2022, 9, 741. [Google Scholar] [CrossRef]
- Hu, Y.; Dai, Y.; Wu, Y.; Yang, C. Time-Domain Feedforward Control for Gust Response Alleviation Based on Seamless Morphing Wing. AIAA J. 2022, 60, 5707–5722. [Google Scholar] [CrossRef]
- Storn, R.; Price, K. Differential Evolution—A Simple and Efficient Heuristic for global Optimization over Continuous Spaces. J. Glob. Optim. 1997, 11, 341–359. [Google Scholar] [CrossRef]
- Moosavian, H.; Mesbahi, P.; Moosavian, N.; Daliri, H. Optimal design of truss structures with frequency constraints: A comparative study of DE, IDE, LSHADE, and CMAES algorithms. Eng. Comput. 2021, 39, 1499–1517. [Google Scholar] [CrossRef]
- Bozyigit, B.; Bozyigit, I.; Prendergast, L.J. Analytical approach for seismic analysis of onshore wind turbines considering soil-structure interaction. Structures 2023, 51, 226–241. [Google Scholar] [CrossRef]
- Kardooni, M.R.; Shishesaz, M.; Moradi, S.; Mosalmani, R. Free Vibrational Analysis of a Functionally Graded Five-Layer Sandwich Plate Resting on a Winkler Elastic Foundation in a Thermal Environment. J. Compos. Sci. 2022, 6, 325. [Google Scholar] [CrossRef]









| Material | Elasticity/GPa | Poisson Ratio | Density/(kg·m−3) |
|---|---|---|---|
| Al | 70 | 0.30 | 2800 |
| Steel | 210 | 0.33 | 7800 |
| Mode | Simulation Results | Experimental Results | ||
|---|---|---|---|---|
| Fully Fixed Support | = 105,920 (N/m3) | = 45,000 () | ||
| 1 | 8.71 | 8.59 | 8.45 | 8.43 |
| 2 | 9.86 | 9.79 | 9.70 | 9.66 |
| 3 | 10.53 | 10.36 | 10.20 | 10.17 |
| Mode | State of the Ground Experiment/Hz | Airborne State/Hz | Difference Before Optimization | Difference After Optimization | |
|---|---|---|---|---|---|
| Before Optimization | After Optimization | ||||
| 1 | 8.45 | 9.96 | 9.32 | 9.3% | 6.4% |
| 2 | 9.70 | 11.85 | 12.35 | 21.5% | 4.2% |
| 3 | 10.20 | 16.02 | 16.62 | 36.3% | 3.7% |
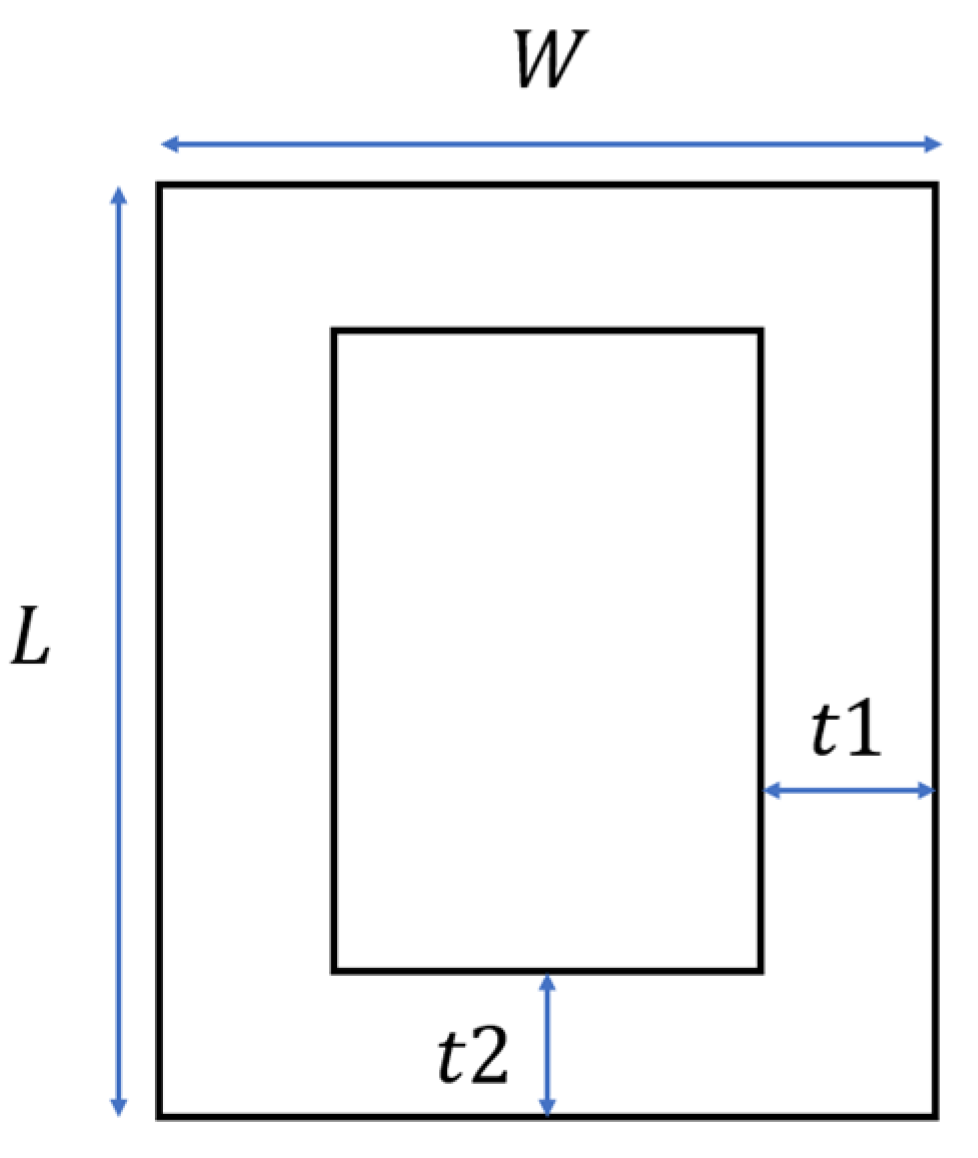
| Structural Parameters | Before Optimization (mm) | After Optimization (mm) |
|---|---|---|
| Width of front mounted structure | 20.00 | 76.16 |
| Height of front mounted structure | 20.00 | 30.58 |
| Thickness of front mount structure | 5.00 | 6.38 |
| Thickness of front mount structure | 5.00 | 3.70 |
| Width of rear mounted structure | 30.00 | 30.04 |
| Height of rear mounted structure | 30.00 | 88.34 |
| Thickness of rear mount structure | 6.00 | 4.39 |
| Thickness of rear mount structure | 6.00 | 3.79 |
| Thickness of upper connection plate | 30.00 | 35.43 |
| Thickness of lower connection plate | 30.00 | 16.92 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, S.; Cai, L.; Cao, Y.; Bai, Y.; Zhang, X.; Huang, H. The Optimization Design of Dynamic Similarity for the Ground Experimental System of an Aircraft Launch Unit. Aerospace 2025, 12, 276. https://doi.org/10.3390/aerospace12040276
Zhang S, Cai L, Cao Y, Bai Y, Zhang X, Huang H. The Optimization Design of Dynamic Similarity for the Ground Experimental System of an Aircraft Launch Unit. Aerospace. 2025; 12(4):276. https://doi.org/10.3390/aerospace12040276
Chicago/Turabian StyleZhang, Sheng, Lin Cai, Yushun Cao, Yuguang Bai, Xiaoshi Zhang, and Hu Huang. 2025. "The Optimization Design of Dynamic Similarity for the Ground Experimental System of an Aircraft Launch Unit" Aerospace 12, no. 4: 276. https://doi.org/10.3390/aerospace12040276
APA StyleZhang, S., Cai, L., Cao, Y., Bai, Y., Zhang, X., & Huang, H. (2025). The Optimization Design of Dynamic Similarity for the Ground Experimental System of an Aircraft Launch Unit. Aerospace, 12(4), 276. https://doi.org/10.3390/aerospace12040276






