Modelling of the Power Demand of Peripheral Aggregates of an Airborne Fuel Cell-Based Power System †
Abstract
1. Introduction
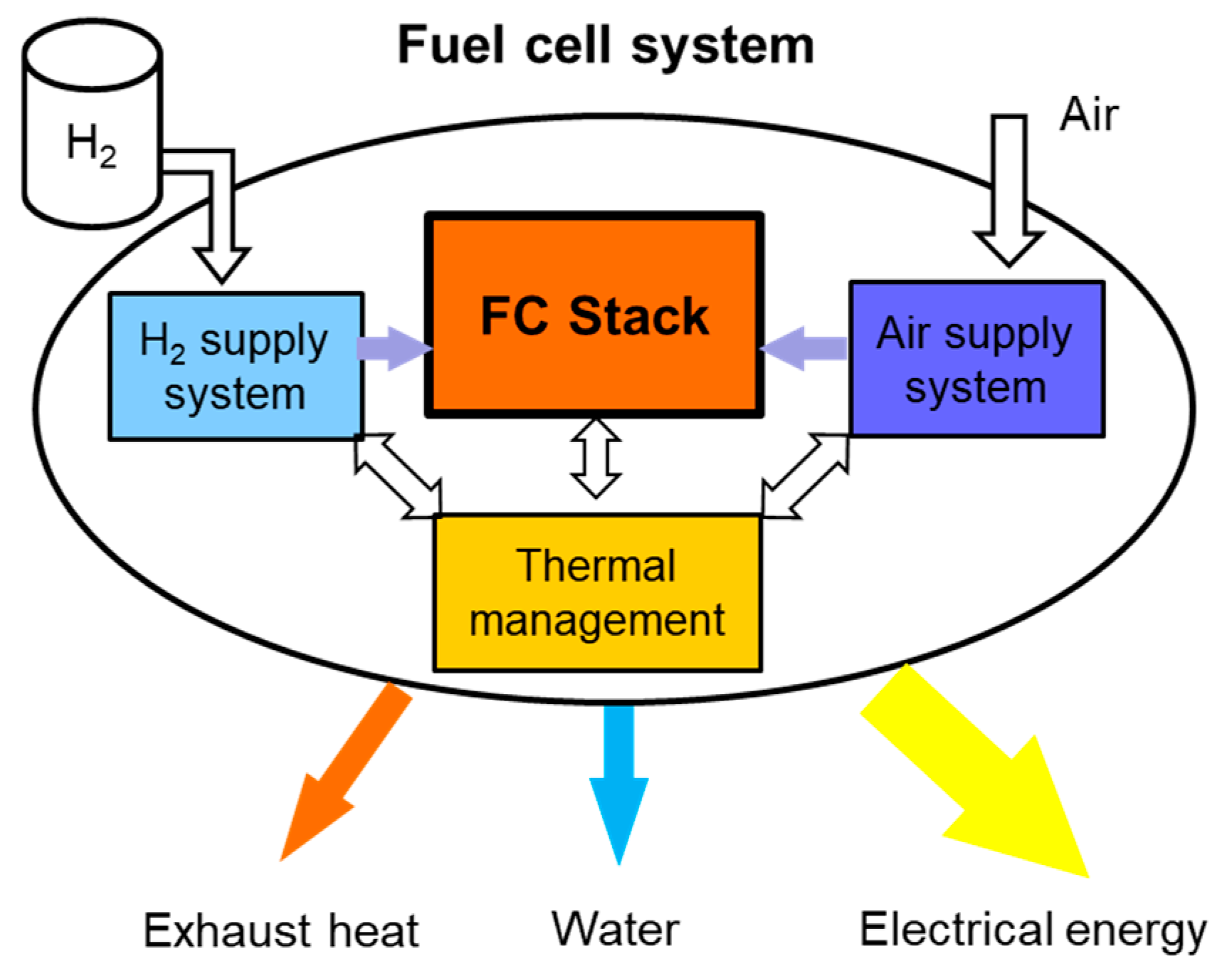
2. Balance of Plant in Fuel Cell Systems
- Hydrogen supply
- Air supply
- Thermal and water management
3. Modelling of Power Demand of Peripheral Aggregates
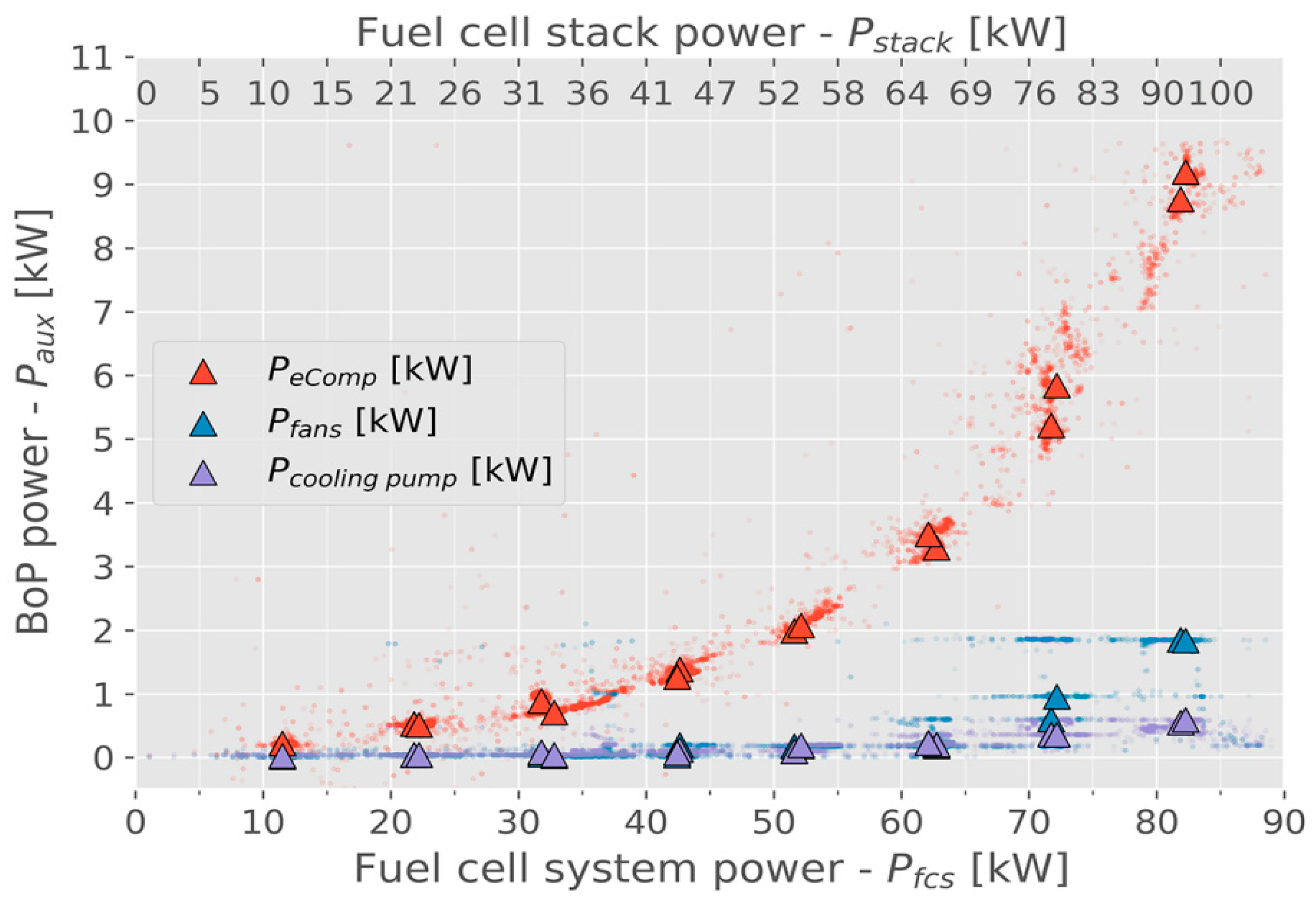
3.1. Deriving BoP Power Demand Based on Experimental Data
3.2. Contribution of Individual BoP Consumer Groups
- Hydrogen supply
- Air supply
- Thermal and water management
- Turbo-compressor (a turbo-compressor is a combination of a compressor and a turbine, which increases the efficiency of the compressor. Both the compressor and the turbine are mounted on the same shaft, and are driven by an electrical motor)
- Cooling pumps
- Fans
- The electric turbo-compressor in the air supply system is a major power consumer, consuming over 80% of the PBoP on average.
- The power consumed by the air supply system is strongly dependent on the power generated by the fuel cell stack.
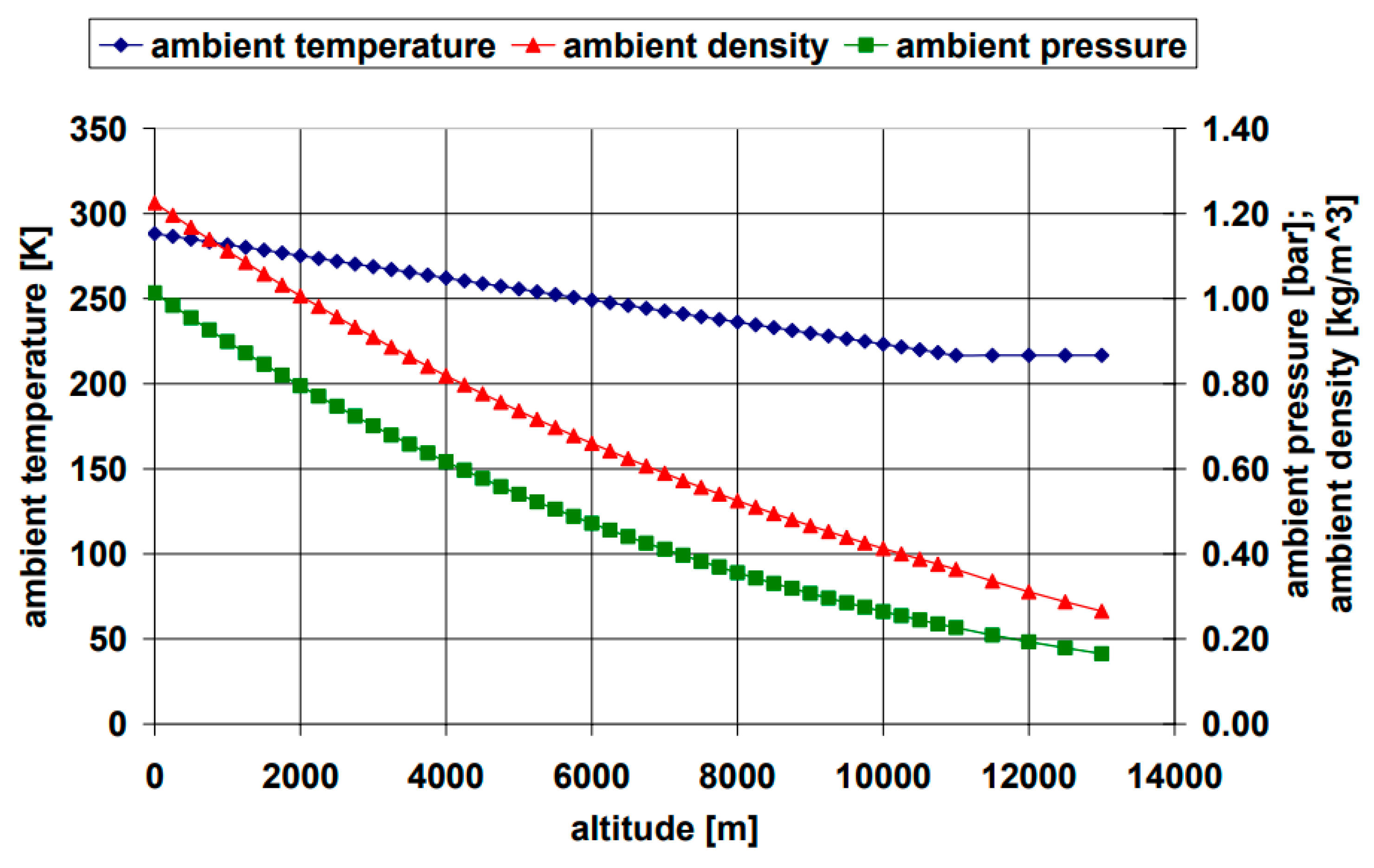
4. Effect of Altitude on Environmental Conditions
4.1. Dependency of Peripheral Aggregates on Environmental Conditions
4.1.1. Hydrogen Supply System and Thermal Management System
4.1.2. Air Supply System
5. Conclusions
- The balance of plant power consumption of a fuel cell system is a third-order polynomial function of the fuel cell power, rather than the linear function presented in the literature [9].
- The air supply system contributes to about 80% of the total BoP power consumption.
- To maintain the fuel cell power generation at a constant level throughout the entire flight mission, the air supply system should be designed for high-altitude conditions. This means that the rated power of the air supply system should be 200% of the amount of power required at ground level.
- At higher altitudes, the ambient pressure and temperature both decrease, but they affect the compressor power demand in opposite ways. However, the effect of the ambient pressure is stronger than that of the ambient temperature.
- At high altitudes, thermal management devices (pumps and fans) consume slightly less power, but this is not enough to compensate for the additional power consumption of the air supply system.
- High altitude has no effect on the power consumption of the hydrogen supply system.
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| BoP | Balance of plant |
| FC | Fuel cell |
| LH2 | Liquid hydrogen |
| LHV | Hydrogen higher heating value |
| PEM | Proton exchange membrane |
References
- Publications Office of the European Union. FlightPath 2050. Available online: https://op.europa.eu/en/publication-detail/-/publication/296a9bd7-fef9-4ae8-82c4-a21ff48be673 (accessed on 14 February 2025).
- Peters, R. Brennstoffzellensysteme in der Luftfahrt; Springer: Berlin/Heidelberg, Germany, 2015. [Google Scholar]
- Colpan, C.O.; Kovač, A. Fuel Cell and Hydrogen Technologies in Aviation; Springer: Berlin/Heidelberg, Germany, 2022; ISBN 978-3-030-99017-6. [Google Scholar]
- Mc Kinsey: Hydrogen-Powered Aviation; A Fact-Based Study of Hydrogen Technology, Economics, and Climate Impact by 2050; Publications Office of the European Union: Luxembourg, 2020; Available online: https://data.europa.eu/doi/10.2843/471510 (accessed on 10 February 2025).
- Karpuk, S.; Freund, Y.; Hanke-Rauschenbach, R. Potential of Hydrogen Fuel Cell Aircraft for Commercial Applications with Advanced Airframe and Propulsion Technologies. Aerospace 2025, 12, 35. [Google Scholar] [CrossRef]
- Schmelcher, M.; Häßy, J. Hydrogen Fuel Cells for Aviation? International Society of Air Breathing Engines (ISABE), Proceedings, 2022. Available online: https://elib.dlr.de/188835/1/ISABE_2022_291_full.pdf (accessed on 14 February 2025).
- Klell, M.; Eichlseder, H.; Trattner, A. Wasserstoff in der Fahrzeugtechnik, 4th ed.; Springer Verlag: Berlin, Germany, 2018. [Google Scholar]
- De Maglie, R.; Mahdavi, N.; Dejan, J. Electronic Controlled Turbo-Compressor for Fuel Cell Air Supply System. In Proceedings of the Towards Sustainable Aviation Summit (TSAS2022), Toulouse, France, 18–20 October 2022. [Google Scholar]
- Jarry, T.; Lacressonnière, F.; Jaafar, A.; Turpin, C.; Scohy, M. Modeling and Sizing of a Fuel Cell—Lithium-Ion Battery Direct Hybridization System for Aeronautical Application. Energies 2021, 14, 7655. [Google Scholar] [CrossRef]
- Sery, J.; Leduc, P. Fuel Cell Behavior and Energy Balance on Board a Hyundai Nexo. Int. J. Engine Res. 2022, 23, 709–720. [Google Scholar] [CrossRef]
- Argonne National Laboratory. Technology Assessment of a Fuel Cell Vehicle: 2017 Toyota Mirai; Report # ANL/ESD-18/12; Argonne National Laboratory: Argonne, IL, USA, 2018. [Google Scholar] [CrossRef]
- Barchewitz, L. In-Flight Air Supply System for PEM Fuel Cells. Ph.D. Thesis, Gottfried Wilhelm Leibniz University Hannover, Hanover, Germany, 2008. [Google Scholar]
- Blunier, B.; Miraoui, A. Air Management in PEM Fuel Cells: State-of-the-Art and Prospectives. In Proceedings of the International Aegean Conference on Electrical Machines and Power Electronics, Bodrum, Turkey, 10–12 September 2007. [Google Scholar]
- Fattoruso, O. Study and Modelling of a Fuel Cell Air Supply System with Turbocharger for a Regional Hybrid Electric Aircraft. Master’s Thesis, Politecnico di Torino, Torino, Italy, October 2023. [Google Scholar]
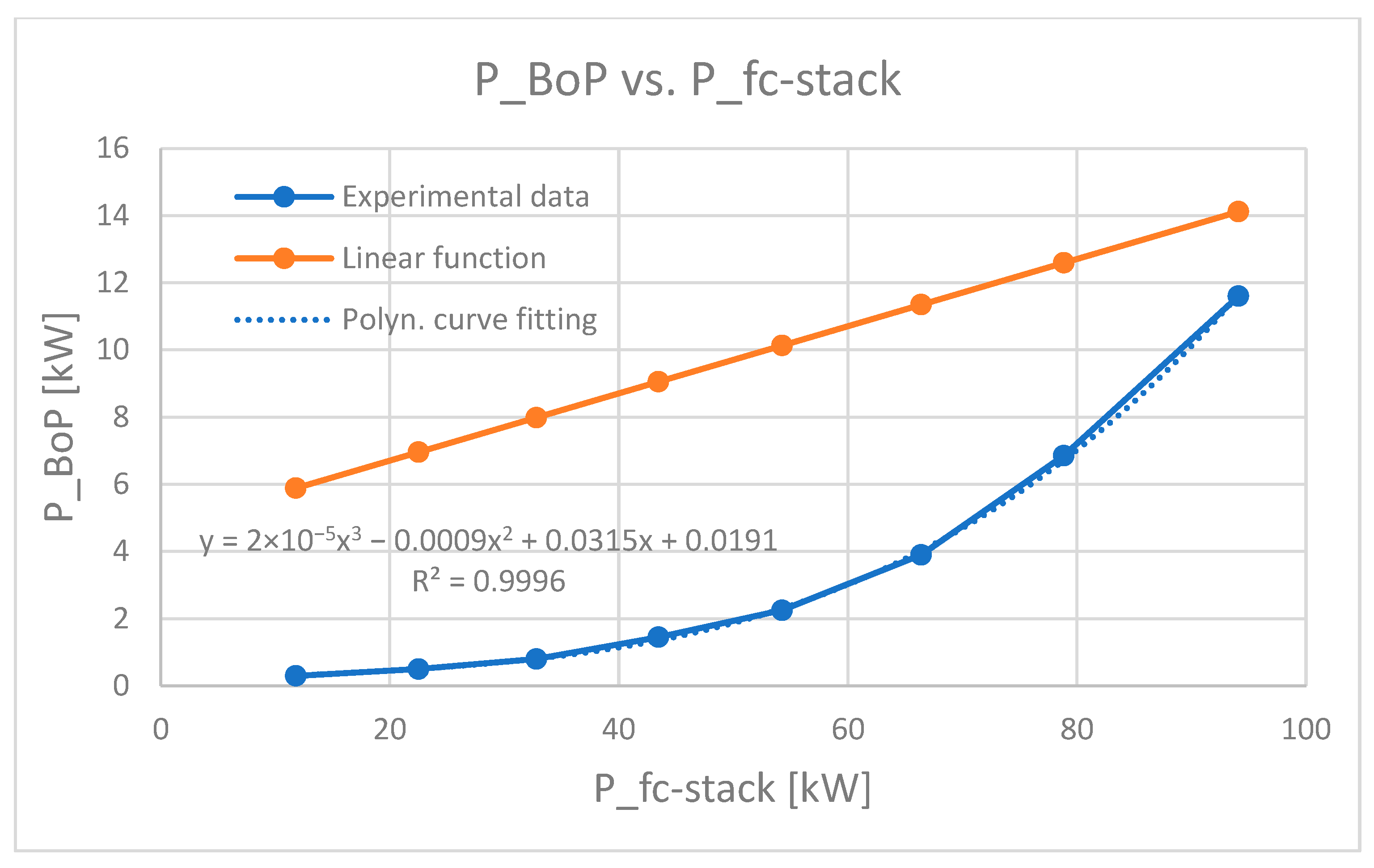
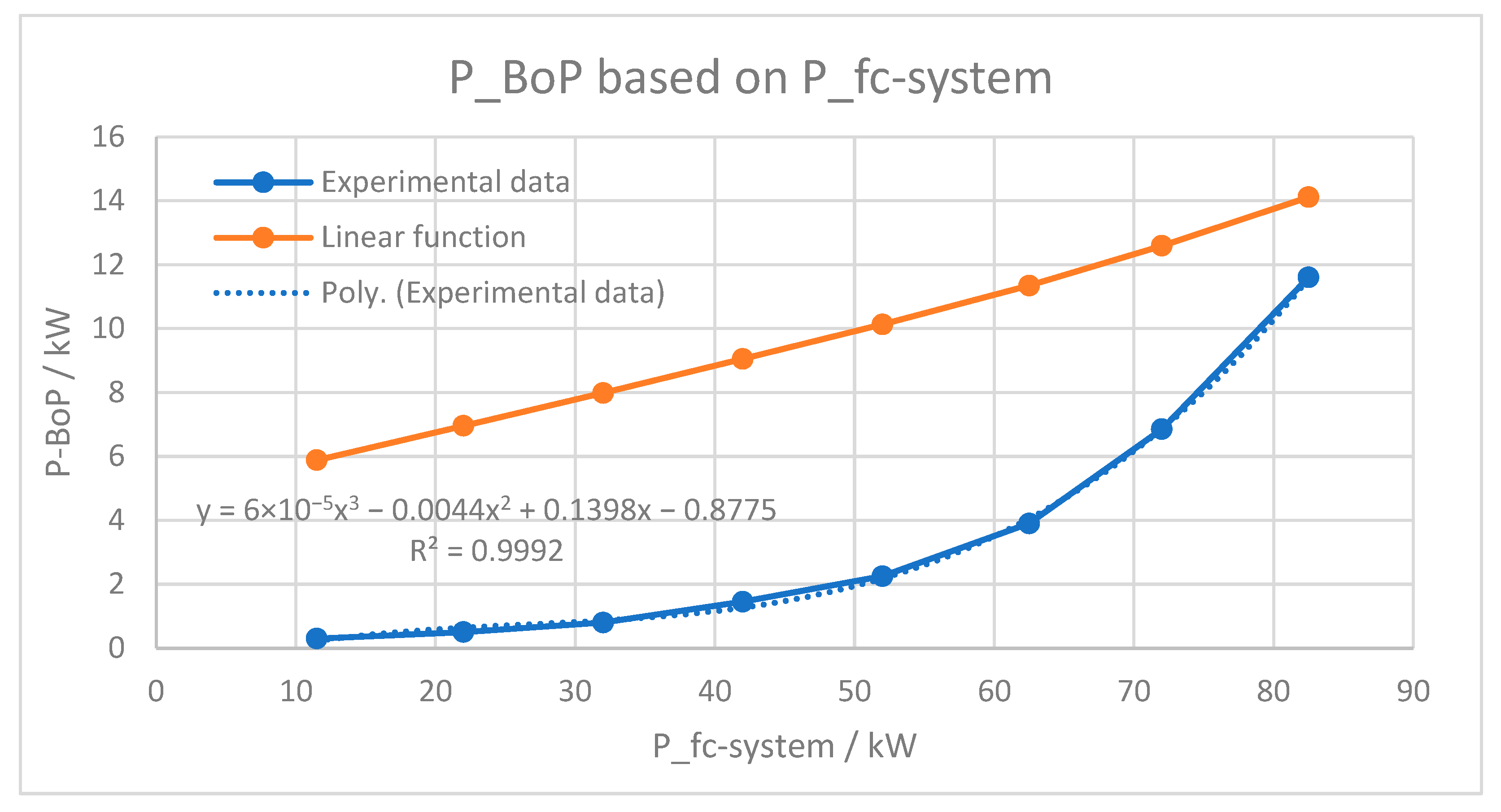

| PFC-St [kW] | PFC-Sys [kW] | PBoP [kW] from Figure 2 | PBoP [kW] from Equation (3) |
|---|---|---|---|
| 11.80 | 11.5 | 0.3 | 5.89 |
| 22.50 | 22.0 | 0.5 | 6.96 |
| 32.80 | 32.0 | 0.8 | 7.99 |
| 43.45 | 42.5 | 1.45 | 9.05 |
| 54.25 | 52.0 | 2.25 | 10.13 |
| 66.40 | 62.5 | 3.9 | 11.35 |
| 78.85 | 72.0 | 6.85 | 12.59 |
| 94.10 | 82.5 | 11.6 | 14.12 |
| PBoP [kW] | PComp [kW] | PComp [%] | PFans [kW] | PFans [%] | PPumps [kW] | PPomps [%] |
|---|---|---|---|---|---|---|
| 0.30 | 0.25 | 83% | 0.03 | 10% | 0.02 | 7% |
| 0.50 | 0.45 | 90% | 0.03 | 6% | 0.02 | 4% |
| 0.80 | 0.70 | 88% | 0.05 | 6% | 0.05 | 6% |
| 1.45 | 1.25 | 86% | 0.10 | 7% | 0.10 | 7% |
| 2.25 | 1.95 | 87% | 0.20 | 9% | 0.10 | 4% |
| 3.90 | 3.35 | 86% | 0.40 | 10% | 0.15 | 4% |
| 6.85 | 5.70 | 83% | 0.80 | 12% | 0.35 | 5% |
| 11.60 | 9.30 | 80% | 1.80 | 16% | 0.50 | 4% |
| Altitude [m] | Pressure [bar] | Temperature [K] | Pc [kW] | Pt [kW] | Pm-tc [kW] |
|---|---|---|---|---|---|
| 0 | 1.01 | 288.15 | 109.15 | 42.96 | 66.19 |
| 1000 | 0.90 | 281.65 | 124.50 | 51.07 | 73.43 |
| 2000 | 0.79 | 275.15 | 140.07 | 59.09 | 80.98 |
| 3000 | 0.70 | 268.65 | 155.86 | 67.02 | 88.85 |
| 4000 | 0.62 | 262.15 | 171.89 | 74.84 | 97.05 |
| 5000 | 0.54 | 255.65 | 188.18 | 82.58 | 105.60 |
| 6000 | 0.47 | 249.15 | 204.74 | 90.21 | 114.53 |
| 7000 | 0.41 | 242.65 | 221.58 | 97.74 | 123.84 |
| 8000 | 0.36 | 236.15 | 238.74 | 105.18 | 133.56 |
| 9000 | 0.31 | 229.65 | 256.22 | 112.51 | 143.71 |
| Power Consumption on Ground | Power Consumption at High Altitudes | |
|---|---|---|
| Air supply system | high | too high (up to 200%) |
| Hydrogen supply system | very low | very low |
| Thermal and water management | moderate | low |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mahdavi, N. Modelling of the Power Demand of Peripheral Aggregates of an Airborne Fuel Cell-Based Power System. Aerospace 2025, 12, 234. https://doi.org/10.3390/aerospace12030234
Mahdavi N. Modelling of the Power Demand of Peripheral Aggregates of an Airborne Fuel Cell-Based Power System. Aerospace. 2025; 12(3):234. https://doi.org/10.3390/aerospace12030234
Chicago/Turabian StyleMahdavi, Nejat. 2025. "Modelling of the Power Demand of Peripheral Aggregates of an Airborne Fuel Cell-Based Power System" Aerospace 12, no. 3: 234. https://doi.org/10.3390/aerospace12030234
APA StyleMahdavi, N. (2025). Modelling of the Power Demand of Peripheral Aggregates of an Airborne Fuel Cell-Based Power System. Aerospace, 12(3), 234. https://doi.org/10.3390/aerospace12030234







