Exploration of Earth’s Magnetosphere Using CubeSats with Electric Propulsion
Abstract
1. Introduction
2. Description and Modeling of CubeSat
2.1. Electric Thruster Mathematical Model
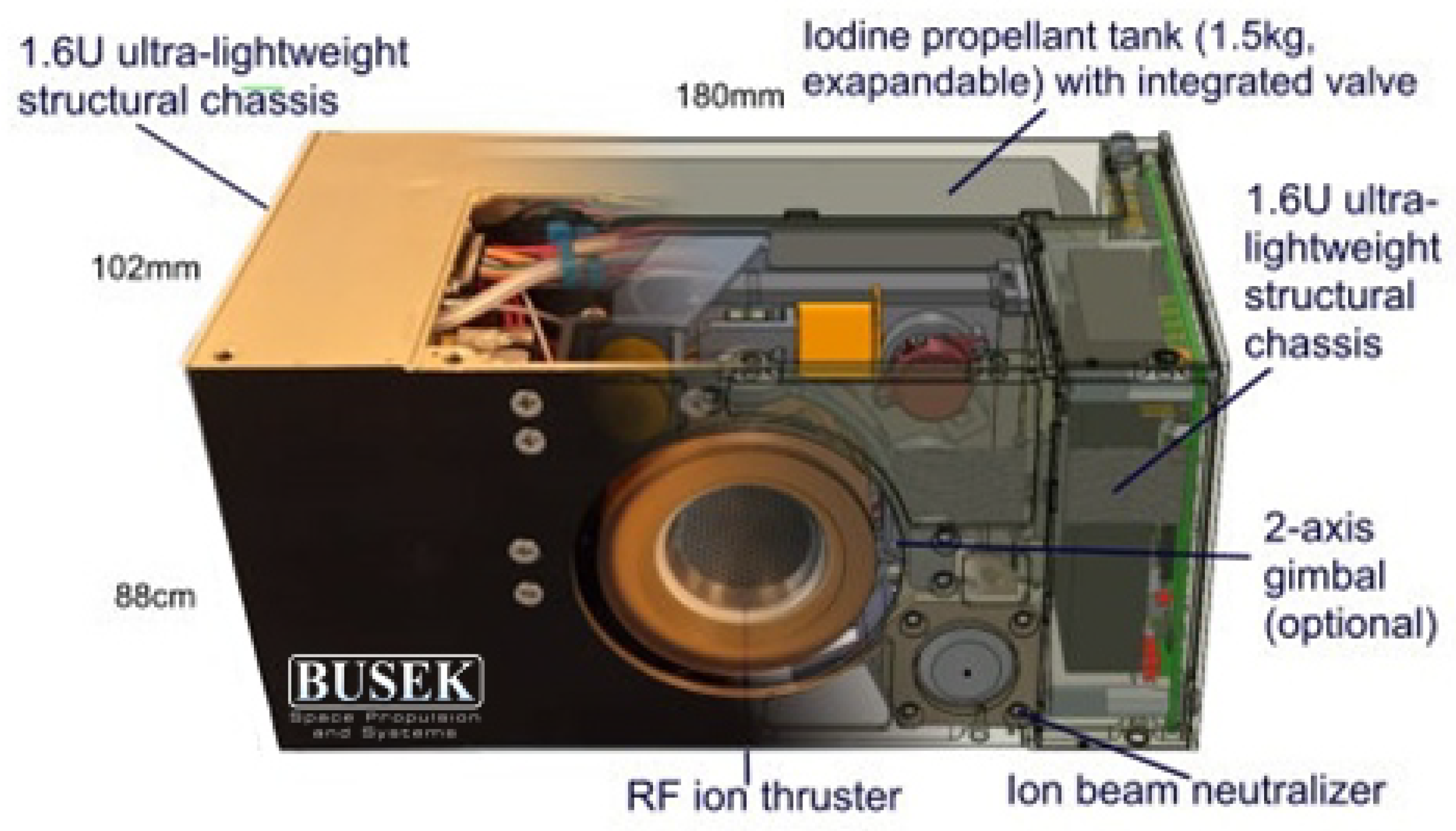
2.2. Potential CubeSat Arrangements and Mass Breakdown Model
3. Spacecraft Dynamics and Guidance Law
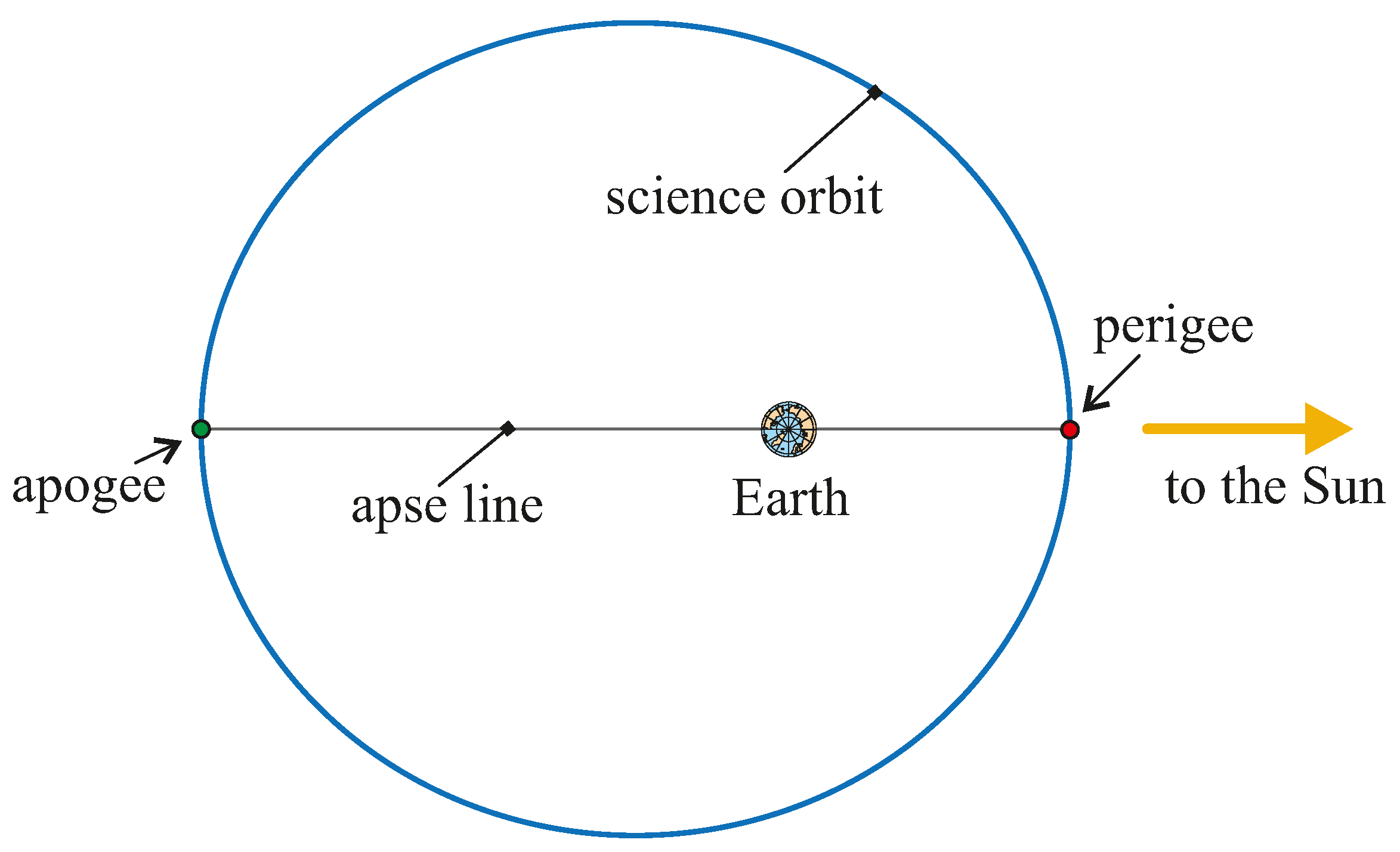
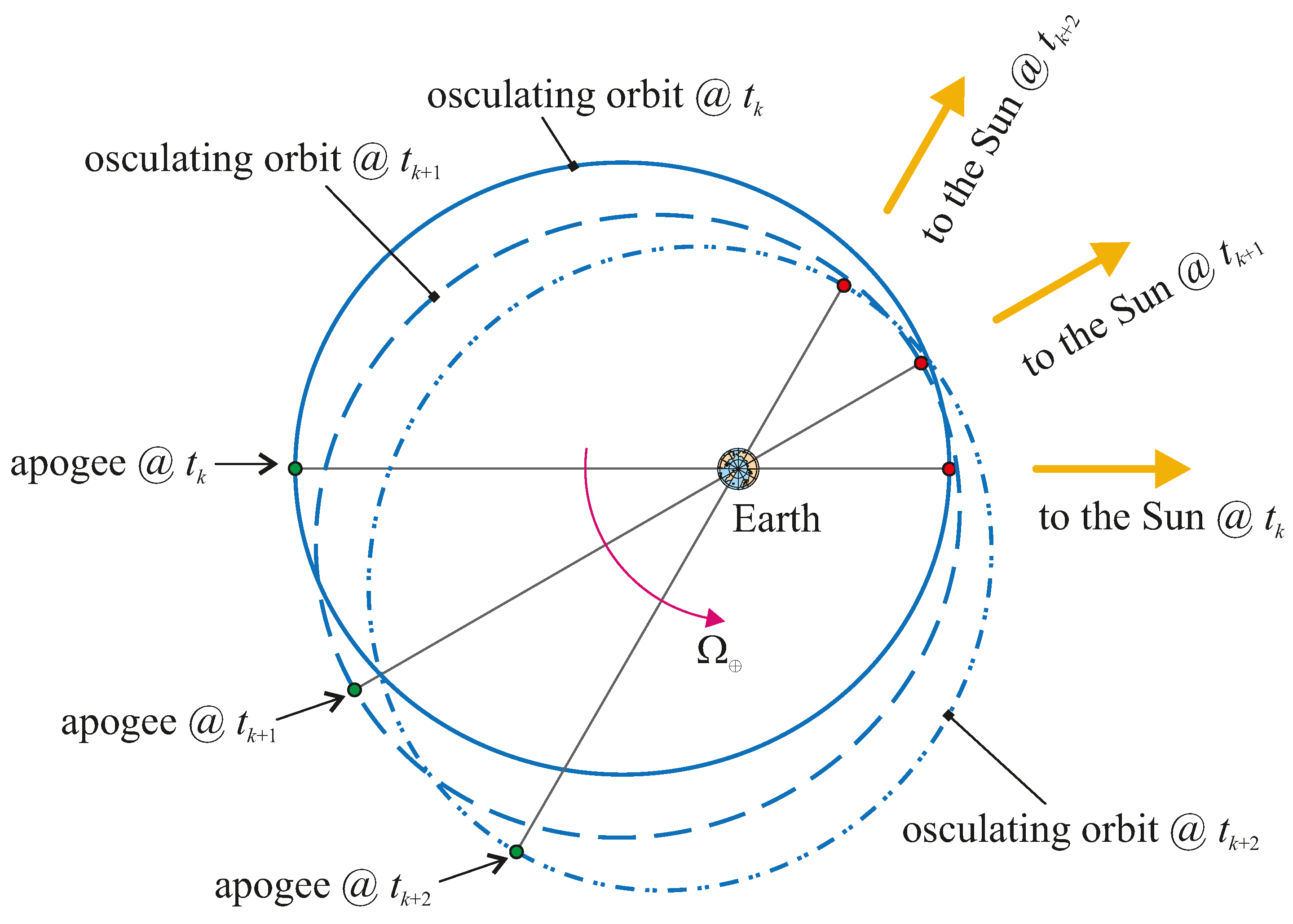
3.1. The Simplified Geocentric Dynamics of the CubeSat
3.2. Trajectory Optimization and Optimal Guidance Laws
4. Numerical Simulations
5. Conclusions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| a | osculating orbit semimajor axis [km] |
| propulsive acceleration vector [mm/s2] | |
| radial component of propulsive acceleration [mm/s2] | |
| transverse component of propulsive acceleration [mm/s2] | |
| auxiliary function; see Equation (27) | |
| e | osculating orbit eccentricity |
| Hamiltonian function | |
| switching function (value of when the thruster array is turned on) | |
| m | CubeSat’s current mass [kg] |
| mass of the thruster array [kg] | |
| mass of the remaining subsystems [kg] | |
| mass of the propellant stored on board the CubeSat [kg] | |
| mass of the scientific payload [kg] | |
| mass of the power generation subsystem [kg] | |
| mass of the additional propellant tanks [kg] | |
| n | number of additional propellant tanks |
| N | number of engine units in the thruster array |
| propellant mass flow rate [g/s] | |
| p | osculating orbit semilatus rectum [km] |
| Earth’s mean reference radius [km] | |
| r | Earth–spacecraft (radial) distance [km] |
| apogee radius [km] | |
| perigee radius [km] | |
| auxiliary function; see Equation (28) | |
| T | thrust magnitude [N] |
| thrust vector [N] | |
| t | time [days] |
| reference time instants with [days] | |
| thrust angle [rad] | |
| adjoint to CubeSat’s state variable i | |
| Earth’s gravitational parameter [km3/s2] | |
| CubeSat’s true anomaly along the osculating orbit [rad] | |
| reference true anomalies with [rad] | |
| constant angular rate of the Sun–Earth line [rad/s] | |
| osculating orbit’s apse line rotation angle [rad] | |
| Subscripts | |
| refers to a single engine unit | |
| 0 | initial science orbit |
| k | at |
References
- El-Alaoui, M.; Walker, R.J.; Weygand, J.M.; Lapenta, G.; Goldstein, M.L. Magnetohydrodynamic Turbulence in the Earth’s Magnetotail From Observations and Global MHD Simulations. Front. Astron. Space Sci. 2021, 8, 620519. [Google Scholar] [CrossRef]
- Wei, Y.; Huang, S.; Rong, Z.; Yuan, Z.; Jiang, K.; Deng, X.; Zhou, M.; Fu, H.; Yu, X.; Xu, S.; et al. Observations of Short-period Current Sheet Flapping Events in the Earth’s Magnetotail. Astrophys. J. Lett. 2019, 874, L18. [Google Scholar] [CrossRef]
- Ergun, R.; Goodrich, K.; Wilder, F.; Ahmadi, N.; Holmes, J.; Eriksson, S.; Stawarz, J.; Nakamura, R.; Genestreti, K.; Hesse, M.; et al. Magnetic Reconnection, Turbulence, and Particle Acceleration: Observations in the Earth’s Magnetotail. Geophys. Res. Lett. 2018, 45, 3338–3347. [Google Scholar] [CrossRef]
- Beyene, F.; Angelopoulos, V. Storm-Time Very-Near-Earth Magnetotail Reconnection: A Statistical Perspective. J. Geophys. Res. Space Phys. 2024, 129, e2024JA032434. [Google Scholar] [CrossRef]
- Nykyri, K.; Di Matteo, S.; Archer, M.; Ma, X.; Hartinger, M.; Sarantos, M.; Zesta, E.; Paterson, W. Could a Low-Frequency Perturbation in the Earth’s Magnetotail Be Generated by the Lunar Wake? Geophys. Res. Lett. 2024, 51, e2024GL110129. [Google Scholar] [CrossRef]
- Hajra, R.; Echer, E.; Franco, A.M.d.S.; Bolzan, M.J.A. Earth’s magnetotail variability during supersubstorms (SSSs): A study on solar wind–magnetosphere–ionosphere coupling. Adv. Space Res. 2023, 72, 1208–1223. [Google Scholar] [CrossRef]
- Li, S.; Poppe, A.; Orlando, T.; Jones, B.; Tucker, O.; Farrell, W.; Hendrix, A. Formation of lunar surface water associated with high-energy electrons in Earth’s magnetotail. Nat. Astron. 2023, 7, 1427–1435. [Google Scholar] [CrossRef]
- Torkar, K.; Nakamura, R.; Roberts, O.; Jeszenszky, H.; Lindqvist, P.A.; Khotyaintsev, Y.; Giles, B.; Barrie, A. Active Spacecraft Potential Control in the MMS Mission: Results from Six Years in Orbit. IEEE Trans. Plasma Sci. 2023, 51, 2461–2467. [Google Scholar] [CrossRef]
- Vaverka, J.; Pavlů, J.; Nouzák, L.; Šafránková, J.; Němeček, Z.; Mann, I.; Ye, S.; Lindqvist, P.A. One-Year Analysis of Dust Impact-Like Events Onto the MMS Spacecraft. J. Geophys. Res. Space Phys. 2019, 124, 8179–8190. [Google Scholar] [CrossRef]
- Williams, T.; Palmer, E.; Godine, D.; Hollister, J.; Ottenstein, N.; Vint, B. MMS extended mission eclipse mitigation and solar wind turbulence science campaign. Adv. Astronaut. Sci. 2020, 171, 829–846. [Google Scholar]
- Williams, T.; Godine, D.; Palmer, E.; Patel, I.; Ottenstein, N.; Winternitz, L.; Petrinec, S. MMS extended mission design: Evaluation of a lunar gravity assist option. Adv. Astronaut. Sci. 2018, 167, 1053–1072. [Google Scholar]
- Svenningsson, I.; Yordanova, E.; Khotyaintsev, Y.; André, M.; Cozzani, G. Classifying the Magnetosheath Using Local Measurements From MMS. J. Geophys. Res. Space Phys. 2025, 130, e2024JA033272. [Google Scholar] [CrossRef]
- Tian, A.; Wang, Z.; Fu, H.; Guo, Z. Evidence of a Magnetic Null in Electron-Only Reconnection. Geophys. Res. Lett. 2025, 52, e2024GL113104. [Google Scholar] [CrossRef]
- Hwang, K.J.; Goldstein, M.; Wendel, D.; Fazakerley, A.; Gurgiolo, C. Cluster observations near reconnection X lines in Earth’s magnetotail current sheet. J. Geophys. Res. Space Phys. 2013, 118, 4199–4209. [Google Scholar] [CrossRef]
- Duan, A.; Cao, J.; Ma, Y.; Wei, X. Cluster observations of large-scale southward movement and dawnward-duskward flapping of Earth’s magnetotail current sheet. Sci. China Technol. Sci. 2013, 56, 194–204. [Google Scholar] [CrossRef]
- Escoubet, C.P.; Hwang, K.J.; Toledo-Redondo, S.; Turc, L.; Haaland, S.E.; Aunai, N.; Dargent, J.; Eastwood, J.P.; Fear, R.C.; Fu, H.; et al. Cluster and MMS Simultaneous Observations of Magnetosheath High Speed Jets and Their Impact on the Magnetopause. Front. Astron. Space Sci. 2020, 6, 78. [Google Scholar] [CrossRef]
- Walia, N.K.; Seki, K.; Amano, T.; Kitamura, N.; Saito, Y.; Ahmadi, T.; Gershman, D.J.; Pollock, C.J.; Giles, B.L.; Fuselier, S.A.; et al. A Study of Slow-mode Shocks in the Near-Earth Magnetotail with MMS Observations and Hybrid Simulations. Astrophys. J. 2024, 977, 117. [Google Scholar] [CrossRef]
- Ovchinnikov, I.; Naiko, D.Y.; Antonova, E. Fluctuations of the Electric and Magnetic Fields in the Plasma Sheet of the Earth’s Magnetotail According to MMS Data. Cosm. Res. 2024, 62, 10–33. [Google Scholar] [CrossRef]
- Zhang, Z.; Lu, S.; Lu, Q.; Wang, R.; Zhan, C.; Li, X.; Artemyev, A.V. Statistical Survey of Thin Current Sheets in Earth’s Magnetotail: MMS Observations. J. Geophys. Res. Space Phys. 2024, 129, e2024JA032575. [Google Scholar] [CrossRef]
- Smith, A.; Sun, W.; Slavin, J.; Rae, I. Ion-Scale Magnetic Flux Ropes and Loops in Earth’s Magnetotail: An Automated, Comprehensive Survey of MMS Data Between 2017 and 2022. J. Geophys. Res. Space Phys. 2024, 129, e2023JA032231. [Google Scholar] [CrossRef]
- Büchner, J.; Kuska, J.P.; Nikutowski, B.; Wiechen, H.; Rustenbach, J.; Auster, U.; Fornacon, K.; Klimov, S.; Petrukovich, A.; Savin, S. Three-dimensional reconnection in the Earth’s magnetotail: Simulations and observations. Geophys. Monogr. Ser. 1998, 104, 313–326. [Google Scholar] [CrossRef]
- Whipple, E.; Puetter, R.; Rosenberg, M. A two-dimensional, time-dependent, near-Earth magnetotail. Adv. Space Res. 1991, 11, 133–142. [Google Scholar] [CrossRef]
- Zhao, P.; Wu, C.; Li, Y. Design and application of solar sailing: A review on key technologies. Chin. J. Aeronaut. 2023, 36, 125–144. [Google Scholar] [CrossRef]
- Gong, S.; Macdonald, M. Review on solar sail technology. Astrodynamics 2019, 3, 93–125. [Google Scholar] [CrossRef]
- Fu, B.; Sperber, E.; Eke, F. Solar sail technology—A state of the art review. Prog. Aerosp. Sci. 2016, 86, 1–19. [Google Scholar] [CrossRef]
- Quarta, A.A. Venus magnetotail long-term sensing using solar sails. Appl. Sci. 2024, 14, 8016. [Google Scholar] [CrossRef]
- Wright, J.L. Space Sailing; Gordon and Breach Science Publishers: Philadephia, PA, USA, 1992; pp. 223–233. ISBN 978-2881248429. [Google Scholar]
- McInnes, C.R. Solar Sailing: Technology, Dynamics and Mission Applications; Springer-Praxis Series in Space Science and Technology; Springer: Berlin/Heidelberg, Germany, 1999; pp. 46–54, 119–120. [Google Scholar] [CrossRef]
- Spencer, D.A.; Johnson, L.; Long, A.C. Solar sailing technology challenges. Aerosp. Sci. Technol. 2019, 93, 105276. [Google Scholar] [CrossRef]
- Berthet, M.; Schalkwyk, J.; Çelik, O.; Sengupta, D.; Fujino, K.; Hein, A.M.; Tenorio, L.; Cardoso dos Santos, J.; Worden, S.P.; Mauskopf, P.D.; et al. Space sails for achieving major space exploration goals: Historical review and future outlook. Prog. Aerosp. Sci. 2024, 150, 101047. [Google Scholar] [CrossRef]
- Lappas, V.; Mengali, G.; Quarta, A.A.; Gil-Fernandez, J.; Schmidt, T.; Wie, B. Practical Systems Design for an Earth-Magnetotail-Monitoring Solar Sail Mission. J. Spacecr. Rocket. 2009, 46, 381–393. [Google Scholar] [CrossRef]
- Macdonald, M.; Hughes, G.W.; McInnes, C.; Lyngvi, A.; Falkner, P.; Atzei, A. GeoSail: An Elegant Solar Sail Demonstration Mission. J. Spacecr. Rocket. 2007, 44, 784–796. [Google Scholar] [CrossRef][Green Version]
- Mengali, G.; Quarta, A.A.; Lappas, V.J. Optimal steering law for the GeoSail mission. J. Guid. Control. Dyn. 2007, 30, 876–879. [Google Scholar] [CrossRef]
- Macdonald, M.; McInnes, C.; Alexander, D.; Sandman, A. GeoSail: Exploring the magnetosphere using a low-cost solar sail. Acta Astronaut. 2006, 59, 757–767. [Google Scholar] [CrossRef]
- Alexander, D.; McInnes, C.R.; Angelopoulos, V.; Sandman, A.W.; Macdonald, M. GeoSail: A novel solar sail mission concept for geospace. In Proceedings of the AIP Space Technology and Applications International Forum—Staif 2002, Albuquerque, NM, USA, 3–6 February 2002. [Google Scholar] [CrossRef]
- McInnes, C.R.; Macdonald, M.; Angelopolous, V.; Alexander, D. GEOSAIL: Exploring the Geomagnetic Tail Using a Small Solar Sail. J. Spacecr. Rocket. 2001, 38, 622–629. [Google Scholar] [CrossRef]
- Levchenko, I.; Goebel, D.; Pedrini, D.; Albertoni, R.; Baranov, O.; Kronhaus, I.; Lev, D.; Walker, M.L.; Xu, S.; Bazaka, K. Recent innovations to advance space electric propulsion technologies. Prog. Aerosp. Sci. 2025, 152, 100900. [Google Scholar] [CrossRef]
- Levchenko, I.; Xu, S.; Mazouffre, S.; Lev, D.; Pedrini, D.; Goebel, D.; Garrigues, L.; Taccogna, F.; Bazaka, K. Perspectives, frontiers, and new horizons for plasma-based space electric propulsion. Phys. Plasmas 2020, 27, 020601. [Google Scholar] [CrossRef]
- Hutchins, M.; Simpson, H.B.; Jiménez, J.P. QinetiQ’s T6 and T5 Ion Thruster Electric Propulsion System Architectures and Performance. In Proceedings of the 34th International Electric Propulsion Conference and 6th Nano-Satellite Symposium, Kobe, Japan, 4–10 July 2015. [Google Scholar]
- Randall, P.N.; Lewis, R.A.; Clark, S.D. QinetiQ T5 based Electric Propulsion System and Architectural Options for Future Applications. In Proceedings of the 35th International Electric Propulsion Conference, Atlanta, GA, USA, 8–12 October 2017. [Google Scholar]
- Grönland, T.A.; Rangsten, P.; Nese, M.; Lang, M. Miniaturization of components and systems for space using MEMS-technology. Acta Astronaut. 2007, 61, 228–233. [Google Scholar] [CrossRef]
- Kabirov, V.; Semenov, V.; Torgaeva, D.; Otto, A. Miniaturization of spacecraft electrical power systems with solar-hydrogen power supply system. Int. J. Hydrogen Energy 2023, 48, 9057–9070. [Google Scholar] [CrossRef]
- Garranzo, D.; Núñez, A.; Laguna, H.; Belenguer, T.; De Miguel, E.; Cebollero, M.; Ibarmia, S.; Martínez, C. APIS: The miniaturized Earth observation camera on-board OPTOS CubeSat. J. Appl. Remote Sens. 2019, 13, 032502. [Google Scholar] [CrossRef]
- Francisco, C.; Henriques, R.; Barbosa, S. A Review on CubeSat Missions for Ionospheric Science. Aerospace 2023, 10, 622. [Google Scholar] [CrossRef]
- Alnaqbi, S.; Darfilal, D.; Swei, S.S.M. Propulsion Technologies for CubeSats: Review. Aerospace 2024, 11, 502. [Google Scholar] [CrossRef]
- O’Reilly, D.; Herdrich, G.; Kavanagh, D.F. Electric Propulsion Methods for Small Satellites: A Review. Aerospace 2021, 8, 22. [Google Scholar] [CrossRef]
- Yeo, S.H.; Ogawa, H.; Kahnfeld, D.; Schneider, R. Miniaturization perspectives of electrostatic propulsion for small spacecraft platforms. Prog. Aerosp. Sci. 2021, 126, 100742. [Google Scholar] [CrossRef]
- Topputo, F.; Wang, Y.; Giordano, C.; Franzese, V.; Goldberg, H.; Perez-Lissi, F.; Walker, R. Envelop of reachable asteroids by M-ARGO CubeSat. Adv. Space Res. 2021, 67, 4193–4221. [Google Scholar] [CrossRef]
- Lockett, T.R.; Castillo-Rogez, J.; Johnson, L.; Matus, J.; Lightholder, J.; Marinan, A.; Few, A. Near-Earth Asteroid Scout Flight Mission. IEEE Aerosp. Electron. Syst. Mag. 2020, 35, 20–29. [Google Scholar] [CrossRef]
- Heaton, A.; Miller, K.; Ahmad, N. Near earth asteroid Scout solar sail thrust and torque model. In Proceedings of the 4th International Symposium on Solar Sailing (ISSS 2017), Kyoyo, Japan, 17–20 January 2017. [Google Scholar]
- Johnson, L.; Castillo-Rogez, J.; Lockett, T. Near Earth asteroid Scout: Exploring asteroid 1991VG using a Smallsat. In Proceedings of the 70th International Astronautical Congress, Washington, DC, USA, 21–25 October 2019. [Google Scholar]
- Busek Co. Inc. BIT-3: Compact and Efficient Iodine Gridded Ion Thruster. 2024. Available online: https://www.busek.com/bit3 (accessed on 12 December 2024).
- Tsay, M.; Model, J.; Barcroft, C.; Frongillo, J. Integrated Testing of Iodine BIT-3 RF Ion Propulsion System for 6U CubeSat Applications. In Proceedings of the 35th International Electric Propulsion Conference, Atlanta, GA, USA, 8–12 October 2017. [Google Scholar]
- Tsay, M.; Frongillo, J.; Hohman, K. Iodine-Fueled Mini RF Ion Thruster for CubeSat Applications. In Proceedings of the 34th International Electric Propulsion Conference, Kobe, Japan, 4–10 July 2015. [Google Scholar]
- Tsay, M.; Frongillo, J.; Model, J.; Zwahlen, J.; Paritsky, L. Maturation of iodine-fueled BIT-3 RF ion thruster and RF neutralizer. In Proceedings of the 52nd AIAA/SAE/ASEE Joint Propulsion Conference, Salt Lake City, UT, USA, 25–27 July 2016. [Google Scholar] [CrossRef]
- Tsay, M.; Frongillo, J.; Model, J.; Zwahlen, J.; Barcroft, C.; Feng, C. Neutralization demo and thrust stand measurement for BIT-3 RF ion thruster. In Proceedings of the 53rd AIAA/SAE/ASEE Joint Propulsion Conference, Atlanta, GA, USA, 10–12 July 2017. [Google Scholar] [CrossRef]
- Quarta, A.A. Preliminary Trajectory Analysis of CubeSats with Electric Thrusters in Nodal Flyby Missions for Asteroid Exploration. Remote Sens. 2025, 17, 513. [Google Scholar] [CrossRef]
- MMA Space. MMA HaWK Solar Arrays. 2024. Available online: https://mmadesignllc.com/next-gen-solar-arrays/ (accessed on 12 December 2024).
- Courtney, D.; Tsay, M.; Demmons, N. Busek SmallSat Technologies. In Proceedings of the Planetary CubeSats Symposium, Greenbelt, MD, USA, 17 August 2018. [Google Scholar]
- Stark, J.P.W.; Swinerd, G.G.; Fortescue, P.W. Spacecraft Systems Engineering; Wiley: Chichester, UK, 2003; Chapter 4; pp. 93–95. [Google Scholar]
- Kulu, E. CubeSats & Nanosatellites–2024 Statistics, Forecast and Reliability. In Proceedings of the 75th International Astronautical Congress, Milan, Italy, 14–18 October 2024. [Google Scholar]
- Betts, J.T. Survey of Numerical Methods for Trajectory Optimization. J. Guid. Control. Dyn. 1998, 21, 193–207. [Google Scholar] [CrossRef]
- Ross, I.M. A Primer on Pontryagin’s Principle in Optimal Control; Collegiate Publishers: San Francisco, CA, USA, 2015; Chapter 2; pp. 127–129. [Google Scholar]
- Prussing, J.E. Optimal Spacecraft Trajectories; Oxford University Press: Oxford, UK, 2018; Chapter 4; pp. 32–40. [Google Scholar]
- Bryson, A.E.; Ho, Y.C. Applied Optimal Control; Hemisphere Publishing Corporation: New York, NY, USA, 1975; Chapter 2; pp. 71–89. ISBN 0-891-16228-3. [Google Scholar]
- Quarta, A.A. Initial costate approximation for rapid orbit raising with very low propulsive acceleration. Appl. Sci. 2024, 14, 1124. [Google Scholar] [CrossRef]

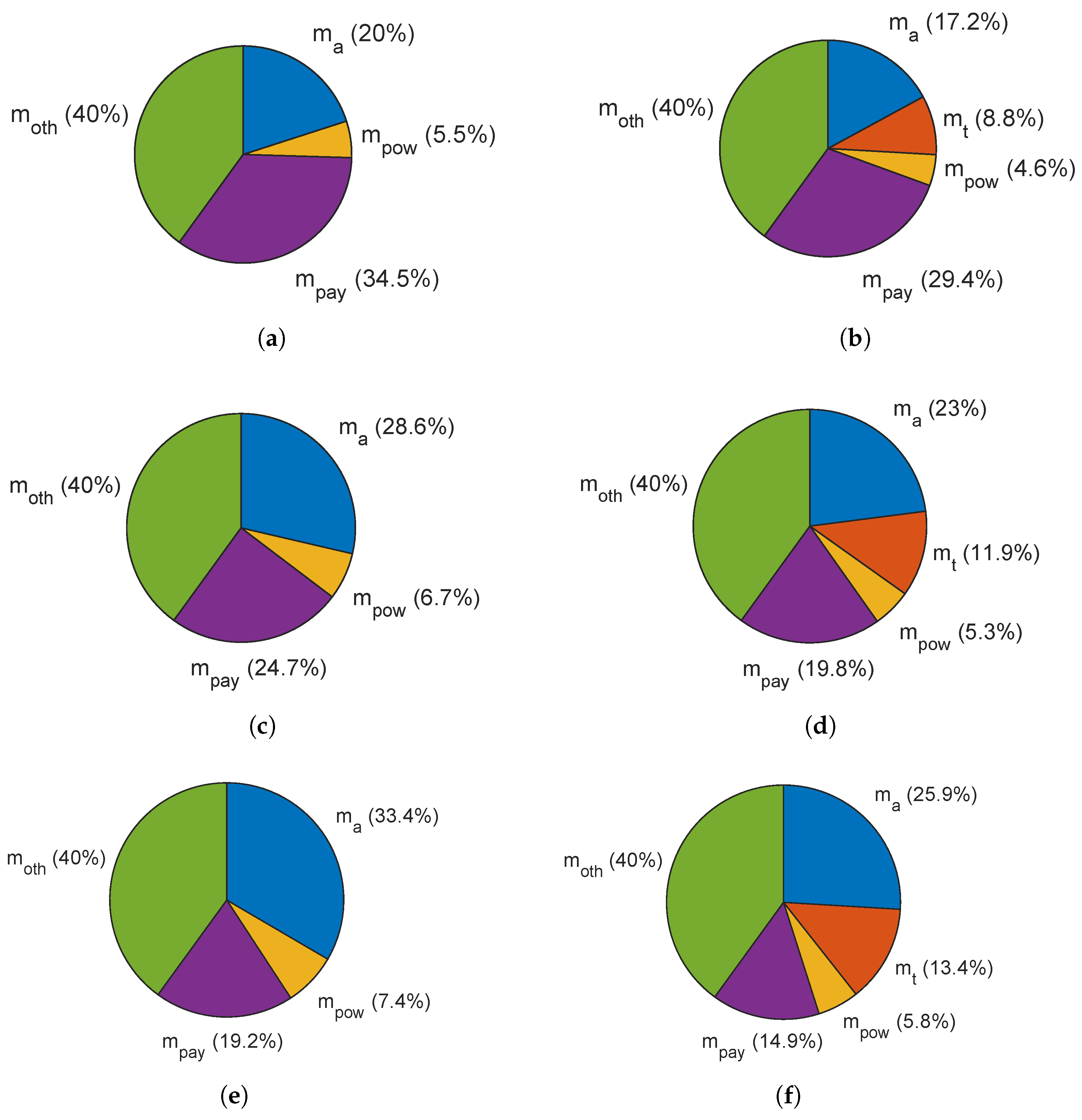
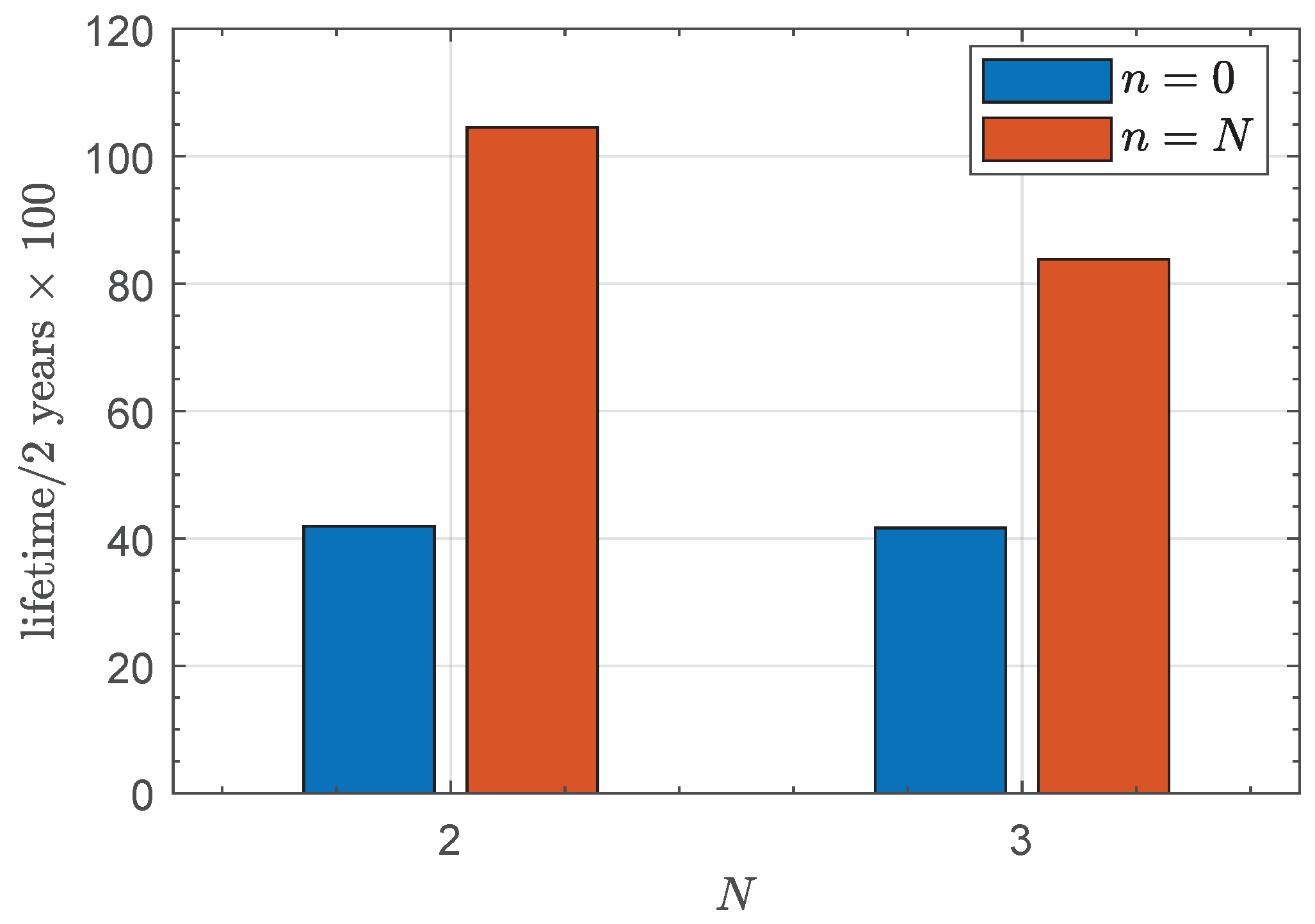
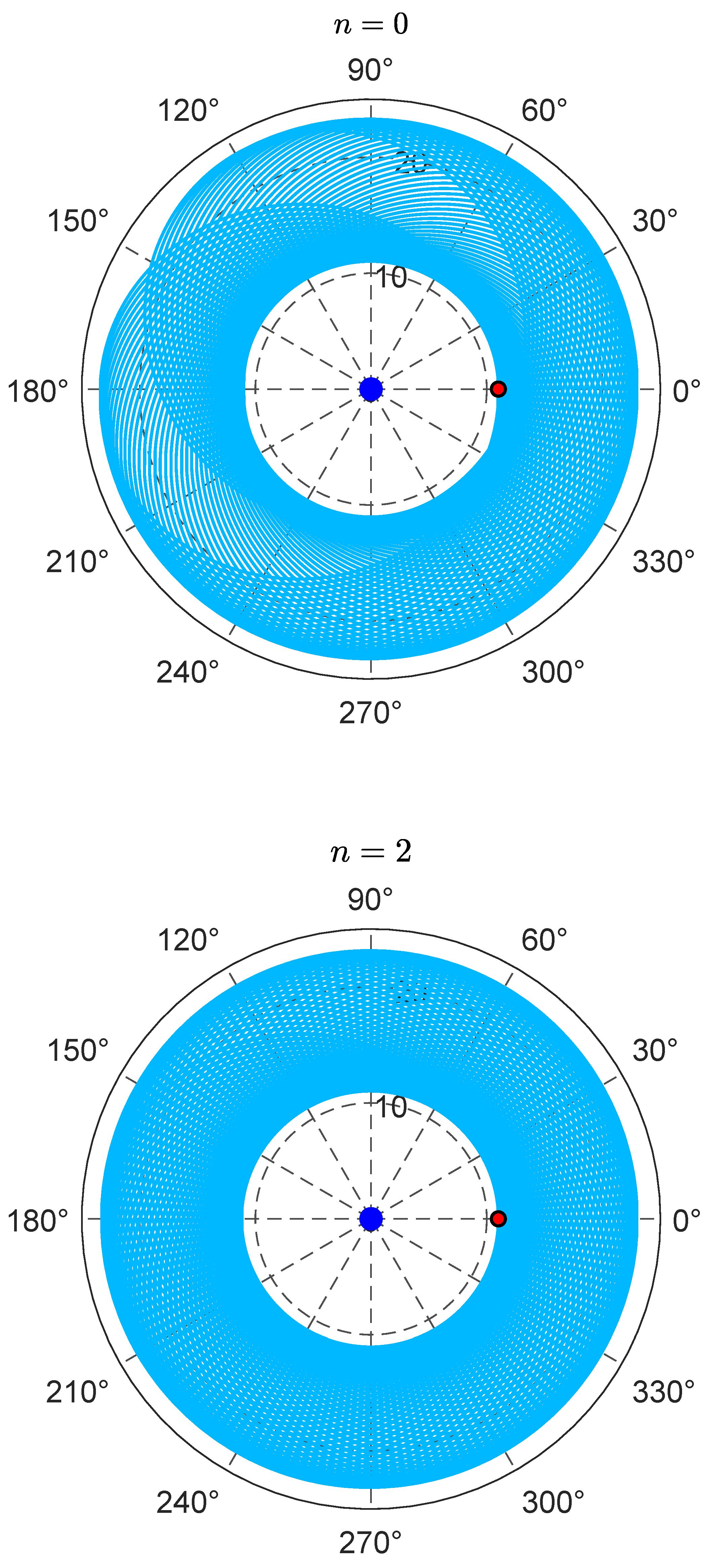


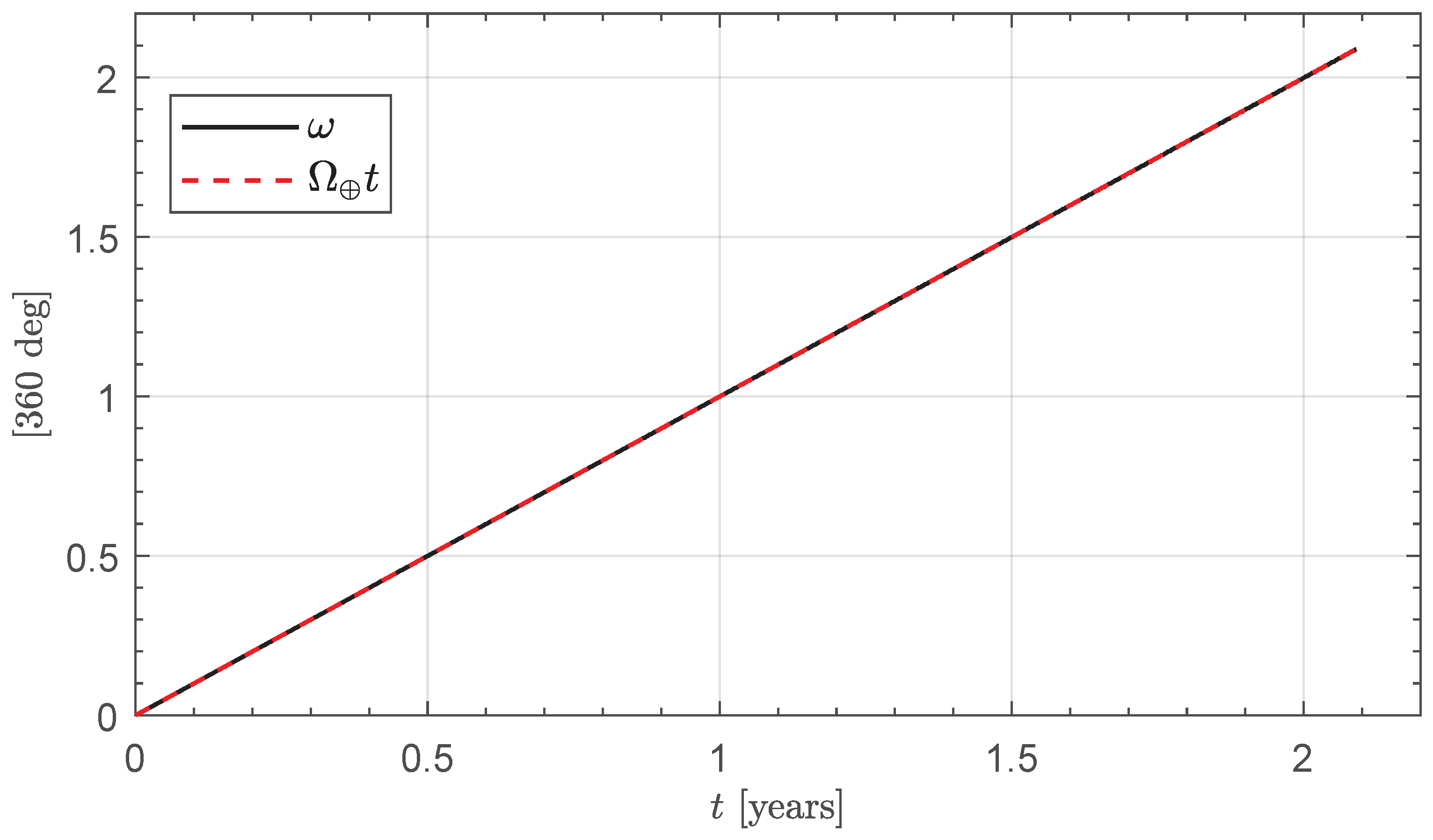
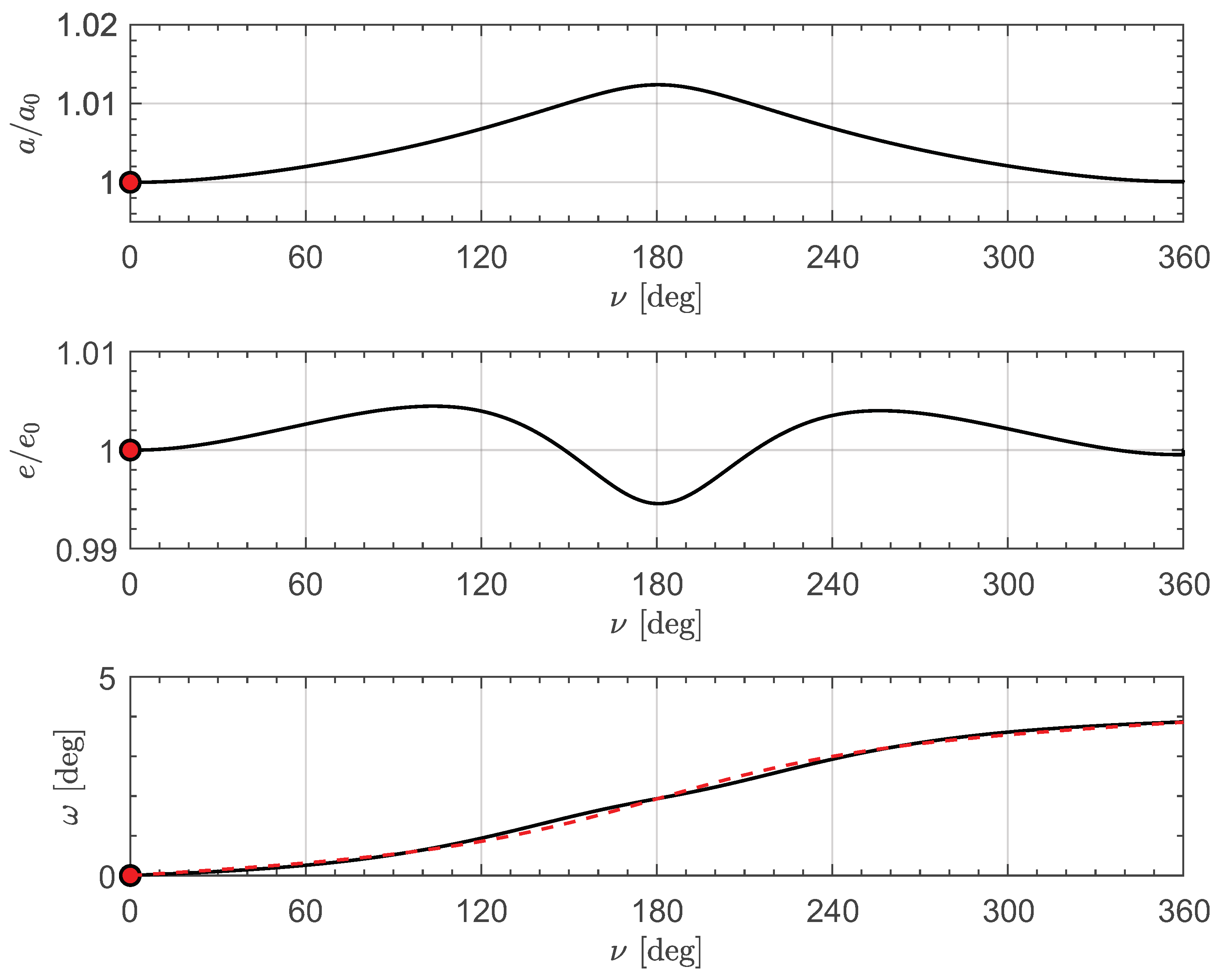


| N | 1 | 2 | 3 |
|---|---|---|---|
| T [mN] | |||
| [mg/day] | |||
| P [W] | 75 | 150 | 225 |
| 26 | |||
| 17 | |||
| — | 31 | ||
| — | — |
| 3 | |||
| 3 | 6 | ||
| — | 6 | ||
| — | — | 9 |
| Lifetime [Days] | Final Mass [kg] | |
|---|---|---|
| and | 306 | |
| and | 763 | |
| and | 304 | |
| and | 612 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Quarta, A.A. Exploration of Earth’s Magnetosphere Using CubeSats with Electric Propulsion. Aerospace 2025, 12, 211. https://doi.org/10.3390/aerospace12030211
Quarta AA. Exploration of Earth’s Magnetosphere Using CubeSats with Electric Propulsion. Aerospace. 2025; 12(3):211. https://doi.org/10.3390/aerospace12030211
Chicago/Turabian StyleQuarta, Alessandro A. 2025. "Exploration of Earth’s Magnetosphere Using CubeSats with Electric Propulsion" Aerospace 12, no. 3: 211. https://doi.org/10.3390/aerospace12030211
APA StyleQuarta, A. A. (2025). Exploration of Earth’s Magnetosphere Using CubeSats with Electric Propulsion. Aerospace, 12(3), 211. https://doi.org/10.3390/aerospace12030211






