An Evaluation of the Accuracy of Existing Empirical and Semi-Empirical Methods for Predicting the Wing Mass of Large Transport Aircraft
Abstract
1. Introduction
2. Empirical and Semi-Empirical Methods of Aircraft Wing Mass Prediction
2.1. Torenbeek Method I (1976)
2.2. Shevell Method (1983)
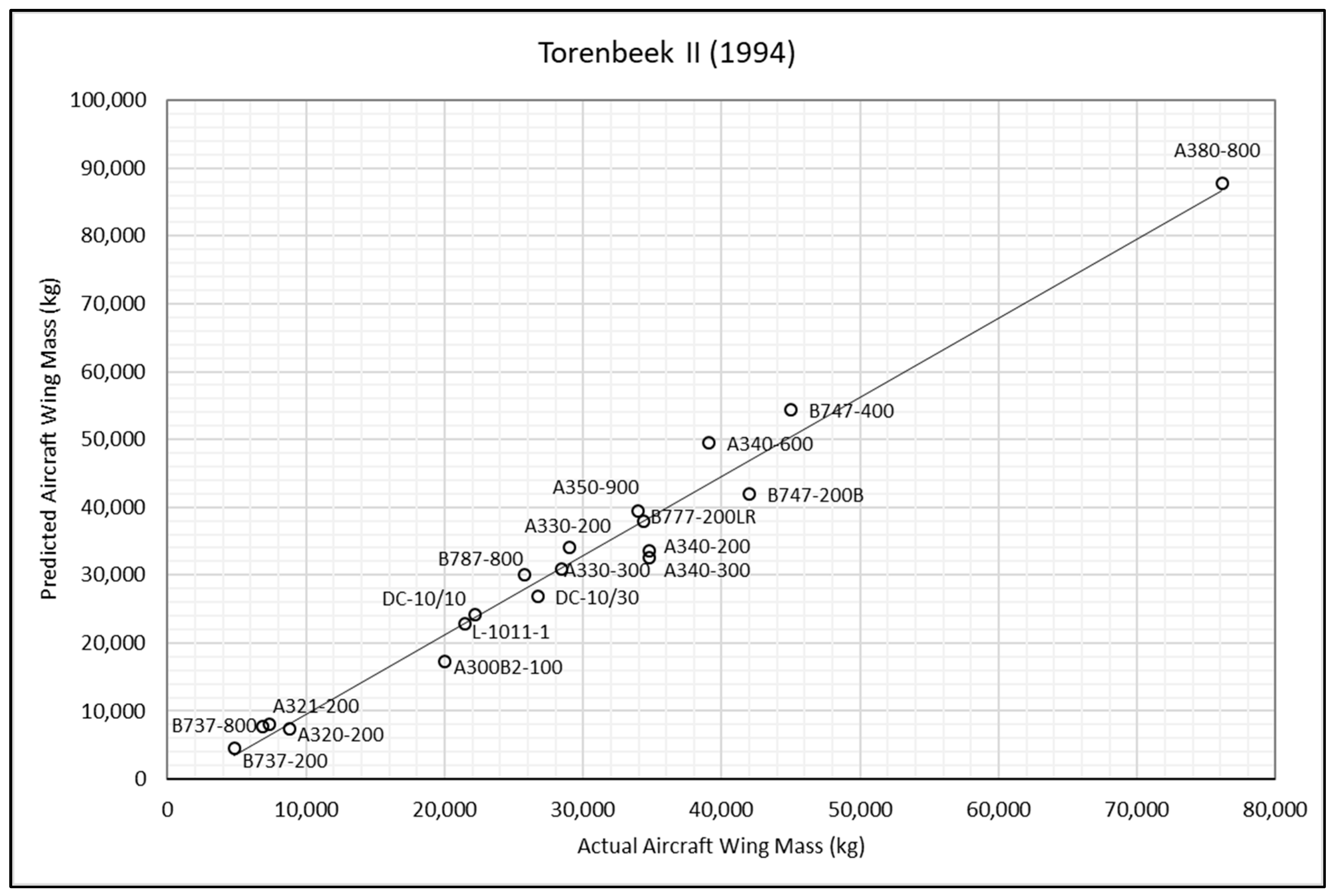
2.3. Torenbeek Method II (1994)
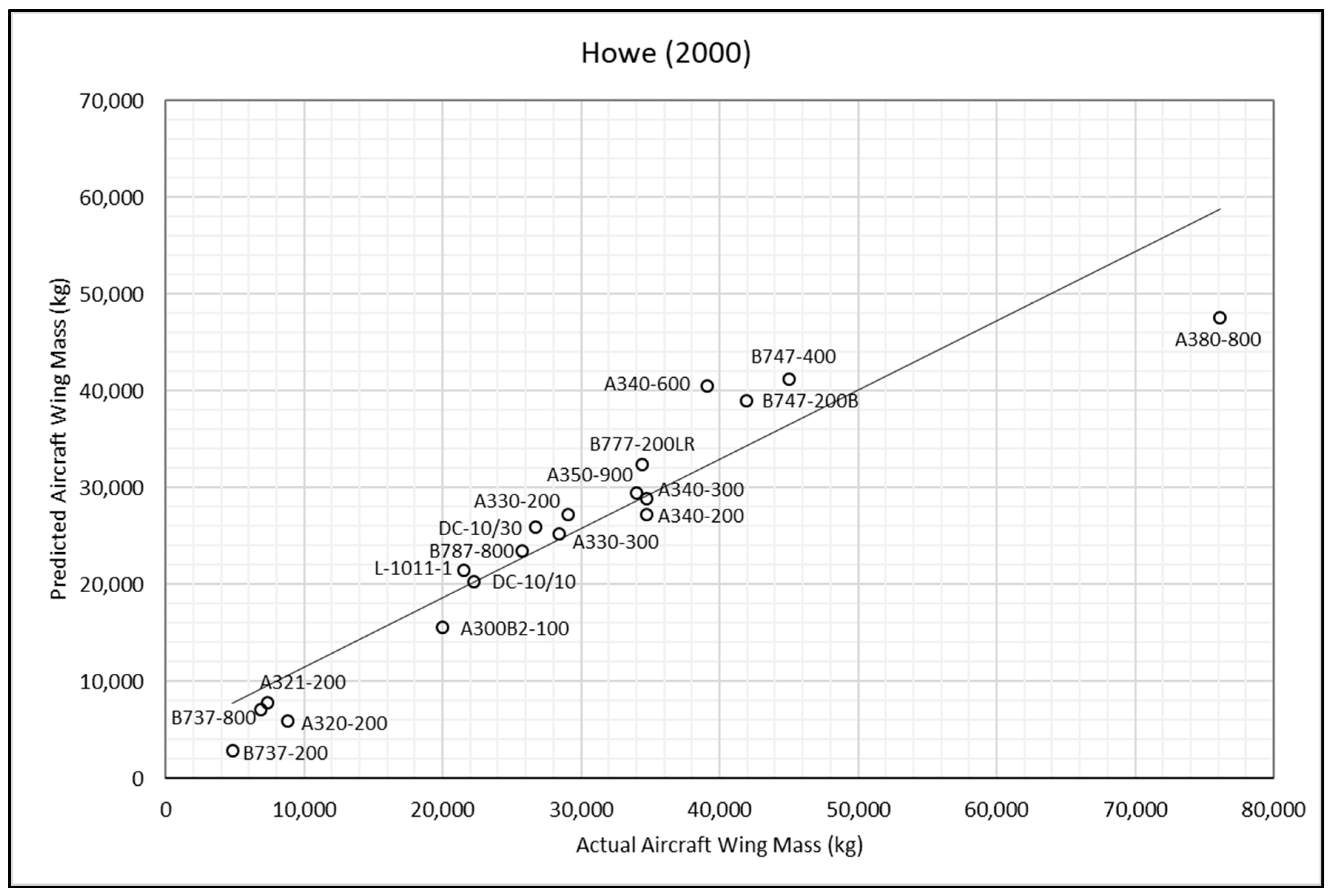
2.4. Howe Method (2000)
- Long range, s > 5000 km
- Short/medium range: ≤ 46,000 kg
- Long-haul jet transports,
- Short-/medium-haul jet transports,
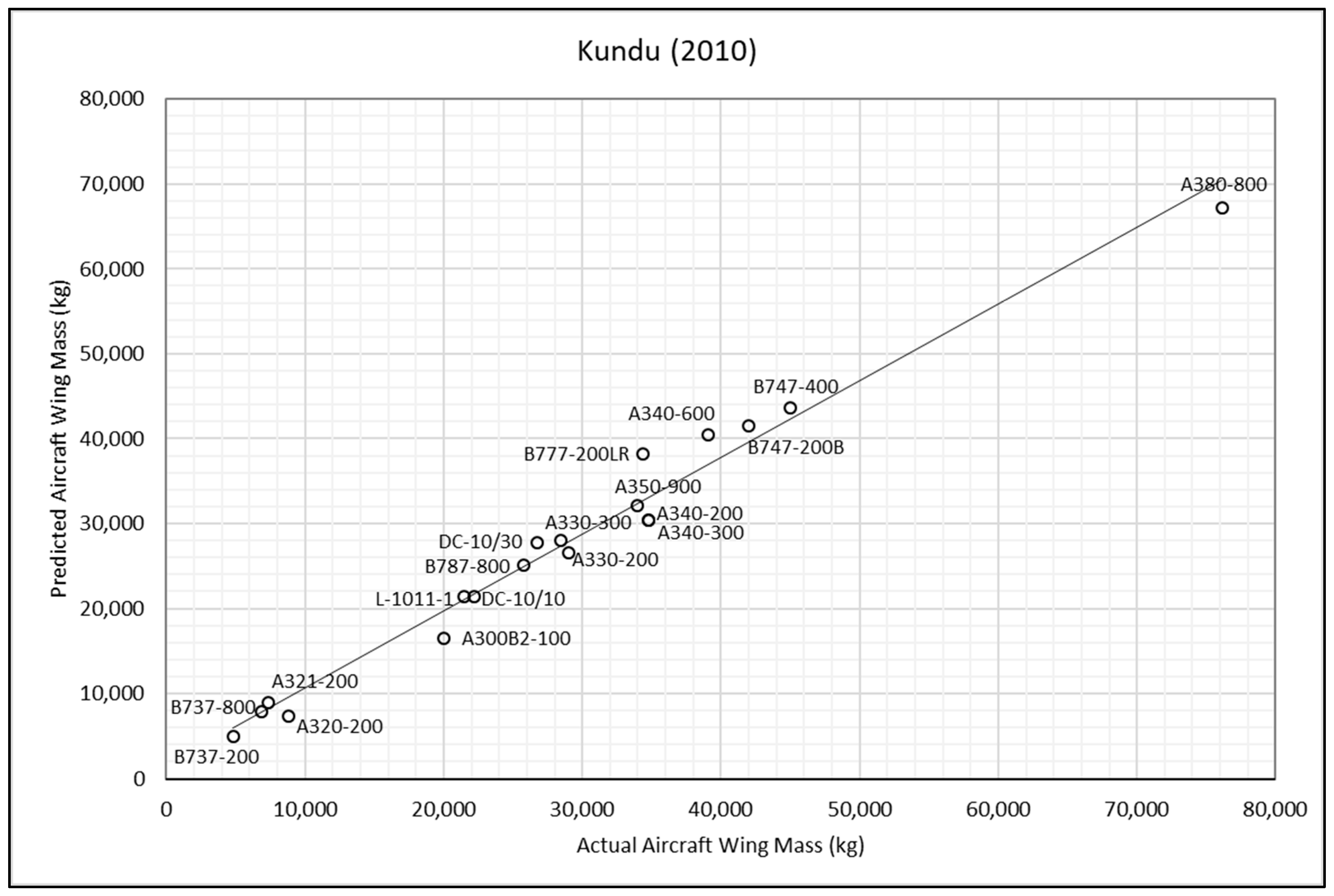
2.5. Kundu Method (2010)
2.6. LTH Method (2011)
2.7. Elham Simple Class I Method (2013)
2.8. Basgall Jet-Transport Method (2020)
3. Application of Methods for Wing-Mass Prediction
4. Comparative Analysis of Investigated Methods of Wing-Mass Estimation
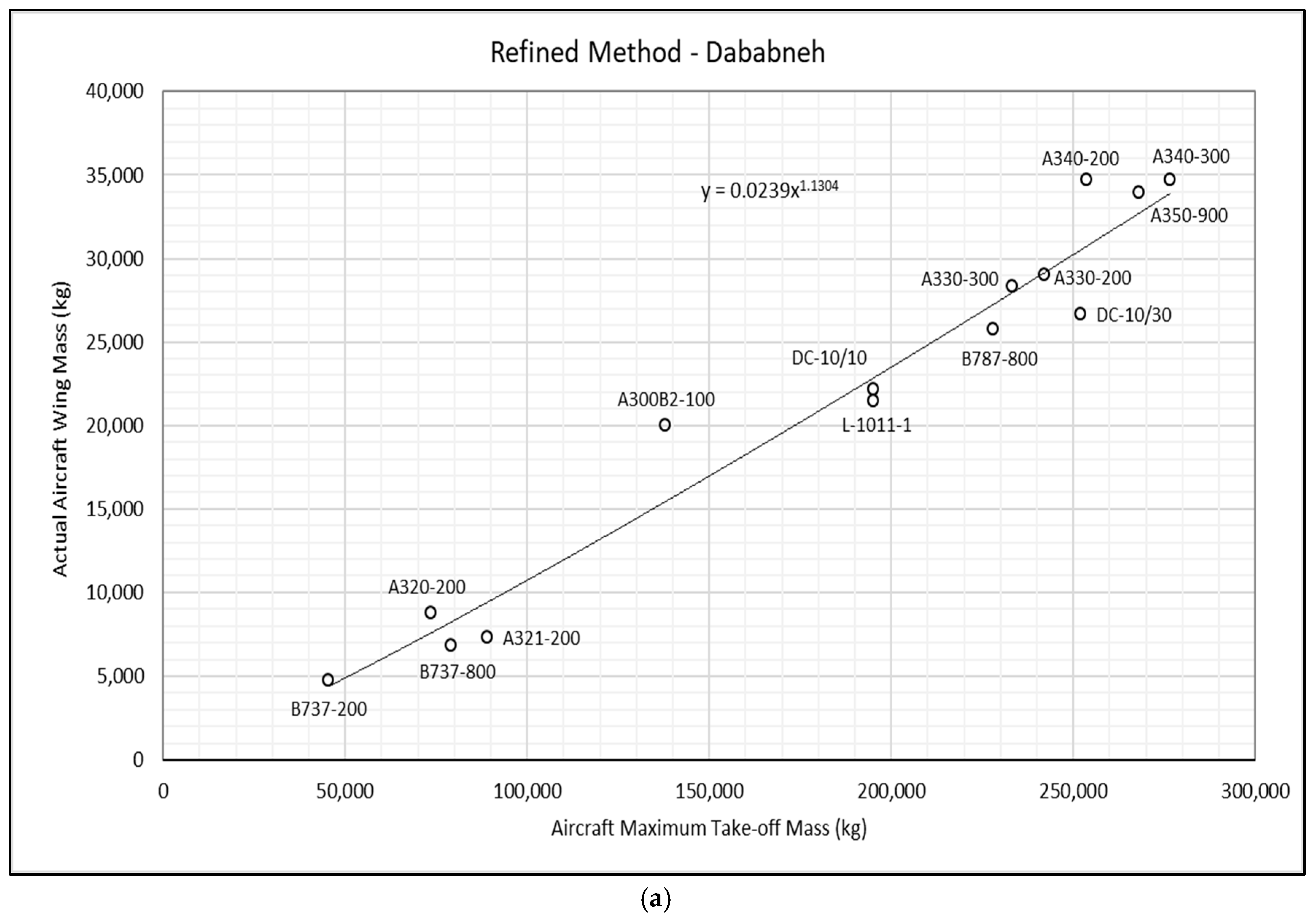
5. Refinement of Methods of Wing-Mass Estimation
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
| Parameter | Units | Aircraft Type—Part A | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| A380-800 | A350-900 | A340-200 | A340-300 | A340-600 | A330-200 | A330-300 | A300B2-100 | A320-200 | A321-200 | ||
| Torenbeek I (1976) [14] | |||||||||||
| kg | 360,911.00 | 189,852.00 | 169,000.00 | 178,000.00 | 245,000.00 | 170,000.00 | 175,000.00 | 116,500.00 | 61,000.00 | 71,500.00 | |
| m | 79.64 | 64.74 | 60.30 | 58.00 | 61.20 | 60.30 | 58.00 | 44.83 | 34.10 | 34.10 | |
| ° | 30.26 | 30.24 | 26.83 | 26.95 | 28.20 | 26.83 | 26.95 | 24.80 | 22.50 | 22.50 | |
| - | 3.75 | 3.75 | 3.75 | 3.75 | 3.75 | 3.75 | 3.75 | 3.75 | 3.75 | 3.75 | |
| m | 2.00 | 1.89 | 1.68 | 1.52 | 1.44 | 1.61 | 1.52 | 1.43 | 0.93 | 0.93 | |
| m2 | 853.21 | 437.17 | 363.10 | 363.11 | 435.26 | 363.1 | 363.11 | 260.00 | 122.57 | 122.57 | |
| Shevell Method (1983) [17] | |||||||||||
| - | 3.75 | 3.75 | 3.75 | 3.75 | 3.75 | 3.75 | 3.75 | 3.75 | 3.75 | 3.75 | |
| ft | 261.29 | 212.40 | 197.83 | 190.29 | 200.79 | 197.83 | 190.29 | 147.08 | 111.88 | 111.88 | |
| lbs | 1,234,588.67 | 590,838.86 | 558,871.83 | 609,578.15 | 811,301.12 | 533,518.67 | 513,677.07 | 304,000.00 | 162,039.76 | 196,211.41 | |
| lbs | 795,672.51 | 418,552.96 | 372,581.22 | 392,422.83 | 540,132.54 | 374,785.85 | 385,808.96 | 256,838.54 | 134,481.98 | 157,630.52 | |
| - | 9.82% | 12.46% | 12.83% | 12.40% | 10.00% | 12.40% | 12.40% | 10.50% | 12.60% | 12.60% | |
| ft2 | 9183.88 | 4705.66 | 3908.38 | 3908.48 | 4685.10 | 3908.38 | 3908.48 | 2798.62 | 1319.33 | 1319.33 | |
| rad | 0.53 | 0.53 | 0.47 | 0.47 | 0.49 | 0.47 | 0.47 | 0.43 | 0.39 | 0.39 | |
| - | 0.20 | 0.18 | 0.25 | 0.22 | 0.20 | 0.25 | 0.22 | 0.30 | 0.21 | 0.21 | |
| Torenbeek II (1994) [16] | |||||||||||
| - | 3.75 | 3.75 | 3.75 | 3.75 | 3.75 | 3.75 | 3.75 | 3.75 | 3.75 | 3.75 | |
| kg | 560,000.00 | 268,000.00 | 253,500.00 | 276,500.00 | 368,000.00 | 242,000.00 | 233,000.00 | 137,892.08 | 73,500.00 | 89,000.00 | |
| kg | 360,910.98 | 189,852.43 | 169,000.00 | 178,000.00 | 245,000.00 | 170,000.00 | 175,000.00 | 116,500.00 | 61,000.00 | 71,500.00 | |
| m | 79.64 | 64.74 | 60.30 | 58.00 | 61.20 | 60.30 | 58.00 | 44.83 | 34.10 | 34.10 | |
| m2 | 853.21 | 437.17 | 363.10 | 363.11 | 435.26 | 363.1 | 363.11 | 260.00 | 122.57 | 122.57 | |
| - | 0.20 | 0.18 | 0.25 | 0.22 | 0.20 | 0.25 | 0.22 | 0.30 | 0.21 | 0.21 | |
| ° | 33.50 | 32.63 | 29.7 | 29.74 | 31.1 | 29.7 | 29.74 | 28 | 24.96 | 24.96 | |
| Howe Method (2000) [21] | |||||||||||
| - | 7.43 | 9.46 | 9.26 | 9.18 | 8.51 | 9.27 | 9.18 | 7.73 | 9.34 | 9.34 | |
| m2 | 853.21 | 437.17 | 363.10 | 363.11 | 435.26 | 363.1 | 363.11 | 260.00 | 122.57 | 122.57 | |
| ° | 33.50 | 32.63 | 29.7 | 29.74 | 31.1 | 29.7 | 29.74 | 28 | 24.96 | 24.96 | |
| - | 0.20 | 0.18 | 0.25 | 0.22 | 0.20 | 0.25 | 0.22 | 0.30 | 0.21 | 0.21 | |
| kg | 560,000.00 | 268,000.00 | 253,500.00 | 276,500.00 | 368,000.00 | 242,000.00 | 233,000.00 | 137,892.08 | 73,500.00 | 89,000.00 | |
| - | 4.13 | 4.13 | 4.13 | 4.13 | 4.13 | 4.13 | 4.13 | 4.13 | 4.13 | 4.13 | |
| m/s | 280.63 | 283.58 | 278.54 | 278.54 | 278.54 | 278.54 | 278.54 | 277.68 | 220.00 | 272.25 | |
| - | 11.26% | 15.49% | 16.00% | 15.30% | 11.97% | 15.00% | 15.30% | 15.00% | 15.21% | 15.21% | |
| 853 | 440 | 420 | 420 | 475 | 380 | 440 | 269 | 179 | 190 | ||
| Kundu Method (2010) [11] | |||||||||||
| - | 12.00% | 12.00% | 12.00% | 12.00% | 11.00% | 11.00% | 12.00% | 12.00% | 10.00% | 10.00% | |
| LTH Method (2011) [19] | |||||||||||
| m2 | 853.21 | 437.17 | 363.10 | 363.11 | 435.26 | 363.1 | 363.11 | 260.00 | 122.57 | 122.57 | |
| kg | 560,000.00 | 268,000.00 | 253,500.00 | 276,500.00 | 368,000.00 | 242,000.00 | 233,000.00 | 137,892.08 | 73,500.00 | 89,000.00 | |
| - | 7.43 | 9.46 | 9.26 | 9.18 | 8.51 | 9.27 | 9.18 | 7.73 | 9.34 | 9.34 | |
| - | 11.26% | 15.49% | 16.00% | 15.30% | 11.97% | 15.00% | 15.30% | 15.00% | 15.21% | 15.21% | |
| - | 9.50% | 11.30% | 12.00% | 11.30% | 9.50% | 12.00% | 11.30% | 12.00% | 11.75% | 11.75% | |
| - | 8.70% | 10.60% | 10.50% | 10.60% | 8.70% | 10.50% | 10.60% | 10.50% | 10.84% | 10.84% | |
| ° | 33.50 | 32.63 | 29.7 | 29.74 | 31.1 | 29.7 | 29.74 | 28 | 24.96 | 24.96 | |
| Elham Simple Class I Method (2013) [8] | |||||||||||
| MTOM | kg | 560,000.00 | 268,000.00 | 253,500.00 | 276,500.00 | 368,000.00 | 242,000.00 | 233,000.00 | 137,892.08 | 73,500.00 | 89,000.00 |
| Basgall Jet Transport Method (2020) [25] | |||||||||||
| MTOM | lbs | 1,234,588.67 | 590,838.86 | 558,871.83 | 609,578.15 | 811,301.12 | 533,518.67 | 513,677.07 | 304,000.00 | 162,039.76 | 196,211.41 |
| Parameter | Units | Aircraft Type—Part B | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| B787-800 | B777-200LR | B747-400 | B747-200B | B737-800 | B737-200 | L-1011-1 | DC-10/10 | DC-10/30 | ||
| Torenbeek I (1976) [14] | ||||||||||
| kg | 160,985.93 | 209,106.08 | 247,207.84 | 238,816.38 | 62,731.82 | 38,555.00 | 145,150.00 | 151,956.00 | 166,922.00 | |
| m | 56.43 | 62.28 | 62.3 | 59.64 | 34.32 | 28.35 | 47.35 | 50.40 | 50.40 | |
| ° | 29.15 | 27.93 | 35.37 | 34.62 | 22.13 | 21.83 | 31.62 | 31.29 | 31.29 | |
| - | 3.75 | 3.75 | 3.75 | 3.75 | 3.75 | 3.75 | 3.75 | 3.75 | 3.75 | |
| m | 1.80 | 1.97 | 1.65 | 2.22 | 0.89 | 0.90 | 1.92 | 2.10 | 2.10 | |
| m2 | 360.77 | 440.09 | 532.74 | 511.00 | 124.74 | 91.04 | 321.50 | 367.70 | 367.70 | |
| Shevell Method (1983) [17] | ||||||||||
| - | 3.75 | 3.75 | 3.75 | 3.75 | 3.75 | 3.75 | 3.75 | 3.75 | 3.75 | |
| ft. | 185.14 | 204.33 | 204.40 | 195.67 | 112.60 | 93.01 | 155.35 | 165.35 | 165.35 | |
| lbs | 502,500.00 | 766,000.00 | 875,000.00 | 833,000.00 | 174,200.00 | 100,000.00 | 430,012.00 | 429,901.00 | 555,036.00 | |
| lbs | 354,913.20 | 461,000.00 | 545,000.00 | 526,500.00 | 138,300.00 | 84,999.20 | 320,001.00 | 335,005.60 | 368,000.00 | |
| - | 11.15% | 12.60% | 9.95% | 12.50% | 12.40% | 12.00% | 10.70% | 11.00% | 11.00% | |
| ft2 | 3883.30 | 4737.09 | 5734.37 | 5500.36 | 1342.69 | 979.95 | 3460.60 | 3957.89 | 3957.89 | |
| rad | 0.51 | 0.49 | 0.62 | 0.60 | 0.39 | 0.38 | 0.55 | 0.55 | 0.55 | |
| - | 0.12 | 0.12 | 0.22 | 0.30 | 0.21 | 0.27 | 0.26 | 0.22 | 0.22 | |
| Torenbeek II (1994) [16] | ||||||||||
| - | 3.75 | 3.75 | 3.75 | 3.75 | 3.75 | 3.75 | 3.75 | 3.75 | 3.75 | |
| kg | 227,930.17 | 347,451.76 | 396,893.32 | 377,842.44 | 79,015.79 | 45,359.24 | 195,050.00 | 195,000.00 | 251,760.00 | |
| kg | 160,985.93 | 209,106.08 | 247,207.84 | 238,816.38 | 62,731.82 | 38,555.00 | 145,150.00 | 151,956.00 | 166,922.00 | |
| m | 56.43 | 62.28 | 62.30 | 59.64 | 34.32 | 28.35 | 47.35 | 50.40 | 50.40 | |
| m2 | 360.77 | 440.09 | 532.74 | 511.00 | 124.74 | 91.04 | 321.50 | 367.70 | 367.70 | |
| - | 0.12 | 0.12 | 0.22 | 0.30 | 0.21 | 0.27 | 0.26 | 0.22 | 0.22 | |
| ° | 32.20 | 31.34 | 38.00 | 37.50 | 25.02 | 25.00 | 35.00 | 35.00 | 35.00 | |
| Howe Method (2000) [21] | ||||||||||
| - | 8.50 | 8.53 | 7.10 | 7.00 | 9.18 | 8.83 | 6.97 | 6.91 | 6.91 | |
| m2 | 360.77 | 440.09 | 532.74 | 511.00 | 124.74 | 91.04 | 321.50 | 367.70 | 367.70 | |
| ° | 32.20 | 31.34 | 38.00 | 37.50 | 25.02 | 25.00 | 35.00 | 35.00 | 35.00 | |
| - | 0.12 | 0.12 | 0.22 | 0.30 | 0.21 | 0.27 | 0.26 | 0.22 | 0.22 | |
| kg | 227,930.17 | 347,451.76 | 396,893.32 | 377,842.44 | 79,015.79 | 45,359.24 | 195,050.00 | 195,000.00 | 251,760.00 | |
| - | 4.13 | 4.13 | 4.13 | 4.13 | 4.13 | 4.13 | 4.13 | 4.13 | 4.13 | |
| m/s | 271.77 | 277.10 | 280.63 | 280.63 | 273.56 | 176.00 | 284.53 | 265.50 | 292.65 | |
| - | 14.67% | 15.00% | 11.40% | 15.00% | 14.50% | 15.00% | 16.00% | 17.90% | 17.90% | |
| - | 381 | 440 | 660 | 480 | 189 | 130 | 400 | 399 | 380 | |
| Kundu Method (2010) [11] | ||||||||||
| - | 11.00% | 11.00% | 11.00% | 11.00% | 10.00% | 11.00% | 11.00% | 11.00% | 11.00% | |
| LTH Method (2011) [19] | ||||||||||
| m2 | 360.77 | 440.09 | 532.74 | 511.00 | 124.74 | 91.04 | 321.50 | 367.70 | 367.70 | |
| kg | 227,930.17 | 347,451.76 | 396,893.32 | 377,842.44 | 79,015.79 | 45,359.24 | 195,050.00 | 195,000.00 | 251,760.00 | |
| - | 8.50 | 8.53 | 7.10 | 7.00 | 9.18 | 8.83 | 6.97 | 6.91 | 6.91 | |
| - | 14.67% | 15.00% | 11.40% | 15.00% | 14.50% | 15.00% | 16.00% | 17.90% | 17.90% | |
| - | 12.09% | 12.30% | 9.46% | 12.00% | 11.75% | 12.00% | 12.00% | 12.00% | 12.00% | |
| - | 6.70% | 10.70% | 9.00% | 10.50% | 11.00% | 10.50% | 7.00% | 8.00% | 8.00% | |
| ° | 32.20 | 31.34 | 38.00 | 37.50 | 25.02 | 25.00 | 35.00 | 35.00 | 35.00 | |
| Elham Simple Class I Method (2013) [8] | ||||||||||
| MTOM | kg | 227,930.17 | 347,451.76 | 396,893.32 | 377,842.44 | 79,015.79 | 45,359.24 | 195,050.00 | 195,000.00 | 251,760.00 |
| Basgall Jet Transport Method (2020) [25] | ||||||||||
| MTOM | lbs | 502,500.00 | 766,000.00 | 875,000.00 | 833,000.00 | 174,200.00 | 100,000.00 | 430,012.00 | 429,901.00 | 555,036.00 |
References
- Kretov, A.; Tiniakov, D. Evaluation of the Mass and Aerodynamic Efficiency of a High Aspect Ratio Wing for Prospective Passenger Aircraft. Aerospace 2022, 9, 497. [Google Scholar] [CrossRef]
- Marano, A.D.; Diodati, G.; Paletta, N.; Di Palma, L.; Belardo, M.; Nour, P.A. Structural Scalability Preliminary Studies for the Next Generation Civil Tiltrotor Composite Wing. Aerospace 2023, 10, 478. [Google Scholar] [CrossRef]
- Dababneh, O.; Kipouros, T. Review of aircraft wing mass estimation methods. Aerosp. Sci. Technol. 2018, 72, 256–266. [Google Scholar] [CrossRef]
- Bravo-Mosquera, P.D.; Catalano, F.M.; Zingg, D.W. Unconventional aircraft for civil aviation: A review of concepts and design methodologies. Prog. Aerosp. Sci. 2022, 131, 100813. [Google Scholar] [CrossRef]
- Zhu, W.; Fan, Z.; Yu, X. Structural mass prediction in conceptual design of blended-wing-body aircraft. Chin. J. Aeronaut. 2019, 32, 2455–2465. [Google Scholar] [CrossRef]
- Takahashi, T.T.; Lemonds, T. Prediction of wing structural mass for transport category aircraft conceptual design. In Proceedings of the 15th AIAA Aviation Technology, Integration, and Operations Conference, Dallas, TX, USA, 22–26 June 2015. [Google Scholar] [CrossRef]
- Chiozzotto, G.P. Wing weight estimation in conceptual design: A method for strut-braced wings considering static aeroelastic effects. CEAS Aeronaut. J. 2016, 7, 499–519. [Google Scholar] [CrossRef]
- Elham, A. Weight Indexing for Multidisciplinary Design Optimization of Lifting Surfaces. Ph.D. Thesis, Delft University of Technology, Delft, The Netherlands, 2013. [Google Scholar]
- Murphy, N. Analytical Wing Weight Prediction/Estimation Using Computer Based Design Techniques. Ph.D. Thesis, Cranfield Institute of Technology, Cranfield, UK, 1987. [Google Scholar]
- Ardema, M.; Chambers, M.; Patron, A.; Hahn, A.; Miura, H.; Moore, M. Analytical Fuselage and Wing Weight Estimation of Transport Aircraft; NASA: Washington, DC, USA, 1996.
- Kundu, A. Aircraft Design; Cambridge University Press: Cambridge, UK, 2010. [Google Scholar]
- Raymer, D.P. Aircraft Design: A Conceptual Approach, 4th ed.; AIAA Education Series; AIAA: Washington, DC, USA, 2006. [Google Scholar]
- Roskam, J. Airplane Design: Part III—Layout Design of Cockpit, Fuselage, Wing and Empennage: Cutaways and Inboard Profiles; Roskam Aviation and Engineering Corporation: Lawrence, KS, USA, 1989. [Google Scholar]
- Torenbeek, E. Synthesis of Subsonic Airplane Design; Delft University Press: Delft, The Netherlands; Kluwer Academic Publishers: Dordrecht, The Netherlands, 1976. [Google Scholar]
- Glatt, C. WAATS—A Computer Program for Weights Analysis of Advanced Transportation Systems; NASA: Washington, DC, USA, 1974. [Google Scholar]
- Torenbeek, E. Optimum Wing Area, Aspect Ratio and Cruise Altitude for Long Range Transport Aircraft; Delft University of Technology: Delft, The Netherlands, 1994. [Google Scholar]
- Shevell, R.S. Fundamentals of Flight; Prentice–Hall: Lansing, MI, USA, 1983. [Google Scholar]
- St. John, R.S. The derivation and application of analytical–statistical weight prediction techniques. In Proceedings of the 28th Annual Conference, San Francisco, CA, USA, 12–16 May 1969; Society of Allied Weight Engineers, Inc.: Melbourne Beach, FL, USA, 1969. SAWE Paper No. 810. [Google Scholar]
- Dorbath, F. Large Civil Jet Transport (MTOM >40t) Statistical Mass Estimation. In DLR-LY/Airbus, LTH MA 401 12-01. 2011. Available online: https://www.researchgate.net/publication/312158007_Large_Civil_Jet_Transport_MTOM_40t_-_Statistical_Mass_Estimation (accessed on 10 February 2025).
- Elham, A.; van Tooren, M.J.L.; La Rocca, G. An Advanced Quasi-Analytical Weight Estimation Method for Airplane Lifting Surfaces. In Proceedings of the 71st International Conference on Mass Properties, Bavaria, Germany, 5–10 May 2012; Society of Allied Weight Engineers, Inc.: Melbourne Beach, FL, USA, 2012. SAWE Paper No. 3571. [Google Scholar]
- Howe, D. Aircraft Conceptual Design Synthesis; Professional Engineering Publishing Limited: London, UK; Bury St. Edmunds, UK, 2000. [Google Scholar]
- Kundu, A.; Price, M.; Riordan, M.D. Conceptual Aircraft Design an Industrial Approach; John Wiley & Sons: Hoboken, NJ, USA, 2019. [Google Scholar]
- Howe, D. The prediction of aircraft wing mass, Part G. J. Aerosp. Eng. 1996, 210, 135–145. [Google Scholar]
- Chiozzotto, G.P. Initial Weight Estimate of Advanced Transport Aircraft Concepts Considering Aeroelastic Effects. In Proceedings of the 55th AIAA Aerospace Sciences Meeting, AIAA SciTech Forum, Grapevine, TX, USA, 9—13 January 2017. [Google Scholar]
- Basgall, B.; Wanbo, L.; Truman, C.; Willem, A.A. Aircraft Design Weight Methods Comparison and Improvement. In Proceedings of the AIAA SciTech Forum, Orlando, FL, USA, 6–10 January 2020. [Google Scholar]
- Horvath, B.L.; Wells, D.P. Comparison of Aircraft Conceptual Design Weight Estimation Methods to the Flight Optimization System. In Proceedings of the 2018 AIAA Aerospace Sciences Meeting, Kissimmee, FL, USA, 8–12 January 2018. [Google Scholar]
- McCullers, L.A. Aircraft Configuration Optimization Including Optimized Flight Profiles, Multi-Disciplinary Analysis and Optimization Part 1; NASA CP-2327; NASA: Washington, DC, USA, 1984.
- Yan, M.J.; Elham, A. Designing high aspect ratio wings: A review of concepts and approaches. Prog. Aerosp. Sci. 2024, 145, 100983. [Google Scholar] [CrossRef]
- Jemitola, P.; Monterzino, G.; Fielding, J. Wing mass estimation algorithm for medium range box wing aircraft. Aeronaut. J. 2013, 117, 329–340. [Google Scholar] [CrossRef]
- Yan, M.J.; Elham, A. Initial weight estimation of twin-fuselage configuration in aircraft conceptual design. In Proceedings of the 80th SAWE International Conference on Mass Properties Engineering, Cocoa Beach, FL, USA, 10–23 October 2021. [Google Scholar]
- Moerland, E.; Pfeiffer, T.; Bohnke, D.; Jepsen, J.; Freund, S.; Liersch, C.M.; Chiozzotto, G.P.; Klein, C.; Scherer, J.; Hasan, Y.J.; et al. On the design of a strut-braced wing configuration in a collaborative design environment. In Proceedings of the 17th AIAA Aviation Technology, Integration, and Operations Conference, American Institute of Aeronautics and Astronautics, Denver, CO, USA, 5–9 June 2017. [Google Scholar] [CrossRef]
- Howe, D. DAeT 9317—Aircraft Mass Prediction, Department of Aerospace Engineering; Cranfield University: Cranfield, UK, 2009. [Google Scholar]
- Effing, T.; Stephan, R.; Stumpf, E. Influence of a Detailed Mass Estimation for a Wing with known Flight Shape in Conceptual Overall Aircraft Design; Deutsche Gesellschaft für Luft- und Raumfahrt-Lilienthal-Oberth e.V.: Bonn, Germany, 2023. [Google Scholar] [CrossRef]
- Castilla, R.A.; Lutz, F.; Jezegou, J.; Benard, E. Wing Structural Model for Overall Aircraft Design of Distributed Electric Propulsion General Aviation and Regional Aircraft. Aerospace 2022, 9, 5. [Google Scholar] [CrossRef]
- Scholz, D. Mass Estimation of Folding Wings. In Proceedings of the 83rd International Conference on Mass Properties Engineering, Online, 20–22 May 2024; Society of Allied Weight Engineers (SAWE): Melbourne Beach, FL, USA, 2024. [Google Scholar] [CrossRef]
- Torenbeek, E. Prediction of wing group weight for preliminary design. Aircr. Eng. Aerosp. Technol. 1971, 43, 16–21. [Google Scholar] [CrossRef]
- Torenbeek, E. Development and Application of a Comprehensive, Design-sensitive Weight Prediction Method for Wing Structures of Transport Category Aircraft; Report LR-693; Delft University of Technology: Delft, The Netherlands, 1992. [Google Scholar]
- Roeseler, W.G.; Sarh, B.; Kismarton, M.U. Composite structures: The first 100 years. In Proceedings of the 16th International Conference on Composite Materials, Kyoto, Japan, 9 July 2007; pp. 1–10. [Google Scholar]
- Gardiner, G. Resin-infused MS-21 wings and wingbox. High Perform. Compos. 2014, 22, 29. [Google Scholar]
- Pierce, R.S.; Falzon, B.G. Simulating Resin Infusion through Textile Reinforcement Materials for the Manufacture of Complex Composite Structures. Engineering 2017, 3, 596–607. [Google Scholar] [CrossRef]
- Nicolai, L.M.; Carichner, G. Fundamentals of Aircraft and Airship Design; AIAA Education: Reston, VA, USA, 2010. [Google Scholar]
- Dababneh, O. Multidisciplinary Design Optimisation for Aircraft Wing Mass Estimation. Ph.D. Thesis, Cranfield University, Cranfield, UK, 2016. [Google Scholar]
- PaceTxtgroup. Pacelab APD. Version 1.1. Aircraft Preliminary Design Software—APD Mass Report; PACE Aerospace & IT: Berlin, Germany, 2009. [Google Scholar]
- Seywald, K. Wing mass prediction considering quasi-static nonlinear aeroelasticity. In PEGASUS—AIAA Student Conference, VIII ed.; Poitiers: Davie, FL, USA, 2012. [Google Scholar]
- Macci, S.H. Semi-analytical method for predicting wing structural mass. In Proceedings of the 54th Annual Conference, Huntsville, AL, USA, 22–24 May 1995. SAWE Paper No. 2282. [Google Scholar]
- Anderson, W.; Udin, S. Wing mass formula for subsonic aircraft. J. Aircr. 1991, 29, 725–727. [Google Scholar]
- Bai, L.; Deng, Z.; Zhang, X.; Xia, M.; Wing, J.W.; Wing, A. Weight Estimation Method Based on Wing-box Beam Design. In SAWE Tech Fair, Virtual Conference; SAWE Paper No. 3709; Society of Allied Weight Engineers, Inc.: Melbourne Beach, FL, USA, 2020. [Google Scholar]
- Hurlimann, F.; Kelm, R.; Dugas, M.; Oltmann, K.; Kress, G. Mass estimation of transport aircraft wingbox structures with a CAD/CAE-based multidisciplinary process. Aerosp. Sci. Technol. 2010, 15, 323–333. [Google Scholar] [CrossRef]
- Masuko, T.; Imamura, T.; Rinoie, K. Conceptual design of biplane with high aspect ratio wings as next generation civil jet transport, SciTech AIAA 2016-1032. In Proceedings of the 54th AIAA Aerospace Sciences Meeting, San Diego, CA, USA, 4–8 January 2016. [Google Scholar]
- Ma, Y.; Karpuk, S.; Elham, A. Conceptual design and comparative study of strut-braced wing and twin-fuselage aircraft configurations with ultra-high aspect ratio wing. Aerosp. Sci. Technol. 2022, 121, 107395. [Google Scholar] [CrossRef]
- Ma, Y.; Yan, J.; Elham, A. Initial weight estimation of twin-fuselage configuration in aircraft conceptual design. Proc. Inst. Mech. Eng. Part G J. Aerosp. Eng. 2023, 23, 130–140. [Google Scholar] [CrossRef]
- booksite.elsevier.com. (n.d.). Butterworth-Heinemann—Civil Jet Aircraft Design—Aircraft Data File—Airbus Aircraft. Available online: https://booksite.elsevier.com/9780340741528/appendices/data-a/table-1/default.htm (accessed on 13 December 2023).
- Airplane Characteristics for Airport Planing. (n.d.). Available online: https://aircraft.airbus.com/sites/g/files/jlcbta126/files/2023-02/Airbus-Commercial-Aircraft-AC-A300-Dec-2009.pdf (accessed on 13 December 2023).
- A350 Aircraft Characteristics Airport and Maintenance Planning AC@A350. (n.d.). Available online: https://aircraft.airbus.com/sites/g/files/jlcbta126/files/2023-02/Airbus-Commercial-Aircraft-AC-A350-900-1000.pdf (accessed on 13 December 2023).
- A380 Aircraft Characteristics Airport and Maintenance Planning AC. (n.d.). Available online: https://aircraft.airbus.com/sites/g/files/jlcbta126/files/2023-12/ac_a380_1223.pdf (accessed on 13 December 2023).
- booksite.elsevier.com. (n.d.). Butterworth-Heinemann—Civil Jet Aircraft Design—Aircraft Data File—Boeing Aircraft. Available online: https://booksite.elsevier.com/9780340741528/appendices/data-a/table-2/table.htm (accessed on 13 December 2023).
- booksite.elsevier.com. (n.d.). Butterworth-Heinemann—Civil Jet Aircraft Design—Aircraft Data File—Boeing Aircraft. Available online: https://booksite.elsevier.com/9780340741528/appendices/data-a/table-3/table.htm (accessed on 13 December 2023).
- booksite.elsevier.com. (n.d.). Butterworth-Heinemann—Civil Jet Aircraft Design—Aircraft Data File—Boeing Aircraft. Available online: https://booksite.elsevier.com/9780340741528/appendices/data-a/table-4/table.htm (accessed on 13 December 2023).
- booksite.elsevier.com. (n.d.). Butterworth-Heinemann—Civil Jet Aircraft Design—Aircraft Data File—Douglas, Boeing and Lockheed Aircraft. Available online: https://booksite.elsevier.com/9780340741528/appendices/data-a/table-6/table.htm (accessed on 13 December 2023).
- 737 Airplane Characteristics for Airport Planning. 2020. Available online: https://www.boeing.com/resources/boeingdotcom/commercial/airports/acaps/737_RevA.pdf (accessed on 13 December 2023).
- Min, K.; Plumley, R.; Brooks, A. Boeing 747 AOE 4124 Spring 2007. 2007. Available online: https://archive.aoe.vt.edu/mason/Mason_f/B747PresS07.pdf?q=747 (accessed on 13 December 2023).
- 787 Airplane Characteristics for Airport Planning. 2018. Available online: https://www.boeing.com/resources/boeingdotcom/commercial/airports/acaps/787.pdf (accessed on 13 December 2023).
- 777-200LR/-300ER/-Freighter Airplane Characteristics for Airport Planning. 2022. Available online: https://www.boeing.com/content/dam/boeing/boeingdotcom/commercial/airports/acaps/777_2_2er_3.pdf (accessed on 10 February 2025).
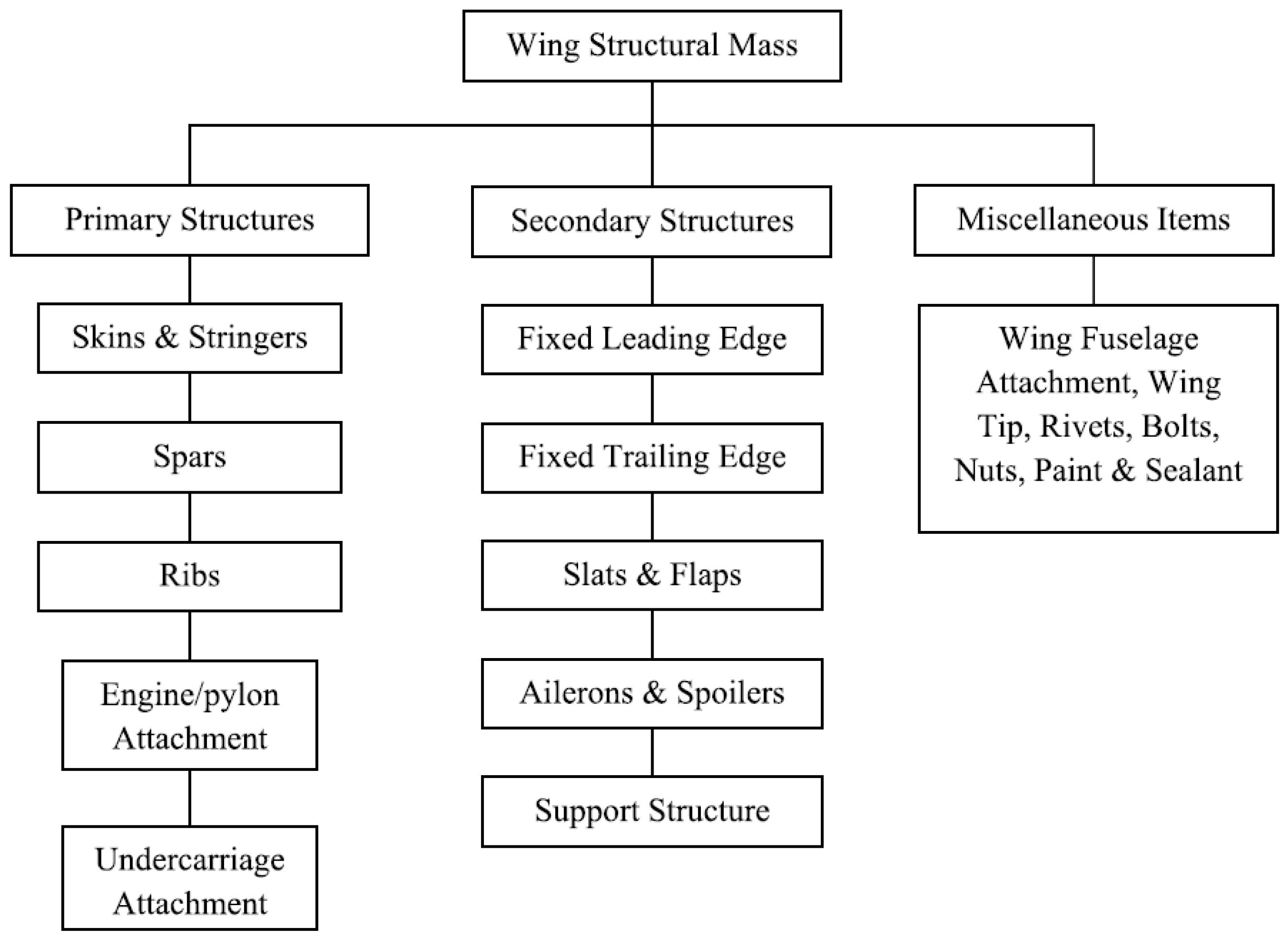
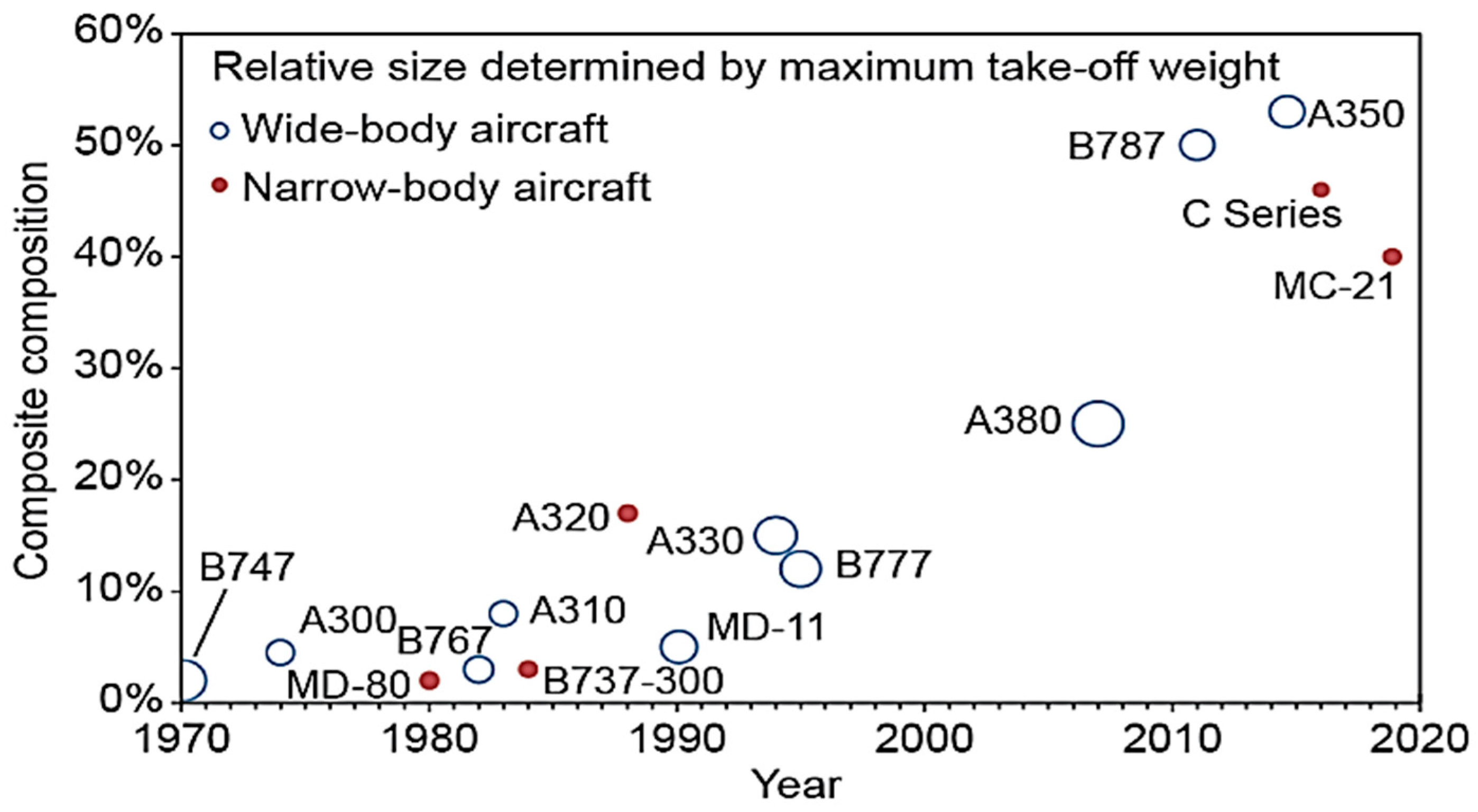
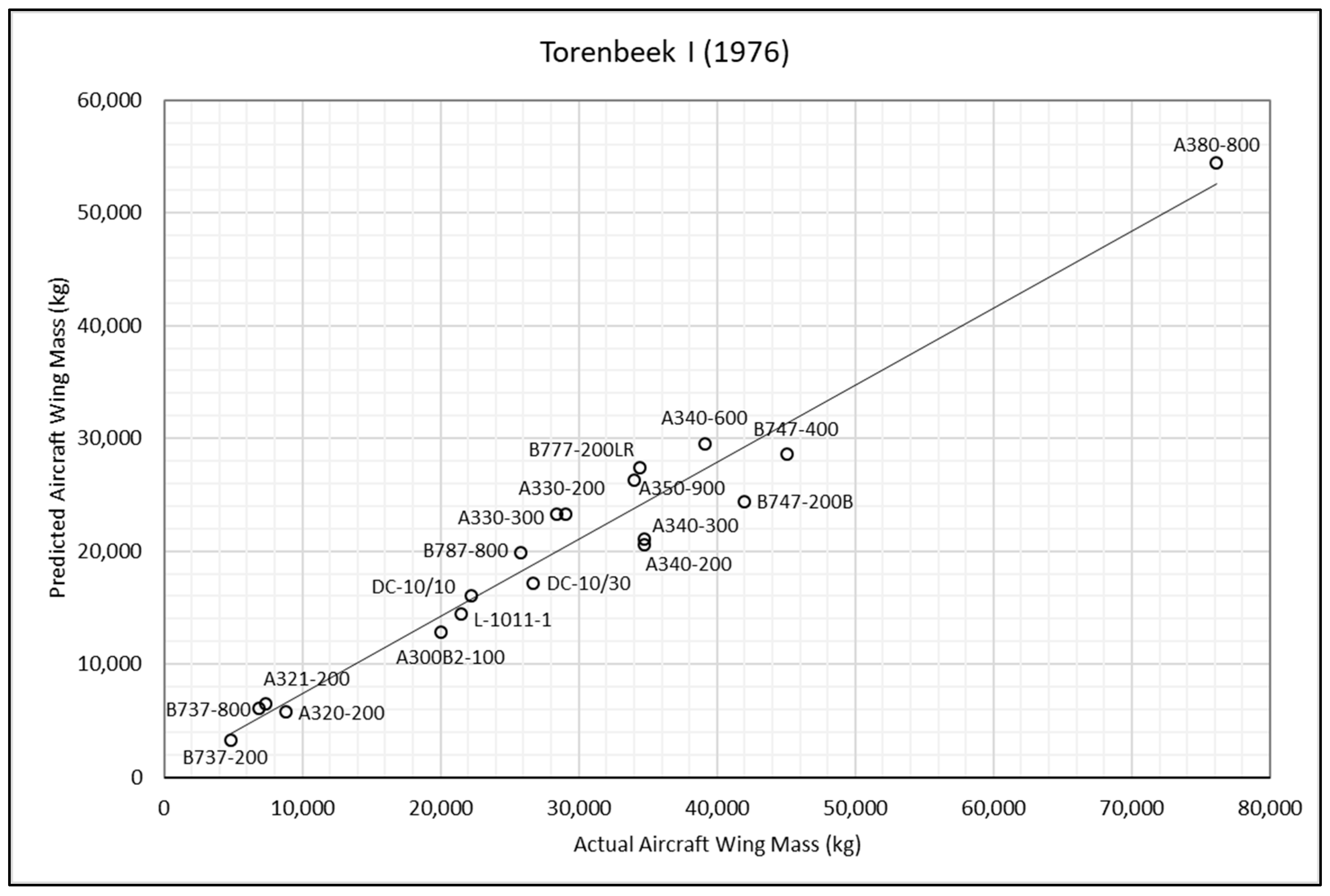
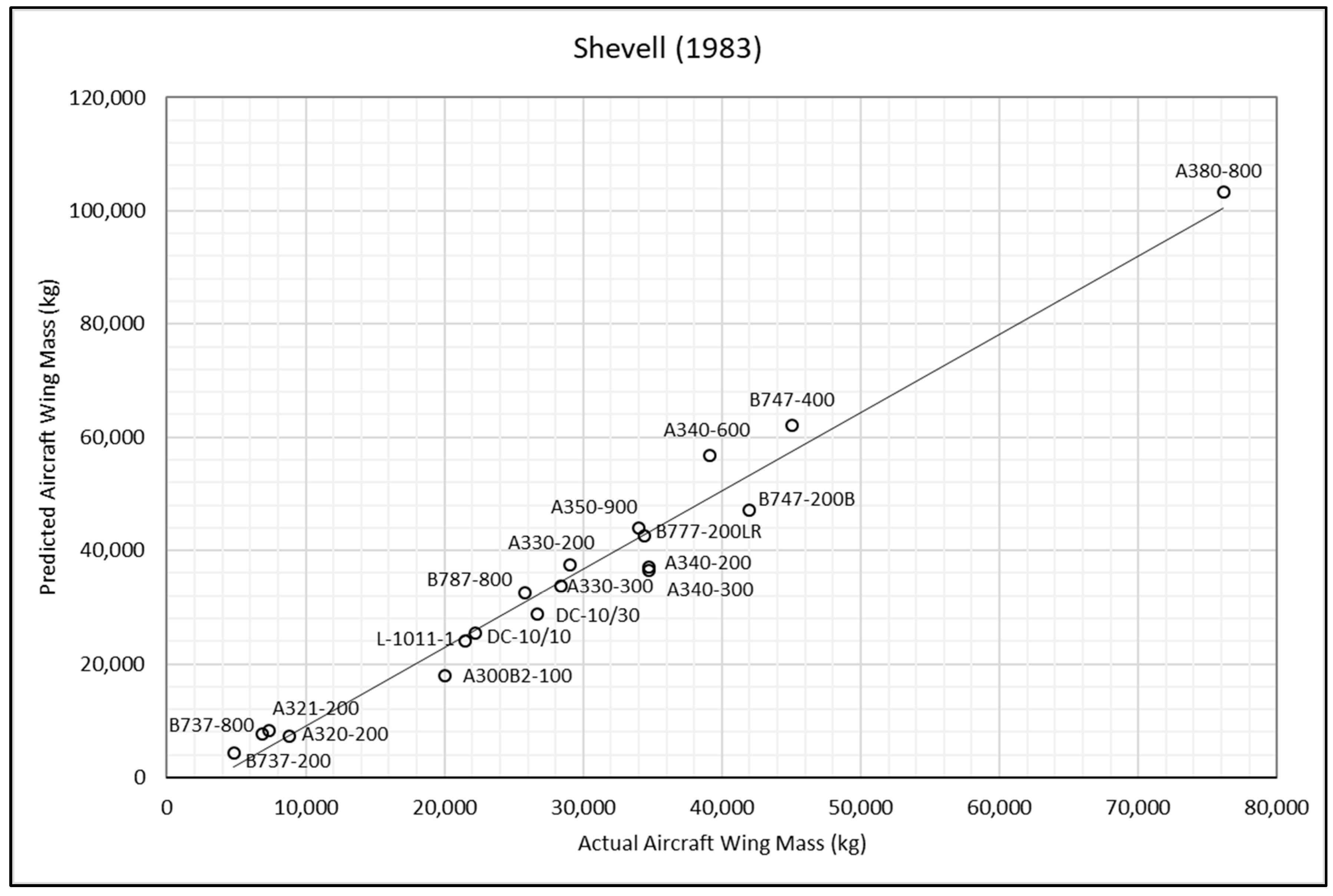




| Author | Mass Fraction | Graphical Regression | Semi-Empirical | Semi-Analytical | Beam Theory | Classical Plate Theory | Strength/ Stiffness Analysis | Finite Element Method |
|---|---|---|---|---|---|---|---|---|
| Murphy [9] | Purely Statistical | Hybrid Analytical | Purely Analytical | |||||
| Ardema et al. [10] | Empirical Regression | Plate Theory | Finite Element Method | |||||
| Kundu [11] | Rapid Method | Graphical Method | Semi-Empirical Method | |||||
| Elham [8] | Class I Method | Class II Method | Class II & 1/2 Method | Class III Method | ||||
| Dababneh [3] | Empirical Method | Analytical Method | Finite Element Analysis Method | |||||
| Group | Mid-Sized Twin-Engine Aircraft | Large Turbofan Aircraft | ||
|---|---|---|---|---|
| Turboprop | Turbofan | 2-Engines | 4-Engines | |
| Wing | 7–9% | 9–11% | 10–12% | 11–12% |
| Variable | Symbol | Valid Range | Unit |
|---|---|---|---|
| Wing mass | MW | 4100–50,300 | [kg] |
| Reference wing area | Sref | 75–550 | [m2] |
| Maximum takeoff mass | Mto | 40,000–400,000 | [kg] |
| Representative thickness-to-chord ratio | 0.10–0.15 | [-] | |
| Aspect ratio | AR | 6.9–9.6 | [-] |
| Quarter-chord sweep angle | 15.0–37.5 | [°] |
| Aircraft Type | Configuration |
|---|---|
| Airbus A380-800 | 4-Engine |
| Airbus A350-900 | 2-Engine |
| Airbus A340-200 | 4-Engine |
| Airbus A340-300 | 4-Engine |
| Airbus A340-600 | 4-Engine |
| Airbus A330-200 | 2-Engine |
| Airbus A330-300 | 2-Engine |
| Airbus A300B2-100 | 2-Engine |
| Airbus A320-200 | 2-Engine |
| Airbus A321-200 | 2-Engine |
| Boeing 787-800 | 2-Engine |
| Boeing 777-200LR | 2-Engine |
| Boeing 747-400 | 4-Engine |
| Boeing 747-200B | 4-Engine |
| Boeing 737-800 | 2-Engine |
| Boeing 737-200 | 2-Engine |
| Lockheed L-1011-1 | 3-Engine |
| McDonnell Douglas DC-10/10 | 3-Engine |
| McDonnell Douglas DC-10/30 | 3-Engine |
| Aircraft Type | MTOM [kg] | Actual Mass * [kg] | Error [%] | |||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Torenbeek I | Shevell | Torenbeek II | Howe | Kundu | LTH | Elham | Basgall | |||
| A380-800 | 560,000 | 76,122 [43] | −28.5 | 35.6 | 15.3 | −37.5 | −11.7 | 8.2 | 37.3 | 7.1 |
| A350-900 | 268,000 | 33,969 [43] | −22.5 | 29.4 | 16.1 | −13.2 | −5.3 | 28.9 | 22.5 | −13.2 |
| A340-200 | 253,500 | 34,757 [19,44] | −40.9 | 6.9 | −3.4 | −21.8 | −12.5 | −0.6 | 11.6 | 17.4 |
| A340-300 | 276,500 | 34,747 [45] | −39.2 | 4.7 | −6.1 | −16.9 | −12.5 | 5.6 | 24.5 | 21.2 |
| A340-600 | 368,000 | 39,096 [43] | −24.5 | 45.5 | 26.7 | 3.7 | 3.5 | 25.6 | 58.2 | −38.0 |
| A330-200 | 242,000 | 29,040 [43] | −19.8 | 29.4 | 17.4 | −6.1 | −8.3 | 18.6 | 26.1 | −4.1 |
| A330-300 | 233,000 | 28,402 [43] | −18.2 | 19.0 | 8.5 | −11.2 | −1.6 | 17.4 | 23.0 | −0.6 |
| A300B2-100 | 137,892 | 20,017 [46] | −36.0 | −10.2 | −13.5 | −22.3 | −17.3 | −23.6 | −9.4 | −0.3 |
| A320-200 | 73,500 | 8811 [45,47] | −34.4 | −17.1 | −16.6 | −32.2 | −16.6 | −3.8 | −6.3 | −7.6 |
| A321-200 | 89,000 | 7332 [43] | −11.9 | 12.0 | 10.8 | 6.2 | 21.4 | 29.7 | 43.0 | −5.1 |
| B787-800 | 227,930 | 25,773 [43] | −22.9 | 26.2 | 16.3 | −8.8 | −2.7 | 18.7 | 31.8 | 7.9 |
| B777-200LR | 347,452 | 34,377 [43] | −20.3 | 24.1 | 10.4 | −5.9 | 11.2 | 24.4 | 67.4 | −18.8 |
| B747-400 | 396,893 | 45,030 [43] | −36.5 | 37.8 | 20.6 | −8.5 | −3.0 | 8.6 | 50.9 | −8.3 |
| B747-200B | 377,842 | 41,696 [45] | −41.9 | 12.3 | −0.2 | −7.1 | −1.0 | −5.6 | 52.2 | −6.6 |
| B737-800 | 79,016 | 6868 [43] | −11.7 | 11.2 | 11.7 | 4.4 | 15.0 | 28.8 | 31.6 | 21.5 |
| B737-200 | 45,359 | 4814 [44] | −32.2 | −9.6 | −5.1 | −39.0 | 3.6 | 2.4 | −6.2 | 17.3 |
| L-1011-1 | 195,050 | 21,502 [10] | −32.9 | 11.9 | 6.1 | 0.0 | −0.2 | −9.8 | 30.1 | 14.3 |
| DC-10/10 | 195,000 | 22,230 [46] | −27.7 | 14.1 | 8.8 | −8.6 | −3.5 | −9.1 | 25.8 | 14.0 |
| DC-10/30 | 251,760 | 26,712 [46] | −35.7 | 7.7 | 0.3 | −3.0 | 3.7 | −13.8 | 44.0 | 10.6 |
| RMSPE | 30 | 22 | 13 | 17 | 10 | 17 | 36 | 15 | ||
| Aircraft Type | MTOM [kg] | Reference Mass [kg] | Predicted Mass [kg] | Error [%] |
|---|---|---|---|---|
| Conventional Transport Type Aircraft | ||||
| DLR-F11 [48] | 230,300 | 27,636 | 27,538 | −0.4 |
| NASA-CRM-Metallic [42] | 260,000 | 30,745 | 31,585 | 2.6 |
| NASA-CRM-Composite [42] | 260,000 | 24,114 | 25,268 | 4.6 |
| Unconventional Transport Type Aircraft | ||||
| Biplane [49] | 153,197 | 18,866 | 17,370 | −8.6 |
| Short Range—Strut Based Wing—SR-SBW [50] | 22,229 | 2103 | 1960 | −7.3 |
| Medium Range—Strut Based Wing—MR-SBW [50] | 67,929 | 9393 | 6927 | −35.6 |
| Long Range—Strut Based Wing—LR-SBW [50] | 262,962 | 47,401 | 31,992 | −48.2 |
| Short Range—Twin Fuselage—SR-TF [50] | 22,945 | 1482 | 2031 | 27.0 |
| Medium Range—Twin Fuselage—MR-TF [51] | 59,218 | 5755 | 5932 | 3.0 |
| Long Range—Twin Fuselage—LR-TF [51] | 243,926 | 39,323 | 29,387 | −33.8 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dababneh, O.; Conway-Smith, J.T. An Evaluation of the Accuracy of Existing Empirical and Semi-Empirical Methods for Predicting the Wing Mass of Large Transport Aircraft. Aerospace 2025, 12, 142. https://doi.org/10.3390/aerospace12020142
Dababneh O, Conway-Smith JT. An Evaluation of the Accuracy of Existing Empirical and Semi-Empirical Methods for Predicting the Wing Mass of Large Transport Aircraft. Aerospace. 2025; 12(2):142. https://doi.org/10.3390/aerospace12020142
Chicago/Turabian StyleDababneh, Odeh, and James T. Conway-Smith. 2025. "An Evaluation of the Accuracy of Existing Empirical and Semi-Empirical Methods for Predicting the Wing Mass of Large Transport Aircraft" Aerospace 12, no. 2: 142. https://doi.org/10.3390/aerospace12020142
APA StyleDababneh, O., & Conway-Smith, J. T. (2025). An Evaluation of the Accuracy of Existing Empirical and Semi-Empirical Methods for Predicting the Wing Mass of Large Transport Aircraft. Aerospace, 12(2), 142. https://doi.org/10.3390/aerospace12020142





