Numerical Prediction of Fatigue Life for Landing Gear Considering the Shock Absorber Travel
Abstract
1. Introduction
2. Research on Fatigue Load Decomposition
2.1. Fatigue Loads of Whole MLG 3D Model
2.2. Equivalent Conversion of MLG Loads
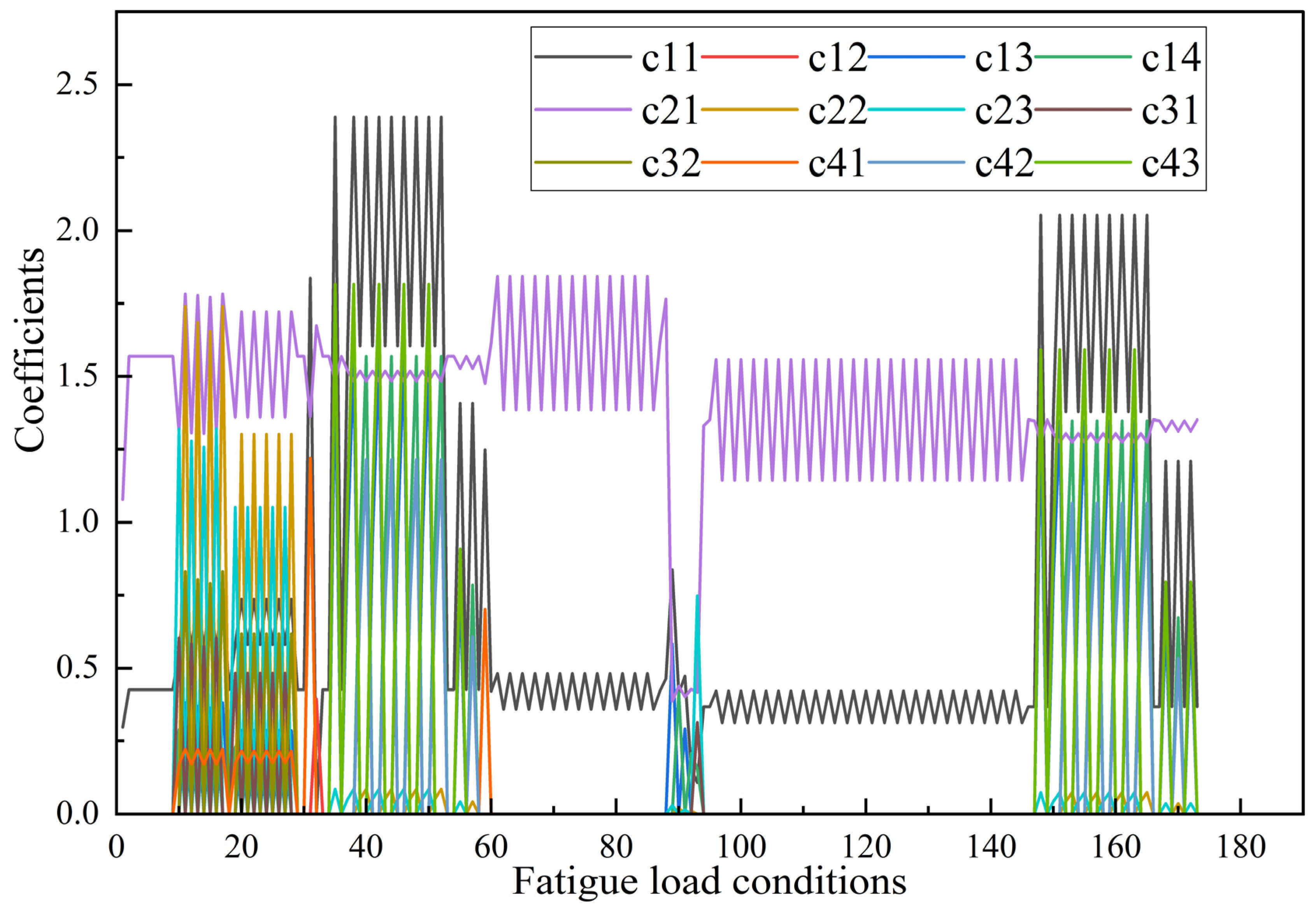
2.3. Load Coefficients for Unit Load Case
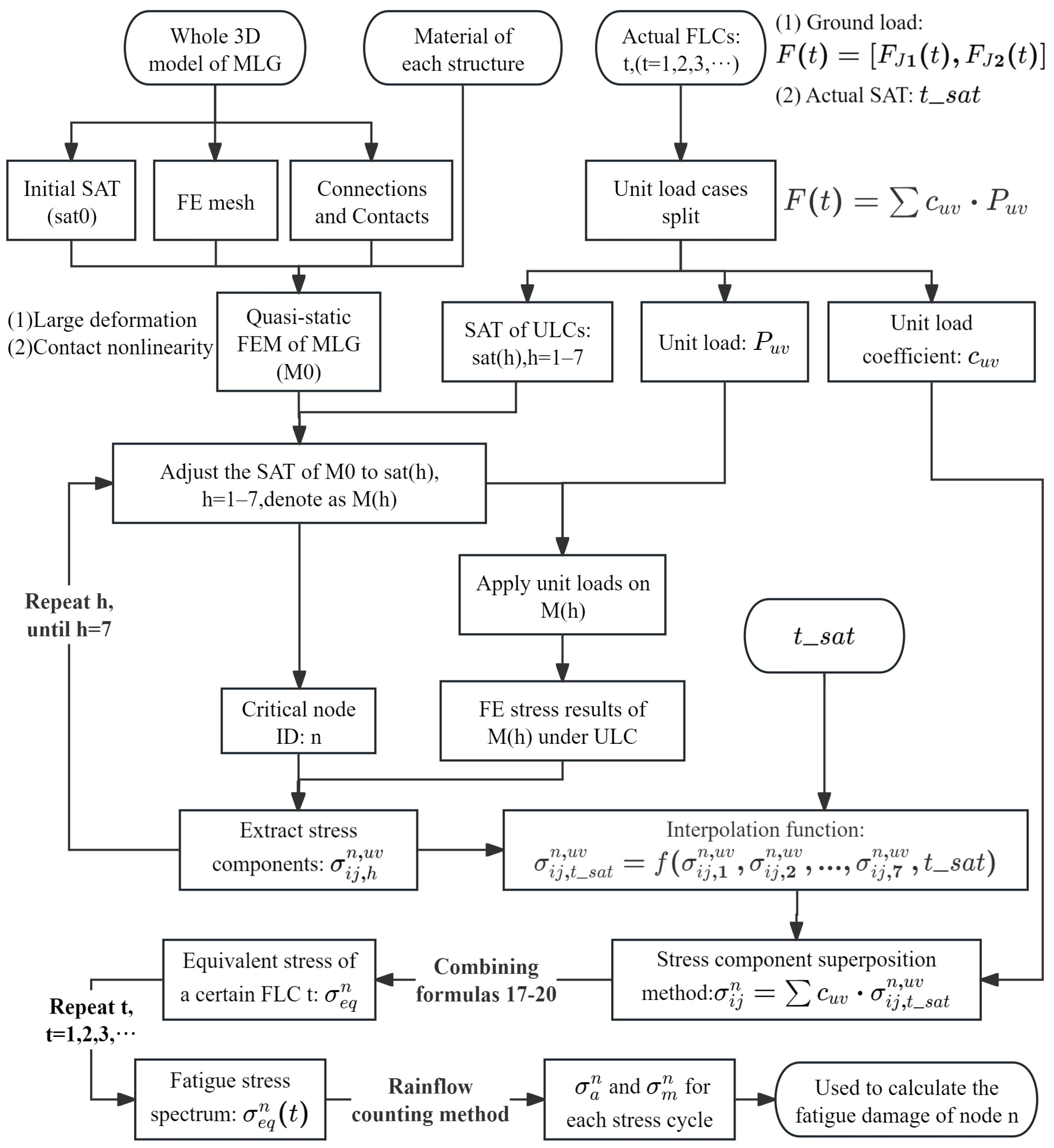
3. Stress Component Superposition Method
3.1. Quasi-Static Finite Element Modeling
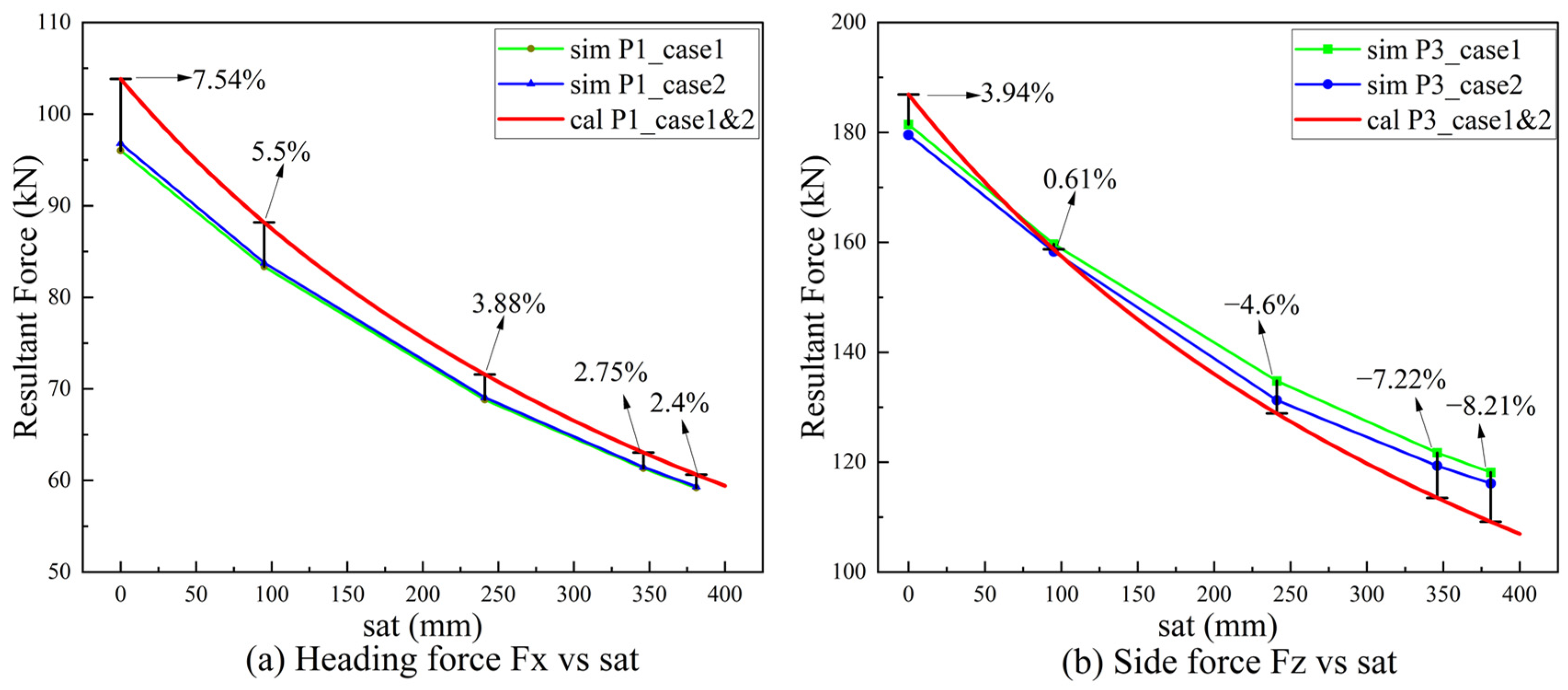
3.2. FEM Internal Force Validation
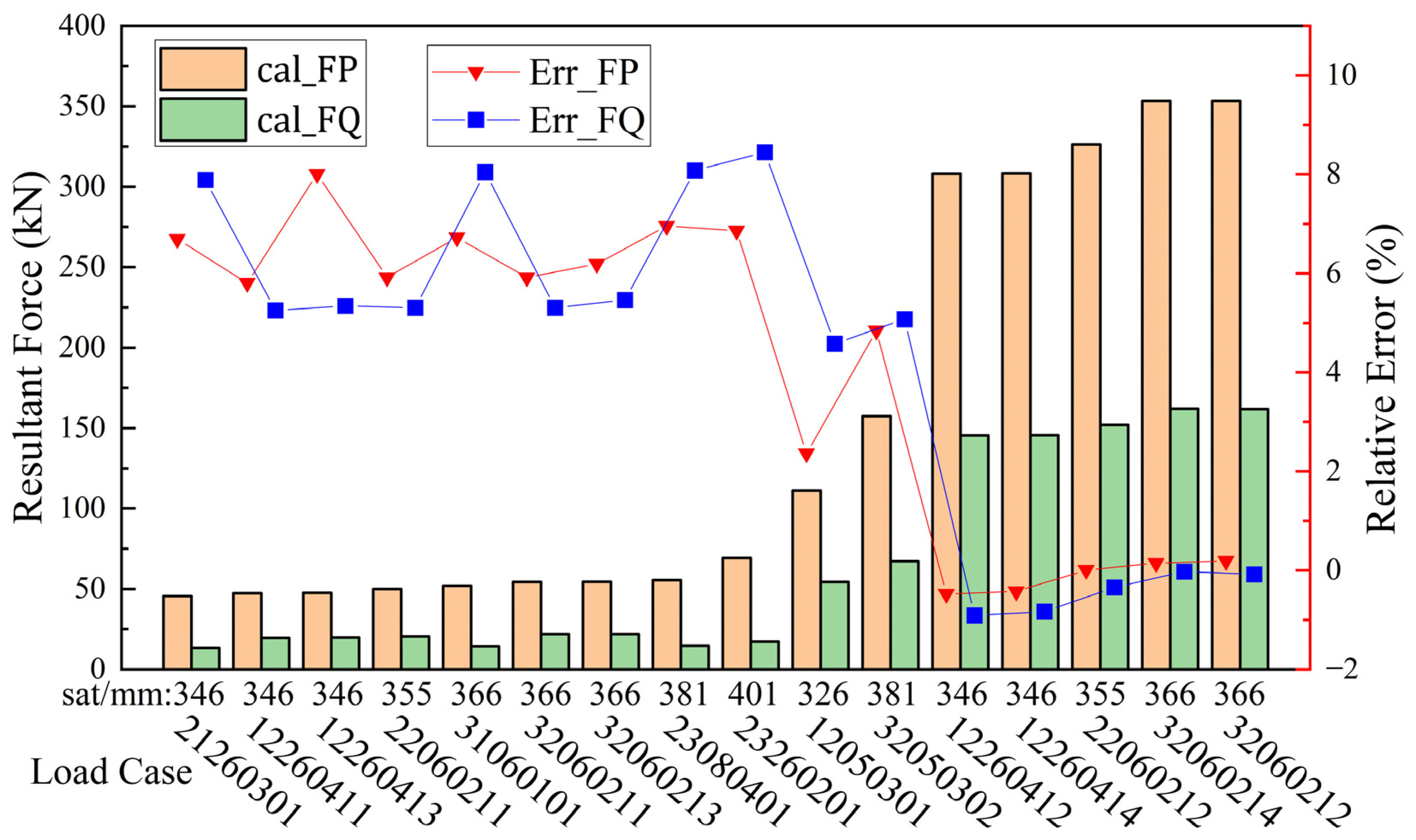
3.3. Method Validation
4. Fatigue Life Evaluation of the Main Fitting
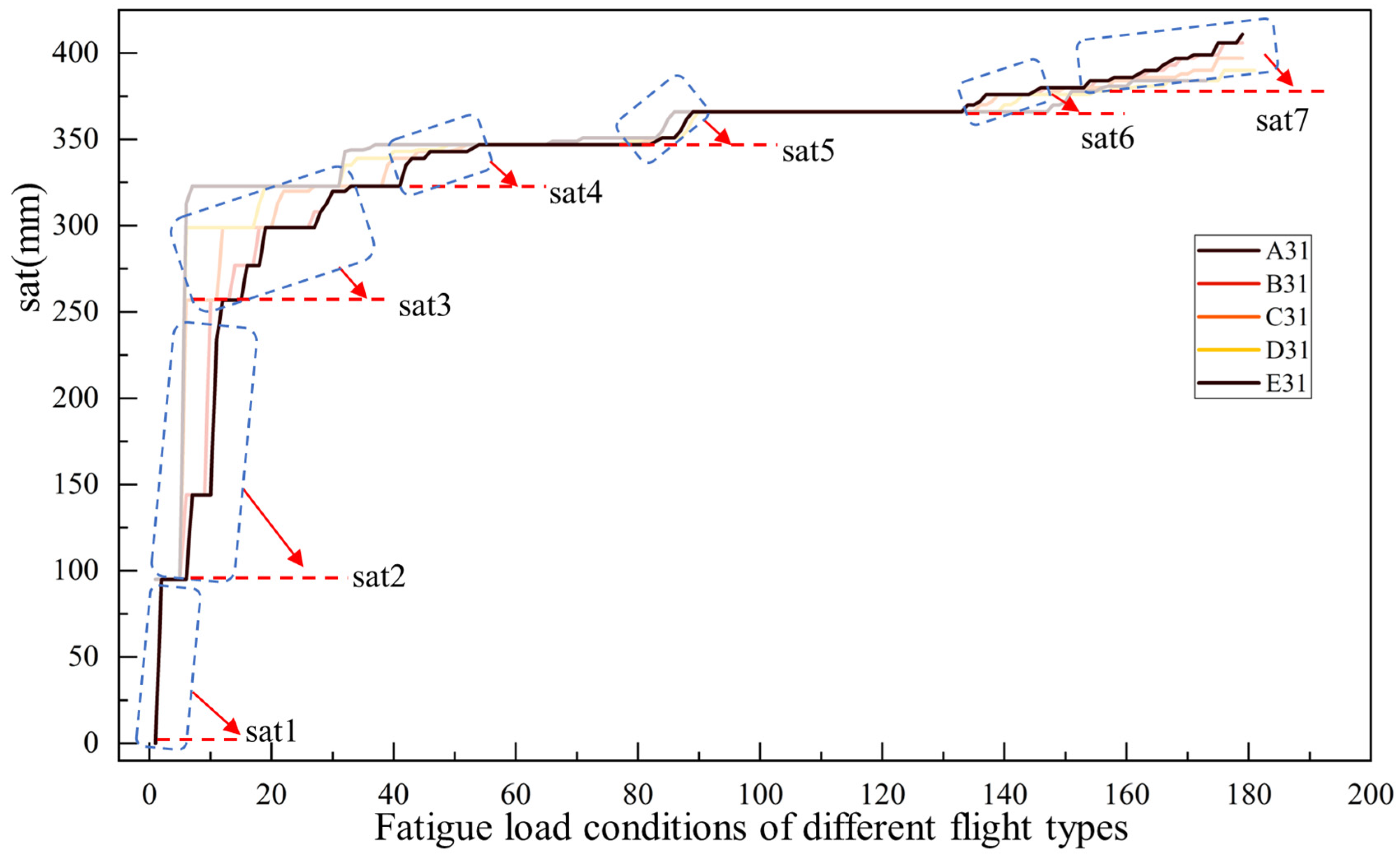
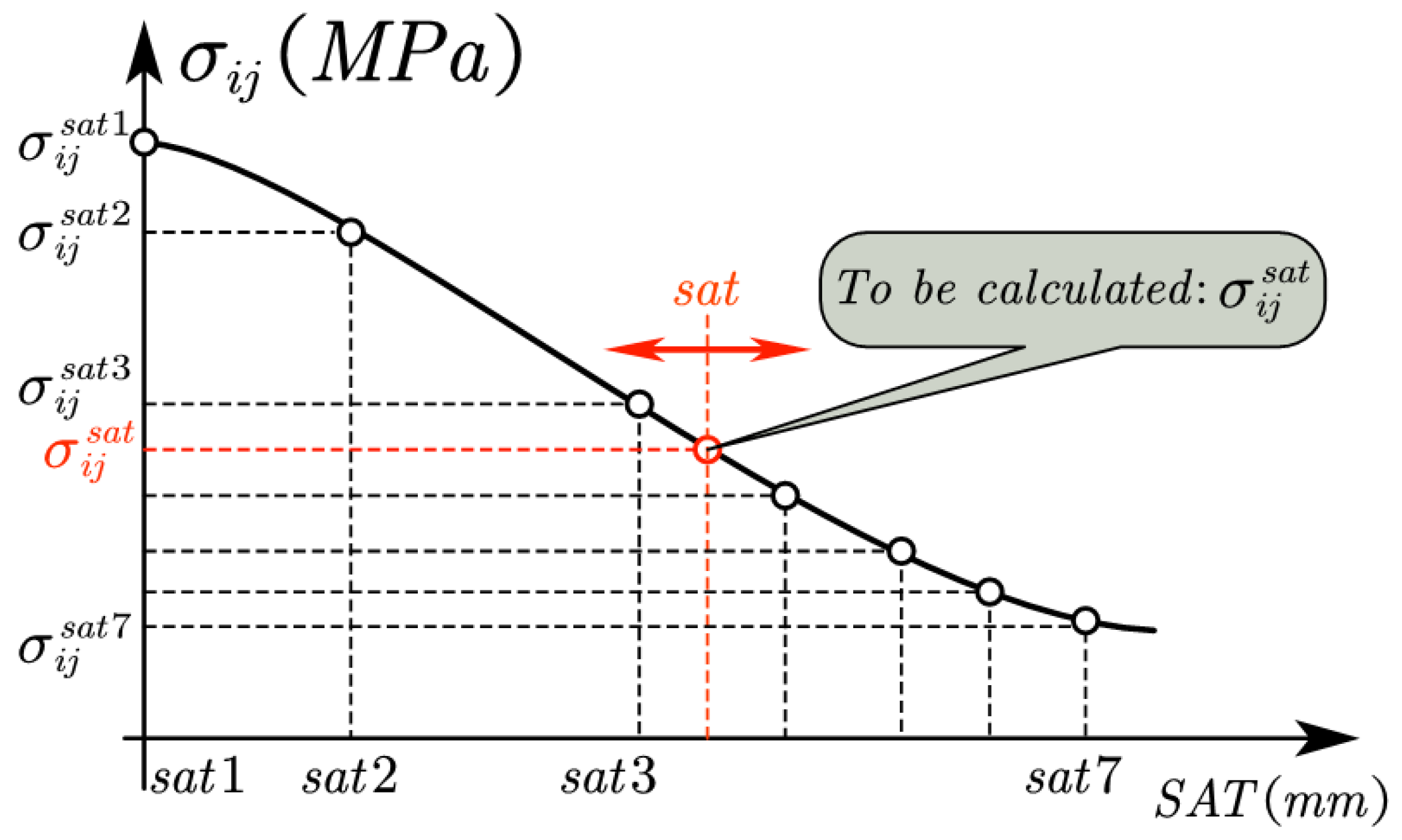
4.1. Equivalent Simplification of SAT
4.2. Computational Methodology for Dynamic Stress
4.3. Fatigue Life Calculation
5. Result Analysis and Discussion
5.1. Effect of SAT on the Load Transmission
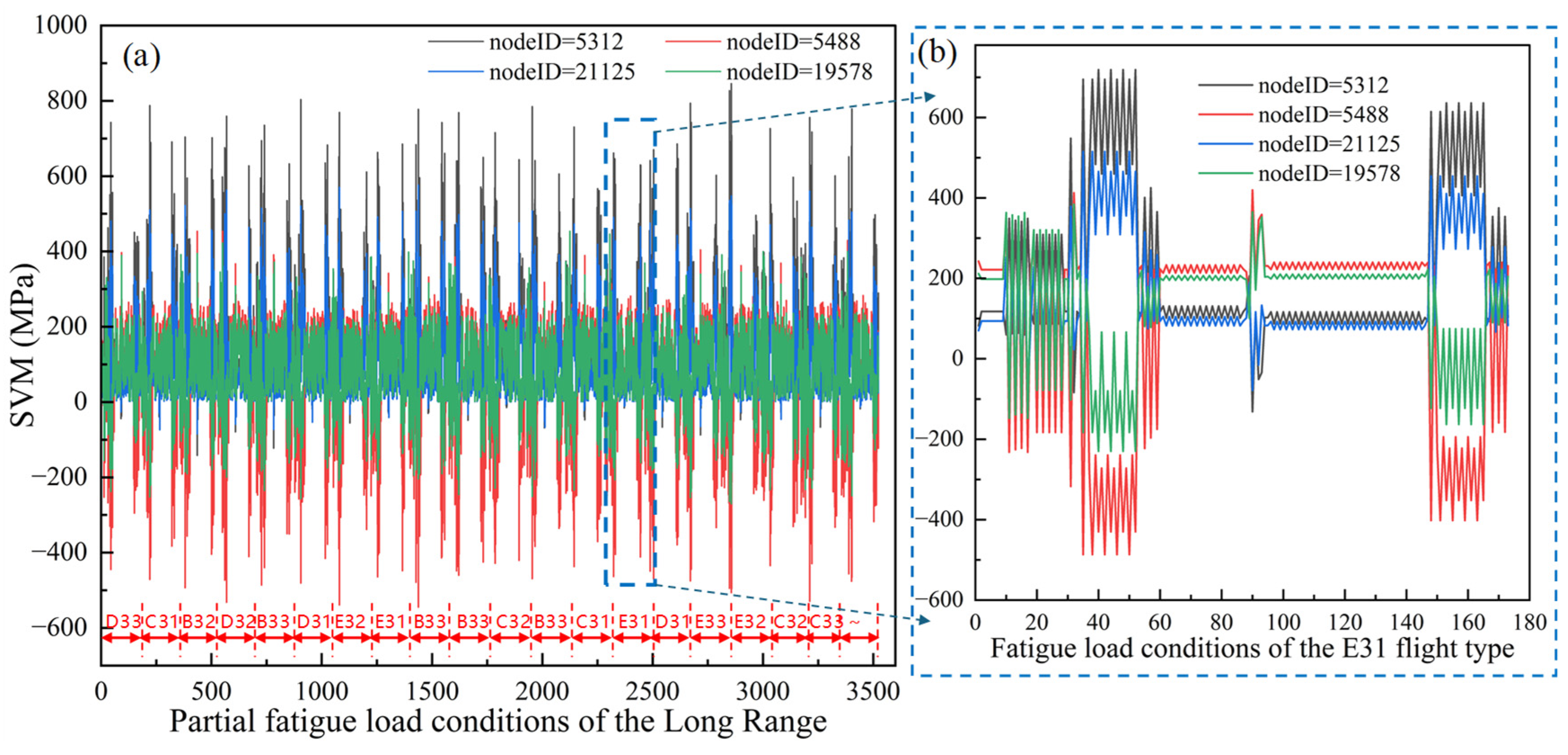
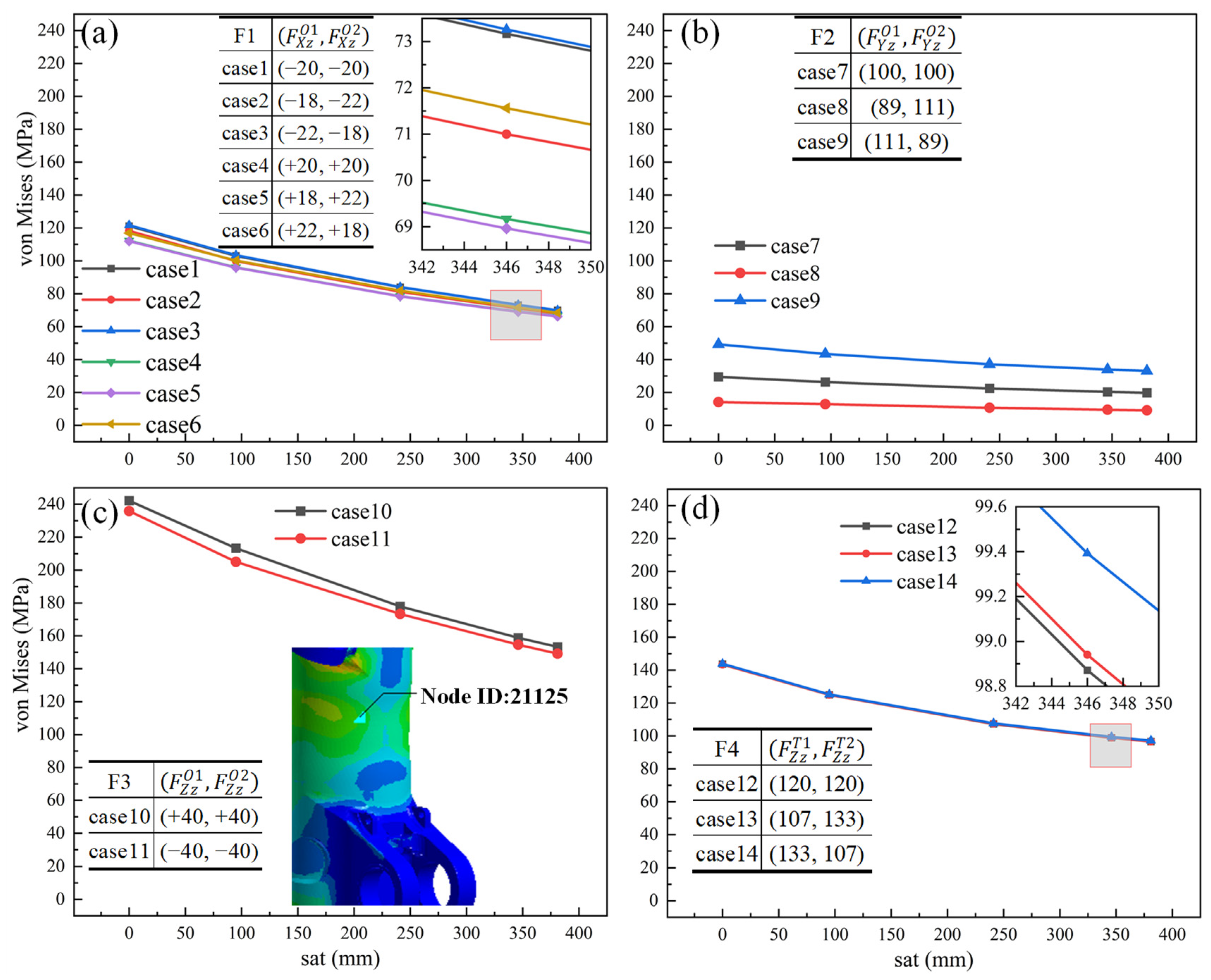
5.2. Effect of SAT on the Node Stress
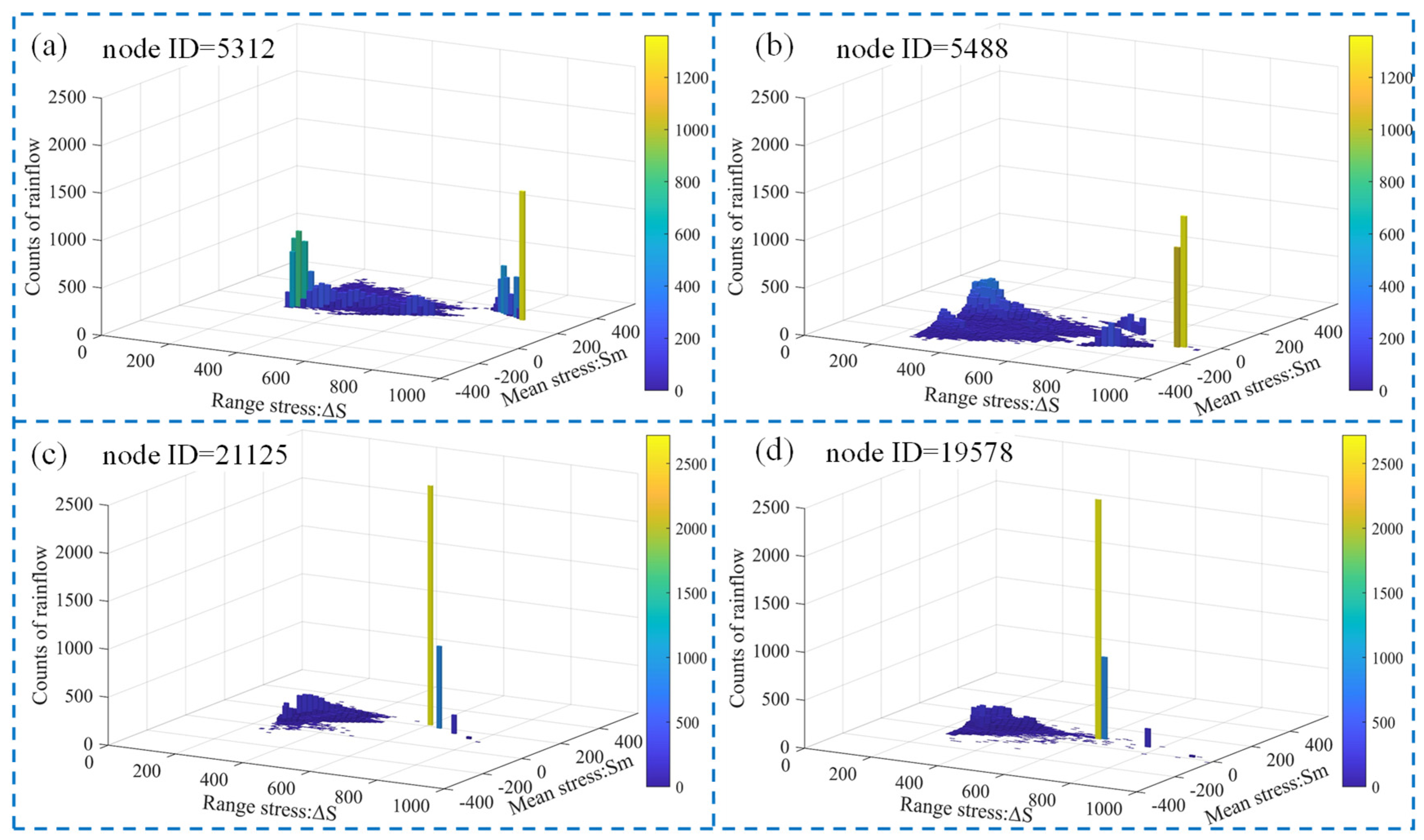
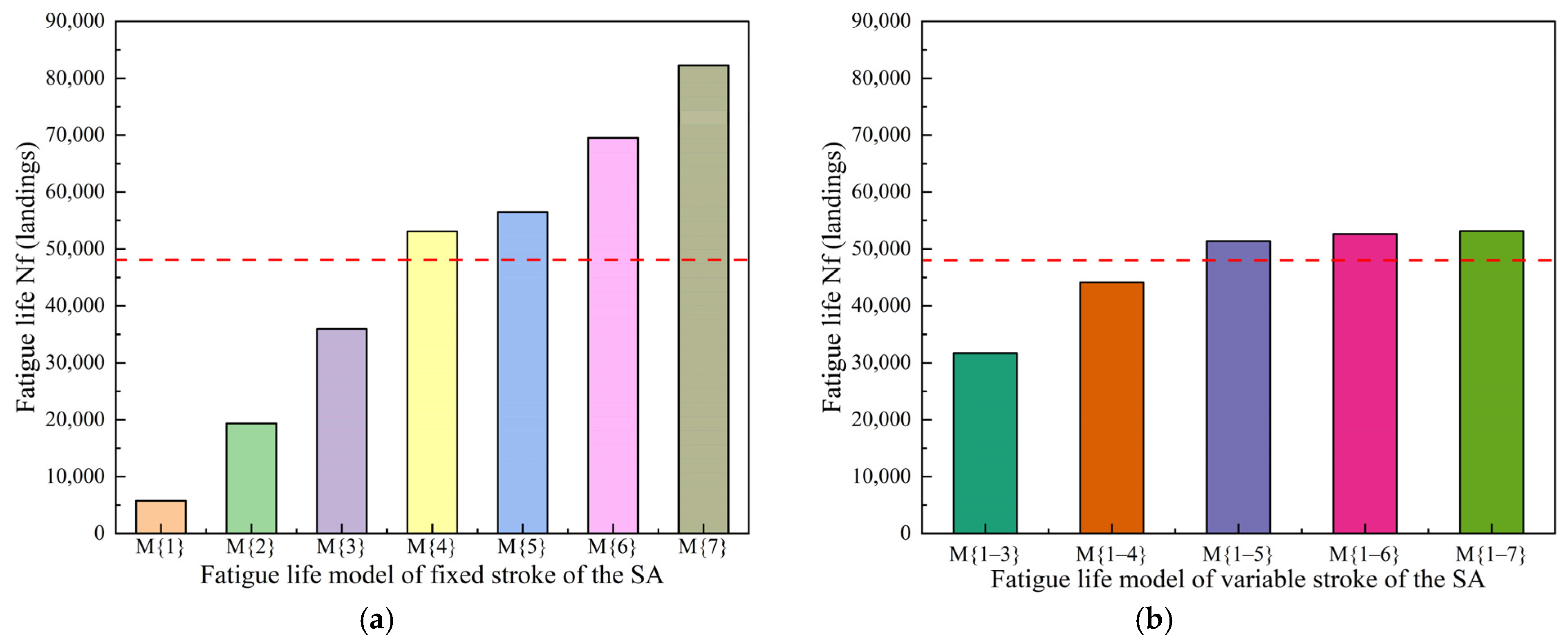
5.3. Effect of SAT on Fatigue Life Evaluation
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Kang, B.-H.; Choi, S.-B. Design, Structure Analysis and Shock Control of Aircraft Landing Gear with MR Damper. Smart Mater. Struct. 2024, 33, 055049. [Google Scholar] [CrossRef]
- Li, W.; Chen, S.; Chu, Y.; Huang, P.; Yan, G. Wide-Range Fiber Bragg Grating Strain Sensor for Load Testing of Aircraft Landing Gears. Optik 2022, 262, 169290. [Google Scholar] [CrossRef]
- Wang, Y.; Zhang, X.; Dong, X.; Yao, W. Multiaxial Fatigue Assessment for Outer Cylinder of Landing Gear by Critical Plane Method. Proc. Inst. Mech. Eng. G J. Aerosp. Eng. 2022, 236, 993–1005. [Google Scholar] [CrossRef]
- Raouf, I.; Kumar, P.; Cheon, Y.; Tanveer, M.; Jo, S.-H.; Kim, H.S. Advances in Prognostics and Health Management for Aircraft Landing-Progress, Challenges, and Future Possibilities. Int. J. Precis. Eng. Manuf. Green Technol. 2024, 12, 301–320. [Google Scholar] [CrossRef]
- Yan, S.; Xue, P.; Liu, L.; Zahran, M.S. Optimization of Landing Gear under Consideration of Vibration Comfort for Civil Aircraft. Aircr. Eng. Aerosp. Technol. 2024, 96, 378–386. [Google Scholar] [CrossRef]
- Fu, Y.; Fu, H.; Zhang, S. A Novel Safe Life Extension Method for Aircraft Main Landing Gear Based on Statistical Inference of Test Life Data and Outfield Life Data. Symmetry 2023, 15, 880. [Google Scholar] [CrossRef]
- Chiariello, A.; Carandente Tartaglia, C.; Arena, M.; Quaranta, V.; Bruno, G.; Belardo, M.; Castaldo, M. Vibration Qualification Campaign on Main Landing Gear System for High-Speed Compound Helicopter. Aerospace 2024, 11, 130. [Google Scholar] [CrossRef]
- Jiang, Y.; Feng, G.; Tang, H.; Zhang, J.; Jiang, B. Effect of Coulomb Friction on Shimmy of Nose Landing Gear under Time-Varying Load. Tribol. Int. 2023, 188, 108828. [Google Scholar] [CrossRef]
- Wang, X.H.; Luo, H.W. Research and Application Progress in Ultra-High Strength Stainless Steel for Aircraft Landing Gear. Cailiao Gongcheng/J. Mater. Eng. 2019, 47, 9. [Google Scholar] [CrossRef]
- Liu, P.; Zhang, J.; Tang, H.; Duan, H.; Jiang, B. Residual Fatigue Life Prediction Based on a Novel Improved-Halford Model Considering Loading Interaction Effect. Heliyon 2024, 10, e36716. [Google Scholar] [CrossRef]
- Skorupka, Z. Dynamic Fatigue Tests of Landing Gears. Fatigue Aircr. Struct. 2020, 2020, 69–77. [Google Scholar] [CrossRef]
- Bag, A.; Delbergue, D.; Ajaja, J.; Bocher, P.; Lévesque, M.; Brochu, M. Effect of Different Shot Peening Conditions on the Fatigue Life of 300 M Steel Submitted to High Stress Amplitudes. Int. J. Fatigue 2020, 130, 105274. [Google Scholar] [CrossRef]
- Wu, B.; Shi, Y.; Yin, Y. Optimization Design of Landing Gear Structure Based on Fatigue Life Constraint. In Proceedings of the Journal of Physics: Conference Series; IOP Publishing: Bristol, UK, 2022; Volume 2403. [Google Scholar]
- Schmidt, R.K. The Design of Aircraft Landing Gear; SAE International: Warrendale, PA, USA, 2021. [Google Scholar]
- El Mir, H.; Perinpanayagam, S. Certification of Machine Learning Algorithms for Safe-Life Assessment of Landing Gear. Front. Astron. Space Sci. 2022, 9, 896877. [Google Scholar] [CrossRef]
- EASA. Certification Specifications and Acceptable Means of Compliance for Large Aeroplanes CS-25. 2018. Available online: https://www.easa.europa.eu/en/document-library/certification-specifications/cs-25-amendment-14 (accessed on 12 December 2024).
- Giannella, V.; Baglivo, G.; Giordano, R.; Sepe, R.; Citarella, R. Structural FEM Analyses of a Landing Gear Testing Machine. Metals 2022, 12, 937. [Google Scholar] [CrossRef]
- Dziendzikowski, M.; Kurnyta, A.; Reymer, P.; Kurdelski, M.; Klysz, S.; Leski, A.; Dragan, K. Application of Operational Load Monitoring System for Fatigue Estimation of Main Landing Gear Attachment Frame of an Aircraft. Materials 2021, 14, 6564. [Google Scholar] [CrossRef]
- Fang, F.; Qiu, L.; Yuan, S.; Ren, Y. Dynamic Probability Modeling-Based Aircraft Structural Health Monitoring Framework under Time-Varying Conditions: Validation in an in-Flight Test Simulated on Ground. Aerosp. Sci. Technol. 2019, 95, 105467. [Google Scholar] [CrossRef]
- Qiu, L.; Yuan, S.; Boller, C. An Adaptive Guided Wave-Gaussian Mixture Model for Damage Monitoring under Time-Varying Conditions: Validation in a Full-Scale Aircraft Fatigue Test. Struct. Health Monit. 2017, 16, 501–517. [Google Scholar] [CrossRef]
- Dai, X.; Liu, L.; Xu, Q.; Xu, F.; Hu, X. Research on the Control Technology of Aircraft Landing Gear Buffer Variable Stroke. J. Phys. Conf. Ser. 2024, 2820, 12010. [Google Scholar] [CrossRef]
- Chen, C.; Hu, B.; Chen, X.; Chai, Y.; Zhang, C. Variable Stroke Fatigue Test for Aircraft Landing Gears. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2024. [Google Scholar] [CrossRef]
- Zhu, Z.H.; Larosa, M.; Ma, J. Fatigue Life Estimation of Helicopter Landing Probe Based on Dynamic Simulation. J. Aircr. 2009, 46, 1533–1543. [Google Scholar] [CrossRef]
- Infante, V.; Fernandes, L.; Freitas, M.; Baptista, R. Failure Analysis of a Nose Landing Gear Fork. Eng. Fail. Anal. 2017, 82, 554–565. [Google Scholar] [CrossRef]
- Freitas, M.; Infante, V.; Baptista, R. Failure Analysis of the Nose Landing Gear Axle of an Aircraft. Eng. Fail. Anal. 2019, 101, 113–120. [Google Scholar] [CrossRef]
- Raković, D.; Simonović, A.; Grbović, A.; Radović, L.; Vorkapić, M.; Krstić, B. Fatigue Fracture Analysis of Helicopter Landing Gear Cross Tube. Eng. Fail. Anal. 2021, 129, 105672. [Google Scholar] [CrossRef]
- Raičević, N.; Grbović, A.; Kastratović, G.; Vidanović, N.; Sedmak, A. Fatigue Life Prediction of Topologically Optimized Torque Link Adjusted for Additive Manufacturing. Int. J. Fatigue 2023, 176, 107907. [Google Scholar] [CrossRef]
- Xie, H.; Yang, G.; Sun, J.; Sai, W.; Zeng, W. Anti-Fatigue Optimization of the Twisting Force Arm of Landing Gear Based on Kriging Approximate Sequential Optimization Method. J. Chin. Inst. Eng. Trans. Chin. Inst. Eng. Ser. A 2024, 47, 2274086. [Google Scholar] [CrossRef]
- Xue, C.J.; Dai, J.H.; Wei, T.; Liu, B.; Deng, Y.Q.; Ma, J. Structural Optimization of a Nose Landing Gear Considering Its Fatigue Life. J. Aircr. 2012, 49, 225–236. [Google Scholar] [CrossRef]
- Shao, H.; Li, D.; Kan, Z.; Zhao, S.; Xiang, J.; Wang, C. Analysis of Catapult-Assisted Takeoff of Carrier-Based Aircraft Based on Finite Element Method and Multibody Dynamics Coupling Method. Aerospace 2023, 10, 1005. [Google Scholar] [CrossRef]
- Candon, M.; Esposito, M.; Fayek, H.; Levinski, O.; Koschel, S.; Joseph, N.; Carrese, R.; Marzocca, P. Advanced Multi-Input System Identification for next Generation Aircraft Loads Monitoring Using Linear Regression, Neural Networks and Deep Learning. Mech. Syst. Signal Process. 2022, 171, 108809. [Google Scholar] [CrossRef]
- Suresh, P.S.; Sura, N.K.; Shankar, K.; Radhakrishnan, G. Synthesis of Landing Dynamics on Land-Base High Performance Aircraft Considering Multi-Variate Landing Conditions. Mech. Based Des. Struct. Mach. 2023, 51, 1946406. [Google Scholar] [CrossRef]
- Di Lorenzo, D.; Rodriguez, S.; Champaney, V.; Germoso, C.; Beringhier, M.; Chinesta, F. Damage Identification Technique by Model Enrichment for Structural Problems. Results Eng. 2024, 23, 102389. [Google Scholar] [CrossRef]
- Marin, M.; Öchsner, A.; Craciun, E.M. A Generalization of the Saint-Venant’s Principle for an Elastic Body with Dipolar Structure. Contin. Mech. Thermodyn. 2020, 32, 269–278. [Google Scholar] [CrossRef]
- Dong, G.; Zhang, M.; Wei, L.; Zhang, Y. Study on High Cycle Fatigue Criteria under Multi-Axial Random Loads of Chassis Parts. Zhongguo Jixie Gongcheng/China Mech. Eng. 2021, 32, 2294. [Google Scholar] [CrossRef]
- Zhang, S.; Le, C.; Gain, A.L.; Norato, J.A. Fatigue-Based Topology Optimization with Non-Proportional Loads. Comput. Methods Appl. Mech. Eng. 2019, 345, 805–825. [Google Scholar] [CrossRef]
- Mi, C.; Gu, Z.; Yang, Q.; Nie, D. Frame Fatigue Life Assessment of a Mining Dump Truck Based on Finite Element Method and Multibody Dynamic Analysis. Eng. Fail. Anal. 2012, 23, 18–26. [Google Scholar] [CrossRef]
- Liu, K.G.; Yan, C.L.; Zhang, S.M. Estimation of Fatigue Damage of Airplane Landing Gear. Front. Mech. Eng. China 2006, 1, 424–428. [Google Scholar] [CrossRef]
- ASTM E1049-85; Standard Practices for Cycle Counting in Fatigue Analysis. ASTM International: West Conshohocken, PA, USA, 2011.
- Zhang, Q.L.; Hu, L.; Gao, X.F. Fatigue Life Prediction of Steel Spiral Cases in Pumped-Storage Power Plants: Factors to Be Considered. Eng. Fail. Anal. 2024, 157, 107908. [Google Scholar] [CrossRef]
- Lian, Y.; Zhao, R.; Yu, K.; Zhu, Y. P-S-N Surfaces of Lifting Lug Structure Based on Extremely Small Samples. Eng. Fail. Anal. 2024, 162, 108457. [Google Scholar] [CrossRef]
- Liu, Y.; Paggi, M.; Gong, B.; Deng, C. A Unified Mean Stress Correction Model for Fatigue Thresholds Prediction of Metals. Eng. Fract. Mech. 2020, 223, 106787. [Google Scholar] [CrossRef]
- Hoole, J.; Sartor, P.; Booker, J.; Cooper, J.; Gogouvitis, X.V.; Schmidt, R.K. Systematic Statistical Characterisation of Stress-Life Datasets Using 3-Parameter Distributions. Int. J. Fatigue 2019, 129, 105216. [Google Scholar] [CrossRef]
- Tan, X.; Li, Q.; Wang, G.; Xie, K. Three-Parameter P-S-N Curve Fitting Based on Improved Maximum Likelihood Estimation Method. Processes 2023, 11, 634. [Google Scholar] [CrossRef]
- Rice, R.; Jackson, J.; Bakuckas, J.; Thompson, S. Metallic Materials Properties Development and Standardization (MMPDS); 2003. Available online: https://ntrl.ntis.gov/NTRL/dashboard/searchResults/titleDetail/PB2003106632.xhtml (accessed on 12 December 2024).

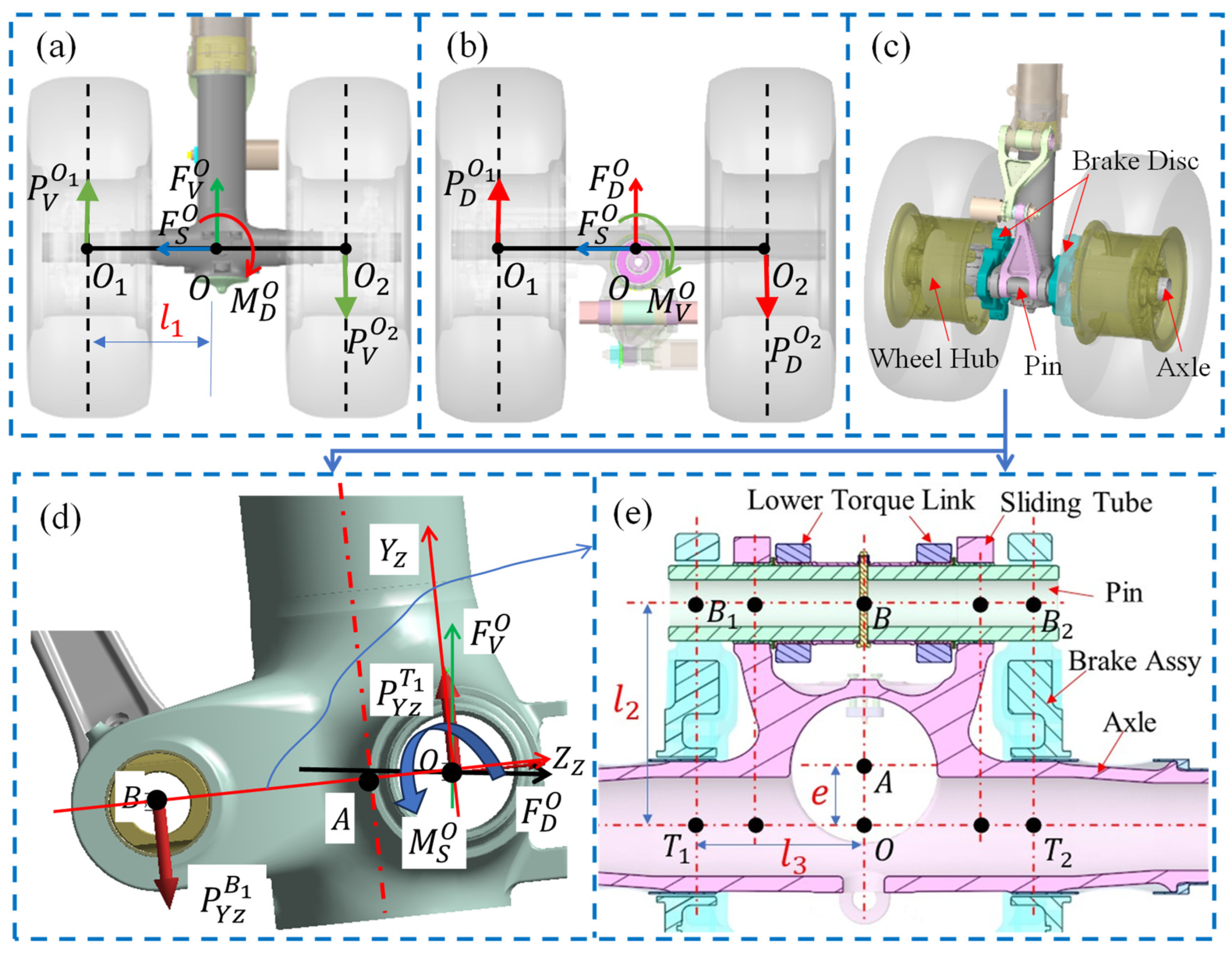



| FLCs No. | /kN | /kN | /kN | /kN·m | /kN·m | /kN·m | Sat /mm | /degree | k1/k2 |
|---|---|---|---|---|---|---|---|---|---|
| 33080302 | 0 | 226.3 | 0 | 0 | 0 | 0 | 320 | 0.18 | 0.5/0.5 |
| 32050501 | 15.9 | 265.2 | 48.3 | −24.6 | 0 | 8.1 | 344 | −0.37 | 0.5/0.5 |
| 32050302 | 21.5 | 358.9 | −63.2 | 30.9 | 0 | 10.5 | 381 | −0.41 | 0.5/0.5 |
| 32060223 | 94.7 | 315.8 | 0 | 14.6 | −4.4 | 47.2 | 366 | −0.32 | 0.45/0.55 |
| 32260431 | 0 | 271.2 | 0 | −12.6 | 0 | 0 | 347 | −0.35 | 0.55/0.45 |
| 32260432 | 67.8 | 271.2 | 0 | −12.6 | 3.1 | 34.5 | 347 | −0.36 | 0.5/0.5 |
| …… | …… | …… | …… | …… | …… | …… | …… | …… | …… |
| Parameter | Description | Value | Unit |
|---|---|---|---|
| Shock absorber travel | - | mm | |
| Aircraft pitch angle | - | degree | |
| MLG inclination angle | 5.6 | degree | |
| MLG stable spacing | 58 | mm | |
| Half of the distance between Wheels | 466 | mm | |
| Distance between Axle and the Pin | 245 | mm | |
| Half of the distance between brake discs | 186 | mm | |
| The distance of QA | 1250 | mm | |
| Initial distance of PA | 715 | mm | |
| Initial gas pressure | 2.92 | MPa | |
| Atmospheric pressure | 0.101 | MPa | |
| Initial gas volume | 11,216,000 | mm3 | |
| Air variability index | 1.1 | - | |
| Pressure area | 23,235 | mm2 |
| Force Type | F1 | F2 | F3 | F4 | F5 | ||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| FLCs No. | |||||||||||
| 33080302 | 10.7 | 10.7 | 112.6 | 112.6 | 0 | 0 | 0 | 0 | 0 | 0 | 248 |
| 32050501 | 19 | 24.4 | 104.8 | 157.3 | 24.2 | 24.2 | 16.5 | 16.5 | −16.5 | −16.5 | 248 |
| 32050302 | 33 | 26 | 210.3 | 144.4 | −31.6 | −31.6 | 21.6 | 21.6 | −21.6 | −21.6 | 248 |
| 32060223 | 54 | 72.8 | 153 | 151.3 | 0 | 0 | 86.7 | 106 | −86.7 | −106 | 248 |
| 32260431 | 14.1 | 14.1 | 134.9 | 134.8 | 0 | 0 | 0 | 0 | 0 | 0 | 248 |
| 32260432 | 49.7 | 45.9 | 117.6 | 145.1 | 0 | 0 | 70.4 | 70.4 | −70.4 | −70.4 | 248 |
| …… | …… | …… | …… | …… | …… | …… | …… | …… | …… | …… | …… |
| Force Location | Unit Load Values | Location of Force Application | |
|---|---|---|---|
| (+40,+40); (−40,−40); (+10,−10); (−10,+10) | Heading force of O1 and O2, other forces set to 0 | ||
| (+100,+100); (+20,−20); (−20,+20) | Vertical force of O1 and O2, other forces set to 0 | ||
| (+40,+40); (−40,−40) | Side force of O1 and O2, other forces set to 0 | ||
| (+100,+100); (100,122); (122,100) | Vertical force of T1, T2, and B1, B2 obtained from formula(6) | ||
| +248; −334 | Point M1, other forces set to 0 |
| Node ID | /Landings |
|---|---|
| 5312 | 53,164 |
| 5488 | 60,272 |
| 21125 | 78,481 |
| 19578 | 83,775 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tang, H.; Liu, P.; Ding, J.; Cheng, J.; Jiang, Y.; Jiang, B. Numerical Prediction of Fatigue Life for Landing Gear Considering the Shock Absorber Travel. Aerospace 2025, 12, 42. https://doi.org/10.3390/aerospace12010042
Tang H, Liu P, Ding J, Cheng J, Jiang Y, Jiang B. Numerical Prediction of Fatigue Life for Landing Gear Considering the Shock Absorber Travel. Aerospace. 2025; 12(1):42. https://doi.org/10.3390/aerospace12010042
Chicago/Turabian StyleTang, Haihong, Panglun Liu, Jianbin Ding, Jinsong Cheng, Yiyao Jiang, and Bingyan Jiang. 2025. "Numerical Prediction of Fatigue Life for Landing Gear Considering the Shock Absorber Travel" Aerospace 12, no. 1: 42. https://doi.org/10.3390/aerospace12010042
APA StyleTang, H., Liu, P., Ding, J., Cheng, J., Jiang, Y., & Jiang, B. (2025). Numerical Prediction of Fatigue Life for Landing Gear Considering the Shock Absorber Travel. Aerospace, 12(1), 42. https://doi.org/10.3390/aerospace12010042





