Chatter and Surface Waviness Analysis in Oerlikon Face Hobbing of Spiral Bevel Gears
Abstract
1. Introduction
2. The Cutting Dynamics in the Spiral Bevel Gear End-Face Hobbing Process
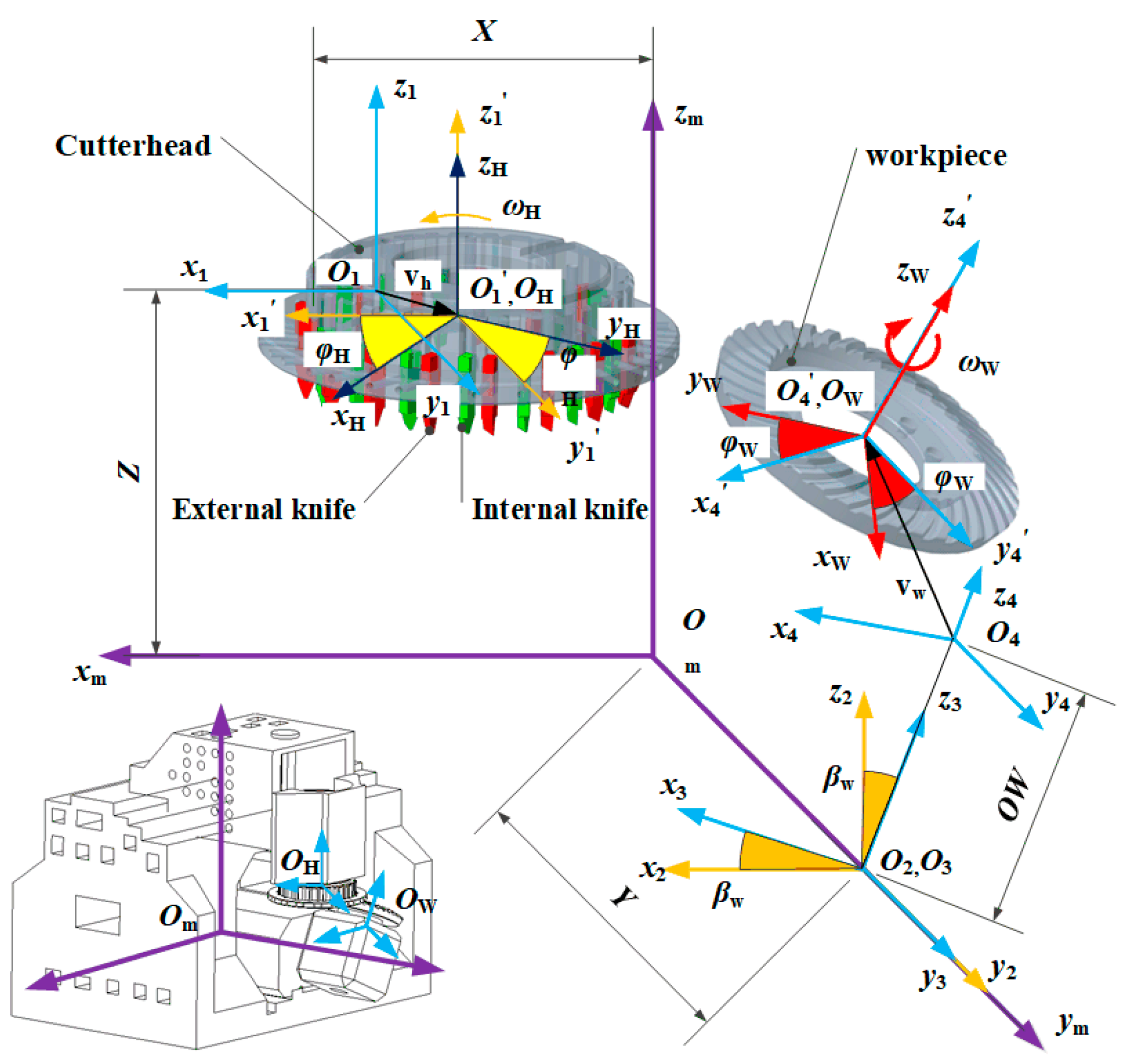
2.1. Kinematics of Machine Tool and Machined Tooth Surface of Gearwheel
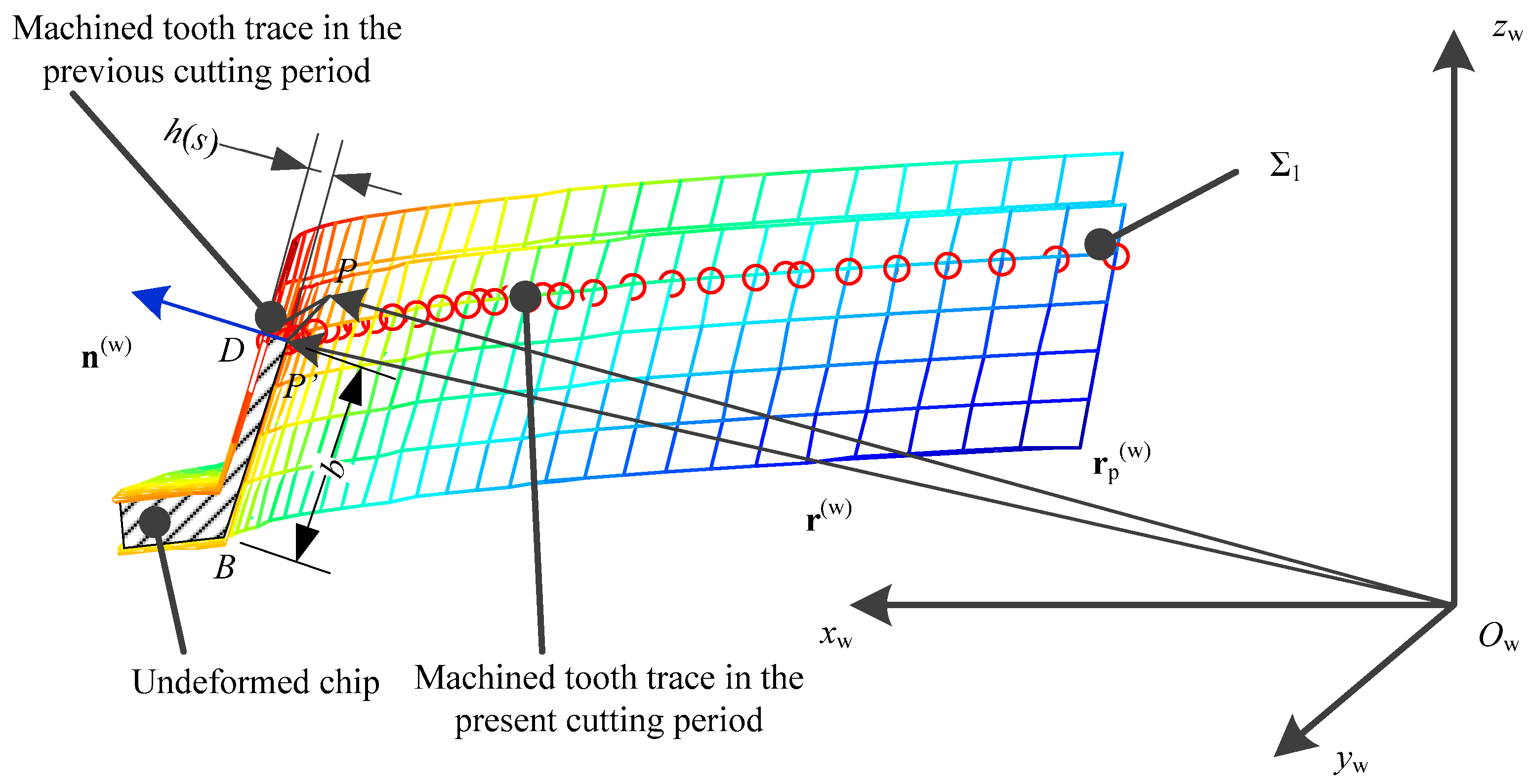
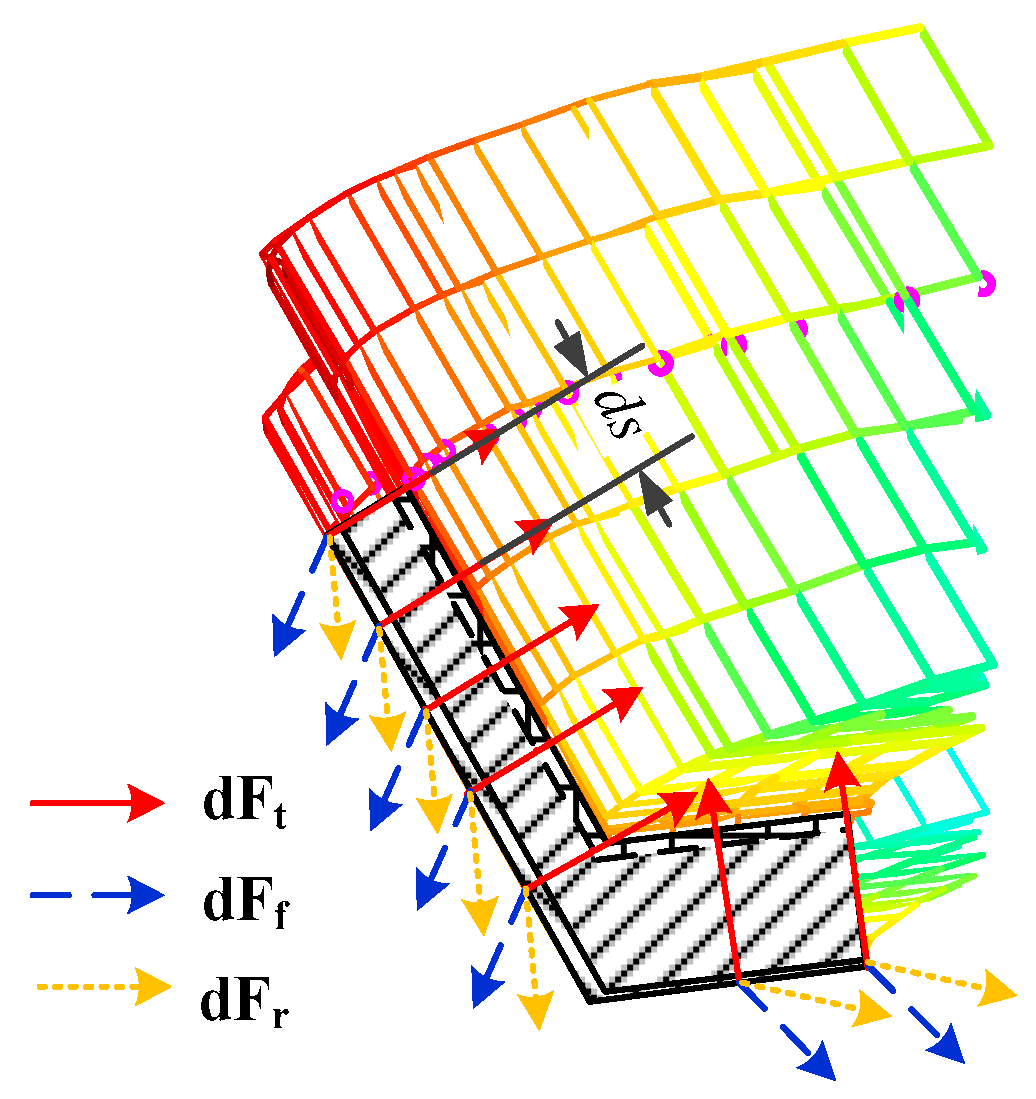
2.2. Undeformed Chip Geometry and End Cutting Forces
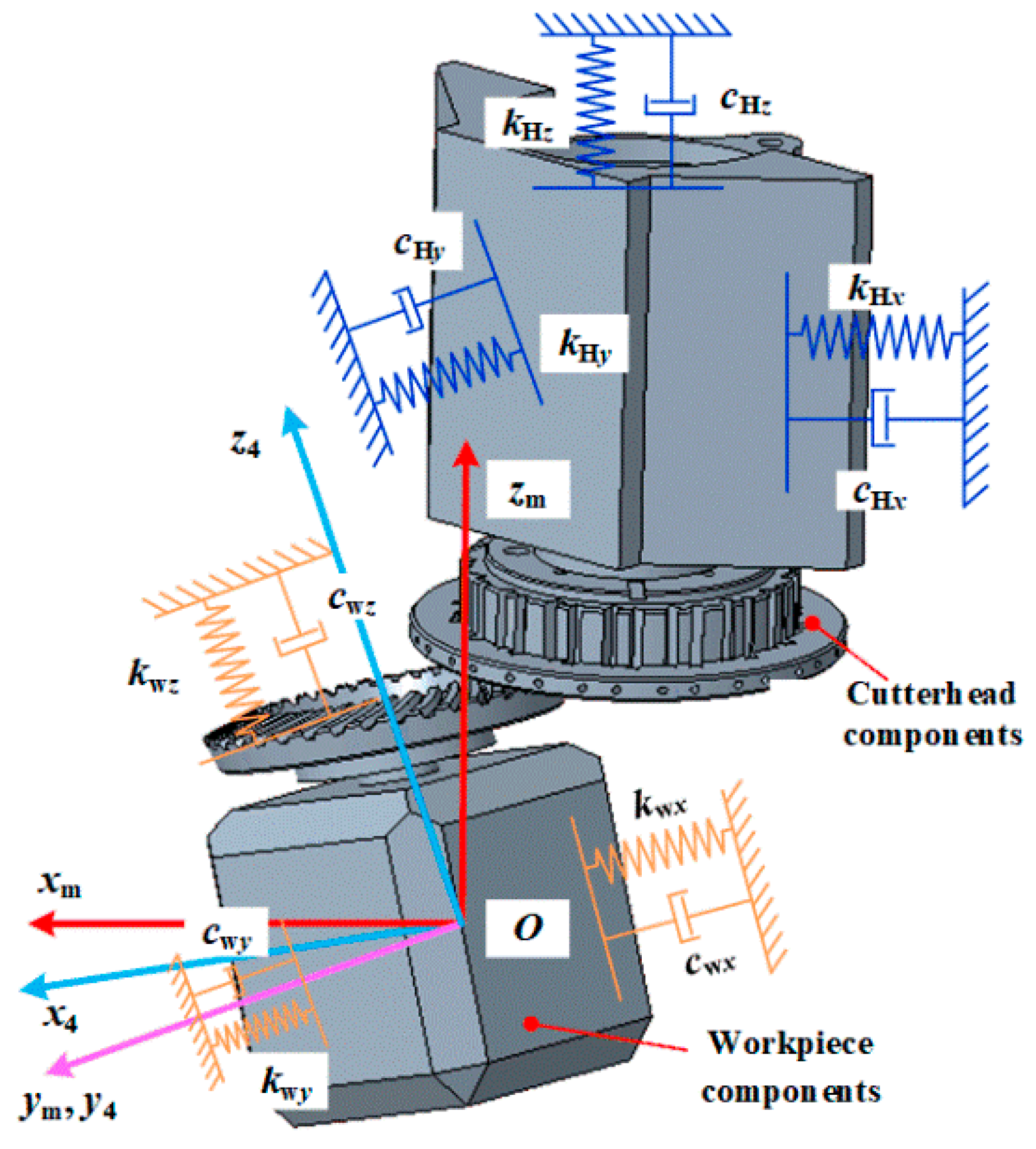
2.3. Cutting Dynamics Analytical Model of Face Hobbing Process
3. Experimental Tests and Verification
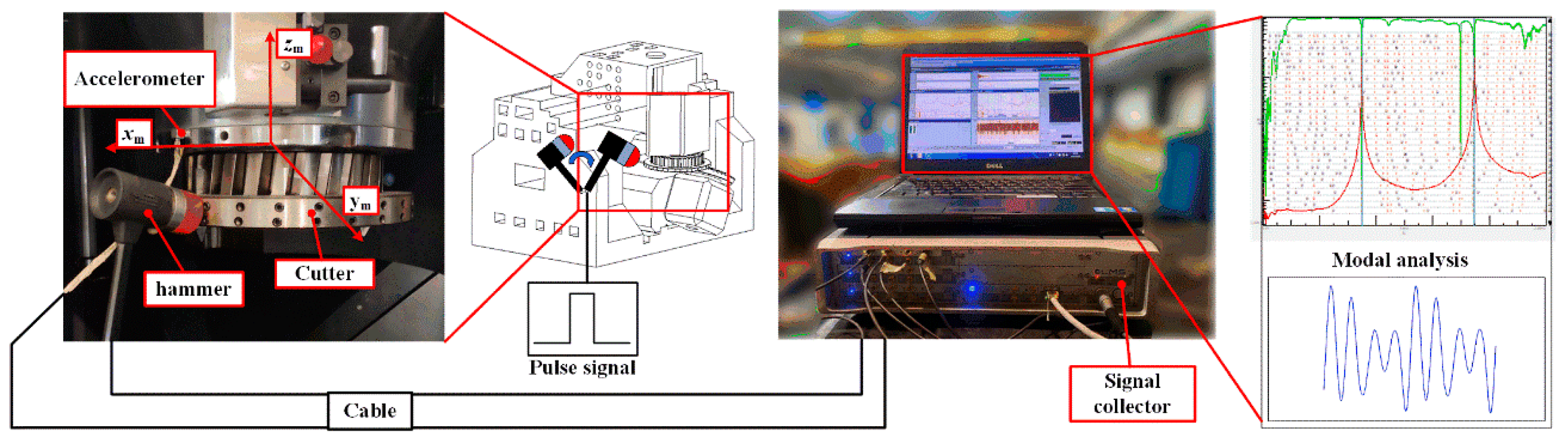
3.1. Hammer Impact Testing
3.2. Experiment on Face-Hobbing of Spiral Bevel Gear
4. Results and Discussion
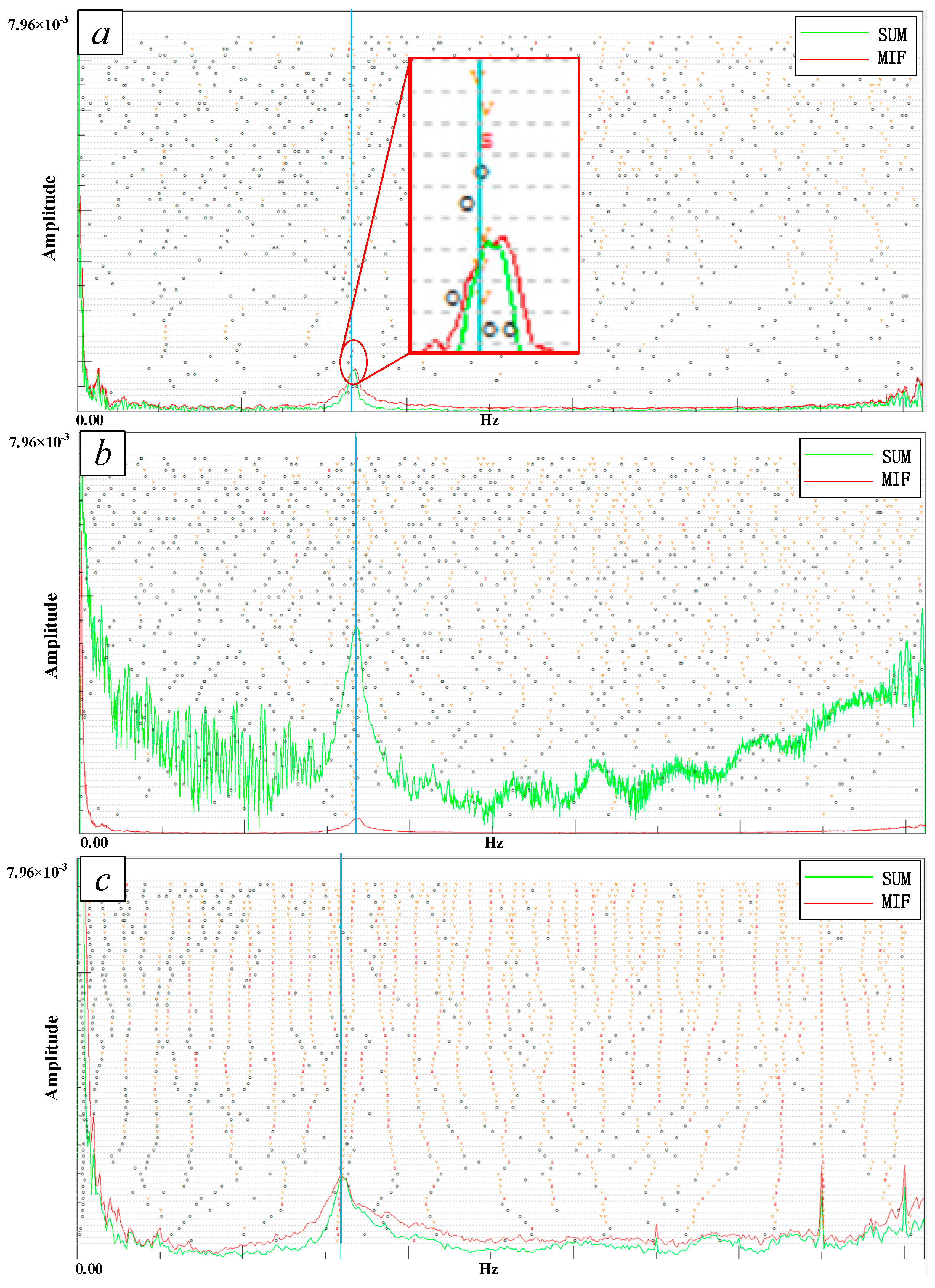
4.1. Experimental Modal Analysis
4.2. Analysis in Time Domain
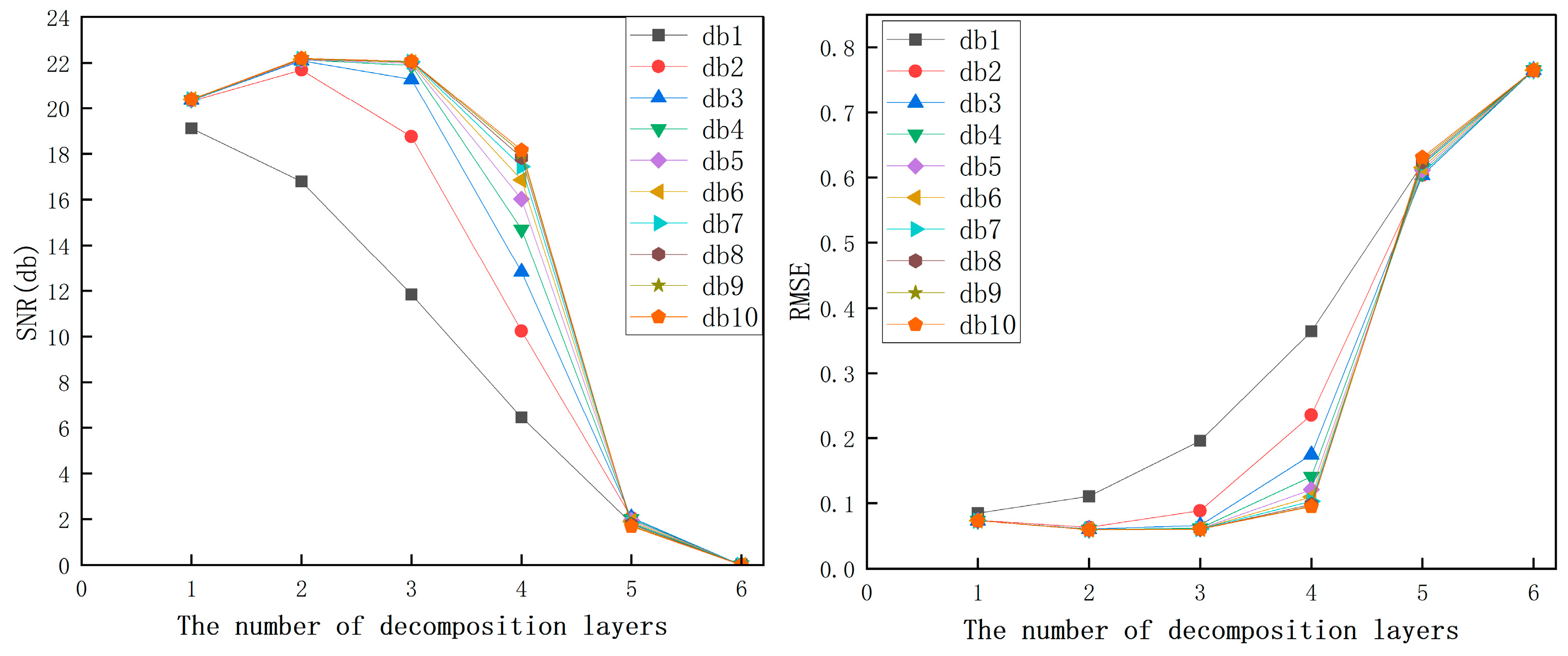
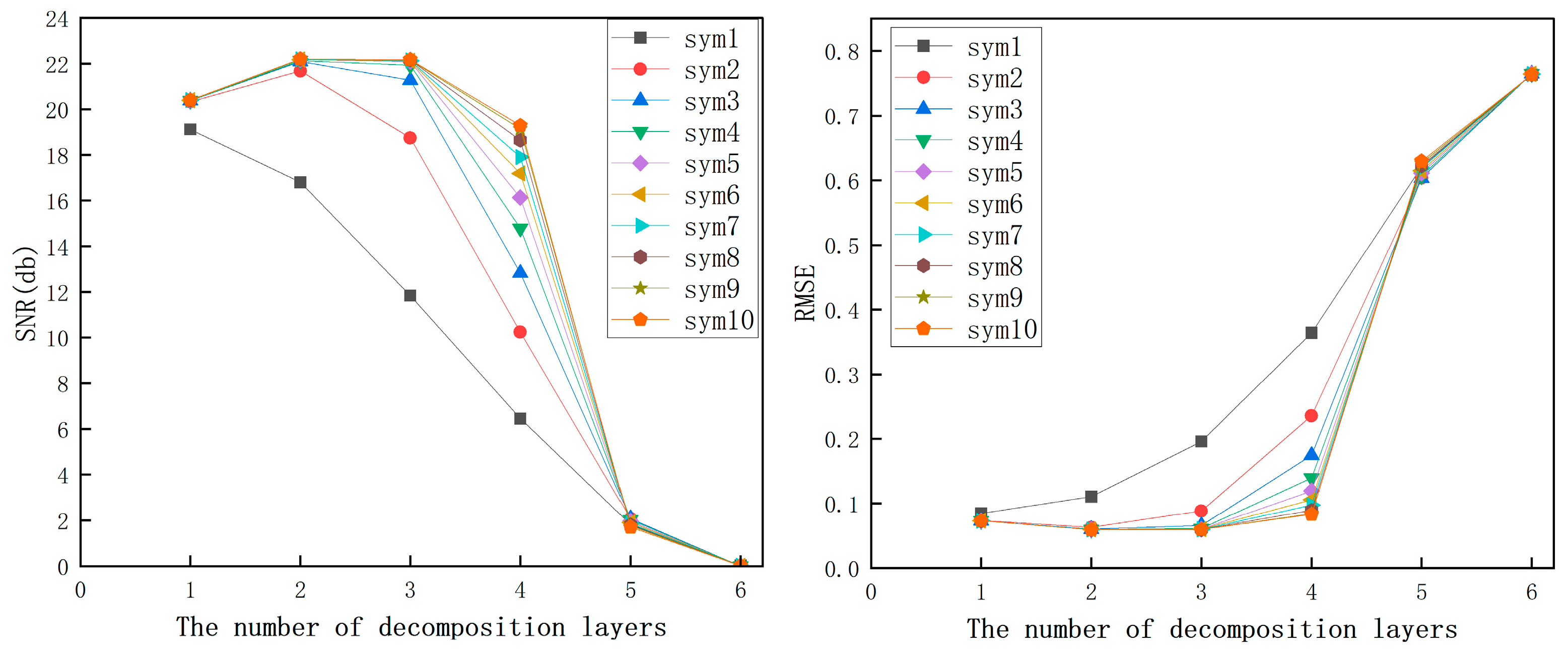
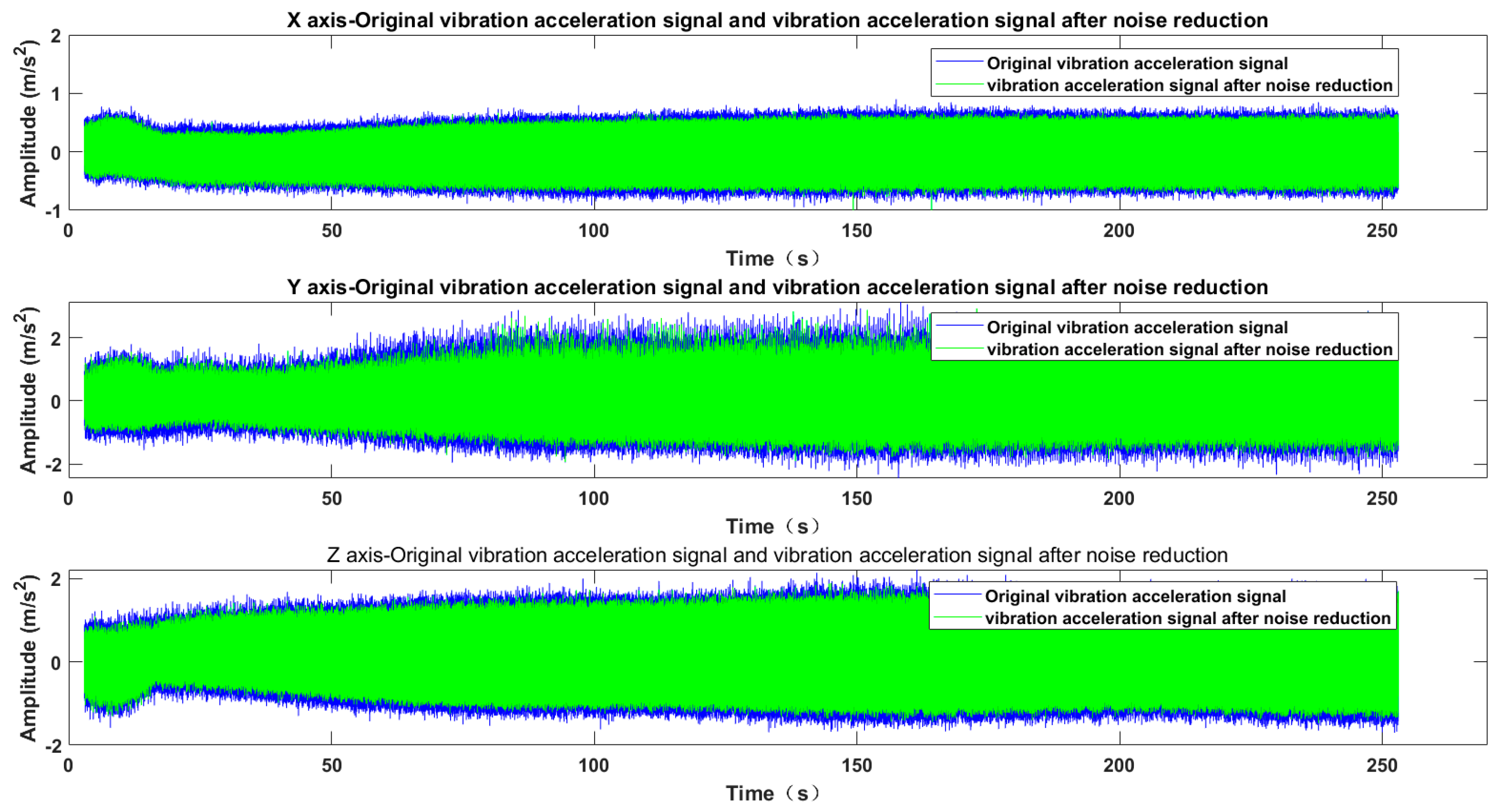
4.2.1. Wavelet Denoising
4.2.2. Feature Extraction in Time Domain
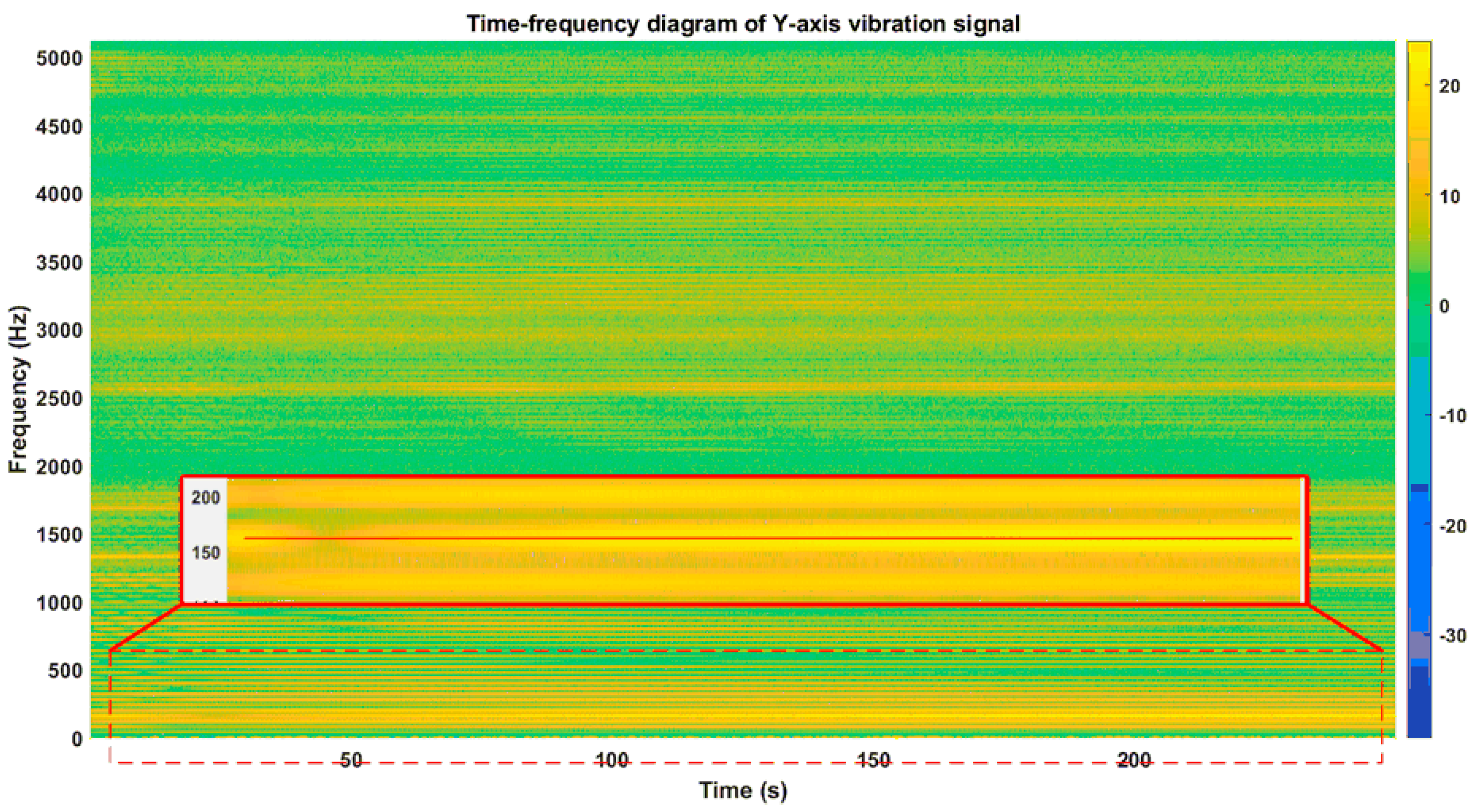
4.3. Frequency Domain Analysis
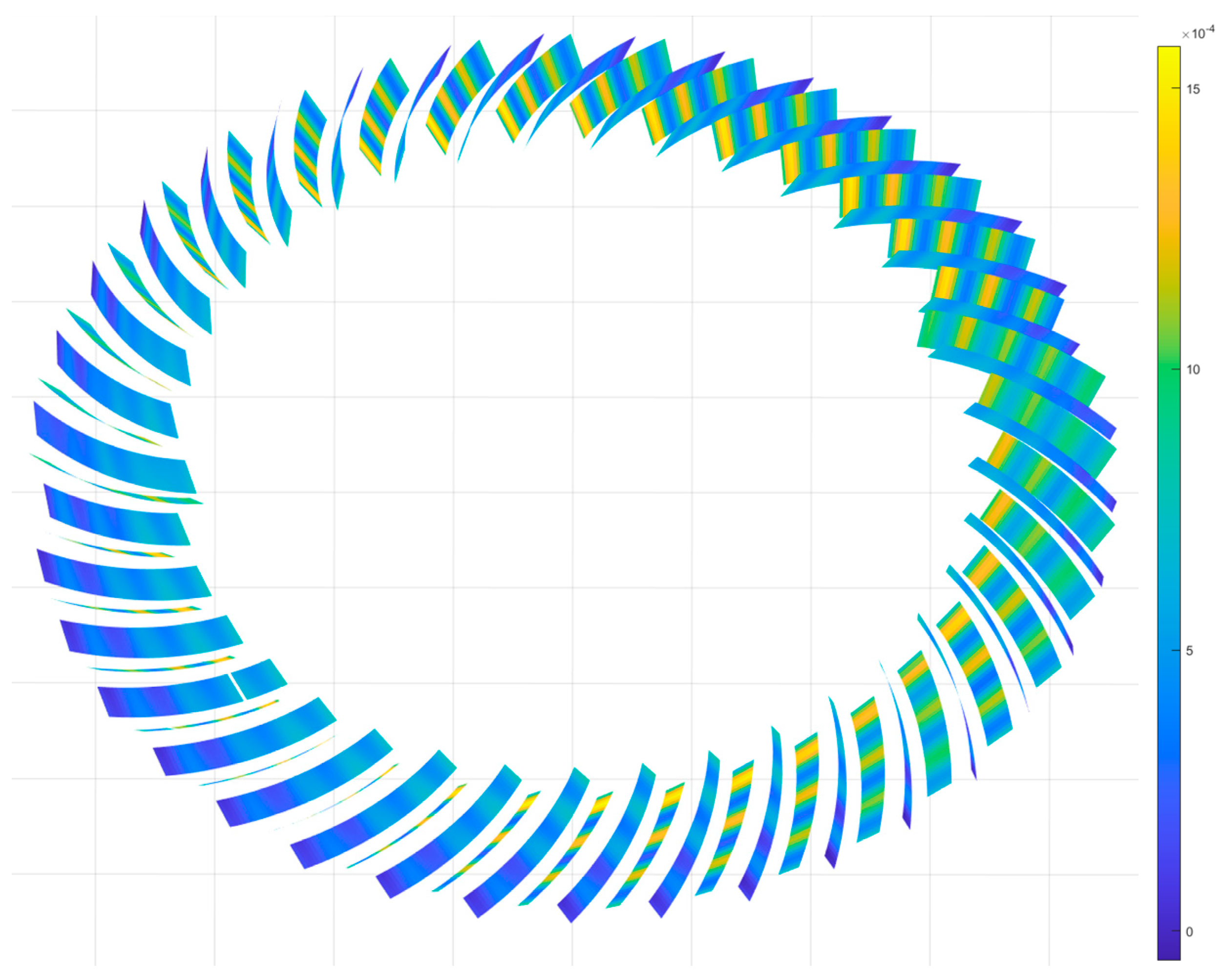
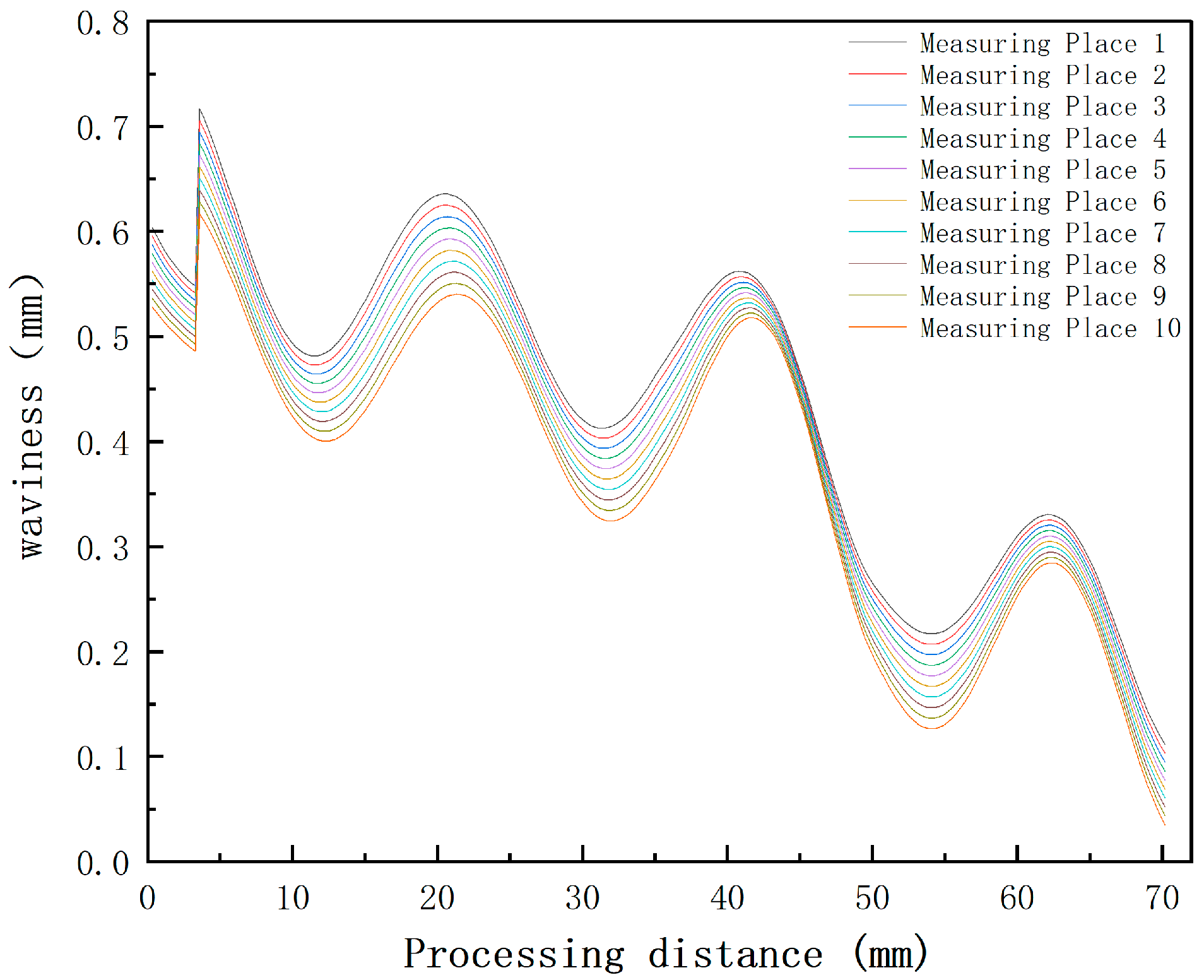
4.4. The Impact of Tool Vibration on the Machining Quality of Spiral Bevel Gear Tooth Surface
5. Conclusions
- (1)
- By comparing the denoising effects of different combinations of wavelet basis functions, decomposition levels, threshold selection rules, and threshold functions, and selecting based on SNR and RMSE, the best denoising effect can be obtained by the combination of using the sym9 wavelet with 2 decomposition levels, applying the Rigrsure rule, and employing a soft thresholding function. This results in a SNR of 22.5046 db, and a RMSE of 0.057393.
- (2)
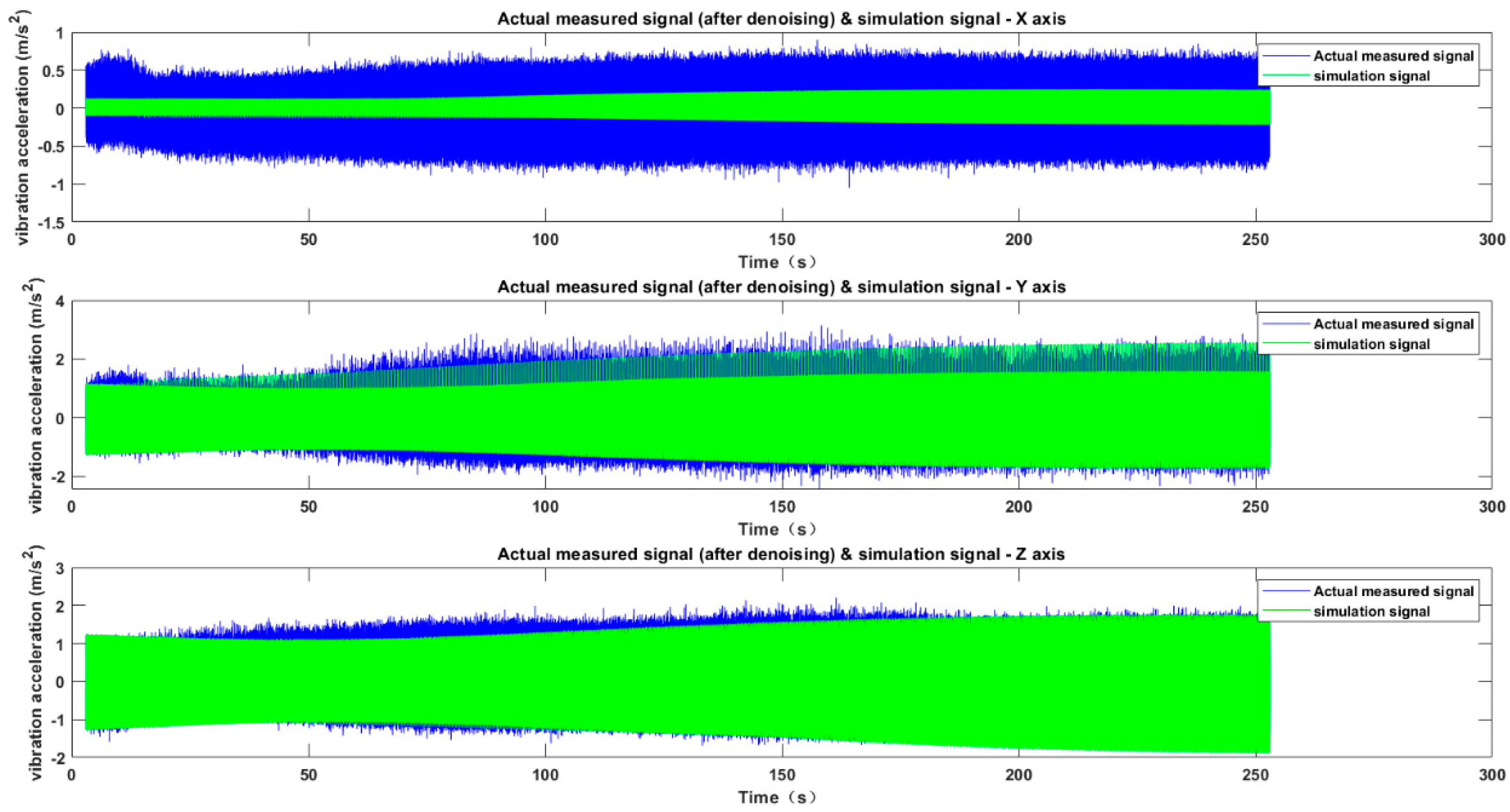
- Through time-domain analysis of both measured vibration signals and simulated signals, a high degree of conformity in the time-domain statistical indicators between the two signal sets was observed, providing evidence at the time-domain level for the accuracy of the cutting dynamics model. For the equipment YKA2260 used in this investigation, the main frequency of cutter head vibration was identified as 159.7799 Hz. The Y-axis vibration was found to be the main cause of machining chatter and the formation of tooth surface chatter marks. The accuracy of the cutting dynamics model at the frequency domain level is confirmed.
- (3)
- With respect to the influence of tool vibration on the machining quality of spiral bevel gears, the comparison between the predicted and actual machined gear surface morphology has validated the reliability of the cutting dynamics model for spiral bevel gear machining. This model can effectively predict the gear surface morphology of spiral bevel gears. The prediction and experimental analysis indicate that the number of tooth surface chatter marks is proportional to the ratio of tool chatter frequency to cutting base frequency. This finding provides promising significance for optimizing the face hobbing process for spiral bevel gears.
- (4)
- This study provides a theoretical foundation and guidance for the design of new non-traditional dynamic vibration absorbers suitable for spiral bevel gear machining machines, aiming to address vibration issues specific to different models of such machines. Additionally, this research is significant for optimizing the face hobbing process of spiral bevel gears. To prevent machining defects caused by vibrations, machining parameter design should be optimized based on the modal parameters of the process system, avoiding multiples of the cutting frequency.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Stadtfeld, H.J. Handbook of Bevel and Hypoid Gears: Calculation-Manufacturing-Optimization; Rochester Institute of Technology: Rochester, NY, USA, 1993. [Google Scholar]
- Stadtfeld, H.J.; Works, G. Gleason Bevel Gear Technology: Basics of Gear Engineering and Modern Manufacturing Methods for Angular Transmissions; Gleason Works: Rochester, NY, USA, 2014. [Google Scholar]
- Fan, Q. Computerized Modeling and Simulation of Spiral Bevel and Hypoid Gears Manufactured by Gleason Face Hobbing Process. In Proceedings of the ASME International Design Engineering Technical Conferences & Computers & Information in Engineering Conference, Philadelphia, PA, USA, 10–13 September 2006. [Google Scholar]
- Fan, Q. Kinematical simulation of face hobbing indexing and tooth surface generation of spiral bevel and hypoid gears. Gear Technol. 2006, 23, 30–38. [Google Scholar]
- Litvin, F.L.; Chaing, W.S.; Kuan, C.; Lundy, M.; Tsung, W.J. Generation and geometry of hypoid gear-member with face-hobbed teeth of uniform depth. Int. J. Mach. Tools Manuf. 1991, 31, 167–181. [Google Scholar] [CrossRef]
- Wu, L.Y.; Wei, H.Q.; Wang, X.C. Analysis on the forming principle and the differential structure of extended epicycloid bevel gear tooth surface. J. Xi’an Jiaotong Univ. 2001, 35, 43–46. [Google Scholar]
- Zhang, Y.; Wu, Z. Geometry of Tooth Profile and Fillet of Face-Hobbed Spiral Bevel Gears. In Proceedings of the ASME 2007 International Design Engineering Technical Conferences and Computers and Information in Engineering Conference, Las Vegas, NV, USA, 4–7 September 2007; pp. 181–192. [Google Scholar]
- Suzuki, A.; Tarutani, I.; Aoyama, T. Design of high-reduction hypoid gears meshing in plane of action. J. Adv. Mech. Des. Syst. Manuf. 2017, 11, JAMDSM0068. [Google Scholar] [CrossRef][Green Version]
- Wang, M.Y.; Li, L.; Sun, Y.H. Tooth flank machining and modelling of line contact spiral bevel gears with the conical finger-type milling cutter. Int. J. Adv. Manuf. Technol. 2023, 125, 3277–3291. [Google Scholar] [CrossRef]
- Habibi, M.; Chen, Z.C. A semi-analytical approach to un-deformed chip boundary theory and cutting force prediction in face-hobbing of bevel gears. Comput.-Aided Des. 2016, 73, 53–65. [Google Scholar] [CrossRef]
- Efstathiou, C.; Tapoglou, N. Simulation of spiral bevel gear manufacturing by face hobbing and prediction of the cutting forces using a novel CAD-based model. Int. J. Adv. Manuf. Technol. 2022, 122, 3789–3813. [Google Scholar] [CrossRef]
- Yan, Y.; Xu, J.; Wiercigroch, M. Modelling of regenerative and frictional cutting dynamics. Int. J. Mech. Sci. 2019, 156, 86–93. [Google Scholar] [CrossRef]
- Kayhan, M.; Budak, E. An experimental investigation of chatter effects on tool life. Proc. Inst. Mech. Eng. Part B J. Eng. Manuf. 2009, 223, 1455–1463. [Google Scholar] [CrossRef]
- Huang, D.; Wang, Z.; Kubo, A. Hypoid gear integrated wear model and experimental verification design and test. Int. J. Mech. Sci. 2020, 166, 105228. [Google Scholar] [CrossRef]
- Park, D.; Kolivand, M.; Kahraman, A. Prediction of surface wear of hypoid gears using a semi-analytical contact model. Mech. Mach. Theory 2012, 52, 180–194. [Google Scholar] [CrossRef]
- Park, D.; Kolivand, M.; Kahraman, A. An approximate method to predict surface wear of hypoid gears using surface interpolation. Mech. Mach. Theory 2014, 71, 64–78. [Google Scholar] [CrossRef]
- Park, D.; Kahraman, A. A Surface Wear Model for Hypoid Gear Pairs; Elsevier: Amsterdam, The Netherlands, 2009. [Google Scholar]
- Das, M.; Knight, W. Critical Assessment of Cutting Force Models in the Analysis of Machine Tool Instability. In Advances in Machine Tool Design and Research, Proceedings of the International Machine Tool Design and Research Conference, Manchester, UK, 15–17 September 1971; Red Globe Press: London, UK, 1971; Volume A, pp. 87–104. [Google Scholar]
- Kaymakci, M.; Kilic, Z.; Altintas, Y. Unified cutting force model for turning, boring, drilling and milling operations. Int. J. Mach. Tools Manuf. 2012, 54, 34–45. [Google Scholar] [CrossRef]
- Merritt, H. Theory of self-excited machine-tool chatter: Contribution to machine-tool chatter research—1. J. Eng. Ind. 1965, 87, 447–454. [Google Scholar] [CrossRef]
- Mohammadi, Y.; Ahmadi, K. Chatter in milling with robots with structural nonlinearity. Mech. Syst. Signal Process. 2022, 167, 108523. [Google Scholar] [CrossRef]
- Kilic, Z.; Altintas, Y. Generalized modelling of cutting tool geometries for unified process simulation. Int. J. Mach. Tools Manuf. 2016, 104, 14–25. [Google Scholar] [CrossRef]
- Kilic, Z.; Altintas, Y. Generalized mechanics and dynamics of metal cutting operations for unified simulations. Int. J. Mach. Tools Manuf. 2016, 104, 1–13. [Google Scholar] [CrossRef]
- Moradi, H.; Movahhedy, M.R.; Vossoughi, G. Bifurcation analysis of milling process with tool wear and process damping: Regenerative chatter with primary resonance. Nonlinear Dyn. 2012, 70, 481–509. [Google Scholar] [CrossRef]
- Moradi, H.; Vossoughi, G.; Movahhedy, M.R.; Ahmadian, M.T. Forced vibration analysis of the milling process with structural nonlinearity, internal resonance, tool wear and process damping effects. Int. J. Non-Linear Mech. 2013, 54, 22–34. [Google Scholar] [CrossRef]
- Feng, J.; Wan, M.; Dong, Z.-Y.; Zhang, W.-H. A unified process damping model considering the varying stiffness of the milling system. Int. J. Mach. Tools Manuf. 2019, 147, 103470. [Google Scholar] [CrossRef]
- Schmitz, T.L.; Couey, J.; Marsh, E.; Mauntler, N.; Hughes, D. Runout effects in milling: Surface finish, surface location error, and stability. Int. J. Mach. Tools Manuf. 2007, 47, 841–851. [Google Scholar] [CrossRef]
- Siddhpura, M.; Paurobally, R. A review of chatter vibration research in turning. Int. J. Mach. Tools Manuf. 2012, 61, 27–47. [Google Scholar] [CrossRef]
- Uhlmann, E.; Mahr, F. A time domain simulation approach for micro milling processes. Procedia CIRP 2012, 4, 22–28. [Google Scholar] [CrossRef]
- Huang, K.; Yu, J.; Wang, J.; Zhang, C.; Wan, L. A vectorization model to closed-form solution for cutting forces prediction during face-hobbing of hypoid gears. Mech. Mach. Theory 2022, 173, 104824. [Google Scholar] [CrossRef]
- Huang, K.; Yu, J.; Luo, H.; Shang, Z.; Huang, S.; Fu, L. An efficient vectorization solution to cutting dynamics modeling for face-hobbing of hypoid gears. Mech. Mach. Theory 2024, 191, 105504. [Google Scholar] [CrossRef]








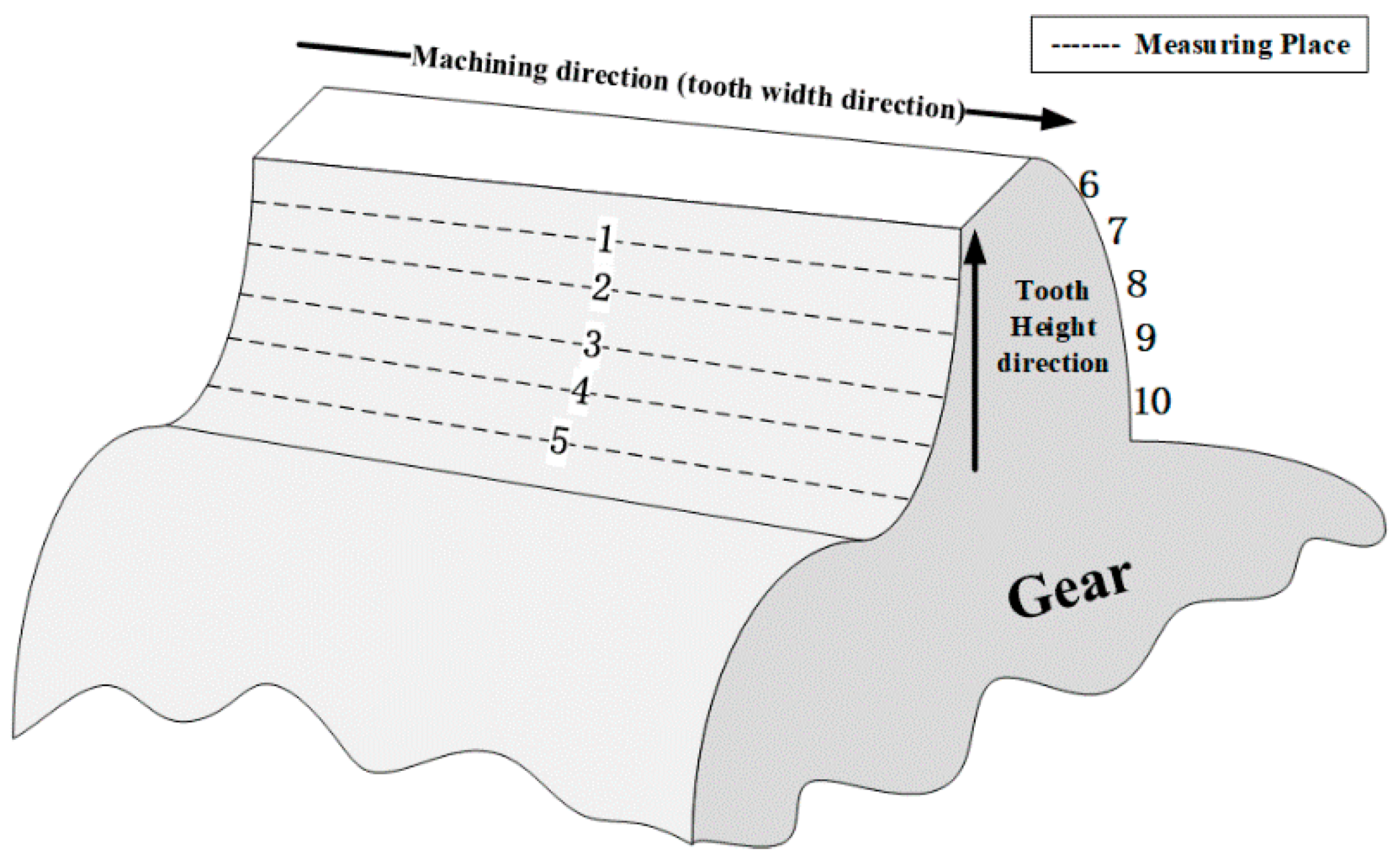


| Name | Codename/Unit | Number | |||
|---|---|---|---|---|---|
| Tilt angle | χ2/deg | 0 | |||
| Aanular position | qp/deg | 45.0943 | |||
| Radial tool position | Ex/mm | 250.9563 | |||
| Workpiece mounting angle | δM/deg | 73 | |||
| Vertical wheel position | Ep2/mm | 0 | |||
| Profile crown wheel radius | rp/mm | 209.7045 | |||
| Machine horizontal coordinate of profile crown wheel | Xp/mm | 97.6625 | |||
| Machine vertical coordinate of profile crown wheel | Yp/mm | −190.4906 | |||
| Position of entry on the x-axis | X/mm | −64.1520 | |||
| Position of entry on the y-axis | Y/mm | −173.6775 | |||
| Angular rotation of axis B (deg) | β/deg | 17 | |||
| Length of workpiece fixture | OW/mm | 292.8 | |||
| Number of teeth in the cutter | Nh | 17 | |||
| Primary cutting edge pressure angle of the outer cutter | αbouter/deg | 23.8851 | |||
| Rake angle of the outer cutter tool | αrouter/deg | 14.1897 | |||
| Mounting inclination angle of the outer cutter | γouter/deg | 8.8 | |||
| Radial offset radius of the outer cutter | R1outer/mm | 64.1299 | |||
| Tangential offset distance of the outer cutter | R2outer/mm | 158.9504 | |||
| Angle between inner and outer cutters | λ/deg | 9.03 | |||
| Primary cutting edge pressure angle of the inner cutter | αbinner/deg | 21.4713 | |||
| Rake angle of the inner cutter tool | αrinner/deg | 5.0874 | |||
| Mounting inclination angle of the inner cutter | γinner/deg | 8.8 | |||
| Cutter head rotation speed | ωh/rpm | 141 | |||
| Processing parameters | Feed stage | 1 | 2 | 3 | 4 |
| Distance from tool tip to tooth bottom (mm) | 20.3943 | 10 | 1 | 0 | |
| Feed speed (mm/min) | 6.0373 | 4.6957 | 2.6832 | 2.3478 | |
| Modal Stiffness (×109 N/m) | Modal Damping (Ns/m) | Modal Mass (kg) | Natural Frequency (Hz) | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| kHx | kHy | kHz | cHx | cHy | cHz | mHx | mHy | mHz | ωHx | ωHy | ωHz |
| 8.56 | 1.11 | 28.2 | 159,460 | 66,338 | 720,196 | 7426.31 | 999.37 | 24847.5 | 166.13 | 167.16 | 162.23 |
| Rule | Sqtwolog | Rigrsure | Heursure | Minmaxi | ||||
|---|---|---|---|---|---|---|---|---|
| Function | Hard | Soft | Hard | Soft | Hard | Soft | Hard | Soft |
| SNR | 22.1877 | 22.1679 | 21.2286 | 22.5046 | 22.1989 | 22.1615 | 22.2362 | 22.1926 |
| RMSE | 0.059525 | 0.059661 | 0.066474 | 0.057393 | 0.059448 | 0.059704 | 0.059193 | 0.059491 |
| Signal | Value | Mean | Variance | Kurtosis | Crest Factor | Shannon Entropy |
|---|---|---|---|---|---|---|
| X-axis | Measured values | 0.16706 | 0.051434 | 3.2649 | 1.3575 | 3.1108 |
| Simulated values | 0.133784 | 0.03785 | 2.451 | 1.2187 | 3.5477 | |
| Error | 24.88% | 35.89% | 33.21% | 11.39% | 14.04% | |
| Y-axis | Measured values | 0.50263 | 0.33961 | 2.1033 | 1.1594 | 3.4626 |
| Simulated values | 0.49427 | 0.3387 | 2.1611 | 1.1775 | 3.4447 | |
| Error | 1.69% | 0.27% | 2.75% | 1.56% | 0.52% | |
| Z-axis | Measured values | 0.46945 | 0.3224 | 2.3353 | 1.2095 | 3.4208 |
| Simulated values | 0.50708 | 0.35297 | 2.0311 | 1.1716 | 3.4642 | |
| Error | 8.02% | 9.48% | 14.9783 | 3.23% | 1.27% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, J.; Qian, J.; Huang, K.; Shang, Z.; Yu, J. Chatter and Surface Waviness Analysis in Oerlikon Face Hobbing of Spiral Bevel Gears. Aerospace 2024, 11, 535. https://doi.org/10.3390/aerospace11070535
Wang J, Qian J, Huang K, Shang Z, Yu J. Chatter and Surface Waviness Analysis in Oerlikon Face Hobbing of Spiral Bevel Gears. Aerospace. 2024; 11(7):535. https://doi.org/10.3390/aerospace11070535
Chicago/Turabian StyleWang, Jingchao, Jun Qian, Kaifeng Huang, Zhentao Shang, and Jianwu Yu. 2024. "Chatter and Surface Waviness Analysis in Oerlikon Face Hobbing of Spiral Bevel Gears" Aerospace 11, no. 7: 535. https://doi.org/10.3390/aerospace11070535
APA StyleWang, J., Qian, J., Huang, K., Shang, Z., & Yu, J. (2024). Chatter and Surface Waviness Analysis in Oerlikon Face Hobbing of Spiral Bevel Gears. Aerospace, 11(7), 535. https://doi.org/10.3390/aerospace11070535






