The Generation Mechanism of the Side Force and Yawing Moment of a Rotating Missile with Wrap-Around Fins
Abstract
1. Introduction
2. Numerical Approach
2.1. Numerical Methods
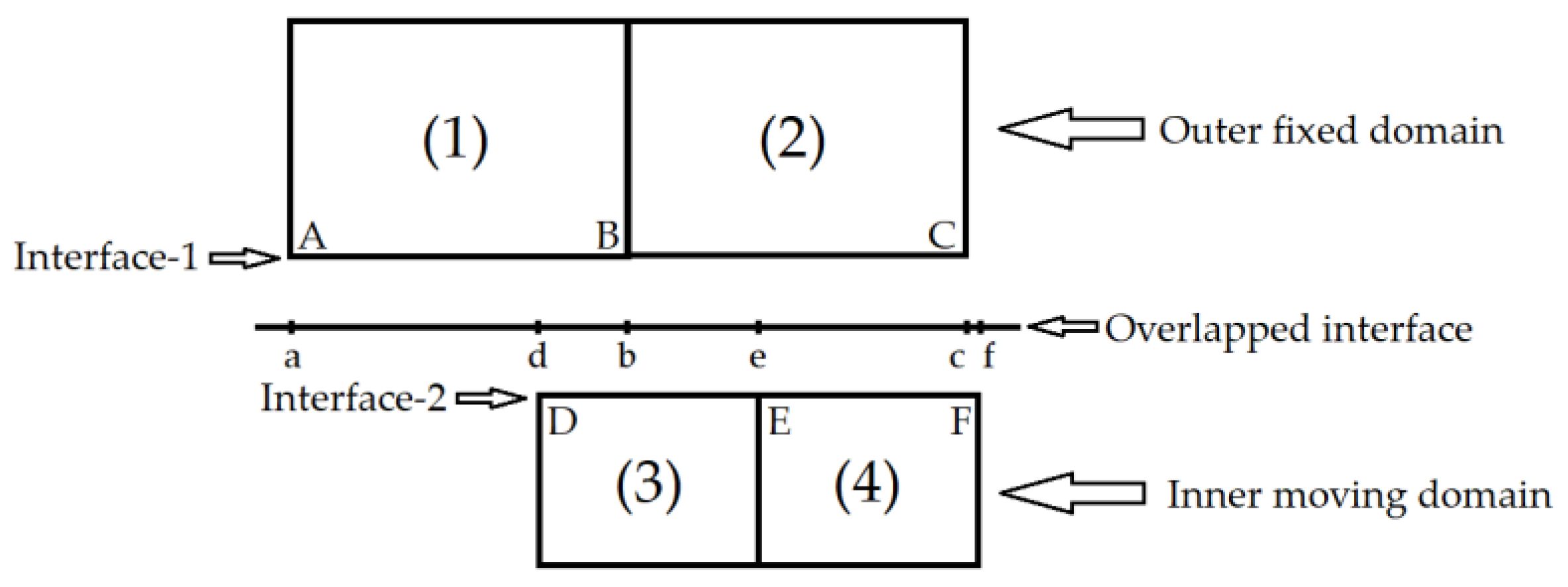
2.2. Sliding Mesh Technique
3. Computational Conditions and Validation
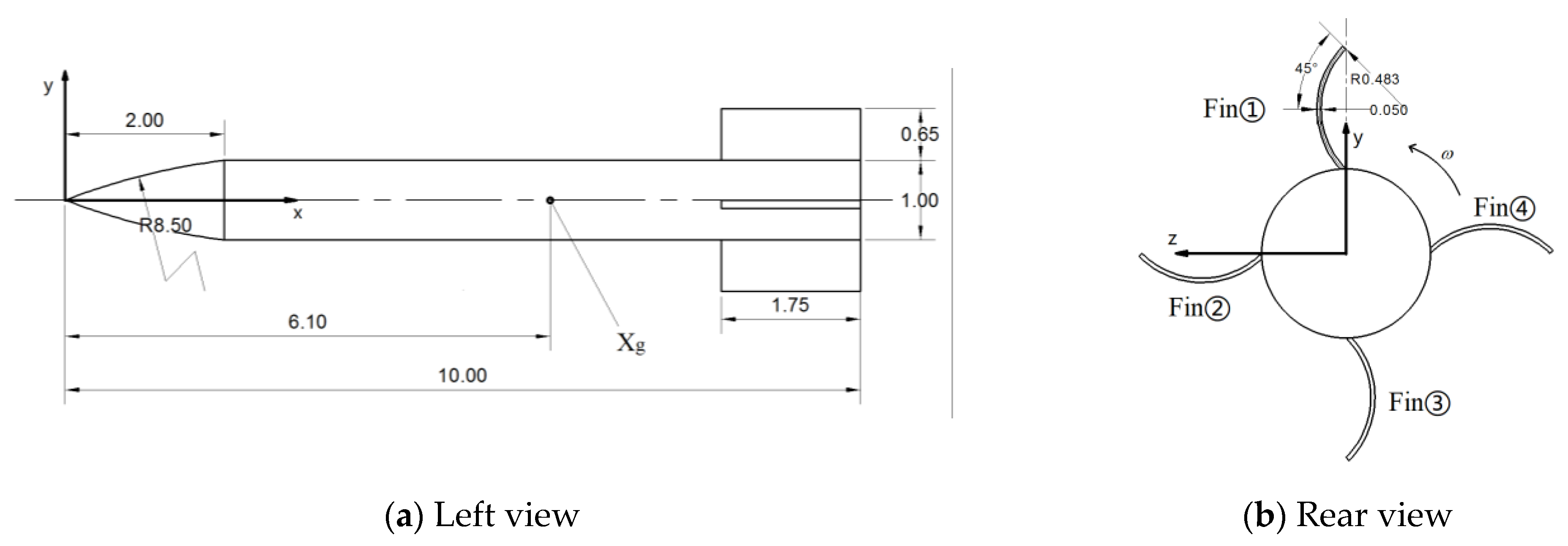
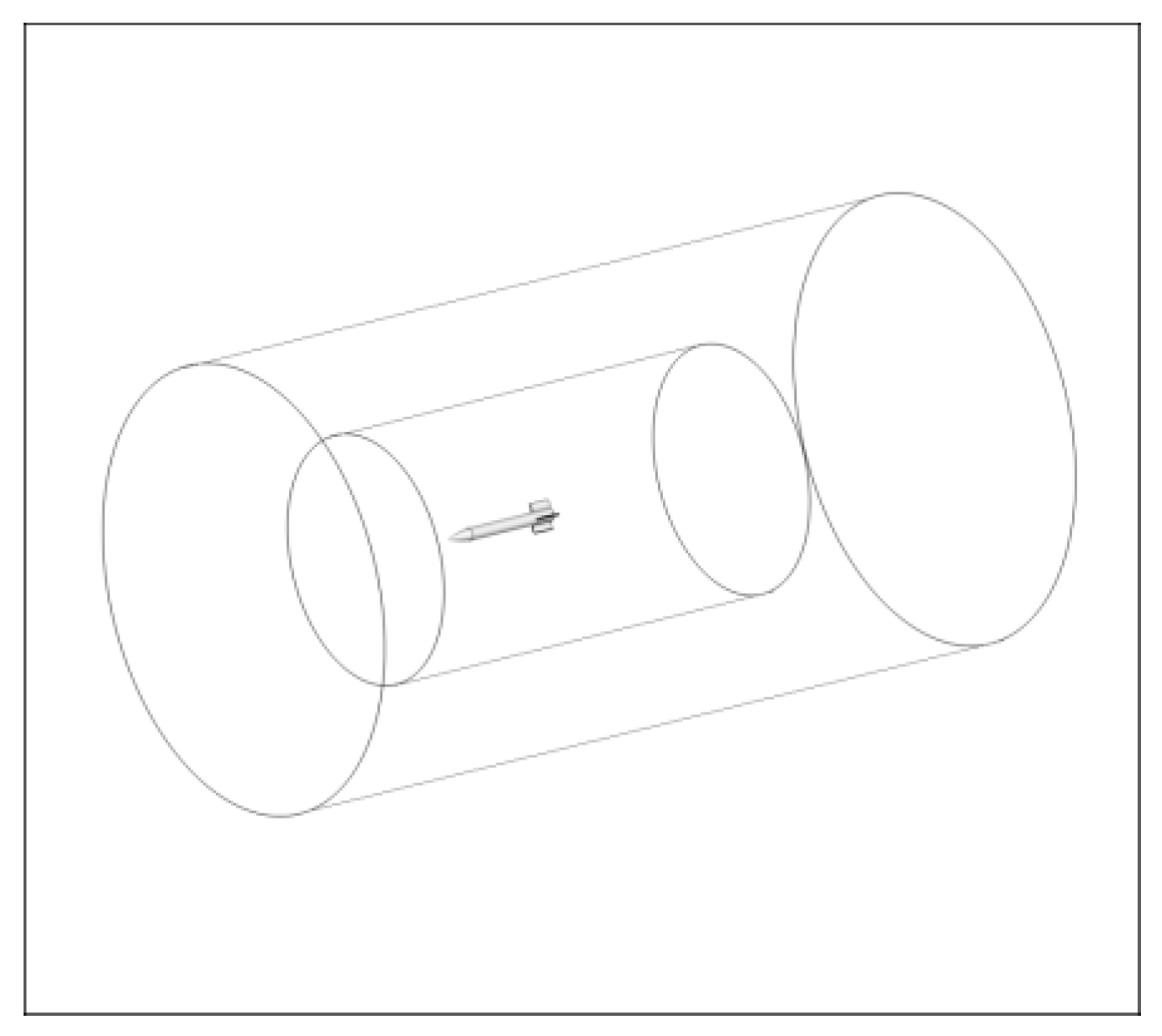
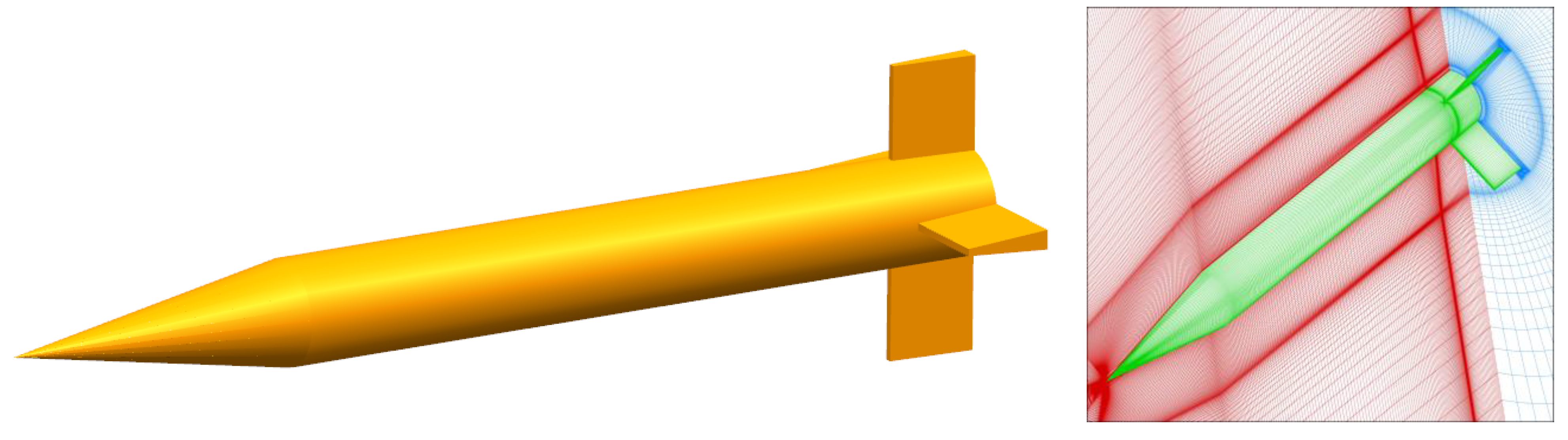
3.1. Models and Grids
3.2. Computational Conditions
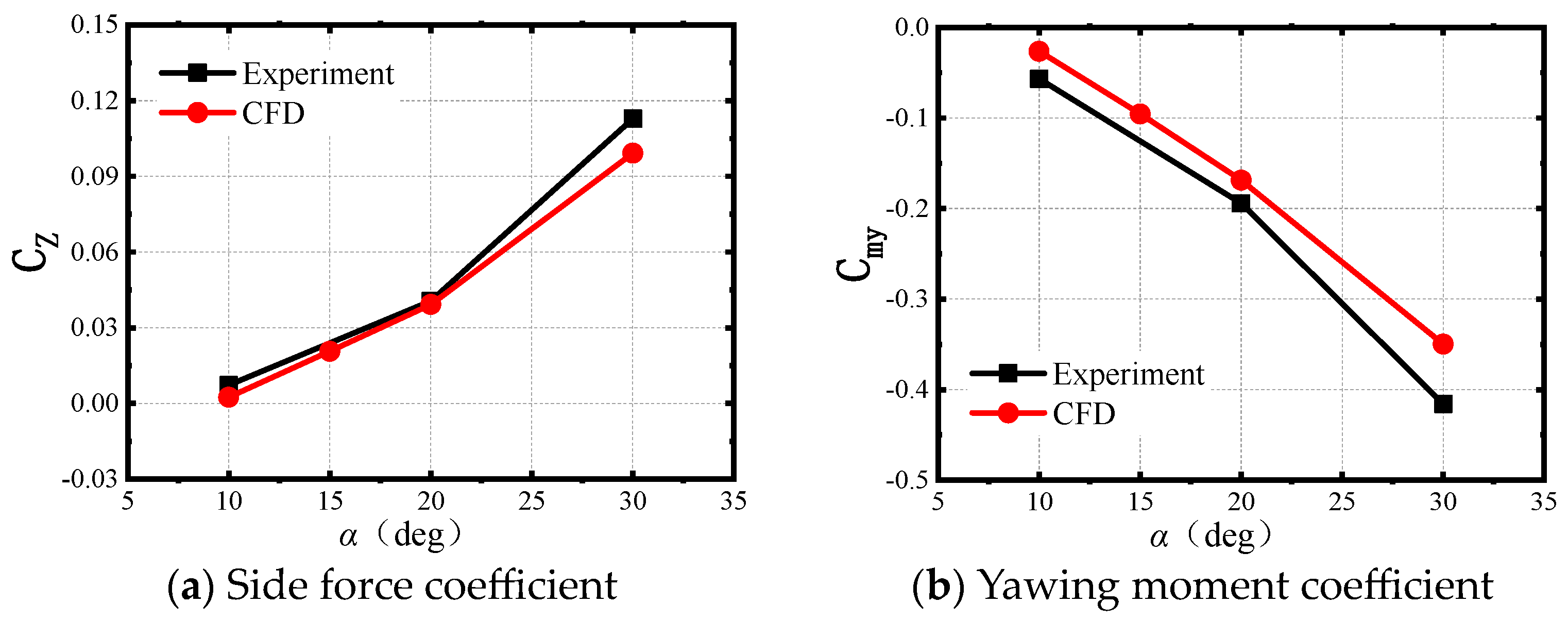
3.3. Validation of Unsteady Numerical Methods
3.3.1. Validation of Rotation Method
3.3.2. Validation of Grid Independence and Time-Step Independence
4. Results and Discussion
4.1. Time-Averaged Side Force and Yawing Moment Properties of Rotating Missile
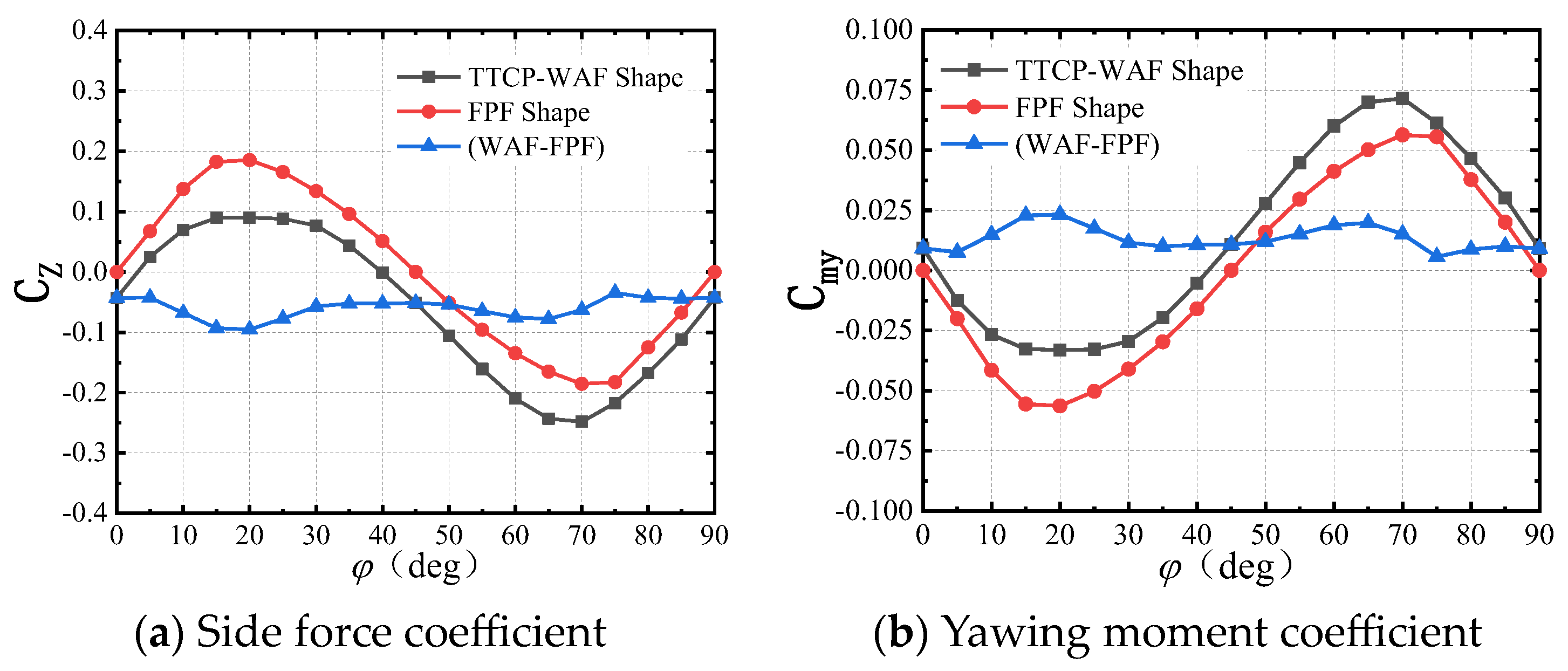
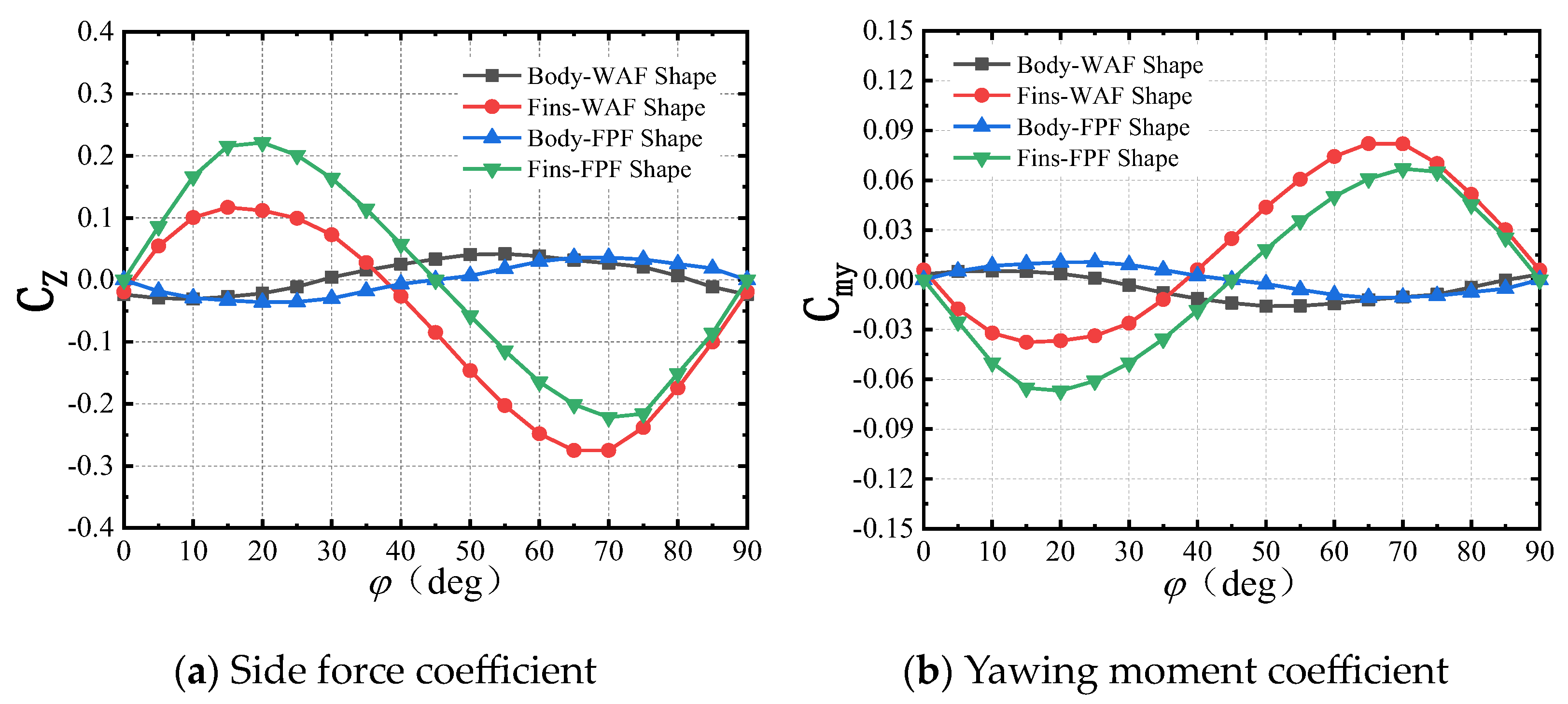
4.1.1. Composition of Side Force and Yawing Moment
4.1.2. Side Force and Yawing Moment Characteristics of Rotating Missile
4.2. Characteristics and Generation Mechanism of Static Side Force and Yawing Moment
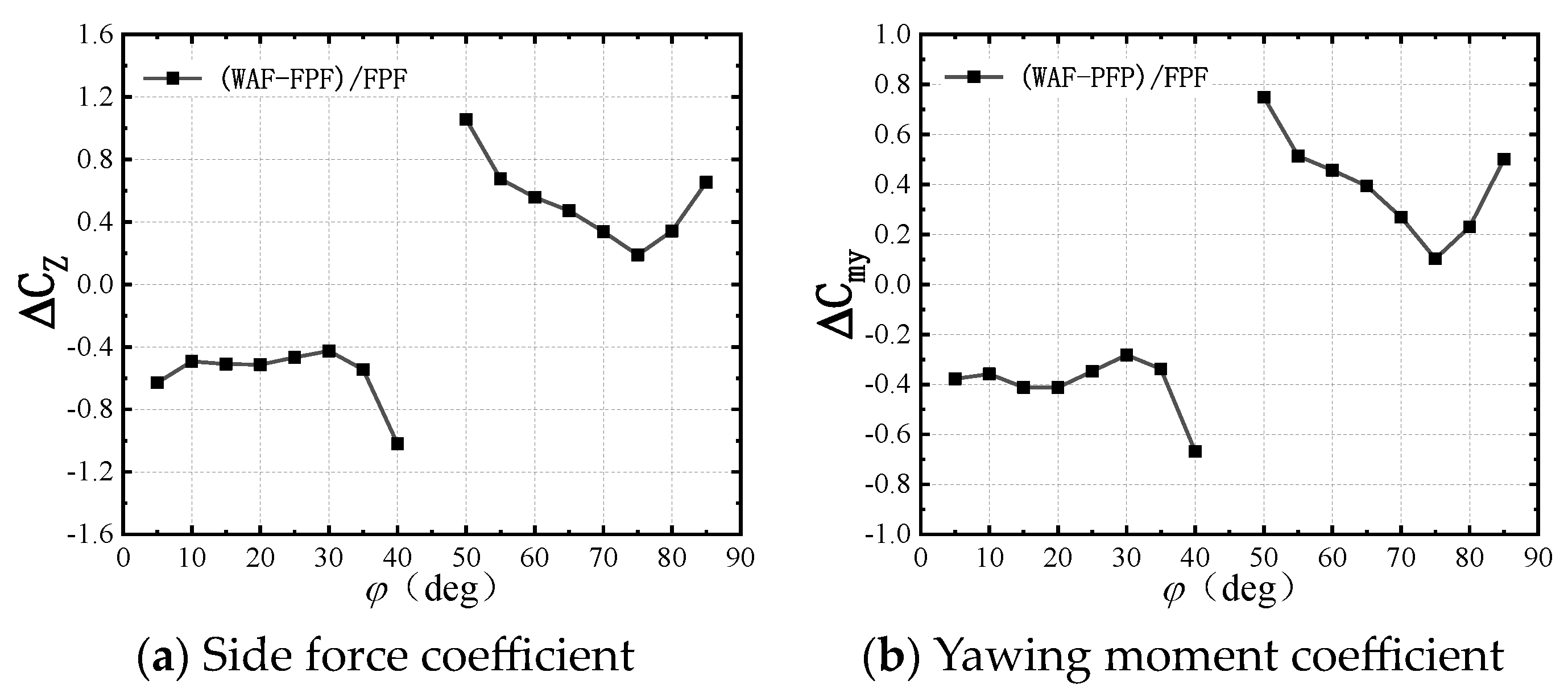
4.2.1. Static Side Force and Yawing Moment
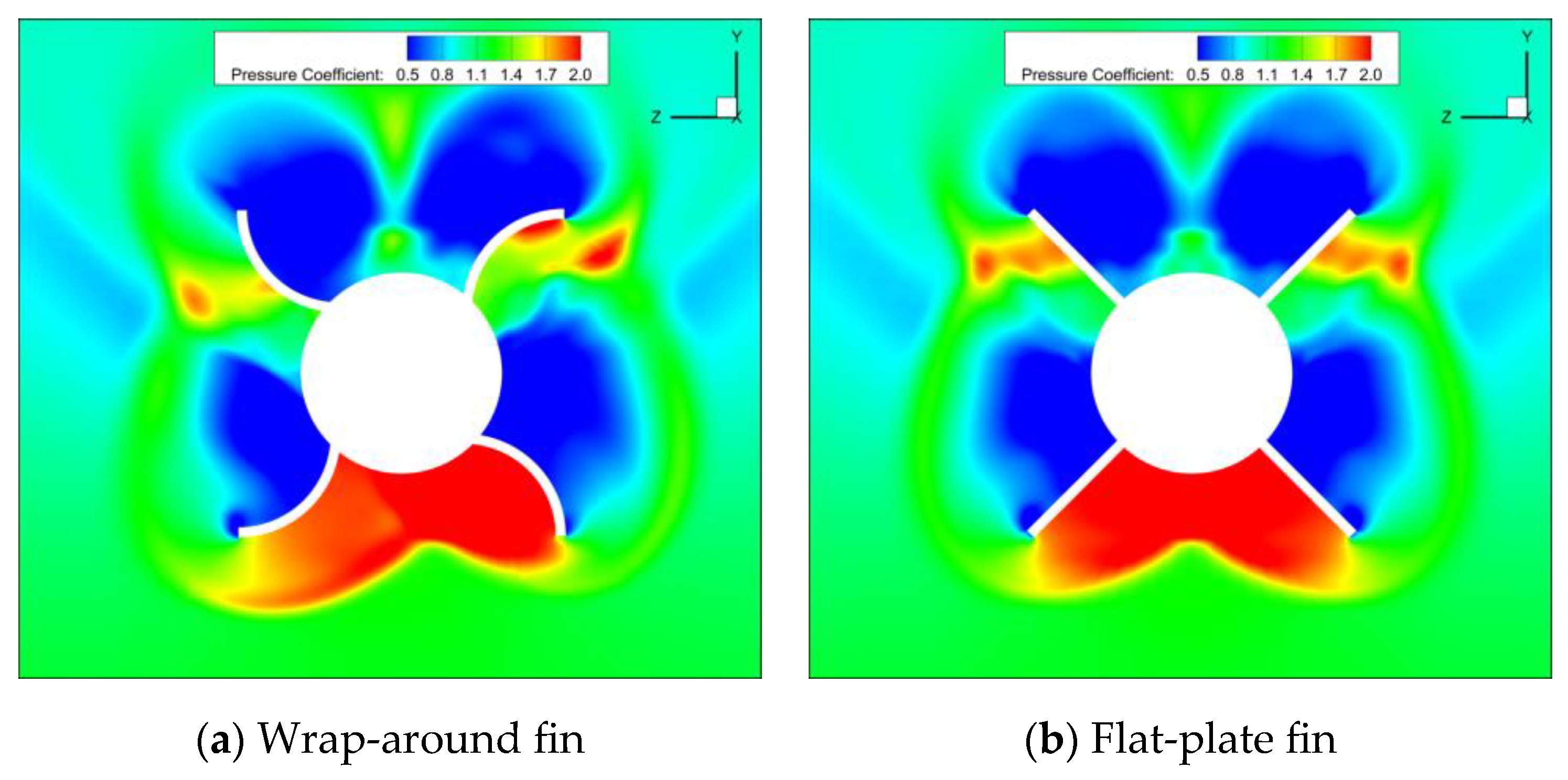
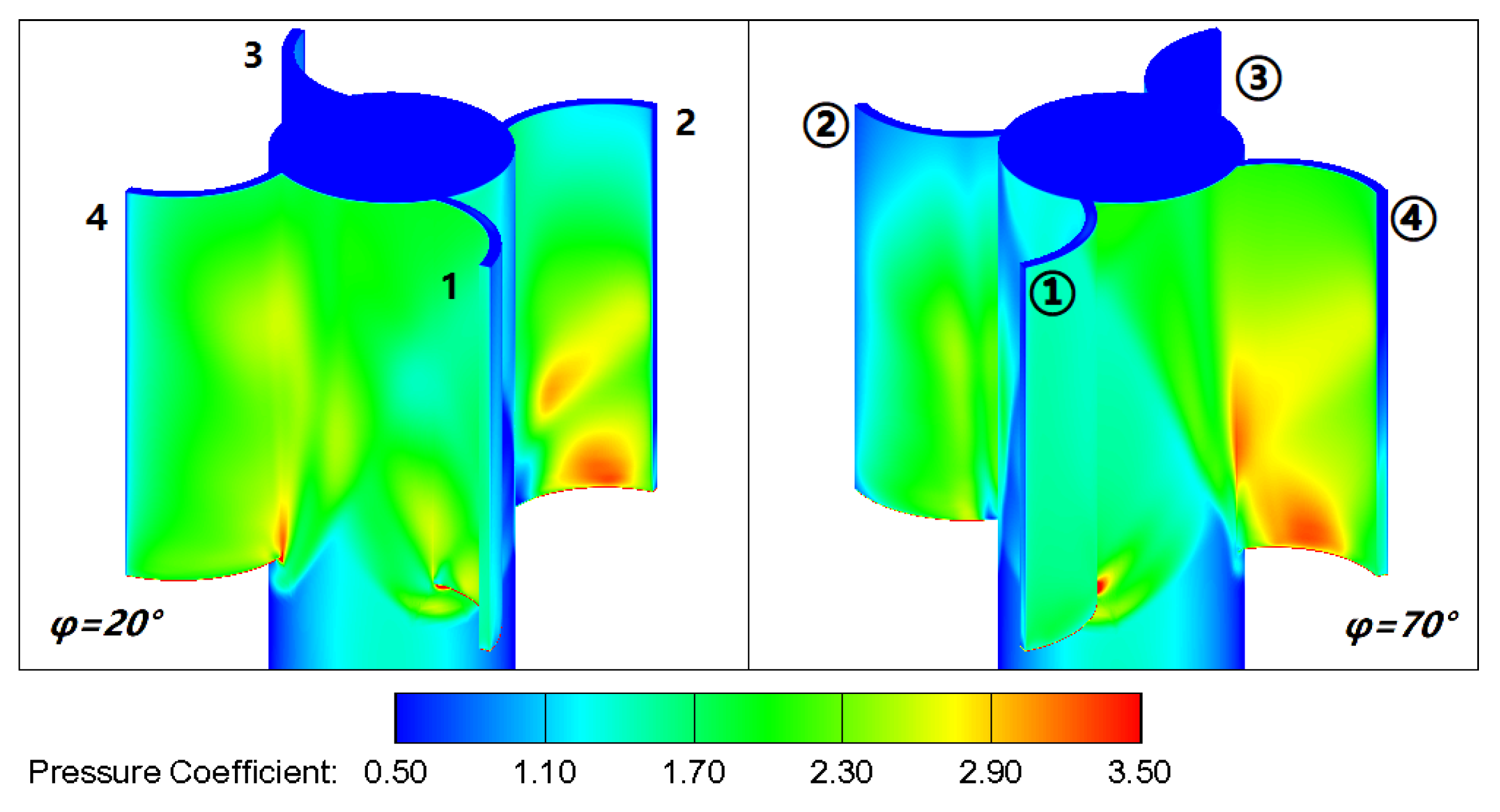
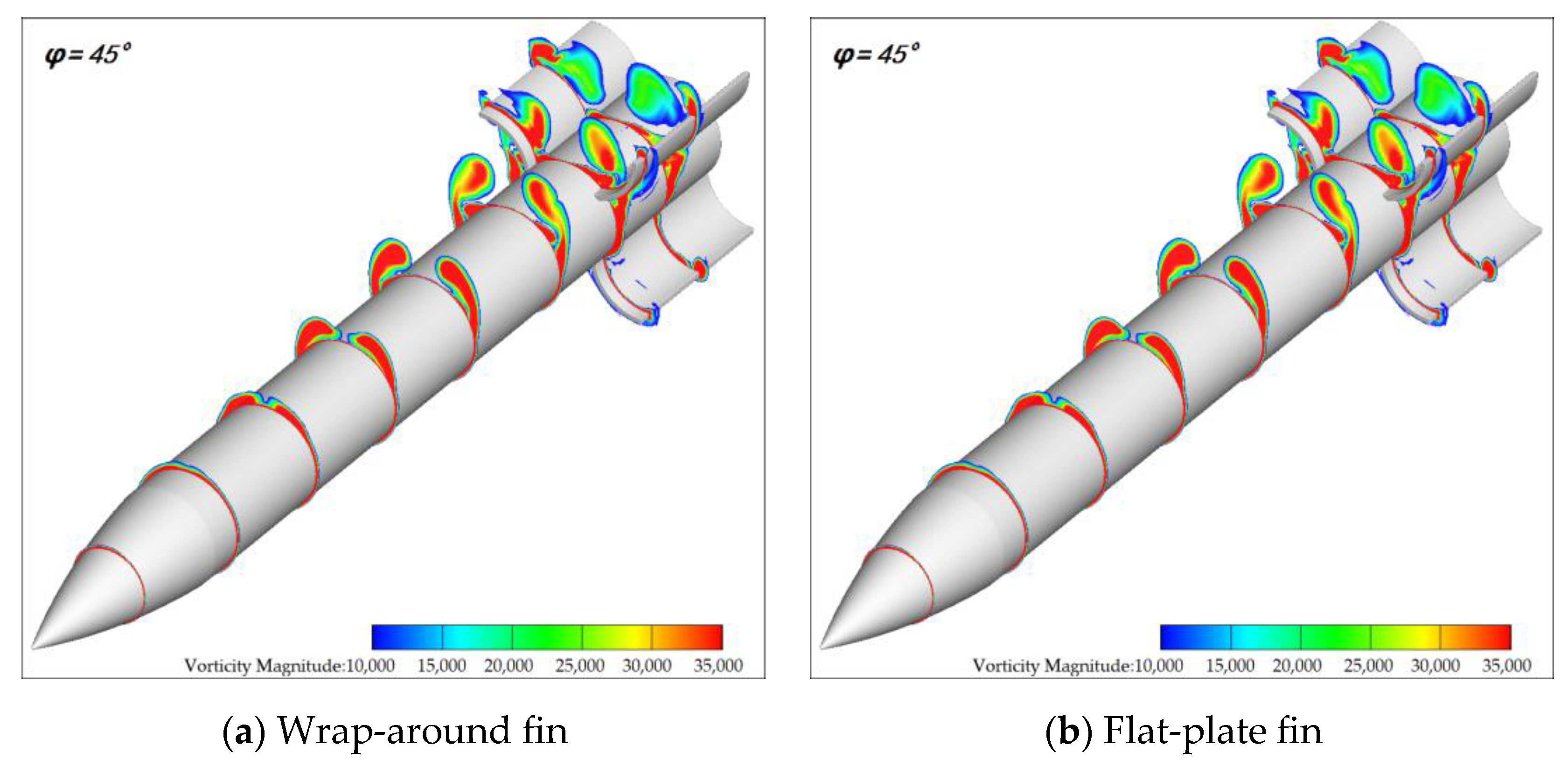
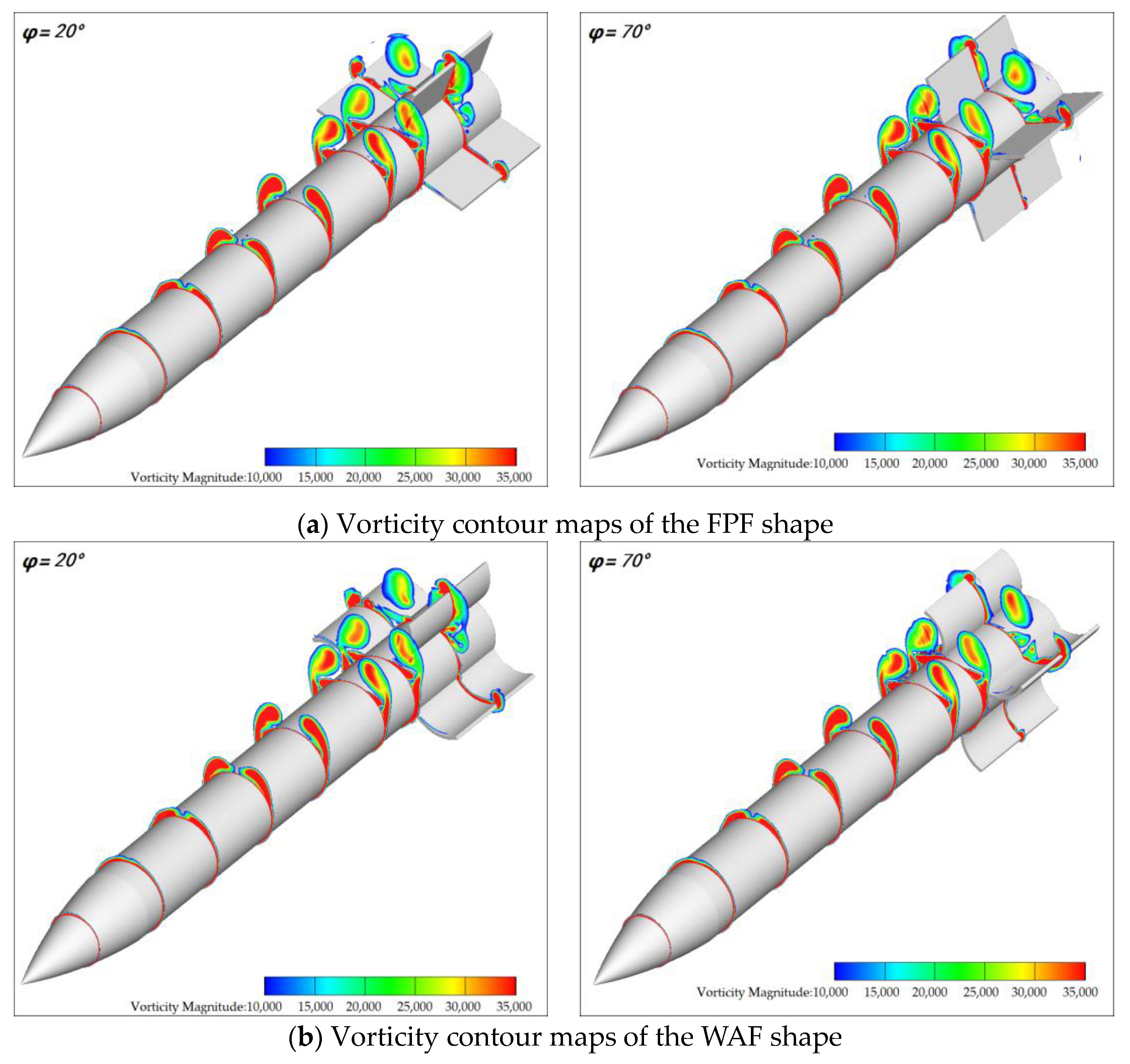
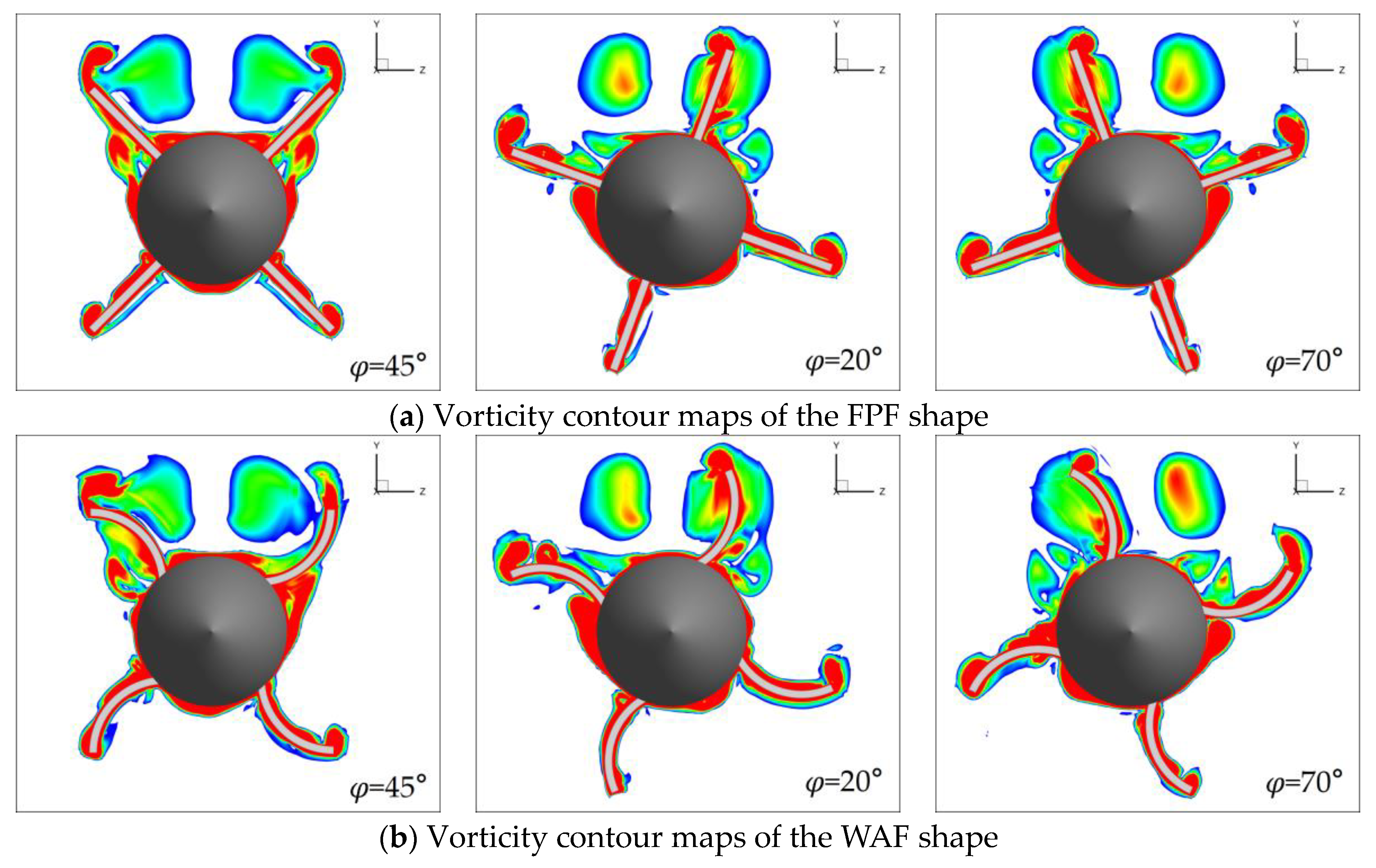
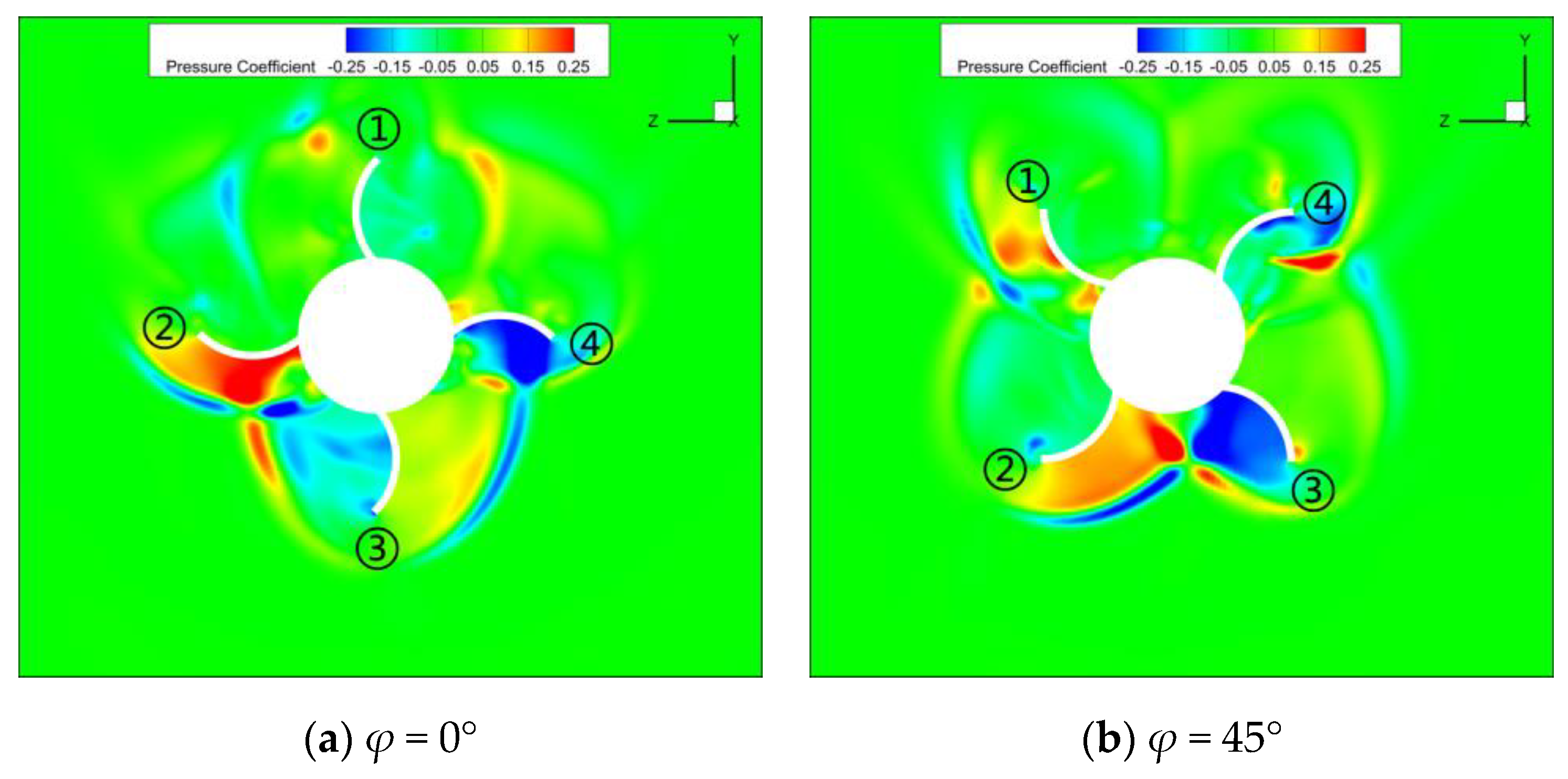
4.2.2. Generation Mechanism of Static Side Force and Yawing Moment of Fins
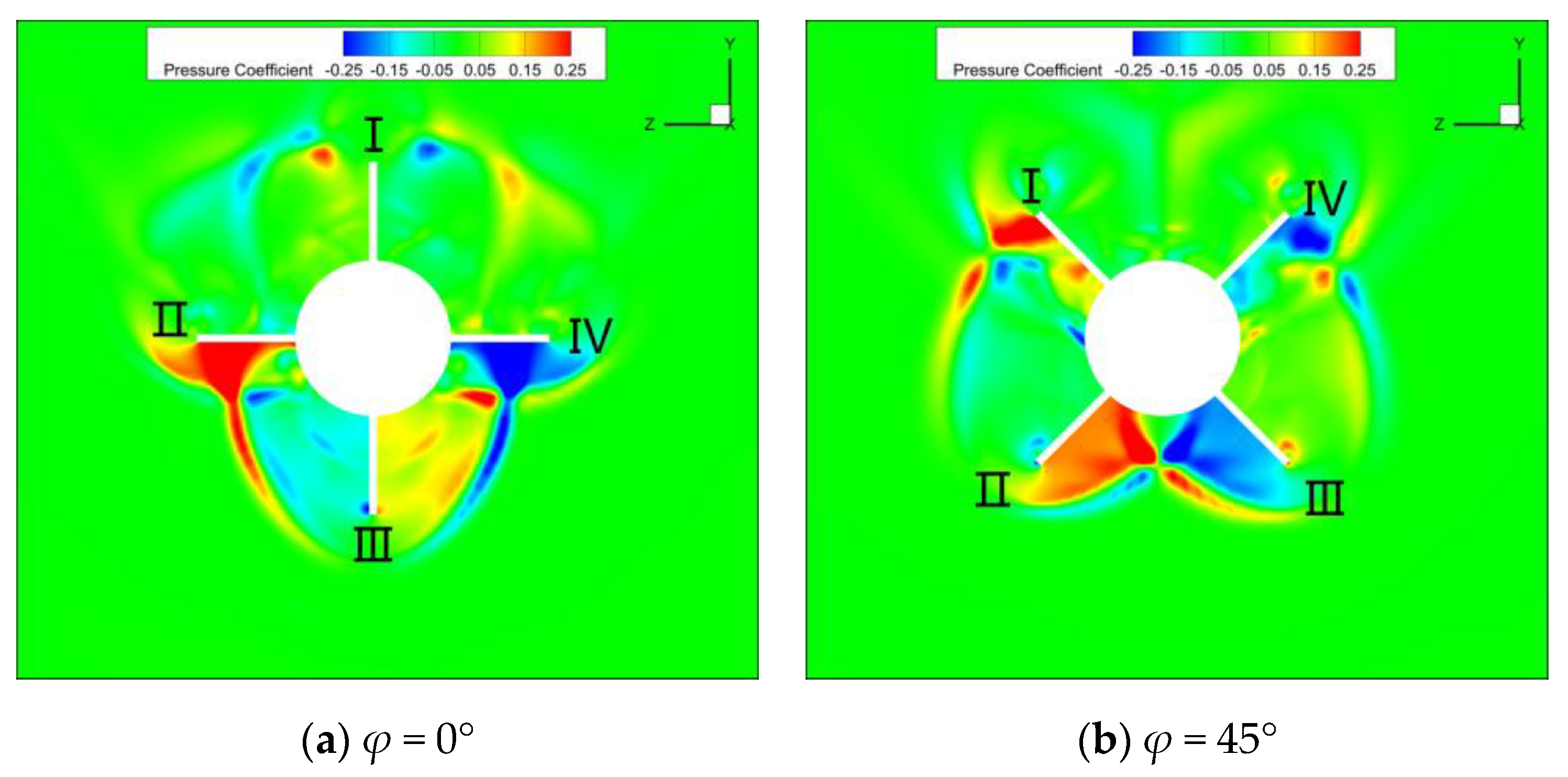
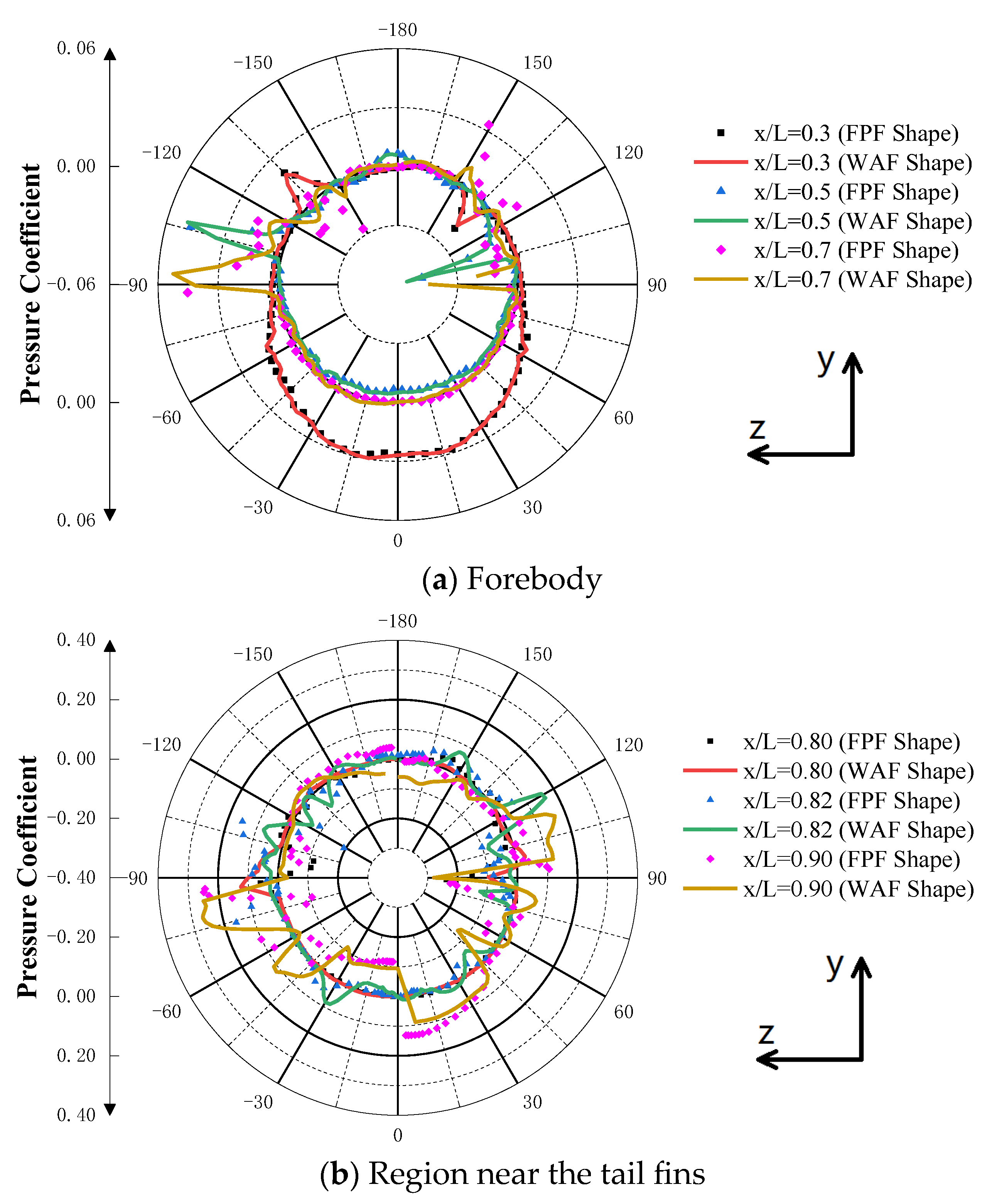
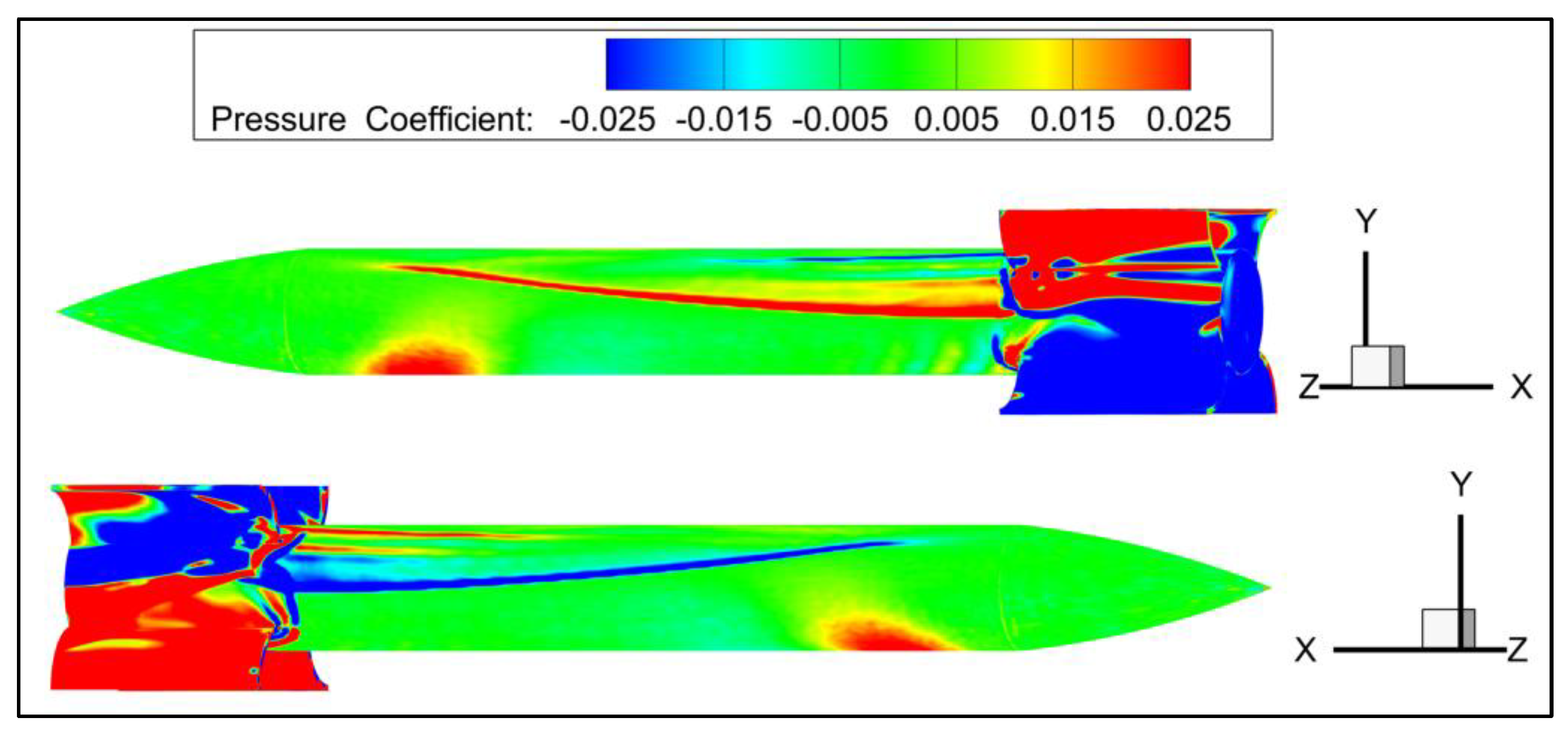
4.2.3. Generation Mechanism of Static Side Force and Yawing Moment of Body
4.3. Side Force and Yawing Moment Induced by Rotation Rate and Their Generation Mechanism
4.3.1. Characteristics of Side Force and Yawing Moment Induced by Rotation Rate
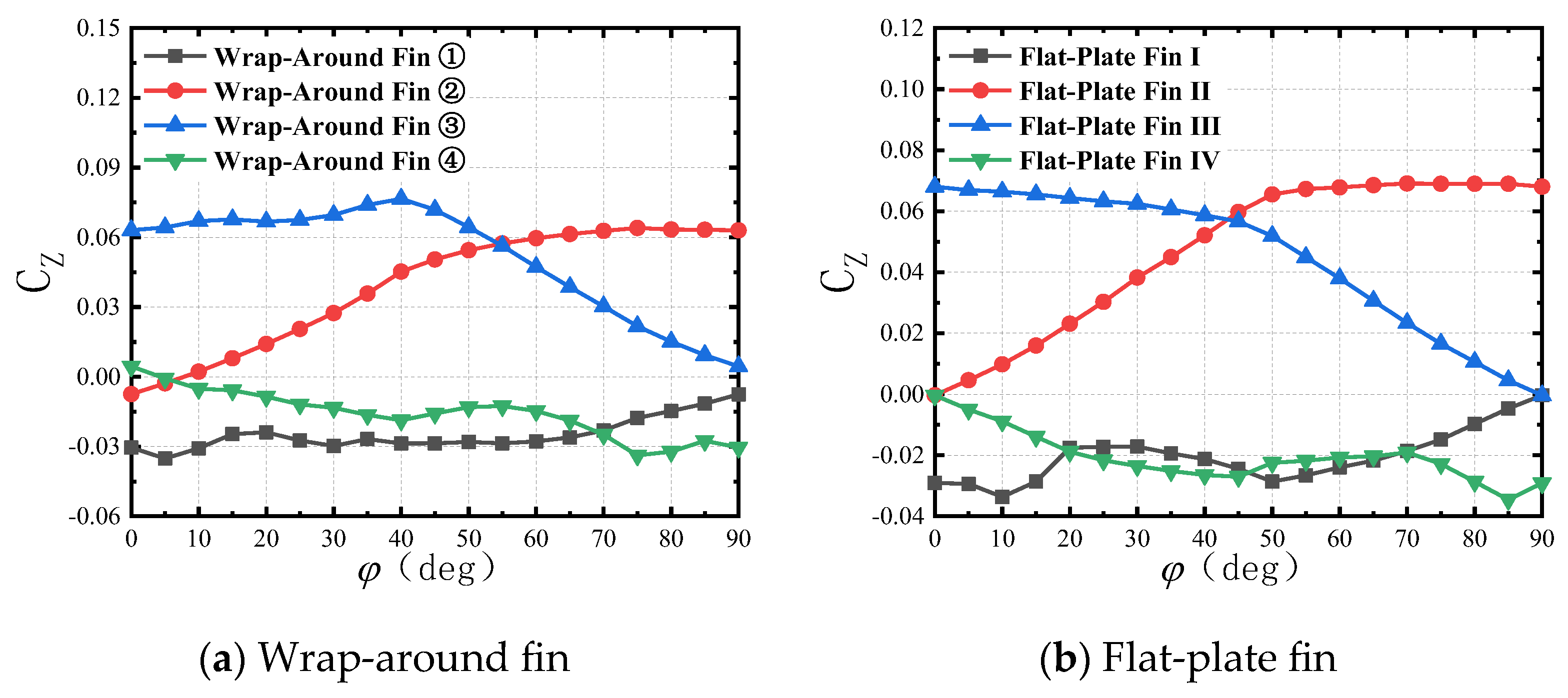
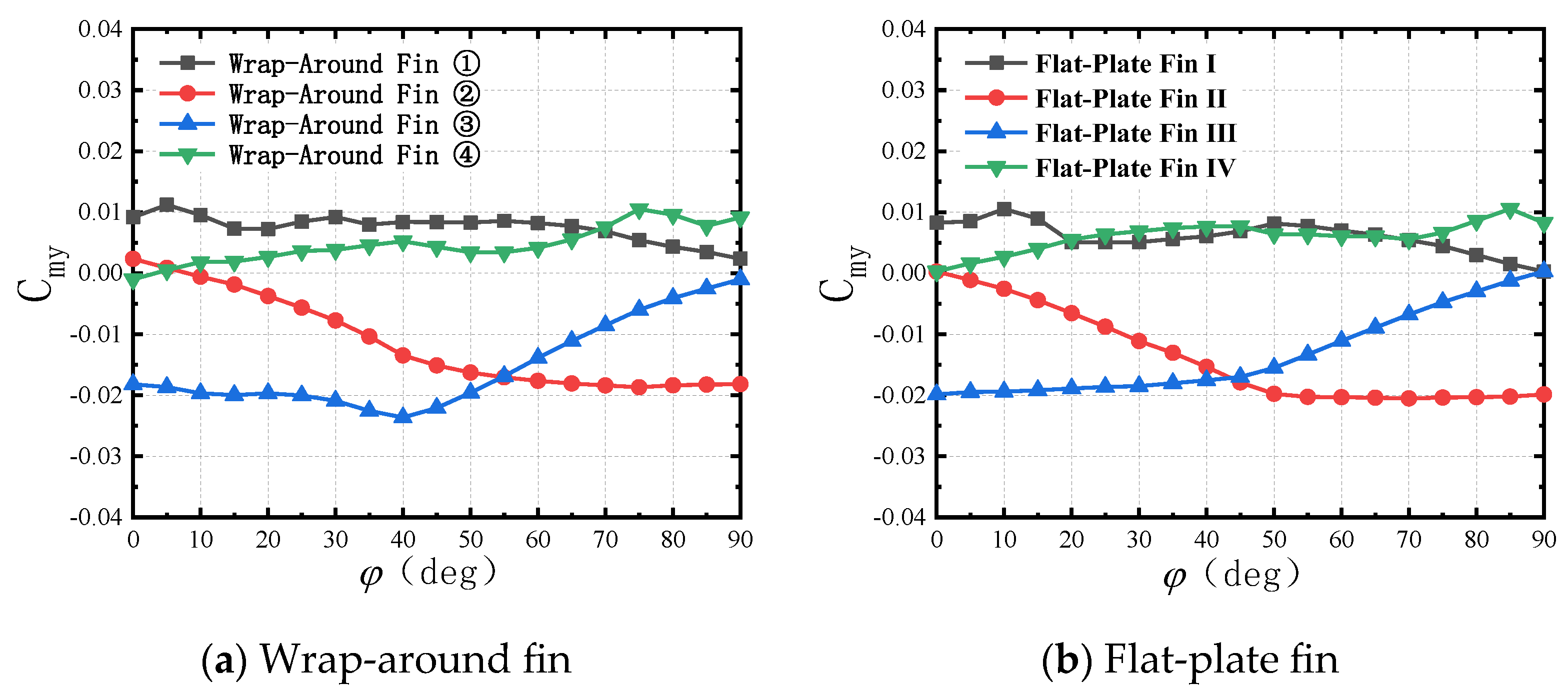
4.3.2. Side Force and Yawing Moment Induced by Rotation Rate on Fins
4.3.3. Side Force and Yawing Moment Induced by Rotation Rate on Body
5. Conclusions
- (1)
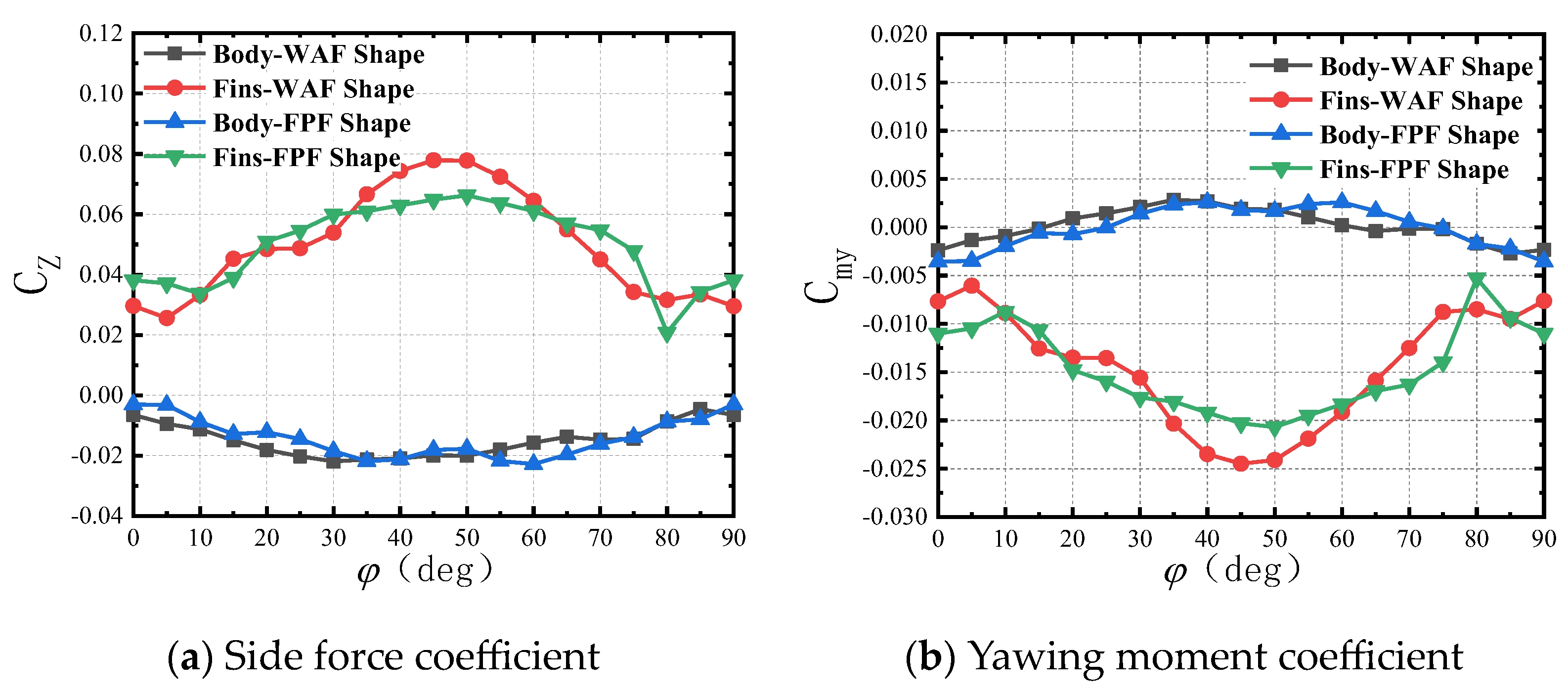
- The side force and yawing moment of WAF and FPF missiles exhibit a good linear relationship with the dimensionless rotation rate. The difference lies in the WAF shape, which exhibits a self-induced side force and yawing moment. For WAF missiles, the static force/moment is of the same order of magnitude as the force/moment induced by rotation; hence, both are crucial aspects that cannot be overlooked in research and design.
- (2)
- There are two main reasons for the generation of time-averaged static side force and yawing moment on the wrap-around-fin missile: flow compression and expansion caused by the convex and concave surfaces resulting from the curvature of the tail fin, and the asymmetric body–fin disturbances caused by the curvature of the tail fin. Therefore, the curvature of the tail fin is the primary influencing factor.
- (3)
- The difference in the side force and yawing moment induced by rotation between the WAF and FPF shapes is very small. The two shapes have the same generation mechanism of this force/moment. The curvature of the tail fin has little effect on the side force and yawing moment induced by rotation.
- (4)
- The fins’ mechanisms of this force/moment are the change in the fins’ effective angle of attack caused by rotation and the force enhancement and attenuation caused by the body’s shielding effect. The body’s mechanisms of this force/moment are the Magnus effect of the forebody and the aerodynamic interference caused by the fins’ rotation. The contribution of the fins is approximately twice that of the body.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| Cmy | Coefficient of yawing moment, My/qLrefSref |
| Cp | Pressure coefficient, |
| Cz | Coefficient of side force, Fz/qSref |
| d | Body diameter, m |
| FZ | Side force, N |
| l | Body length, m |
| Lref | Reference length, m |
| Ma | Mach number |
| My | Yawing moment, |
| Freestream static pressure, Pa | |
| q | Dynamic pressure, |
| Sref | Reference area,πd2/4, m2 |
| t | Rotation period, s |
| Time step, s | |
| Freestream static temperature, K | |
| Freestream velocity, m/s | |
| y+ | Non-dimensional wall distance |
| Angle of attack, deg | |
| Circumferential angle, deg | |
| Density, kg/m3 | |
| φ | Rotation angle, deg |
| ω | Rotational rate, rad/s |
| Ω | Dimensionless rotational rate, |
References
- Sturek, W.B.; Dwyer, H.A.; Kayser, L.D.; Nietubicz, C.J.; Reklis, R.P.; Opalka, K.O. Computations of Magnus Effects for a Yawed, Spinning Body of Revolution. AIAA J. 1978, 16, 687–692. [Google Scholar] [CrossRef]
- Mikhail, A.G. In-Flight Flexure and Spin Lock-in for Antitank Kinetic Energy Projectiles. J. Spacecr. Rocket. 1996, 33, 657–664. [Google Scholar] [CrossRef]
- Guidos, B.J.; Garner, J.M.; Newill, J.F.; Livecchia, C.D. Measured in-flight rod flexure of a 120-mm M829E3 kinetic energy (KE) projectile steel model. US Army Research Laboratory, Aberdeen Proving Ground, Adelphi, MD, USA. 2002; to be published. [Google Scholar]
- Martin, J.C. On Magnus Effects Caused by the Boundary-Layer Displacement Thickness on Bodies of Revolution at Small Angles of Attack. J. Aeronaut. Sci. 1957, 24, 421–429. [Google Scholar] [CrossRef]
- Fiebig, M. Laminar Boundary Layer on a Spinning Circular Cone in Supersonic Flow at a Small Angle of Attack; Cornell University: Ithaca, NY, USA, 1956. [Google Scholar]
- Sedney, R. Laminar Boundary Layer on a Spinning Cone at Small Angles of Attack in a Supersonic Flow. J. Aeronaut. Sci. 1957, 24, 430–436. [Google Scholar] [CrossRef]
- Jacobson, I.D. Contribution of a Wall Shear Stress to the Magnus Effect on Nose Shapes. AIAA J. 1974, 12, 1003–1005. [Google Scholar] [CrossRef]
- Platou, A.S. Magnus Characteristics of Finned and Nonfinned Projectiles. AIAA J. 1965, 3, 83–90. [Google Scholar] [CrossRef]
- Jenke, L.M. Experimental Roll-Damping, Magnus, and Static-Stability Characteristics of Two Slender Missile Configurations at High Angles of Attack (0 to 90 Deg) and Mach Numbers 0.2 through 2.5; Arnold Engineering Development Center; Arnold AFB: Tullahoma, TN, USA, 1976. [Google Scholar] [CrossRef]
- Jiasheng, W.; Xianming, J.; Ruisheng, M. Advances in the research for aerodynamic characteristics of Wrap-Around fins. Adv. Mech. 1995, 25, 102–113. [Google Scholar] [CrossRef]
- Jiasheng, W.; Xianming, J.; Wenxi, X. Self-Rolling Characteristics of Wrap Around Fin-Body Combinations. Trans. Beijing Inst. Technol. 1993, 2, 180–186. [Google Scholar]
- Eastman, D.; Wenndt, D. Aerodynamics of Maneuvering Missiles with Wrap-around Fins. In Proceedings of the 3rd Applied Aerodynamics Conference, Colorado Springs, CO, USA, 14–16 October 1985; American Institute of Aeronautics and Astronautics: Reston, VA, USA, 1985. [Google Scholar] [CrossRef]
- Abdullah, N.A.; Ismail, N.I.; Ismail, I.I.; Azami, M.H.; Nordin, N.H.; Sukindar, N.A.; Abidin, Z.Z.; Bakar, A.J.A.; Dani, N. Flow Behaviour Assessment of Smokey SAM Rocket Prototype. CFD Lett. 2021, 13, 42–56. [Google Scholar] [CrossRef]
- Wu, Y.; Huang, J.; Ji, B.; Song, L. Aerodynamic Shape Optimization of Subsonic/Supersonic Flows Integrating Variable-Fidelity Longitudinal Trim Analysis. Aerospace 2024, 11, 143. [Google Scholar] [CrossRef]
- Edge, H. Computation of the Roll Moment Coefficient for a Projectile with Wrap-around Fins. In Proceedings of the 31st Aerospace Sciences Meeting, Reno, NV, USA, 11–14 January 1993; American Institute of Aeronautics and Astronautics: Reston, VA, USA, 1993. [Google Scholar] [CrossRef]
- Abate, G.; Cook, T. Analysis of Missile Configurations with Wrap-around Fins Using Computational Fluid Dynamics. In Flight Simulation and Technologies; American Institute of Aeronautics and Astronautics: Reston, VA, USA, 1993. [Google Scholar] [CrossRef]
- Edge, H.L. Computation of the Roll Moment for a Projectile with Wrap-around Fins. J. Spacecr. Rocket. 1994, 31, 615–620. [Google Scholar] [CrossRef]
- Dahlke, C.W. A Review and Status of Wrap-Around Fin Aerodynamics. In Tenth Navy Symposium on Aeroballistics; Naval Surface Weapons Center: Dahlgren, VA, USA, 1975; Volume 1, pp. 279–324. [Google Scholar]
- Dahlke, C.W.; Flowers, L.D. The Aerodynamic Characteristics of Wrap-Around Fins, Including Fold Angle at Mach Numbers from 0.5 to 3.0; Army Missile Research, Development and Engineering Laboratory: Adelphi, MD, USA, 1974. [Google Scholar] [CrossRef]
- Tilmann, C.P.; Buter, T.A.; Bowersox, R.D.W. Characterization of the Flowfield near a Wrap-Around Fin at Mach 2.8. J. Aircr. 1998, 35, 868–875. [Google Scholar] [CrossRef]
- Zhang, G.Q.; Yu, S.C.M.; Schlüter, J. Aerodynamic Characteristics of a Wrap-around Fin Rocket. Aircr. Eng. Aerosp. Technol. 2016, 88, 82–96. [Google Scholar] [CrossRef]
- Yuwei, X.; Jie, L. Rolling Characteristics Investigation on Missile with Wrap-around Fins. J. Proj. Rocket. Missiles Guid. 2015, 35, 127–132. [Google Scholar] [CrossRef]
- Song, Q.; Yang, S.X.; Xu, Y.; Wang, F. Numerical simulation on aerodynamic characteristics of rolling rocket with Wrap Around Fins. J. Solid. Rocket. Technol. 2008, 31, 552–554+560. [Google Scholar]
- Wentao, Z.; Sijiang, C. Numerical analysis of aerodynamic characteristics of free-rotating wraparound fins projectile. J. Harbin Inst. Technol. 2023, 55, 113–122. [Google Scholar]
- Roe, P.L. Characteristic-Based Schemes for the Euler Equations. Annu. Rev. Fluid. Mech. 1986, 18, 337–365. [Google Scholar] [CrossRef]
- Menter, F.R. Two-Equation Eddy-Viscosity Turbulence Models for Engineering Applications. AIAA J. 1994, 32, 1598–1605. [Google Scholar] [CrossRef]
- Rivera, C.A.; Heniche, M.; Bertrand, F.; Glowinski, R.; Tanguy, P.A. A Parallel Finite Element Sliding Mesh Technique for the Simulation of Viscous Flows in Agitated Tanks. Int. J. Numer. Methods Fluids 2012, 69, 653–670. [Google Scholar] [CrossRef]
- Steijl, R.; Barakos, G. Sliding Mesh Algorithm for CFD Analysis of Helicopter Rotor–Fuselage Aerodynamics. Int. J. Numer. Methods Fluids 2008, 58, 527–549. [Google Scholar] [CrossRef]

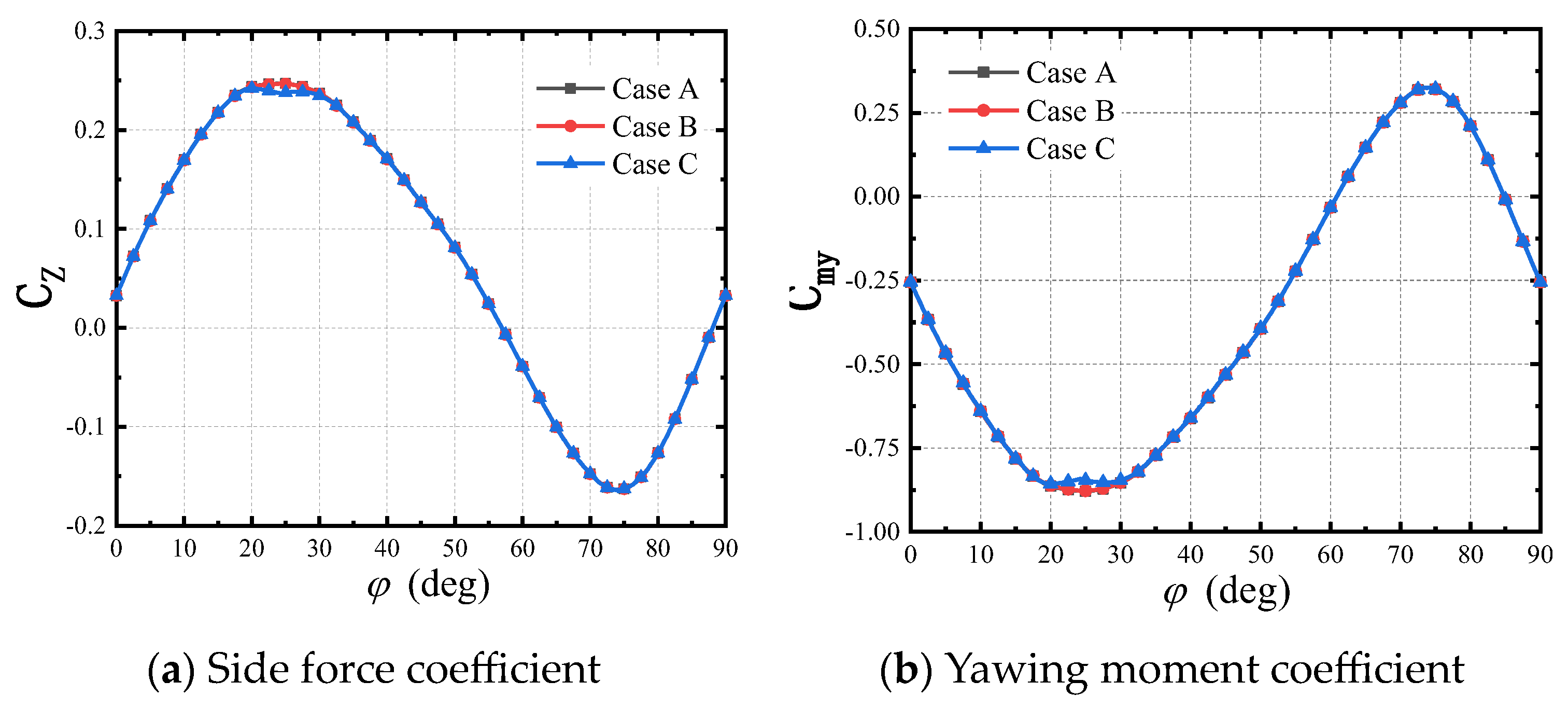
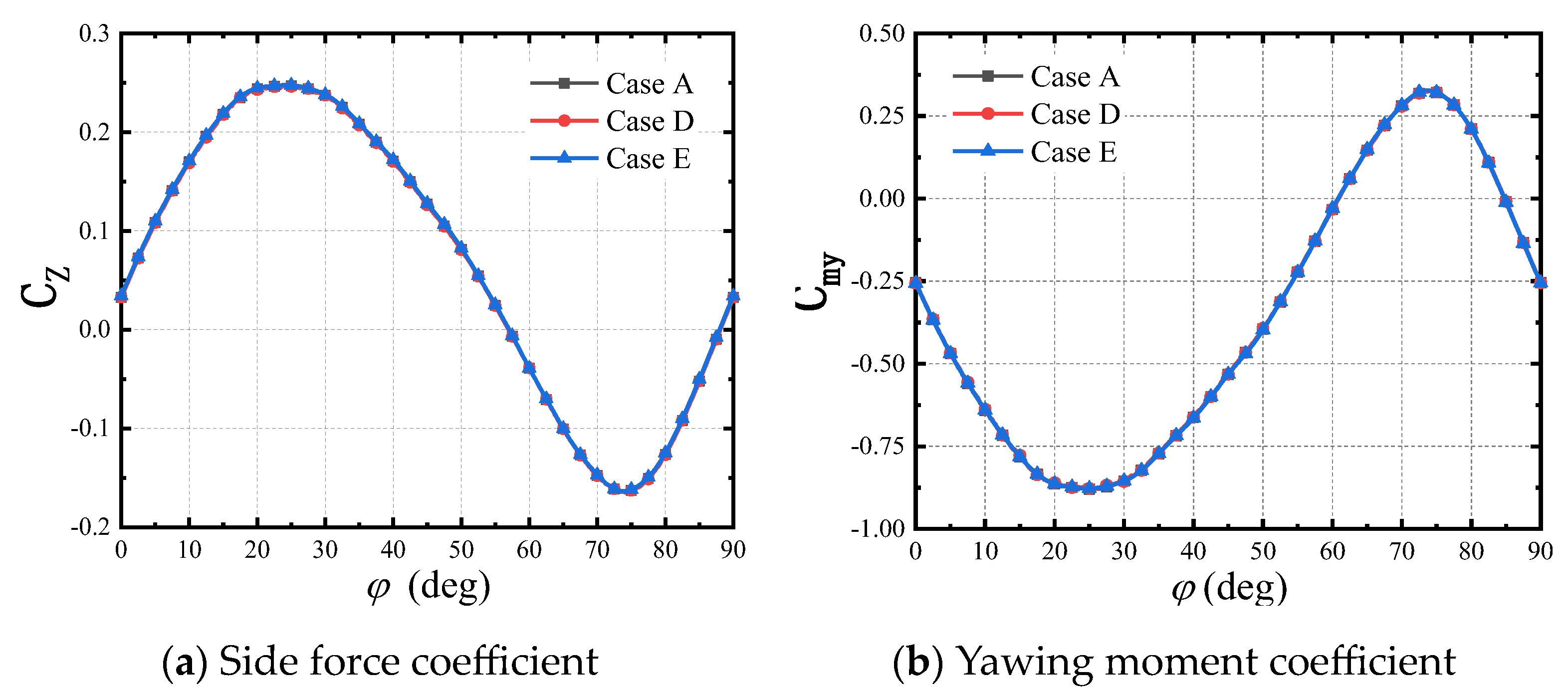






| Case | Longitudinal | Circular | Spanwise | Step Size (s) | Total Cells (Million) |
|---|---|---|---|---|---|
| A | 339 | 168 | 23 | T/1440 | 13.3 |
| B | 431 | 214 | 30 | T/1440 | 24.7 |
| C | 269 | 134 | 18 | T/1440 | 7.20 |
| D | 339 | 168 | 23 | T/720 | 13.3 |
| E | 339 | 168 | 23 | T/2880 | 13.3 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yong, Z.; Lei, J.; Yin, J. The Generation Mechanism of the Side Force and Yawing Moment of a Rotating Missile with Wrap-Around Fins. Aerospace 2024, 11, 765. https://doi.org/10.3390/aerospace11090765
Yong Z, Lei J, Yin J. The Generation Mechanism of the Side Force and Yawing Moment of a Rotating Missile with Wrap-Around Fins. Aerospace. 2024; 11(9):765. https://doi.org/10.3390/aerospace11090765
Chicago/Turabian StyleYong, Zheng, Juanmian Lei, and Jintao Yin. 2024. "The Generation Mechanism of the Side Force and Yawing Moment of a Rotating Missile with Wrap-Around Fins" Aerospace 11, no. 9: 765. https://doi.org/10.3390/aerospace11090765
APA StyleYong, Z., Lei, J., & Yin, J. (2024). The Generation Mechanism of the Side Force and Yawing Moment of a Rotating Missile with Wrap-Around Fins. Aerospace, 11(9), 765. https://doi.org/10.3390/aerospace11090765






