Research on a Honeycomb Structure for Pyroshock Isolation at the Spacecraft–Rocket Interface
Abstract
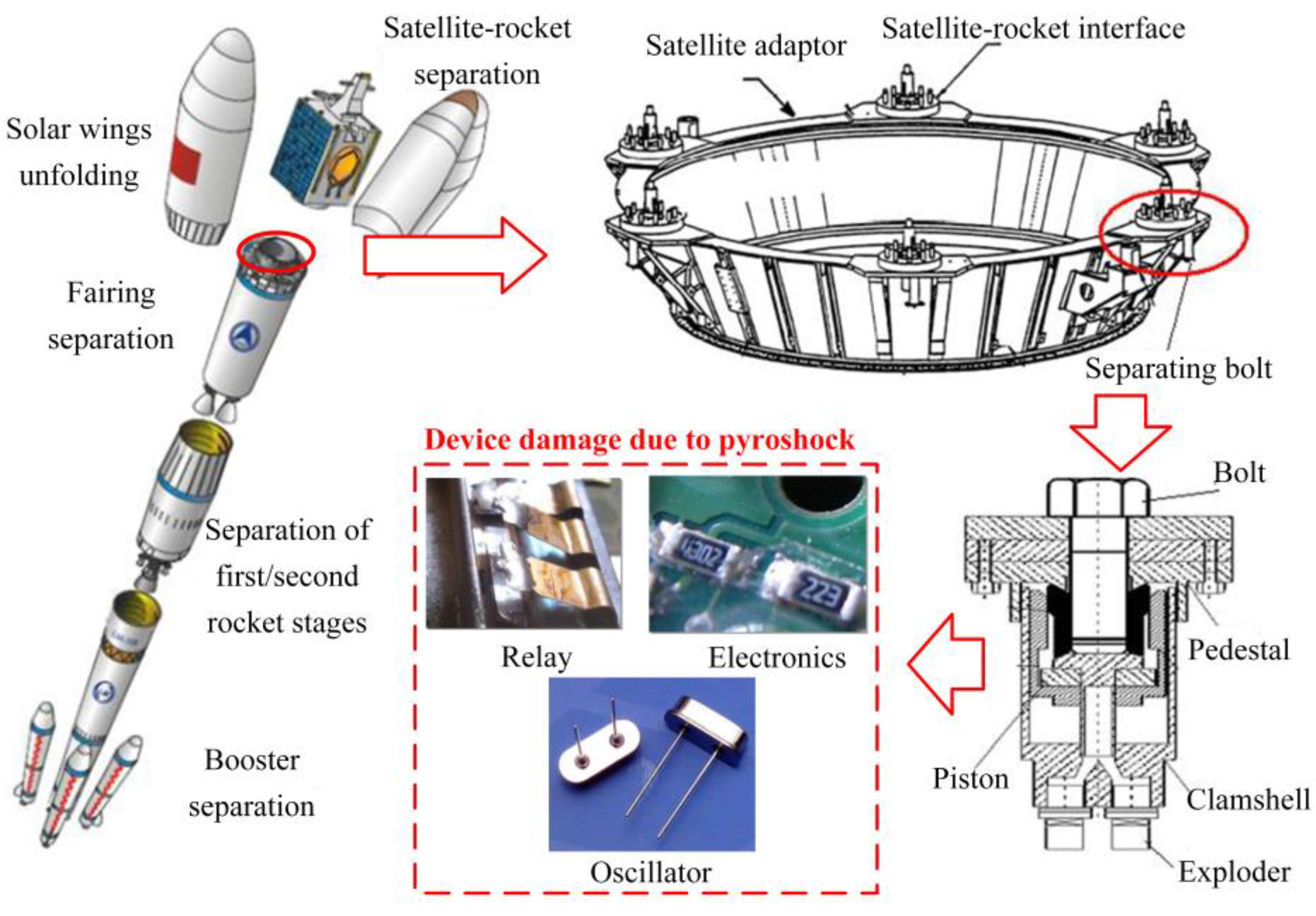
1. Introduction
2. Transmission Mechanism of Pyroshock
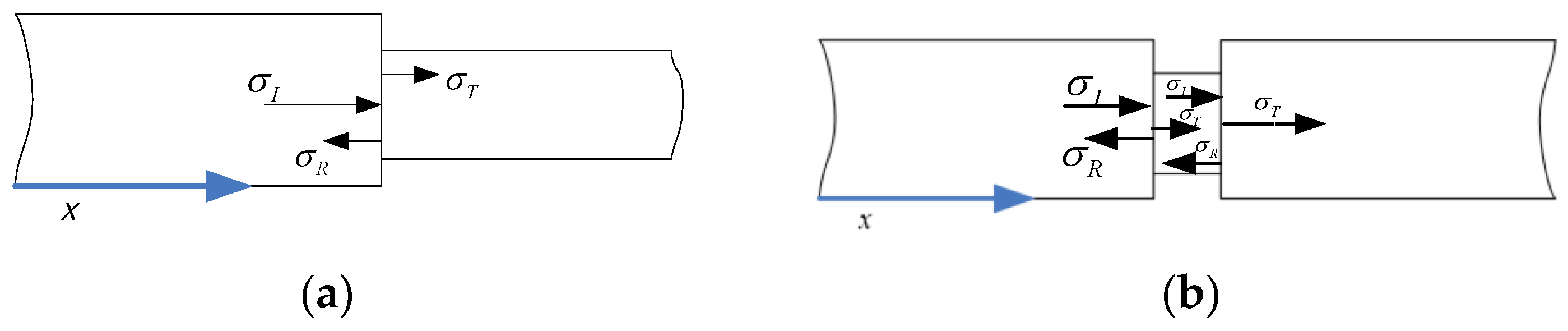
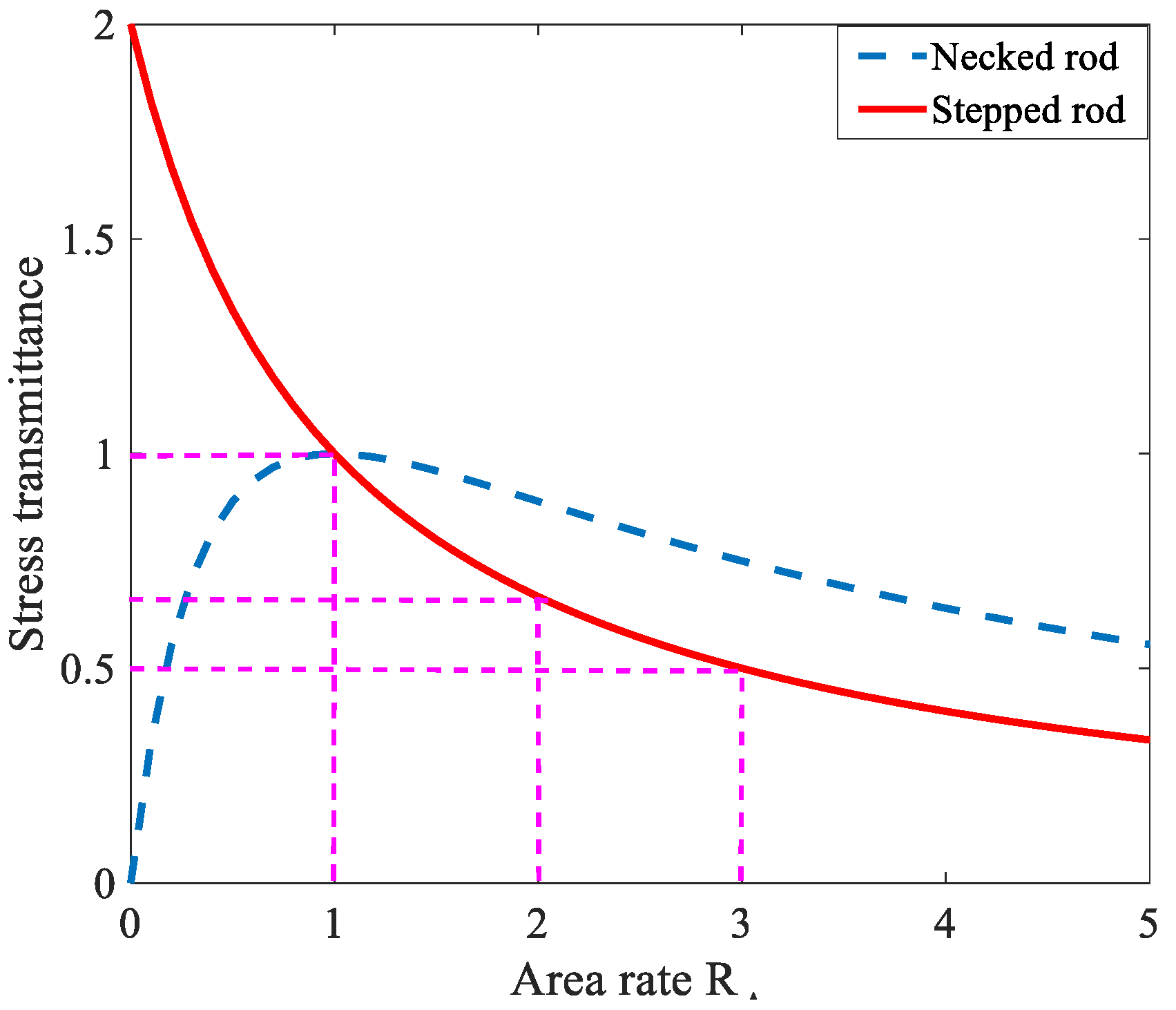
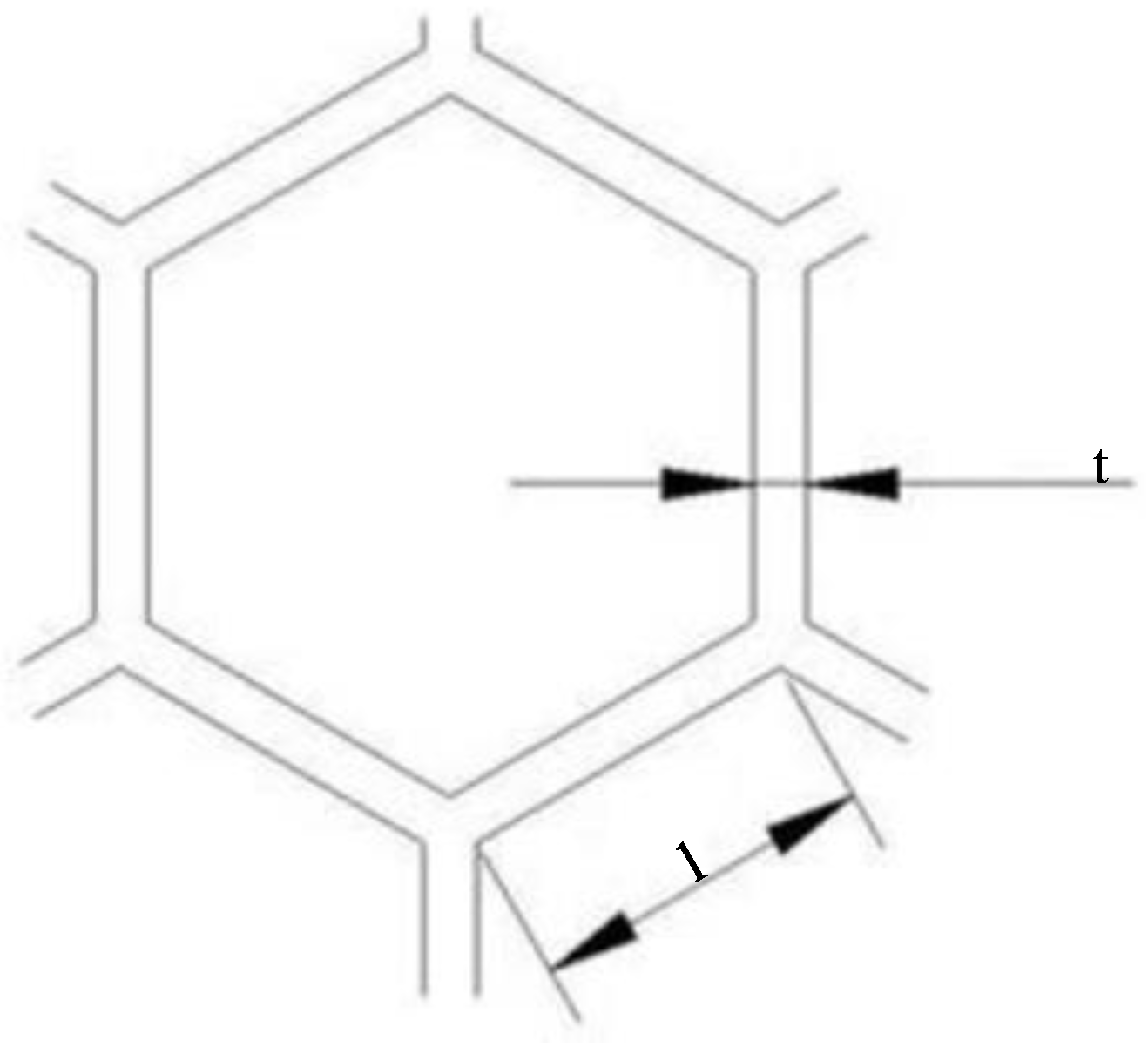
2.1. Theoretical Analysis of Honeycomb Structure
2.2. Pyroshock Isolation Strategies
3. Finite Element Analysis
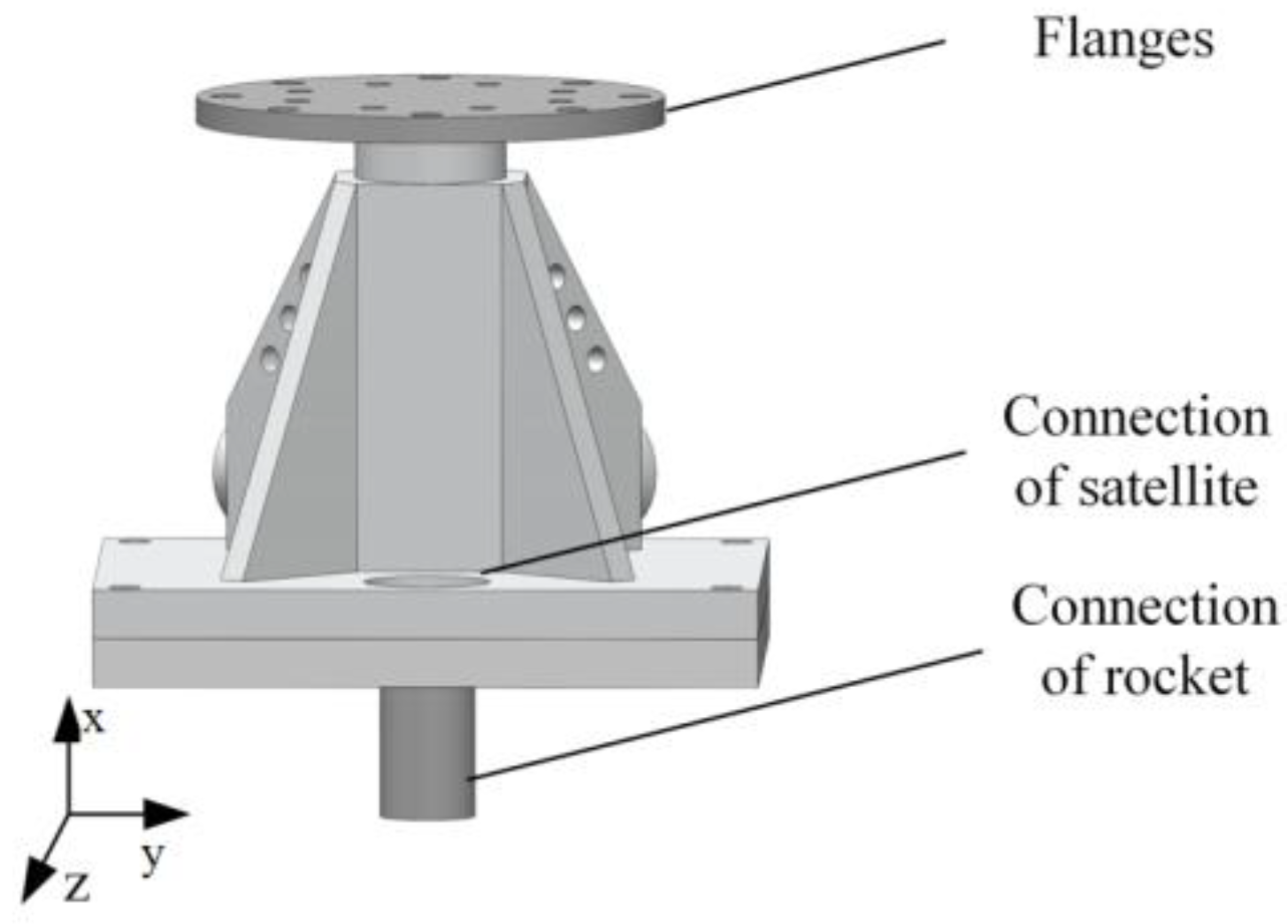
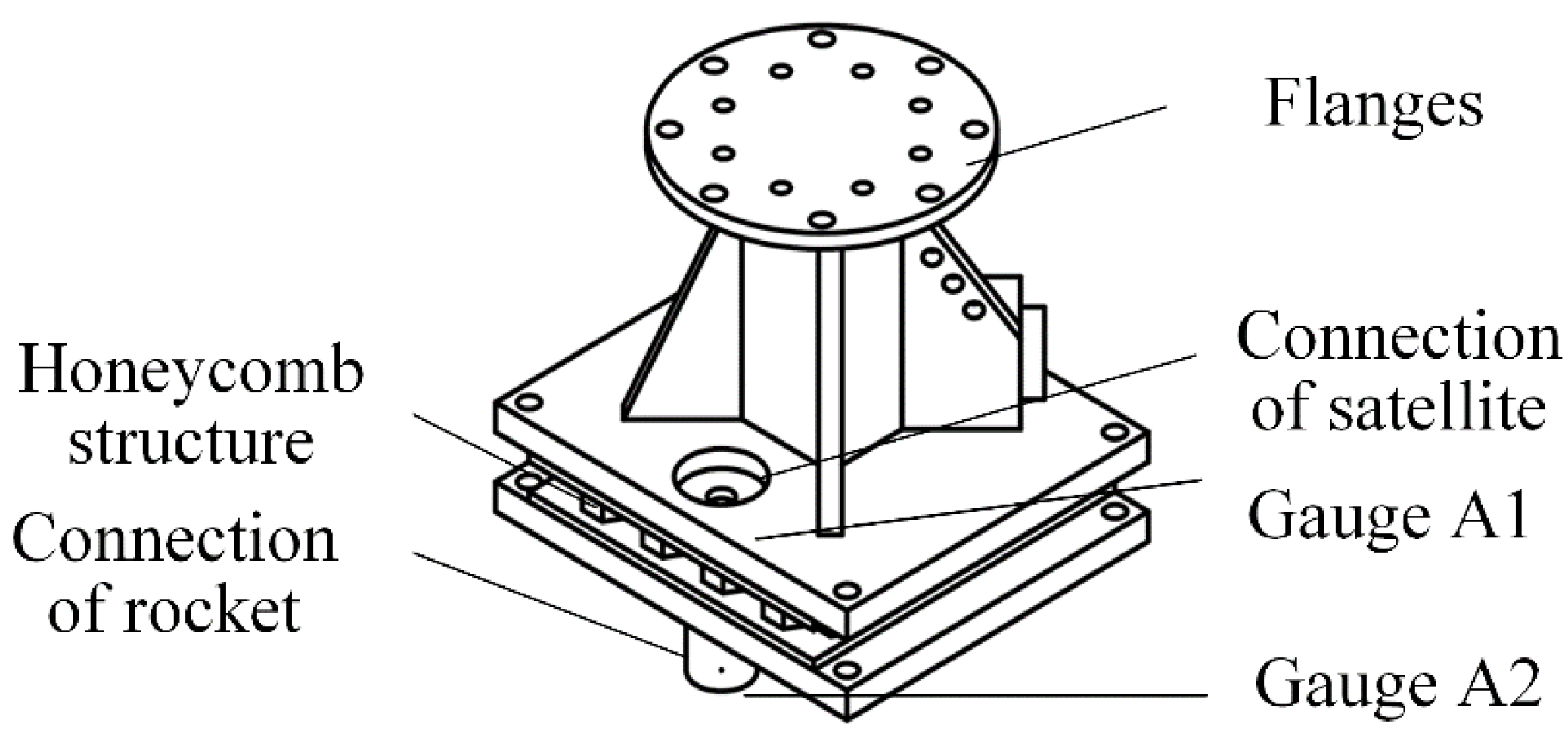
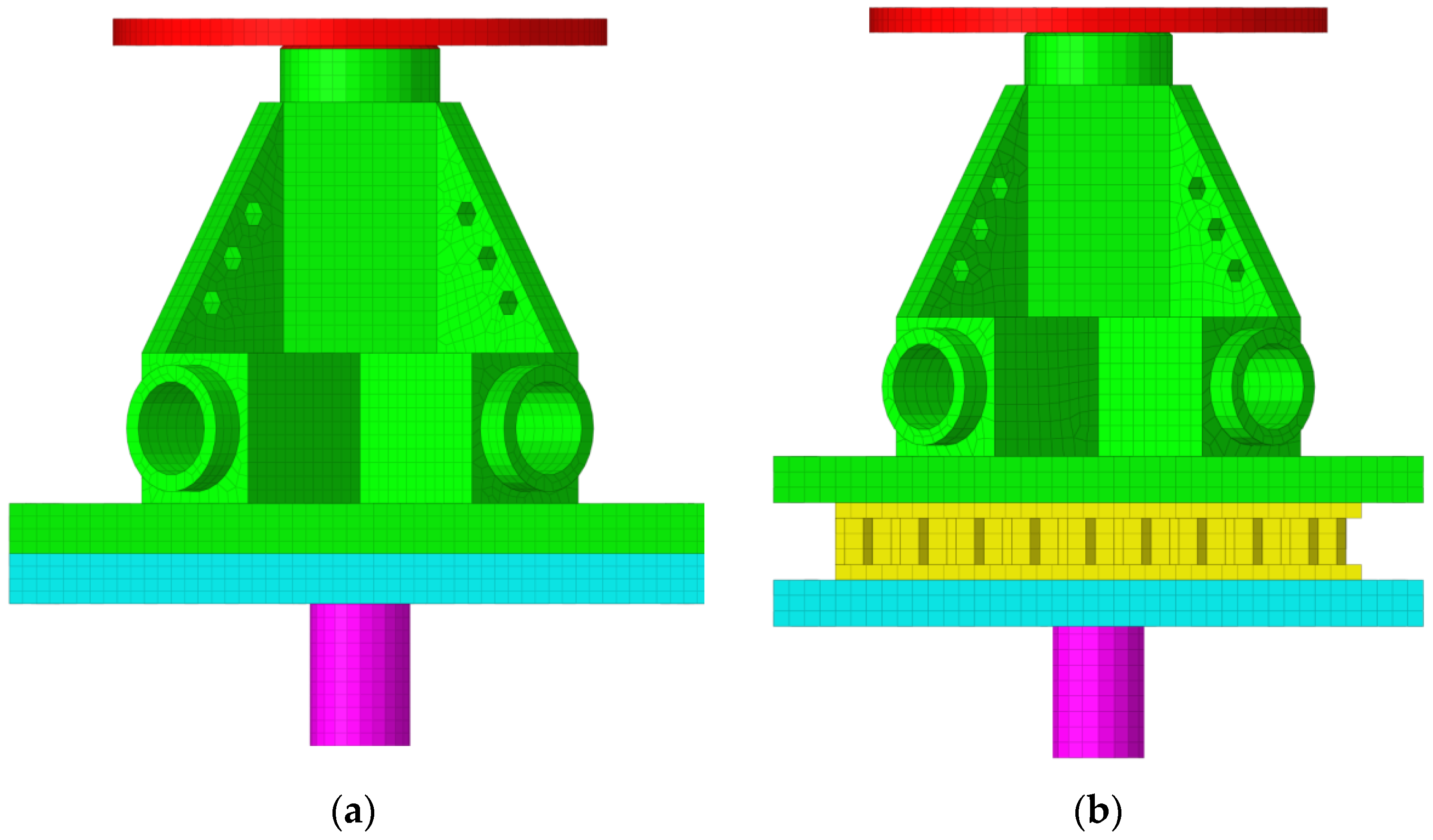
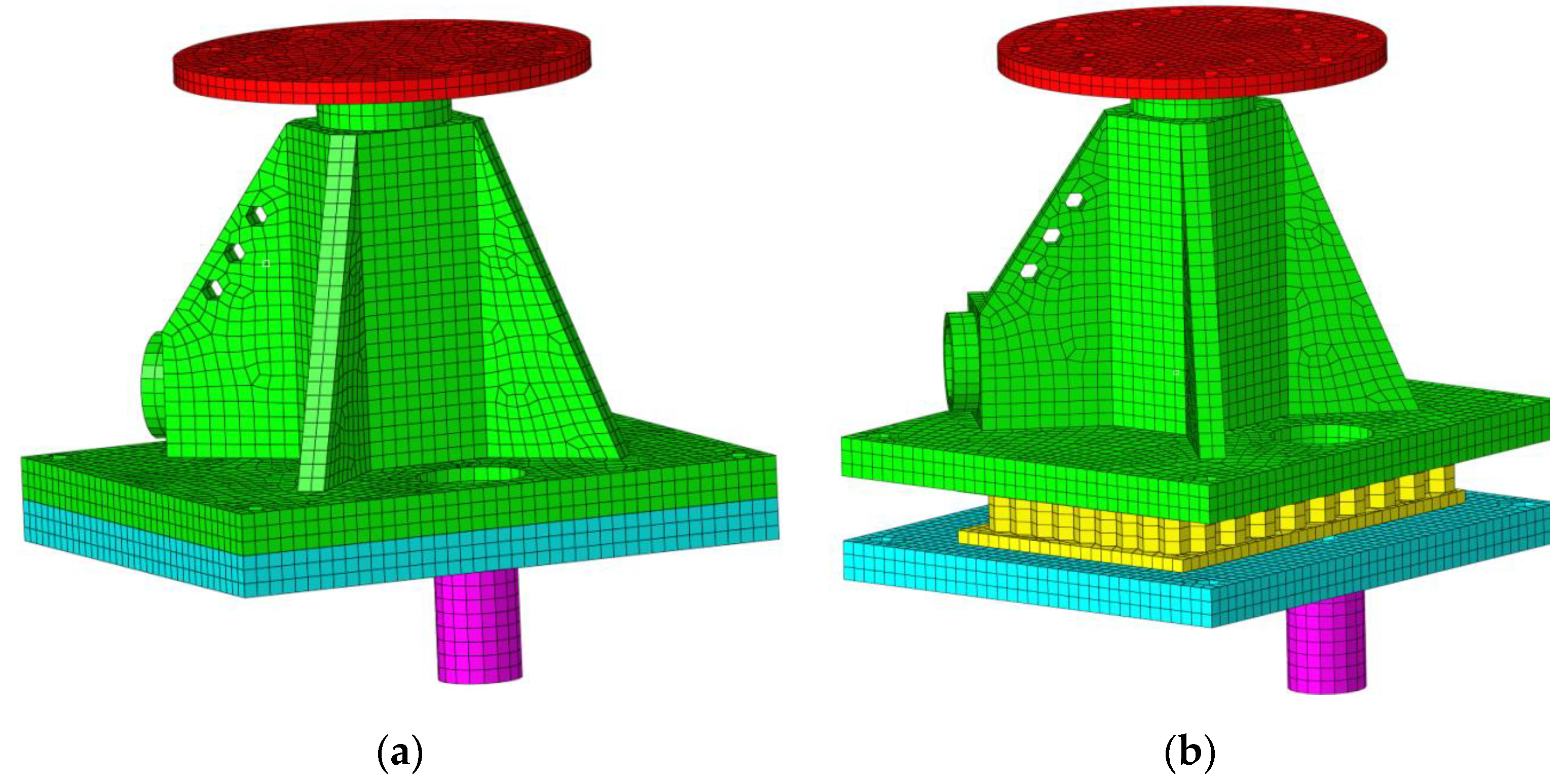
3.1. Model Description
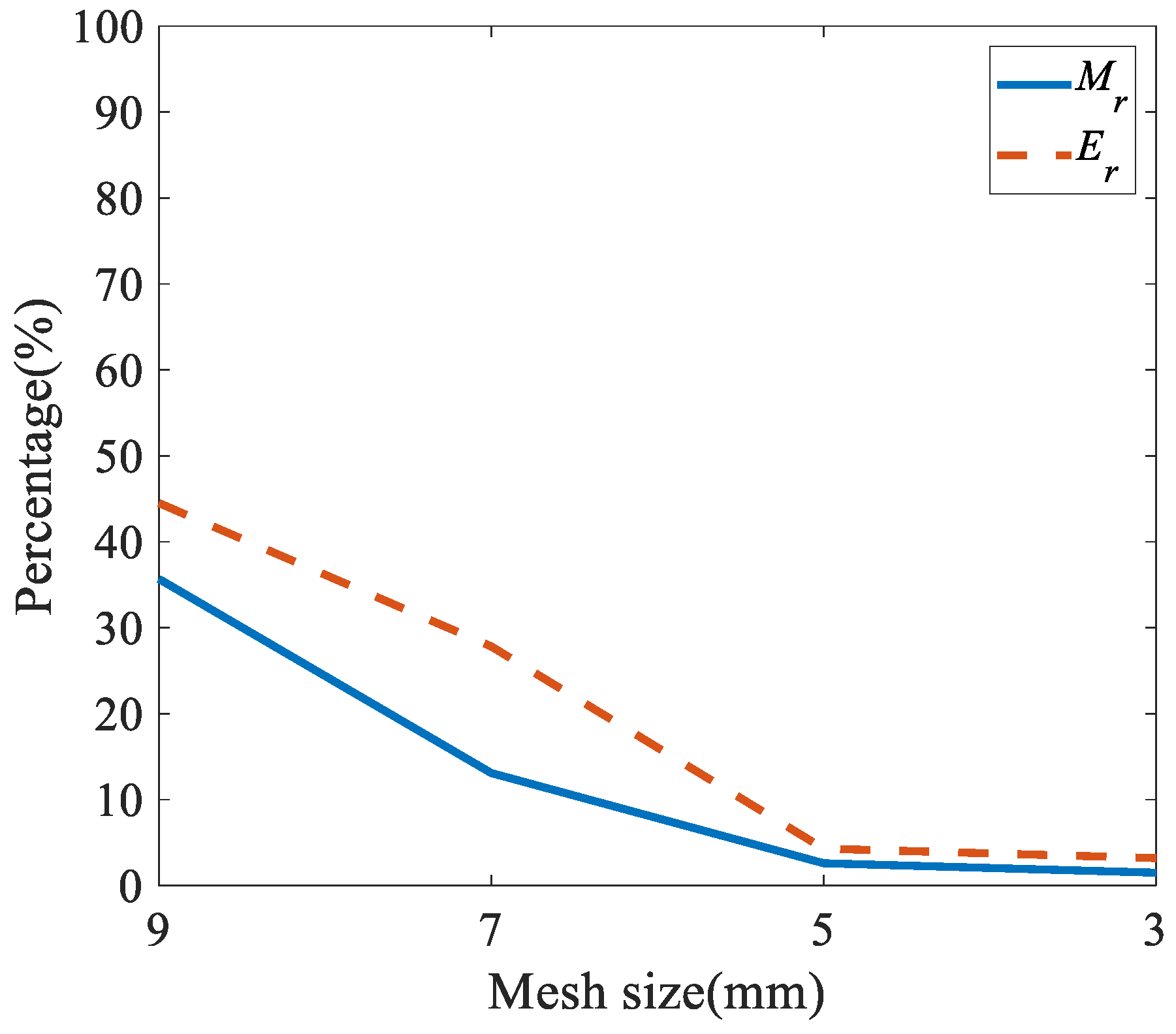
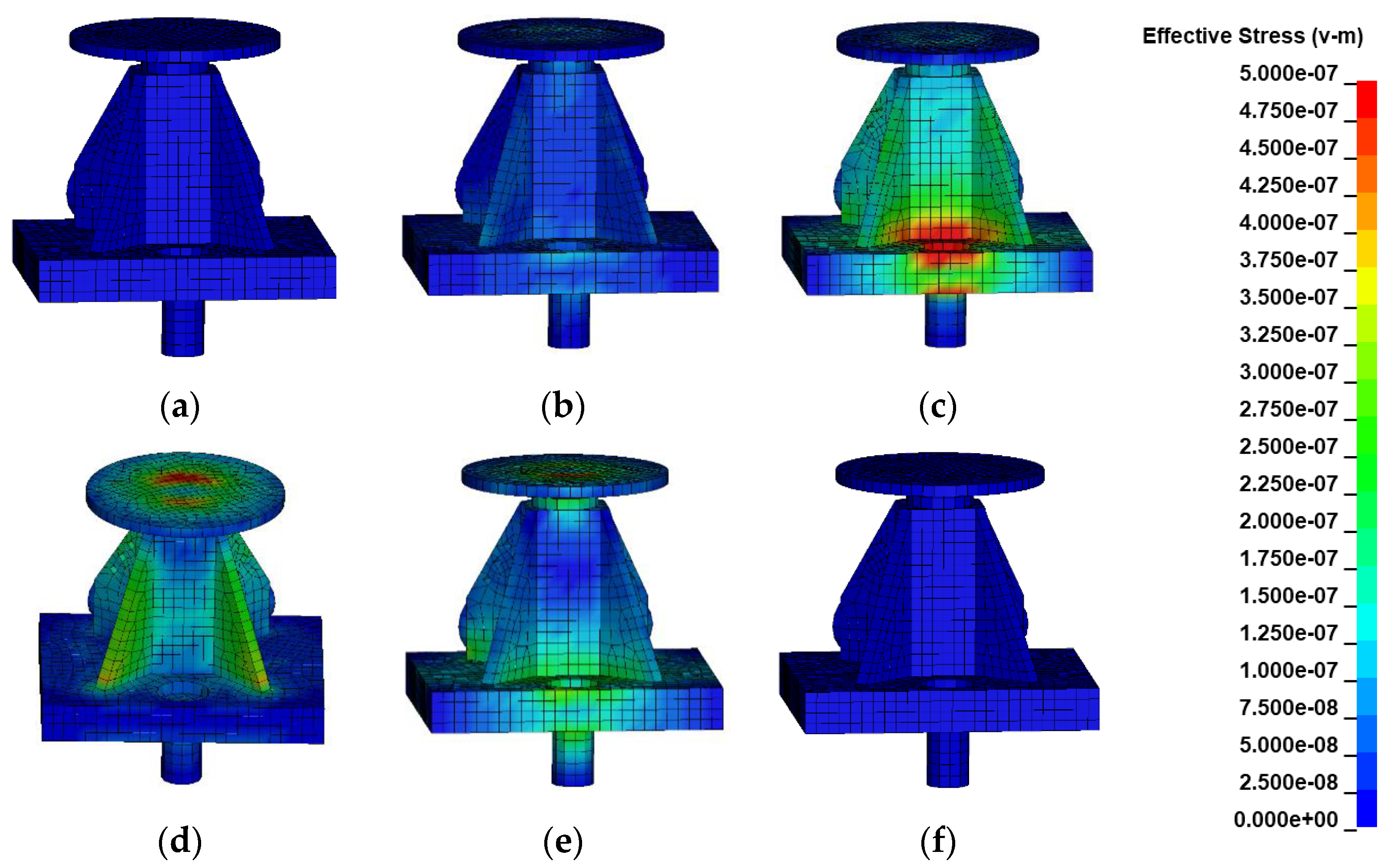
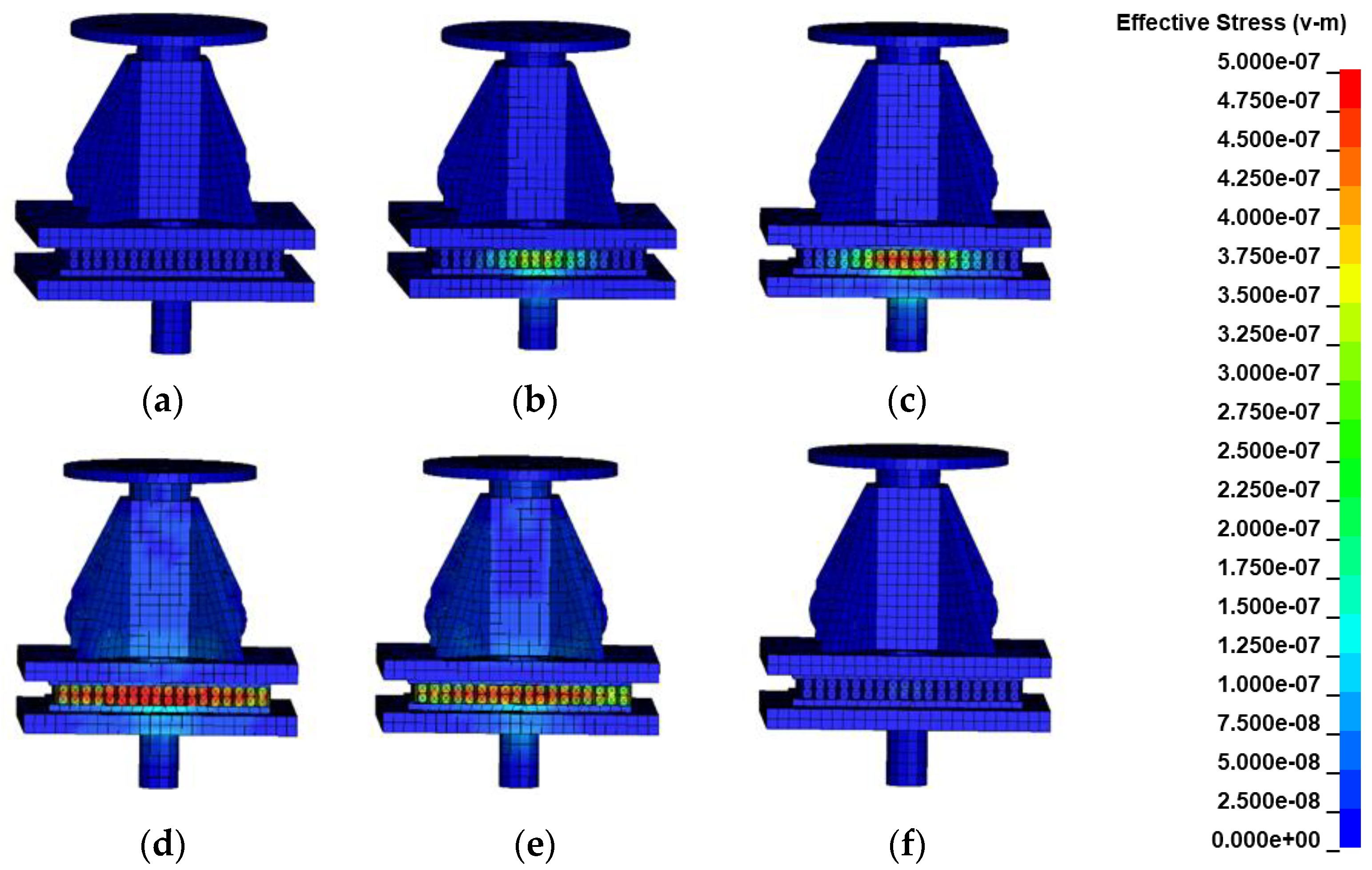
3.2. Simulation and Discussion
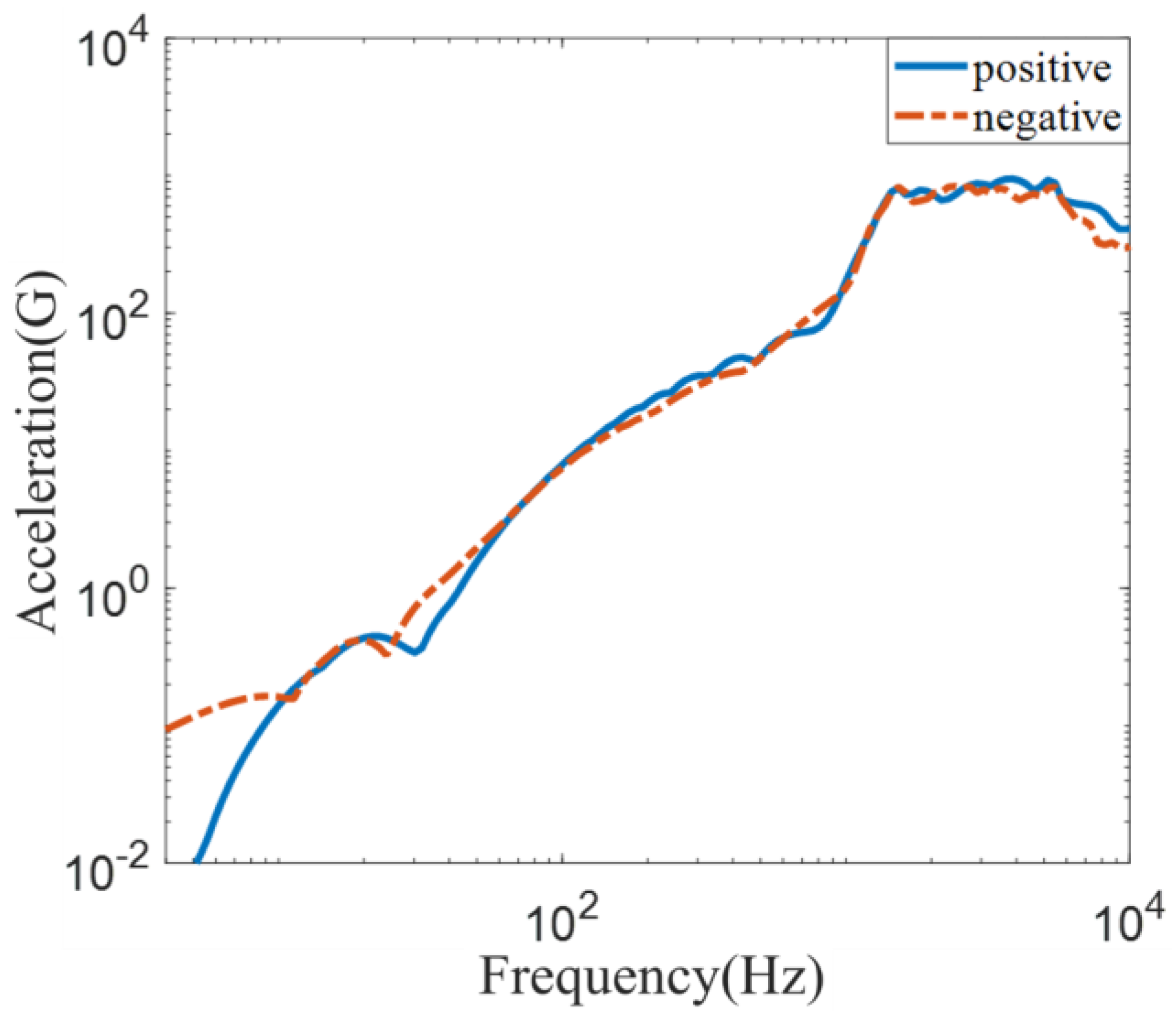
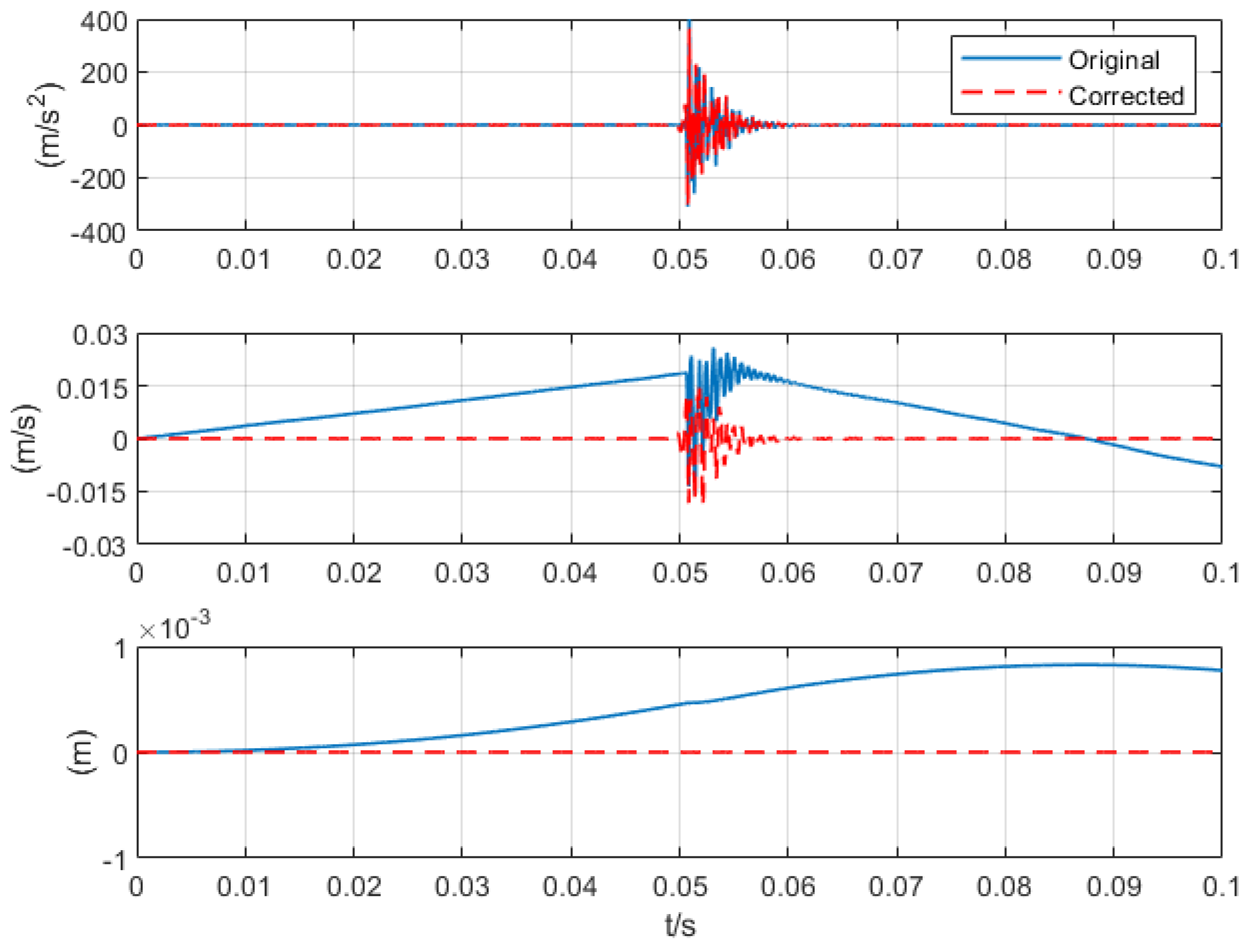
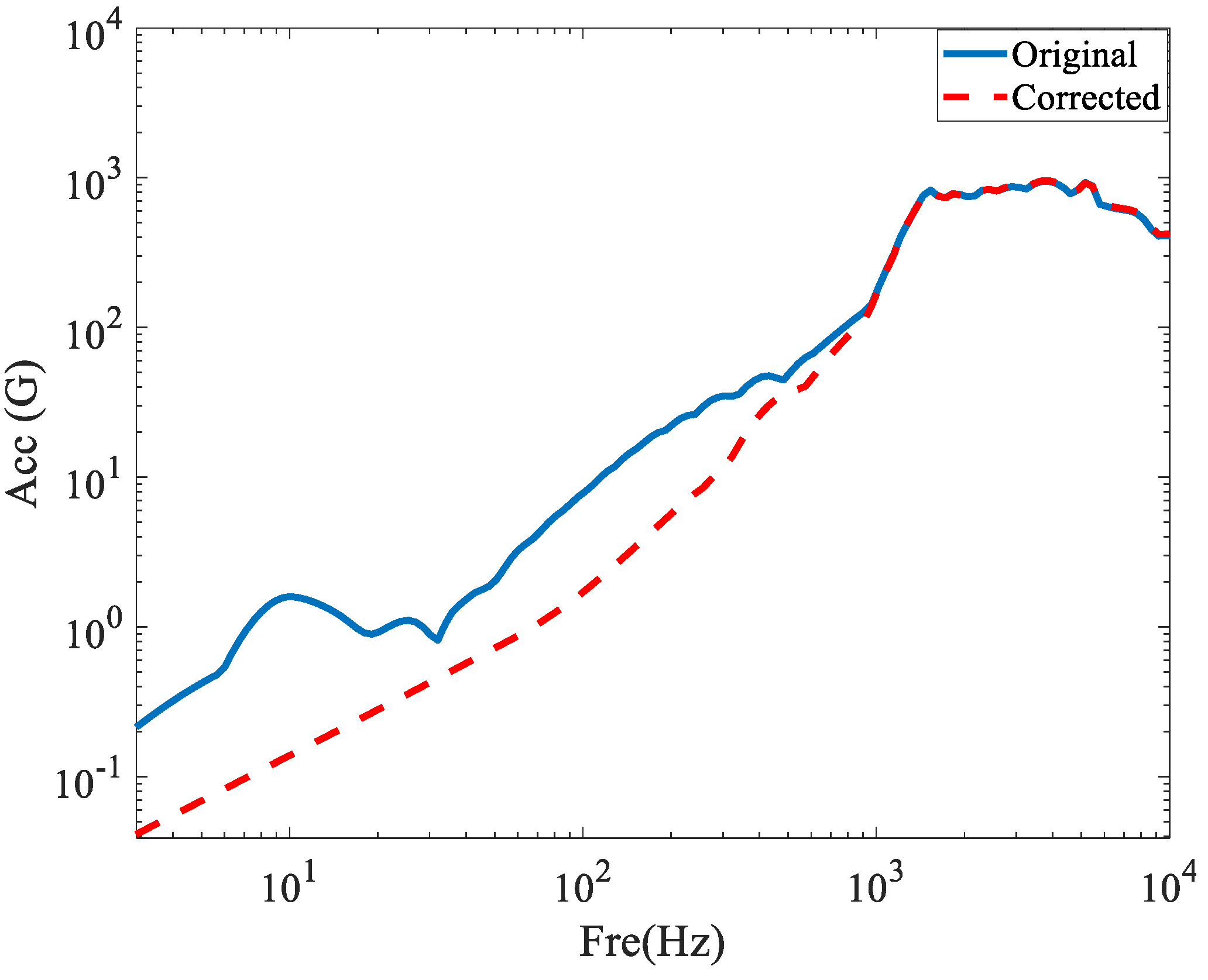
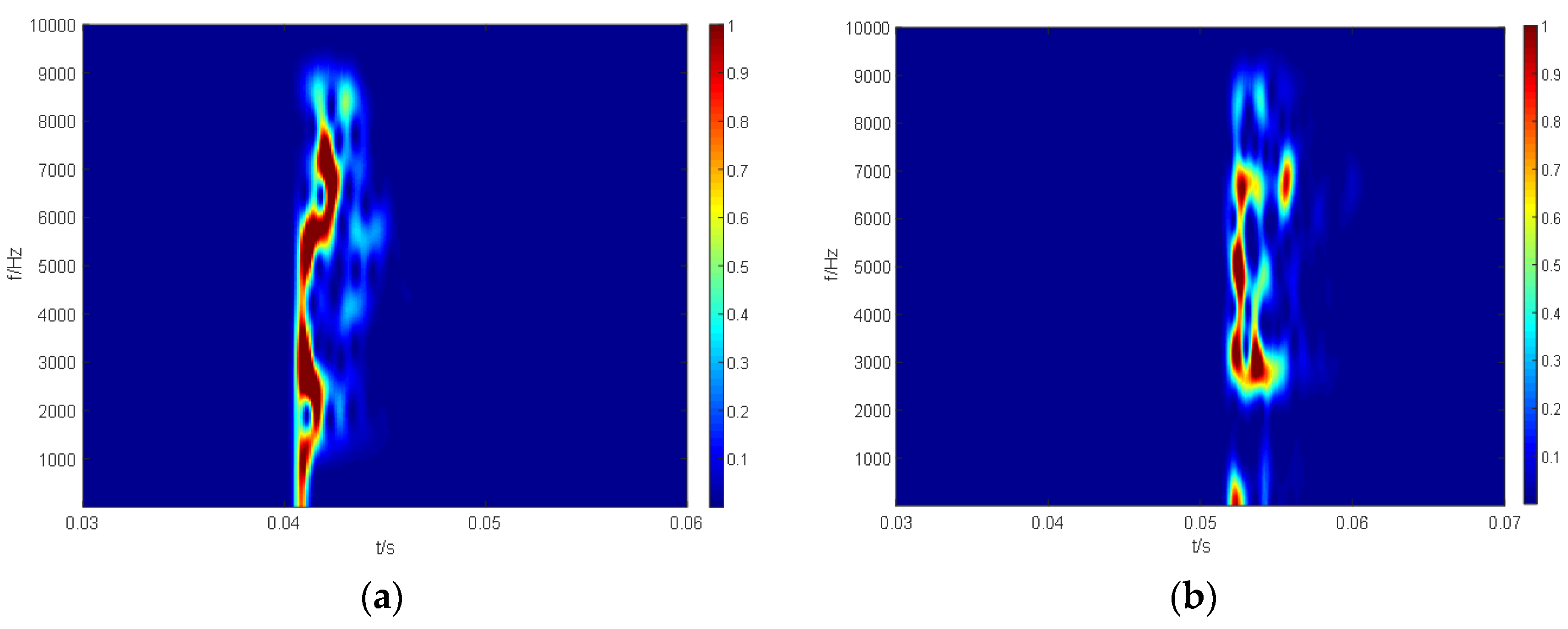
3.3. Experimental Setup
3.4. Experimental Results
4. Results and Discussion
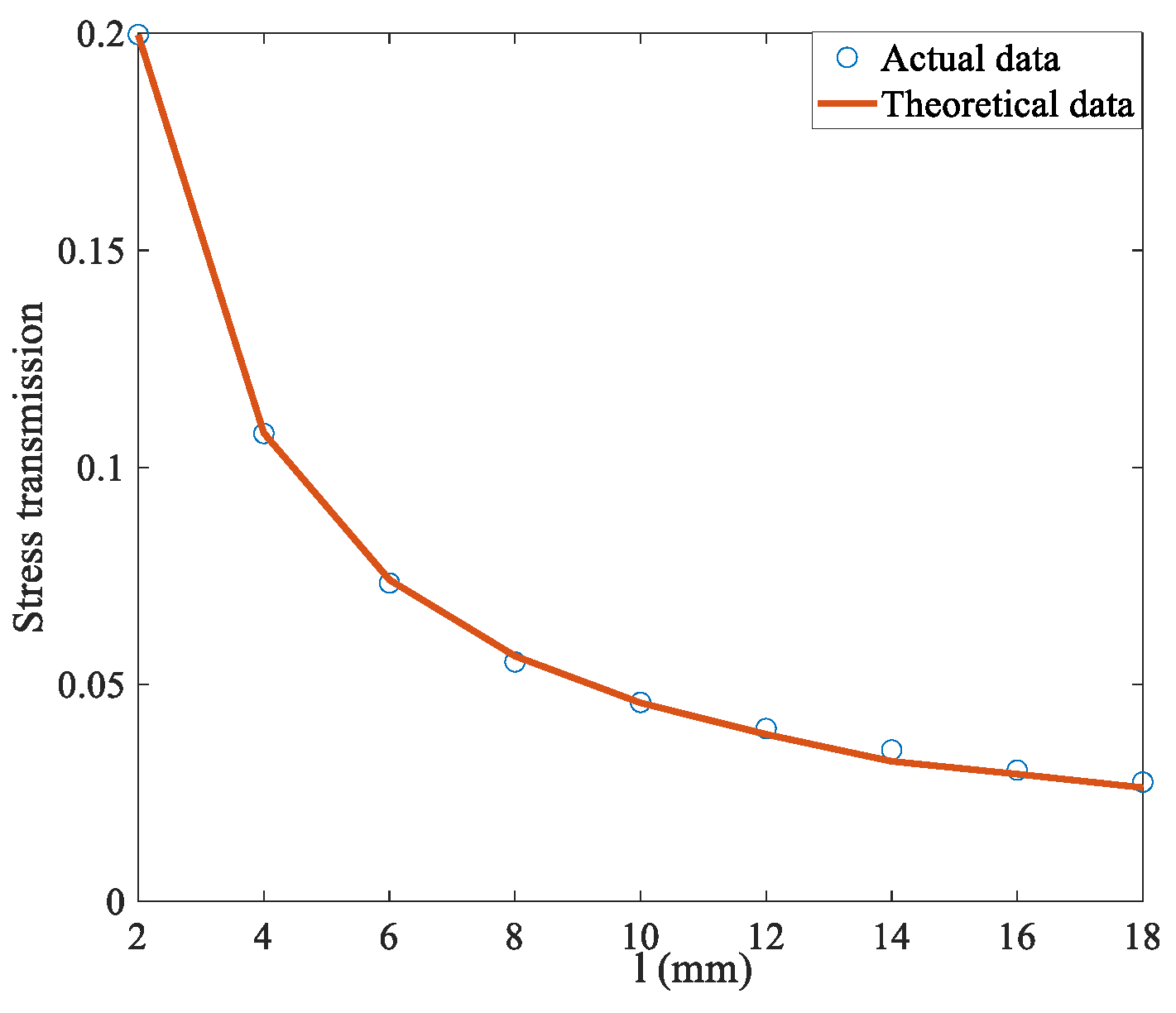
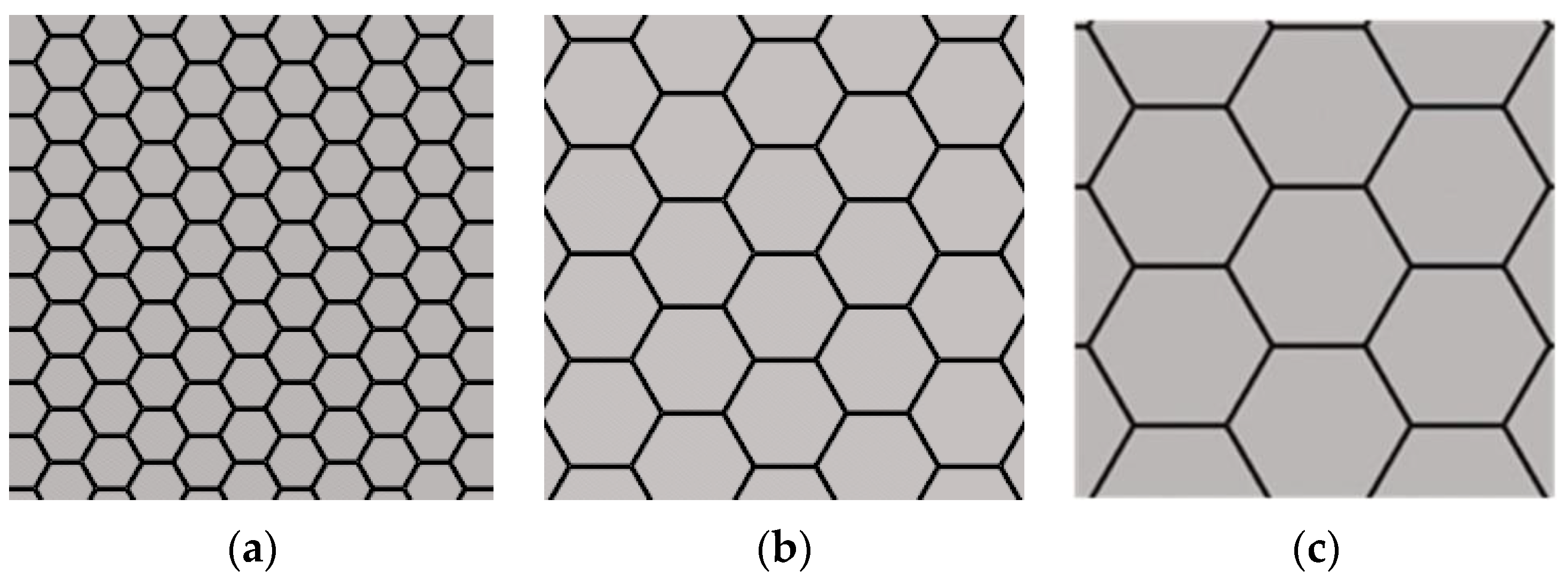
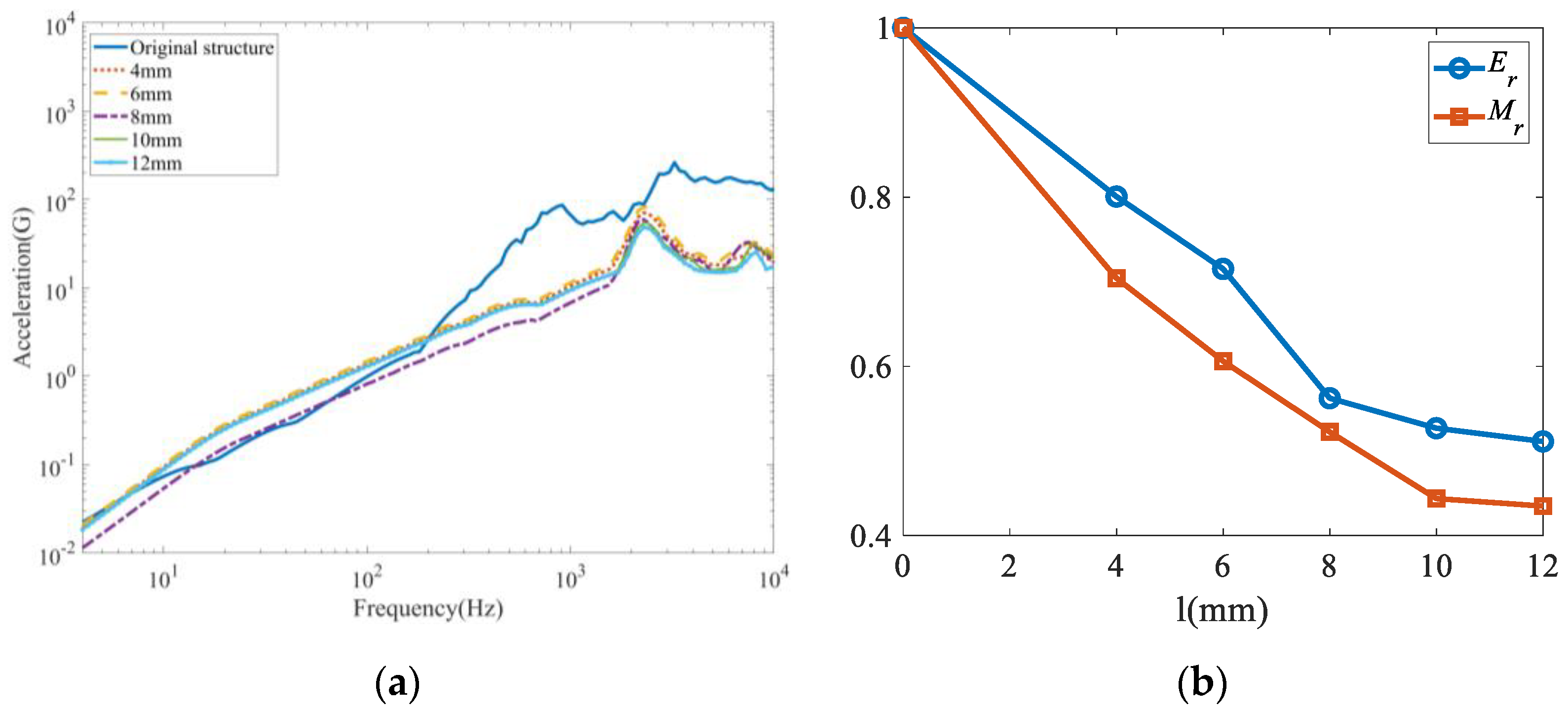
4.1. Effect of Honeycomb Cell Wall Length on Stress Propagation
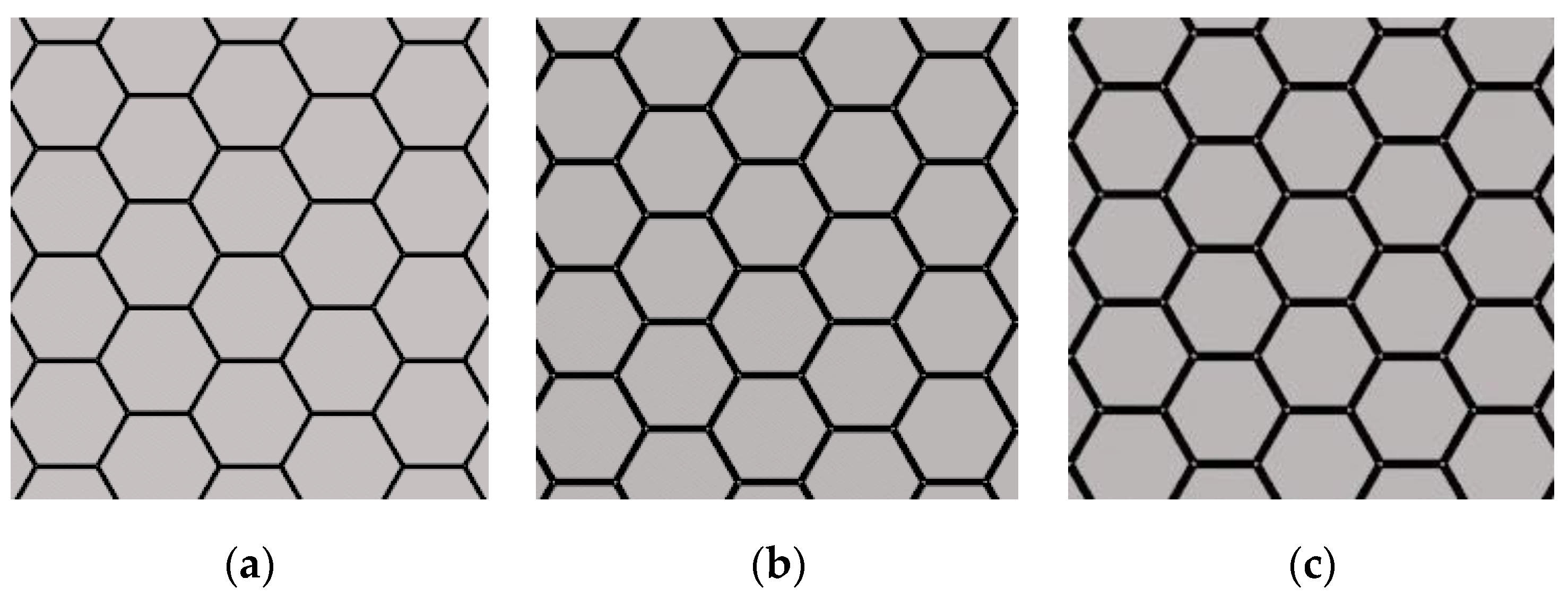
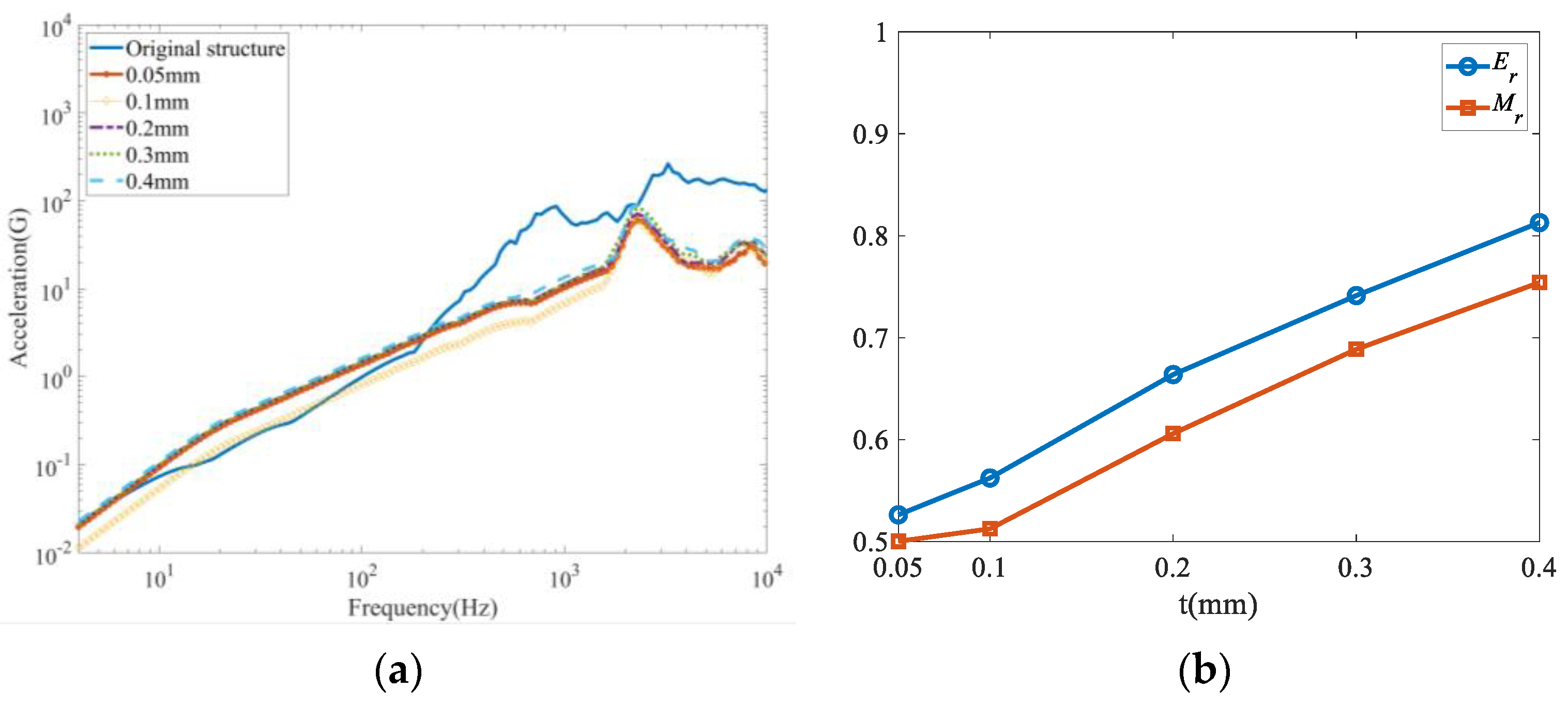
4.2. Effect of Honeycomb Cell Wall Thickness on Stress Propagation
5. Conclusions
- The new kind of spacecraft–rocket interface is conducive to achieving shock isolation, and the results of the FE model and the experimental results are in good agreement. The honeycomb structure can effectively reduce the propagation of stress waves, which can achieve a reduction in Mr of more than 65%.
- The SRS decreases with the increase in cell wall length thickness and the decrease in cell wall length, which indicates that small thickness and greater length is beneficial for shock isolation.
- Based on this study, the optimized geometric dimension of the honeycomb structure against Pyroshock is obtained when the cell wall length and cell wall thickness of the honeycomb are 8 mm and 0.1 mm, respectively.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Lee, J.R.; Chia, C.C.; Kong, C.W. Review of Pyroshock wave measurement and simulation for space systems. Measurement 2012, 45, 631–642. [Google Scholar] [CrossRef]
- Moening, C. Pyrotechnic shock flight failures. In Institute of Environmental Sciences Pyrotechnic Shock Tutorial Program, 31st Annual Technical Meeting; Citeseer: Las Vegas, NV, USA, 1985; pp. 4–5. [Google Scholar]
- Daniel, R. Pyroshock Test Criteria. NASA-STD-7003A; NASA Technical Standard; NASA: Washington, DC, USA, 2011. Available online: https://www.docin.com/p-369312963.html (accessed on 3 September 2024).
- Girard, A.; Pont, C.; Courau, E. Very first results from pyroshock database for satellites, Spacecraft Structures. In Proceedings of the Spacecraft Structures, Materials and Mechanical Testing 2005, Noordwijk, The Netherlands, 10–12 May 2005; p. 127. [Google Scholar]
- Smallwood, D.O. An Improved Recursive Formula for Calculating Shock Response Spectra; S.N. Laboratories: Albuquerque, NM, USA, 1981. [Google Scholar]
- Bateman, V.; Merritt, R. Validation of Pyroshock Data. J. IEST 2012, 55, 40–56. [Google Scholar] [CrossRef]
- Anderson, A.; Bait, J.; Bell, D. MIL-STD-810F-2000 Test Method Standard for Environmental Engineering Considerations and Laboratory Tests; The US Department of Defense: Washington, DC, USA, 2000.
- Yan, H.; Qin, Z.; Chu, F.; Zhang, W.; Yang, Z.; Ming, A.; Wang, X. Dynamic Response of Aluminum Honeycomb Panels to High-Frequency Laser Shock Excitations. J. Spacecr. Rocket. 2020, 57, 198–201. [Google Scholar] [CrossRef]
- Iwasa, T.; Shi, Q. Simplified Analysis Model for Predicting Pyroshock Responses on Composite Panel. J. Space Eng. 2008, 1, 79–90. [Google Scholar] [CrossRef]
- Velmurugan, R.; Najeeb, E.M. Study of Far-Field Pyroshock Responses of Composite Panels. J. Vib. Acoust.-Trans. ASME 2014, 136, 031014. [Google Scholar] [CrossRef]
- Ding, J.F. Shock isolation of satellite-rocket separation and its test verification. Struct. Environ. Eng. 2016, 43, 17–24. [Google Scholar]
- Park, H.S.; Hwang, D.H.; Han, J.H.; Yang, J.Y. Development of shock-absorbing insert for honeycomb sandwich panel. Aerosp. Sci. Technol. 2020, 104, 105930. [Google Scholar] [CrossRef]
- Ma, Y.; Zhang, Q.; Zhang, D.; Scarpa, F.; Liu, B.; Hong, J. The mechanics of shape memory alloy metal rubber. Acta Mater. 2015, 96, 89–100. [Google Scholar] [CrossRef]
- Lu, X.; Jiang, K.; Cheng, S.; Su, D.; Wang, H. Prediction and isolation of pyroshock in typical pyrotechnic device based on coupled modeling technique. Thin-Walled Struct. 2022, 177, 109393. [Google Scholar] [CrossRef]
- Ciepielewski, R.; Gieleta, R.; Miedzinska, D. Experimental Study on Static and Dynamic Response of Aluminum Honeycomb Sandwich Structures. Materials 2022, 15, 1793. [Google Scholar] [CrossRef] [PubMed]
- Norris, C.B. An Analysis of the Compressive Strength of Honeycomb Cores for Sandwich Construction; No. NACA-TN-1251; National Advisory Committee for Aeronautics: Washington, DC, USA, 1947. [Google Scholar]
- Gunes, R.; Arslan, K. Development of numerical realistic model for predicting low-velocity impact response of aluminium honeycomb sandwich structures. J. Sandw. Struct. Mater. 2016, 18, 95–112. [Google Scholar] [CrossRef]
- Venugopal, A.; Venugopal, P.; Thomas, B.G.; Koshy, C.P.; Varghese, J.T. Numerical Study on Aluminium 5056 Alloy Hexagonal Honeycomb Cells as a Possible Material for Energy Absorption During Vehicle Crash. Trans. Indian Inst. Met. 2023, 76, 2515–2524. [Google Scholar] [CrossRef]
- Qi, C.; Jiang, F.; Yang, S. Advanced honeycomb designs for improving mechanical properties: A review. Compos. Part B-Eng. 2021, 227, 109393. [Google Scholar] [CrossRef]
- Lin, Y.L.; Zhang, Z.F.; Chen, R.; Li, Y.; Wen, X.J.; Lu, F.Y. Cushioning and Energy Absorbing Property of Combined Aluminum Honeycomb. Adv. Eng. Mater. 2015, 17, 1434–1441. [Google Scholar] [CrossRef]
- Najeeb, E.M.; Velmurugan, R. Effect of fiber orientations of composite panels under far-field Pyroshock. Polym. Compos. 2019, 40, 255–262. [Google Scholar] [CrossRef]
- NASA-HDBK-7005; Dynamic Enviromental Criteria. Jet Propulsion Laboratory, National Aeronautics and Space Administration: Pasadena, CA, USA, 2017. Available online: https://www.abbottaerospace.com/downloads/nasa-hdbk-7005/ (accessed on 3 September 2024).
- Mao, Y.J.; Yue, X.H.; Huang, H.Y.; Niu, B.L.; Huang, H.J. Experimental study on Pyroshock responses of a conical shell. Appl. Mech. Mater. 2010, 20, 1458–1462. [Google Scholar] [CrossRef]
- Bement, L.J.; Schimmel, M.L. A Manual for Pyrotechnic Design, Development and Qualification; NASA Technical Reports Server: Hampton, VA, USA, 1995.
- Harris, C.M. Harris’ Shock and Vibration Handbook; McGraw-Hill: New York, NY, USA, 2017. [Google Scholar]
- Lyu, X.; Sheng, H.; He, M.; Ding, Q.; Tang, L.; Yang, T. Satellite vibration isolation using periodic acoustic black hole structures with ultrawide bandgap. J. Vib. Acoust. 2023, 145, 014501. [Google Scholar] [CrossRef]
- Lee, J.; Han, J.H.; Lee, Y.; Lee, H. Separation characteristics study of ridge-cut explosive bolts. Aerosp. Sci. Technol. 2014, 39, 153–168. [Google Scholar] [CrossRef]
- IEST-RP-DTE032; IEST Design, Test, and Evaluation Division (WG-DTE032). Pyroshock Testing Techniques. 2. Recommended Practice for Pyroshock Testing. ANSI: Washington, DC, USA, 2009. Available online: https://webstore.ansi.org/standards/iest/iestrpdte032 (accessed on 3 September 2024).
- MIL-STD-810G; Environmental Test Methods and Engineering Guides. United States Military Standard: Washington, DC, USA, 2009.
- Thomson, W. Theory of Vibration with Applications; CRC Press: Boca Raton, FL, USA, 1996. [Google Scholar]
- Qin, Z.Y.; Yan, S.Z.; Chu, F.L. Analytical modeling of clamp band joint under external bending moment. Aerosp. Sci. Technol. 2013, 25, 45–55. [Google Scholar] [CrossRef]







| Parameters | AISI1045 | ASTM201 | Units |
|---|---|---|---|
| Density (ρ) | 7.8 | 7.8 | g/cm3 |
| Elasticity moduls (E) | 210 | 190 | GPa |
| Poisson ratio (μ) | 0.33 | 0.305 | |
| Yield stress (σY) | 0.355 | 0.269 | GPa |
| Tangential moduls | 21 | 19 | GPa |
| Parameters | Symbol | Value | Units |
|---|---|---|---|
| Height of connection | Hj | 145 | mm |
| Length of interface | Lj | 210 | mm |
| Width of interface | Wj | 180 | mm |
| Thickness of interface | Tj | 15 | mm |
| Diameter of interface | ∅j | 45 | mm |
| Interface area | Ao | 37,800 | mm2 |
| Thickness of the honeycomb cell wall | T | 0.8 | mm |
| Length of the honeycomb cell wall | L | 8 | mm |
| Heihgt of the honeycomb cell wall | H | 15 | mm |
| Thickness of the honeycomb damping insert | Th | 139 | mm |
| Length of the honeycomb damping insert | Lh | 169 | mm |
| Heihgt of the honeycomb damping insert | Hh | 240 | mm |
| Parameters | Value | Units |
|---|---|---|
| Sensitivity ± 10% | 0.5 | mV/g |
| Sampling Frequency | 20 | kHz |
| Maximum Range | 10,000 | g |
| Mounted Resonant Frequency | >90 | kHz |
| Frequency Response, ±5% | 0.49–10,000 | Hz |
| Frequency Response, ±10% | 0.33–20,000 | Hz |
| Frequency Response, ±3 db | 0.16–50,000 | Hz |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, X.; Gao, Z.; Cheng, D.; Deng, X.; Yu, T.; Qin, Z.; Chu, F. Research on a Honeycomb Structure for Pyroshock Isolation at the Spacecraft–Rocket Interface. Aerospace 2024, 11, 756. https://doi.org/10.3390/aerospace11090756
Wang X, Gao Z, Cheng D, Deng X, Yu T, Qin Z, Chu F. Research on a Honeycomb Structure for Pyroshock Isolation at the Spacecraft–Rocket Interface. Aerospace. 2024; 11(9):756. https://doi.org/10.3390/aerospace11090756
Chicago/Turabian StyleWang, Xixiong, Zhibo Gao, Dong Cheng, Xuchen Deng, Tao Yu, Zhaoye Qin, and Fulei Chu. 2024. "Research on a Honeycomb Structure for Pyroshock Isolation at the Spacecraft–Rocket Interface" Aerospace 11, no. 9: 756. https://doi.org/10.3390/aerospace11090756
APA StyleWang, X., Gao, Z., Cheng, D., Deng, X., Yu, T., Qin, Z., & Chu, F. (2024). Research on a Honeycomb Structure for Pyroshock Isolation at the Spacecraft–Rocket Interface. Aerospace, 11(9), 756. https://doi.org/10.3390/aerospace11090756






