Heat Transfer Model Based on Flow Pattern during Flow Boiling in Rectangular Microchannels
Abstract
1. Introduction
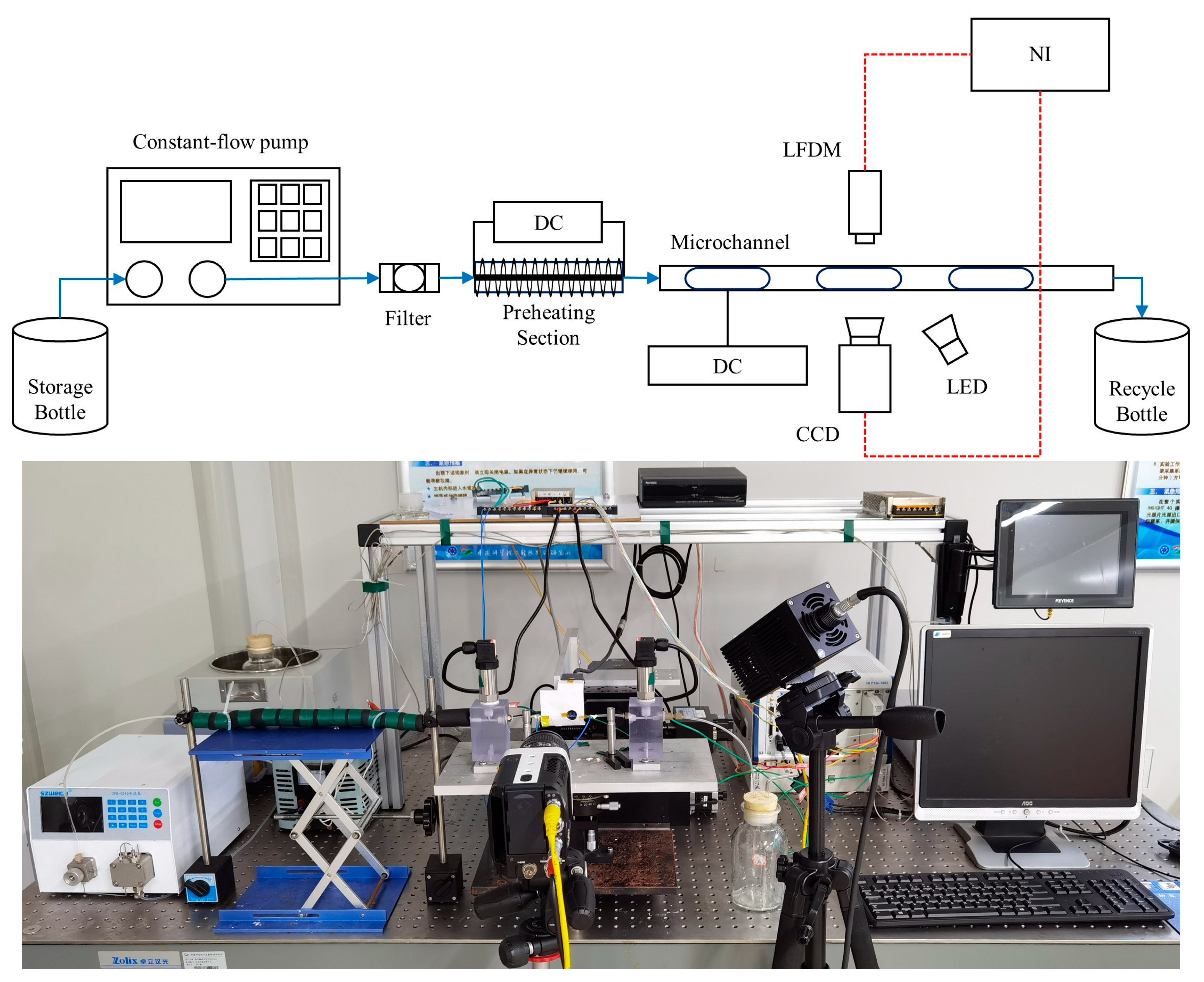
2. Experimental System and Procedure
2.1. Experimental System
2.2. Experimental Procedure
2.3. Experimental Data Reduction
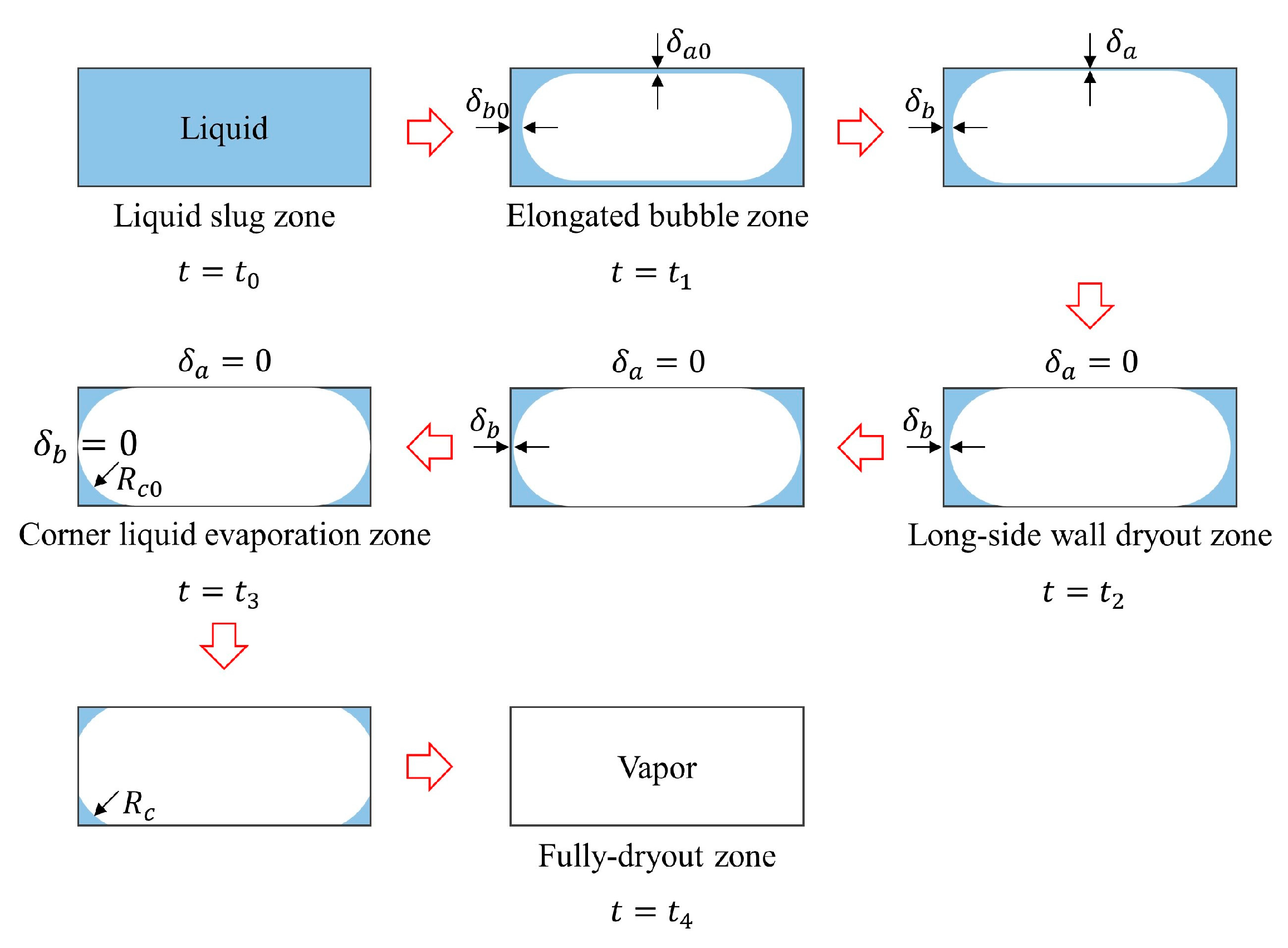
3. The Five-Zone Flow Boiling Model
3.1. Characterization of Five-Zone Flow Boiling Model
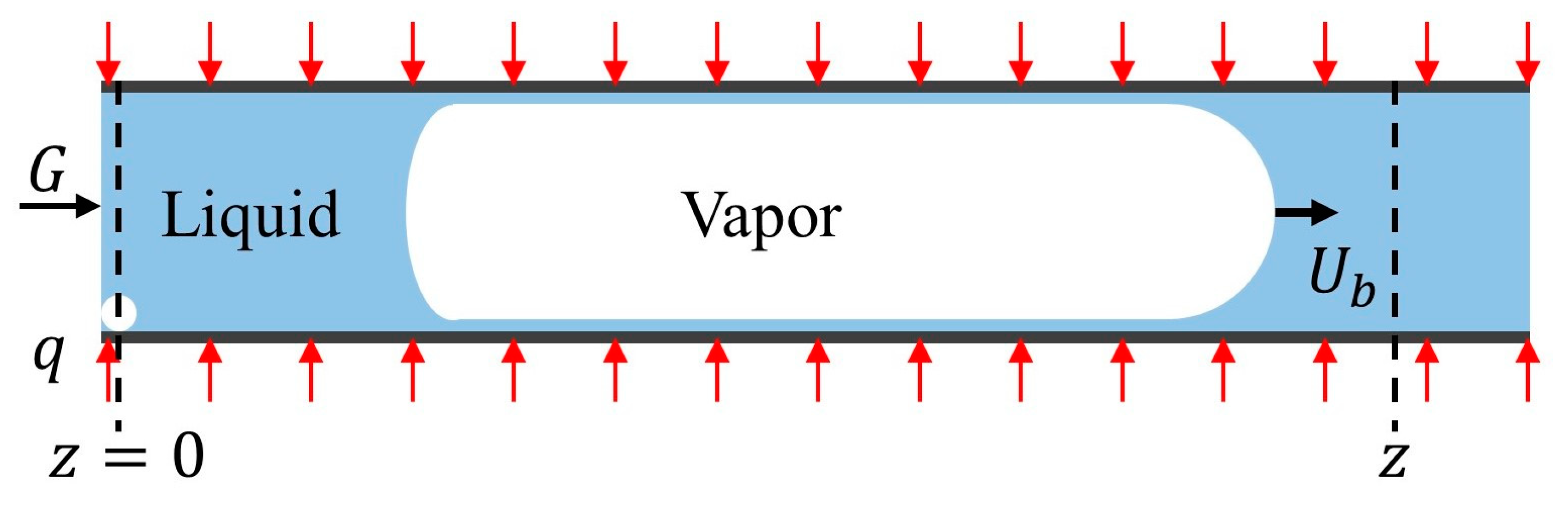
3.2. Assumptions
3.3. Initial Conditions and Basic Parameter Calculation
3.4. Five Zone Heat Transfer Coefficient Calculation
3.4.1. Heat Transfer Coefficients in the Liquid Slug Zone and Full Dryout Zone
3.4.2. Heat Transfer Coefficient in the Elongated Bubble Zone
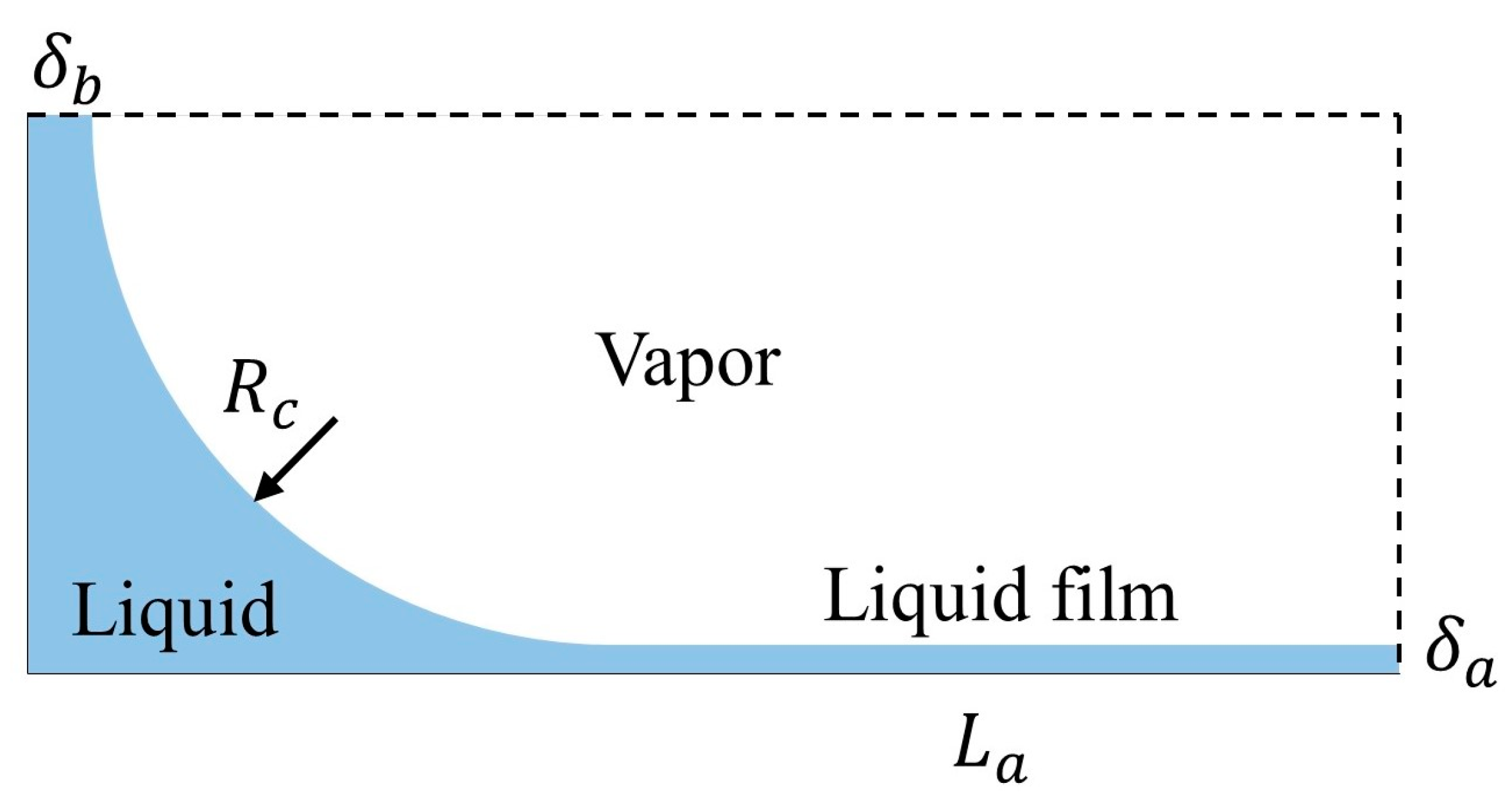
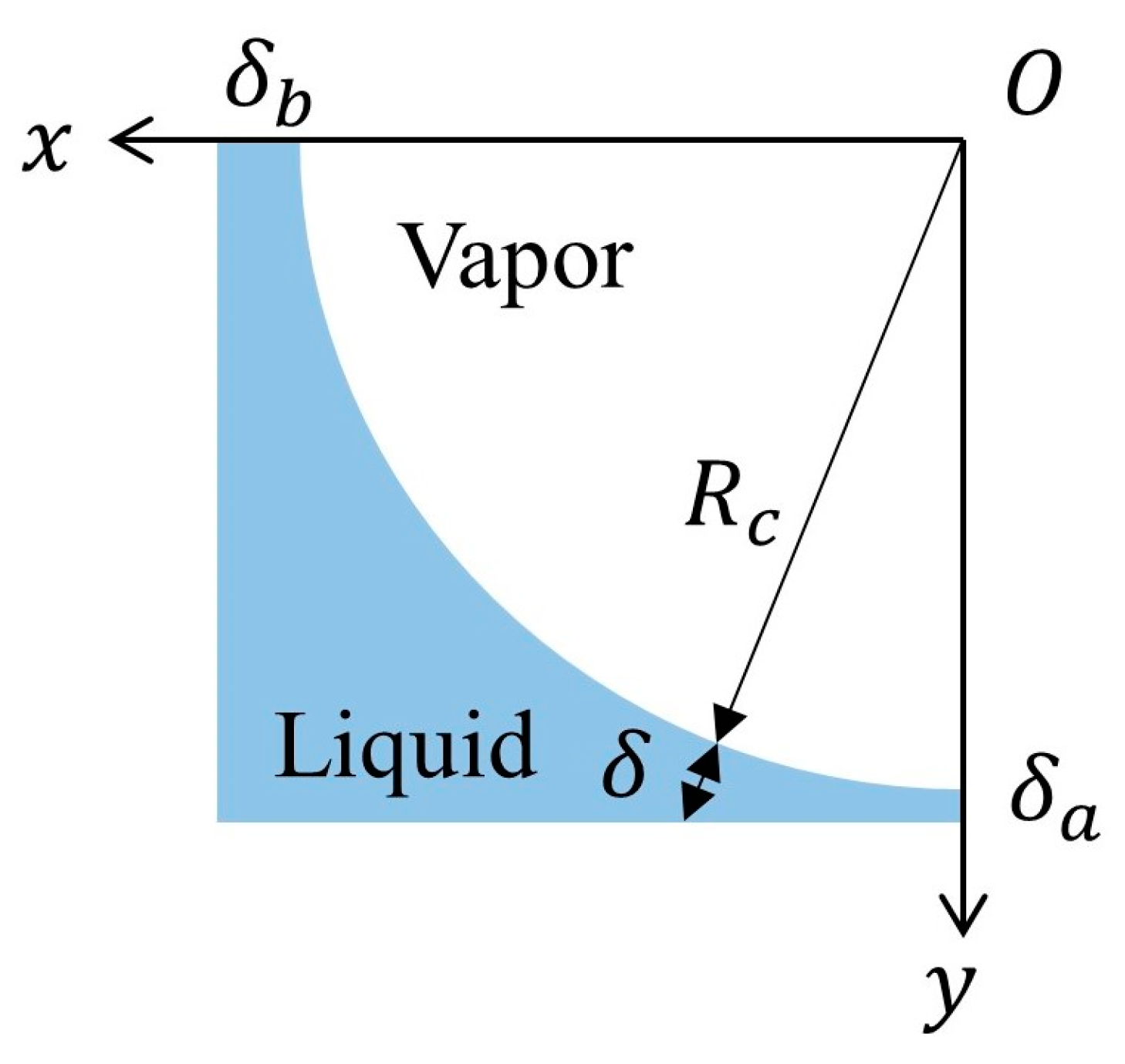
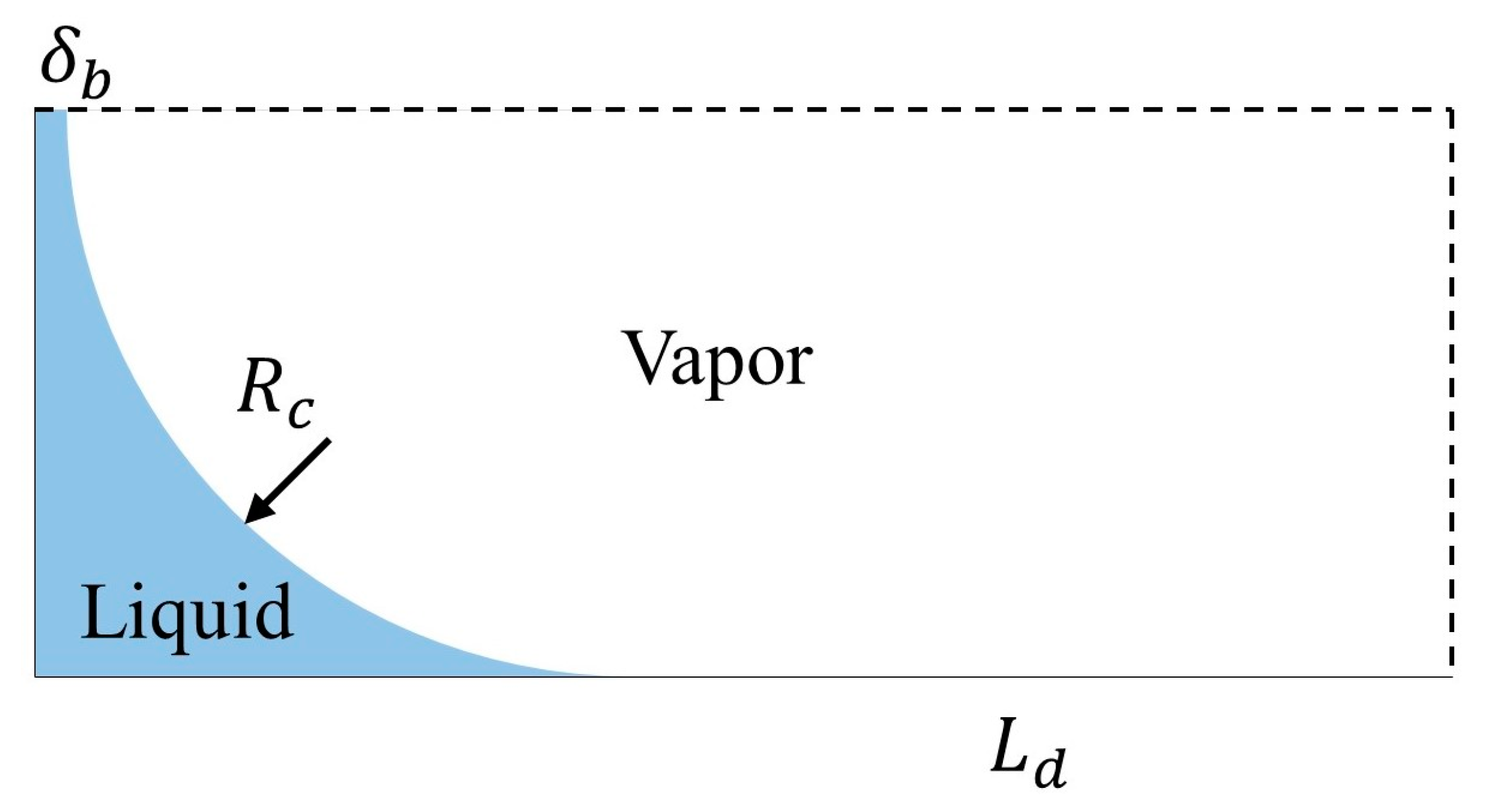
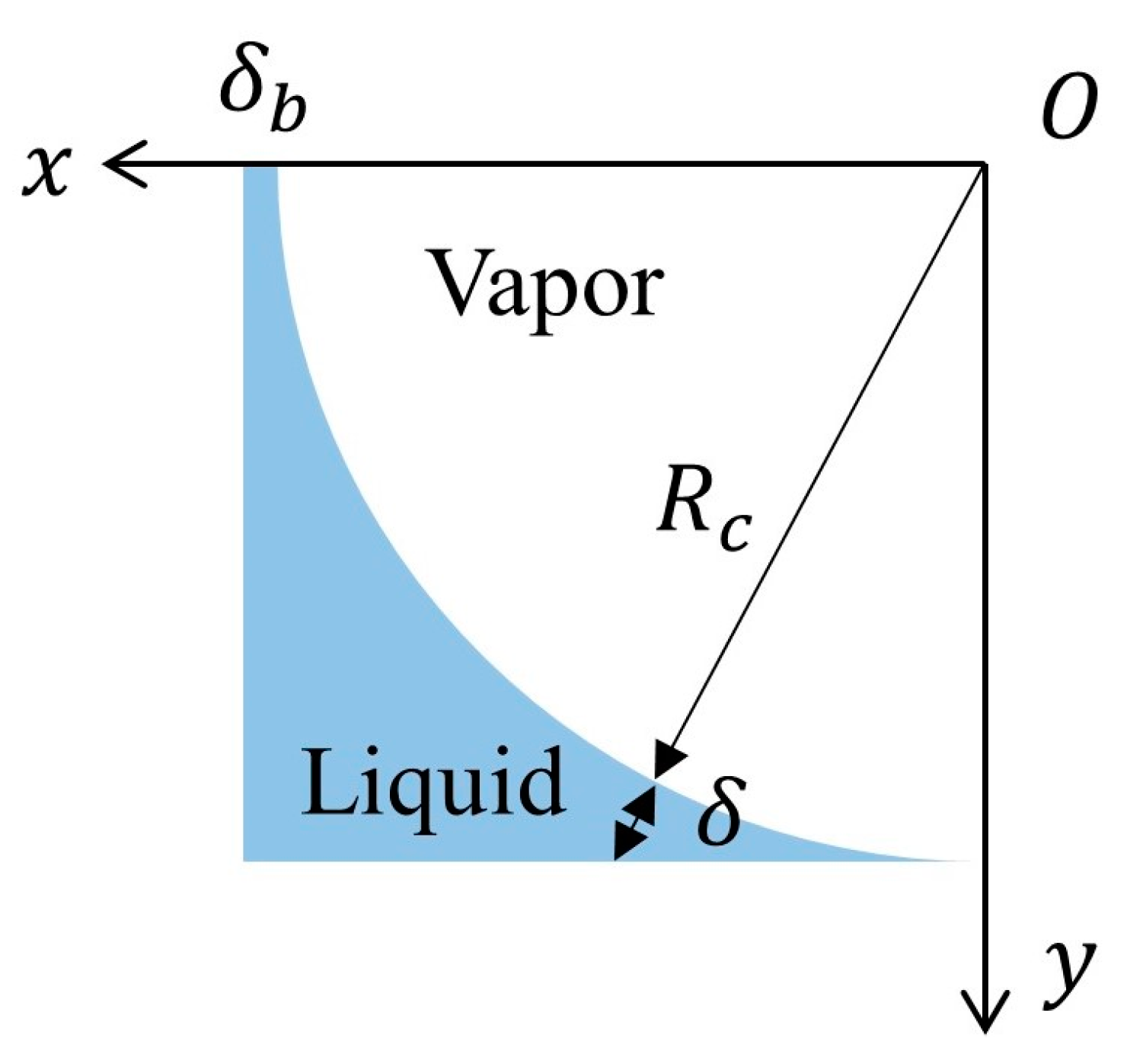
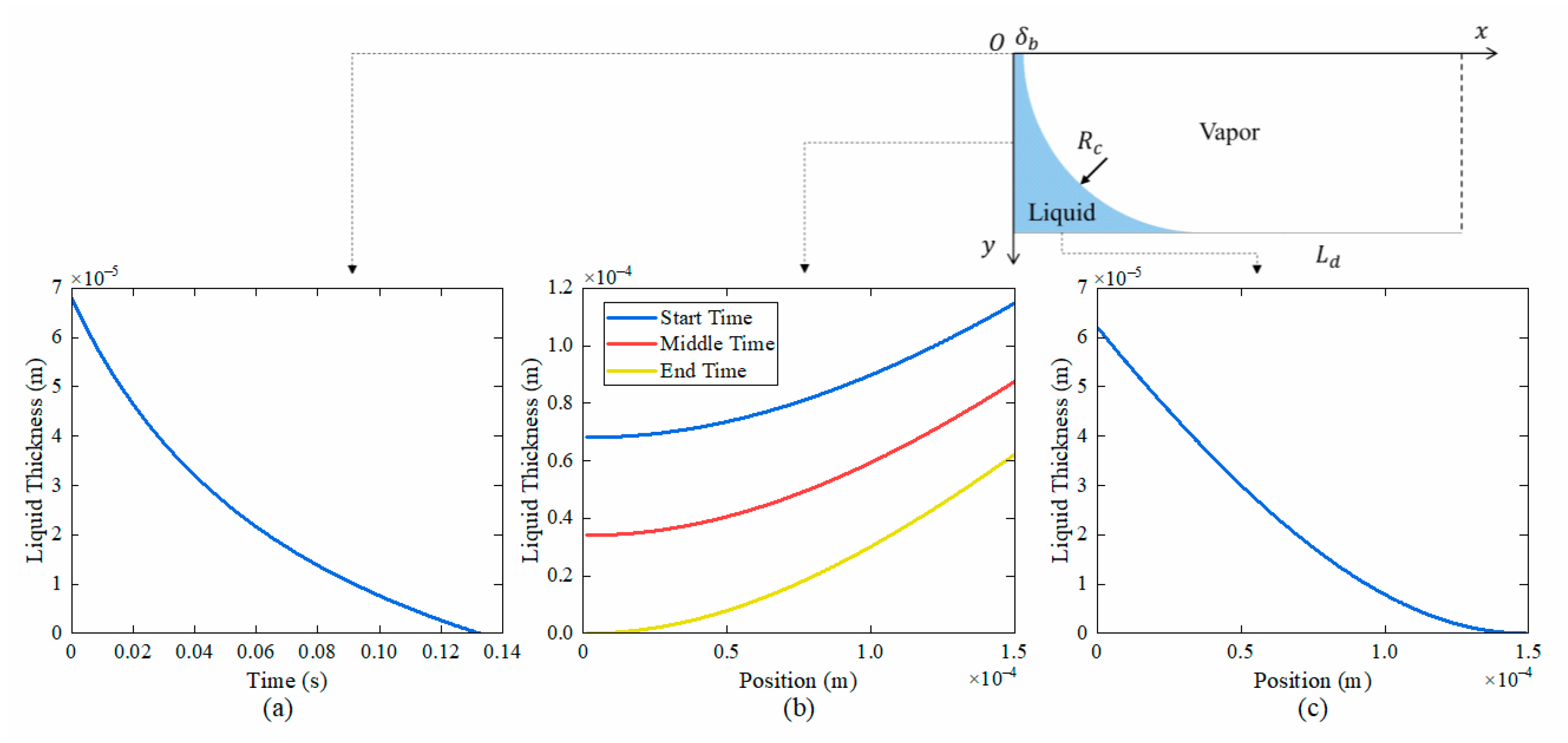
3.4.3. Heat Transfer Coefficient in the Long-Side Wall Dryout Zone
3.4.4. Heat Transfer Coefficient in the Corner Liquid Evaporation Zone
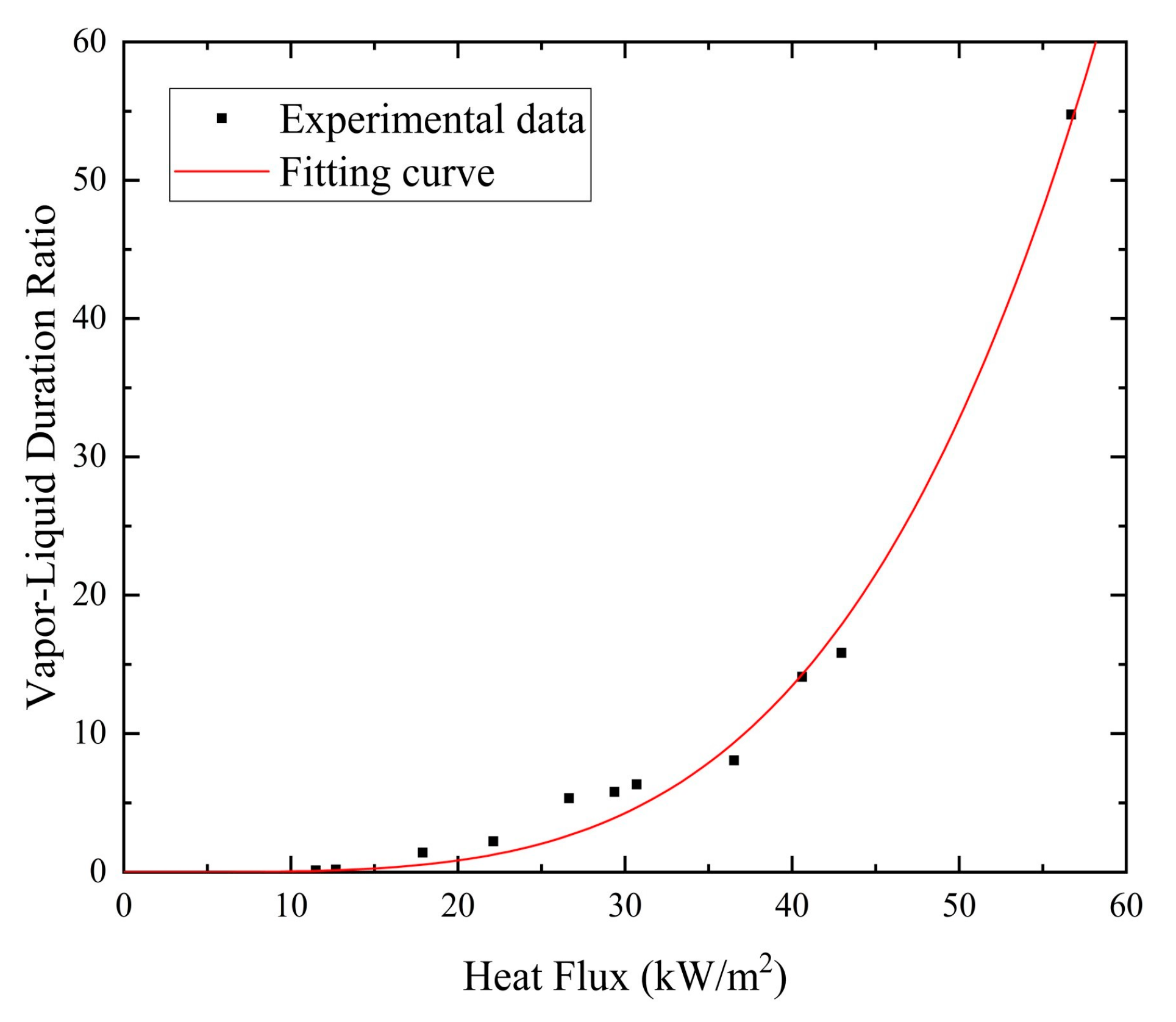
3.5. Analysis of Five Zone Duration
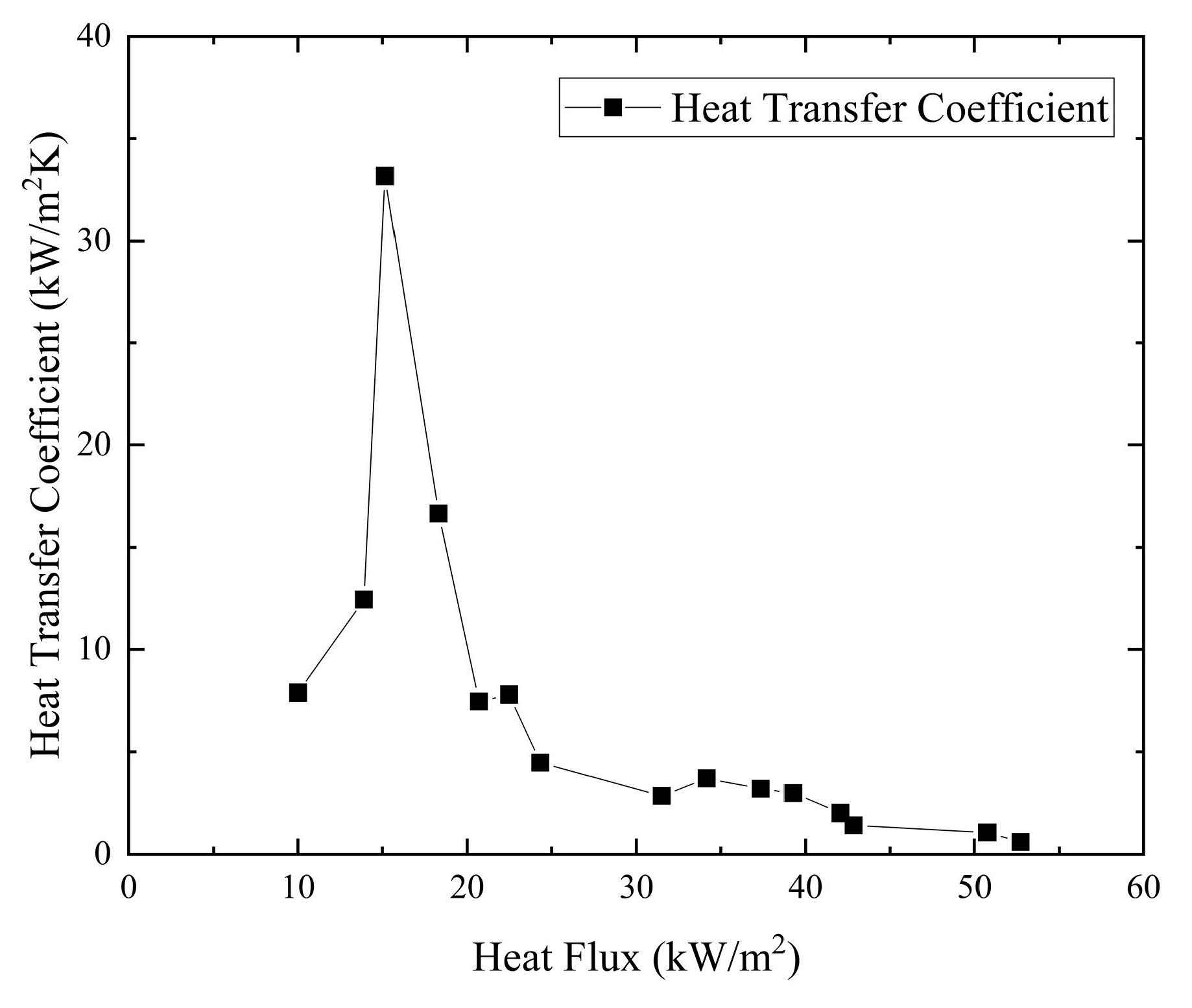
4. Results and Discussion
4.1. Experimental Results
4.2. Numerical Results of Five-Zone Model
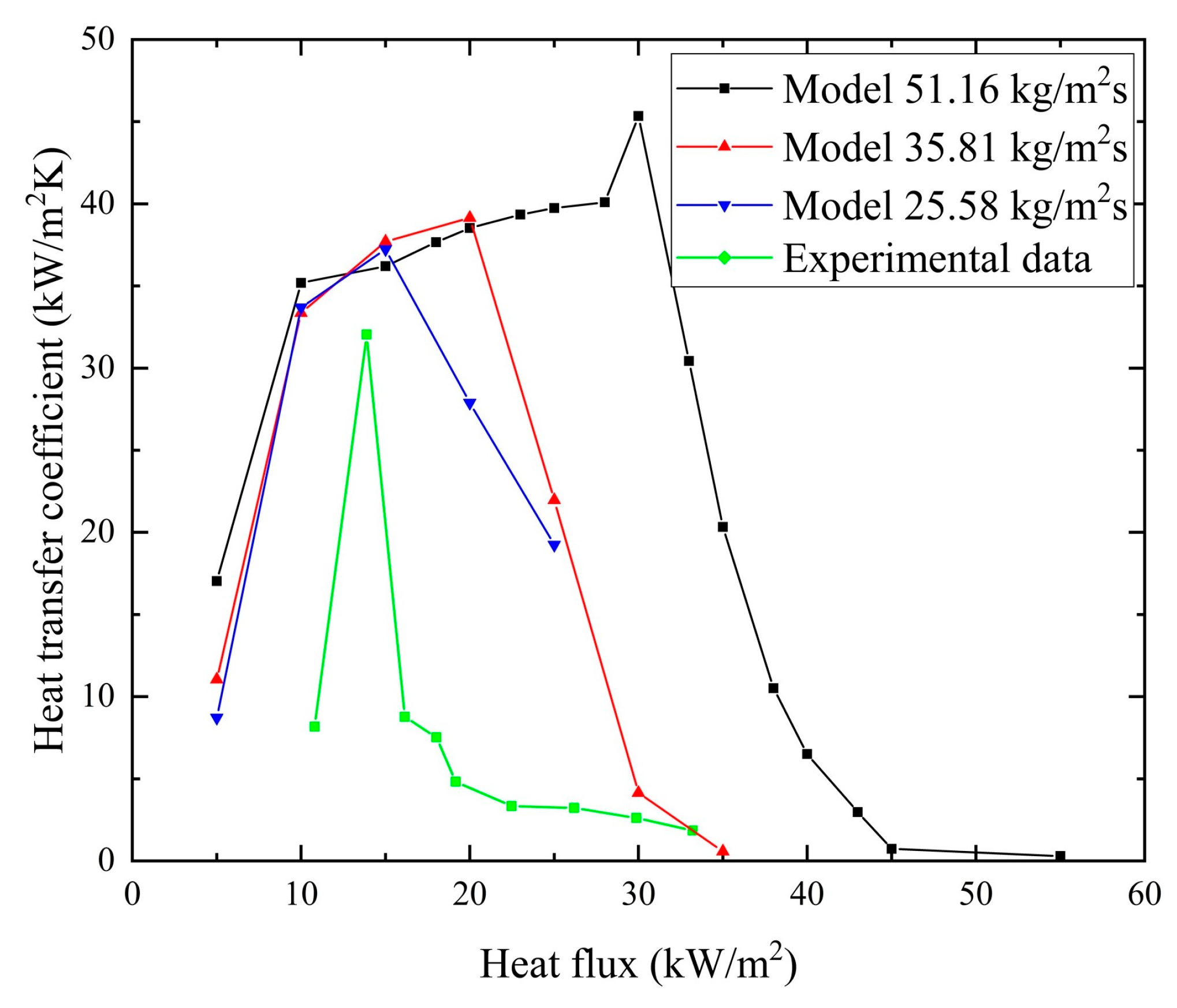
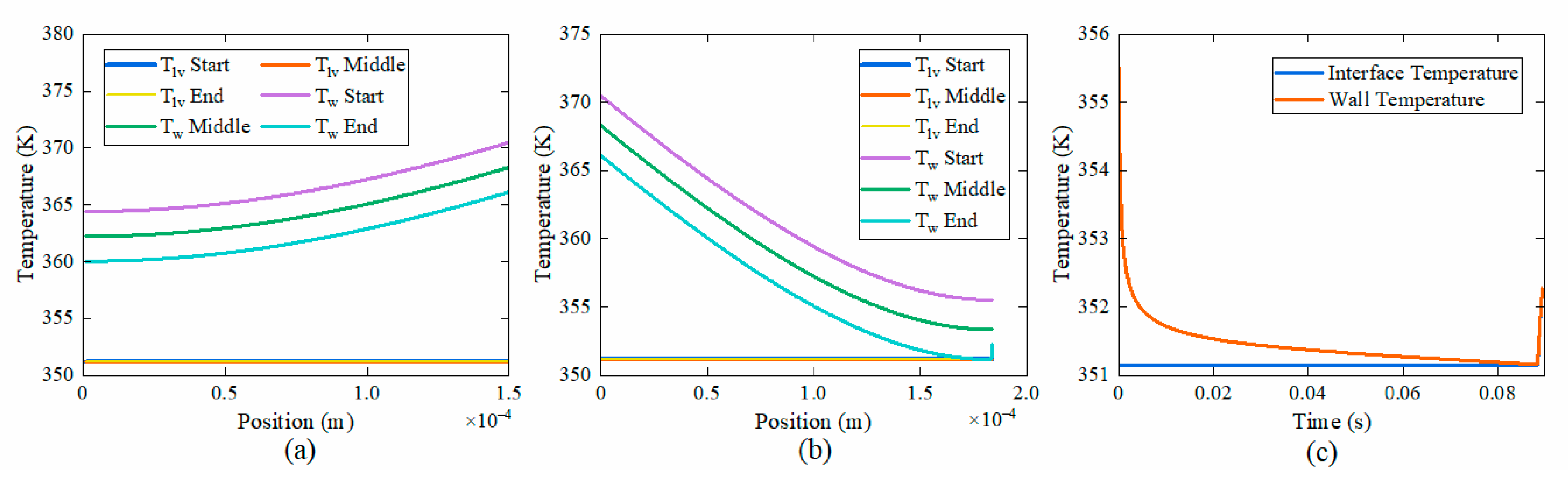
4.2.1. Model Validation
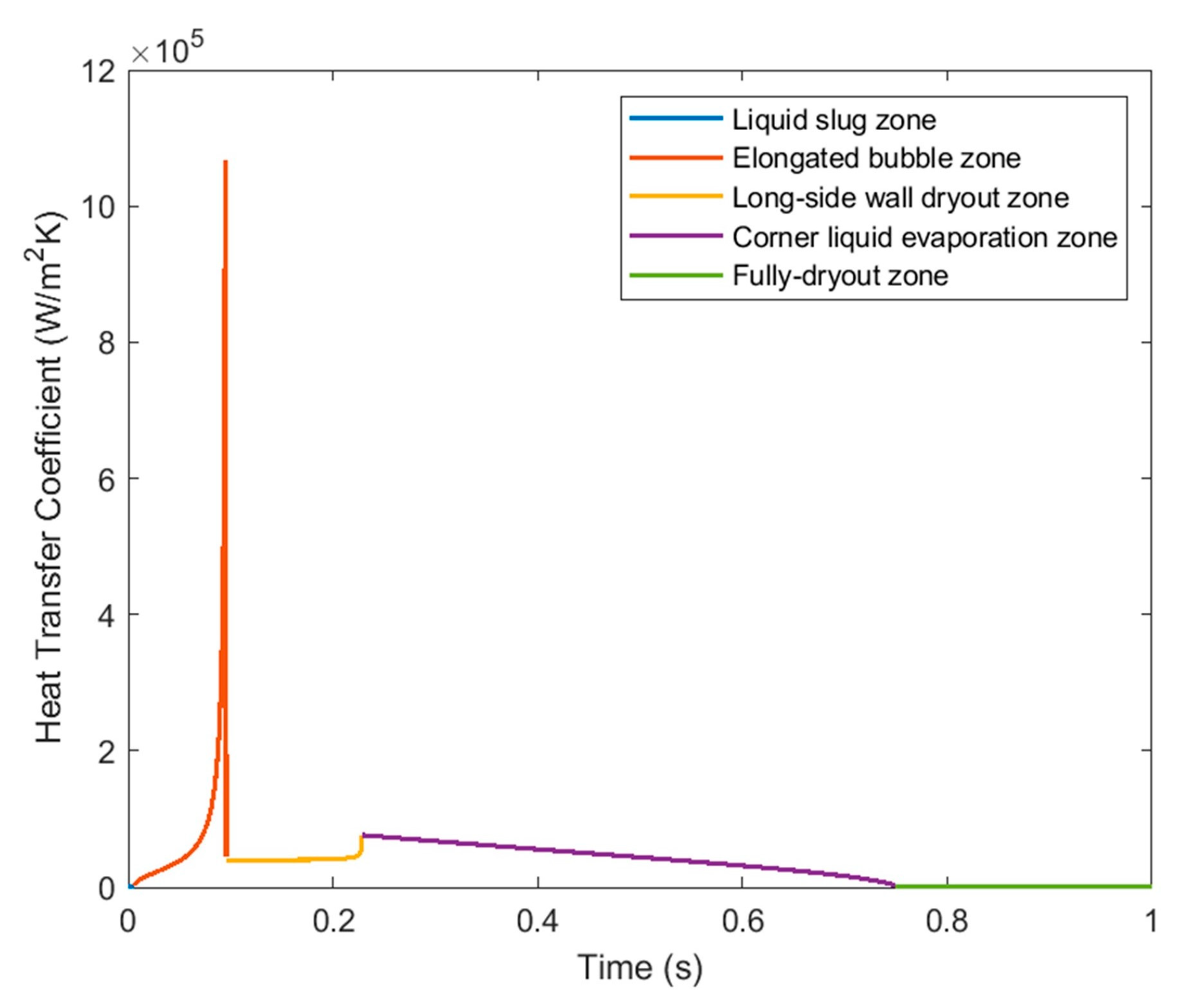
4.2.2. The Spatially Averaged Heat Transfer Coefficient during a Bubble Formation Period
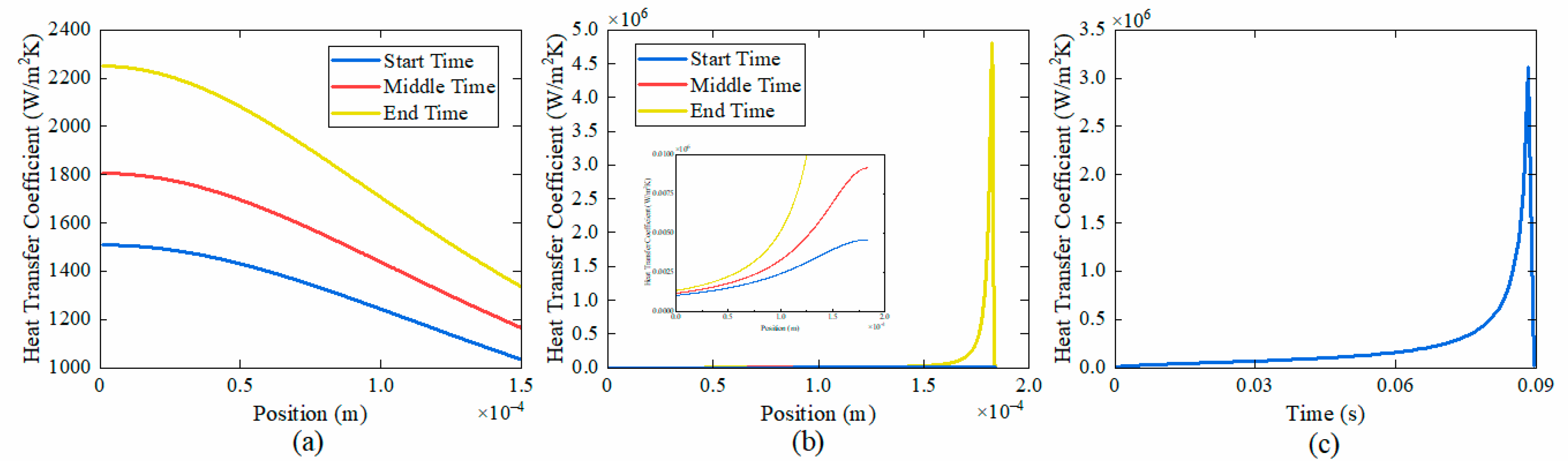
4.2.3. The Elongated Bubble Zone
4.2.4. The Long-Side Wall Dryout Zone
4.2.5. The Corner Liquid Evaporation Zone
4.3. Influence Factor of Heat Transfer Performance
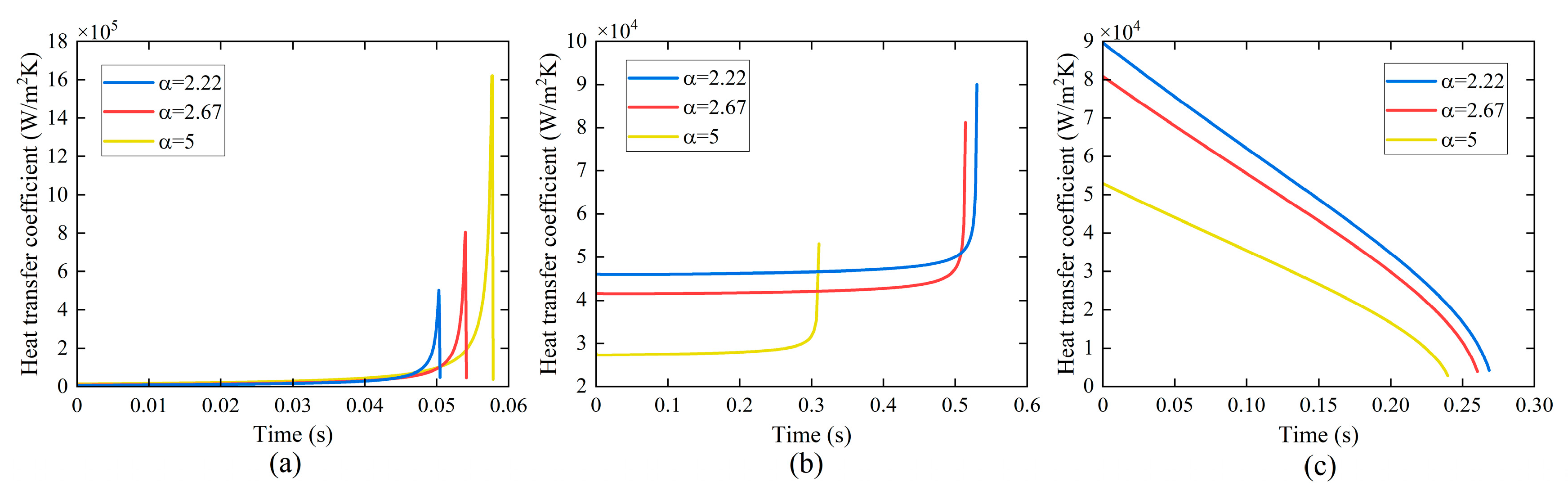
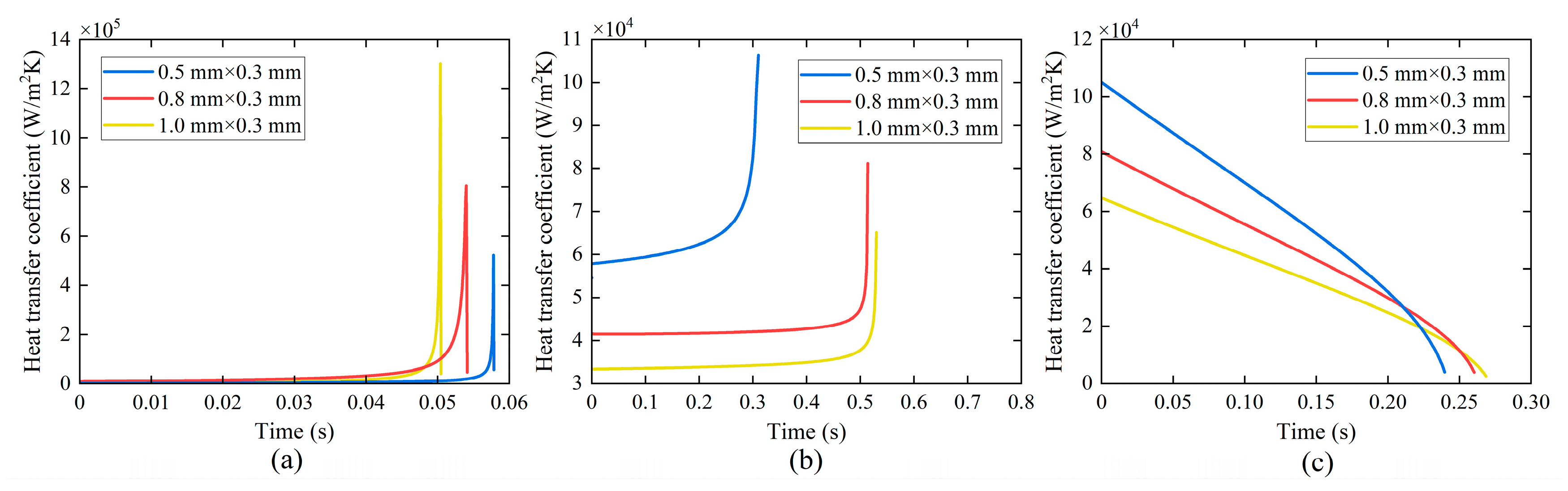
4.3.1. Effect of Aspect Ratio
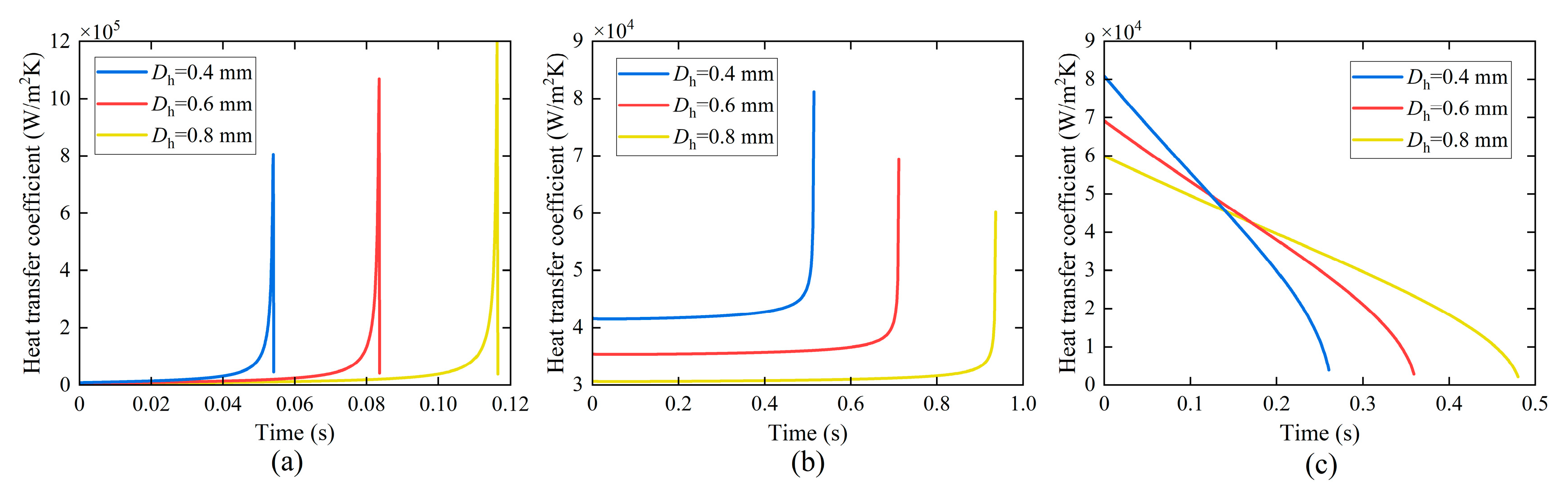
4.3.2. Effect of Hydraulic Diameter
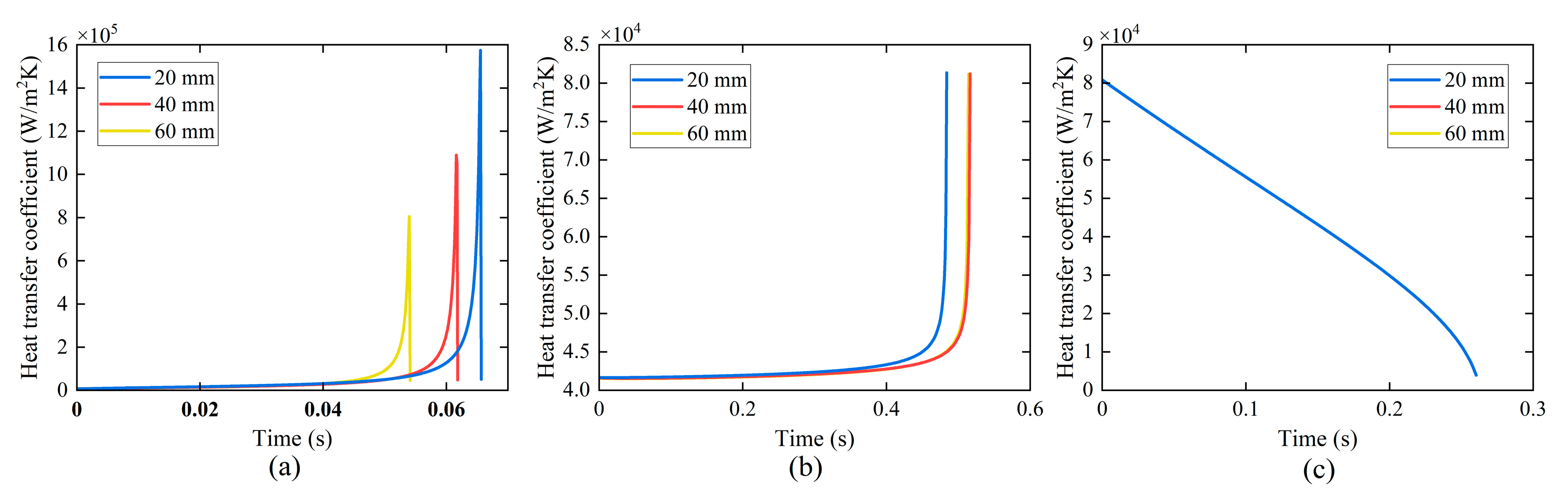
4.3.3. Effect of Measurement Point
4.3.4. Effect of Mass Flux
4.3.5. Effect of Heat Flux
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| A | area, mm2 |
| Bo | boiling number, (Bo = q/Ghlv) |
| Dh | hydraulic diameter, mm |
| G | mass flux, kg/(m2·s) |
| h | heat transfer coefficient, kW/(m2·K) |
| hlv | latent heat, kJ/kg |
| H | height, mm |
| K | curvature, 1/m |
| L | length, mm |
| La | half-length of long-side wall, mm |
| m″ | evaporative mass flux, kg/m2 |
| M | molecular weight, kg/kmol |
| Nu | Nusselt number, (Nu = hDh/λ) |
| q | heat flux, kW/m2 |
| R | hydraulic radius, mm |
| R | universal gas constant, J/(kmol·K) |
| t | time, ms |
| T | temperature, °C |
| u | velocity, m/s |
| U | velocity, m/s |
| W | width, mm |
| z | axial location, mm |
| Greek symbols | |
| α | aspect ratio |
| δ | liquid film thickness, μm |
| λ | thermal conductivity, W/(m·K) |
| μ | dynamic viscosity, μPa∙s |
| ρ | density, kg/m3 |
| τ | time, s |
| χ | vapor quality |
| Subscripts | |
| 0 | initial state |
| a | long side of rectangular microchannels cross-section |
| b | short side of rectangular microchannels cross-section |
| b | bubble |
| c | corner |
| l | liquid |
| out | outer |
| sat | saturation |
| v | vapor |
| w | wall surface |
References
- Zu, Y.Q.; Yan, Y.Y.; Gedupudi, S.; Karayiannis, T.G.; Kenning, D.B.R. Confined Bubble Growth During Flow Boiling in a Mini-/Micro-Channel of Rectangular Cross-Section Part Ii: Approximate 3-D Numerical Simulation. Int. J. Therm. Sci. 2011, 50, 267–273. [Google Scholar] [CrossRef]
- Ferrari, A.; Magnini, M.; Thome, J.R. Numerical Analysis of Slug Flow Boiling in Square Microchannels. Int. J. Heat Mass Transf. 2018, 123, 928–944. [Google Scholar] [CrossRef]
- Cheng, L.; Xia, G. Fundamental Issues, Mechanisms and Models of Flow Boiling Heat Transfer in Microscale Channels. Int. J. Heat Mass Transf. 2017, 108, 97–127. [Google Scholar] [CrossRef]
- Chennu, R. Numerical Analysis of Compact Plate-Fin Heat Exchangers for Aerospace Applications. Int. J. Numer. Methods Heat Fluid Flow 2018, 28, 395–412. [Google Scholar] [CrossRef]
- Careri, F.; Khan, R.H.U.; Todd, C.; Attallah, M.M. Additive Manufacturing of Heat Exchangers in Aerospace Applications: A Review. Appl. Therm. Eng. 2023, 235, 121387. [Google Scholar] [CrossRef]
- Karayiannis, T.G.; Mahmoud, M.M. Flow Boiling in Microchannels: Fundamentals and Applications. Appl. Therm. Eng. 2017, 115, 1372–1397. [Google Scholar] [CrossRef]
- Tibiriçá, C.B.; Ribatski, G. Flow Boiling in Micro-Scale Channels—Synthesized Literature Review. Int. J. Refrig. 2013, 36, 301–324. [Google Scholar] [CrossRef]
- Kandlikar, S.G. Fundamental Issues Related to Flow Boiling in Minichannels and Microchannels. Exp. Therm. Fluid Sci. 2002, 26, 389–407. [Google Scholar] [CrossRef]
- Chen, T.; Garimella, S.V. Measurements and High-Speed Visualizations of Flow Boiling of a Dielectric Fluid in a Silicon Microchannel Heat Sink. Int. J. Multiph. Flow 2006, 32, 957–971. [Google Scholar] [CrossRef]
- Harirchian, T.; Garimella, S.V. Effects of Channel Dimension, Heat Flux, and Mass Flux on Flow Boiling Regimes in Microchannels. Int. J. Multiph. Flow 2009, 35, 349–362. [Google Scholar] [CrossRef]
- Thome, J.R.; Bar-Cohen, A.; Revellin, R.; Zun, I. Unified Mechanistic Multiscale Mapping of Two-Phase Flow Patterns in Microchannels. Exp. Therm. Fluid Sci. 2013, 44, 1–22. [Google Scholar] [CrossRef]
- Bao, Z.Y.; Fletcher, D.F.; Haynes, B.S. Flow Boiling Heat Transfer of Freon R11 and Hcfc123 in Narrow Passages. Int. J. Heat Mass Transf. 2000, 42, 3347–3358. [Google Scholar] [CrossRef]
- Yu, W.; France, D.M.; Wambsganss, M.W.; Hull, J.R. Two-Phase Pressure Drop, Boiling Heat Transfer, and Critical Heat Flux to Water in a Small-Diameter Horizontal Tube. Int. J. Multiph. Flow 2002, 28, 927–941. [Google Scholar] [CrossRef]
- Lee, H.J.; Lee, S.Y. Heat Transfer Correlation for Boiling Flows in Small Rectangular Horizontal Channels with Low Aspect Ratios. Int. J. Multiph. Flow 2001, 27, 2043–2062. [Google Scholar] [CrossRef]
- Qu, W.; Mudawar, I. Flow Boiling Heat Transfer in Two-Phase Micro-Channel Heat Sinks––I. Experimental Investigation and Assessment of Correlation Methods. Int. J. Heat Mass Transf. 2003, 46, 2755–2771. [Google Scholar] [CrossRef]
- Tran, T.N.; Wambsganss, M.W. Small Circular- and Rectangular-Channel Boiling with Two Refrigerants. Int. J. Multiph. Flow 1996, 22, 485–498. [Google Scholar] [CrossRef]
- Yen, T.-H.; Shoji, M.; Takemura, F.; Suzuki, Y.; Kasagi, N. Visualization of Convective Boiling Heat Transfer in Single Microchannels with Different Shaped Cross-Sections. Int. J. Heat Mass Transf. 2006, 49, 3884–3894. [Google Scholar] [CrossRef]
- Ali, R.; Palm, B.; Maqbool, M.H. Flow Boiling Heat Transfer of Refrigerants R134a and R245fa in a Horizontal Micro-Channel. Exp. Heat Transf. 2012, 25, 181–196. [Google Scholar] [CrossRef]
- Bigham, S.; Moghaddam, S. Microscale Study of Mechanisms of Heat Transfer During Flow Boiling in a Microchannel. Int. J. Heat Mass Transf. 2015, 88, 111–121. [Google Scholar] [CrossRef]
- Okajima, J.; Stephan, P. Numerical Simulation of Liquid Film Formation and Its Heat Transfer through Vapor Bubble Expansion in a Microchannel. Int. J. Heat Mass Transf. 2019, 136, 1241–1249. [Google Scholar] [CrossRef]
- Mukherjee, A.; Kandlikar, S.G.; Edel, Z.J. Numerical Study of Bubble Growth and Wall Heat Transfer During Flow Boiling in a Microchannel. Int. J. Heat Mass Transf. 2011, 54, 3702–3718. [Google Scholar] [CrossRef]
- Jacobi, A.M.; Thome, J.R. Heat Transfer Model for Evaporation of Elongated Bubble Flows in Microchannels. J. Heat Transf. 2002, 124, 1131–1136. [Google Scholar] [CrossRef]
- Thome, J.R.; Dupont, V.; Jacobi, A.M. Heat Transfer Model for Evaporation in Microchannels. Part I: Presentation of the Model. Int. J. Heat Mass Transf. 2004, 47, 3375–3385. [Google Scholar] [CrossRef]
- Dupont, V.; Thome, J.R.; Jacobi, A.M. Heat Transfer Model for Evaporation in Microchannels. Part Ii: Comparison with the Database. Int. J. Heat Mass Transf. 2004, 47, 3387–3401. [Google Scholar] [CrossRef]
- Consolini, L.; Thome, J.R. A Heat Transfer Model for Evaporation of Coalescing Bubbles in Micro-Channel Flow. Int. J. Heat Fluid Flow 2010, 31, 115–125. [Google Scholar] [CrossRef]
- Costa-Patry, E.; Thome, J.R. Flow Pattern-Based Flow Boiling Heat Transfer Model for Microchannels. Int. J. Refrig. 2013, 36, 414–420. [Google Scholar] [CrossRef]
- Wang, H.; Garimella, S.V.; Murthy, J.Y. Characteristics of an Evaporating Thin Film in a Microchannel. Int. J. Heat Mass Transf. 2007, 50, 3933–3942. [Google Scholar] [CrossRef]
- Harirchian, T.; Garimella, S.V. Flow Regime-Based Modeling of Heat Transfer and Pressure Drop in Microchannel Flow Boiling. Int. J. Heat Mass Transf. 2012, 55, 1246–1260. [Google Scholar] [CrossRef]
- Wang, G.; Hao, L.; Cheng, P. A Four-Zone Model for Saturated Flow Boiling in a Microchannel of Rectangular Cross-Section. Int. J. Heat Mass Transf. 2010, 53, 3439–3448. [Google Scholar] [CrossRef]
- Magnini, M.; Thome, J.R. An Updated Three-Zone Heat Transfer Model for Slug Flow Boiling in Microchannels. Int. J. Multiph. Flow 2017, 91, 296–314. [Google Scholar] [CrossRef]
- Jain, S.; Jayaramu, P.; Gedupudi, S. Modeling of Pressure Drop and Heat Transfer for Flow Boiling in a Mini/Micro-Channel of Rectangular Cross-Section. Int. J. Heat Mass Transf. 2019, 140, 1029–1054. [Google Scholar] [CrossRef]
- Sun, Y.; Guo, C.; Jiang, Y.; Wang, T.; Zhang, L. Transient Film Thickness and Microscale Heat Transfer During Flow Boiling in Microchannels. Int. J. Heat Mass Transf. 2018, 116, 458–470. [Google Scholar] [CrossRef]
- Guo, C.; Tao, J.; Jiang, Y.; Sun, Y.; Wang, T. Measurement and Theoretical Analysis of Transient Liquid Film During Micro-Channel Flow Boiling. Int. J. Multiph. Flow 2020, 130, 103365. [Google Scholar] [CrossRef]
- Zhang, P.; Wang, T.; Jiang, Y.; Guo, C. Measurement of Transient Liquid Film and Its Effect on Flow Boiling Heat Transfer in Non-Circular Microchannels. Int. J. Therm. Sci. 2023, 184, 108004. [Google Scholar] [CrossRef]
- Shah, R.K.; London, A.L. Laminar Flow Forced Convection in Ducts: A Source Book for Compact Heat Exchanger Analytical Data; Academic Press: New York, NY, USA, 1978; pp. 78–138. [Google Scholar]
- Wayner, P.C., Jr. The Effect of Interfacial Mass Transport on Flow in Thin Liquid Films. Colloids Surf. 1991, 52, 71–84. [Google Scholar] [CrossRef]
- Bretherton, F.P. The Motion of Long Bubbles in Tubes. J. Fluid Mech. 1961, 5, 166–188. [Google Scholar] [CrossRef]
- Aussillous, P.; Quéré, D. Quick Deposition of a Fluid on the Wall of a Tube. Phys. Fluids 2000, 12, 2367–2371. [Google Scholar] [CrossRef]
- Zhang, P.; Wang, T.; Jiang, Y.; Yu, C.; Guo, C.; Tan, S. Experimental Study of Initial Liquid Film Thickness in Square Microchannel Two-Phase Flow. Heat Transf. Res. 2022, 53, 51–69. [Google Scholar] [CrossRef]
- Hazel, A.L.; Heil, M. The Steady Propagation of a Semi-Infinite Bubble into a Tube of Elliptical or Rectangular Cross-Section. J. Fluid Mech. 2002, 470, 91–114. [Google Scholar] [CrossRef]
- Revellin, R.; Thome, J.R. A New Type of Diabatic Flow Pattern Map for Boiling Heat Transfer in Microchannels. J. Micromech. Microeng. 2007, 17, 788–796. [Google Scholar] [CrossRef]











Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhu, J.; Zhang, P.; Tan, S.; Wang, T.; Guo, C.; Jiang, Y. Heat Transfer Model Based on Flow Pattern during Flow Boiling in Rectangular Microchannels. Aerospace 2024, 11, 733. https://doi.org/10.3390/aerospace11090733
Zhu J, Zhang P, Tan S, Wang T, Guo C, Jiang Y. Heat Transfer Model Based on Flow Pattern during Flow Boiling in Rectangular Microchannels. Aerospace. 2024; 11(9):733. https://doi.org/10.3390/aerospace11090733
Chicago/Turabian StyleZhu, Jiamin, Peng Zhang, Sicong Tan, Tao Wang, Chaohong Guo, and Yuyan Jiang. 2024. "Heat Transfer Model Based on Flow Pattern during Flow Boiling in Rectangular Microchannels" Aerospace 11, no. 9: 733. https://doi.org/10.3390/aerospace11090733
APA StyleZhu, J., Zhang, P., Tan, S., Wang, T., Guo, C., & Jiang, Y. (2024). Heat Transfer Model Based on Flow Pattern during Flow Boiling in Rectangular Microchannels. Aerospace, 11(9), 733. https://doi.org/10.3390/aerospace11090733





