An Endurance Equation for Hybrid-Electric Aircraft
Abstract
1. Introduction
2. Hybrid-Electric Aircraft Configuration
3. Derivation of Hybrid-Electric Endurance Equation
- For the series hybrid-electric aircraft:
- For the parallel hybrid-electric aircraft:
- Limiting cases:
- At φ = 1, the derived endurance equation (Equation (36)) recedes to the endurance equation [20] for the fully electric aircraft.
- Fully fueled case or (φ = 0):
- Fully electric case (or φ = 1):
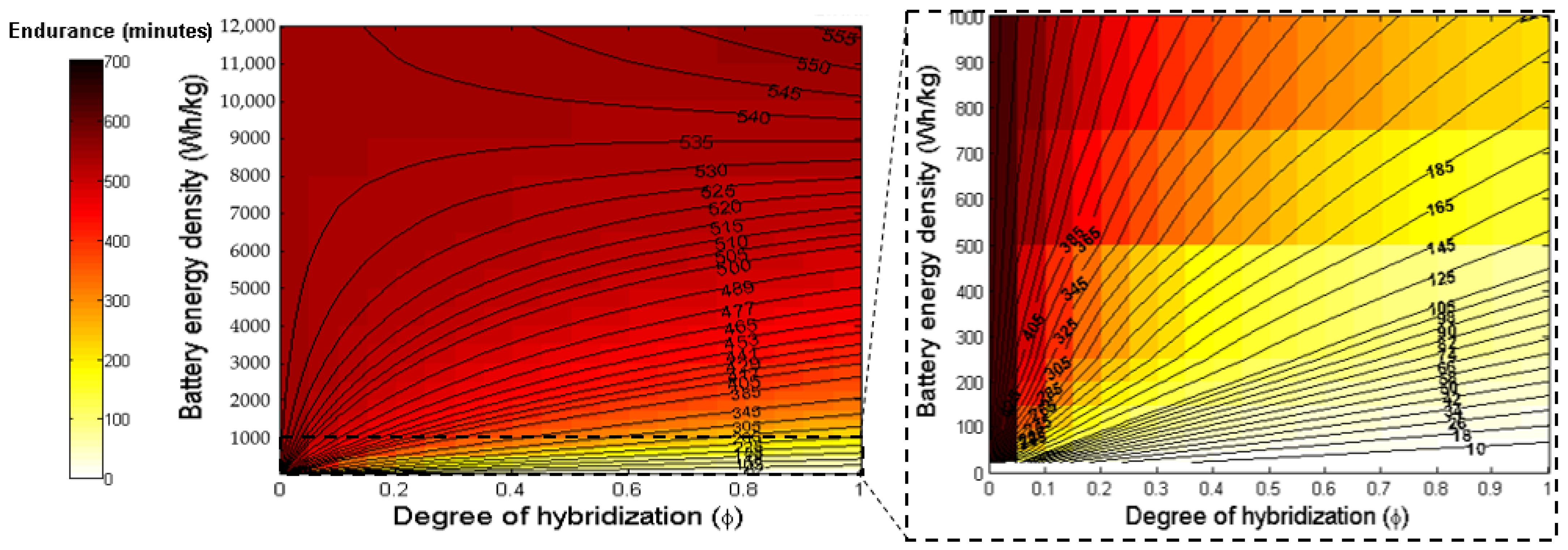
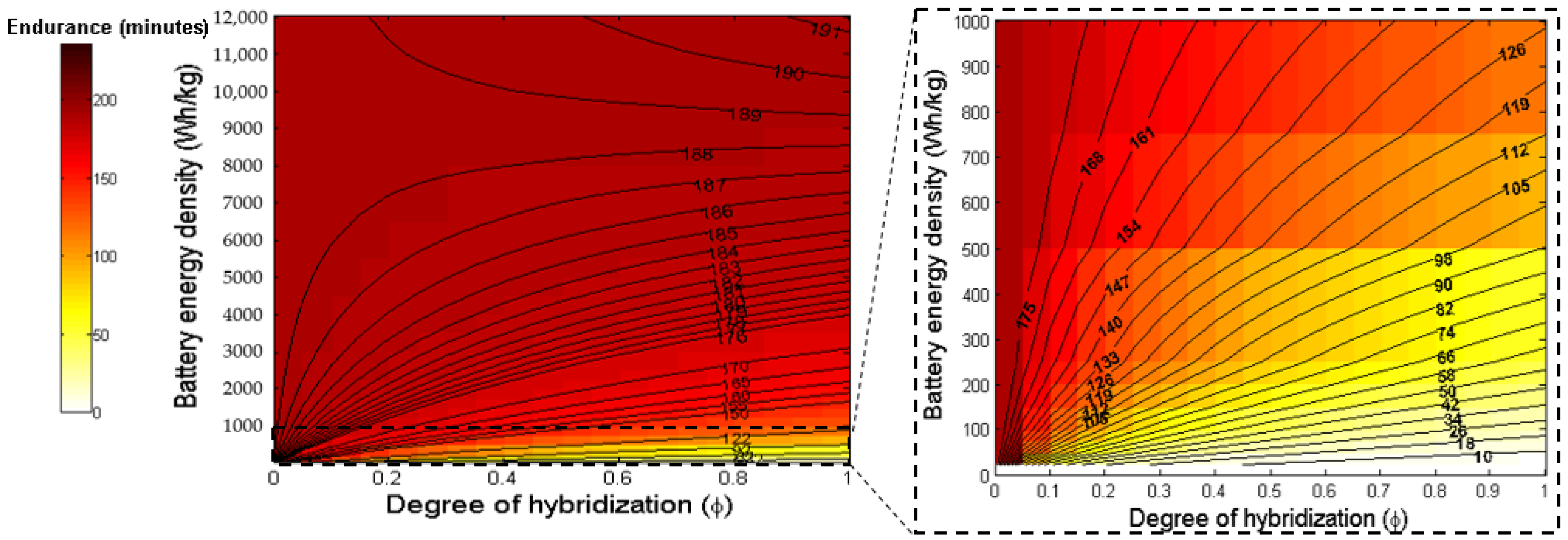
4. Case Study
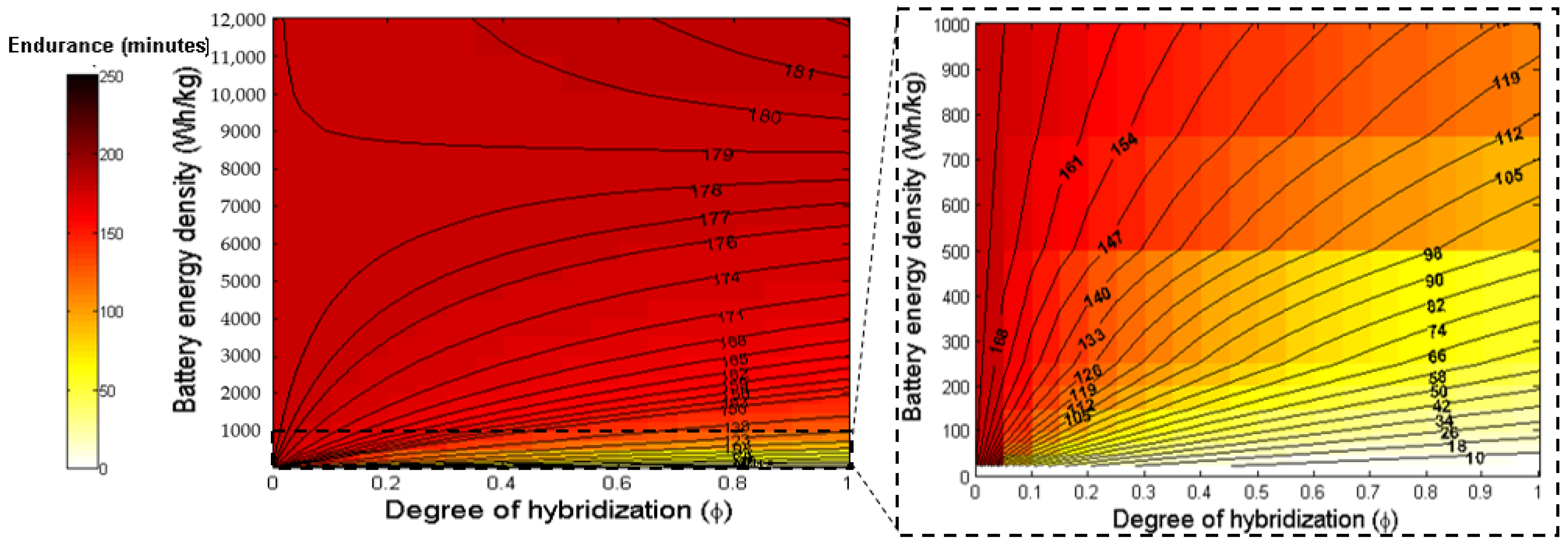
- It was observed that the parallel hybrid has marginally higher endurance as compared to the series hybrid. One reason can be that the series hybrid has a larger efficiency penalty due to the generator and also due to motor power conversion. On the other hand, parallel hybrid is connected to a motor powered by an energy source and, henceforth, is not affected by this efficiency penalty. It was also observed that the deviation in the configurations enhances with the increase in the degree of hybridization, as showcased in Table 3. Both cases converge if the efficiencies of both the electric motor and generator are considered to be one.
| Parallel Hybrid | Series Hybrid | ||||
|---|---|---|---|---|---|
| Battery Energy Density | 500 Wh/kg | 1000 Wh/kg | 500 Wh/kg | 1000 Wh/kg | |
| Degree of hybridization | 0.3 | 285.6 min | 385.8 min | 278.5 min | 372 min |
| 0.6 | 183.5 min | 294.4 min | 181.6 min | 287.4 min | |
| 0.9 | 124.2 min | 226.3 min | 130.3 min | 230.8 min | |
- In the cases of parallel and series hybrid configurations, it is observed that there is an energy density threshold (EDT), below which the endurance reduces with an increase in degree of hybridization. Conversely, above EDT, endurance enhances with an increase in degree of hybridization. For any aircraft configuration, the EDT is not constant with degree of hybridization. For the parallel hybrid, the value ranges between 8960 < EDT < 9030 Wh/kg (in terms of the battery energy density), whereas in the series hybrid, it ranges between 8650 < EDT < 8950 Wh/kg (also in terms of the battery energy density). The higher value for the parallel hybrid is due to its higher dependence on a battery energy source. The exact values depend on the specific aircraft parameters given in Table 2. At the EDT, aircraft endurance is not dependent on degree of hybridization at all. This is probably because the effect of battery energy density and corresponding efficiencies becomes more prominent than the fuel energy density and corresponding efficiencies.
- The significance of the value of the EDT becomes apparent when considering the current battery energy storage technologies. The current energy density of batteries lies at approximately 228 Wh/kg. This is expected to double approximately every 23 years [9] and, thus, this point lies way beyond the theoretical limits of battery energy densities. However, the endurance theory would still hold if another energy source would be able to fill the gap.
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| AoA | Angle of Attack |
| cL | Coefficient of Lift |
| cD | Coefficient of Drag |
| D | Drag [N] |
| e | Specific Energy [J/kg] |
| E | Energy [J] |
| EDT | Energy Density Threshold |
| g | Gravitational acceleration [m/s2] |
| L | Lift [N] |
| P | Power [W] |
| S | Planform area [m2] |
| t | Time [s] |
| T | Thrust [N] |
| v | Velocity [m/s] |
| W | Weight [N] |
| γ | Flight path angle [rad] |
| η | Efficiency |
| φ | Degree of hybridization |
| ρ | Density [kg/m3] |
| Subscripts | |
| 0 | Start of the mission |
| 1,2,3 | Powertrain branch indices |
| bat | Battery |
| eg | Electrical generator |
| em | Electrical motor |
| end | End of mission segment |
| f | Fuel |
| gt | Gas turbine |
| gb | Gearbox |
| OE | Operating Empty |
| p | Propeller |
| PL | Payload |
| start | Start of mission segment |
| tot | Total |
References
- Batra, A.; Raute, R.; Camilleri, R. On the range equation for a hybrid-electric aircraft. Aerospace 2023, 10, 687. [Google Scholar] [CrossRef]
- Intergovernmental Panel on Climate Change (IPCC) 2022 Report. Available online: https://www.ipcc.ch/report/ar6/wg2/ (accessed on 24 April 2023).
- International Civil Aviation Organization (ICAO). Post-COVID-19 Forecasts Scenarios. Available online: https://www.icao.int/sustainability/Pages/Post-Covid-Forecasts-Scenarios.aspx (accessed on 24 December 2023).
- European Comission. Flightpath 2050 CARE2050. Europe’s Vision for Aviation. Available online: https://ec.europa.eu/transport/sites/transport/files/modes/air/doc/flightpath2050.pdf (accessed on 25 April 2023).
- European Comission. European Green Deal. Available online: https://ec.europa.eu/commission/presscorner/detail/en/ip_23_2389 (accessed on 22 December 2023).
- Schwab, A.; Thomas, A.; Bennett, J.; Robertson, E.; Cary, S. Electrification of Aircraft: Challenges, Barriers, and Potential Impacts; NREL/TP-6A20-80220; National Renewable Energy Laboratory (NREL): Golden, CO, USA, 2021. [Google Scholar]
- Hoelzen, J.; Silberhorn, D.; Zill, T.; Bensmann, B.; Rauschenbach, R.H. Hydrogen-Powered Aviation and Its Reliance on Green Hydrogen Infrastructure—Review and Research Gaps; Elsevier: Amsterdam, The Netherlands, 2022; Volume 47, pp. 3108–3130. [Google Scholar] [CrossRef]
- Zhang, L.; Butler, T.L.; Yang, B. Recent trends, opportunities and challenges of sustainable aviation fuel. In Green Energy to Sustainability: Strategies for Global Industries; John Wiley & Sons Ltd.: Hoboken, NJ, USA, 2020; pp. 85–110. [Google Scholar]
- Schäfer, A.W.; Barrett, S.R.H.; Doyme, K.; Dray, L.M.; Gnadt, A.R.; Self, R.; O’Sullivan, A.; Synodinos, A.P.; Torija, A. Technological, economic and environmental prospects of all-electric aircraft. Nat. Energy 2019, 4, 160–166. [Google Scholar] [CrossRef]
- Viswanathan, V.; Epstein, A.H.; Chiang, Y.; Takeuchi, E.; Bradley, M.; Langford, J.; Winter, M. The challenges and opportunities of battery-powered flight. Nat. Perspect. 2022, 601, 519–525. [Google Scholar] [CrossRef] [PubMed]
- Rossi, N.; Trainelli, L.; Riboldi, C.E.D.; Rolando, A. Conceptual Design of Hybrid-Electric Aircraft. Politechnico Di Milano, 2016–2017. Available online: https://hdl.handle.net/10589/139491 (accessed on 20 January 2024).
- Wheeler, P.; Sirimanna, A.S.; Bozhko, S.; Haran, K.S. Electric/Hybrid-Electric Aircraft Propulsion Systems. Proc. IEEE 2021, 109, 1115–1127. [Google Scholar] [CrossRef]
- Marciello, V.; Ruocco, M.; Nicolosi, F.; Stasio, M.D. Markey Analysis, TLARS selection and preliminary design investigations for a regional hybrid-electric aircraft. In Proceedings of the 33rd Congress of the International Council of the Aeronautical Sciences (ICAS), Stockholm, Sweden, 4–9 September 2022. [Google Scholar]
- Vries, R.D.; Hoogreef, M.F.M.; Vos, R. Range Equation for Hybrid-Electric Aircraft with Constant Power Split. J. Aircr. 2020, 57, 552–557. [Google Scholar] [CrossRef]
- Diehl, W.S. Three Methods of Calculating Range and Endurance of Airplanes; US Government Printing Office: Washington, DC, USA, 1925.
- Raymer, D. Aircraft desing: A conceptual approach. In AIAA Education Series, 4th ed.; American Institute of Aeronautics and Astronautics: Reston, VA, USA, 2006. [Google Scholar]
- Traub, L.W. Range and endurance estimates for battery-powered aircraft. J. Aircr. 2011, 48, 703–707. [Google Scholar] [CrossRef]
- Sadraey, M.H. Aircraft Performance: An Engineering Approach, 2nd ed.; CRC Press: Boca Raton, FL, USA, 2023. [Google Scholar] [CrossRef]
- Donateo, T.; Luigi, S. Fuel economy of hybrid electric flight. Appl. Energy 2017, 206, 723–738. [Google Scholar] [CrossRef]
- Nygren, C.K.P.; Major, R.R.S. Breguet’s Formulas for Aircraft Range and Endurance an Application of Integral Calculus. In Proceedings of the 1996 Annual Conference, Washington, DC, USA, 23 June 1996; pp. 1–90. [Google Scholar]
- Xie, Y.; Savvarisal, A.; Tsourdos, A.; Zhang, D.; Gu, L. Review of hybrid electric powered aircraft, its conceptual design and energy management methodologies. Chin. J. Aeronaut. 2021, 34, 432–450. [Google Scholar] [CrossRef]
- Finger, D.F.; Braun, C.; Bil, C. Comparative Assessment of Parallel-Hybrid-Electric Propulsion Systems for Four Different Aircraft. J. Aircr. 2020, 57, 843–853. [Google Scholar] [CrossRef]
- Zamboni, J.; Vos, R.; Emeneth, M.; Schneegans, A. A Method for the Conceptual Design of Hybrid Electric Aircraft. In Proceedings of the AIAA Scitech 2019 Forum, San Diego, CA, USA, 7–11 January 2019. [Google Scholar] [CrossRef]
- Bai, M.; Yang, W.; Li, J.; Kosuda, M.; Fozo, L.; Kelemen, M. Sizing methodology and Energy Management of an Air-Ground Aircraft with Turbo-Electric Hybrid Propulsion System. Aerospace 2022, 9, 764. [Google Scholar] [CrossRef]
- Taylor, A.E. L’Hospital’s Rule. Am. Math. Mon. 1952, 59, 20–24. [Google Scholar] [CrossRef]
- Project Report. Periodic Reporting for Period 3—GAM-2020-REG (REGIONAL AIRCRAFT 2020–2023). Available online: https://cordis.europa.eu/project/id/945548 (accessed on 20 January 2024).
- Clean Aviation. Available online: https://www.clean-aviation.eu/hybrid-electric-regional-aircraft (accessed on 2 April 2024).
- ATR Aircraft. Available online: https://www.atr-aircraft.com/aircraft-services/aircraft-family/atr-72-600/ (accessed on 2 January 2024).
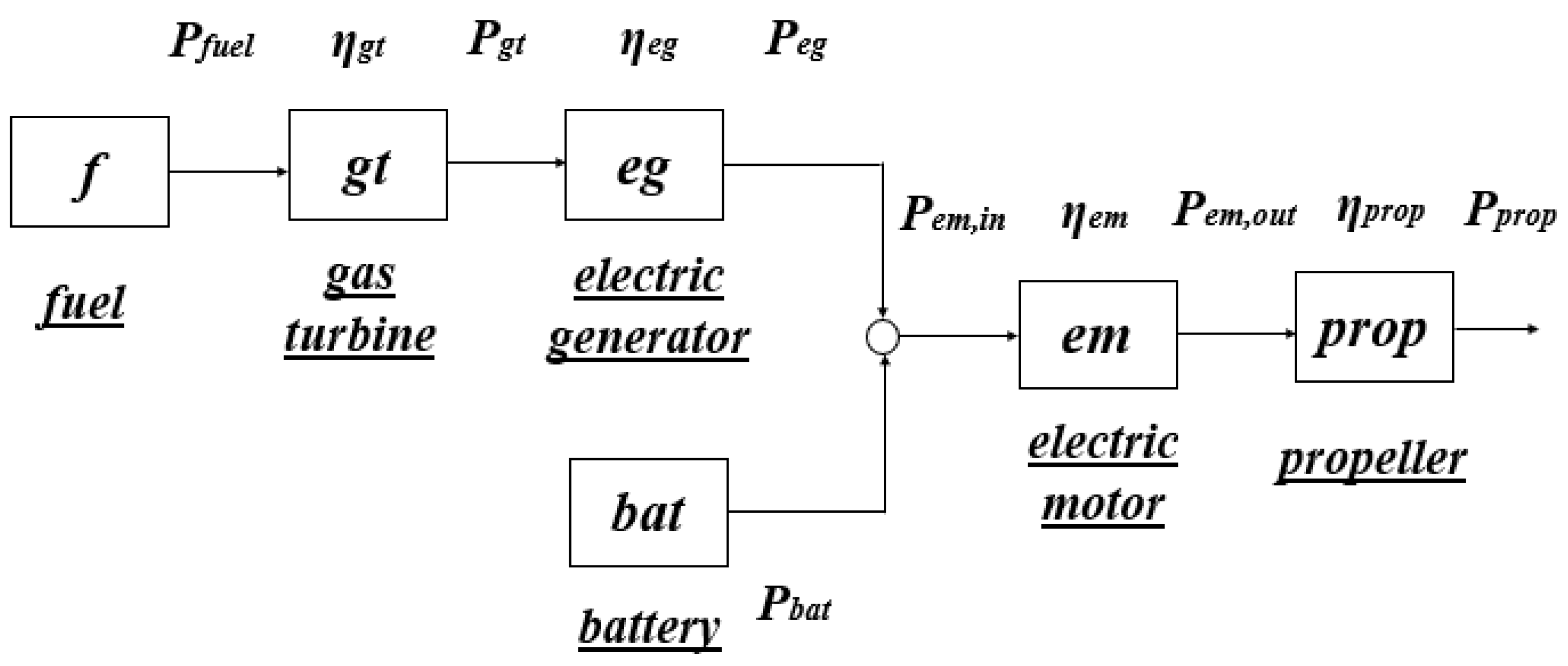

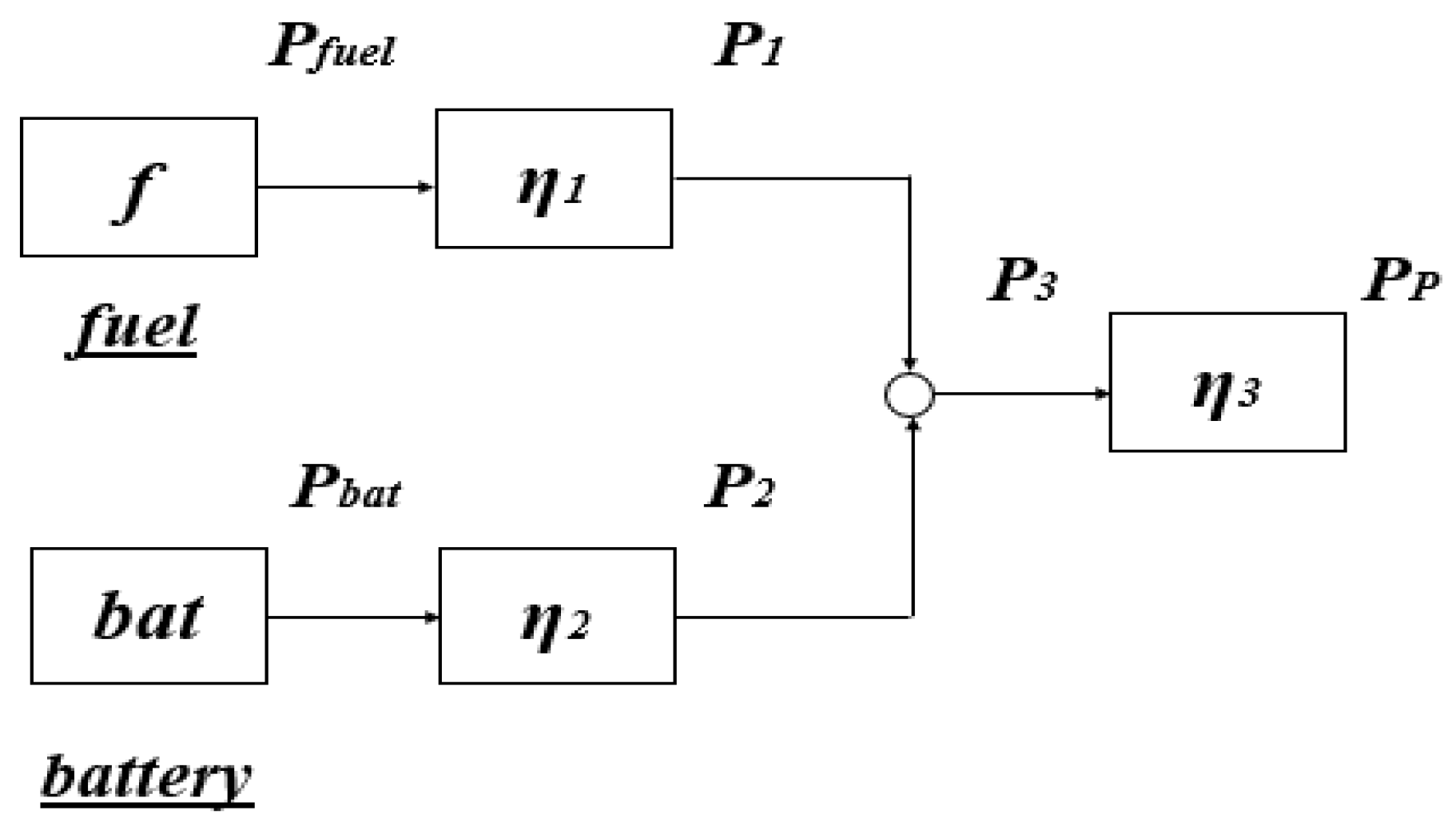

| Simplified Representation (Figure 3) | Parallel Configuration (or Mechanical-Node Architecture) (Figure 2) | Series Configuration (or Electrical-Node Architecture) (Figure 1) |
|---|---|---|
| 1 | ||
| Variables | Values |
|---|---|
| Empty weight (WOE [N]) | 50,000 |
| Payload weight (WPL [N]) | 20,000 |
| Total input energy (Eo,tot [GJ]) | 25 |
| Gas turbine efficiency (ηgt) | 0.35 |
| Electric motor efficiency (ηem) | 0.95 |
| Electric generator efficiency (ηeg) | 0.98 |
| Propulsive efficiency (ηp) | 0.80 |
| Gearbox efficiency (ηgb) | 0.95 |
| Energy density of aviation fuel (ef [Wh/kg]) | 11,900 |
| Acceleration due to gravity (g [m/s2]) | 9.81 |
| Coefficient of lift | 0.6391 |
| Coefficient of drag | 0.0572 |
| Planform area [m2] | 61 |
| Density (at cruise altitude) [kg/m3] | 0.5579 |
| Cruise velocity [m/s] | 141.67 |
| Variables | Values |
|---|---|
| Empty weight (WOE [N]) | 127,040 |
| Payload weight (WPL [N]) | 68,670 |
| Total input energy (Eo,tot [GJ]) | 25 |
| Gas turbine efficiency (ηgt) | 0.35 |
| Electric motor efficiency (ηem) | 0.95 |
| Electric generator efficiency (ηeg) | 0.98 |
| Propulsive efficiency (ηp) | 0.80 |
| Gearbox efficiency (ηgb) | 0.95 |
| Energy density of aviation fuel (ef [Wh/kg]) | 11,900 |
| Acceleration due to gravity (g [m/s2]) | 9.81 |
| Coefficient of lift | 0.6391 |
| Coefficient of drag | 0.0572 |
| Planform area [m2] | 61 |
| Density (at cruise altitude) [kg/m3] | 0.5579 |
| Cruise velocity [m/s] | 141.67 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Batra, A.; Raute, R.; Camilleri, R. An Endurance Equation for Hybrid-Electric Aircraft. Aerospace 2024, 11, 698. https://doi.org/10.3390/aerospace11090698
Batra A, Raute R, Camilleri R. An Endurance Equation for Hybrid-Electric Aircraft. Aerospace. 2024; 11(9):698. https://doi.org/10.3390/aerospace11090698
Chicago/Turabian StyleBatra, Aman, Reiko Raute, and Robert Camilleri. 2024. "An Endurance Equation for Hybrid-Electric Aircraft" Aerospace 11, no. 9: 698. https://doi.org/10.3390/aerospace11090698
APA StyleBatra, A., Raute, R., & Camilleri, R. (2024). An Endurance Equation for Hybrid-Electric Aircraft. Aerospace, 11(9), 698. https://doi.org/10.3390/aerospace11090698








