Stability of Single-Channel Homing Rolling Aerospace Vehicle
Abstract
1. Introduction
- The analysis of the stability of single-channel homing missiles with slow roll using the Frank–Wall stability criterion. This analysis follows the influence of the main parameters in the missile’s guidance system, and for some of them, restrictions and stability domains will be defined.
- The determination of the Frank–Wall (F-W) stability parameters for fourth-order polynomials; the calculation procedures are detailed in Annex D of this paper.
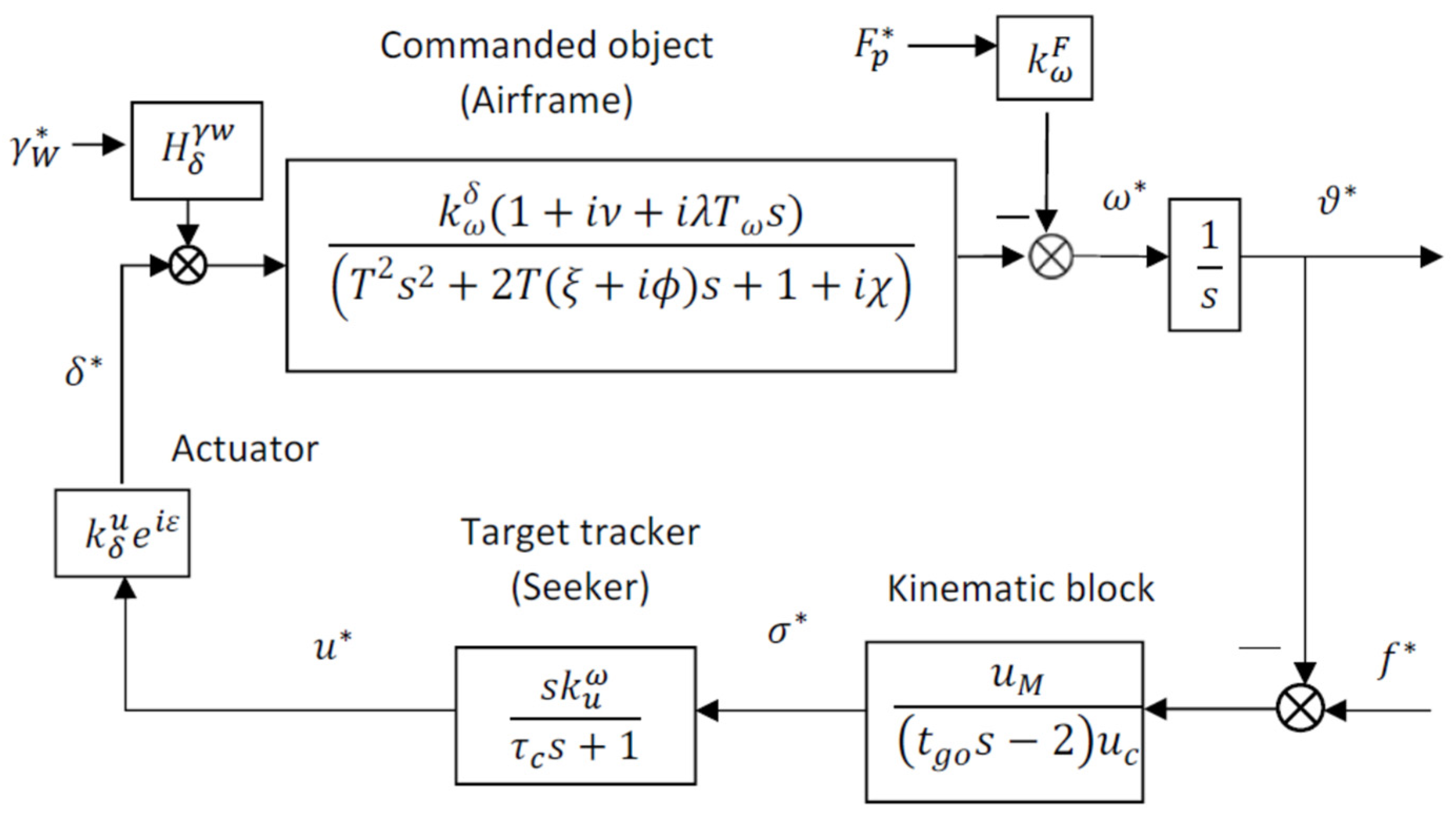
- The detailed structural scheme of commanded movement for a slow-rolling missile.
- The detailed structural scheme for the homing slow-rolling missile with a single channel.
- The analysis of the stability and command derivative magnitude from the relation (61) and of flight quality parameters from relations (63), (64), (66), (81) and (83).
- The expression of average equivalent canard deflection in nonlinear (147) and linear form (148).
- The validation of linear analysis with the F-W criterion using a nonlinear model (Section 10.4)
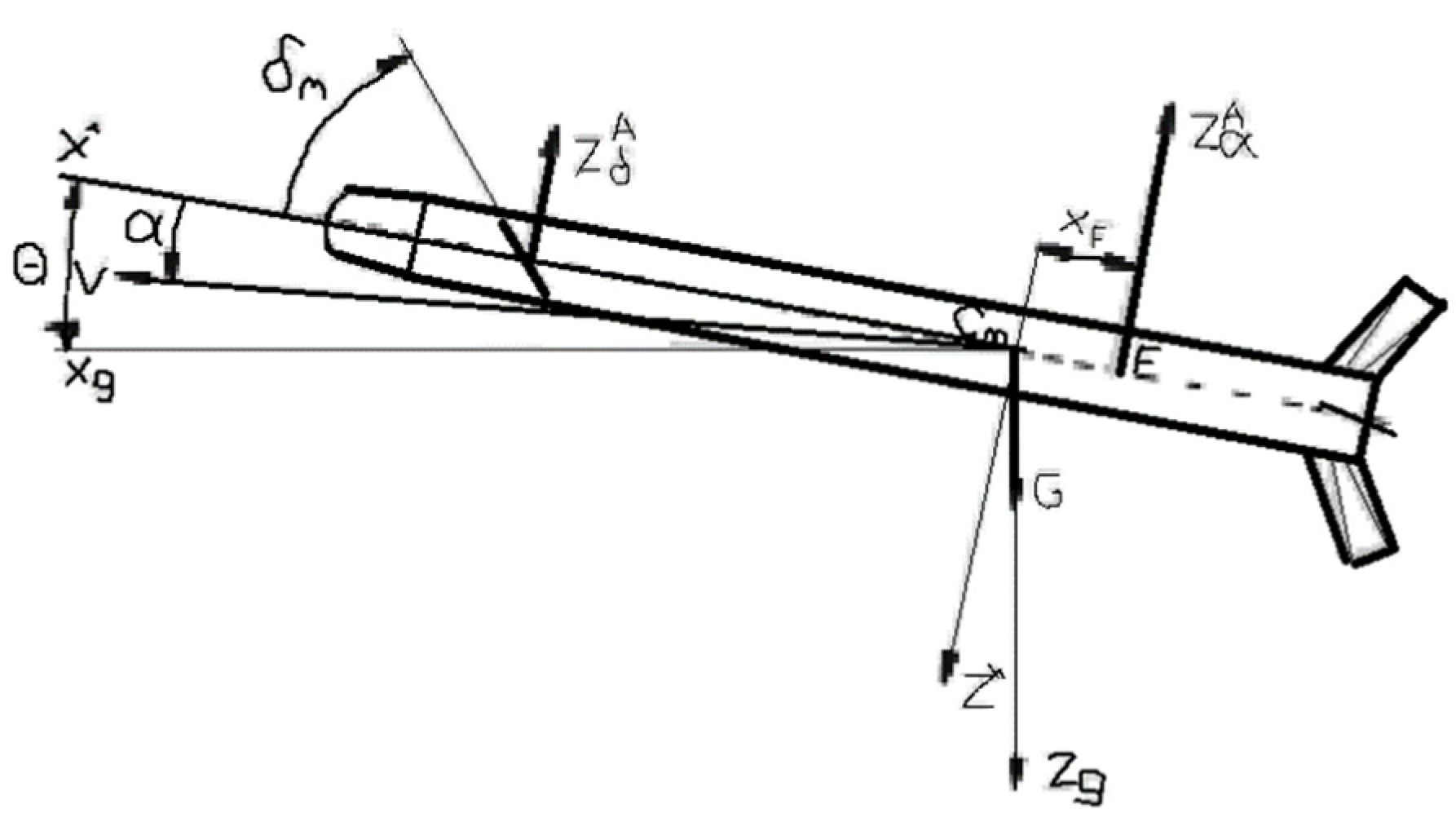
2. Equations of the General Motion in the Body Frame
2.1. Kinematic Equations Using Attitude Angles
2.2. Dynamic Equations of Motion in the Body Frame
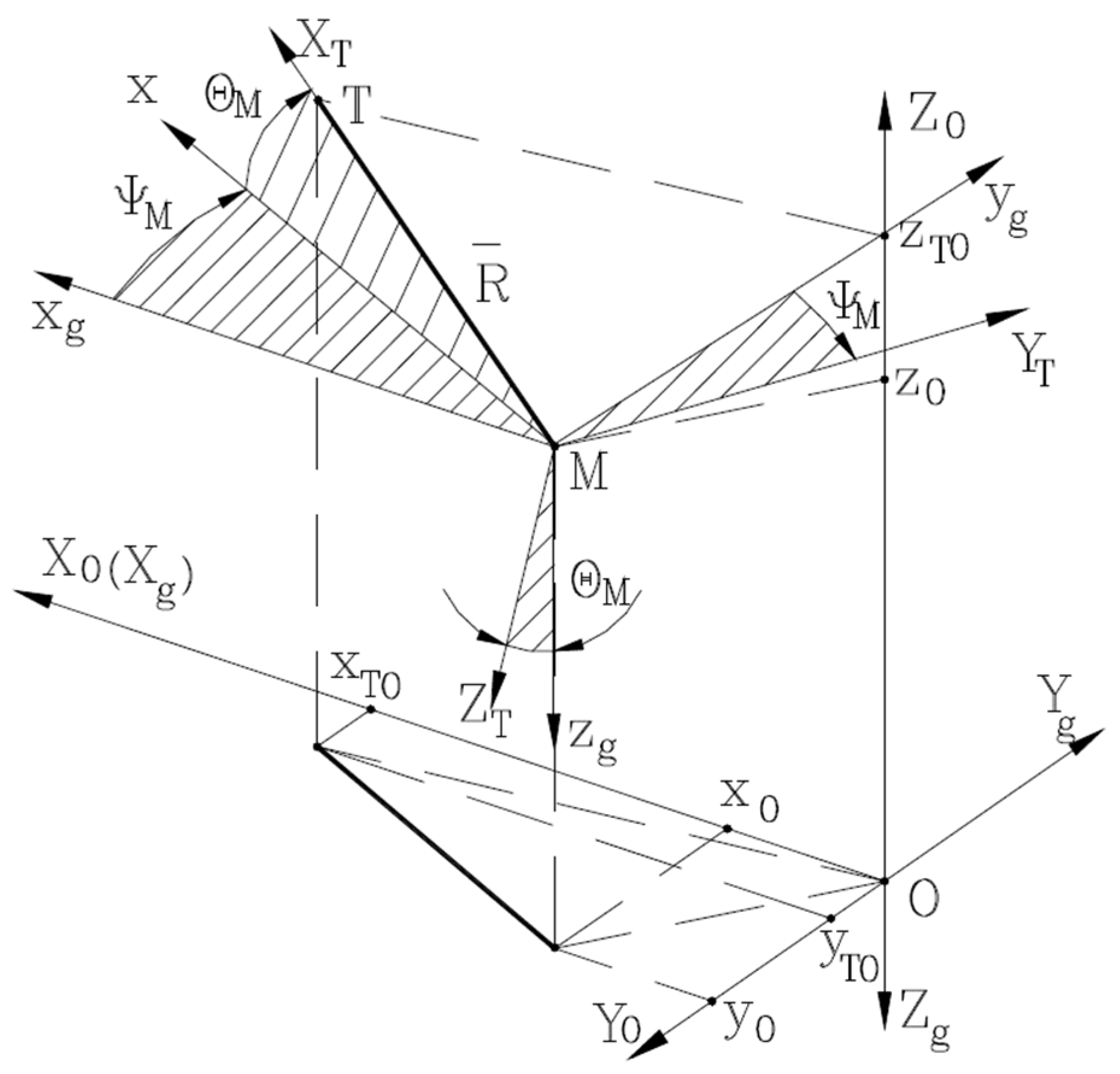
3. Equations of the General Motion in the Resal Frame
4. Basic Movement and Maximum Maneuverability
4.1. Basic Movement
4.2. Maximum Maneuverability
5. The Linear Form of the Equations of Rapid Motion around the Center of Mass
6. The Flight Quality Parameters
7. The Guided Flight Model
7.1. Kinematic Guidance Equations
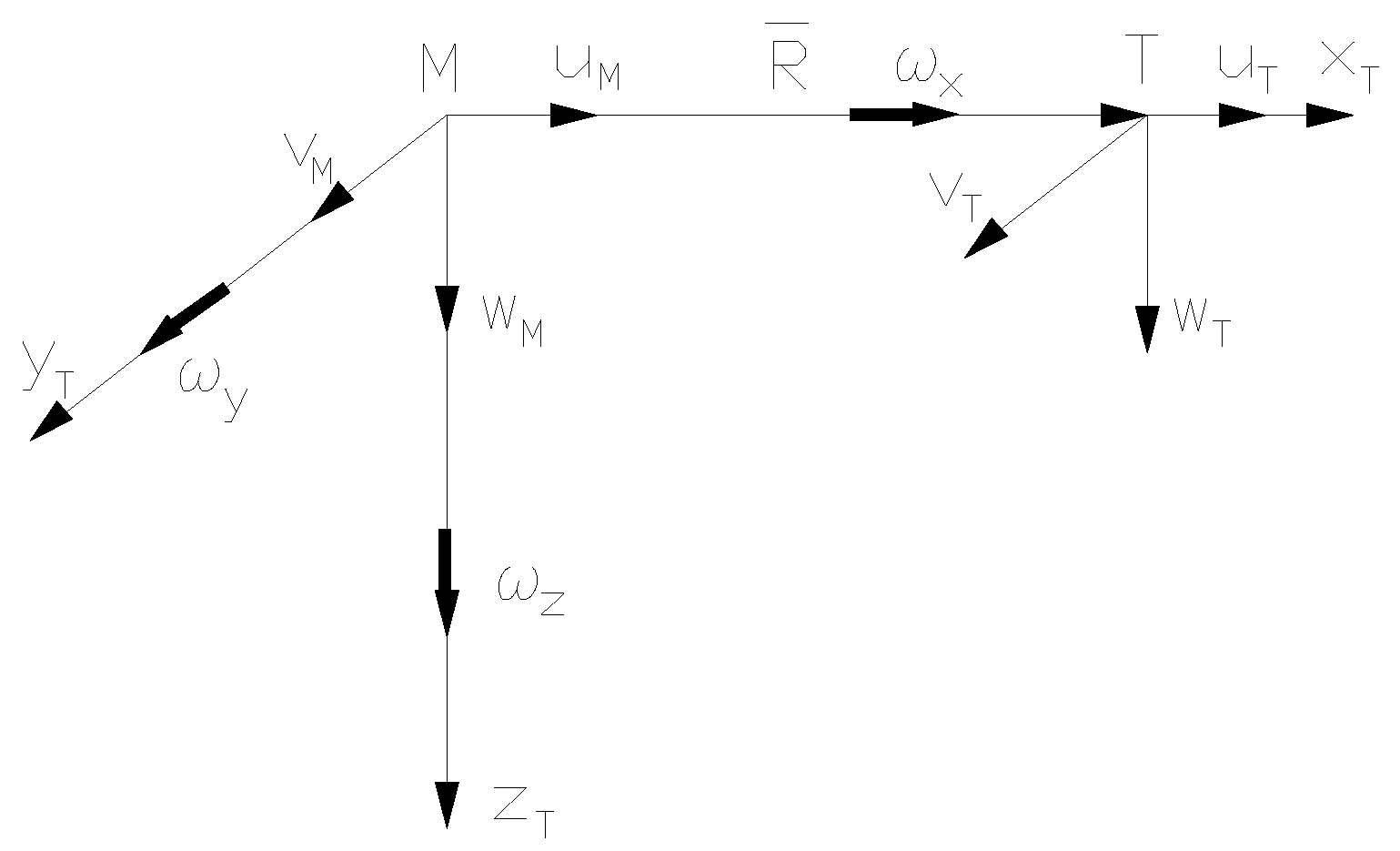
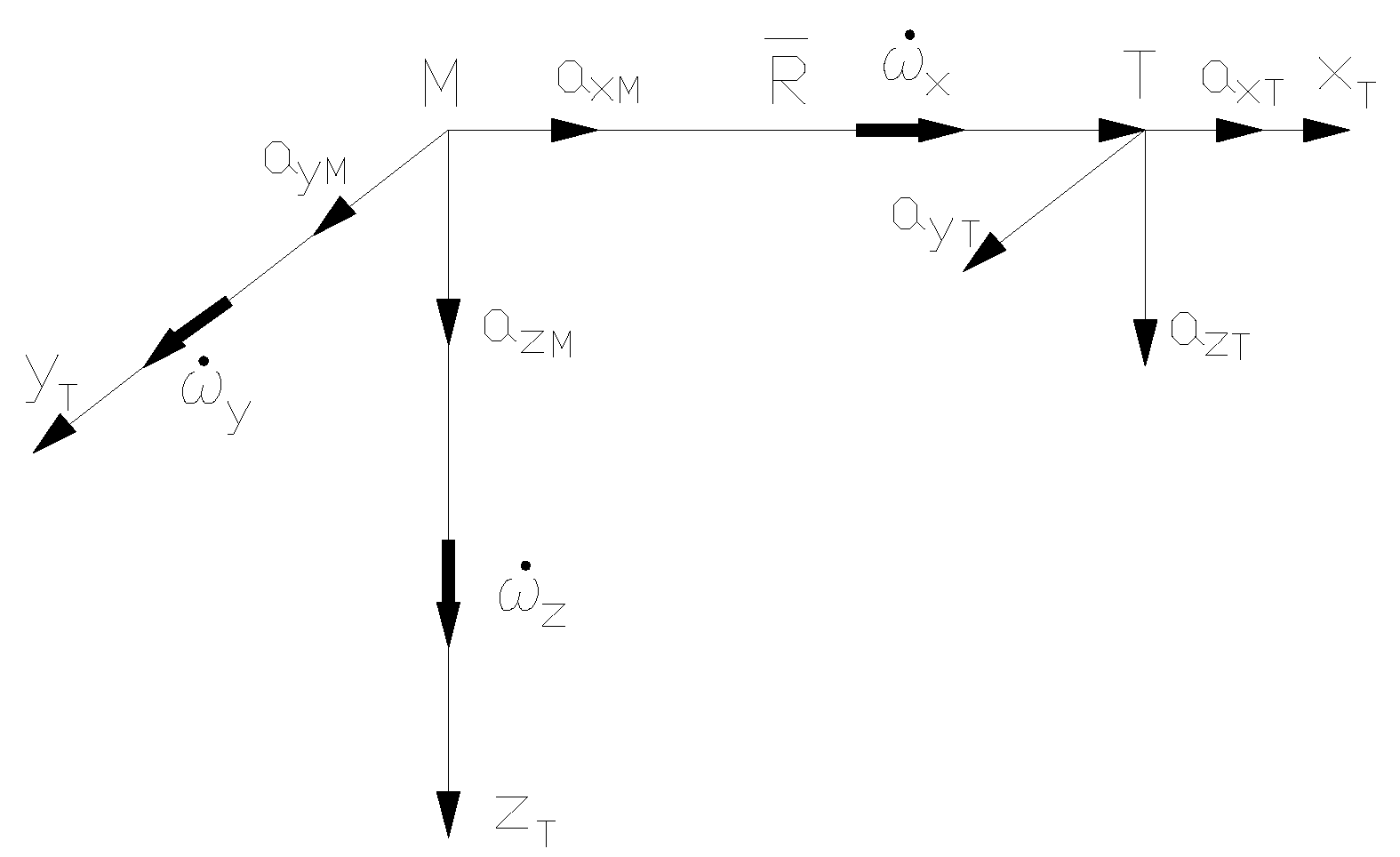
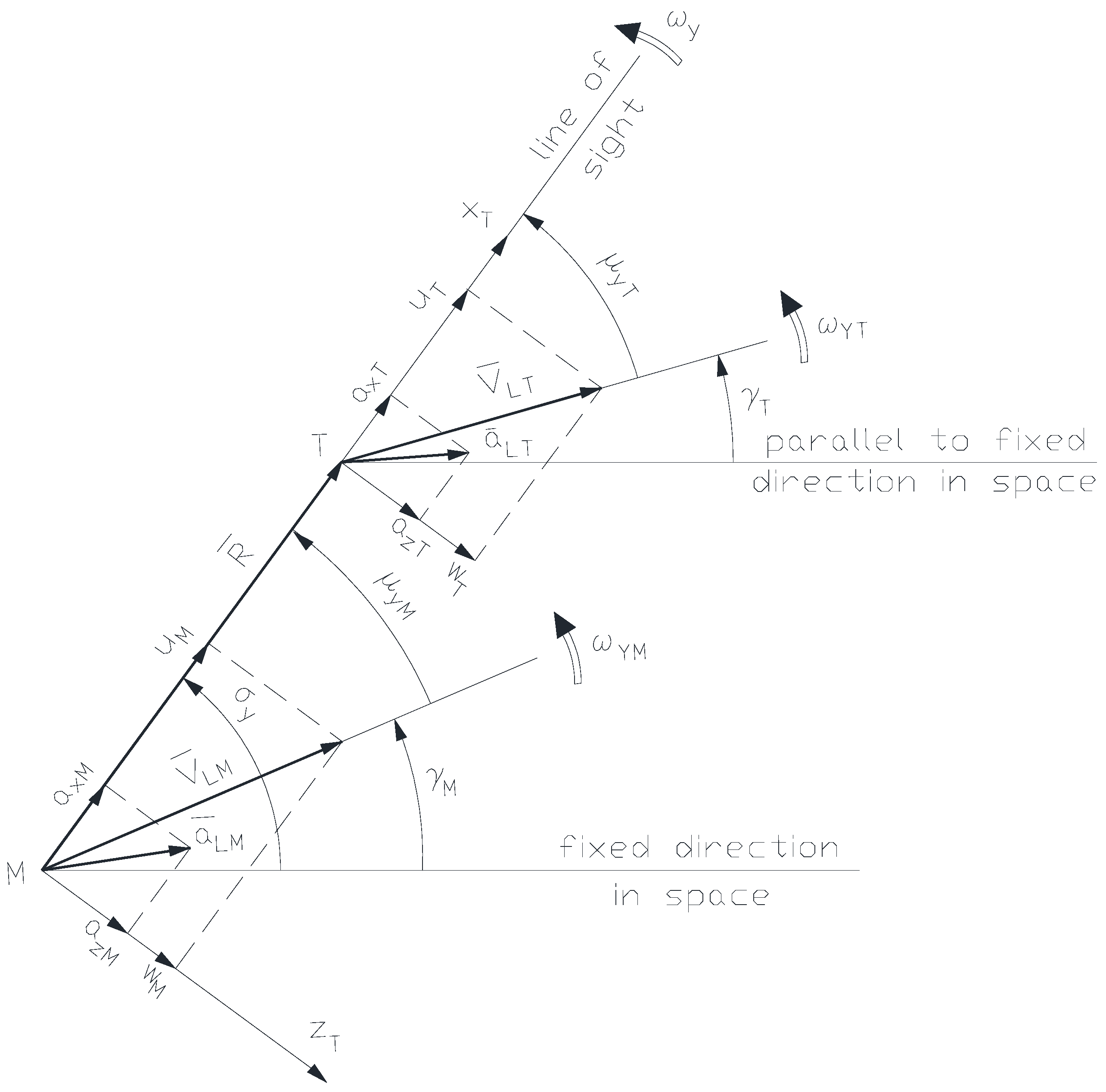
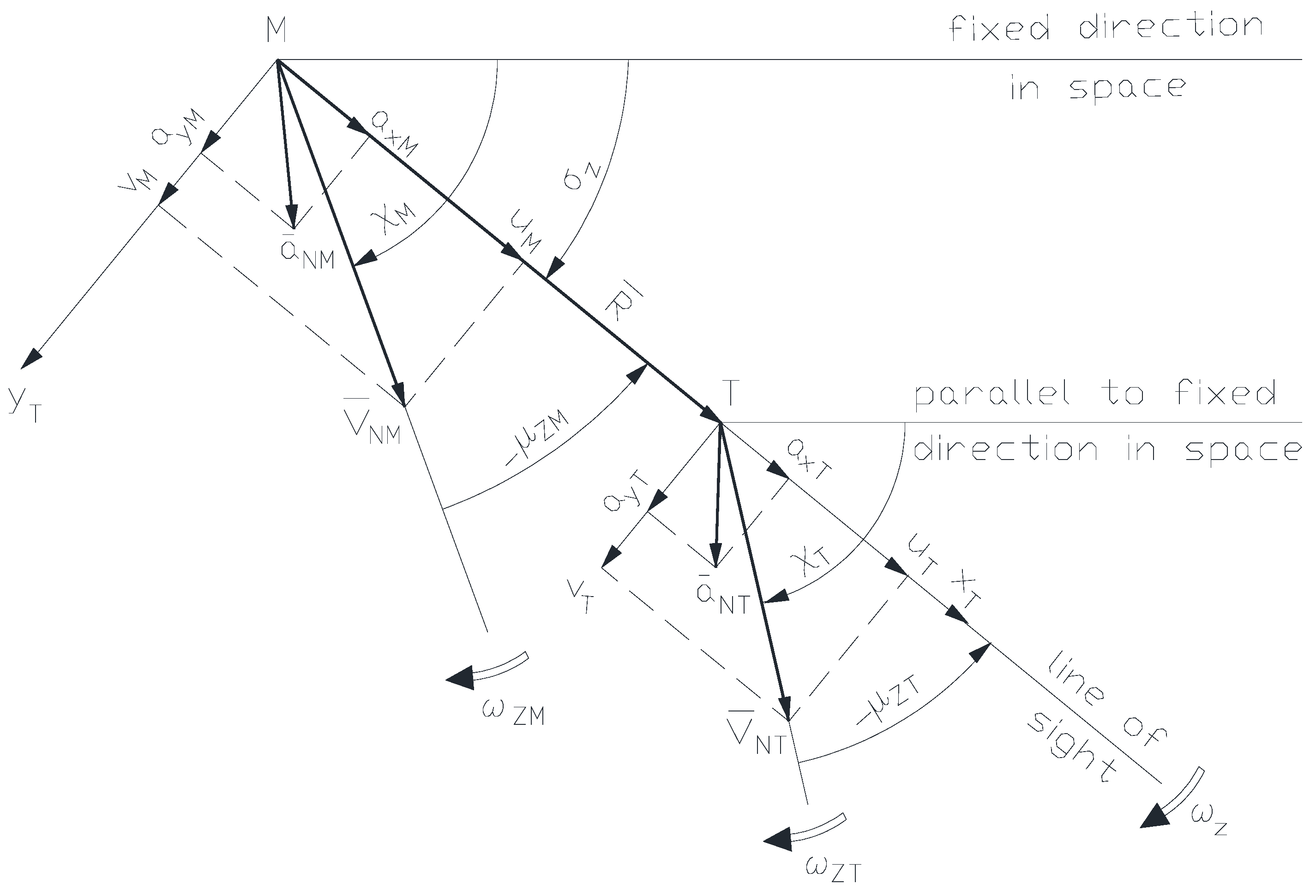
7.2. Linear Form of Kinematic Guidance Equations
7.3. Target Tracker Equations
7.4. The Command for Slow-Rolling Single-Channel Missile
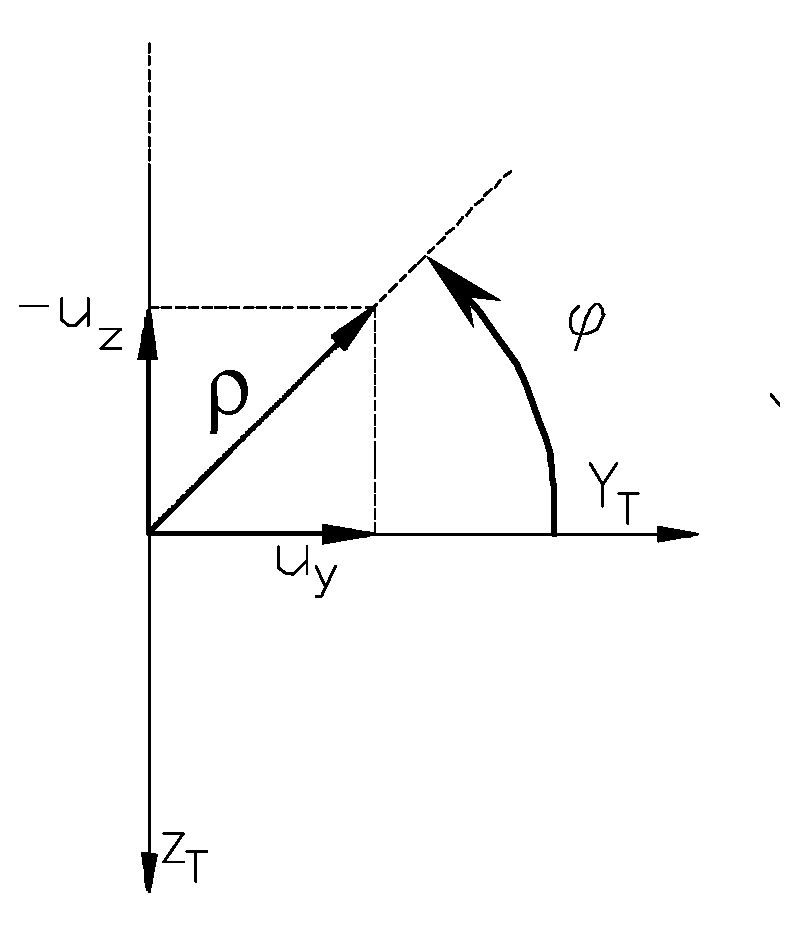
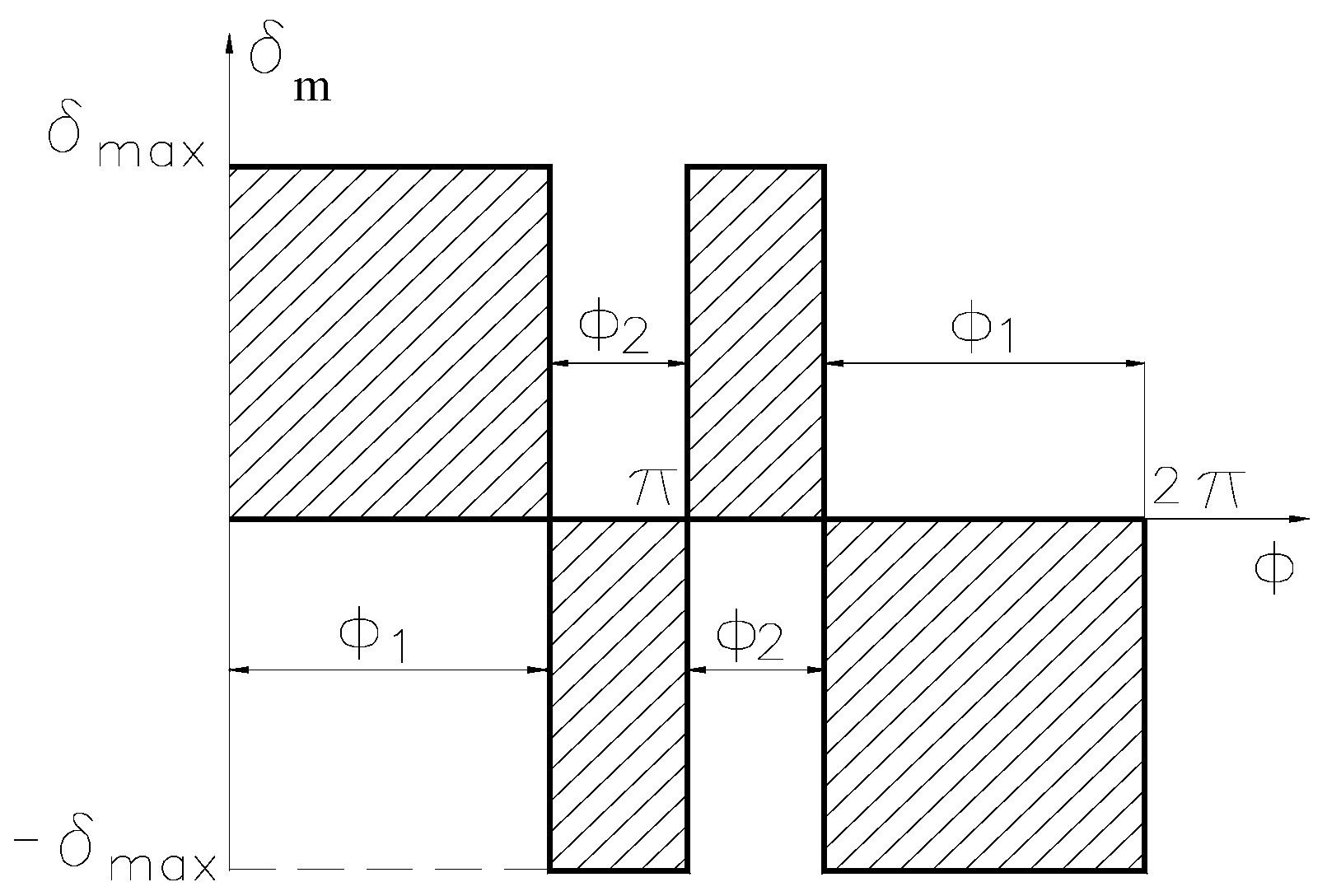
Observation
8. The Guided Flight Stability in the Linear Model
8.1. Determination of Stability Parameters
8.2. Non-Rotational Case Analysis
9. Calculus Model
9.1. Aerodynamic Characteristics
9.2. Mechanical and Reference Characteristics
9.3. Thrust Characteristic
9.4. Time Constants and Controller Gains
- Constant navigation indicated as the optimal value for the PN method [28];
- The time constant for the target tracker ;
- Time to reach the target starting from the second phase of flight ;
- Rolling rotational velocity close to that indicated in Section 10.4 ;
- Calculation altitude ;
- Phase shift .
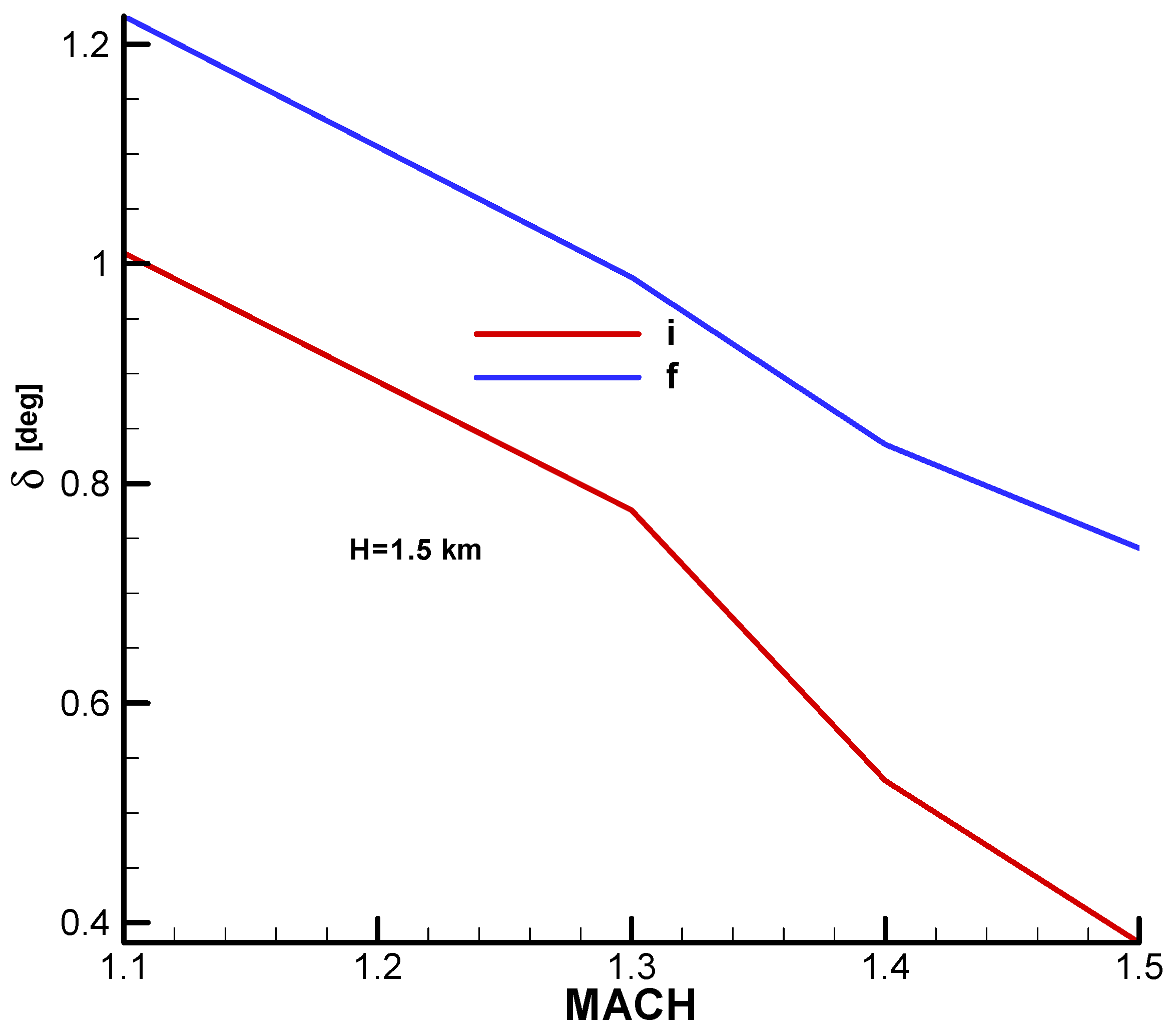
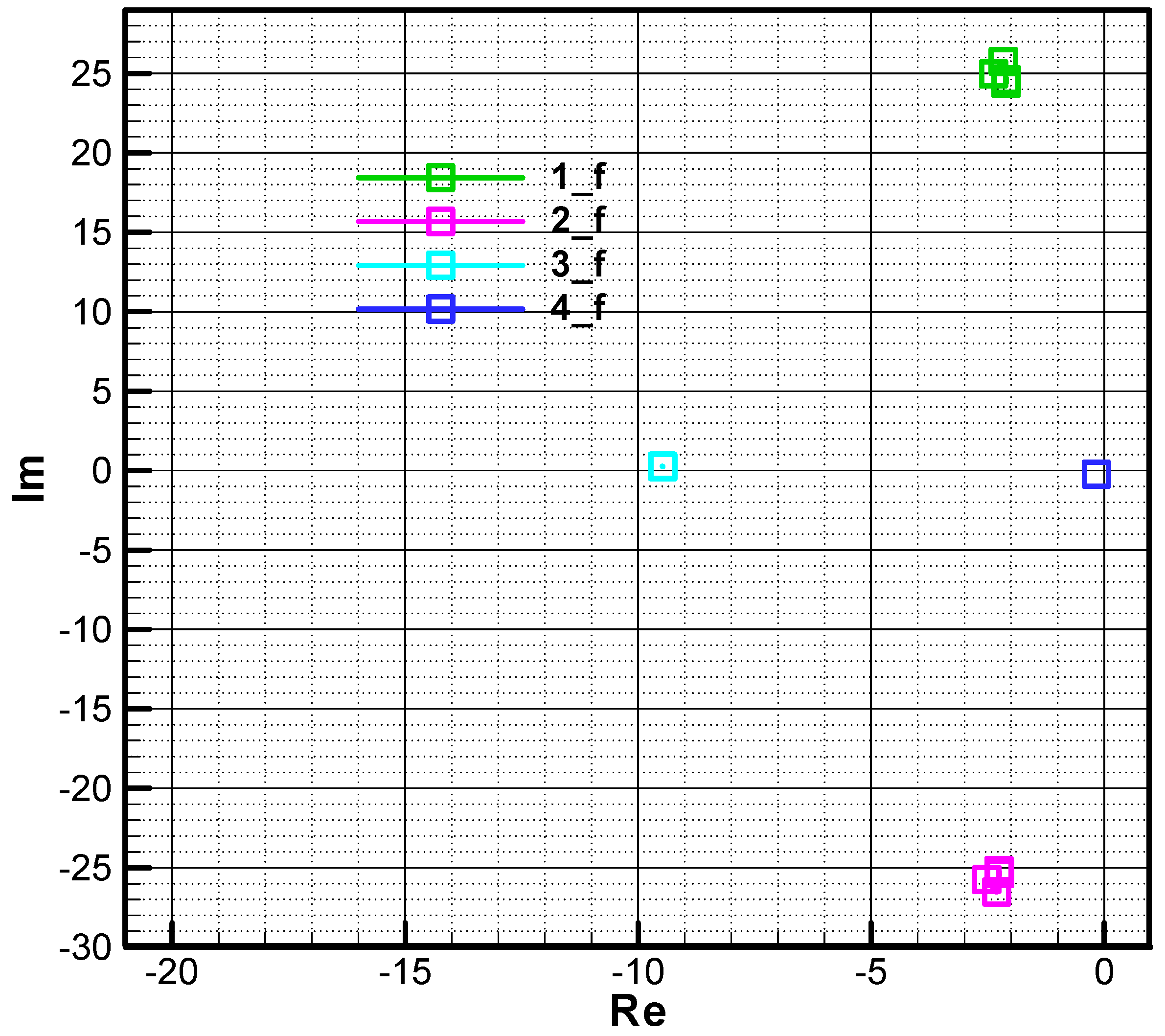
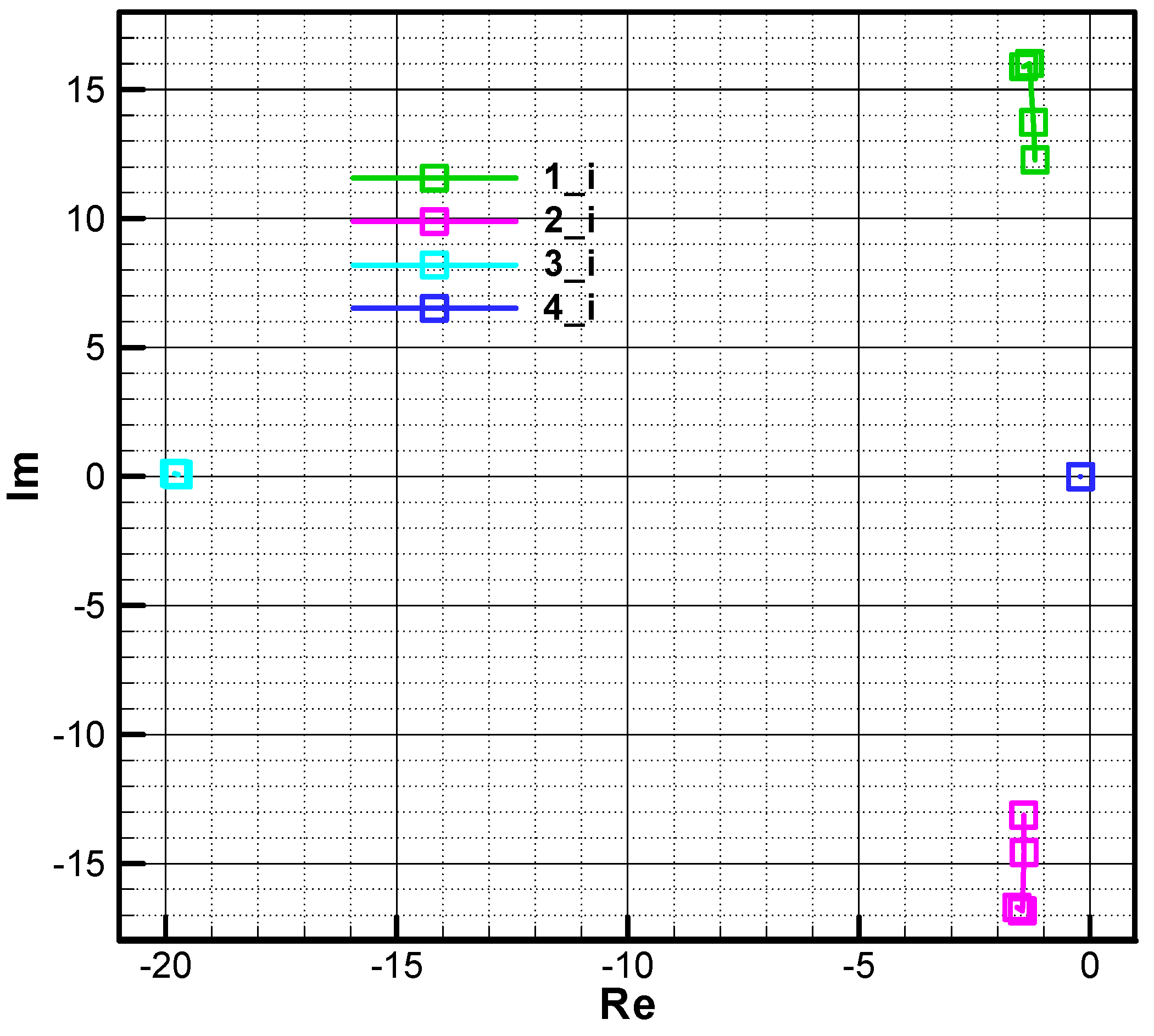
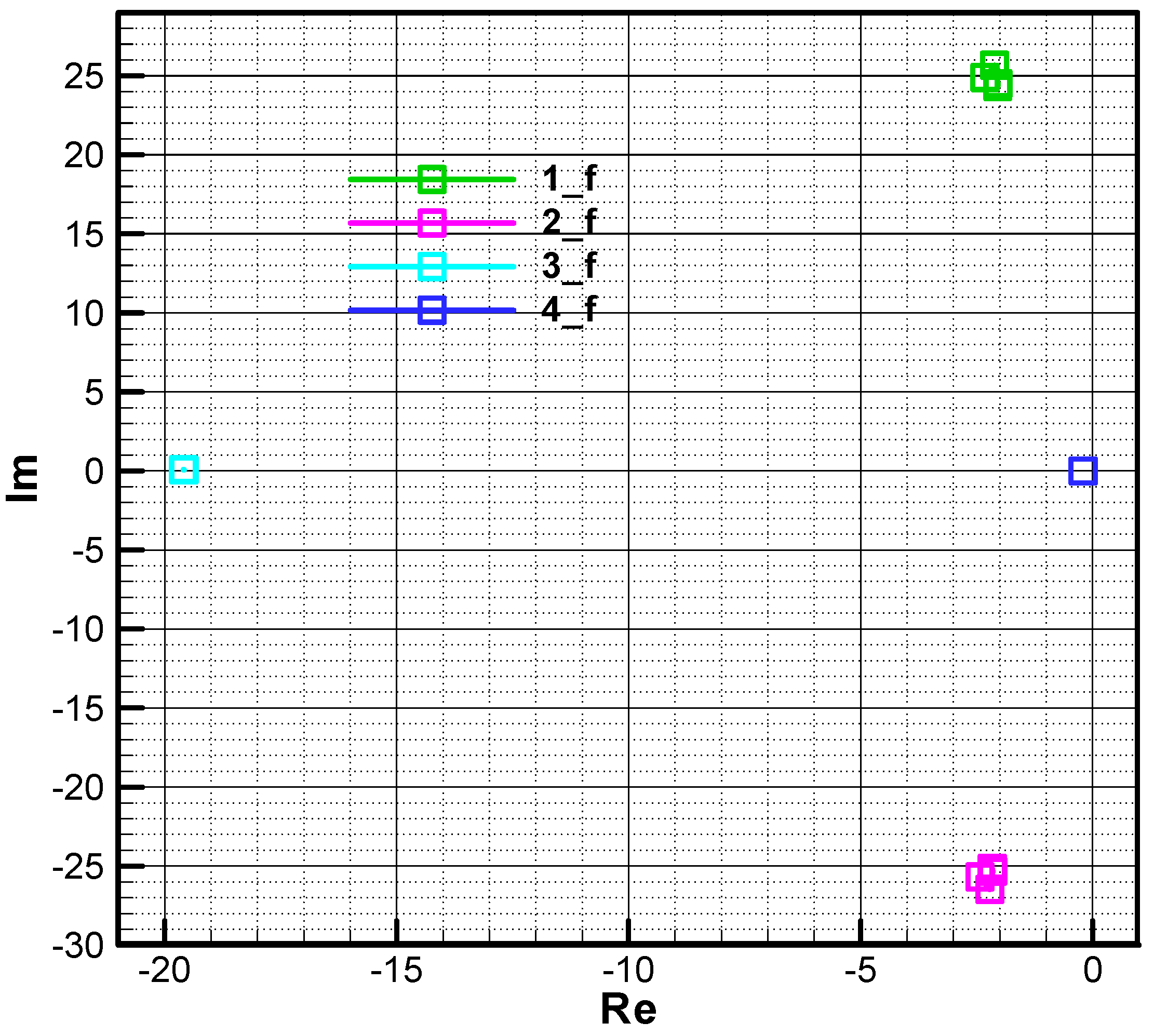
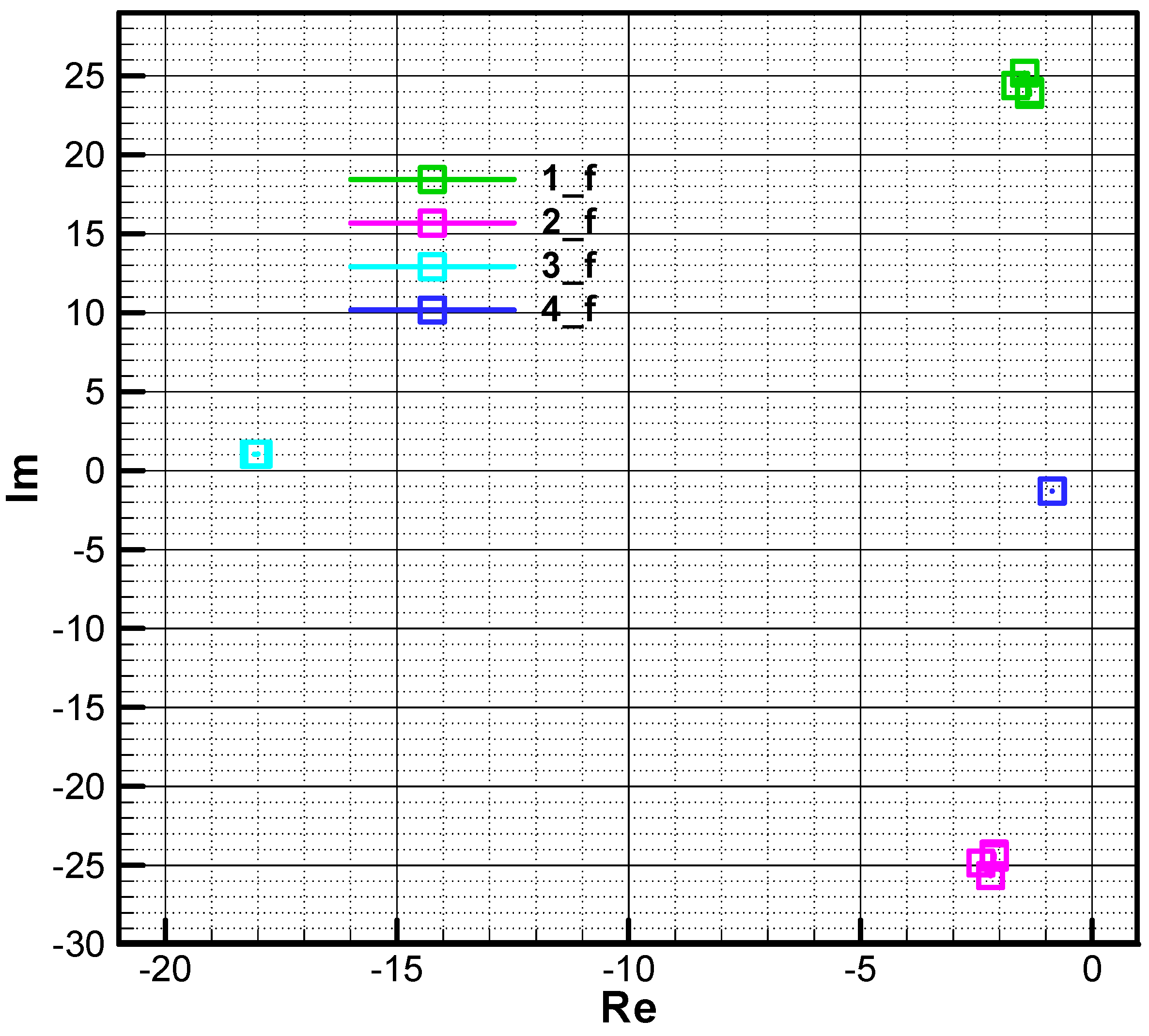
10. Stability Analysis of the Single-Channel Homing Slow-Rolling Missile
10.1. Organization of Results
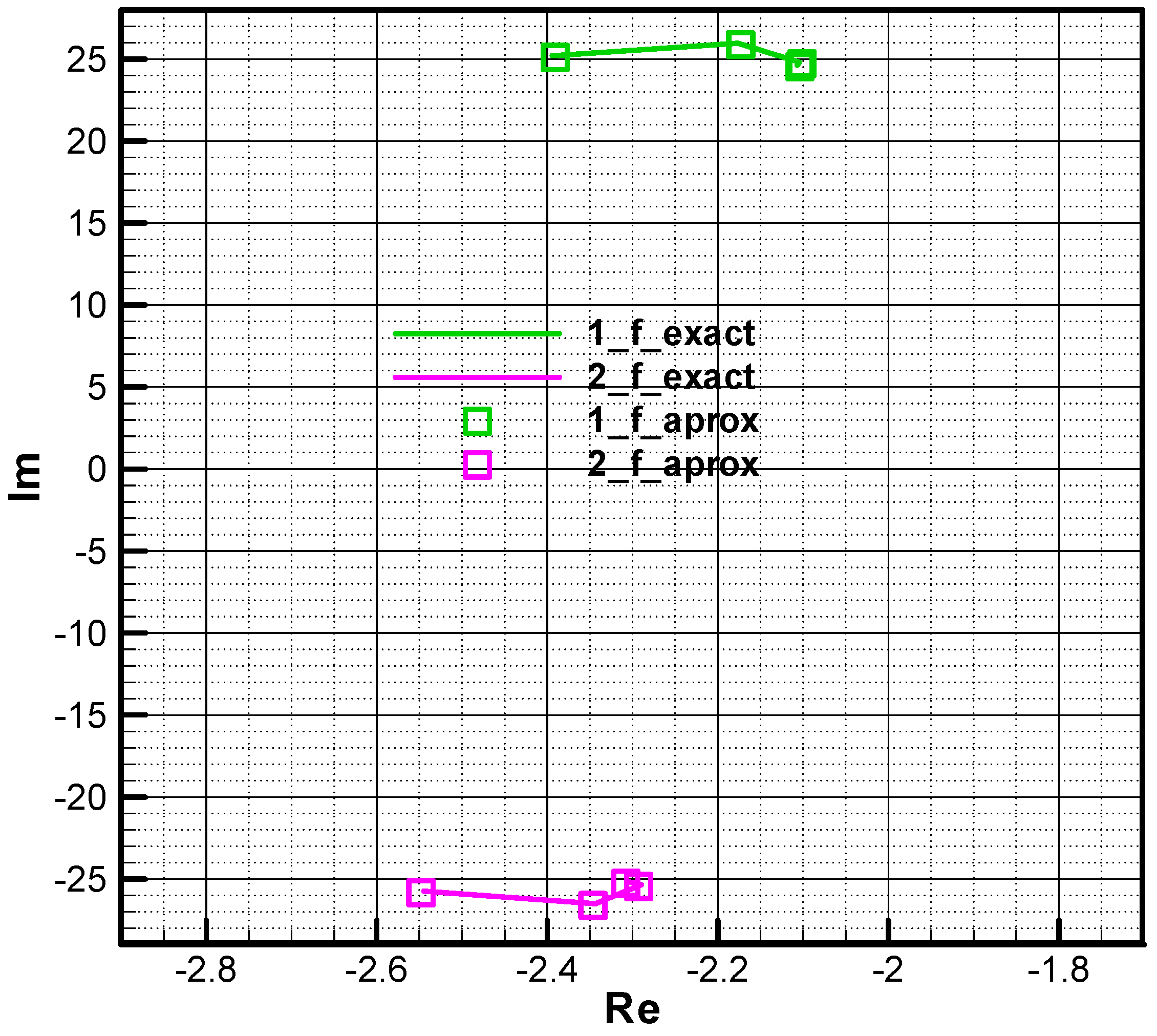
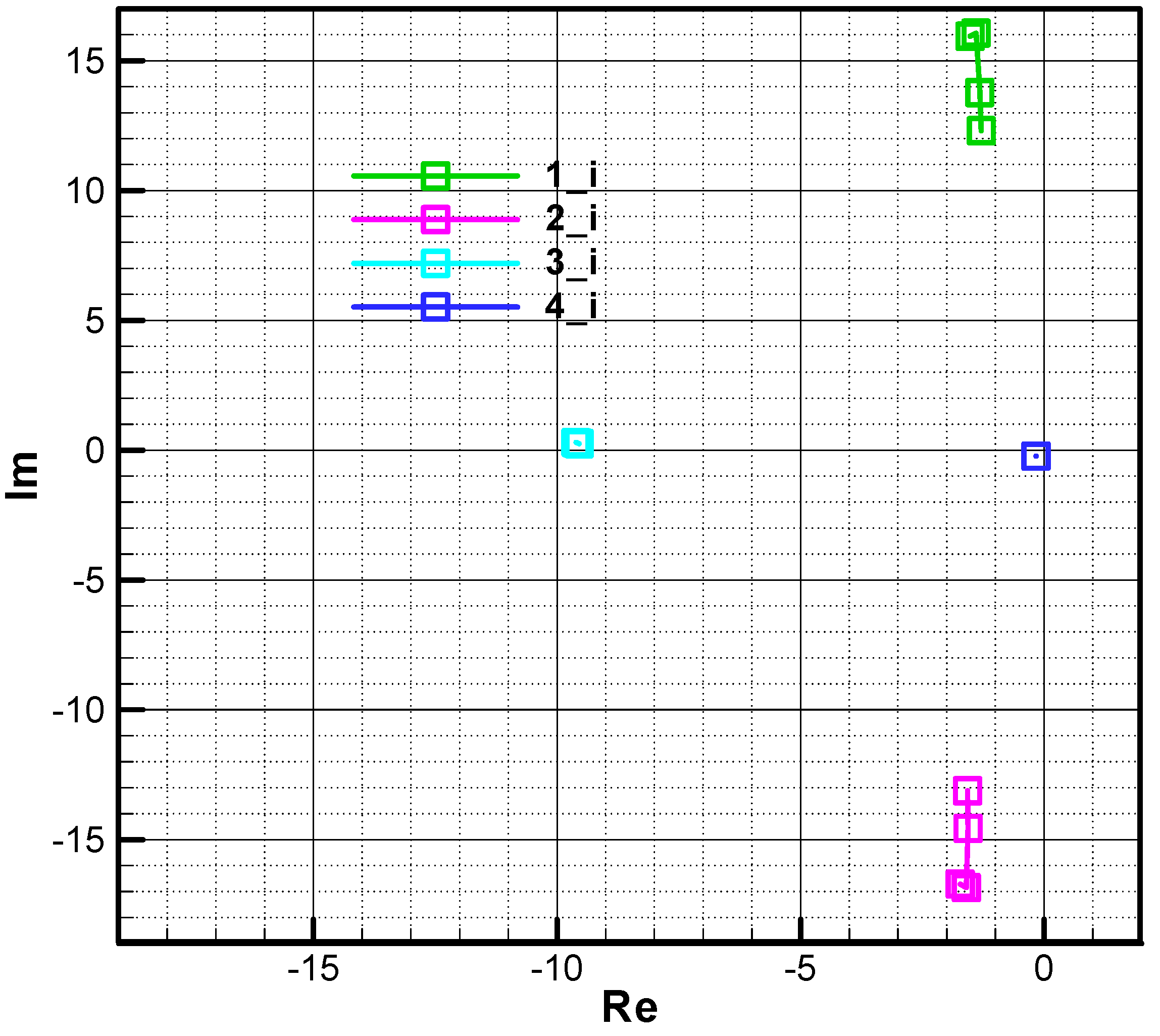
10.2. The Root Locus
- -
- -
- Root 3 is large in the module, with a negative real part and a small complex part, due to the target tracker response time.
- -
- Root 4 is small in the module, with a negative real part, with a small complex part due to the guidance loop.
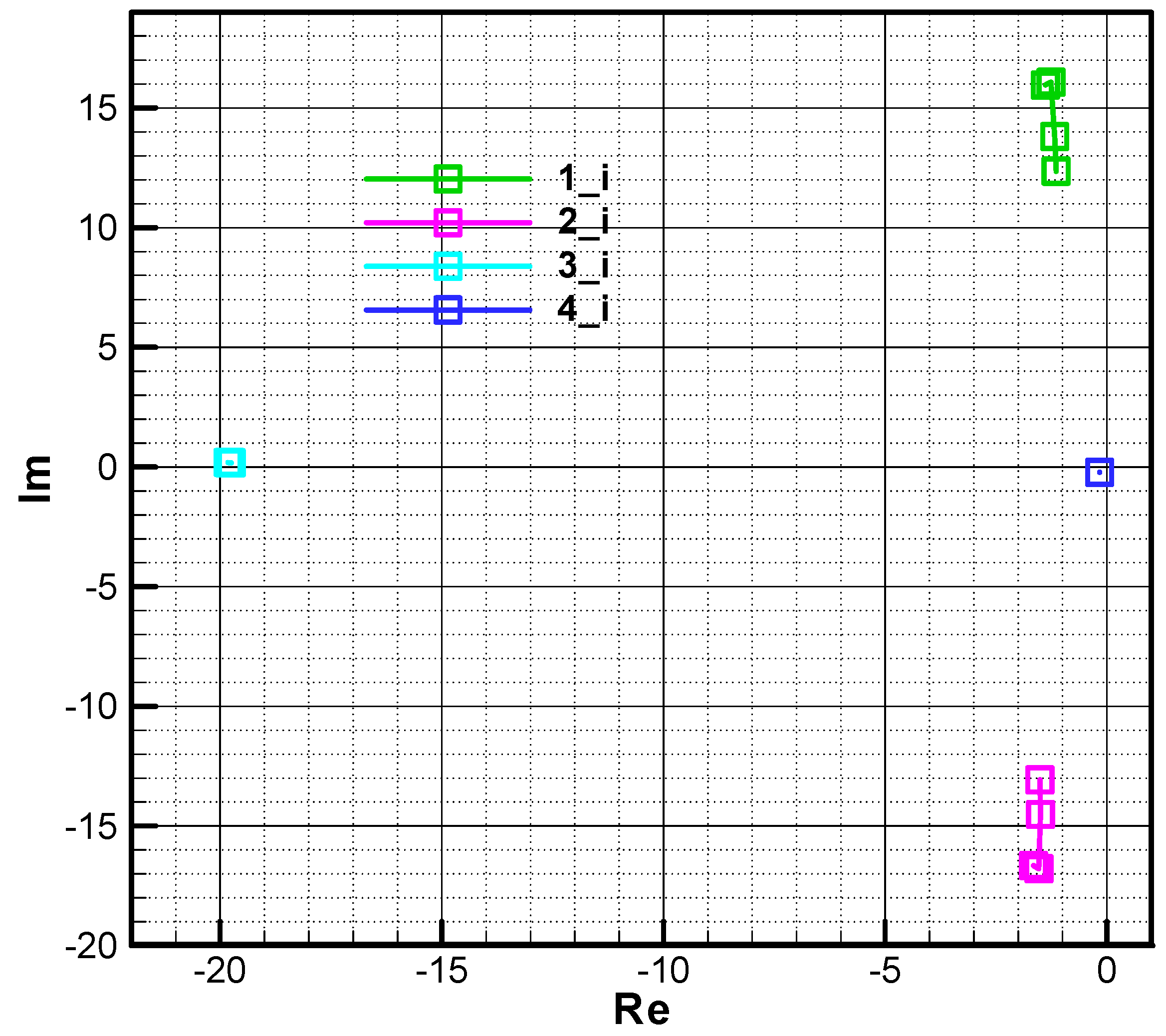
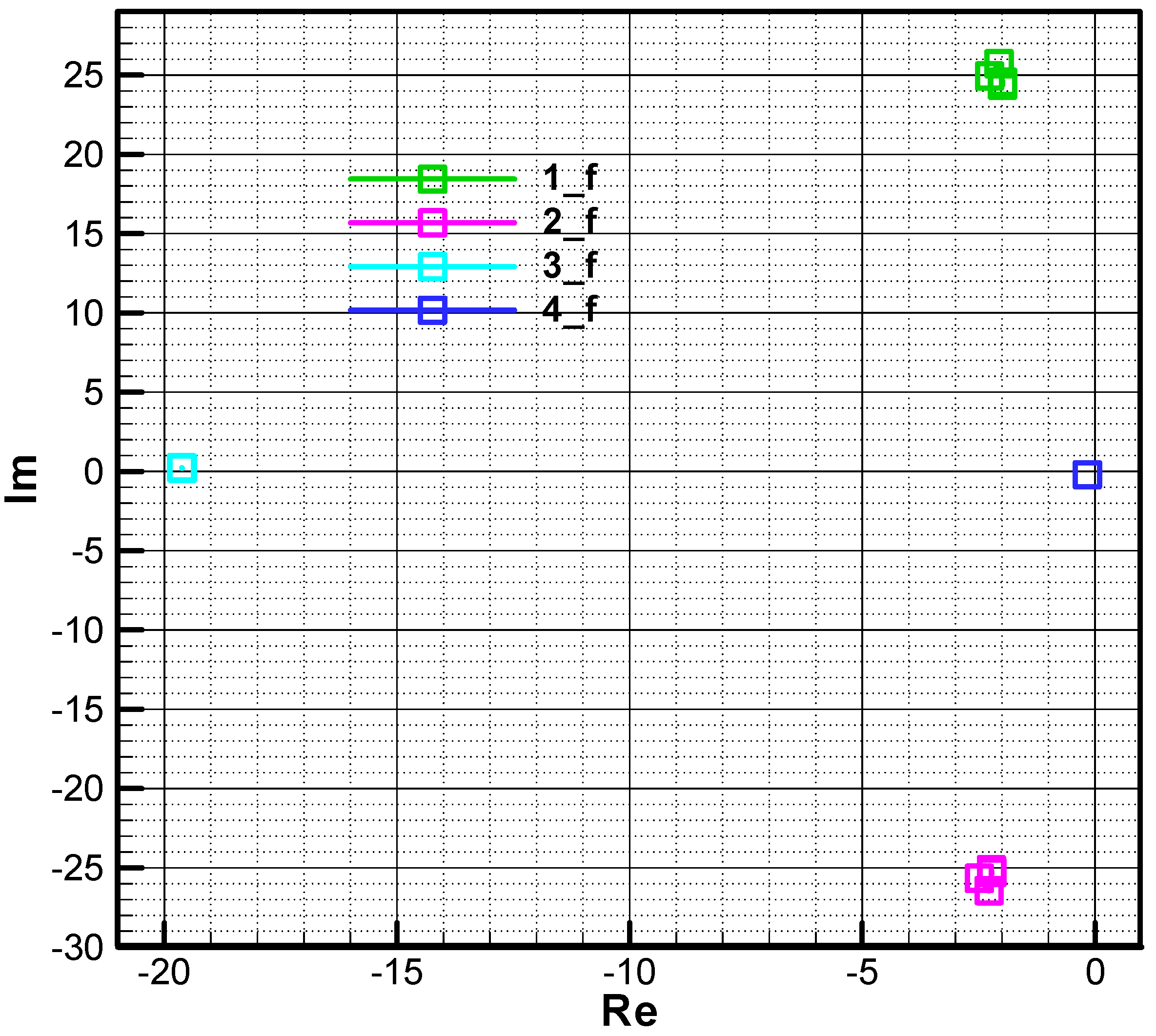
10.3. Constraints Due to Stability Conditions
10.3.1. Time to Hit the Target
10.3.2. Navigation Constant
10.4. Stability Analysis Based on the Nonlinear Model

11. Conclusions
12. Notations
Reference Frames
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
Appendix A. Aerodynamics

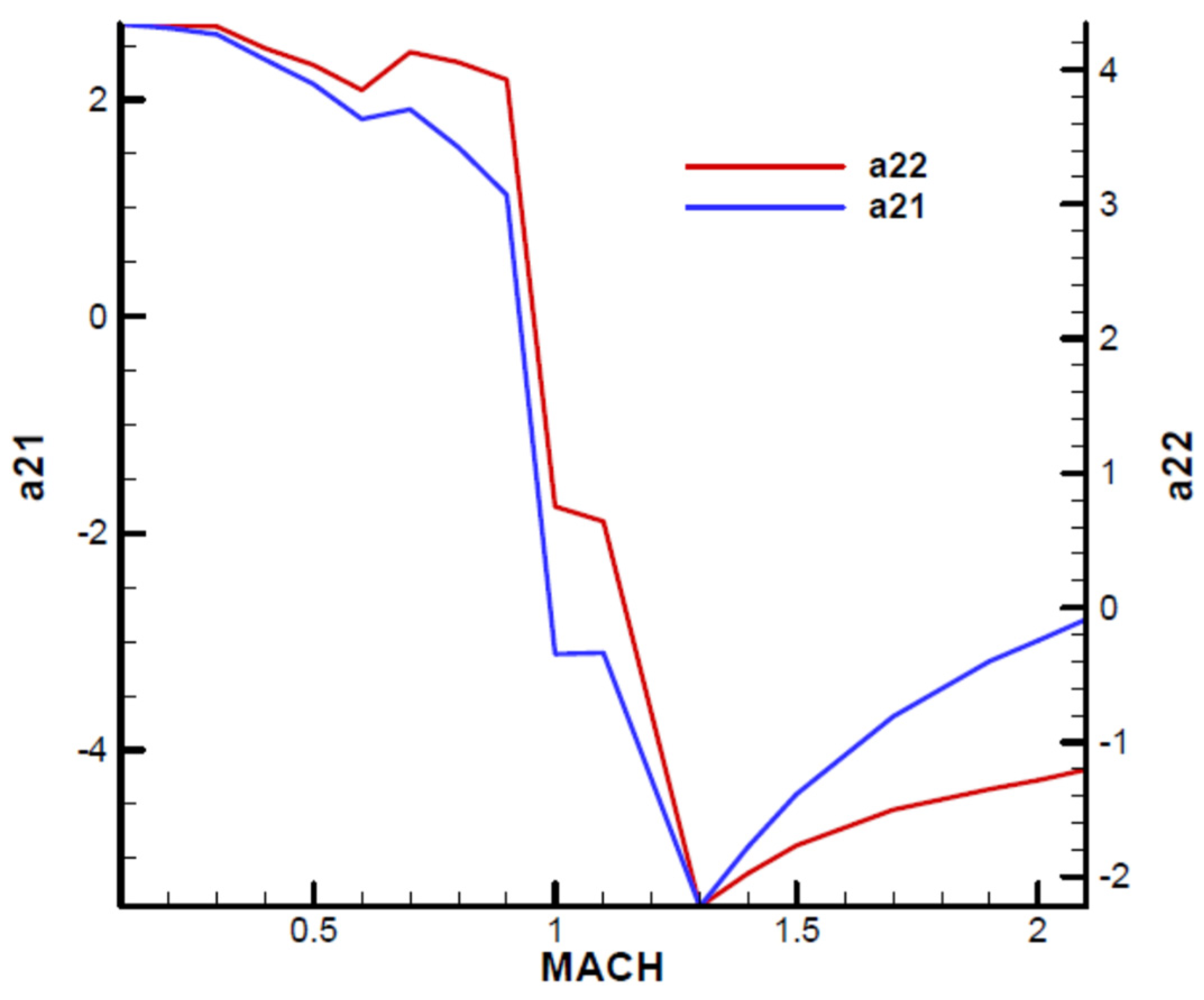
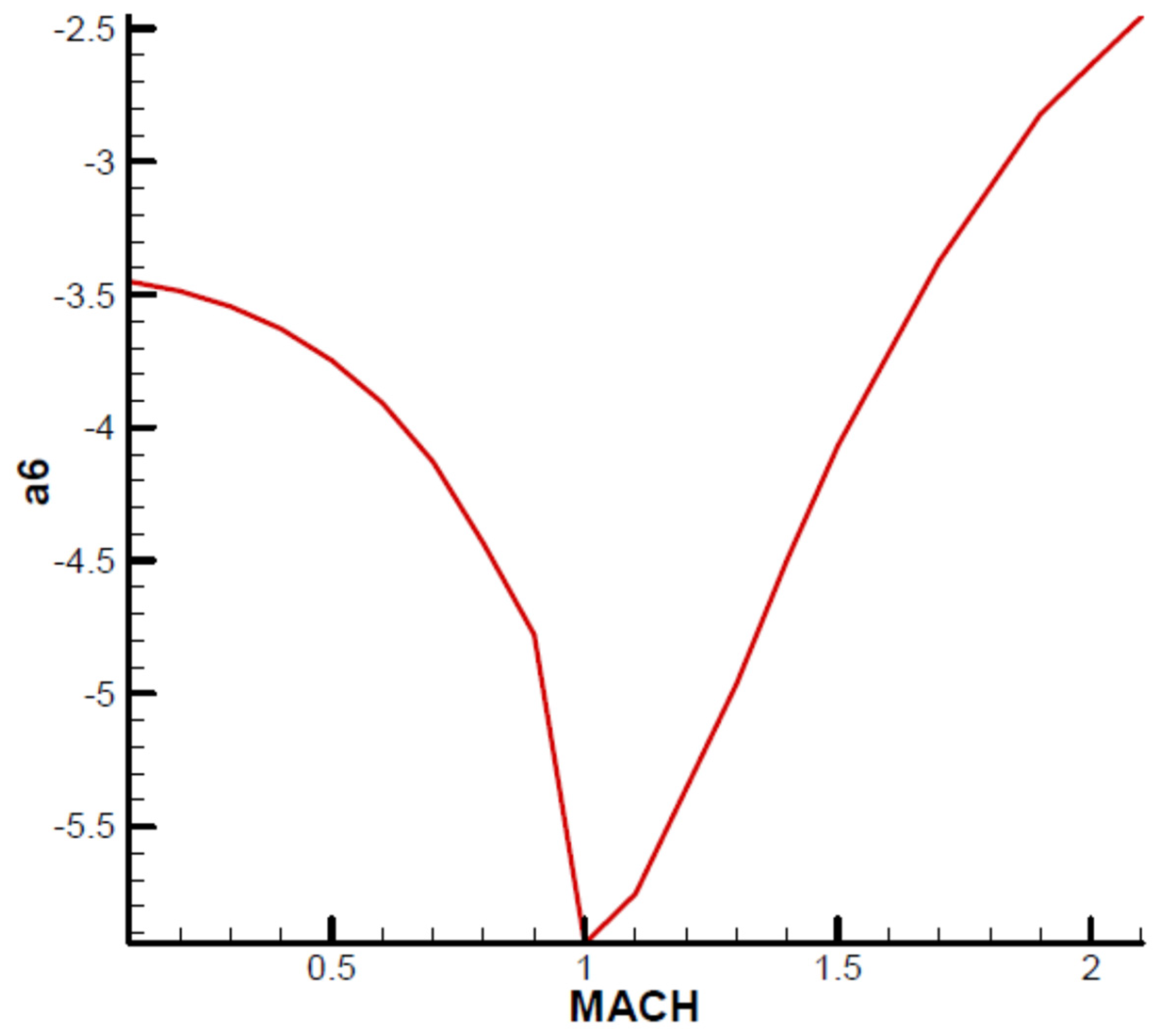
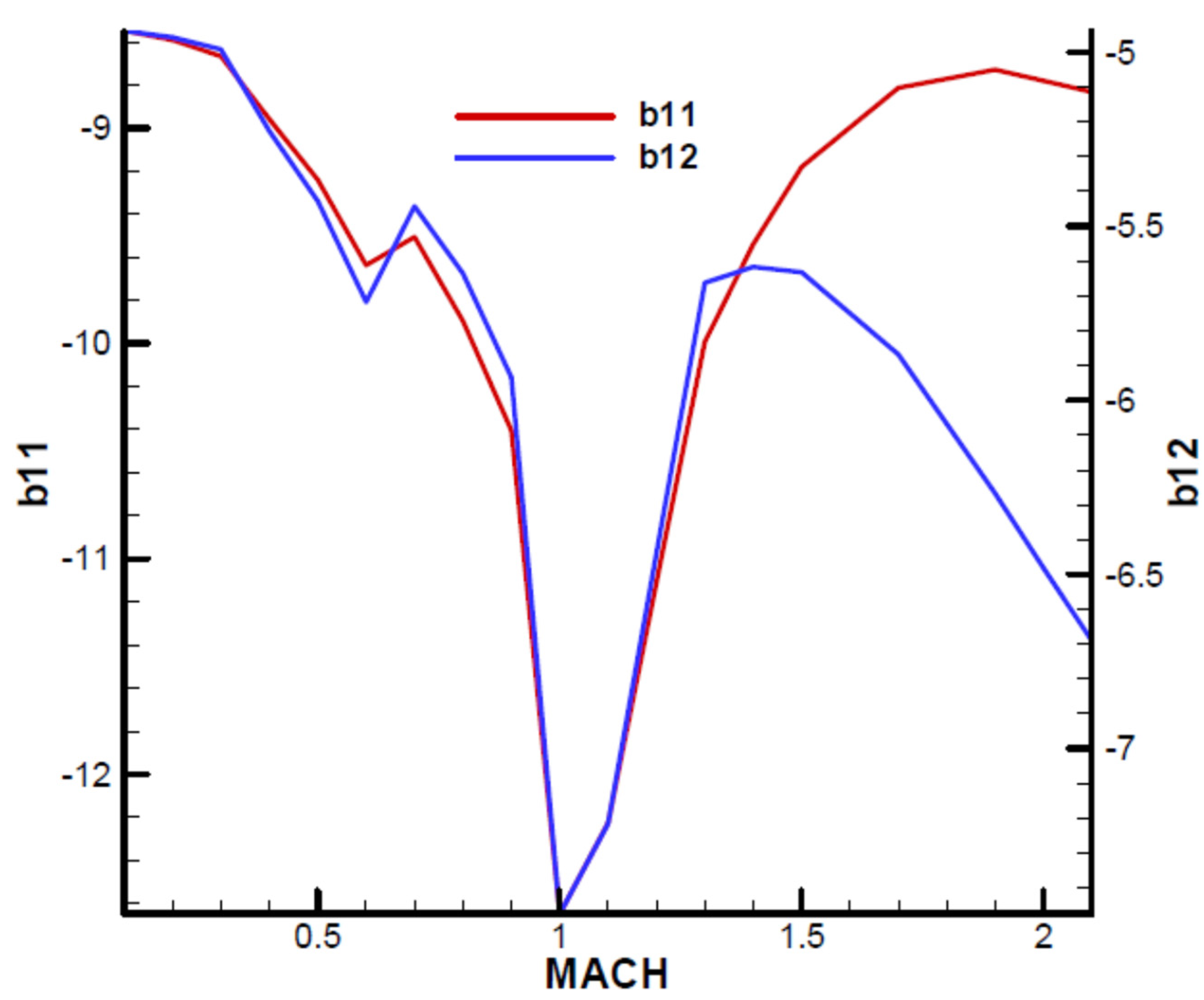

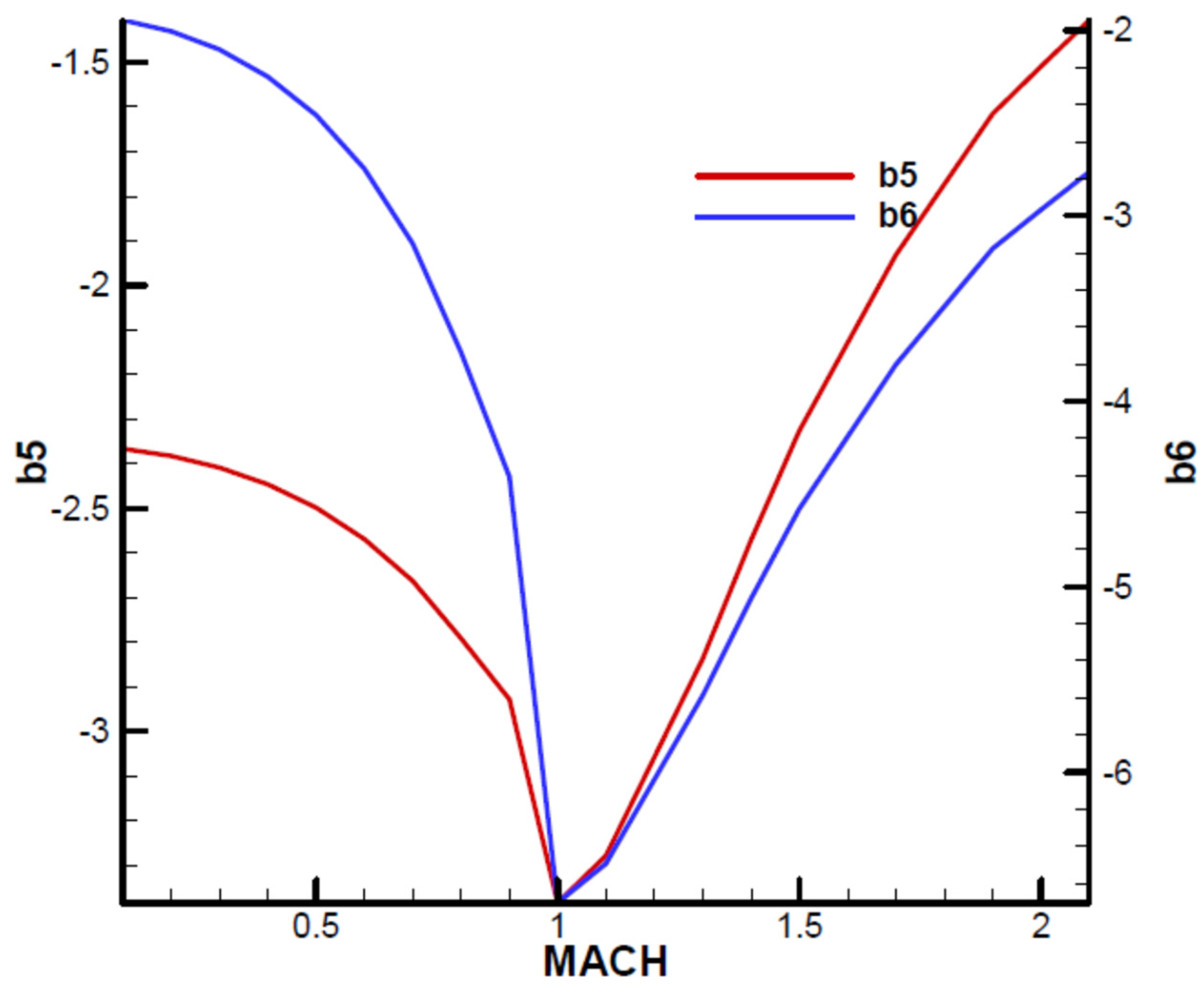

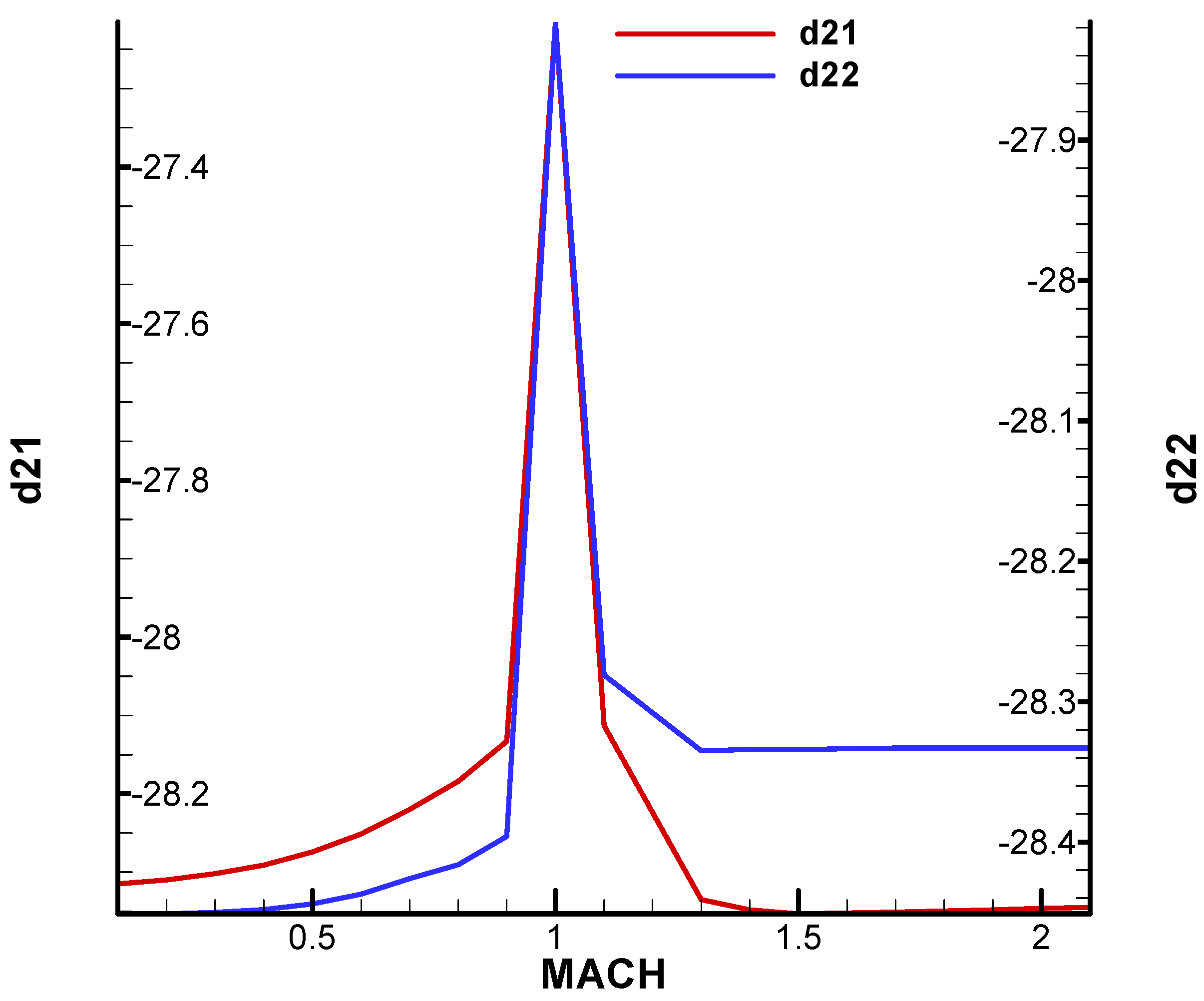
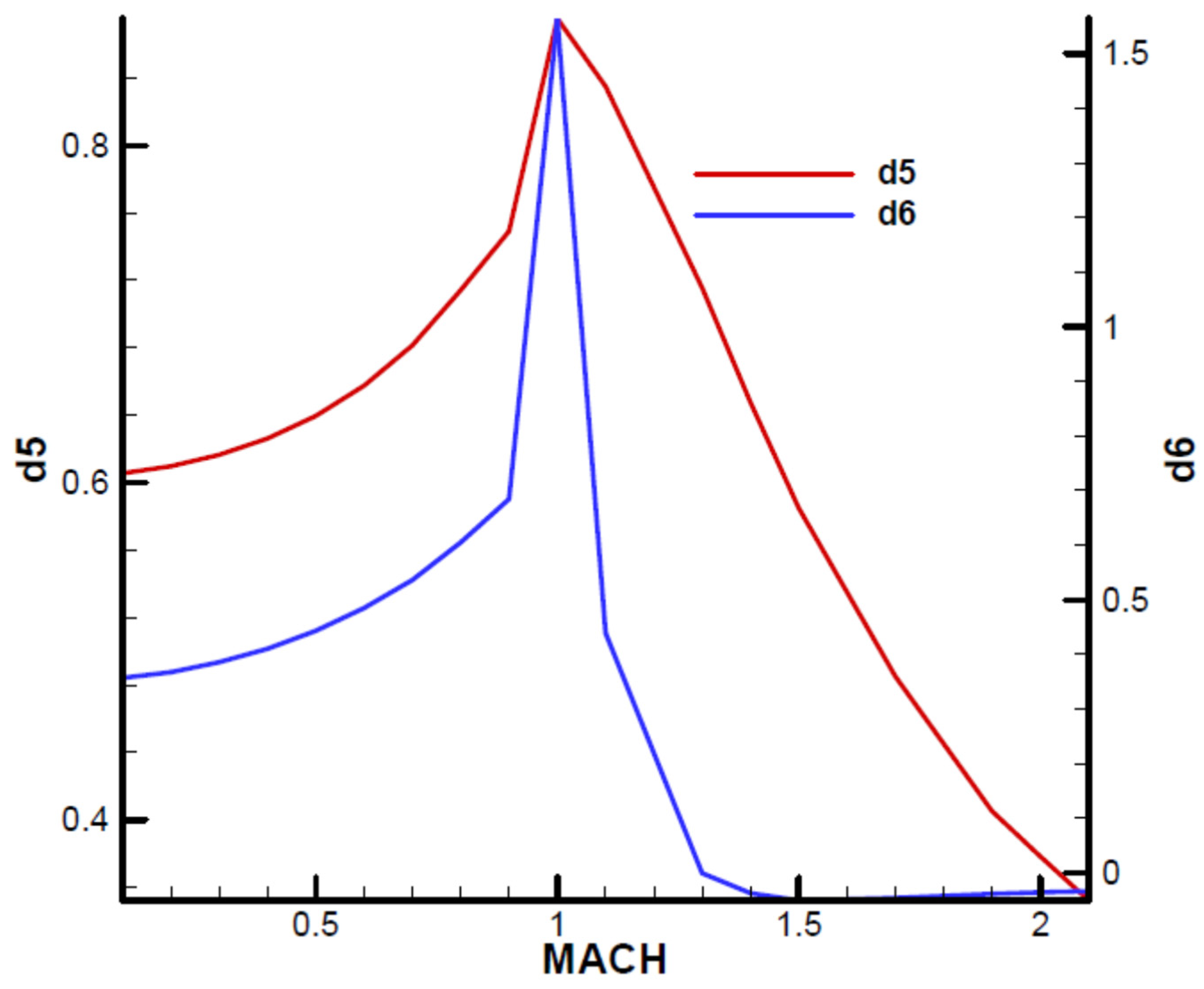
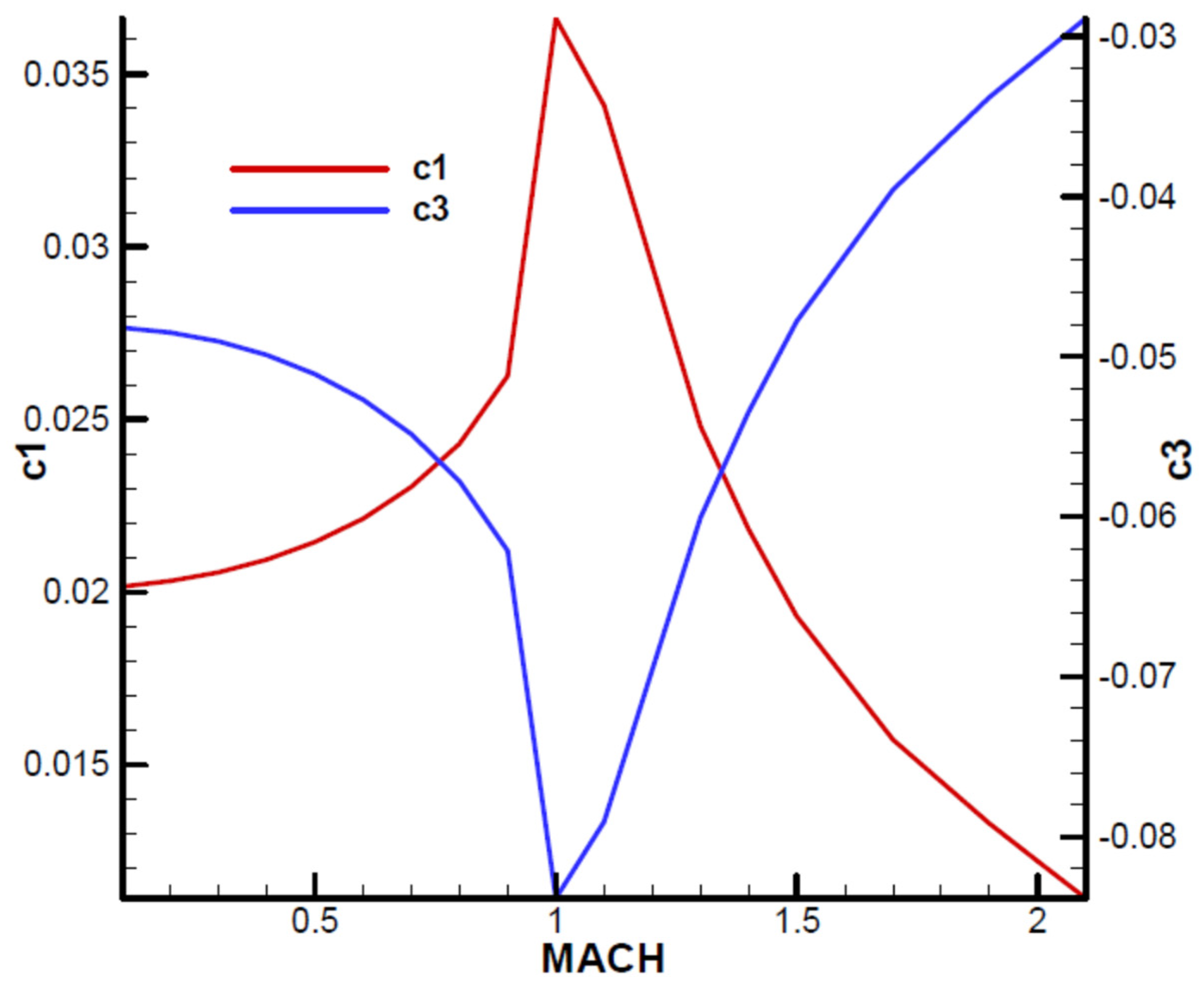
Appendix B
Appendix B.1. Basic Movement
Appendix B.2. Stability Matrix





Appendix B.3. Command Matrix
Appendix B.4. Flight Quality Parameters


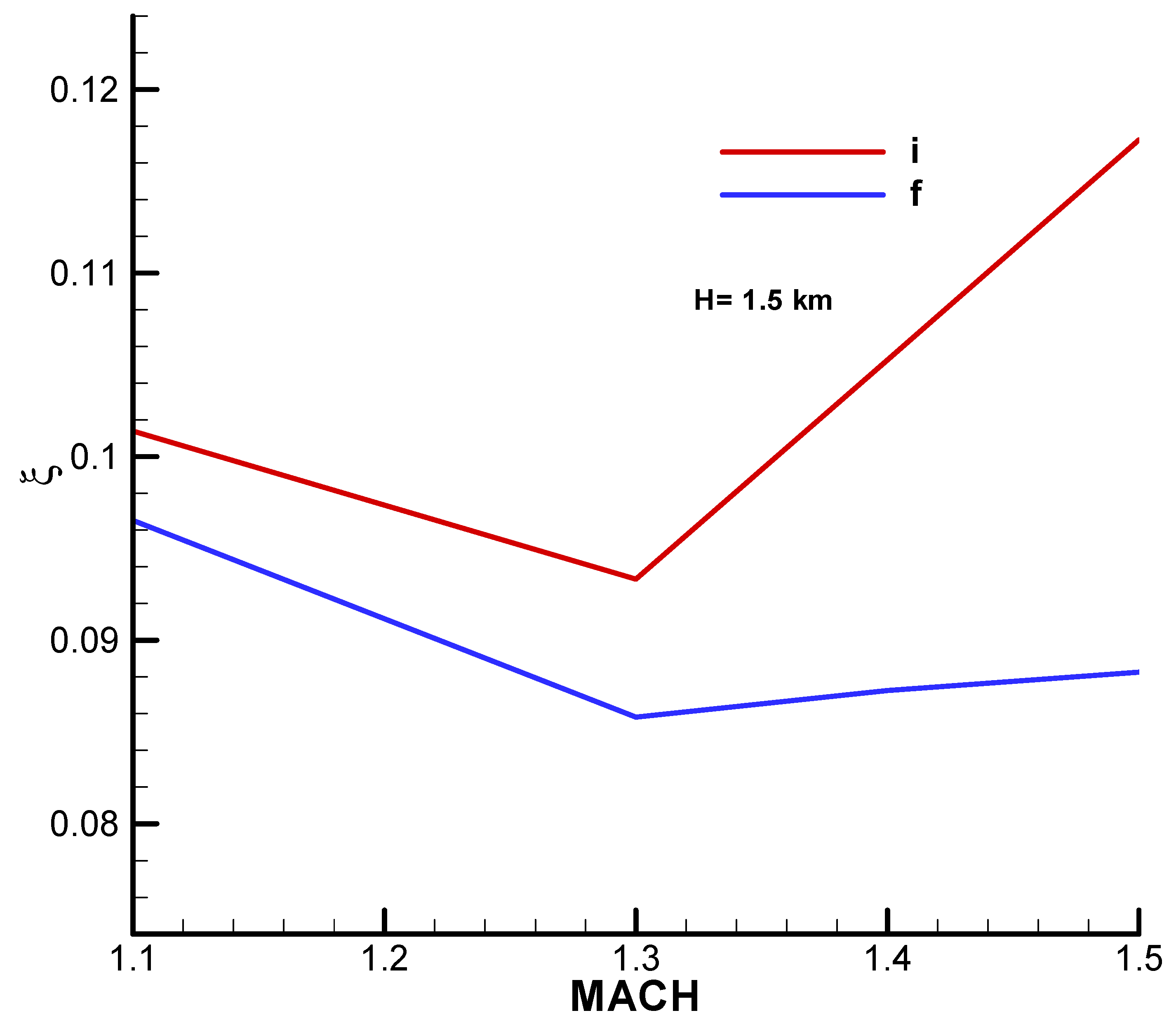
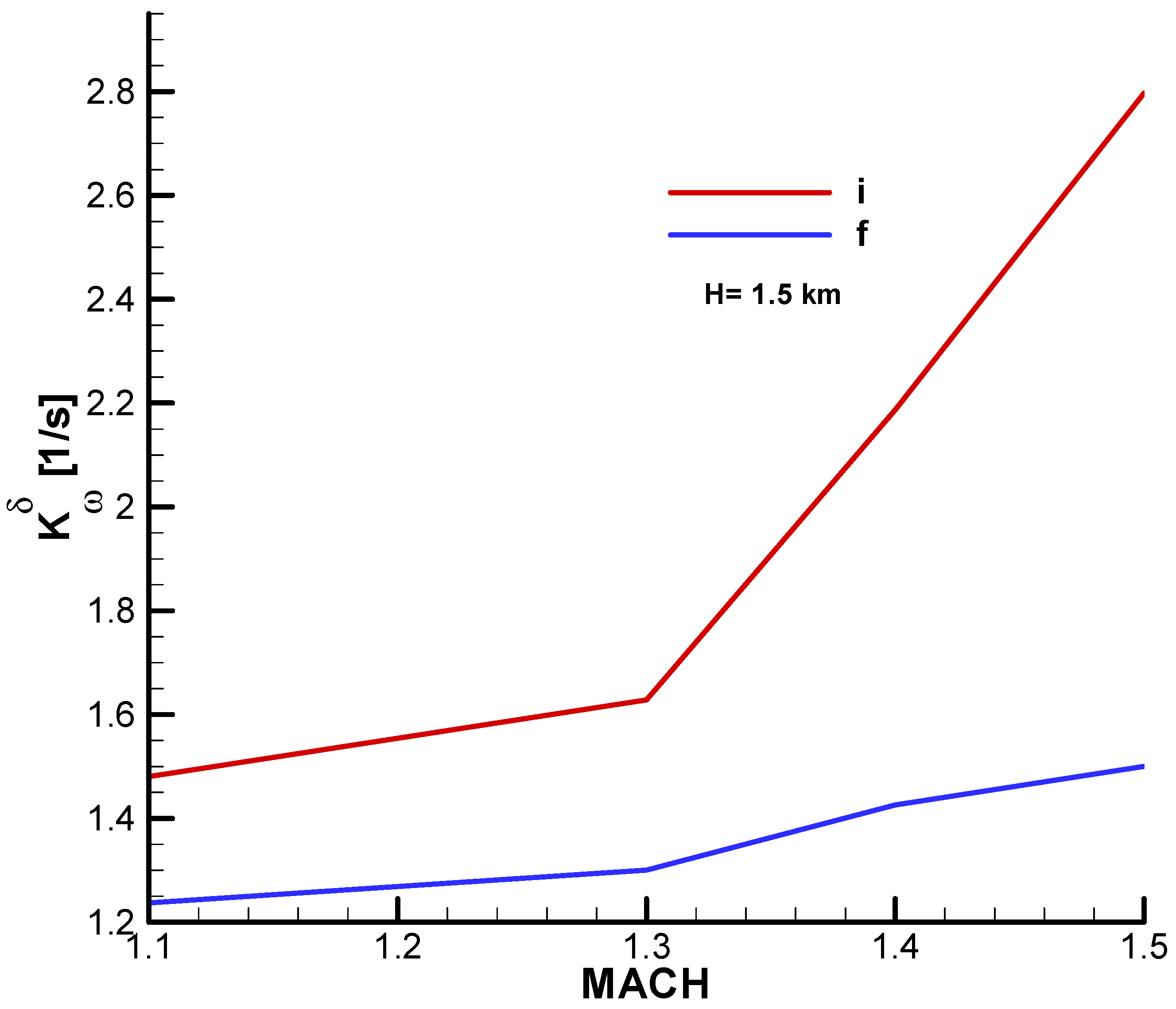


Appendix C
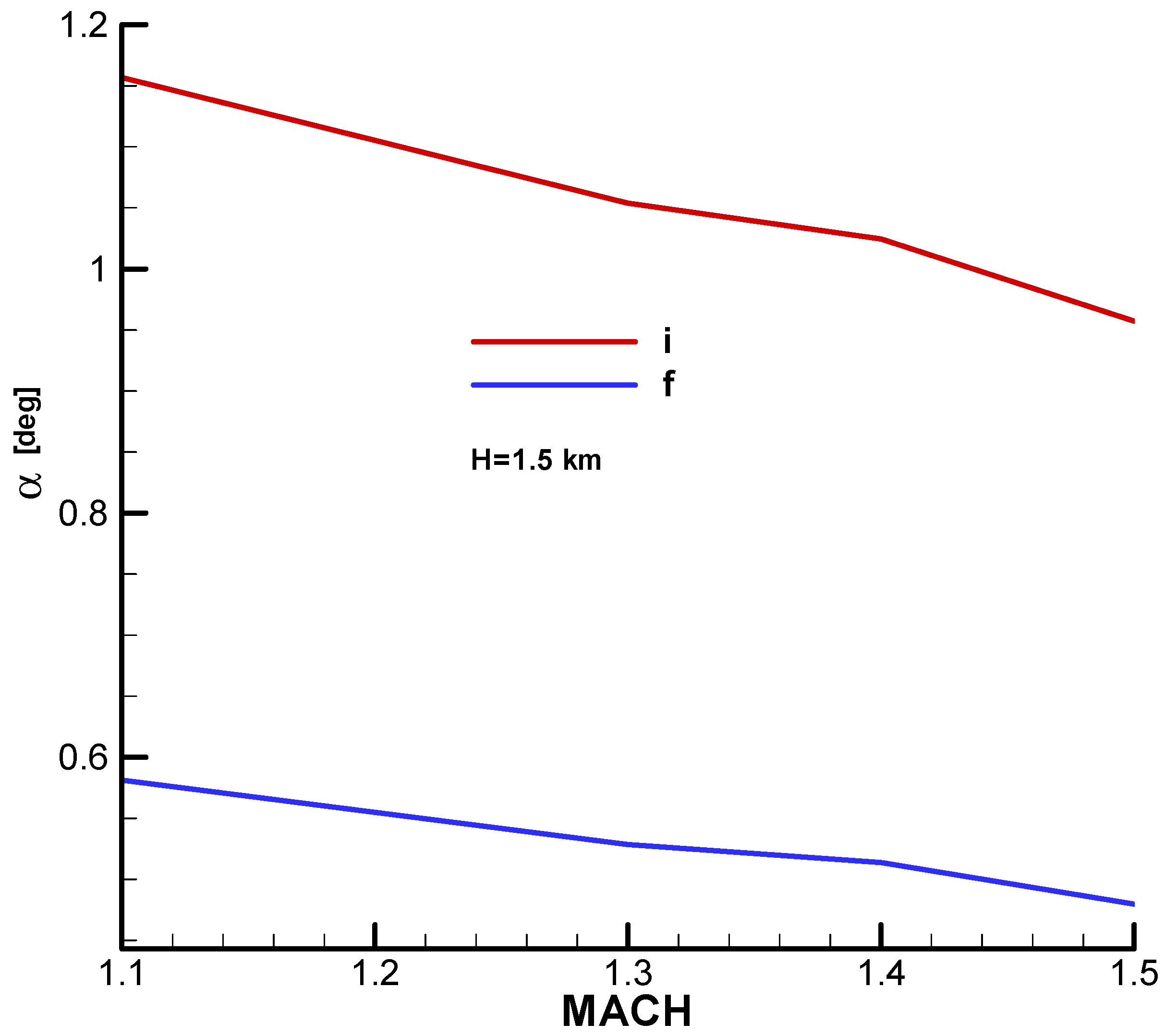
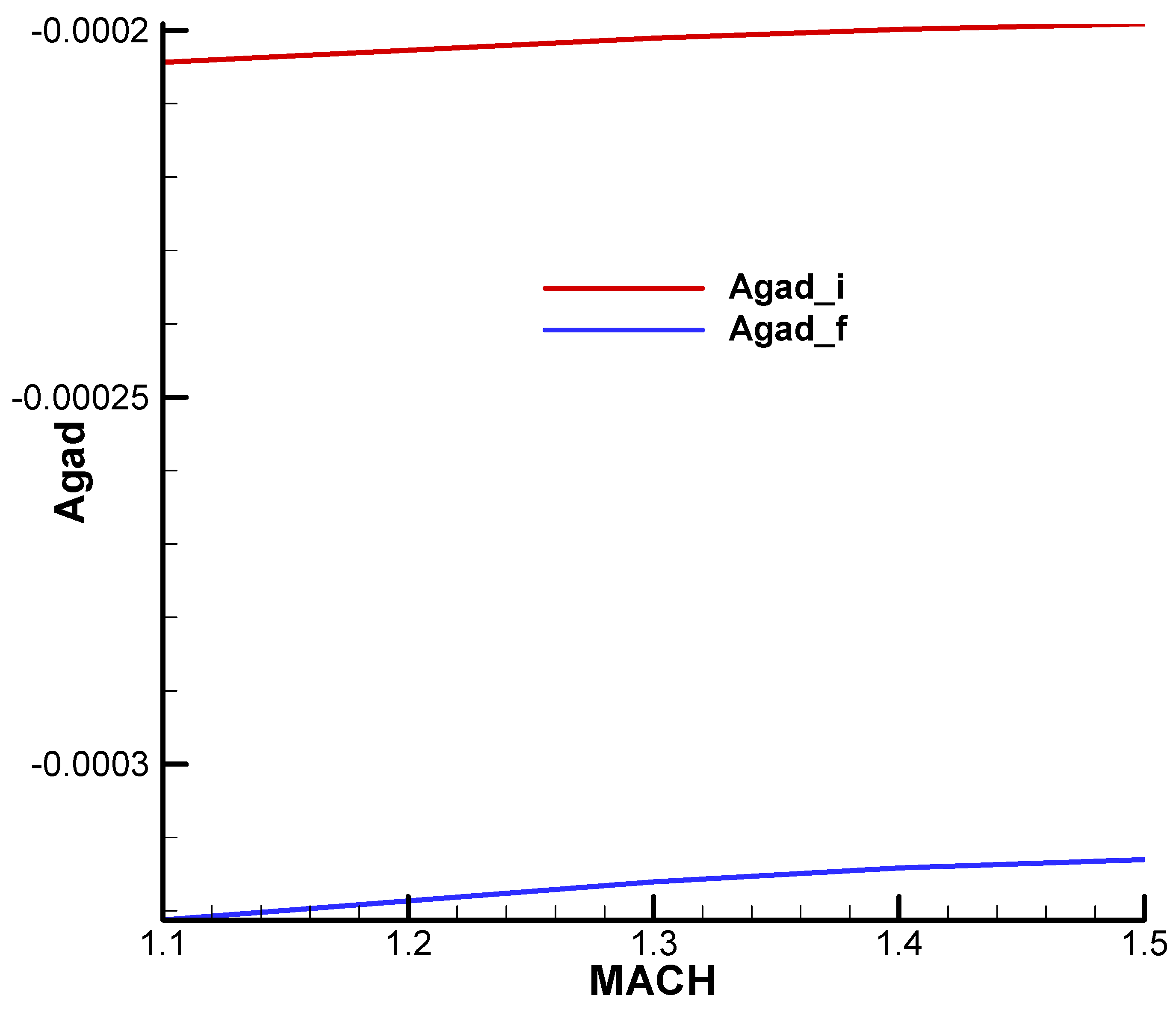
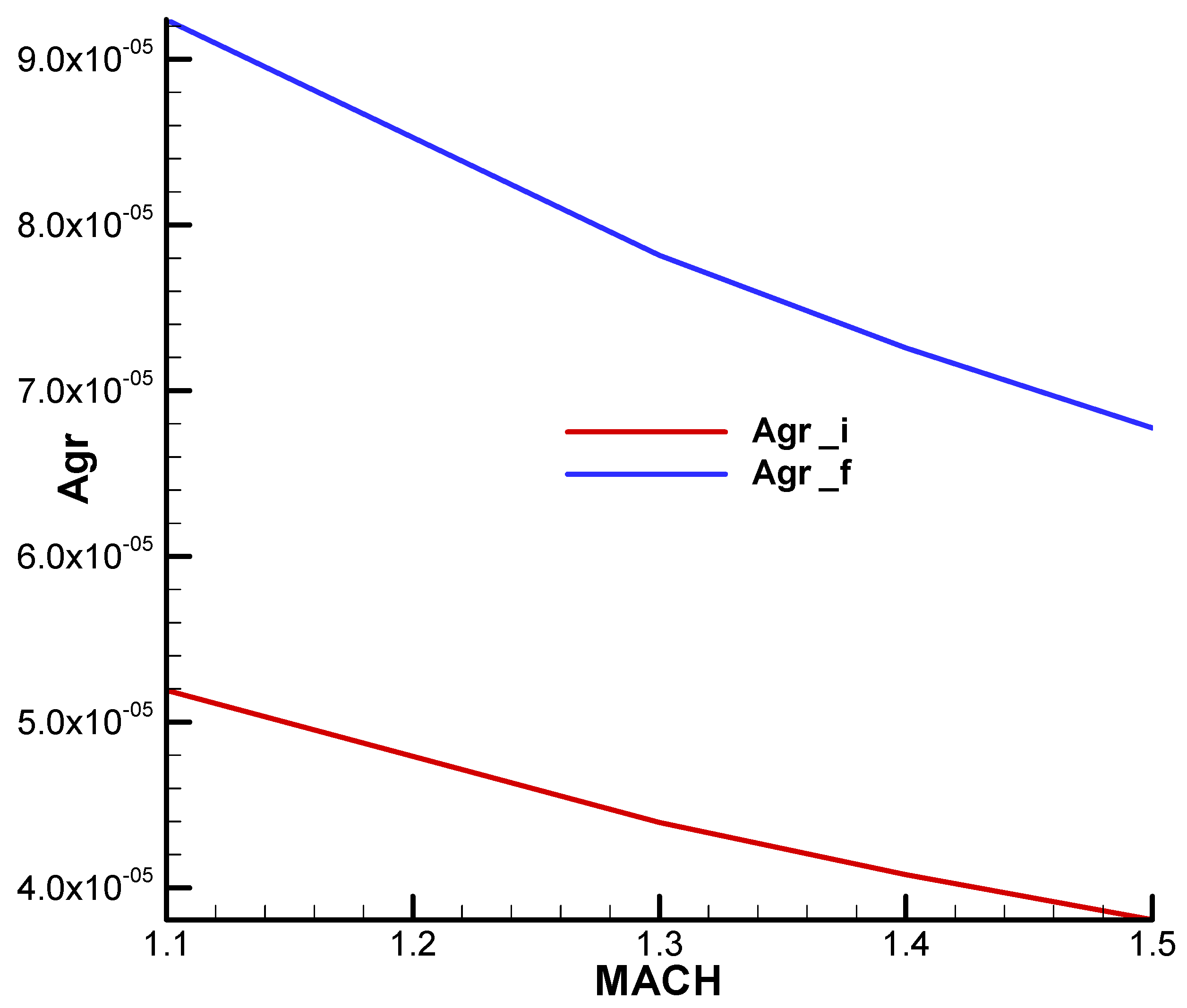
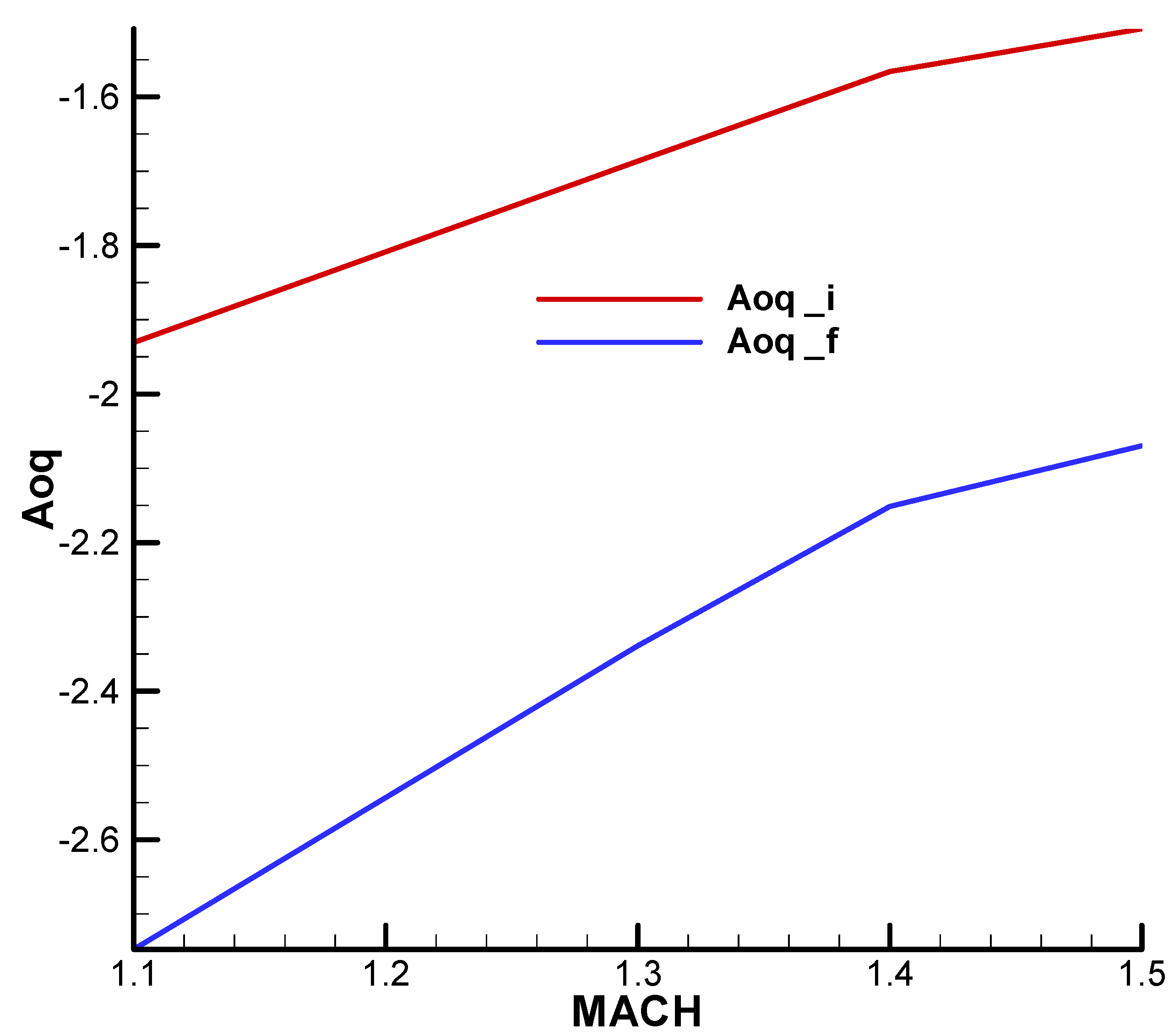
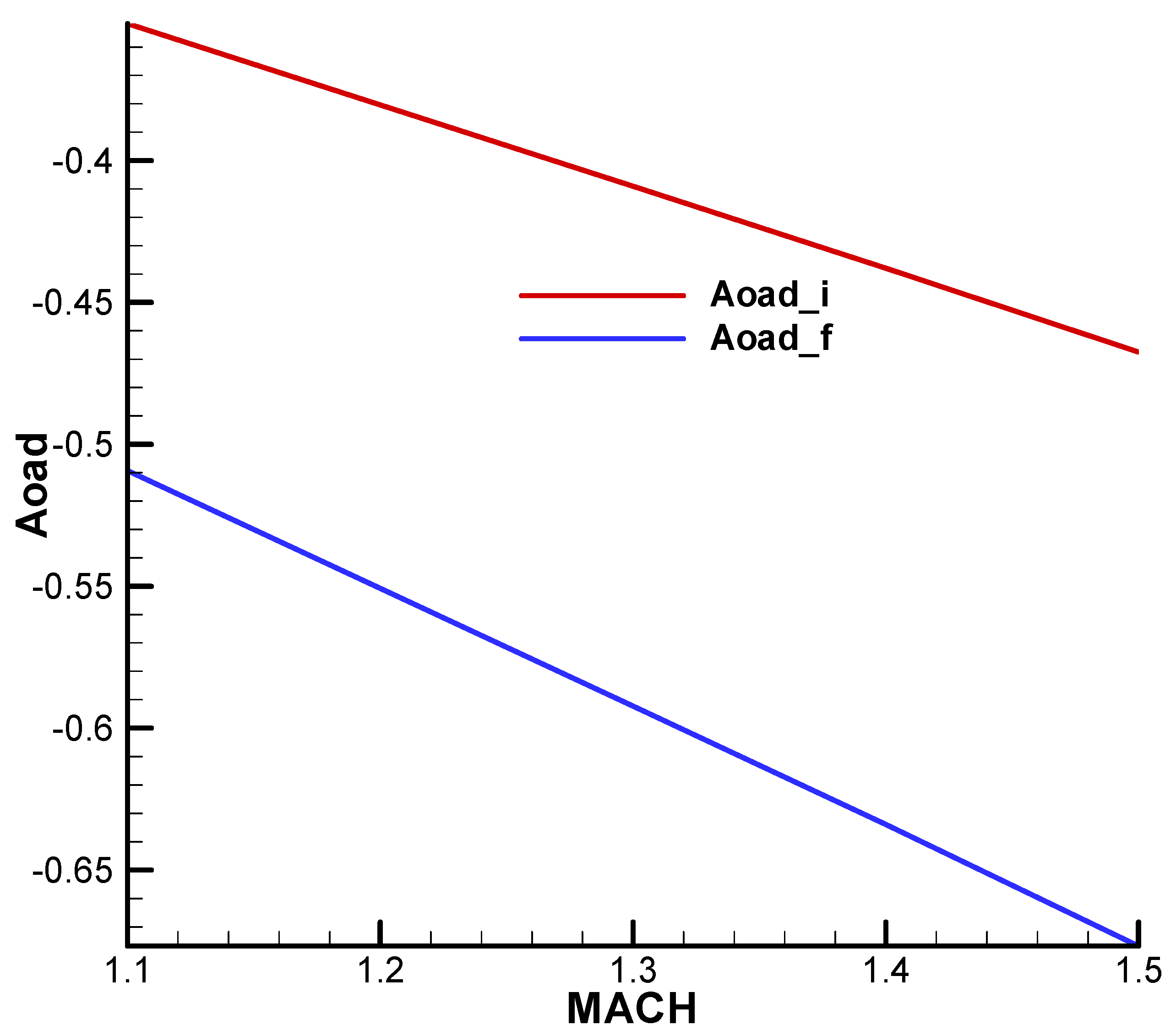
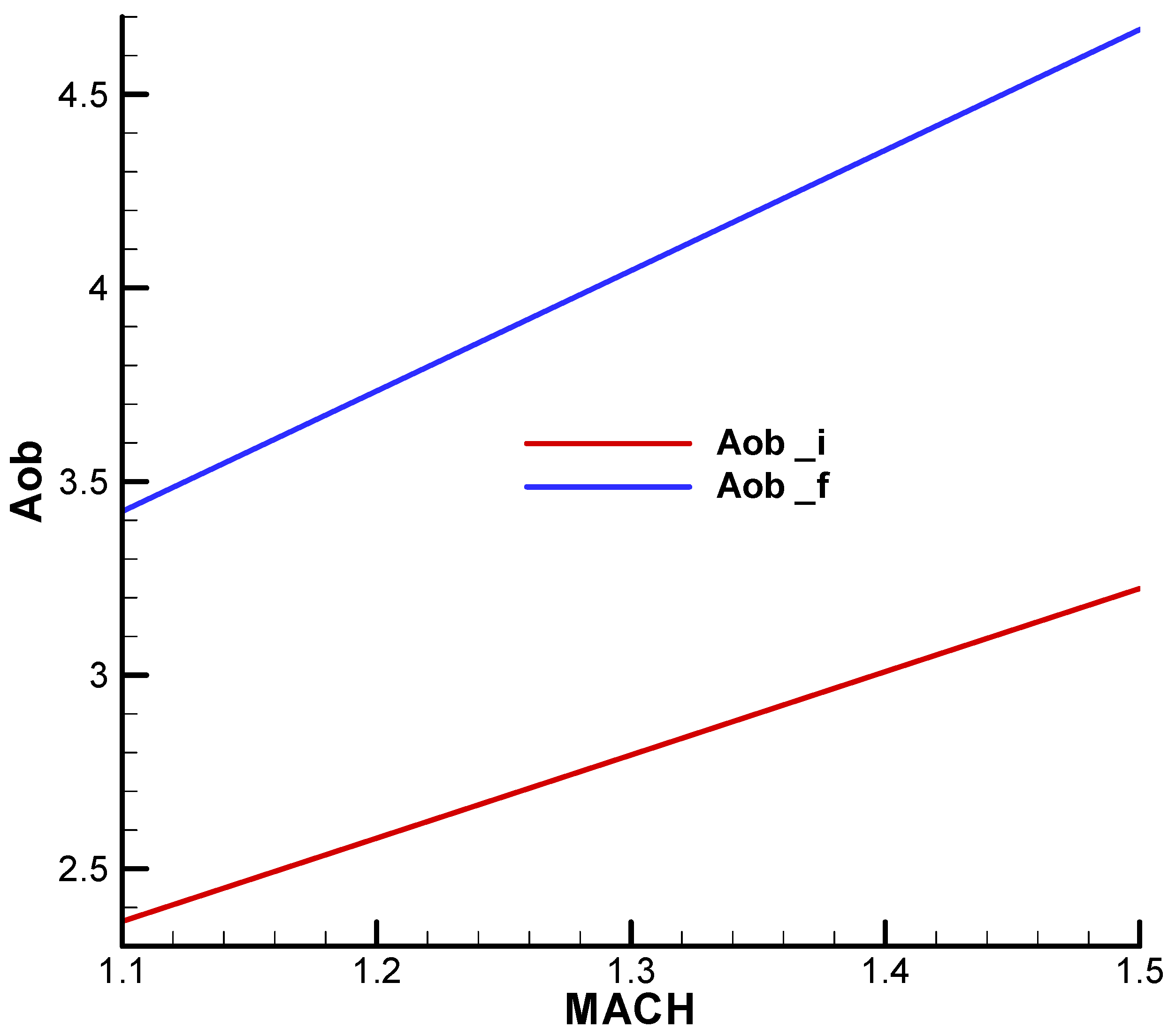
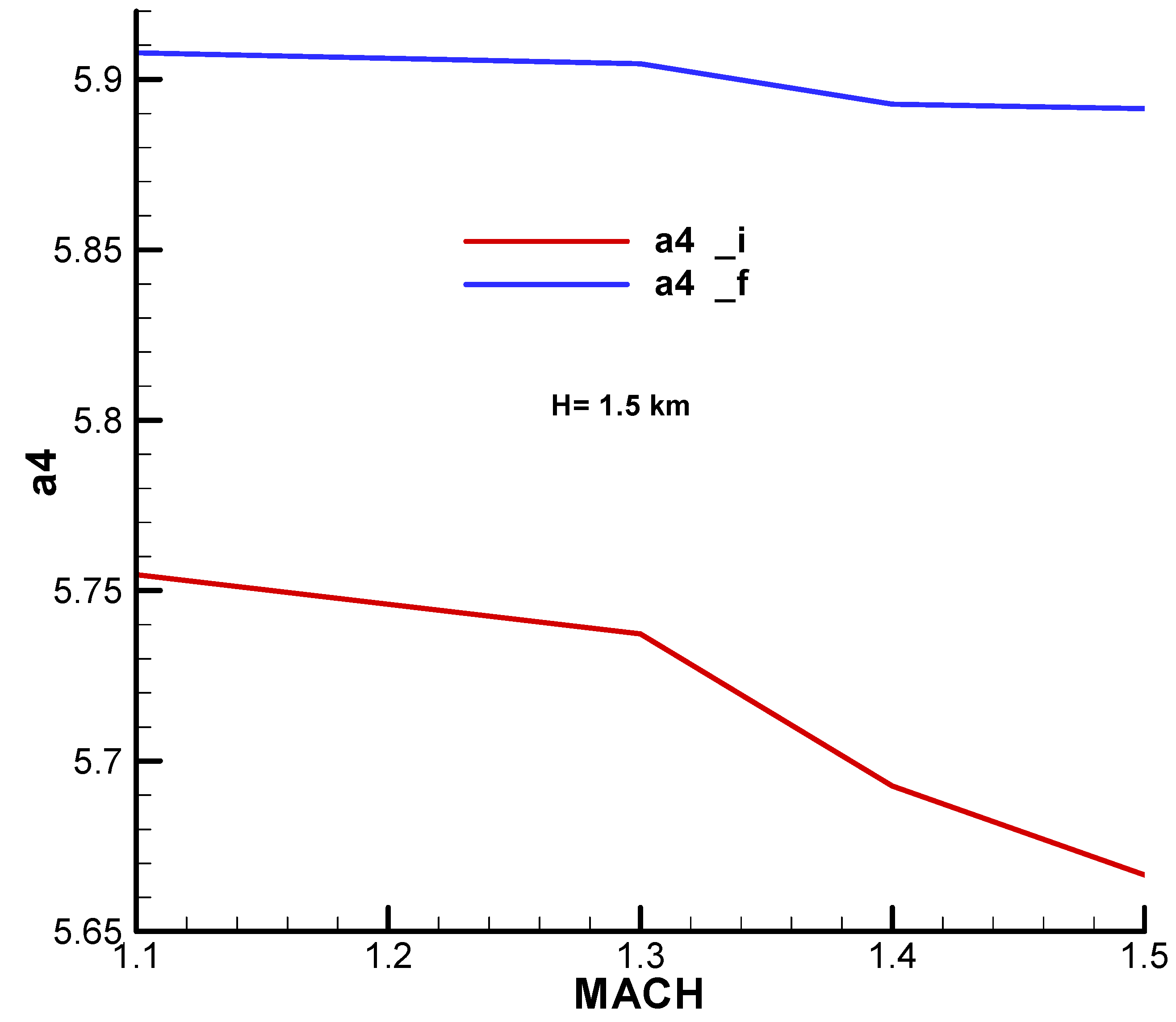
Appendix C.1. Coefficients of the Characteristic Polynomial: i—Initial; f—Final
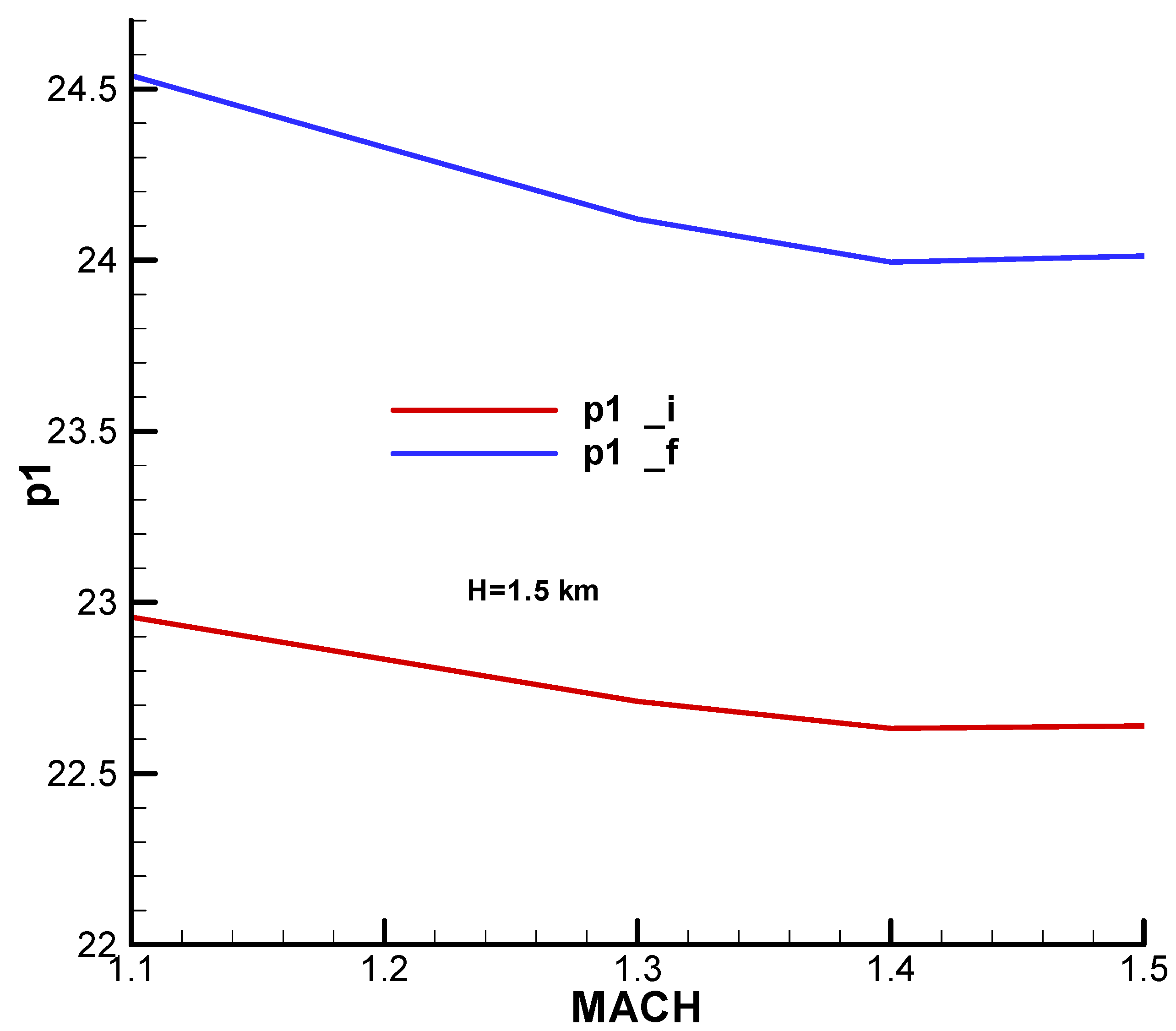
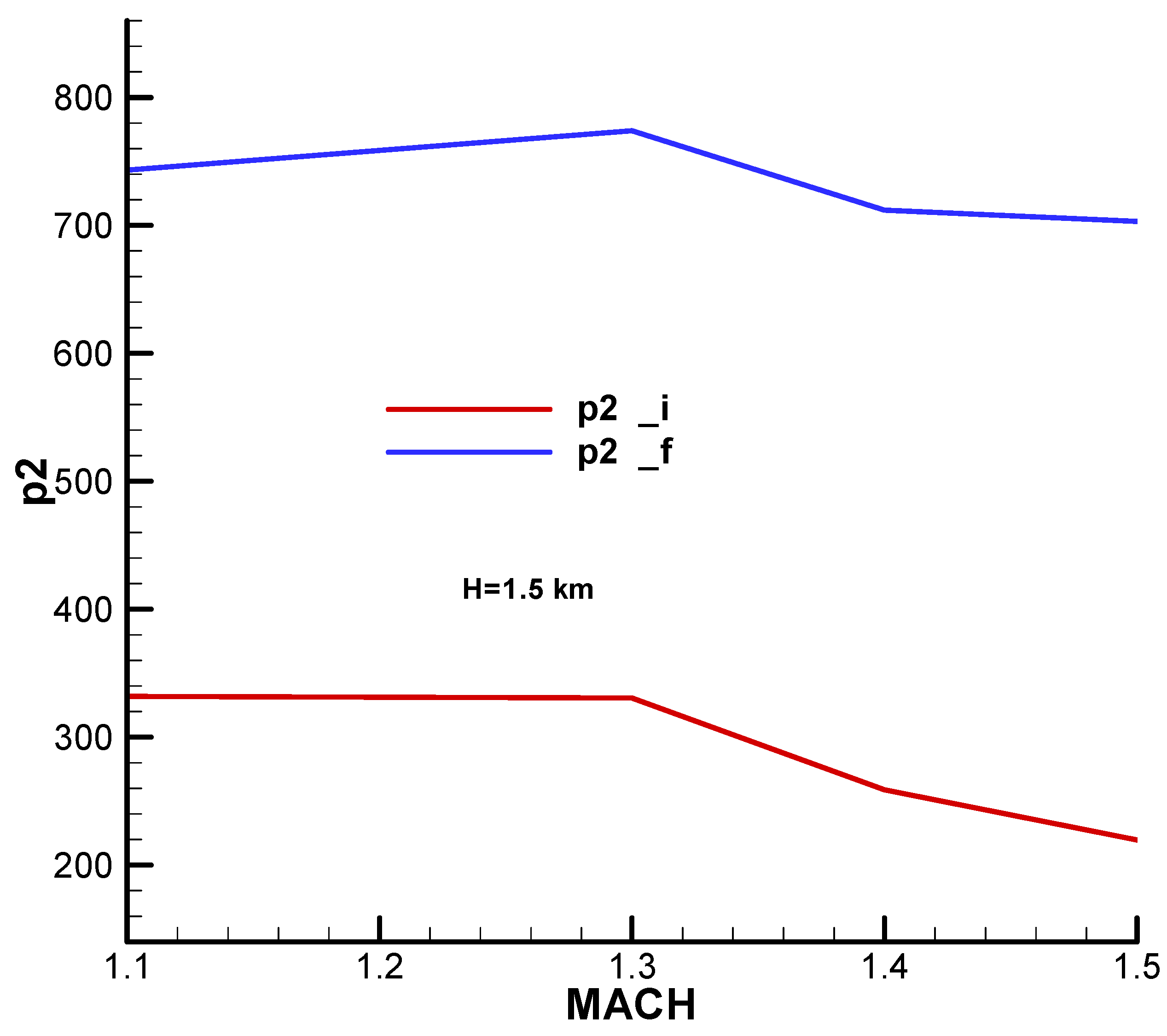

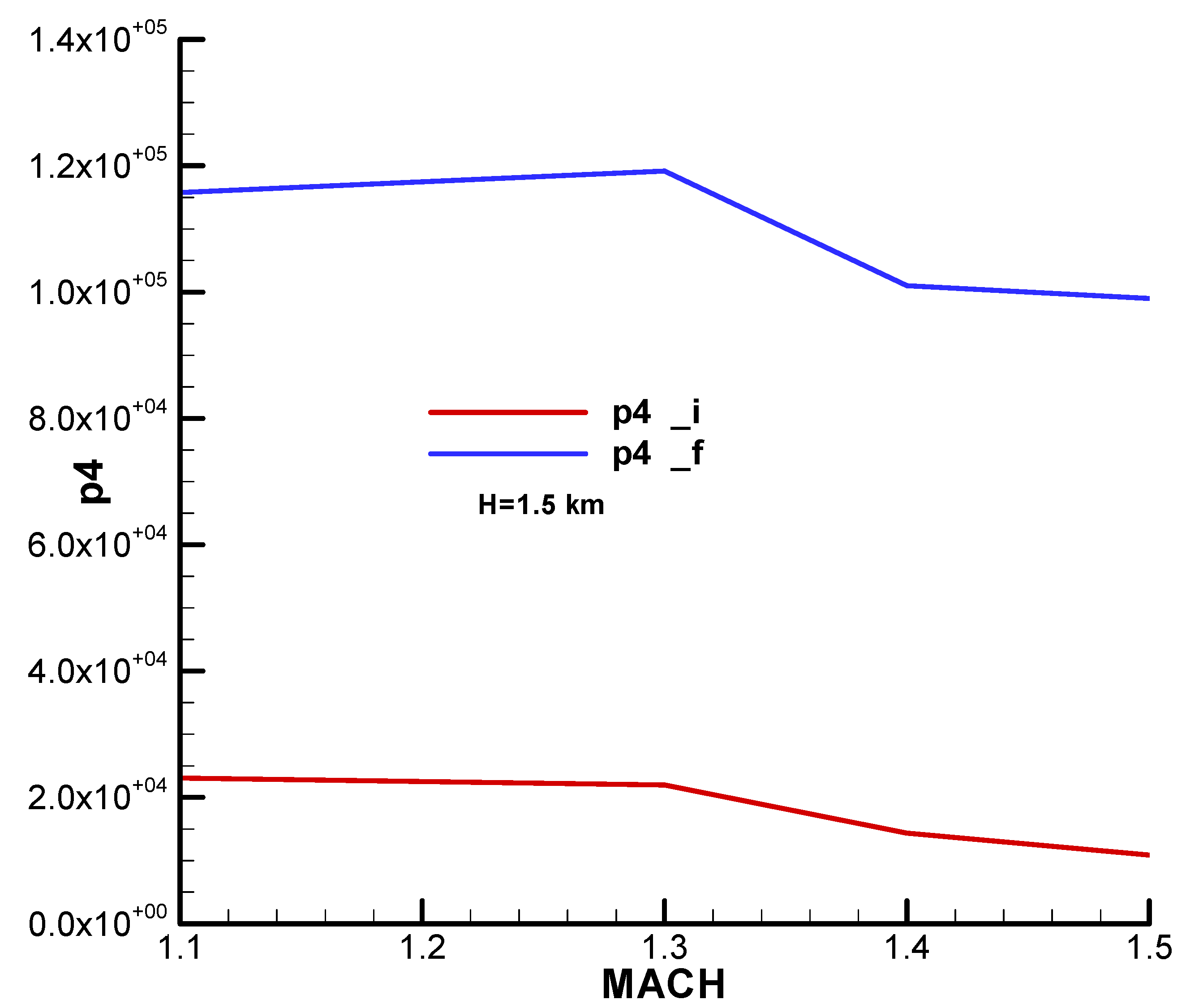

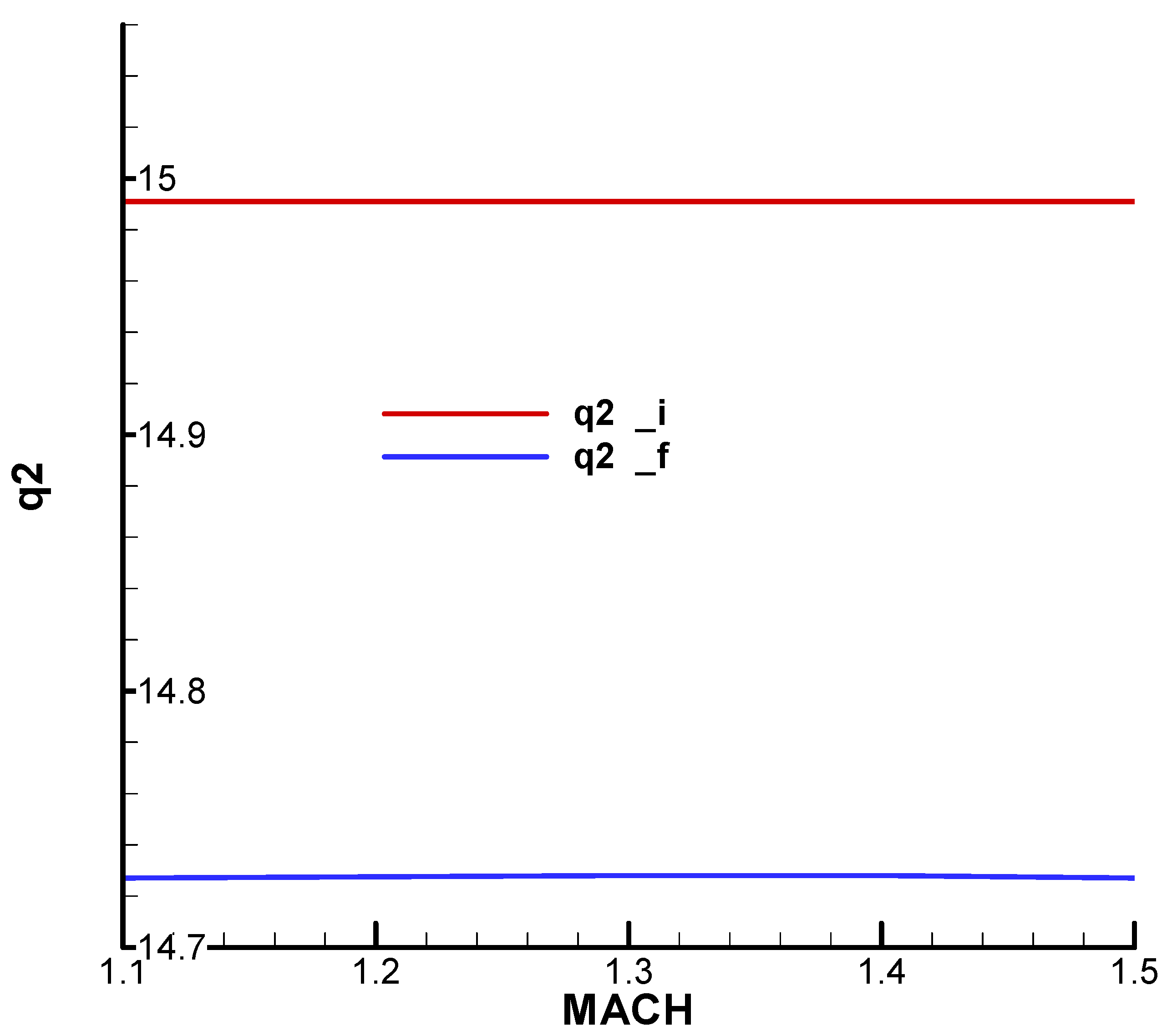

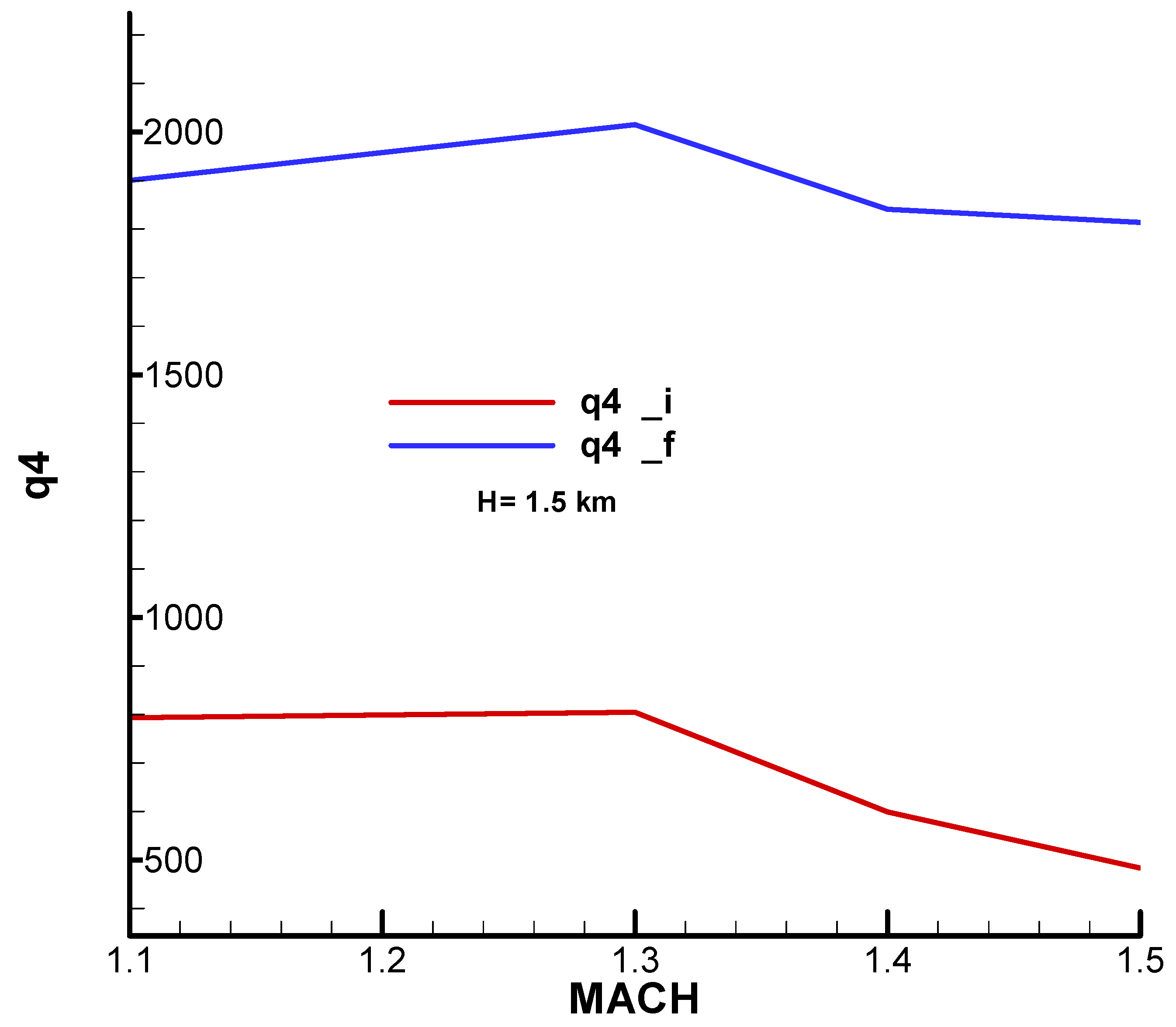
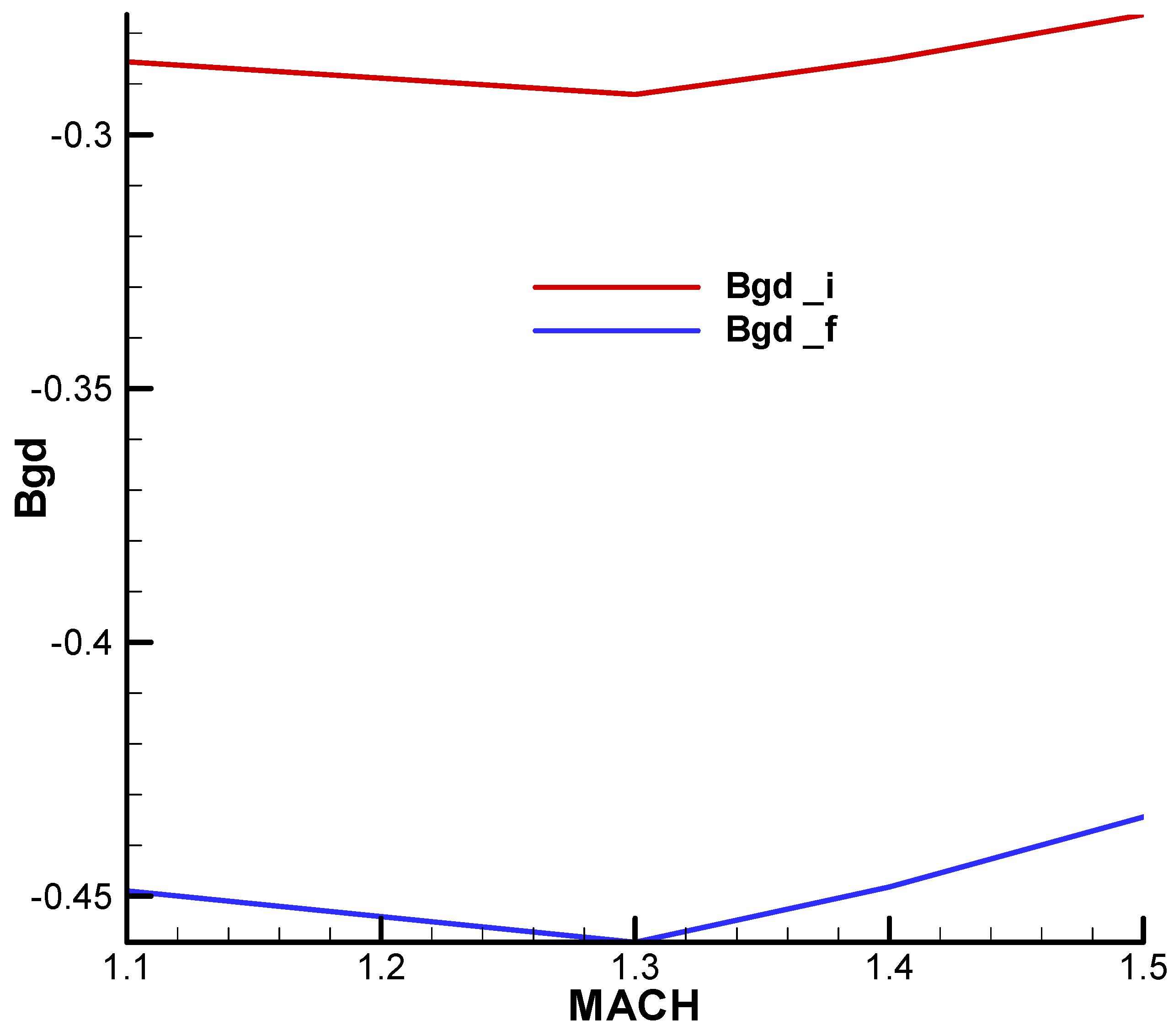
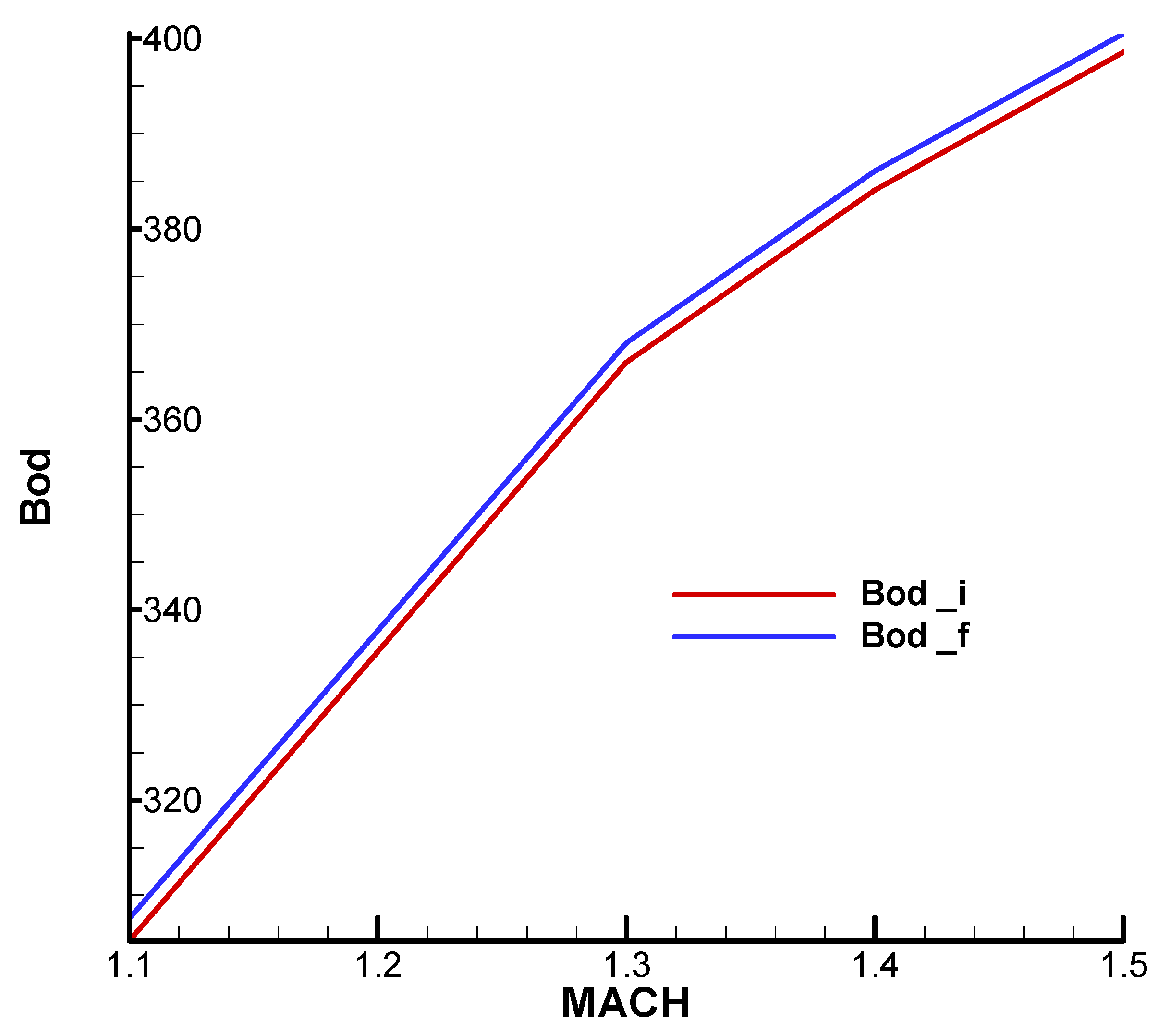
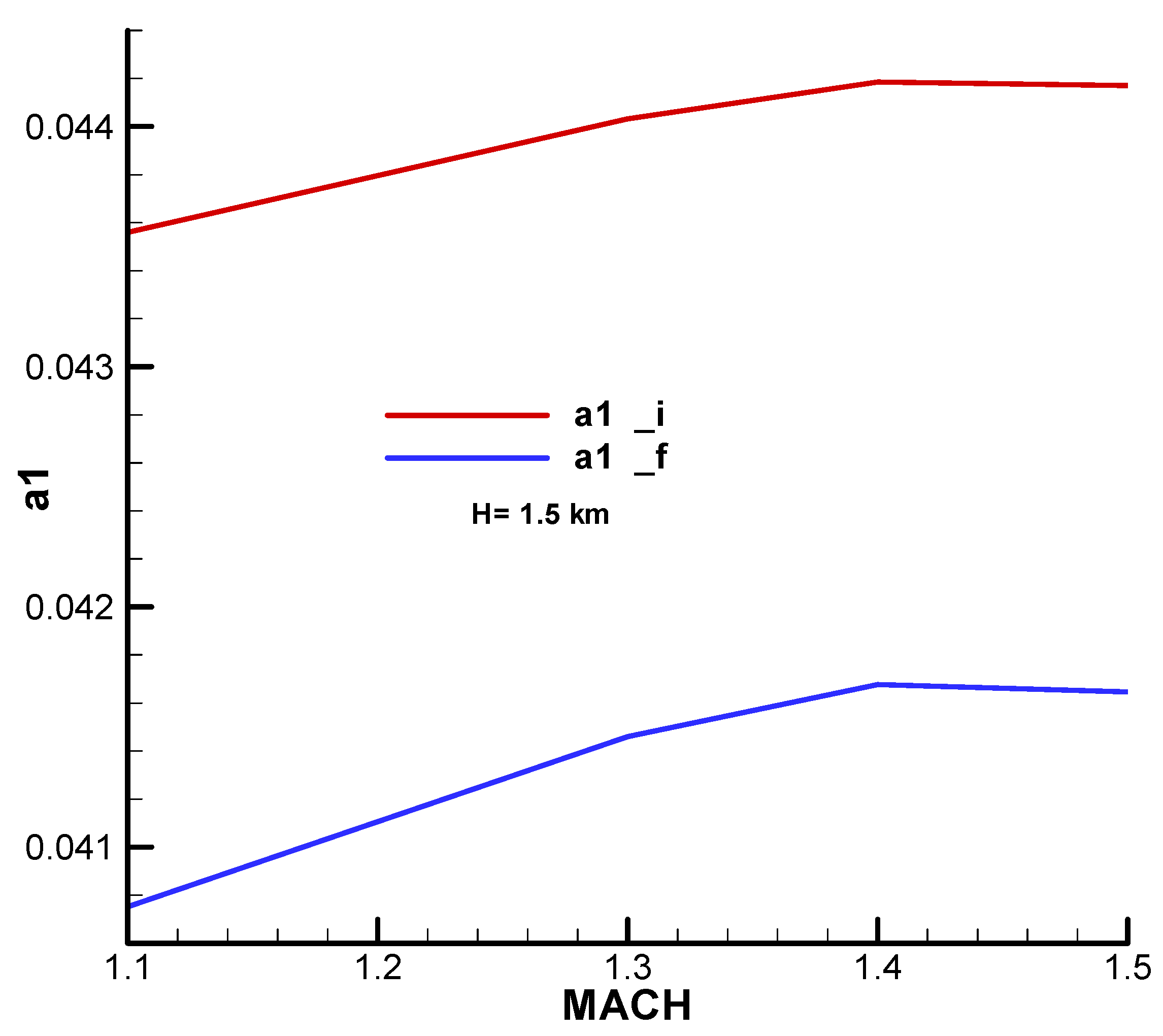
Appendix C.2. Stability Parameters F-W: i-Initial; f- Final


Appendix D. Frank–Wall Stability Criterion for the 4th-Order Polynomial
Check for Non-Rolling Case
References
- Hu, X.; Yang, S. Coning motion instability of spinning missiles induced by the delay of strap-down seeker. Chin. J. Aeronaut. 2021, 33, 3360–3368. [Google Scholar] [CrossRef]
- Hu, S.; Wang, J.; Wang, Y.; Tian, S. Stability Limits for the Velocity Orientation Autopilot of Rolling Missiles. IEEE Access 2021, 9, 110940–110951. [Google Scholar] [CrossRef]
- Hu, X.; Yang, S.; Xiong, F.; Zhang, G. Stability of spinning missile with homing proportional guidance law. Aerosp. Sci. Technol. 2017, 71, 546–555. [Google Scholar] [CrossRef]
- Suiçmez, E.C.; Kutay, A.T. Single channel digital controller design for a high spinning rate rolling airframe missile. Aeronaut. J. 2022, 126, 1815–1833. [Google Scholar] [CrossRef]
- Karimi, J. A new closed form solution for dynamic stability analysis of rolling airframes having one pair on-off actuator. Aviat. Open Access 2021, 25, 92–103. [Google Scholar] [CrossRef]
- Botez, R.M.; Chelaru, V.; Parvu, P.; Gheorghe, C. Calculus model for a rolling guided missile. J. Vib. Control. 2001, 7, 863–889. [Google Scholar] [CrossRef]
- Chelaru, T.-V.; Constantinescu, C.E. Theoretical and experimental solutions for multistage quasi-guided rocket. In Proceedings of the International Astronautical Congress, IAC, Washington, DC, USA, 21–25 October 2019. [Google Scholar]
- Zheng, Q.; Zhou, Z. Flight Stability of Canard-Guided Dual-Spin Projectiles with Angular Rate Loops. Int. J. Aerosp. Eng. 2020, 2020, 2705175. [Google Scholar] [CrossRef]
- Zheng, D.; Lin, D.; Wang, J.; Xu, X. Dynamic stability of rolling missiles employing a two-loop autopilot with consideration for the radome aberration parasitic feedback loop. Aerosp. Sci. Technol. 2017, 61, 1–10. [Google Scholar] [CrossRef]
- Tian, S.; Lin, D.; Wang, J.; Li, B. Dynamic stability of rolling missiles with angle-of-attack feedback three-loop autopilot considering parasitic effect. Aerosp. Sci. Technol. 2017, 71, 592–602. [Google Scholar] [CrossRef]
- Zheng, D.; Lin, D.; Xu, X.; Tian, S. Dynamic stability of rolling missile with proportional navigation & PI autopilot considering parasitic radome loop. Aerosp. Sci. Technol. 2017, 67, 41–48. [Google Scholar]
- Qin, S.-J.; Zhang, F.-X. A Combination of Silicon Micro-gyroscope that Application Rotary Missile Attitude Control System. In Proceedings of the 2011 International Conference on Physics Science and Technology (Icpst), Hong Kong, China, 28–30 December 2011. [Google Scholar]
- Yan, X.; Chen, G.; Tian, X. Two-Step Adaptive Augmented Unscented Kalman Filter for Roll Angles of Spinning Missiles Based on Magnetometer Measurements. Meas. Control. 2018, 51, 73–82. [Google Scholar] [CrossRef]
- Jahangir, E.; Howe, R.M. Time-Optimal Attitude Control Scheme for a Spinning Missile. J. Guid. Control. Dyn. 1993, 16, 346–353. [Google Scholar] [CrossRef][Green Version]
- Kocetkov, V.T.; Polovko, A.M.; Ponomarev, B.M. Theory of Self-Guided and Remote-Controlled Missile Systems; Nauka: Moskva, Russian, 1964. [Google Scholar]
- Frank, E. On the zeros of polynomials with complex coefficients. Bull. Amer. Math. Soc. 1946, 52, 144–157. [Google Scholar] [CrossRef]
- ISO 1151-75; Flight Dynamics—Concepts, Quantities and Symbols—Part 5: Quantities Used in Measurements. ISO: Geneva, Switzerland, 1987.
- STP M 40406-99; The Commanded Missile System—Terminology and Symbols. Ministry of National Defence (MoND): Bucharest, Romania, 1998.
- Niţă, M.M.; Andreescu, D. ŞT. Rocket Flight; Militara: Bucharest, Romania, 1964. [Google Scholar]
- Chelaru, T.V. Dynamics of Flight—Guided Missile, 2nd ed.; Revised and Added; Printech: Bucharest, Romania, 2004; p. 434. [Google Scholar]
- Kuzovkov, N.T. Flight Stabilization Systems, Ballistic Missile and Anti-Aircraft Missile; Visaia Skola: Moskva, Russian, 1976. [Google Scholar]
- Belea, C.; Lungu, R.; Cismaru, C. Gyroscopic Systems and Their Applications; Scrisul Romanesc: Craiova, Romania, 1986. [Google Scholar]
- Hacker, T. Stability and Control in Flight Theory; Romanian Academy: Bucharest, Romania, 1968. [Google Scholar]
- STP M 40455-99; The Guided Missile System—Terminology and Symbols. Ministry of National Defence (MoND): Bucharest, Romania, 1998.
- Carpantier, R. Guidance des Avions et des Missiles Aerodynamiques; Tom I,II,III, lit; ENSAE: Paris, France, 1989. [Google Scholar]
- Nielsen, J. Missile Aerodynamics; McGraw-Hill Book Company, Inc.: New York, NY, USA; Toronto, ON, Canada; London, UK, 1960. [Google Scholar]
- Sviatoduh, V. Dynamics of Spatial Motion of Guided Missiles; Masinostroenie: Moskva, Russian, 1969. [Google Scholar]
- Ben-Asher, J.Z.; Isaac, Y. Advanced in Missile Guidance Theory; American Institute of Aernautics and Astronautics: Preston, VA, USA, 1998. [Google Scholar]
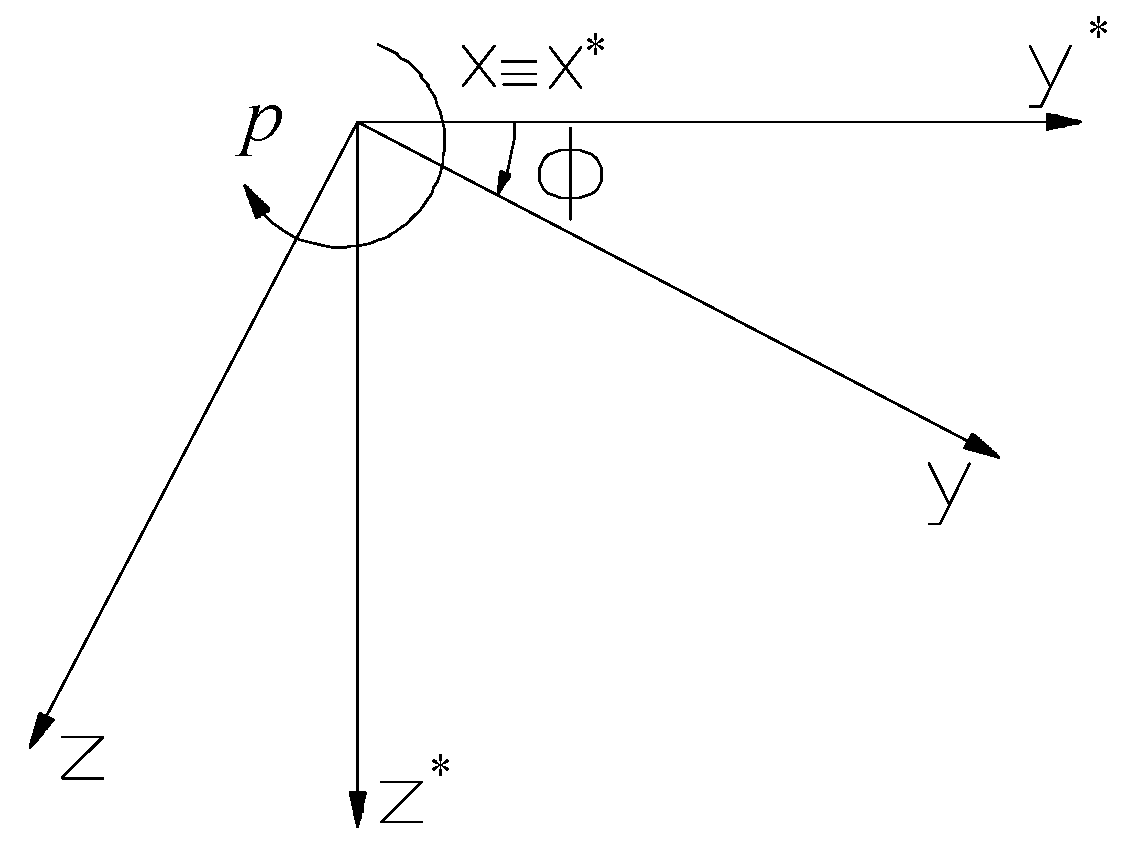



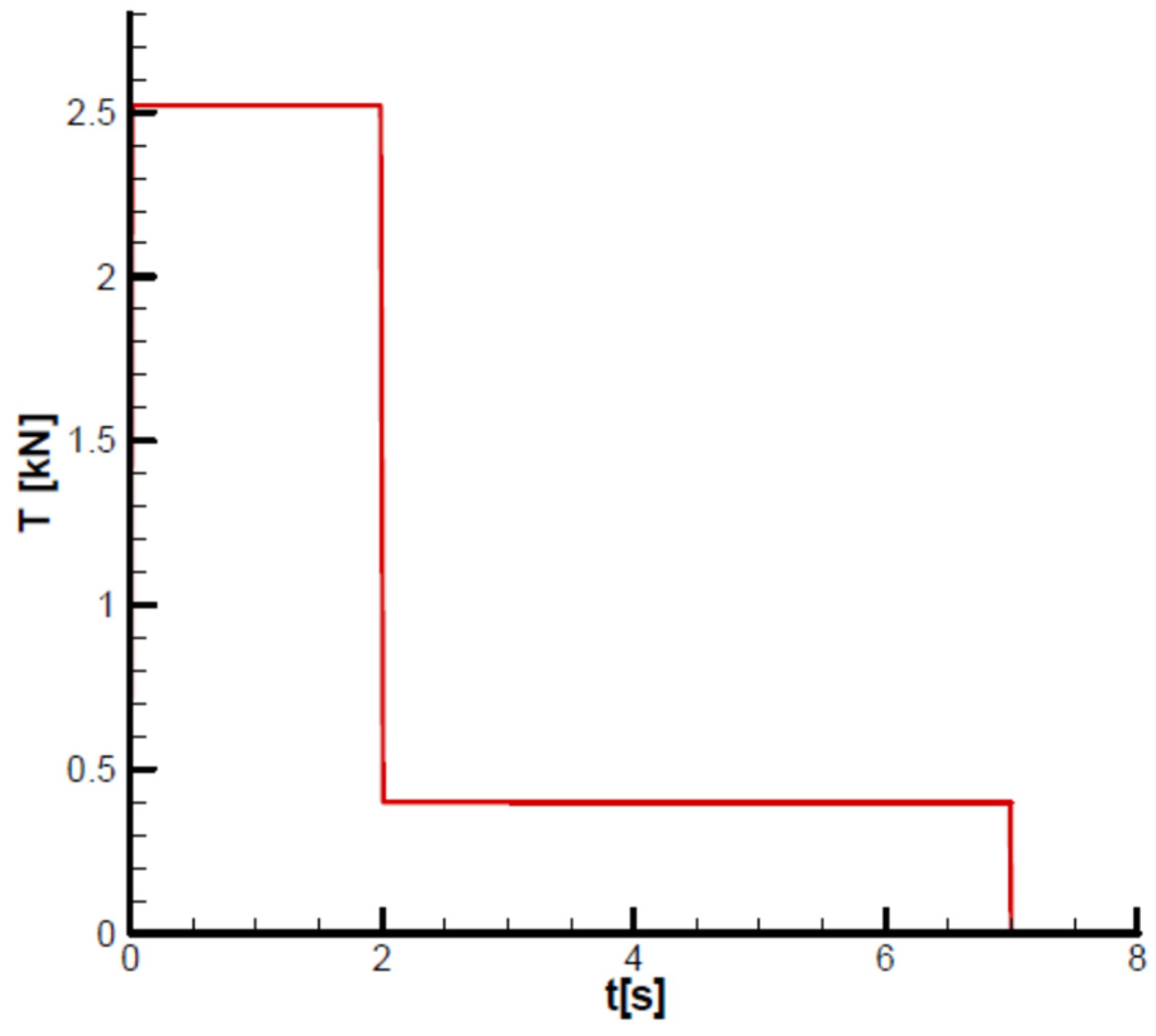





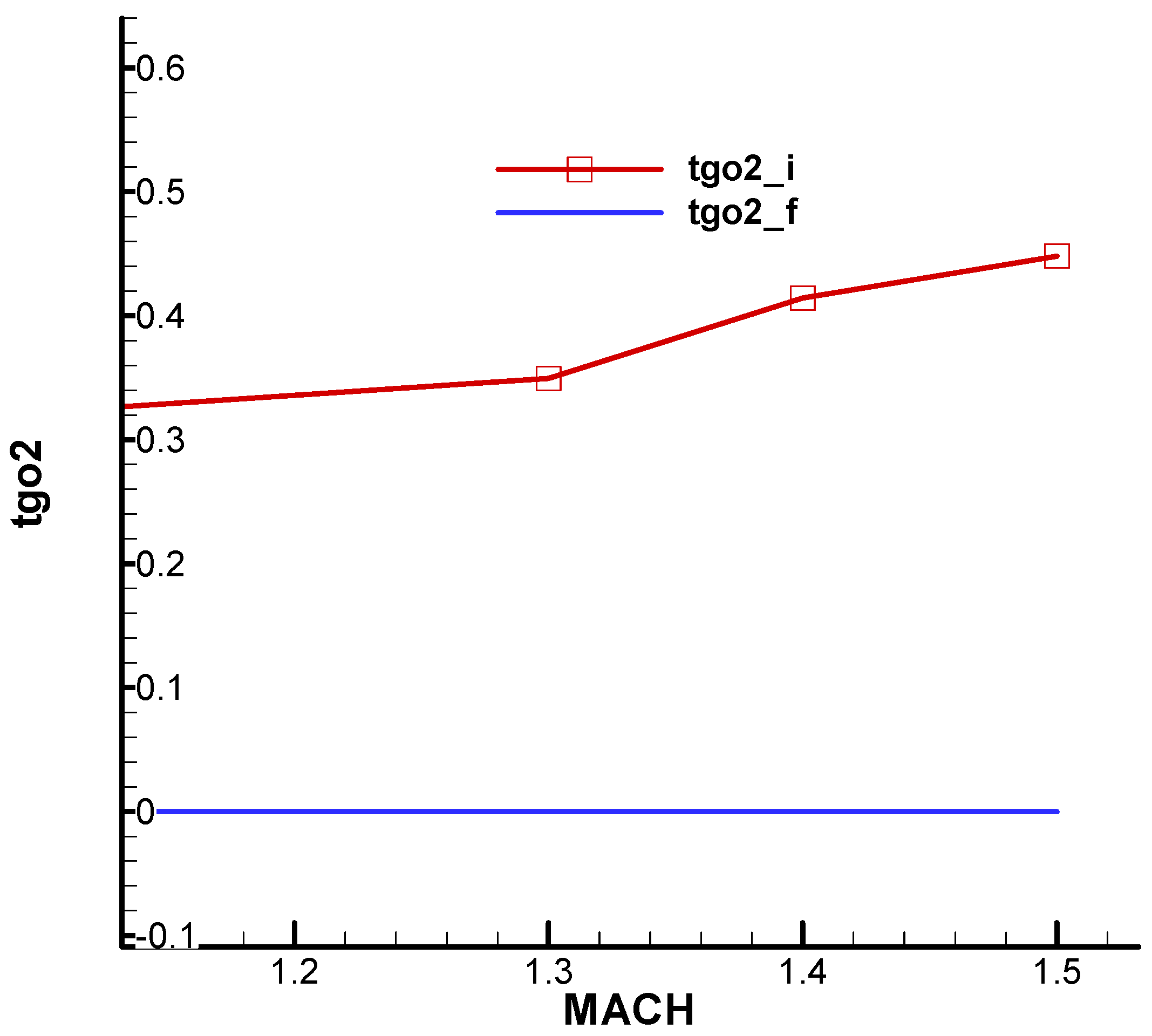


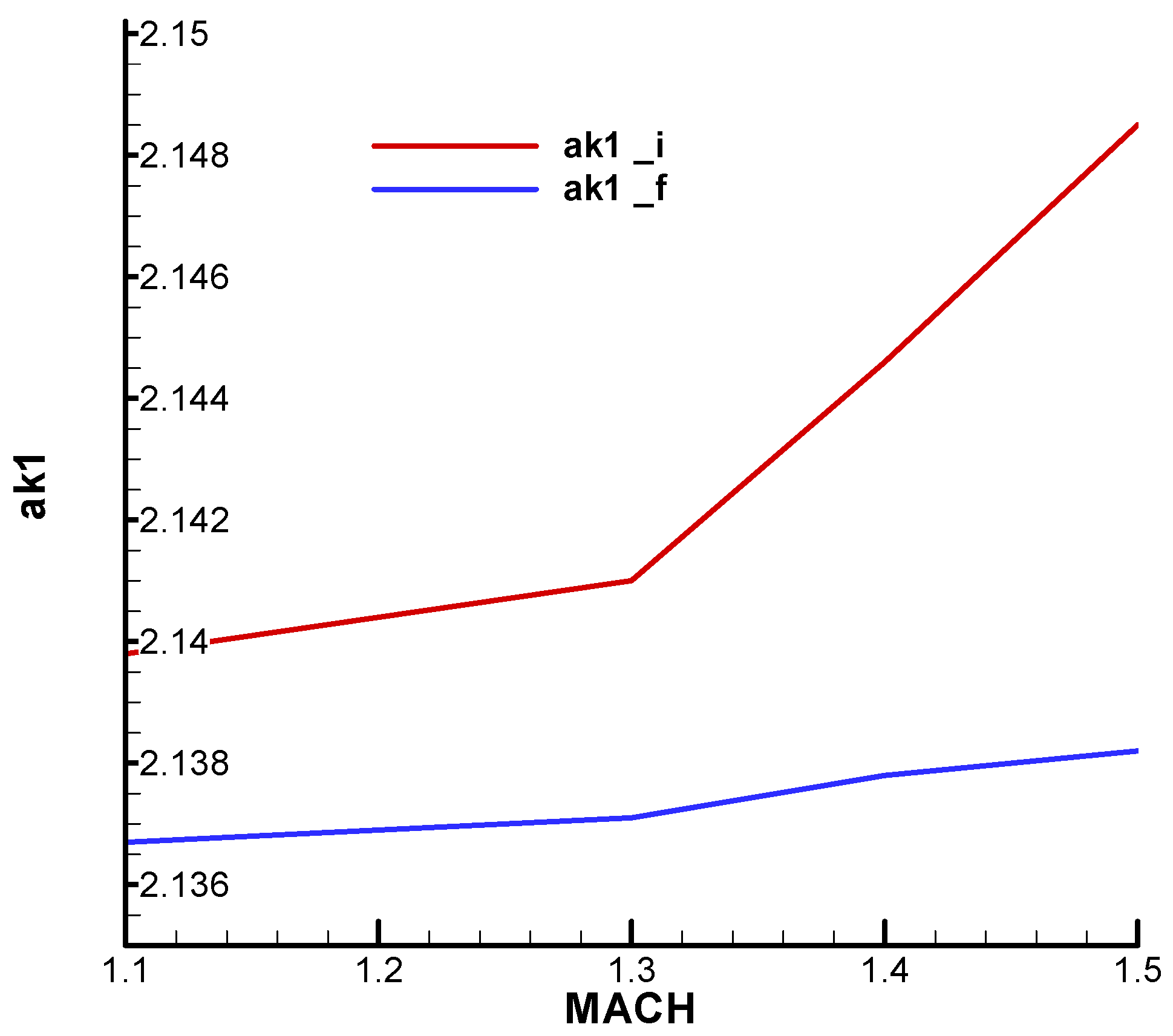

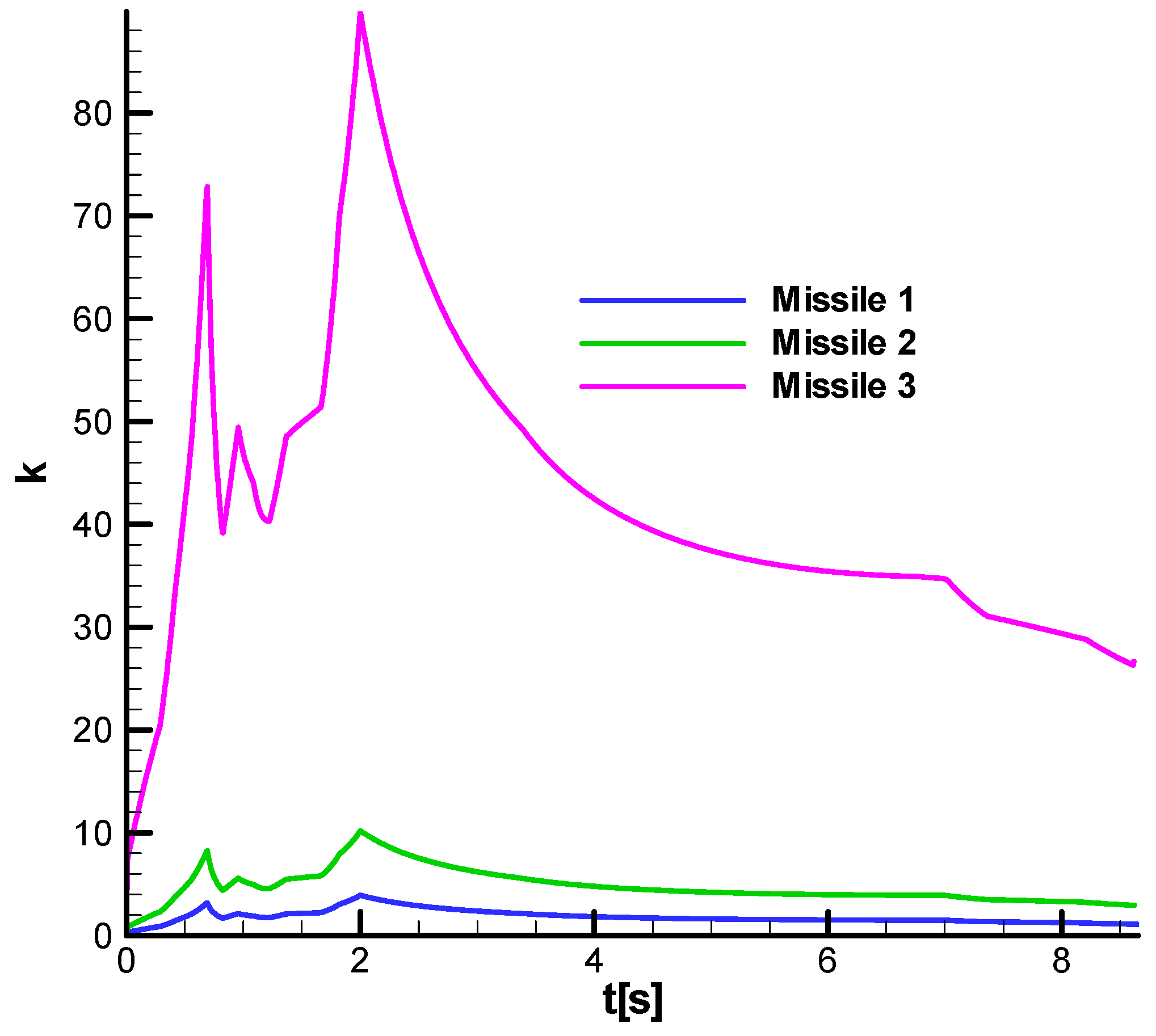
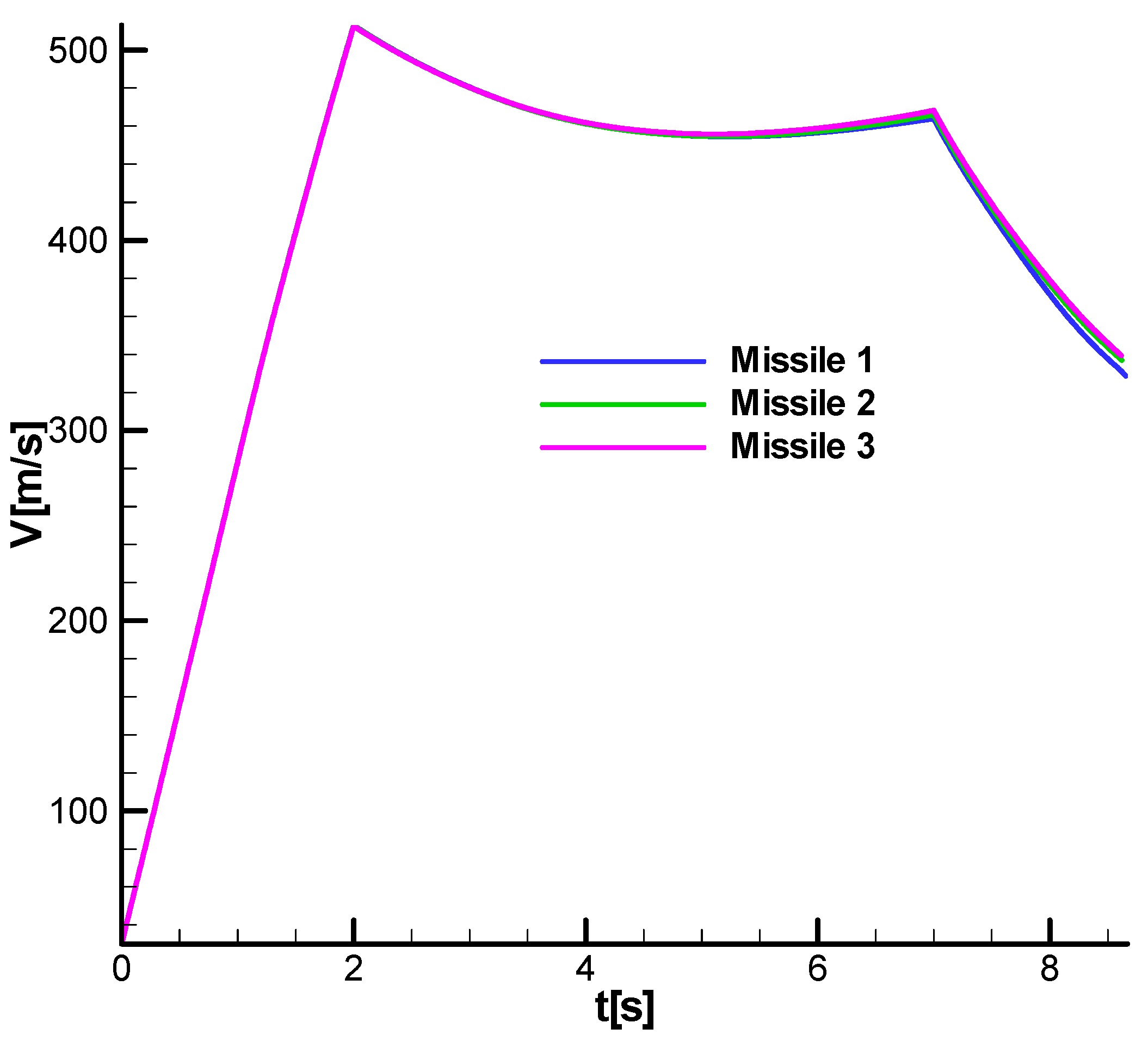
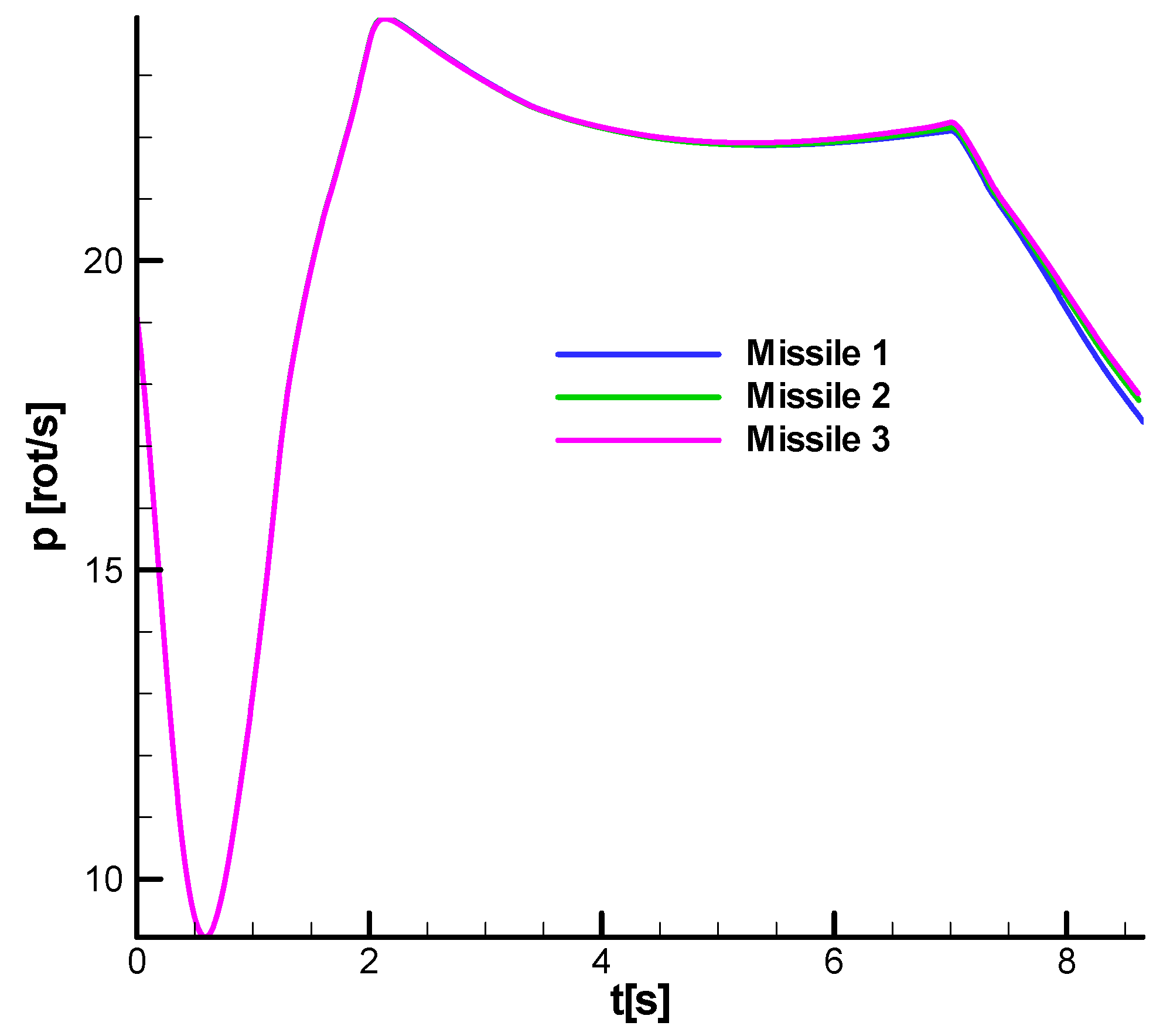


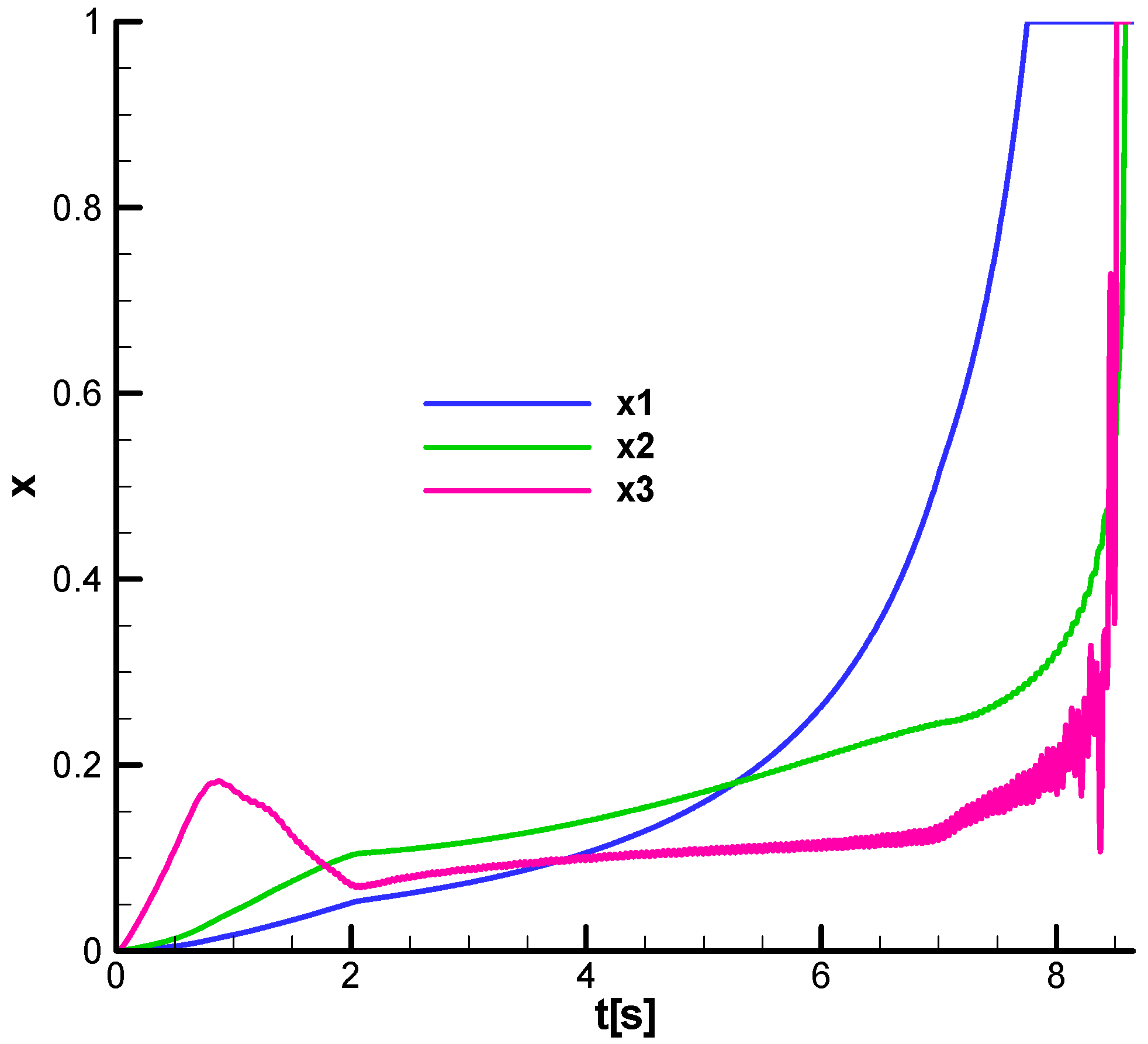
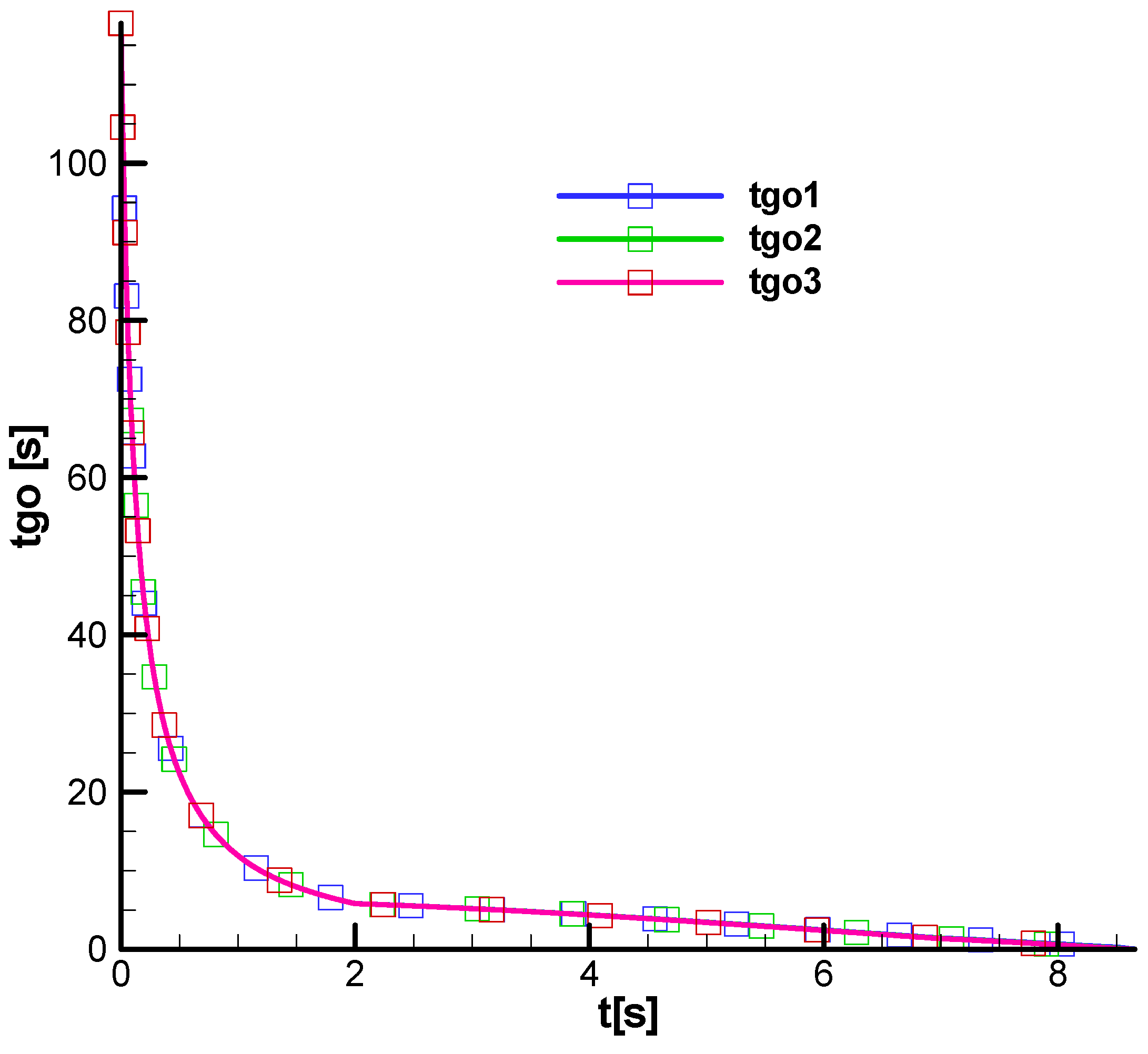
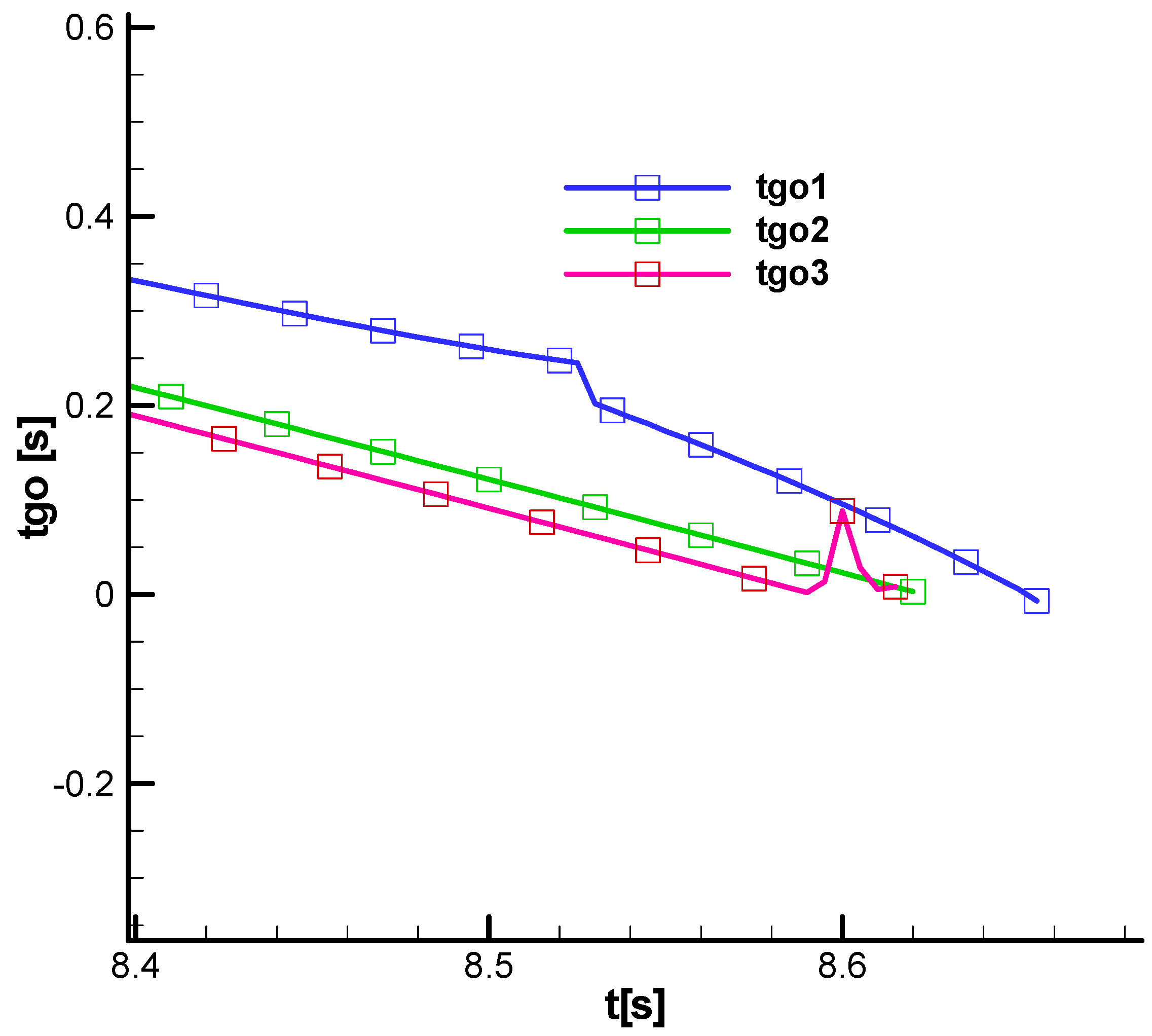
| Phase | Mass M [kg] | Center of Mass Xcm [m] | Roll Inertial Moment A [kgm2] | Yaw/Pitch Inertial Moment B [kgm2] | Time [s] |
|---|---|---|---|---|---|
| Initial | 10 | 0.7 | 0.008 | 1.4 | 0 |
| Intermediate 1 | 9 | 0.7 | 0.007 | 1.3 | 2 |
| Final | 6 | 0.6 | 0.005 | 1.0 | 7 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chelaru, T.-V.; Constantinescu, C.E.; Pană, V.; Ene, C.; Chelaru, A. Stability of Single-Channel Homing Rolling Aerospace Vehicle. Aerospace 2024, 11, 660. https://doi.org/10.3390/aerospace11080660
Chelaru T-V, Constantinescu CE, Pană V, Ene C, Chelaru A. Stability of Single-Channel Homing Rolling Aerospace Vehicle. Aerospace. 2024; 11(8):660. https://doi.org/10.3390/aerospace11080660
Chicago/Turabian StyleChelaru, Teodor-Viorel, Cristian Emil Constantinescu, Valentin Pană, Costin Ene, and Adrian Chelaru. 2024. "Stability of Single-Channel Homing Rolling Aerospace Vehicle" Aerospace 11, no. 8: 660. https://doi.org/10.3390/aerospace11080660
APA StyleChelaru, T.-V., Constantinescu, C. E., Pană, V., Ene, C., & Chelaru, A. (2024). Stability of Single-Channel Homing Rolling Aerospace Vehicle. Aerospace, 11(8), 660. https://doi.org/10.3390/aerospace11080660






