Test Scheme Design and Numerical Simulation of Composite Thrust Reverser Cascade
Abstract
1. Introduction
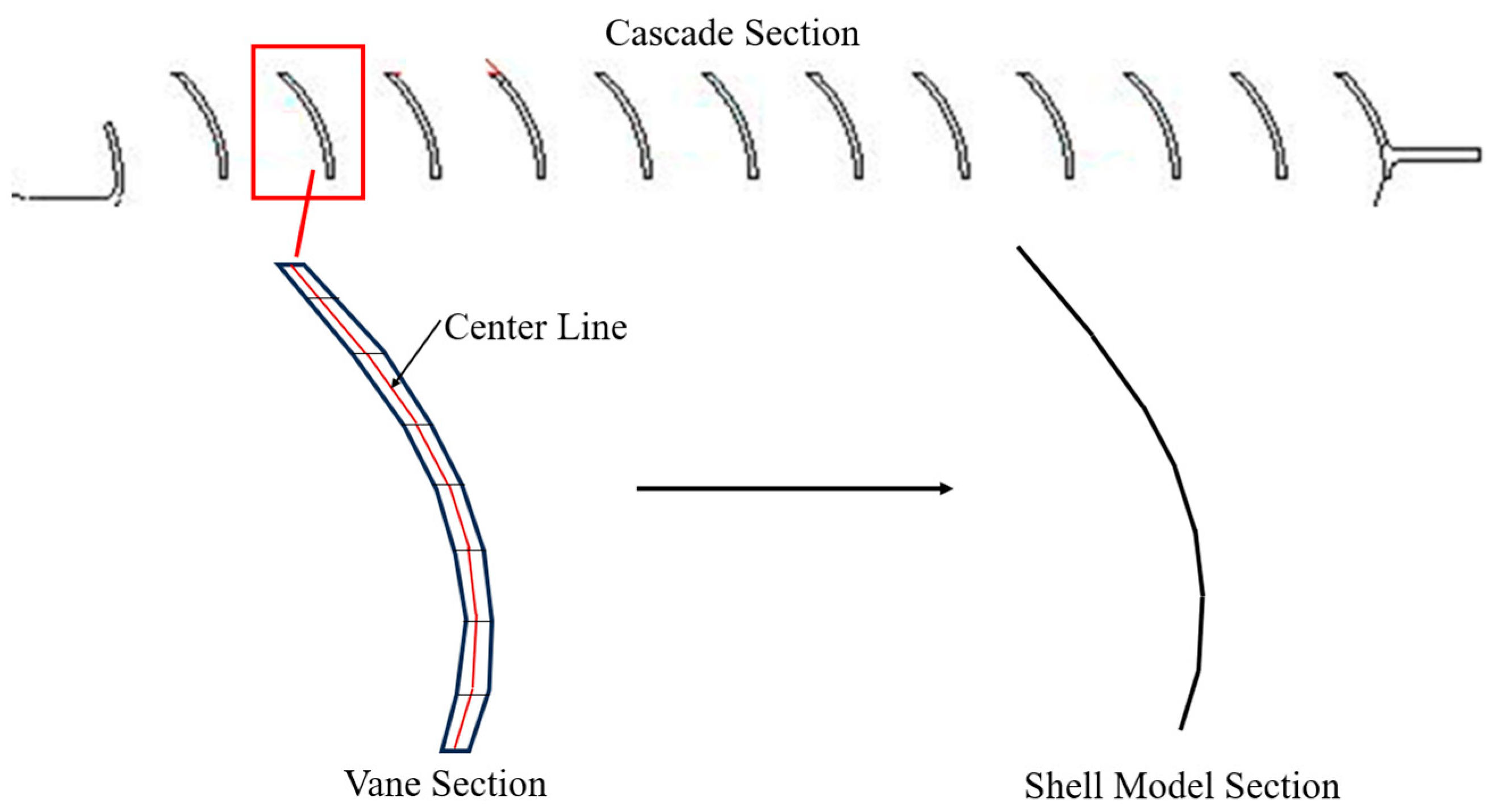
2. Composite Thrust Reverser Cascade Structure
3. Finite Element Modeling of Composite Thrust Reverser Cascade
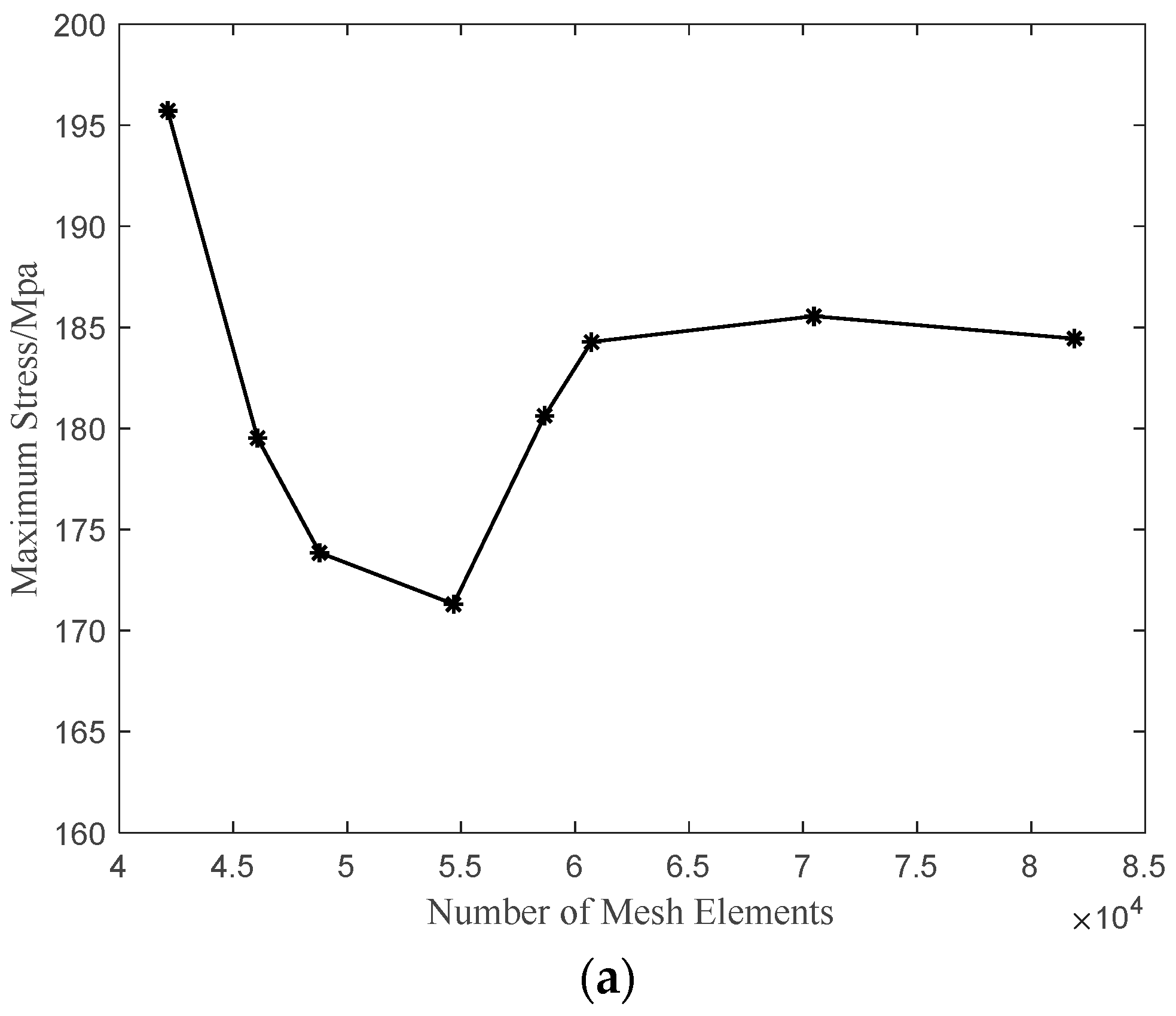
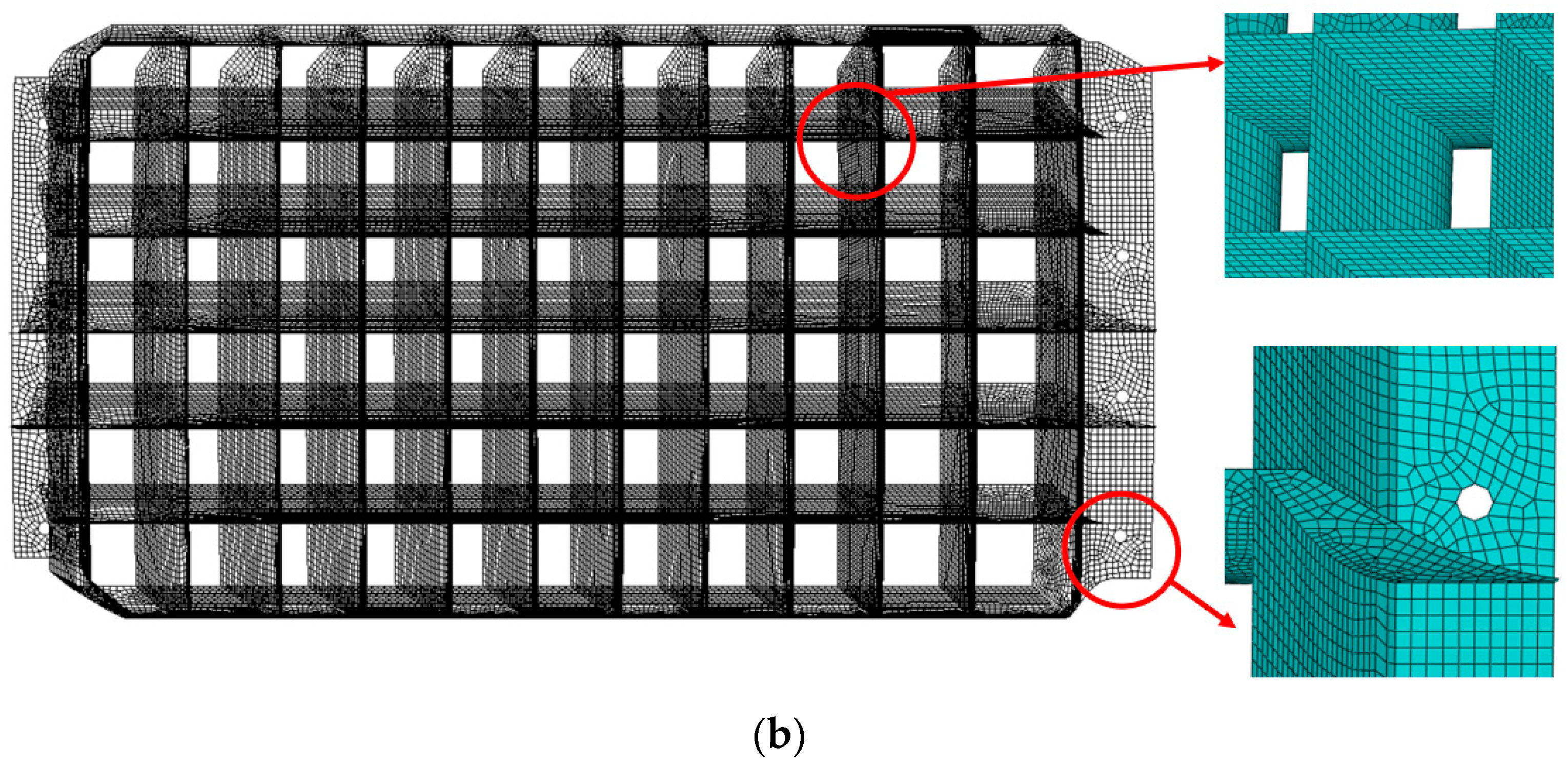
3.1. Structure and Mesh Modeling
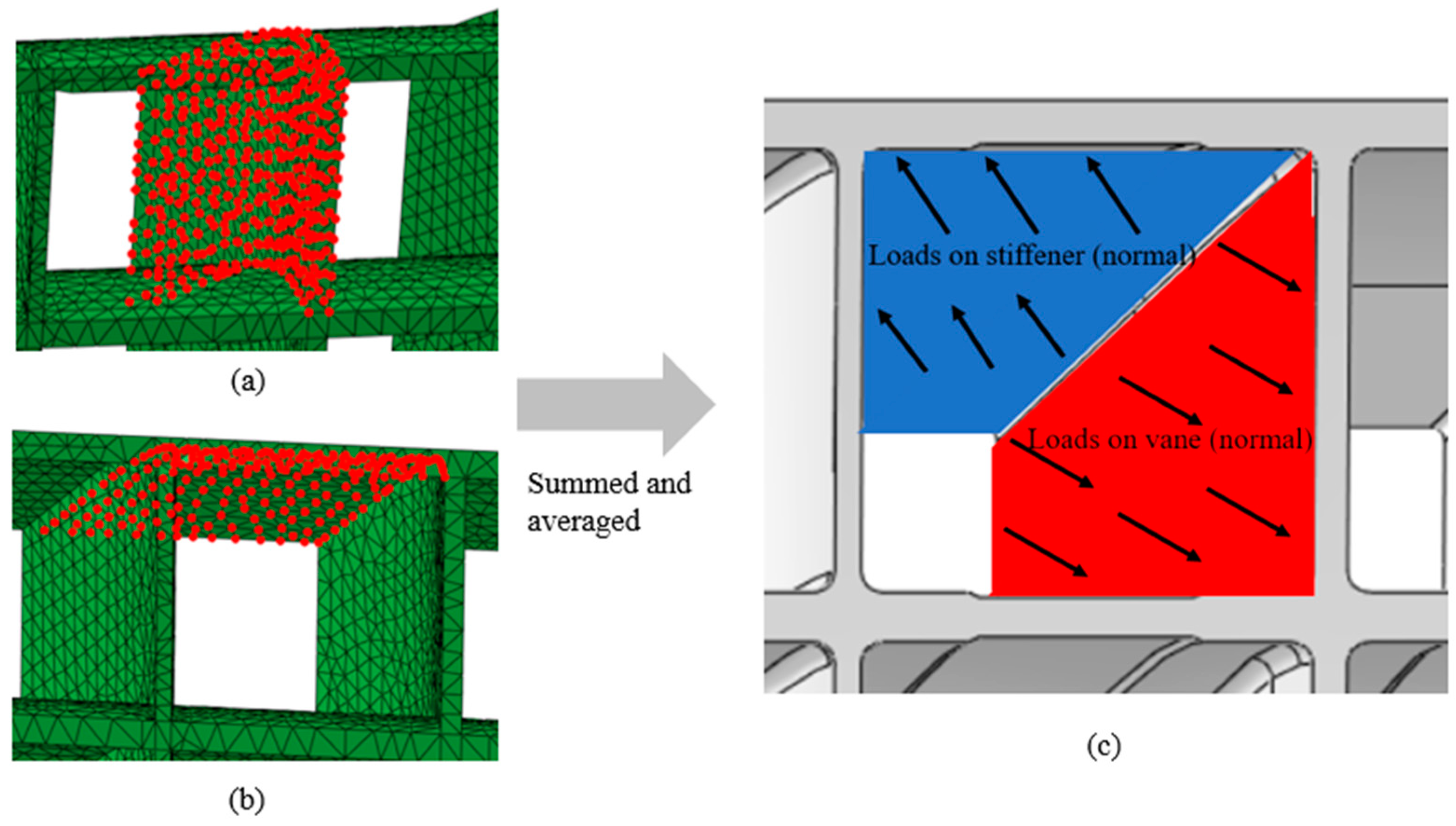
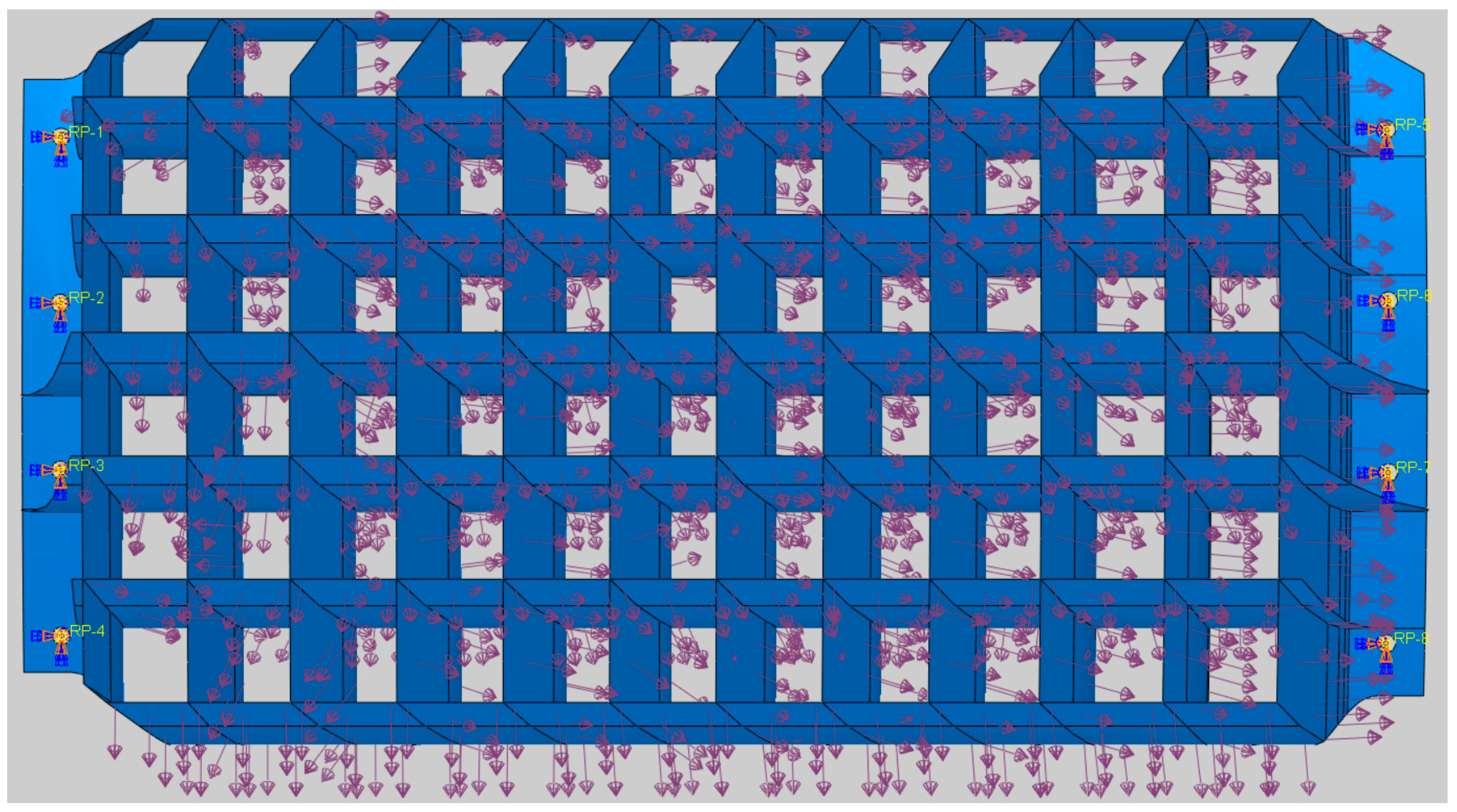
3.2. Boundary Conditions and Loads Simplification
3.3. Constitutive Model
4. Numerical Results of Composite Thrust Reverser Cascade
4.1. Operational Condition Results
4.2. Ultimate Load Condition Results
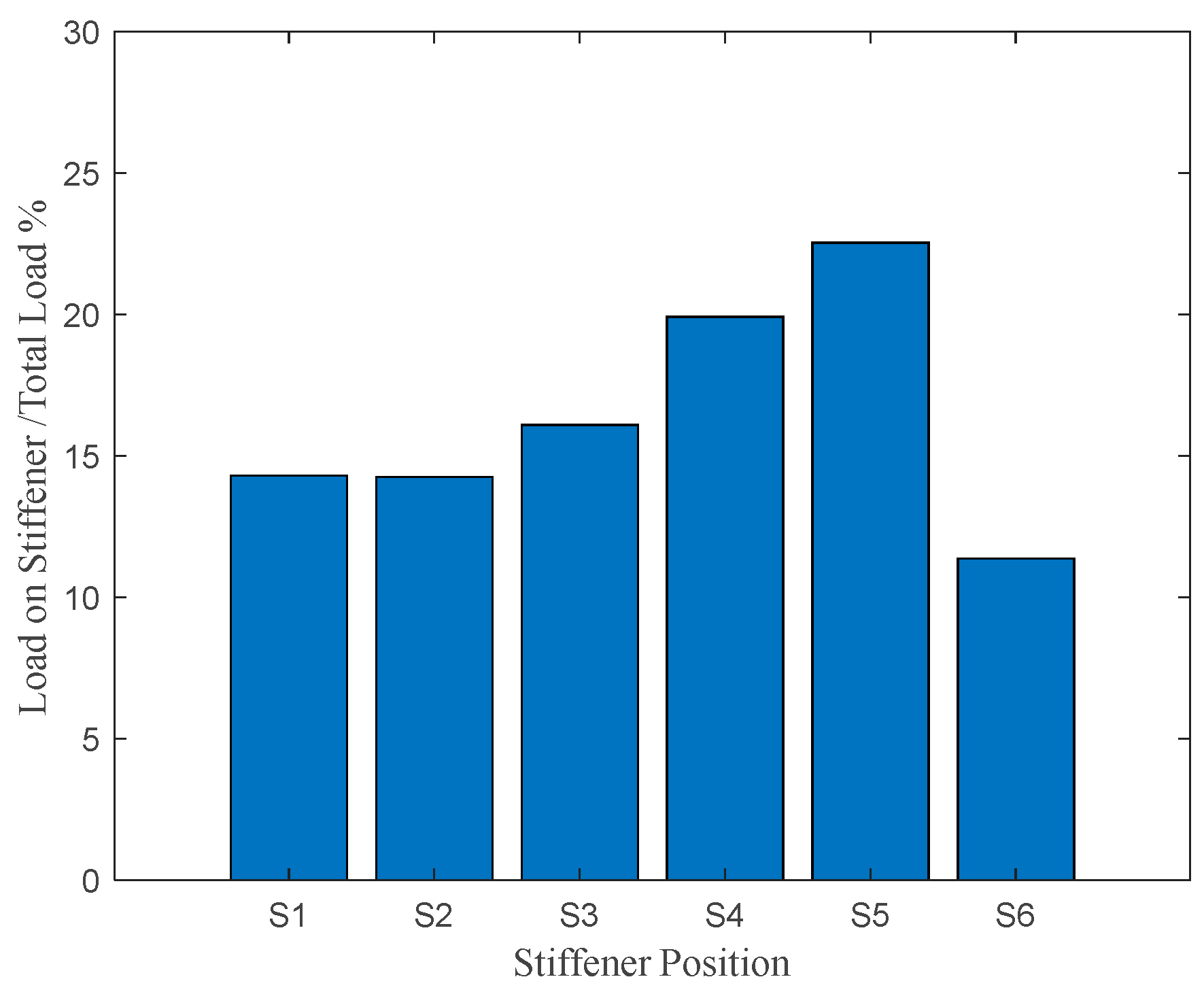
4.2.1. Global Model Results
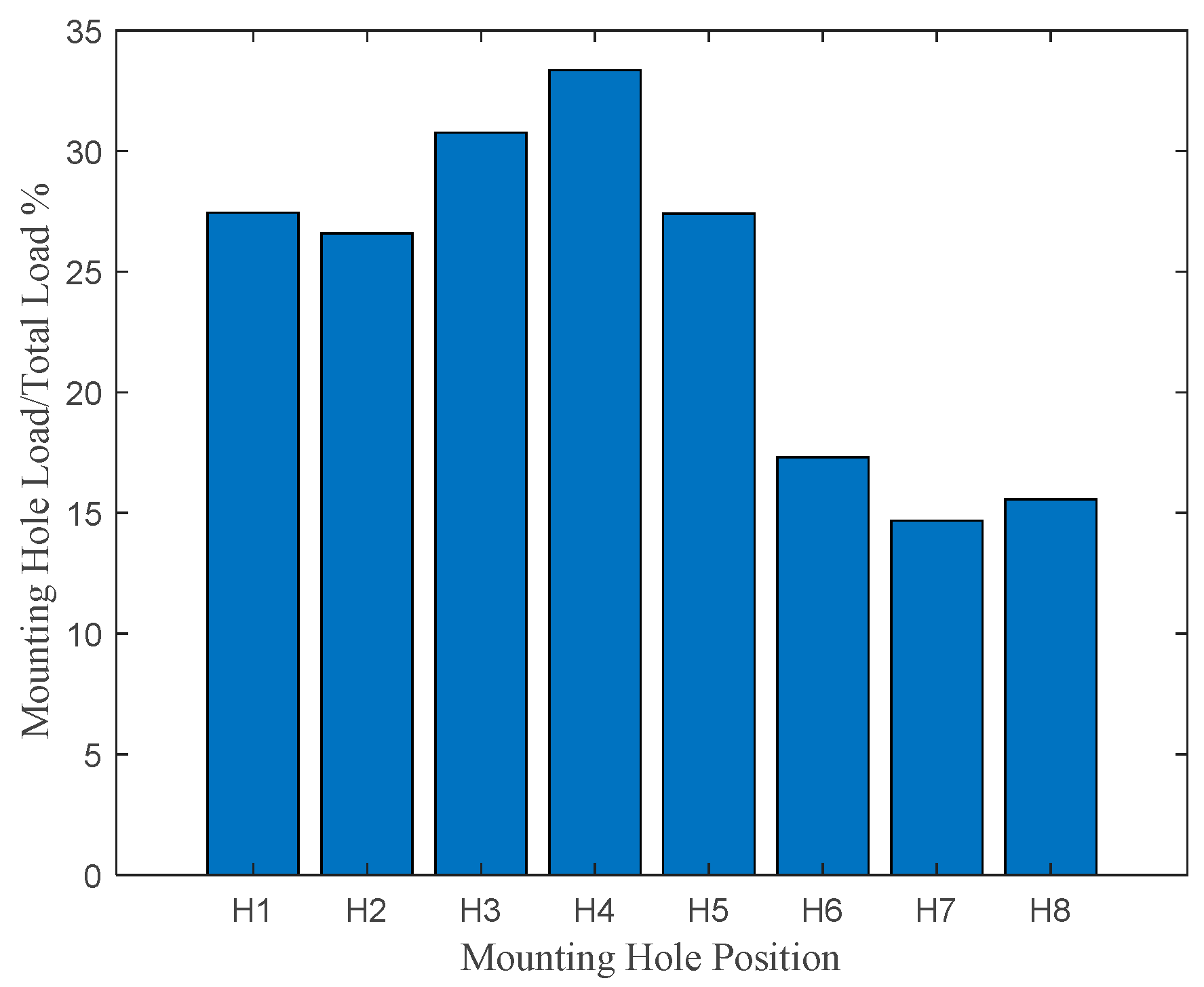
4.2.2. Sub-Model of the Mounting Hole
5. Composite Thrust Reverser Cascade Test Scheme Design
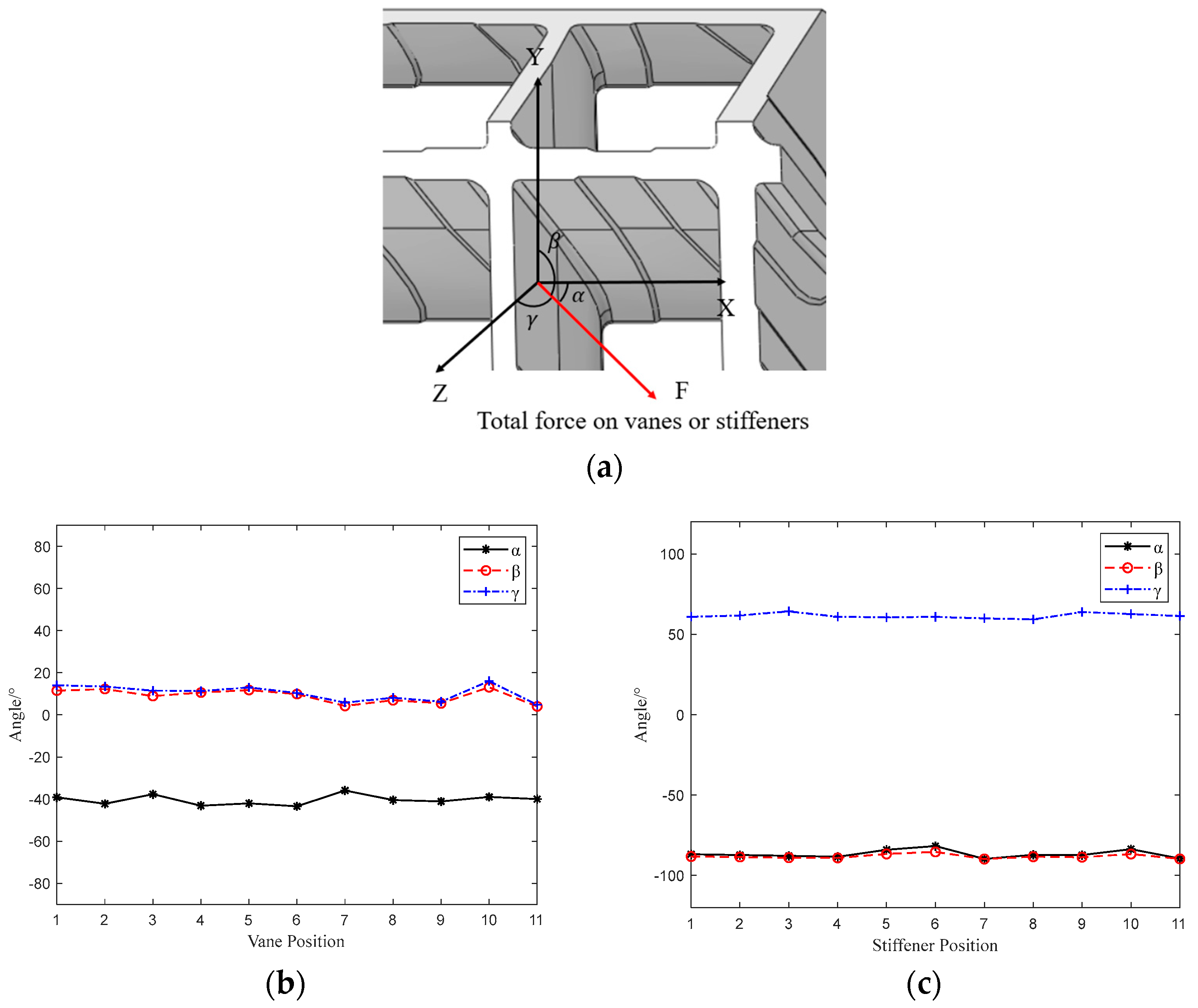
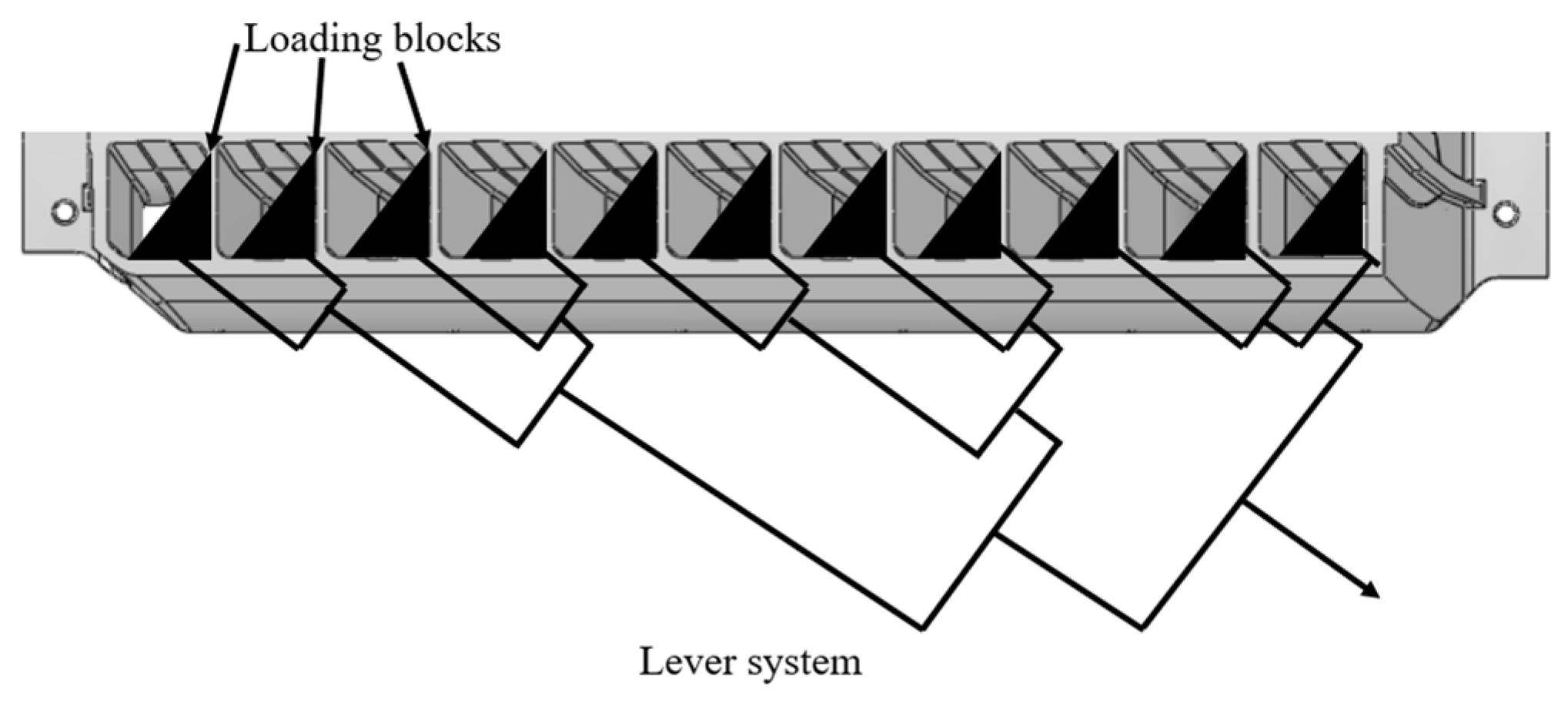
5.1. Load Simplification and Load Scheme Design
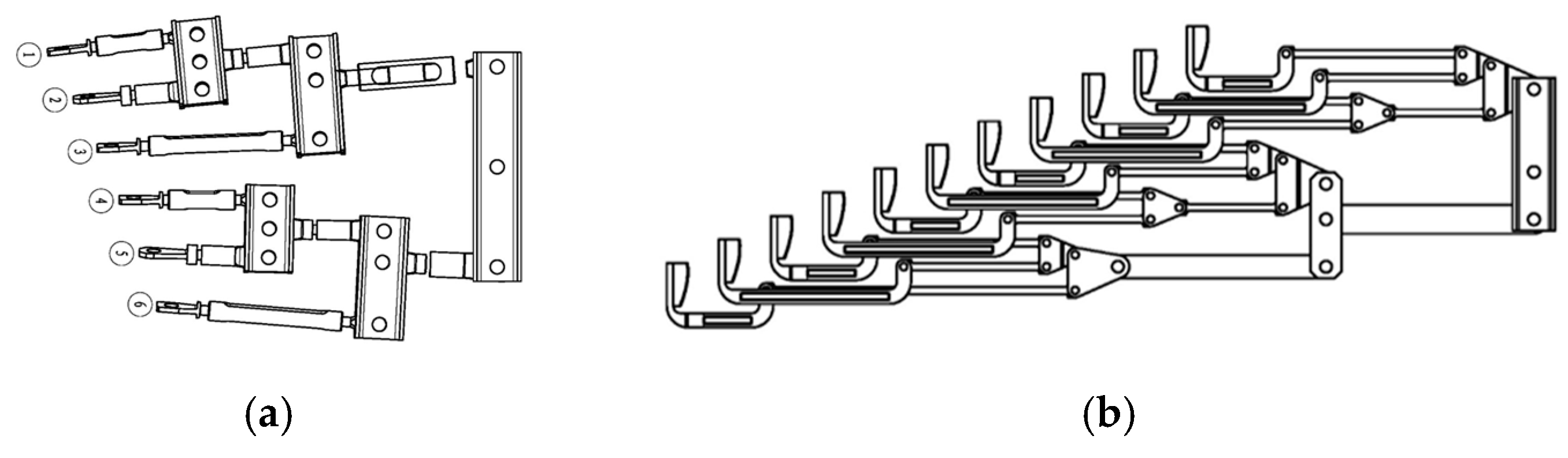
5.2. Fixture Design
5.3. Summary
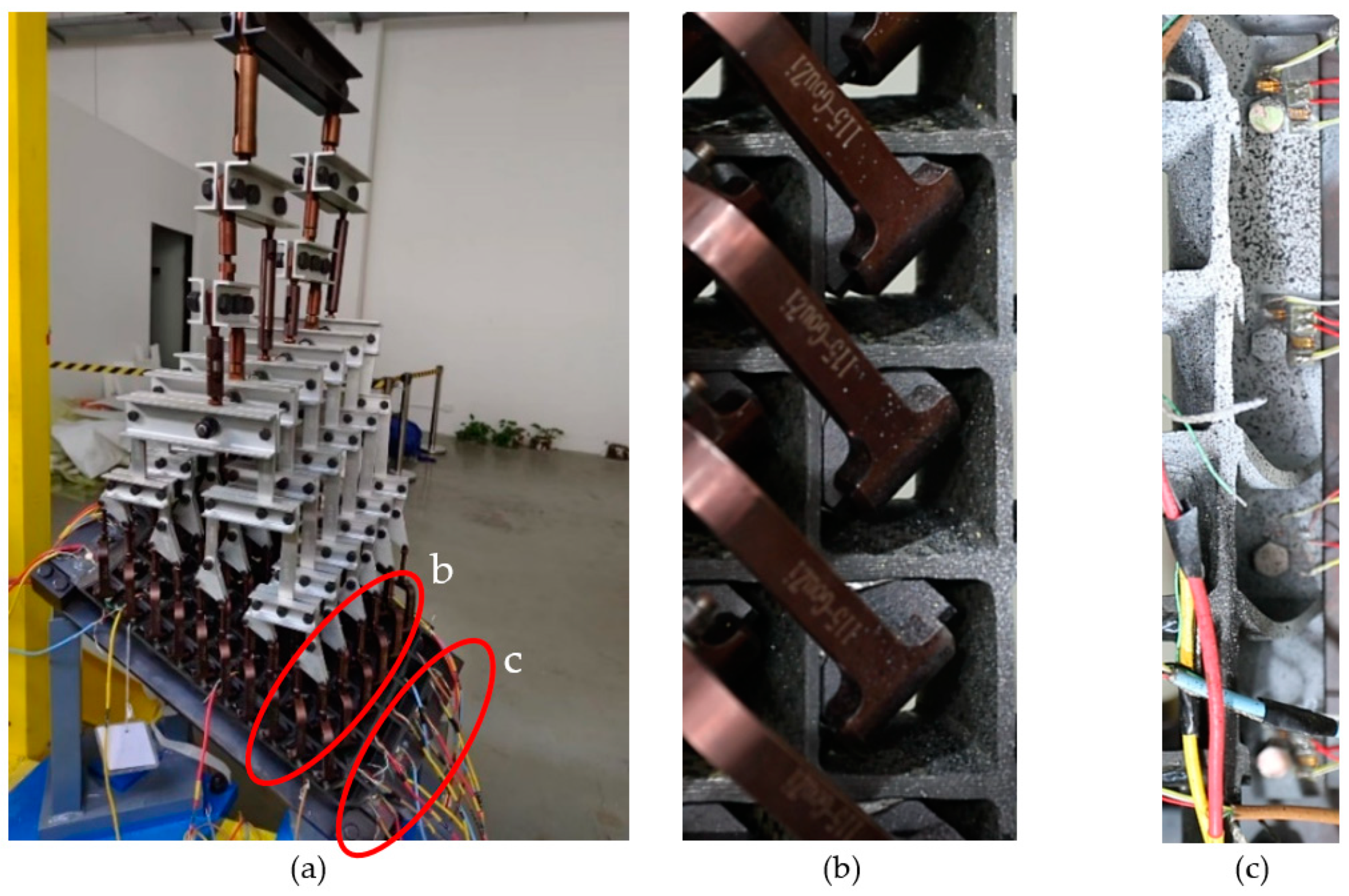
6. Test Results and Discussion
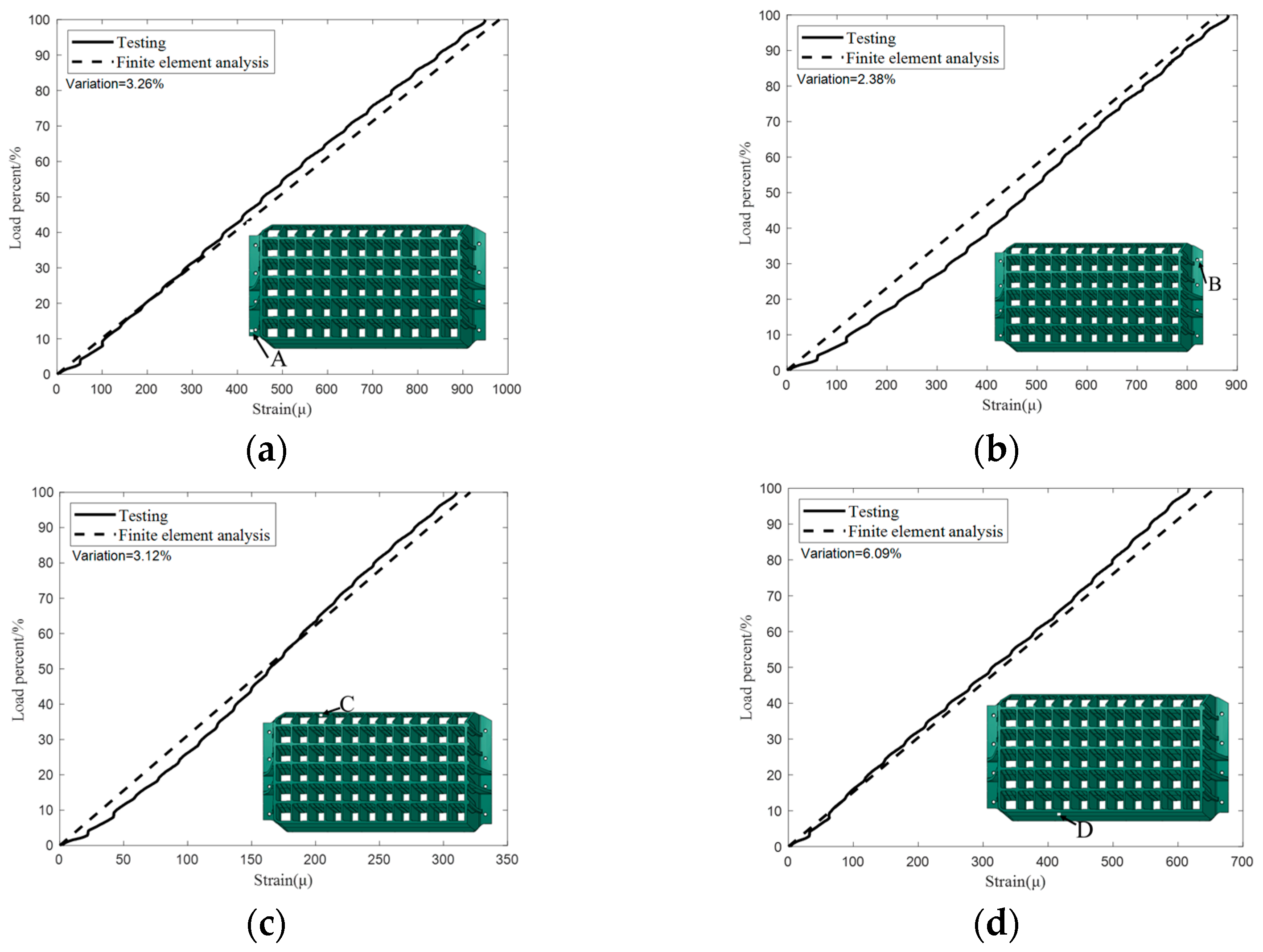
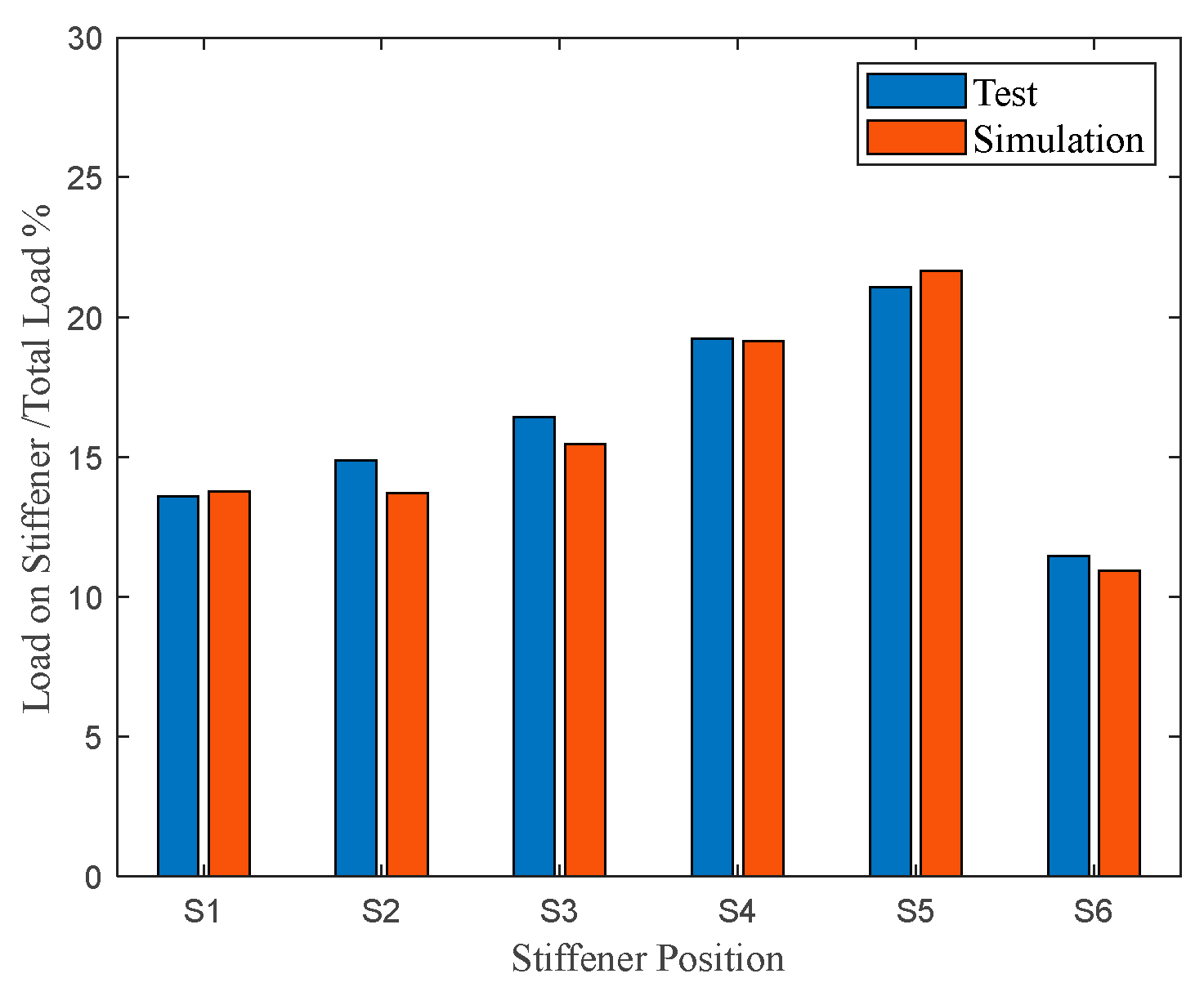
6.1. Operational Condition Test Results and Discussion
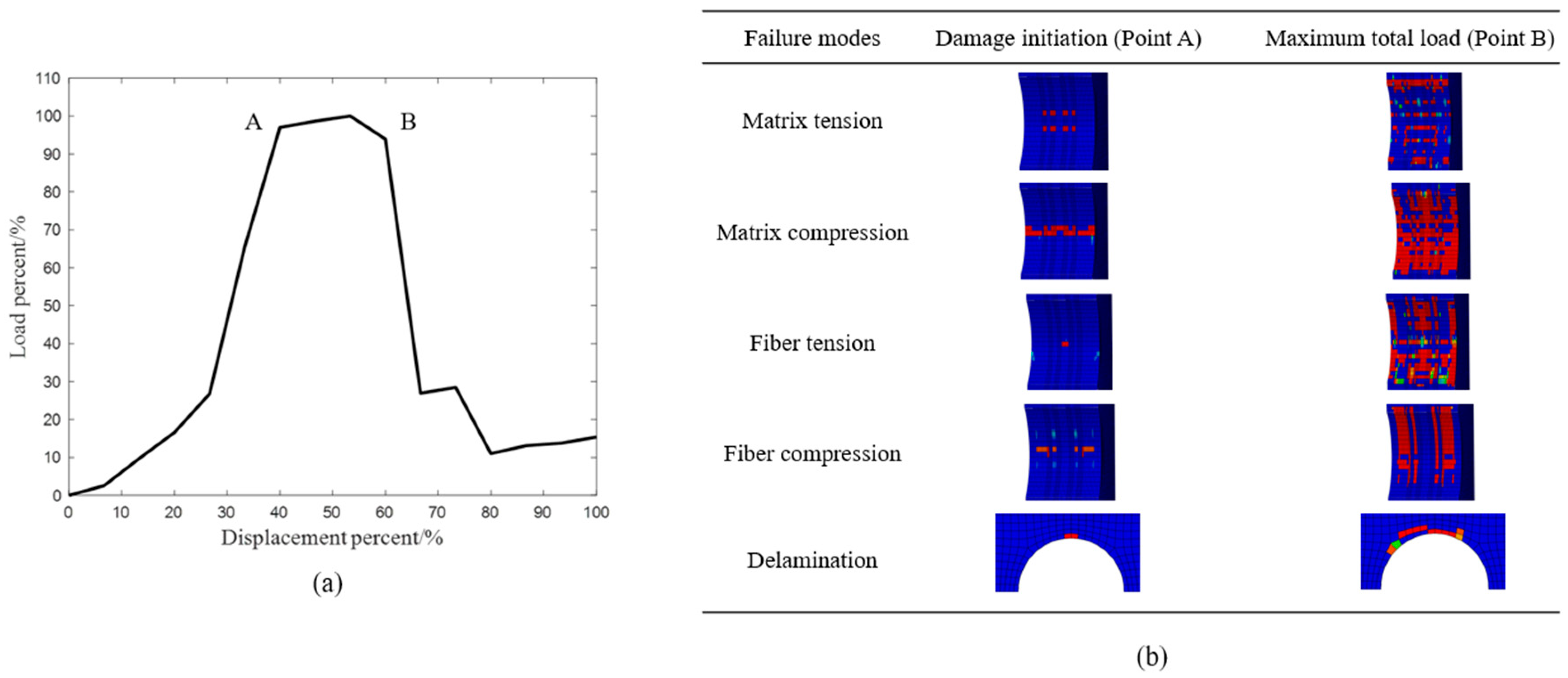
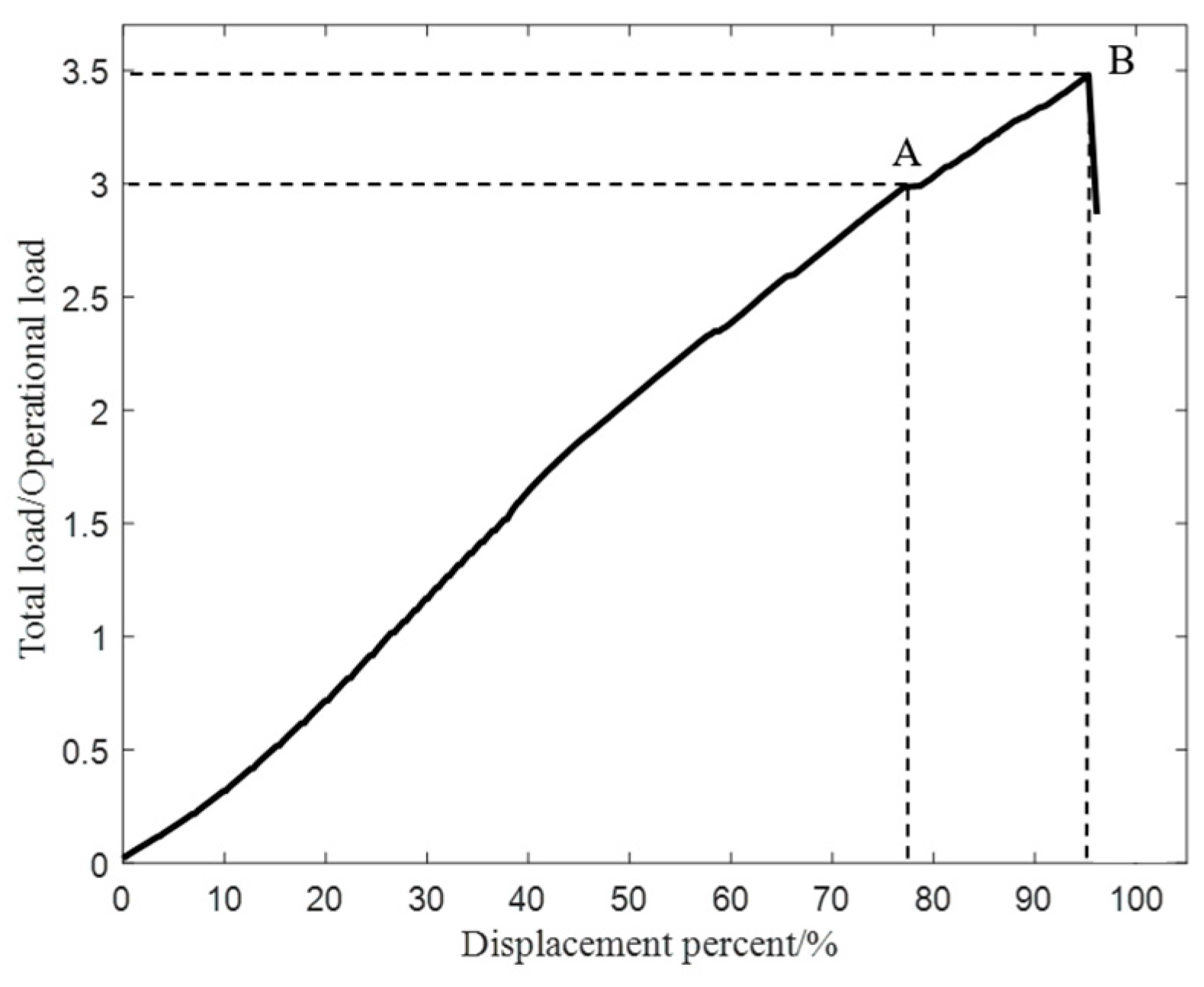
6.2. Ultimate Load Condition Test Results and Discussion
7. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Yetter, J.A. Why Do Airlines Want and Use Thrust Reversers? A Compilation of Airline Industry Responses to a Survey Regarding the Use of Thrust Reversers on Commercial Transport Airplanes; NASA: Hampton, Virginia, 1995. [Google Scholar]
- Atanasov, G.; Silberhorn, D. Hybrid aircraft for improved off-design performance and reduced emissions. In Proceedings of the AIAA Scitech 2020 Forum, Orlando, FL, USA, 6–10 January 2020; p. 0753. [Google Scholar] [CrossRef]
- Santin, M.A.; Barbosa, J.R.; Bontempo, L.P.; Silva, F.D.S.; Jesus, A.B.; Trapp, L.G.; Oliveira, G.L. Overview on thrust reverser design. In Proceedings of the 18th International Congress of Mechanical Engineering, Minas Gerais, Brazil, 6–11 November 2005; pp. 1–8. [Google Scholar]
- Filippatos, A.; Markatos, D.; Tzortzinis, G.; Abhyankar, K.; Malefaki, S.; Gude, M.; Pantelakis, S. Sustainability-driven design of aircraft composite components. Aerospace 2024, 11, 86. [Google Scholar] [CrossRef]
- Saboktakin, A. 3d textile preforms and composites for aircraft strcutures: A review. Int. J. Aviat. Aeronaut. Aerosp. 2019, 6, 2. [Google Scholar] [CrossRef]
- Zimmermann, N.; Wang, P.H. A review of failure modes and fracture analysis of aircraft composite materials. Eng. Fail. Anal. 2020, 115, 104692. [Google Scholar] [CrossRef]
- Parveez, B.; Kittur, M.I.; Badruddin, I.A.; Kamangar, S.; Hussien, M.; Umarfarooq, M.A. Scientific advancements in composite materials for aircraft applications: A review. Polymers 2022, 14, 5007. [Google Scholar] [CrossRef] [PubMed]
- Sioutis, I.; Tserpes, K. A literature review on crack arrest features for composite materials and composite joints with a focus on aerospace applications. Aerospace 2023, 10, 137. [Google Scholar] [CrossRef]
- Falaschetti, M.; Rondina, F.; Zavatta, N.; Troiani, E.; Donati, L. Effective implementation of numerical models for the crashworthiness of composite laminates. Eng. Fail. Anal. 2024, 160, 108196. [Google Scholar] [CrossRef]
- Zavatta, N.; Rondina, F.; Falaschetti, M.P.; Donati, L. Effect of thermal ageing on the mechanical strength of carbon fibre reinforced epoxy composites. Polymers 2021, 13, 2006. [Google Scholar] [CrossRef]
- Nobel, T.; Wooden, P.; Arledge, R. Experimental thrust reverser design with computational analysis. In Proceedings of the 32nd Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 10–13 January 1994; p. 21. [Google Scholar] [CrossRef]
- Strash, D.J.; Summa, J.M.; Frank, J.H.; Standish, R. Aerodynamic analysis of an installed thrust reverser. J. Propuls. Power 2000, 16, 10–15. [Google Scholar] [CrossRef]
- Yao, H.; Raghunathan, S.; Cooper, R.; Benard, E.; Tweedie, J.; Riordan, D. Numerical simulation on flow fields of the nature blockage thrust reverser. In Proceedings of the 43rd AIAA Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 10–13 January 2005; p. 631. [Google Scholar] [CrossRef]
- Bdeiwi, H.; Ciarella, A. Computational fluid dynamics investigations of a thrust reverser unit flowfield. In Proceedings of the AIAA Scitech Forum, Online, 11–15 January 2021; p. 0244. [Google Scholar] [CrossRef]
- Bi, S.; Mao, J.; Han, X.; Cai, K.; Wang, F. Study of the aerodynamic characteristics on the computed flowfield during thrust reversers operation. J. Appl. Fluid Mech. 2021, 14, 1497–1509. [Google Scholar] [CrossRef]
- Zhao, J.; Wang, X.; Meng, C.; Song, H.; Luo, Z.; Han, Q. Dynamic modeling and analysis of thrust reverser mechanism considering clearance joints and flexible component. Aerospace 2022, 9, 611. [Google Scholar] [CrossRef]
- Liu, C.; Deng, J.; Su, S. Design of loading system for thrust reverser ground simulation test. In IOP Conference Series: Earth and Environmental Science; IOP Publishing: Bristol, UK, 2018; Volume 170, p. 042093. [Google Scholar] [CrossRef]
- Shi, G.-L.; Pan, J. Electro-hydraulic force servo system for simulating thrust in reverse of experimental systems on the ground for airplane. China Mech. Eng. 2010, 21, 42. [Google Scholar]
- Henzel, J.G., Jr.; McArdle, J.G. Preliminary Performance Data of Several Tail-Pipe-Cascade-Type Model Thrust Reversers; NASA: Washington, DC, USA, 1955. [Google Scholar]
- Anderson SB Cooper, G.E.; Faye, A.E., Jr. Flight Measurements of the Effect of a Controllable Thrust Reverser on the Flight Characteristics of a Single-Engine Jet Airplane; NASA: Washington, DC, USA, 1959. [Google Scholar]
- Romine, J.; Johnson, W. Performance investigation of a fan thrust reverser for a high by-pass turbofan engine. In Proceedings of the 20th Joint Propulsion Conference, Cincinnati, OH, USA, 11–13 June 1984; p. 1178. [Google Scholar] [CrossRef]
- Scott, C.A.; Jeffrey, A.Y. Static Performance of Six Innovative Thrust Reverser Concepts for Subsonic Transport Applications-Summary of the Nasa Langley Innovative Thrust Reverser Test Program; NASA: Washington, DC, USA, 2000. [Google Scholar]
- Butterfield, J.; Yao, H.; Price, M.; Armstrong, C.; Raghunatan, S.; Riordan, D.; Cathers, G. Methodologies for structural optimisation of a thrust reverser cascade using a multidisciplinary approach. In Proceedings of the 41st Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 6–9 January 2003; p. 107. [Google Scholar] [CrossRef]
- Butterfield, J.; Yao, H.; Benard, E.; Price, M.; Cooper, R.; Armstrong, C.; Raghunathan, S.; Monaghan, D. Optimisation of a thrust reverser cascade: An assessment of dynamic response during reverse thrust. In Proceedings of the AIAA’s 3rd Annual Aviation Technology, Integration, and Operations Forum, Denver, CO, USA, 17–19 November 2003; p. 6748. [Google Scholar] [CrossRef]
- Butterfield, J.; Yao, H.; Curran, R.; Price, M.; Armstrong, C.; Raghunathan, S. Integration of aerodynamic, structural, cost and manufacturing cosiderations during the conceptual design of a thrust reverser cascade. In Proceedings of the 42nd AIAA Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 5–8 January 2004; p. 282. [Google Scholar] [CrossRef]
- Butterfield, J.; Yao, H.; Price, M.; Armstrong, C.; Raghunathan, S.; Bénard, E.; Cooper, R.; Monaghan, D. Enhancement of thrust reverser cascade performance using aerodynamic and structural integration. Aeronaut. J. 2004, 108, 621–628. [Google Scholar] [CrossRef][Green Version]
- Yao, H.; Butterfield, J.; Raghunathan, S.; Cooper, R.; Benard, E. The aerodynamic performance of a thrust reverser cascade. In Proceedings of the 24th International Congress of Aeronautical Sciences, Yokohama, Japan, 29 August–3 September 2004; Volume 4. [Google Scholar]
- Yao, H.; Butterfield, J.; Raghunathan, S.; Wang, J.; Cooper, R.; Benard, E. Optimization design for thrust reverser cascade with a view to reduce noise. Multidiscip. Model. Mater. Struct. 2005, 1, 63–72. [Google Scholar] [CrossRef]
- Butterfield, J.; Yao, H.; Curran, R.; Price, M.; Armstrong, C.; Raghunathan, S. Integrated methods for the design of a thrust reverser cascade. J. Propuls. Power 2006, 22, 862–871. [Google Scholar] [CrossRef]
- Yang, J.S.; Qi, H.Y.; Yang, X.G.; Shi, D.Q. Progressive failure analysis of an integral composite joint for thrust reverser cascade. Key Eng. Mater. 2019, 795, 325–332. [Google Scholar] [CrossRef]
- Huicheng, Y.; Qingzhen, Y.; Canliang, W.; Jin, B. Parametric design optimization of reverse thrust blades and analysis of fluid-solid coupling. Front. Energy Res. 2022, 10, 860337. (In English) [Google Scholar] [CrossRef]
- Hashin, Z.; Rotem, A. A fatigue failure criterion for fiber reinforced materials. J. Compos. Mater. 1973, 7, 448–464. [Google Scholar] [CrossRef]
- Hashin, Z. Fatigue failure criteria for unidirectional fiber composites. J. Appl. Mech. 1980, 47, 329–334. [Google Scholar] [CrossRef]









| Properties | Values | Properties | Values |
|---|---|---|---|
| Longitudinal tensile modulus | 125,000 MPa | Longitudinal tensile strength | 1504.7 MPa |
| Longitudinal compression modulus | 120,000 MPa | Longitudinal compression strength | 1120.5 MPa |
| Transversal tensile modulus | 19,500 MPa | Transversal tensile strength | 60.9 MPa |
| Transversal compression modulus | 9900 MPa | Transversal compression strength | 207.7 MPa |
| Shear modulus | 5300 MPa | Longitudinal shear strength | 86.6 MPa |
| Poisson’s ratio | 0.3 | Transversal shear strength | 49.2 MPa |
| Properties | Values | Properties | Values |
|---|---|---|---|
| Longitudinal tensile modulus | 65,000 MPa | Longitudinal tensile strength | 538.7 MPa |
| Longitudinal compression modulus | 60,000 MPa | Longitudinal compression strength | 697.4 MPa |
| Transversal tensile modulus | 63,600 MPa | Transversal tensile strength | 534.2 MPa |
| Transversal compression modulus | 57,700 MPa | Transversal compression strength | 595.2 MPa |
| Shear modulus | 3800 MPa | Longitudinal shear strength | 59.2 MPa |
| Poisson’s ratio | 0.04 | Transversal shear strength | 72.2 MPa |
| Area | 0° | 90° | 45° | −45° | 0/90° | ±45° | Thickness/mm |
|---|---|---|---|---|---|---|---|
| Mounting edges | 43.48% | 8.70% | 0.00% | 0.00% | 21.74% | 26.09% | 4.72 |
| Vanes | 5.88% | 11.76% | 11.76% | 11.76% | 47.06% | 11.76% | 2.255 |
| Stiffeners | 11.11% | 11.11% | 0.00% | 0.00% | 44.44% | 33.33% | 2.59 |
| Surround | 43.48% | 8.70% | 0.00% | 0.00% | 21.74% | 26.09% | 4.03 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, D.; Chen, X.; Qiu, H. Test Scheme Design and Numerical Simulation of Composite Thrust Reverser Cascade. Aerospace 2024, 11, 641. https://doi.org/10.3390/aerospace11080641
Wu D, Chen X, Qiu H. Test Scheme Design and Numerical Simulation of Composite Thrust Reverser Cascade. Aerospace. 2024; 11(8):641. https://doi.org/10.3390/aerospace11080641
Chicago/Turabian StyleWu, Dingzhou, Xiuhua Chen, and Hongyan Qiu. 2024. "Test Scheme Design and Numerical Simulation of Composite Thrust Reverser Cascade" Aerospace 11, no. 8: 641. https://doi.org/10.3390/aerospace11080641
APA StyleWu, D., Chen, X., & Qiu, H. (2024). Test Scheme Design and Numerical Simulation of Composite Thrust Reverser Cascade. Aerospace, 11(8), 641. https://doi.org/10.3390/aerospace11080641





